Q.1.
A particle with certain charge enters a region of constant, uniform and mutually orthogonal fields E and B with a velocity u perpendicular to both E and B and comes out without any change in magnitude or direction u. This means that
-
0%
Force acting on particle is Inclined at an angle of $$180^o$$
-
0%
Force acting on particle is highly directional
-
0%
Force acting on charged particle is zero
-
0%
Charge on particle is too high
Q.2.
If flow of electric current is parallel to magnetic field, force will be:
-
0%
Negative
-
0%
Maximum
-
0%
Zero
-
0%
None of these
Q.3.
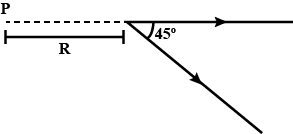
A long straight wire carrying current $$I$$ is bent at its mid-point to form an angle of $$45^{\circ}$$. The field at point $$P$$ as shown in the figure is

-
0%
$$\dfrac {\mu_{0}I}{4\pi R}(\sqrt {2} - 1)$$
-
0%
$$\dfrac {\mu_{0}I}{4\pi R}(\sqrt {2} + 1)$$
-
0%
$$\dfrac {\mu_{0}I}{4\sqrt {2}\pi R}(\sqrt {2} + 1)$$
-
0%
$$\dfrac {\mu_{0}I}{4\sqrt {2}\pi R}(\sqrt {2} - 1)$$
Q.4.
The ratio of the magnetic field at the centre of a current carrying circular wire and the magnetic field at the centre of a square coil made from the same length of wire is
-
0%
$$\dfrac {\pi}{2\sqrt {2}}$$
-
0%
$$\dfrac {\pi}{4\sqrt {2}}$$
-
0%
$$\dfrac {\pi^{2}}{4\sqrt {2}}$$
-
0%
$$\dfrac {\pi^{2}}{8\sqrt {2}}$$
Q.5.
Potential energy of a bar magnet, of magnetic moment M is placed in a magnetic filed of induction B such that it makes an angle $$\theta$$ with the direction of B is?
-
0%
$$MB\,sin\, \theta$$
-
0%
$$-MB\,cos\, \theta$$
-
0%
$$MB(1-cos\theta)$$
-
0%
$$MB(1+cos\theta)$$
Q.6.
In the given figure, what is the magnetic field induction at point O.


-
0%
$$\displaystyle\frac{\mu_0l}{4\pi r}$$
-
0%
$$\displaystyle\frac{\mu_0l}{4r}+\frac{\mu_0l}{2\pi r}$$
-
0%
$$\displaystyle\frac{\mu_ol}{4r}+\frac{\mu_0l}{4\pi r}$$
-
0%
$$\displaystyle\frac{\mu_0l}{4r}-\frac{\mu_0l}{4\pi r}$$
Q.7.
A magnetic dipole is under the influence of two magnetic fields. The angle between the field directions is $$60^0$$ and one of the fields has a magnitude of $$1.2 \times 10^{-2}$$T. If the dipole comes to stable equilibrium at an angle of $$30^0$$ with this field, then the magnitude of the field is
-
0%
$$1.2 \times 10^{-4}$$T
-
0%
$$2.4 \times 10^{-4}$$T
-
0%
$$1.2 \times 10^{-2}$$T
-
0%
$$2.4 \times 10^{-2}$$T
Q.8.
Assertion : Diamagnetism is universal, it is present in all materials.
Reason : Field due to induced magnetic moment is opposite to the magnetising field
Reason : Field due to induced magnetic moment is opposite to the magnetising field
-
0%
If both assertion and reason are true and reason is the correct explanation of assertion.
-
0%
If both assertion and reason are true and reason is not the correct explanation of assertion.
-
0%
If assertion is true but reason is false.
-
0%
If both assertion and reason are false
Q.9.
In an inertial frame of reference, the magnetic force on a moving charged particle is $$\bar{F}$$. Its value in another internal frame of reference will be
-
0%
remain same
-
0%
changed due to change in the amount of charge
-
0%
changed due to change in velocity of charged particle
-
0%
changed due to change in field direction
Q.10.
Imagine yourself to be swimming in the wire in the direction of the current and facing a magnetic needle, then the north pole of the needle is deflected towards your left hand. Which is this rule?
-
0%
Ampere's rule
-
0%
Faraday right hand rule
-
0%
Faraday left hand rule
-
0%
Maxwell's rule
Q.11.
Two free parallel wires carrying currents in opposite directions.
-
0%
Do not affect each other
-
0%
Attract each other
-
0%
Repel each other
-
0%
None of these
Q.12.
A short bar magnet placed with its axis at $$30^0$$ with a uniform external magnetic field of $$0.35T$$ experiences a torque of magnitude equal to $$4.5 \times 10^{-2}$$N m. The magnitude of magnetic moment of the given magnet is
-
0%
$$26 JT^{-1}$$
-
0%
$$2.6 JT^{-1}$$
-
0%
$$0.26 JT^{-1}$$
-
0%
$$0.026 JT^{-1}$$
Q.13.
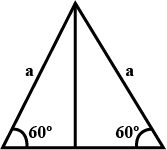
A uniform conduction wire of length $$10 \,g$$ and resistance $$R$$ is wound up into four turns as a current carrying coil in the shape of equilateral triangle of side $$a$$. If current $$I$$ is flowing through the coil then the magnetic moment of the coil is

-
0%
$$\dfrac{\sqrt3}{2}a^2I$$
-
0%
$$\dfrac{a^2I}{\sqrt3}$$
-
0%
$$\sqrt3a^2I$$
-
0%
$$\dfrac{2a^2I}{\sqrt3}$$
Q.14.
Ampere's circuital law is given by:
-
0%
$$\oint \bar{H} . \bar{dl} = \mu _0 I_{enc}$$
-
0%
$$\oint \bar{B} . \bar{dl} = \mu _0 I_{enc}$$
-
0%
$$\oint \bar{B} . \bar{dl} = \mu _0 I$$
-
0%
$$\oint \bar{H} . \bar{dl} = \mu _0 I$$
Q.15.
A circular coil of magnetic moment $$0.355$$J $$T^{-1}$$ rests with its plane normal to an external field of magnitude $$5.0\times 10^{-2}$$T. The coil is free to turn about an axis in its plane perpendicular to the field direction. When the coil is turned slightly and released, it oscillates about its stable equilibrium with a frequency of $$2$$Hz. The moment of inertia of the coil about its axis of rotation is?
-
0%
$$1.13\times 10^{-1}$$kg $$m^2$$
-
0%
$$1.13\times 10^{-2}$$kg $$m^2$$
-
0%
$$1.13\times 10^{-3}$$kg $$m^2$$
-
0%
$$1.13\times 10^{-4}$$kg $$m^2$$
Q.16.
Amperes circuital law is given by
-
0%
$$\oint\bar H. \bar {dl} =\mu_0 I_{enc}$$
-
0%
$$\oint\bar B. \bar {dl} =\mu_0 I$$
-
0%
$$\oint\bar B. \bar {dl}=\mu_0 J$$
-
0%
$$\oint\bar H. \bar {dl}=\mu_0 J$$
Q.17.
An electron of energy 1800 eV describes a circular path in magnetic field of flux density 0.4 T. The radius of path is (q = 1.6 X $$10^{-19}$$ C, $$m_e$$ = 9.1 X $$10^{-31}$$ kg)
-
0%
2.58 X $$10^{-4}$$m
-
0%
3.58 X $$10^{-4}$$m
-
0%
2.58 X $$10^{-3}$$m
-
0%
3.58 X $$10^{-3}$$m
Q.18.
The magnetic induction at a point $$1$$ $$\overset{o}{A}$$ away from a proton measured along its axis of spin is (magnetic moment of the proton is $$1.4\times 10^{-26}$$A $$m^2$$).
-
0%
$$0.28$$ mT
-
0%
$$28$$ mT
-
0%
$$0.028$$ mT
-
0%
$$2.8$$ mT
Q.19.
A 200 turn closely wound circular coil of radius 15 cm carries a current of 4 A. The magnetic moment of this coil is:
-
0%
$$36.5 A\,m^2$$
-
0%
$$56.5 A\,m^2$$
-
0%
$$66.5A\,m^2$$
-
0%
$$108A\,m^2$$
Q.20.
If an electron is moving with velocity $$\bar{v}$$ produces a magnetic field $$\bar{B}$$, then
-
0%
the direction of field $$\bar{B}$$ will be same as the direction of velocity $$\bar{v}$$
-
0%
the direction of field $$\bar{B}$$ will be opposite as the direction of velocity $$\bar{v}$$
-
0%
the direction of field $$\bar{B}$$ will be perpendicular as the direction of velocity $$\bar{v}$$
-
0%
the direction of field $$\bar{B}$$ does not depend upon the direction of velocity $$\bar{v}$$
Q.21.
The torque and magnetic potential energy of a magnetic dipole in most stable position in a uniform magnetic field($$\bar{B}$$) having magnetic moment ($$\bar{m}$$) will be.
-
0%
$$-$$mB, zero
-
0%
mB, zero
-
0%
Zero, mB
-
0%
Zero, $$-$$mB
Q.22.
Two charged particles traverse identical helical j paths in a completely opposite sense in a uniform magnetic field $$\bar B=B_0\hat {k}$$.
-
0%
They have equal z-components of momenta.
-
0%
They must have equal charges.
-
0%
They necessarily represent a particle-antiparticle pair.
-
0%
The charge to mass ratio satisfy:
$${\left (\dfrac{e}{m} \right )}_1+{\left (\dfrac{e}{m} \right )}_2=0.$$
Q.23.
Similar pole each of pole strength m are placed at a distance of $$1, 2, 4, 8$$,_________ meters from the origin on the x-axis. Where do you place a similar pole on the other side of the origin so that the origin becomes a neutral point.
-
0%
$$0.5$$m
-
0%
$$0.5774$$m
-
0%
$$0.866$$m
-
0%
$$1$$m
Q.24.
The magnetic field at the centre of a circular loop of area A is B. The magnetic moment of the loop is
-
0%
$$\dfrac{BA^2}{\mu_0\pi}$$
-
0%
$$\dfrac{BA\sqrt A}{\mu}$$
-
0%
$$\dfrac{BA\sqrt A}{\mu_0\pi}$$
-
0%
$$\dfrac{2BA\sqrt A}{\mu_0\sqrt\pi}$$
Q.25.
The magnetic moment of a short bar magnet placed with its magnetic axis at $$30^o$$ to an external field of $$900$$G and experiences a torque of $$0.02$$N m is?
-
0%
$$0.35$$ A $$m^2$$
-
0%
$$0.44$$ A $$m^2$$
-
0%
$$2.45$$ A $$m^2$$
-
0%
$$1.5$$ A $$m^2$$
Q.26.
A straight steel wire of length $$l$$ has magnetic moment $$m$$. If the wire is bent in the form of a semicircle, the new value of the magnetic dipole moment is _______
-
0%
$$m$$
-
0%
$$\cfrac { m }{ 2 } $$
-
0%
$$\cfrac { m }{ \pi } $$
-
0%
$$\cfrac { 2m }{ \pi } $$
Q.27.
The magnetic moment associated with a circular coil of 35 turns and radius 25 cm, if it carries a current of 11 A is:
-
0%
$$72.2 A\,m^2$$
-
0%
$$70.5 A\,m^2$$
-
0%
$$74.56A\,m2$$
-
0%
$$75.56A\,m^2$$
Q.28.
A circular coil of $$300$$ turns and diameter $$14$$ cm carries a current of $$15$$A. The magnitude of magnetic moment associated with the loop is?
-
0%
$$51.7$$J $$T^{-1}$$
-
0%
$$69.2$$J $$T^{-1}$$
-
0%
$$38.6$$J $$T^{-1}$$
-
0%
$$19.5$$J $$T^{-1}$$
Q.29.
Biot-Savart law indicates that the moving electrons (velocity $$\bar v$$ ) produce a magnetic field $$\bar B$$ such that:
-
0%
$$\bar B \perp \bar v$$
-
0%
$$\bar B \parallel \bar v$$
-
0%
it obeys inverse cube law.
-
0%
it is along the line joining the electron and point of observation.
Q.30.
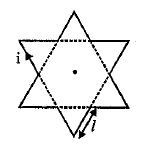
A star shaped loop (with l = length of each edge)then the magnetic field at the centroid of the loop is:

-
0%
$$\frac{3\mu_0i}{\pi l}$$
-
0%
$$\frac{3\mu_0i}{2\pi l}$$
-
0%
$$(3 -\sqrt3)\frac{\mu_0i}{\pi l}$$
-
0%
$$(3 +\sqrt3)\frac{3\mu_0i}{\pi l}$$
Q.31.
Fill in the blanks
A charge is moving through a magnetic field. The force acting on the charge is maximum when the angle between the velocity of charge and the magnetic field is ______
A charge is moving through a magnetic field. The force acting on the charge is maximum when the angle between the velocity of charge and the magnetic field is ______
-
0%
$$\pi$$
-
0%
$$\pi/3$$
-
0%
$$\pi/2$$
-
0%
$$\pi/4$$
Q.32.
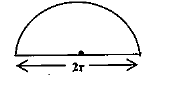
A magnetised wire of magnetic movement M and length l is bent in the form of a semicircle of radius r. The new magnetic moment is:

-
0%
M
-
0%
$$\frac{2M}{\pi}$$
-
0%
$$\frac{M}{\pi}$$
-
0%
None of the above
Q.33.
The incorrect statement of the following is:
-
0%
Work done on a moving charge in a magnetic field is zero
-
0%
The kinetic energy of a moving charge in magnetic field is conserved
-
0%
Magnetic field is not produced due to electron moving in a straight line
-
0%
Kinetic energy of a moving charge in an electric field changes
Q.34.
A conducting square loop of side $$L$$ and resistance $$R$$ moves in its plane with a uniform velocity $$v$$ perpendicular to one of its sides. A magnetic induction $$B$$, constant in time and space, pointing perpendicular and into the plane of the loop exists everywhere, then the current induced in the loop is


-
0%
$$BLv/R$$ clockwise
-
0%
$$BLv/R$$ anticlockwise
-
0%
$$2 BLv/R$$ anticlockwise
-
0%
$$0$$
Q.35.
A proton and an alpha particle are separately projected in a region , where a uniform magnetic field exists. The initial velocities are perpendicular to the direction of magnetic field . If both the particles move along circles of equal radii , the ratio of momentum of proton to alpha particle( $$\dfrac{P_p}{P_a}$$)
-
0%
1
-
0%
1/2
-
0%
2
-
0%
1/4
Q.36.
A charged particle moves in a uniform magnetic field perpendicular to it, with a radius of curvature 4 cm. On passing through a metallic sheet it looses half of its kinetic energy, then the radius of curvature of the particle is :
-
0%
2 cm
-
0%
4 cm
-
0%
8 cm
-
0%
$$2\sqrt2$$ cm
Q.37.
Potential energy of a bar magnet of magnetic moment $$M$$ placed in a magnetic field of induction $$B$$ Such that it makes an angle $$\theta$$ with the direction of $$B$$ is (take $$\theta={90}^{o}$$ as datum)
-
0%
$$-M\ B \sin {\theta}$$
-
0%
$$-M\ B \cos {\theta}$$
-
0%
$$M\ B (1-\cos {\theta})$$
-
0%
$$M\ B (1+\cos {\theta})$$
Q.38.
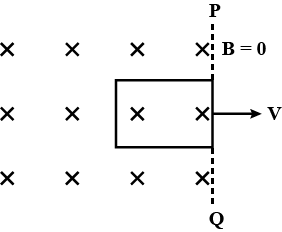
Figure shows a square loop of side $$1m$$ and resistance $$1\Omega$$. The magnetic field on left side of line PQ has a magnitude $$B=1.0T$$. The work done in pulling the loop out of the field uniformly in $$1s$$ is

-
0%
$$1J$$
-
0%
$$10J$$
-
0%
$$0.1J$$
-
0%
$$100J$$
Q.39.
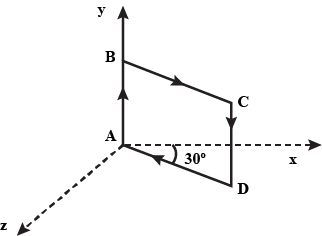
Figure shows a square current-carrying loop $$ABCD$$ of side $$2\ m$$ and current $$I=\dfrac { 1 }{ 2 } A$$. The magnetic moment $$\vec { M }$$ of the loop is:

-
0%
$$\left( \hat { i } -\sqrt { 3 } \hat { k } \right) A-{ m }^{ 2 }$$
-
0%
$$\left( \hat { j } -\hat { k } \right) A-{ m }^{ 2 }$$
-
0%
$$\left( \sqrt { 3 } \hat { i } -\hat { k } \right) A-{ m }^{ 2 }$$
-
0%
$$\left( \hat { i } -\hat { k } \right) A-{ m }^{ 2 }$$
Q.40.
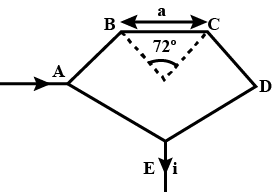
Magnetic field strength at the centre of regular pentagon made of a conducting wire of uniform cross section area as shown in figure is : (i amount of current enters at A and leaves at E)

-
0%
$$\frac{5\mu_0 i}{4\pi a} [2sin \frac{72^0}{2}]$$
-
0%
0
-
0%
$$\frac{3\mu_0 i}{4\pi a} [2sin \frac{72^0}{2}]$$
-
0%
$$\frac{\mu_0 i}{4\pi a} [2sin \frac{72^0}{2}]$$
Q.41.
The correct Biot-Savart law in vector form is?
-
0%
$$d\vec{B}=\dfrac{\mu_0}{4\pi}\dfrac{I(d\vec{l}\times \vec{r})}{r^2}$$
-
0%
$$d\vec{B}=\dfrac{\mu_0}{4\pi}\dfrac{I(d\vec{l}\times \vec{r})}{r^3}$$
-
0%
$$d\vec{B}=\dfrac{\mu_0}{4\pi}\dfrac{Id\vec{l}}{r^2}$$
-
0%
$$d\vec{B}=\dfrac{\mu_0}{4\pi}\cdot \dfrac{Id\vec{l}}{r^3}$$
Q.42.
A long straight wire of circular cross-section is made of a non-magnetic material. The wire is of radius $$a$$.The wire carries a current $$I$$ which is uniformly distributed over its cross-section. The energy stored per unit length in the magnetic field contained within the wire is
-
0%
$$U=\dfrac {\mu_{0}I^{2}}{8 \pi}$$
-
0%
$$U=\dfrac {\mu_{0}I^{2}}{16 \pi}$$
-
0%
$$U=\dfrac {\mu_{0}I^{2}}{4 \pi}$$
-
0%
$$U=\dfrac {\mu_{0}I^{2}}{2 \pi}$$
Q.43.
The current carrying wire of length $$l$$ and current $$I$$ is coiled into a circular coil of $$n$$ number of turns. The magnetic dipole moment of the coil is
-
0%
$$\cfrac{Il^{2}}{{\pi}n}$$
-
0%
$$\cfrac{Il^{2}}{2{\pi}n}$$
-
0%
$$\cfrac{nIl^{2}}{4\pi}$$
-
0%
$$\cfrac{Il^{2}}{4{\pi}n}$$
Q.44.
When a charged particle enters in a uniform magnetic field, then its kinetic energy
-
0%
remains constant
-
0%
increases
-
0%
decreases
-
0%
becomes zero
Q.45.
A circular coil of radius 4 cm and of 20 turns carries a current of 3 amperes. It is placed in a magnetic field of intensity of $$0.5 \ weber /m^2$$. The magnetic dipole moment of the coil is
-
0%
$$0.15 \ ampere-m^2$$
-
0%
$$0.3 \ ampere-m^2$$
-
0%
$$0.45 \ ampere-m^2$$
-
0%
$$0.6 \ ampere-m^2$$
Q.46.
Two long thin charged rods with charge density $$ \lambda $$ each are placed parallel to each other at a distance d apart. The force per unit length exerted on one rod by the other will beWhere $$\left( K=\dfrac { 1 }{ 4\pi \varepsilon_ 0 }\right) $$:
-
0%
$$ \dfrac { K2\lambda }{ d } $$
-
0%
$$ \dfrac { K2\lambda ^ 2 }{ d } $$
-
0%
$$ \dfrac { K2\lambda }{ d^ 2 } $$
-
0%
$$ \dfrac { K2\lambda ^ 2 }{ d^ 2 } $$
Q.47.
The maximum energy of a deuteron coming out a cyclotron is $$20\ MeV$$. The maximum energy of proton that can be obtained from this accelerator is:
-
0%
$$10\ MeV$$
-
0%
$$20\ MeV$$
-
0%
$$30\ MeV$$
-
0%
$$40\ MeV$$
Q.48.
A particle of mass m, charge $$q$$ and kinetic energy $$t$$ enters a transverse uniform magnetic field of induction B. After 3 s, the kinetic energy of the particle will be
-
0%
$$3T$$
-
0%
$$2T$$
-
0%
$$T$$
-
0%
$$4T$$
Q.49.
A magnetic moment m oscillating freely in earth's horizontal magnetic field makes a oscillation per minute. If the magnetic moment is quadrupled and the earth's field doubled, the number of oscillation made per minute would becomes
-
0%
$$\dfrac{n}{2\sqrt{2}}$$
-
0%
$$\dfrac{n}{\sqrt{2}}$$
-
0%
$$2\sqrt{2}n$$
-
0%
$$\sqrt{2}n$$
Q.50.
The magnetic field near a current carrying conductor is given by
-
0%
Coulomb's law
-
0%
Lenz' law
-
0%
Biot-Savart's law
-
0%
Kirchhoff's law