MCQ Questions for Class 11 Maths Quiz with Answers Chapter Wise PDF Download
CBSE Multiple Choice Type Questions for 11th Class Maths PDF formatted study resources are available for free download. These Grade 11 Maths CBSE MCQ Mock Test helps you learn & practice the concepts in a fun learning way.
Class 11 Maths MCQs Multiple Choice Questions with Answers
Here are the chapterwise CBSE MCQ Quiz Test Questions for Class 11th Maths in pdf format that helps you access & download so that you can practice online/offline easily.
- Binomial Theorem Class 11 Engineering Maths MCQ Questions
- Complex Numbers And Quadratic Equations Class 11 Engineering Maths MCQ Questions
- Conic Sections Class 11 Engineering Maths MCQ Questions
- Introduction To Three Dimensional Geometry Class 11 Engineering Maths MCQ Questions
- Limits And Derivatives Class 11 Engineering Maths MCQ Questions
- Linear Inequalities Class 11 Engineering Maths MCQ Questions
- Mathematical Reasoning Class 11 Engineering Maths MCQ Questions
- Permutations And Combinations Class 11 Engineering Maths MCQ Questions
- Principle Of Mathematical Induction Class 11 Engineering Maths MCQ Questions
- Probability Class 11 Engineering Maths MCQ Questions
- Relations And Functions Class 11 Engineering Maths MCQ Questions
- Sequences And Series Class 11 Engineering Maths MCQ Questions
- Sets Class 11 Engineering Maths MCQ Questions
- Statistics Class 11 Engineering Maths MCQ Questions
- Straight Lines Class 11 Engineering Maths MCQ Questions
- Trigonometric Functions Class 11 Engineering Maths MCQ Questions
Binomial Theorem Class 11 Engineering Maths MCQ Quiz
Quiz 1
Quiz 2
Quiz 3
Quiz 4
Quiz 5
Quiz 6
Quiz 7
Quiz 8
Quiz 9
Quiz 10
Quiz 11
Quiz 12
Quiz 13
Quiz 14
Quiz 15
Binomial Theorem Questions and Answers
| Binomial Theorem Quiz Question | Answer |
|---|---|
| The sum of coefficient of integral powers of $$x$$ in the binomial expansion of $$(1-2\sqrt x)^{50}$$ is : | $$\dfrac {1}{2}(3^{50}+1)$$ |
| If $$(2+\dfrac {x}{3})^{55}$$ is expanded in the ascending powers of $$x$$ and the coefficients of powers of $$x$$ in two consecutive terms of the expansion are equal, then these terms are : | $$8^{th}$$ and $$9^{th}$$ |
| If the sum of odd numbered terms and the sum of even numbered terms in the expansion of $$(x + a)^{n}$$ are $$A$$ and $$B$$ respectively, then the value of $$(x^{2} - a^{2})^{n}$$ is | $$A^{2} - B^{2}$$ |
| $${ _{ }^{ 15 }{ C } }_{ 3 }+{ _{ }^{ 15 }{ C } }_{ 5 }+....+{ _{ }^{ 15 }{ C } }_{ 15 }$$ will be equal to | $${2}^{14}-15$$ |
| Find the sum of coefficient of middle terms of the expansion $$\left(3x-\dfrac{x^3}{6}\right)^7$$: | $$\dfrac {595}{48}$$ |
| The total number of terms in the expansion of $$(x+y)^{50}+(x-y)^{50}$$ is | $$26$$ |
| In the expansion of $$(\sqrt 2+\sqrt [3]{5})^{20}$$ the number of rational terms will be: | $$4$$ |
| What is $$\displaystyle\sum _{ r=0 }^{ n }{ C\left( n,r \right) } $$ equal to? | $${2}^{n}$$ |
| The number of rational terms in the expansion of $$(9^{1/4} + 8^{1/6})^{1000}$$ is: |
$$501$$ |
| The $$3rd$$ term of $${\left( {3x - \dfrac{{{y^3}}}{6}} \right)^4}$$ is | $$^4{C_2}{\left( {3x} \right)^2}{\left( { - \dfrac{{{y^3}}}{6}} \right)^2}$$ |
Complex Numbers And Quadratic Equations Class 11 Engineering Maths MCQ Quiz
Complex Numbers And Quadratic Equations Questions and Answers
| Complex Numbers And Quadratic Equations Quiz Question | Answer |
|---|---|
| Express $$\dfrac{1}{(1 - cos \theta + 2 i sin \theta)}$$ in the form $$x + iy$$ | $$\left(\displaystyle \frac{1}{5 + 3 cos \theta}\right) + \left(\displaystyle \frac{-2 cot \theta/2}{5 + 3 cos \theta}\right)i$$ |
| If $$z = x + iy$$ and $$\omega = \dfrac{(1 -iz)}{(z-i)}$$, then $$\left|\omega\right| = 1$$ implies that in the complex plane | z lies on the real axis |
| If $$a, b$$ and $$c$$ are real numbers then the roots of the equation $$(x - a)(x - b) + (x - b)(x - c) + (x - c)(x - a) = 0$$ are always | Real |
| If $$(x+iy)(2-3i)=4+i$$ then (x, y) = | $$\left ( \dfrac{5}{13},\dfrac{14}{13} \right )$$ |
| If $$z =3+5i$$, then $$z^3+z+198=$$ | $$3 + 15i$$ |
| If $$z=2-3i$$ then $$z^2-4z+13=$$ | $$0$$ |
| The complex number $$\displaystyle \frac{1+2i}{1-i}$$ lies in the quadrant : |
II |
| $$\sqrt{-3}\sqrt{-75}=$$ | $$-15$$ |
| The sum of two complex numbers $$a + ib$$ and $$c +id$$ is a real number if | $$b + d = 0$$ |
| The locus of complex number z such that z is purely real and real part is equal to - 2 is | The point (-2, 0) |
Conic Sections Class 11 Engineering Maths MCQ Quiz
Conic Sections Questions and Answers
| Conic Sections Quiz Question | Answer |
|---|---|
| If the lines $$2\mathrm{x}+3\mathrm{y}+1=0$$ and $$\mathrm{3x}- \mathrm{y}-4=0$$ lie along diameters of a circle of circumference $$ 10\pi$$, then the equation of the circle is: |
$$\mathrm{x}^{2}+\mathrm{y}^{2}-2\mathrm{x}+2\mathrm{y}-23=0$$ |
The circle $$x^{2}+y^{2}-8x=0$$ and hyperbola $$\dfrac{x^{2}}{9}-\dfrac{y^{2}}{4}=1$$ intersect at the points $$A$$ and $$B$$. then the equation of the circle with $$AB$$ as its diameter is |
$$x^{2}+y^{2}-12x+24=0$$ |
| The length of the latus rectum of the parabola $$169 \left[(x-1)^2+(y-3)^2\right]=(5x-12y+17)^2$$ is: | $$\displaystyle \frac { 28 }{ 13 } $$ |
| Equation of the ellipse in its standard form is $$\displaystyle \frac{x^2}{a^2}-\frac{y^2}{b^2}=1$$ | False |
| The equation of the circle touching $$x = 0, y = 0$$ and $$x = 4$$ is | $$x^2 + y^2 - 4x - 4y + 16 = 0$$ |
| The radius of the circle with center (0,0) and which passes through (-6,8) is | 10 |
| Assertion is incorrect but Reason is correct | |
| The equation of circle with its centre at the origin is $$x^2+y^2=r^2$$ | True |
| Which of the following equations of a circle has center at (1, -3) and radius of 5? | $$\displaystyle \left ( x-1 \right )^{2}+\left ( y+3 \right )^{2}=25$$ |
| Determine the area enclosed by the curve $$\displaystyle x^{2}-10x+4y+y^{2}=196$$ | $$225\pi$$ |
Introduction To Three Dimensional Geometry Class 11 Engineering Maths MCQ Quiz
Introduction To Three Dimensional Geometry Questions and Answers
| Introduction To Three Dimensional Geometry Quiz Question | Answer |
|---|---|
| Distance between $$A(4,5,6)$$ from origin $$O$$ is | $$\sqrt {77}$$ |
In the given figure, co-ordinates of the midpoint of $$AB$$ are  |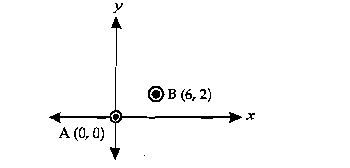
$$(3,1)$$ |
| Name three undefined terms. |
All of the above |
State the following statement is True or False If two distinct lines are intersecting each other in a plane then they cannot have more than one point in common. |
True |
| Distance between the points $$(12,4,7)$$ and $$(10,5,3)$$ is | $$\sqrt{21}$$ |
| The three planes divides the space into | eight parts |
| A point on XOZ-plane divides the join of $$(5, -3, -2)$$ and $$(1, 2, -2)$$ at | $$\left(\displaystyle\frac{13}{5}, 0, -2\right)$$ |
| In three dimensions, the coordinate axes of a rectangular cartesian coordinate system are | three mutually perpendicular lines |
| If G is centroid of $$\triangle ABC$$, then | $$3\overrightarrow {G}=\overrightarrow {a}+ \overrightarrow {b}+\overrightarrow {c}$$ |
| Find the distance between $$(12,3,4)$$ and $$(4,5,2)$$ | $$\sqrt {72}$$ |
Limits And Derivatives Class 11 Engineering Maths MCQ Quiz
Quiz 1
Quiz 2
Quiz 3
Quiz 4
Quiz 5
Quiz 6
Quiz 7
Quiz 8
Quiz 9
Quiz 10
Quiz 11
Quiz 12
Quiz 13
Quiz 14
Quiz 15
Quiz 16
Limits And Derivatives Questions and Answers
| Limits And Derivatives Quiz Question | Answer |
|---|---|
| Differentiation gives us the instantaneous rate of change of one variable with respect to another. | True |
| The value of '$$a$$' in order $$f(x)=\sqrt{3}\sin x-\cos x -2ax+b$$ decrease for all real values of $$x$$, is given by | $$a>1$$ |
| $$\displaystyle \frac{d(\tan x.)}{dx}$$ | $$\sec^2 x$$ |
| $$\displaystyle \frac{d(\sin x)}{dx}.$$ | $$\cos x$$ |
| Differentiate $$\displaystyle \cos x$$ |
$$\displaystyle -\sin x$$ |
| $$\displaystyle \dfrac{d}{dx} \sec x=$$ | $$\sec x \tan x$$ |
State if the given statement is True or False Derivative of $$y= \cos x$$ with respect to $$x$$ is $$\sin x$$. |
False |
| For instantaneous speed, the distance traveled by the object and the time taken are both equal to zero. | False |
| Derivative of $$2\tan x - 7\sec x$$ with respect to $$x$$ is: |
$$\sec x (2 \sec x - 7 \tan x)$$ |
| $$\displaystyle \frac{d}{dx}(\sin^{2}x)$$ | $$sin2x$$ |
Linear Inequalities Class 11 Engineering Maths MCQ Quiz
Linear Inequalities Questions and Answers
| Linear Inequalities Quiz Question | Answer |
|---|---|
Which equation has the solution shown on the number line? |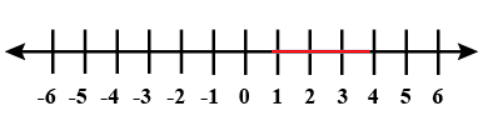
$$1\leq x < 4$$. |
| Ordered pair that satisfy the equation $$x + y + 1 < 0$$ is: | Both (B) and (C) |
| Which of the following number line represents the solution of the inequality $$15 < 4x + 3 \le 31$$ ?  |
|
Which equation has the solution shown on the number line? |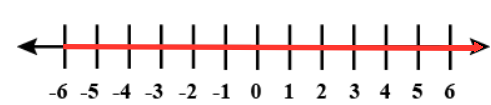
$$x \geq -6$$ |
| If you multiply an inequality by a negative number, when should you reverse the inequality symbol? | Always |
Which equation has the solution shown on the number line? |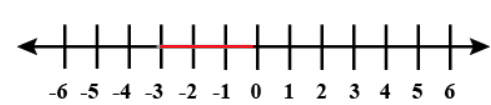
$$-3\leq x < 0 $$. |
| Find solution of following inequality also show it graphically. $$x<5,x\in W$$. |
|
| Solve the following inequality and show it graphically: $$-2<x+3<5,x\in Z$$ |
|
| Which of the following number line represents the solution of the inequality $$2x+1 \ge 9$$? | |
| Find the solution of following inequality, also show it graphically: $$x<4,x\in R$$ |
Mathematical Reasoning Class 11 Engineering Maths MCQ Quiz
Mathematical Reasoning Questions and Answers
| Mathematical Reasoning Quiz Question | Answer |
|---|---|
| The contrapositive of the following statement, "If the side of a square doubles, then its area increases four times"' is: | If the area of a square does not increase four times, then its side is not doubled |
| Consider the following two statements: P: If $$7$$ is an odd number, then $$7$$ is divisible by $$2$$. Q: If $$7$$ is a prime number, then $$7$$ is an odd number. If $$V_{1}$$ is the truth value of the contrapositive of P and $$V_{2}$$ is the truth value of contrapositive of Q, then the ordered pair $$(V_{1}, V_{2})$$ equals: |
$$(F, T)$$ |
| The Boolean expression $$( ( p \wedge q ) \vee ( p \vee \sim q ) ) \wedge ( \sim p \wedge \sim q )$$ is equivalent to : | $$(\sim { p })\wedge (\sim { q })$$ |
| The negation of $$\sim s \vee (\sim r\wedge s)$$ is equivalent to | $$S\wedge\ r$$ |
| The contrapositive of the statement 'If I am not feeling well, then I will go to the doctor' is: | If I will not go to the doctor, then I am feeling well |
| The contrapositive of the statement 'I go to school if it does not rain' is: | If I do not go to school, it rains. |
| The contrapositive of the statement "If it is raining, then I will not come", is : | If I will come, then it is not raining. |
| The contrapositive of "If $$x\in A\cup B$$, then $$x\in A$$ or $$x\in B$$", is |
If $$x\not\in A$$ and $$x\not\in B$$, then $$x\not\in A\cup B$$. |
| Earth is a planet. Choose the option that is a negation of this statement. |
Earth is not a planet |
| The converse of "if $$x\in A\cap B$$ then $$x\in A$$ and $$x\in B$$", is |
If $$x\in A$$ and $$x\in B$$, then $$x\in A\cap B$$. |
Permutations And Combinations Class 11 Engineering Maths MCQ Quiz
Quiz 1
Quiz 2
Quiz 3
Quiz 4
Quiz 5
Quiz 6
Quiz 7
Quiz 8
Quiz 9
Quiz 10
Quiz 11
Quiz 12
Quiz 13
Quiz 14
Permutations And Combinations Questions and Answers
| Permutations And Combinations Quiz Question | Answer |
|---|---|
| If $$\displaystyle { S }_{ { n } }=\sum _{ { r }=0 }^{ { n } } \frac { 1 }{ ^{ n }{ { C }_{ r } } } $$ and $$\displaystyle { t }_{ { n } }=\sum _{ { r }=0 }^{ { n } } \frac { { r } }{ ^{ n }{ { C }_{ r } } } $$, then $$\displaystyle \frac { { t }_{ { n } } }{ { s }_{ { n } } } =$$ | $$\displaystyle \frac{1}{2}{n}$$ |
| The value of $$^{50}C_{4}+ \sum _{r=1}^{6}\ ^{56-r}C_{3}$$ is | $$^{56}C_{4}$$ |
| If all the words, with or without meaning, are written using the letters of the word QUEEN and are arranged as in English dictionary, then the position of the word QUEEN is. | $$46^{th}$$ |
| Statement-l: $$\displaystyle \sum_{r=0}^{n}(r+1)^{n}C_{r}=(n+2)2^{n-1}$$ Statement-2: $$\displaystyle \sum_{r=0}^{n}(r+1)^{n}C_{r}x^{r}=(1+x)^{n}+nx(1+x)^{n-1}$$ |
Statement-1 is true, Statement-2 is true; Statement-2 is a correct explanation for Statement-1. |
| The total number of 4-digit numbers in which the digits are descending order is | $${ _{ }^{ 10 }{ C } }_{ 4 }$$ |
| An automobile dealer provides motorcycles and scooters in three body patterns and $$4$$ different colors each. The number of choices open to a customer is | $$4\times 3\times 2$$ |
| There are $$'m'$$ copies each of $$'n'$$ different books in a university library. The number of ways in which one or more than one book can be selected is | $$(m+1)^n - 1$$ |
| In a crossword puzzle, $$20$$ words are to be guessed of which $$8$$ words have each an alternative solution also. The number of possible solutions will be | $$ 256 $$ |
| $$ ^xC_7 - ^xC_5 = 0 $$, then $$x = $$ | 12 |
| $$15$$ buses operate between Hyderabad and Tirupathi.The number of ways can a man go to Tirupathi from Hyderabad by a bus and return by a different bus is | $$210$$ |
Principle Of Mathematical Induction Class 11 Engineering Maths MCQ Quiz
Principle Of Mathematical Induction Questions and Answers
| Principle Of Mathematical Induction Quiz Question | Answer |
|---|---|
| Statement-l: For every natural number $$n\geq 2,\ \displaystyle \frac{1}{\sqrt{1}}+\frac{1}{\sqrt{2}}+\ldots\ldots+\frac{1}{\sqrt{n}}>\sqrt{n}$$. Statement-2: For every natural number $$n\geq 2,\ \sqrt{n(n+1)}<n+1$$. |
Statement-1 is true, Statement-2 is true; Statement-2 is not a correct explanation for Statement-1. |
| Let $$S(k) = 1 + 3 + 5 + .... + (2k - 1) = 3 + k^2$$. Then which of the following is true? | $$S (k)$$ $$\Rightarrow$$ $$S (k + 1)$$ |
| Mathematical Induction is the principle containing the set |
N |
| Let $$P(n)$$ be a statement and $$P(n)=P(n+1) \forall n\in N$$, then $$P(n)$$ is true for what values of $$n$$? |
For all $$n$$ |
| State whether the following statement is true or false. cos x + cos 2x + .... + cos nx = $$\dfrac{cos\left (\dfrac{n \, + \, 1 }{2} \right )x sin \dfrac{nx}{2}}{sin\dfrac{x}{2}}$$ |
True |
| $$1+3+5+....+(2n-1)=n^2$$. | True |
A bag contains $$3$$ red and $$2$$ black balls. One ball is drawn from it at random. Find the probability of drawing red ball is $$\dfrac 35$$ |
True |
| Let $$P(n)$$ be the statement $$"3^n>n"$$. If $$P(n)$$ is true, $$P(n+1)$$ is true. | True |
| Let $$P(n)= 5^{n}-2^{n}$$. $$P(n)$$ is divisible by $$ 3\lambda$$ where $$\lambda$$ and $${n}$$ both are odd positive integers, then the least value of $$n$$ and $$\lambda$$ will be |
$$1$$ |
| For every integer $$n\geq 1, (3^{2^{n}}-1)$$ is always divisible by |
$$2^{n+2}$$ |
Probability Class 11 Engineering Maths MCQ Quiz
Probability Questions and Answers
| Probability Quiz Question | Answer |
|---|---|
| A set $$A$$ is containing $$n$$ elements. A subset $$P$$ of $$A$$ is chosen at random. The set is reconstructed by replacing the elements of $$P$$. A subset $$Q$$ of $$A$$ is again chosen at random. The probability that $$P$$ and $$Q$$ have no common elements is: | $$\left (\dfrac {3}{4}\right )^n$$ |
| If events $$A$$ and $$B$$ are independent and $$P(A)=0.4, P(A\cup B)=0.6$$, then $$P(B)=$$ |
$$\dfrac{1}{3}$$ |
| Let $$S$$ and $$T$$ are two events defined on a sample space with probabilities $$P(S)=0.5,P(T)=0.69,P(S/T)=0.5$$ Event $$S$$ and $$T$$ are | independent |
| If $$P(A)=\dfrac {1}{8}$$ and $$P(B)=\dfrac {5}{8}.$$ Which of the following statements is not correct? | $$P(\overline A\cap B)\leq \dfrac {5}{8}$$ |
| If events $$A$$ and $$B$$ are independent and $$P(A)=0.15, P(A\cup B)=0.45,$$ then $$P(B)$$ is: | $$\dfrac {6}{17}$$ |
| If events $$A$$ and $$B$$ are independent and $$P(A)=0.15, P(A\cup B)=0.45$$, then $$P(B)=$$ | $$\dfrac {6}{17}$$ |
| A bag contains four tickets marked with $$112, 121, 211, 222$$, one ticket is drawn at random from the bag. Let $$E_i(i=1, 2, 3)$$ denote the event that $$i^{th}$$ digit on the ticket is $$2$$ then : | $$E_3$$ and $$E_1$$ are independent |
| A coin is tossed 15 times and the outcomes are recorded as follows: $$H\, T\, T\, H\, T\, H\, H\, H\, T\, T\, H\, T\, H\, T\, T$$ The chance of occurrence of a head is $$50$$ per cent. |
False |
| One or more outcomes of an experiment make an event. | True |
| E and F are two independent events,The probability that both E and F happen is 1/12 and the probability that neither E nor F happens is 1/2.Then, | $$P(E)=1/3,P(F)=1/4$$ |
Relations And Functions Class 11 Engineering Maths MCQ Quiz
Relations And Functions Questions and Answers
| Relations And Functions Quiz Question | Answer |
|---|---|
| For nonnegative integers s and r, let $$\begin{pmatrix} s \\ r \end{pmatrix}$$ $$ \left\{\begin{matrix} \dfrac{s!}{r!(s - r)!} & if \ r \le s\\ 0 & if \ r > s \end{matrix}\right.$$ For positive integers m and n, let $$g(m, n) = \sum_{p = 0}^{m+n} \dfrac{f(m, n, p)}{\begin{pmatrix} n + p \\ p \end{pmatrix}}$$ where for any nonnegative integer p, $$f(m, n, p) = \sum_{i = 0}^p \begin{pmatrix} m \\ i \end{pmatrix} \begin{pmatrix} n + i \\ p \end{pmatrix} \begin{pmatrix} p + n \\ p - i\end{pmatrix}$$. Then which of the following statements is/are TRUE? |
$$g(2m, 2n) = (g(m, n))^2$$ for all positive integers $$m, n$$ |
| $$A$$ and $$B$$ are two sets having $$3$$ and $$4$$ elements respectively and having $$2$$ elements in common. The number of relations which can be defined from $$A$$ to $$B$$ is: | $$2^{12}$$ |
| Let $$R$$ be a relation from a set $$A$$ to a set $$B$$, then: | $$R\subseteq A\times B$$ |
| If $$\displaystyle a - \frac{1}{a} = 8$$ and $$\displaystyle a \neq 0$$; find :$$\displaystyle a + \frac{1}{a}$$. | $$\displaystyle \pm 2 \sqrt{17}$$ |
| If $$f(x)=3x+1, g(x)= x^{3}+2,$$ then $$(f+g)(0)-f(0)g(0)=$$ |
$$1$$ |
Let $$x$$ be a real number $$\left [ x \right ]$$ denotes the greatest integer function, and $$\left \{ x \right \}$$ denotes the fractional part and $$(x)$$ denotes the least integer function,then solve the following. $$\left [ 2x \right ]-2x=\left [ x+1 \right ]$$ |
$$\left \{ -1,\displaystyle-\frac{1}{2} \right \}$$ |
| If $$A=\left \{x:x^2-3x+2=0\right \}$$ and $$B=\left \{x:x^2+4x-5=0\right \}$$ then the value of A-B is | $$\left \{2\right \}$$ |
| If R={$$(x,y)/3x+2y=15$$ and x,y $$\displaystyle \epsilon $$ N}, the range of the relation R is________ | $$\{3,6\}$$ |
| If $$x$$ co-ordinate of a point is $$2$$ and $$y$$ co-ordinate is $$0$$, then ordered pair for its coordinate on $$XY$$ plane is | $$(2, 0)$$ |
| If $$(x+3, 4-y)=(1,7)$$, then $$(x-3, 4+y)$$ is equal to | $$(-5, 1)$$ |
Sequences And Series Class 11 Engineering Maths MCQ Quiz
Sequences And Series Questions and Answers
| Sequences And Series Quiz Question | Answer |
|---|---|
| The value of $$\displaystyle \sum_{r = 16}^{30}{ (r + 2)(r - 3) } $$ is equal to : | $$7780$$ |
Let $$\mathrm{a}_{1},\ \mathrm{a}_{2},\ \mathrm{a}_{3},\ \ldots,\ \mathrm{a}_{11}$$ be real numbers satisfying $$\mathrm{a}_{1}=15,27-2\mathrm{a}_{2}>0$$ and $$\mathrm{a}_{\mathrm{k}}=2\mathrm{a}_{\mathrm{k}-1}-\mathrm{a}_{\mathrm{k}-2}$$ for $$\mathrm{k}=3,4,\ \ldots, 11.$$ If $$\displaystyle \frac{a_{1}^{2}+a_{2}^{2}+\ldots+a_{11}^{2}}{11}=90$$, then the value of $$\displaystyle \frac{a_{1}+a_{2}+\ldots+a_{11}}{11}$$ is equal to |
$$0$$ |
| $$x\left (x + \dfrac {1}{x}\right )^{2} + \left (x^{2} + \dfrac {1}{x^{2}}\right )^{2} + \left (x^{3} + \dfrac {1}{x^{3}}\right )^{2}$$ .... upto $$n$$ terms is | $$\dfrac {x^{2n} - 1}{x^{2} - 1}\times \dfrac {x^{2n + 2} + n}{x^{2n}} + 2n$$ |
| Which letter will be the fifth from the right if the first and second, the third and fourth and so on are interchanged among each other in the word "COMPANIONATE"? | I |
| If the sum of the first n natural numbers is 1/5 times the sum of the their squares, then the value of n is | 7 |
| Mean of the first $$n$$ terms of the A.P. $$a, (a + d), (a + 2d), ........$$ is | $$\displaystyle a + \frac{(n - 1)d}{2}$$ |
| The sum of $$n$$ terms of the series whose $$n^{th}$$ term is $$n(n + 1)$$ is equal to. | $$\dfrac {n(n + 1)(n + 2)}{3}$$ |
| The sum of nn is equal to | $$\dfrac {1}{4}n(n+1)(n+2)(n+3)$$ |
| In numbers from $$1 \ to\ 100$$ the digit "$$0$$" appears ____________times. | $$11$$ |
The spread sheet on the right contains 20 cells. A cell In a spread sheet can be identified fIrst by the column letter and then by the row number. For example,. the number 10 is found in cell CIf the number in cell A3 =B4-3(E2 +D4) then which of the following must be the number in cell E2? |
-12 |
Sets Class 11 Engineering Maths MCQ Quiz
Sets Questions and Answers
| Sets Quiz Question | Answer |
|---|---|
| If P is true and Q is false, then Bi-implication of p and q is | False |
| For any two sets $$ A$$ and $$B, A = B$$ is equivalent to |
$$A \cup C = B \cup C$$ and $$A \cap C = B \cap C $$ for any set $$C$$ |
| If A = {1, 2, 3, 4, 5, 6, 7, 8} and B = {1, 3, 5, 7}, then find $$A - B$$ and $$A \cap B$$ |
{2, 4, 6, 8} and {1, 3, 5, 7} |
Suppose $${ A }_{ 1 },{ A }_{ 2 },...,{ A }_{ 30 }$$ are thirty sets, each with five elements and $${ B }_{ 1 },{ B }_{ 2 },...,{ B }_{ 30 }$$ are $$n$$ sets ecah with three elements. Let $$\displaystyle \bigcup _{ i=1 }^{ 30 }{ { A }_{ i }= } \bigcup _{ j=1 }^{ n }{ { B }_{ j } } =S$$ If each element of $$S$$ belongs to exactly ten of the $${ A }_{ i }'s$$ and exactly none of the $${ B }_{ j }'s$$ then $$n=$$ |
45 |
| A $$\bigcup \phi = $$ |
A |
| If $$A=\left \{ 1,2,3 \right \};B=\left \{ 2,3,4 \right \},$$ then $$A-B=$$ | $$\left \{ 1 \right \}$$ |
Let $$n$$ be a fixed positive integer. Let a relation $$R$$ defined on $$I$$ (the set of all integers) as follows: $$aRb$$ iff $$n/(a-b)$$, that is, iff $$a-b$$ is divisible by $$n$$, then, the relation $$R$$ is |
An equivalence relation |
| Let $$A=\left\{ 1,2,3,4 \right\} $$ and $$B=\left\{ 2,3,4,5,6 \right\} $$ then $$A \triangle\ B$$ is equal to | $$\left\{ 1,5,6 \right\} $$ |
| If X $$=$$ (multiples of 2), Y $$=$$ (multiples of 5), Z $$=$$ (multiples of 10), then $$X \cap ( Y \cap Z )$$ is equal to |
multiples of 10 |
In a locality two-thirds of the people have cable TV one-fifth have Dish TV and one-tenth have both What is the fraction of people having either cable TV or Dish TV? |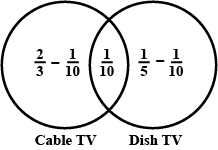
$$\displaystyle \frac{2}{3}$$ |
Statistics Class 11 Engineering Maths MCQ Quiz
Quiz 1
Quiz 2
Quiz 3
Quiz 4
Quiz 5
Quiz 6
Quiz 7
Quiz 8
Quiz 9
Quiz 10
Quiz 11
Quiz 12
Quiz 13
Quiz 14
Quiz 15
Statistics Questions and Answers
| Statistics Quiz Question | Answer |
|---|---|
| The mean of $$x, y, z$$ is $$y$$, then $$x+z =$$ | $$2y$$ |
| Value of the middle-most observation(s) is called: | Median |
| The ________ is the difference between the greatest and the least value of the variate. | Range |
| Median divides the total frequency into _____ equal parts. | $$2$$ |
| The modal value is the value of the variate which divides the total frequency into two equal parts. | False |
| The difference between the maximum and the minimum obervations in data is called the ____________. | range of the data |
| The variance is the _______ of the standard deviation. | Square |
| State true or false: The mode is the most frequently occurring observation. |
True |
| A child says that the median of $$3, 14, 18, 20, 5$$ is $$18$$. What concept does the child missed about finding the median? |
The order of numbers. |
| Median of $$15, 28, 72, 56, 44, 32, 31, 43\ and\ 51\ is\ 43.$$ | True |
Straight Lines Class 11 Engineering Maths MCQ Quiz
Quiz 1
Quiz 2
Quiz 3
Quiz 4
Quiz 5
Quiz 6
Quiz 7
Quiz 8
Quiz 9
Quiz 10
Quiz 11
Quiz 12
Quiz 13
Quiz 14
Straight Lines Questions and Answers
| Straight Lines Quiz Question | Answer | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Area of a triangle formed by the points A(5, 2), B(4, 7) and C(7, -4) is _____. | 2 sq. units | ||||||||||
| $$A(0, 0), B(7, 2), C(7, 7)$$ and $$D(2, 7)$$ are the vertices of a quadrilateral. The respective slopes of diagonals $$AC$$ and $$BD$$ are |
$$1, -1$$ | ||||||||||
Let $$\mathrm{P}(\mathrm{x}_{1},\mathrm{y}_{1})\mathrm{b}\mathrm{e}$$ any point on the cartesian plane then match the following lists:
|
$$A - 2, B - 4, C - 3$$ | ||||||||||
| If A and B are the points $$(-6,7)$$ and $$(-1,-5)$$ respectively, then the distance 2AB is equal to |
$$26$$ | ||||||||||
| The vertices of a triangle are $$(-2,0) ,(2,3)$$ and $$(1, -3)$$ , then the type of the triangle is | scalene | ||||||||||
| The vertices P, Q, R, and S of a parallelogram are at (3,-5), (-5,-4), (7,10) and (15,9) respectively The length of the diagonal PR is | $$\displaystyle \sqrt{241}$$ | ||||||||||
| The distance between (a,-b) and (-a, -b) is given by | 2a | ||||||||||
| The area of a triangle whose vertices are $$(a, c+a), (a, c) $$ and $$(-a, c-a) $$ are | $$\displaystyle a^{2}$$ | ||||||||||
| Equation of the line y = 0 represents | x-axis | ||||||||||
| The points $$(-2, -1), (1, 0),(4, 3),$$ and $$(1, 2)$$ are the vertices | Parallelogram |
Trigonometric Functions Class 11 Engineering Maths MCQ Quiz
Quiz 1
Quiz 2
Quiz 3
Quiz 4
Quiz 5
Quiz 6
Quiz 7
Quiz 8
Quiz 9
Quiz 10
Quiz 11
Quiz 12
Quiz 13
Quiz 14
Quiz 15
Quiz 16
Quiz 17
Trigonometric Functions Questions and Answers
| Trigonometric Functions Quiz Question | Answer |
|---|---|
| The number of $$x\epsilon [0, 2\pi]$$ for which $$|\sqrt {2\sin^{4} x + 18\cos^{2}x} - \sqrt {2\cos^{4} x + 18\sin^{2} x}| = 1$$ is: | $$8$$ |
| For $$x \in (0, \pi)$$, the equation $$\sin x + 2 \sin 2 x - \sin 3x =3$$, has | no solution |
| If $$0^{\circ}\leq \theta\leq 90^{\circ}$$ and $$\sqrt{3} tan\theta - sec\theta=1$$, then $$\theta$$ has the value |
$$60^{\circ}$$ |
| The of value of $$\quad \sin60^{\small\circ}\cos30^{\small\circ} + \cos60^{\small\circ}\sin30^{\small\circ}$$ is equal to |
$$\sin 90^0$$ |
| Which one of the following relations is true ? |
$$\sec^2 \theta - \tan^2 \theta=1$$ |
| Which is true for all values of $$\theta$$? |
$$\text{cosec}^2\theta- \cot^2 \theta=1$$ |
| If $$0^{\circ} \leq \theta \leq 90^{\circ}$$ and $$\sqrt{2} tan \theta - sec \theta =0$$, then the value of $$(\sqrt{2} sin \theta + 2 tan \theta)$$ is - |
3 |
| The value of $$ 1+ \cot^2 A$$ is |
$$\text{cosec}^2 A$$ |
| $$sin^2x+cos^2x=$$ |
1 |
| The solution of equation $$\cos^2 \theta +\sin \theta+1=0$$ lies in the interval | $$\displaystyle \left ( \frac{5\pi}{4}, \frac{7\pi}{4} \right )$$ |
Maths MCQ Questions for Class 11 - Practice Test with Solutions
Do you want to overcome your drawbacks while attempting the quizzes or MCQ tests like time consumption, approaching questions, etc.? Take the advantage of practicing with MCQExams.com MCQ Questions for Standard 11 Maths Test. As it is a time-based approach and also provides answers to all questions.
One should practice the MCQs in this way for a better assessment of their preparation level. All chapters CBSE Class 11 Maths MCQ Quiz Questions with Solutions PDF free download links are available for easy access & quick reference.
How to Use MCQExams.com Chapterwise 11th Maths MCQ Interactive Quiz?
Guys do you love to share your practice hacks and tips with your friends? If yes, then our 11tth standard CBSE Maths MCQ interactive quiz help you do the same. Excited to know the process then jump into the below steps right away:
- Go with the respective chapter class 11 Maths MCQ quiz link from the above
- Now, you will find the MCQ quiz boxes for the Binomial Theorem chapter along with the interactive quiz windows.
- Click on the CBSE 11th Class Binomial Theorem MCQ Interactive Quiz and it will redirect you to another window where it displays the questions with options in stories format.
- Answer the question one after another and learn the answers right away this helps you to do a quick assessment of your knowledge.
- You can also share this cool MCQ Interactive Quiz Questions of Plus One Maths topicwise with your friends by just tapping on the send arrow located at the top left corner of the story.
- After clicking the button, you can opt for the copy link option and easily paste the link on your friend's chat or else in your whatsapp story too. Isn’t it cool!!
- Keep passing this interesting approach of practicing Plus One CBSE Maths Binomial Theorem MCQ Questions to your co-students and help them in attempting the entrance exams like JEE & NEET.