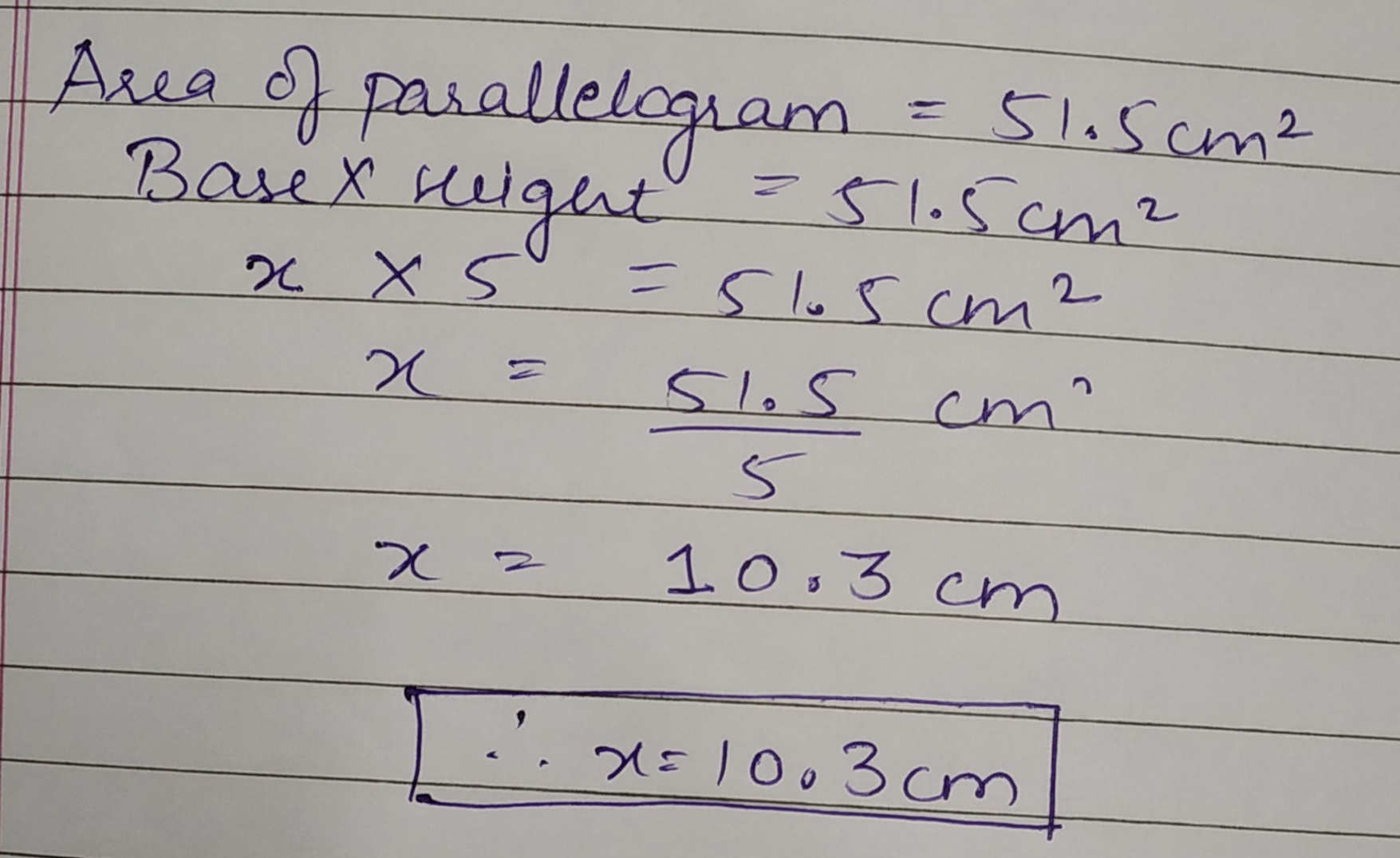Areas Of Parallelograms And Triangles - Class 9 Maths - Extra Questions
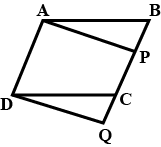
The area of parallelogram ABCD is 36 cm2. Calculate the height of parallelogram ABEF if AB = 4.2 cm.
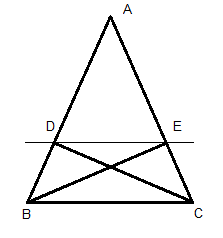
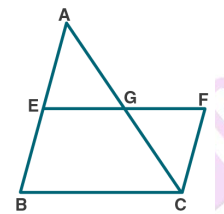
In the given figure, DE∥BC and DE:BC=4:5. Calculate the ratio of the areas of △ADE and the trapezium △CEDB.
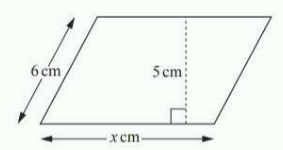
The area of this parallelogram is 51.5cm2.
Work out the value of x.
In the given figure, DE∥BC. If DE=5 cm,BC=10 cm and ar(△ADE)=20 cm2, find the area of △ABC.
Find the area of following parallelograms:

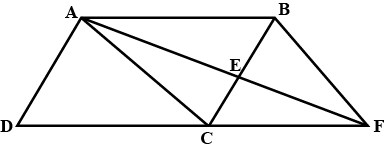
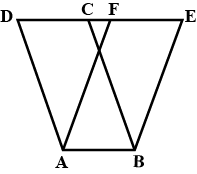
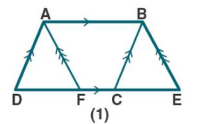
In the given figure,
AD||BE||CF.
Prove that :
Area(△AEC)=Area(△DBF)
The given figure shows a pentagon ABCDE .EG drawn parallel to DA meets BA producedat G and CF drawn parallel to DB meets AB produced at F .
Prove that the area of pentagon ABCDE is equal to the area of triangle GDF .
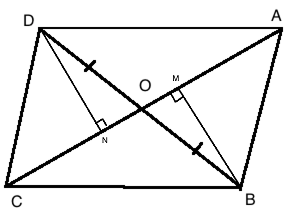
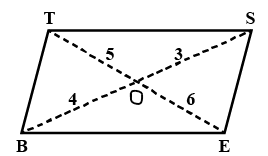
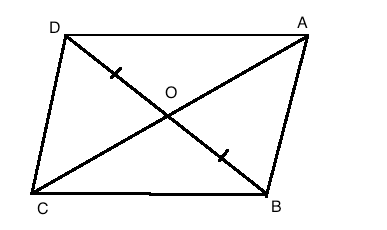
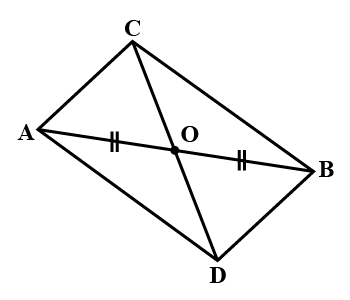
In the given figure, the diagonals AC and BD intersect at point O. If OB = OD and AB//DC, show that :Area(ΔDCB)=Area(ΔACB).
P and Q are any two points lying on the sides DC and AD respectively of a parallelogram ABCD. Show that area (ΔAPB)=area(ΔBQC)
D, E and F are the mid-points of the sides BC, CA and AB respectively of triangle ABC. Prove that area of BDEF is half the area of ΔABC.
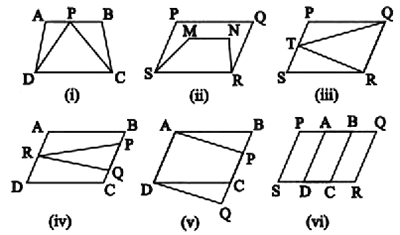
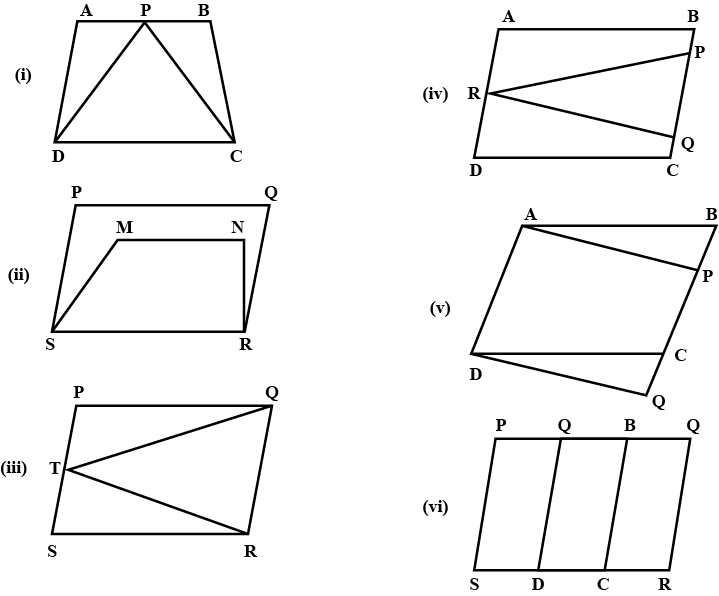
Which of the following figures lie on the same base and between the same parallels. In such a case, write the common base and the two parallels
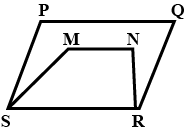
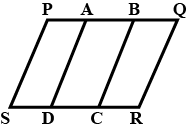
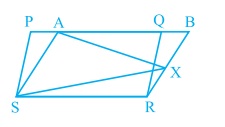
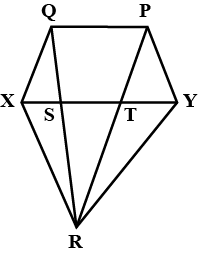
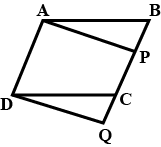
In fig, PQRS and ABRS are parallelogram and X is any point on side BR. Show that
(i) area(PQRS)=area(ABRS)
(ii) area(AXS)=12area(PQRS)
(i) area(PQRS)=area(ABRS)
(ii) area(AXS)=12area(PQRS)
In the given figure, DE is parallel BC.
Show that :Area (ΔBOD) = Area (ΔCOE)

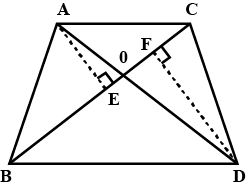
If E, F, G and H are respectively the midpoints of the sides AB, BC, CD and AD of a parallelogram ABCD, show that ar(EFGH)=12ar(ABCD)

Parallelogram ABCD and rectangle ABEF are on the same base AB and have equal areas. Show that the perimeter of the parallelogram is greater than that of the rectangle.
D and E are points on sides AB and AC respectively of △ABC such that area(DBC)=area(EBC). Prove that DE∥BC
A villager Itwarri has a plot of land of the shape of a quadrilateral. The Gram Panchayat of the village decided to take over some portion of his plot from one of the corners to construct a Health Centre. Itwarri agrees to the above proposal with the condition that he should be given equal amount of land in lieu of his land adjoining his plot so as to form a triangular plot. Explain how this proposal will be implemented
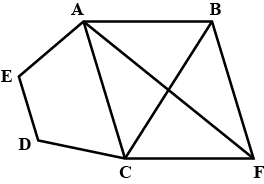
In fig, ABCDE is a pentagon. A line through B parallel to AC meets DC produced at F. Show that
(i) ar(ACB)=ar(ACF)
(ii) ar(AEDF)=ar(ABCDE)

In Fig, AP∥BQ∥CR. Prove that ar(AQC)=ar(PBR)
In the figure, ABCD is a quadrilateral. AC is the diagonal and DE||AC and also DE meets BC produced at E. Show that ar(ABCD)=ar(△ABE).
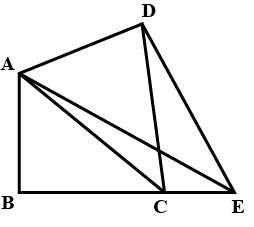
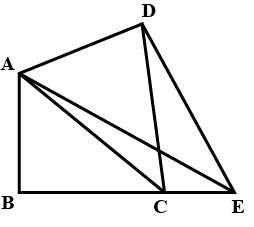
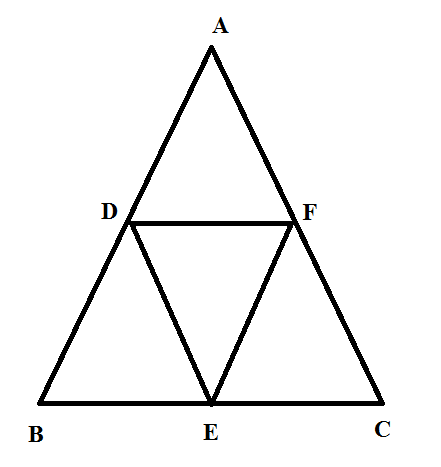
In fig, ABC and BDE are two equilateral triangles such that D is the mid-point of BC. If AE intersects BC at F, show that:
(i) ar(BDE)=14ar(ABC)
(ii) ar(BDE)=12ar(BAE)
(iii) ar(ABC)=2ar(BEC)
(iv) ar(BFE)=ar(AFD)
(v) ar(BFE)=2ar(FED)
(vi) ar(FED)=18ar(AFC)
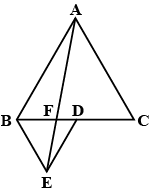
A farmer was having a field in the form of a parallelogram PQRS. She took any point A on RS and joined it to points P and Q. In how many parts the fields is divided? What are the shapes of these parts? The farmer wants to sow wheat and pulses in equal portions of the field separately. How should she do it?
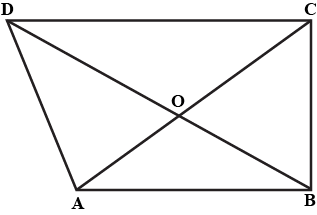
In the figure, diagonals AC and BD of a trapezium ABCD with AB || DC intersect each other at O. Prove that area(\triangle AOD) = area (\triangle BOC).
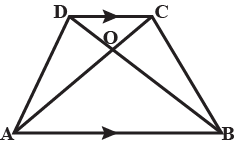
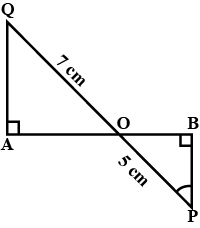
In the given figure drawn, seg BE \perp seg AB and seg BA \perp seg AD. If BE=8 and AD=12, find \dfrac { A\left( \Delta ABE \right) }{ A\left( \Delta BAD \right) } .
The ratio of the areas of two triangles with common base in 4 : 3. Height of the larger triangle is 20 cm, then find the corresponding height of the smaller triangle
In the figure, \triangle ABC and \triangle ABD are two triangles on the same base AB. If line segment CD is bisected by \overline{AB} at O, show that area(\triangle ABC) = area (\triangle ABD).
A villager Ramayya has a plot of land in the shape of a quadrilateral. The grampanchayat of the village decided to take over some portion of his plot from one of the corners to construct a school. Ramayya agrees to the above proposal with the condition that he should be given equal amount of land in exchange of his land adjoining his plot so as to form a triangular plot. Explain how this proposal will be implemented.
Find the number of shot in an incomplete square pile of 16 courses when the number of shot in the upper course is 1005 less than in the lowest course.
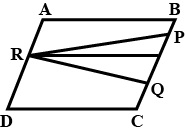
In the given figure , QP || XY, QX || PR and PY || QR. prove that ar(\bigtriangleupQXR) = ar(\bigtriangleupPQR)
In the following figure RP : PK = 3 : 2, then find the value of A(\Delta TRP) : A(\Delta TPK).

ar(\triangle ACB) = ar (\triangle ACF)
In a parallelogram ABCD, AB = 8 cm. The altitudes corresponding to sides AB and AD are respectively 4 m and 5 cm. Find AD.

In a parallelogram ABCD, AB = 10 cm. The altitudes corresponding to the sides AB and AD are respectively 7 cm and 8 cm. Find AD.
A point E is taken on the side BC of a parallelogram ABCD, AE and DC produced to meet at F. Prove that area (\Delta ADF) = Area (ll^m \,ABFC).
Find the permeter of the shaded region if ABCD is a square if sides 21cm and APB & CPD are semicircles.

In\Delta ABC, D E,F, are mid point of side AB, BC and AC reaspectively. If area(CEDF)=24cm^{2}, find area (BEFD):
If base a parallelogram is 8 cm and height is 5 cm then find its area.
In a figure X and Y are the mid point of AC and AB respectively, QP \parallel BC and CYQ and BXP are straight lines. Prove that ar (\triangle ABP)=ar(\triangle ACQ).

How wc can find the area of
Parallelogram = ?
Rhombus = ?
Trapezium = ?
Solve for p: p ^ {2} - 10 p + 25=0
The height of a parallelogram is one-third of its base. If the area of the parallelogram is 108\ cm^2 then find its base and height.
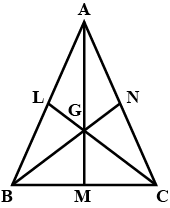
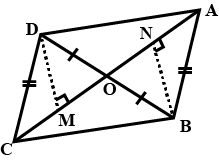
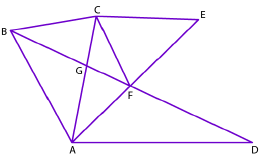
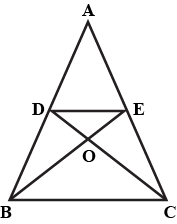
If the medians of a triangle ABC intersect at G, prove that:
ar.\left( {\Delta AGB} \right) = ar.\left( {\Delta AGC} \right) = ar\left( {\Delta BGC} \right) = \dfrac{1}{3}ar\left( {\Delta ABC} \right)
Find the area of rhombus with diagonals 4cm,6cm
Find the area of the quadrilateral whose Base is 5m and Height is 4m
In figure , ABCDE is any pentagon . BP drawn parallel to AC meets DC produced at P and EQ drawn parallel to AD meets CD produced at Q .
Prove that ar(ABCDE) = ar(APQ) .
O is any point on the diagonal PR of parallelogram PQRS. Prove that ar(\triangle PSO)=ar(\triangle PQO)
Does the following figure lie on the same base and between the same parallels? In such a case, write the common base and two parallels.
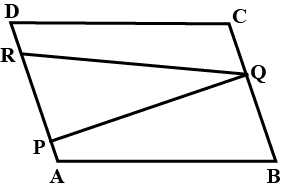
Can the following figures be parallelograms. Justify your answer.
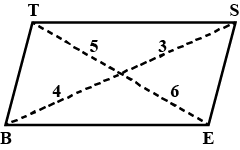
Does the following figure lie on the same base and between the same parallels? In such a case, write the common base and two parallels.
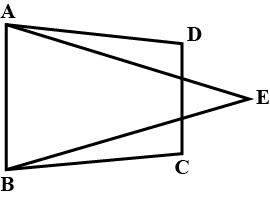
Can the following figures be parallelograms. Justify your answer.
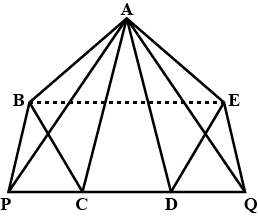
ABC is a triangle and through A, B, C lines are drawn parallel to BC, CA and AB respectively intersecting at P,Q and R. Prove that the perimeter of \Delta PQR is double the perimeter of \Delta ABC.
Does the following figure lie on the same base and between the same parallels? In such a case, write the common base and two parallels.
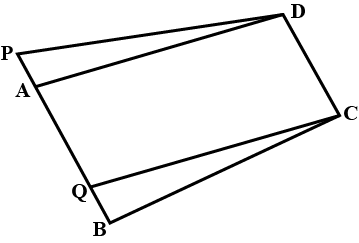
Does the following figure lie on the same base and between the same parallels? In such a case, write the common base and two parallels.
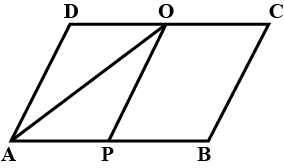
Does the following figure lie on the same base and between the same parallels? In such a case, write the common base and two parallels.
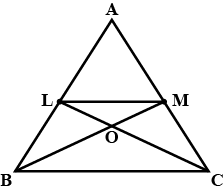
In a \triangle ABC, if L and M are points on AB and AC respectively such that LM||BC.Prove that:
ar(\triangle LOB)=ar (\triangle MOC)
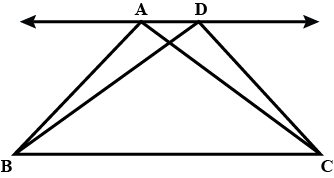
Does the following figure lie on the same base and between the same parallels? In such a case, write the common base and two parallels.

In the adjoining figure, ABCD is a quadrilateral. A line through D, parallel to AC, meets BC produced in P. Prove that ar(\triangle ABP)=ar(quad\ ABCD).
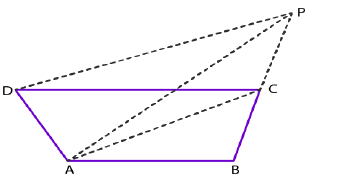
In the adjoining figure, DE||BC. Prove that
ar(\triangle ACD)=ar (\triangle ABE)
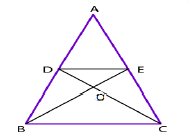
In the adjoining figure, ABCD is a trapezium in which AB||DC and its diagonals AC and BD intersect at O. Prove that ar(\triangle AOD)=ar(\triangle BOC).
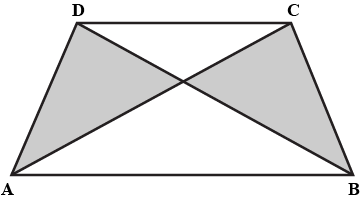
In a trapezium ABCD,\ AB||DC and M is the midpoint of BC. Through M a line PQ|| AD has been drawn meets AB in P and DC produced in Q, as shown in the adjoining figure. Prove that ar(ABCD)=ar(APQD).
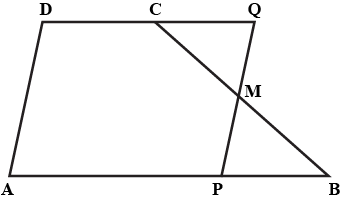
In a triangle ABC, the medians BE and CF intersect at G. Prove that ar(\triangle BCG)=ar(AFGE).
In the adjoining figure, CE || AD and CF || BA. Prove that ar(\triangle CBG)=ar(\triangle AFG).
In the adjoining figure, \triangle ABC and \triangle DBC are on the same base BC with A and D on opposite sides of BC such that ar(\triangle ABC)=ar(\triangle DBC). Show that BC bisects AD.
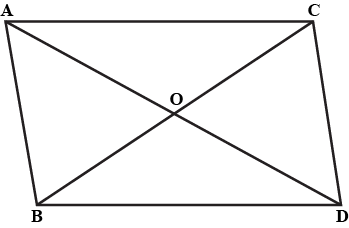
In the adjoining figure, the diagonals AC and BD of a quadrilateral ABCD intersect at O. If BO=OD, prove that ar(\triangle ABC)=ar(\triangle ADC)
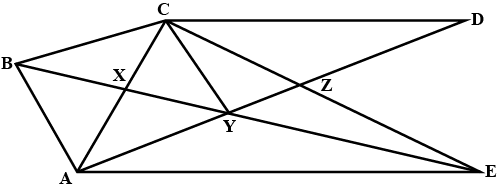
In Fig. 9.24, CD \parallel AE and CY \parallel BA. Prove that ar (\Delta CBX) = ar (\Delta AXY)
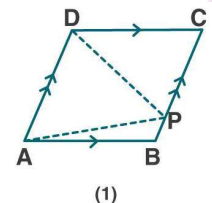
In the figure ( 1 ) given below, A B C D is a parallelogram and P is any point in BC.
Prove that: Area of \triangle \mathrm{ABP}+ area of \Delta \mathrm{DPC}= Area of \triangle \mathrm{APD} .
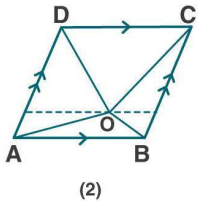
In the figure ( 2 ) given below, O is any point inside a parallelogram ABCD.
Prove that:
(i) area of \Delta \mathrm{OAB}+ area of \Delta \mathrm{OCD}=1 / 2 area of \| \mathrm{gm} \mathrm{ABCD}
(ii) area of \Delta \mathbf{O B C}+ area of \Delta \mathbf{O A D}=1 / 2 area of \| \mathbf{g m} \mathbf{A B C D}
Perimeter of a parallelogram shaped land is 96 \, \text{cm} and its area is 270\ \text{cm}^2. If one of the sides of this parallelogram is 18\, \text{cm}, find the length of the other side. Also, find the lengths of altitudes l and m (Fig).

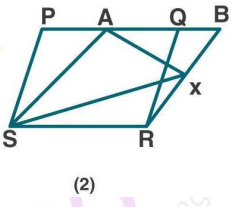
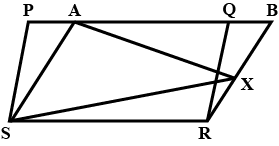
In the figure ( 2 ) given below, PQRS and ABRS are parallelograms and X is any point on the side BR. Show that area of \Delta \mathrm{AXS}=1 / 2 area of \| \mathrm{gm} PQRS.
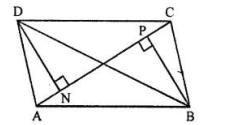
In the given figure, A B C D is a parallelogram. Perpendiculars DN and BP are drawn on diagonal AC. Prove that:
(i) \Delta \mathrm{DCN} \cong \Delta \mathrm{BAP}
(ii) \mathrm{AN}=\mathrm{CP}
In the figure ( 1 ) given below, area of parallelogram A B C D is 29 \mathrm{cm}^{2} . Calculate the height of parallelogram ABEF if \mathrm{AB}=5.8 \mathrm{cm}
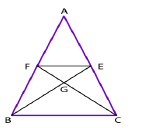
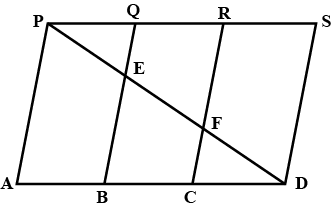
In Fig.9.11, PSDA is a parallelogram. Points Q and R are taken as PS such that PQ = QR = RS and PA \parallel QB \parallel RC. Prove that ar(PQE) = ar (CFD).
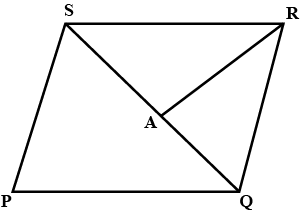
PQRS is a parallelogram whose area is 180\,cm^2 and A is any point on the diagonal QS. The area of \Delta ASR = 90 \,cm^2. Write True or False and justify your answer:
Which of the following figures lie on the same base and between the same parallels. In such a case, write the common base and the two parallels.
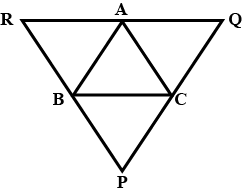
In the figure given, ABCD and AEFG are two parallelograms. Prove that area of \| \mathrm{gm} \mathrm{ABCD}= area of \| \mathrm{gm} AEFG.
In the fig. (3) Given below, ABCD is a parallelogram. P is a point on DC such that area of \Delta \mathbf{D A P}=25 \mathrm{cm}^{2} and area of \Delta \mathbf{B C P}=15 \mathrm{cm}^{2} . Find
(i) area of \| gm A B C D
(ii) DP: PC.

In the fig. ( 2) Given below, the side A B of the parallelogram ABCD is produced toE. A straight line through A is drawn parallel to C E to meet C B produced at F and parallelogram BFGE is Completed prove that area of \| gm \mathrm{BFGE}= Area of \| \mathrm{gm} ABCD.
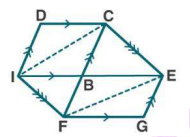
Which of the following figures lie on the same base and between the same parallels. In such a case, write the common base and the two parallels.
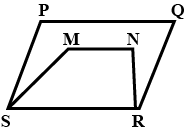
Which of the following figures lie on the same base and between the same parallels. In such a case, write the common base and the two parallels.
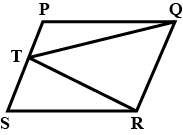
Which of the following figures lie on the same base and between the same parallels. In such a case, write the common base and the two parallels.
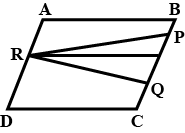
Show that:
A diagonal divides a parallelogram into two triangles of equal area.
ABCD and BCEF are parallelograms. If area of triangle EBC =480\ cm^1, AB=30cm and BC=40cm;
Calculate:
Area of parallelogram ABCD
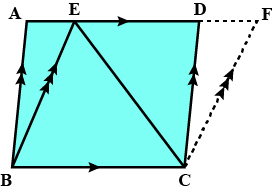
In the adjoining figure, \mathbf{E} is mid-point of the side A B of a triangle A B C and EBCF is a parallelogram. If the area of \Delta \mathrm{ABC} is 25 \mathrm{sq} . units, find the area of \| \mathrm{gm} EBCF.
In figure, PQRS and ABRS are parallelograms and X is any point on side BR. Show that
ar. (PQRS)=ar. ( \square ABRS)
P and Q are any two points lying on the sides DC and AD respectively of a parallelogram ABCD. Show that ar(PAB)=ar (BQC).

Which of the following figures lie on the same base and between the same parallels. In such a case, write the common base and the two parallels.

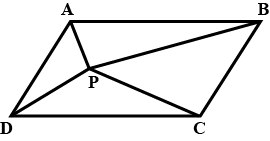
In figure, P is a point in the interior of a parallelogram ABCD, Show that
ar. (ABP)=ar(PCD)=\dfrac 12 ar ( \square ABCD)
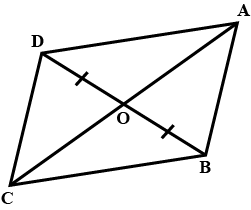
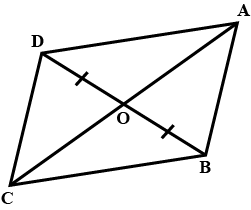
In Fig, diagonals AC and BD of quadrilateral ABCD intersect at O such that OB=OD. If AB=CD, then show that:
ar.(\triangle DOC)=ar.(\triangle AOB)
[Hint: From D and B, draw perpendiculars to AC.]
P and Q are respectively the mid points of sides AB and BC of a triangle AABC and R is the mid point of AP, show that
ar. (\triangle PBQ)= ar. (\triangle ARC)

In figure, P is a point in the interior of a parallelogram ABCD, Show that
ar. (ABD)=ar(PBC)=ar (APB)+ar(PCD)
(Hint: Through P, draw a line parallel to AB).

In figure, PQRS and ABRS are parallelograms and X is any point on side BR. Show that
ar. (AXS)+=\dfrac 12ar. ( \square PQRS)

Show that the diagonals of a parallelogram divide it into four triangles of equal area.

In Fig, diagonals AC and BD of quadrilateral ABCD intersect at O such that OB=OD. If AB=CD, then show that:
ar.(\triangle DCB)=ar.(\triangle ACB)
[Hint: From D and B, draw perpendiculars to AC.]
If the ratio of the altitude and the area of the parallelogram is 2:11, then find the length of the base of the parallelogram.
Draw the following figure which is on the same base and in between the pair of parallel lines in your notebook.
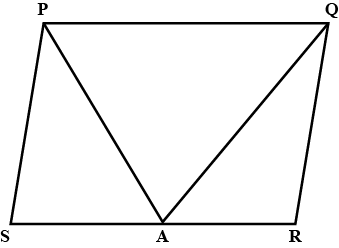
(i) One obtuse angled triangle and a trapezium.
(ii) A parallelogram and an isosceles triangle.
(iii) A square and a parallelogram.
(iv) A rectangle and a rhombus.
(v) A rhombus and a parallelogram.
If heights of two triangles are in the ratio 4:9 then find the ratio of their areas.
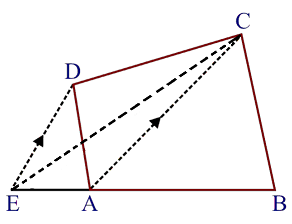
The side AB of a parallelogram ABCD is produced to any point P. A line through A and parallel to CP meets CB produced at Q and then parallelogram PBQR is completed. Show that ar.(ABCD)=ar(PBQR).
[Hint: Join AC and PQ. Now compare ar. (\triangle ACQ) and ar.(\triangle APQ)]
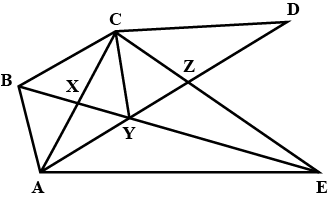
In the following figure, CD \parallel AE and CY \parallel BA. Prove that ar (\triangle CBX)= ar ( \triangle AXY).
Which of the following figures lie on the same base and between the same parallels? In such a case, write the common base and two parallels.
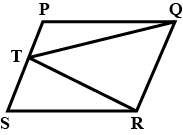
In a figure, PQRS and EFRS are two parallelograms then, prove that ar (MFR)= \dfrac12 ar (PQRS).

Solve the following questions:
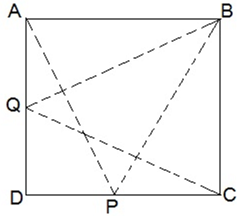
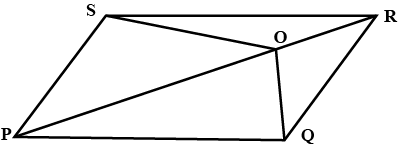
PB and QA are perpendicular at A and B of line segment AB. If P and Q lies on two sides AB and by joining P and Q. It intersects AB at O and PO=5cm,QO=7cm, ar(\triangle POB)=150{cm}^{2} then find ar(\triangle QOA)
In the following figure, two triangles ABC and DBC are formed on same base BC. If AD,BC intersect at point O then show that
\cfrac { ar.\left( ABC \right) }{ ar.\left( DBC \right) } =\cfrac { AO }{ DO }
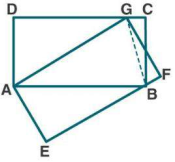
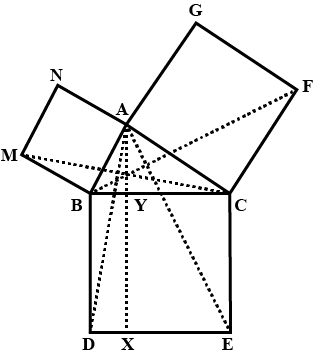
In figure ABC is a right triangle right angled at A, BCED, ACFG and ABMN are square on the sides BC, CA and AB respectively. Line segment AX\bot DE meets BC at Y. Show that:
ar.(BCED)=ar.(ABMN)+ar.(ACFG)
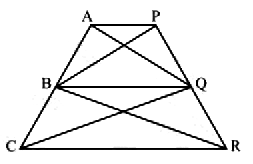
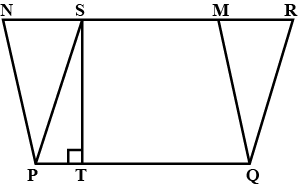
Two parallelograms PQRS and PQMN have common base PQ as shown in figure. PQ= 9 \ cm, SM= 3 \ cm and ST= 5 \ cm. Find the area of PQRN.
ABCD is a parallelogram, E and F are the mid-points of BC and CD respectively.
Prove that: ar(\triangle AEF)= \dfrac38 ar(||gm \ ABCD).
In the adjoining figure, DE||BC. Prove that
ar(\triangle OCE)=ar (\triangle OBD)
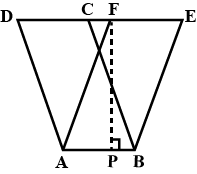
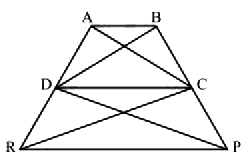
In the given fig, ar(DRC) = ar(DPC) and ar(BDP) = ar(ARC). Show that both the quadrilaterals ABCD and DCPR are trapeziums.
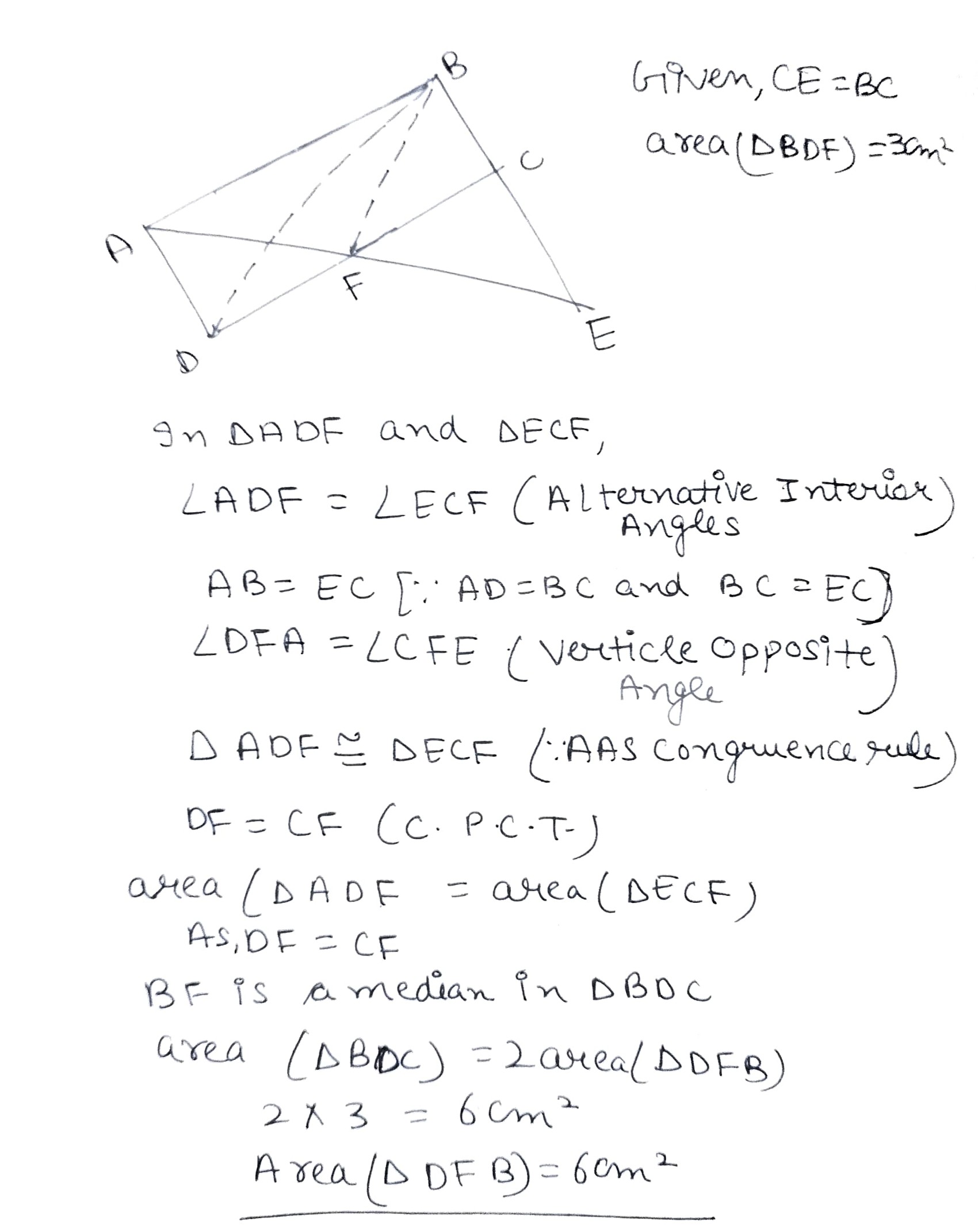
In fig. 2, ABCD is a parallelogram in which BC is produced to E such that CE= BC. AE intersects CD at F. If area of \triangle BDF= 3 cm^2, find the area of parallelogram ABCD.
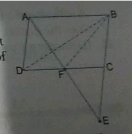
D and E are points on sides AB and AC respectively of \triangle ABC such that ar(\triangle BCD)=ar(\triangle BCE). Prove that DE || BC.
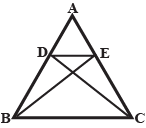
Find the missing term in the following on the basis of given diagram.
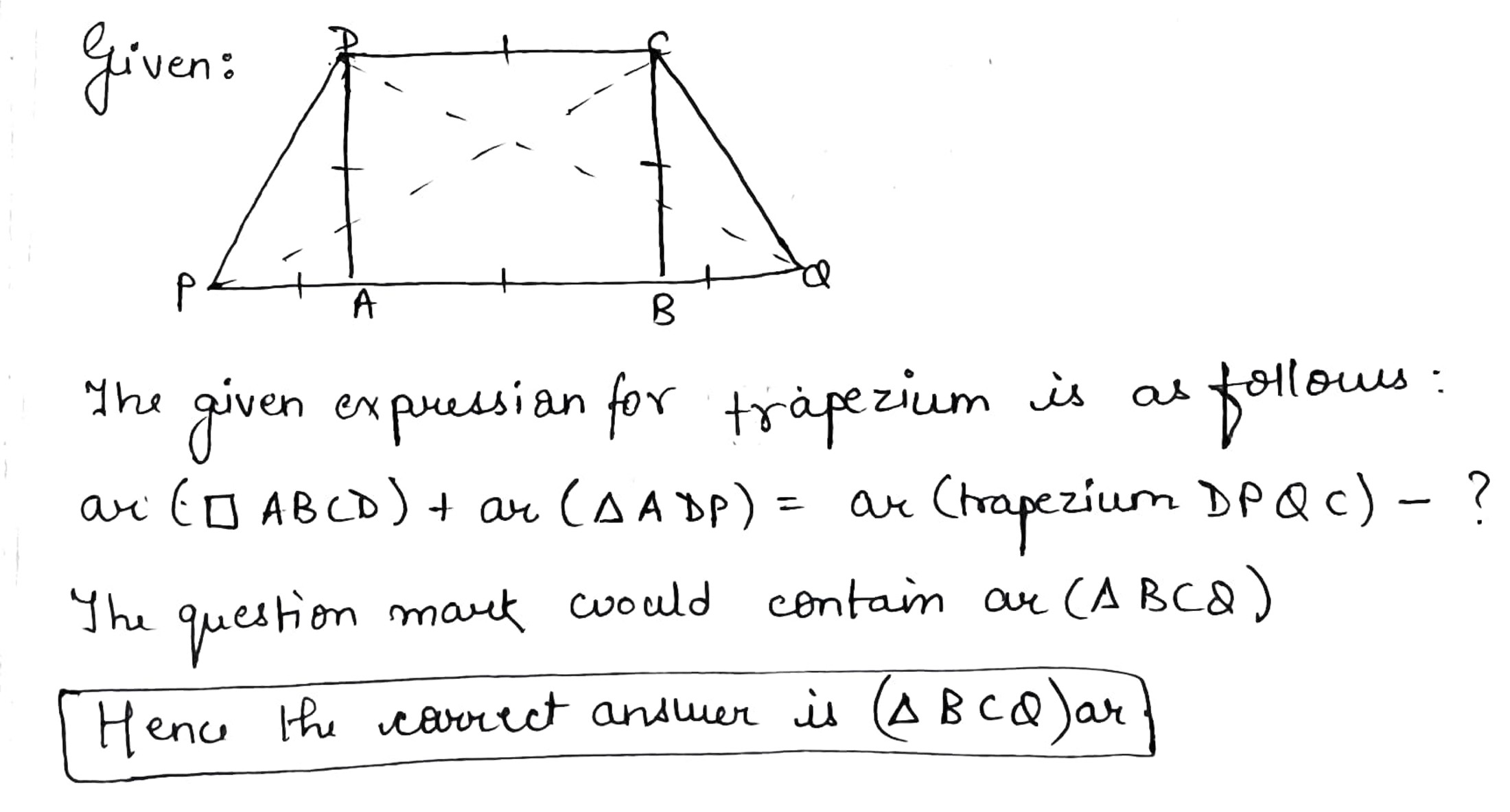
ar(\square ABCD) + ar(\triangle ADP) = ar(\trapezium DPQC) - ?
Class 9 Maths Extra Questions
- Areas Of Parallelograms And Triangles Extra Questions
- Circles Extra Questions
- Constructions Extra Questions
- Coordinate Geometry Extra Questions
- Heron'S Formula Extra Questions
- Introduction To Euclid'S Geometry Extra Questions
- Linear Equations In Two Variable Extra Questions
- Lines And Angles Extra Questions
- Number Systems Extra Questions
- Polynomials Extra Questions
- Probability Extra Questions
- Quadrilaterals Extra Questions
- Statistics Extra Questions
- Surface Areas And Volumes Extra Questions
- Triangles Extra Questions