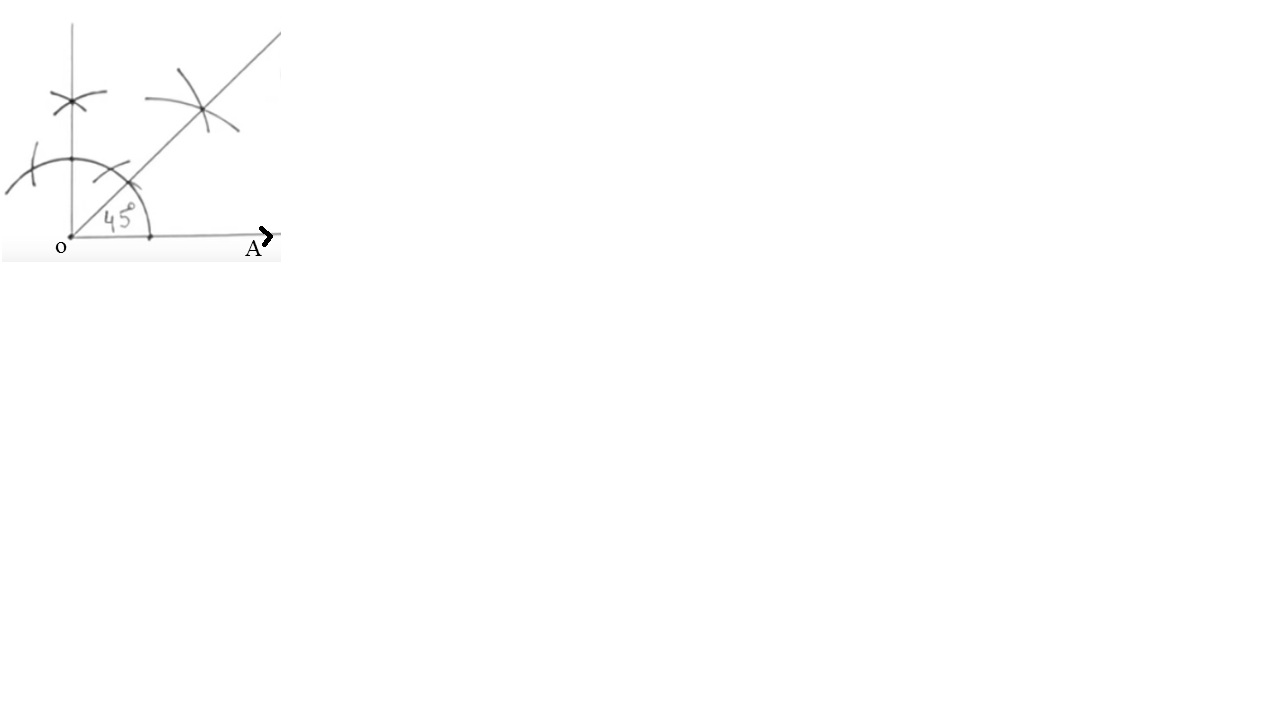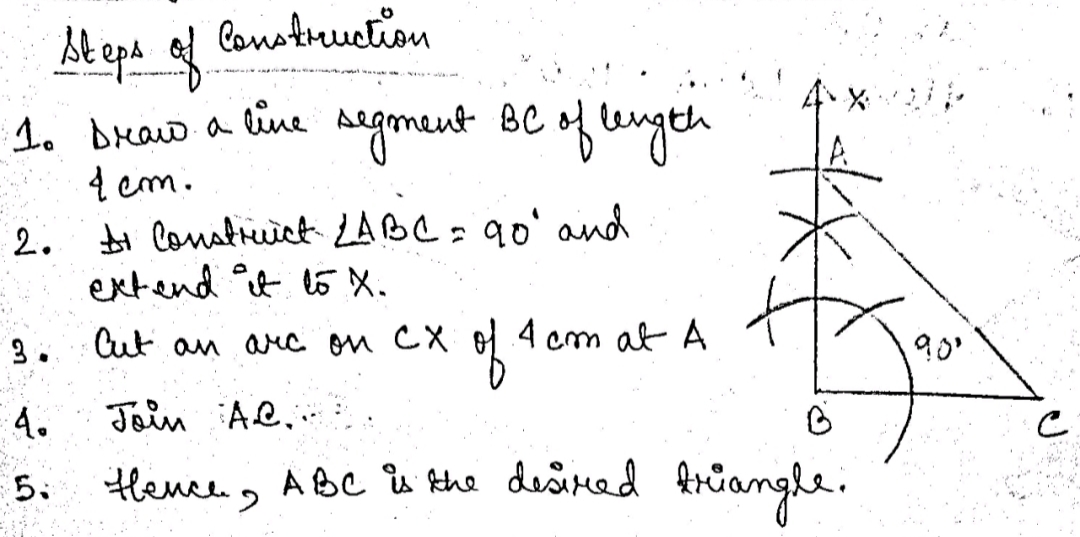Constructions - Class 9 Maths - Extra Questions
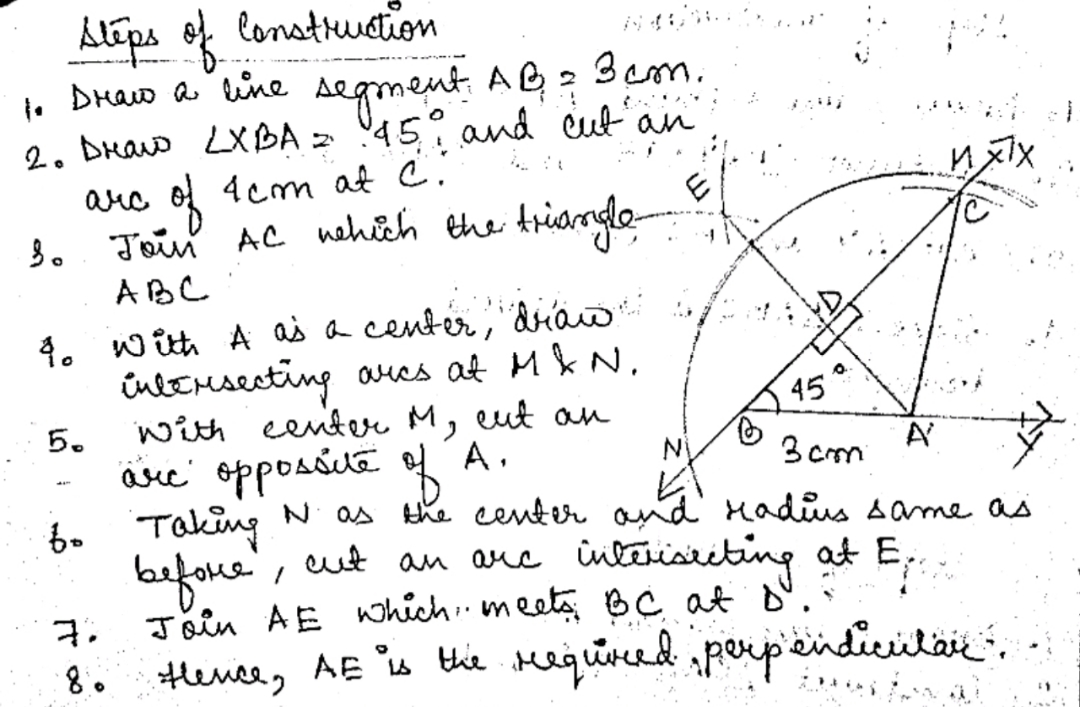
Construct a scalene triangle ABC, given base AB=3 cm, base angle =90o and sum of the lengths BC+AC=9 cm.
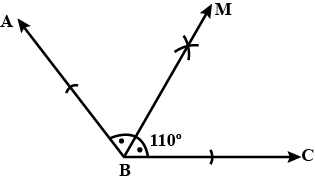
Draw ∠ABC of measure 110∘ and bisect it.
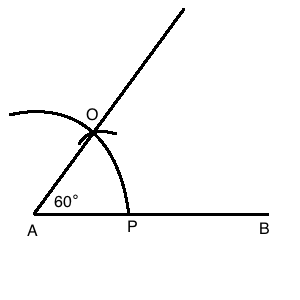
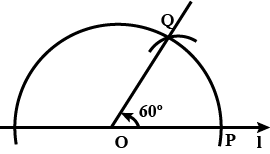
Construct a bisector of an angle of 60o.
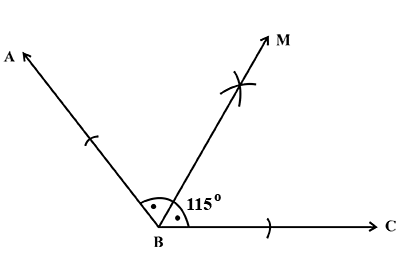
Draw ∠ABC of measure 115∘ and bisect it.
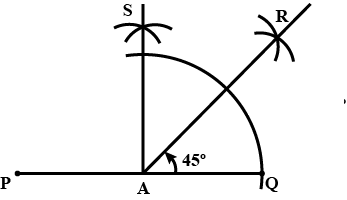
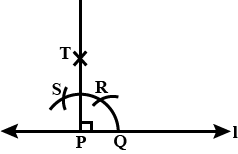
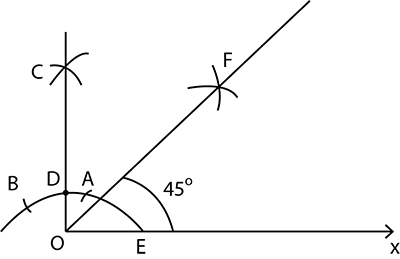
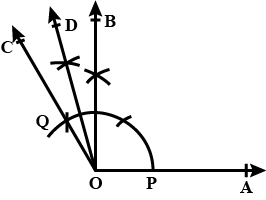
Construct an angle of 45o using compass.
Draw the following angles using ruler and compasses. Also label them.
45o
Construct ΔPQR, such that QR=6.5cm, ∠PQR=600 and PQ−PR=2.5cm.
The construction of ΔPQR given that QR=5.2 cm angle Q=50∘. Is it possible when the difference of PQ and PR is 3.5 cm? justify.
Draw the following angles using ruler and compasses. Also label them.
90o
How many of the given letters have perpendicular lines?
MATHS
Draw an angle of measure 45o and bisect it.
Draw a right angle.
Draw ∠POQ of measure 75o and find its line of symmetry.
Draw the following angles using ruler and compasses. Also label them.
30∘
Draw a line segment XY=7cm and draw ∠AXY=90∘
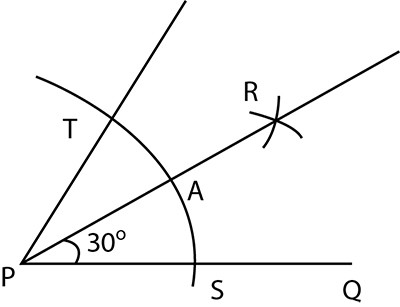
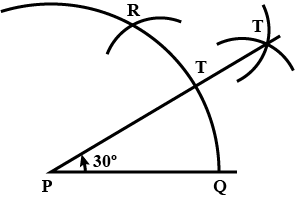
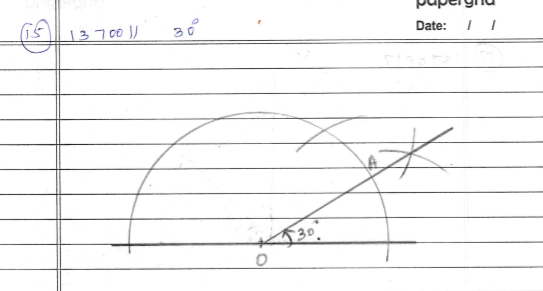
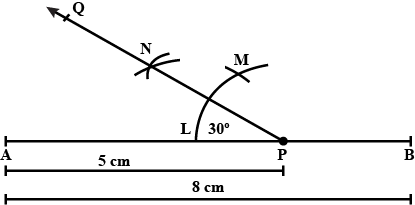
Construct the angle of 30o using a ruler and compass.
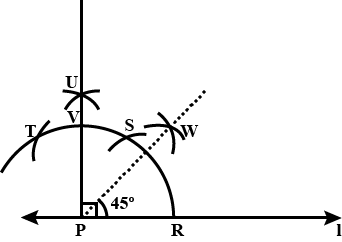
Construct an angle of the measure 45∘ with ruler and compasses.
Construct with ruler and compasses, angle of the following measure:
30o
Construct angles of following measures using ruler and compasses.
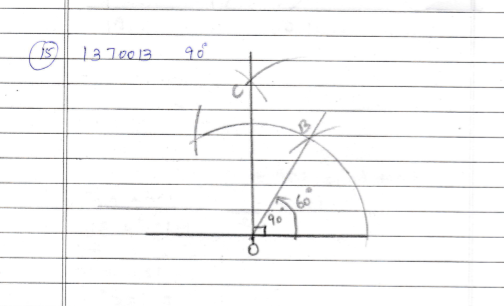
90o
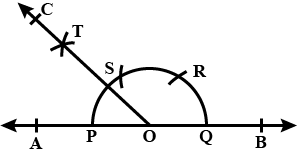
What is an angle bisector ?
Construct with ruler and compasses, angle of the following measure:
90o
Construct angles of following measures using ruler and compasses.
60o
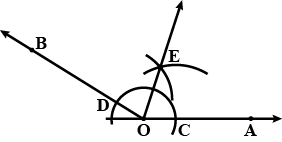
Construct a bisector of an angle.
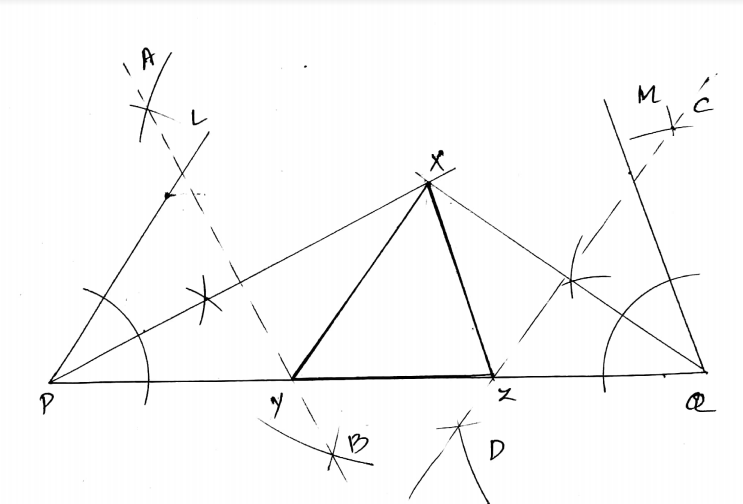
Construct a triangle ABC whose perimeter 12 cm and who base angles are 50∘ and 80∘.
Construct with ruler and compasses, angle of following measures.
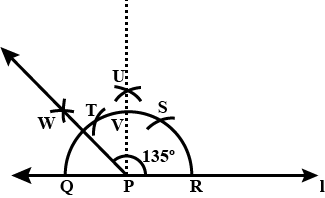
135o.
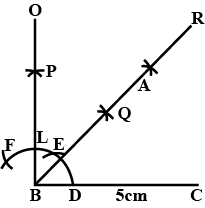
Construct an angle of 45∘ at the initial point of a given ray OA, using a ruler and compasses.
Construct with ruler and compasses, angle of following measures.
60o.
Construct with ruler and compasses, angle of following measures.
90o.
Draw an angle of measure 135o and bisect it.
Construct with ruler and compasses, angle of following measures.
30o.
Construct with ruler and compasses, angle of following measures.
120o.
Draw a right angle and construct its bisector.
Construct with ruler and compasses, angle of following measures.
45o.
Draw an angle of 45∘, using a pair of compasses.
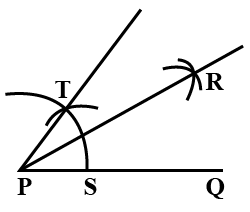
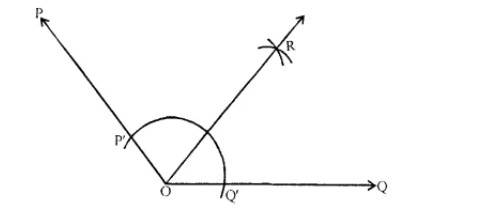
In this question, use a straight edge and compasses only and show all your construction arcs. Construct the bisector of angle ABC.
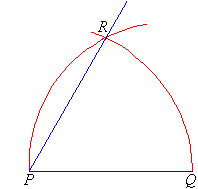
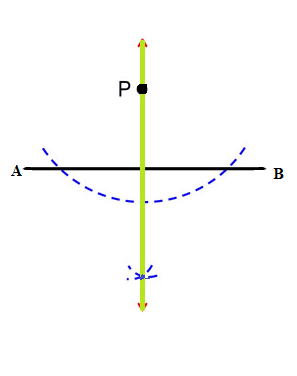
In this question, use a straight edge and compasses only and show all your construction arcs. Construct the perpendicular bisector of PQ.

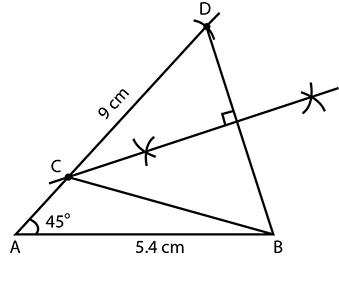
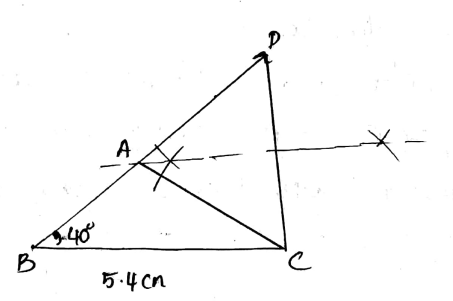
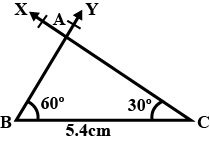
Construct a △ABC in which: AB=5.4 cm, ∠CAB=450 and AC+BC=9 cm.Then the length of AC (in cm.) is:
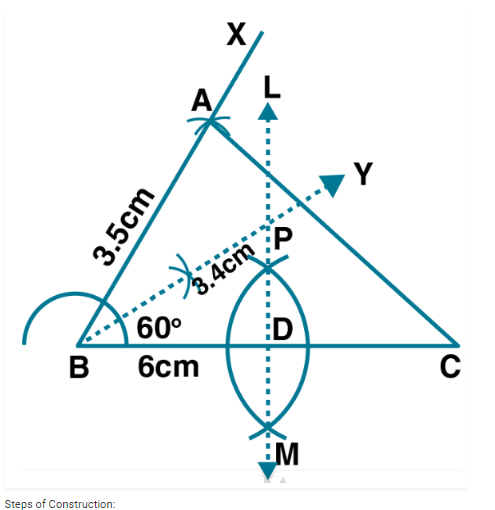
Construct a triangle PQR in which ∠Q=60o and ∠R=45o and PQ+QR+PR=11 cm.
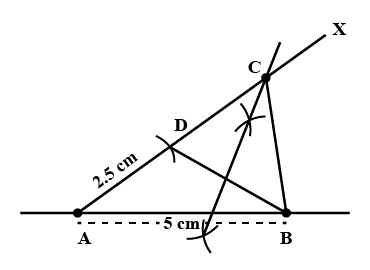
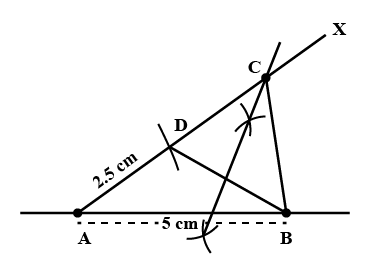
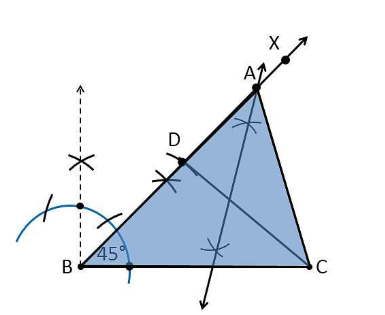
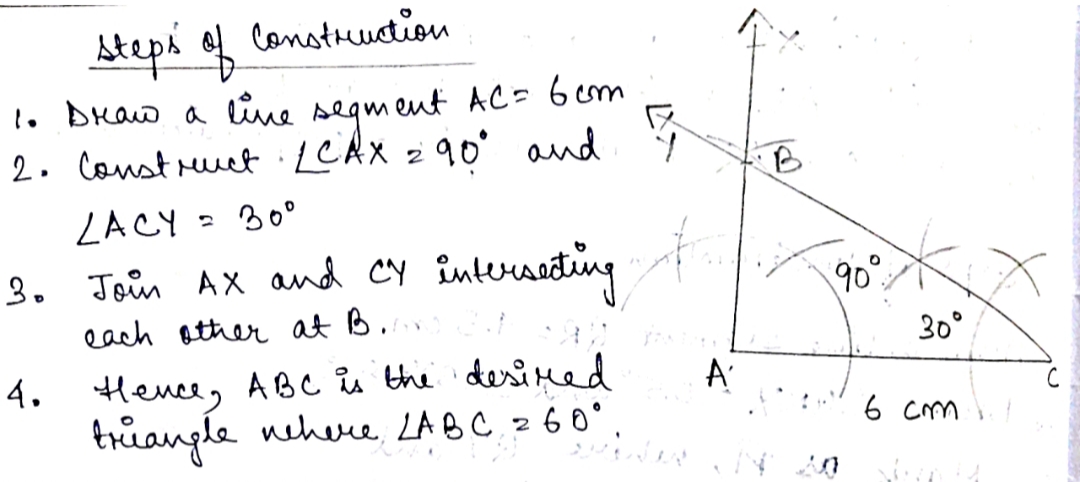
Construct a △ABC in which AC=5 cm, and ∠BAC=60∘ and AB−BC=1.2 cm.
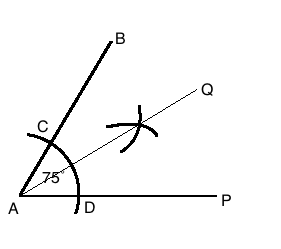
Construct the bisector of an angle 75o.
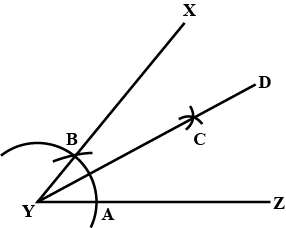
Construct an angle of 60o using compass.
Construct a triangle whose perimeter is 9.8 cm and the base angles are 45∘ and 60∘.
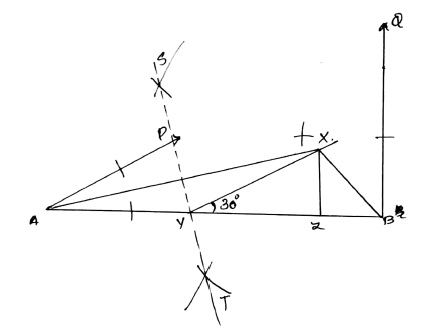
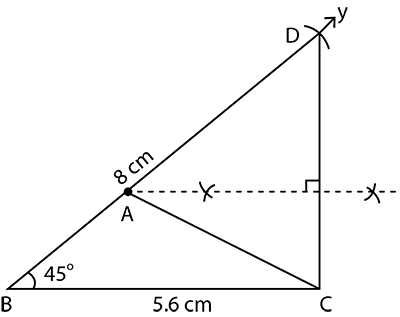
Construct a triangle ABC in which BC=5.6 cm, ∠B=45∘ and AB+AC=8 cm.
Construct a △ PQR, such that ∠ Q=70o, ∠R=70o and PQ+QR+RP =10 cm.
Construct a triangle ABC in which BC=6 cm,∠A=45∘ and median AD=5 cm.
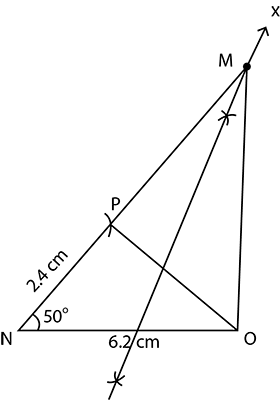
Construct △MNO such that NO=6.2 cm, ∠N=50∘ and MO−MN=2.4 cm.
Perimeter of △ABC is 14cm, AB=4.5 cm and ∠A=80o. Construct △ABC.
Construct an angle of 30o using compass.
Construct △MNO where base NO=6.7 cm, ∠MNO=45∘ and MO−MN=2.8 cm.
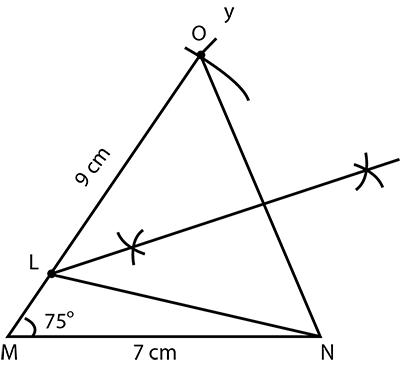
Construct △LMN in which base MN=7 cm, ∠LMN=75∘ and LM+LN=9 cm.
Construct a triangle XYZ in which ∠Y=30o, ∠Z=90o and XY+ZX+YZ=11cm.
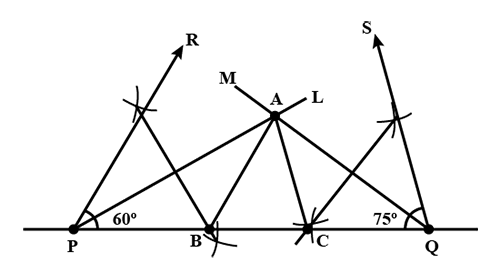
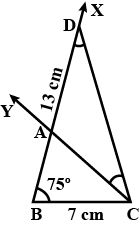
Construct a triangle ABC in which BC=7cm, ∠B=75o and AB+AD=13 cm.
Construct △PQR such that perimeter of △PQR is 16 cm and ∠Q=90∘ and QR=5 cm.
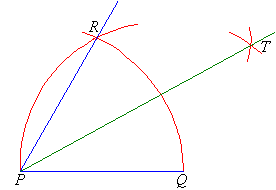
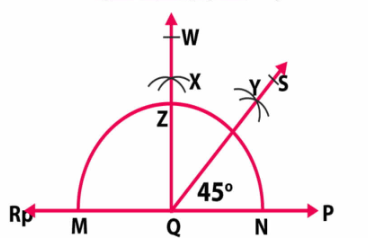
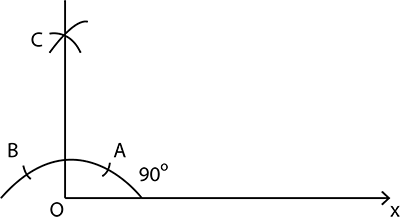
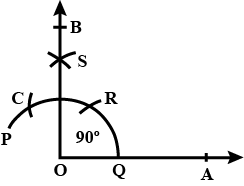
Construct an angle of 90∘ at the initial point of a given ray and justify the construction.
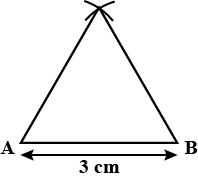
Construct an equilateral triangle, given its side =3cm and justify the construction.
Construct a triangle PQR in which QR=6cm, ∠Q=60o and PQ−PO=2cm.
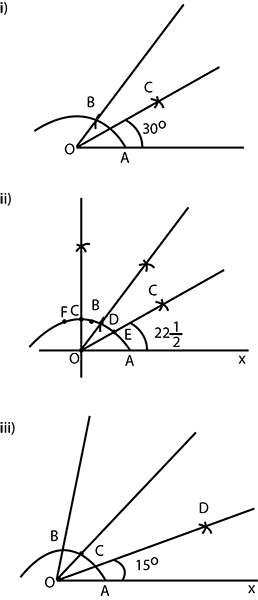
Construct the angles of the following measurements:
(i)30∘
(ii)2212∘
(iii)15∘
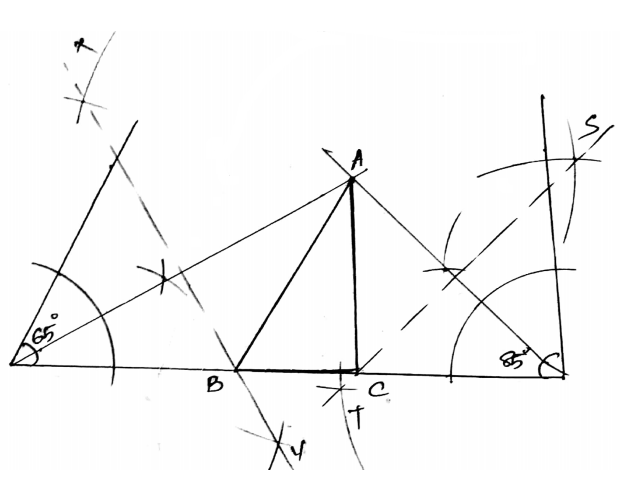
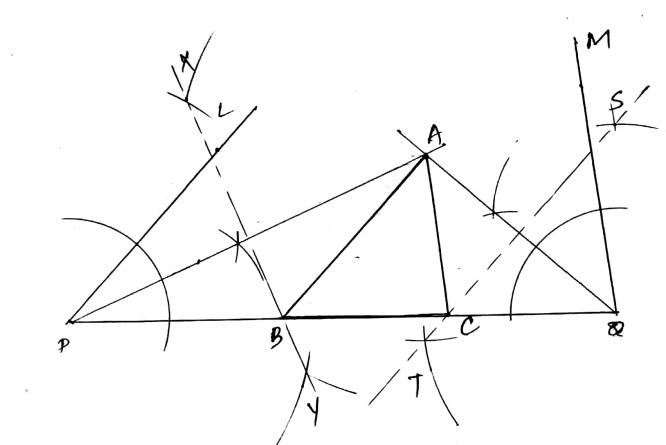
Construct a triangle ABC whose perimeter 12cm and whose base angles are 65∘ and 85∘
Construct a triangle ABC whose perimeter 12cm and whose base angles are 50∘ and 80∘
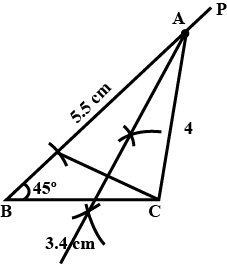
Construct triangle ABC in which BC=3.4cm,AB−AC=1.5cm and ∠B=45∘
Construct a triangle ABC, whose perimeter is 12cm and whose sides are in the ratio 3:4:5
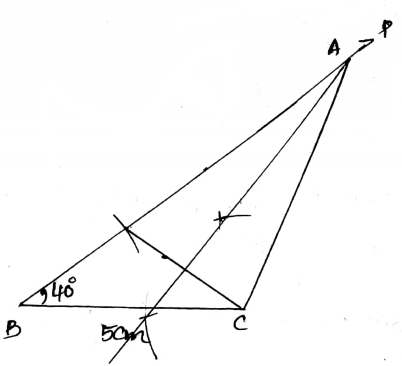
Construct triangle ABC in which BC=5cm,AB−AC=2.8cm and ∠B=40∘
Construct triangle ABC in which BC=6cm,AB−AC=3.1cm and ∠B=30∘
Construct a triangle ABC in which BC=3.6cm,AB+AC=4.8cm and ∠B=60∘
Construct a triangle ABC in which AB=5.6cm,BC=5.4cm and ∠B=40∘
Construct a triangle ABC, whose perimeter is 12cm and whose sides are in the ratio 2:3:4.
Construct a triangle XYZ whose perimeter 15 cm and whose base angles are 60∘ and 70∘
Draw ∠ABC of measure 120∘ and bisect it.
Construct a triangle ABC in which AB=5.8 cm,BC+CA=8.4 cm and ∠B=60∘
Using a ruler and compass only.
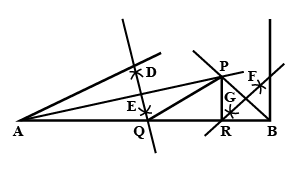
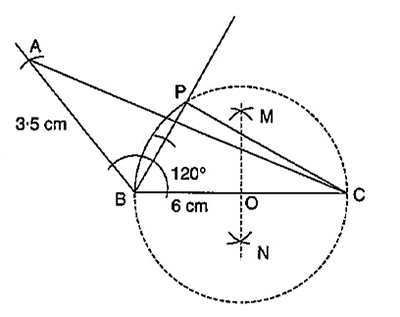
(i) Construct a △ABC with the following data.
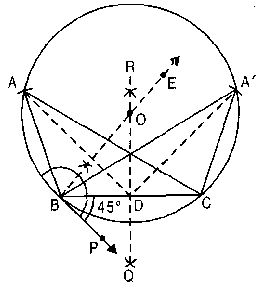
AB=3.5 cm,BC=6 cm and ∠ABC=120∘
(ii) In the same diagram, draw a circle with BC as diameter. Find a point P on the circumference of the circle which is equidistant from AB and BC.
(iii) Measure ∠BCP.
Construct a triangle ABC in which base AB=5cm,∠A=30∘ and AC−BC=2.5cm
Construct a triangle ABC whose perimeter is 12.5cm and whose base angles are 60∘ and 75∘
Construct an angle of 45o and bisect it.
Draw ∠ ABC of measure 125o and bisect it.
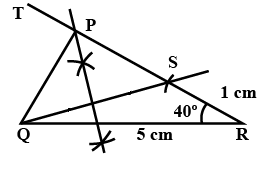
Construct a triangle PQR in which QR=5 cm,∠R=40o and PR−PQ=1 cm.
Construct a triangle ABC in which BC=7cm,∠B=75o and AB+AC=13cm
Construct an angle of 90o at the initial point of a given ray and justify the construction.
If the measure of an angle is equal to 225∘, then find the fraction of one complete turn?
Construct △ABC in which BC=6.3 cm ∠B=75o and AB+AC=9 cm.
Construct △XYZ in which ∠Y=30o,∠Z=60o and XY+YZ+ZX=10 cm.
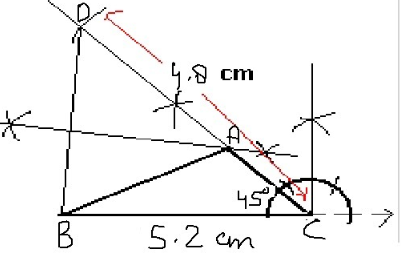
Write the steps to construct △ABC, in which BC=5.2 cm, ∠ACB=45∘ and perimeter of △ABC is 10 cm.
Construct an angle of 45o at the initial point of a given ray and justify the construction.
Construct ΔABC, such that BC=6cm,∠ABC=1000 and AC−AB=2.5cm.
Construct an angle of 45∘ at the initial point of a given point of a given ray and justify the construction.
Construct an angle of 90o at the point of a given ray and justify the construction.
Draw the following angles using ruler and compasses. Also label them.
60o
Draw the following angles using ruler and compasses. Also label them.
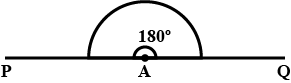
180o
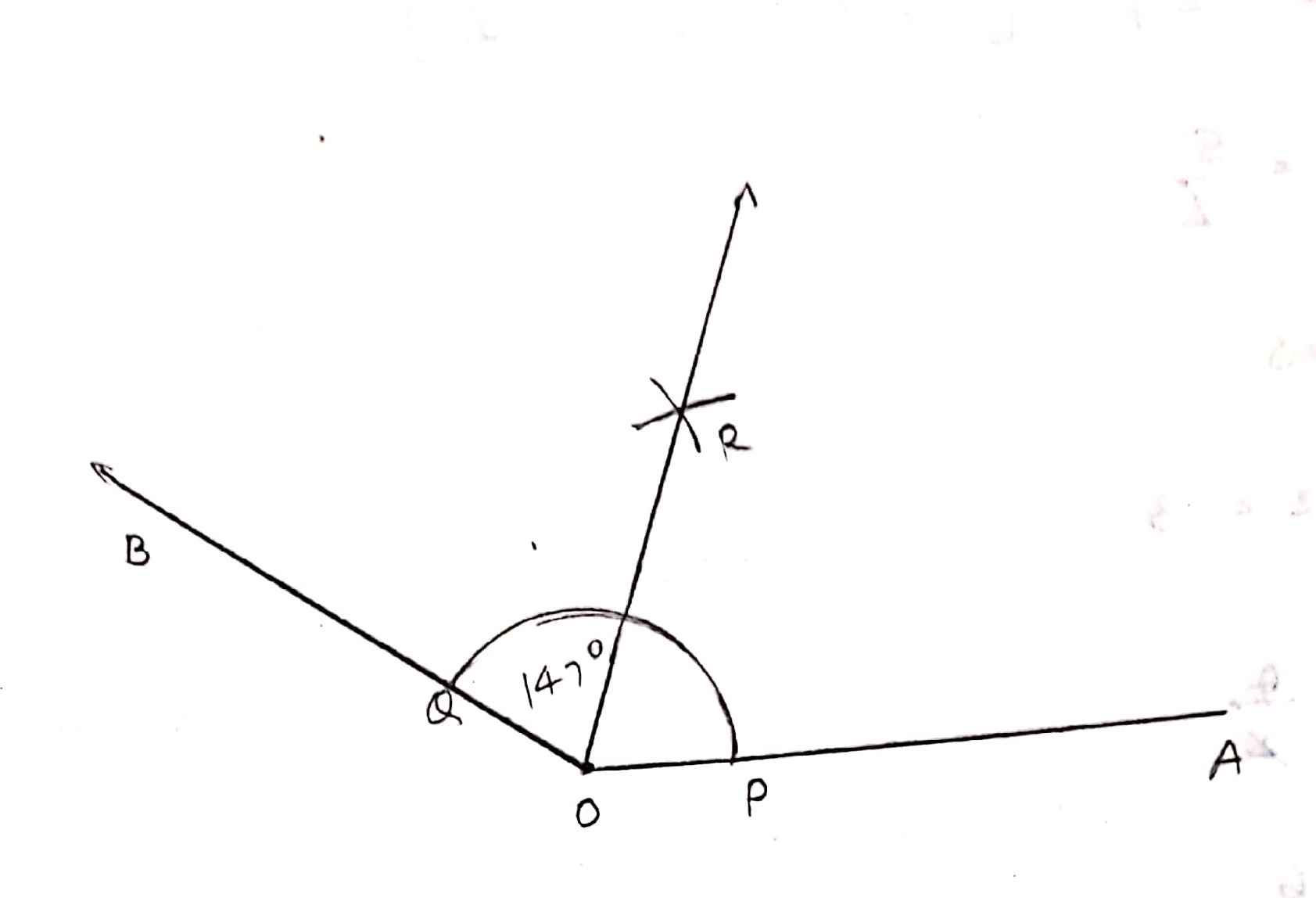
Draw an angle of measure 147∘ and construct its bisector.
Draw an angle of measure 147∘ and construct its bisector
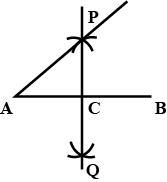
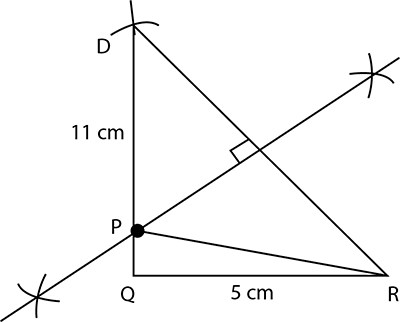
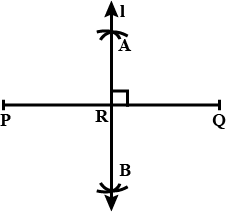
Draw a line segment PQ=6.2 cm. Draw the perpendicular bisector of PQ.

Construct a triangle ABC in which BC=8cm,∠B=45∘ and AB−AC=3.5cm
Construct a △ABC in which BC=5.6 cm,∠B=30o and the difference between the other sides is 3 cm.
Construct triangle ABC having given BC=7 cm, AB−AC=1 cm and ∠ ABC=45o.
Construct a perpendicular line from point p to any line AB.
Use ruler and compasses only for this question.
Construct a △ABC,AB=3.5cm,BC=6cm,&∠ABC=60∘
Mark the point P which is equidistant from AB, BC and also equidistant from B and C.
Construct a triangle ABC in which BC=8cm,∠B=45∘andAB−AC=3.5cm.
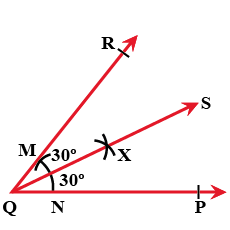
Draw an angle of 60∘, using a pair of compasses. Bisect it to make an angle of 30∘.
Draw any angle with vertex O. Take a point A on one of its arms and B on another such that OA=OB. Draw the perpendicular bisectors of ¯OA and ¯OB. Let them meet at P. Is PA=PB?
Without using set-squares or protractor construct:
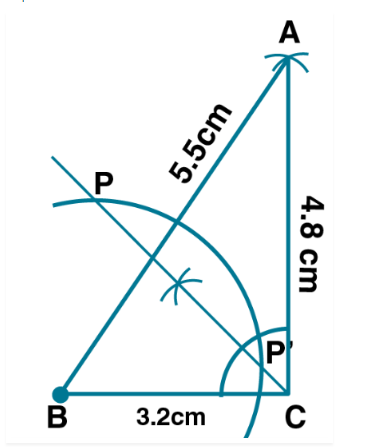
Triangle ABC, in which AB=5.5 cm, BC=3.2 cm and CA=4.8 cm.
Draw the locus of a point which moves so that it is equidistant from the sides BC and CA.
BM and CN are perpendiculars to a line passing through the vertex A of a triangle ABC. If L is the mid-point of BC, prove that LM=LN.
Construct a ΔABC in which BC=5 cm, ∠B=45 and AB-AC=2.8cm
ABC is a triangle. D is a point on AB such that AD=14AB and E is a point on AC such that AE=14AC. Prove that DE=14BC.
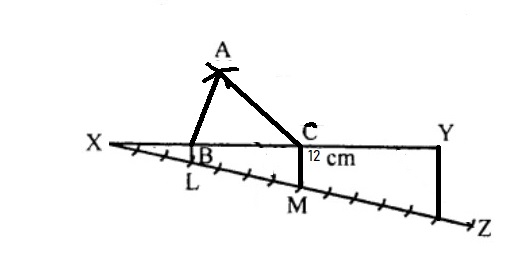
Draw a right triangle having hypotenuse of length 5.4cm, and one of the acute angles of measure 30o.
Draw an angle of measure 127∘ and construct its bisector.
Without using set-squares or protractor construct:
construct a △ABC,AB=5.5cm,BC=3.2cm,&AC=4.8cm
Find the locus which is equidistant from sides BC&AC and also at a distance of 2.5cm from B
Mark the point of intersection of the loci with the letter P and measure PC.
Bisect the ∠XYZ and write the steps of construction as well.
Draw a line segment AB=8 cm. Mark a point P in AB so that AP=5 cm. At P, construct ∠APQ=30∘.
Construct angle ABC=45o in which BC=5cm and AB=4.6cm.
Construct an angle of 45∘ from a horizontal line and justify the construction.
Using a protractor, draw ∠BAC of measure 45∘. Take a point P in the interior of ∠BAC. From P draw line segments PM and PN such that PM⊥AB and PN⊥AC, Measure ∠MPN.
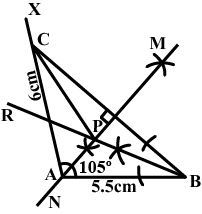
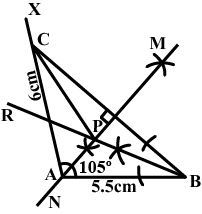
Construct a triangle ABC with AB=5.5\ cm,AC=6\ cm and \angle BAX=105^{o}
Hence :
Construct the locus of point equidistant from B and C.
In triangle ABC; angle A=90^{o}, side AB=x\ cm,\ AC=(x+5)\ cm and area =150\ cm^{2}. Find the sides of the triangle.
Construct a triangle ABC with AB=5.5\ cm,AC=6\ cm and \angle BAX=105^{o}
Hence :
Construct the locus of points equidistant from BA and BC .
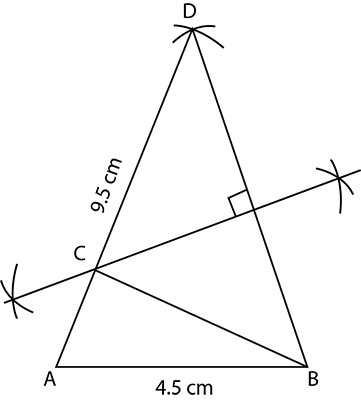
Construct a \triangle A B C in which B C = 3.6 cm, A B = 5 cm and A C = 5.4 cm. Draw the perpendicular bisector of the side B C.
Construct a triangle XYZ in which \angle Y = {30}^0 , \angle Z = {90}^0 and XY + YZ + ZX = 11 cm .
Draw a triangle ABC in which BC=4cm, AB=3cm and \angle B=45^o. Also, draw a perpendicular from A on BC.
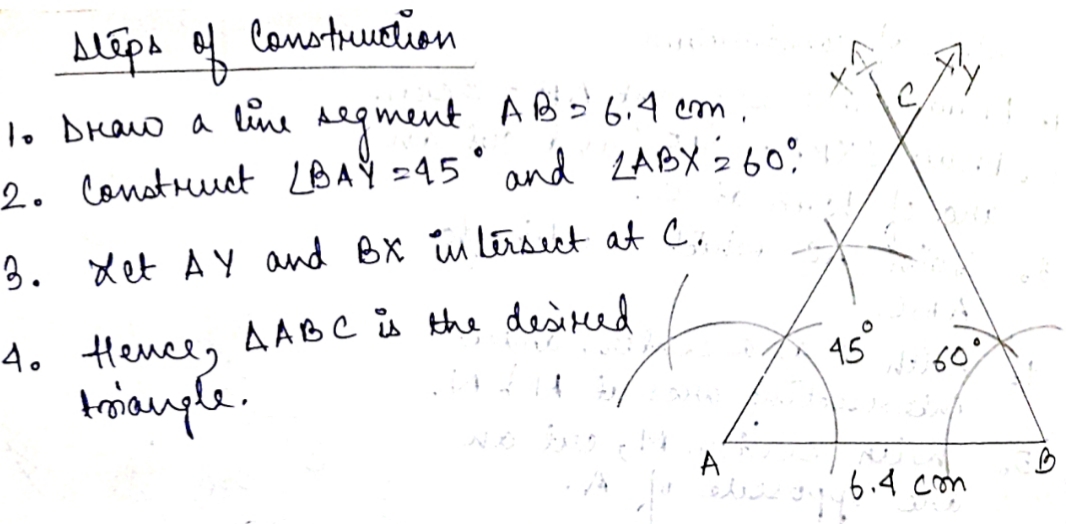
Construct \DeltaABC in which AB=6.4cm, \angle A=45^o and \angle B=60^o.
Construct the following angles with the help of ruler and compasses only:150^{\circ}
Construct the following angles with the help of ruler and compasses only:105^{\circ}
Draw \DeltaABC in which AC=6cm, \angle A=90^o and \angle B=60^o.
Draw \DeltaABC in which \angle C=90^o and AC=BC=4cm.
Using ruler and compasses only, draw a right angle.
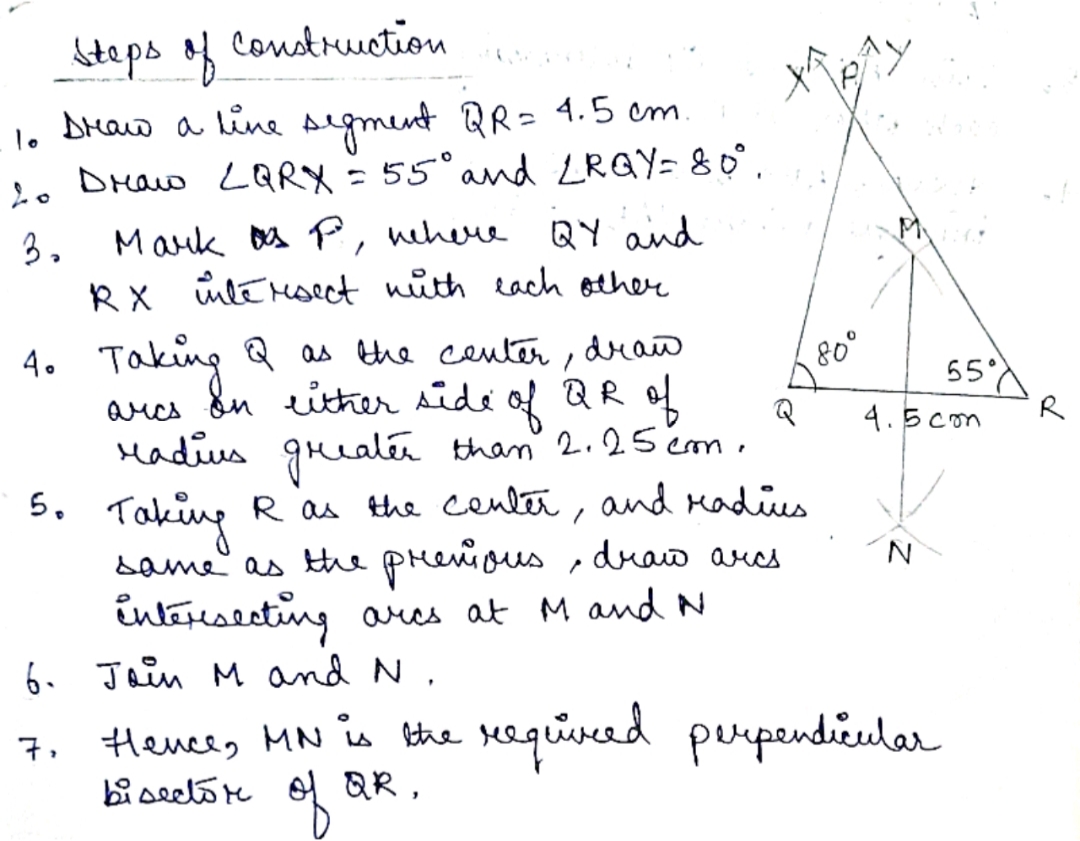
Draw \DeltaPQR in which \angle Q=80^o, \angle R=55^o and QR=4.5cm. Draw the perpendicular bisector of side QR.
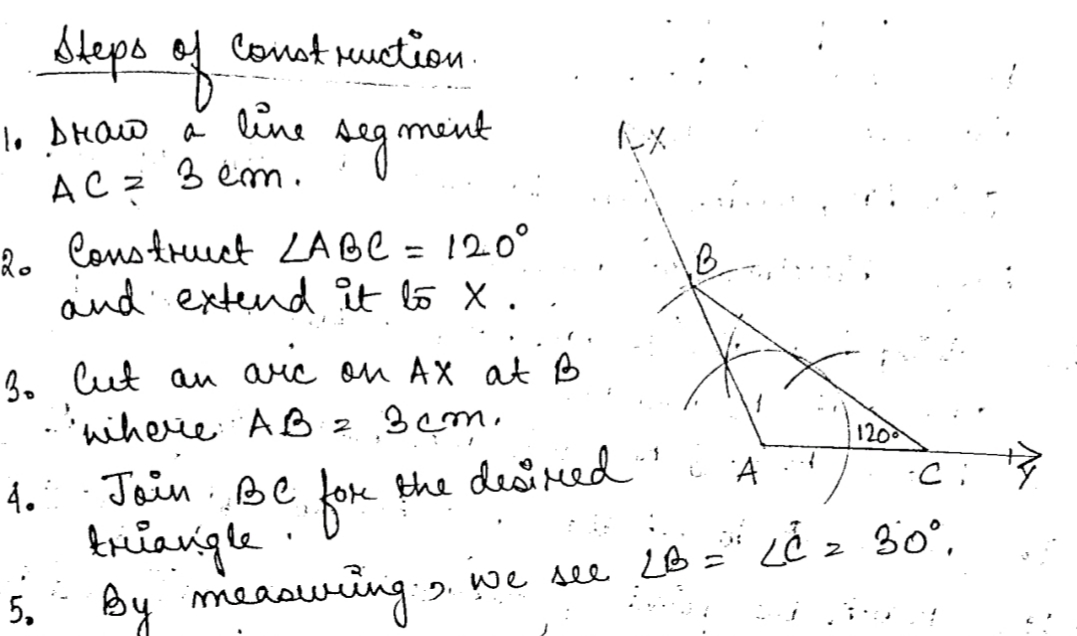
Draw \DeltaABC in which \angle A=120^o, AC=AB=3cm. Measure \angle B and \angle C.
Class 9 Maths Extra Questions
- Areas Of Parallelograms And Triangles Extra Questions
- Circles Extra Questions
- Constructions Extra Questions
- Coordinate Geometry Extra Questions
- Heron'S Formula Extra Questions
- Introduction To Euclid'S Geometry Extra Questions
- Linear Equations In Two Variable Extra Questions
- Lines And Angles Extra Questions
- Number Systems Extra Questions
- Polynomials Extra Questions
- Probability Extra Questions
- Quadrilaterals Extra Questions
- Statistics Extra Questions
- Surface Areas And Volumes Extra Questions
- Triangles Extra Questions