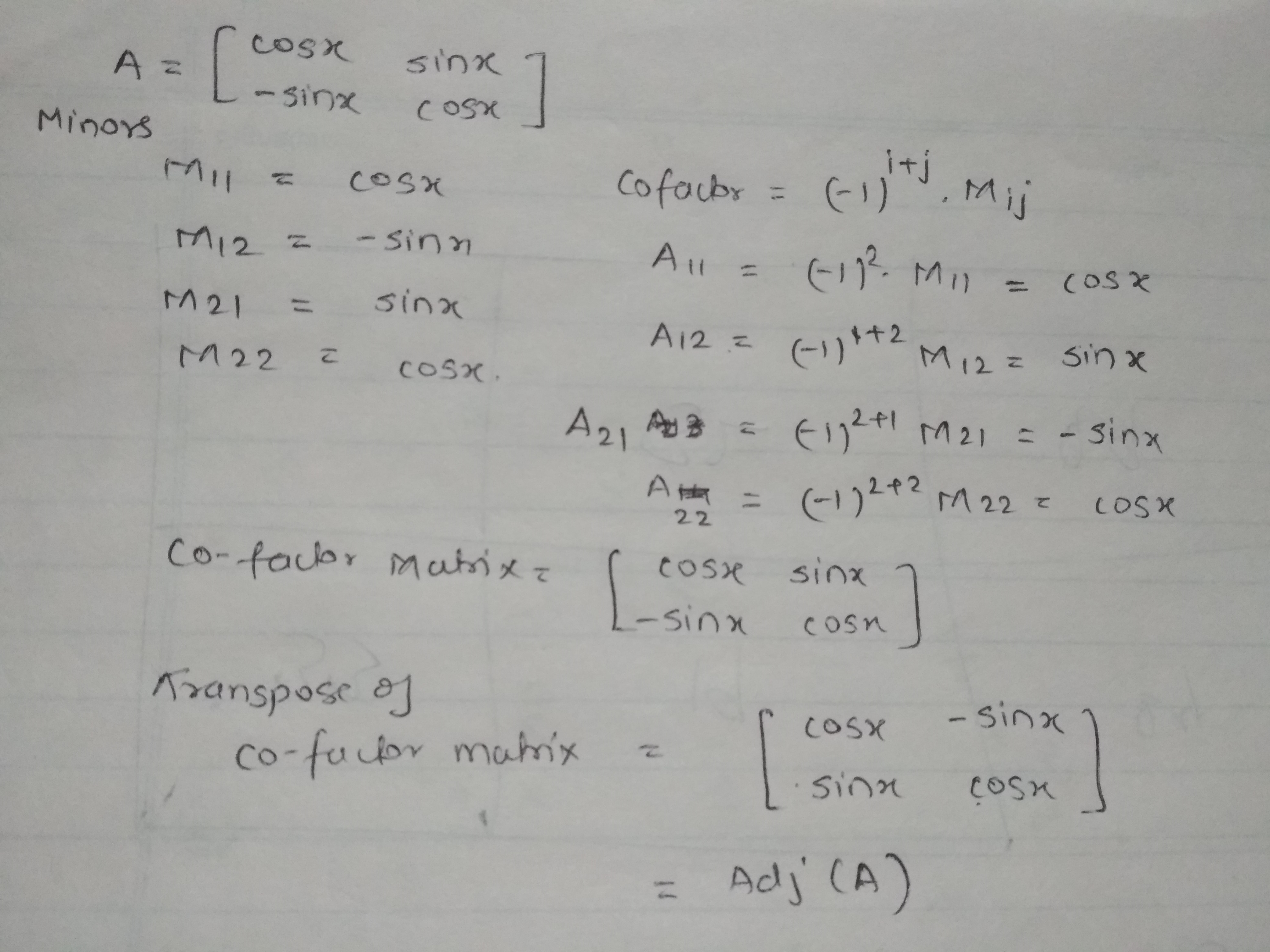Determinants - Class 12 Engineering Maths - Extra Questions
Find the value of determinant |24−5−1|.
Find the Adjoint matrix of the matrix |123232334|
If ω is one of the imaginary cube roots of unity, find the value of
|1ω3ω2ω31ωω2ω1|.
Find determinant Dc=|11−21−232−1−1|
Find the relation between a and b. If the points P(1,2),Q(0,0) and P(a,b) are collinear
If the matrix A = [6x22−12−1052]is a singular matrix. Find the value of x.
Find ′x′ if
|4x6234111|=10
If △=|3572−31112|, find it's value.
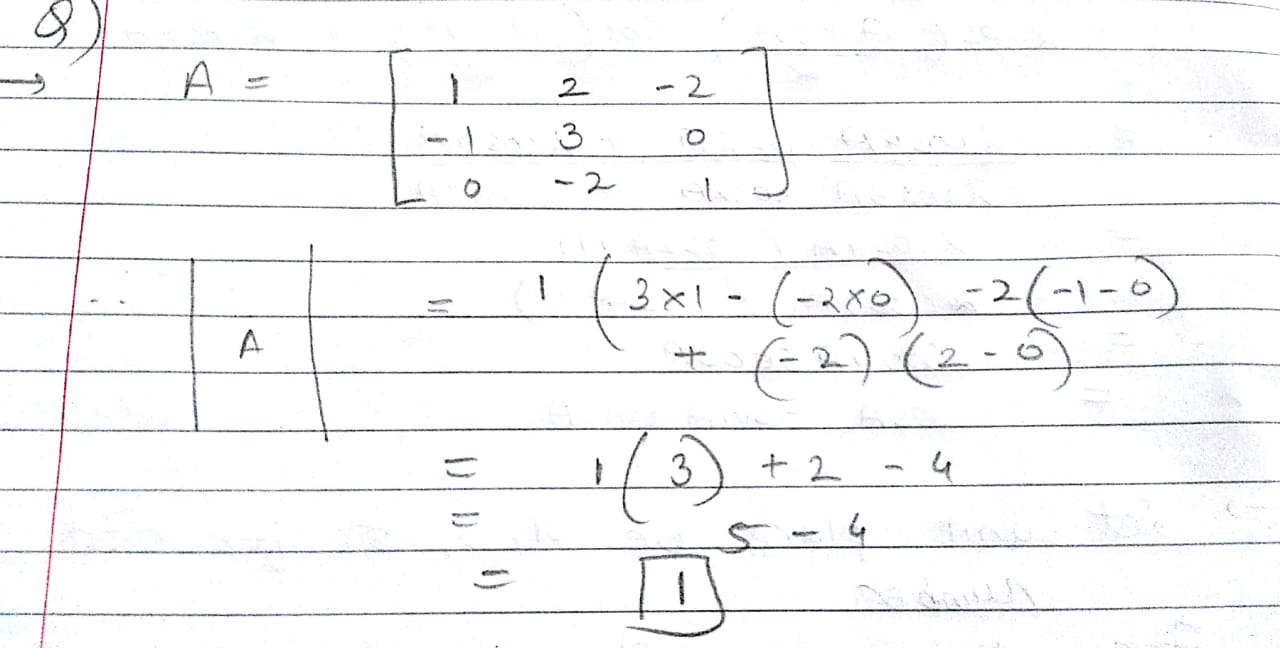
How do I find
A=[12−2−1300−21]=|A|=?
Find ad-joint of
A=[cosxsinx−sinxcosx]
Find the values of x, if
|x+1x−1x−3x+2|=|4−113|
Find the values of x, if |2x58x|=|6583|
Expand:|1−735−6012−3|
Find the values of x, if
|x+1x−1x−3x+2|=|4−113|
Show |axbyczx2y2z2111|=|abcxyzyzzxxy|
For a fixed positive integer n, if D=|n!(n+1)!(n+2)!(n+1)!(n+2)!(n+3)!(n+2)!(n+3)!(n+4)!| then show[D(n!)3−4] is divisible by n.
If u=ax2+2bxy+cy2,u′=a′x2+2b′xy+c′y2, then prove that |y2−xyx2abca′b′c′|=|ax+bybx+cya′x+b′yb′x+c′y|=−1y|uu′ax+bya′x+b′y|
If ω is one of the imaginary cube roots of unity, find the value of
|1ωω2ωω21ω21ω|.
Prove the following :
|2aba2b2a2b22abb22aba2|=−(a3+b3)2.
Let A be the matrix of order 3×3 such that |A|=1, B=2A−1 and C=(adjA)3√2, then the value of |AB2.C3| is
[Note : |A| represent determinant value of matrix A.]
Prove the following :
|a2+b2cccab2+c2aabbc2+a2b|=4abc
If A = [ab−a]3×1[ab−a]1×3 then find wheather A−1 exists or not.
Solve: |xx2yzyy2zxzz2xy|=(x−y)(y−z)(z−x)(xy+yz+zx).
Find the value of x for which the determinant |2x−3x−2x−1x−22x−2xx−1x2x−1| vanishes if
Show that:|abca−bb−cc−ab+cc+aa+b|=a3+b3+c3−3abc
Find the maximum value of
|11111+sinθ1111+cosπ|
Find the equation of line passing through the points (3,2) and (−1,3) by using determinants.
Find the determinant of the matrix
[3152]
Find the value of the determinant |2i−3ii3−2is| where i=√−i
prove that |x+1352x+2523x+4|=(x−1)2(x+9)
If |2−49d−3|=4, then find the value of d.
|cos15∘sin15∘sin75∘cos75∘|
Find the value of x if|3410x83−14|=0
For what value of x the matrix A is singular?
A=[1+x73−x8]
Evaluate the following determinant : |ahghbfgfc|
Prove that: |a+b+2cabcb+c+2abcac+a+2b|=2(a+b+c)3
If the point (x,y),(a,0),0,b) are collinear, prove that
xa+yb=1
If Dr=|2r−12(3r−1)4(5r−1)xyz2n−13n−15n−1| then prove that n∑r=1Dr=0
The trace of a square matrix is defined to be the sum of its diagonal entries. If A is a 2×2 matrix such that the trace of A is 3 and the trace of A3 is −18, then the value of the determinant of A is ______.
Class 12 Engineering Maths Extra Questions
- Continuity And Differentiability Extra Questions
- Determinants Extra Questions
- Differential Equations Extra Questions
- Integrals Extra Questions
- Inverse Trigonometric Functions Extra Questions
- Relations And Functions Extra Questions
- Three Dimensional Geometry Extra Questions
- Vector Algebra Extra Questions