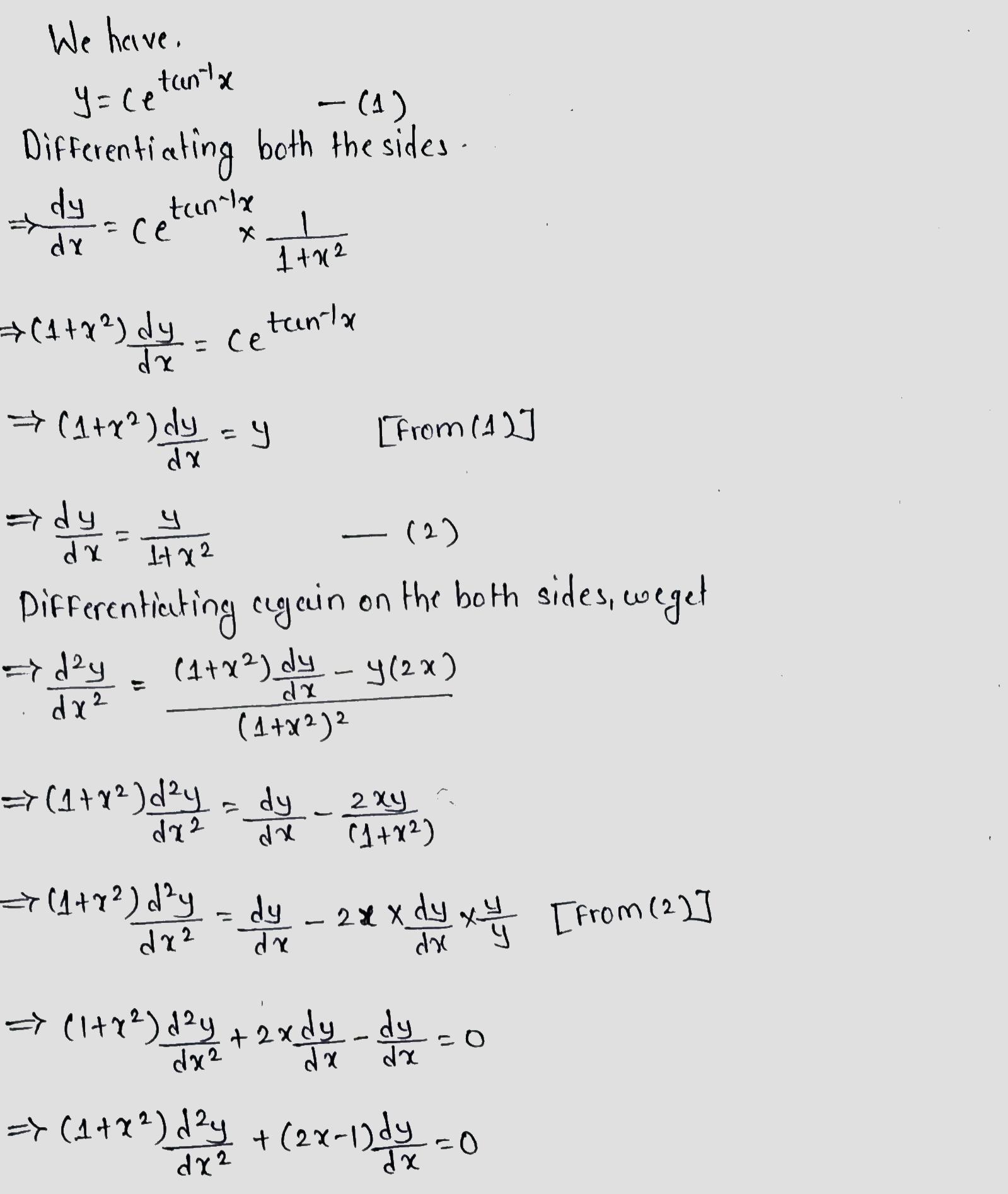Differential Equations - Class 12 Engineering Maths - Extra Questions
Find the order and degree of the differential equations.
a) y′′+3y′2+y3=0
b) (dydx)2+1dydx=2
c) d2ydx2+4y=0
cos(dydx)=a(a∈R);y=1 when x=0.Find y?
Show that y=cx+ac is a solution of the differential equation y=xdydx+adydx.
Write the integrating factor of the differential equation:
cosxdydx+y=sinx;0≤x<π2.
Find the integrating factor of dydx+yx=x2.
Find the differential coefficient of tan−1x w.r to x.
Consider the following equation, dydx+P(x)y=Q(x)
(i) If two particular solutions of given equation u(x) and v(x) are known, find the general solution of the same equation in terms of u(x) and v(x).
(ii) If α and β are constants such that the linear combinations α⋅u(x)+β⋅v(x) is a solution of the given equation, find the relation between α and β.
(iii) If w(x) is the third particular solution different from u(x) and v(x) then find the ratio v(x)−u(x)w(x)−u(x).
Find the general solution of the differential equation:
xdy−ydx=√x2+y2dx
Verify that y=4sin3x is the solution of the differential equation d2ydx2+9y=0.
Find order and degree of the following differential equations .
(i) dydx+y=1dydx
(ii)e[dydx−d3ydx3]=ln[d5ydx5+1]
(iii)[(dydx)1/3+y]2=d2ydx2
Find the particular solution of the difference equation
(1−y2)(1+logx)dx+2xydy=0
given that y=0 when x=1
Solve: cos2xdydx+y=tanx.
Find the order of the differential equation obtained by eliminating the arbitrary constants b and c from xy=cex+be−x+x2.
Solve: dydx=cos(x+y)+sin(x+y)
If y=(cos−1x)2, prove that (1−x2)d2ydx2−xdydx−2=0. Hence find y2 when x=0.
If xy=ex−y, show that dydx=ylogxx(logx+1)
Write the order of the differential equation 1+(dydx)2=7(d2ydx2)3.
Show that xy=aex+be−x+x2 is a solution of the differential equation xd2ydx2+2dydx−xy+x2−2=0.
Verify that y=Acos2x−Bsin2x is the general solution of the differential equation d2ydx2+4y=0.
Verify that y=cetan−1x is a solution of the differential equation (1+x2)d2ydx2+(2x−1)dydx=0.
The number of arbitrary constants in the general solution of a differential equation of order three is ______ .
Solve the following differential equation:
x2dydx=y2+2xy
Given that : y=1, when x=1.
Find the particular solution of the differential equation dydx=x(2logx+1)siny+ycosy given that y=π2 where x = 1.
Class 12 Engineering Maths Extra Questions
- Continuity And Differentiability Extra Questions
- Determinants Extra Questions
- Differential Equations Extra Questions
- Integrals Extra Questions
- Inverse Trigonometric Functions Extra Questions
- Relations And Functions Extra Questions
- Three Dimensional Geometry Extra Questions
- Vector Algebra Extra Questions