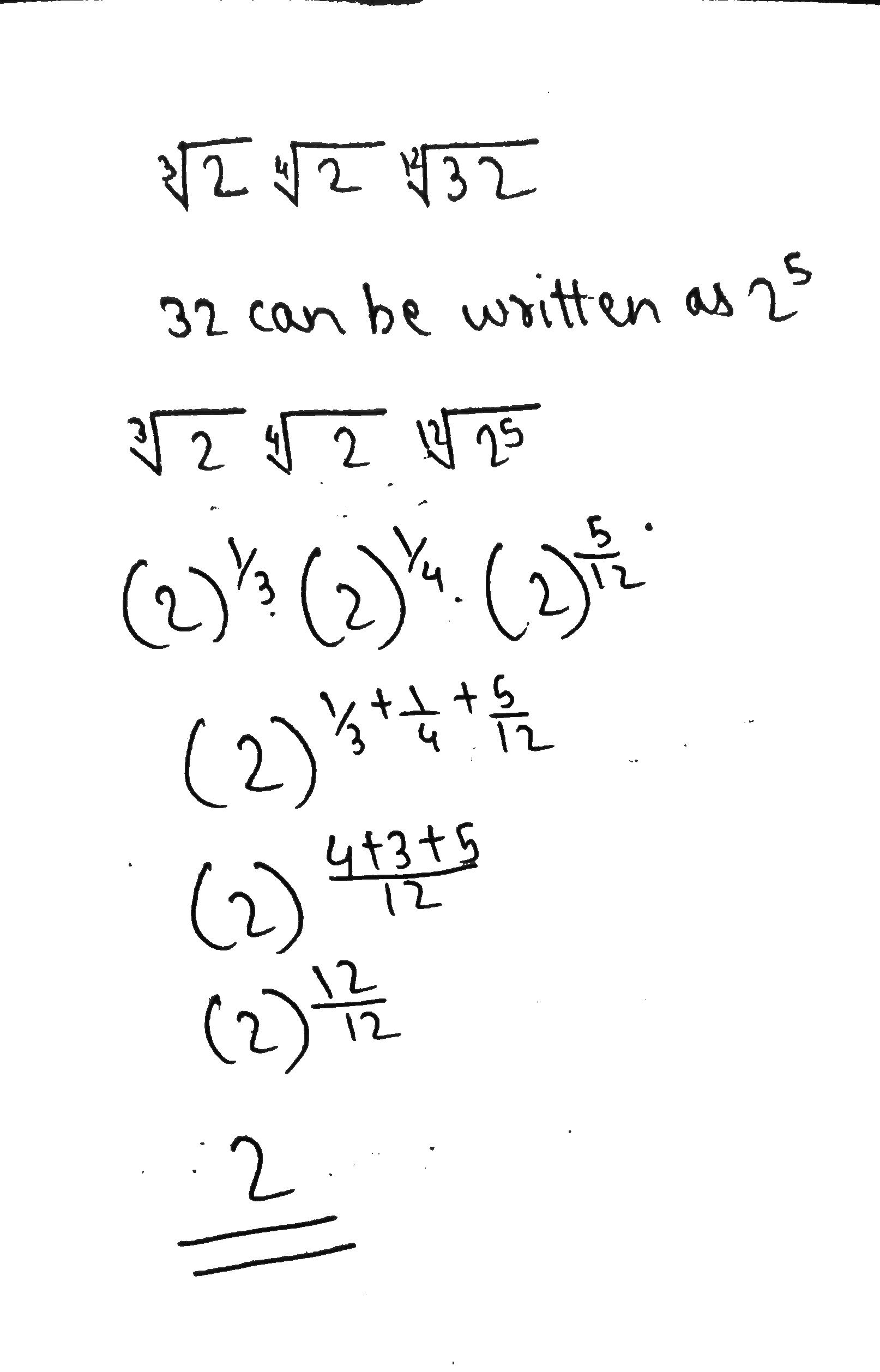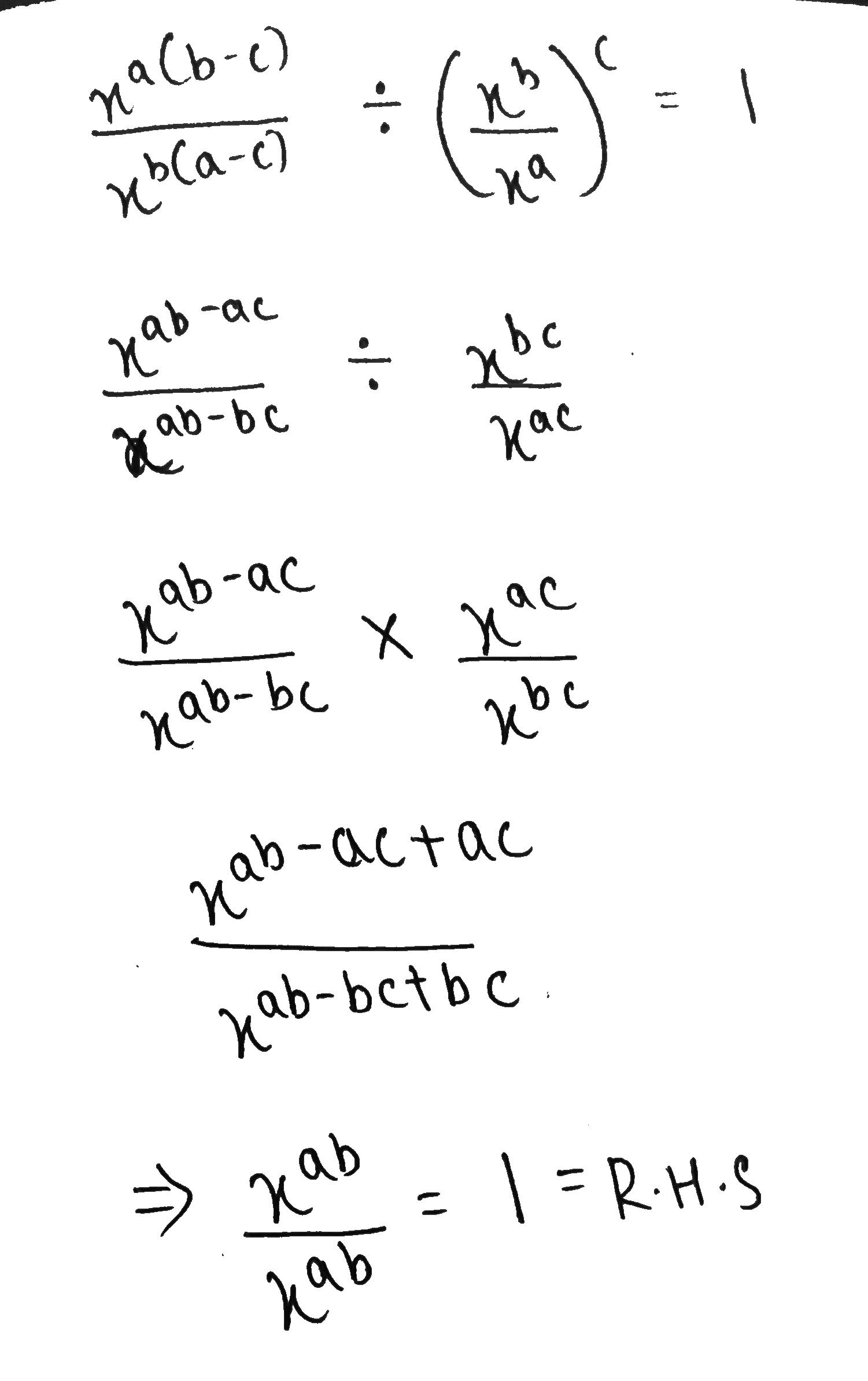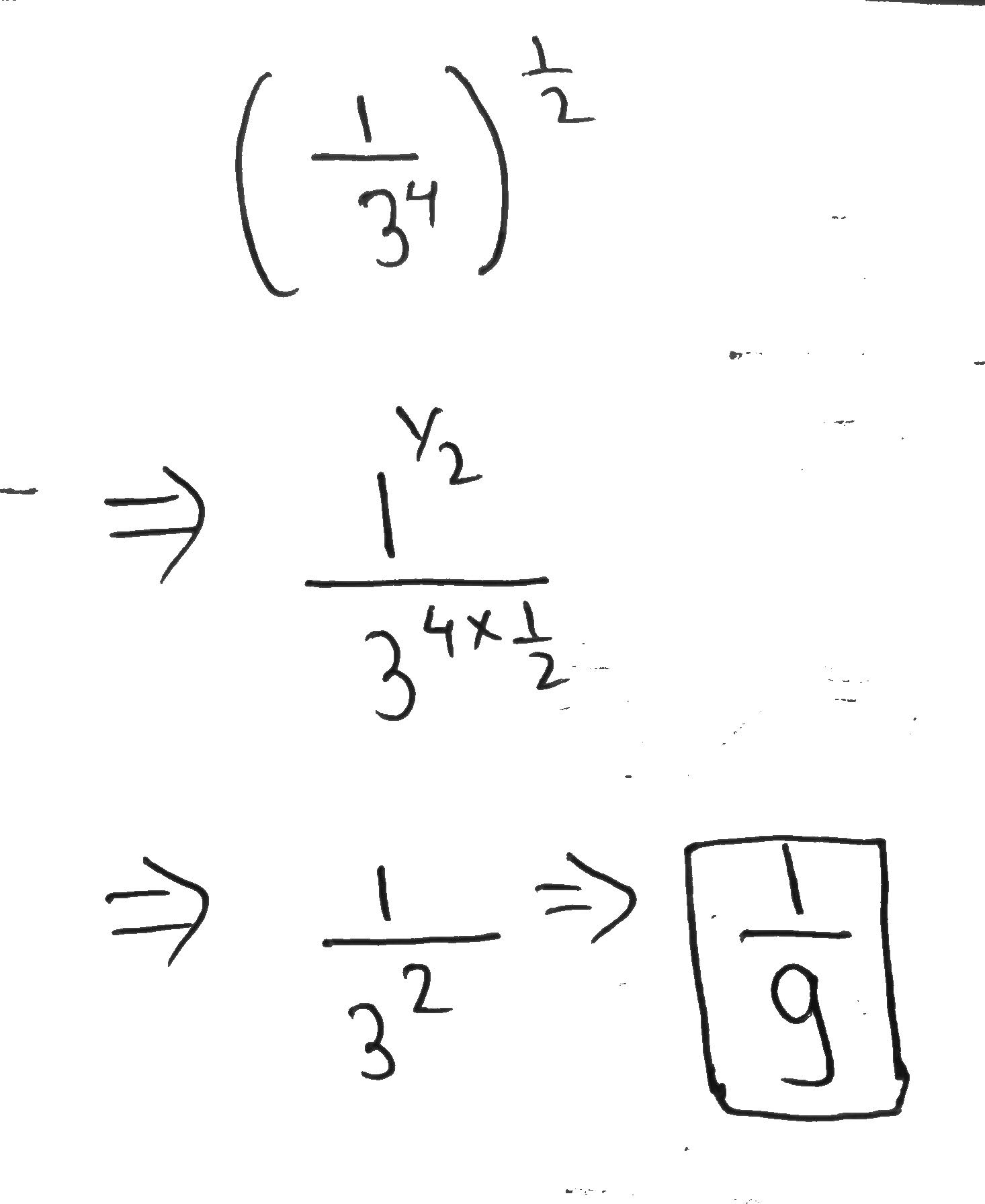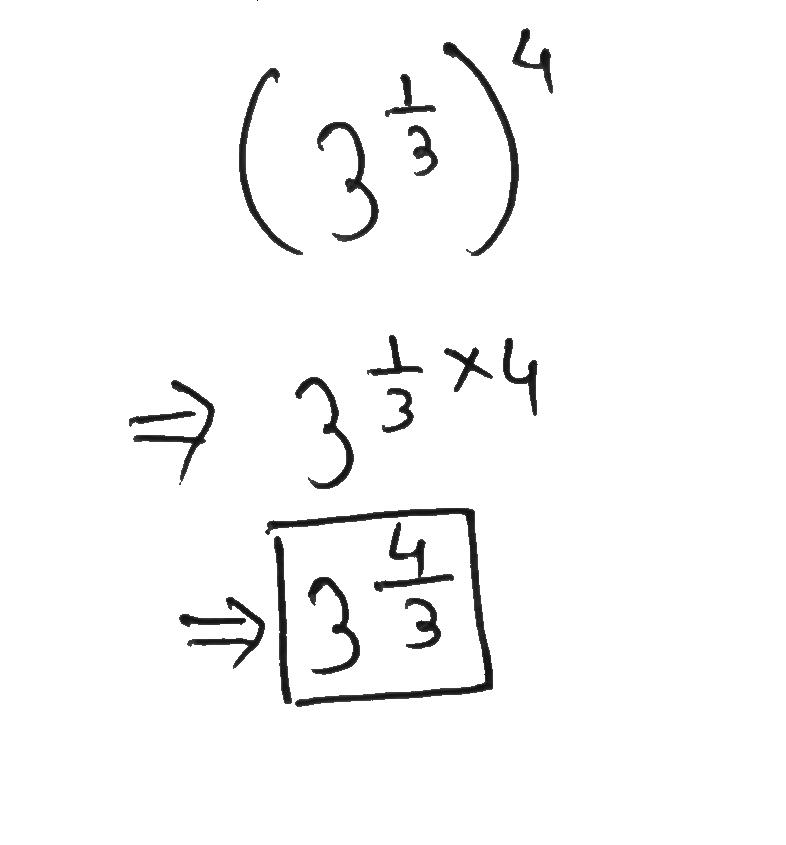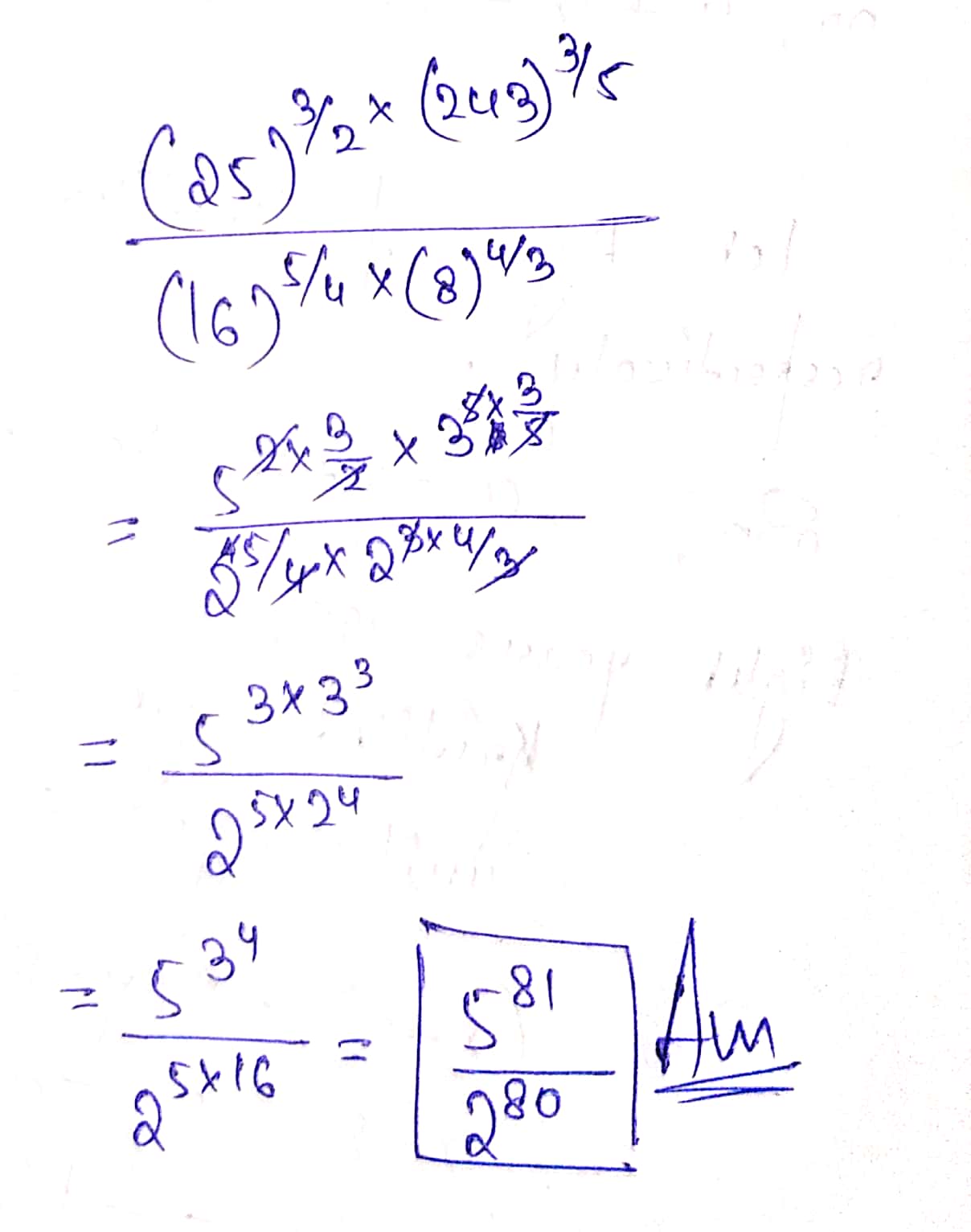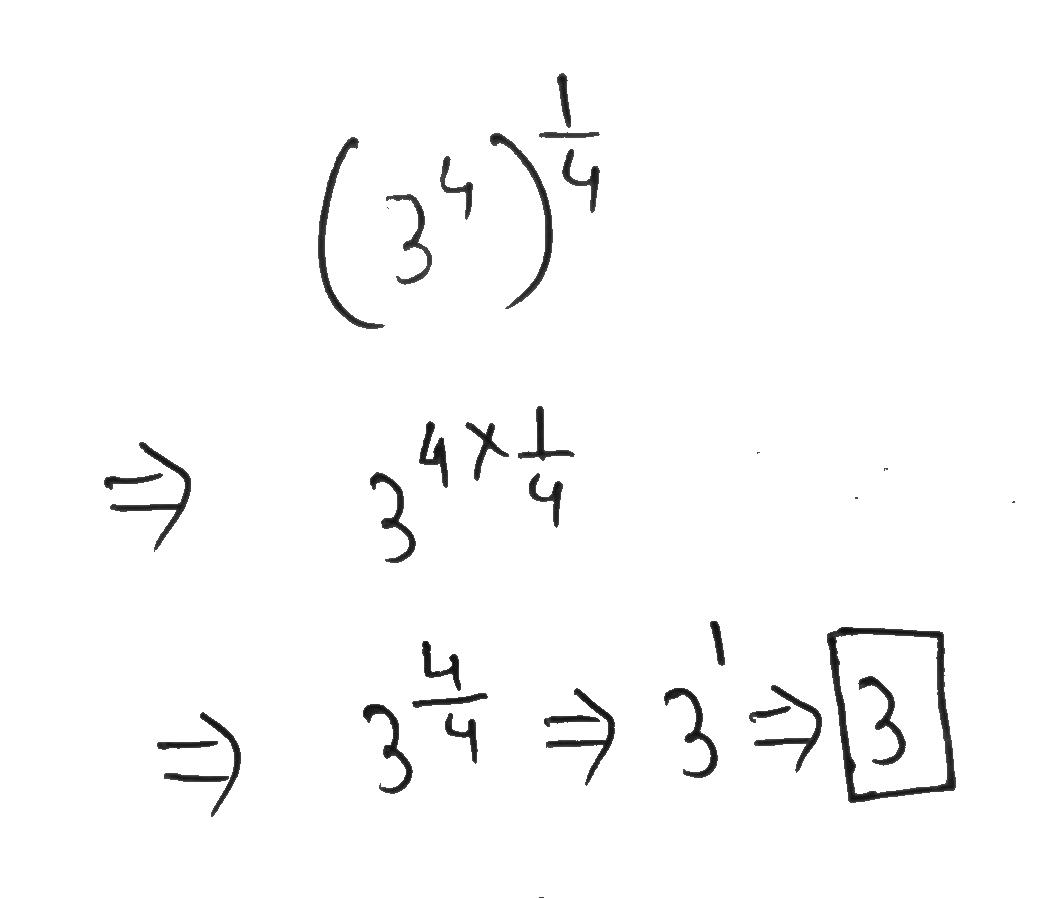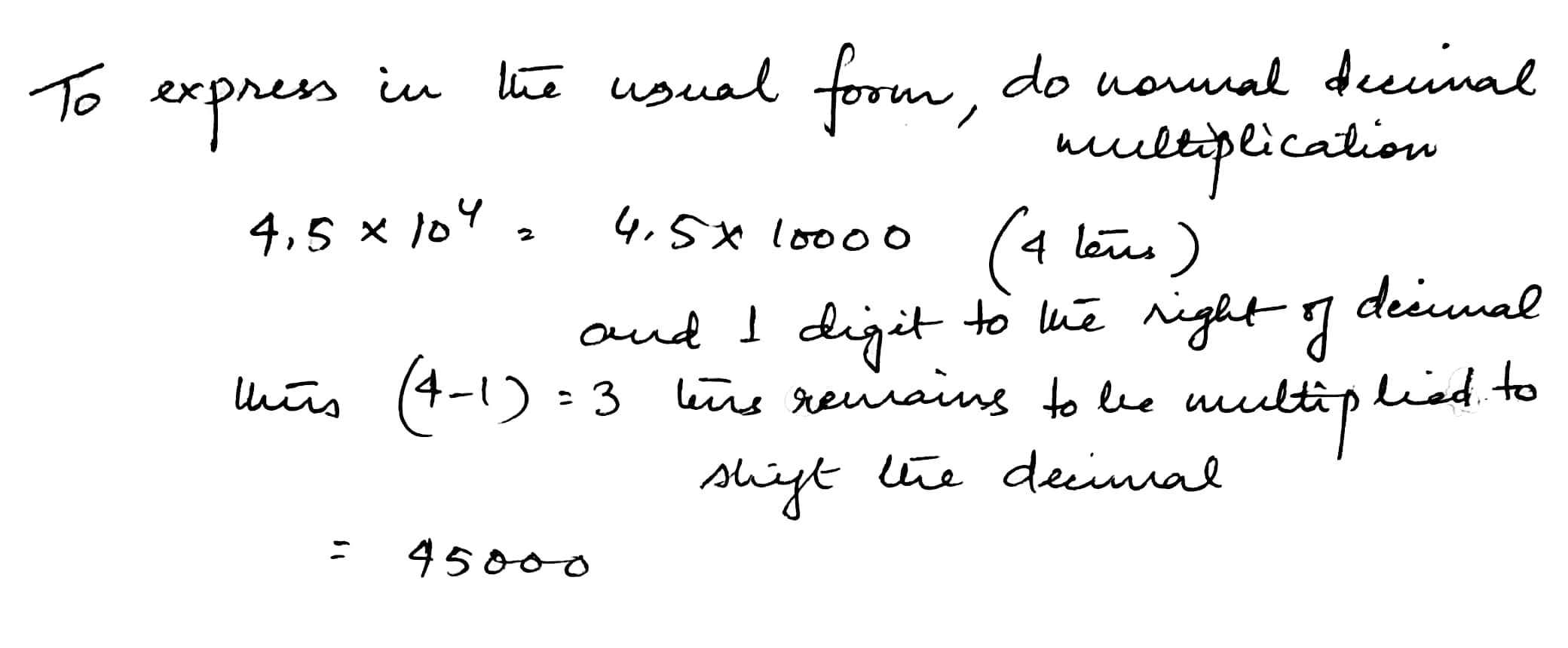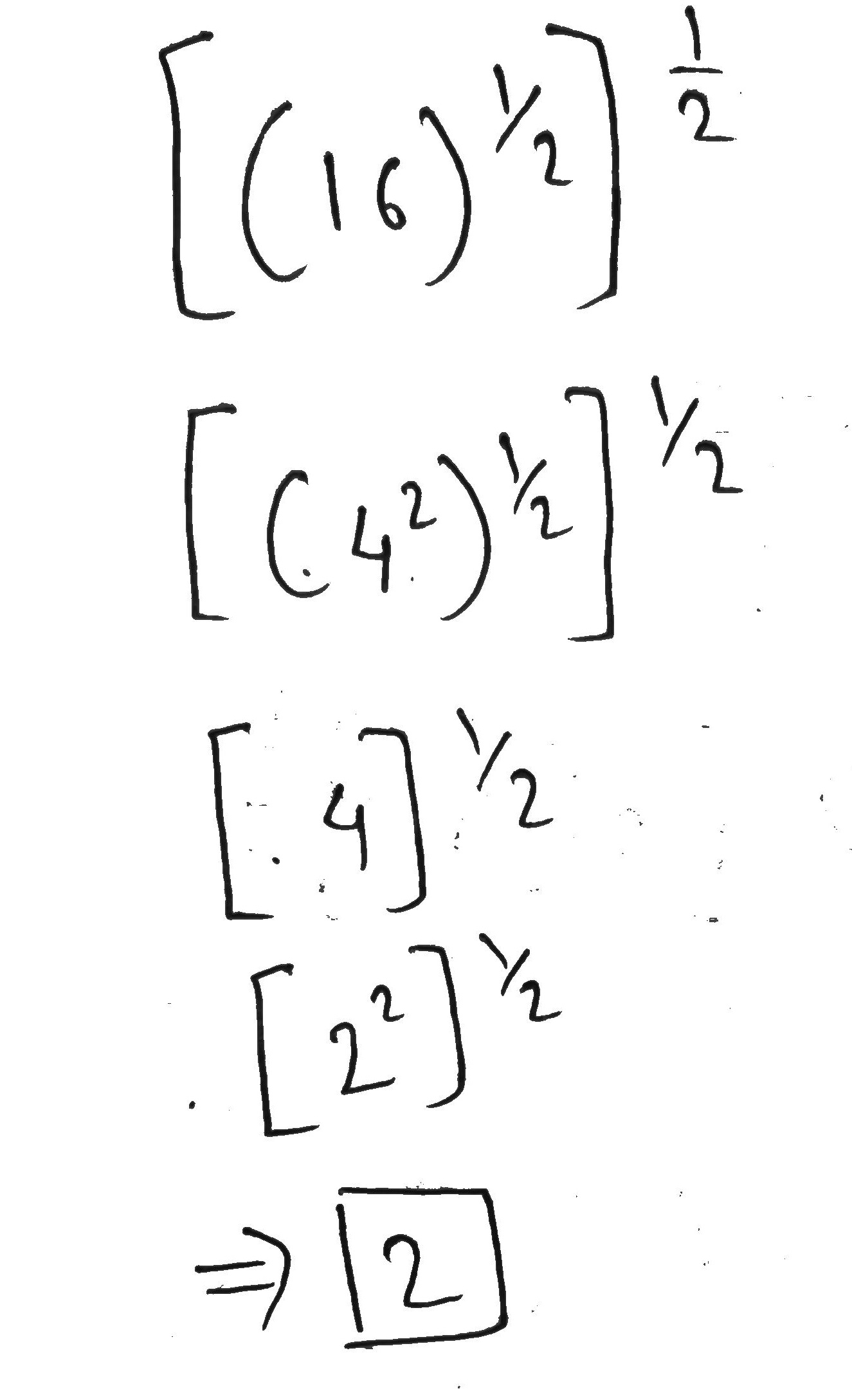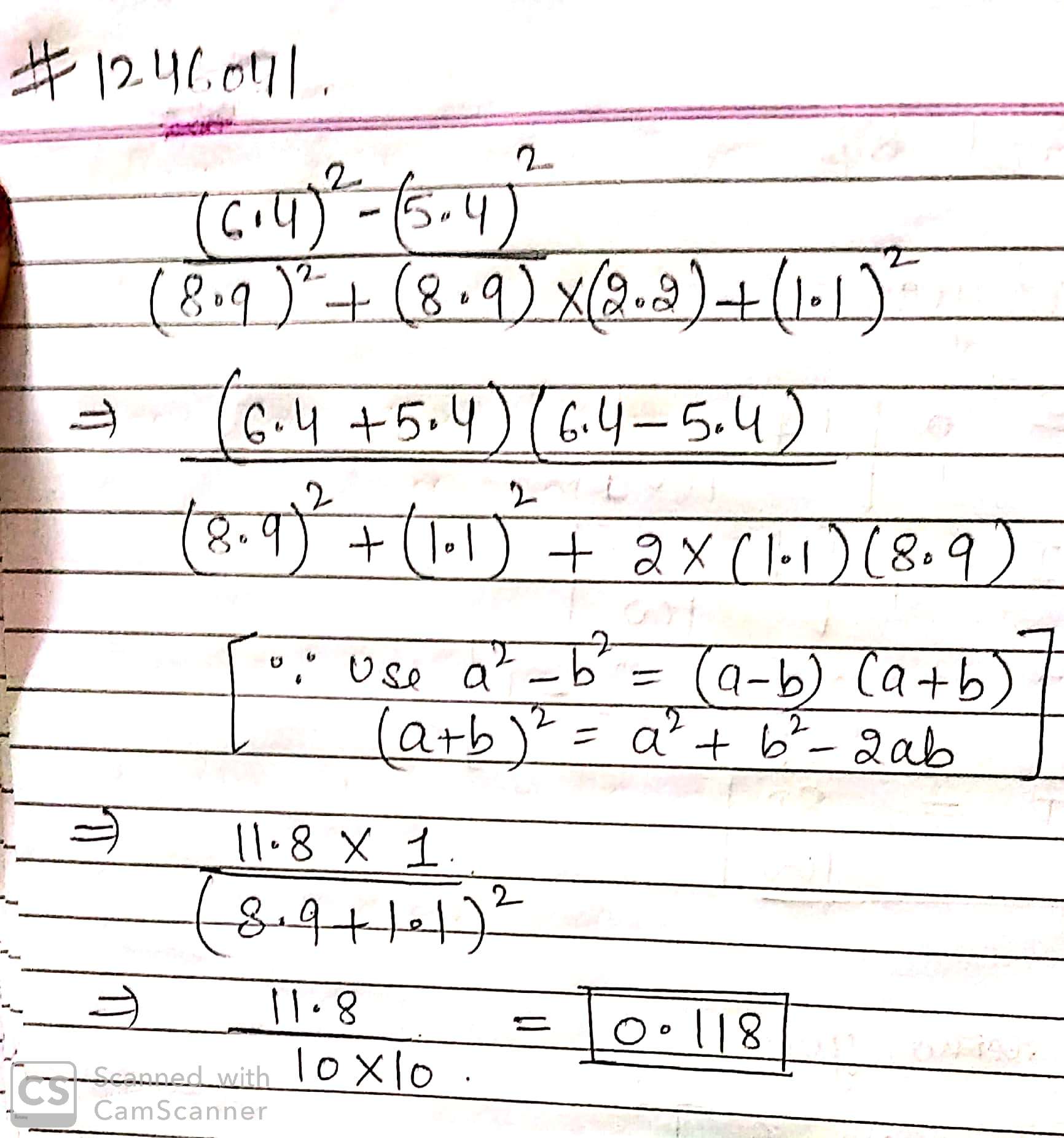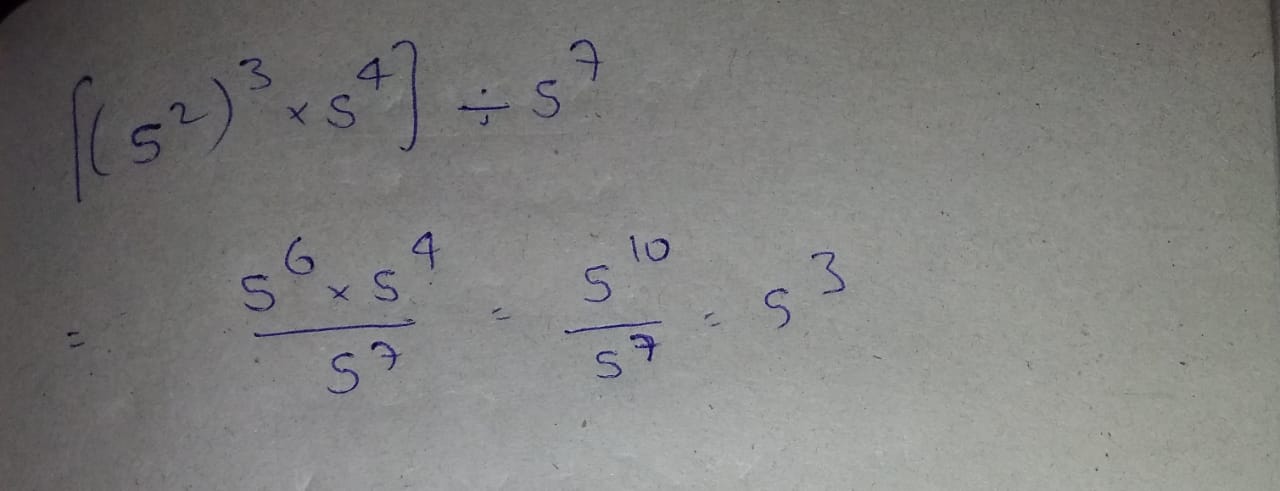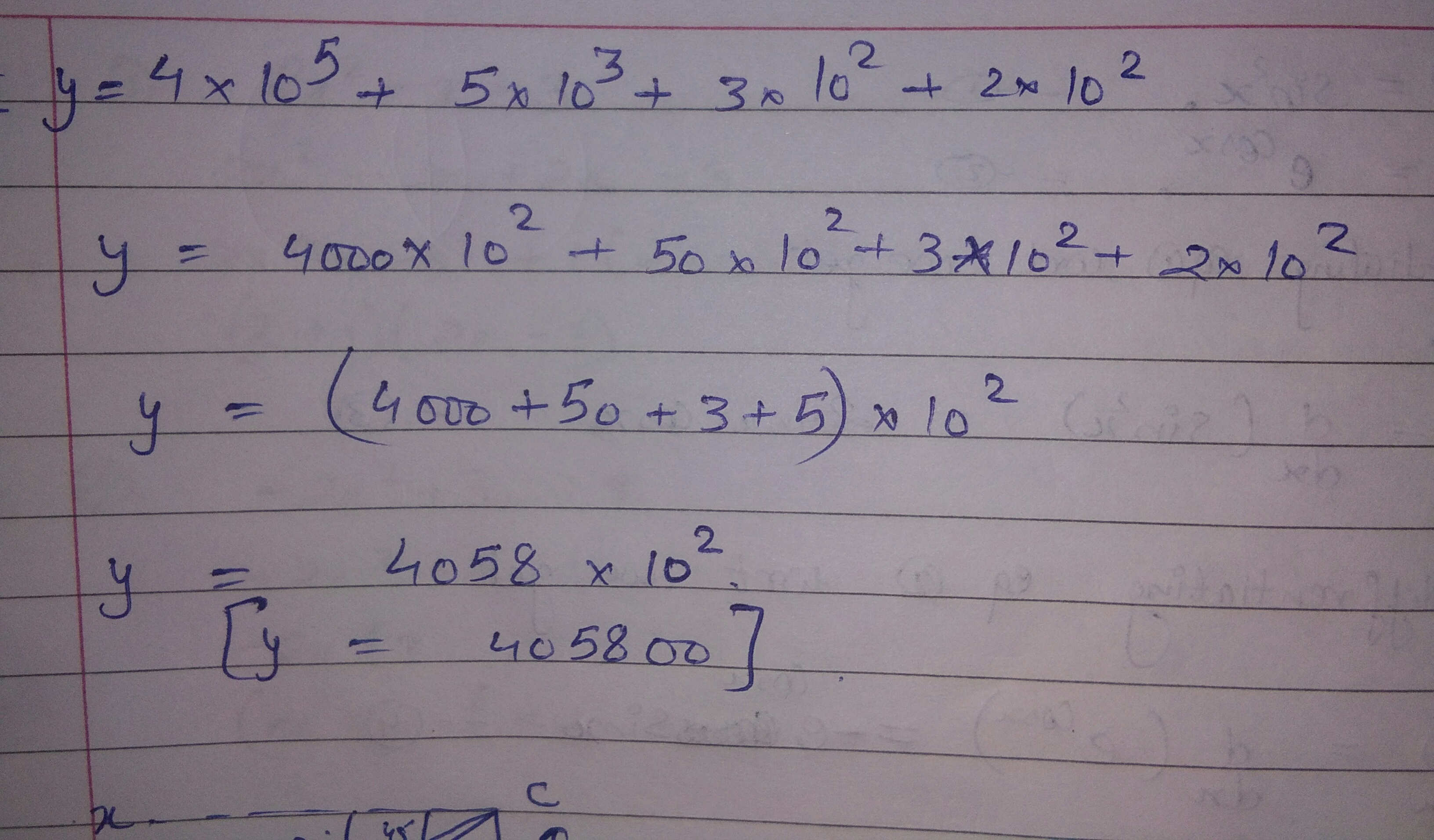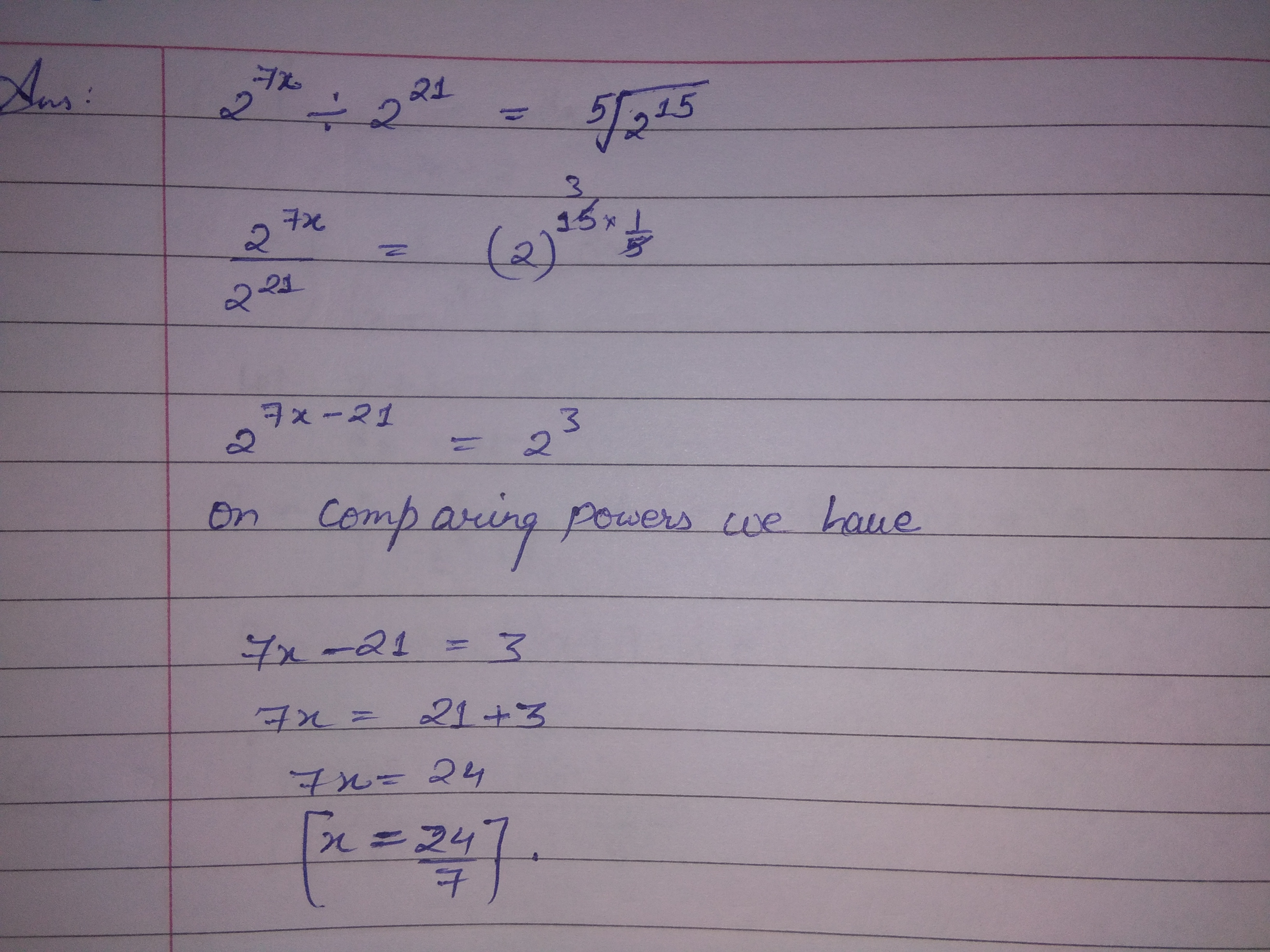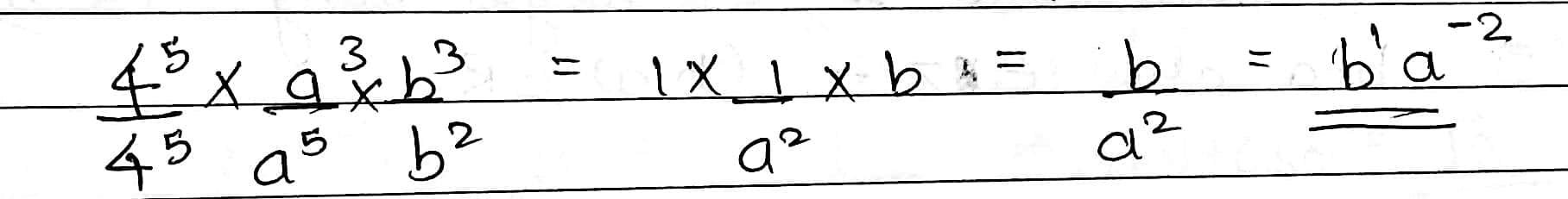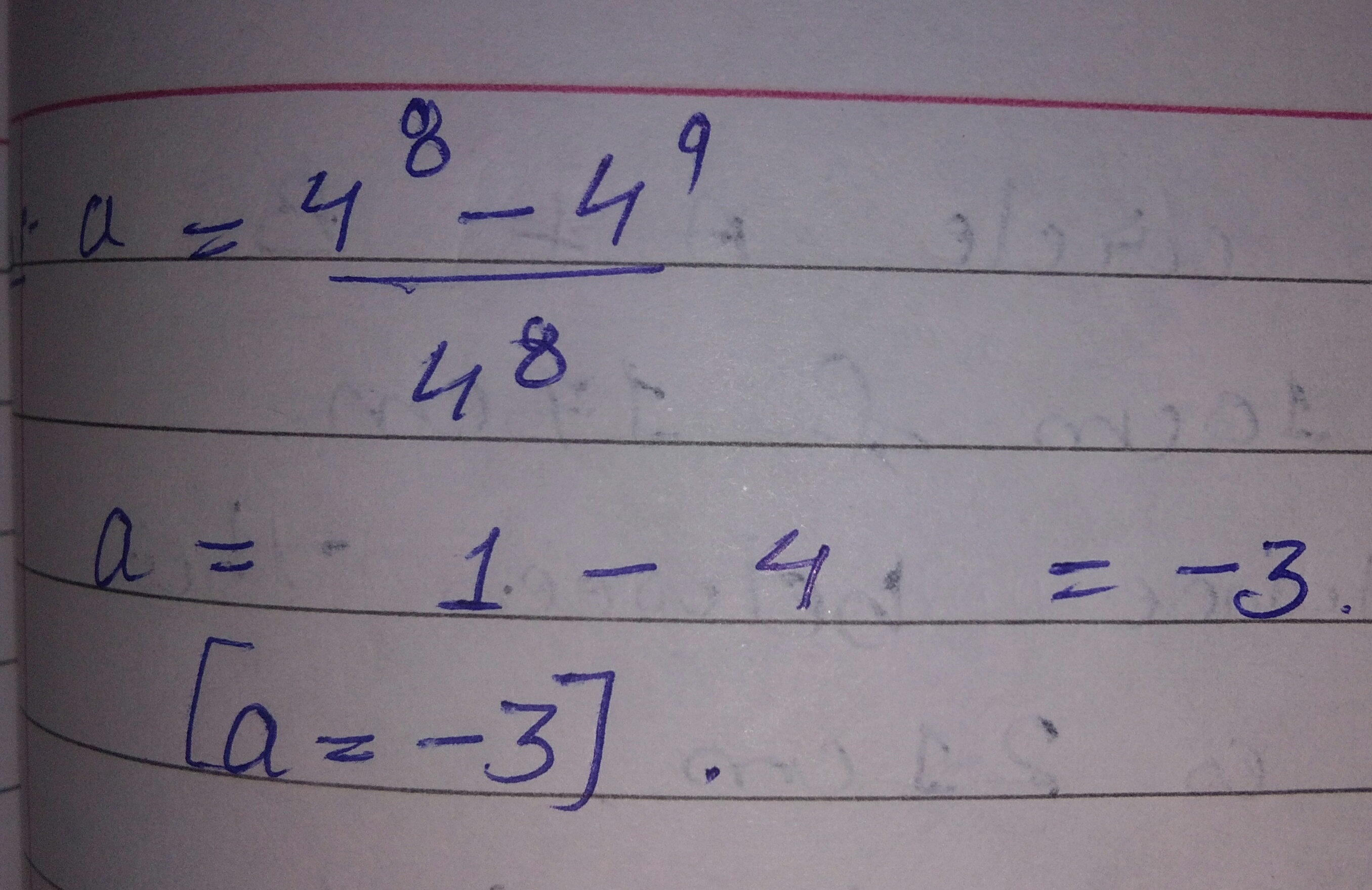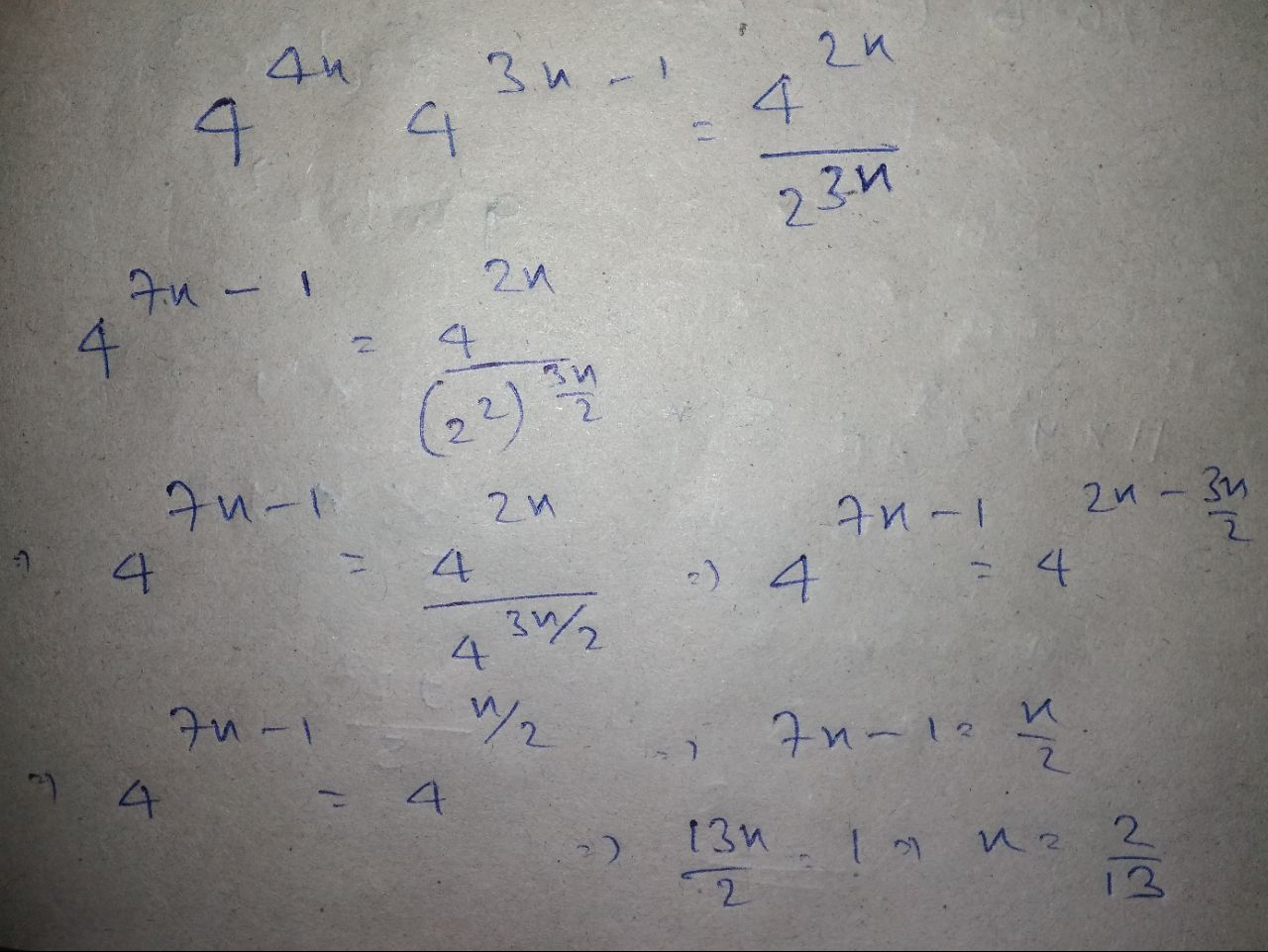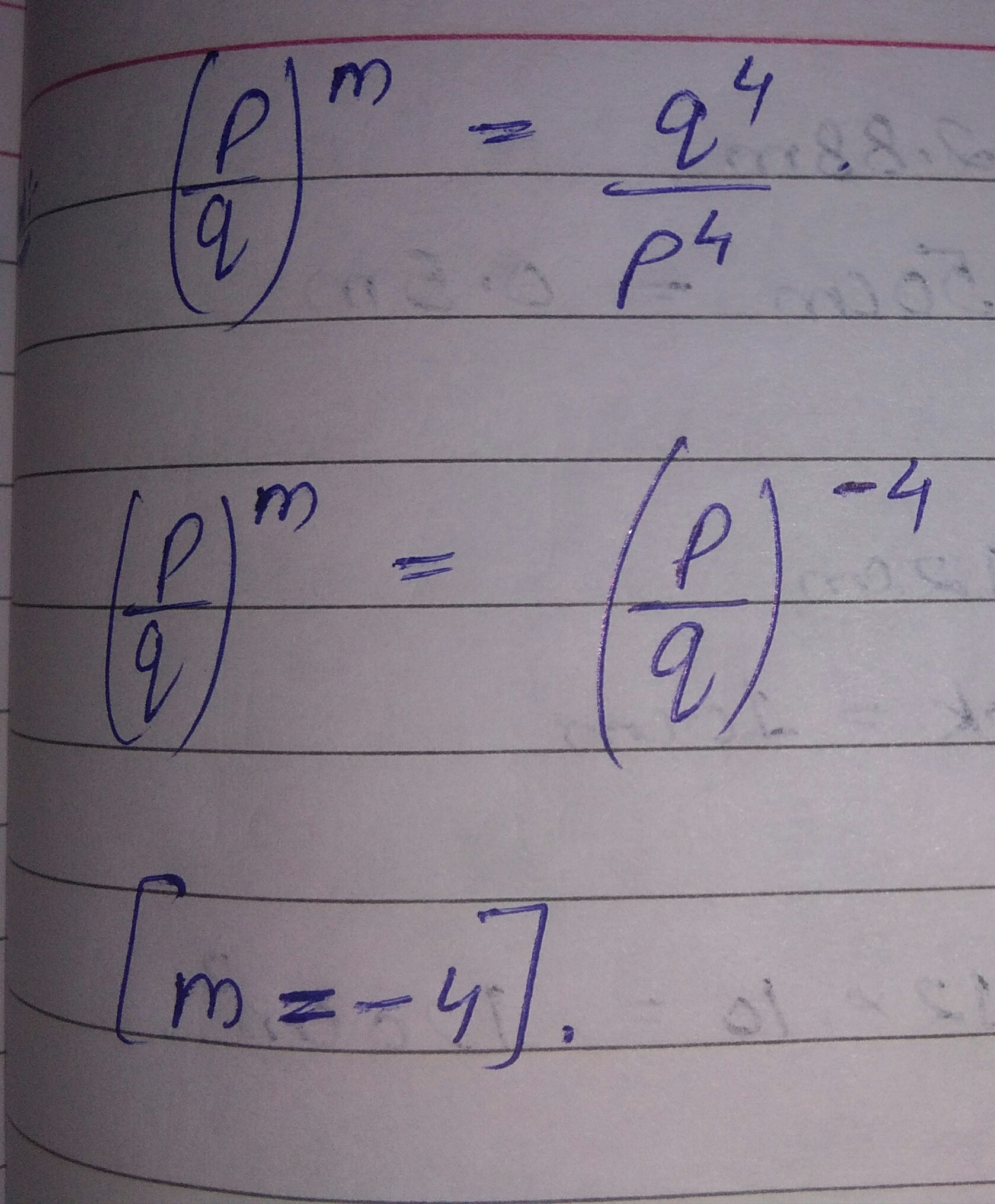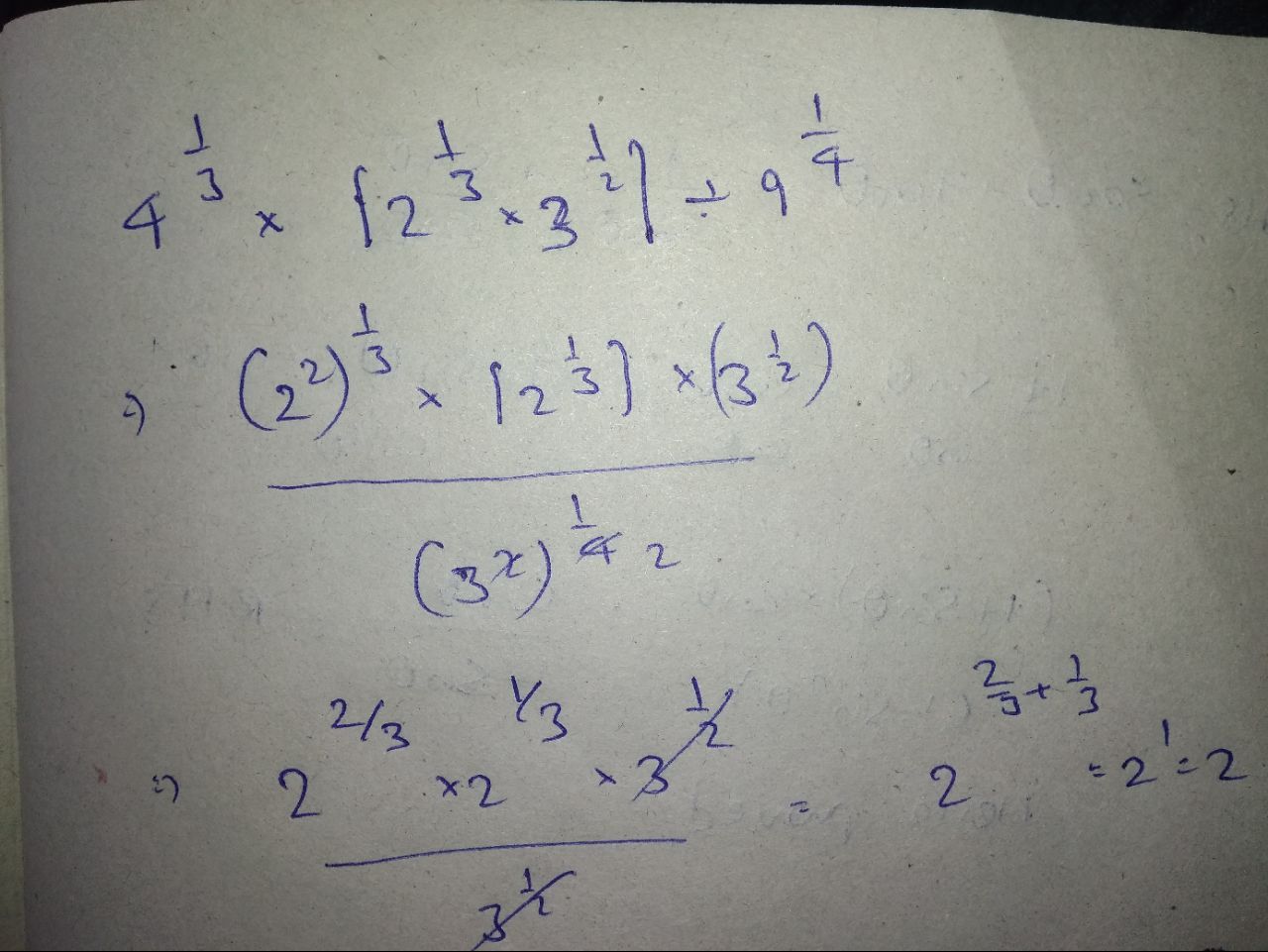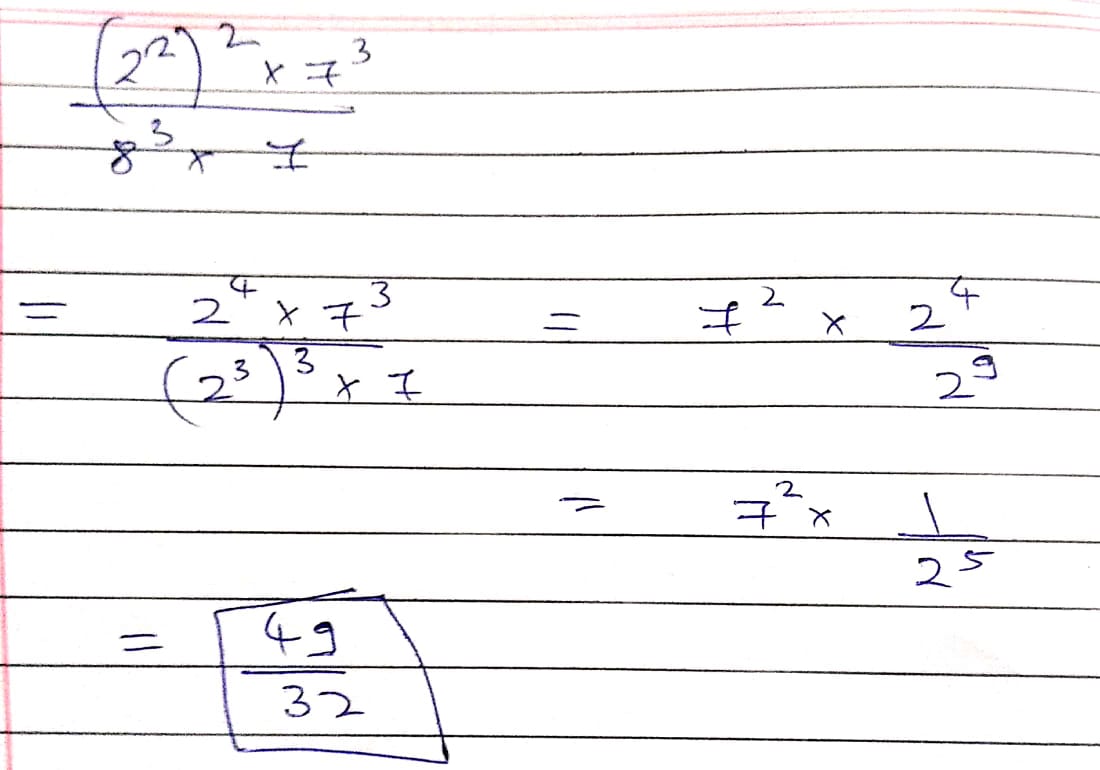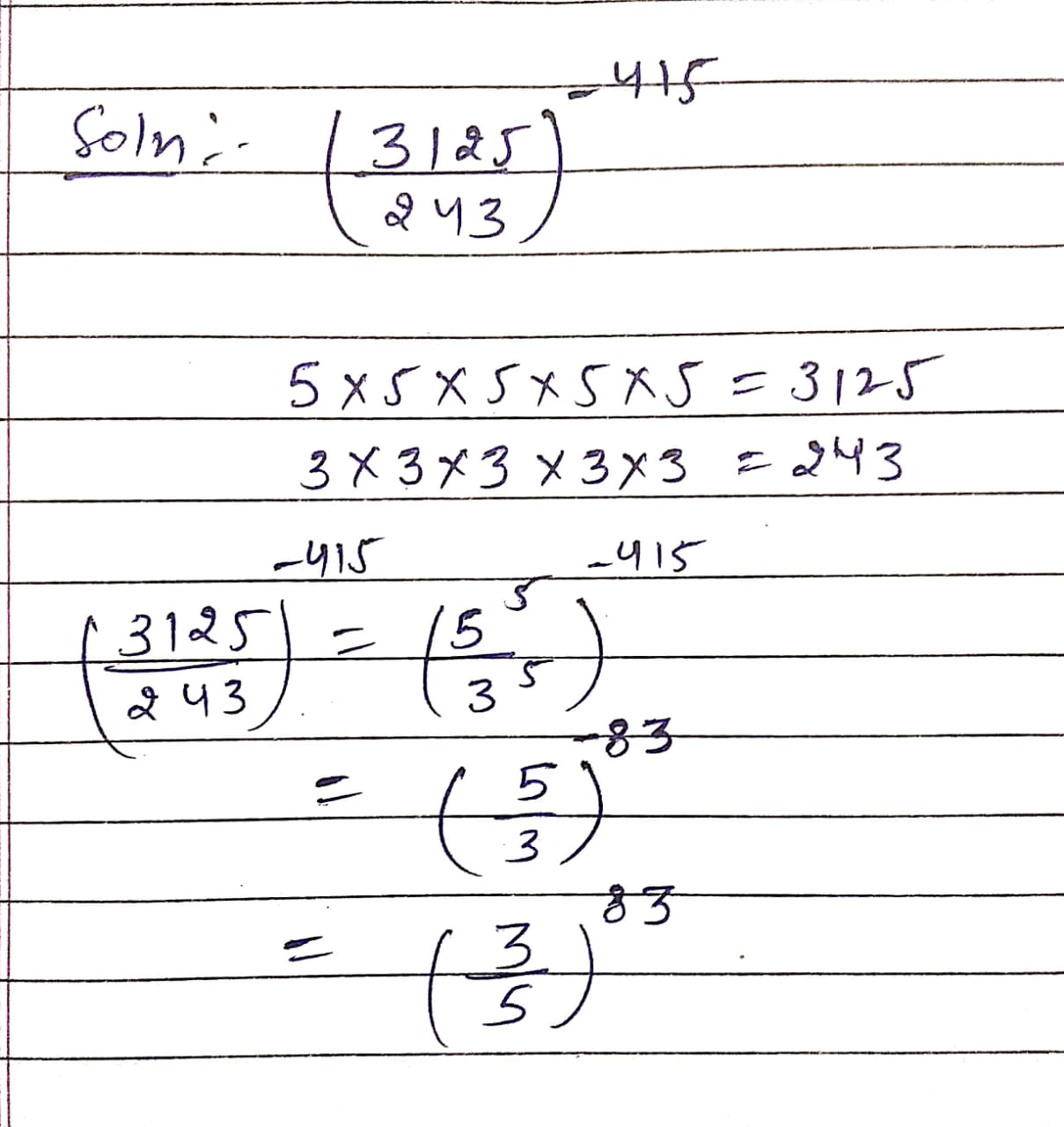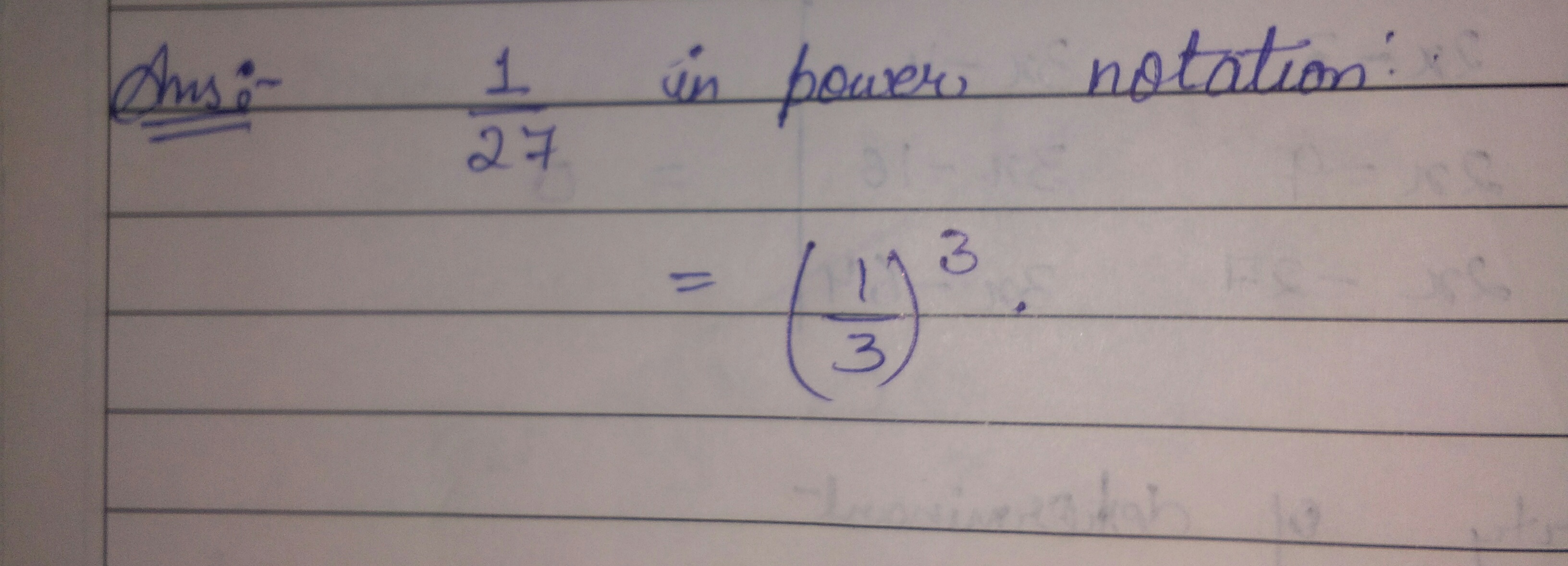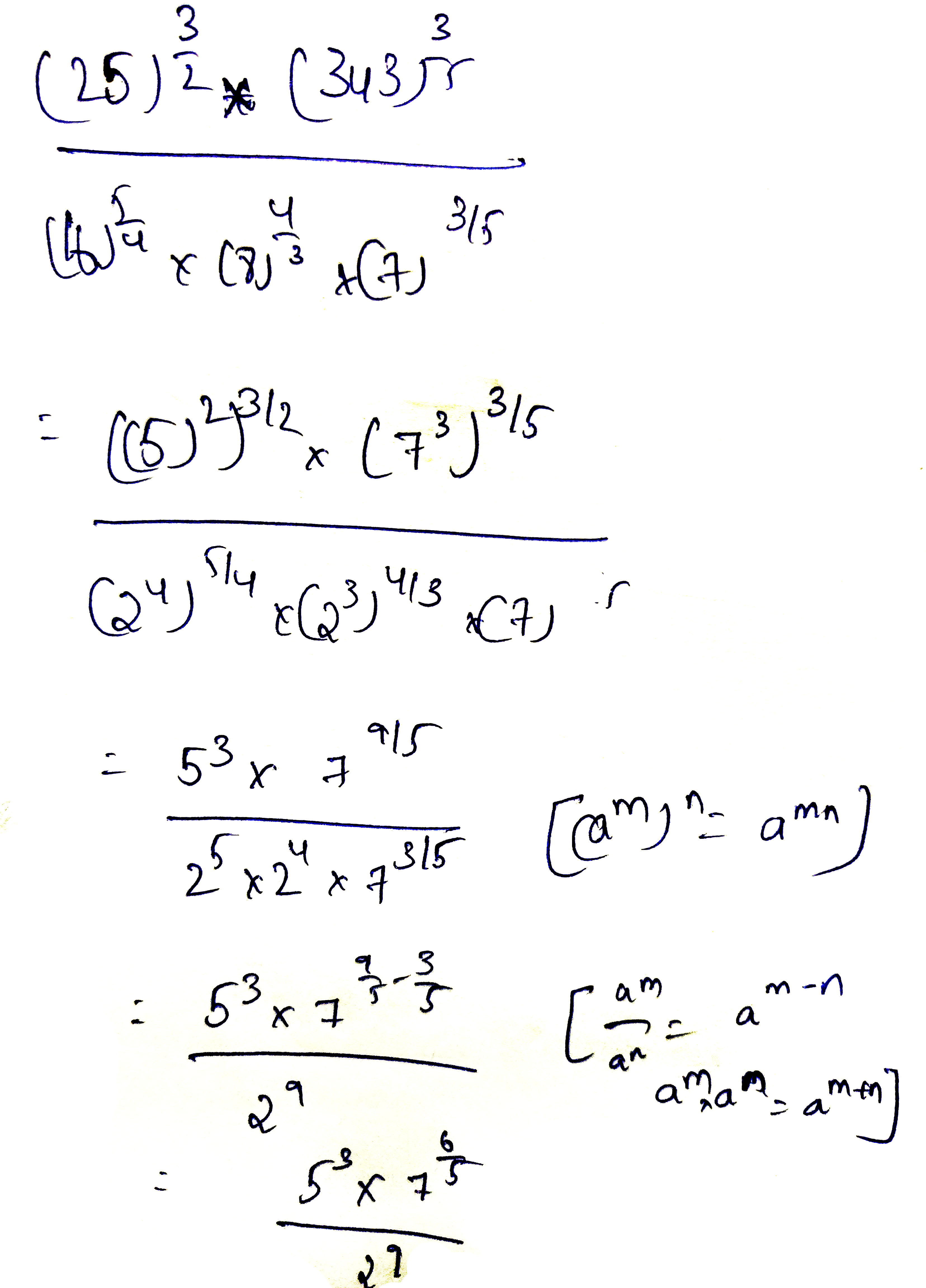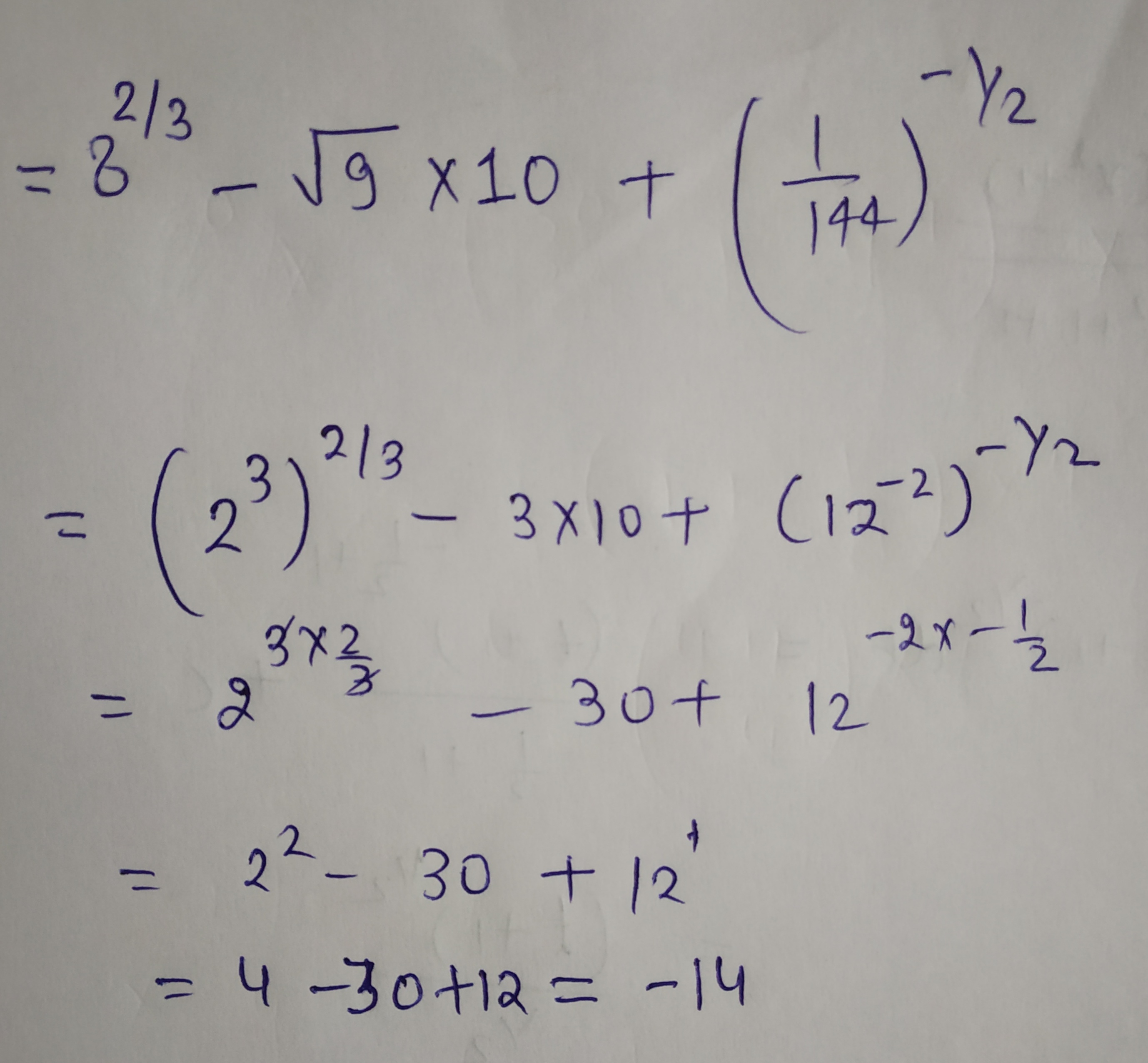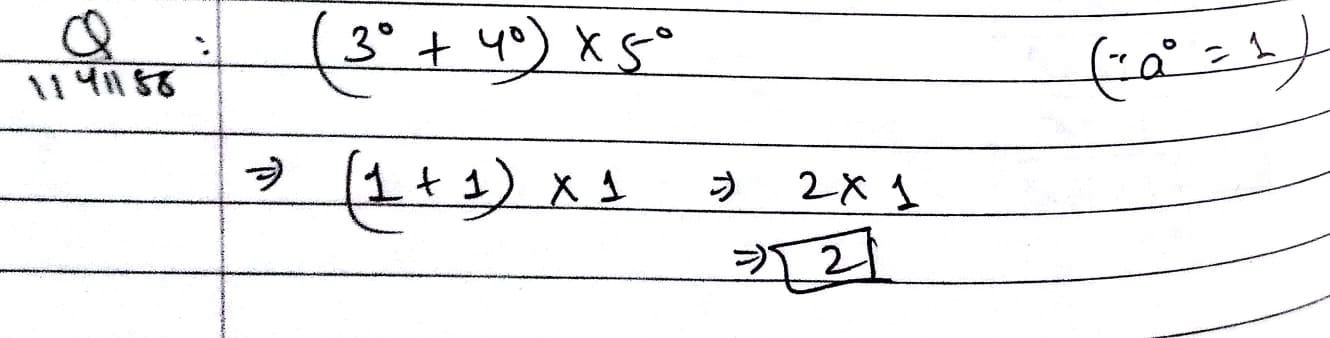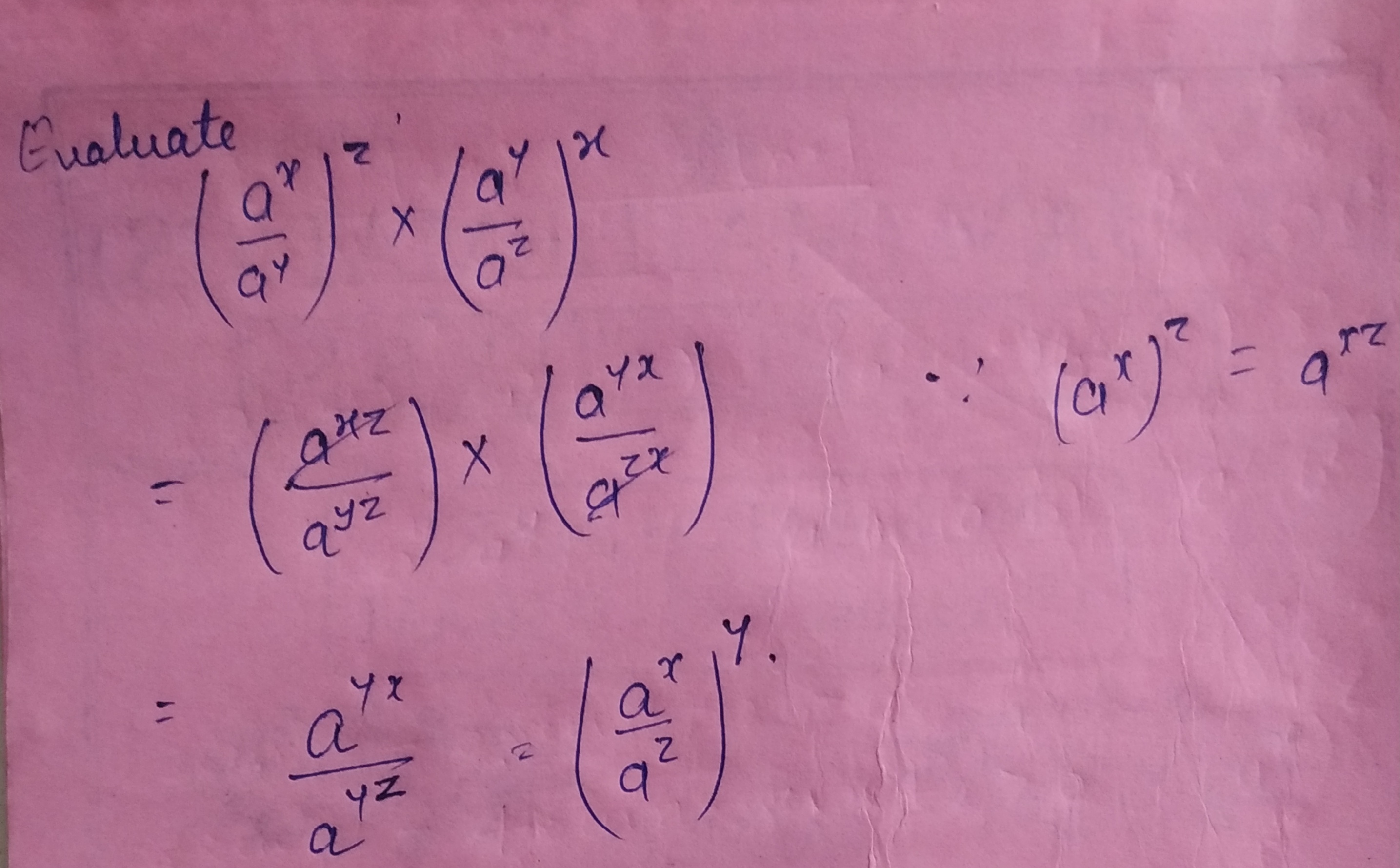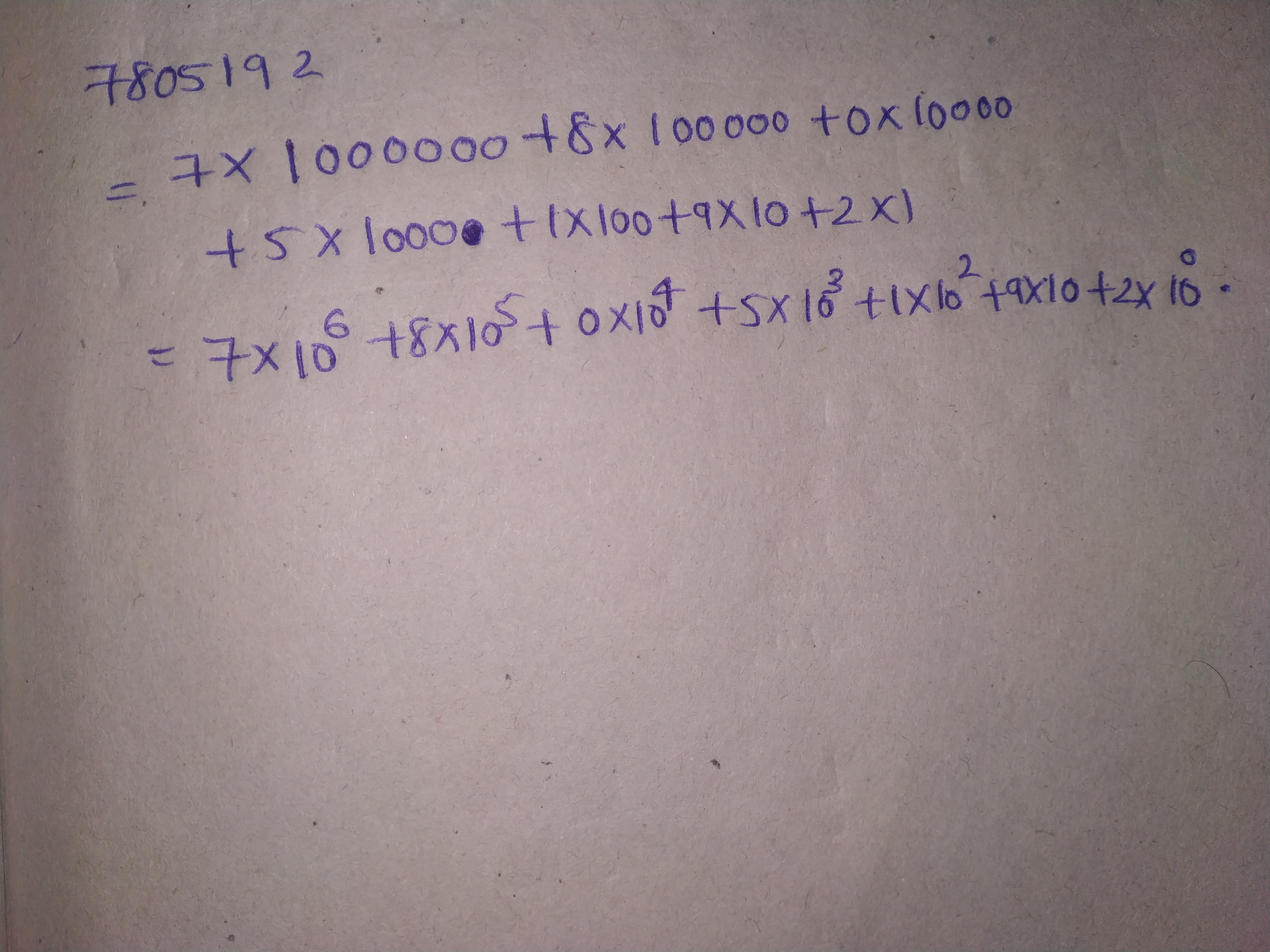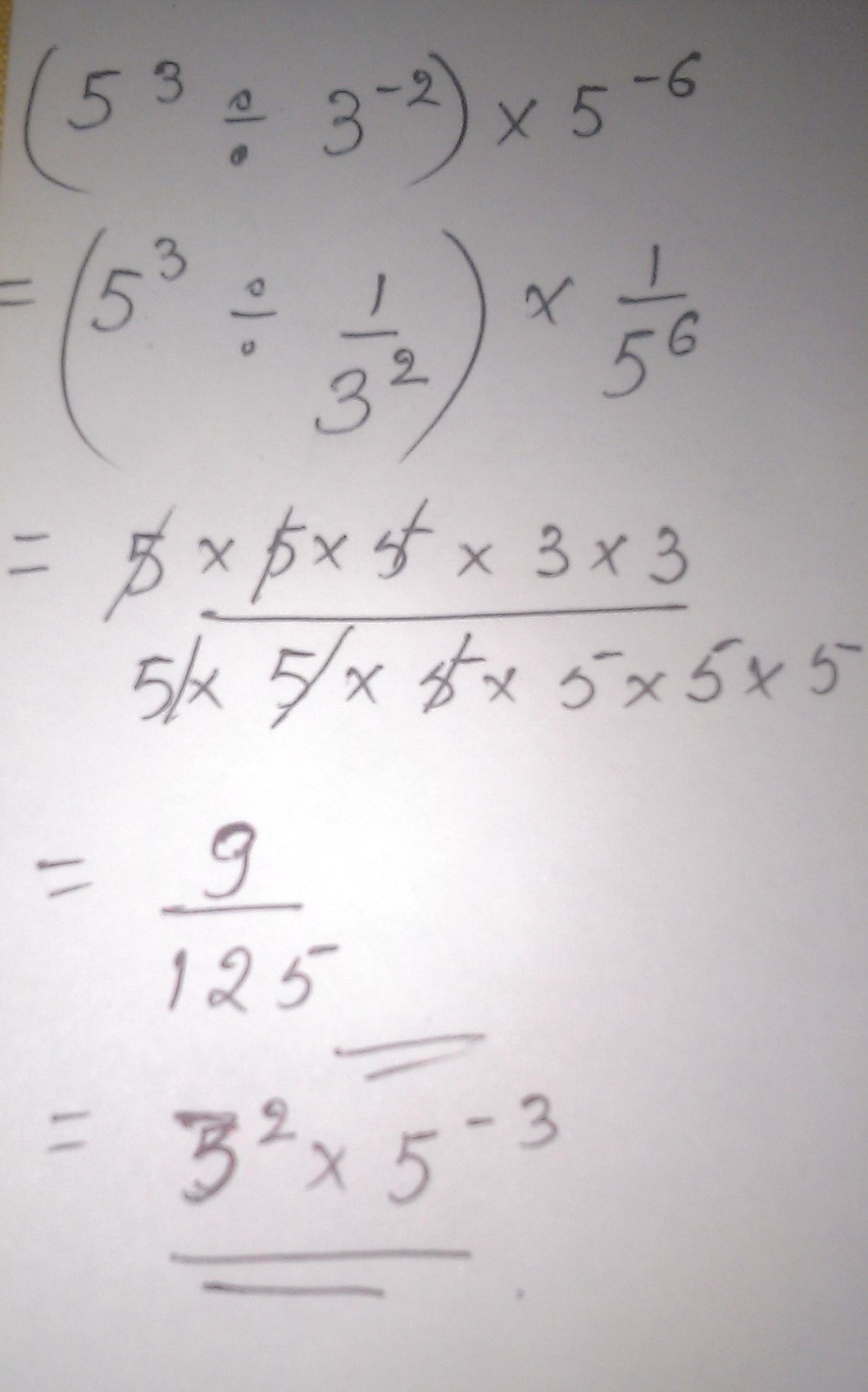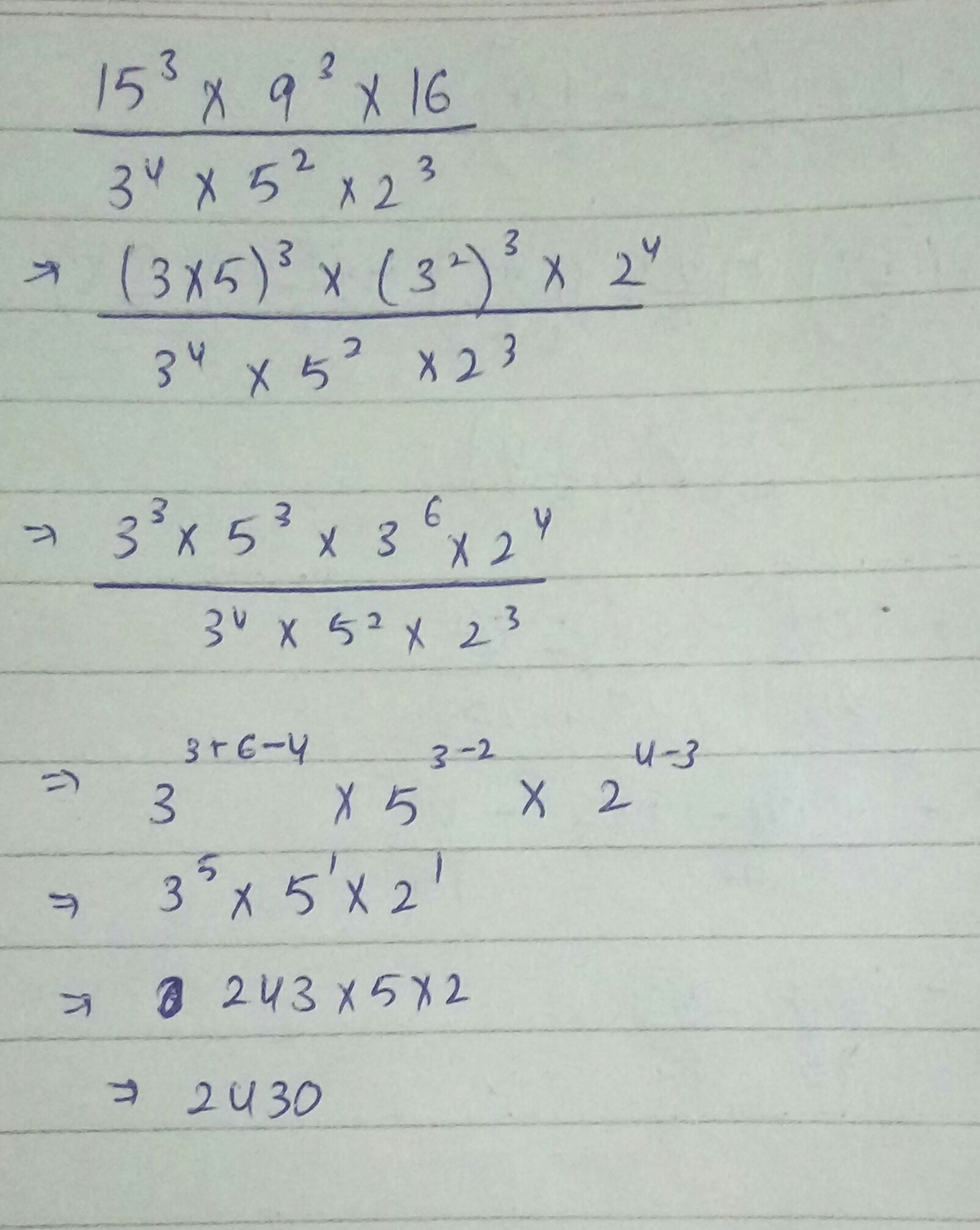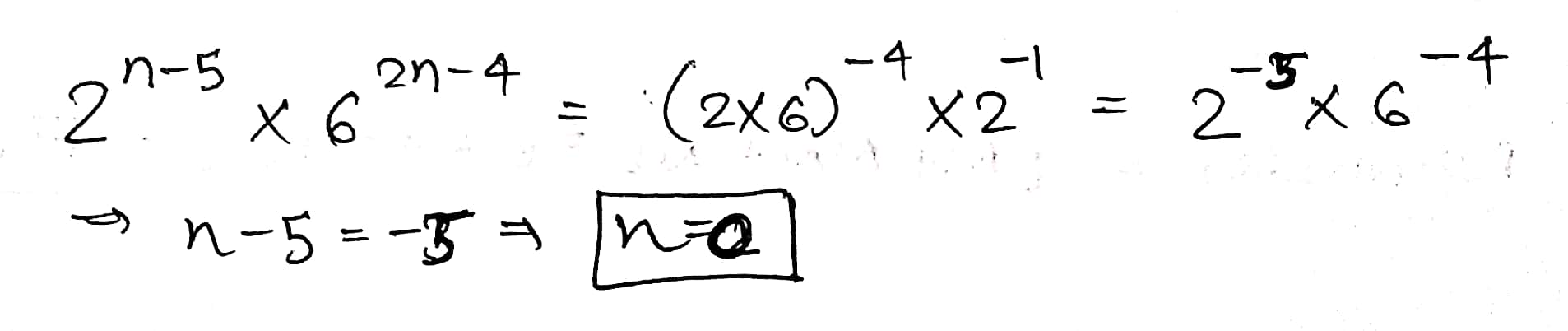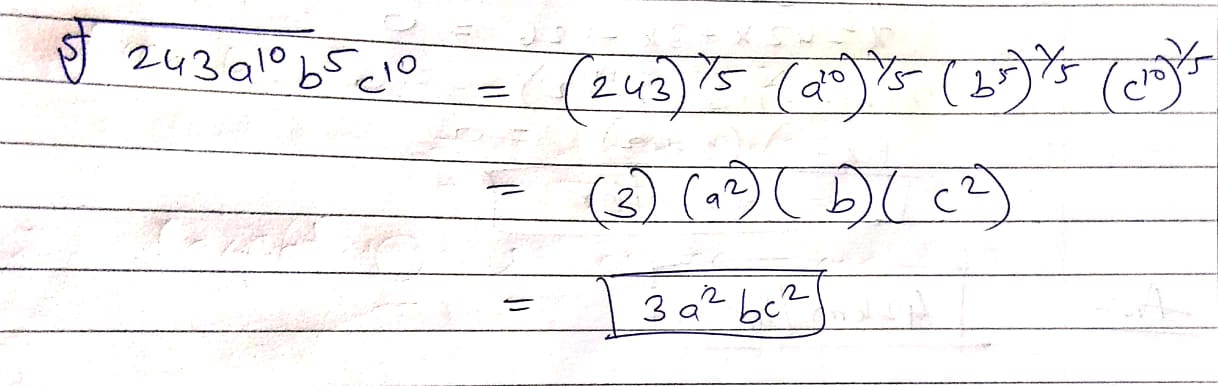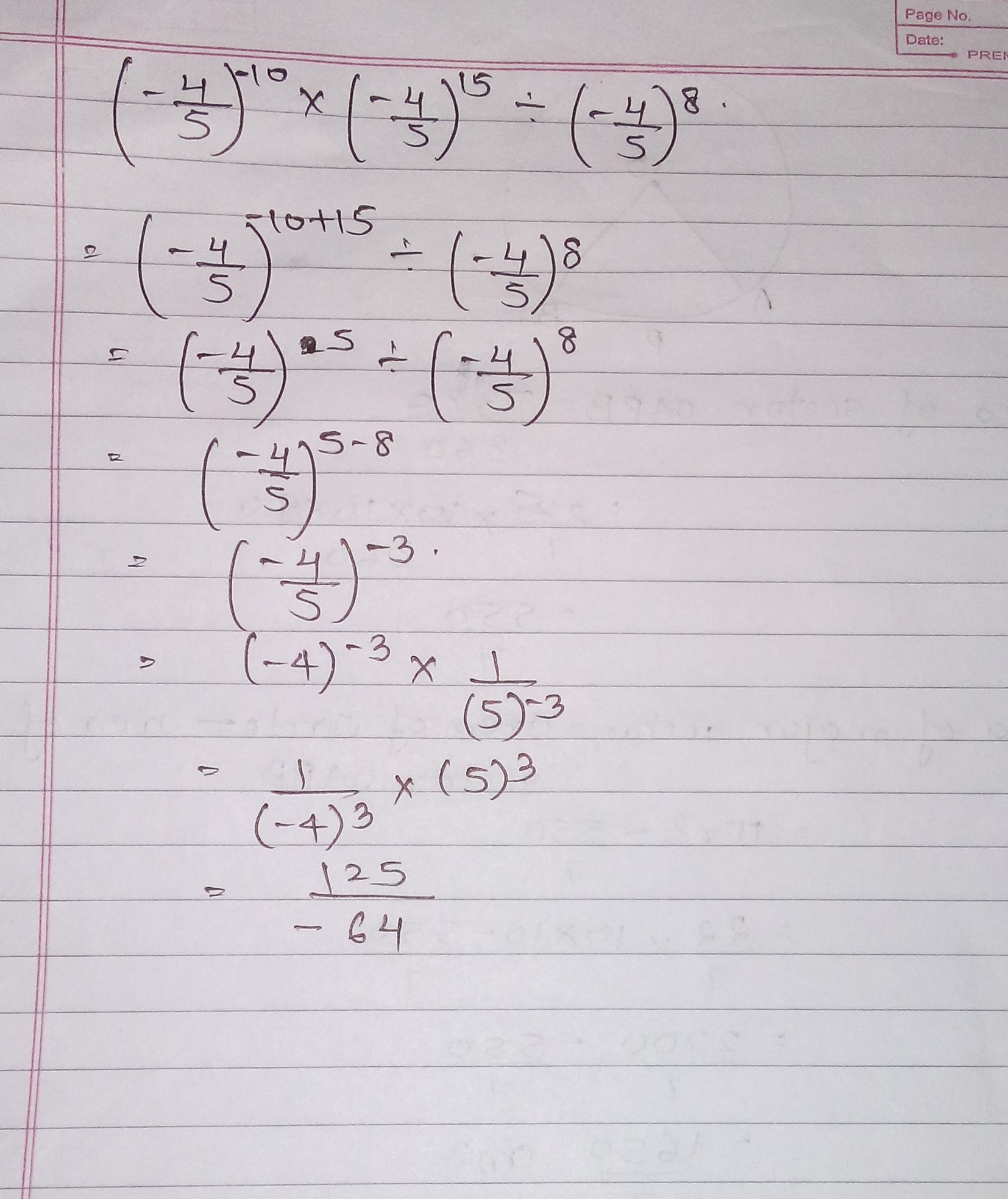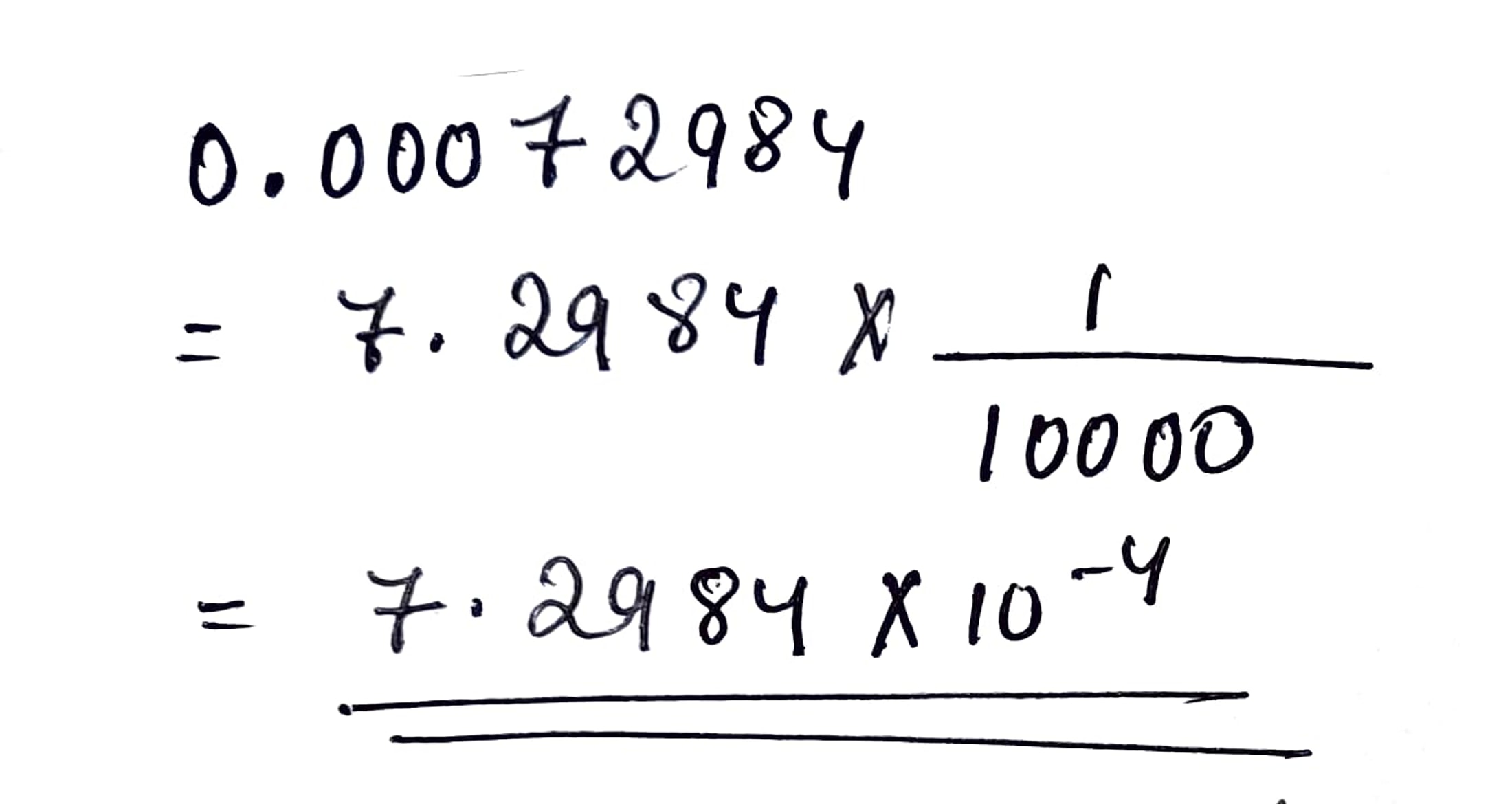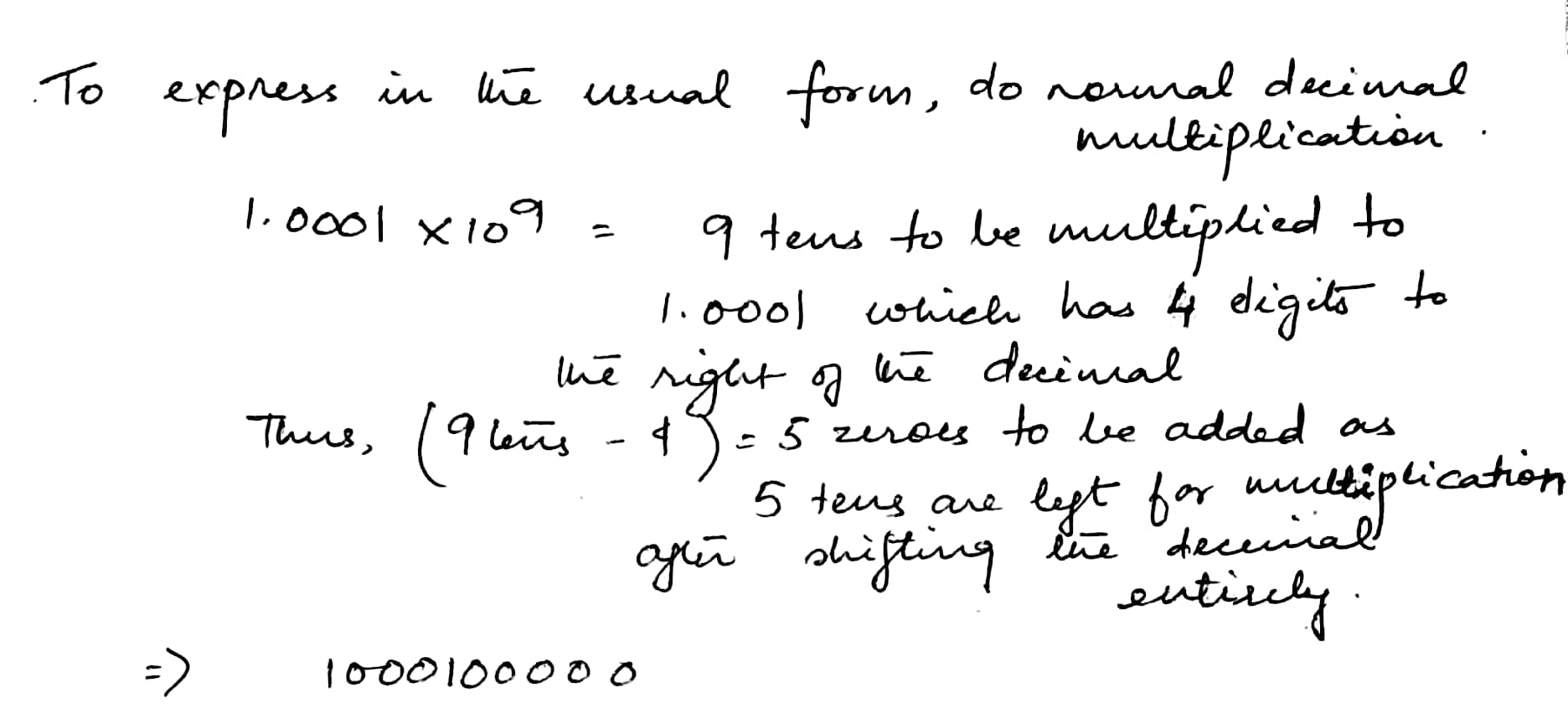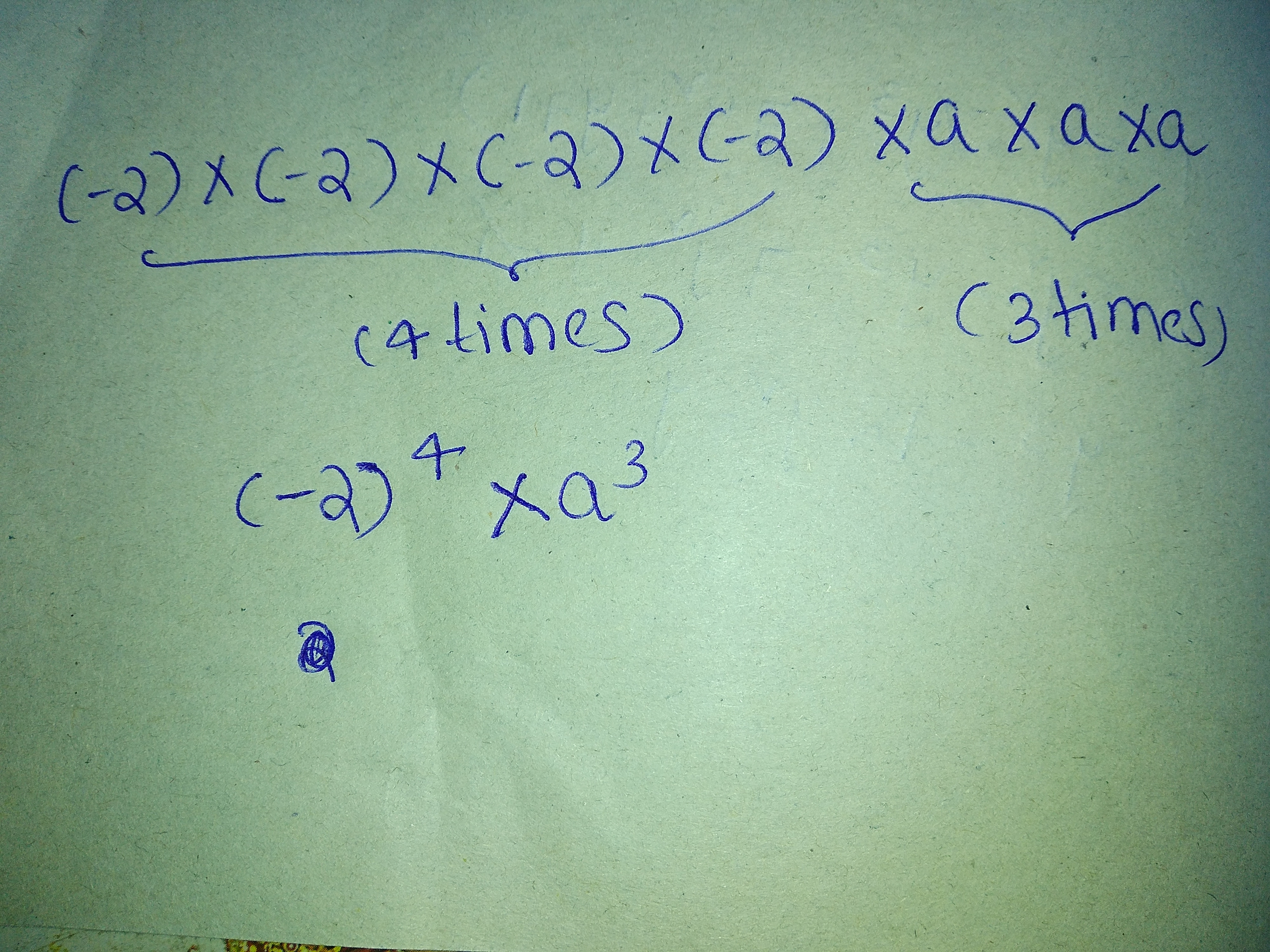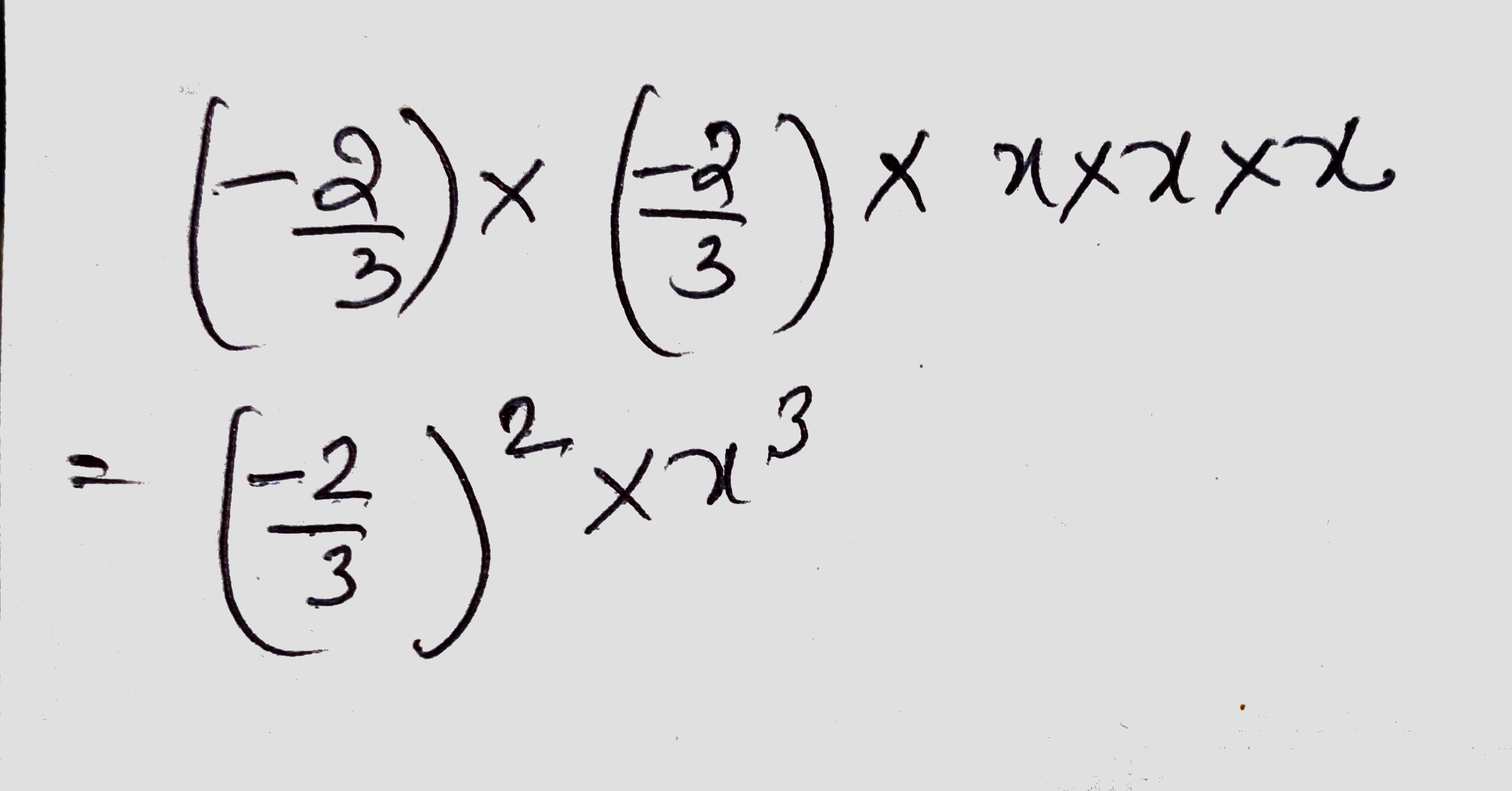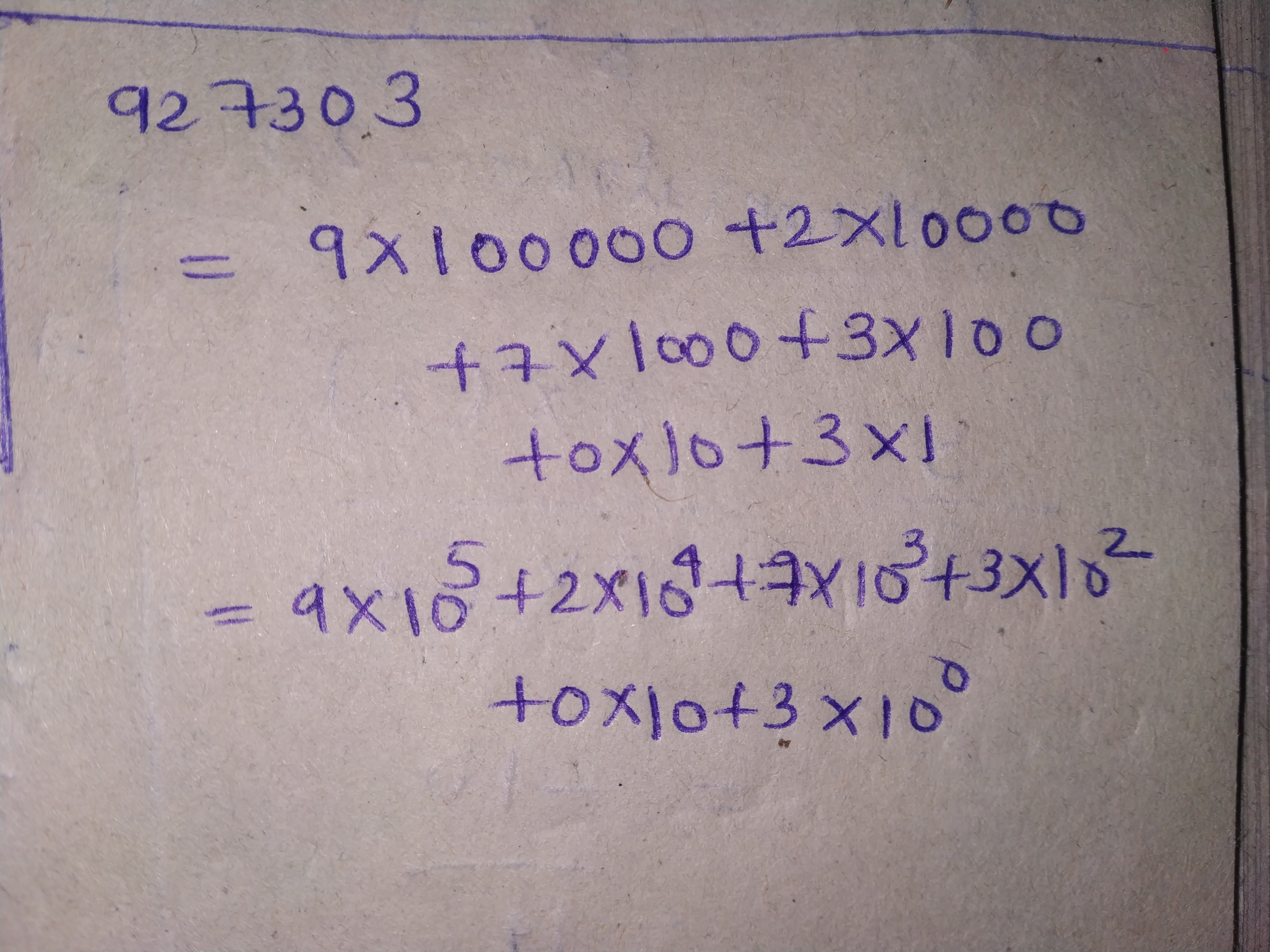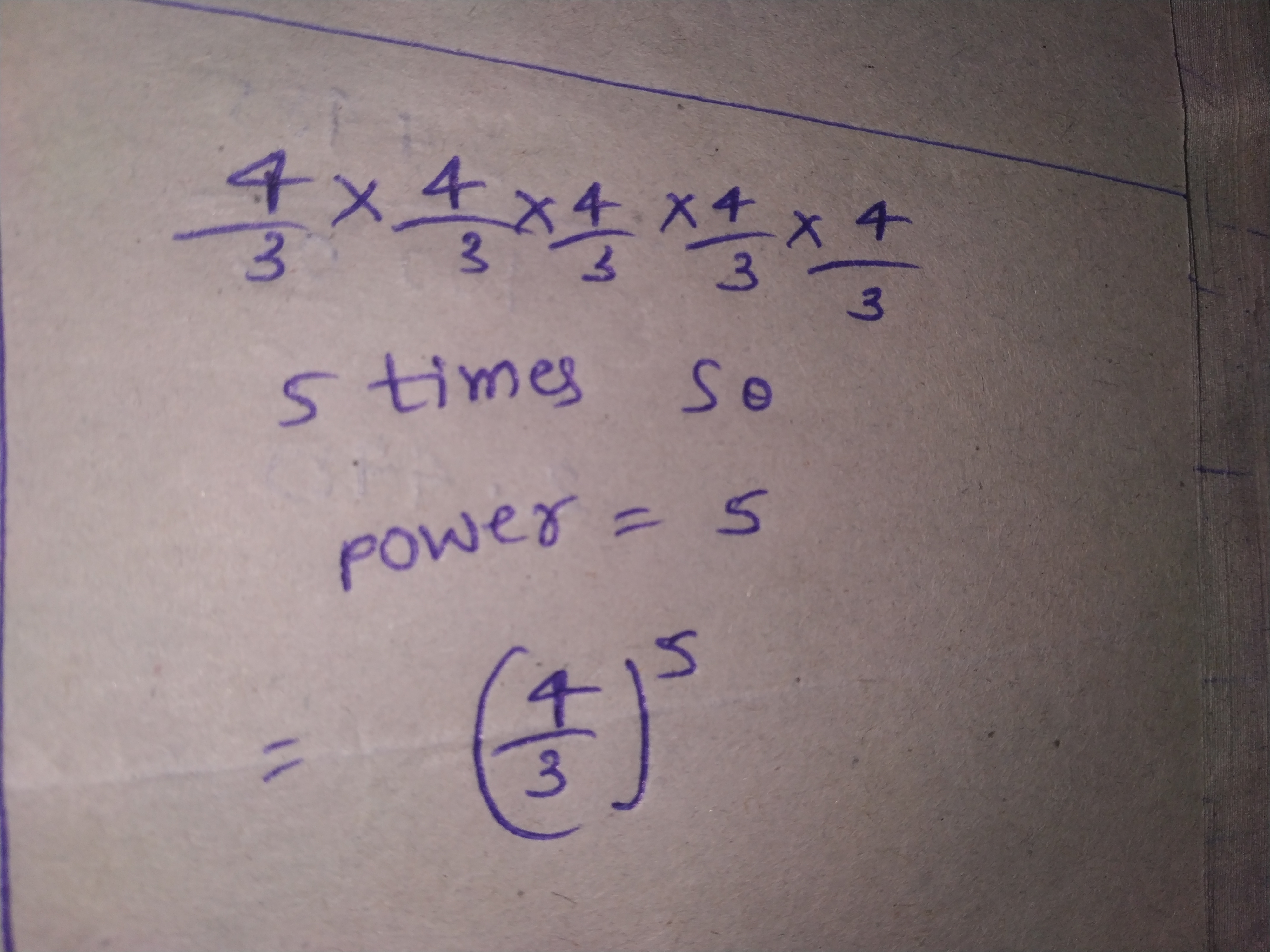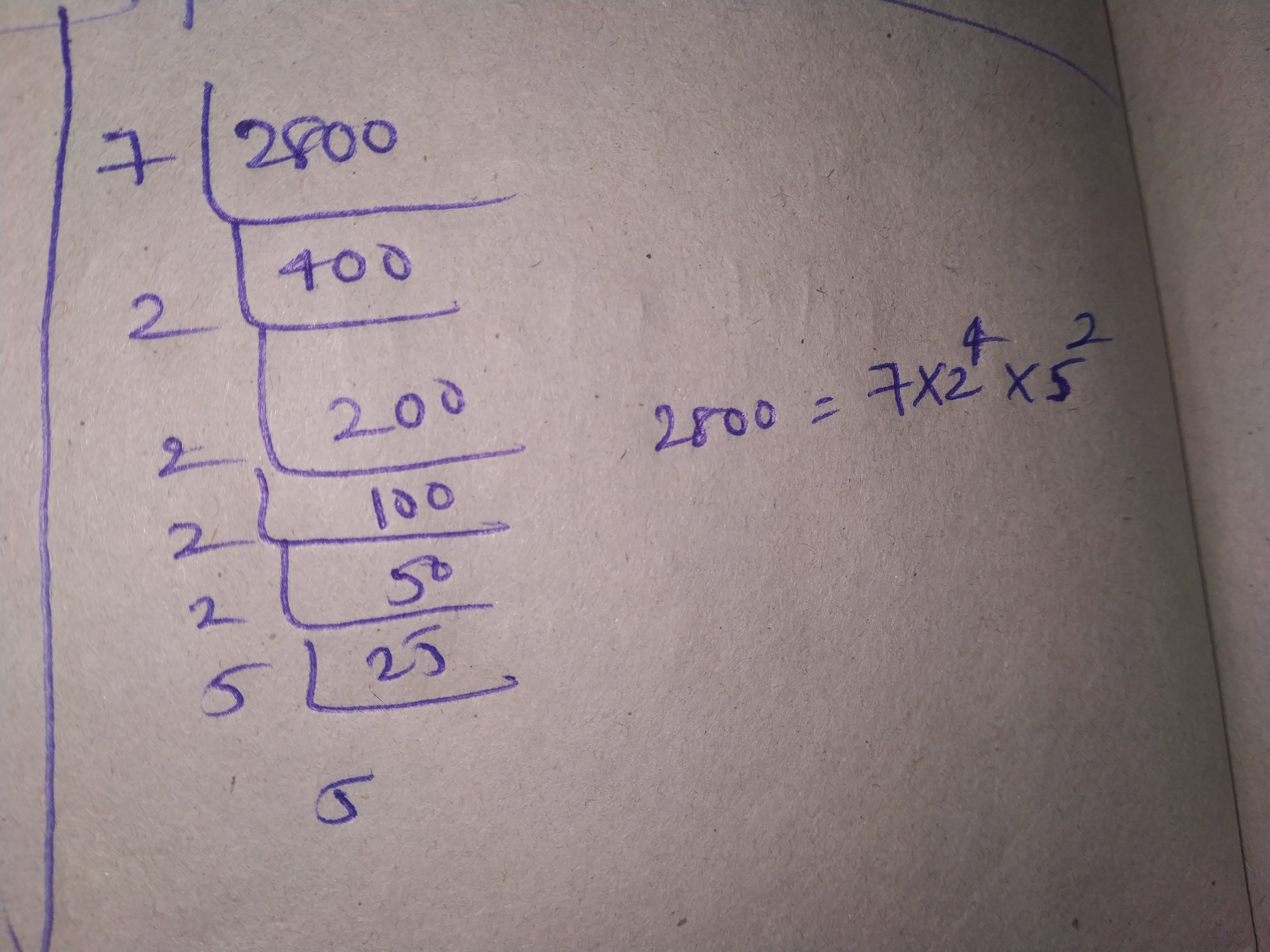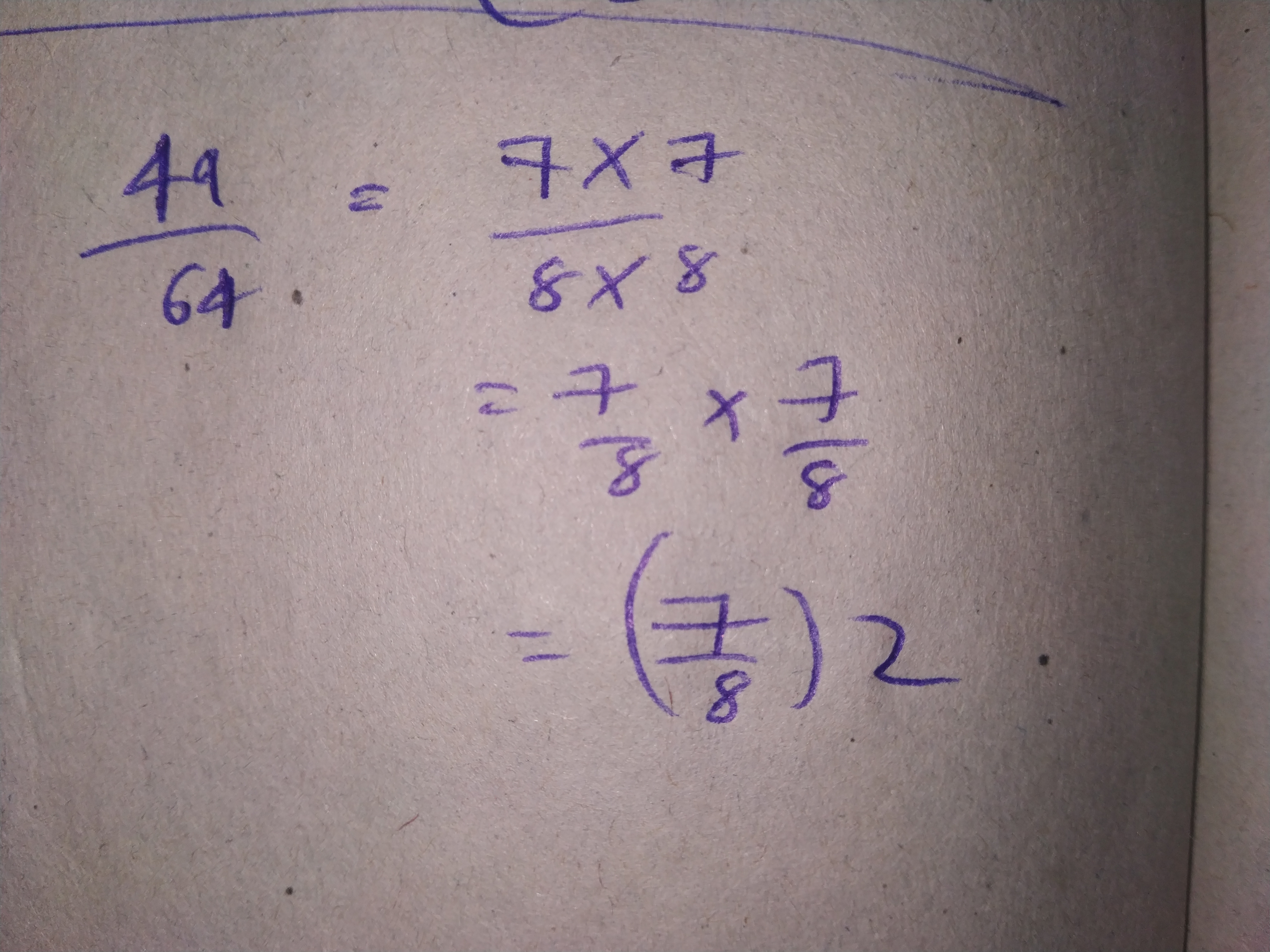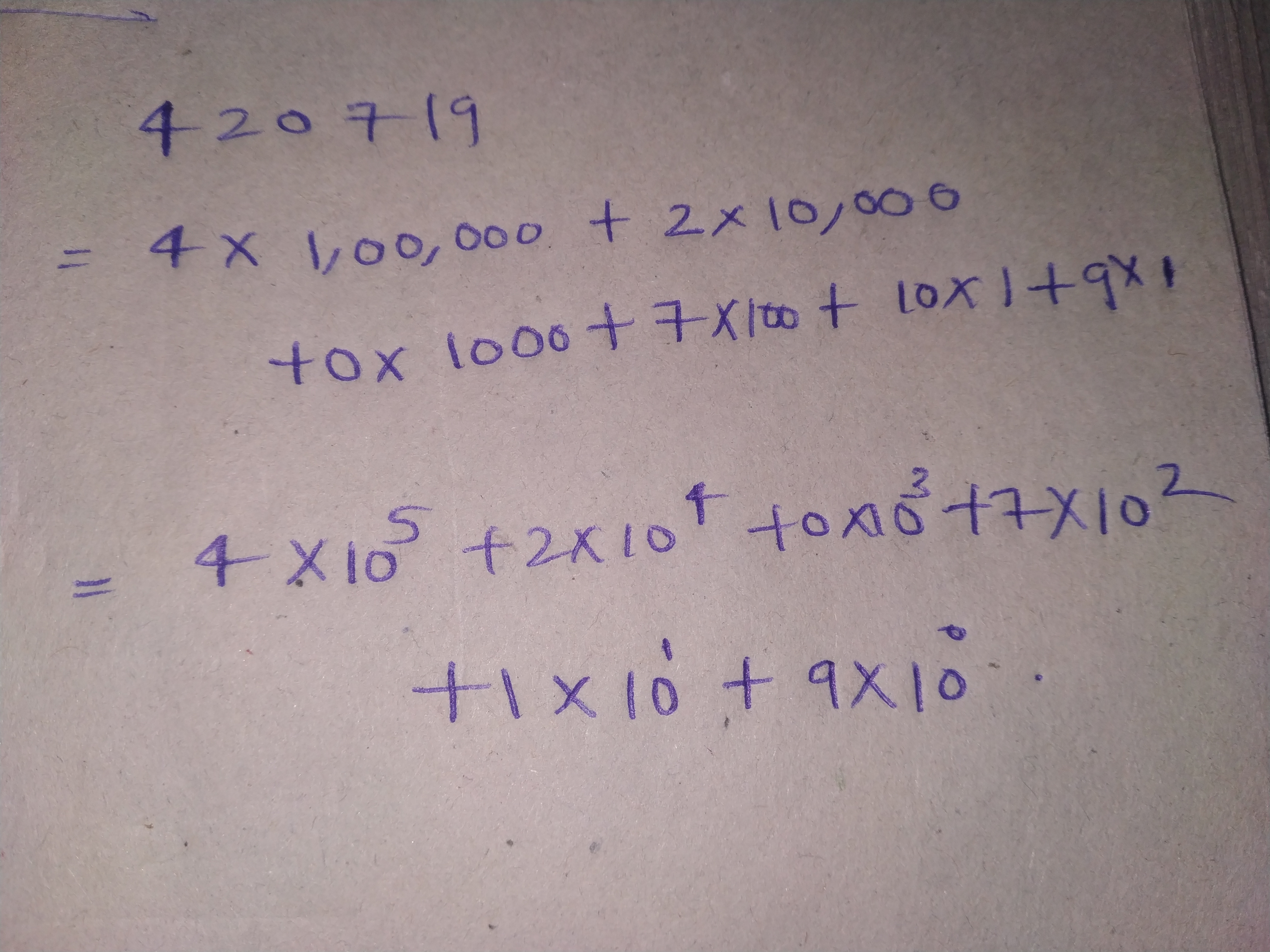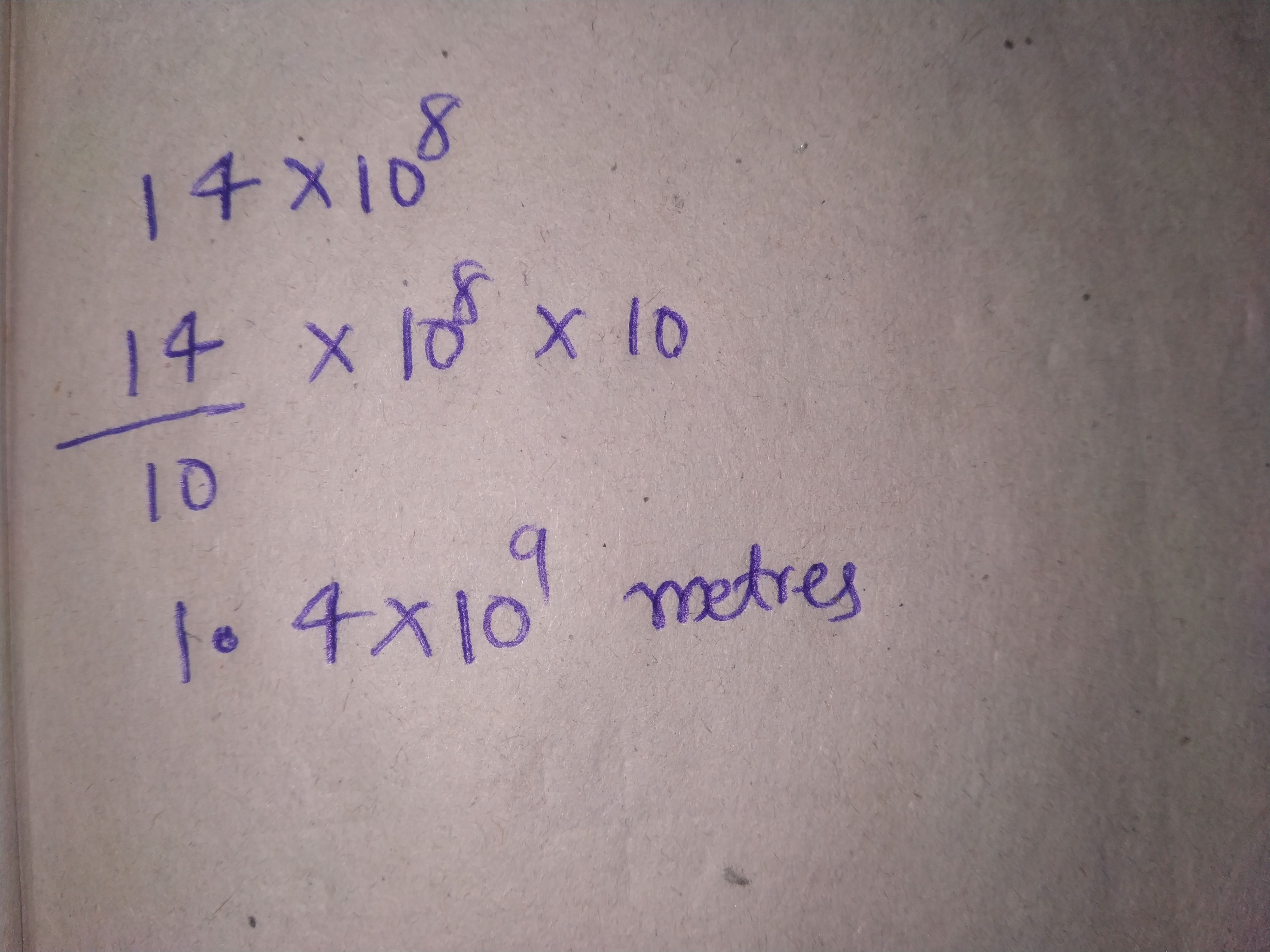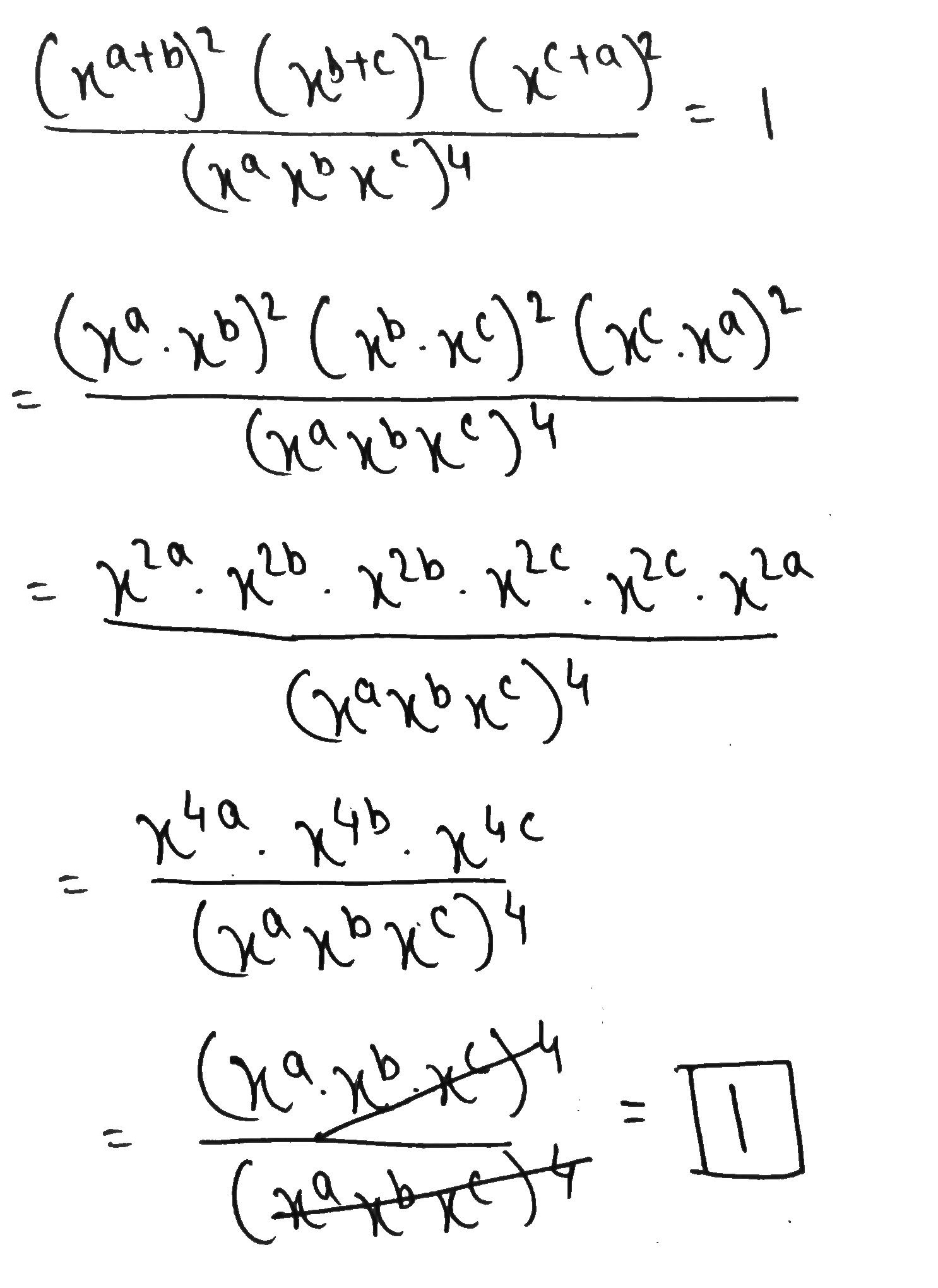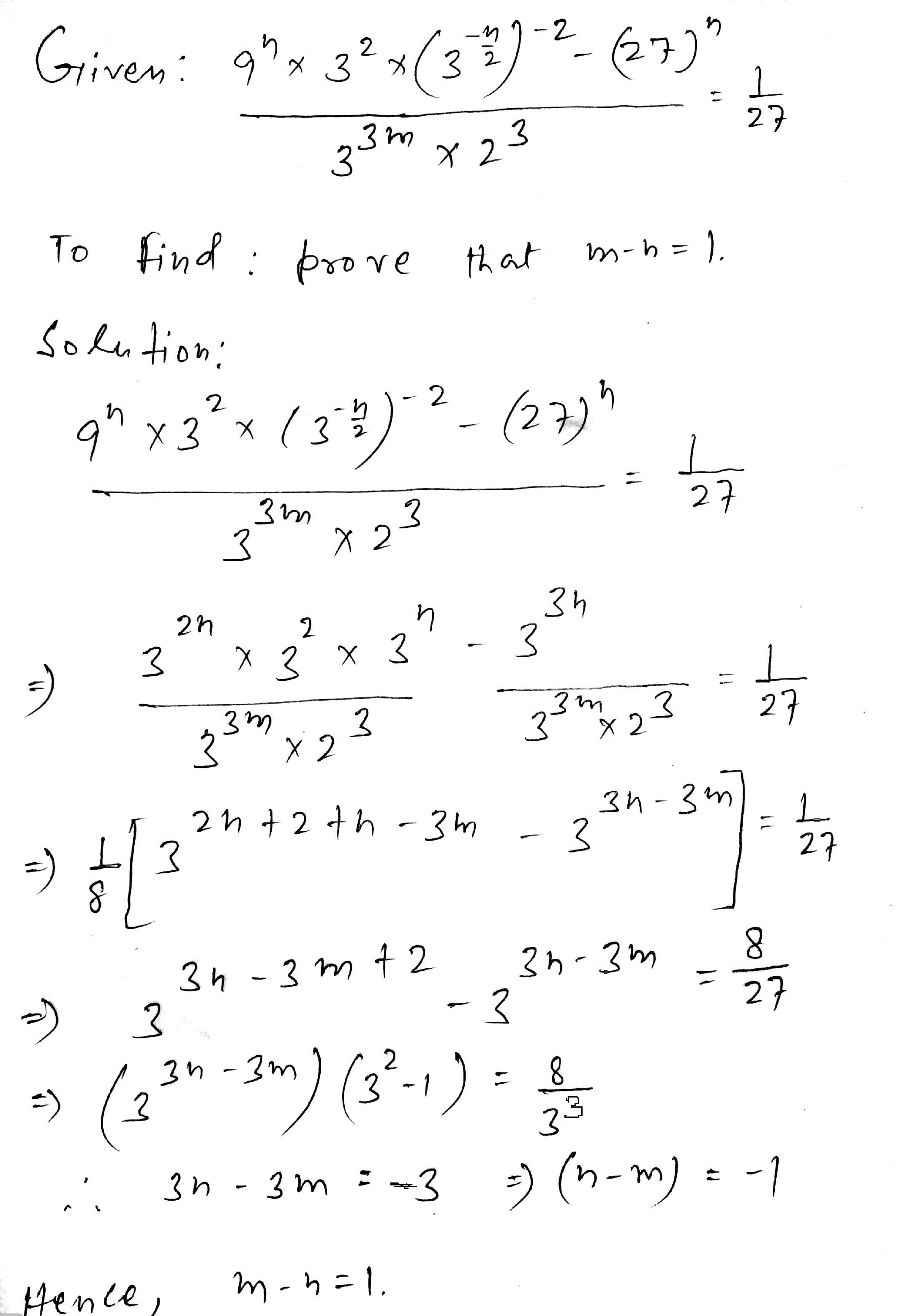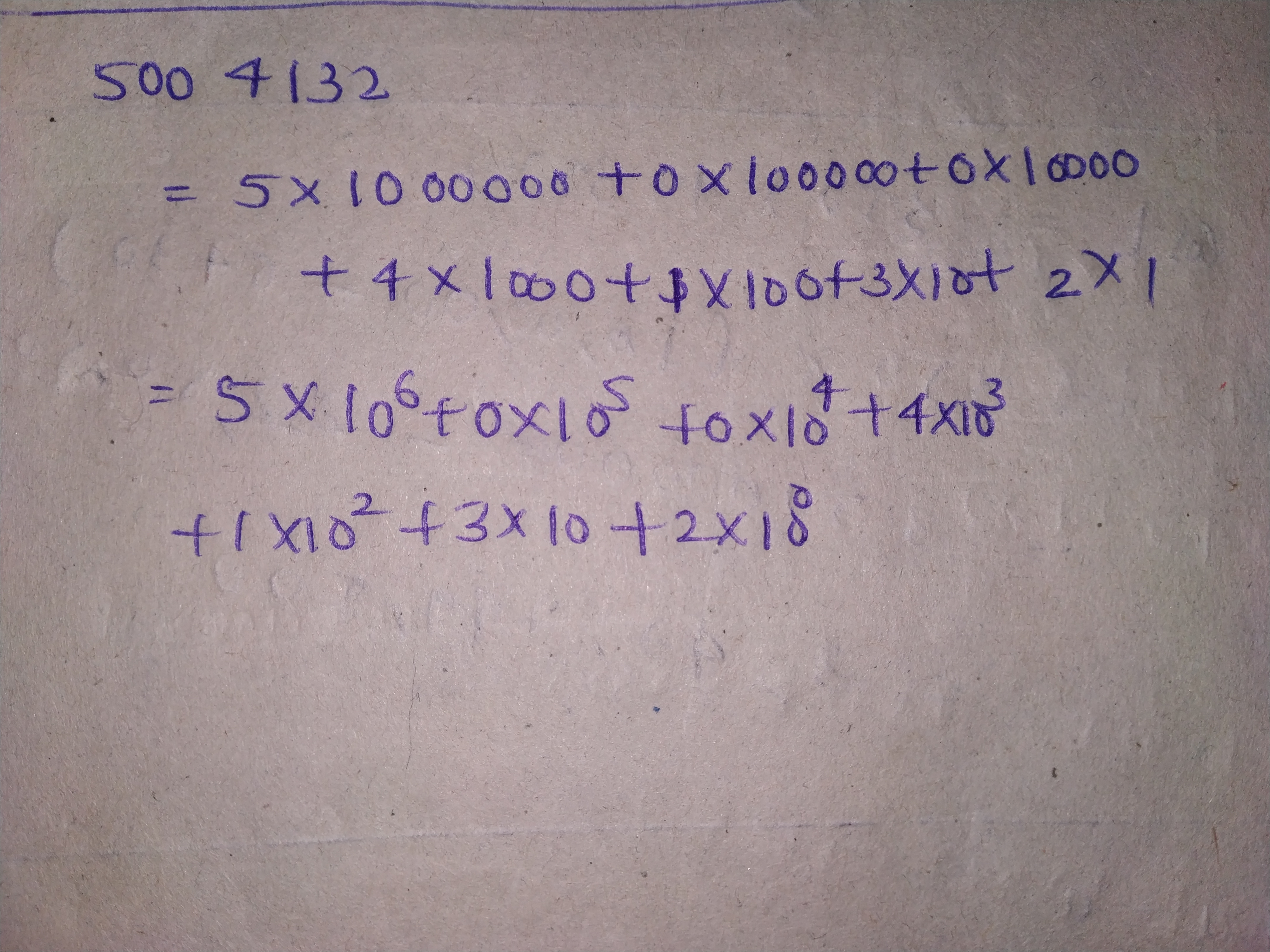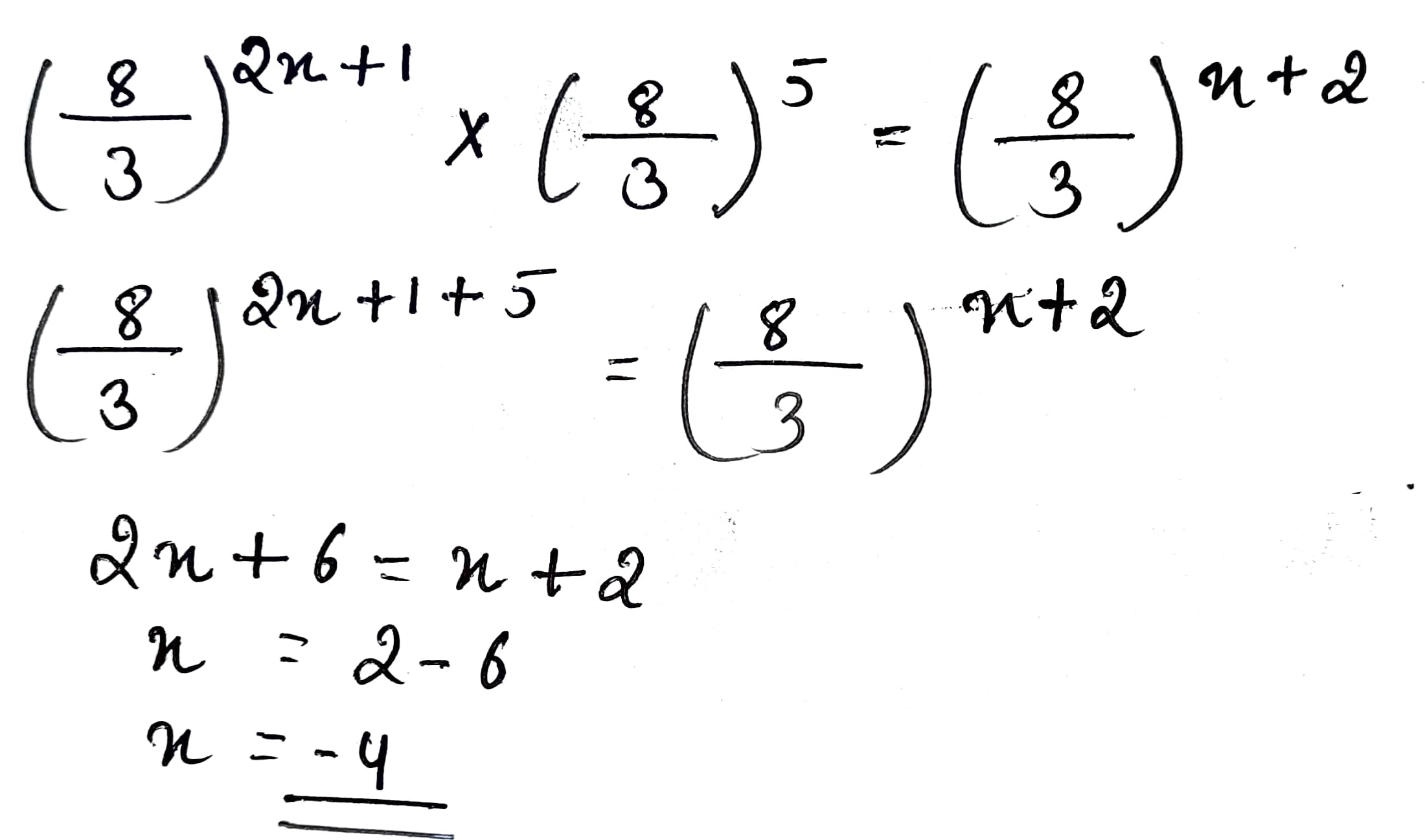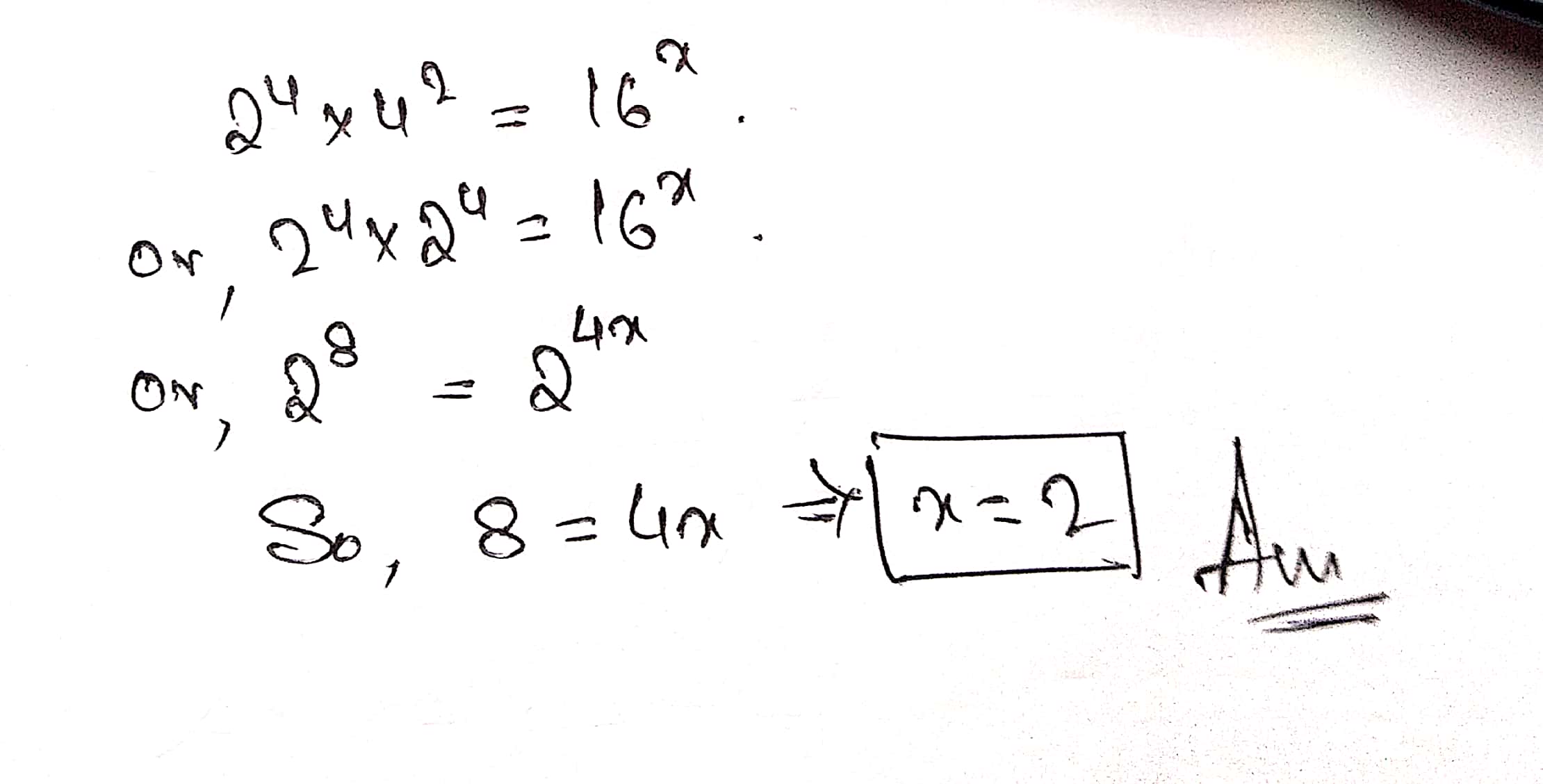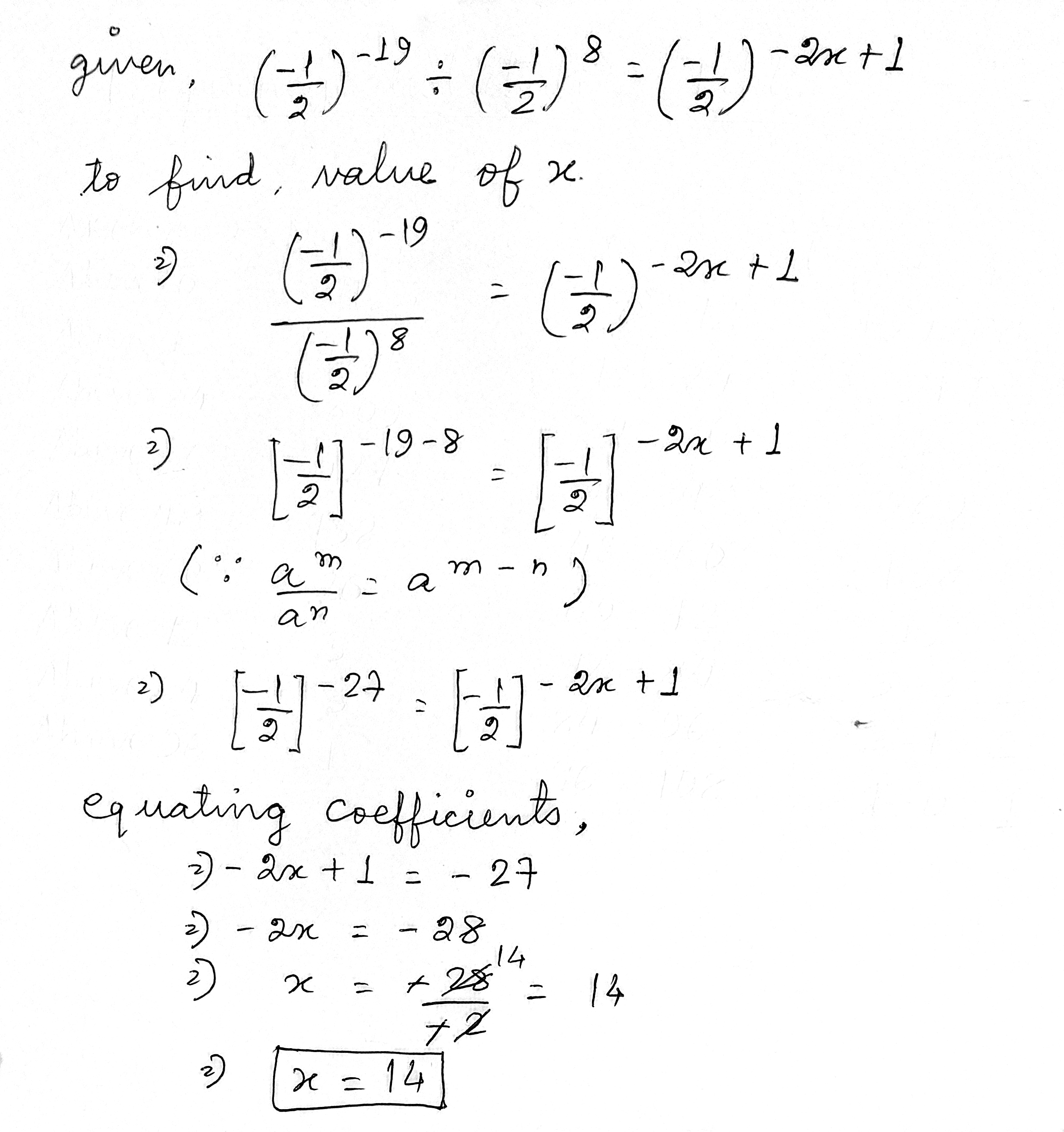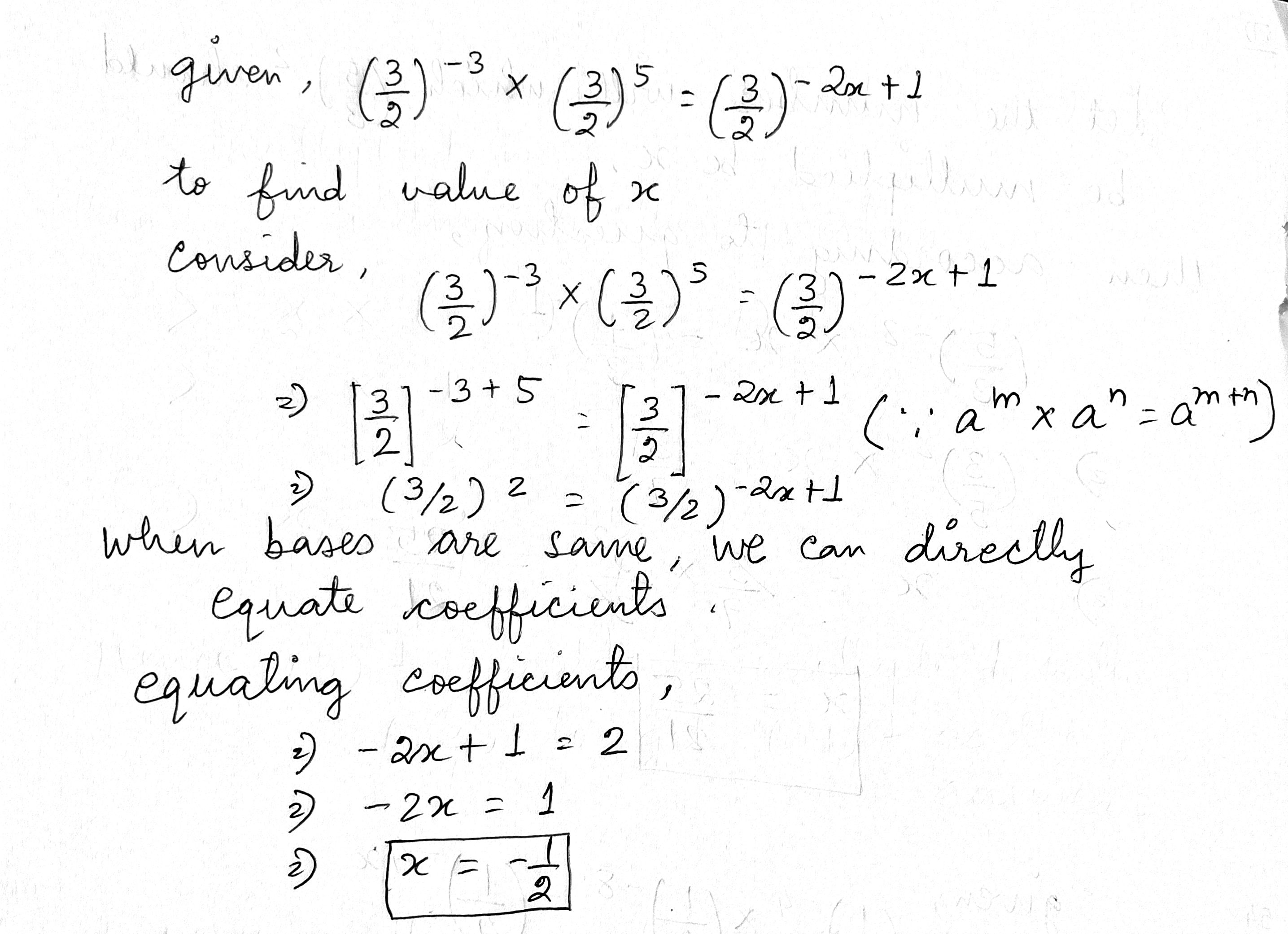Exponents And Powers - Class 7 Maths - Extra Questions
Solve: (712)8(146)8.
Solve: 0.00000000657×1015
Solve: (5−45−6)×53
Evaluate using product rule properties: 27×3=
Express the following as a rational number:
(5/7)−1×(7/4)−1
Express the following as a rational number:
(1/4)−4
Express the following as a rational number:
(−3)−1×(1/3)−1
Find the value of the following:
(23)4
Fill in the blanks to make the statements true.
The square of 500 will have ______ zeroes.
Simplify:
(32)−1÷(−25)−1
Simplify:
(−35)−3
Fill in the blanks:
(513)10÷[(513)3]2=(513)......
Fill in the blanks:
In the expression (−5)9, exponent = ........ and base = ........
Simplify and write the exponential form with negative exponent:
(−7)3×(1/−7)−9÷(−7)10
Express the number appearing in the following statement in standard form:
The diameter of a wire on a computer chip is 0.000003 m.
Express the number appearing in the following statement in standard form:
The thickness of a piece of paper is 0.0016 cm.
Express the number appearing in the following statement in standard form:
Mass of molecule of hydrogen gas is about 0.00000000000000000000334 tons.
Express the number appearing in the following statement in standard form:
The distance form the Earth of the Sun is 149,600,000,000 m.
Express the number appearing in the following statement in standard form:
The human body has 1 trillion cells which vary in shapes and size.
Express 1024 as a power of 2.
Express
343 as a power of 7.
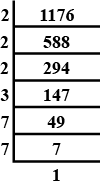
Express each of the following in exponential form:
1176
Express each of the following in exponential form:
1250
Which is greater:
54 or 43
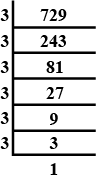
Express
729 as a power of 3
Evaluate
23÷28
Fill in the blanks
If index =3x and base =2y,the number = ………
Evaluate
(26)0
Evaluate
(35×47×58)0
Evaluate
28÷23
Fill in the blanks
If index = 3x and base = 2y, the number =
Evaluate
(30)6
Simplify, giving answers with positive index:
(a10)10(16)10
Evaluate:
(54)2
Express 10,100,1000,10000,100000 is exponential form.
Evaluate:
(114)2
Express each of the following as a product of prime factors only in exponential form:
729×64
Express the following as a rational number:
(1/3)−1
Simplify the product 3√2×4√2×12√32.
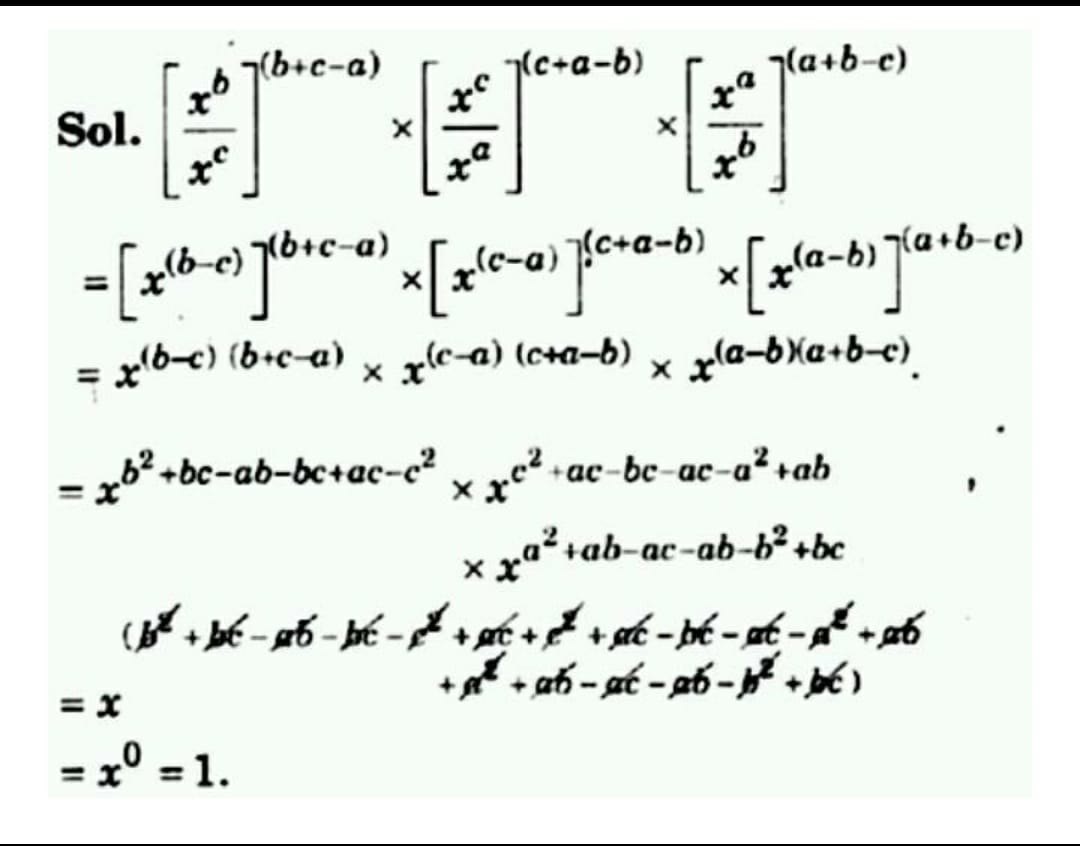
Prove that xa(b−c)xb(a−c)÷(xbxa)c=1.
Simplify (134)12.
Simplify (313)4.
Simplify (25)32×(243)35(16)54×(8)43
Simplify and express the following as a rational number:
(−7/8)−3×(−7/8)2
Express the following as a rational number:
(−2)−3
Express the following as a rational number:
5−3
Simplify and express the following as a rational number:
(4/9)6×(4/9)−4
Express the following as a rational number:
(4)−1
Express the following as a rational number:
(−3/4)−3
Solve for x when 22x+1+29=210
If 27x=93x, find x.
Express each of the following as a product of prime factors only in exponential form:
270
Express the following numbers in standard from:
846×107
Express the following as a rational number:
(−8/5)3
Simplify (34)14.
Express the following as a rational number:
(1/6)3
Express the following as a rational number:
(−13/11)2
Write the following numbers in the usual form:
4.5×104
Express the following in a rational number:
(−1/2)5
Evaluate [(16)12]12.
Finf the value of m: (−23)4×(−23)3×(−23)=(−23)m
Simplify the following using law of exponents.
[(−56)2]5
Express the following as a rational number:
(2/3)5
Simplify the following using law of exponents.
(102)3
Simplify 1123×1113
Simplify
1315×1715
Prove that xa(b−c)xb(a−c)÷(xbcxac)=1
If (−4)8÷(−4)4 is (−4)m, then find the value of m.
Express the number 4.5×103 in usual form:
Evaluate : [(−43)2]3
Which is larger (2.5)6 or (1.25)12?
Write the following numbers without scientific notation:
(A) 1.234×105
(B) 5.45×10−3
Simplify (1024)3×(81)4(243)2×(128)4
Suppose b is a positive integer such that 24b2 is also an integer. What are the possible values of b?
Express the number 1728 in the exponential form:
Simplify: 23×32×5433×52×24
Simplify:
(15)−3×(−12)45−6×362
Simplify:
68×53103×34
simplify in exponent form:36a4b6c36√108
Express the following as a rational number:
(−2/3)−1
Which is larger: (34×23) or (25×32)?
Simplify:
(25)6×(33)2(26)5×(32)3
What is the least positive integer n such that (30)3(35)2n is also integer?
Simplify: 21/241/6
Find the value of (811/2×82/3×322/5)1/2
Rakesh solved some problems of exponents in the following way. Do you agree with the solutions? If not why? Justify your argument.
x3x2=x4
Rakesh solved some problems of exponents in the following way. Do you agree with the solutions? If not why? Justify your argument.
3x−1=13x
Find the value of (1000π)2/3×(512π)4/3
Simplify and obtain a numerical value (32)−45.
Prove that :
(i) 3x+13x(x+1)×(3x3)x+1=1
(ii) (xmxm)m+n⋅(xnxl)n+l⋅(xlxm)l+m=1
The value of (0.001)13 is
Find the value of (−10m2n2)×(−20 mn15) for m=1.2 and n=−1.
If 102x=25 then find 10−x.
If ax−1=bc,by−1=ac,cz−1=ab, then find the value of xy+yz+zx−xyz.
Write each of the following in scientific notation:
(i) 92.43
(ii) 0.9243
(iii) 9243
(iv) 924300
(v) 0.009243
(vi) 0.09243
Find the value of the following:
[(23)2]2
Write the value of 0.2642E05+0.3781E05.
Simplify :
83a×25×22a4×211a×2−2a
Simplify:(c−2)2/3×c3/2÷c−3 is equal to cpq . If p snd q are co prime fiind the value of p+q.
Find the radix of the scale in which the numbers denoted by 479,698,907 are in arithmetical progression.
Simplify:(3r2)×(9r2)3/2÷(27r−3)1/3 and find the power of r
Express the following in exponential form
(i) log2128=7
(ii) log100.0001=−4
(iii) log816=43
[(−3)2]5 = ..............
Solve for x:3x5+3x−1010=84.
Solve: 4x−9.2x+23=0.
Express in exponential notation:A) (−72)11×(−72)5
B) (1317)4÷(1317)7
If ax=b,by=c,cz=a then show that xyz=1.
Simply with the help of exponential notation :
(i) (22×33)2
(ii) (1516)3÷(98)2
Find the value of 5√324.
Prove that: (ab)m(ab)n=(ab)m−n
Simplify:
[40+42−25]×3−2
Simplify:
[(2)−1+(4)−1+(3)−1]−1
Find the value of 4−3 in fractional form:
Simplify:
[(4)−1−(5)−1]2×(58)−1
Find n
(23)3×(23)5=(23)n−2
Evaluate :√14+(0.01)−12−(27)23
Simplify:
[(5)2−(14)−2]×(34)−2
If x=(100)a,y=(10000)b and z=(10)c, express log10√yx2z3 in terms of a,b,c
Find the value of (6561)0.25
(23)4=(22)x, find x.
Express each of the following numbers using expotential notation: i) 512ii) 343iii) 729iv) 3125
Find the highest power of p when 81p4−1 is divided by 3p−1.
Simplify: 8583
Solve: {\left( {25} \right)^2}
Express the following in exponential form
(i)-1331
(ii)\dfrac{-64}{125}
(iii)\dfrac{-345}{1024}
(ii)\dfrac{-64}{125}
(iii)\dfrac{-345}{1024}
Calculate:
\dfrac{{{4^3} \times {6^6} \times {2^5}}}{{{2^3} \times {4^2} \times {3^2}}}
Simplify \sqrt[4]{(81)^{-2}}.
Simplify:
{2^{\cfrac{2}{3}}}{.2^{\cfrac{1}{5}}}
Find the value of x for the given equation {\left( {\dfrac{3}{4}} \right)^3}{\left( {\dfrac{4}{3}} \right)^{ - 7}} = \left( {\dfrac{3}{4}} \right)2x
If a=2, b=3 find the value of (ab)^a.
81 in exponential form as
a) base 9
b) base 3
Find the value of n when :
i) 5^{2n} \times 5^3 = 5^9
ii) 8 \times 2^{n + 2} = 32
Solve
\dfrac{4^5 a^8b^3}{ 4^5 a^5b^2}
Simplify:{\left( { - 3} \right)^4} \times {\left( {\dfrac{5}{3}} \right)^4}
Find the value of 4^3
Solve:\dfrac{{{3^0} \times {5^2}}}{{{3^4} \times {5^3} \times {2^4}}}
Find the value of : {\left( { - \dfrac{1}{{27}}} \right)^{ - \dfrac{2}{3}}}
Find the value of \left(\frac{9}{8}\right)^{-3}\times \left(\frac{8}{9}\right)^{-2}
a = \dfrac{{{2^{x - 1}}}}{{{2^{-x - 2}}}} and b = \dfrac{{{2^{ - x}}}}{{{2^{x + 1}}}}, find the value of x.
Find the value of x , if
{(\dfrac{5}{7})^6}{(\dfrac{7}{5})^{ - 9}} = {(\dfrac{5}{7})^{3x}}
Simplify :
(-5)^4 + (2)^3
Show that
\dfrac{{{{\left( {{3^a}} \right)}^2} \times {{\left( {{2^{a - 2}}} \right)}^2}}}{{{9^{a - 1}} \times {4^{a + 2}}}} = \dfrac{9}{{256}}
Solve 84{ a }^{ 2 }{ b }^{ 5 }\div 12{ a }^{ 2 }{ b }^{ 2 }
What is {10^{ - 10}} equals to
Find Y-
27^y = \dfrac{9}{{{3^y}}}.
Express in the form of a^{m}b^{m}c^{m} as appropriate: 343\times 216
Simplify 11^{8} \div 11^{2}
If {\left( {\frac{5}{9}} \right)^{ - 4}} \times {\left( {\frac{7}{5}} \right)^{ - 4}} = \left( {\frac{p}{q}} \right), find {\left( {\frac{p}{q}} \right)^{ - 2}}
Find value of 12x^{3}+x^{2}+x if x=7
If a= b^{2x}, b= c^{2y} & c= a^{2z} , prove that xyz= \frac{1}{8}
Find x, if {\left( {{3^6}} \right)^4} = {3^{12x}}
Write the base and exponent of the following :
\left( \dfrac {-4}{7} \right)^6
Write the base and exponent of the following :
\dfrac{-2}{3}
Express the following in exponential form :
\left( \dfrac {9}{2} \right)^4
Express the following in exponential form :
\left( \dfrac {-7}{8} \right)^3
Write the base and exponent of the following :
\left( \dfrac {2}{9} \right)^5
Write the base and exponent of the following :
\left( \dfrac {15}{19} \right)^3
Express the following in exponential form.
\dfrac{9}{2}\times \dfrac{9}{2}\times \dfrac{9}{2}\times \dfrac{9}{2}\rightarrow\left(\dfrac{9}{2}\right)^4.
if 4^x - 4^{x-1} = 24 then find the value of (2x)^x .
Express the following in exponential form :
\left( \dfrac {5}{6} \right)^2
Solve : \dfrac{7525}{5^2} + 3125 \, \times \, 5^{-4} - 105 \, \times \, 2 = ?
Simplify:
16^{\frac{3}{2}}
Simplify:
32^{\frac{1}{5}}
Express the following in exponential form :
\left( \dfrac {11}{12} \right)^6
Express the following in exponential form :
\left( 1.8 \right)^7
Find the value of { \left[ { \left( 16 \right) }^{ \frac { 1 }{ 2 } } \right] }^{ \frac { 1 }{ 2 } }
Prove that : \left(\dfrac{2^a}{2^b} \right)^{(a +b)} . \left(\dfrac{2^b}{2^c} \right)^{(b + c)} . \left(\dfrac{2^c}{2^d} \right)^{(c + d)}.\left(\dfrac {2^d}{2^a}\right)^{(d+a)} = 1
Simplify:
625^{\frac{1}{4}}
Simplify:
243^{\frac{2}{5}}
Find the value of K if {5^6}\times{5^k} = {\left( 5 \right)^{3k}}
Evaluate 8^{9}\div8^{3}
Is {\left( {{a^m}} \right)^n} = {\left( {{a^n}} \right)^m} Explain.
\dfrac{\left ( -3^{2} \right )\times \left ( -6^{2} \right )\times 7^{2}}{\left ( 12 \right )^{1}\times \left ( 14 \right )^{2}}
Express \dfrac{1.5 \times 10^6}{2.5 \times 10^{-4}} in the standard form.
Simplify: 125^{\frac{2}{3}}.
Find the value of \sqrt{\left(81\right)^{-2}}.
Find 9x^3 + x^3 + x =? if x = 7.
Write the following numbers in the usual form:
4.83\times 10^7
Express each of the following numbers using exponential notations:
- 512
- 343
- 729
- 3125
- 512
- 343
- 729
- 3125
If 2^{n}=1024, then n=?
Solve:
\dfrac{{2}^{8}\times {a}^{6}}{{4}^{3}\times {a}^{3}}
If \frac{(2^{-a})^{-2}8^{a}+32^{a}}{2^{3b}4}=2^{-4}, then 3b - 5 a =
If 27^{n}=81, find \dfrac {27}{4}\times n\dfrac {3n}{4}
Simplify :- 62.5×(10)−122.5×(10)862.5×(10)−122.5×(10)8
Solve:3a^2 \times 8a^4
Simplify \dfrac{7^{-5}\times 10^{-5}\times 75}{5^{-7}\times (14)^{-5}}
Solve : [1-\left\{1-(1-n)^{-1}\right\}]^{-1}
Solve
\dfrac{6^{7}}{2^{3}\times 3^{7}}
If b = \sqrt [ n ] { a ^ { m } }, then will a = \sqrt [ m ] { b ^ { n } }.
How to calculate the value of 10^{2}?
a^ {x}=b^ {y}=c^ {x} and b^ {2}=ac prove that y=\dfrac {2xz}{x+z}
Write the base and exponent of
(2xy)^{3}
Find the value of :-
\cfrac{{{{\left( {6.4} \right)}^2} - {{\left( {5.4} \right)}^2}}}{{{{\left( {8.9} \right)}^2} + \left( {8.9} \right) \times \left( {2.2} \right) + {{\left( {1.1} \right)}^2}}}
Write the base and exponent of
(7y)^{2}
Simplify and express the result in power notation with a positive exponent.
\left( \dfrac { 1 } { 2 ^ { 3 } } \right) ^ { 2 }
Express in exponential form:0.000169
Find the value of 3 ^ { 2 } \times 3 ^ { 4 } \times 3 ^ { 3 } = ?
Evaluate
(-6)^8 \div (-6)^2
Find the value of x, if (5)^{x-3} \times (3)^{2x-8}=225
solve [(5^2)^3 \times 5^4] \div 5^7
The exponential form of \dfrac{-64}{125} is
Solve the following equation for x :4^{2x}=\dfrac{1}{32}
Solve
{ \left( \dfrac { 5 }{ 3 } \right) }^{ -4 }\times { \left( \dfrac { 5 }{ 3 } \right) }^{ -5 }
Using laws of exponents, simplify and write the answer in exponential form : \dfrac {{ 6 }^{ 15 }} {{ 6 }^{ 10 }}
Express 27^{-2} as a power with base 3.
Using laws of exponents, simplify and write the answer in exponential form: a^{ 4 }\times b^{ 4 }
4\times 10^{5}+5\times 10^{3}+3\times 10^{2}+2\times 10^{2}=
Solve:2^{7x}\div 2^{21}=\sqrt[5]{2^{15}}
Simplify and express following in exponential form :
\dfrac{4^{5}\times a^{3}b^{3}}{4^{5}\times a^{5}b^{2}}
What is the value of \dfrac{4^{8}-4^{9}}{4^{8}}?
Write the following in standard form 0.00000564.
Solve { (-2) }^{ x }=-128.
Let us solve :
4^{4x}.4^{3x-1}=\dfrac{4^{2x}}{2^{3x}}
Find the value of x for which 7^{10}\times 7^8 = \left ( \dfrac{1}{7} \right )^x
Find the product of 3 ^ { 1 / 2 } and \sqrt { 3 }.
Find the value of m if \left ( \dfrac{p}{q} \right )^{m}=\dfrac{q^{4}}{p^{4}}
Write exponential form for 8 \times 8 \times 8 \times 8 taking base as 2
Let us solve :
49^{x}=7^{3}
Write the base and the exponential :
3^{4}
Prove that:
4^{\dfrac {1}{3}}\times [2^{\dfrac {1}{3}}\times 3^{\dfrac {1}{2}}]\div 9^{\dfrac {1}{4}}.
Divide
8\sqrt { 15 } by 2\sqrt { 3 } .
Express each of the following as product of powers of their prime factors:
540
Write the value of (\frac{x^{a}}{x^{b}})^{a+b}\times (\frac{x^{b}}{x^{c}})^{b+c}\times (\frac{x^{c}}{x^{a}})^{c+a}
Simplify
\dfrac { (2^{ 2 })^{ 2 }\times 7^{ 3 } }{ { 8 }^{ 3 }\times 7 }
Compare the following numbers:
4\times{10}^{14};3\times{10}^{17}
Find value of { \left( -\dfrac { 1 }{ 27 } \right) }^{ -\tfrac { 2 }{ 3 } }.
Simplify and express the following in exponential form:
{2}^{0}\times{3}^{0}\times{4}^{0}
Express each of the following as product of powers of their prime factors:
3,600
Solve \frac { 6 ^ { 4 } \times 36 \times 216 } { 6 ^ { 7 } }
Simplify:{8}^{\tfrac{2}{3}}
Write 2016 in standard form.
Write the following numbers in the usual form:
302\times 10^{6}
Express the following as a rational number:
\left(\dfrac{-4}{7}\right)^3
Express the following as a rational number:
(-6)^{-1}
Simplify the following and express as a rational number:
(3/2)^4\times (1/5)^2
Express the following as a rational number:
(-1)^9
Simplify the following and express as a rational number:
(2/3)^2\times (-3/5)^3\times (7/2)^2
Simplify the following and express as a rational number:
\left\{ (-3/4)^3- (-5/2)^3\right\} \times 4^2
Simplify the following and express as a rational number:
(-1/2)^5\times 2^3 \times (3/4)^2
Simplify the following and express as a rational number:
(-2/3)^5\times (-3/7)^3
Fill in the blanks to make the statement true:
(213 \times 657)^{-1} = 213^{-1} \times ...............
Simplify and express the following as a rational number:
(\dfrac{4}{3})^{-3} \times (\dfrac{4}{3})^{-2}
Write the prime factorization of the following number in exponential form:
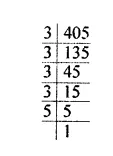
405
Write the prime factorization of the following number in exponential form:
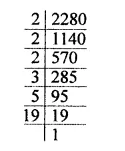
2280
Write the prime factorization of the following number in exponential form:
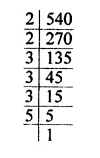
540
Write the prime factorization of the following number in exponential form:
360
Write the prime factorization of the following number in exponential form:
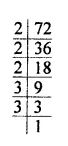
72
Simplify : (256)^{-\left (4^{-\frac {3}{2}}\right )}
Write the prime factorization of the following number in exponential form:
3600
Fill in the blanks:
(-3)^8 \div (-3)^5 = (-3)^{.....}
Express the number appearing in the following statement in scientific notation (or standard form):
In a galaxy there are 100000000000 stars on an average.
In a galaxy there are 100000000000 stars on an average.
Write the prime factorization of the following number in exponential form:
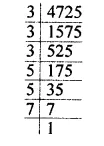
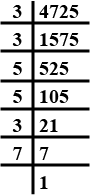
4725
Write 1.205 \times 10^5 in the usual decimal notation.
Write the prime factorization of the following number in exponential form:
8400
Fill in the blanks:
In 5^2 = 25, base = .......... and index = ...........
Evaluate:
(-1)^{4}
Evaluate:
2^{1}
Evaluate:
0^{3}.
Find the sum of 8 \times {10}^{13} and 12.3 \times {10}^{12} and express the answer in scientific notation.
Fill in the blank with "equal to " or "greater than" or "less than"39 \times {10}^4 is _______ 3.9 \times {10}^5
Which of the following numbers is least?
3.47 \times {10}^4 , 34.7 \times {10}^4 and 347 \times {10}^4
Choose the correct answers for number in exponential form 9^8
Fill in the blank:
The value of exponential 5^4 is _____.
Write the exponential form when base is 11 and exponent is 7.
Fill in the blank:
The base in the exponential 6^2 is ____.
Exponent of a^{5}\, \times\, a^0\, \times\, a^5 is equal to
Simplify
\cfrac { { 15 }^{ 4 }\times { 14 }^{ 2 } }{ { 21 }^{ 3 }\times { 10 }^{ 3 } }
Express the following numbers using scientific notation:
(A) 1,234.56
(B) 0.0876
Simplify: { \left( \cfrac { 2 }{ 5 } \right) }^{ -3 }\times { \left( \cfrac { 25 }{ 4 } \right) }^{ -2 }
Simplify: \cfrac { { \left( { 5 }^{ -3 } \right) }^{ 2 }\times { \left( { 3 }^{ 4 } \right) }^{ } }{ { \left( { 3 }^{ -2 } \right) }^{ -3 }\times { \left( { 5 }^{ 3 } \right) }^{ -2 } }
Simplify:
\cfrac { { \left( 0.22 \right) }^{ 4 }\times { \left( 0.222 \right) }^{ 3 } }{ { \left( 0.2 \right) }^{ 5 }\times { \left( 0.2222 \right) }^{ 2 } }
Find all positive integers m,n such that { \left( { 3 }^{ m } \right) }^{ n }={ 3 }^{ m }\times { 3 }^{ n }\quad
Can it happen that for some integer m\ne 0, { { \left( \cfrac { 4 }{ 25 } \right) }^{ m } }={ \left( \cfrac { 2 }{ 5 } \right) }^{ { m }^{ 2 } }\quad ?
Simplify:
\cfrac { { \left( 0.0006 \right) }^{ 9 } }{ { \left( 0.015 \right) }^{ -4 } }
If { \left( { 2 }^{ m } \right) }^{ 4 }={ 4 }^{ 6 }, find the value of m.
Simplify:
{ \left( 1.8 \right) }^{ 6 }\times { \left( 4.2 \right) }^{ -3 }
Which is larger: 0.25^4 or 0.35^3
Find the value of the following:
\left (\dfrac {-1}{2}\right )^{5}
Simplify: 10^{9} \div 10^{6}
Simplify: (2^{5})^{2}
Is \cfrac { { \left( { 10 }^{ 4 } \right) }^{ 3 } }{ { 5 }^{ 13 } } an integer? Justify your answer.
Simplify:
\left [\left \{(3^{5/2} \times 5^{3/4})\div 2^{-5/4}\right \} \div \left \{16\div (5^{2}\times 2^{1/4} \times 3^{1/2})\right \}\right ]^{1/5}
Simplify the following using laws of indices:
(6.25)^{0.5}\times 10^{2}\times (100)^{-1/2}
Find the value of the expression
\left [3^{1/3}\left \{5^{-1/2} \times 3^{-1/3} \times (225^{2})^{1/3} \right \}^{-1/2}\right ]^{6}
Write the given \sqrt [3]{108} in its simplest form
Write the given surd \sqrt [4]{5000} in its simplest form
Write the given surd \sqrt {76} in its simplest form
Express the following information in the standard form:
Size of the bacteria is 0.0000004 m
Simplify \left(\frac{3125}{243}\right) ^{-415}
Simplify: \root 4 \of {\root 3 \of {{2^2}} }
Simplify the following expression.
(8p^{-3})^{2/3} \times (4p^2)^{3/2} \div p^{-3} and find the power of p
(8p^{-3})^{2/3} \times (4p^2)^{3/2} \div p^{-3} and find the power of p
Simplify: (x^{0})^{4}
Simplify: (2\times 3)^{4}
Simplify: \left (\dfrac {3}{2}\right )^{5}
Simplify the following using law of exponents.
\dfrac{(-6)^9}{(-6)^5}
Simplify the following using law of exponents.
(3^2)^2
Solve the following equation:
\displaystyle\, \left ( \dfrac{5}{3} \right )^{x + 1}\cdot \left ( \dfrac{9}{25} \right )^{x^2 + 2x - 11} = \left ( \dfrac{5}{3} \right )^9
Represent the following mixed infinite decimal periodic fractions as common fractions (1 4)
\displaystyle\frac{\sqrt[3]{a^5 b^{1/2}}\sqrt[4]{a^{-1}}}{(a^2 \sqrt[5]{ab^3})^2}
Represent the following mixed infinite decimal periodic fractions as common fractions:
Simplify the following expressions.
\displaystyle\frac{(\sqrt[5]{a^{4/3}})^{3/2}}{(\sqrt[5]{a^4})^3} \cdot \frac{(\sqrt{a \sqrt[3]{a^2b}})^4}{(\sqrt[3]{a\sqrt{b}})^4}
If (7+4 \sqrt3)^n = p + \beta , where n and p are positive integers, and \beta a proper fraction, show that ( 1 - \beta ) ( p + \beta ) = 1
Simplify the following using law of exponents.
\dfrac{(4)^6}{(4)^3}
Solve the following equation:
\displaystyle\, 9^{|3x - 1|} = 3^{8x - 2}
Solve the following equations.
\displaystyle\, 3.4^x + \frac{1}{3} \cdot 9^{x + 2} = 6.4^{x + 1} - \frac{1}{2} \cdot 9^{x + 1}
4^x \, - \, 3^{x \, - \, \dfrac{1}{2}} \, = \, 3^{x \, + \, \dfrac{1}{2}} \, - \, 2^{2x \, - \, 1} find the value of x
Multiply -
\sqrt {27{a^3}{b^2}{c^4}} \times \root 3 \of {128{a^7}{b^9}{c^2}} \times \root 6 \of {729a{b^{12}}{c^2}}
Solve the following equation:
\displaystyle 81^{\sin^2 \, x} \, + \, 81^{\cos^2 \, x} \, = \, 30
Evaluate \dfrac{{{{(36)}^{\dfrac{{ - 7}}{2}}} - {{(36)}^{\dfrac{{ - 9}}{2}}}}}{{{{\left( {36} \right)}^{\dfrac{{ - 5}}{2}}}}}.
{27^x} = {\dfrac{9} {{3^x}}} find x
Solve the following equations. \left (\displaystyle\, 7.3^{x + 1} - 5^{x + 2} \right ) = \left (3^ {x + 4} - 5 ^{x + 3} \right )
Simplify: 2\root 5 \of {\root 4 \of {{{({2^3})}^4}} }
Prove that
{\dfrac{a + b + c}{{a^{ - 1}}{b^{ - 1}} + {b^{ - 1}}{c^{ - 1}} + {c^{ - 1}}{a^{ - 1}}}} = abc
Solve: \left( \cfrac{125}{343} \right)^{1/3}
Simplify:
\left(\dfrac{x^b}{x^c}\right)^a\times\left(\dfrac{x^c}{x^a}\right)^b\times\left(\dfrac{x^a}{x^b}\right)^c
Simplify : 2^{\frac{1}{4}} . 4^{\frac{1}{8}} . 8^{\frac{1}{16}} . 16^{\frac{1}{32}} ......
Convert into power notation
\dfrac { 1 }{1000 }
Find m. \dfrac { { \left( 3 \right) }^{ 2m+1 } }{ 27 } ({ 9 }^{ 2 })={ \left( \left( { -3 }^{ 8 } \right) { 3 }^{ 4 } \right) }^{ 5 } ?
Convert into power notation
\dfrac {-1 }{32 }
Convert into power notation
\dfrac {-1 }{343 }
Convert in to power notation
\dfrac{1}{27}
Convert into power notation
\dfrac { -27 }{125 }
If \dfrac{9^n \times 3^2 \times 3^n - (27)^n}{3^{3m} \times 2^3} = 3^{-3}, prove that (m - n) = 1.
Convert into power notation
\dfrac { -1 }{64 }
Convert into power notation
\dfrac {49 }{81}
Convert into power notation
156
Convert into power notation
343
If x=y^{2}, y=z^{2}, z=p^{2} where p =2^{36} \times 9^{48}, then \sqrt{x} is_____
Let a+b+c=0, then find the value of \sqrt[bc]{\dfrac{x^{a^2}}{x^{bc}}}\times\sqrt[ca]{\dfrac{x^{b^2}}{x^{ca}}}\times\sqrt[ab]{\dfrac{x^{c^2}}{x^{ab}}}.
Simplify:
\dfrac{m^{2} -n^{2}}{(m + n)^{2}} \times \dfrac{m^{2} +mn + n^{2}}{m^{3} - n^{3}}
Convert into power notation
169
Evaluate \dfrac { \left( { 3 }^{ -2 } \right) ^{ 2 }\times \left( { 5 }^{ 2 } \right) ^{ -3 }\times \left( { t }^{ -3 } \right) ^{ 1 } }{ \left( { 3 }^{ -2 } \right) ^{ 5 }\times \left( { 5 }^{ 3 } \right) ^{ -2 }\times \left( { t }^{ -4 } \right) ^{ 3 } }
Simplify:
\left(\dfrac{5^{-1} \times 7^2}{5^2 \times 7^{-4}}\right)^{7/2} \times \left(\dfrac{5^2 \times 7^3}{5^3 \times 7^{-5}}\right)^{-5/2}.
Write in exponential form: {2}^{3}+{2}^{5}+{2}^{6}-{2}^{2}
Solve:
\sqrt {{2^{x + 3}}} = 16
\sqrt {{2^{x + 3}}} = 16
Value of \dfrac{(81)^{3\times6}\times (9)^{2\times 7}}{(81)^{4\times 2}\times (3)} is?
Simplify:- \left[\dfrac {(-3)^{2}}{2}\right]^{3}
Solve \frac{8^{x} + 27^{x}}{12^{x} +18^{x}} =\frac{7}{6}
{ \left( 64 \right) }^{ 2/3 }+\sqrt [ 3 ]{ 125 } +{3}^{o}+\dfrac { 1 }{ { 2 }^{ -5 } } +{ \left( 27 \right) }^{ -2/3 }\times { \left( \dfrac { 25 }{ 9 } \right) }^{ -12 }
Exponential
{(34)}^{3}
If the radian measures of two angles of a triangle are as given below . find the radian measure and the degree measure of the third angle then.
\dfrac{3\pi}{5},\dfrac{4\pi}{15}
If{ 2 }^{ 3 }\frac{2}{3}^x=8\frac { 8 }{ 27 }Find the value of x?
Given 4725={ 3 }^{ a }{ 5 }^{ b }{ 7 }^{ c }, find
i) the integral values of a,b and c.
ii) the value of { 2 }^{ -a }{ 3 }^{ b }{ 7 }^{ c }
Express positive exponents{(\dfrac {5}{7})}^{-3} and {2}^{-5}
Simplify:
\dfrac{{{{3}^{30}} + {3^{29}} + {3^{25}}}}{{{3^{31}} + {{3}^{30}} - {3^{29}}}} + \dfrac{{{2^{30}} + {2^{29}} + {2^{25}}}}{{{2^{31}} + {2^{30}} - {2^{29}}}}
Simplify and find the value of x:-
{e^{x + 2\log x}}
Solve : \dfrac{25^{\frac{3}{2}} \times 343^{\frac{3}{5}}}{16^{\frac{5}{4}} \times 8^{\frac{4}{3}} \times 7^{\frac{3}{5}}}
\left(\dfrac{5}{7}\right)^3\times \left(\dfrac{21}{25}\right)^2.
The number of zeroes at the end of the sum {101^{11}} - 1
Express the following in exponential form:
(i) 6 \times 6 \times 6 \times 6 (ii) t \times t (iii) b \times b \times b \times b
(iv) 5 \times 5 \times 7 \times 7 \times 7 (v) 2 \times 2 \times a \times a (vi) a \times a \times a \times c \times c \times c \times c \times d
If a=2-\sqrt{5}/2+\sqrt{5} and b=2+\sqrt{5}/2-\sqrt{5} find (a+b)^3.
Simplify
{8^{\frac{2}{3}}} - \sqrt 9 \times 10 + {\left( {\frac{1}{{144}}} \right)^{ - \frac{1}{2}}}
Write the base and exponent of the following :
\left( \dfrac {-1}{3} \right)^3
Express the following in exponential from: \dfrac{4}{3}\times \dfrac{4}{3}\times \dfrac{4}{3}\times \dfrac{4}{3}\times \dfrac{4}{3}
What we should multiply with \dfrac {\sqrt{x^3} \sqrt[3]{x^5}}{\sqrt[5]{x^3}} . \sqrt[30]{x^{77}} to get 1
Evaluate; \dfrac {^{3}\sqrt {-125 \times 343}}{^{3}\sqrt {(-27)}\times (-64)}
Simplify and express of the following in exponential form.
25^4\div 5^3.
Simplify and express the following in the positive exponent form.
\left( 5{ \times 25 }^{ n-1 } \right) \div \left( { 5 }^{ n-1 }{ \times 25 }^{ n-1 }\times 25 \right)
Solve for x:2^{2x+1}+2^9=2^{10}.
Write the base and exponent of the following :
(-15)^4
If (a^m)^m=a^m, then m =
Find the value of :
(3^{0}+4^{0})\times 5^{0}
Simplify :
128^{\dfrac{-2}{7}}-(625^{-3})^{\dfrac{-1}{4}}+ {14(2401)^{\dfrac{-1}{4}}}
Express the following numbers in the exponential form:
(i) 1728
(ii) \dfrac{1}{512}
(iii) 0.000169
Solve the following equation and find the value of m in 3^{m}=\left[\dfrac{1}{9}\right].
Write the following numbers using base 10 and exponents:
(i) 12345
(ii) 1010.0101
(iii) 0.1020304
Value of x, satisfying {(\sqrt{3}+1)}^{2x}+{(\sqrt{3}-1)}^{2x}={2}^{3x}, is equal to
Solve the following equation and find the value of m in 7^{m}=343
Evaluate \left( \frac { a ^ { x } } { a ^ { y } } \right) ^ { z } \times \left( \frac { a ^ { y } } { a ^ { z } } \right) ^ { x }
Prove \left(\dfrac{2^a}{2^b} \right)^{a + b} \times \left(\dfrac{2^b}{2^c} \right)^{b + c} \times \left(\dfrac{2^c}{2^a} \right)^{c + a} = 1
Find {\left( {\dfrac{{16}}{{100}}} \right)^{ - 1/2}}
Express the following in exponential form:
b\times b\times b\times b
What number should {(-3)}^{7} be divided by so that the quotient may be equal to {(-3)}^{4}?
Solve
3.2\times { 10 }^{ 6 }\times 4.1\times { 10 }^{ -1 }
Find the value of x so that-
{3}^{2}\times{(-4)}^{2}={(-12)}^{2x}
Simplify:
{ \left[ { \left( \dfrac { 2 }{ 3 } \right) }^{ 2 } \right] }^{ 3 }\times { \left( \dfrac { 2 }{ 3 } \right) }^{ -4 }\times { 3 }^{ -1 }\times \dfrac { 1 }{ 6 }
Prove that \left( \frac { 1 } { x ^ { a - b } } \right) ^ { \frac { 1 } { a - c } } \left( x ^ { \frac { 1 } { b - c } } \right) ^ { \frac { 1 } { b - a } } \left( x ^ { \frac { 1 } { c - a } } \right) ^ { \frac { 1 } { c - b } } = 1
{a}^{\cfrac{4}{3}}\div {a}^{-\cfrac{2}{3}}=
If \left(x^{n^2}\right)^n=\left(x^{2^n}\right)^2 then prove that \sqrt[n+1]{n^3}=2.
Find the greatest:2^{300},3^{200}.
(9^{\dfrac43}\div 27^{\dfrac23})\times 3^{\dfrac32}
Solve: {\left( {81} \right)^{ - 1/4}} \times \sqrt[4]{{81}}
Solve
\dfrac { 1.5\times { 10 }^{ 6 } }{ 2.5\times { 10 }^{ -4 } }
Write the following numbers in the expanded exponential forms: 7805192
Evaluate :
\dfrac{3^{4}\times 12^{3}\times 36}{2^{5}\times 6^{3}}
Write the following in the form of 4 ^ { n } :
(i) 16 (ii) 8
Solve the equation 9^{x+2}-6\times3^{x+1}+1=0
(5^{3}\div 3^{-2})\times 5^{-6}
Simplify and express in exponential form: \left[ \left( \dfrac { - 3 } { - 5 } \right) ^ { 4 } \right] ^ { 3 }
Find the approximant value of (36)^{\dfrac{1}{6}}.
Simplify:\left(\dfrac{625}{256}\right)^{-\dfrac{3}{4}}.
If {5}^{a}={25}^{5} then a is equal to
Solve:{5}^{3x+1}={25}^{x+2}
The population of India is 125,00000000. Write in exponential form.
If {\left( {2.3} \right)^x} = {\left( {0.23} \right)^y} = 1000 then find the value of \frac{1}{x} - \frac{1}{y}.
Solve:{2}^{{x}^{2}}:{2}^{2x}=8:1
Using laws of exponents, simplify and write the answer in exponential form: { \left( { 3 }^{ 4 } \right) }^{ 3 }
{ e }^{ \left( 1+{ sin }^{ 2 }x+{ sin }^{ 4 }x+....\infty \right) log2 }=16 then
Simplify: \dfrac{15^{3}\times 9^{3}\times 16}{3^{4}\times 5^{2}\times 2^{3}}
Which is greatest {2}^{12} or {3}^{8}
Find the value of x if {0.6}^{x}{\left(\dfrac{25}{9}\right)}^{{x}^{2}-12}={\left(\dfrac{27}{125}\right)}^{3}
Find the value of x
{ \left( \dfrac { 5 }{ 3 } \right) }^{ -4 }\times { \left( \dfrac { 5 }{ 3 } \right) }^{ -5 }={ \left( \dfrac { 5 }{ 3 } \right) }^{ 3x }
Find the unit's place in the expansion {2}^{2007}
If {3}^{x+y}=81 and {81}^{x-y}=3, then find the values of x and y
Find the unit's place in the expansion {7}^{131}
Using laws of exponents, simplify and write the answer in exponential form: \dfrac{{\left({5}^{2}\right)}^{3}}{{5}^{3}}
Find the unit's place in the expansion {3}^{2006}
Solve :[{(2^{-1})^{-1}}^{-1}]^{-1}
9x+5={ e }^{ 10b }
9x+5=2.688\times { 10 }^{ 43 }
Find the value of n, where n is an integer and 2^{n-5}\times 6^{2n-4}=\dfrac{1}{12^{4}\times 2}
Evaluate:
\dfrac {3\times 27^{n+1}+9\times 3^{n-1}}{8\times 3^{3n}-5\times 27^{n}}
Using laws of exponents, simplify and write the answer in exponential form: \dfrac{{8}^{t}}{{8}^{2}}
If a=3,\,b=7, then find the value of {a}^{b}-{b}^{a}
Find the value of m-n such that a^m=a^n.
Evaluate \sqrt [ 4 ]{ \cfrac { 256 }{ 625 } } -\sqrt { \cfrac { 9 }{ 25 } }
Change 8.3705\times 10^{6} in usual form.
Find the value of n :
\left(\dfrac{3}{2}\right)^{6}\times\left(\dfrac{3}{2}\right)^{2n+1}\times \left(\dfrac{3}{2}\right)^{-3}=1
\dfrac { (2{ p }^{ 2 }{ q }^{ 9 })^{ 2 }(4pq)^{ 3 } }{ (4{ p }^{ 6 }{ q }^{ 10 })^{ 2 } }
Express 512 in exponential form
Express in exponential form:
\sqrt [ 3 ]{ { xy }^{ 2 } } \div { x }^{ 2 }y\\
Find the values:
2^{3+\log_{2}3}
{\left( {{{81} \over {16}}} \right)^{{{ - 3} \over 4}}} \times {\left( {{{25} \over 9}} \right)^{{{ - 3} \over 2}}}
Express the following numbers in standard from:
3759\times 10^{4}
Simplify: (81 / 16)^{-3 / 4} \times\left[(25 / 9)^{-3 / 2} \div(5 / 2)^{-3}\right]
Solve : { 3 }^{ 5}\times { 3 }^{ -6 }
Simplify \sqrt [4]{81}-8\sqrt [3]{216}+15\sqrt [3]{8}+\sqrt {225}
Solve for x.
5^{x+1} + 5^{1-x} = 26
Solve : \dfrac { { 3 }^{ -5 }\times{ 10 }^{ -5 }\times {125} }{ { 5 }^{ -7 }\times { 6 }^{ -5 } }
Prove that : \frac{2^{30}+2^{29}+2^{28}}{2^{31}+2^{30}-2^{29}}=\frac{7}{10}
Simplify \sqrt [ 5 ]{ 243{ a }^{ 10 }{ b }^{ 5 }{ c }^{ 10 } }
Express in exponential form: \dfrac { 81 }{ 625 }
Evaluate : \left (\dfrac{-4}{5}\right)^{-10} \times \left (\dfrac{-4}{5}\right)^{15} \div \left (\dfrac{-4}{5}\right)^{8}
Evaluate using law of exponents: 25^{4}\div 5^{3}
By what number should { 3 }^{ -4 } be multiplied so that the products is 729
Given 4725 = 3^{a}5^{b}7^{c}, find the value of 2^{a}3^{b}7^{c}
If 5^{3x} = 125 and 10^{y} = 0.001 then find x + y.
Express each of the following as a product of prime factors only in exponential form:
768
Prove that :
\left ( \dfrac{x^{a}}{x^{b}} \right )^{a^{2}+ab+b^{2}}\times \left ( \dfrac{x^{b}}{x^{c}} \right )^{b^{2}+bc+c^{2}}\times \left ( \dfrac{x^{c}}{x^{a}} \right )^{c^{2}+ca+a^{2}} = 1
Express the following numbers in standard from:
0.00072984
Write the given expression in the product form.
9xy^2z^2
3.25\times 10^{2} in the usual form is 325
Enter 1 if it is True or 0 if it is False
3\times 10^{8} in the usual form is 300000000Enter 1 if it is True or 0 if it is False.
Write the given expression in the product form.
6x^7y.
Write the following numbers in the usual form:
5.8\times 10^2
Write the following numbers in the usual form:
3.61492\times 10^6
Write the given product in exponential form.
5\times a\times a\times a \times b\times b\times c\times c\times c.
Write the given expression in the product form.
7a^3b^4.
Write the following numbers in the usual form:
1.0001\times 10^9
Express each of the following in exponential from: (-2)\times (-2)\times(-2)\times (-2) \times a \times a \times a
Express the following in exponential form: \dfrac{-5}{7}\times \dfrac{-5}{7}\times \dfrac{-5}{7}\times \dfrac{-5}{7}
Express each of the following in exponential from: \left ( \dfrac{-2}{3} \right )\times \left ( \dfrac{-2}{3} \right )\times x \times x \times x
Write the following numbers in the expanded exponential forms: 927303
In a large hall there are 4x^2 rows of benches. If each row has 5x^2y^3 benches and each bench can accommodate xy^2 persons, determine the total number of persons if its is full up to its capacity.
Simplify: \left ( \dfrac{-2}{3} \right )^{4}
Express each of the following in exponential from: \dfrac{4}{3}\times \dfrac{4}{3}\times \dfrac{4}{3}\times \dfrac{4}{3}\times \dfrac{4}{3}
Express the following in exponential form: x\times x \times x\times x \times a \times a \times b \times b \times b
Write down the given expression in the product form.
10a^3b^3c^3.
Express each of the following rational numbers in power notation:
-\dfrac{1}{216}
Write 9 \times 9 \times 9 \times 9\times 9 in exponential form with base 3.
Write the following number in the expanded exponential forms: 20068
Express each of the following numbers as a product of powers of their prime factors: 2800
Express each of the following rational numbers in power notation:
\dfrac{49}{64}
Write the following numbers in the expanded exponential forms: 420719
Express the following number as a product of powers of their prime factors: 2800
Express the following number as a product of powers of their prime factors: 450
Express the number appearing in the standard form:
Diameter of the Sun is 1,400,000,000 metres.
Diameter of the Sun is 1,400,000,000 metres.
If x is a positive real number and exponents are rational numbers, simplify \left(\dfrac{x^b}{x^c}\right)^{b+c-a}\times \left(\dfrac{x^c}{x^a}\right)^{c+a-b}\times \left(\dfrac{x^a}{x^b}\right)^{a+b-c}.
Prove that \dfrac{(x^{a+b})^2(x^{b+c})^2(x^{c+a})^2}{(x^ax^bx^c)^4}=1.
If \dfrac{9^n\times 3^2\times (3^{-\tfrac{n}{2}})^{-2}-(27)^n}{3^{3m}\times 2^3}=\dfrac{1}{27}, prove that m-n=1.
Write the following numbers in the expanded exponential forms: 5004132
What number must be subtracted from
1,02,59756 to get 77,63,835 ?
The sale receipt of a company during a year was Rs.30587850. Next year it increased by Rs.6375490. What was the total sale receipt of the company during these two years ?
Express the following as a rational number:
((5)^{-1}-(7)^{-1})^{-1}
Express 16/81 and -16/81 as powers of a rational number.
Solve the following:
\left( \dfrac 12 \right)^{-2}\div \left( \dfrac 12\right)^{-3}
Express 27/64 and -27/64 as powers of a rational number.
Simplify :
(2^5 \div 2^8) \times 2^{-7}
Express as a power of a rational number with negative exponent.
(2^5 \div 2^8)\times 2^{-7}
The usual form of 2.39461 \times 10^6 is _______.
Simplify :
(2^{-1}+4^{-1}+6^{-1}+8^{-1})^x=1
Find the value of x^{-3} if x=(100)^{1-4}\div (100)^0.
The usual form of 3.41 \times 10^6 is _______.
3^5 \div 3^{-6} can be simplified as ________ .
To add the numbers given in the standard form, we first convert them into numbers with _____ exponents.
The usual form for 2.3 \times 10^{-10} is _____.
The value of 3 \times 10^{-7} is equal to _______.
On dividing 8^5 by ________ we get 8
Mass of Mars is 6.42\times 10^{29}\ kg and mass of the Sun is 1.99\times 10^{30}\ kg. What is the total mass?
By what number should \left( \dfrac{-3}{2}\right)^{-3} be divided so that the quotient may be \left( \dfrac{4}{27}\right)^{-2}?
A new born bear weights 4\ kg. How many kilograms might a five year old bear weight if its weight increases by the power of 2 in 5 years?
This table shows the mass of one atom for five chemical elements. Use it to answer the question given.
| Element | Mass of atom (kg) |
| Titanium | 7.95\times 10^{-26} |
| Lead | 3.44\times 10^{-25} |
| Silver | 1.79\times 10^{-25} |
| Lithium | 1.15\times 10^{-26} |
| Hydrogen | 1.674\times 10^{-27} |
Planet A is at a distance of 9.35\times 10^6\ km from Earth and planet B is 6.27\times 10^7\ km from Earth. Which planet is nearer to Earth?
By what number should (-15)^{-1} be divided so that quotient may be equal to (-15)^{-1}?
\Big\{\Big[6^2 +(8^2)^{\frac{1}{2}}\Big]\Big\}^3
This table shows the mass of one atom for five chemical elements. Use it to answer the question given.
| Element | Mass of atom (kg) |
| Titanium | 7.95\times 10^{-26} |
| Lead | 3.44\times 10^{-25} |
| Silver | 1.79\times 10^{-25} |
| Lithium | 1.15\times 10^{-26} |
| Hydrogen | 1.674\times 10^{-27} |
Use the properties of exponents to varify that the statement is true.
25(5^{n-2})=5^n
Find a single repeater machine that will do the same work as the hook -up.
Find the value of:
(-3\dfrac{1}{2})^{3}
This table shows the mass of one atom for five chemical elements. Use it to answer the question given.
| Element | Mass of atom (kg) |
| Titanium | 7.95\times 10^{-26} |
| Lead | 3.44\times 10^{-25} |
| Silver | 1.79\times 10^{-25} |
| Lithium | 1.15\times 10^{-26} |
| Hydrogen | 1.674\times 10^{-27} |
The given table shows the crop production of a state in the year 2008 and 2009. Observe the table table given below and answer the given questions.
| Crop | 2008 Harvest ( Hectare) | Increase/ decrease ( Hectare ) in 2009 |
| Bajra | 1.4\times 10^3 | -100 |
| Jowar | 1.7\times 10^6 | -440,000 |
| Rice | 3.7\times 10^3 | -100 |
| Wheat | 5.1\times 10^5 | +190,000 |
Find three repeater machines that will do the same work as a(\times 64) machine. Draw them, or describe those using exponents.
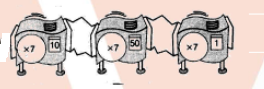
What will the following machine do to a 2\ cm long piece of chalk?
At the end of the 20th century, the world population was approximately 6.1\times 10^9 people. Express this population in usual form. How would you say this number in words?
The volume of the Earth is approximately 7.67\times 10^{-7} times the volume of the Sun. Express this figure in usual form.
Sanchay put a 1\ cm stick of gum through a(1\times 3^{-2}) machine. How long was the stick when it came out ?
What happens when 1\ cm worms are sent through these hook-ups?
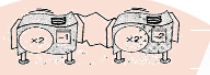
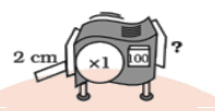
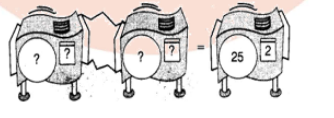
Find a single machine that will do the same job as the given hook-up.
A(\times 2^4) machine followed by \left[ \times \left( \dfrac{1}{2}\right)^2\right] machine.
For the hook-up, Find the output for 3\ unit input workIs other single repeater machine possible which can produce same amount of work.
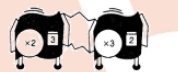
If possible, find a hook-up of prime base number machine that will do the same work as the given stretching machine. Do not use (\times 1) machines.

If possible, find a hook-up of prime base number machine that will do the same work as the given stretching machine. Do not use (\times 1) machines.
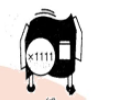
Find a repeater machine that will do the same work as a \left( \times \dfrac{1}{8}\right) machine.
Find the two repeater machines that will do the same work as (\times 81) machine.
If possible, find a hook-up of prime base number machine that will do the same work as the given stretching machine. Do not use (\times 1) machines.

Neha needs to stretch some sticks to 25^2 times their original lengths, but her (\times 25) machine is broken. Find a hook-up of two repeater machines that will do the same work as (\times 25^2) machine. To get started, think about the hook-up you could use to replace the (\times 25) machine.
Express the following in exponential form:
\dfrac{-125}{343}
Express the following in exponential form:
\dfrac{400}{3969}
Compare the following numbers:
3.7662 \times 10^{17}, 3.7671 \times 10^{17}
Find x.
\left( -\dfrac 17\right)^{-5}\div \left( -\dfrac 17\right)^{-7}=(-7)^x
The distance between the Sun and the Earth is 1.496\times 10^8\ km and distance between the Earth and the Moon is 3.84\times 10^8\ m. During solar eclipse the Moon comes in between the Earth and the Sun. What is the distance between the Moon and the Sun at that particular time?
Express the following in exponential form:
\dfrac{-1296}{14641}
By what number should (-15)^{-1} be divided so that the quotient may be equal to (-5)^{-1}?
Express the following in exponential form:
\dfrac{-625}{10000}
Find the value of (-6)^{4}
Find the value of (\frac{-2}{3})^{5}
In the expression 3^7, base = ........ and exponent = ........
Find the value of (-2)^{9}
Find the value of 5^{5}
Find the number from the expanded form:
8 \times 10^7 + 3 \times 10^4 + 7 \times 10^3+ 5 \times 10^2+8 \times 10^1
Find the number from the expanded form:
4 \times 10^5 + 5 \times 10^3 + 3 \times 10^2 + 2 \times 10^0
Identify the greater number in 4^5 or 5^4
Fill in the blanks:
In the expression (\frac{2}{5})^{11}, base = ...... and exponent = .......
Compare the following numbers:
1.439 \times 10^{12}, 1.4335 \times 10^{12}
Compare the following numbers:
5.976 \times 10^{24}, 8.689 \times 10^{23}
Simplify:
\bigg(\dfrac{-1}{2}\bigg)^{5} \times 2^6 \times \bigg(\dfrac{3}{4}\bigg)^{3}
Simplify and write the exponential form with negative exponent:
5^{3}\times (4/5)^3
Write the following numbers in expanded from using exponents:
2789.453
Simplify and write the following exponential form:
3^{-5}\times 3^2\div 3^{-6}+(2^2 \times 3)^2 +\left(\dfrac{2}{3}\right)^{-1}+2^{-1} +\left(\dfrac{1}{19}\right)^{-1}
Simplify and write in exponential form with positive exponent:
\dfrac{[8^{-1}\times 5^3]}{2^{-4}}
Simplify and write in exponential form with positive exponent:
(2/7)^2\times (7/2)^{-3}\div \left\{(7/5)^{-2}\right\}^{-4}
Simplify and write the exponential form with negative exponent:
[(3/7)^{-2}]^{-3}
Simplify and write in exponential form with positive exponent:
(4/5)^2 \times 5^4\times (2/5)^{-2}\div (5/2)^{-3}
Write the following number in expanded from using exponents:
3007.805
Simplify and write the following exponential form:
((-2)^3)^2 +5^{-3}\div 5^{-5}-(-1/2)^0
Simplify and write the exponential form with negative exponent:
(5/9)^{-2}\times (5/3)^2\div (1/5)^{-2}
Simplify and write the exponential form with negative exponent:
2^{-1}\bigg [\bigg( \dfrac 53\bigg)^4 +\bigg( \dfrac 35\bigg) ^{-2}\bigg ]\div \dfrac {17}{9}
Express the number appearing in the following statement in standard form:
A helium atom has a diameter of 22/100000000000 m.
Express the number appearing in the following statement in standard form:
The mass of a proton is 0.000000000000000000000001673 gram.
The number of red blood cells per cubic millimeter of blood is approximately 5.5 million. If the average body contains 5 liters of blood, what is the total number of red cell in the body? \left(1\ liter=1,00,000\ mm^{3}\right)
Express the number appearing in the following statement in standard form:
Express 7 hectares in cm^{2}.
Compare the following:
Size of a plant cell to the diameter of a wire on a computer chip.Given size of plant cell =0.00001275\ m=1.275\times 10^{-5}\ mDiameter of a wire on a computer chip =0.000003\ m=3.0\times 10^{-6}\ m
Given size of plant cell =0.00001275\ m=1.275\times 10^{-5}\ m
Diameter of a wire on a computer chip =0.000003\ m=3.0\times 10^{-6}\ m
Compare the following:
The thickness of a piece of paper to the diameter of wire on a computer chip.
Given Thickness of a piece of paper =0.0016\ cm
Diameter of a wire on a computer chip =0.000003\ m
Express the number appearing in the following statement in standard form:
The speed of light is 300,000,000\ m/sec.
Express the number appearing in the following statement in standard form:
A sugar factory has annual sales of 3 billion 720 million kilograms of sugar.
Express the number appearing in the following statement in standard form:
Express 3 years in seconds.
Compare the following:
Size of a plant cell to the thickness of a piece of paper.Given size of plant cell =0.00001275\ m=1.275\times 10^{-5}\ mThickness of a piece of paper =0.0016\ cm=1.6\times 10^{-3}cm
Given size of plant cell =0.00001275\ m=1.275\times 10^{-5}\ m
Thickness of a piece of paper =0.0016\ cm=1.6\times 10^{-3}cm
Which is greater:
2^3 or 3^2
Express each of the following in exponential form:
1350
Express each of the following in exponential form:
3600
Express each of the following in exponential form:
512
Which is greater:
4^3 or 3^4
Which is greater:
2^5 or 5^2
Simplify, giving answers with positive index:
(2a^3)^4 (4a^2)^2
Simplify, giving answers with positive index:
(10^2)^3 (x ^8)^{12}
Express in simplest exponential form:
25*125.
Express in simplest exponential form:
16*64
9^{0}\times 4^{-1}\div 2^{-4}
Express in simplest exponential form:
128\div 32
Can you find two integers m,n such that { 2 }^{ m+n }={ 2 }^{ mn }?
Simplify the following using laws of indices:
(3^{-\tfrac 12} \times 2^{-\tfrac 13}) \div (3^{-\tfrac 34} \times 2^{-\tfrac 56})
Express the following in exponential form:
t\times t
In a particular year, a company manufactured 8570435 bicycles and next year it
manufactured 8756430 bicycles. In which year more bicycles were manufacture and by how many?
Find x, if
\left (\dfrac {8}{3}\right)^{2x+1} \times \left (\dfrac {8}{3}\right)^{5} = \left (\dfrac {8}{3}\right)^{x+2}
If 2^4\times 4^2=16^x, then find the value of x.
Find x, if
\left (\dfrac {-1}{2}\right)^{-19} \div \left (\dfrac {-1}{2}\right)^{8} = \left (\dfrac {-1}{2}\right)^{-2x+1}
Find x, if
\left (\dfrac {3}{2}\right)^{-3} \times \left (\dfrac {3}{2}\right)^{5} = \left (\dfrac {3}{2}\right)^{-2x+1}
Find x, if
\left (\dfrac {5}{4}\right)^{-x} \div \left (\dfrac {5}{4}\right)^{-4} = \left (\dfrac {5}{4}\right)^{5}
If \displaystyle a^m\cdot a^n=a^{mn}, then m(n-2)+n(m-2) is?
Class 7 Maths Extra Questions
- Algebraic Expressions Extra Questions
- Comparing Quantities Extra Questions
- Data Handling Extra Questions
- Exponents And Powers Extra Questions
- Fractions And Decimals Extra Questions
- Integers Extra Questions
- Lines And Angles Extra Questions
- Perimeter And Area Extra Questions
- Practical Geometry Extra Questions
- Rational Numbers Extra Questions
- Simple Equations Extra Questions
- Symmetry Extra Questions
- The Triangle And Its Properties Extra Questions
- Visualising Solid Shapes Extra Questions