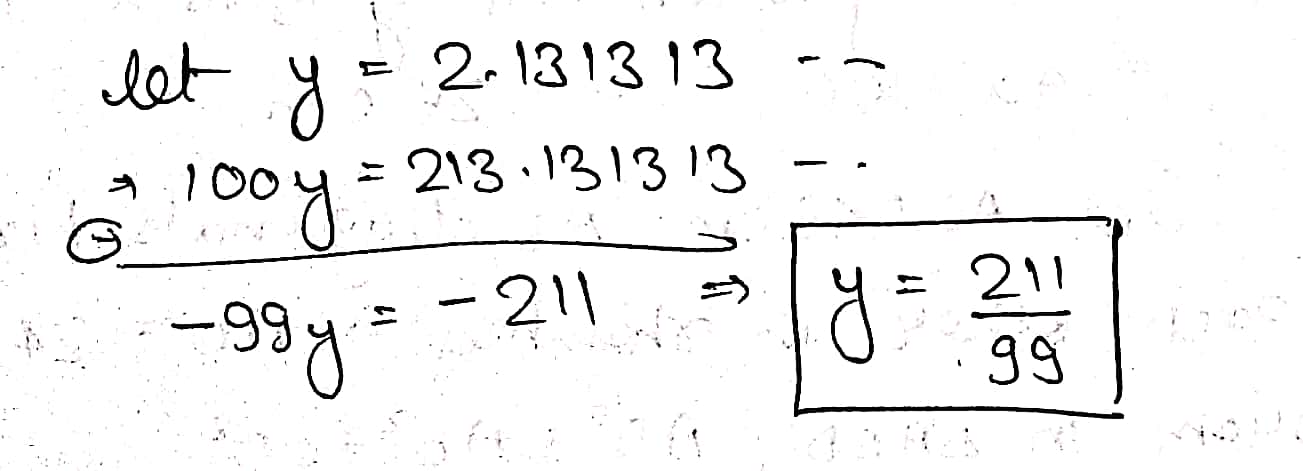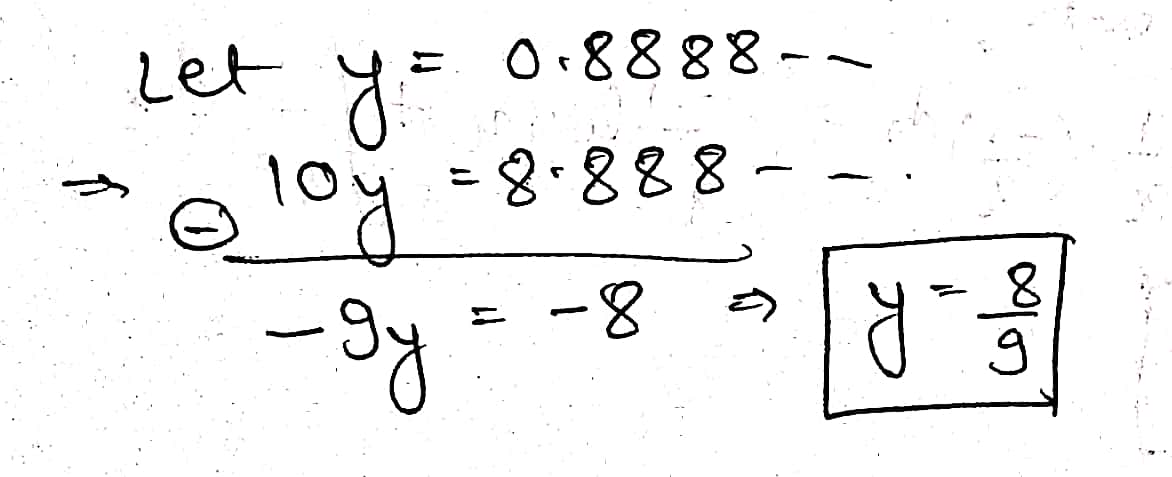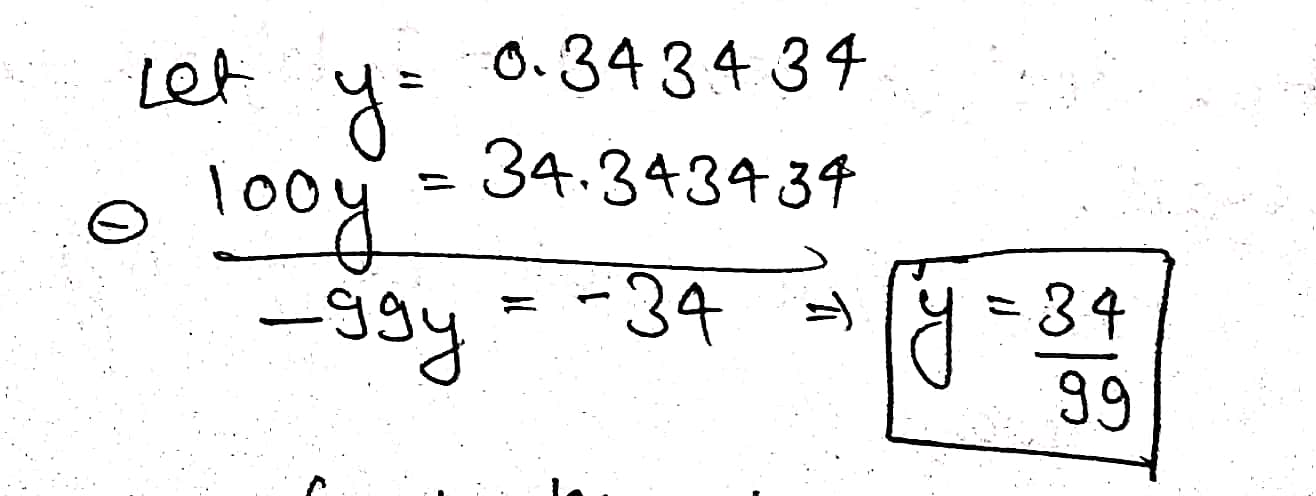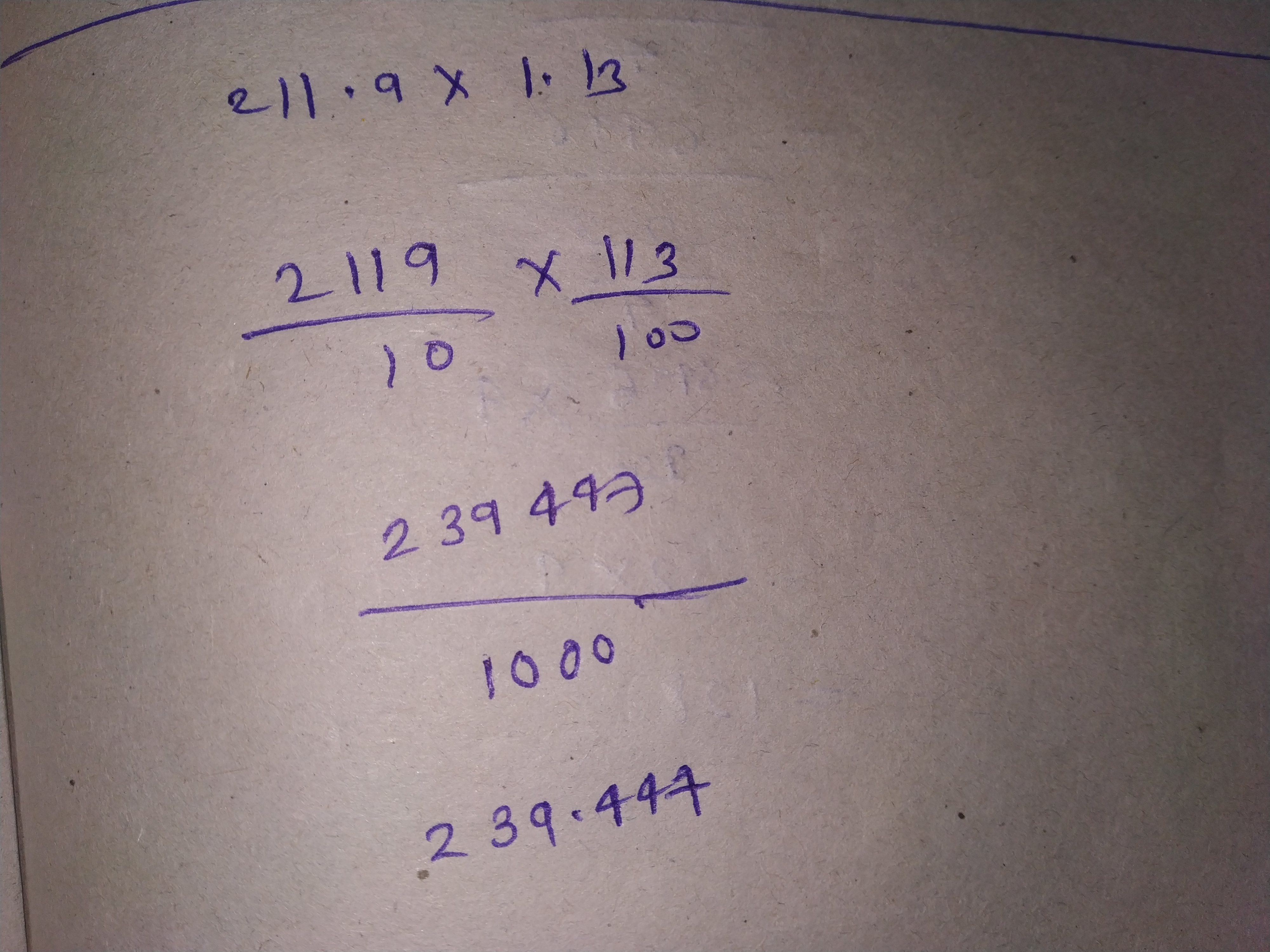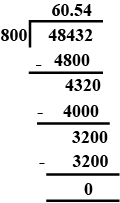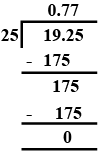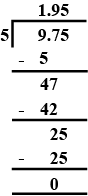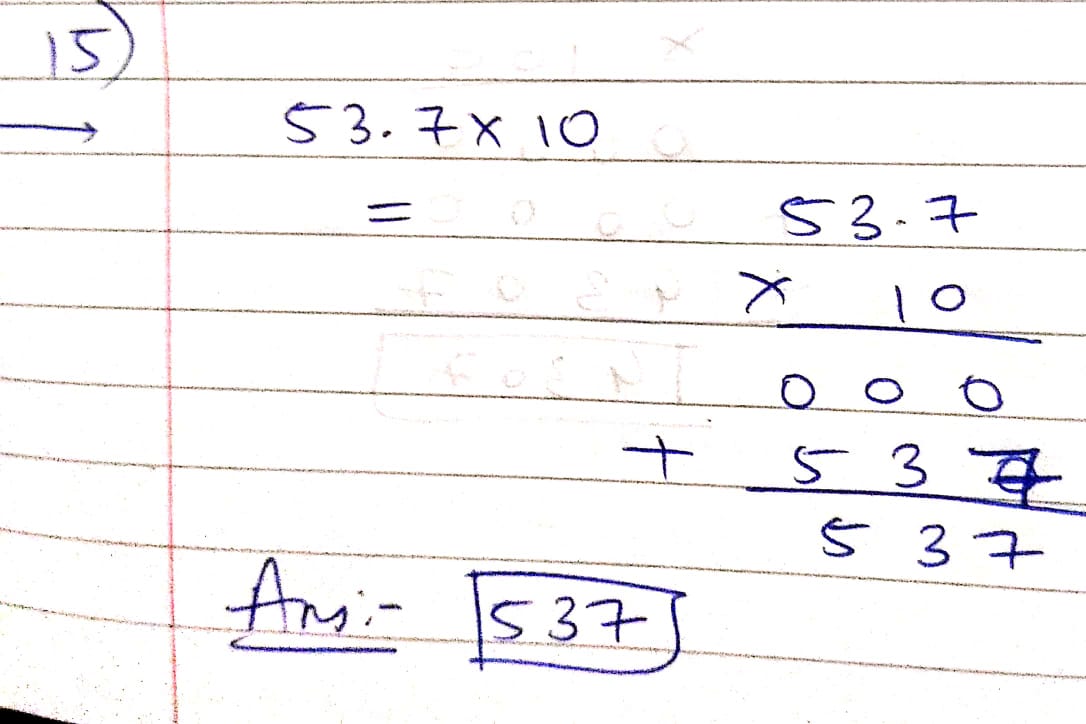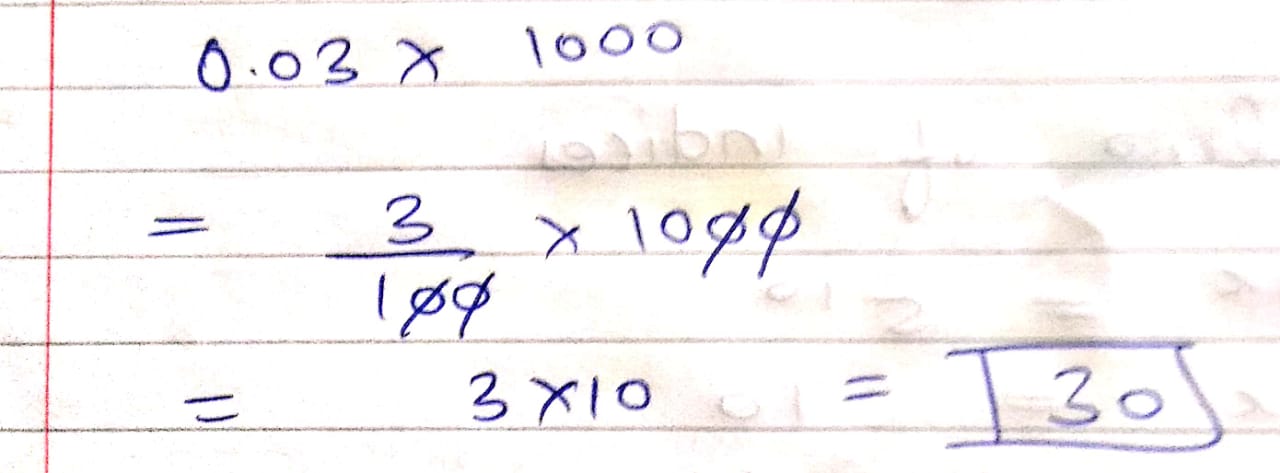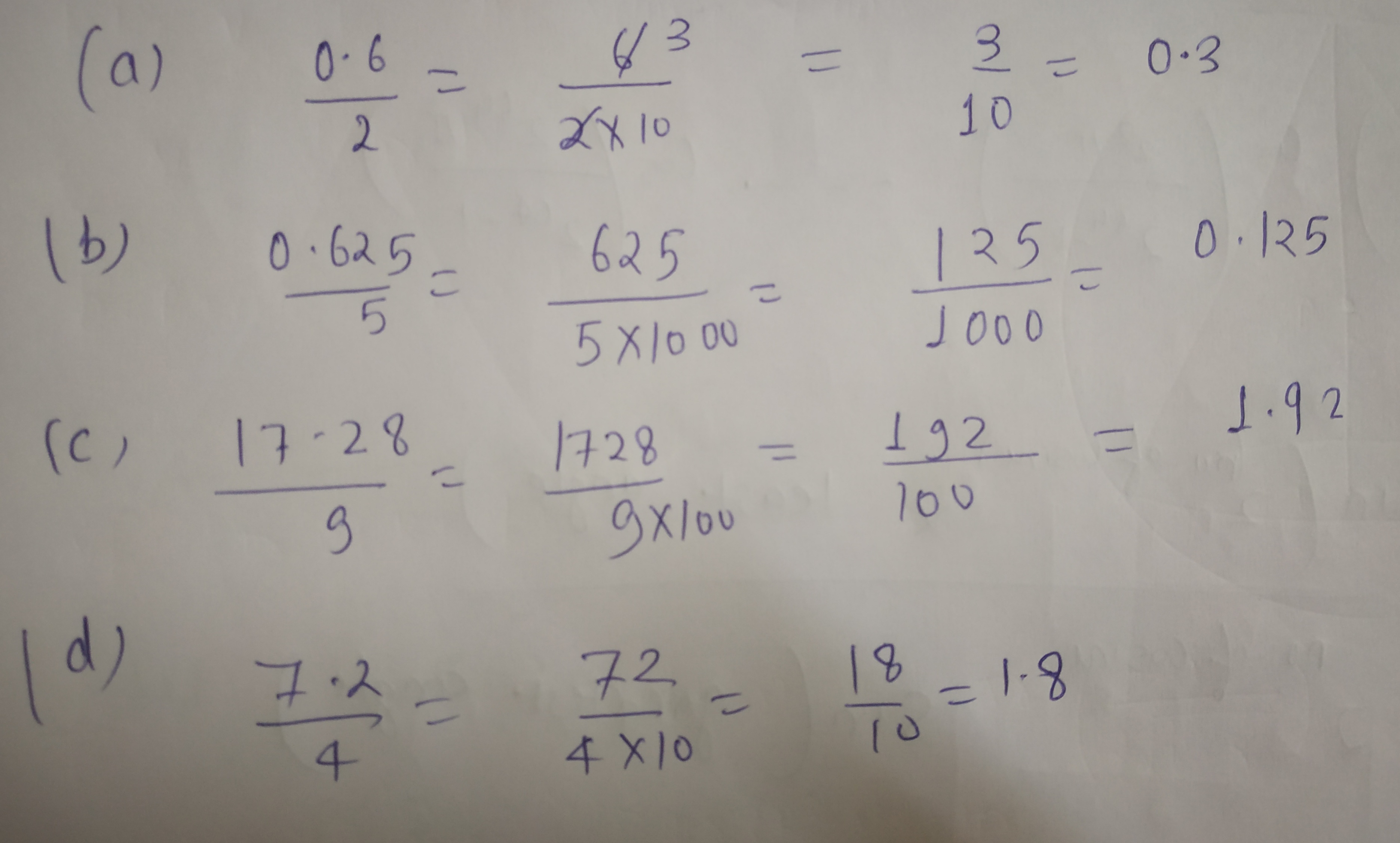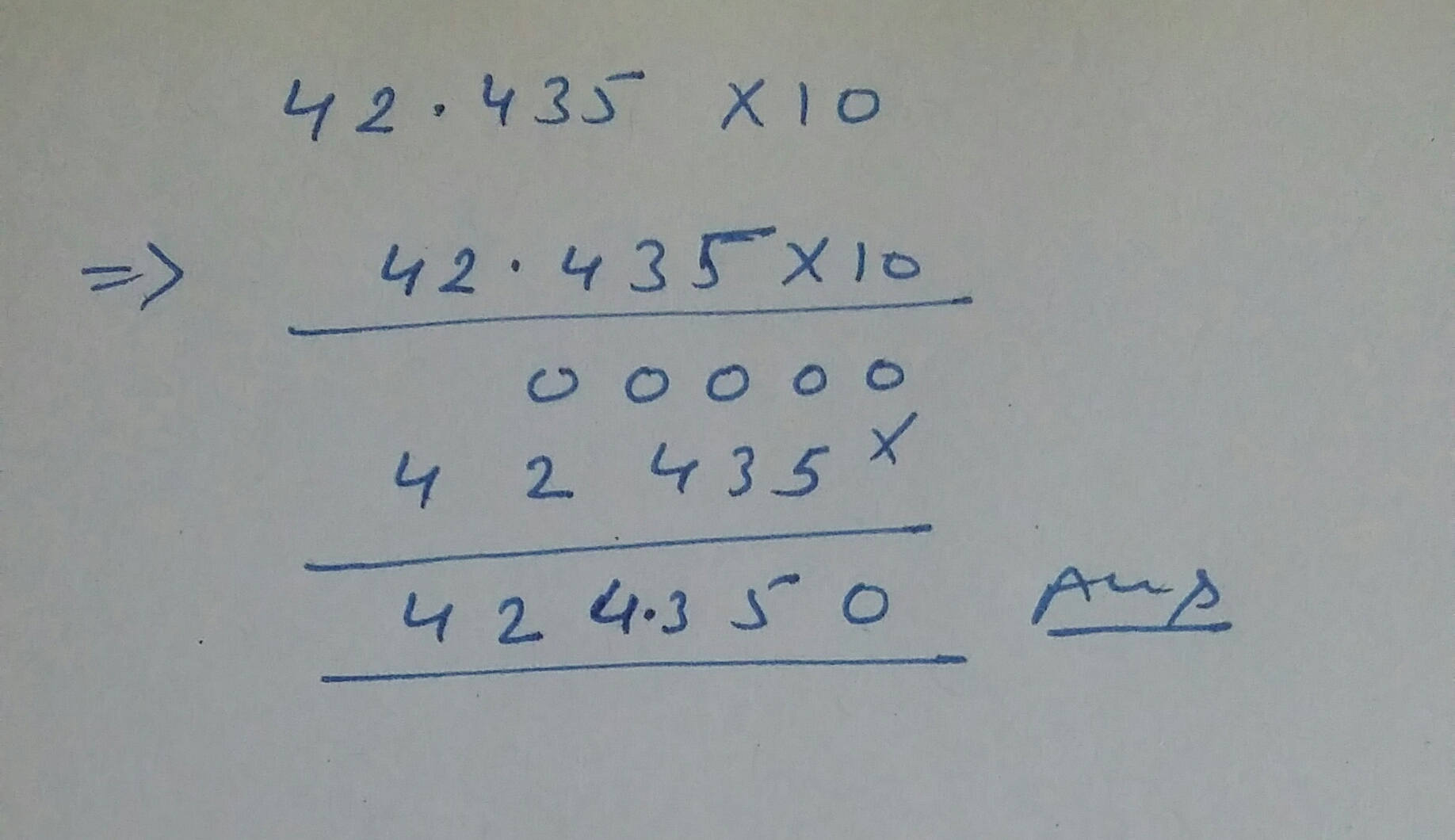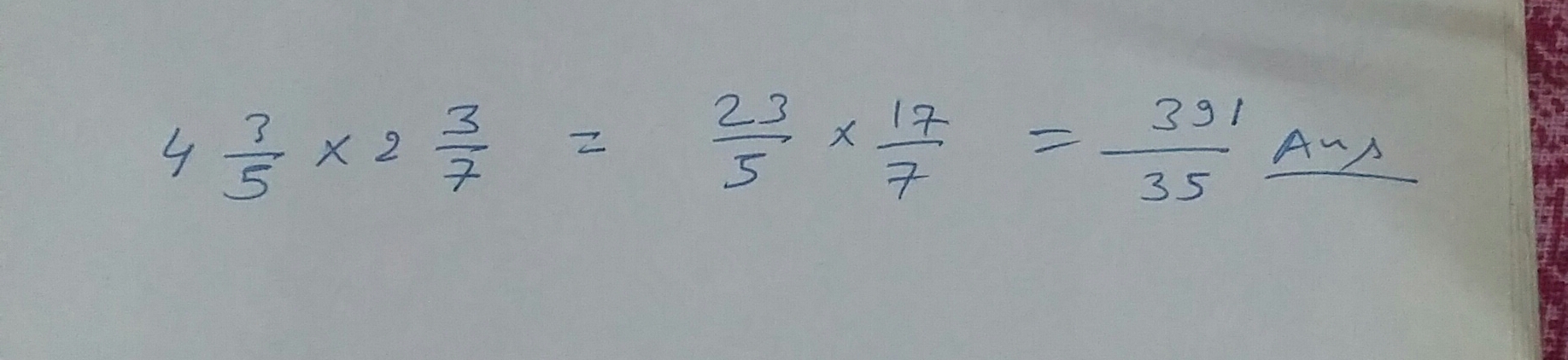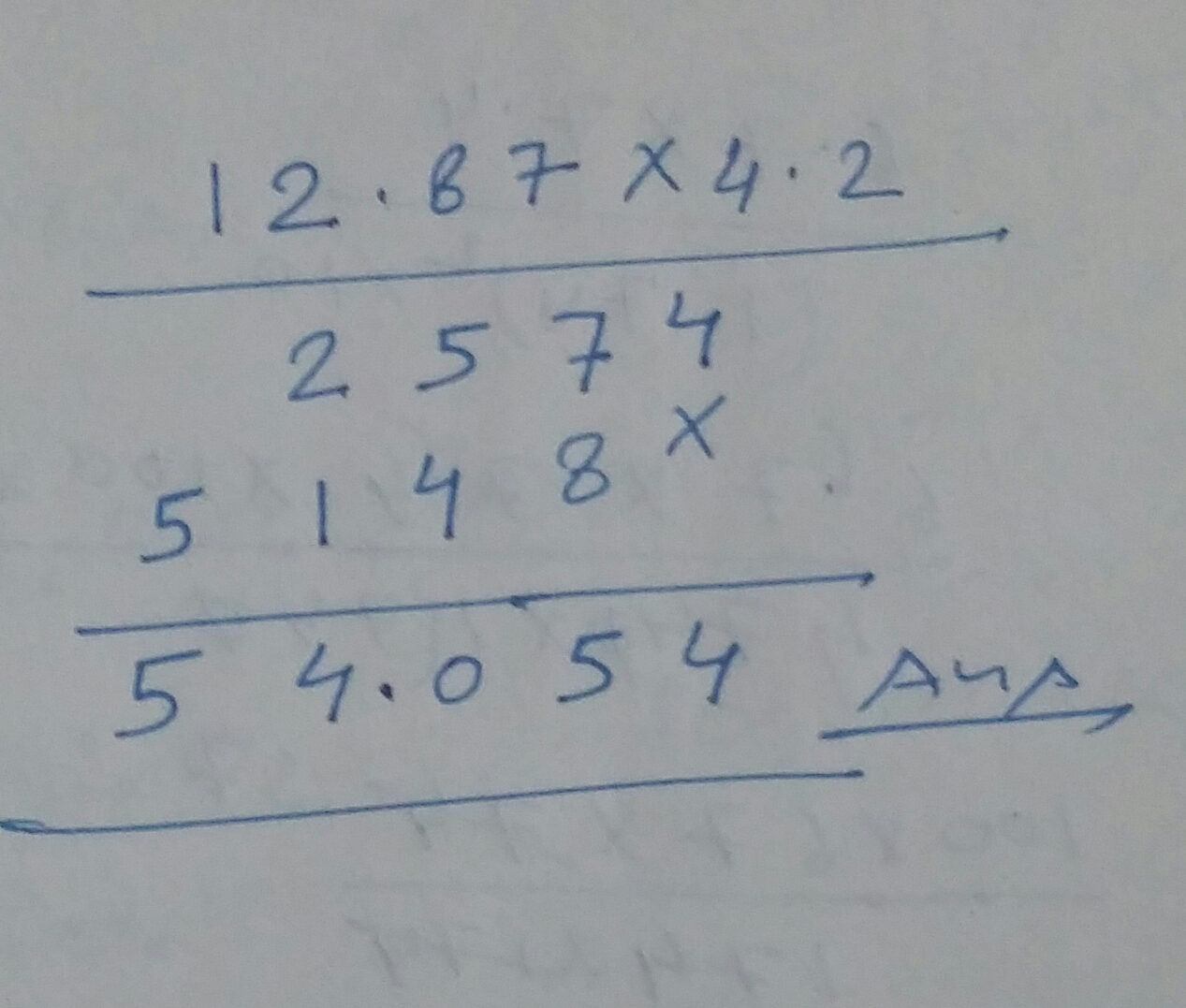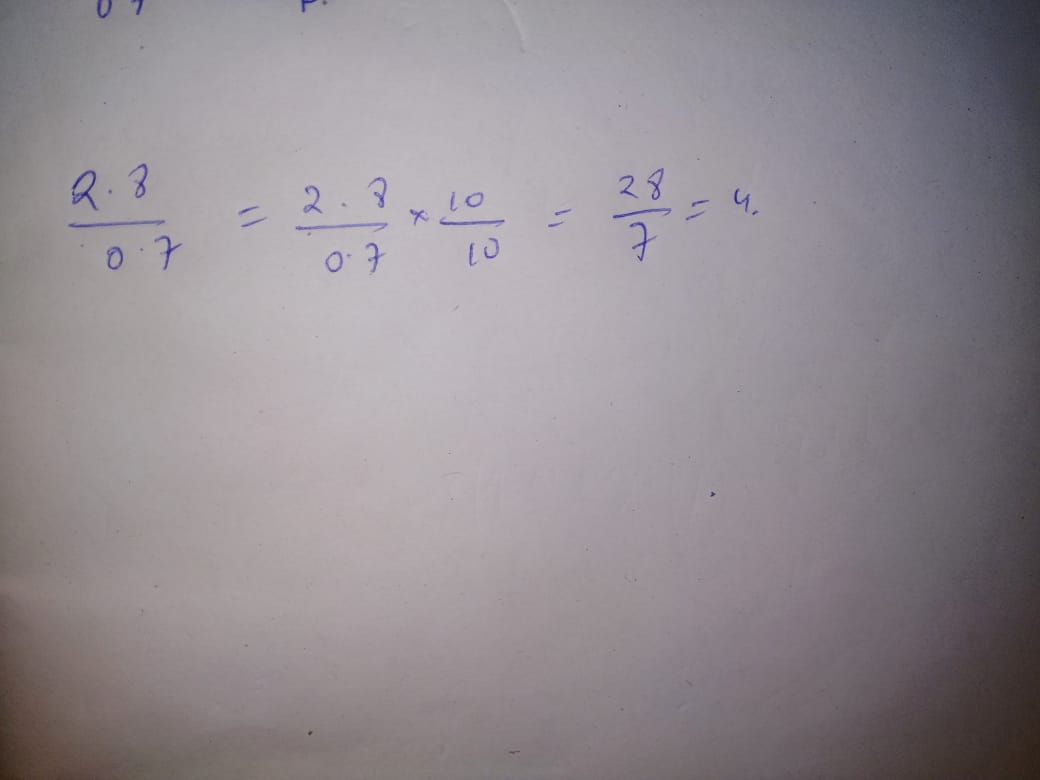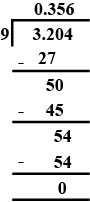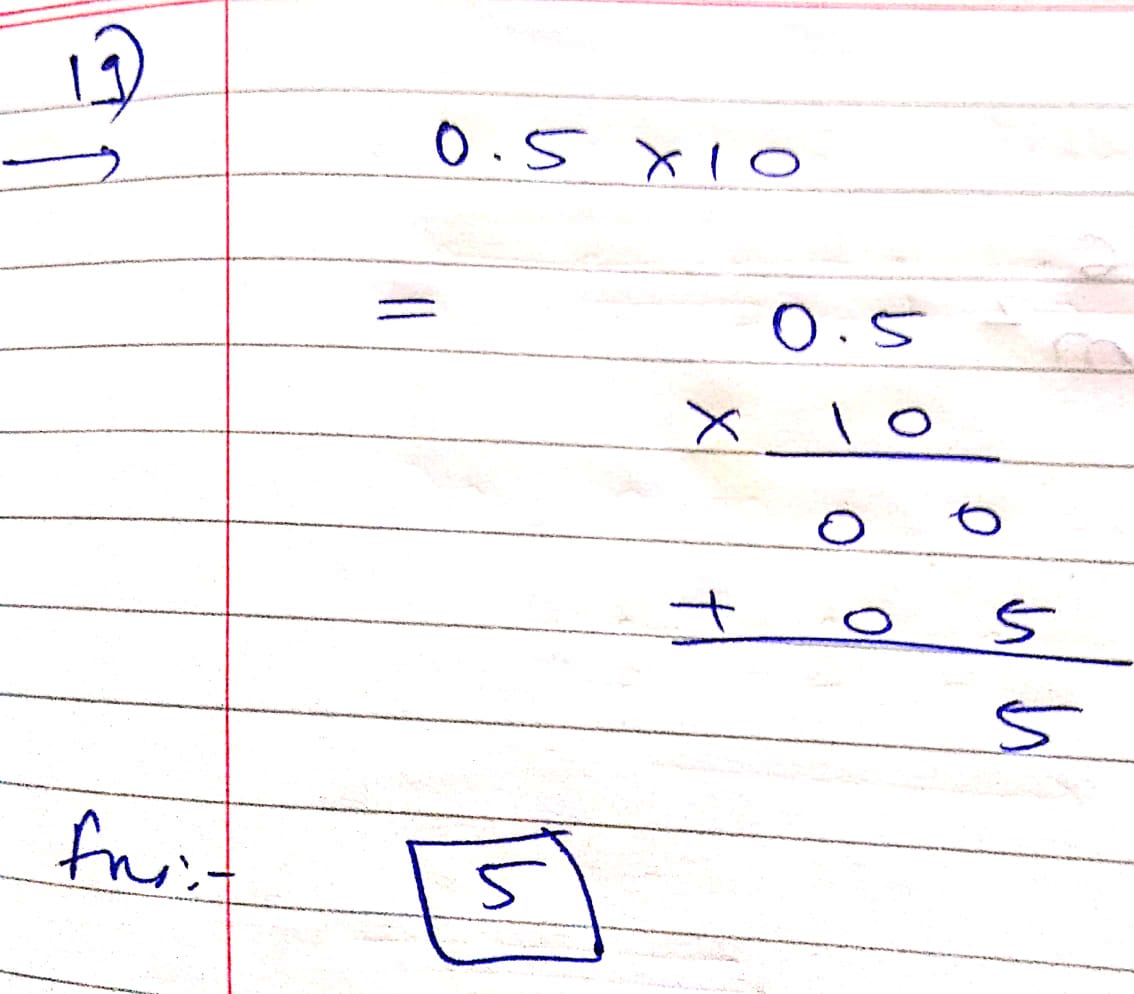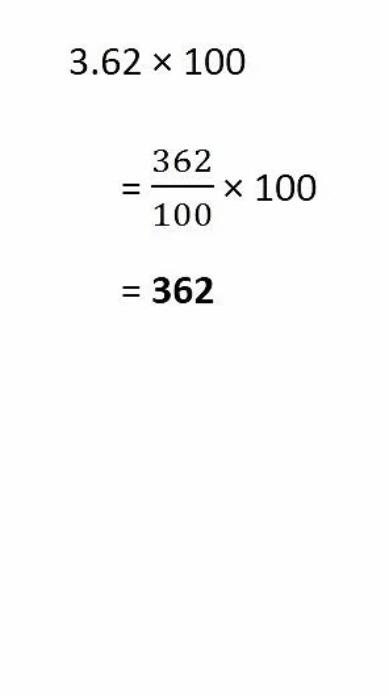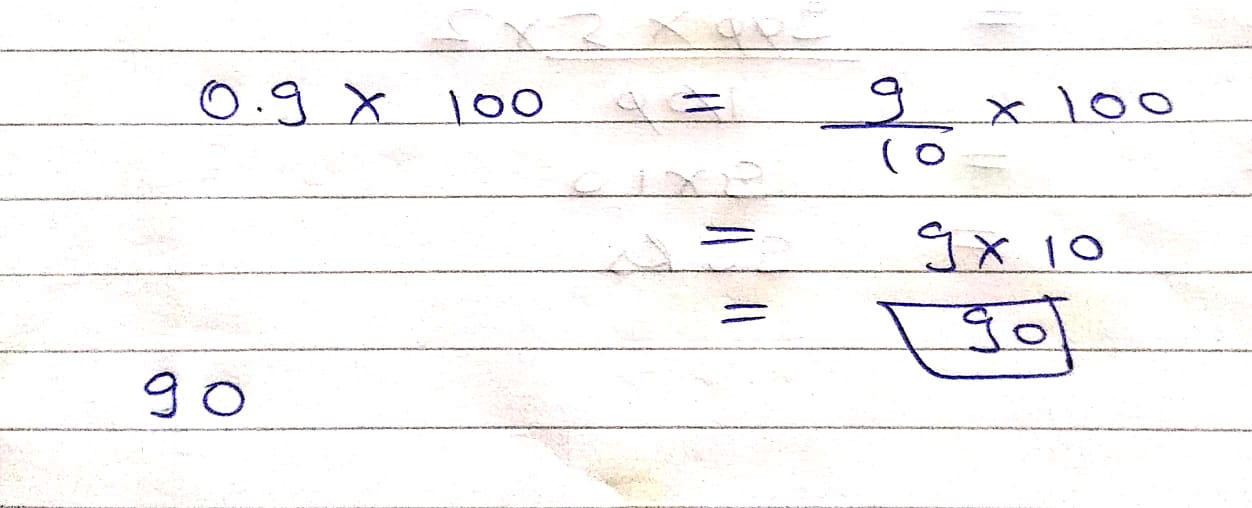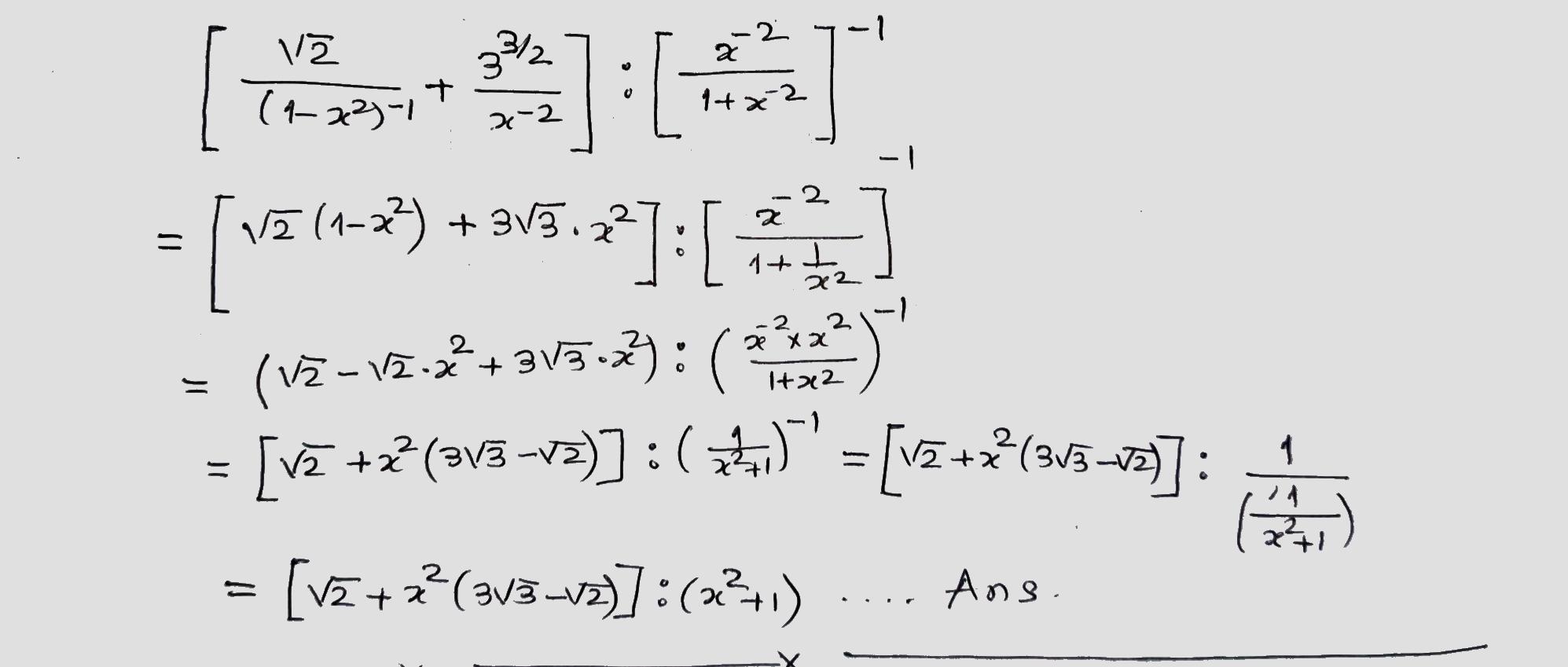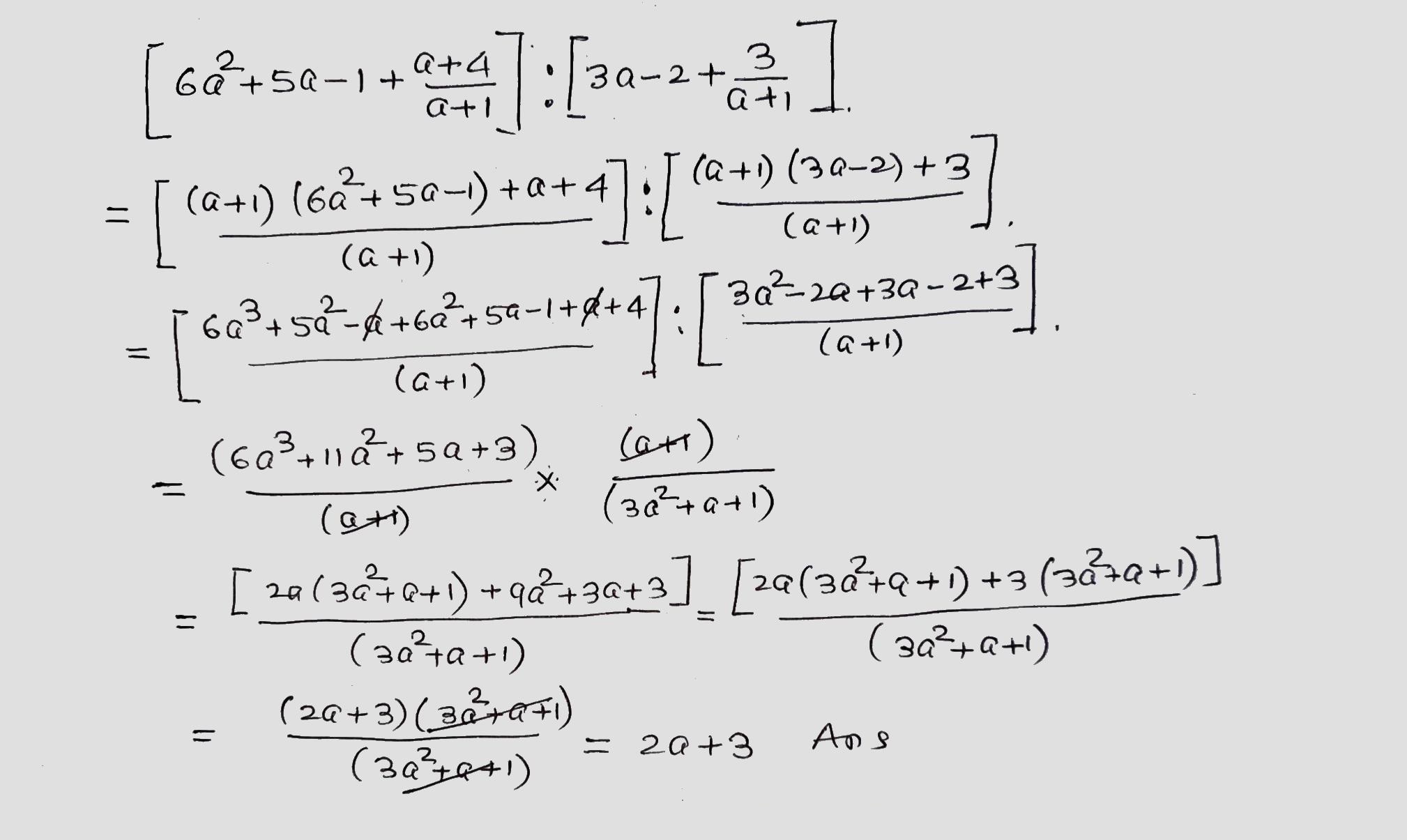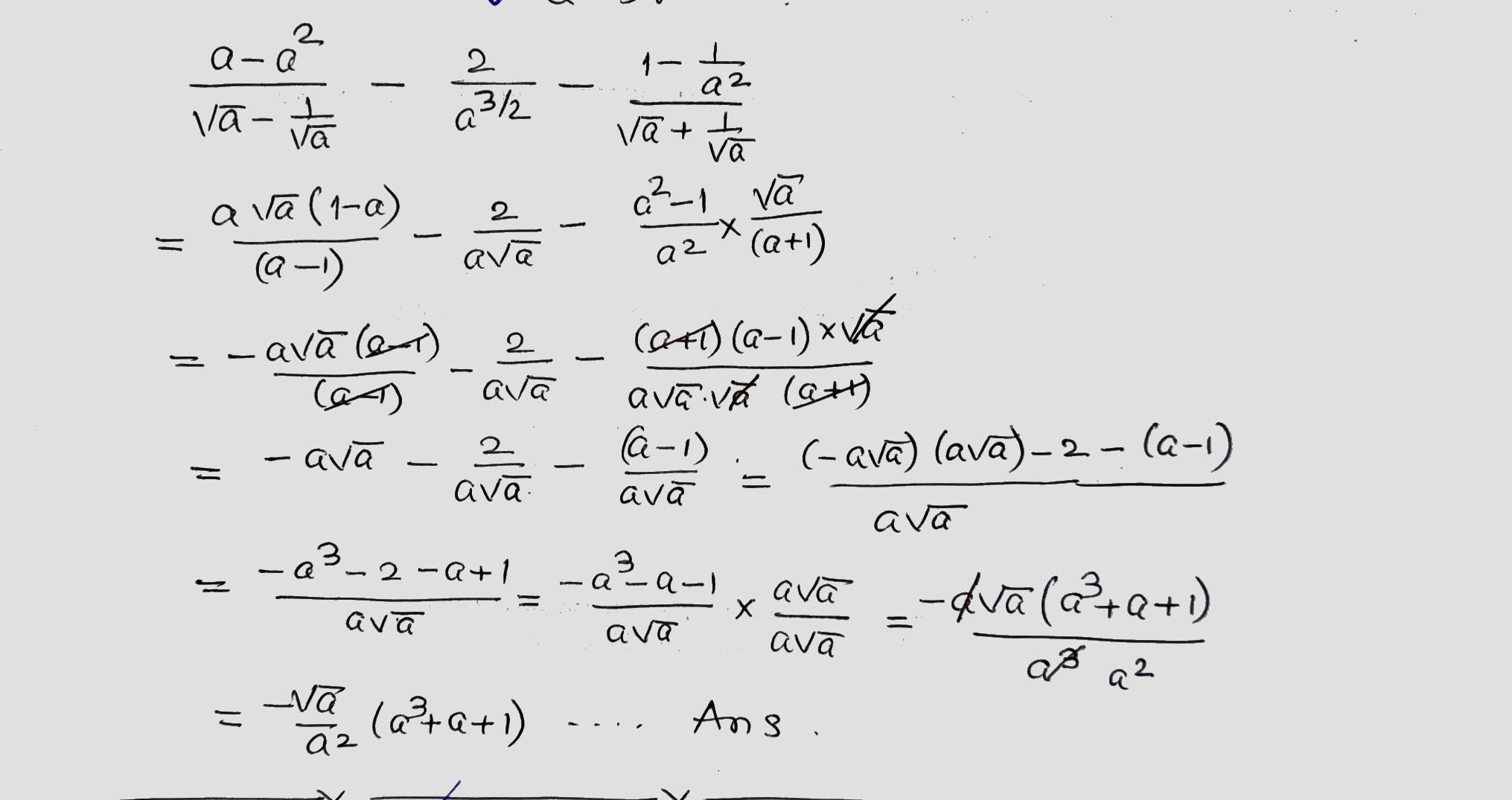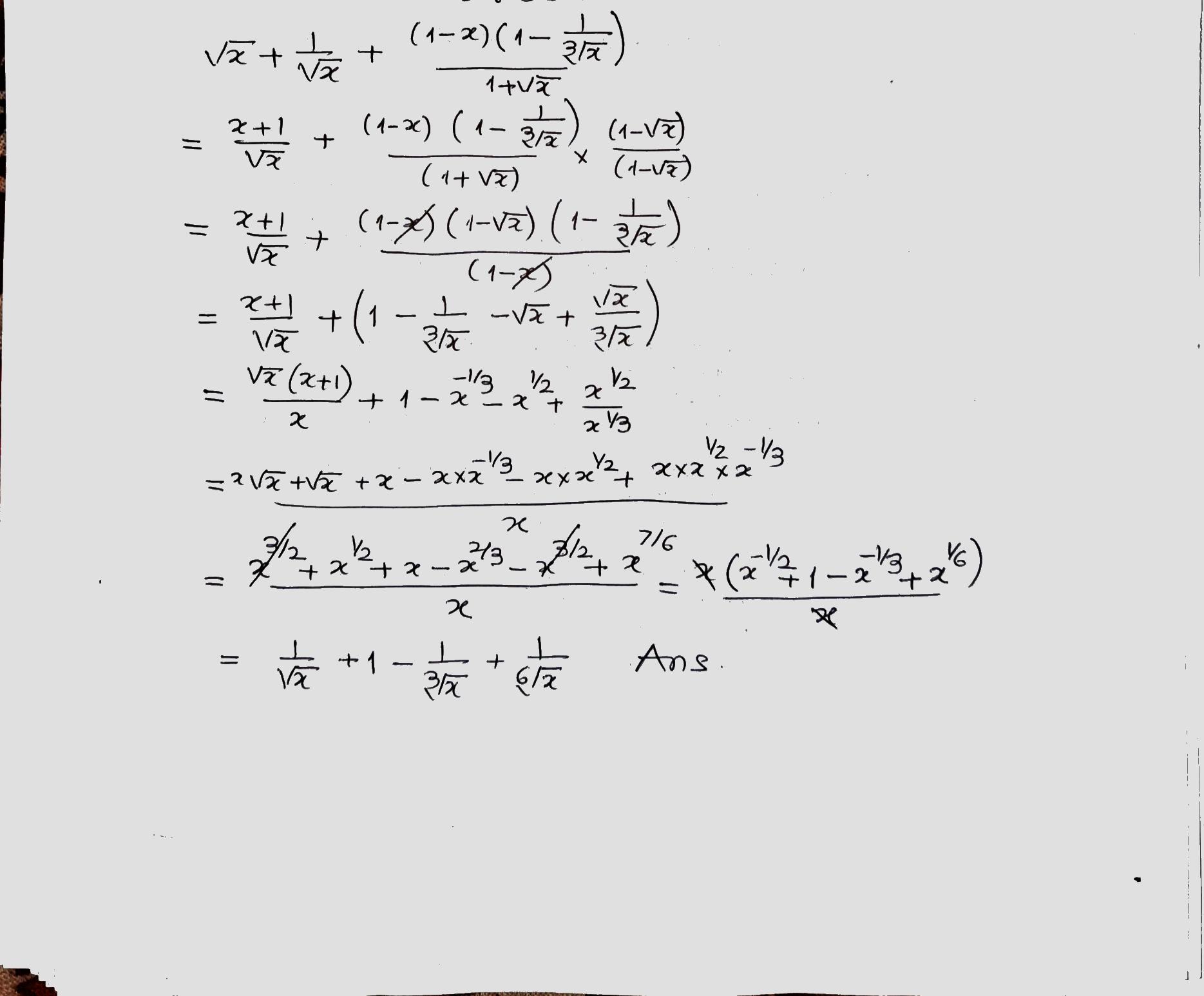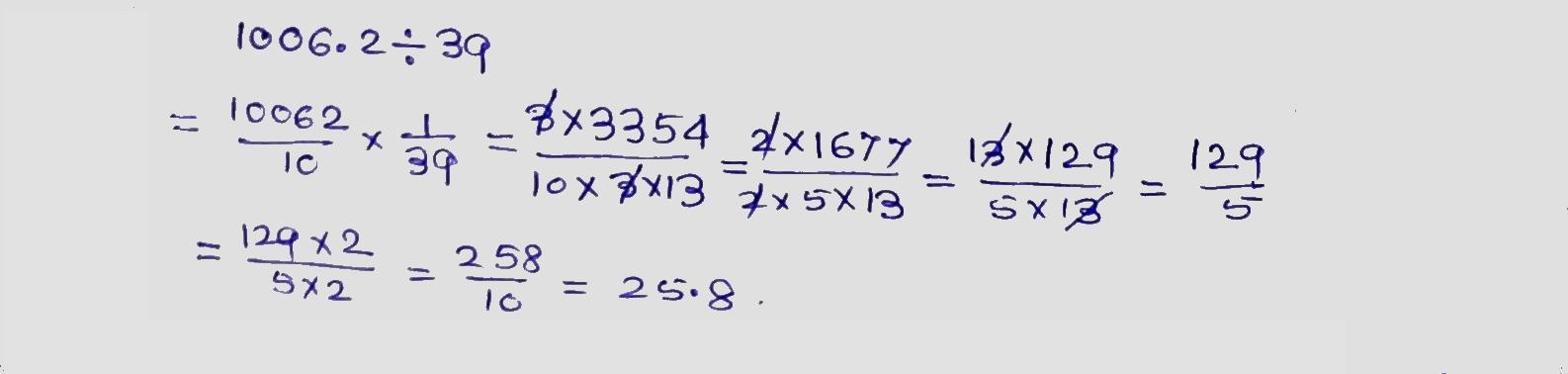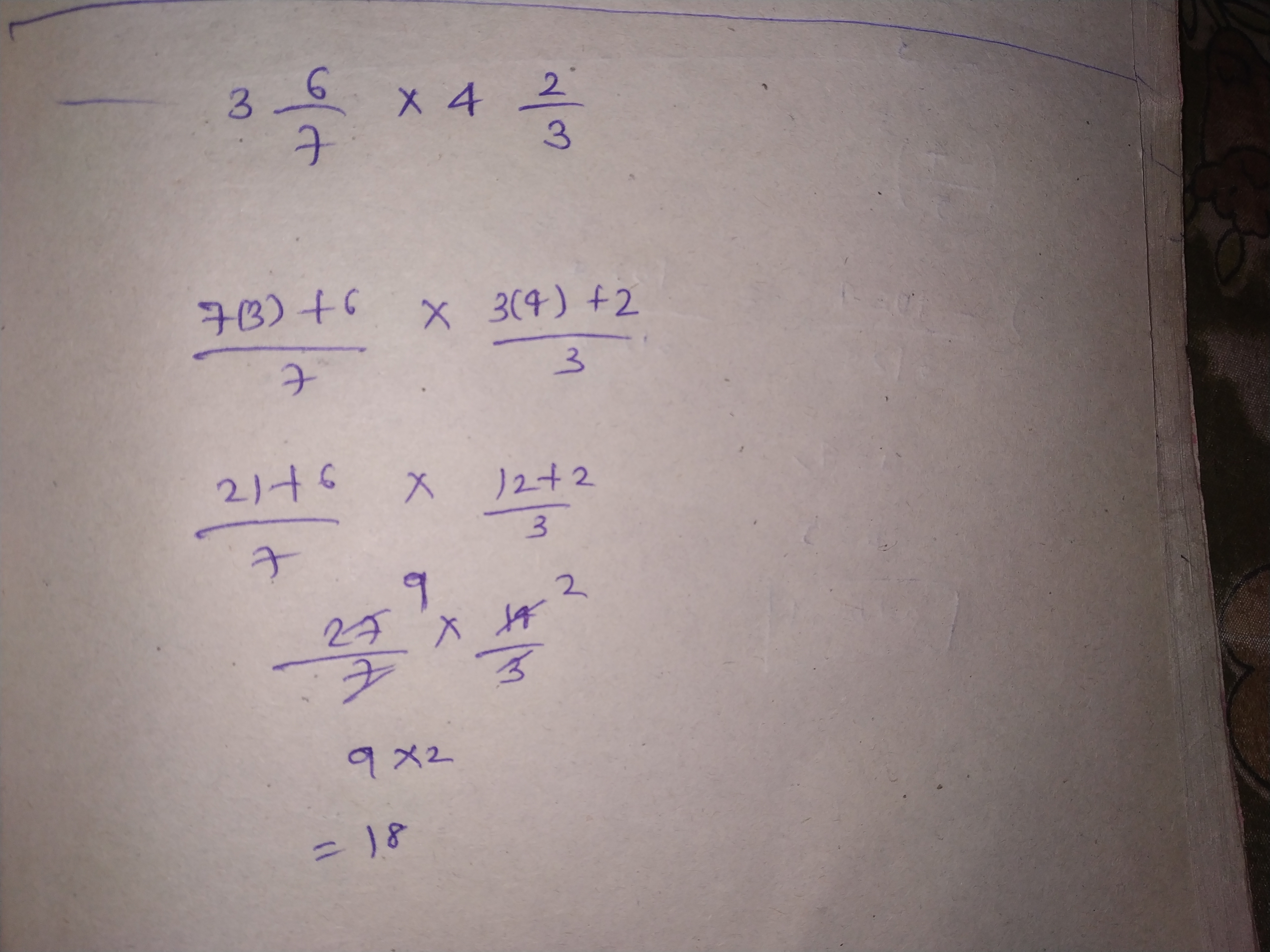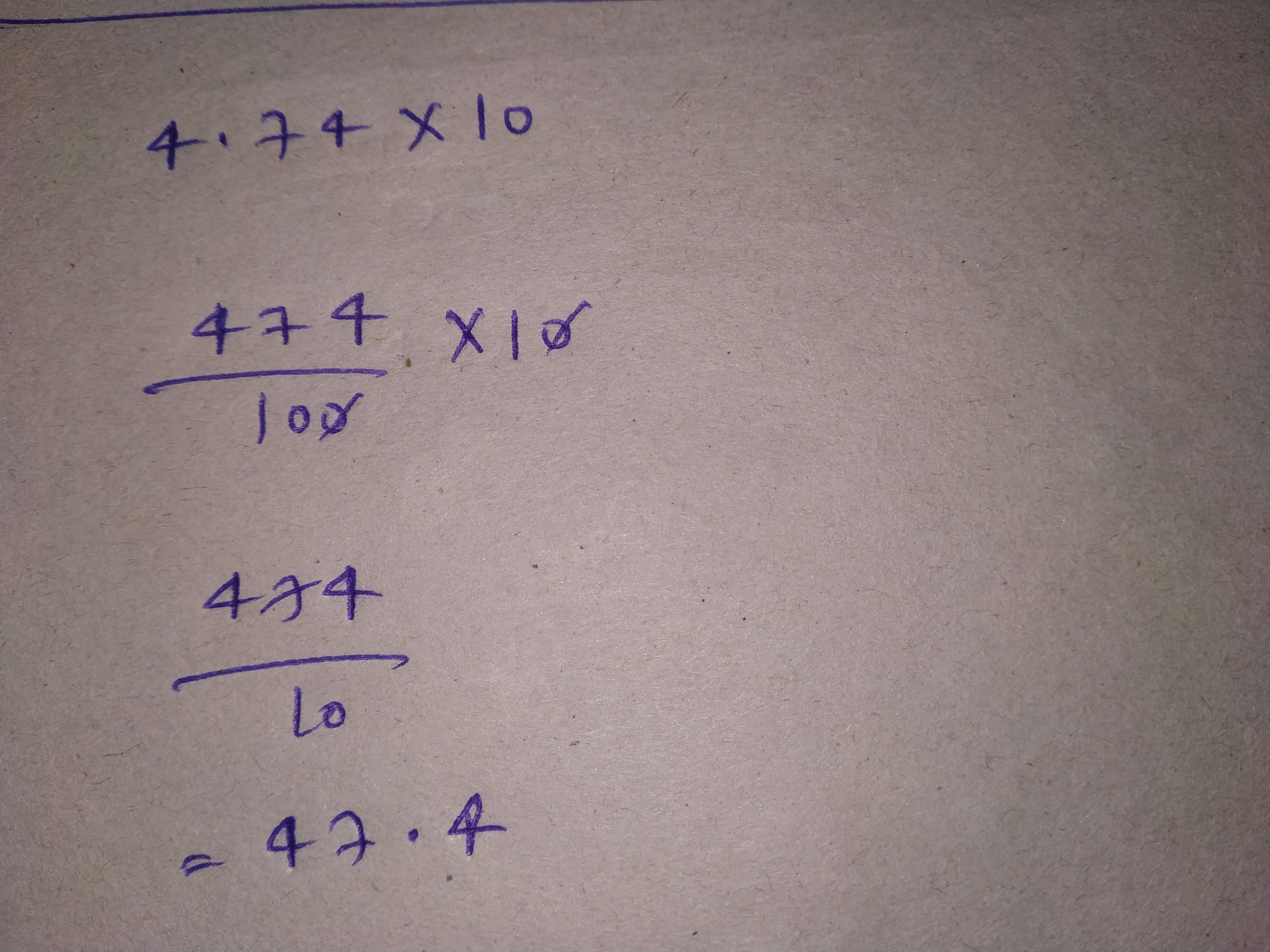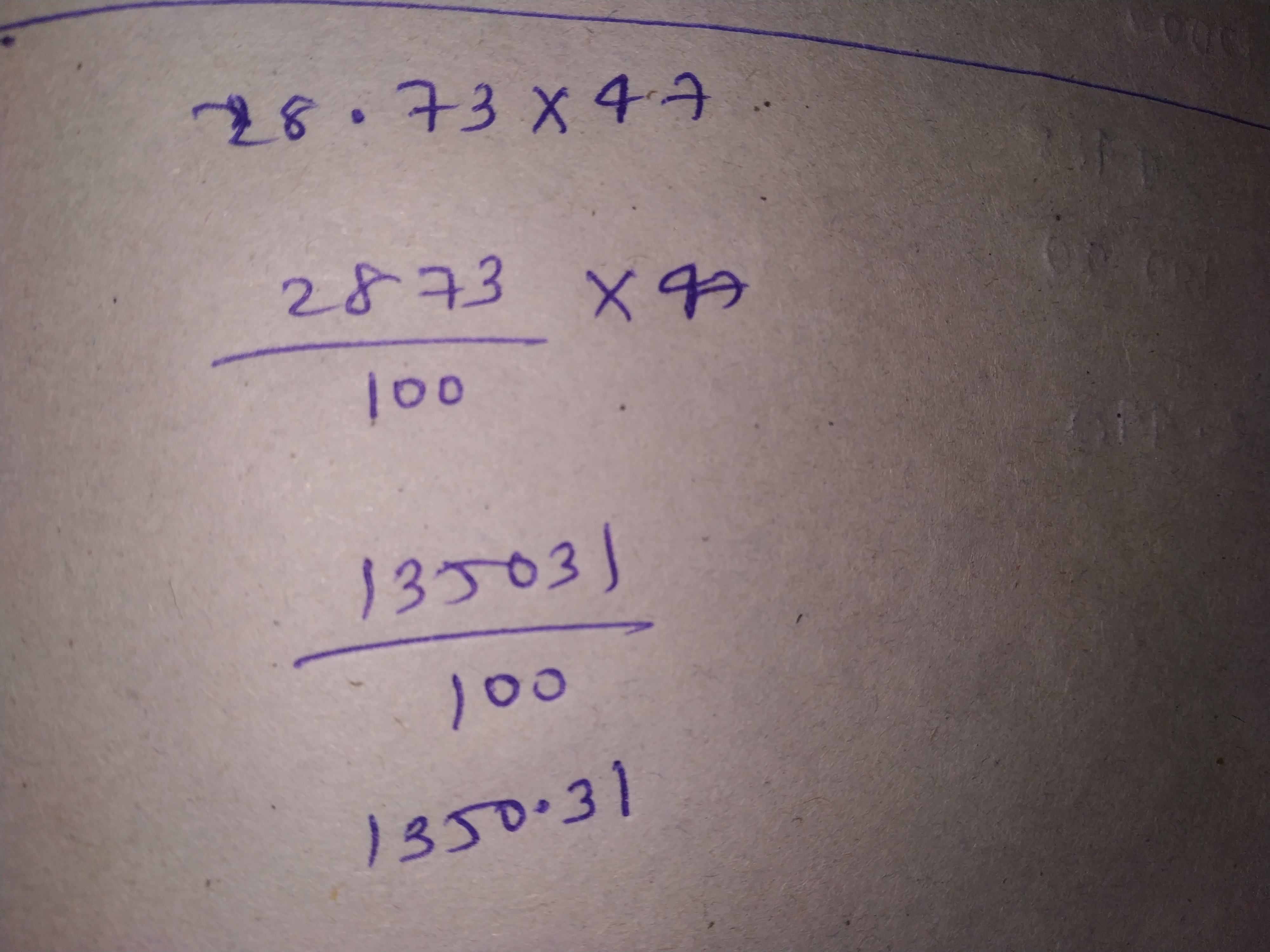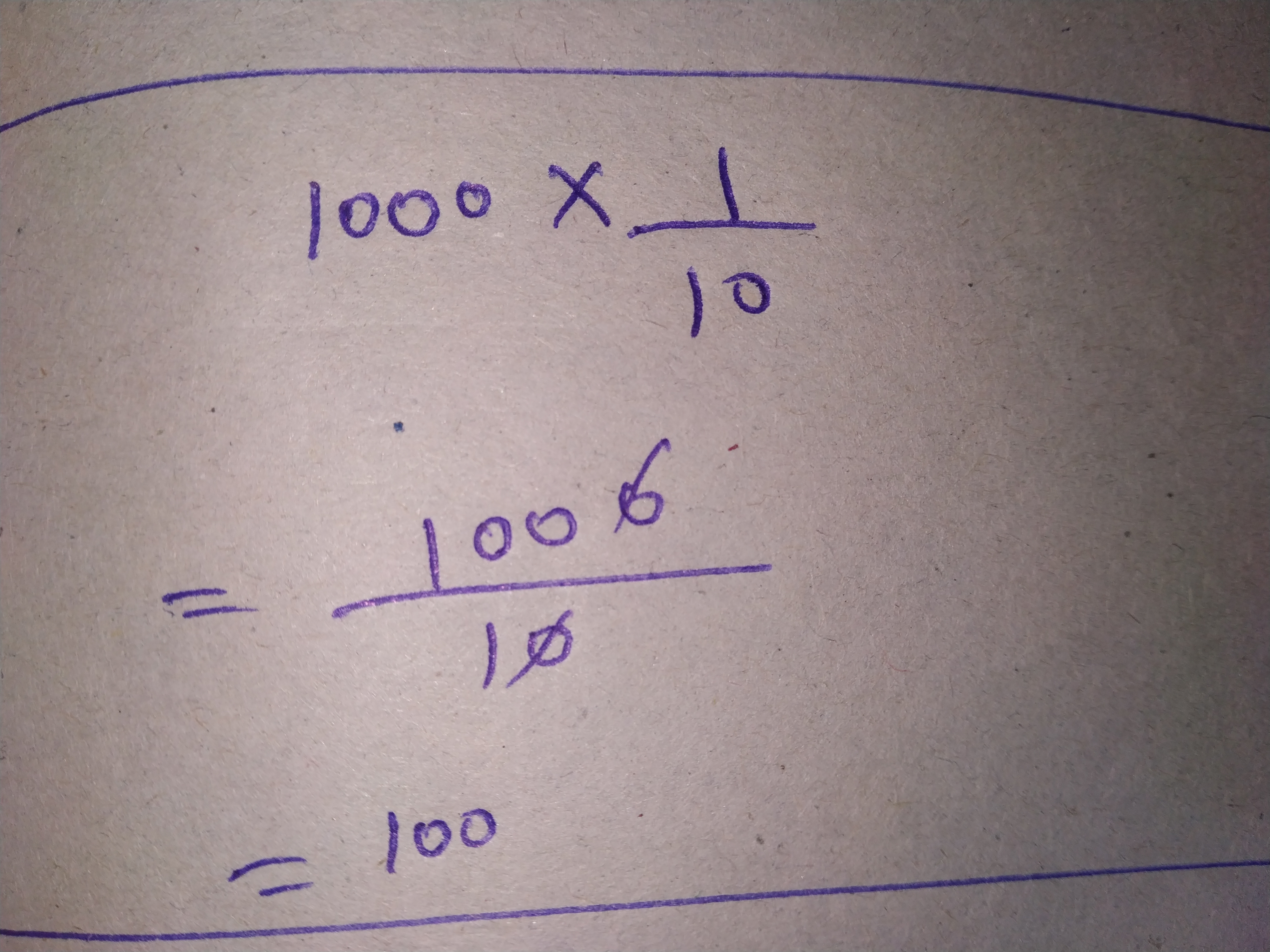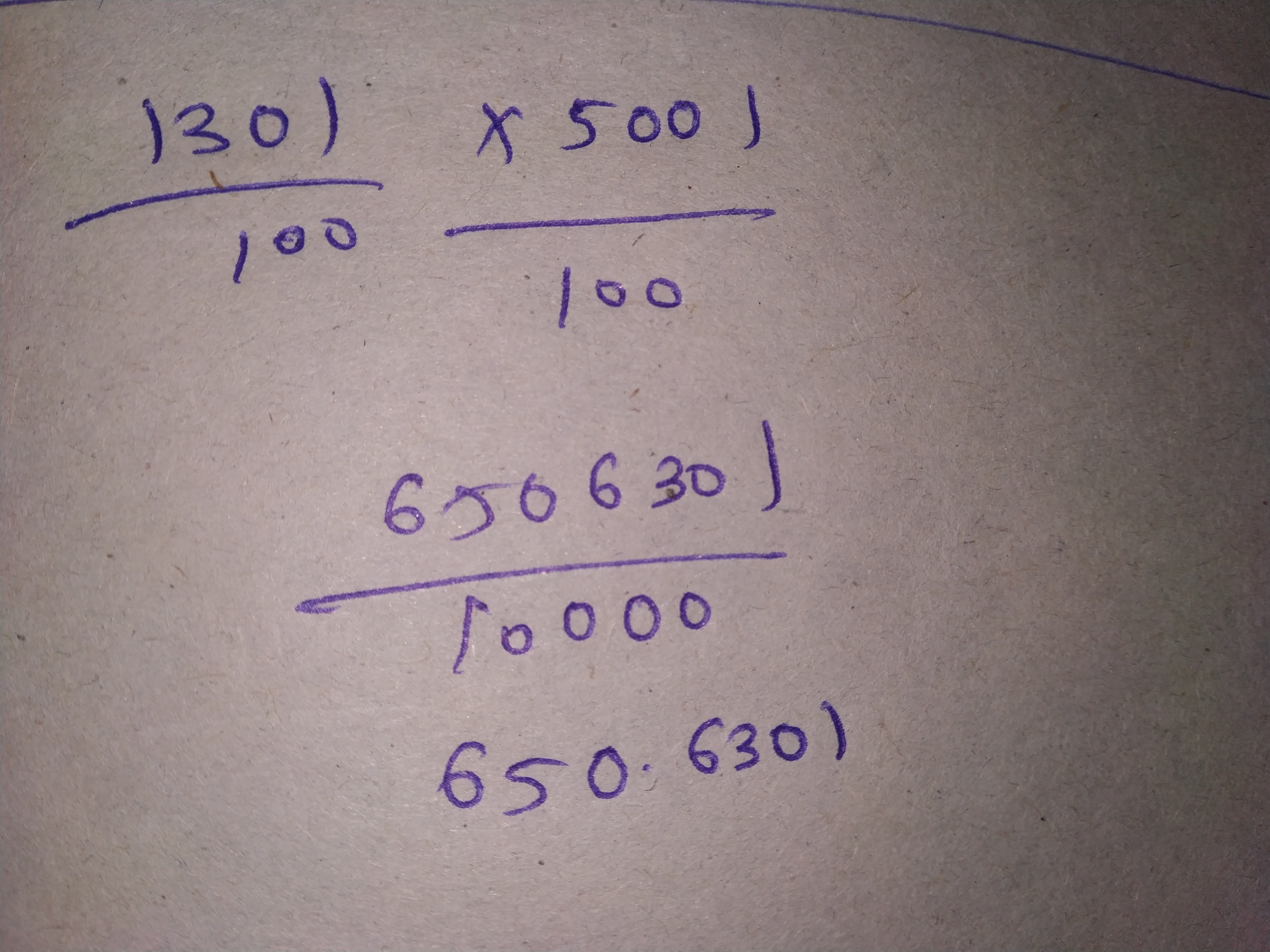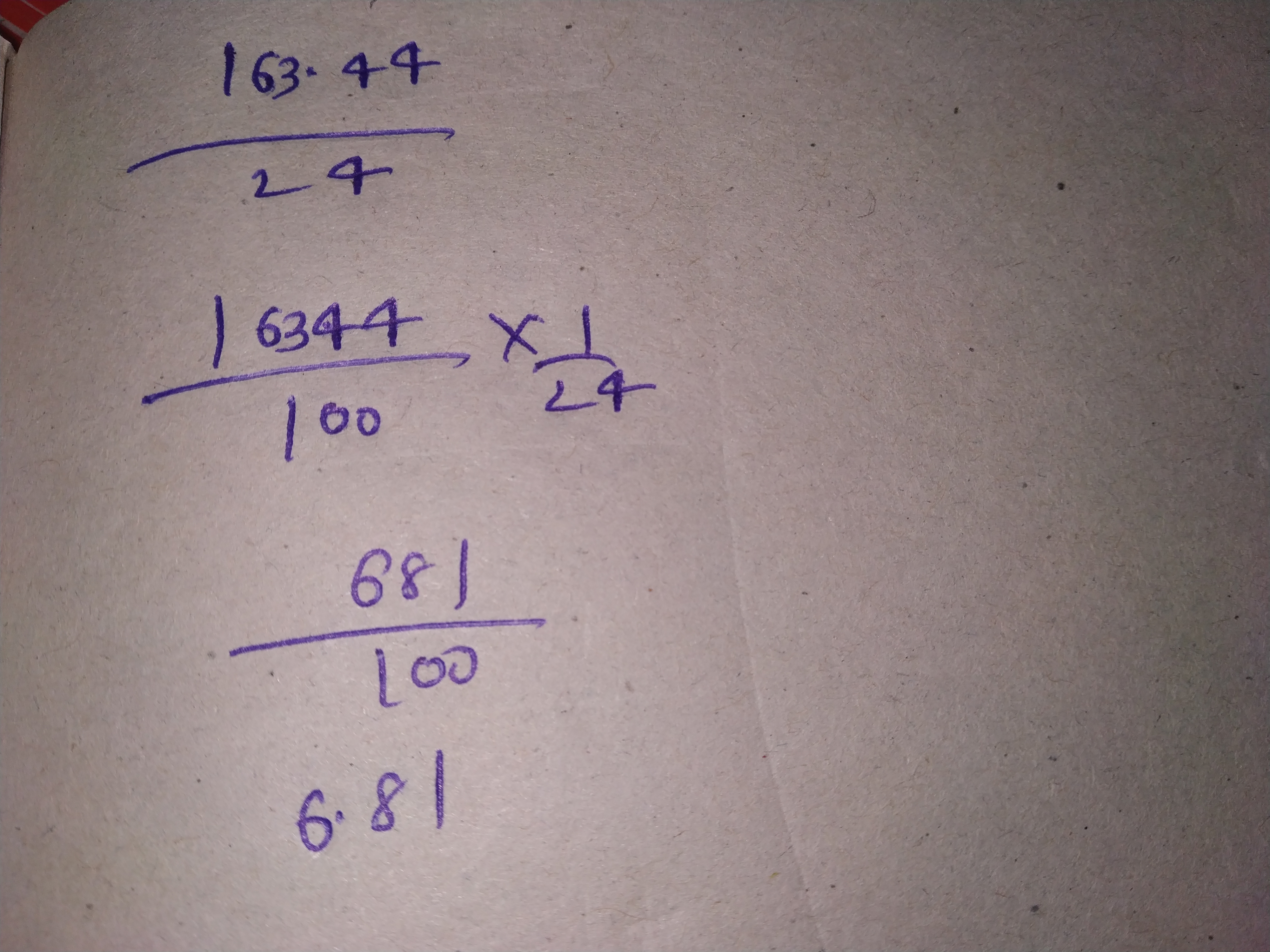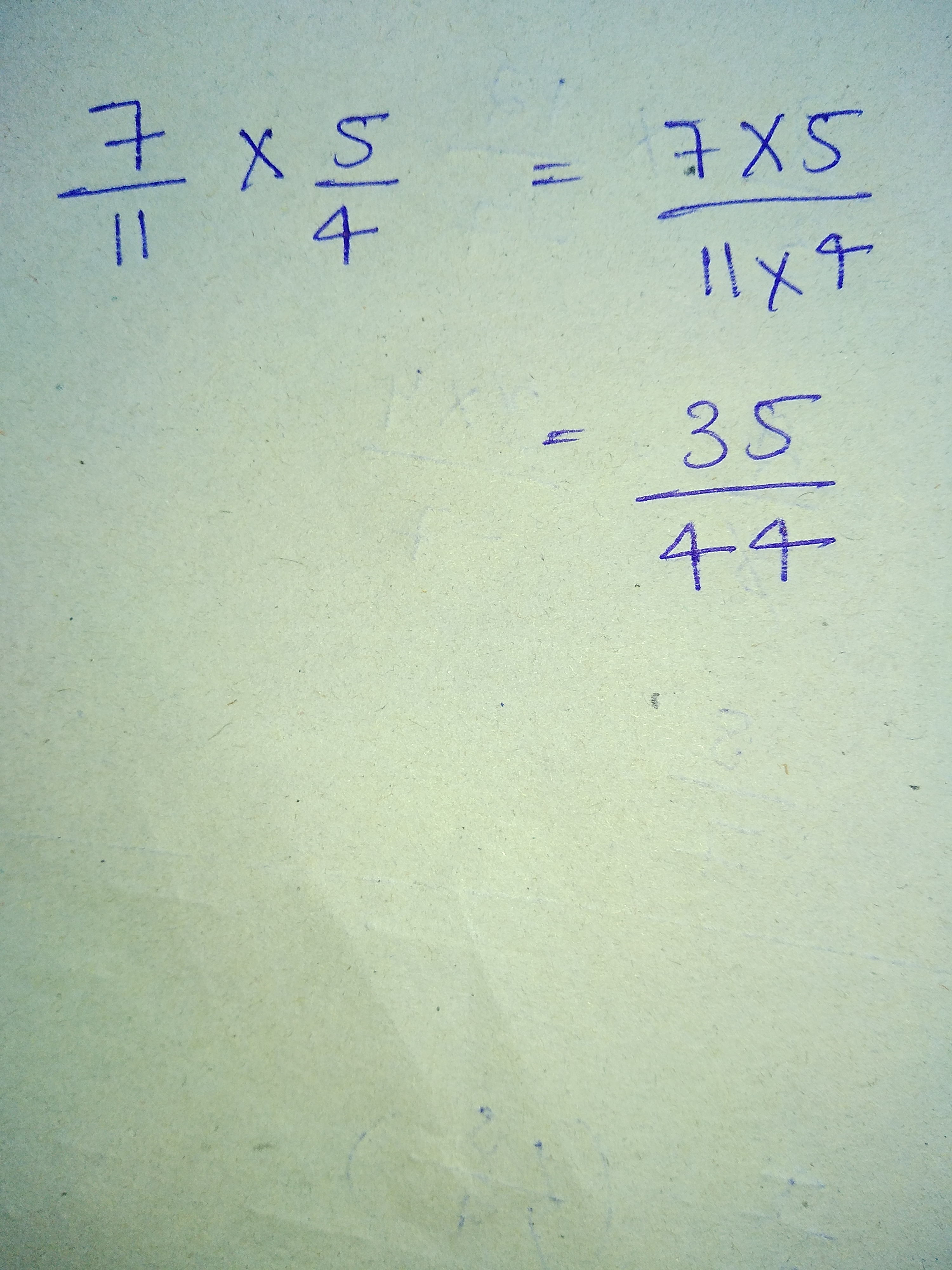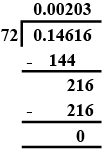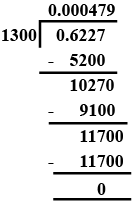Fractions And Decimals - Class 7 Maths - Extra Questions
23.2÷100=x. Find 1000x
Convert the given mixed fractions into improper
215
Convert the given mixed fractions into improper
212
Convert the given mixed fractions into improper
316
Convert the given mixed fractions into improper
729
Convert the given mixed fractions into improper
6110
Convert the given mixed fractions into improper
423
Find 212 of 320 people.
find : 2.5×0.3
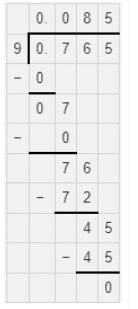
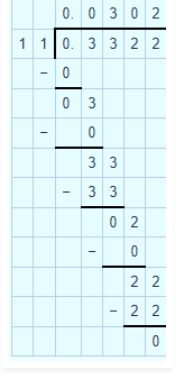
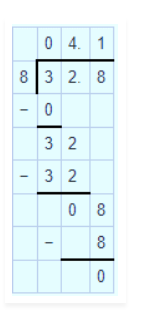
18.368÷14.
Divide each of the given numbers by 10,100,1000 and 10000:2.18.645.010.09060.125111.11
8.64
5.01
0.0906
0.125
111.11
Solve : 0.5×0.05
Solve
(i) 1.5×0.3
Solve: 1.07×0.02
Solve: 4.3×3.4
Solve: 0.1×47.5
Solve:10.05×1.05
Solve : 70.01×1.1
Find:
0.2×6
Find
651.2÷4
Convert the following into a vulgar fraction:
2.¯13
Fill in the blank.
1.1×100=______
Convert the following into a vulgar fraction:
0.ˉ8
Multiply : 0.1×51.7
Simplify:9510
Convert the following into a vulgar fraction:
0.¯34
Fill in the blank.
0.345×1000=______
Fill in the blank.
5.9×1000=_____
Fill in the blank.
10.056×100=______
Fill in the blank.
5.76×10=________
What is the value when 442.36 km is divided by 2.
What is 10 times of 50.30kg.
Convert 877 into mixed fraction.
To multiply a decimal number by 1000, we move
the decimal point in the number to the right by three places.
Represent the following mixed infinite decimal periodic fractions as common fractions:
(a+a1/2b1/2)(a+b)−1(√a(√a−√b)−1−(√a+√b√b)−1)
Convert the following into a vulgar fraction:
0.ˉ6
2.4÷10=
Simplify:
3.24÷0.0016
Divide 0.57 by 10.
Find:
211.9×1.13
Divide 54.25 by 10.
Divide 0.004 by 10.
Divide:
0.45 by 9
Divide 0.48 by 100.
Divide:
40.32 by 9.6
Divide 0.71 by 1000.
Divide 3.8 by 1000.
Divide 74.3 by 100.
Divide 0.7 by 100.
Divide:
217.44 by 9
Divide: 319.2 by 2.28
Divide 29.5 by 1000.
Divide:
31.92 by 228
Divide:
403.2 by 96
Divide:
0.765 by 0.9
Divide:
15.68 by 20
Divide:
0.08by10
Divide:
23.4by100
Divide:
137.2by100
Divide:
32.56by10
Divide:
0.062by10
Express the following improper fractions as mixed fractions:
25/6
Express the following improper fractions as mixed fractions:
18/5
Express the following mixed fractions as improper fractions:
314
Express the following improper fractions as mixed fractions:
22/5
Express the following improper fractions as mixed fractions:
38/5
Multiply each number by (i)10,(ii)100,(iii)1000:
0.112
Multiply each number by 10,100,1000:
0.5
Multiply:
0.568 by 6.4
Express the following mixed fractions as improper fractions:
12711
Multiply each number by (i)10,(ii)100,(iii)1000:
4.8
Multiply:
16.32 by 28
Divide:
324.76 by 1000
Divide:
4.8432 by 0.08
Evaluate :
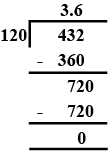
0.125÷25
Multiply each number by (i)10,(ii)100,(iii)1000:
16.27
Evaluate:
5.897×2.3
Multiply each number by (i)10,(ii)100,(iii)1000:
234.8
Evaluate :
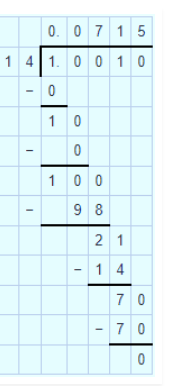
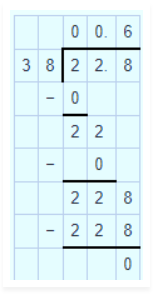
20.64÷16
Divide:
27.918 by 9
Multiply each number by (i)10,(ii)100,(iii)1000:
0.0359
Evaluate :
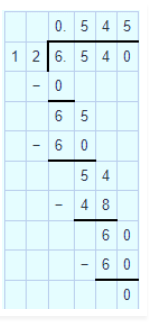
19.25÷25
Evaluate :
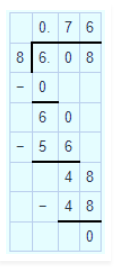
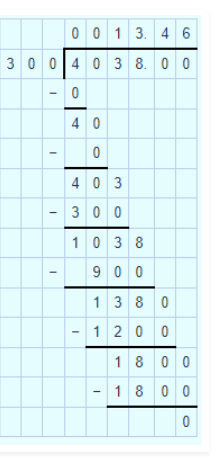
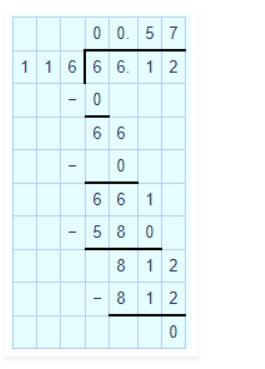
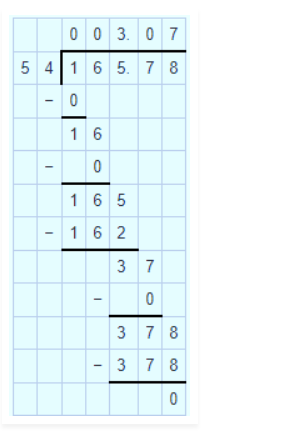
257.894÷0.169
Solve : 1356×1526
Divide the following numbers by 10,100 and 1000 ( orally):
3.45
Evaluate :
9.75÷5
For the celebration class VII bought sweets for Rs.740.25 and cold drink for Rs.70. If 35 students contributed equally what amount was contributed by each student.
Fill in the blank.
32.4×10=______
Fill in the blank.
0.369×10=________
Fill in the blank.
2.35×1000=____
Fill in the blank.
0.25×100=_____
Divide 0.043 by 10.
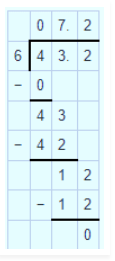
8.892÷19=x. Find 1000x
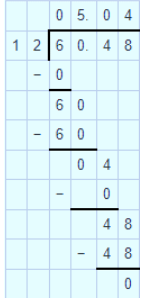
324.4÷100=x. Find 1000x
35.7÷10=x. Find 100x
If 0.24÷0.6=x. Find 10x
Find 1.5÷0.05=
If cost of a pen is Rs.6.25 then the cost of 14 such pens is
Find 3.6÷0.6.
0.2÷10=x. Find 100x
Solve 6.69÷3=x. Find 100x
Solve : 946×723
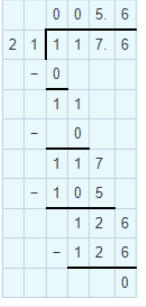
2.214÷18=x. Find 1000x
Express the septenary fraction 15522626 as a denary vulgar fraction in its lowest terms.
Solve the following:
168.07×100
Solve the following:
131.1×100
Solve the following:
53.7×10
Solve the following:
36.8×10
Solve the following:
21.3×10
Solve the following:
0.08×100
Solve the following:
0.03×1000
Solve the following:
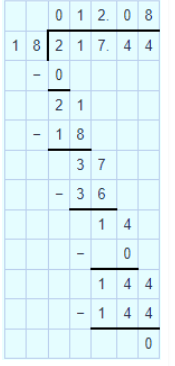
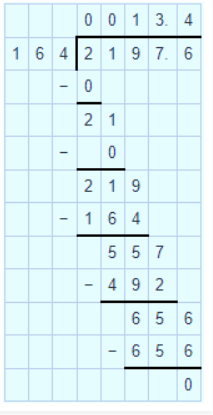
88.5÷0.15
615×518= x9. Find x.
Solve 5012×4912.
910×59×1525= x10. Find x.
Calculate 4.203×15000.
Calculate 0.035×1200.
Evaluate 2.25×4.
3.4×10= ________.
2.97×100= _______.
3.4×1000= _______.
Calculate 3.5×24.
0.46×100= _________.
0.5×10= ____________.
Calculate 6.54×50.
0.25×1000= __________.
Solve 2.1×100= ______.
Calculate 7.604×0.
14.63÷11=x. Find 100x
13.92÷12=x. Find 100x
48.3÷14=x. Find 100x
45.645÷15=x. Find 1000x
39.39÷13=x. Find 100x
Solve 2.24×100= ______.
24.6÷100=x. Find 1000x
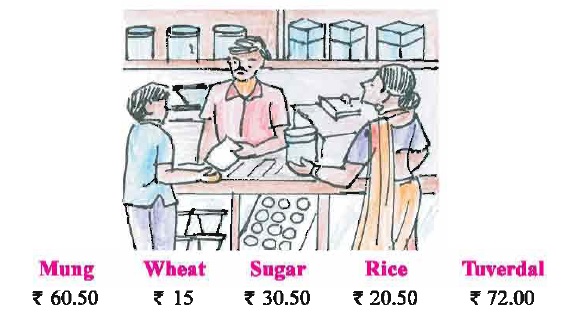
Hitesh and Hiral goes to market to buy some items for their home. Rate of items are in kg. Answer the following question on its base. How much is the price of 4 kg Rice in rupees?
620.5÷10=x. Find 100x
Represent the following mixed infinite decimal periodic fractions as common fractions:
x1/2+1x+x1/2+1:1x1/2−1
Divide 3.96 by 4
Convert each of the following into mixed fraction :
(1)175 (2)627 (3)1018
(4)9513 (5)8111 (6)8716
(7)11312 (8)11720
Simplify 712×313
Evaluate 9111×10112
Find the value of :
(i) (82)2−(18)2
(ii) (128)2−(72)2
(iii) 197×203
(iv) 198×198−102×10296
(v) (14.5×15.3)
(vi) (8.63)2−(1.37)2
Solve 45.30÷15
Multiply: 45×614
Find
(i) 0.4÷2
(ii) 0.35÷5
(iii) 2.48÷4
(iv) 65.4÷6
(v) 651.2÷4
(vi) 14.49÷7
(vii) 3.96÷4
(viii) 0.80÷5
Find
(i) 7.9÷1000
(ii) 128.9÷10
Work out the following division.
a. 0.6÷2
b. 0.625÷5
c. 17.28÷9
d. 7.2÷4
Rishi bought six school bags for Rs. 712.02. What is the cost of each bag?
solve:
(i)614÷113
(ii) 12÷134
(iii)13×213
Multiply:
2.57 by 1.8
Find the product of 1215 & 1410
0.0364
Find the value of
3.852÷1.8
Multiply:
1.5 by 0.9
412:118
Solve: 42.435×10=?
Convert 4117 into improper fraction:
Solve:435×237=235×177=?
Find the product :
3.762×10
6.4÷0.4
Simplify: 516−314+313+4
Solve:12.87×4.2
A student in a school pays 315 as a fund. If the amount collected is 40625, find the number of students in the school.
Divide : 312÷314
Simplify
227÷1411×249
If in mixed fraction form 196 is equal to 3x6, then what is the value of x ?
Find the product :
19.638×10
Divide the following
2.8÷0.7
Solve 78+512×910−14.
Write these numbers in order, starting with the smallest.
0.55 611 5412%
_______ < _______ < _______
Find :
211.02×4
Find:
20.1×4
Find :
1.3×10
Find :
0.05×5
Find:
153.7×10
Find :
2.71×5
Find :
2×0.86
Find :
11.2×0.15.
Find :
1.3×3.1.
Find :
168.07×10.
Find :
0.2×316.8.
Find :
0.5×10.
Find :
156.1×100.
Find :
31.3×100.
Find :
0.08×10
Divide:
43.2by6
Divide:
60.48by12
Divide:
117.6by21
Divide:
2.575by25
Divide:
0.765by9
Find the product: 58 × 47
Divide:
6.08by8
Divide:
217.44by18
Evaluate the following:
12.08×9.3
Evaluate the following:
238.06×7.5
Evaluate the following:
3.7×4.5
Work out the following:
72.8÷0.04
Work out the following:
8.64÷3.6
Multiply the following number by 10,100 and 1000 orally:
.234
Work out the following:
0.144÷0.02
Multiply the following number by 10,100 and 1000 orally:
3.45
Work out the following:
70.756÷4
Work out the following:
2.46÷6
Evaluate the following:
0.79×32.4
Work out the following:
3.016÷8
Convert the following mixed number into improper fractions :
(i)7211
(ii)3548
(iii)13764
(iv)723
Divide the following numbers by 10,100 and 1000 ( orally):
23.01
Evaluate the following :
(i)25×37
(ii)35×89
(iii)7×123
Divide the following numbers by 10,100 and 1000 ( orally):
4.7
Multiply:
0.943 and 62
If length of one bed-cover is 2.1m, find the total length of 17 bed-covers.
Multiply:
5.6 and 8
Multiply:
38.46 and 9
Multiply:
0.0453 and 35
Multiply:
7.5 and 2.5
Multiply:
0.87 by 10
Multiply:
6.4 by 1000
Multiply:
2.948 by 100
Multiply:
5.8 by 4
Divide:
12.8 by 4
Divide:
7.8 by 100
Evaluate:
0.01×0.001
Divide:
4.32 by 1.2
Divide:
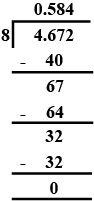
4.672 by 8
Divide:
54.9 by 10
Multiply:
5.037 by 8
Multiply:
4.6 by 2.1
Evaluate:
0.894×87
Evaluate :
3.204÷9
Evaluate :
27.69÷30
Evaluate :
4.4064÷4
Divide:
7.644 by 1.4
If we multiply the fractions 12 and 13 we will get 16 .If true enter 1 else 0.
Solve the following:
0.5×10
Solve the following:
3.62×100
A motor bike covers a distance of 62.5km consuming one litre of petrol. How much distance does it cover for 10 litres of petrol? (In km)
Solve the following:
0.9×100
Represent the following mixed infinite decimal periodic fractions as common fractions:
(√2(1−x2)−1+33/2x−2):(x−21+x−2)−1
Represent the following mixed infinite decimal periodic fractions as common fractions:
0.23(7)+434500.5(61)−113495
Represent the following mixed infinite decimal periodic fractions as common fractions:
(1a+1b−2cab)(a+b+2c):(1a2+1b2+2ab−4c2a2b2)
Represent the following mixed infinite decimal periodic fractions as common fractions:
\displaystyle\left ( 6a^2 + 5a - 1 + \frac{a + 4}{a + 1} \right ) : \left ( 3a - 2 + \frac{3}{a + 1} \right )
Remove the irrationality in the denominator.
\displaystyle\frac{1}{1 + \sqrt{2} + \sqrt{3}}
Represent the following mixed infinite decimal periodic fractions as common fractions:
\displaystyle( \sqrt{\sqrt{3} + \sqrt{2}} - (\sqrt{3} - \sqrt{2})^{1/2}) ((\sqrt{3} + \sqrt{2})^{1/2} + \sqrt{\sqrt{3} - \sqrt{2}})^{-1} - \cos \frac{5\pi }{4}
Represent the following mixed infinite decimal periodic fractions as common fractions:
\displaystyle\frac{x^{1/2} + x^{-1/2}}{1 - x} + \frac{1 - x^{-1/2}}{1 + \sqrt{x}}
Represent the following mixed infinite decimal periodic fractions as common fractions:
\displaystyle\, \frac{2 \sqrt{b}}{\sqrt{a} + \sqrt{b}} + \left ( \frac{a^{3/2} + b^{3/2}}{\sqrt{a} + \sqrt{b}} - \frac{1}{(ab)^{-1/2}} \right )(a - b)^{-1}
Represent the following mixed infinite decimal periodic fractions as common fractions:
\displaystyle\, \frac{a - a^2}{a^{1/2} - a^{-1/2}} - \frac{2}{a^{3/2}} - \frac{1 - a^{-2}}{a^{1/2} + a^{-1/2}}
Represent the following mixed infinite decimal periodic fractions as common fractions:
\displaystyle\frac{a \sqrt{a} + b \sqrt{b}}{(\sqrt{a} + \sqrt{b})(a - b)} + \frac{2\sqrt{b}}{\sqrt{a} + \sqrt{b}} - \frac{\sqrt{ab}}{a -b}
Represent the following mixed infinite decimal periodic fractions as common fractions:
\displaystyle\, x^{1/2} + x^{-1/2} + \frac{(1 - x)(1 - x^{-1/3})}{1 + \sqrt{x}}
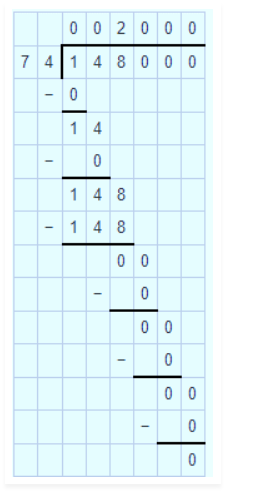
1006.2 \div 39
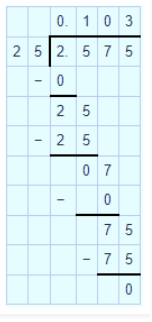
Express 32.12\bar{35} in the form of \dfrac{p}{q} where p and q are integers.
Convert each of the following into mixed fraction :
(1)\frac{17}{5} (2)\frac{62}{7} (3)\frac{101}{8} (4)\frac{95}{13}
(5)\frac{81}{11} (6)\frac{87}{16} (7)\frac{113}{12} (8)\frac{117}{20}
Find 0.1\times 71.3
In order to make a shirt 2\,m\,\,\,25\,cm cloth is needed. What length of cloth is required to make 18 such shirt ?
Find: 100.01\times 0.01
Find 101.01 \times 0.01
Find 0.2\times 312.4
5.04 \div 0.8=?
Find 3.3\times 0.3
In mixed fraction form \dfrac {35}{9} is equal to 3\dfrac {y}{9}.
What is the value of y ?
Solve:
84.9 \div 30
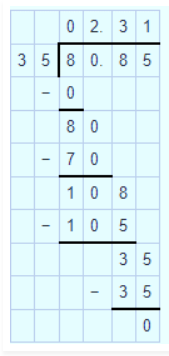
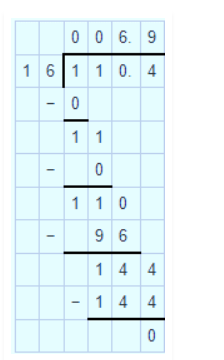
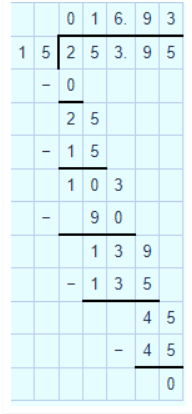
14.9\div 0.64.
Find :
1) \dfrac{2}{5} \times \dfrac{1}{8}
ii) \dfrac{1}{5} \times \dfrac{3}{4}
iii) \dfrac{5}{8} \times \dfrac{8}{15}
2\dfrac {1}{2}:3\dfrac {1}{3} > 3.5:4.5
State true or false.
Draw representations to multiply :
(a) 3 and 2.6
(b) 4 and 1.3
Multiply of the following numbers by 10,\ 100 and 1000 :
(i) 3.9
(ii) 2.89
(iii) 0.0829
(iv) 40.3
(v) 0.3725
Express the following as improper fractions:
10\dfrac {3}{5}
The following fractions represent just three different numbers. Separate them in to three groups of equal fractions by changing each one to its simplest form:
\dfrac {15}{75}
Change the following fraction into its simplest form:
\dfrac {4}{25}
Multiply :
3\dfrac{1}{8} \times 4\dfrac{10}{11}
Find the product:
3\dfrac{6}{7}\times 4\dfrac{2}{3}
Find the product:
7\dfrac{1}{2}\times 2\dfrac{4}{15}
Find the product:
4.74\times 10
Find the product:
42.5 \times 100
Find the product:
0.0054 \times 10
Find the product:
0.45 \times 10
Find the product:
35.853 \times 100
Find the product:
12.075 \times 100
Find the product:
0.0529 \times 1000
Find the product:
28.73 \times 47
Find the product:
20.708 \times 1000
Find the product:
100 \times 0.005
Find the product:
1000 \times 0.1
Find:
13.01 \times 50.01
Divide 142.45 by 10.
Find the product:
0.0415 \times 59
Divide 3.45 by 10.
Find the area of a square plot of land whose each side measures 8\dfrac {1}{2} meters.
Divide:
16.64 by 20
Divide:
164.6 by 200
Divide:
403.80 by 30
Divide:
163.44 by 24
Multiply:\dfrac{7}{11} by \dfrac{5}{4}
Find the product :
7.54 \times 10
Find the product :
2.397 \times 100
Find the product :
0.08 \times 100
Find the product :
6.83 \times 100
Find the product :
0.7 \times 10
Find the product :
0.83 \times 10
Find the product :
73.92 \times 10
Find the product :
84.003 \times 10
Find the product :
0.032 \times 10
Find the product :
0.076 \times 1000
Find the product :
6.25 \times 1000
Find the product :
0.03 \times 100
Find the product:
4.8 \times 1000
Find the product :
5.4 \times 1.6
Find the product :
0.182 \times 1000
Find the product :
0.06 \times 1000
Find the product :
0.6 \times 100
Find the product :
6.7314 \times 1000
Find the product :
3.65 \times 19
Find the product :
7.6 \times 2.4
Find the product :
0.54 \times 0.27
Find the product :
6.032 \times 124
Find the product :
0.0146 \times 69
Find the product :
3.45 \times 6.3
Find the product :
0.854 \times 12
Find the product :
0.00125 \times 327
Find the product :
4.125 \times 86
Find the product :
36.73 \times 48
Find the product :
104.06 \times 75
Find the product :
0.623 \times 0.75
Find the product :
0.06 \times 0.38
Divide:
4.7 \quad by \quad 100
Find the product :
54.5 \times 1.76
Find the product :
1.245 \times 6.4
Find the product :
0.014 \times 0.46
Find the product :
0.568 \times 4.9
Find the product :
6.54 \times 0.09
Find the product :
3.87 \times 1.25
Find the product :
0.045 \times 2.4
Divide:
131.6 \quad by \quad 10
Divide:
0.3 \quad by \quad 100
Divide:
0.34 \quad by \quad 10
Divide:
4.38 \quad by \quad 10
Divide:
0.58 \quad by \quad 100
Multiply:
\cfrac98 by \cfrac{32}3
Multiply:
\cfrac34 by \cfrac57
Divide:
4.6 \quad by \quad 1000
Divide:
354.16 \quad by \quad 1000
Divide:
38.9 \quad by \quad 1000
Divide:
1286.5 \quad by \quad 1000
Simplify: =\dfrac{3}{20}\times \dfrac{4}{5}
Divide:
0.8 \quad by \quad 1000
Divide:
0.02 \quad by \quad 100
Divide:
0.768 \quad by \quad 16
Divide:
0.477 \quad by \quad 18
Divide:
0.3322 \quad by \quad 11
Divide:
403.8 \div 30
Divide:
6.54 \hspace{2mm} by \hspace{2mm}12
Divide:
1.001 \quad by \quad 14
Divide:
16.46 \div 20
Divide:
0.175 \quad by \quad 25
Divide:
5.52 \quad by \quad 16
Divide:
2.13 by 15
Find the product:
\left(3\cfrac{6}{7}\right) \times\left(4\cfrac{2}{3}\right)
Divide:
18.08 \div 400
Find the product:
\left(4\cfrac{1}{8} \right)\times\left(2\cfrac{10}{11}\right)
Divide:
3.28 \div 0.8
Find the product:
\left(9\cfrac{1}{2}\right) \times\left(1\cfrac{9}{19}\right)
Find the product:
\left(5\cfrac{5}{6}\right)\times\left(1\cfrac{5}{7}\right)
Divide:
156.8 \div 200
Divide:
12.8 \div 500
Divide:
19.2 \div 80
Divide:
0.8085 \quad by \quad 0.35
Divide:
148 \quad by \quad 0.074
Divide:
6.612 \quad by \quad 11.6
Divide:
21.976 \quad by \quad 1.64
Divide:
0.288\ by\ 0.9
Divide:
11.04 \quad by \quad 1.6
Divide:
0.076 \quad by \quad 0.19
Divide:
16.578 \quad by \quad 5.4
Divide:
25.395 by 1.5
Divide:
0.228 by 0.38
The total cost of 24 chairs is 9255.60. Find the cost of each chair.
8 \frac{2}{7} Is equal to the improper fraction_____.
Write \dfrac{129}{8} as a mixed fraction.
Grip size of a tennis racquet is 11 \dfrac{9}{80} cm . Express the size as an improper fraction.
Convert the following fractions into mixed fractions:
(i) \dfrac{73}{8}
(ii) \dfrac{94}{13}
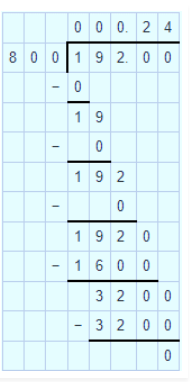
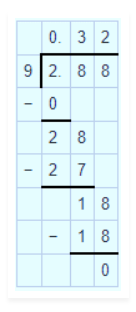
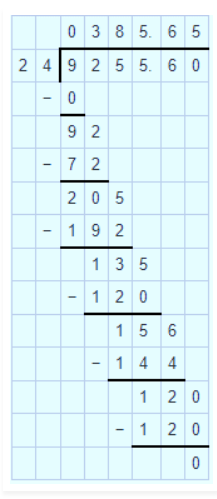
When 0.004 is divided by 0.02964, what will be the quotient?
Convert the following fractions into improper fractions :
(i) 2 \dfrac{7}{9}
(ii) 5 \dfrac{4}{11}
Find the following:
(i) 10.8 \div 4
(ii) 126.35 \div 7
(iii) 22.5 \div 1.5
(iv) 4.28 \div 0.02
(v) 3.645 \div 1.35
(vi) 0.728 \div 0.04
(vii) 13.06 \div 0.08
(viii) 58.635 \div 4.5
Multiply each of the following numbers by 10, 100 and 1000 (orally):
(i) 5.9
(ii) 3.76
(iii) 0.549
Find the following:
(i) 2.7 \times 4
(ii) 2.71 \times 5
(iii) 2.5 \times 0.3
(iv) 2.3 \times 4.35
(v) 238.06 \times 7.5
(vi) 0.79 \times 32.4
(vii) 1.07 \times 0.02
(viii) 10.05 \times 1.05
What is the cost of 27.5 m of cloth at 53.50 per metre?
What will be the total length of cloth required to make 5 shirts, if 2.15 m of cloth is needed for each shirt?
The cost of a fountain pen is Rs 13.25. Find the cost of 8 such pens.
Find the area of a rectangle whose length is 5.7 \ cm and breadth is 3.5 \ cm.
If Rs. 242.46 are to be distributed among 6 children equally, find the share of each.
83 note-books are sold at Rs 15.25 each. Find the total money (in rupees) obtained by selling these note-books.
The cost of one metre cloth is Rs. 38.50. Find the cost of 3.6 m cloth.
The length of an iron rod is 10.32 m. The rod is divided into 4 pieces of equal lengths. Find the length of each piece.
The cost of 25 identical articles is Rs 218.25. Find the cost of one article
If the product of two decimal numbers is 38.745 and one of the numbers is 2.7, find the other.
Change the following mixed fractions to improper fractions:
(i) 2\frac { 1 }{ 5 }
(ii) 3\frac { 1 }{ 4 }
(iii) 7\frac { 1 }{ 8 }
(iv) 2\frac { 1 }{ 11 }
Express the following improper fractions as mixed fractions:
7/4
Express the following mixed fractions as improper fractions:
2 \dfrac{5}{48}
Express the following mixed fractions as improper fractions:
2 \dfrac{4}{9}
Express the following mixed fractions as improper fractions:
7 \dfrac{5}{13}
Express the following as improper fractions:
a) 7\dfrac{3}{4} b) 5\dfrac{6}{7} c) 2\dfrac{5}{6} d) 10\dfrac{5}{3} e) 9\dfrac{3}{7} f)8\dfrac{4}{9}
Evaluate :
0.14616 \div 72
Evaluate :
0.6227 \div 1300
Express the following as mixed fraction:
a) \displaystyle \frac{20}{3} b) \displaystyle \frac{11}{5} c) \displaystyle \frac{17}{7} d) \displaystyle \frac{28}{5} e) \displaystyle \frac{19}{6} f) \displaystyle \frac{35}{9}
a) \displaystyle \frac{20}{3} b) \displaystyle \frac{11}{5} c) \displaystyle \frac{17}{7} d) \displaystyle \frac{28}{5} e) \displaystyle \frac{19}{6} f) \displaystyle \frac{35}{9}
Represent the following mixed infinite decimal periodic fractions as common fractions:
\displaystyle\frac{2b + a - \frac{4a^2 - b^2}{a}}{b^3 + 2ab^2 - 3a^2b} \cdot \frac{a^3b - 2a^2b^2 + ab^3}{a^2 - b^2}
Represent the following mixed infinite decimal periodic fractions as common fractions:
\displaystyle\left ( \frac{1}{a - \sqrt{2}} - \frac{a^2 + 4}{a^3 - \sqrt{8}} \right ) : \left ( \frac{a}{\sqrt{2}} + 1 + \frac{\sqrt{2}}{a} \right )^{-1}
Represent the following mixed infinite decimal periodic fractions as common fractions:
\displaystyle\frac{(5 \sqrt{3} + \sqrt{50})\, (5 - \sqrt{24})}{\sqrt{75} - 5 \sqrt{2}}
Represent the following mixed infinite decimal periodic fractions as common fractions:
\displaystyle\left ( \frac{1}{3} \right )^{-10}\, \cdot 27^{-3} + 0.2^{-4} \cdot 25^{-2} + (64^{-1/9})^{-3}
Represent the following mixed infinite decimal periodic fractions as common fractions:
\displaystyle\, \frac{\sqrt{x} + 1}{1 + \sqrt{x} + x} : \frac{1}{x^2 - \sqrt{x}}
Represent the following mixed infinite decimal periodic fractions as common fractions:
\displaystyle\, \left ( \frac{1}{m - \sqrt{mn}} + \frac{1}{m + \sqrt{mn}} \right )\cdot \frac{m^3 - n^3}{m^2 + mn + n^2}
Represent the following mixed infinite decimal periodic fractions as common fractions:
\displaystyle\, \left ( \frac{a^{1/2} + 2}{a + 2a^{1/2} + 1 } - \frac{a^{1/2} - 2}{a - 1} \right ) \cdot \frac{a^{1/2} + 1}{a^{1/2}}
Represent the following mixed infinite decimal periodic fractions as common fractions:
\displaystyle\, \frac{a -b}{a + b + 2\sqrt{ab}} : \frac{a^{-1/2} - b^{-1/2}}{a^{-1/2} + b^{-1/2}}
Represent the following mixed infinite decimal periodic fractions as common fractions:
\displaystyle\, \frac{b -x}{\sqrt{b} - \sqrt{x}} - \frac{b^{3/2} - x^{3/2}}{b - x}
Represent the following mixed infinite decimal periodic fractions as common fractions:
\displaystyle\,(x + \sqrt{x^2 - 1})^2 + (x + \sqrt{x^2 - 1})^{-2} + 2 (1 -2x^2)
Represent the following mixed infinite decimal periodic fractions as common fractions:
\displaystyle\,\left ( \frac{x^{1/2} + y^{1/2}}{x^{1/2} - y^{1/2}} - \frac{x^{1/2} - y^{1/2}}{x^{1/2} + y^{1/2}} \right ) (y^{-1/2} - x^{-1/2})
Represent the following mixed infinite decimal periodic fractions as common fractions:
\displaystyle\, \left ( \frac{3}{\sqrt{1 + a}} + \sqrt{1 -a} \right ) : \left ( \frac{3}{\sqrt{1 - a^2}} + 1 \right )
A flower garden is 22.50 m long. Sheela wants to make a border along one side using bricks that are 0.25 m long. How many bricks will be needed?
Represent the following mixed infinite decimal periodic fractions as common fractions:
\displaystyle\, \left ( \frac{\sqrt{a}}{2} - \frac{1}{2 \sqrt{a}} \right )^2 \left ( \frac{\sqrt{a} - 1}{\sqrt{a} + 1} - \frac{\sqrt{a} + 1}{\sqrt{a} - 1} \right )
Represent the following mixed infinite decimal periodic fractions as common fractions:
\displaystyle\, \left ( \frac{P^{3/2} + q^{3/2}}{p - q} - \frac{p - q}{P^{1/2} + q^{1/2}} \right )\left ( \sqrt{pq} \frac{\sqrt{p} + \sqrt{q}}{p - q} \right )^{-1}
Class 7 Maths Extra Questions
- Algebraic Expressions Extra Questions
- Comparing Quantities Extra Questions
- Data Handling Extra Questions
- Exponents And Powers Extra Questions
- Fractions And Decimals Extra Questions
- Integers Extra Questions
- Lines And Angles Extra Questions
- Perimeter And Area Extra Questions
- Practical Geometry Extra Questions
- Rational Numbers Extra Questions
- Simple Equations Extra Questions
- Symmetry Extra Questions
- The Triangle And Its Properties Extra Questions
- Visualising Solid Shapes Extra Questions