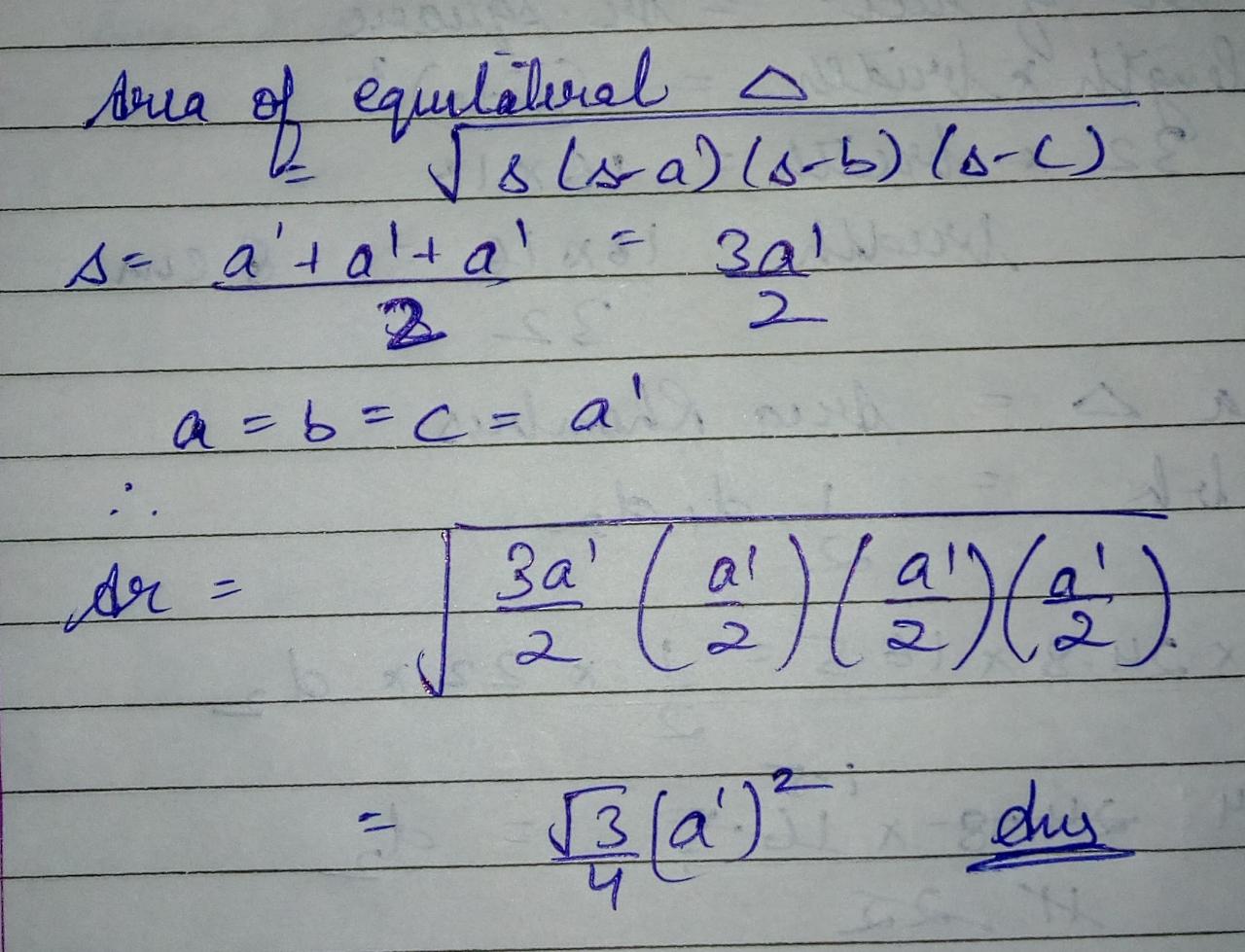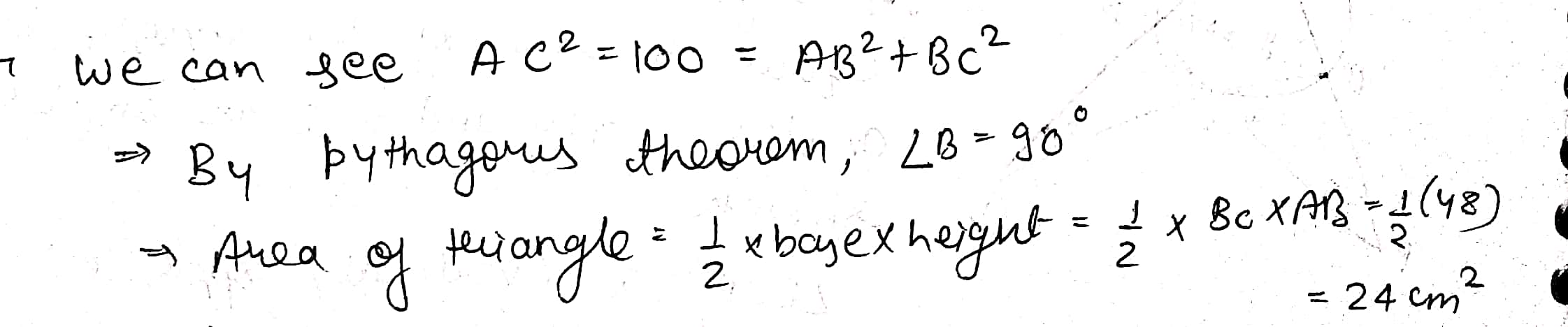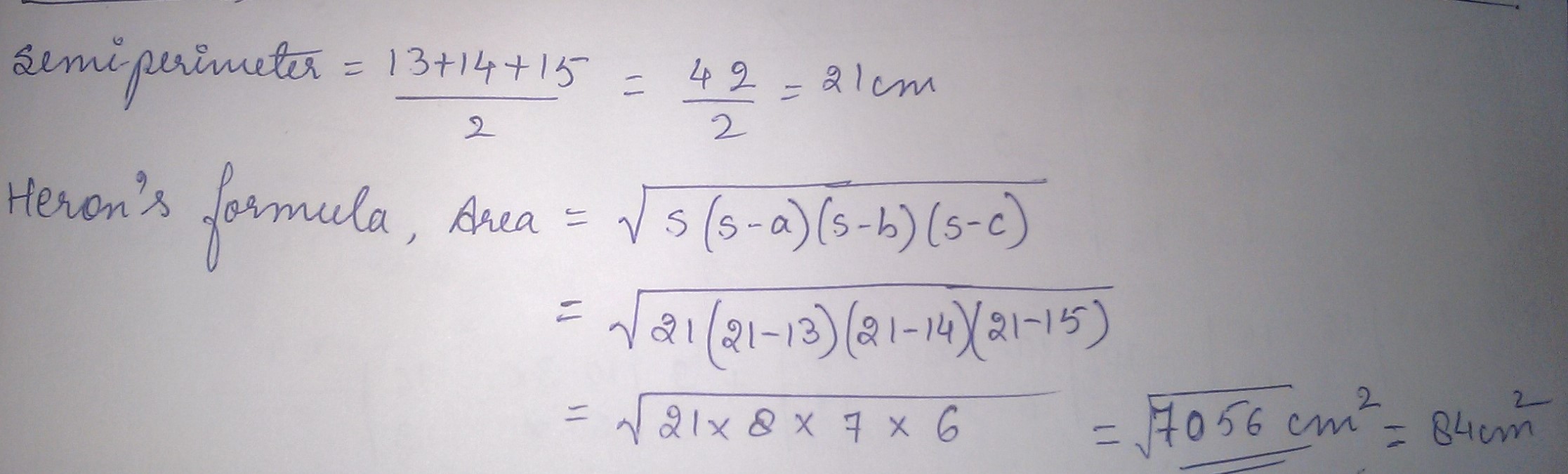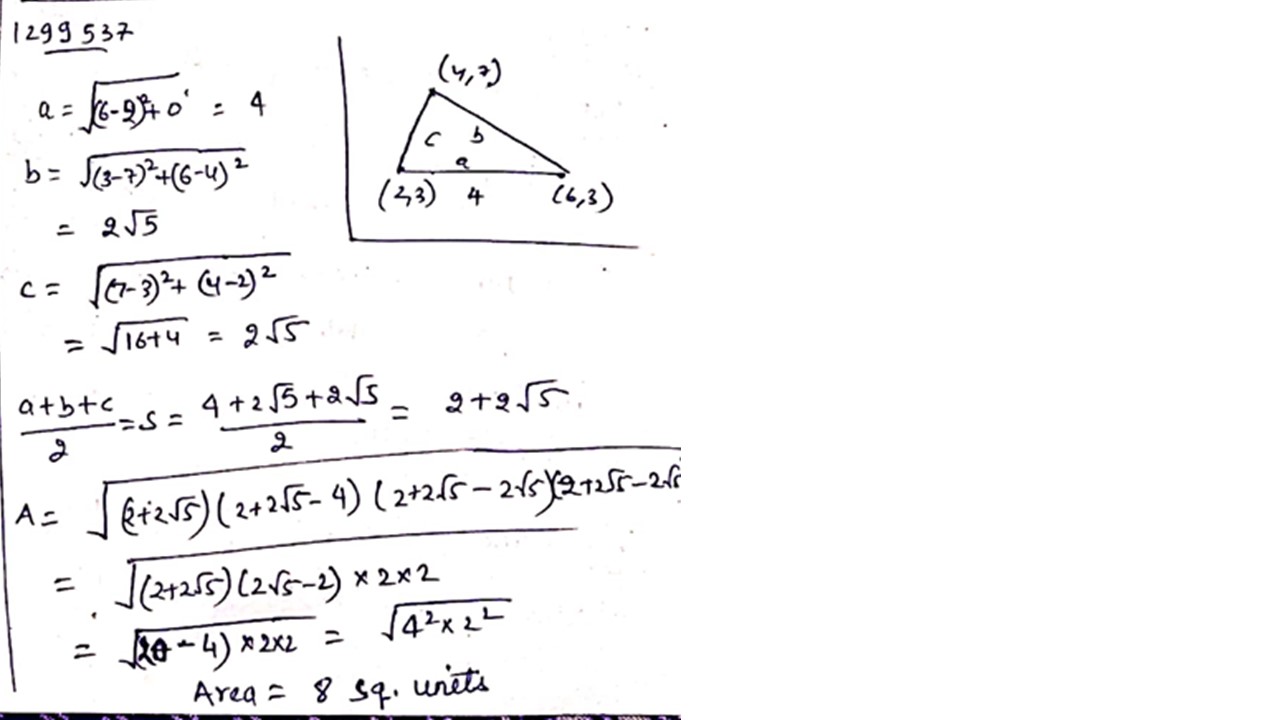Heron'S Formula - Class 9 Maths - Extra Questions
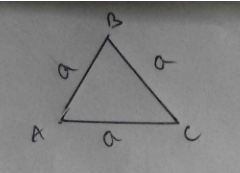
Find area of an equilateral triangle whose each side is a′.
The area of an equilateral triangle is (16×√3)cm2.Find the length of each side of triangle.
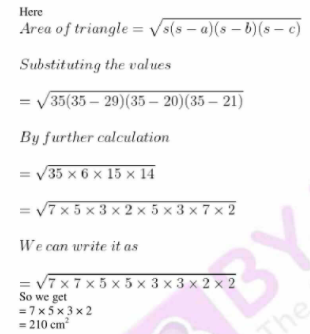
Sides of a triangle are 70 cm, 80 cm and 90 cm. Find its area.
The area of the triangle whose sides are 6,8,10 is ..........
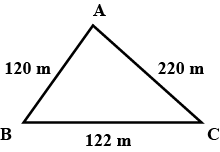
The longest side of a right angled triangle is 125 m and one of the remaining two sides is 100 m. Find its area using Heron's formula.
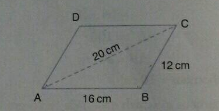
In a parallelogram ABCD, AB=16 cm,BC=12 cm and diagonal AC=20 cm. Find the area of the parallelogram.
The sides of a triangle are 12cm,16cm and 20cm. Find its area.
Find the perimeter of triangle with sides :
6cm,7cm,3cm
Find the area of the triangle whose sides are 42 cm, 34 cm and 20 cm in length. Find the height corresponding to the longest side.
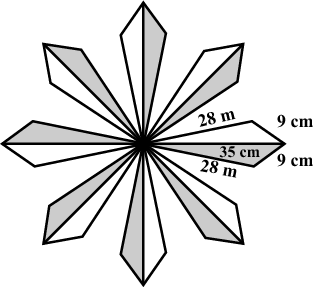
A floral design on a floor is made up of 16 tiles which are triangular, the sides of the triangle being 9cm, 28cm and 35cm. Find the cost of polishing the tiles at the rate of 50p per cm2.
Find the area of the triangle whose sides are 50 m, 78 m and 112 m and also find the length of the perpendicular from the opposite vertex to the side of length 112 m.
Write the Heron's formula used to calculate the area of a triangle whose side are a,b and c.
Side of a triangle in the ratio 7:12:8 and its perimeter is 270 cm, find its area.
Find the cost of laying grass in a triangular field of sides 50m,65m and 65m at the rate of Rs7 per m2.
Find the area of a triangle two sides of which are 18 cm and 10 cm and the perimeter is 42 cm.
From a point in the interior of an equilateral triangle, perpendiculars are drawn on the three sides. The lengths of the perpendiculars are 14cm, 10cm and 6cm. Find the area of the triangle.
Find the area of triangle whose sides are 4 cm,6 cm,8 cm
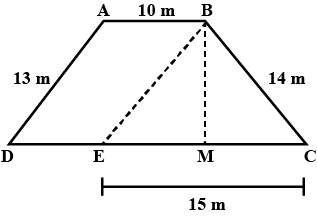
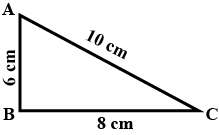
Find the area of the following triangle.
The triangular side walls of a flyover have been used for advertisements. The sides of the walls are 13m, 14m and 15m. The advertisements yield an earning of Rs. 2000 per m2 a year. A company hired one of its walls for 6 months. How much rent did it pay?
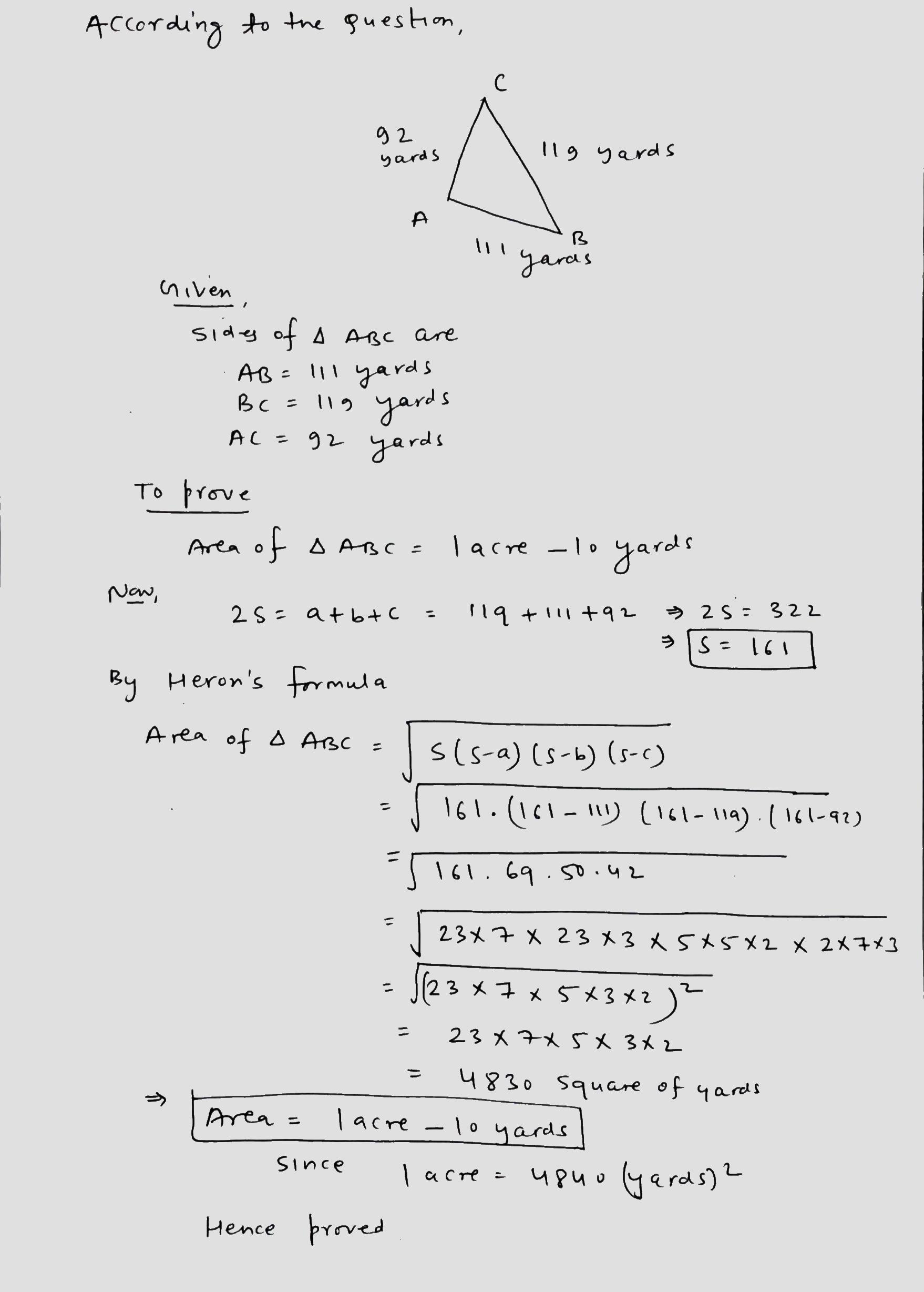
The sides of a triangle are 119,111 and 92 yards; prove that its area is 10 sq. yards less than an acre.
Find the area of the isosceles triangle whose
i) each of the equal sides is 8 cm and the base is 9 cm;
Write Heron's formula.
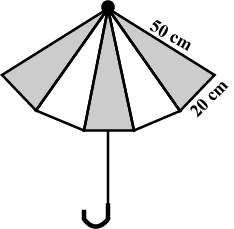
An umbrella is made by stitching 10 triangular pieces of cloth of 5 different colour each piece measuring 20cm, 50cm and 50cm. How much cloth of each colour is required for one umbrella?
The sides of the triangle are 78m, 112m and 50m respectively. What is the area of the triangle?
Find the area of a Δ whose sides are 8 m, 17 m and 15 m.
Prove: In ΔABC r1−ra+r2−rb=cr3
Find the area of the triangle with sides 35cm,54cm and 61cm.
The base of an isosceles triangle is 10 cm and one of its equal sides is 13 cm. Find its area using Heron's Formula.
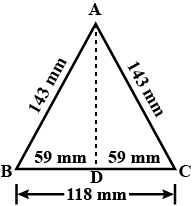
An isosceles triangle has a base 118mm long and two equal sides are each 143mm long. calculate the are of the triangle.
Give an triangle with an area of 3.94 sq.units a perimeter of 16 units and sides a=3 units, b=7 units. Find c in units.
The semi- perimeter of a triangle is 96cm and its sides are in the ratio 3:4:5. Find the area of the triangle.
The sides of Δ are 6cm ,8cm,10cm find its area using heron's formulas
A farmer has a triangular field with sides 240 m and 360 m, where he grew wheat. In another triangular field with sides 240 m, 320 m and 400 m adjacent to the previous field, he wanted to grow potatoes and onions. He divided the field into two parts by joining the mid point of the longest side to the opposite vertex and grew potatoes in one part and onions in the other part. How much area (in hectares) has been used for wheat, potatoes and onions ?

The perimeter of an isosceles triangle is equal to 14 cm, the ratio of the lateral side to the base is 5:4, then the area of the triangle is:
Find area of the triangle with sides 5 cm, 12 cm, 13 cm.
The sides of a triangular field are in the ratio 5:3:4 and its perimeter is 180m. Find:
(i) its area
(ii) altitude of the triangle corresponding to its largest side
(iii) the cost of levelling the field at the rate of Rs. 10 per square metre
An umbrella is made by stitching 6 triangular pieces of cloth of red and black colours each measuring 20cm, 50cm, and 50cm. How much cloth of each colour is required for umbrella?
Find the area of an isosceles triangle each of whose equal sides measures 13 cm and whose base measures 20 cm.
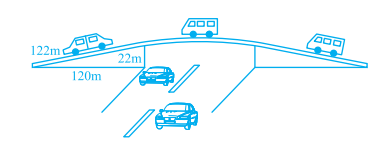
The triangular side walls of a flyover have been used for advertisements.The sides of the walls are 122m,22m,120m. The advertisements yield an earning of RS. 5000 per m2. A company hired one of its walls for 3 months. How much rent did it pay?
The triangular side walls of a flyover have been used for advertisements. The sides of the walls are 13 m, 14 m and 15 m. The advertisements yield an earning of Rs 2000 per m2 a year. A company hired one of its walls for 6 months. How much rent did it pay?
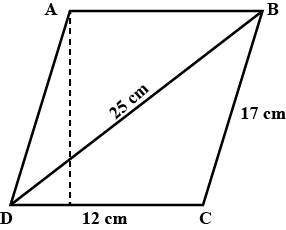
Find the area of a parallelogram given in Fig. 12.Also find the length of the altitude from vertex A on the side DC.(in cm2)
The perimeter of a triangle is 50 cm. One side of a triangle is 4 cm longer than the smaller side and the third side is 6 cm less than twice the smaller side, then the area of the triangle is 20√30cm2
If true then enter 1 and if false then enter 0
Find the area of a triangle in mm2, whose sides are: 18mm,24mm and 30mm.
Find the area of a triangle in cm2, whose sides are: 10cm,24cm and 26cm
The sides of a triangle are 16 cm, 12 cm and 20 cm. Find area of the triangle
The lengths of the sides of a triangle are in the ratio 4:5:3 and its perimeter is 96 cm. Find its area (in sq. cm).
If a triangle with sides (in units) of measure a,b and c has an area of ′A′ sq units and a triangle with sides measuring a+b,b+c,c+a has an area of ′1′ sq units, then prove that T≥4A.
The sides of a triangular field are in the ratio 5:3:4 and its perimeter is 180 m, then find the altitude of the triangle corresponding to its largest side (in m.)
The sides of a triangular field are in the ratio 5:3:4 and its perimeter is 180 m, then find the cost of levelling the field at the rate of Rs. 10 per square meter.
Find the area of a triangle in cm2; whose sides are: 21cm,28cm and 35cm.
The lengths of the sides of a triangle are in the ratio 3:4:5. Find the area of the triangle if its perimeter is 144 cm .
The area of a triangle is 57 sq. cm. If the two sides are 8 cm and 19 cm respectively, then what is the third side approximately?
Find the length of the perpendicular from the vertex opposite to the side 72 m of the triangular field, whose other two sides are 30 m and 78 m.
Radha made a picture of an aeroplane with coloured paper as shown in Fig 12.15. Find the total area of the paper used.
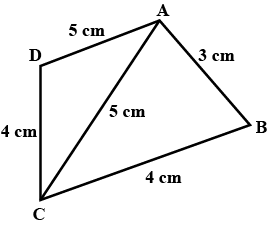
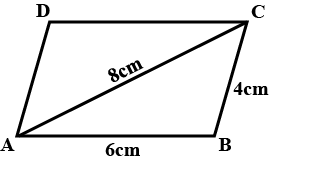
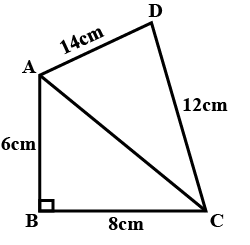
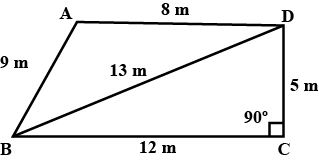
Find the area of a quadrilateral ABCD in which AB=3 cm, BC=4 cm, CD=4 cm, DA=5 cm and AC=5 cm
A park, in the shape of a quadrilateral ABCD, has ∠C=90o, AB=9 m, BC=12 m, CD=5 m and AD=8 m. How much area does it occupy?
An isosceles triangle has perimeter 30cm and each of the equal sides is 12cm. Find the area of the triangle.
Find the area of a triangle two sides of which are 18 cm and 10 cm and the perimeter is 42 cm.
Find the area of a triangle whose sides are 16 cm, 19 cm, and 21 cm in length.
In an isosceles triangle , length of the congruent sides is 13 cm and its base is 10 cm . Find the distance between the vertex opposite the base and the centroid
A traffic signal board, indicating ′SCHOOL AHEAD′, is an equivalent triangle with side 'a'. Find the area of the signal board, using Heron's formula. If its perimeter is 180 cm, what will be the area of the signal board?
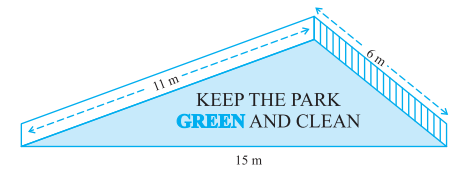
There is a slide on a park. One of its side walls has been painted in some colour with a message "KEEP THE PARK GREEN AND CLEAN" (see figure). If the sides of the wall are 15 m,11 m and 6 m, find the area painted in colour.
Sides of a triangle are in the ratio of 12:17:25 and its perimeter is 540 cm. Find its area.
The triangular side walls of a flyover have been used for advertisements. The sides of the walls are 122 m,22 m and 120 m (see figure). The advertisements yield an earning of Rs.5000 per m2 per year. A company hired one of its walls for 3 months. How much rent did it pay?
An umbrella is made by stitching 10 triangular pieces of cloth of two different colours (see figure), each piece measuring 20 cm, 50 cm and 50 cm. How much cloth of each colour is required for the umbrella?
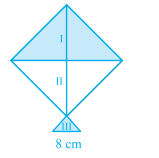
A kite in the shape of square with a diagonal 32cm and an isosceles triangle of base 8cm and sides 6cm each is to be made of three different shades as shown in figure. How much paper if each shade has been used in it?
A floral design on a floor is made up of 16 tiles which are triangular, the sides of the triangle being 9 cm, 28 cm and 35 cm (see figure). Find the cost of polishing the tiles at the rate of 50 p per cm2.
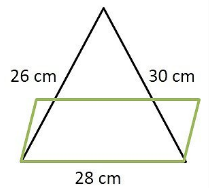
A triangle and a parallelogram have the same base and the same area. If the sides of the triangle are 26cm,28cm and 30cm and the parallelogram stands on the base 28cm, find the height of the paraollelogram.
In ΔABC, BC=11 cm, CA=13 cm and the median to side AB is 10 cm. The area of the Δle is:
Find area of a triangle whose sides measures 13 cm,14cm,15cm.
Find the area of a triangle if its sides are 9cm,12cm and 15cm
r1(s−b)(s−c)+r2(s−c)(s−a)+r3(s−a)(s−b)=3r
Find the area of \triangle two sides are 18\ cm, 10\ cm if perimeter is 42\ cm
The sides of a triangle are x, x + 1, 2x - 1 and its area is x\sqrt{10}. What is the value of x?
A triangle has sides of 35\ cm, 54\ cm and 61\ cm long. Find its area and its smallest altitude.
A kite in the shape of a square with a diagonal 40\ \text{cm} and an isosceles triangle of base 10\ \text{cm} and equal sides 13\ \text{cm} each, is to be made up of three different shades as shown in the figure. How much paper of each shade has been used to make the kite?
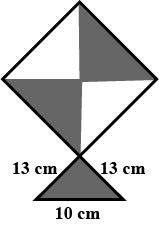
The perimeter of right angled triangle is 12 cm and its hypotenuse is of length 5 cm. Find the other two sides and calculate its area. Verify the result using heron' formula.
Each of equal sides of an isosceles triangle is 4 cm greater than its height. If the base of the triangle is 24 cm, calculate the perimeter and the area of the triangle.
The length of the sides of a triangle are in the ratio 3:4:5 and its perimeter is 144 cm. Find the area of the triangle and the height corresponding to the longest side
In the given figure, ABCD is a rectangle, where AB = 8 cm, BC = 6 cm and the diagonals bisect each other at O. Find the area of the shaded region by Heron's formula.

A school started a campaign against the habit of taking junk food. Ten students were asked to prepare banners in a triangular shape. The sides of the banner are 112, 78 AND 50\ cm. if cost of banners is Rs 3 per 100\ cm2, find the total cost of banners. What valye is being promoted in this question?
find the area of the triangle whose are (-5,-1) (3,-5) (5,2).
Find the area of triangle between the co-ordinate axis of line 3x-4y-12=0
A triangle and a parallelogram have the same base and the same area. If the sides of the triangle are 26cm, 28cm and 30cm and the parallelogram stands on the base 28cm, find the height of the parallelogram.
Find the area of triangle with sides 3 cm, 7 cm and 8 cm.
Find the area of this \triangle by hero's formula.
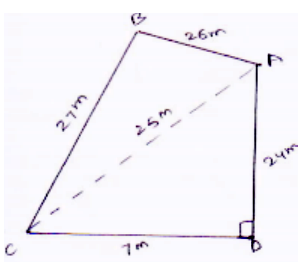
The sides of quadrangular field taken in order are 26,27,7,24m respectively. The angle contained by the last 2 sides is a right angle. Find its area.
Find the area of a triangle with sides a=3cm, b=2cm, c=4cm.
If base of an isosceles triangle is 12\ cm and its perimeter is 35\ cm, find the area of the triangle.
Derive a formula for area of equilateral triangle in terms of its side using Heron's formula.
Find the area of triangle whose sides are 13 cm, 14 cm and 15 cm.
The area of a parallelogram with a base 24 \text{ cm} is equal to the area of a triangle of sides 10 \text{ cm},\ 24 \text{ cm} and 26 \text{ cm}. Find the altitude of the parallelogram.
Get the formula \triangle =\sqrt {s(s-a)(s-b)(s-c)} for the area of a triangle by use of vectors.
Using Heron's formula, find the area of a triangle whose sides are 5\ cm, 12\ cm and 13\ cm.
Find the area of the triangle whose vertices are (1, 2), (3, 7) and (5, 3),
Find the altitude of the triangle if its area is 25\ acres and base is 20\ m.
A parallelogram has sides 6\ cm and 4\ cm and one of its diagonals is 8\ cm then its area is:-
Find the area of the triangle in which a=91\ m,\ b=98\ m,\ c=105\ m, where a,b,c are sides of triangle.
Find the area of the triangle in which a=52\ cm,\ b=56\ cm,\ c=60\ cm, where a,b,c are sides of triangle.
Find the area of a triangle plot whole side are 61 cm, 60 cm, 11 cm

Find the area of a triangle whose sides are 13 cm, 14 cm and 15 cm.
A park is in the shape of quadrilateral ABCD as shown. Given that angle C=90^{o} Also, AB=9\ m,\ BC=12\ m,\ CD=5\ m and AD=8\ m. Using Pythagoras Theorem find the length of diagonal BD. Also, find the total area of the given quadrilateral by using Heron's Formula.
An isosceles triangle has perimeter 30\ cm and each of the equal side is 12\ cm. Find the area of the triangle.
Find the area of a triangle whose sides re in the ratio 5:12:13 and its perimeter is 60\ cm.
What is Heron's formula?
Plot the points (2,3)(6,3)and(4,7) in a graphsheet.join them to make it a triangle find the area of the triangle
The sides of a triangle-shaped sheet are 5\ cm, 12\ cm and 13\ cm. Find the cost of painting on the sheet at the rate of Rs.\ 30\ per\ cm^ {2}.
In a quadrilateral ABCD, CO and DO are the bisectors of \angle C and \angle D respectively. Prove that \angle COD=\dfrac{1}{2}(\angle A+\angle B).
Calculated the area of the triangle whose are 18 cm, 24 cm and 30 cm in length. also find the length of the altitude corresponding is the smallest side.
Find the area of the triangle whose side are 42 cm, 34 cm and 20 cm in length. hence find the height corresponding to the longest side.
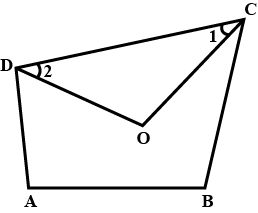
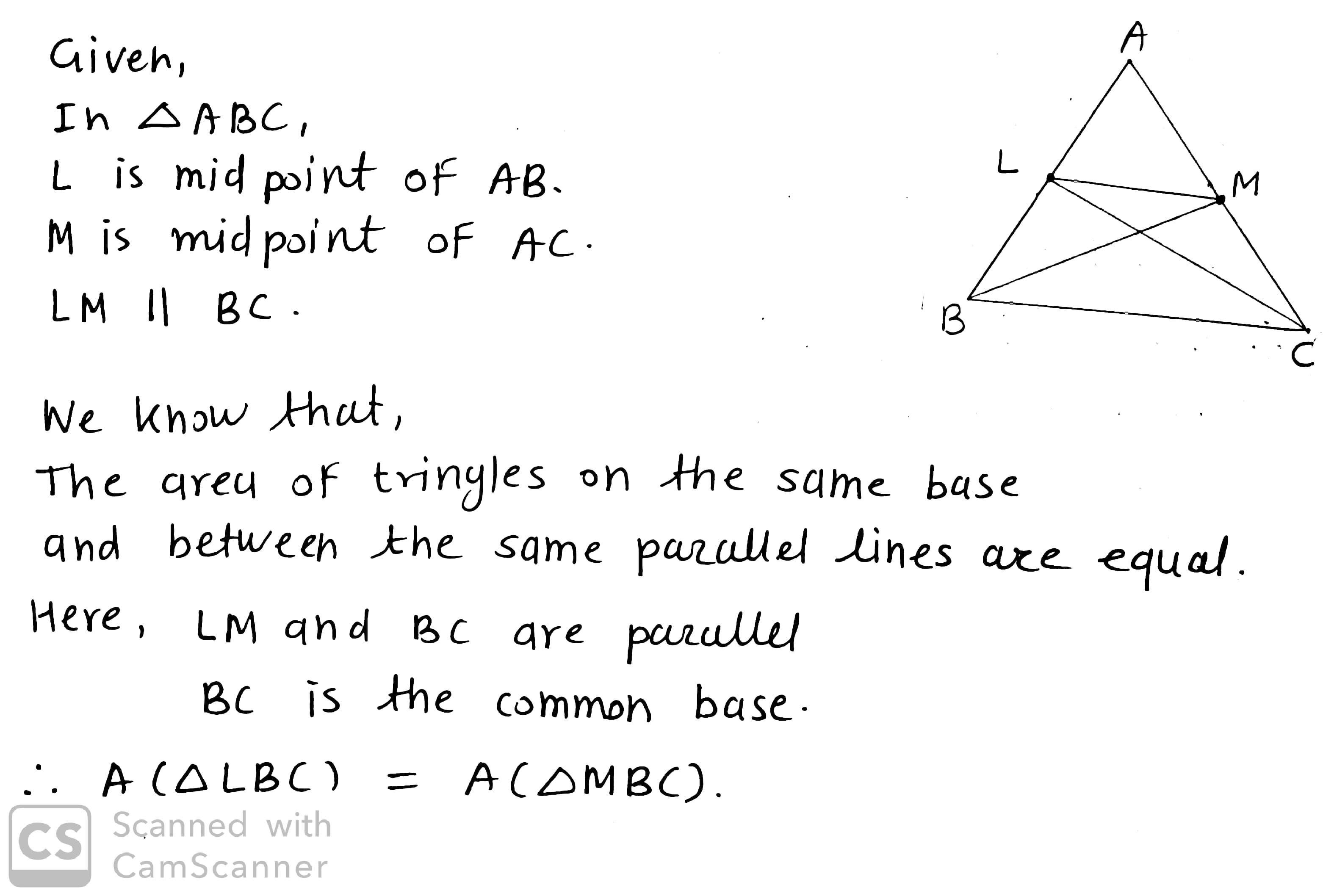
In a \triangle ABC, if L and M are points on AB and AC respectively such that LM||BC.Prove that:
ar(\triangle LBC)=ar (\triangle MBC)
Find the area and perimeter of an isosceles right triangle, each of whose equal sides measures 10 cm. [Given: \sqrt{2} = 1 .41]
Find the area of the triangle whose sides are 18 cm, 24 cm and 30 cm. Also, find the height corresponding to the smallest side.
Each of the equal sides of an isosceles triangle measures 2 cm more than its height, and the base of the triangle measures 12 cm. Find the area of the triangle.
The height of an equilateral triangle is 6 cm. Find its area. [Take \sqrt{3} = 1 .73]
If the area of an equilateral triangle is 81\sqrt{3}cm^{2}, find its height.
The base of a right-angled triangle measures 48 cm and its hypotenuse measures 50 cm. Find the area of the triangle.
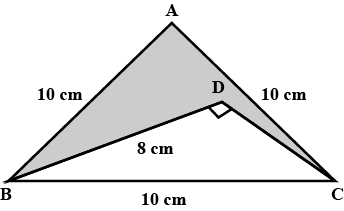
In the given figure, \Delta ABC is an equilateral triangle the length of whose side is equal to 10 cm, and \Delta DBC is right-angled at D and BD = 8\,cm. Find the area of the shaded region. [Take \sqrt{3} = 1.732.]
Each side of an equilateral triangle is 10 cm. Find (i) the area of the triangle and (ii) the height of the triangle.
The sides of a triangle are in the ratio 5: 12 : 13, and its perimeter is 150 m. Find the area of the triangle.
In Fig., area of triangle PQR is 20\, cm^2 and area of triangle PQS is 44\, cm^2. Find the length RS. if PQ is perpendicular to QS and QR is 5\,cm.

The sides of a quadrilateral ABCD taken in order are 6\ cm,\ 8\ cm,\ 12\ cm, and 14\ cm respectively and the angle between the first two sides is a right angle. Find its area. (Use \sqrt{6}=2.45)
Using Herons formula, find the area of an equilateral triangle the length of whose one side is x.
Find the area of a triangle whose sides are
29 cm, 20 cm , and 21 cm
Find the area of a triangle whose sides are
3 cm,4 cm, and 5 cm
The perimeter of a triangular field is 540\text{ m} and its sides are in the ratio of 25: 17: 12. Find the area of the field. Also, find the cost of plowing the field at \text{Rs. } 5 \text{ per m}^2.
In the given triangles of Fig, perimeter of triangle ABC = perimeter of triangle PQR. Find the area of triangle ABC.
The ratio of the area of triangle WXY to the area of triangle WZY is 3:4 (Fig). If the area of triangle WXZ is 56 cm^2 end WY =8\, cm, find the lengths of XY and YZ.
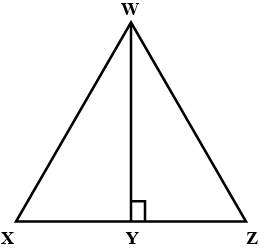
The perimeter of an isosceles triangle is 32cm. The ratio of the equal side to its base is 3:Find the area of the triangle.
An umbrella is made by stitching 12 triangular pieces of cloth, each measuring (50\ cm\times 20\ cm \times 50\ cm). Find the area of the cloth used in it.

If the perimeter of an equilateral triangle is 36 cm,calculate its area
Find the area of a triangle whose sides are 12 cm, 9.6 cm, and 7.2 cm
Find the area of an equilateral triangle whose side is 8 cm. Give your answer correct to two decimal places.
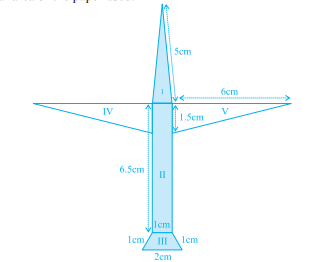
How much paper of yellow shade is needed in cm^2 to make a kite given in Fig, in which ABCD is a square with diagonal 44 cm.

The legs of a right triangle are 12 cm and 5 cm long. Find the distance between the centres of the inscribed and the circumscribed circle.
Find to the three places of decimals the radius of the circle whose area is the sum of the areas of two triangles whose sides are 35, 53, 66 and 33, 56, 65 measured in centimetres. (Use \pi = 22/7).
A triangular park in a city has dimensions 30m\times 26m\times 28m. A gardener has to plant grass inside the park at Rs.150\quad per\quad { m }^{ 2 }. Find the amount to be paid to the gardener.
In the given figure, ABC is a right angle triangle right-angled at A. Find the area of shaded region, if AB = 6m, BC = 10m and O is the centre of the incircle of triangle ABC (Use \pi = 3.14)
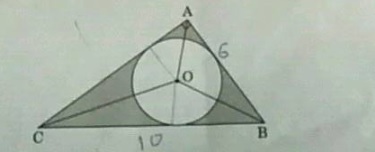
Find the area of a triangle whose sides are 8 cm, 15 and 17 cm.
A field is in the shape of a trapezium whose parallel sides are 25\ m and 10\ m. The non-parallel sides are 14\ m and 13\ m. Find the area of the field.
Find the area of a triangle field of sides 18, 24m and 30m. Also find the altitude corresponding to the shortest side.
If each sides of a triangle is double, then find the ratio of area of the new triangle thus formed and the given triangle.
A park, in the shape of a quadrilateral ABCD, has \angle{C}={90}^{o}, AB=9\ m, BC=12\ m, CD=5\ m and AD=8\ m. How much area does it occupy?
Find the area of a triangle whose sides. are 18 cm , 10 cm and 14 cm.
\triangle ABC \sim \triangle PQR,M is mid point of BC and N is mid point of QR. If the area of \triangle AC=100\ cm^{2}, the area of \triangle PQR=144\ cm^{2} and AM=4\ cm, then find the length of PN
Find the area of the triangle in which
a = 13 m, b = 14 m and c = 15 m
In the triangle ABC with vertices A(2,\ 3),\ B(4,\ -1) and C(1,\ 2). find the equation and the length of the altitude from the vertex A.
Find the area of a triangle whose sides are 34 cm, 20 cm and 42 cm . hence the length of the altitude corresponding to the shortest side.
In figure ABCD is a ||^{gm}. O is any point on AC. PQ||AB and LM||AD. Prove that ar(||^{gm} DLOP)=ar (||^{gm} BMOQ).
Class 9 Maths Extra Questions
- Areas Of Parallelograms And Triangles Extra Questions
- Circles Extra Questions
- Constructions Extra Questions
- Coordinate Geometry Extra Questions
- Heron'S Formula Extra Questions
- Introduction To Euclid'S Geometry Extra Questions
- Linear Equations In Two Variable Extra Questions
- Lines And Angles Extra Questions
- Number Systems Extra Questions
- Polynomials Extra Questions
- Probability Extra Questions
- Quadrilaterals Extra Questions
- Statistics Extra Questions
- Surface Areas And Volumes Extra Questions
- Triangles Extra Questions