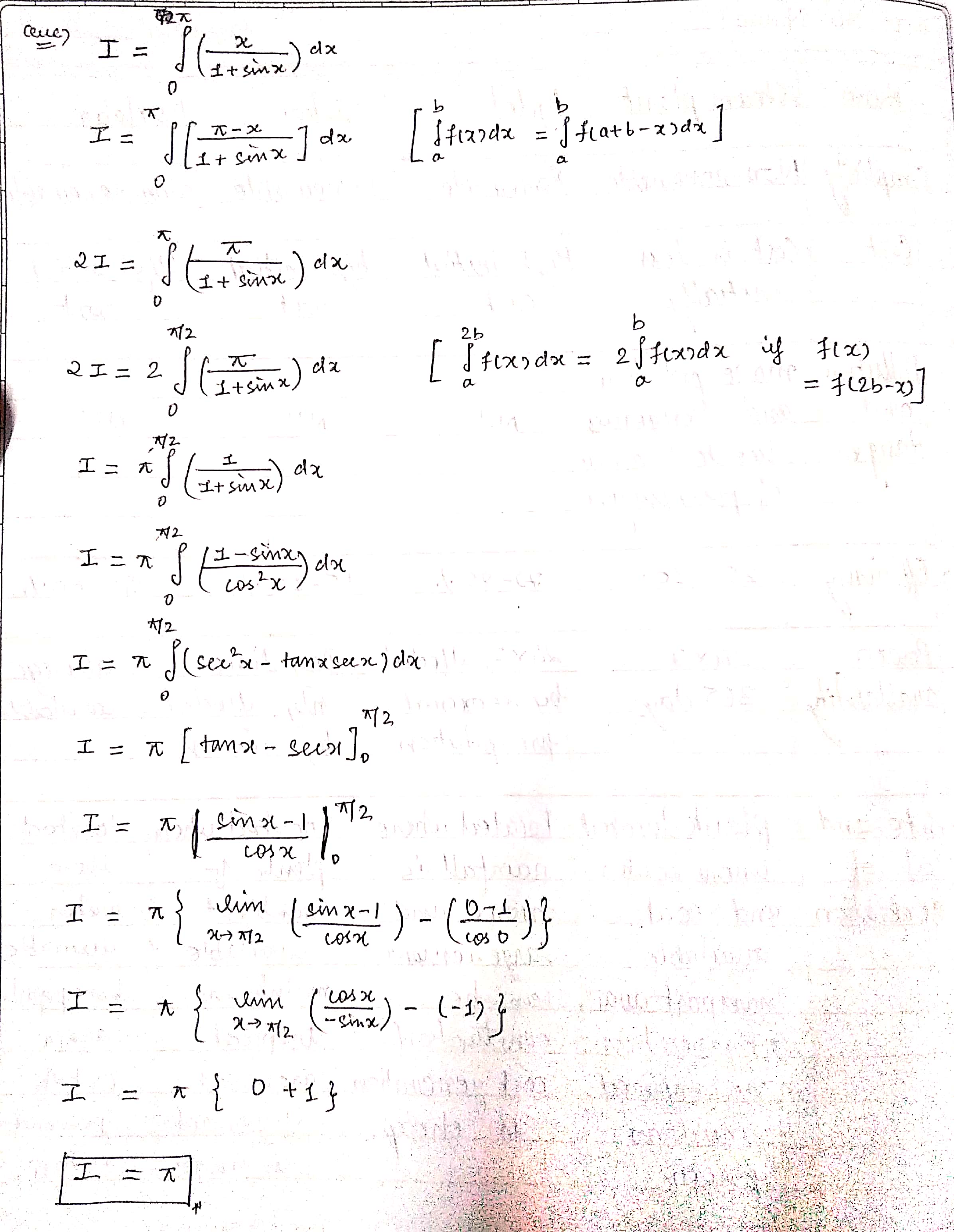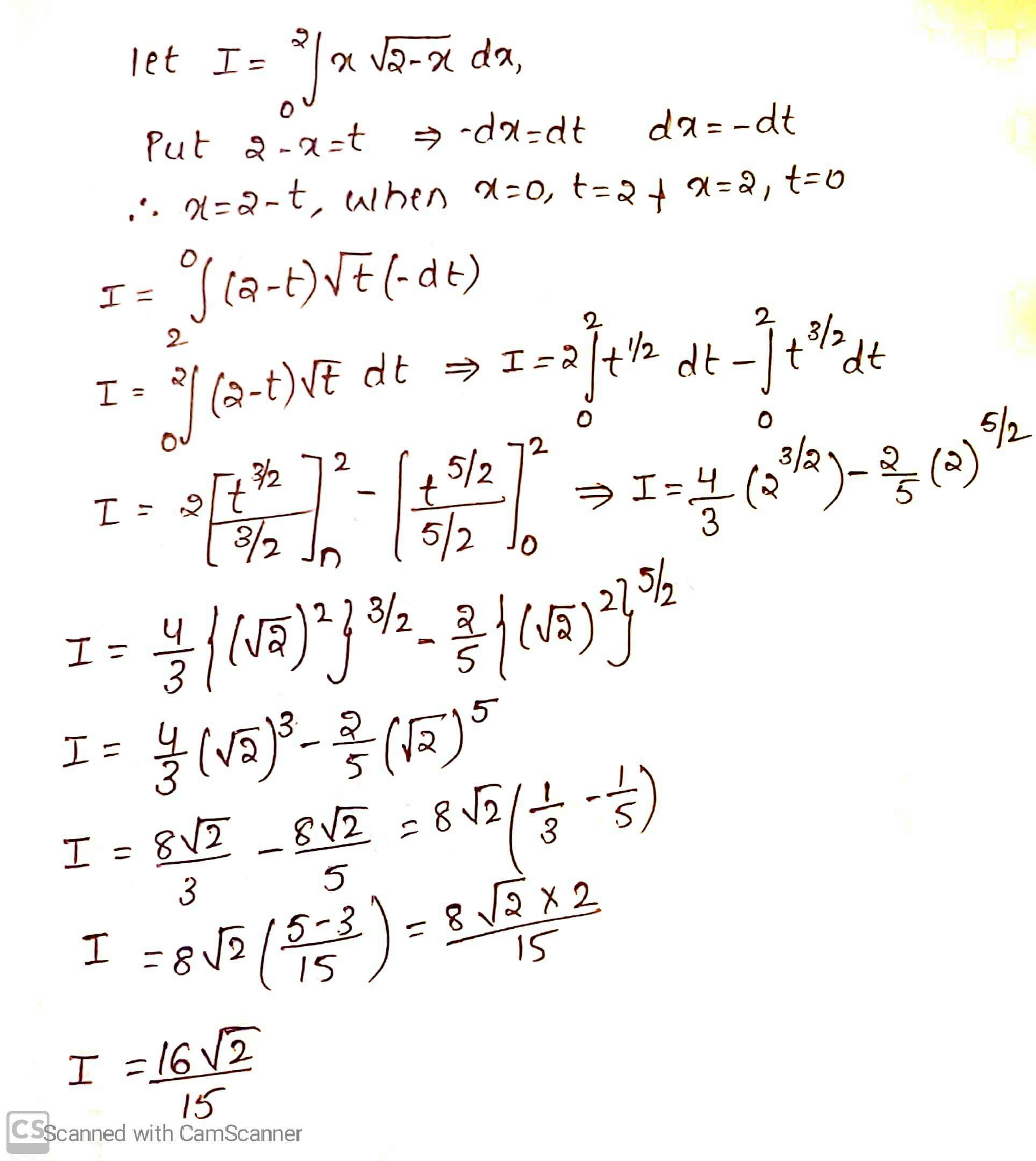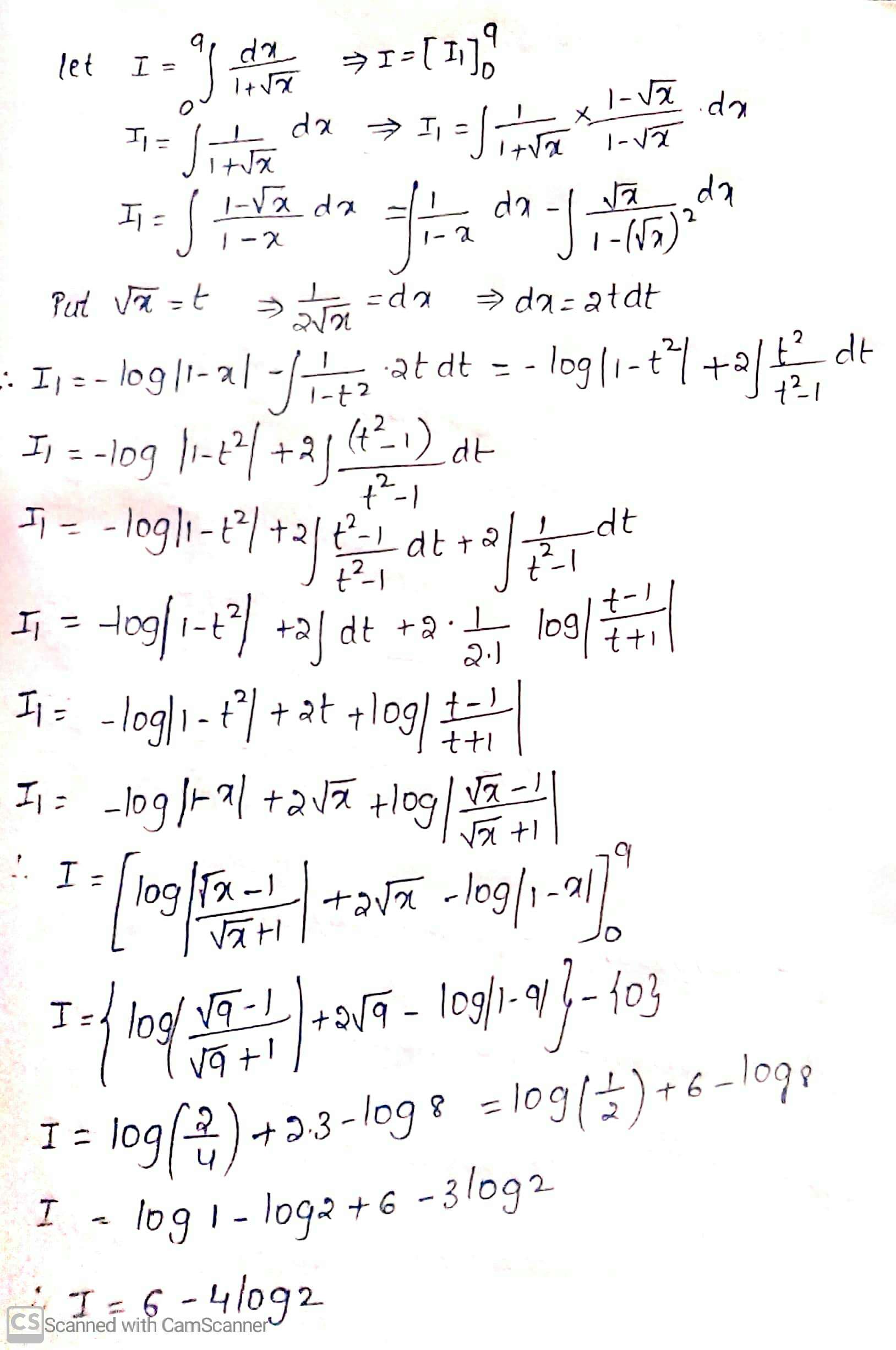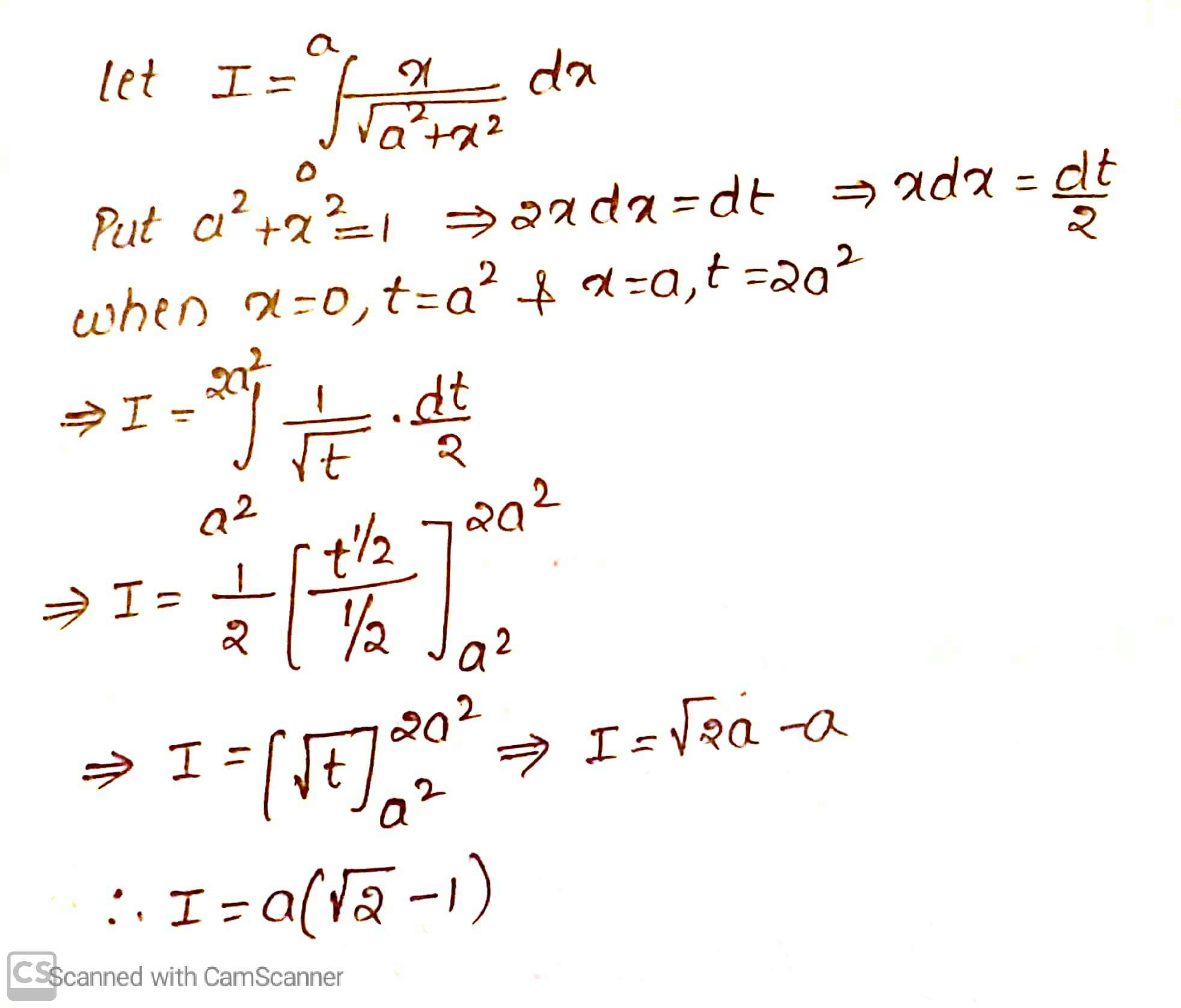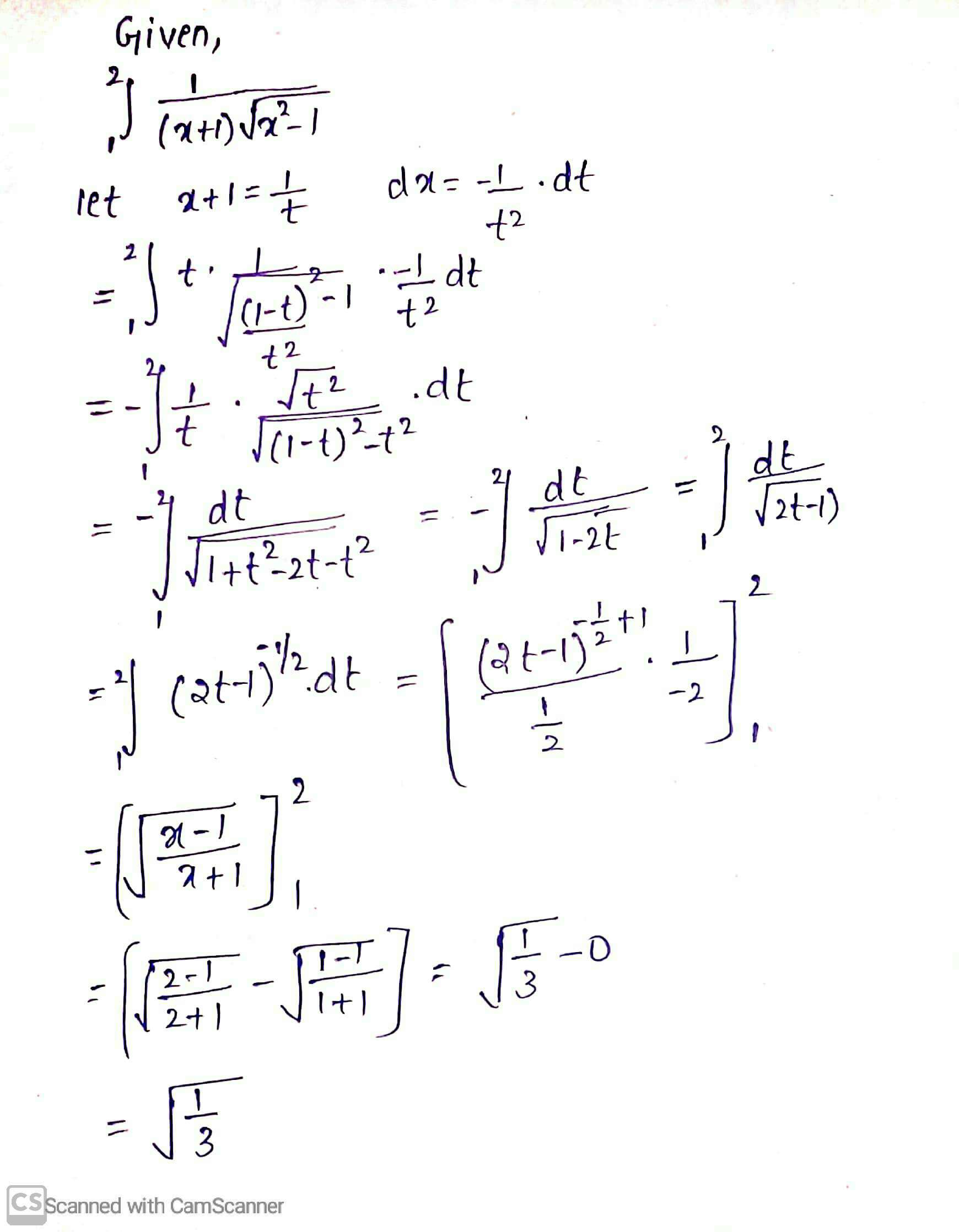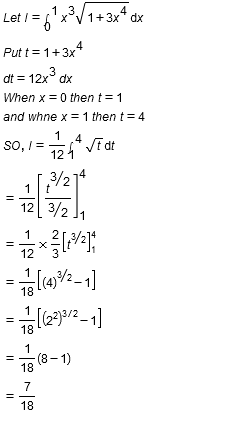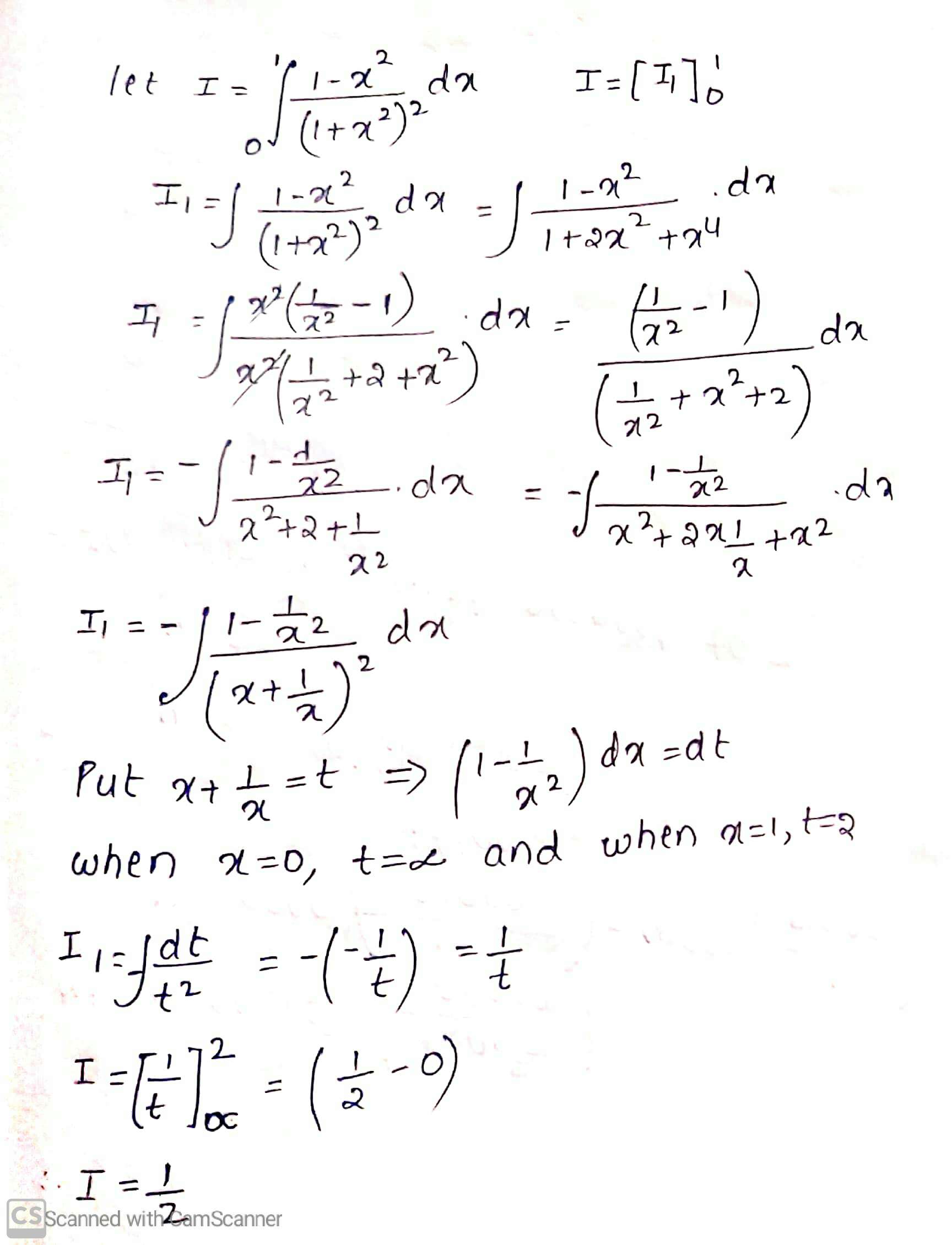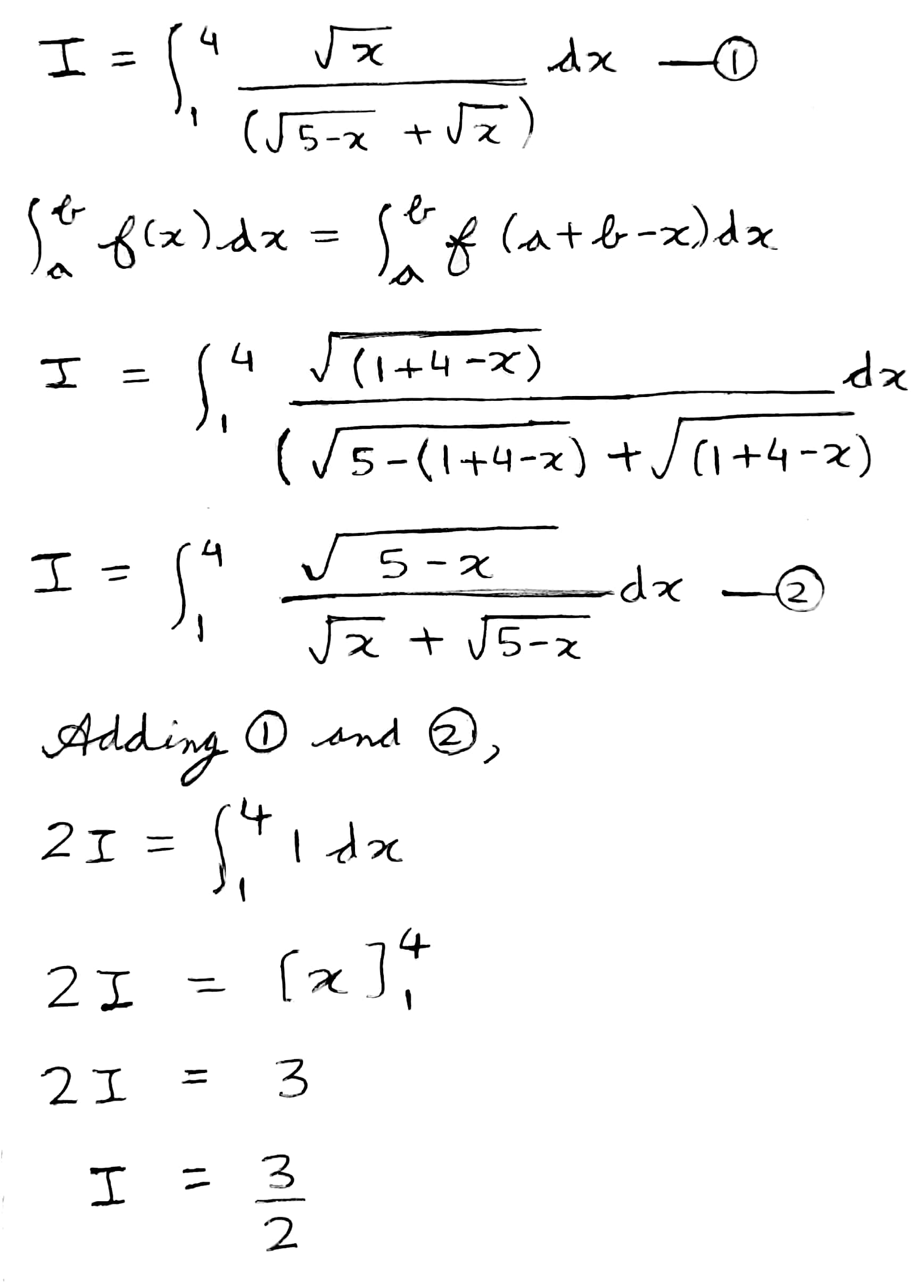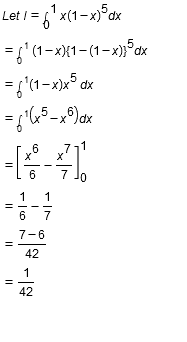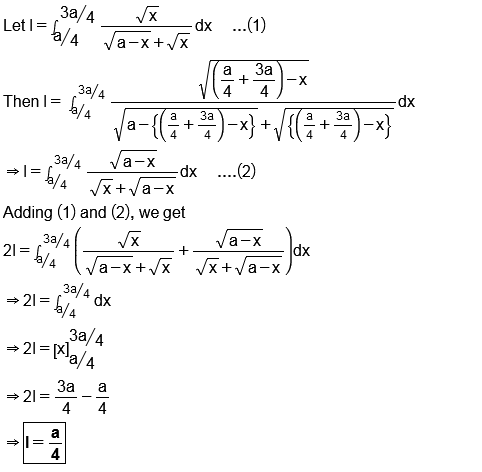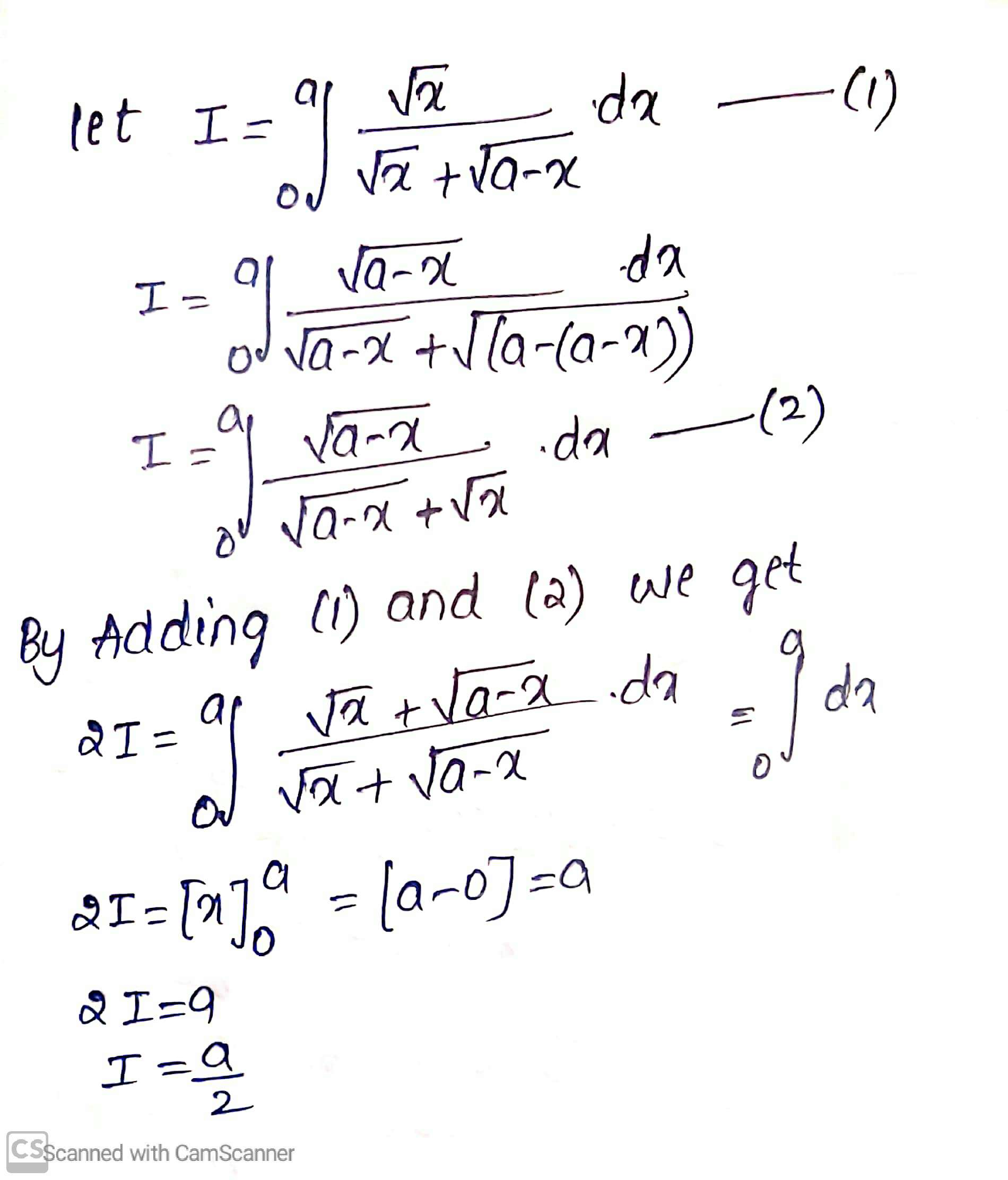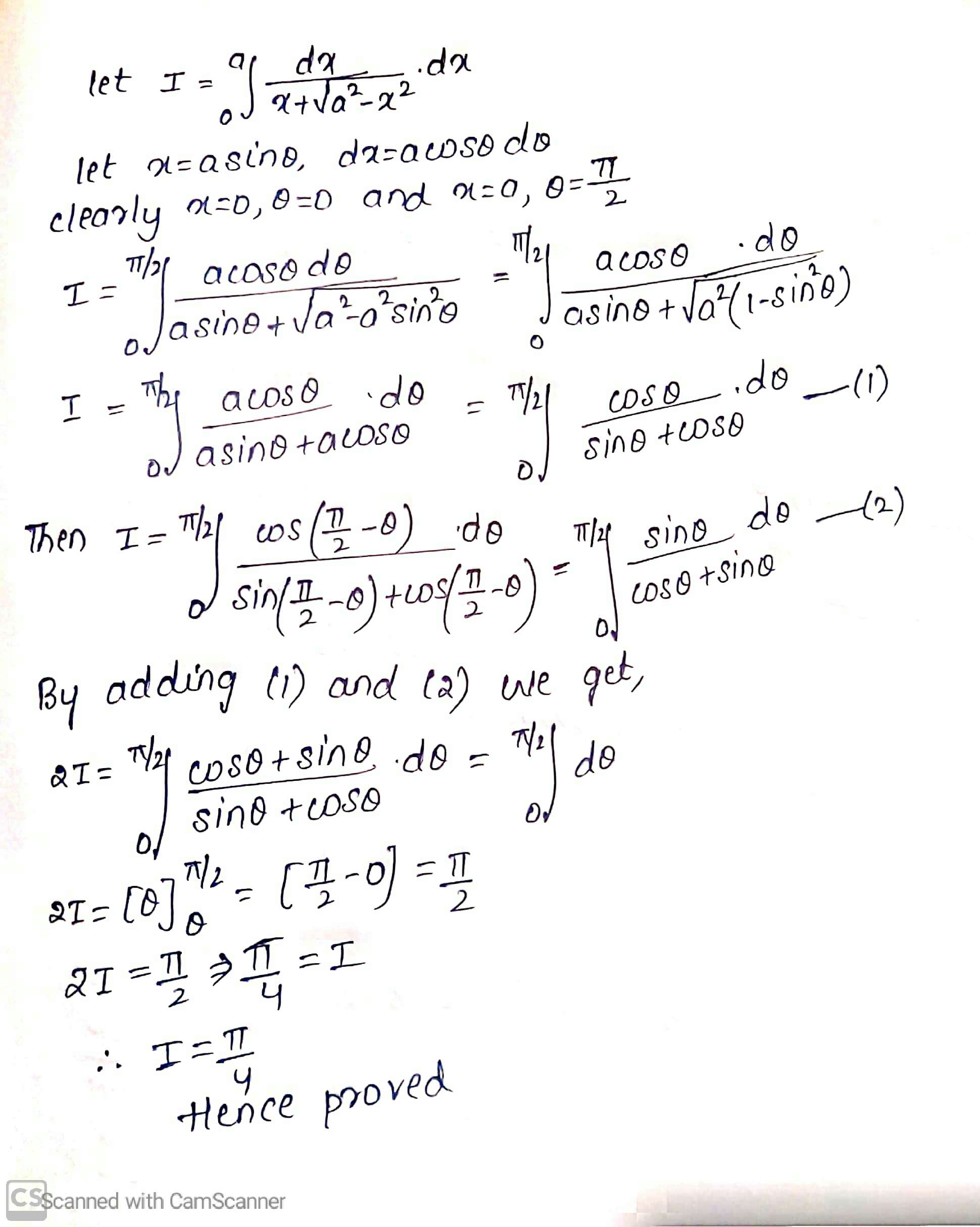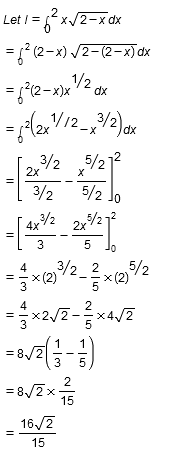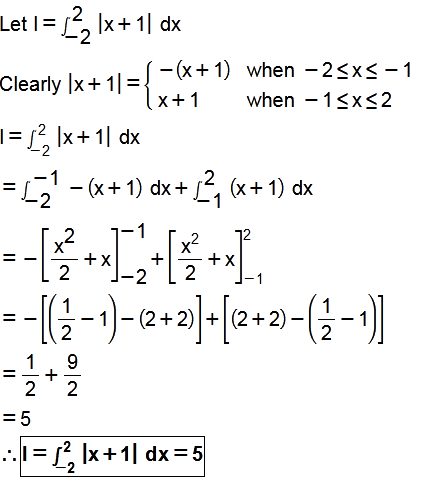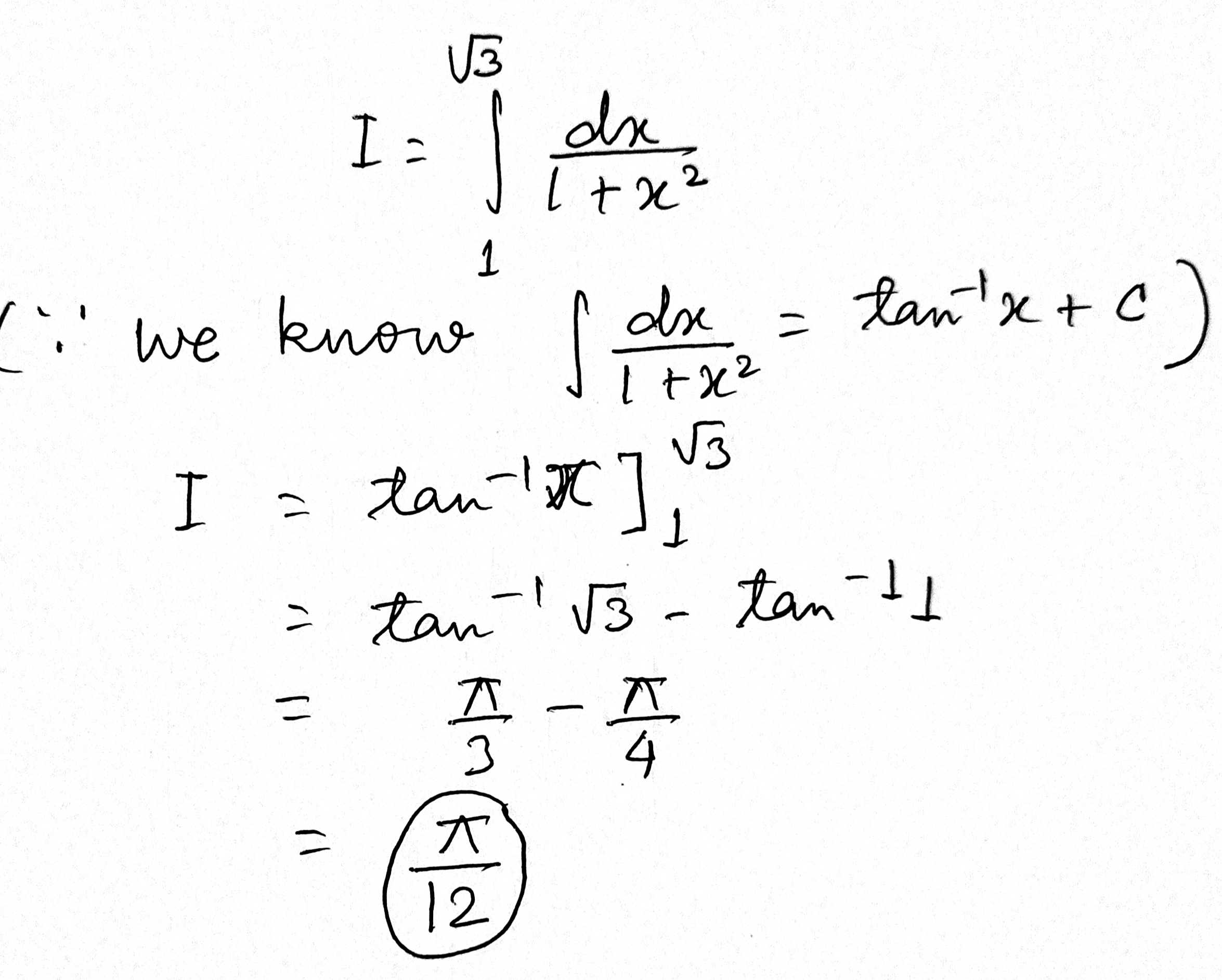Class 12 Engineering Maths - Integrals

The value of the definite integral, $I = \int _{ 0 }^{ \sqrt { 10 } }{ \dfrac { x }{ { e }^{ { x }^{ 2 } } } } dx$ is equal to

$I = \displaystyle \int_0^{\sqrt{10}} \frac{x}{e^{x^2}}dx \quad x^2 = t$

$\displaystyle \Rightarrow 2x . dx = dt \Rightarrow x \, dx = \frac{dt}{2}$

$I = \displaystyle \int_0^{\sqrt{10}} \frac{dt}{2\times e^t} =\frac{1}{2}\int_0^{\sqrt{10}} e^{-t} \, dt$

$I \displaystyle =\frac{-1}{2}[e^{-t}]_0^{\sqrt{10}} = \frac{-1}{2}[e^{-x^2}]_0^{\sqrt{10}}$

$I \displaystyle =\frac{-1}{2} \left[e^{-10}- e^0\right] = \frac{-1}{2}\left[[\frac{1}{e^2}]^5 - 1\right]$

$\displaystyle = 0.499$

$\displaystyle \int_0^{2\pi}\cos^5x\,\,dx$

$I=\int _{ 0 }^{ 2\pi }{ \cos ^{ 5 }{ x } } dx.......(i)\\ I=\int _{ 0 }^{ 2\pi }{ \cos ^{ 5 }{ (2\pi -x) } } dx\\ I=-\int _{ 0 }^{ 2\pi }{ \cos ^{ 5 }{ x } } dx.....(ii)$

Adding

$(i)$ and

$(ii)$

$\Rightarrow 2I=0\\ \Rightarrow I=0$

Write the value of $\displaystyle\int \dfrac{1-\sin x}{\cos^2x}dx$

$I=\int \dfrac{1-\sin x}{\cos^2x} dx$

$I=\int (\dfrac{1}{\cos^2x}-\dfrac{\sin x}{\cos^2x})dx$

$=\int \sec^2x dx-\int \sec x \tan x \ dx$

$=\tan x -\sec x +C$

$\sqrt{2}\displaystyle \int _{ 0 }^{ 2\pi }{ \sqrt { 1-\sin { x } } dx } =$

$\sqrt 2 \int_0^{2\pi } {\sqrt {1 - \sin x} } dx$

$= \mathop \smallint \nolimits^ \sqrt 2 \sin \left( {\frac{{2x - \pi }}{4}} \right){\mkern 1mu} {\rm{d}}x$

$= - {2^{\frac{3}{2}}}\cos \left( u \right)$

$= - {2^{\frac{3}{2}}}\cos \left( {\frac{{2x - \pi }}{4}} \right)$

$= \sqrt 2 \mathop \smallint \nolimits^ \sqrt {1 - \sin \left( x \right)} {\mkern 1mu} {\rm{d}}x$

$= - 4\cos \left( {\frac{{2x - \pi }}{4}} \right) + C$

$\sqrt 2 \int_0^{2\pi } {\sqrt {1 - \sin x} dx} = 8$

Prove that:
$\displaystyle \int_{0}^{\pi}\dfrac {xdx}{1+\sin x} = \pi$

$I=\displaystyle\int_0^{\pi}\left(\dfrac{x}{1+\sin x}\right)dx$

$I=\displaystyle\int_0^{\pi}\left[\dfrac{x}{1+\sin x}\right]dx$

$\left[\displaystyle\int_a^b f(x)dx=\displaystyle\int_a^b f(a+b-x)dx\right]$

$2I=\displaystyle\int_0^{\pi}\left(\dfrac{x}{1+\sin x}\right)dx$

$2I=\displaystyle\int_0^{\pi}\left[\dfrac{x}{1+\sin x}\right]dx$

$\left[\displaystyle\int_a^{2b}f(x)dx=2\int_a^bf(x)dx if f(x )=7(2b-x) \right]$

$I=\displaystyle\int_0^{\pi/2}\left(\dfrac{1}{1+\sin x}\right)dx$

$I=\displaystyle\int_0^{\pi/2}\left(\dfrac{1-\sin x}{\cos^2x}\right)dx$

$I=\displaystyle\int_0^{\pi/2}(\sec^2x-\tan x\sec x)dx$

$I=\pi [\tan x- \sec x]^{\pi/2}_0$

$I=\pi\left|\dfrac{\sin x-1}{\cos x}\right|^{\pi/2}_0$

$I=\pi\left\{\displaystyle\lim_{x\rightarrow \pi/2}\left(\dfrac{\sin x-1}{\cos x}\right)\left(\dfrac{0-1}{\cos 0}\right)\right\}$

$I=\pi\left\{\displaystyle\lim_{x\rightarrow \pi/2}\left(\dfrac{\cos x}{-\sin x}\right)-(-1)\right\}$

$I=\pi\left\{0+1\right\}$

$I=\pi$ .

1173018_1346787_ans_16ec191de5294914a2a5e91f4a67b447.jpg

Evaluate the following definite integral:

$\displaystyle\int_{4}^{12}x\ dx$

$\displaystyle\int_{4}^{12}x\ dx$

$\implies\left.\dfrac {x^2}2\right|_4^{12}$

$\implies\dfrac {12^2}2 -\dfrac {4^2}2$

$\implies\dfrac {144}2-\dfrac {16}2$

$\implies72-8=64$

Evaluate the following definite integral:
$\displaystyle\int_{0}^{\pi/2} \sin x \cos x\ dx$

consider,

$I=\displaystyle\int_{0}^{\pi/2} \sin x \cos x\ dx$

Let

$t=\sin x \implies dt=\cos x dx$

$x \to 0 \to \pi/2$

$t \to 0 \to 1$

$\Rightarrow I= \displaystyle \int _0^1 t \ dt$

$\Rightarrow$

$\left [\dfrac {t^2}2 \right] _0^1 =\dfrac 12$

Evaluate the following definate integral:
$\displaystyle\int_{0}^{2} 3x+2\ dx$

consider,

$I=\displaystyle\int_{0}^{2} 3x+2\ dx$

$I=\left[ 3\dfrac{x^2}2+2x \right]_0^2$

$I=6+4-0-0=10$

$\displaystyle \int_{0}^{1}\frac{dx}{(1+x)\sqrt{(2+x-x^{2})}}=\frac{1}{k}\sqrt{2}$ . Find the value of $k$ .

Let

$\displaystyle I=\int { \dfrac { 1 }{ \left( 1+x \right) \sqrt { 2+x-{ x }^{ 2 } } } } =\int { \dfrac { 1 }{ \sqrt { \dfrac { 9 }{ 4 } -{ \left( x-\dfrac { 1 }{ 2 } \right) }^{ 2 } } \left( x+1 \right) } dx }$

Substitute

$\displaystyle x-\dfrac { 1 }{ 2 } =t\Rightarrow dx=dt$

$\displaystyle I=\int { \dfrac { 1 }{ \left( t+\dfrac { 3 }{ 2 } \right) \sqrt { \dfrac { 9 }{ 4 } -{ t }^{ 2 } } } } dt$

Substitute

$\displaystyle t=\dfrac { 3 }{ 2 } \sin { u } \Rightarrow dt=\dfrac { 3\cos { u } }{ 2 } dx$

$\displaystyle \therefore I=\int { \dfrac { 1 }{ \dfrac { 3\sin { u } }{ 2 } +\dfrac { 3 }{ 2 } } } dt$

Substitute

$\displaystyle p=\tan { \dfrac { u }{ 2 } } \Rightarrow dp=\dfrac { 1 }{ 2 } \sec ^{ 2 }{ \dfrac { u }{ 2 } du }$

$\displaystyle \therefore I=\int { \dfrac { 4 }{ 3{ p }^{ 2 }+6p+3 } dp } =4\int { \dfrac { 1 }{ { \left( \sqrt { 3 } p+\sqrt { 3 } \right) }^{ 2 } } dp }$

$\displaystyle =-\dfrac { 4 }{ 3p+3 } =\dfrac { 2\left( x-2 \right) }{ 3\sqrt { -{ x }^{ 2 }+x+2 } }$

$\displaystyle \therefore \int _{ 0 }^{ 1 }{ \dfrac { 1 }{ \left( 1+x \right) \sqrt { 2+x-{ x }^{ 2 } } } dx } =\dfrac { \sqrt { 2 } }{ 3 }$

Integrate $\displaystyle\int _{ 0 }^{ 1 }{ \sin ^{ -1 }{ \left( \dfrac { 2x }{ 1+{ x }^{ 2 } } \right) dx } }$

Let,

$I = \int_0^1 {si{n^{ - 1}}\left( {\dfrac{{2x}}{{1 + {x^2}}}} \right)dx}$

Let

$x=tan{\theta}$

$dx=sec^2{\theta}d{\theta}$

When,

$x=0, {\theta}=0$ &

$x=1, {\theta}=\frac{\pi}{4}$

Now,

$I = \int_0^{\frac{\pi }{4}} {2\theta .{{\sec }^2}\theta .d\theta }$

Integrating by parts , Taking

$sec^2{\theta}$ as a first function.

$=\dfrac{\pi}{2}-\log 2$

Evaluate: $\displaystyle \int _{ 0 }^{ 1 }{ \frac { { x }^{ 4 }{ \left( 1-x \right) }^{ 4 } }{ 1+{ x }^{ 2 } } } dx$

Consider the given integral.

$I=\int_{0}^{1}{\dfrac{{{x}^{4}}{{\left( 1-x \right)}^{4}}}{1+{{x}^{2}}}}dx$

$I=\int_{0}^{1}{\dfrac{{{x}^{4}}{{\left( 1-x \right)}^{2}}{{\left( 1-x \right)}^{2}}}{1+{{x}^{2}}}}dx$

$I=\int_{0}^{1}{\dfrac{{{x}^{4}}\left( 1+{{x}^{2}}-2x \right)\left( 1+{{x}^{2}}-2x \right)}{1+{{x}^{2}}}}dx$

$I=\int_{0}^{1}{\dfrac{{{x}^{4}}\left( 1+{{x}^{2}}-2x+{{x}^{2}}+{{x}^{4}}-2{{x}^{3}}-2x-2{{x}^{3}}+4{{x}^{2}} \right)}{1+{{x}^{2}}}}dx$

$I=\int_{0}^{1}{\dfrac{{{x}^{4}}\left( 1+6{{x}^{2}}+{{x}^{4}}-4{{x}^{3}}-4x \right)}{1+{{x}^{2}}}}dx$

$I=\int_{0}^{1}{\dfrac{\left( {{x}^{4}}+6{{x}^{6}}+{{x}^{8}}-4{{x}^{7}}-4{{x}^{5}} \right)}{1+{{x}^{2}}}}dx$

$I=\int_{0}^{1}{\dfrac{\left( {{x}^{8}}-4{{x}^{7}}+6{{x}^{6}}-4{{x}^{5}}+{{x}^{4}} \right)}{{{x}^{2}}+1}}dx$

$I=\int_{0}^{1}{\left( {{x}^{6}}-4{{x}^{5}}+5{{x}^{4}}-4{{x}^{2}}+4-\dfrac{4}{1+{{x}^{2}}} \right)}dx$

$I=\left[ \dfrac{{{x}^{7}}}{7}-\dfrac{4{{x}^{6}}}{6}+\dfrac{5{{x}^{5}}}{5}-\dfrac{4{{x}^{3}}}{3}+4x-4{{\tan }^{-1}}x \right]_{0}^{1}$

$I=\left[ \dfrac{1}{7}-\dfrac{4}{6}+\dfrac{5}{5}-\dfrac{4}{3}+4-4{{\tan }^{-1}}\left( 1 \right)-\left[ 0 \right] \right]$

$I=\left[ \dfrac{1}{7}-\dfrac{2}{3}+1-\dfrac{4}{3}+4-4{{\tan }^{-1}}\left( \tan \dfrac{\pi }{4} \right) \right]$

$I=\left[ \dfrac{1}{7}-\dfrac{2}{3}-\dfrac{4}{3}+5-\dfrac{4\pi }{4} \right]$

$I=\left[ \dfrac{1}{7}-2+5-\pi \right]$

$I=\left[ \dfrac{1}{7}-3-\pi \right]$

$I=-\left( \dfrac{20}{7}+\pi \right)$

Hence, this is the answer.

$\displaystyle\int^1_0\cot^{-1}(1+x^2-x)dx$ .

$\begin{array}{l} \int _{ 0 }^{ 1 }{ { { \tan }^{ -1 } } }(\frac { { x+\left( { 1-x } \right) } }{ { 1+{ x^{ 2 } }-x } } )dx \\ \int _{ 0 }^{ 1 }{ { { \tan }^{ -1 } } }(\frac { { x-\left( { -1+x } \right) } }{ { 1+x\left( { x+1 } \right) } } )dx \\ \int _{ 0 }^{ 1 }{ \left[ { { { \tan }^{ -1 } }x-{ { \tan }^{ -1 } }\left( { x-1 } \right) } \right] }dx \\ \int _{ 0 }^{ 1 }{ { { \tan }^{ -1 } }xdx }-\int _{ 0 }^{ 1 }{ { { \tan }^{ -1 } } }\left( { x-1 } \right) dx \\ \left[ { \frac { 1 }{ { 1+{ x^{ 2 } } } } } \right] _{ 0 }^{ 1 }+\left[ { \frac { 1 }{ { \left[ { 1+\left( { x-1 } \right) } \right] } } } \right] _{ 0 }^{ 1 } \\ \left( { \frac { 1 }{ 2 } -1 } \right) -\left( { 1-\frac { 1 }{ 2 } } \right) \\ -\frac { 1 }{ 2 } -\frac { 1 }{ 2 } =-1 \end{array}$

Prove that $\displaystyle\int^{\tan x}_{1/e}\dfrac{t}{1+t^2}dt+\displaystyle\int^{\cot x}_{1/e}\dfrac{1}{t(1+t^2)}dt=1$ .

To prove

$\int _{ \cfrac { 1 }{ e } }^{ \tan { x } }{ \cfrac { t }{ 1+{ t }^{ 2 } } dt } +\int _{ \cfrac { 1 }{ e } }^{ \cot { x } }{ \cfrac { 1 }{ t(1+{ t }^{ 2 }) } dt }$

Let

${ I }_{ 1 }=\int _{ \cfrac { 1 }{ e } }^{ \tan { x } }{ \cfrac { t }{ 1+{ t }^{ 2 } } dt } \\ { I }_{ 2 }=\int _{ \cfrac { 1 }{ e } }^{ \cot { x } }{ \cfrac { 1 }{ t(1+{ t }^{ 2 }) } dt }$

and

$I={ I }_{ 1 }+{ I }_{ 2 }$

also,

$\int _{ \cfrac { 1 }{ e } }^{ \cot { x } }{ \cfrac { 1 }{ t(1+{ t }^{ 2 }) } dt }$

Let

$t=\cfrac { 1 }{ z }$

$dt=\cfrac { -1 }{ { z }^{ 2 } } dz$

So,

${ I }_{ 1 }=\int _{ e }^{ \tan { x } }{ \cfrac { -zdz }{ { z }^{ 2 }(1+\cfrac { 1 }{ { z }^{ 2 } } ) } } =\int _{ \tan { x } }^{ e }{ \cfrac { zdz }{ 1+{ z }^{ 2 } } }$

So,

${ I }_{ 2 }=\int _{ \tan { x } }^{ e }{ \cfrac { tdt }{ 1+{ t }^{ 2 } } }$

So,

$I={ I }_{ 1 }+{ I }_{ 2 }$

$I=\int _{ \cfrac { 1 }{ e } }^{ e }{ \cfrac { tdt }{ 1+{ t }^{ 2 } } } \\ =\cfrac { 1 }{ 2 } \int _{ \cfrac { 1 }{ e } }^{ e }{ \cfrac { 2t }{ 1+{ t }^{ 2 } } dt } \\ I=\cfrac { 1 }{ 2 } { \log { (1+{ t }^{ 2 }) } }_{ \cfrac { 1 }{ e } }^{ e }\\ I=\cfrac { 1 }{ 2 } \log { (1+{ e }^{ 2 }) } -\cfrac { 1 }{ 2 } \log { (1+\cfrac { 1 }{ { e }^{ 2 } } ) } \\ I=\cfrac { 1 }{ 2 } [\log { (\cfrac { 1+{ e }^{ 2 } }{ 1+\cfrac { 1 }{ { e }^{ 2 } } } ) } ]\\ I=\cfrac { 1 }{ 2 } \log { { e }^{ 2 } } \\ I=\cfrac { 1 }{ 2 } \times 2\\ I=1$

Evaluate:
$\int _ { 0 } ^ { \pi / 4 } \dfrac { \sin x + \cos x } { 9 + 16 \sin 2 x } d x$

$\displaystyle \int_{0}^{\pi/4} \dfrac{sinx+cosx}{9+16sin2x}dx$

express deno. in terms of sinx-cosx.

$sin 2x = 1-(sinx-cosx)^{2}$

$\displaystyle \Rightarrow \int_{0}^{\pi/4} \dfrac{sinx+cosx}{9+16(1-(sinx-cosx)^{2})}dx$

$\displaystyle \Rightarrow \int_{0}^{\pi/4} \dfrac{sinx+cosx}{25-16(sinx-cosx^{2})}dx$

Put

$sinx - cosx = t$

$\displaystyle I = \int_{-1}^{0}\dfrac{dt}{25-16t^{2}}$

$\displaystyle = \frac{1}{16} \times \dfrac{1}{2.\dfrac{5}{4}}[log\dfrac{5/4+t}{5/4-t}]_{-1}^{0}$

$= \dfrac{1}{40}log 9.$

Evaluate:
$\displaystyle \int\limits_2^0 {\dfrac{1}{{\sqrt {4 - x^2} }}} dx$

1353297_1270642_ans_b8aa8492c8844000812c3ac00ff12a7b.PNG

EVALUATE
$\int_{2}^{3} \frac{1}{x+5} d x$

$\displaystyle\int ^{3}_{2}\dfrac{1}{x+5}d x=[\ln |x+5|]^3_2=\ln |3+5|-\ln |2+5|=\ln 8-\ln 7=\ln \dfrac{8}{7}$

Evaluate the definite integral:
$\displaystyle\int_{0}^{1}\dfrac{1-x^{2}}{(1+x^{2})^{2}}dx$

$I=\displaystyle\int_{0}^{1}\dfrac{1-x^{2}}{(1+x^{2})^{2}}dx$

Dividing by

$x^2$ in both numerator and denominator

$I=\displaystyle\int_{0}^{1}\dfrac{\dfrac{1} {x^{2}}-1}{\left(x+\dfrac{1}{x}\right)^{2}}\ dx$

Let

$x+\dfrac{1}{x}=t$ .

Then,

$d\left(x+\dfrac{1}{x}\right)=dt\Rightarrow \left(1-\dfrac{1}{x^{2}}\right)dx=dt$

Clearly,

$x=0\Rightarrow t=\infty$

$x=1\Rightarrow t=2$

$\therefore \displaystyle\int_{\infty}^{2}-\dfrac{1}{t^{2}}dt=\left[\dfrac{1}{t}\right]_{\infty}^{2}=\dfrac{1}{2}$

Evaluate: $\displaystyle\int \dfrac {1}{2x+3}dx$

Let

$t=2x+3 \implies dt=2 dx$

$\Rightarrow \displaystyle \int \dfrac 1{2t }dt$

$\Rightarrow \dfrac12 \log t$

$\Rightarrow \dfrac12[\log 2x+3]$

Evaluate the following integrals:
$\int { \sqrt { 2{ x }^{ 2 }+3x+4 } } dx$

Let

$I=\displaystyle\int \sqrt{2 x^2+3 x+4} d x$

$=\displaystyle\int \sqrt{2\left(x^2+2(x)\bigg(\dfrac{3}{4}\bigg)+2\right)}$

$=\sqrt{2}\displaystyle\int \sqrt{x^2+2(x)\bigg(\dfrac{3}{4}\bigg)+\bigg(\dfrac{3}{4}\bigg)^2-\bigg(\dfrac{3}{4}\bigg)^2+2}\ d x$

$=\sqrt{2}\displaystyle\int \sqrt{\bigg(x+\dfrac{3}{4}\bigg)^2+\dfrac{23}{16}}\ d x$

$(\because (a+b)^2=a^2+2 a b+b^2)$

Put

$t=x+\dfrac{3}{4}\implies d t=d x$

$I=\sqrt{2}\displaystyle\int \sqrt{t^2+\bigg(\dfrac{\sqrt{23}}{4}\bigg)^2}\ d t$

As we know that

$\displaystyle\int \sqrt{a^2+x^2} d x=\dfrac{x}{2}\sqrt{x^2+a^2}+\dfrac{a^2}{2}\ln |x+\sqrt{x^2+a^2}|+C$

Here

$a=\dfrac{\sqrt{23}}{4}$

$\implies I=\sqrt{2}\left(\dfrac{t}{2}\sqrt{t^2+\dfrac{23}{16}}+\dfrac{23/16}{2}\ln \left|t+\sqrt{t^2+\dfrac{23}{16}}\right|+C\right)$

$\implies I=\dfrac{1}{2}\bigg(x+\dfrac{3}{4}\bigg)\sqrt{2\bigg(x+\dfrac{3}{4}\bigg)^2+\dfrac{23}{16}}+\dfrac{23\sqrt{2}}{32}\ln \left|x+\dfrac{3}{4}+\sqrt{\bigg(x+\dfrac{3}{4}\bigg)^2+\dfrac{23}{16}}\ \right|+C$

$\implies I=\dfrac{1}{8}(4 x+3)\sqrt{2 x^2+3 x+4}+\dfrac{23\sqrt{2}}{32}\ln \left|x+\dfrac{3}{4}+\sqrt{x^2+\dfrac{3}{2} x+2}\ \right|+C$

Evaluate the following integral:
$\int { \left( x+1 \right) } \sqrt { 2{ x }^{ 2 }+3 } dx$

Let

$I=\displaystyle\int (x+1)\sqrt{2 x^2+3}\ d x$

$\implies I=\displaystyle\int (x+1)\sqrt{2\bigg(x^2+\dfrac{3}{2}\bigg)}\ d x$

$\implies I=\sqrt{2}\displaystyle\int (x+1)\sqrt{x^2+\dfrac{3}{2}}\ d x$

$\implies I=\sqrt{2}\displaystyle\int x\sqrt{x^2+\dfrac{3}{2}}\ d x+\sqrt{2}\displaystyle\int \sqrt{x^2+\bigg(\sqrt{\dfrac{3}{2}}\bigg)^2}\ d x$

As we know that

$\displaystyle\int \sqrt{a^2+x^2} d x=\dfrac{x}{2}\sqrt{x^2+a^2}+\dfrac{a^2}{2}\ln |x+\sqrt{x^2+a^2}|+C$

$\implies I=\sqrt{2}\displaystyle\int x\sqrt{x^2+\dfrac{3}{2}}\ d x+\sqrt{2}\left(\dfrac{x}{2}\sqrt{x^2+\dfrac{3}{2}}+\dfrac{3/2}{2}\ln \bigg| x+\sqrt{x^2+\dfrac{3}{2}}\ \bigg|\right)+C$

Put

$x^2+\dfrac{3}{2}=y\implies 2 x d x=d y\implies x d x=\dfrac{dy}{2}$

$\implies I=\sqrt{2}\displaystyle\int \sqrt{y}\times \dfrac{d y}{2}+\dfrac{x}{\sqrt{2}}\sqrt{x^2+\dfrac{3}{2}}+\dfrac{3}{2\sqrt{2}}\ln \bigg| x+\sqrt{x^2+\dfrac{3}{2}}\ \bigg|+C$

$\implies I=\dfrac{1}{\sqrt{2}}\displaystyle\int \sqrt{y}\ d y+\dfrac{x}{\sqrt{2}}\sqrt{x^2+\dfrac{3}{2}}+\dfrac{3}{2\sqrt{2}}\ln \bigg| x+\sqrt{x^2+\dfrac{3}{2}}\ \bigg|+C$

$\implies I=\dfrac{1}{\sqrt{2}}\times \dfrac{y^{3/2}}{3/2}+\dfrac{x}{\sqrt{2}}\sqrt{x^2+\dfrac{3}{2}}+\dfrac{3}{2\sqrt{2}}\ln \bigg| x+\sqrt{x^2+\dfrac{3}{2}}\ \bigg|+C$

$\left(\because \displaystyle\int x^n\ d x=\dfrac{x^{n+1}}{n+1}+c\right)$

$\implies I=\dfrac{\sqrt{2}}{3}\ y^{3/2}+\dfrac{\sqrt{2}x}{2}\sqrt{x^2+\dfrac{3}{2}}+\dfrac{3}{2\sqrt{2}}\ln \bigg| x+\sqrt{x^2+\dfrac{3}{2}}\ \bigg|+C$

$\implies I=\dfrac{\sqrt{2}}{3}\ \left(x^2+\dfrac{3}{2}\right)^{3/2}+\dfrac{\sqrt{2}x}{2}\sqrt{x^2+\dfrac{3}{2}}+\dfrac{3}{2\sqrt{2}}\ln \bigg| x+\sqrt{x^2+\dfrac{3}{2}}\ \bigg|+C$

$\left(\because y=x^2+\dfrac{3}{2}\right)$

$\implies I=\dfrac{\sqrt{2}}{3}\ \left(\dfrac{2 x^2+3}{2}\right)^{3/2}+\dfrac{x}{2}\sqrt{2 x^2+3}+\dfrac{3}{2\sqrt{2}}\ln \bigg| x+\sqrt{x^2+\dfrac{3}{2}}\ \bigg|+C$

$\implies I=\dfrac{1}{6}\ \left(2 x^2+3\right)^{3/2}+\dfrac{x}{2}\sqrt{2 x^2+3}+\dfrac{3}{2\sqrt{2}}\ln \bigg| x+\sqrt{x^2+\dfrac{3}{2}}\ \bigg|+C$

Evaluate the following integrals:
$\int { \sqrt { 2ax-{ x }^{ 2 } } } dx\quad$

Let

$I=\displaystyle\int \sqrt{2 a x-x^2}\ d x$

$\implies I=\displaystyle\int \sqrt{-(-2(a)(x)+x^2)}\ d x$

$\implies I=\displaystyle\int \sqrt{a^2-(a^2-2(a)(x)+x^2}\ d x$

$\implies I=\displaystyle\int \sqrt{a^2-(a-x)^2}\ d x$

Put

$t=a-x\implies d t=-d x$

$\implies I=-\displaystyle\int \sqrt{a^2-t^2}\ d t$

As we know that

$\displaystyle\int \sqrt{a^2-x^2}\ d x=\dfrac{x}{2}\sqrt{a^2-x^2}+\dfrac{a^2}{2}\text{sin}^{-1} \left(\dfrac{x}{a}\right)+C$

$I=-\dfrac{t}{2}\sqrt{a^2-t^2}-\dfrac{a^2}{2}\text{sin}^{-1} \left(\dfrac{t}{a}\right)+C$

$I=-\dfrac{a-x}{2}\sqrt{a^2-(a-x)^2}-\dfrac{a^2}{2}\text{sin}^{-1} \left(\dfrac{a-x}{a}\right)+C$

$I=\dfrac{1}{2}(x-a)\sqrt{2 a x-x^2}-\dfrac{a^2}{2}\text{sin}^{-1} \left(\dfrac{a-x}{a}\right)+C$

Evaluate the following definite integral:
$\displaystyle\int_{0}^{\pi/4}\sin^{3}2t\cos 2t\ dt$ .

Consider,

$I=\displaystyle\int_{0}^{\pi/4}\sin^{3}2t\cos 2t\ dt$

Let

$\sin 2t=u$ .

Then,

$d(\sin 2t)=du$

$\Rightarrow 2\cos 2t\ dt=du$

$\Rightarrow \cos 2t dt=\dfrac{1}{2}du$

Also,

$t=0\Rightarrow u=\sin 0=0$

$t=\dfrac{\pi}{4}$

$\Rightarrow u=\sin\dfrac{\pi}{2}=1$

$\therefore I=\dfrac{1}{2}\displaystyle\int_{0}^{1}u^{3}du$

$I=\dfrac{1}{8}\left[u^{4}\right]_{0}^{1}=\dfrac{1}{8}$

Evaluate the following integral:
$\displaystyle\int^2_0x\sqrt{2-x}dx$ .

1548009_1706409_ans_ee11ccf7842548df99ee6e8d91cd53db.jpg

Evaluate the following integral:
$\displaystyle\int^9_0\dfrac{dx}{(1+\sqrt{x})}$ .

1548623_1706440_ans_1a2af81ff3da463dbbb5ee56f765851e.jpg

Evaluate the following integral:
$\displaystyle\int^3_2\dfrac{(2-x)}{\sqrt{5x-6-x^2}}dx$ .

1548628_1706450_ans_0b7eb7ecd8ef425e87a283aafc1259e6.jpg

Evaluate the following integral:
$\displaystyle\int^a_0\dfrac{x}{\sqrt{a^2+x^2}}dx$ .

1548008_1706408_ans_668d63b38a7d4e62abcbbf5702c12e88.jpg

Evaluate the following integral:
$\displaystyle\int^2_1\dfrac{dx}{(x+1)\sqrt{x^2-1}}$ .

1548626_1706445_ans_0c04428214c7465c87342ae3e304f78c.jpg

Evaluate the following integral:
$\displaystyle\int^1_0x^3\sqrt{1+3x^4}dx$ .

1546857_1706441_ans_9ec97e3304b94dee9076cf63d5a4d806.jpg

Evaluate the following integral:
$\displaystyle\int^a_0\dfrac{x^4}{\sqrt{a^2-x^2}}dx$ .

1548006_1706407_ans_7cbfdb6903e4402b97fee10092688f13.jpg

Evaluate the following integral:
$\displaystyle\int^1_0\dfrac{(1-x^2)}{(1+x^2)^2}dx$ .

$\therefore I=\dfrac12$
1548625_1706444_ans_06e905af0bb24e839eef368784f9fd8c.jpg

Prove that $\displaystyle\int^4_1\dfrac{\sqrt{x}}{(\sqrt{5-x}+\sqrt{x})}dx=\dfrac{3}{2}$ .

1548747_1706591_ans_71e83b25ba9846c59774ec55dabf0ac6.jpg

Prove that $\displaystyle\int^1_0x(1-x)^5dx=\dfrac{1}{42}$ .

1546876_1706527_ans_aa38366ff8964ef68f0fe50d5e81be85.jpg

Prove that $\displaystyle\int^{\infty}_0\dfrac{x}{(1+x)(1+x^2)}dx=\dfrac{\pi}{4}$ .

1548816_1706554_ans_734f2bd1b54341b3a87ef641bc38ad8e.jpg

Prove that $\displaystyle\int^{3a/4}_{a/4}\dfrac{\sqrt{x}}{(\sqrt{a-x}+\sqrt{x})}dx=\dfrac{a}{4}$ .

1564749_1706587_ans_91f130031cab4805b774f5c9c8b59594.png

Prove that $\displaystyle\int^8_0|x-5|dx=17$ .

1549298_1706610_ans_caa28685b8d149898fac0e2943a8bcf9.jpg

Prove that $\displaystyle\int^a_0\dfrac{\sqrt{x}}{(\sqrt{x}+\sqrt{a-x})}dx=\dfrac{a}{2}$ .

1548822_1706558_ans_32e7ce247af74b30a5cdbd11bd2809b4.jpg

Prove that $\displaystyle\int^a_0\dfrac{dx}{x+\sqrt{a^2-x^2}}=\dfrac{\pi}{4}$ .

1548819_1706556_ans_a30516ff255e428f9d08334b579dbde7.jpg

Prove that $\displaystyle\int^2_0x\sqrt{2-x}dx=\dfrac{16\sqrt{2}}{15}$ .

1546883_1706530_ans_302bf98eef7c433791acdd4115f33839.jpg

Prove that $\displaystyle\int^2_{-2}|x+1|dx=5$ .

1564788_1706608_ans_42d8688e808d46ada7da35b4838f5622.png

The value of the integral $9999\displaystyle \int_{0}^{\infty }\displaystyle \frac{dx}{\left ( x+\sqrt{1+x^{2}} \right )^{100}}$ is

Let

$\displaystyle I=9999\int _{ 0 }^{ \infty } \frac { dx }{ \left( x+\sqrt { 1+x^{ 2 } } \right) ^{ 100 } }$

Put

$\log { \left( x+\sqrt { 1+x^{ 2 } } \right) } =t$

$\Rightarrow \left( x+\sqrt { 1+x^{ 2 } } \right) ={ e }^ t$

$\Rightarrow \ { { x }^{ 2 }+1 } ={ e }^{ 2t }-2x{ e }^{ t }+{ x }^{ 2 }$

$\displaystyle \therefore x=\frac { { e }^{ 2t }-1 }{ 2{ e }^{ t } } =\frac { { e }^{ t }+{ e }^{ -t } }{ 2 }$

$\Rightarrow dx=\dfrac { { e }^{ t }-{ e }^{ -t } }{ 2 } dt$

when

$x\rightarrow 0,t\rightarrow 0$ and

$x\rightarrow \infty ,t\rightarrow \infty$

$\displaystyle \therefore I=9999\int _{ 0 }^{ \infty }{ \left( \frac { { e }^{ t }+{ e }^{ -t } }{ 2{ e }^{ 100t } } \right) dt } =\frac { 9999 }{ 2 } \int _{ 0 }^{ \infty }{ \left( { e }^{ -99t }+{ e }^{ -101t } \right) dt }$

$\displaystyle =\frac { 9999 }{ 2 } \lim _{ a\rightarrow \infty }{ { \left( \frac { { e }^{ -99t } }{ -99 } -\frac { { e }^{ -101t } }{ 101 } \right) }_{ 0 }^{ a } }$

$\displaystyle =\frac { 9999 }{ 2 } \lim _{ a\rightarrow \infty }{ \left( \frac { 1 }{ -99{ e }^{ 99a } } -\frac { 1 }{ 101{ e }^{ 101a } } -\frac { 1 }{ -99 } +\frac { 1 }{ 101 } \right) }$

$\displaystyle =\frac { 9999 }{ 2 } \left( \frac { 1 }{ 99 } +\frac { 1 }{ 101 } \right) =100$

Let $\displaystyle I=\int_{0}^{1}\frac{dx}{\sqrt{4-x^{2}-x^{3}}}$ and $\displaystyle I_{1}=\int_{0}^{1/2}\frac{dx}{\sqrt{1-x^{4}}}$

$0\leq x\leq 1\Rightarrow 4-x^{ 2 }\geq 4-x^{ 2 }-x^{ 3 }\geq 4-2x^{ 2 }\Rightarrow \int _{ 0 }^{ 1 } \frac { dx }{ \sqrt { 4-x^{ 2 } } } \leq I\leq \int _{ 0 }^{ 1 } \frac { dx }{ \sqrt { 4-2x^{ 2 } } }$

$\int _{ 0 }^{ 1 } \frac { dx }{ \sqrt { 4-x^{ 2 } } } =\left. \sin ^{ -1 } \frac { x }{ 2 } \right| _{ 0 }^{ 1 }=\frac { \pi }{ 6 } \\ \int _{ 0 }^{ 1 } \frac { dx }{ \sqrt { 4-2x^{ 2 } } } =\frac { 1 }{ \sqrt { 2 } } \int _{ 0 }^{ 1 } \frac { dx }{ \sqrt { 2-x^{ 2 } } } =\left. \frac { 1 }{ \sqrt { 2 } } \sin ^{ -1 } \frac { x }{ \sqrt { 2 } } \right| _{ 0 }^{ 1 }=\frac { \pi }{ 4\sqrt { 2 } }$
And as

$0\leq x\leq 1/2\Rightarrow \sqrt { 1-x^{ 2 } } \leq \sqrt { 1-x^{ 4 } } \leq 1$

$\Rightarrow \frac { 1 }{ 2 } =\int _{ 0 }^{ 1/2 } 1dx\leq I_{ 1 }\leq \int _{ 0 }^{ 1/2 } \frac { dx }{ \sqrt { 1-x^{ 2 } } } =\frac { \pi }{ 6 }$
A)

$I<\frac { \pi }{ 4\sqrt { 2 } } <\frac { \pi }{ 4 } <1$
B)

$I>\frac { \pi }{ 6 } >\frac { 1 }{ 2 } >\frac { 1 }{ 4 }$
C)

${ I }_{ 1 }<\frac { \pi }{ 6 } <\frac { \pi }{ 4 } <1$
D)

${ I }_{ 1 }>\frac { 1 }{ 2 } >\frac { 1 }{ 4 }$

Find : $\displaystyle \int_{0}^{\tfrac {\pi}{2}} \dfrac {dx}{4 + 5\cos x} dx$

Let

$I = \displaystyle \int _0^{\tfrac{\pi}{2}} \cfrac{dx}{4 + 5\cos x}$

Substituting

$\cos x = \cfrac{1 - \tan^2{\dfrac{x}{2}}}{1 + \tan^2{\dfrac{x}{2}}}$ , we have

$I = \displaystyle \int _0^{\tfrac{\pi}{2}} \cfrac{\left(1 + \tan^2{\cfrac{x}{2}}\right)dx}{9 - \tan^2{\cfrac{x}{2}}}$

Let

$t = \tan{\cfrac{x}{2}}$

$\Rightarrow 2dt = \left(1 + \tan^2{\cfrac{x}{2}}\right) dx$

$\therefore I = \displaystyle \int _0^{1} \cfrac{2dt}{9 - t^2}$

$\therefore I = \displaystyle \int _{0}^{1} \cfrac{(3 + t + 3 - t)}{3(3 + t)(3 - t)}$

$= \displaystyle \int _0^{1} \cfrac{dt}{3(3 - t)} + \displaystyle \int _0^{1} \cfrac{dt}{3(3 + t)}$

$= [\ln(3 - t)]_0^{1} \times \cfrac{-1}{3} + [\ln(3 + t)]_0^{1} \times \cfrac{1}{3}$

$= (\ln 3 - \ln 2 + \ln 4 - \ln 3) \times \cfrac{1}{3}$

$= \cfrac{1}{3} \times \ln 2$

$\int _{ 0 }^{ \pi /4 }{ \frac { (sinx+cosx) }{ 9+16sin2x } } dx$

$I=\displaystyle\int_{0}^{\frac{\pi}{4}}{\dfrac{\sin{x}+\cos{x}}{9+16\sin{2x}}dx}$

Let

$\sin{x}-\cos{x}=t$

$\left(\cos{x}+\sin{x}\right)dx=dt$

Again

${\left(\sin{x}-\cos{x}\right)}^{2}={t}^{2}$

$\Rightarrow {\sin}^{2}{x}+{\cos}^{2}{x}-2\sin{x}\cos{x}={t}^{2}$

$\Rightarrow 1-\sin{2x}={t}^{2}$

$\Rightarrow \sin{2x}=1-{t}^{2}$

When

$x=0\Rightarrow t=\sin{0}-\cos{0}=-1$

When

$x=\dfrac{\pi}{4}\Rightarrow t=\sin{\dfrac{\pi}{4}}-\cos{\dfrac{\pi}{4}}=\dfrac{1}{\sqrt{2}}-\dfrac{1}{\sqrt{2}}=0$

$\therefore I=\displaystyle\int_{-1}^{0}{\dfrac{dt}{9+16\left(1-{t}^{2}\right)}}$

$=\displaystyle\int_{-1}^{0}{\dfrac{dt}{9+16-16{t}^{2}}}$

$=\displaystyle\int_{-1}^{0}{\dfrac{dt}{25-16{t}^{2}}}$

$=\dfrac{1}{16}\displaystyle\int_{-1}^{0}{\dfrac{dt}{\dfrac{25}{16}-{t}^{2}}}$

$=\dfrac{1}{16}\left[\dfrac{1}{2\times\dfrac{5}{4}}\log{\left|\dfrac{\dfrac{5}{4}+t}{\dfrac{5}{4}-t}\right|}\right]_{-1}^{0}$

$=\dfrac{1}{4}\left[\dfrac{1}{10}\log{\left|\dfrac{5+4t}{5-4t}\right|}\right]_{-1}^{0}$

$=\dfrac{1}{40}\left[\log{\left|\dfrac{5+0}{5-0}\right|}-\log{\left|\dfrac{5-4}{5+4}\right|}\right]$

$=\dfrac{1}{40}\left[\log{1}-\log{\left(\dfrac{1}{9}\right)}\right]$

$=\dfrac{1}{40}\left[0+\log{9}\right]$

$=\dfrac{\log{9}}{40}$

$\int^2_1 \dfrac{x}{\sqrt{2x^2+1}} dx$

$\displaystyle\int_{1}^{2}{\dfrac{xdx}{\sqrt{2{x}^{2}+1}}}$

$=\dfrac{1}{4}\displaystyle\int_{1}^{2}{\dfrac{4xdx}{\sqrt{2{x}^{2}+1}}}$

Let

$t=2{x}^{2}+1\Rightarrow dt=4xdx$

$=\dfrac{1}{4}\displaystyle\int_{1}^{2}{\dfrac{dt}{\sqrt{t}}}$

$=\dfrac{1}{4}\displaystyle\int_{1}^{2}{{t}^{\frac{-1}{2}}dt}$

$=\dfrac{1}{4}\left[\dfrac{{t}^{\frac{-1}{2}+1}}{\dfrac{-1}{2}+1}\right]_{1}^{2}$

$=\dfrac{2}{4}\left[\sqrt{t}\right]_{1}^{2}$

$=\dfrac{1}{2}\left(\sqrt{2}-1\right)$

Evaluate: $\int_{1}^{\sqrt{3}} \frac{d x}{1+x^{2}}$

2040901_1453406_ans_4c8ff73261304a4d89d036f06b10a3b3.jpg

Evaluate: $\displaystyle \int_{0}^{\frac{\pi}{2}} \dfrac{\sin x \cdot \cos x}{1+\sin^4 x}\cdot dx$ .

$\displaystyle \overset{\pi/2}{\underset{0}{\int}} \frac{\sin x \cdot \cos x}{1 + \sin^4 x} \cdot dx$ .

Let

$\sin^2 n = t$

$2 \sin x \,\cos x \,dx = dt$

When

$x = 0, t = 0$

$x = \dfrac{\pi}{2}, t = 1$

$= \dfrac{1}{2} \displaystyle \overset{dt/2}{\underset{1 + t^2}{\int}} \frac{dt}{1 + t^2}$

$= \dfrac{1}{2} \displaystyle \overset{1}{\underset{0}{\int}} \frac{dt}{1 + t^2}$

$= \dfrac{1}{2} [\tan^{-1} - \tan^{-1} 0]$

$= \dfrac{1}{2} \left[\dfrac{\pi}{4} - 0 \right]$

$= \dfrac{\pi}{8}$

If the value of the definite integral $\int_{0}^{207} C_{7} x^{200} \cdot(1-x)^{7} d x$ is equal to $\dfrac{1}{k}$ where $k \in N\\$ , thenthe value of $k / 26$ is

(8) Let

$I=\int_{0}^{1} 207 C_{7} \cdot \underbrace{x^{200}}_{I I} \cdot \underbrace{(1-x)^{7}}_{I} d x\\$

$\begin{aligned}I=^{207} C_{7}[\left.\underbrace{(1-x)^{7} \cdot \dfrac{x^{201}}{201}}\right|_{0} ^{1}&\left.+\dfrac{7}{201} \int_{0}^{1}(1-x)^{6} \cdot x^{201} d x\right] \\&=^{207} C_{7}: \dfrac{7}{201} \int_{0}^{1}(1-x)^{6} \cdot x^{201} d x\end{aligned}\\$
Integrating by parts again 6 more times

$\begin{array}{l}=^{207} \mathrm{C}_{7} \cdot \dfrac{7 !}{201.202 .203 .204 .205 .206 .207} \int_{0}^{1} x^{207} d x \\=\dfrac{(207) !}{7 !(200) !} \cdot \dfrac{7 !}{201.202 \cdots 207} \cdot \dfrac{1}{208} \\=\dfrac{(207) !}{(207) ! 7 !} \cdot \dfrac{7 !}{208}=\dfrac{1}{208}=\dfrac{1}{k} \Rightarrow k=208\end{array}\\$

Integrals - Class 12 Engineering Maths - Extra Questions

The value of the definite integral, I=∫√100xex2dxI = \int _{ 0 }^{ \sqrt { 10 } }{ \dfrac { x }{ { e }^{ { x }^{ 2 } } } } dx is equal to

∫2π0cos5xdx\displaystyle \int_0^{2\pi}\cos^5x\,\,dx

Write the value of ∫1−sinxcos2xdx\displaystyle\int \dfrac{1-\sin x}{\cos^2x}dx

√2∫2π0√1−sinxdx=\sqrt{2}\displaystyle \int _{ 0 }^{ 2\pi }{ \sqrt { 1-\sin { x } } dx } =

Prove that:∫π0xdx1+sinx=π\displaystyle \int_{0}^{\pi}\dfrac {xdx}{1+\sin x} = \pi

Evaluate the following definite integral:∫124x dx\displaystyle\int_{4}^{12}x\ dx

Evaluate the following definite integral:∫π/20sinxcosx dx\displaystyle\int_{0}^{\pi/2} \sin x \cos x\ dx

Evaluate the following definate integral:∫203x+2 dx\displaystyle\int_{0}^{2} 3x+2\ dx

∫10dx(1+x)√(2+x−x2)=1k√2\displaystyle \int_{0}^{1}\frac{dx}{(1+x)\sqrt{(2+x-x^{2})}}=\frac{1}{k}\sqrt{2}. Find the value of kk.

Integrate ∫10sin−1(2x1+x2)dx\displaystyle\int _{ 0 }^{ 1 }{ \sin ^{ -1 }{ \left( \dfrac { 2x }{ 1+{ x }^{ 2 } } \right) dx } }

Evaluate: ∫10x4(1−x)41+x2dx\displaystyle \int _{ 0 }^{ 1 }{ \frac { { x }^{ 4 }{ \left( 1-x \right) }^{ 4 } }{ 1+{ x }^{ 2 } } } dx

∫10cot−1(1+x2−x)dx\displaystyle\int^1_0\cot^{-1}(1+x^2-x)dx.

Prove that ∫tanx1/et1+t2dt+∫cotx1/e1t(1+t2)dt=1\displaystyle\int^{\tan x}_{1/e}\dfrac{t}{1+t^2}dt+\displaystyle\int^{\cot x}_{1/e}\dfrac{1}{t(1+t^2)}dt=1.

Evaluate:∫π/40sinx+cosx9+16sin2xdx\int _ { 0 } ^ { \pi / 4 } \dfrac { \sin x + \cos x } { 9 + 16 \sin 2 x } d x

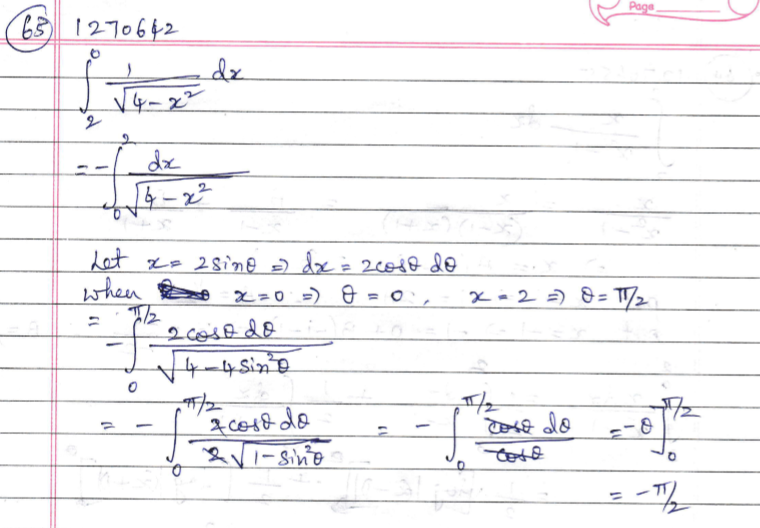
Evaluate:0∫21√4−x2dx\displaystyle \int\limits_2^0 {\dfrac{1}{{\sqrt {4 - x^2} }}} dx

EVALUATE ∫321x+5dx \int_{2}^{3} \frac{1}{x+5} d x

Evaluate the definite integral:∫101−x2(1+x2)2dx\displaystyle\int_{0}^{1}\dfrac{1-x^{2}}{(1+x^{2})^{2}}dx

Evaluate: ∫12x+3dx\displaystyle\int \dfrac {1}{2x+3}dx

Evaluate the following integrals:∫√2x2+3x+4dx\int { \sqrt { 2{ x }^{ 2 }+3x+4 } } dx

Evaluate the following integral:∫(x+1)√2x2+3dx\int { \left( x+1 \right) } \sqrt { 2{ x }^{ 2 }+3 } dx

Evaluate the following integrals:∫√2ax−x2dx\int { \sqrt { 2ax-{ x }^{ 2 } } } dx\quad

Evaluate the following definite integral:∫π/40sin32tcos2t dt\displaystyle\int_{0}^{\pi/4}\sin^{3}2t\cos 2t\ dt.

Evaluate the following integral:∫20x√2−xdx\displaystyle\int^2_0x\sqrt{2-x}dx.

Evaluate the following integral:∫90dx(1+√x)\displaystyle\int^9_0\dfrac{dx}{(1+\sqrt{x})}.

Evaluate the following integral:∫32(2−x)√5x−6−x2dx\displaystyle\int^3_2\dfrac{(2-x)}{\sqrt{5x-6-x^2}}dx.

Evaluate the following integral:∫a0x√a2+x2dx\displaystyle\int^a_0\dfrac{x}{\sqrt{a^2+x^2}}dx.

Evaluate the following integral:∫21dx(x+1)√x2−1\displaystyle\int^2_1\dfrac{dx}{(x+1)\sqrt{x^2-1}}.

Evaluate the following integral:∫10x3√1+3x4dx\displaystyle\int^1_0x^3\sqrt{1+3x^4}dx.

Evaluate the following integral:∫a0x4√a2−x2dx\displaystyle\int^a_0\dfrac{x^4}{\sqrt{a^2-x^2}}dx.

Evaluate the following integral:∫10(1−x2)(1+x2)2dx\displaystyle\int^1_0\dfrac{(1-x^2)}{(1+x^2)^2}dx.

Prove that ∫41√x(√5−x+√x)dx=32\displaystyle\int^4_1\dfrac{\sqrt{x}}{(\sqrt{5-x}+\sqrt{x})}dx=\dfrac{3}{2}.

Prove that ∫10x(1−x)5dx=142\displaystyle\int^1_0x(1-x)^5dx=\dfrac{1}{42}.

Prove that ∫∞0x(1+x)(1+x2)dx=π4\displaystyle\int^{\infty}_0\dfrac{x}{(1+x)(1+x^2)}dx=\dfrac{\pi}{4}.

Prove that ∫3a/4a/4√x(√a−x+√x)dx=a4\displaystyle\int^{3a/4}_{a/4}\dfrac{\sqrt{x}}{(\sqrt{a-x}+\sqrt{x})}dx=\dfrac{a}{4}.

Prove that ∫80|x−5|dx=17\displaystyle\int^8_0|x-5|dx=17.

Prove that ∫a0√x(√x+√a−x)dx=a2\displaystyle\int^a_0\dfrac{\sqrt{x}}{(\sqrt{x}+\sqrt{a-x})}dx=\dfrac{a}{2}.

Prove that ∫a0dxx+√a2−x2=π4\displaystyle\int^a_0\dfrac{dx}{x+\sqrt{a^2-x^2}}=\dfrac{\pi}{4}.

Prove that ∫20x√2−xdx=16√215\displaystyle\int^2_0x\sqrt{2-x}dx=\dfrac{16\sqrt{2}}{15}.

Prove that ∫2−2|x+1|dx=5\displaystyle\int^2_{-2}|x+1|dx=5.

The value of the integral 9999∫∞0dx(x+√1+x2)100 9999\displaystyle \int_{0}^{\infty }\displaystyle \frac{dx}{\left ( x+\sqrt{1+x^{2}} \right )^{100}} is

Let I=∫10dx√4−x2−x3\displaystyle I=\int_{0}^{1}\frac{dx}{\sqrt{4-x^{2}-x^{3}}} and I1=∫1/20dx√1−x4\displaystyle I_{1}=\int_{0}^{1/2}\frac{dx}{\sqrt{1-x^{4}}}

Find : ∫π20dx4+5cosxdx\displaystyle \int_{0}^{\tfrac {\pi}{2}} \dfrac {dx}{4 + 5\cos x} dx

∫π/40(sinx+cosx)9+16sin2xdx\int _{ 0 }^{ \pi /4 }{ \frac { (sinx+cosx) }{ 9+16sin2x } } dx

∫21x√2x2+1dx\int^2_1 \dfrac{x}{\sqrt{2x^2+1}} dx

Evaluate: ∫√31dx1+x2 \int_{1}^{\sqrt{3}} \frac{d x}{1+x^{2}}

Evaluate: ∫π20sinx⋅cosx1+sin4x⋅dx\displaystyle \int_{0}^{\frac{\pi}{2}} \dfrac{\sin x \cdot \cos x}{1+\sin^4 x}\cdot dx.

If the value of the definite integral∫2070C7x200⋅(1−x)7dx\int_{0}^{207} C_{7} x^{200} \cdot(1-x)^{7} d x is equal to 1k\dfrac{1}{k} where k∈Nk \in N\\, thenthe value of k/26k / 26 is

Class 12 Engineering Maths Extra Questions

Support mcqexams.com by disabling your adblocker.

The value of the definite integral, $I = \int _{ 0 }^{ \sqrt { 10 } }{ \dfrac { x }{ { e }^{ { x }^{ 2 } } } } dx$ is equal to

$\displaystyle \int_0^{2\pi}\cos^5x\,\,dx$

Write the value of $\displaystyle\int \dfrac{1-\sin x}{\cos^2x}dx$

$\sqrt{2}\displaystyle \int _{ 0 }^{ 2\pi }{ \sqrt { 1-\sin { x } } dx } =$

Prove that:
$\displaystyle \int_{0}^{\pi}\dfrac {xdx}{1+\sin x} = \pi$

Evaluate the following definite integral:

$\displaystyle\int_{4}^{12}x\ dx$

Evaluate the following definite integral:
$\displaystyle\int_{0}^{\pi/2} \sin x \cos x\ dx$

Evaluate the following definate integral:
$\displaystyle\int_{0}^{2} 3x+2\ dx$

$\displaystyle \int_{0}^{1}\frac{dx}{(1+x)\sqrt{(2+x-x^{2})}}=\frac{1}{k}\sqrt{2}$ . Find the value of $k$ .

Integrate $\displaystyle\int _{ 0 }^{ 1 }{ \sin ^{ -1 }{ \left( \dfrac { 2x }{ 1+{ x }^{ 2 } } \right) dx } }$

Evaluate: $\displaystyle \int _{ 0 }^{ 1 }{ \frac { { x }^{ 4 }{ \left( 1-x \right) }^{ 4 } }{ 1+{ x }^{ 2 } } } dx$

$\displaystyle\int^1_0\cot^{-1}(1+x^2-x)dx$ .

Prove that $\displaystyle\int^{\tan x}_{1/e}\dfrac{t}{1+t^2}dt+\displaystyle\int^{\cot x}_{1/e}\dfrac{1}{t(1+t^2)}dt=1$ .

Evaluate:
$\int _ { 0 } ^ { \pi / 4 } \dfrac { \sin x + \cos x } { 9 + 16 \sin 2 x } d x$

Evaluate:
$\displaystyle \int\limits_2^0 {\dfrac{1}{{\sqrt {4 - x^2} }}} dx$

EVALUATE
$\int_{2}^{3} \frac{1}{x+5} d x$

Evaluate the definite integral:
$\displaystyle\int_{0}^{1}\dfrac{1-x^{2}}{(1+x^{2})^{2}}dx$

Evaluate: $\displaystyle\int \dfrac {1}{2x+3}dx$

Evaluate the following integrals:
$\int { \sqrt { 2{ x }^{ 2 }+3x+4 } } dx$

Evaluate the following integral:
$\int { \left( x+1 \right) } \sqrt { 2{ x }^{ 2 }+3 } dx$

Evaluate the following integrals:
$\int { \sqrt { 2ax-{ x }^{ 2 } } } dx\quad$

Evaluate the following definite integral:
$\displaystyle\int_{0}^{\pi/4}\sin^{3}2t\cos 2t\ dt$ .

Evaluate the following integral:
$\displaystyle\int^2_0x\sqrt{2-x}dx$ .

Evaluate the following integral:
$\displaystyle\int^9_0\dfrac{dx}{(1+\sqrt{x})}$ .

Evaluate the following integral:
$\displaystyle\int^3_2\dfrac{(2-x)}{\sqrt{5x-6-x^2}}dx$ .

Evaluate the following integral:
$\displaystyle\int^a_0\dfrac{x}{\sqrt{a^2+x^2}}dx$ .

Evaluate the following integral:
$\displaystyle\int^2_1\dfrac{dx}{(x+1)\sqrt{x^2-1}}$ .

Evaluate the following integral:
$\displaystyle\int^1_0x^3\sqrt{1+3x^4}dx$ .

Evaluate the following integral:
$\displaystyle\int^a_0\dfrac{x^4}{\sqrt{a^2-x^2}}dx$ .

Evaluate the following integral:
$\displaystyle\int^1_0\dfrac{(1-x^2)}{(1+x^2)^2}dx$ .

Prove that $\displaystyle\int^4_1\dfrac{\sqrt{x}}{(\sqrt{5-x}+\sqrt{x})}dx=\dfrac{3}{2}$ .

Prove that $\displaystyle\int^1_0x(1-x)^5dx=\dfrac{1}{42}$ .

Prove that $\displaystyle\int^{\infty}_0\dfrac{x}{(1+x)(1+x^2)}dx=\dfrac{\pi}{4}$ .

Prove that $\displaystyle\int^{3a/4}_{a/4}\dfrac{\sqrt{x}}{(\sqrt{a-x}+\sqrt{x})}dx=\dfrac{a}{4}$ .

Prove that $\displaystyle\int^8_0|x-5|dx=17$ .

Prove that $\displaystyle\int^a_0\dfrac{\sqrt{x}}{(\sqrt{x}+\sqrt{a-x})}dx=\dfrac{a}{2}$ .

Prove that $\displaystyle\int^a_0\dfrac{dx}{x+\sqrt{a^2-x^2}}=\dfrac{\pi}{4}$ .

Prove that $\displaystyle\int^2_0x\sqrt{2-x}dx=\dfrac{16\sqrt{2}}{15}$ .

Prove that $\displaystyle\int^2_{-2}|x+1|dx=5$ .

The value of the integral $9999\displaystyle \int_{0}^{\infty }\displaystyle \frac{dx}{\left ( x+\sqrt{1+x^{2}} \right )^{100}}$ is

Let $\displaystyle I=\int_{0}^{1}\frac{dx}{\sqrt{4-x^{2}-x^{3}}}$ and $\displaystyle I_{1}=\int_{0}^{1/2}\frac{dx}{\sqrt{1-x^{4}}}$

Find : $\displaystyle \int_{0}^{\tfrac {\pi}{2}} \dfrac {dx}{4 + 5\cos x} dx$

$\int _{ 0 }^{ \pi /4 }{ \frac { (sinx+cosx) }{ 9+16sin2x } } dx$

$\int^2_1 \dfrac{x}{\sqrt{2x^2+1}} dx$

Evaluate: $\int_{1}^{\sqrt{3}} \frac{d x}{1+x^{2}}$

Evaluate: $\displaystyle \int_{0}^{\frac{\pi}{2}} \dfrac{\sin x \cdot \cos x}{1+\sin^4 x}\cdot dx$ .

If the value of the definite integral $\int_{0}^{207} C_{7} x^{200} \cdot(1-x)^{7} d x$ is equal to $\dfrac{1}{k}$ where $k \in N\\$ , thenthe value of $k / 26$ is