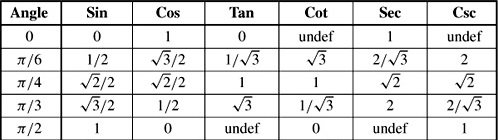
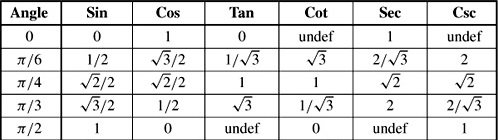
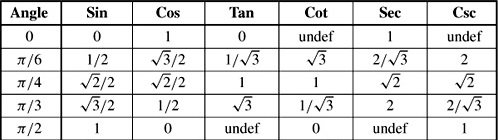
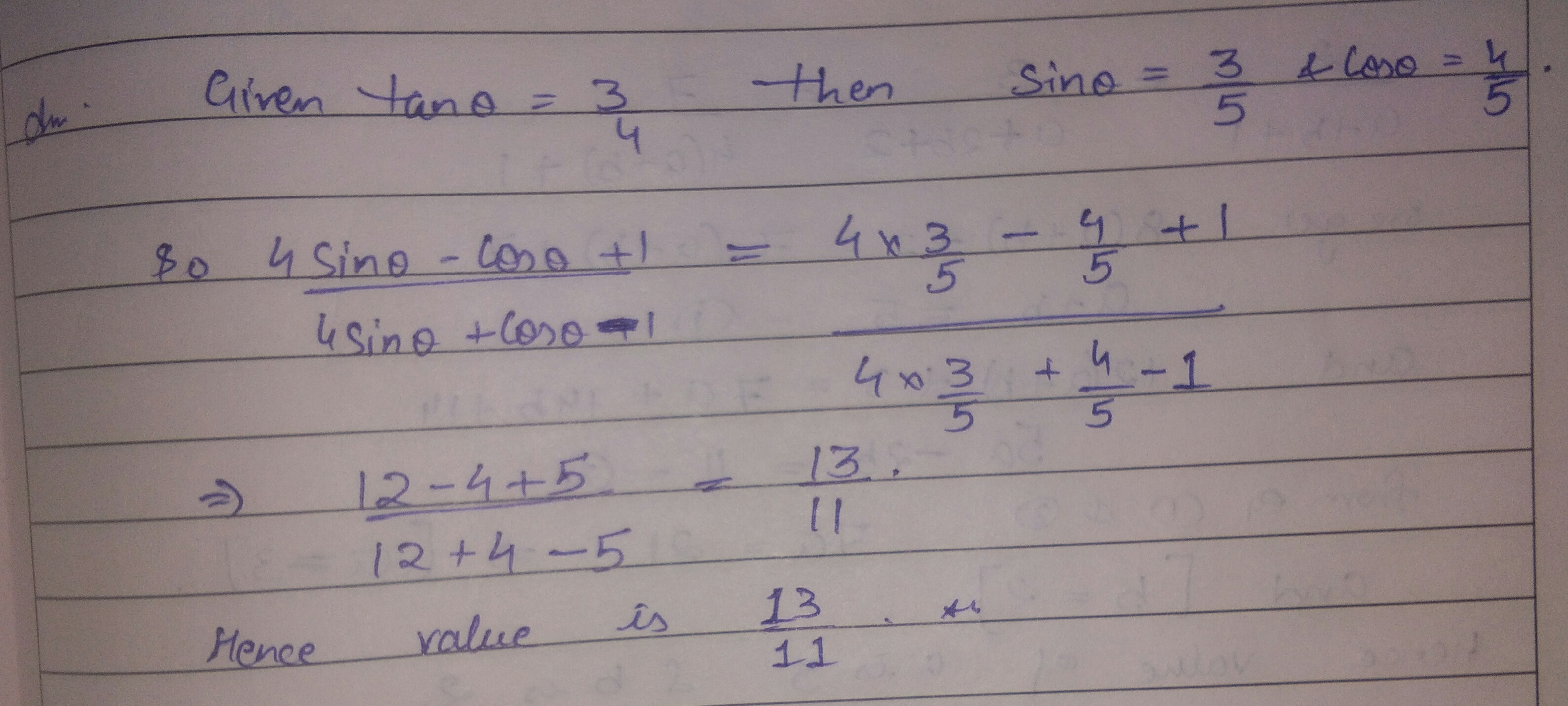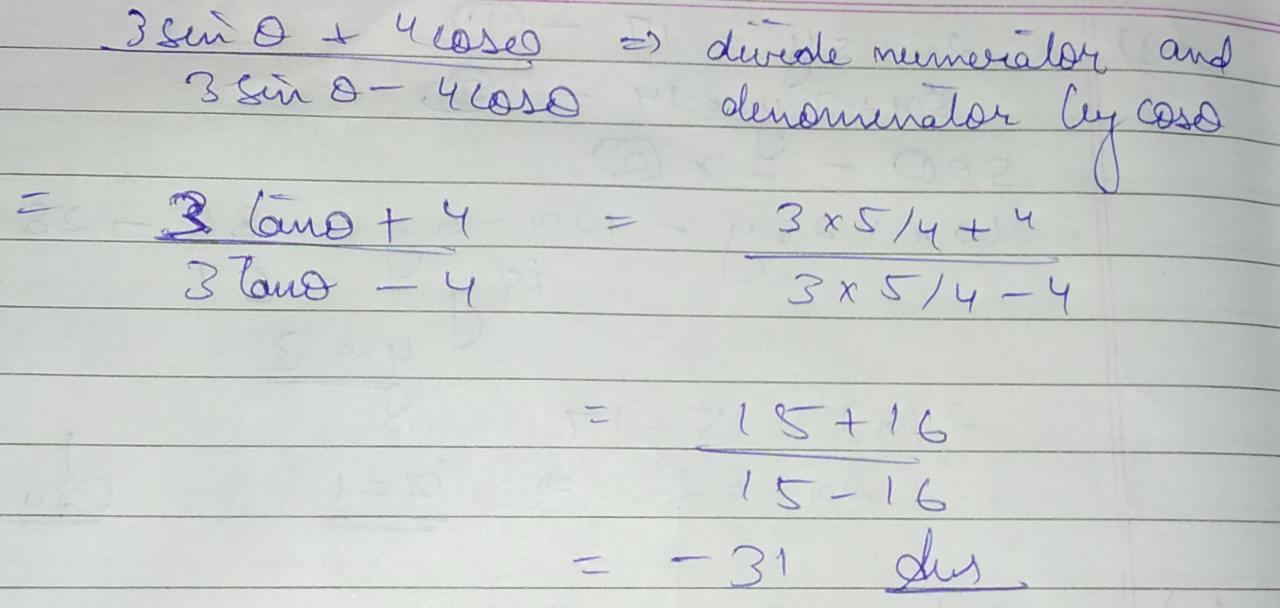Introduction To Trigonometry - Class 10 Maths - Extra Questions
Find value of 10x
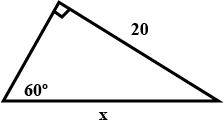
Value of 100x is 3464,
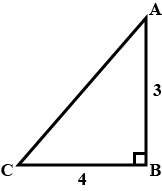
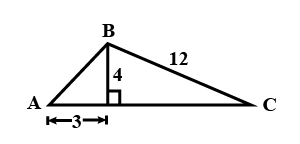
From the given figure, find the value of m if, tan C is m4.
1−cos2Asin2A=tan2A
If above statement is true then mention answer as 1, else mention 0 if false
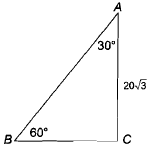
Find side c of the triangle ABC, given that A=300,B=600 and b=20√3
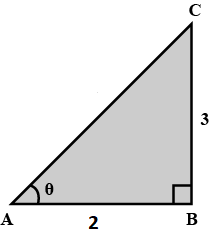
If 2cosθ=1 and θ is acute angle, then what is θ equal to?
Find the value of the following:
tan260o+2tan245o
Prove that cosθ⋅cosec θ=cotθ
Find the value of sin245o+cos245o.
Find the value of (sinθ+cosθ)2+(sinθ−cosθ)2
If cosθ=1, then find the value of acute angle θ.
sin(60∘+30∘)= _______.
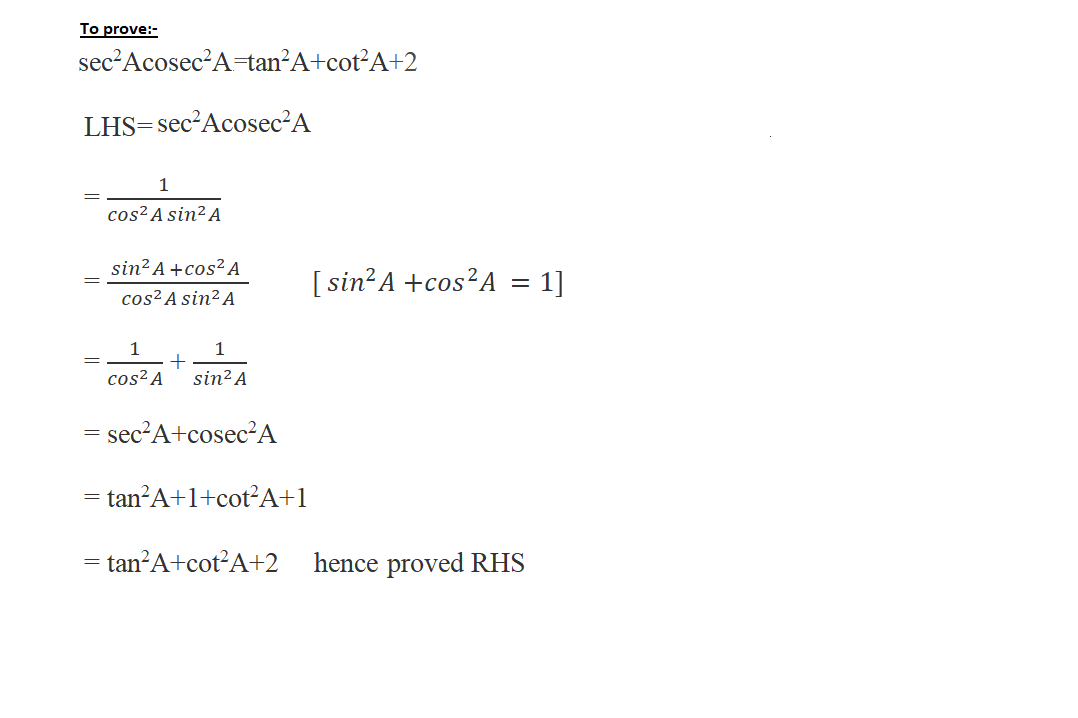
(1+tanA⋅tanB)2+(tanA−tanB)2=sec2A⋅sec2B.
Find the value of sin230∘+cos260∘
Prove that sin2A+11+tan2A=1.
Prove that (1+cot2A)sin2A=1.
Prove the following :
(iv) cos(90∘−A)sin(90∘−A)tan(90∘−A)=sin2A
Prove that cosecθ√1−cos2θ=1.
Evaluate the following :
cos37∘sin53∘
Evaluate the following :
tan10cot80
Evaluate the following :
sec11cosec79
sec11cosec79
Prove that (secθ+cosθ)(secθ−cosθ)=tan2θ+sin2θ.
Prove that (sec2θ−1)(cosec2θ−1)=1
Evaluate the following :
tan54∘cot36∘
Evaluate the following:
2tan53∘cot37∘−cot80∘tan10∘
2tan53∘cot37∘−cot80∘tan10∘
Verify that cot260o+sin245o+sin230o+cos290o=1312
If tanθ=2021, then prove that 1−sinθ+cosθ1+sinθ+cosθ=37
Express each of the following in trigonometric ratios of angles between 0∘and45∘ :
sin85∘+cosec85∘
(tanx+cotx)2=sec2x+csc2x
Prove that:1+secAsecA=sin2A1−cosA
Calculate : (cos40)2+(sin40)2
Prove that cotA−cosAcotA+cosA=cosecA−1cosecA+1
Prove that: 1secA−1+1secA+1=2cscAcotA
Prove that 1+cosθsin2θ=11−cosθ
Evaluate
tan45ocosec30o+sec60ocot45o−5sin90o2cos0o
Evaluate : sin290.cos245+4tan230+12sin2a−2cos290+124
Simplify:
sinθ.csc(90−θ).tanθcsc(90−θ).cosθ.cot(90−θ)
For any angle of measure θ prove that 1+tan2θ=sec2θ.
Find the value of cos30otan60o+sin60ocos60ocosec30osec60o−cosec60ocot30o
Prove that: √sec2A+csc2A=tanA+cotA
If A=15o, verify that 4sin2A.cos4A.sin6A=1
Evaluate
sin2θ(tan2θ+1)=sec2θ−1
Evaluate
tanθ.sinθ+cosθ=secθ
Simplify: sec x + tan xsec x - tan x
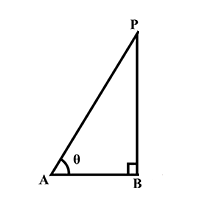
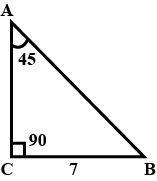
In the figure, find the value of BC
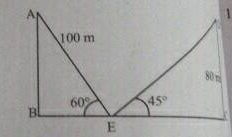
Solve cos45∘sec30∘+cosec30∘
Find value of:
3\cos ^{ 2 }{ { 30 }^{ o } } +\sec ^{ 2 }{ { 30 }^{ o } } +2\cos ^{ 2 }{ { 0 }^{ o } } +3\sin ^{ 2 }{ { 90 }^{ o } } -\tan ^{ 2 }{ { 60 }^{ o } }
Evaluate: \dfrac{\cos\ 3A-2\cos(4A)}{\sin\ 3A+2\sin(4A)}; when A=15^{o}
Solve:-
\dfrac{{2\tan {{45}^ \circ }}}{{1 + {{\tan }^2}{{45}^ \circ }}}
Prove that \cos \theta \sin(90^o-\theta)+\sin \theta \cos(90^o-\theta)=1
Without using trigonometric tables, evaluate the following: \left ( \sin 35^{0}\cos 55^{0}+\cos 35^{0}\sin 55^{0} \right )/\left ( cosec^{2}10^{0}-\tan ^{2}80^{0} \right ).
Prove that following identities, where the angles involved are acute angles for which the trigonometric ratios as defined: \left ( 1+\tan ^{2}A \right )\left ( 1-\sin A \right )\left ( 1+\sin A \right )=1.
Verify that
\tan^{2} 30^{\circ} + \tan^{2} 45^{\circ} + \tan^{2} 60^{\circ} = 4\dfrac {1}{3}.
A 15 m long ladder touches the top of vertical wall. If this ladder makes an angle of 60^{0} with the wall, then height of the wall is :
Prove that following identities, where the angles involved are acute angles for which the trigonometric ratios as defined: 1/\left ( 1+\cos A \right )+1/\left ( 1-\cos A \right )=2cosec^{2}A.
Verify that
\cos 45^{\circ} \cos 60^{\circ} - \sin 45^{\circ} \sin 60^{\circ} = -\dfrac {\sqrt {3} - 1}{2\sqrt {2}}.
Verify that
cosec^{2} 45^{\circ} \cdot \sec^{2} 30^{\circ} \cdot \sin^{3} 90^{\circ} \cdot \cos 60^{\circ} = 1\dfrac {1}{3}.
Find the value of \cfrac{\tan 47^{0}}{\cot 43^{0}}
Simplify: (1 + \tan^{2} \theta) (1 - \sin \theta) (1 + \sin \theta).
State true or false:\sin^2A+ \cos^2A= 1
If \sin A, \cos A and \tan A are in geometric progression, then \cot^6A-\cot^2A=........
\tan 30^{\circ} \: \cot 30^{\circ}=
\text{cosec}\, ^{2} 60^{0}-\tan ^{2} 30^{0}=
If the angle \theta = 60^{\circ} and \displaystyle cos \theta = \frac{1}{2}, find the value of sec \theta.
Find the value of : \displaystyle \dfrac{2 \tan 53^o}{\cot 37^o} - \dfrac{\cot 80^o}{\tan 10^o}
If \displaystyle \sin \theta = \cfrac{\sqrt{2}}{3}, \cos \theta = \cfrac{1}{3}, then \displaystyle \tan \theta = \sqrt{m}. The value of m is
If \displaystyle tan \theta = \frac{2}{5}, then \displaystyle cot \theta = \frac{p}{2}, p is
If \tan 2A = \cot (A - 18^o) then find the value of A where (2A) and (A - 18^o) are acute angles
The value of \displaystyle \left ( \dfrac{\tan 60^{0}+1}{\tan 60^{0}-1} \right )^{2}= \dfrac{1+\cos 30^{0}}{1-\cos 30^{0}}
if true then enter 1 and if false then write 0
if true then enter 1 and if false then write 0
The value of \displaystyle \tan \left ( 2 \times 30^{0}\right )= \frac{2 \tan 30^{0}}{1-\tan ^{2} 30^{0}}
if true then enter 1 and if false then write 0
if true then enter 1 and if false then write 0
The value of \cos ^{2} 30^{0}-\sin ^{2} 30^{0}= \cos 60^o
If above statement is true then enter 1 and if false then write 0.
\sin ^{2} 30^{0}+\cos ^{2} 30^{0}
The value of \cos 30^{\circ} \cos 60^{\circ}-\sin 30^{\circ} \sin 60^{\circ} is equal to
ABC is an isosceles right-angled triangle. Assuming AB= BC= x, find the value of each of the following trigonometric ratios:\sin 45^{0} is \displaystyle \frac{1}{\sqrt{2}}
If true then enter 1 and if false then enter 0

If true then enter 1 and if false then enter 0
Find the value of \text{cosec}^{2} 45^{o}-\cot^{2} 45^{o}.
The value of \displaystyle \cos \left ( 2 \times 30^{0}\right )= \frac{1-\tan ^{2} 30^{0}}{1+\tan ^{2} 30^{0}}
If true then enter 1 and if false then write 0
If true then enter 1 and if false then write 0
The value of \displaystyle \sin \left ( 2 \times 30^{0}\right )= \frac{2\tan 30^{0}}{1+\tan ^{2} 30^{0}}
if true then enter 1 and if false then write 0
if true then enter 1 and if false then write 0
If A= 30^{0}, then the value of\sin \left ( 60^{0}+A \right )-\sin \left ( 60^{0}-A \right )= \sin AIf true then enter 1 and if false then write 0
If \tan \theta= \cot \theta and 0^{0}\leq \theta \leq 90^{0}, state the value of \theta in degrees
The value of \sin 60^{0}=2 \sin 30^{0} \cos 30^{0}
if true then enter 1 and if false then write 0
if true then enter 1 and if false then write 0
If \sqrt{3}= 1.732, (correct to two decimal places) the value of \sin 60^{0} is 0.87.If true then enter 1 and if false then enter 0.
If \sqrt{3}= 1.732, (correct to two decimal places) the value of \sin 60^{0} is 0.87.
If true then enter 1 and if false then enter 0.
If x= 15^{0}, evaluate:
\displaystyle 12 \sin 3x \cos 3x+5 \tan ( 2x+ 15^{0})-3 \cot ^{2}3x
If \sin x= \cos x and x is acute, state the value of x in degrees.
If A= 60^{0} and B= 30^{0}, find the value of \left ( \sin A \cos B+ \cos A\sin B \right )^{2}+\left ( \cos A \cos B-\sin A \sin B \right )^{2}
If x= 15^{0}, evaluate:
4 \cos 2x \sin 4x\tan 3x-1
If \sqrt{3}= 1.732, find (correct to two decimal places) the value of the following:
\displaystyle \dfrac{2}{\tan 30^{0}} is 3.46
If true then enter 1 and if false then enter 0
\displaystyle \dfrac{2}{\tan 30^{0}} is 3.46
If true then enter 1 and if false then enter 0
If A= 30^{0}, then\displaystyle \cos 2A= \cos ^{2} A-\sin ^{2} A= \dfrac{1-\tan ^{2}A}{1+\tan ^{2}A}If true enter 1 else 0
Given A= 60^{0} and B= 30^{0}, then
\displaystyle \tan \left ( A-B \right )= \dfrac{\tan A-\tan B}{1+\tan A \tan B}If true enter 1 else 0
If A= 30^{0}, then 2\cos ^{2} A-1= 1-2\sin ^{2}AIf true enter 1 else 0
If A=B= 45^{0}, then \sin \left ( A-B \right )=\sin A \cos B-\cos A \sin BIf true enter 1 else 0
Solve: \sec 4A = \csc (A-20^{\circ})
\displaystyle \cos ^2\ 10^{\circ}-\sin^2 80+\tan ^2 45^{\circ}
State true or false
Given A= 60^{0} and B= 30^{0}, then\sin \left ( A+B \right )= \sin A\cos B+\cos A\sin B
If true enter 1 else 0
Given A= 60^{0} and B= 30^{0}, then
\sin \left ( A+B \right )= \sin A\cos B+\cos A\sin B
Find the value of: \displaystyle \sqrt{\frac{1-\sin ^{2}60^{0}}{1-\cos ^{2}60^{0}}} is \displaystyle \frac{1}{\sqrt{m}}, m is
Given A= 60^{0} and B= 30^{0}, then
\cos \left ( A-B \right )= \cos A\cos B+\sin A\sin BIf true or false:
If A= 60^{0} and B= 30^{0}; \displaystyle \frac{\sin \left ( A-B \right )}{\sin A \sin B}= \cot B-\cot A
If true enter 1 else 0
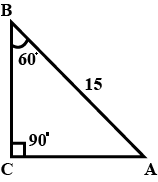
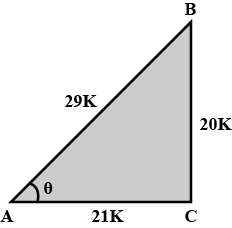
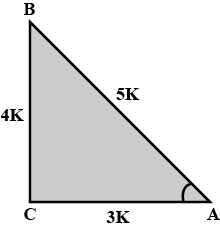
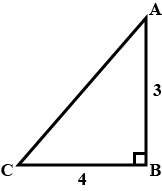
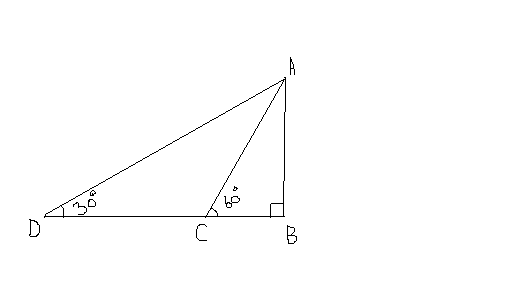
Refer to the triangle given in figure.\sec C is \displaystyle \frac{3\sqrt{2}}{m}, m is
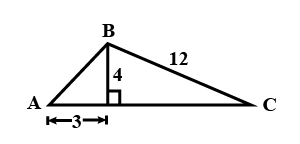
Consider the following figure:
\sin B is \displaystyle \frac{12}{m}, m is
\displaystyle 3-\sin ^2 48^{\circ}+\cos ^2 42^{\circ}
Consider the following figure:
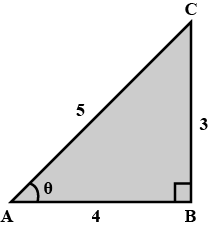
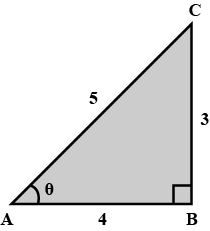
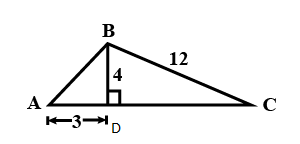
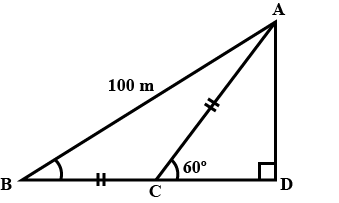
\tan C is \displaystyle \frac{m}{4}, m is
Refer to the triangle given in the figure.
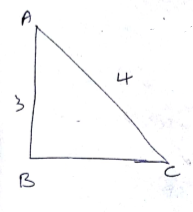
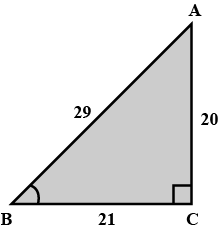
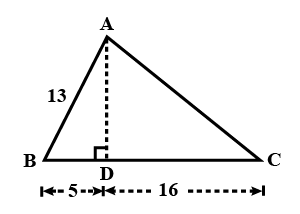
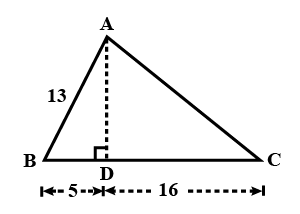
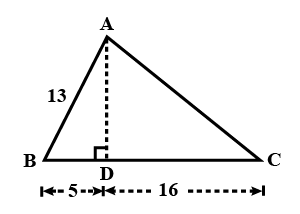
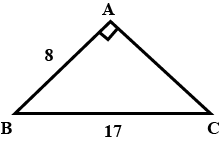
If \cos A is equal to \displaystyle \frac{m}{5}, find m.
If \cos A is equal to \displaystyle \frac{m}{5}, find m.
If \cos A is \displaystyle \frac{3}{m}, then m is

From the following figure, find the values of:
\sec ^{2}B\, -\, \tan ^{2}B
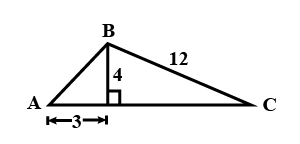
If \sin C is \displaystyle \frac{1}{m}, then m is:
Refer to the triangle given in figure.
If cosec\: A is \displaystyle \cfrac{5}{m}, then m is:
Also find the value of : \sin B\cdot \cos C\, +\, \cos B\cdot \sin C.
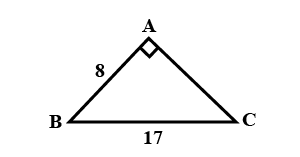
From the figure, \sin x^{0} is \displaystyle \frac{\sqrt{m}}{2}. The value of m is:
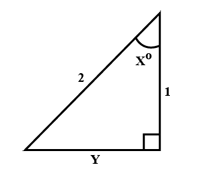
In triangle ABC, AD is perpendicular to BC. \sin B= 0.8, BD = 9 cm and \tan C= 1. Find the length of AB
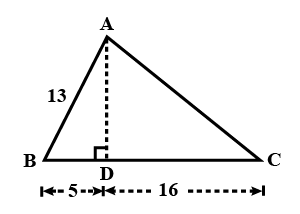
In triangle ABC, AB= AC= 15 cm and BC= 18 cm. Find :
m if \sin C is \displaystyle \dfrac{4}{m},
m if \sin C is \displaystyle \dfrac{4}{m},
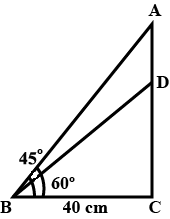
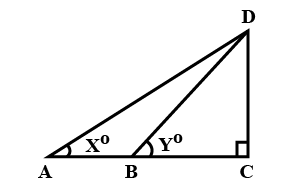
Find the length of AD. If \angle ABC = 60^{\circ},\ \angle DBC = 45^{\circ} and BC = 40\ cm.
From the following figure, find the value of:
\sin ^{2}C\, +\, \cos ^{2}C
From the following figure, find the value of:
\cos ^{2}A\, +\, \sin ^{2}A

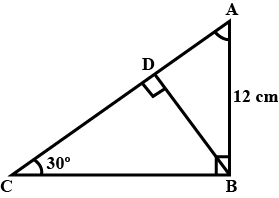
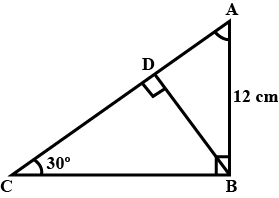
Find AB, if:
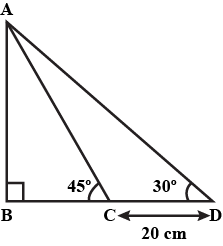
Find AC
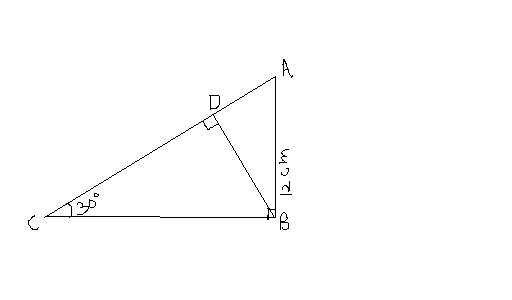
Find AB
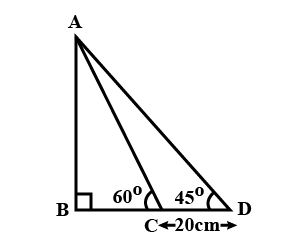
The value of BC in the closest integer (in cm.) is:
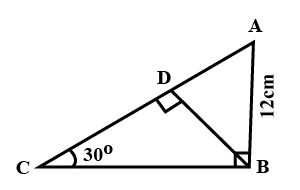
Find \space BC \space and \space AB

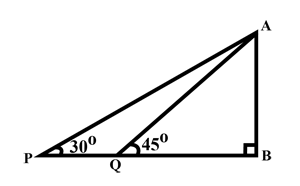
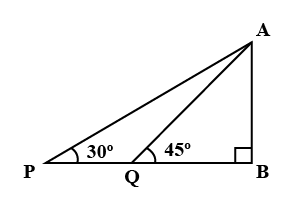
Find PQ, if \displaystyle AB = 150\ m, \angle P = 30^{\circ} and \displaystyle \angle Q = 45^{\circ}
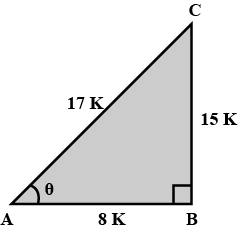
From the given figure, find the value of m, if cos A =\displaystyle \dfrac{3}{m},
If \tan x^{\circ} = \dfrac {5}{12}, \tan y^{\circ} = \dfrac {3}{4} and AB = 48\ m; find the length of CD.
From the given figure, find the value of \sin^{2}B\, +\, \cos^{2}B
In the following figure; angle \displaystyle B=90^{\circ},\angle ADB=30^{\circ} and \displaystyle \angle ACB=60^{\circ}.
If \displaystyle CD = 40\ m, then AB is 34.64 m
If true then enter 1 and if false then enter 0
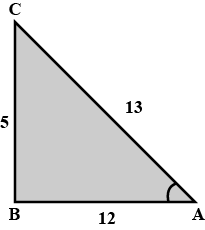
cos A is \displaystyle \frac{12}{m}, m is
sin A is \displaystyle \frac{m}{13}, m is
From the given figure, find the value of sin B.cos C + cos B.sin C

sin B is \displaystyle \frac{m}{5}, m is
In triangle ABC, AD is perpendicular to BC.If \sin B = 0.8, BD = 9 cm and \tan C = 1. Find the length of AB
If \sin\, 30^{\circ}\, \cos\, 30^{\circ} is \displaystyle \dfrac{\sqrt{3}}{m}, then m is:
If 3 \tan A - 5 \cos B = \sqrt{3} and B = 90^{\circ}, find the value of A in degrees.
AB is 17.32 cm
If true then enter 1 and if false then enter 0

Find PQ, if AB = 150 m, \angle P\, =\, 30^{\circ}\, and\, \angle Q\, =\, 45^{\circ}
Ab is 47.32 cm
If true then enter 1 and if false then enter 0

\cos^{2}\, 10^{\circ}\, -\, \sin^{2}\, 80^{\circ}\, +\, \tan^{2}\, 45^{\circ}
AB is 27.32 cm
If true then enter 1 and if false then enter 0

sin\,x^{\circ} is \displaystyle\,\frac{\sqrt{3}}{m}
value of m is
The value of x is m\sqrt{2}, so m is equal to:

Use cos\,x^{\circ} to find the value of y.
If \sec A \tan B+\tan A \sec B=91, then the value of (\sec A \sec B+\tan A \tan B)^2 is equal to
If \displaystyle \cos \theta =\frac{3}{4} then the value of \displaystyle \sqrt{\frac{\sec \theta -cosec \theta }{\sec \theta +cosec \theta }} is ____
Find the value of \displaystyle \frac{2\sin 68^{\circ}}{\cos 22^{\circ}}-\frac{2\cot 15^{\circ}}{5\tan 75^{\circ}}-\frac{3\tan 45^{\circ}\tan 20^{\circ}\tan 40^{\circ}\tan 50^{\circ}\tan 70^{\circ}}{5}.
Find the value of \displaystyle \frac{\cot 54^{\circ}}{\tan 36^{\circ}}+\frac{\tan 20^{\circ}}{\cot 70^{\circ}}-2.
The value of \displaystyle \cos ^{2}5^{0}+\cos ^{2}10^{0}+\cos ^{2}15^{0}+\cos ^{2}20^{0}+\cos ^{2}70^{0}+\cos ^{2}75^{0}+\cos ^{2}80^{0}+\cos ^{2}85^{0} is equal to
Eliminate \theta, \displaystyle x=a\cos ^{3}\theta\ y=b\sin ^{3}\theta
Find the relation obtained by eliminating \displaystyle \theta from the equations x = r \displaystyle \cos \theta +s\sin \theta and y=r\displaystyle \sin \theta -s\cos \theta
Show that : (i) \tan 48^{\circ} \tan 23^{\circ} \tan 42^{\circ} \tan 67^{\circ} = 1 (ii) \cos 38^{\circ} \cos 52^{\circ} - \sin 38^{\circ} \sin 52^{\circ} = 0.
Evaluate:
(i) \displaystyle \frac { \sin { { 1 }8^{ \circ } } }{ \cos { { 72 }^{ \circ } } }
(ii) \displaystyle \frac { \tan { { 26 }^{ \circ } } }{ \cot { { 64 }^{ \circ } } }
(iii) \displaystyle \cos { { 48 }^{ \circ } } -\sin { 42^{ \circ } }
(iv) \displaystyle \csc \ { 31 }^{ \circ }-\sec { { 59 }^{ \circ } }
If \cos{x} = \dfrac{24}{25}, then \sec{x} =
If \sin { \theta } =\cfrac { 1 }{ 2 } and \cos { \theta } =-\cfrac { \sqrt { 3 } }{ 2 } , find the values of the other 4 trignometric functions.
If cosec\ {x} = \dfrac{25}{15}, \sin{x} =
If \tan{x} = \dfrac{7}{24}, \cot{x} =
If \cot{A} = \dfrac{8}{15} and \sin{A} = \dfrac{15}{17} then, \cos{A} =
If \sin{x} = \dfrac{3}{5}, then \text{ cosec} \ x = ?
What trigonometric ratios of angles from {0}^{o} to {90}^{o} are not defined?
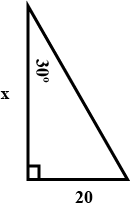
Find the value of 'x'
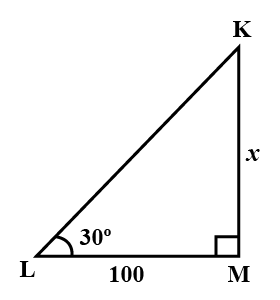
Find the value of 'x'
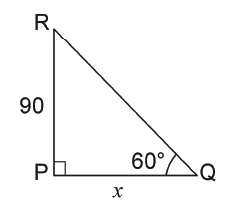
If A={ 60 }^{ o },B={ 30 }^{ o } then prove that \cos { \left( A+B \right) } =\cos { A } \cos { B } -\sin { A } \sin { B }
What trigonometric ratios of angles from {0}^{o} to {90}^{o} are equal?
What trigonometric ratios of angles from {0}^{\circ} to {90}^{\circ} are equal to 0?
If A={ 60 }^{ o } and B={ 30 }^{ o }, then prove that \tan { \left( A-B \right) } =\dfrac { \tan { A } -\tan { B } }{ 1+\tan { A } \tan { B } }
What trigonometric ratios of angles from {0}^{o} to {90}^{o} are equal to 1?
Prove that \cos { \theta } \cdot \text{cosec} { \theta } =\cot { \theta }
Find the value of 'x'
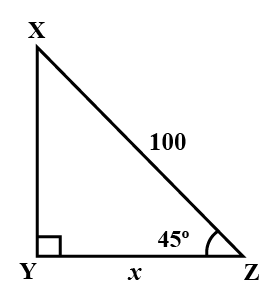
Prove that \dfrac { \sin { \theta } }{ 1-\cot { \theta } } +\dfrac { \cos { \theta } }{ 1-\tan { \theta } } =\cos { \theta } +\sin { \theta } .
Evaluate without using trigonometric tables
\cos^226^\circ+\cos\,64^\circ \sin\,26^\circ+\dfrac{\tan\,36^\circ}{\cot\,54^\circ}
Without using tables evaluate 3\cos 80^o.\text{cosec}\, 10^o+2\sin 59^o.\sec 31^o.
If \cos { 7A } =\sin { \left( A-{ 6 }^{ o } \right) } , where 7A is an acute angle. Find the value of A.
Without using trigonometric tables evaluate :\dfrac { \sin { { 63 }^\circ } }{ \cos { { 27 }^\circ } } +\dfrac { \cos { { 39 }^\circ } }{ \sin { { 51 }^\circ } } -\sin { { 23 }^\circ } \cdot \sec { { 67 }^\circ } +\csc ^{ 2 }{ { 30 }^\circ }
If \cos{\theta}=\dfrac{1}{2}, then find the value of acute angle \theta.
Prove that: \dfrac {\cos A}{1 + \sin A} + \tan A = \sec A
If \theta = 60^{\circ}, find the value of \cos \theta
Prove that \displaystyle \frac{\tan^2\theta}{(\sec\,\theta-1)^2}=\frac{1+\cos\,\theta}{1-\cos\,\theta}
Without using trigonometric tables, evaluate \sin^{2} 34^{\circ} + \sin^{2} 56^{\circ} + 2\tan 18^{\circ} \tan 72^{\circ} - \cot^{2} 30^{\circ}
Verify the following equalities.
\cos 60^{\circ} = 1 - 2\sin^{2} 30^{\circ} = 2\cos^{2} 30^{\circ} - 1
Verify the following equalities.
\sin^{2}30^{\circ} + \cos^{2} 30^{\circ} = 1
Verify the following equalities.
\cos 90^{\circ} = 1 - 2\sin^{2} 45^{\circ} = 2\cos^{2} 45^{\circ} - 1
Prove that \sqrt {\dfrac {1 + \cos \theta}{1 - \cos \theta}} = cosec \theta + \cot \theta
Prove that
\dfrac {\sin (90^{\circ} - \theta)}{1 + \sin \theta} + \dfrac {\cos \theta}{1 - \cos (90^{\circ} - \theta)} = 2\sec \theta
Verify the following equalities.
1 + \tan^{2} 45^{\circ} = \sec^{2} 45^{\circ}
Verify the following equalities.
\tan^{2} 60^{\circ} - 2\tan^{2} 45^{\circ} - \cot^{2} 30^{\circ} + 2\sin^{2} 30^{\circ} + \dfrac {3}{4} cosec^{2} 45^{\circ} = 0
Verify the given equalities.
\sin 30^{\circ} \cos 60^{\circ} + \cos 30^{\circ} \sin 60^{\circ} = \sin 90^{\circ}
Verify the following equalities.
\dfrac {1 - \tan^{2} 60^{\circ}}{1 + \tan^{2} 60^{\circ}} = 2\cos^{2} 60^{\circ} - 1
Verify the following equalities.
4\cot^{2} 45^{\circ} - \sec^{2} 60^{\circ} + \sin^{2} 60^{\circ} + \cos^{2} 60^{\circ} = 1
Simplify \dfrac {\cos 80^{\circ}}{\sin 10^{\circ}} + \cos 59^{\circ} cosec 31^{\circ}
Verify the following equalities.
\dfrac {\sec 30^{\circ} + \tan 30^{\circ}}{\sec 30^{\circ} - \tan 30^{\circ}} = \dfrac {1 + \sin 30^{\circ}}{1 - \sin 30^{\circ}}
Verify the following equalities.
\dfrac {\cos 60^{\circ}}{1 + \sin 60^{\circ}} = \dfrac {1}{\sec 60^{\circ} + \tan 60^{\circ}}
Show that: \tan 35^{\circ} \tan 60^{\circ} \tan 55^{\circ} \tan 30^{\circ} = 1
Prove that: \sec^2\theta =1+\tan^2\theta.
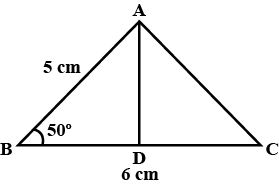
Length of two sides of a triangle are 6 centimetres and 5 centimetres. If their included angle is {50}^{o}. What is the area of the triangle?
Also find the length of its third side.
\left( \sin { { 50 }^{ o } } =0.77,\cos { { 50 }^{ o } } =0.64,\tan { { 50 }^{ o } } =1.19 \right)
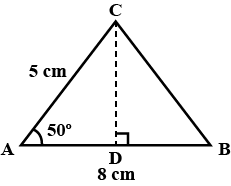
In \Delta ABC, AB = 8 cm, AC = 5 cm and m \angle A = 50^o. Then
(a) What is the length of the perpendicular from C to AB?
(b) Find the length of BC.
[sin 50^o = 0.7660, cos 50^o = 0.6428, tan 50^o = 1.1918]
Without using trigonometric tables, evaluate the following:
2\left (\dfrac {\cos 58^{\circ}}{\sin 32^{\circ}}\right ) - \sqrt {3}\left (\dfrac {\cos 38^{\circ} \text{cosec }52^{\circ}}{\tan 15^{\circ} \tan 60^{\circ} \tan 75^{\circ}}\right ).
Evaluate : \displaystyle \, \frac{cosec 13^{\circ}}{\sec 77^{\circ}} \, - \, \frac{\cot 20^{\circ}}{\tan 70^{\circ}}
Calculate
\displaystyle\, \frac{\sin \, \alpha}{\sin^3 \, \alpha \, + \, \cos^3 \, \alpha} if \tan \alpha =2.
If \cos\theta +\sin\theta =\sqrt{2}\cos\theta, show that \cos\theta -\sin\theta=\sqrt{2}\sin\theta
Show that:\left( 1+\cot { A } +\tan { A } \right) \left( \sin { A } -\cos { A } \right) =\cfrac { \sec { A } }{\text{cosec} ^{ 2 }{ A } } -\cfrac {\text{cosec }{ A } }{ \sec ^{ 2 }{ A } } =\sin { A } \tan { A } -\cot { A } \cos { A }
Prove that \dfrac{\tan^2A}{1 + \tan^2A} + \dfrac{\cot^2A}{1 +\cot^2A} = 1
Prove that \dfrac{\tan A \, + \, \tan B}{\cot A \, + \, \cot B} \, = \, \tan A \, \tan B
Prove the following statements.
\dfrac {\cot A + \tan B}{\cot B + \tan A} = \cot A \tan B.
Evaluate the following : sin 45^{\circ} sin 30^{\circ} + cos 45^{\circ} cos 30^{\circ}
Prove that \tan^2A + \cot^2A = \sec^2A.cosec^2A \, - \, 2
Prove that \dfrac{\cos^2 \theta}{\sin \theta} - cosec \theta + \sin \theta = 0.
Evaluate \sin 60^{\circ} \cos 30^{\circ} + \cos 60^{\circ} \sin 30^{\circ}.
Evaluate the following :
cos 60^{\circ} cos 45^{\circ} - sin 60^{\circ} sin 45^{\circ}
Prove that \dfrac{(1 + \tan^2\theta) \cot \theta}{co\sec^2 \theta} = \tan \theta
Prove that \dfrac{\sin 70^{\circ}}{\cos 20^{\circ}} + \dfrac{\text{cosec} 20^{\circ}}{\sec 70^{\circ}} - 2 \cos 70^{\circ} \text{cosec} 20^{\circ} = 0
Express the following in terms of trigonometric ratios of angles lying between 0^\circ and 45^\circ:
\cot 85^\circ + \cos 75^\circ
If A = 30^{\circ} and B = 60^{\circ}, verify that
(i) \sin(A + B) = \sin A \cos B + \cos A \sin B
(ii) \cos(A + B) = \cos A \cos B - \sin A \sin B
Express the following in terms of trigonometric ratios of angles lying between 0 and 45:
\text{cosec} 54^0 + \sin 72^0
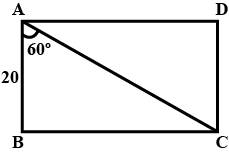
In a rectangle ABCD, AB = 20 cm, \angleBAC = 60^{\circ}, calculate side BC and diagonals AC, BD.
In a right \triangle \text{ABC}, right angled at \text{C}, if \angle \text{B} = 60^{\circ} and \text{AB} = 15 units. Find the remaining angles and sides.
Prove the following :\dfrac{\cos(90^{\circ} \, - \, \theta) \, \sec \, (90^{\circ} \, - \, \theta) \, \tan \, \theta}{\text{cosec}(90^{\circ} \, - \, \theta) \, \sin(90^{\circ} \, - \, \theta) \, \cot(90^{\circ} \, - \, \theta)} \, + \ \dfrac{\tan(90^{\circ} \, - \, \theta)}{\cot \, \theta} \, = \, 2
If \Delta ABC is a right triangle such that \angle C = 90^{\circ}, \, \angle A = 45^{\circ} and BC = 7 units. Find \angle B, AB and AC.
Prove that \sin 48^{\circ} \sec 42^{\circ} + \cos 48^{\circ} \text{cosec} 42^{\circ} = 2
Prove that: \tan 20^{\circ} \tan 35^{\circ} \tan 45^{\circ} \tan 55^{\circ} \tan 70^{\circ} = 1
Prove that: \dfrac{{\tan \theta }}{{1 - \cot \theta }} + \dfrac{{\cot \theta }}{{1 - \tan \theta }} = 1 + \sec \theta\ {cosec}\ \theta
If 3x\sin 60^o.\cos^2 30^o=\dfrac{\tan^2 45^o.\sec 60^o}{cosec 60^o.\sin 45^o}, then find the value of x.
If a \cos \theta - b \sin \theta = x and a \sin \theta + b \cos \theta = y then find \dfrac{a^{2} + b^{2}}{x^{2} + y^{2}}.
Express each of the following in trigonometric ratios of angles between 0^{\circ} \, and \, 45^{\circ} :
sin 72^{\circ} \, + \, cot 72^{\circ}
Prove that :
sin 35^{\circ} \, sin 55^{\circ} \, - \, cos 35^{\circ} \, cos 55^{\circ} \, = \, 0
The value of \left( 1+\cos { \dfrac { \pi }{ 8 } } \right) \left( 1+\cos { \dfrac { 3\pi }{ 8 } } \right) \left( 1+\cos { \dfrac { 5\pi }{ 8 } } \right) \left( 1+\cos { \dfrac { 7\pi }{ 8 } } \right) =\dfrac { a }{ 256 } . Find a.
Find the value of:
\dfrac{1 + \cos A -\sin A}{1 + \cos A +\sin A} -\sec A + \tan A
Prove that \dfrac{1+2\cos^2\,A}{1+3\cot^2\,A}=\sin^2\,A
Find the value of (\sin \theta + \sec \theta)^{2} + (\cos \theta + \text{cosec} \theta)^{2} - (1 + \sec \theta \text{cosec} \theta)^{2}
If cosec^{6} \theta -cot^{6} = a cot^{4}\theta + b cot^{2}\theta + c then a + b + c =
If \dfrac{\tan^3\alpha}{1+\tan^2\alpha}= \sec\alpha \sin\alpha- a \sin\alpha \cos\alpha. Find a
If x= r \cos\theta. \sin\phi, y= \sin\theta . \sin\phi, z= r \cos\phi Prove that x^2+y^2+z^2=r^2
Prove that \sqrt { \dfrac { \sin { A } +1 }{ 1-\sin { A } } } +\sqrt { \dfrac { 1-\sin { A } }{ \sin { A } +1 } } =2\sec { A }
Solve: \cos^{2}\theta +\cos^{2}\theta \cot^{2}\theta-\cot^{2}\theta
\dfrac { \cos { x } }{ { \left( \cos { x } +\sin { x } \right) }^{ 2 } }
If (\sec A+ \tan A -1)(\sec A-\tan A+1)= a\tan A.Find a
If \dfrac{\tan\theta}{(1+\tan^2\theta)^2}+ \dfrac{\cot\theta}{(1+ \cot^2\theta)^2}= m\sin \theta \cos\theta. Find m
If \sin\theta =\dfrac{3}{4} , show that \sqrt{\dfrac{\text{cosec}^2\theta - \cot^2\theta}{\sec^2\theta-1}}=\dfrac{\sqrt m}{3}..Find m
si{n^2}{30^ \circ } + co{s^2}60 + {\tan ^2}{45^ \circ } + se{c^2}{60^ \circ } - co{\sec ^2}{30^ \circ } = \frac{3}{2}
If \dfrac{\sin\theta - \cos\theta +1}{\sin\theta + \cos\theta -1}= \dfrac{m}{\sec\theta - \tan\theta}, Find m
If x= \text{cosec}A + \cos A and y= \text{cosec}A- \cos A then prove that \left(\dfrac{2}{x+y}\right) ^2+ \left(\dfrac{x-y}{2}\right)^2-1=0.
If {\cos}^{4}{\theta}+{\cos}^{2}{\theta}=1 then show that
i) {\sec}^{4}{\theta}-{\sec}^{2}{\theta}=1
ii) {\cot}^{4}{\theta}-{\cot}^{2}{\theta}=1
If A+ B =90^{\circ} then prove \displaystyle \sqrt{\frac{ \tan A\tan B + \tan A\cot B}{\sin A \sec B} -\frac{\sin^{2}B}{\cos^{2}A}} = \tan A
Solve 7cos^{2}\theta + 3 sin^{2}\theta =4.
Evaluate
\dfrac{{\sin {{90}^ \circ }}}{{\cos {{45}^ \circ }}} + \dfrac{1}{{\cos ec{{30}^ \circ }}}
Prove \dfrac{1+\cos{A}+\sin{A}}{1+\cos{A}-\sin{A}}=\dfrac{1+\sin{A}}{\cos{A}}
If \tan\theta =\dfrac{1}{\sqrt{7}}, show that \dfrac{cosec^2\theta -\sec^2\theta}{cosec^2\theta +\sec^2\theta}=\dfrac{3}{4}.
2x\tan ^{ 2 }{ { 60 }^{ o }+3x\sin ^{ 2 }{ { 30 }^{ o } } } =\dfrac { 27\cos ^{ 2 }{ { 45 }^{ o } } }{ 4\sin ^{ 2 }{ { 60 }^{ o } } }
Prove: 1- \dfrac{cos^2A}{1+SinA}= SinA.
Prove:\cfrac{{\cot \theta - \cos \theta }}{{\cot \theta + \cos \theta }} = \cfrac{{{\mathop{\rm cosec}\nolimits} \theta - 1}}{{{\mathop{\rm cosec}\nolimits} \theta + 1}}
If \cot x =2, find the value of \dfrac{(2+2\sin x)(1-\sin x)}{(1+\cos x)(2-2\cos x)}
\dfrac{2}{3}\text{cosec}^258^0 -\dfrac{2}{3}\cot 58^0 \tan32^0-\dfrac{5}{3}\tan 13^0\tan37^0\tan45^0\tan53^0\tan 77^0=-m.Find m
{\sec ^4}x - {\sec ^2}x = {\tan ^4}x + {\tan ^2}x
Prove:\left( {1 + \,{{\tan }^2}\,\theta } \right)\,{\sin ^2}\,\theta \, = \,{\tan ^2}\,\theta
Prove: \sec ^{ 4 }{ \theta } -\tan ^{ 4 }{ \theta } =2\sec ^{ 2 }{ \theta } -1
Prove that \dfrac{\sin \theta-\cos \theta+1}{\sin \theta+ \cos \theta-1}=\dfrac{1}{\sec \theta- \tan \theta } [use the identity \sec^{2} \theta=1+\tan^{2} \theta.]
Prove that \dfrac{2}{1-\sin A}\times \dfrac{1}{1+\sin A}=2\sec^2 A
Evaluate \dfrac {2\cos^{2}90^{o}+4\cos^{2}45^{o}+\tan^{2}60^{o}+3csc^{2}60^{o}+1}{3csc 60^{o}-\dfrac {7}{2}\sec^{2}45^{o}+2\csc 30^{o}-1}
If \sec{\theta}=x+\cfrac{1}{4x} then prove \sec{\theta}+\tan{\theta}=2x
Evaluate:
{ \sin }^{ 2 }{ 5 }^{ o }+{ \sin }^{ 2 }{ 10 }^{ o }+..........{ \sin }^{ 2 }{ 85 }^{ o } ?
Prove: { \left( \tan { \theta } +\sec { \theta } \right) }^{ 2 }+{ \left( \tan { \theta } -\sec { \theta } \right) }^{ 2 }=\cfrac { 2\left( 1+\sin ^{ 2 }{ \theta } \right) }{ \cos ^{ 2 }{ \theta } }
\tan^{2}{\phi}-\sin^{2}{\phi}=\tan^{2}{\phi}.\sin^{2}{\phi}
Solve :
2\tan^{2}{45^{0}}+\cos^{2}{45^{0}}-\sin^{2}{45^{0}}
Solve {\sec ^6}x - {\tan ^6}x - 3{\sec ^2}x.{\tan ^2}
To prove that
\left(\dfrac {1}{\sec^{2}x-\cos^{2}x}+\dfrac {1}{\csc^{2}x-\sin^{2}x}\right)\sin^{2}x.\cos^{2}x
=\dfrac {1-\sin^{2}x \cos^{2}x}{2+\sin x\cos^{2}x}
Find the values of the trigonometric ratio
\tan \theta=11
Show that (\text{cosec}{\theta}-\cot{\theta})^{2}=\dfrac{1-\cos{\theta}}{1+\cos{\theta}}
\dfrac{\cos{A}-1}{2-\sec^{2}{A}}=\dfrac{\cot{A}}{1+\tan{A}}Find A.
If x=a\sin\theta and y=b\tan\theta, then prove that \dfrac{a^{2}}{x^{2}}-\dfrac{b^{2}}{y^{2}}=1.
Express \sec{\theta} in terms of \cot{\theta}
If x=r\sin A\cos C, y=r\sin A\sin C and z=r\cos A, prove that r^{2}=x^{2}+y^{2}+z^{2}.
If \dfrac{\cos{A}}{\cos{B}}=m and \dfrac{\cos{A}}{\sin{B}}=n.Prove:- \left(m^{2}+n^{2}\right)\cos^{2}B=n
Show that \dfrac{{\tan A}}{{1 - \cot A}} + \dfrac{{\cot A}}{{1 - \tan A}} = 1 + \sec A cosecA
Prove that \dfrac { 1 + \cos A } { \sin A } + \dfrac { \sin A } { 1 + \cos A } = 2 \csc A
Evaluate:
\tan ^{ 2 }{ { 30 }^{ o } } \sin { { 30 }^{ o } } +\cos { { 60 }^{ o } } \sin ^{ 2 }{ { 90 }^{ o } } \tan ^{ 2 }{ { 60 }^{ o } } -2\tan { { 45 }^{ o } } \cos ^{ 2 }{ { 0 }^{ o } } \sin { { 90 }^{ o } }
Given sec\: \theta = \dfrac {13}{12}, calculate all other trigonometric ratios
In fig. \triangle PQR right angled at Q,PQ=6cm and PR=12cm. Determine \angle QPR and \angle PRQ.
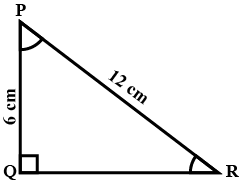
If \tan \theta + \sec \theta =1.5, find \sin \theta.
Evaluate \dfrac { \sin 18 ^ { \circ } } { \cos 72 ^ { \circ } }.
Prove that: \cfrac { \sin { \theta } }{ \left( 1-\cot { \theta } \right) } +\cfrac { \cos { \theta } }{ \left( 1-\tan { \theta } \right) } =\cos { \theta } +\sin { \theta }
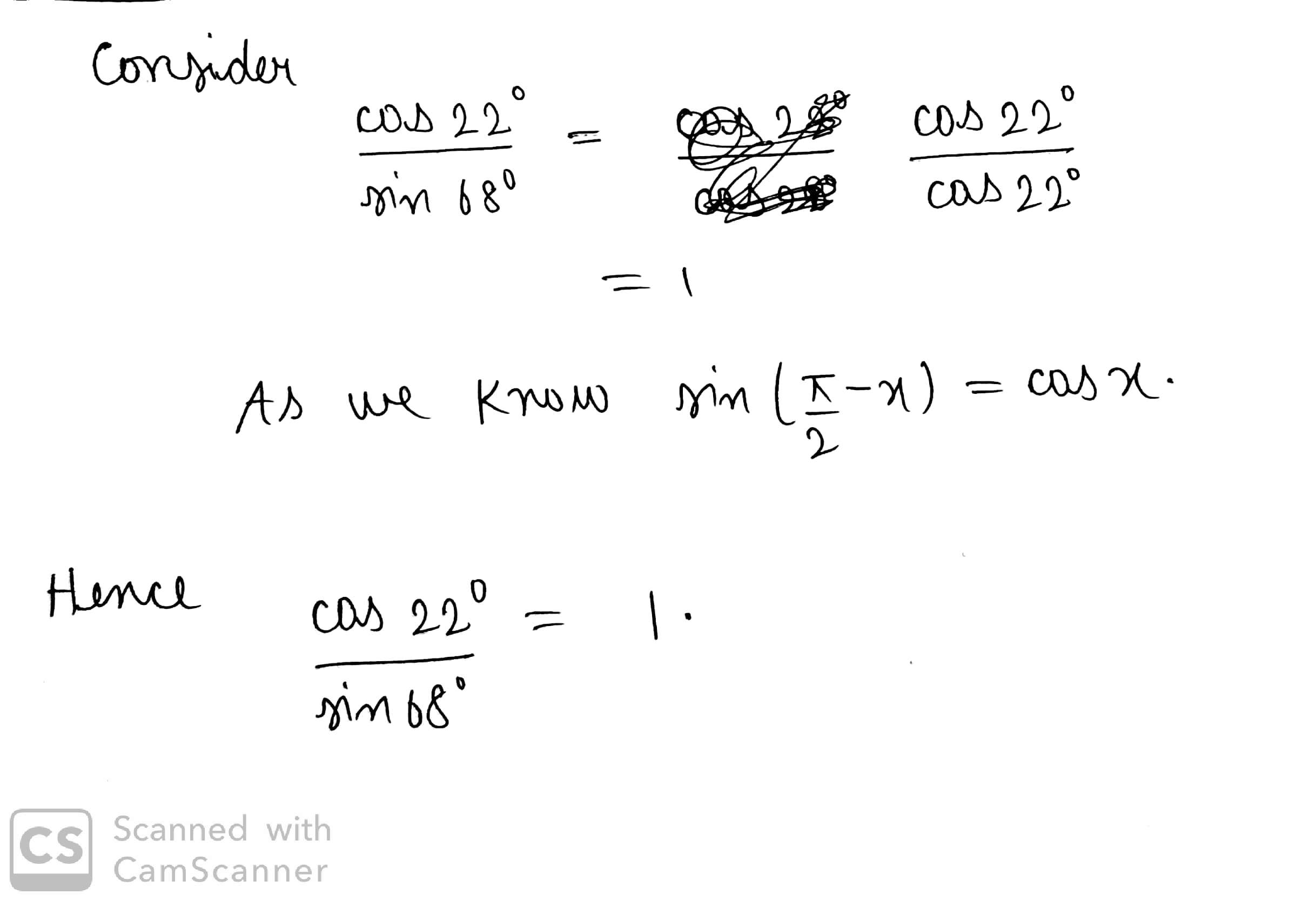
Evaluate : \dfrac{ cos 22^0}{sin 68^0}
If 4tan\theta =3, evaluate \left( \dfrac { 4sin\theta -cos\theta +1 }{ 4sin\theta +cos\theta -1 } \right)
If \cot\theta=3x-\dfrac{1}{12x} then show that \csc\theta+\cot\theta=6x or -\dfrac{1}{6x}.
Evaluate : \dfrac { 4 }{ { cot }^{ 2 }{ 30 }^{ 0 } } +\dfrac { 1 }{ { sin }^{ 2 }{ 30 }^{} } -2{ cos }^{ 2 }{ 45 }^{ 0 }-{ sin }^{ 2 }{ 0 }^{ 0 }
If \cot \theta=\dfrac {15}{8}, find \cos \theta and \csc \theta.
Find the value of 6\tan^{ 2 }\theta -6\sec^{ 2 }\theta .
Evaluate :
\left( 3-\cot{ 30 }^{ \circ } \right) -{ \tan }^{ 3 }{ 60 }^{ \circ }+2\tan{ 60 }^{ \circ }
Prove that :
(\sin\theta+\cos\theta)(\tan\theta+\cot\theta)=\sec\theta+\csc\theta.
The value of tan^2 60^{0} is ?
Evaluate:
\sin {25^0}\cos {65^0} + \cos {25^0}\;\sin {65^0}
If A and B are acute angles such that \tan A = \dfrac {1}{3}, \tan B = \dfrac{1}{2} and \tan(A + B) = \dfrac {\tan A + \tan B}{1 - \tan A \tan B}, show that A + B = 45^{\circ}.
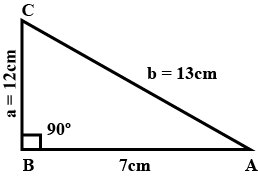
If in \Delta A B C , \angle B = 90 ^ { \circ } , a = 12 \mathrm { cm } , b = 13 \mathrm { cm } , then write all the trigonometric ratios for \angle A and \angle C
Without using tables, evaluate:
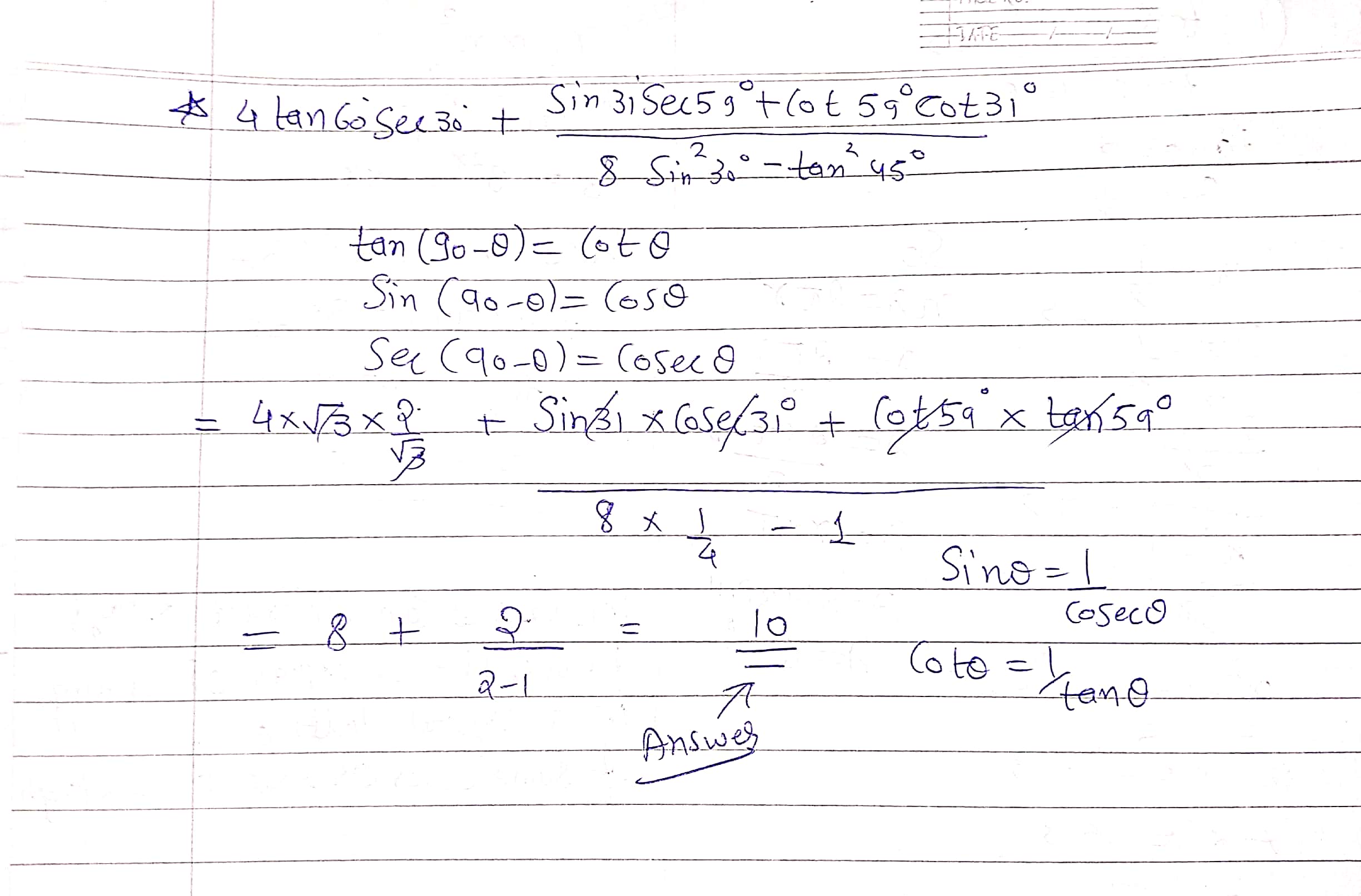
4 \tan 60 ^ { \circ } \sec 30 ^ { \circ } + \dfrac { \sin 31 ^ { \circ } \sec 59 ^ { \circ } + \cot 59 ^ { \circ } \cot 31 ^ { \circ } } { 8 \sin ^ { 2 } 30 ^ { \circ } - \tan ^ { 2 } 45 ^ { \circ } }
In \Delta { ABC } , if \dfrac { \cos { A } } { a } = \dfrac { \cos { B } } { b }, then show that it is an isosceles triangle.
If the angles (2x-10)^{\circ} and (x-5)^{\circ} are complementary angles, find x (in \, degrees).
If \tan \theta = \dfrac { 5 } { 4 }, then find the value of \dfrac { 3 \sin \theta + 4 \cos \theta } { 3 \sin \theta - 4 \cos \theta }.
If sec \theta = 5/4 , show that \left( \dfrac { sin\theta -2cos\theta }{ tan\theta -cos\theta } \right) = \dfrac{12}{7}
If tan \theta = \dfrac{20}{21} ,show that \left( \dfrac { 1-sin\theta +cos\theta }{ 1+sin\theta +cos\theta } \right) = \dfrac{3}{7}
If tan \theta = \dfrac{1}{ \sqrt 7} show that \dfrac{(cosec^2\theta - sec^2 \theta)}{cosec^2 \theta + sec^2 \theta)} = \dfrac{3}{4}
In a right \triangle ABC , right-angled at B, if \tan A = 1, then verify that, 2 sin A. cos A = 1
In a \triangle ABC angle B = 90 degree, AB = 12 cm and BC = 5 cm
Find (i) cos A (ii) cosec A (iii) cos C (iv) cosec C
If 3 cot \theta = 2 ,show that \left( \dfrac { 4sin\theta -3cos\theta }{ 2sin\theta +6cos\theta } \right) = \dfrac{1}{3}
If cot \theta = 3/4, show that
\left( \frac { sec\theta -cos\theta }{ sec\theta +cosec\theta } \right) = \frac{1}{\sqrt{7}}
If sin \theta = \dfrac34, show that \sqrt { \dfrac { cosec^ 2\theta -cot^ 2\theta }{ sec^ 2\theta -1 } } = \dfrac{\sqrt{7}}{3} .
If cos \theta = \dfrac{3}{5} , show that \dfrac { \left( sin\theta -cot\theta \right) }{ 2tan\theta } = \dfrac{3}{160}
If tan \theta = 4/3 , show that (sin \theta + cos \theta) = 7/5
In a \triangle ABC , in which \angle = 90^o , \angle ABC = \theta^o, BC = 21 units, AB = 29 units, show that (cos^2 \theta - sin^2 \theta ) = \dfrac{41}{841}
If sec \theta = \dfrac{17}{8}, verify that \dfrac{3-4 sin^2 \theta}{4 cos^2 \theta - 3} = \dfrac{3- tan^2 \theta}{1 - 3tan^2 \theta}
If 3 \cot \theta = 4 , show that \dfrac{(1-\tan^2 \theta)}{(1+\tan^2 \theta)}=(\cos^2 \theta - \sin^2 \theta) .
Without using trigonometric tables, prove that:
\tan ^{ 2 }{ { 66 }^{ o } } -\cot ^{ 2 }{ { 24 }^{ o } } =0
Without using trigonometric tables, prove that:
\left( \sin { { 65 }^{ o } } +\cos { { 25 }^{ o } } \right) \left( \sin { { 65 }^{ o } } -\cos { { 25 }^{ o } } \right) =0
Without trigonometric tables, prove that:
\cos { { 54 }^{ o } } \cos { { 36 }^{ o } } -\sin { { 54 }^{ o } } \sin { { 36 }^{ o } } =0\quad
Without using trigonometric tables, prove that:
\sin ^{ o }{ { 48 }^{ o } } +\sin ^{ o }{ { 42 }^{ o } } =1
Without using trigonometric tables, prove that:
co\sec ^{ 2 }{ { 72 }^{ o } } -\tan ^{ 2 }{ { 18 }^{ o } } =1
Without using trigonometric tables, prove that:
\cos ^{ 2 }{ { 57 }^{ o } } -\sin ^{ 2 }{ { 33 }^{ o } } =0
Without trigonometric tables, prove that:
\sin { { 53 }^{ o } } \cos { { 37 }^{ o } } +\cos { { 53 }^{ o } } \sin { { 37 }^{ o } } =1
Without trigonometric tables, prove that:
\sec { { 70 }^{ o } } \sin { { 20 }^{ o } } +\cos { { 20 }^{ o } } co\sec { { 70 }^{ o } } =2
Without using trigonometric tables, prove that:
\cos ^{ 2 }{ { 75 }^{ o } } +\cos ^{ 2 }{ { 15 }^{ o } }=1
Without trigonometric tables, prove that:
\sin { { 35 }^{ o } } \sin { { 55 }^{ o } } -\cos { { 35 }^{ o } } \cos { { 55 }^{ o } } =0
Without trigonometric tables, prove that:
\left( \sin { { 72 }^{ o } } +\cos { { 18 }^{ o } } \right) \left( \sin { { 72 }^{ o } } -\cos { { 18 }^{ o } } \right) =0
Prove that:\sec^{2}\theta \textrm{cosec}^{2}\theta = \tan^{2}\theta + \cot^{2}\theta + 2
Prove the following statement:
(\sec A + \cos A) (\sec A - \cos A) = \tan^{2}A + \sin^{2}A.
Prove that
\cfrac { \cos { { 80 }^{ o } } }{ \sin { { 10 }^{ o } } } +\cos { { 59 }^{ o } } co\sec { { 31 }^{ o } } =2
Prove the following statements.
\dfrac {1 + \tan^{2}A}{1 + \cot^{2}A} = \dfrac {\sin^{2}A}{\cos^{2}A}.
Prove that,
\dfrac {\sec A - \tan A}{\sec A + \tan A} = 1 - 2\sec A \tan A + 2\tan^{2}A.
Without trigonometric tables, prove that:
\tan { { 48 }^{ o } } \tan { { 23 }^{ o } } \tan { { 42 }^{ o } } \tan { { 67 }^{ o } } =1
Prove that
\cfrac { \sin { { 70 }^{ o } } }{ \cos { { 20 }^{ o } } } +\cfrac { co\sec { { 20 }^{ o } } }{ \sec { { 70 }^{ o } } } -2\cos { { 70 }^{ o } } co\sec { { 20 }^{ o } } =0
Prove that
\cfrac { 2\sin { { 68 }^{ o } } }{ \cos { { 22 }^{ o } } } -\cfrac { 2\cot { { 15 }^{ o } } }{ 5\tan { { 75 }^{ o } } } -\cfrac { 3\tan { { 45 }^{ o } } \tan { { 20 }^{ o } } \tan { { 40 }^{ o } } \tan { { 50 }^{ o } } \tan { { 70 }^{ o } } }{ 5 } =1\quad
Prove the following statements.
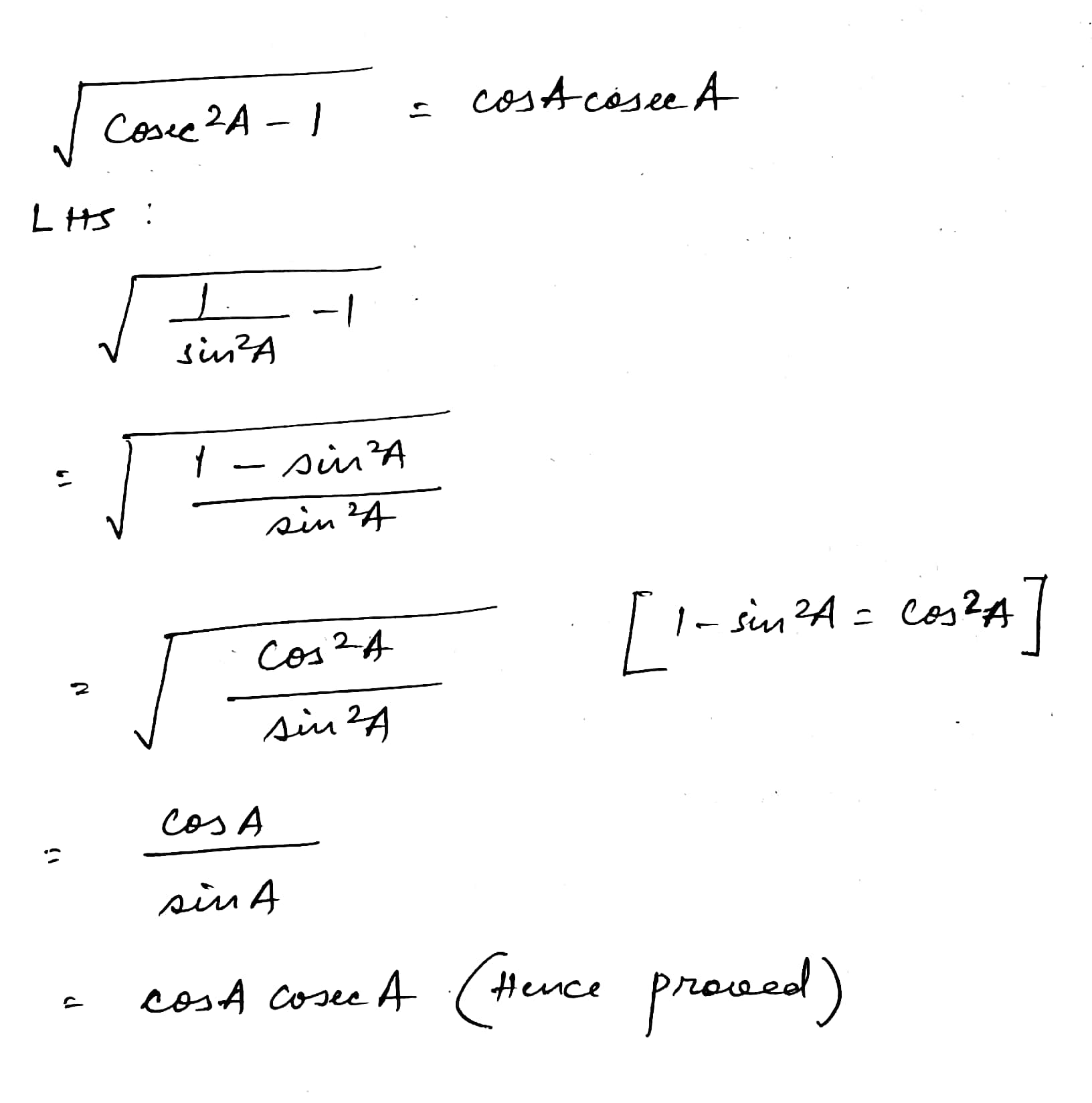
\sqrt {co\sec^{2}A - 1} = \cos A\cdot \csc A.
Prove the following statements.
\tan^{2}A - \sin^{2}A = \sin^{4} A \sec^{2}A.
Prove that following identities, where the angles involved are acute angles for which the trigonometric ratios as defined: \left ( 1-\tan ^{2}A \right )/\left ( \cot ^{2}A-1 \right )=\tan ^{2}A.
If \tan A=1/\sqrt{3}, find all other trigonometric ratios of angle A.
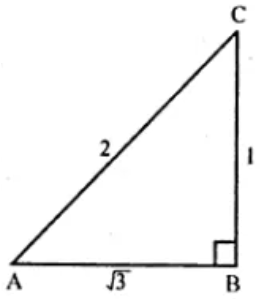
Prove that following identities, where the angles involved are acute angles for which the trigonometric ratios as defined: \cos \theta /\left ( 1-\tan \theta \right )-\sin ^{2}\theta /\left ( \cos \theta -\sin \theta \right )=\cos \theta +\sin \theta .
Prove that:
\left (\dfrac {1}{\sec^{2}\alpha - \cos^{2}\alpha} + \dfrac {1}{\text{cosec}^{2}\alpha - \sin^{2}\alpha}\right ) \cos^{2} \alpha \sin^{2}\alpha = \dfrac {1 - \cos^{2} \alpha \sin^{2} \alpha}{2 + \cos^{2} \alpha \sin^{2} \alpha}.
Without using trigonometric tables, evaluate the following: \left ( \sin 29^{0}/cosec61^{0} \right )+2\cot 8^{0}\cot 17^{0}\cot 45^{0}\cot 73^{0}\cot 82^{0}-3\left ( \sin ^{2}38^{0}+\sin ^{2}52^{2} \right ).
Without using trigonometric tables, evaluate the following: \frac{cosec^{2}\left ( 90-\theta \right )-\tan ^{2}\theta }{2\left ( \cos ^{2}48^{0}+\cos ^{2}42^{0} \right )}-\frac{2\tan ^{2}30^{0}\sec ^{2}52^{0}\sin ^{2}38^{0}}{cosec^{2}70^{0}-\tan ^{2}20^{0}}.
Show that \dfrac {4}{3} \cot^{2} 30^{\circ} + 3\sin^{2} 60^{\circ} - 2cosec^{2} 60^{\circ} - \dfrac {3}{4} \tan^{2} 30^{\circ} = 3\dfrac {1}{3}.
Verify that
\sin^{2} 30^{\circ} + \sin^{2} 45^{\circ} + \sin^{2} 60^{\circ} = \dfrac {3}{2}.
Prove the following statement.
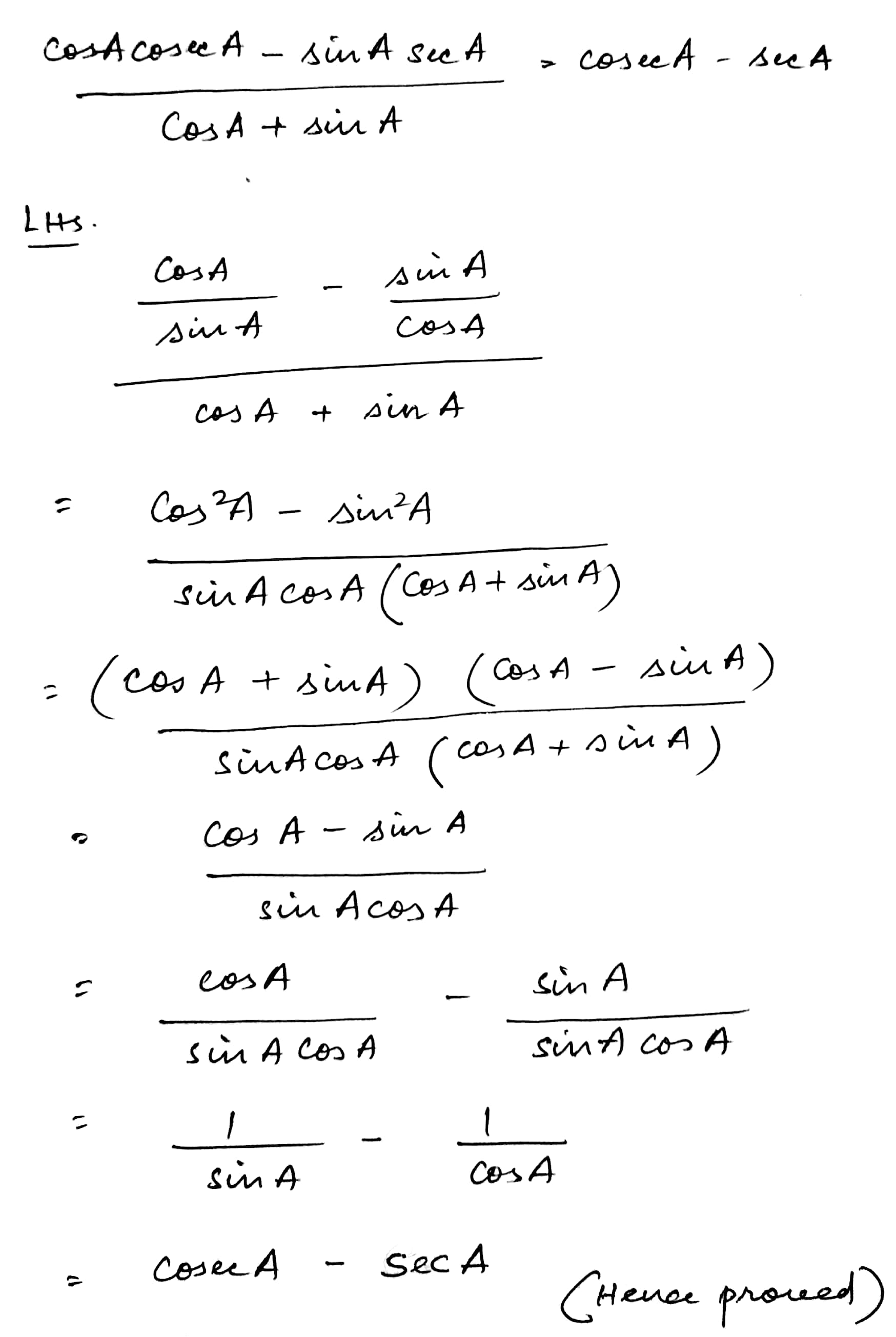
\dfrac {\cos A cosec A - \sin A \sec A}{\cos A + \sin A} = cosec A - \sec A.
Show that
\tan 48^{\circ} \tan 16^{\circ} \tan 42^{\circ} \tan 74^{\circ} = 1
Prove the following identities:
\dfrac{sec A -1}{secA +1} = \dfrac{1-cosA}{1+ cosA}
Show that
\cos 36^{\circ} \cos 54^{\circ} - \sin 36^{\circ} \sin 54^{\circ} = 0
Prove the following identities:
\dfrac{1+sinA}{1-sinA}= \dfrac{cosec A + 1}{cosec A -1}
Evaluate:
3 \dfrac{sin 72^\circ}{cos 18^\circ}- \dfrac{sec 32^\circ}{cosec58^\circ}
Evaluate
\sin 15^{\circ} \sec 75^{\circ}
Evaluate
\tan 26^{\circ} \tan 64^{\circ}
If \sec \theta =\cfrac{13}{5}, show that \cfrac { 2\sin { \theta } -3\cos { \theta } }{ 4\sin { \theta } -9\cos { \theta } } =3
Evaluate
\dfrac{\tan 36^{\circ}}{\cot 54^{\circ}}
2 \dfrac{tan57^\circ}{cot 33^\circ} - \dfrac{cot 70^\circ}{tan20^\circ} - \sqrt{2} cos 45^\circ
\dfrac{cot^2 41^\circ}{tan^2 49^\circ} - 2 \dfrac{sin^2 75^\circ}{cos^2 15^\circ}
Find the value of:
\dfrac{3 sin 72^\circ}{ cos 18^\circ} - \dfrac{sec 32^\circ}{cosec 58^\circ}
Find AD(Refer figure)
\dfrac{5 \ sin 66^\circ}{cos 24^\circ}- \dfrac{2 \ cot 85^\circ}{tan 5^\circ}
sin 27^\circ \ sin 63^\circ- cos 63^\circ \ cos 27^\circ
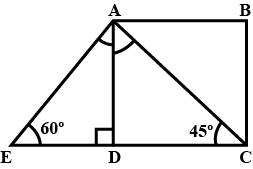
In the given figure, AB and EC are parallel to each other. Sides AD and BC are 2\ cm each and are perpendicular to AB.
Given that \angle AED = 60^{\circ} and \angle ACD = 45^{\circ}. Calculate AB.
Find the length of AB.

Evaluate:
sec 26^\circ \ sin 64^\circ + \dfrac{cosec 33^\circ}{sec 57^\circ}
Evaluate:
2 \left( \dfrac{tan 35^\circ}{cot 55^\circ} \right)^2+ \left(\dfrac{cot 55^\circ}{tan 35^\circ} \right)^2 - 3 \left( \dfrac{sec 40^\circ}{cosec 50^\circ} \right)
Find AD.(Refer figure)

Evaluate: \dfrac{\cos 22^{0}}{\sin68^{0}}
Find AD.(Refer figure)
Find BC.(Refer figure)
Refer given figure, Find AB and BC

Find PQ, if AB = 150\ m, \angle P = 30^{\circ} and \angle Q = 45^{\circ}.
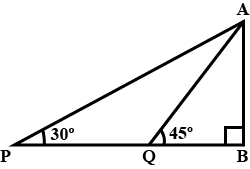
Refer given figure, Find AB and BC.

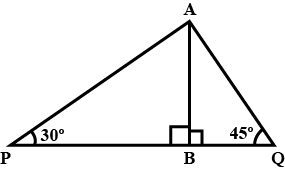
Find PQ, if AB = 150\ m, \angle P = 30^{\circ} and \angle Q = 45^{\circ}.
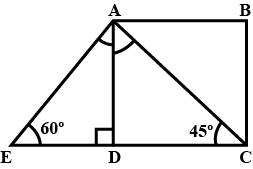
In the given figure, AB and EC are parallel to each other. Sides AD and BC are 2\ cm each and are perpendicular to AB.
Given that \angle AED = 60^{\circ} and \angle ACD = 45^{\circ}. Calculate: AE.

In the given figure, AB and EC are parallel to each other. Sides AD and BC are 2\ cm each and are perpendicular to AB.
Given that \angle AED = 60^{\circ} and \angle ACD = 45^{\circ}. Calculate AC.
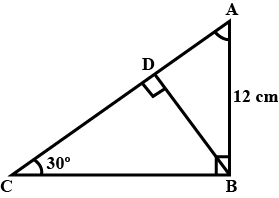
Find AC.(Refer figure)
Evaluate:
\dfrac{\cos55^{0}}{\sin35^{0}}+\dfrac{\cot35^{0}}{\tan55^{0}}
Evaluate:
\dfrac{\cot54^{0}}{\tan36^{0}}+\dfrac{\tan20^{0}}{\cot70^{0}}-2
Evaluate:
\sin42^{0}\sin48^{0}-\cos42^{0}\cos48^{0}
Show that:
\sin42^{0}\sec48^{0}+\cos42^{0}cosec48^{0}=2
Evaluate:
\dfrac{2\tan53^{0}}{\cot37^{0}}-\dfrac{\cot80^{0}}{\tan10^{0}}
Evaluate: \dfrac{\sec75^{0}}{cosec15^{0}}
Evaluate:
3\dfrac{\sin72^{0}}{\cos18^{0}}-\dfrac{\sec32^{0}}{cosec58^{0}}
Show that:
\tan10^{0}\tan15^{0}\tan75^{0}\tan80^{0}=1
Evaluate:
\dfrac{\sin80^{0}}{\cos10^{0}}+\sin59^{0}\sec31^{0}
Evaluate: \dfrac{\tan47^{0}}{\cot43^{0}}
What is the meaning of trigonometry?
Evaluate:
2\dfrac{\tan57^{0}}{\cot33^{0}}-\dfrac{\cot70^{0}}{\tan20^{0}}-\sqrt{2}\cos45^{0}
Find the value of the following :
\dfrac{\cos 37^o}{\sin 53^o}
Evaluate :
cos 48^0 - sin 42^0
Find the value of the following :
\dfrac{\cos 19^o}{\sin 71^o}
Find the value of the following :
\cot 34^o- \tan 56^o
Evaluate:
\dfrac{\cos70^{0}}{\sin20^{0}}+\dfrac{\cos59^{0}}{\sin31^{0}}-8\sin^{2}30^{0}
Find the value of the following :
\dfrac{\tan 10^o}{\cot 80^o}
Evaluate:
\left( \dfrac{\sin 27^o}{\cos 63^o} \right)^2 + \left( \dfrac{\cos 63^o}{\sin 27^o}\right)^2
Prove that
\sin \theta \, cosec \theta + \cos \theta \sec \theta = 2 .
Solve:
\sqrt {\dfrac {\sec \theta +1}{\sec \theta -1}}=\cot \theta +\csc \theta
Find the value of the following :
\dfrac{\sin 36^o}{\cos 54^o} - \dfrac{\sin 54^o}{\cos 36^o}
Evaluate:
\sin 70^o \sin 20^o - \cos 20^o \cos 70^o
Evaluate:
\dfrac{2 \cos 67^o}{\sin 23^o}- \dfrac{\tan 40^o}{\cot 50^o}- \cos 60^o
Evaluate:
\left( \dfrac{\sin 35^o}{\cos 55^o} \right)^2 + \left( \dfrac{\cos 55^o}{\sin 35^o}\right)^2 -2 \cos 60^o
Prove :
\tan \theta + \tan \left( {{{90}^ \circ } - \theta } \right) = \sec \theta \sec \left( {{{90}^ \circ } - \theta } \right)
If \displaystyle \frac {9x}{\cos\theta}+\frac {5y}{\sin\theta}=56 and \displaystyle \frac {9x \sin\theta}{\cos^2\theta}-\cfrac {5y \cos\theta}{\sin^2\theta}=0, then the value of [(9x)^{2/3}+(5y)^{2/3}]^3 is
Express the trigonometric ratios \sin A, \sec A and \tan A in terms of \cot A.
If \text{cosec} \theta -\sin \theta =m and \sec \theta - \cos \theta =n, then find the value of (m^{2}n)^{2/3} + (mn^{2})^{2/3}.
Class 10 Maths Extra Questions
- Areas Related To Cricles Extra Questions
- Arithmetic Progressions Extra Questions
- Circles Extra Questions
- Constructions Extra Questions
- Coordinate Geometry Extra Questions
- Introduction To Trigonometry Extra Questions
- Pair Of Linear Equations In Two Variables Extra Questions
- Polynomials Extra Questions
- Probability Extra Questions
- Quadratic Equations Extra Questions
- Real Numbers Extra Questions
- Some Applications Of Trigonometry Extra Questions
- Statistics Extra Questions
- Surface Areas And Volumes Extra Questions
- Triangles Extra Questions