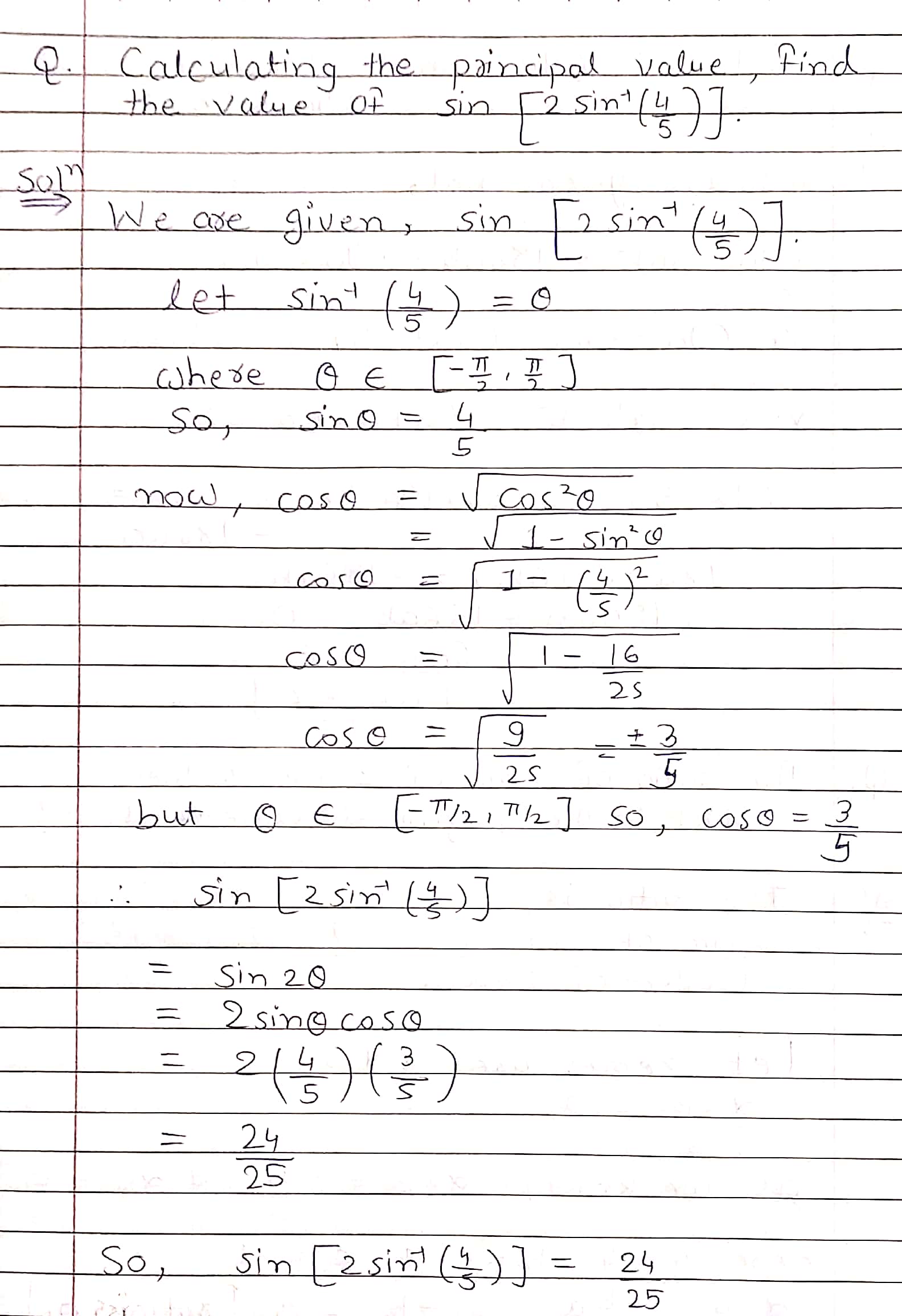Inverse Trigonometric Functions - Class 12 Engineering Maths - Extra Questions
sin−1x+cos−1x= ________
If xy<1, tan−1x+tan−1y= _______.
Prove that tan−1(1)+tan−1(2)+tan−1(3)=π
If f:(−π2,π2)→(−∞,∞) is defined by f(x)=tanx, then f−1(√3)=
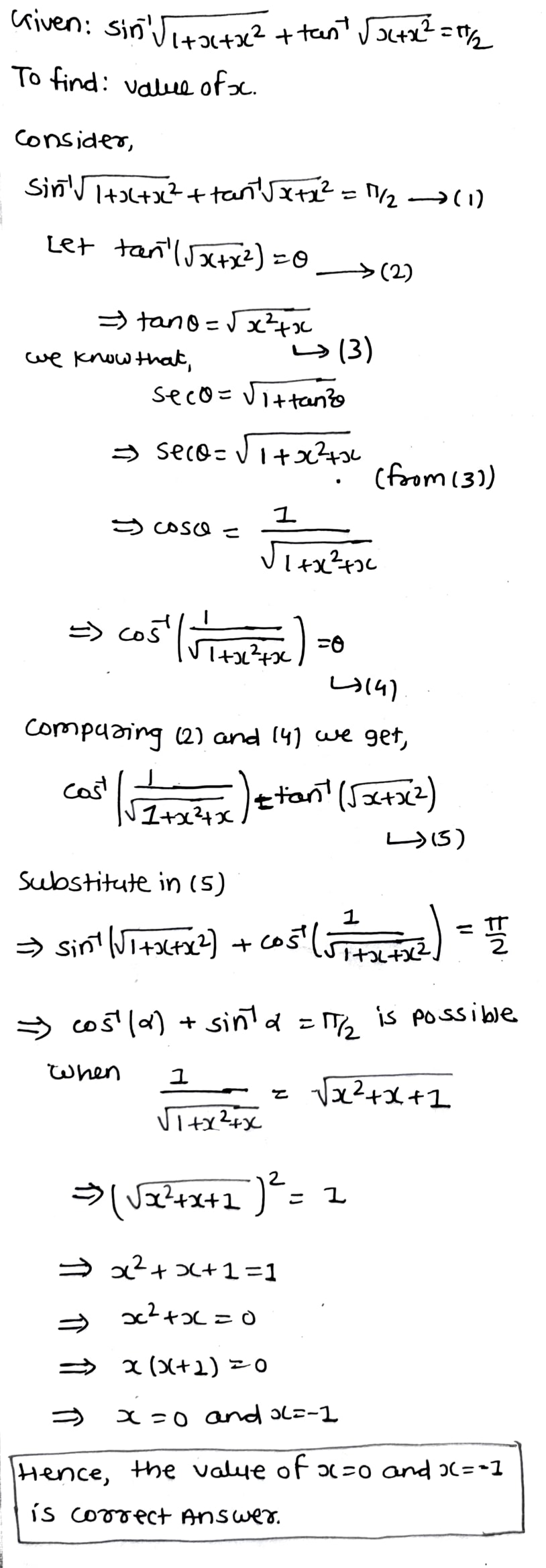
If sin−1√1+x+x2+tan−1√x+x2=π2
thenx=
Simplify sin(sin−1(15)+cos−1(x))=1
Solve sin−1√x
If tan−1(12i2)=t, then tant equals
Prove tan−134+tan−135−tan−1819=π4.
Evaluate : cos[cos−1(−√32)+π6]
If sin−1x=π5, for some x∈(−1,1), then find the value of cos−1x.
Solve sin−1(cosx)
Evaluate: tan−1√3−sec−1(−2)+cosec−12√3.
Prove that sincot−1tancos−1x=sincosec−1cottan−1x=x where xϵ(0,1]
Write the function in the simplest form:
tan−11√x2−1,|x|>1
Prove: 2tan−112+tan−117=tan−13117
Prove: tan−1211+tan−1724=tan−112
If tan−1x−1x−2+tan−1x+1x+2=π4, then find the value of x.
If sin(sin−115+cos−1x)=1, then find the value of x.
Find the value of tan12[sin−12x1+x2+cos−11−y21+y2],|x|<1,y>0 and xy<1
Prove: 9π8−94sin−113=94sin−12√23
If A=11cot−1(11)+12cot−1(12)+13cot−1(13) and B=1cot−11+2cot−12+3cot−13 then |B−A| is equal to aπb+cdcot−13 where a,b,c,d∈N and are in their lowest form then a+b+c+d equal to
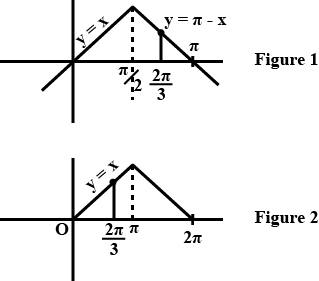
Solve the equation: tan−1x+2cot−1x=2π3.
Write the simplest form of tan−1(cosx−sinxcosx+sinx),0<x<π2.
Write tan−1[√1+x2−1x],x≠0 in the simplest form.
If sin(sin−115+cos−1x)=1 then find the value of x.
Inverse circular functions,Principal values of sin−1x,cos−1x,tan−1x.
tan−1x+tan−1y=tan−1x+y1−xy, xy<1
π+tan−1x+y1−xy, xy>1.
(a) cos−1(cos7π6)
(b) cos−1(cos4π3)
Inverse circular functions,Principal values of sin−1x,cos−1x,tan−1x.
tan−1x+tan−1y=tan−1x+y1−xy, xy<1
π+tan−1x+y1−xy, xy>1.
(a) tan−12mnm2−n2+tan−12pqp2−q2=tan−12MNM2−N2
where M=mp−nq,N=np+mq
(b) 23tan−13mn2−m3n3−3m2n+23tan−13pq2−p3q3−3p2q=tan−12MNM2−N2
Where M=−mp+nq,N=np+mq and all the letters are +ive quantities.
Solve the equation tan−1√x2+x+sin−1√x2+x+1=π2
If cot−1(√1+sinx+√1−sinx√1+sinx−√1−sinx)=xm,x∈(0,π4).Find m
If (Tan−1x)2+(Cot−1x)2=5π28,then x=
find the value of the following:
(i) sin−1(−12)
(ii) cos−1(√32)
(iii) cosec−1(2)
(iv) tan−1(−√3)
(v) cos−1(−12)
(vi)tan−1(−1)
Differentiate cos−1(4x2−3x);xϵ(12,1)
Solve the following for x:tan−1(x−2x−3)+tan−1(x+2x+3)=π4,|x|<1
Simplify : cot−1[√1+x2−x]
Does sin−1(2√5)=tan−1(2) & please give value.
Find the domain and range of the real function x+2x2−8x−4
Solve y=tan−15axa2−6x2, find dydx
Calculating the principal value, find the value of sin[2sin−1(45)].
Find sin−1(√3+12√2)=
Prove that sin−135−sin−1817=cos−1(8485)
2tan−1(1+x1−x)+sin−1(1−x21+x2)=
Prove
4\tan^{-1}\left(\dfrac{1}{5}\right)-\tan^{-1}\left(\dfrac{1}{70}\right)+\tan^{-1}\left(\dfrac{1}{99}\right)=\dfrac{\pi}{4}.
Find the value of \sin\left(\dfrac{1}{2}\cot^{-1}\left(-\dfrac{3}{4}\right)\right).
if\int \sin^{-1}\left ( \dfrac{2x+2}{\sqrt{4x^2+8x+13}} \right )dx=(x + 1)tan^{-1}\left ( \dfrac{2x+2}{3}\right )+\lambdaln(4x^2+8x+13)+C
then find the value of - 4\lambda
If 0< \cos^{-1} x< 1 and 1+\sin (\cos^{-1}x)+\sin^{2}(\cos^{-1}x)+\sin^{3} (\cos^{-1}x)+.........\infty =2, then find 2\sqrt{3}x.
Find the number of values of x of the form 6n, where n is an integer , in the domain of the function f(x)=x\ln|x-1|+\displaystyle \frac{\sqrt{64-x^{2}}}{\sin x}
If \displaystyle 0< \cos ^{-1}x< 1 and \displaystyle 1+\sin(\cos^{-1}x)+\sin^{2}(\cos^{-1}x)+\sin^{3}(\cos^{-1}x)+...infinity= 2 then the value of \displaystyle 12 x^{2} is
If the domain of the function \displaystyle f(x)= \sqrt{3\cos^{-1}(4x)-\pi} is [a,b] then the value of \displaystyle (4a+64b) is
If \displaystyle x= \sin^{-1}(a^{6}+1)\cos^{-1}(a^{4}+1)-\tan^{-1}(a^{2}+1),a\epsilon R , then the value of \displaystyle \sec^{2}x is
If range of the function \displaystyle f(x)= \sin^{-1}x+2\tan^{-1}x+x^{2}+4x+1 is [p,q], then the value of (p+q) is
Prove that
2\tan^{-1} \dfrac {1}{2} + \tan^{-1}\dfrac {1}{7} = \tan^{-1} \dfrac {31}{17}
Prove that \tan^{-1} \left [\dfrac {\sqrt {1 + x} + \sqrt {1 - x}}{\sqrt {1 + x} - \sqrt {1 - x}}\right ] = \dfrac {\pi}{4} + \dfrac {1}{2} \cos^{-1} x, 0 < x < 1
The number of real solutions of the equation
\sin^{-1}\left(\displaystyle\sum^{\infty}_{i=1}x^{i+1}-x\displaystyle\sum^{\infty}_{i=1}\left(\displaystyle\dfrac{x}{2}\right)^i\right)=\dfrac{\pi}{2}-\cos^{-1}\left(\displaystyle\sum^{\infty}_{i=1}\left(-\displaystyle\frac{x}{2}\right)^i-\displaystyle\sum^{\infty}_{i=1}(-x)^i\right) lying in the interval \left(-\displaystyle\frac{1}{2}, \frac{1}{2}\right) is
Using principal values, evaluate the following
\cos^{-1}(\cos\dfrac{2\pi}{3})+ \sin^{-1}(\sin\dfrac{2\pi}{3})
\dfrac{d}{dx}\sin ^{-1}\left(2x\sqrt{1-x^2}\right)=.......|x|>\dfrac{1}{\sqrt{2}}
Find the value of \sin (\cfrac{1}{4}\sin^{-1}\cfrac{\sqrt 63}{8})
Prove that \tan^{-1}\dfrac{1}{7}+\tan^{-1}\dfrac{1}{13}-\tan^{-1}\dfrac{2}{9}=0
{\sin ^{ - 1}}\sqrt {1 + x + {x^2}} + {\tan ^{ - 1}}\sqrt {x + {x^2}} = \frac{\pi }{2}
then\,x =
\tan^{-1}\left(\dfrac{5-x}{6x^2-5x-3}\right).
\cot^{-1} \dfrac {1 - x}{1 + x}.
\tan^{-1}\left(\dfrac{4x}{1+5x^2}\right)+\tan^{-1}\left(\dfrac{2+3x}{3-2x}\right).
Find the value of \sin{\left[\dfrac{1}{2}\cot^{-1}{\left(\dfrac{-3}{4}\right)}\right]}.
Class 12 Engineering Maths Extra Questions
- Continuity And Differentiability Extra Questions
- Determinants Extra Questions
- Differential Equations Extra Questions
- Integrals Extra Questions
- Inverse Trigonometric Functions Extra Questions
- Relations And Functions Extra Questions
- Three Dimensional Geometry Extra Questions
- Vector Algebra Extra Questions