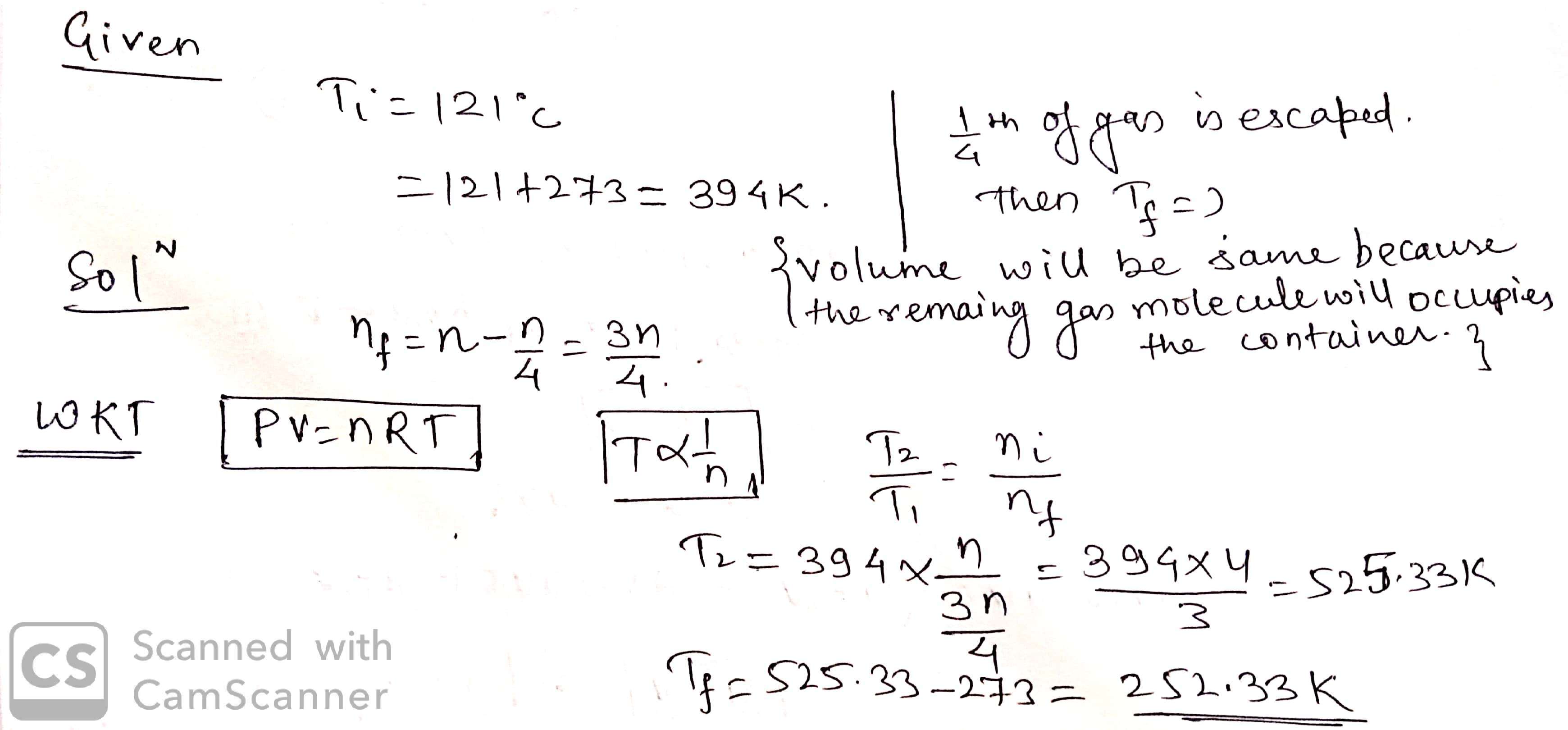Kinetic Theory - Class 11 Medical Physics - Extra Questions
Gas A four times denser than gas B. Find the ratio between their rates of diffusion.
State Charles law at constant pressure and constant volume
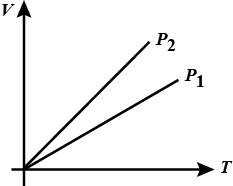
State whether P1>P2 or P2>P1 for given mass of a gas ?
An ideal gas is taken through a complete cycle in three steps: adiabatic expansion with work equal to 125 J, isothermal contraction at 325 K and increase in pressure at constant volume.
Is it transferred to or from the gas?
The temperature of 3.00 mol of a gas with Cv=6.00 cal/mol K is to be raised 50.0 K. If the process is at constant volume, If the process is at constant pressure, what are ΔK?
An ideal gas initially at 300 K is compressed at a constant pressure of 25 N/m2 from a volume of 3.0 m3 to a volume of 1.8 m3. In the process 75 J is lost by the gas as heat. What are the change in internal energy of the gas?
Two ideal gas thermometers A and B use oxygen and hydrogen respectively. The following observations are made
| Temperature | Pressure thermometer A | Pressure thermometer B |
| Triple-point of water | 1.250×105Pa | 0.200×105Pa |
| Normal melting point of sulphur | 1.797×105Pa | 0.287×105Pa |
(b) What do you think is the reason behind the slight difference in answers of thermometers A and B ? (The thermometers are not faulty) What further procedure is needed in the experiment to reduce the discrepancy between the two readings ?
Describe an experiment to verify the Boyles law.
How will you show that air supports burning?
Fill in the empty values of the cells in the table:
| V1 | T1 | V2 | T2 | |
| A | 840 K | 1070 ml | 147 K | |
| B | 3250 ml | 4750 | 500 | |
| C | 10 Litre | 15 Litre | 500 |
The volume of an air bubble becomes three times as it rises from the bottom of a lake to its surface. Assuming atmospheric pressure to be 75 cm of Hg and the density of water to be 1/10 of the density of mercury, the depth of the lake.
The temperature of 3.00 mol of a gas with Cv=6.00 cal/mol K is to be raised 50.0 K. If the process is at constant volume, If the process is at constant pressure, what are W?
The temperature of 3.00 mol of a gas with Cv=6.00 cal/mol K is to be raised 50.0 K. If the process is at constant volume, If the process is at constant pressure, what are Q.
During a compression at a constant pressure of 250 Pa, the volume of an ideal gas decreases from 0.80 m3 to 0.20 m3. The initial temperature is 360 K, and the gas loses 210 J as heat. What are the final temperature of the gas?
An ideal gas initially at 300 K is compressed at a constant pressure of 25 N/m2 from a volume of 3.0 m3 to a volume of 1.8 m3. In the process 75 J is lost by the gas as heat. What are the final temperature of the gas?
When air is pumped into a cycle tyre the volume and pressure of the air in the tyre both are increased. What about Boyle's law in this case?
We have 0.5g of hydrogen gas in a cubic chamber of size 3cm kept at NTP. The gas in the chamber is compressed keeping the temperature constant till a final pressure of 100atm. Is one justified in assuming the ideal gas law, in the final state? (Hydrogen molecules can be consider as sphere of radius 1˚A)
An ideal gas under goes adiabatic expansion to twice original volume. If the gas was initially at 40∘C, calculate its temperature after the process.
[Take1=53]
A gas is found to obey PV2=Constant. The initial volume and temperature of the gas are Vi and Ti respectively. If the final volume of the gas is Vf, find its final temperature (Tf).
State and explain Charles law.
Review. At 25.0 m below the surface of the sea, where the temperature is 5.000C, a diver exhales an air bubble having a volume of 1.00CM3. If the surface temperature of the sea is 20.00C, what is the volume of the bubble just before it breaks the surface?
One mole of an ideal gas goes through a process in which the entropy of the gas changes with temperature T as S=aT++Cv, In T, where a is a positive constant. Cv, is the molar heat capacity of this gas at constant volume. Find the volume dependence of the gas temperature in this process if T=T0 at V=V0.
A room of volume V contains air having equivalent molar mass M (in g/mol). If the temperature of the room is raised from T1 to T2, what mass of air will leave the room? Assume that the air pressure in the room is maintained at P0.
Air in a thundercloud expands as it rises. If its initial temperature is 300 K and no energy is lost by thermal conduction on expansion, what is its temperature when the initial volume has doubled?
We have two vessels of equal volume, one filled with hydrogen and the other with an equal mass of Helium. The common temperature is 27∘C.
If the temperature of Helium is kept at 27∘C and that of hydrogen is changed, at what temperature will its pressure become equal to that of helium? The molecular weights of hydrogen and helium are 2 and 4 respectively.
A gas is filled in a container of volume V at 121C. To what temperature should it be heated in order that 14th of the gas may escape out of the vessel?
An air bubble of volume 20 cm3 is at the bottom of a lake 40 m deep, where the temperature is 4.0oC. The bubble rises to the surface, which is at a temperature of 20oC. Take the temperature of the bubble's air to be the same as that of the surrounding water. Just as the bubble reaches the surface, what is its volume?
In the temperature range 310 K to 330 K, the pressure p of a certain nonideal gas is related to volume V and temperature T by
p=(24.9 J/K)TV−(0.00662 J/K2)T2V.
How much work is done by the gas if its temperature is raised from 315 K to 325 K while the pressure is held constant?
Give the laws of Boyle, Charles, Gay-Lussac and Dalton by writing the postulates of kinetic theory of gases.
Class 11 Medical Physics Extra Questions
- Gravitation Extra Questions
- Kinetic Theory Extra Questions
- Laws Of Motion Extra Questions
- Mechanical Properties Of Fluids Extra Questions
- Mechanical Properties Of Solids Extra Questions
- Motion In A Plane Extra Questions
- Motion In A Straight Line Extra Questions
- Oscillations Extra Questions
- Systems Of Particles And Rotational Motion Extra Questions
- Thermal Properties Of Matter Extra Questions
- Units And Measurement Extra Questions
- Waves Extra Questions
- Work, Energy And Power Extra Questions