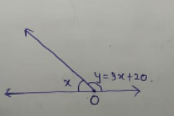
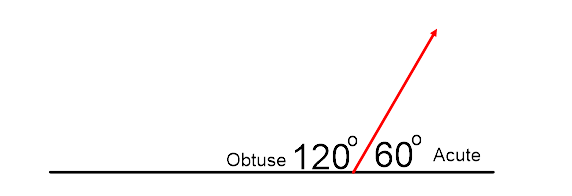
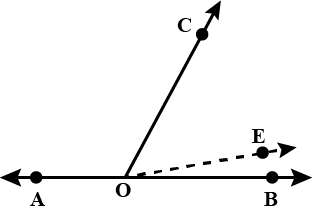
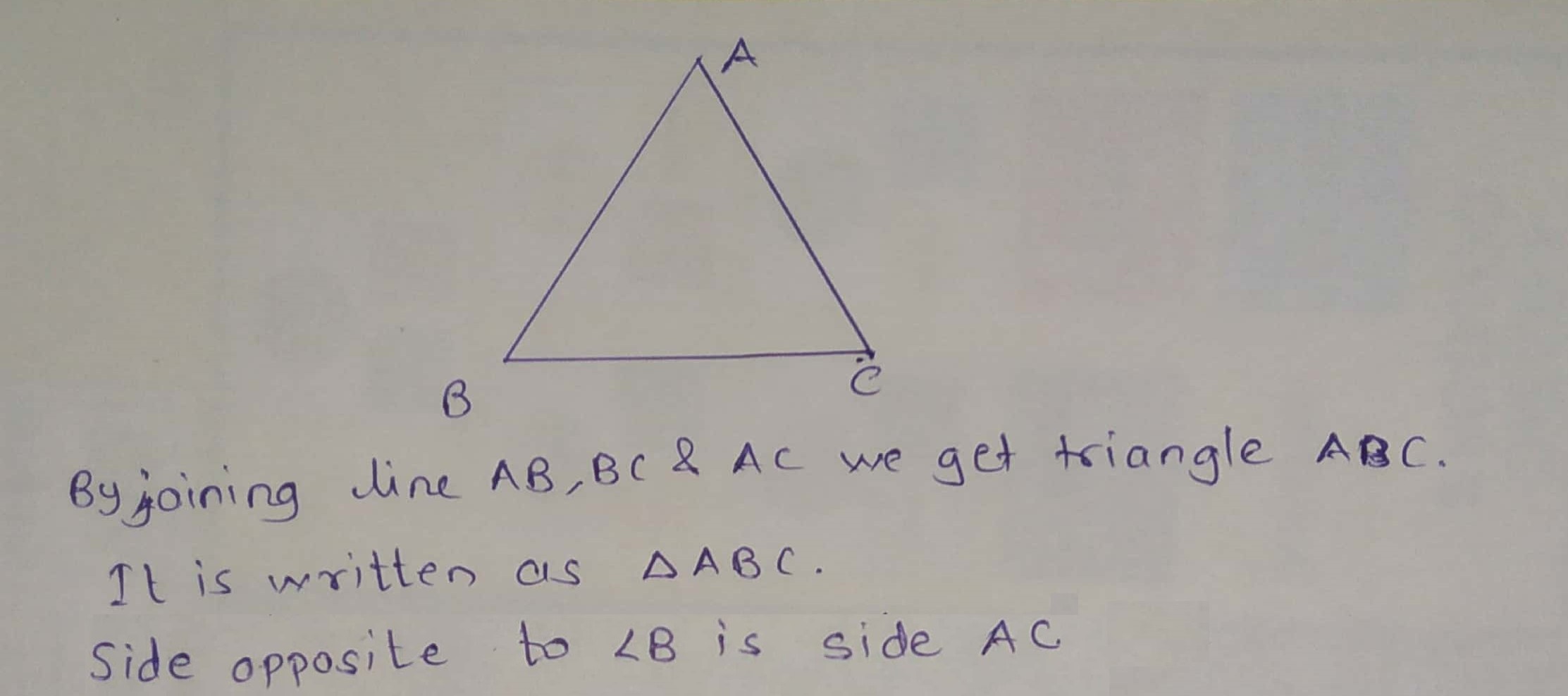
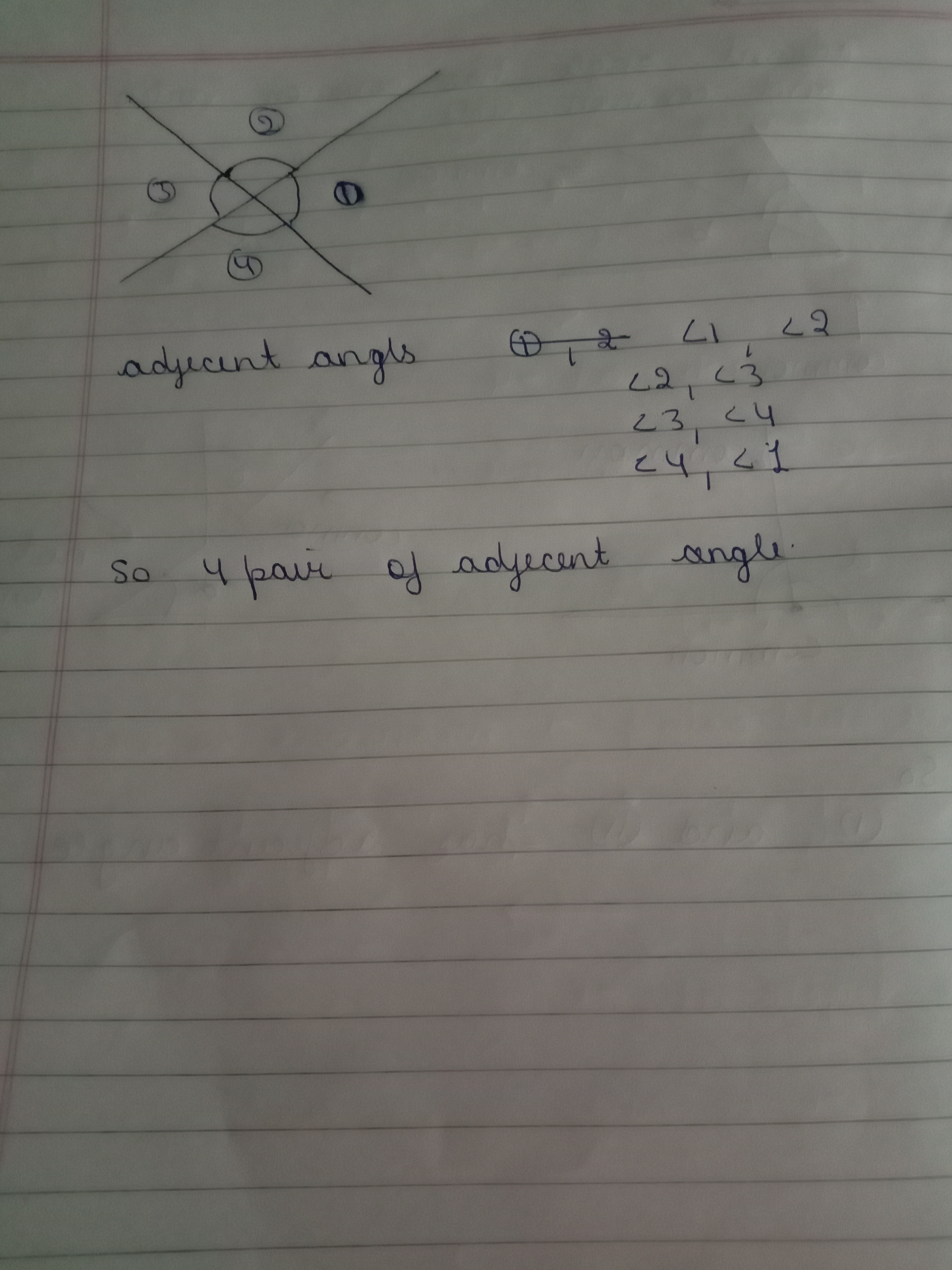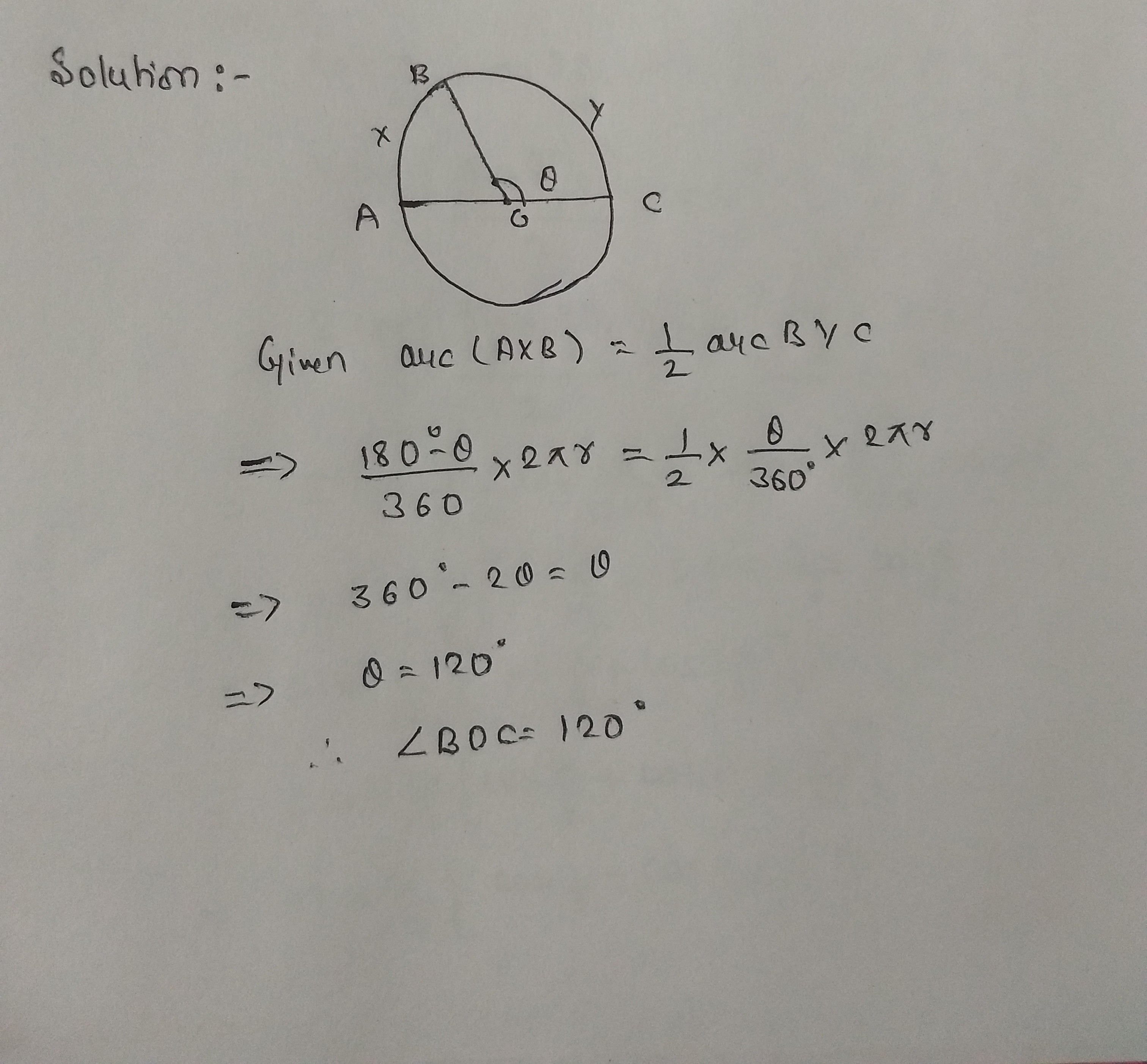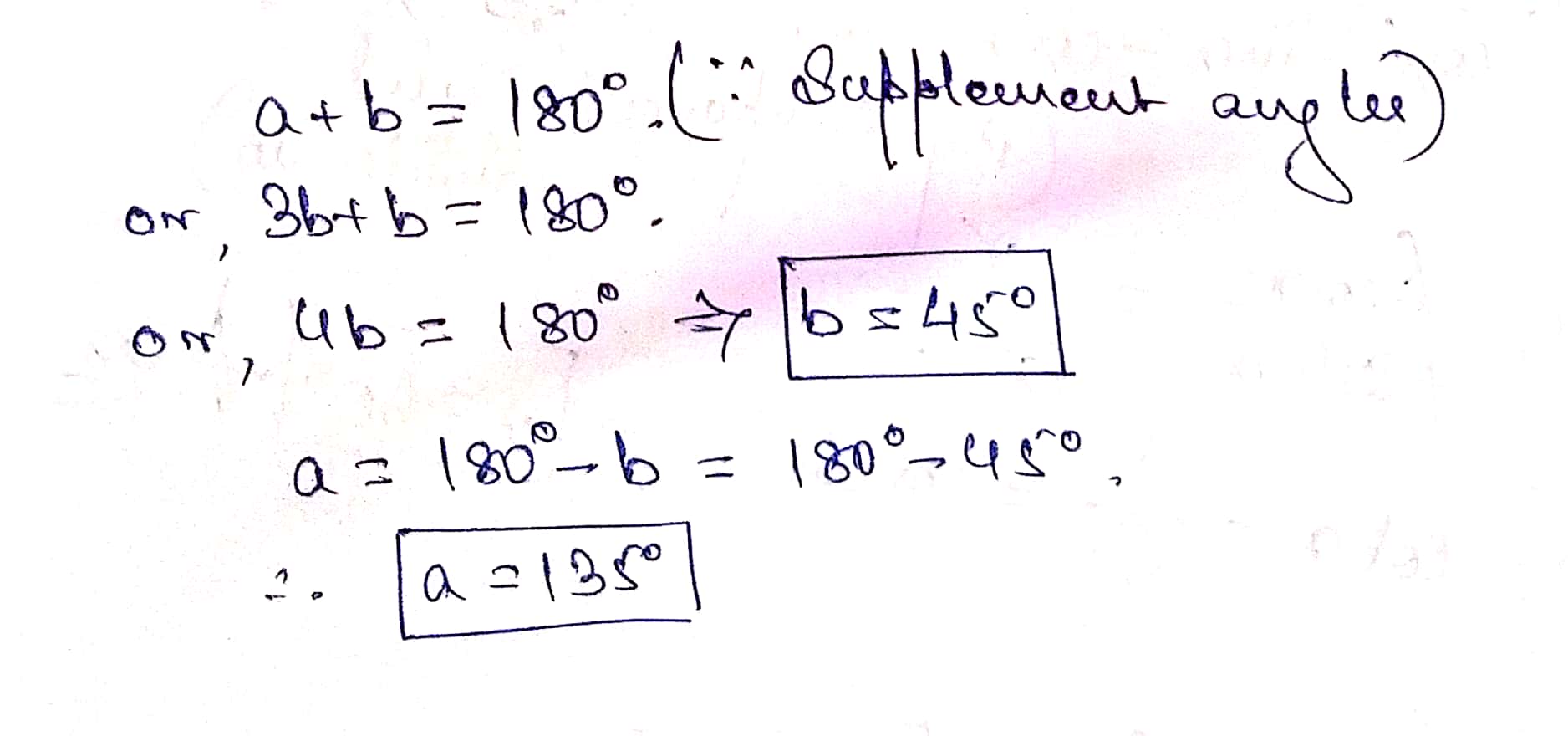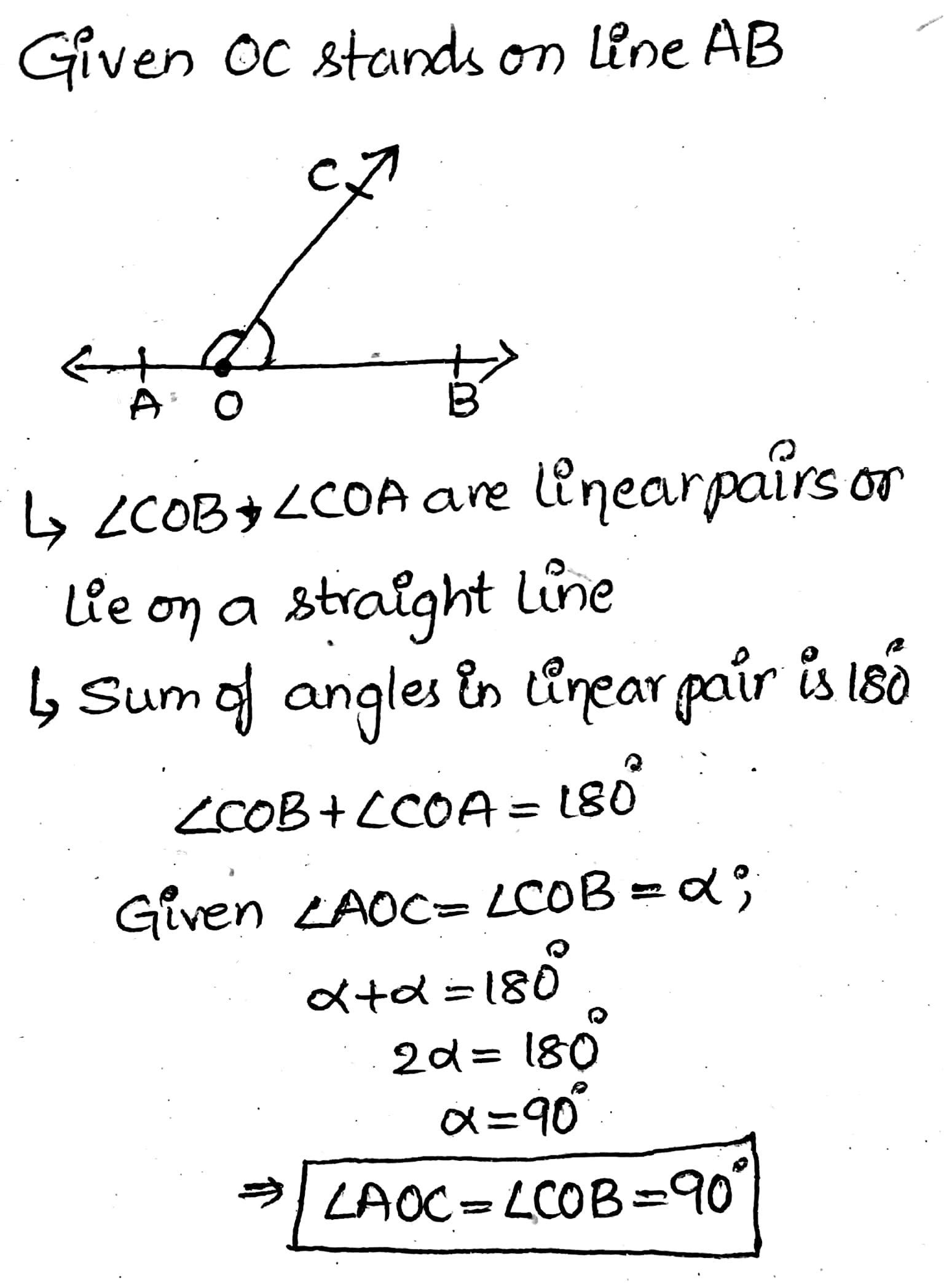Lines And Angles - Class 9 Maths - Extra Questions
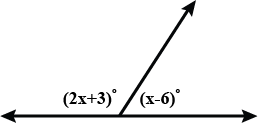
Draw a line segment AB of 4 cm in length. Draw a line perpendicular to AB through A and B, respectively. Are these lines parallel?
If true write 1 and if false then write 0
Can two acute angles be complement to each other always?
If true, then enter 1 and if false, then enter 0.
Can two acute angles be complement to each other always?
If true then enter 1 and if false then enter 0
Can two obtuse angles be adjacent angles?
If true then enter 1 and if false then enter 0
Can two right angles be supplementary?
If true then enter 1 and if false then enter 0
Can two adjacent angles be supplementary?
Measure of an angle is given below. Find the measure of it's complementary angle:
58^o.
If true then enter 1 and if false then enter 0
Can two acute angles form a linear pair?
Measures of some angles are given below. Find the measures of their supplementary angles.
108^o
Measures of some angles are given below. Find the measure of their complementary angle:
56^o
Measures of some angles are given below. Find the measures of their complementary angles:
16^o.
Measures of some angles are given below. Find the measures of their complementary angles:
68^o
Find the measure of the supplementary angle pair of 60^o. (in degrees)
Measures of some angle is given below. Find the measures of its complementary angle:
If the angle is \displaystyle \frac{2}{3}rd of a right angle.
Find the angle which is equal to its complement.(in ^\circ)
Find the measures of the third angle in degrees \\ 92^{\circ}, 27^{\circ},----
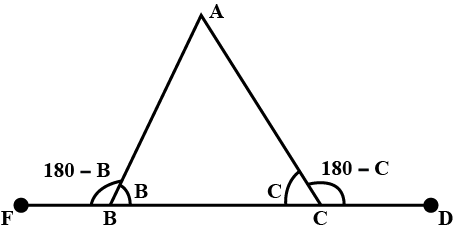
Side BC of a \Delta ABC is produced in both the directions. Prove that the sum of the two exterior angles so formed is greater than 180^o.
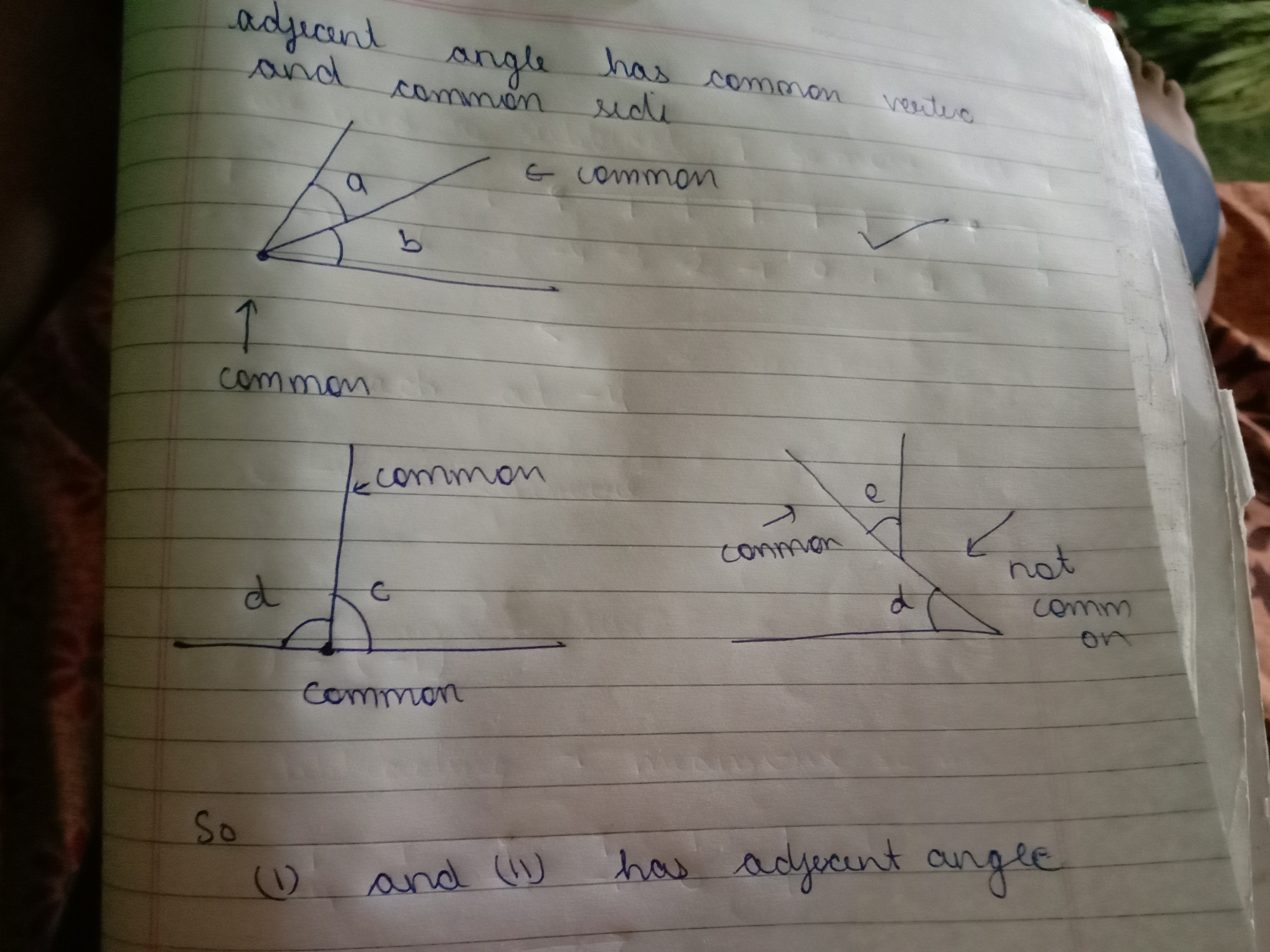
When do you say two angles are adjacent?
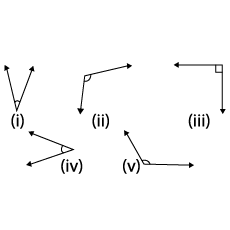
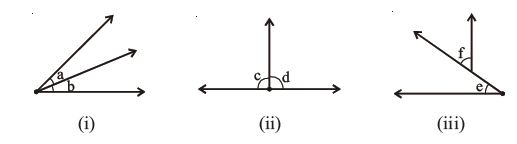
Observe the following figures and identify the type of angles in them
Identify the following given angles as acute , right or obtuse.
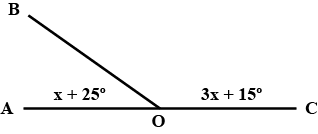
In each of the following figure AB || CD. Find the value of x in each case.
Find the supplement of the following angles.
58^{\circ}
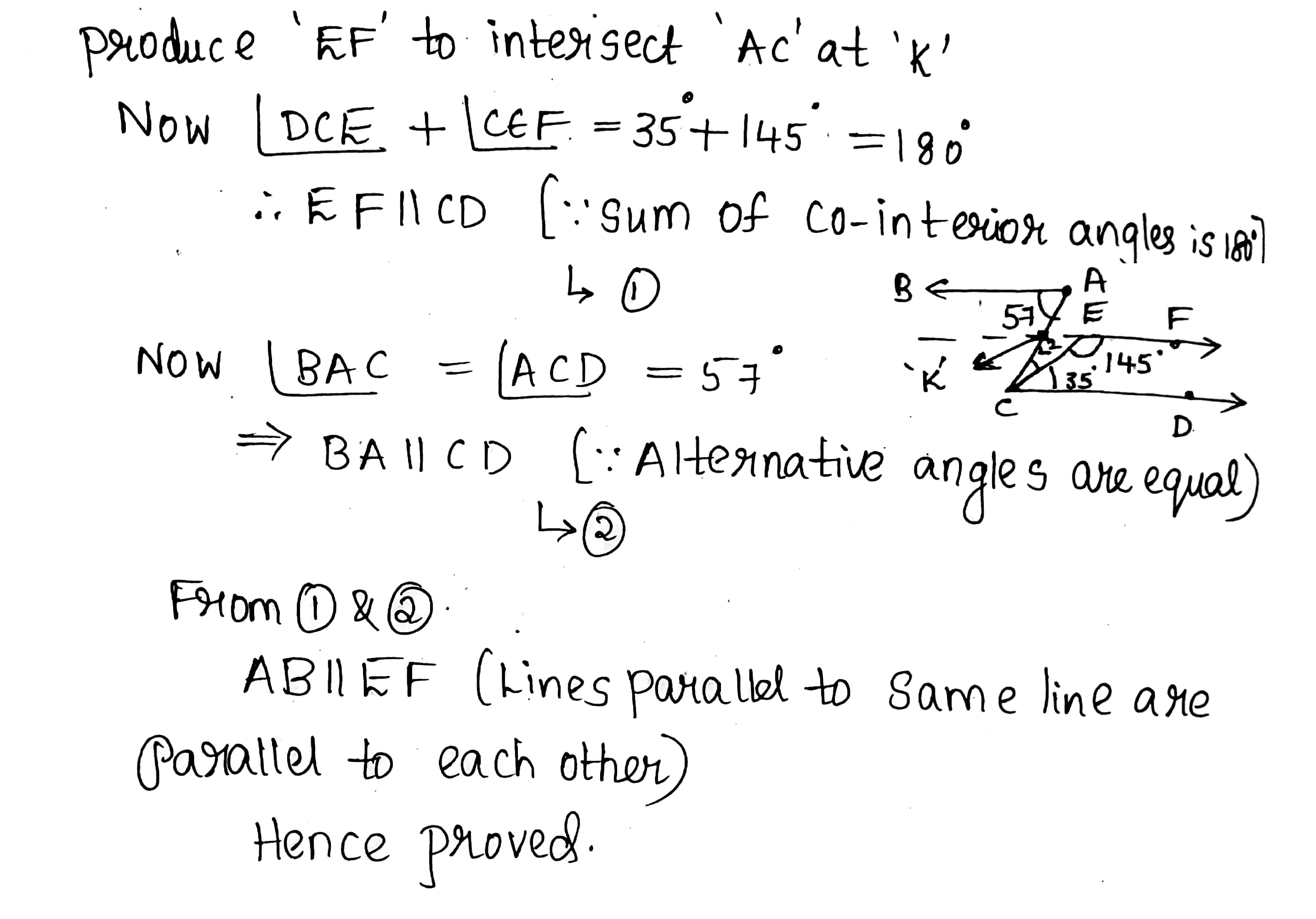
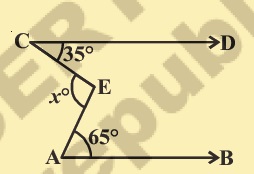
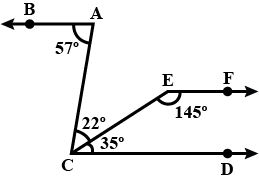
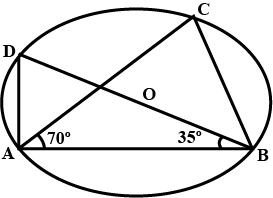
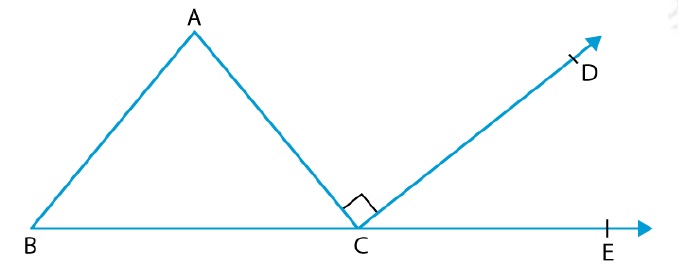
In the given figure if AB || DE, \angle BAC = 35^o and \angle CDE = 53^o, find \angle DCE.

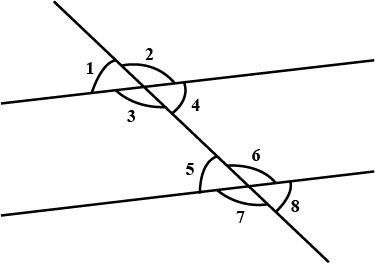
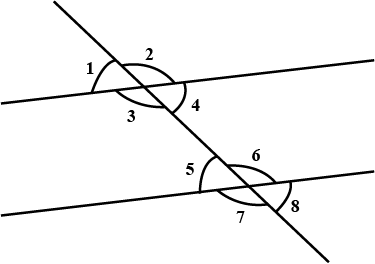
To prove: \angle 1 is supplementary to \angle 8. Write reasons for the statement.
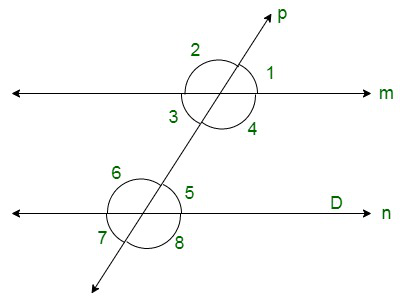
Statement Reason
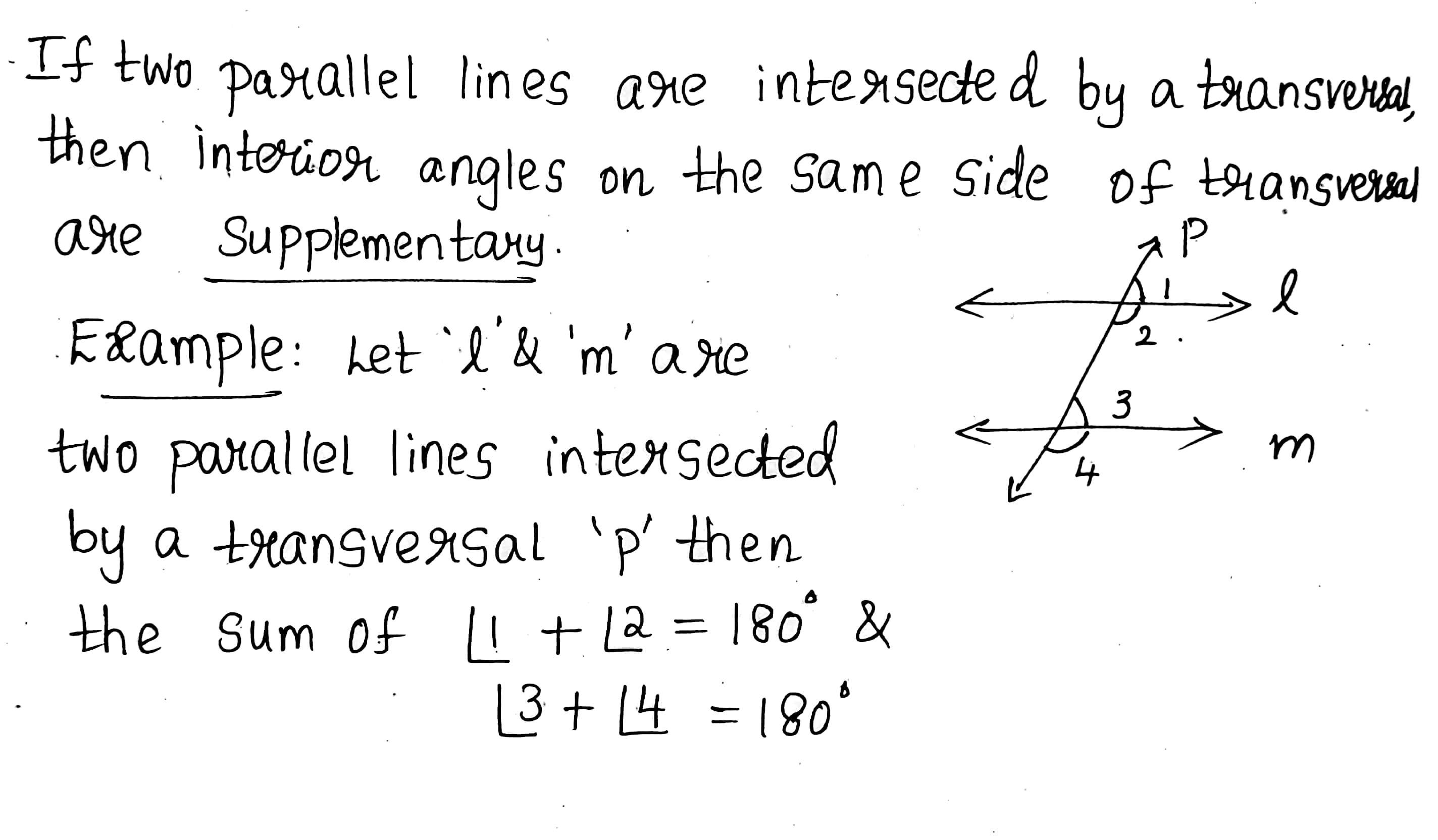
\angle 1 + \angle 8 = 180^o .......................

Identify the angles which are more than right angles and which are less than right angle?
In each of the following figure AB || CD. Find the value of x in each case.
Find the complementary angle of 36^{\circ}.
Can two adjacent angles be complementary ? Draw figure .
Can two adjacent angles be supplementary ? Draw figure .
Write the measure of supplementary angle of the given angles:
47^{\circ}.
Which of the following pairs of angles are supplementary ?
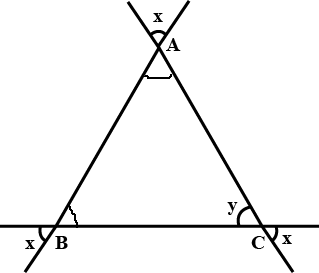
Find the value of unknown 'x' in the following triangle:
Fill up the table on finding complementary angle of given angle:
| Angle - 1 | Angle - 2 | |
| (1) | 50^{\circ} | _________ |
| (2) | ____________ | 63^{\circ} |
| (3) | 47^{\circ} | _________ |
| (4) | 56^{\circ} | _________ |
| (5) | __________ | 12^{\circ} |
| (6) | 67^{\circ} | _________ |
Write the measure of supplementary angle of the given angles:
75^{\circ}.
Measure of one angle of linear pair is given. Find the measure of another angle:
111^{\circ}.
Write the measure of the supplementary angle of the given angle:
112^{\circ}.
Write the measure of supplementary angle of the given angles:
100^{\circ}.
Write the measure of supplementary angle of the given angles:
81^{\circ}.
Write the measure of supplementary angle of the given angles:
109^{\circ}.
Write the measure of supplementary angle of the given angles:
90^{\circ}.
Write the measure of supplementary angle of the given angles:
132^{\circ}.
Write the measure of supplementary angle of the given angles:
60^{\circ}.
Measure of one angle of linear pair is given. Find the measure of another angle:
20^{\circ}.
Measure of one angle of linear pair is given. Find the measure of another angle:
130^{\circ}.
Make a pair of angles forming linear pair from the following angles:
27^{\circ}, 90^{\circ}, 130^{\circ}, 80^{\circ}, 35^{\circ}, 50^{\circ}, 145^{\circ}, 100^{\circ}, 90^{\circ}, 153^{\circ}.
m\angle XVW = 120^{\circ}, then
(i) m\angle WVY = ______ and
(ii) m\angle XVZ = ______.
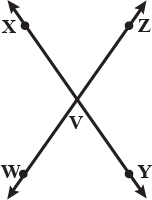
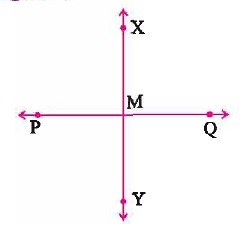
How may pairs are formed of angles of linear pair?
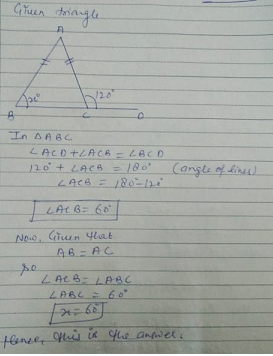
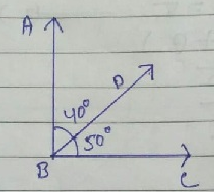
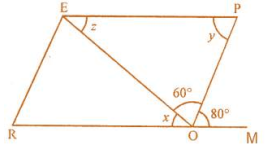
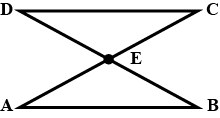
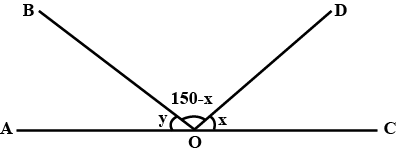
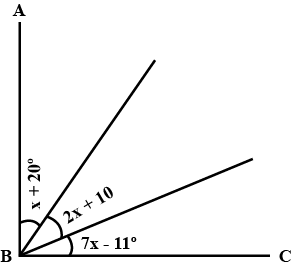
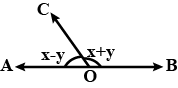
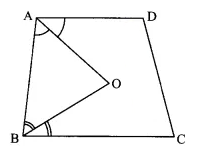
Find the angles x and y in the figure. AO=OB

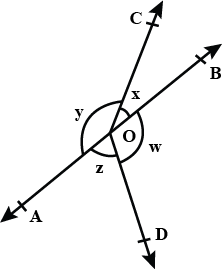
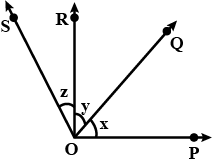
Find x, y, z.
In the figures given, state whether the lines l and m are parallel or not.

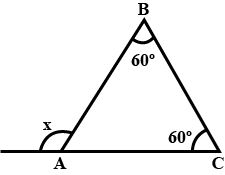
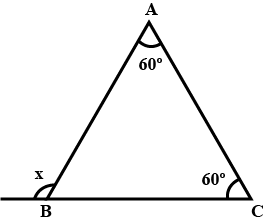
Show that the angles of an equilateral triangle are 60^o each.
Can two obtuse angles be supplementary?
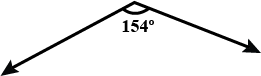
Find the supplement of 105.
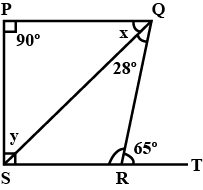
If PQ\bot PS,\ PQ\parallel SR,\ \angle SOR = 28^{o} and \angle QRT = 65^{o}, then find x & y.
Find the supplementary of 37^028' and 52^0 33'.
In Fig. if AB || DE, \angle BAC = 35^o and angle CDE = 53^o, find \angle DCE.
If the sum of the measures of two angles is {90^\circ}, then the angles are called _____ angles.
Find the angles, when:(i)The angles are complementary and the smaller is 40^ \circ less than the larger. (ii)The angles are complementary and the larger is four times the smaller.
In the figure \angle ABC={80}^{o}. Find \angle DGC and \angle DEF, given AB||DE and BC||EF.
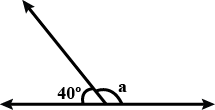
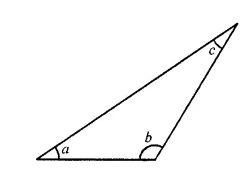
Find a.
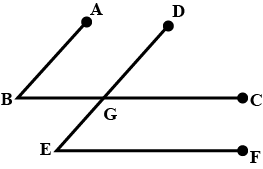
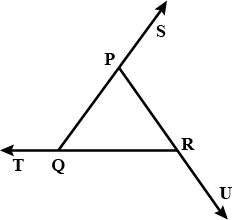
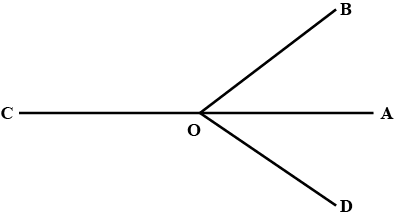
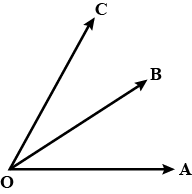
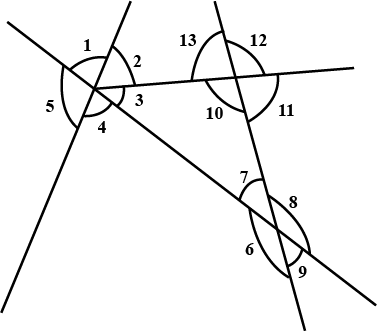
Name the pairs of adjacent angles in the figures.

Manasa says, "Each angle in any pair of complementary angles is always acute". Do you agree? Give reason.
AE||BD, AC||ED & AB=AC find a,b,c
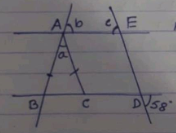
Find \angle APB, if AE || BR.

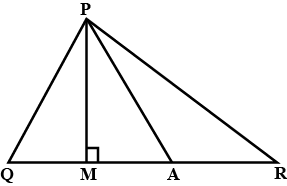
In the figure,\Delta PQR is a right angled at Q, \overline {ML} ||\overline {RQ} and \angle LMR = {130^ \circ }. Find \angle LPM and \angle PML

Find the measure of an angle which measures same as its complement.
AD is an altitude of an isosceles triangle ABC in which AB=AC show that, (i) AD bisects BC (ii) AD bisects \angle A.
If A,B,C are the angles of a triangle, show that
\sin{B}\cos{(C+A)}+\cos{B}\sin{(C+A)}=0
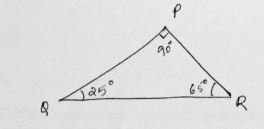
In \triangle\ PQR,\angle\ Q=40^{o},\angle\ R=75^{o}, then find the shortest and the longest side of the triangle
In the adjoining figure, name the following pairs of angles.
Equal supplementary angles

Explain:Can two acute angles be supplementary
Can two right angles be supplementary?
Two complementary angles are in the ratio of 2:1. Find the measure of the angles.
In the adjoining figure, name the following pairs of angles.
Unequal supplementary angles

In the following figure, is \angle 1 adjacent to \angle 2? Given reasons.
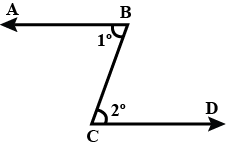
Find the angles of a triangle that are in ratio 2 : 2 : 5.
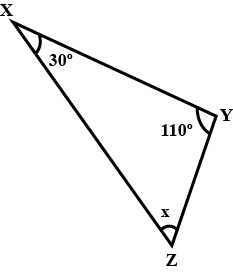
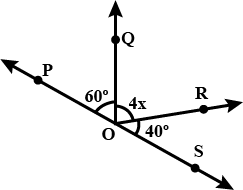
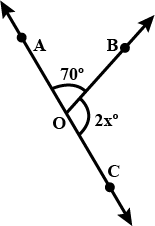
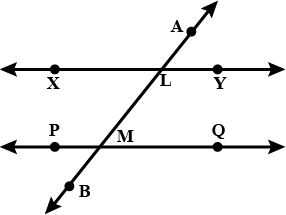
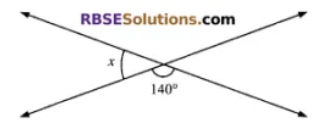
Find the value of x

Two adjacent angle of ||gm are 2x-15^o and 7x-75^o.Find all angles of ||gm ?
Find each one of lettered angles giving reason
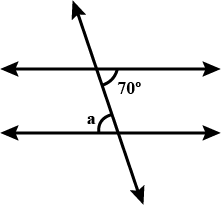
Find x in the following figure (AB || CD) :

Find the complements of the following angles:-
a) {45^ \circ }
b) {8^ \circ }
c) {20^ \circ }
d) {25^ \circ }
e) {78^ \circ }
Calculate the value of x :

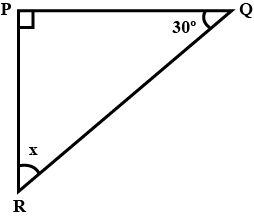
Find the value of x?
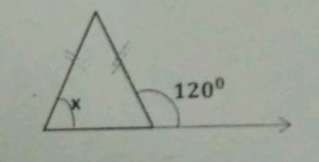
Find x in the following figures :
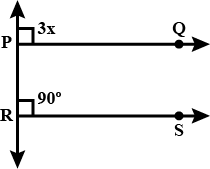
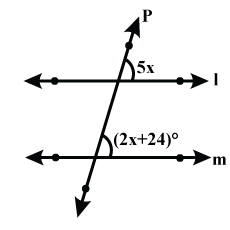
In the figure l\parallel m and p is a transversal, find the value of x, giving necessary reasons.
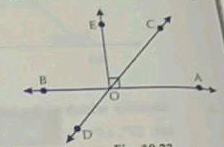
In figure given alongside AB and CD are intersecting lines. Name the following pair of angles.
Adjacent angles that do not form a linear pair and each less than 180^{o}.
From the adjoining figure find x, i AOB is a straight line. Hence complete the following
Which is an obtuse angle?
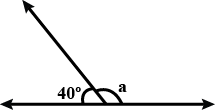
The supplementary angle of 40^{o} is
From the adjoining figure find x, i AOB is a straight line. Hence complete the following
Which is an acute angle?
Define adjacent angle.
Fill in the blank.
The sum of the measure of supplementary angle is ______
What do you mean by linear pair of angles ?
Find the supplement of the angle whose measure is {60}^{\circ}
In figure given alongside AB and CD are intersecting lines. Name the following pair of angles.
Equal supplementary angles

In Fig. If x+y=w+z then prove that A O B is a line.
If the ratio between two complementary angles 2:3, then find the angles.
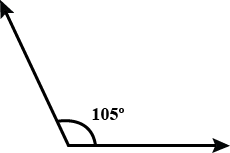
Find the supplementary angle of 105^{o}.
In the figure.

Find the supplementary angle of 115^{o}.
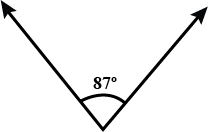
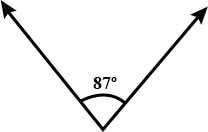
Find the supplementary angle of 87^{\circ}
Find the supplementary angle of 40^o.
For an angle { x }^{ 2 }, find: The complementary angle.
Find the measure of an angle which is 36^\circ more than its complement.
Classify the following angle as right, straight, acute, obtuse or reflex.

Classify the following angle as right, straight, acute, obtuse or reflex.

Draw a rough sketch of a quadrilateral KLMN. State two pairs of adjacent angles.
Write down the measures of some acute angles.(give at least two examples)
Find five situations where obtuse angles are made.
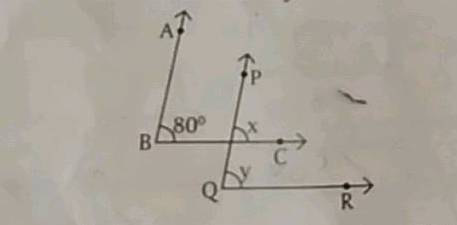
In the given figure, \angle ABC and \angle PQR are given such that AB\parallel PQ and BC\parallel QR. Find the value of \angle x and \angle y
Write down the measures of some obtuse angles.(give at least two examples)
List ten situations where the angles made are acute.
Identify whether the following pairs of angles are complementary or supplementary:
45^o, 45^o
Identify whether the following pairs of angles are complementary or supplementary:
112^o,68^o
Fill in the blanks:
If two adjacent angles are supplementary, they form a ______.
Identify whether the following pairs of angles are complementary or supplementary:
63^o, 27^o
Fill in the blanks:
If two angles are supplementary, then the sum of their measures is ______.
Identify whether the following pairs of angles are complementary or supplementary:
130^o, 50^o
Fill in the blanks:
If two lines intersect a point, then the vertically opposite angles are always ______.
Find the value of x.
Find the complement of the following angle: 35^o.
In Fig., write all pairs of adjacent angles and all the linear pairs.

Find the complementary angle of the following angle: 85^o.
Fill in the blanks in each of the following to make the statement true:
Two lines parallel to the same line are ______ to each other.
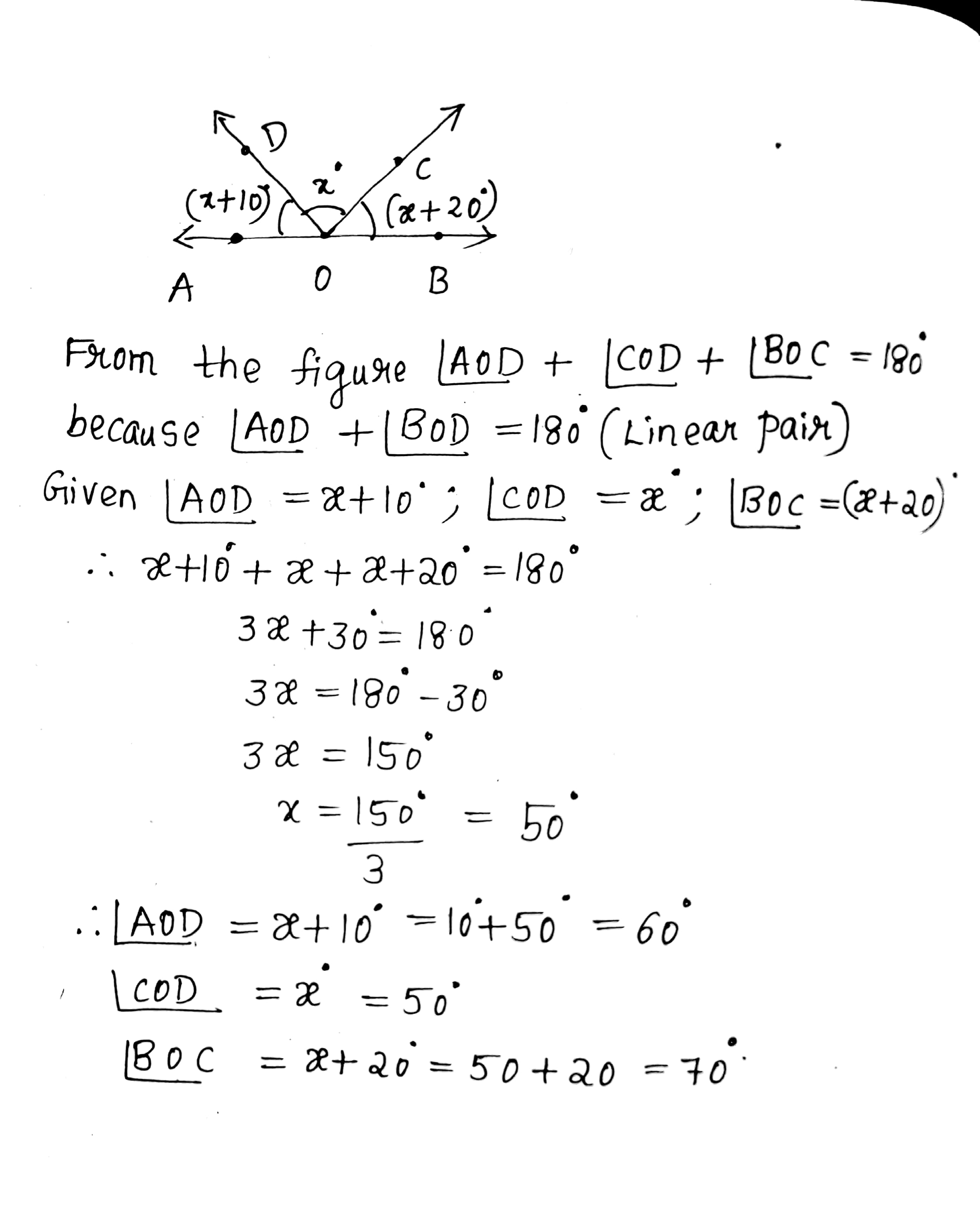
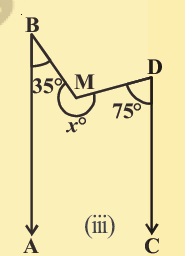
In Fig., find x. Further find \angle BOC, \angle COD and \angle AOD.

If two straight lines intersect each other, prove that the ray opposite to the bisector of one of the angles thus formed bisects the vertically opposite angle.
Find the supplement of the following angle.
70^o.
Identify the following pairs of angles are complementary or supplementary:
80^o,10^o
Find the supplement of the following angle:
90^o.
Can two angles be supplementary, if both of them be acute?
Identify the complementary and supplementary pairs of angles from the following pair.
100^o, 80^o.
Can two angles be supplementary, if both of them be obtuse?
Can two angles be supplementary, if both of them be right?
Identify the complementary pair of angles from the following pair:
63^o, 27^o.
Find the supplement of the following angle.
135^o.
Identify the complementary pair of angles from the following pair:
25^o, 65^o.
Find the supplement of the following angle.
120^o.
Classify the angle whose magnitude is given below:
215^{o}
Classify the angles whose magnitudes are given below:
89^{o}
Classify the angles whose magnitudes are given below:
180^{o}
Draw rough diagrams of two angles such that they have
One point in common.
Classify the angle whose magnitude is given below:
328^{o}
Classify the angles whose magnitudes are given below:
125^{o}
Fill in the blanks:
Two different lines in a plane either ...... at exactly one point or are parallel.
Classify the angles whose magnitudes are given below:
56^{o}
State which of the following angles with a small letter in the following diagrams are acute, which obtuse and which are reflex:
Fill in the blanks
An angle whose measure is less than that of a right angle is ......
Classify the angle whose magnitude is given below:
178^{\circ}
Classify the angle whose measure is given below:
125^{\circ}
Classify the angles whose measures are given below:
56^{o}
Name the following triangles with regards to angles:
Classify the angles whose measures are given below:
180^{o}
Write down each pair of adjacent angles shown in the above figures:
If sum of measures of two angles is 180^{o}, then the are _______.
The supplement of an acute is always ________ angle.
Two lines in a plane which do not meet at a point anywhere are called ________ lines.
Write down each pair of adjacent angles shown in the above figures:
Two angles forming a ________ pair are supplementary.
If two angles have a common vertex and their arms form opposite rays Fig., Then, write all the pairs of vertically opposite angles.

In each of the above figure write, if any, (i) each pair of vertically opposite angles, and (ii) each linear pair.

Write down each pair of adjacent angles shown in the above figures:

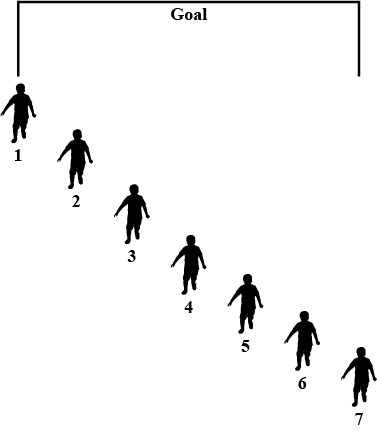
The drawing below Fig., show angles formed by the goalposts at different positions of a football player.The greater the angle, the better chance the player has of scoring a goal. For example, the player has a better chance of scoring a goal from Position A than from POsition B.
In Parts (a) and (b) given below it may help to trace the diagram and draw and measure angles.
Estimate atleast two situations such that the angles formed by different positions of two player are complement to each other.
Name the pairs of supplementary angles in the above figure:
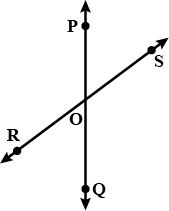
If two angles have a common vertex and their arms form opposite rays Fig., Then, how many angles are formed?

In Fig., OR \bot \ OP.
Name all the pairs of adjacent angles.
In Fig. P,Q and R are collinear points and TQ \bot PR,
Name:
Four pair of adjacent angles.
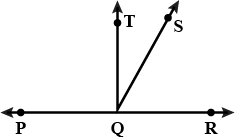
In Fig., are the above pair of angle adjacent? Justify your answer.

In the figure, OR \bot \ OP.
Name all the pairs of complementary angles.

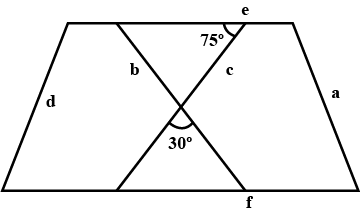
Iron roads a,\ b,\ c,\ d,\ e and f are making a design in a bridge as shown in Fig., in which a \parallel b,\ c \parallel d,\ e \parallel \ f. Find the marked angles between b and c
In Fig., are the above pair of angle adjacent? Justify your answer.
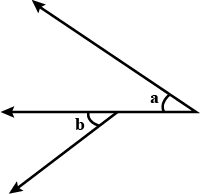
What is the type of other angle of a linear pair if one of its angle is right?
Write the complement angle of: x^{o}.
In Fig., are the above pair of angle adjacent? Justify your answer.
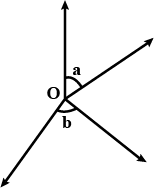
In Fig. write all the pairs of supplementary angles.

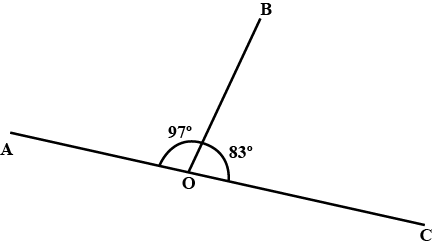
In Fig., OB is perpendicular to OA and \angle BOC=49^o. Find \angle AOD.

Write the supplement angle of:
49^{o}
Write the complement angle of: 45^{o}.
In Fig., are the above pair of angle adjacent? Justify your answer.

Write the supplement angle of:
111^{o}
Prove that the bisectors of two adjacent supplementary angles include a right angle.
The larger of two supplementary angles exceeds thrice the smaller by 20 degrees.
Find them.
Write the supplement angle of:
20^{o}+y^{o}
For an angle x^{o}, find:
the supplementary angle
Write the supplement angle of:
(x-30)^{o}
Figure given below shows a pair of adjacent angles AOB and BOC. Find whether or not the exterior arms OA and OC are in the same straight line.
If true then enter 1 and if false then enter 0
Can two obtuse angles be complement to each other?
If true then enter 1 and if false then enter 0
Can an acute angle be adjacent to an obtuse angle?
If true then enter 1 and if false then enter 0
Can two obtuse angles be supplementary?
If true then enter 1 and if false then enter 0
Can two right angles be complement to each other?
If true then enter 1 and if false then enter 0
Can two acute angles be supplementary?
If true then enter 1 and if false then enter 0
Can two obtuse angles form a linear pair?
The adjacent angles on a straight line are (5x-6)^o and 7(x+6)^o. Find the value of x.
The measures of two adjacent angles on a straight line are x^o and (2x-27)^o. Find the smallest angle.
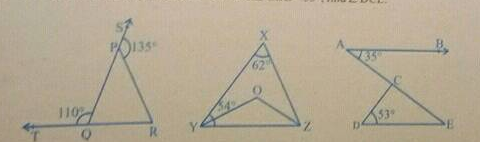
The side EF, FD and DE of a triangle DEF are produced in order forming three exterior angles DFP, EDQ and FER respectively. Prove that \angle DFP+\angle EDQ+\angle FER=360^o.
Prove that if the sum of two adjacent angles is 180^o, then the non-common arms are two opposite rays.
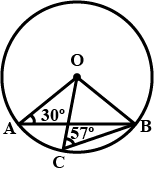
In Fig. 10.16 \displaystyle \angle OAB=30^{\circ}\,and\,\angle OCB=57^{\circ} Find \displaystyle \angle BOC\,and\,\angle AOC
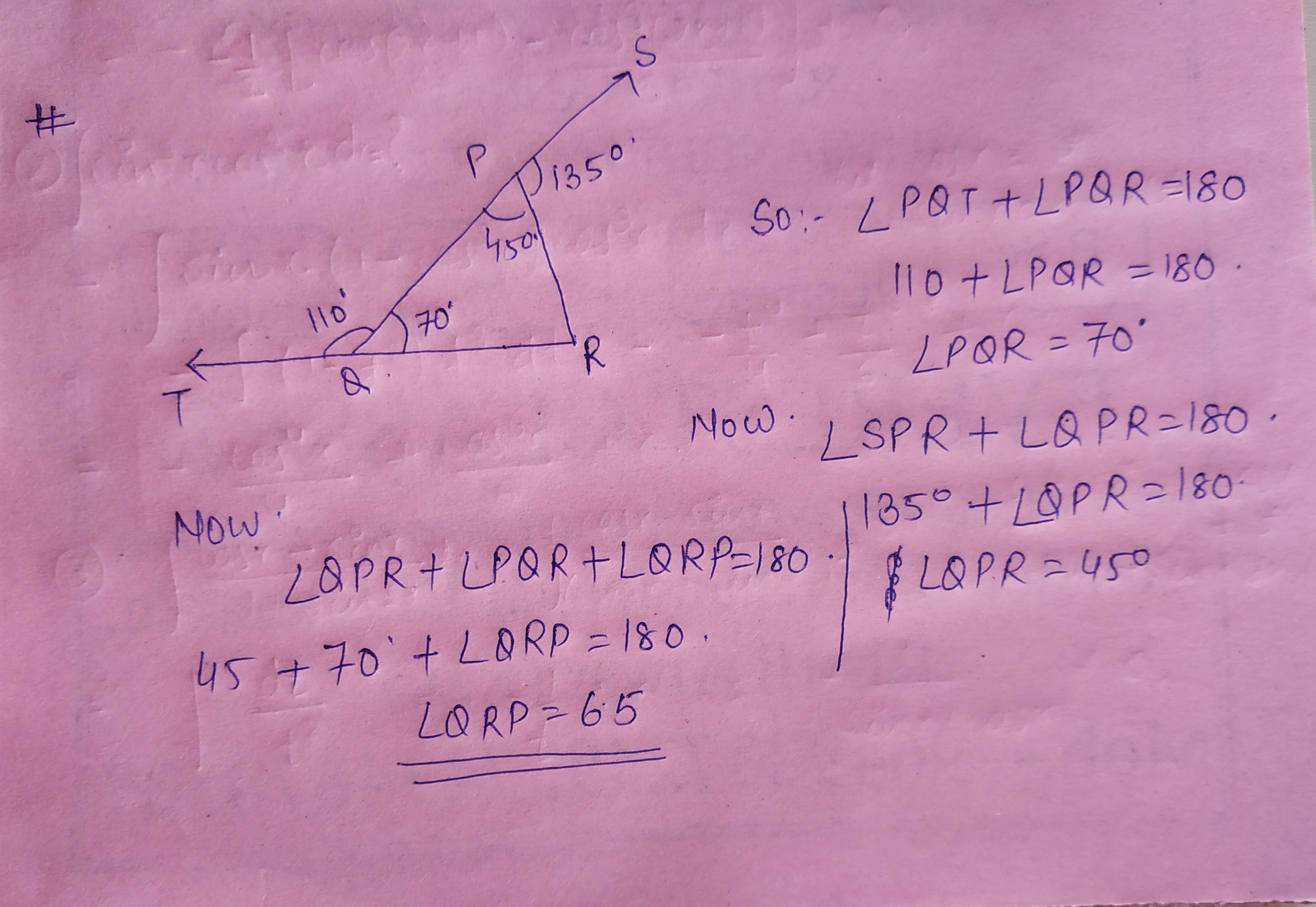
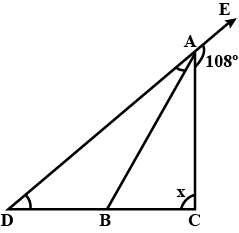
In the figure, sides QP and RQ of \Delta PQR are produced to points S and T respectively. If \angle SPR={ 135 }^{o } and \angle PQT={ 110 }^{ o}, find \angle PRQ.
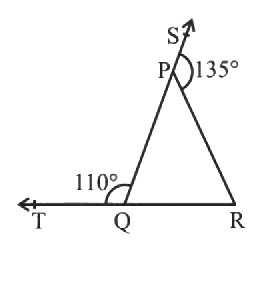
It is given that \angle XYZ={ 64 }^{ } and XY is produced to point P. Draw a figure from the given information. If ray YQ bisects \angle ZYP, find \angle XYQ and reflex \angle QYP.
In the figure, lines XY and MN intersect at O. If \angle POY={ 90 }^{o } and a : b = 2 : 3, find c.
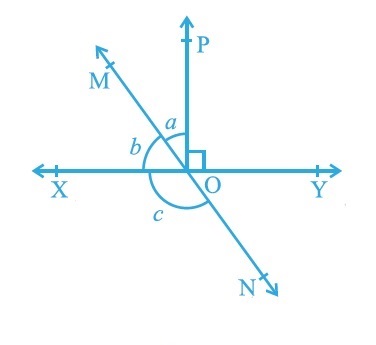
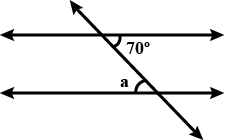
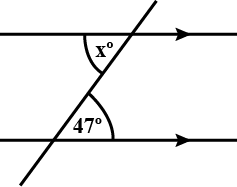
The line d is parallel to e, as shown in the figure above. Find the value of y .
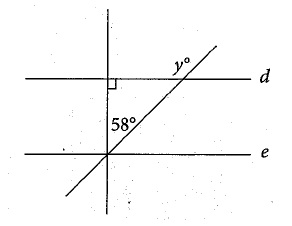
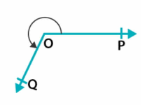
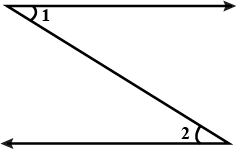
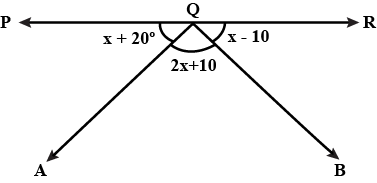
Which pair of angles are supplementary in the following diagram? Are there supplementary rays?
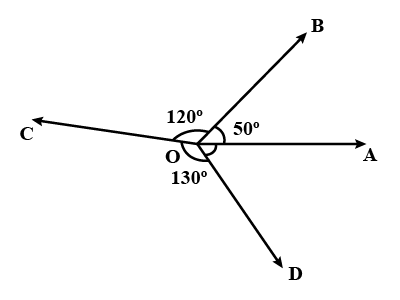
Two complementary angles differ by {12}^{o}, find the angles?
Draw diagrams illustrating each of the following situation:
(a) Three straight lines which do not pass through a fixed point.
(b) A point and rays emanating from that point such that the angle between any two adjacent rays is an acute angle.
(c) Two angles which are not adjacent angles, but still supplementary.
(d) Three points in the plane which are equidistant from each other.
Find the value of x in the following diagram:
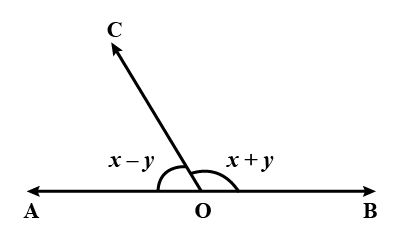
Statement
\angle 1 is supplement to \angle 8 .
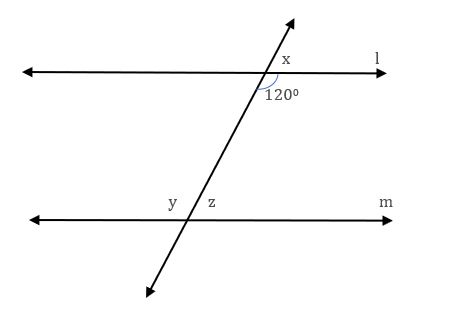
Provide appropriate reason for the same if l is parallel to m and a transversal intersects these parallel lines.

In the figure given above the sides, AB and AC of \triangle ABC are produced to points E and D respectively. If bisectors BO and CO of \angle CBE and \angle BCD respectively meet at point O, then prove that \angle BOC = 90^o - \dfrac{1}{2} \angle BAC.
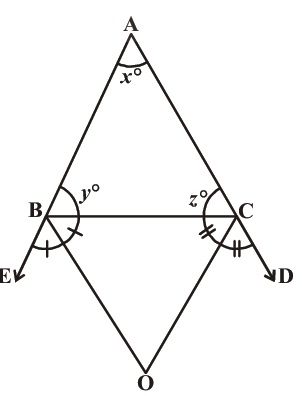
\triangle{PQR} is an isosceles triangle with PQ=PR, QP is produced to S and PT bisects the extension angle 2{ x }^{ o }. Prove that \angle Q={ x }^{ o } and hence prove that PT\parallel QR.
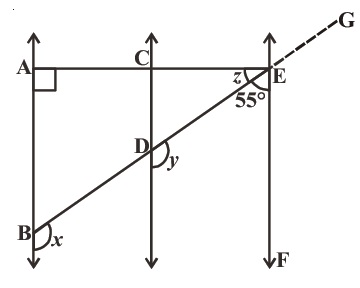
In the given figure AB || CD and CD || EF. Also EA \perp AB. If \angle BEF = 55^\circ find the values of x, y and z.
Using information given in the adjacent figure, find the values of x and y.
In the adjacent figure PQ and RS are two mirrors placed parallel to each other. An incident ray \overrightarrow{AB} strikes the mirror PQ at B, the reflected ray moves along the path \overrightarrow{BC} and strikes the mirror RS at C and again reflected back along \overrightarrow{CD}. Prove that AB || CD.

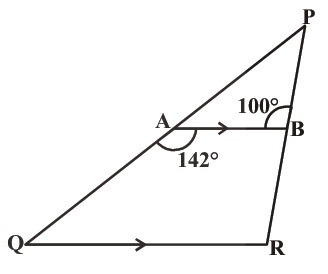
In the given above figure, AB || QR, \angle BAQ = 142^o and \angle ABP = 100^o. Find \angle APB.
Find the supplementary angle of the given angle(in degrees).
105^0
Look at the pictures. Mark the angles and colour them as indicated below.(a) If the angle is right angle then mark it in blue.
(b) If an angle is less than a right angle then mark it in red.
(c) If an angle is more than a right angle then mark it in black.
(b) If an angle is less than a right angle then mark it in red.
(c) If an angle is more than a right angle then mark it in black.
Find the number of pairs of adjacent angles in the figure .

How many of the following are adjacent angles ?
What is the measure of complementary angle of 20^{\circ}?
(1) 60^{\circ}
(2) 70^{\circ}
(3) 80^{\circ}.
What is the measure of complementary angle of 55^{\circ}?
(1) 25^{\circ}
(2) 15^{\circ}
(3) 35^{\circ}
What is the measure of complementary angle of 83^{\circ}?
(1) 7^{\circ}
(2) 17^{\circ}
(3) 27^{\circ}.
Write the measure of supplementary angle of the given angles:
145^{\circ}.
Measure of one angle of linear pair is given as 50^{\circ}. Find the measure of another angle.
Two angles are supplementary angles. If measure of one angle is 66^{\circ}, then find the measure of another angle.
Find complementary angle of 23^{\circ}.
One angle of linear pair is a right angle, then find the measure of another angle.
Measure of one angle of linear pair is given. Find the measure of another angle:
85^{\circ}.
From the given figure write two pairs of supplementary angles:
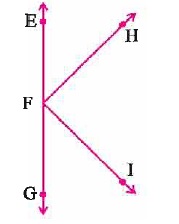
Measure of one angle of linear pair is given. Find the measure of another angle:
107^{\circ}.
Measure of one angle of linear pair is 82^{\circ}, find the measure of another angle.
Measure of one angle of linear pair is given. Find the measure of another angle:
155^{\circ}.
Measure of one angle of linear pair is 108^{\circ}, then find the measure of another angle.
Write all pairs of angles of linear pair.

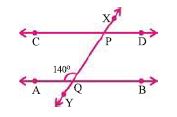
\overleftrightarrow{AB} || \overleftrightarrow{CD} in the following figure. \overleftrightarrow{XY} is the transversal. If m \angle PQA = 140^o, find the measure of remaining angles:
In the following figure, ABCD and PORS are two parallelograms such that \angle D=120^{o} and \angle Q=70^{o}. Find the value of x
Find \angle a,\angle b,\angle c, \angle d and \angle e in the figure? Give reasons.

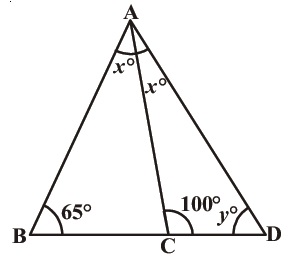
In \Delta PQR,\angle P = 2\angle Q and \angle R = 3\angle Q, calculate the angles of \Delta PQR
Observe the figure and find x.
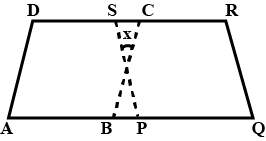
In fig. if AB || CD then find the value of x.
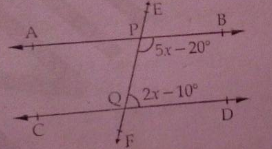
In parallelogram ABCD two points P and Q are taken on diagonal BD such that DP=BQ (see fig. 8.20). Show that: AP = CQ
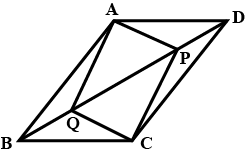
From the figure given above, find the values of angles x, y and z.

In figure, m||n and p||q. If \angle 1=75^{o}, prove that \angle 2=\angle 1+\dfrac {1}{3} of a right angle.

If two lines intersect, prove that the vertically opposite angles are equal.
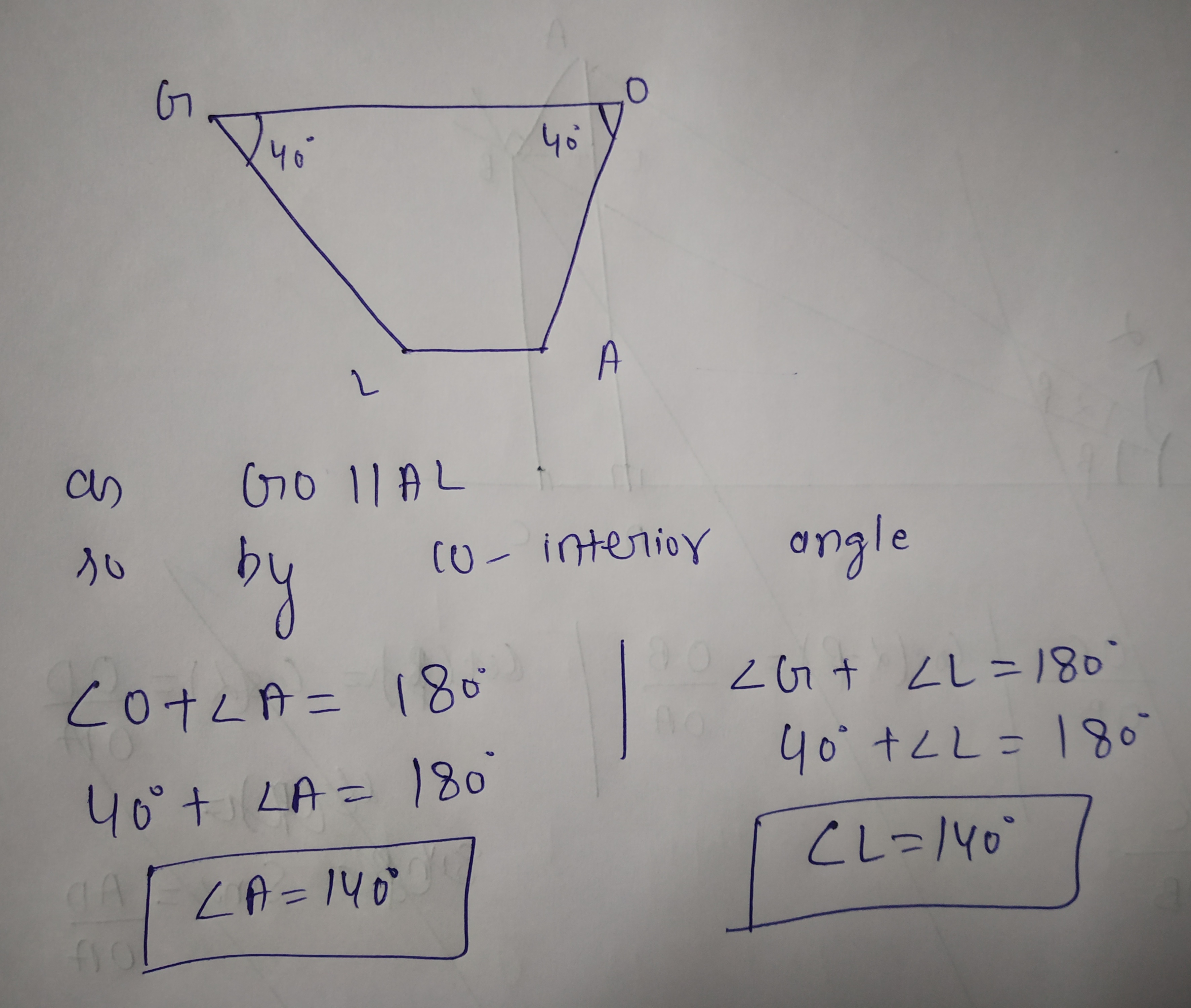
GOAL is a quadrilateral in which GO||AL. If \angleG=\angleO=40^o. What are the measure of \angleA and \angleL.
An angle is equal to four times its complement. Determine its measure.
Ray PQ and ray PR are perpendicular to each other. Points B and A are in the interior and exterior of \angle QPR respectively. Ray PB and ray PA are perpendicular to each other. Draw a figure all this rays and write-A pair of complementary angles A pair of supplementary anglesA pair of congruent angles.
In Figure, AB||CD and EF is a transversal. Find the measure of all the other angles that are formed.
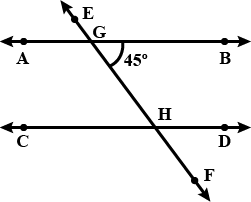
Find the value of x in the above figure.
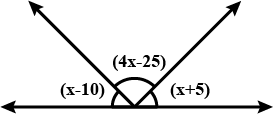
An angle is \dfrac {2}{3} of its supplementary. What is its magnitude?
In the figure, find x.

Find the angles in a straight line
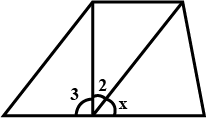
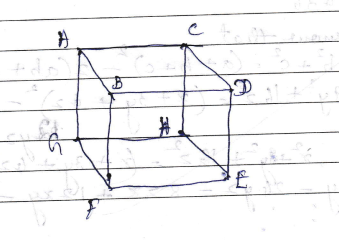
How many sets of parallel line segments can you find in a cube?
Find the value of x:
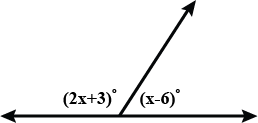
Two complementary angles differ by 10^{o}. Find the angles.
Two complementary angles differ by {6^ \circ }. Find the measure of the two angles.
On the sides, AB and AC of triangle ABC, equilateral triangles ABD and ACE are drawn. Prove that: \angle {\text{CAD}} = \angle {\text{BAE}}
In figure, l || m and p || q. Find the values of x, y, z and t

One of the acute angles of a right triangle is 40°. Find the other acute angle.
Two parallel lines l and m are cut by a transversal t. If the interior angles of the same side of t are (2x-8)^\circ and (3x-7)^\circ, find the measure of each of these angles.
Find the complement of: \dfrac{1}{2} of 40^0.
An angle 10^{0} more than three times its complementary angle. Then find the angles.
In \Delta PQR S is a point on PQ such that SR = QR = SP. and \angle PSR=120^{\circ} .Find \angle P .

Prove that the line segments joining the points of contact of two parallel tangents of a circle,passes through its centre ?
If
A,B,C are the interior angles of triangle ABC, then prove that \text{cosec}\left( {\dfrac{{C + A}}{2}} \right) = \sec \left( {\dfrac{B}{2}} \right)
An angle is 14^{o} more than its complementary angle. What is the measure?
In the given figure line AB and CD intersect at O . \angle BOC = {36^ \circ }. Find \angle x , \angle y and \angle z

In the above sided figure,if QT \bot PR.,\angle TQR=40^o and \angle SPR=30^o,find x and y

In the joining figure, name the following pairs of angles.
Adjacent angles that do not form a linear pair.

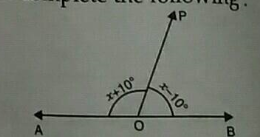
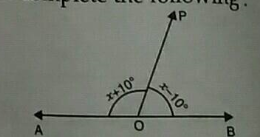
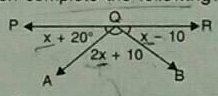
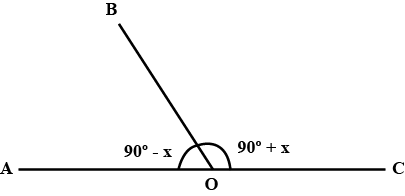
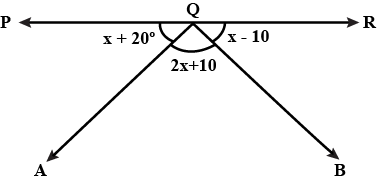
\angle{A} and \angle{B} are supplementary angles. \angle{A}=x+{10}^{o} and \angle{B}=x-{10}^{o}, find \angle{A} and \angle{B}
The measure of an angle is twice the measure of its supplementary angle. Find its measure.
Find the value of x in each of the following figures ?

Write the complement of { 51 }^{ \circ } ,28^{ \circ },13^{ \circ } ?
The two angles \left(3x+10\right)^{o} and \left(2x+30\right)^{o} will make a straight line then find x?
In the figure.A B \parallel C D , \angle P T B = 55 ^ { \circ } and \angle D V S = 45 ^ { \circ }. Find sum of \angle \mathrm { CUQ } and \angle R T P is :

Fill in the blanks
An angle which measure is 180^{o} is called ..
In the given figure \Box PTQR and PS is the bisector of \angle P. If \angle Q = 60^o and \angle R = 30^o, find \angle TPS.
Three angles which add upto 180^o are in the ratio 2:3:7. Find them.
Prove that the sum of angles of a triangle is 180.
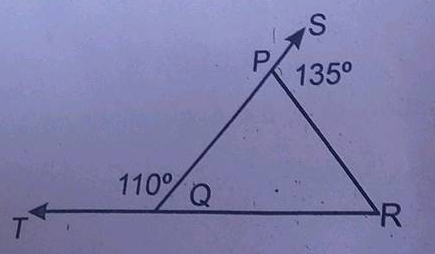
In fig, sides QP and RQ of triangle PQR are produced to point S and T respectively. If angle S P R = 135 ^ { \circ } and angle P Q T = 110 ^ { \circ } \text { ,find } \angle P R Q.
Two supplementary angles differ by 22 ^ { 0 }. Find the angles.
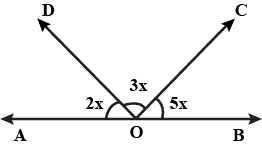
OC and OD are two rays from point O on straight line AOB. \angle AOC =2x, \angle COD = 3x, \angle BOC=5x.
Find each angle : \angle AOC , \angle COD, \angle DOB
Find each angle : \angle AOC , \angle COD, \angle DOB
Find the measure of the angles of a triangle in each of the following cases:
One of the acute angles of a right triangle is 63^{\circ}. Find the other angle.
Find all the angle of triangle ABC

Find \angle x = ?
Find the angles in each of the following:
The angles are complementary and the smaller is 40 ^ { \circ } less than the larger.
In the given figure , AOC is the diameter of the circle , with centre O.
If arc AXB is half of arc BYC , find \angle BOC.

Observe the figure and find the value of x.
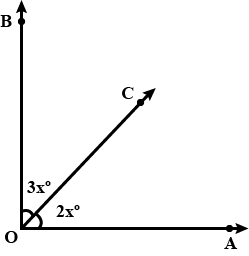
Find the angle which is supplementary of itself.
The measure of an angle which is 9 times its supplement is?
Find the value of x
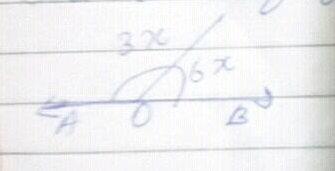
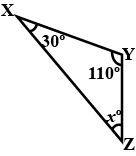
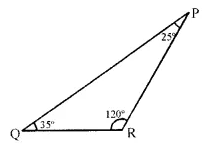
Angles Q and R of a \Delta PQR are 25^{\circ} and 65^{\circ}. Write which of the following is true:
1. PQ^2 +QR^2 = RP^22. PQ^2 +RP^2 = QR^23. RP^2 +QR^2 = PQ^2
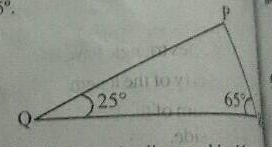
The measures of two complementary angles are x and 4x. Find the measure of each of these angles.
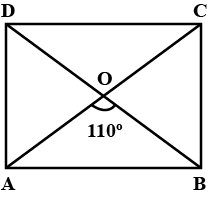
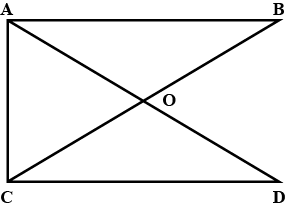
In the adjoining figure,ABCD is a rectangle whose diagonals AC and BD intersect at O. If \angle AOB=110^{o}, find the measure of:\angle AOD and \angle BOC.
An angle is \dfrac{2}{3} of its complement. What is its magnitude?
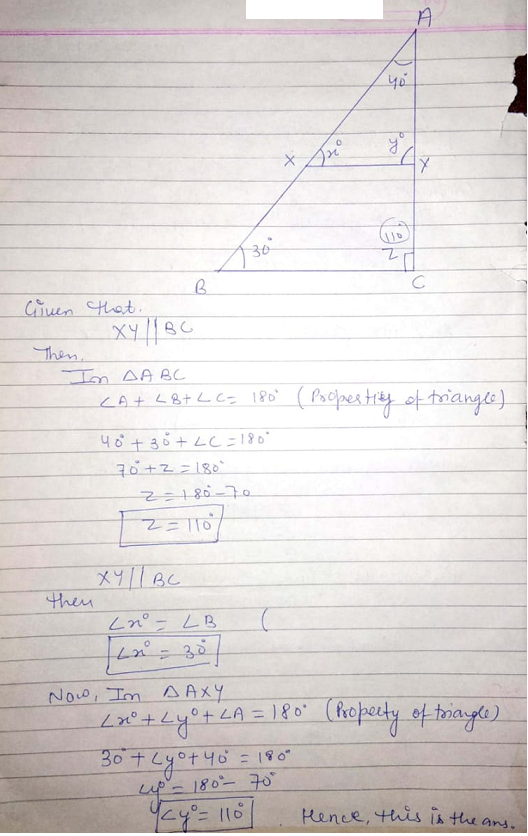
In the adjoining Figure, \overline{XY}\parallel \overline {BC}. Find the values of x, y, z.
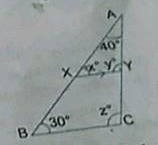
Two angles are equal and they form a pair of supplementary angles. Find their magnitude.
Find the value of x in each of the following figure :
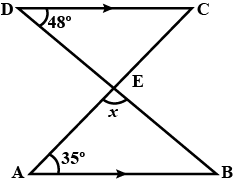
In fig name all the pairs of adjacent angles.

Find the values of x and y in the given figure
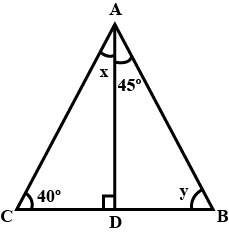
Find the value of x

Find the angle which is \dfrac{1}{5} of its complement.
If the complement of an angle is 27^{\circ}, then find its supplement.
Two complementary angles are in the ratio 2:3.Find the supplement of a larger angle.
In Fig.,\Delta ODC \sim \Delta OBA,\angle BOC = {125^ \circ } and \angle CDO = {70^ \circ }. Find \angle DOC,\angle DCO and \angle OAB

Write down each pair of adjacent angles shown in fig.

Find the complementary angle of 45\dfrac{1}{2}^o.
In the adjoining figure, identify the pair of corresponding angles.

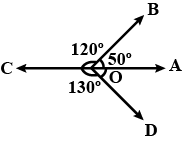
In the figure, if a-b=80^{o}, find a and b.
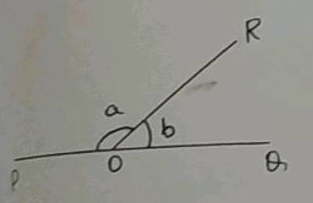
In the adjoining figure, identify the pair of alternate interior angles.

An angle is 28^{o} less than its complement, find its measure.
Find the complementary angle of 37\dfrac{1}{2}^o.
An angle is equal to 5 times its complement. Find the measure of each angle.
What is an Obtuse Angle ?
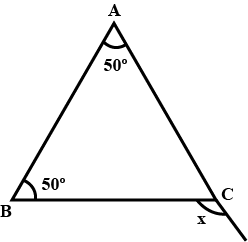
One of the angle of a triangle is { 50 }^{ \circ } and the other two angles are equal. Find the measure of each of the equal angles.
If the angle ( 2 x - 10 ) ^ { \circ } and ( x - 5 ) ^ { \circ } are complementary angles. Find the angles.
Find the complementary angle of {28}^{\circ}.
Show that the lines 2x+y+1=0,\,\,x-2y+1=0 are perpendicular.
Find the complement of: {23}^{\circ}{19}^{\prime}{32}^{\prime\prime}.
Find the complement of: {58}^{\circ}{20}^{\prime}{25}^{\prime\prime}.
In Fig. 7.12, if AB ||DC and E is the mid - point of side AC, then prove that E is the mid-point of side BD.
The difference between the angle and its complement is {36}^{\circ}. Find the angles.
The difference in the measures of the two complementary angles is 12^o. Find the measure of the angles.
Find the measure of an angle which is {18}^{\circ}{2}^{\prime}{10}^{\prime\prime} less than its complement.
What are complementary and supplementary angles ?
In the figure if Line l || m and the line n is the transversal then find the value of a and b

Find the complementary angle of 22\dfrac {1}{2}^{o}.
In the given figure, line l || line m and line n || p . Find \angle a,\angle b,\angle c. from the given measure of an angle .

Which of the pair given is complementary and which are not:
a) { 15 }^{ \circ },{ 75 }^{ \circ }
b) 76^{ \circ },{ 47 }^{ \circ }
c) 64^{ \circ },{ 26 }^{ \circ }
In fiq. AB divides \angle DAC in the ratio 1:3 and AB=DB. Determine the value of x.
Find the complementary of the angle 30^o.
The difference between the measures of the two angles of a complementary pair is { 40 }^{ \circ }. Find the measures of the two angles.
In the figure, AB = AC and OB and OC are angle bisectors of \angle B and \angle C. Prove that \angle BOC = \angle ACD.

For what value of x+y in the given figure will ABC be a line? Justify you answer.
Two adjacent angles are equal. Is it necessary that each of these angles will be a right angle. Justify your answer.
Find the complementary of 75^{\circ}.
One angle of a linear pair is twice the other. How much is each?
Two angles measure \left( 55 ^ { \circ } + 3 a \right) and \left( 115 ^ { \circ } - 2 a \right) \cdot If each is supplement of the other, then calculate the value of a .
Define Adjacent angles, Linear pair of angles. What is the sum of a linear pair of angles
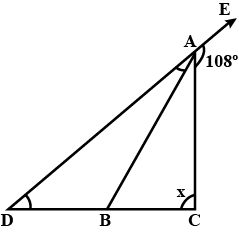
In the figure, PQR is a straight line. Find x. Then complete the following :
(i) \angle AQB=
(ii) \angle BQP=
(iii) \angle AQR=
Write the complementary, supplementary angles of 75^\circ .
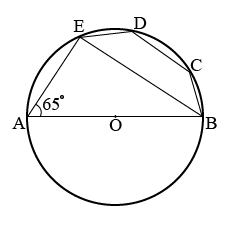
In the given figure, AB is diameter of a circle with centre O and chord ED is parallel to AB and \angle EAB={ 65 }^{ o }. Calculate (i)\angle EAB (ii) \angle BED (iii) \angle BCD
Write down the measures of
(a) some acute angles
(b) some obtuse angles
(give at least two examples of each).
Sushma says, "Complementary angle of { 48 }^{ 0 } is { 42 }^{ 0 }". Do you agree with her? Give reasons.
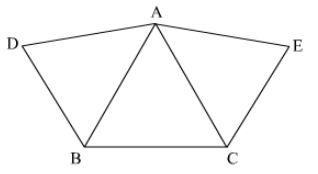
In the figure, show that \angle A + \angle B + \angle C + \angle D + \angle E + \angle F = 360^{\circ}.
In a right-angled triangle, one of the acute angles measures 53^{\circ}. Find the measure of each angle of the triangle.
If \left(2x-14\right) and 11\left( x+7 \right) are supplementary angles then find the angles.
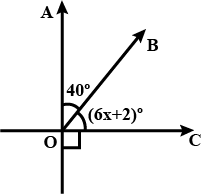
If a=3b, find a and b. (in degree)

Find the value of x from the adjacent figure
Find the complement of each of the following angle: { 55 }^{ \circ }.
Write the supplement of each of the following angles :
{ 54 }^{ \circ }
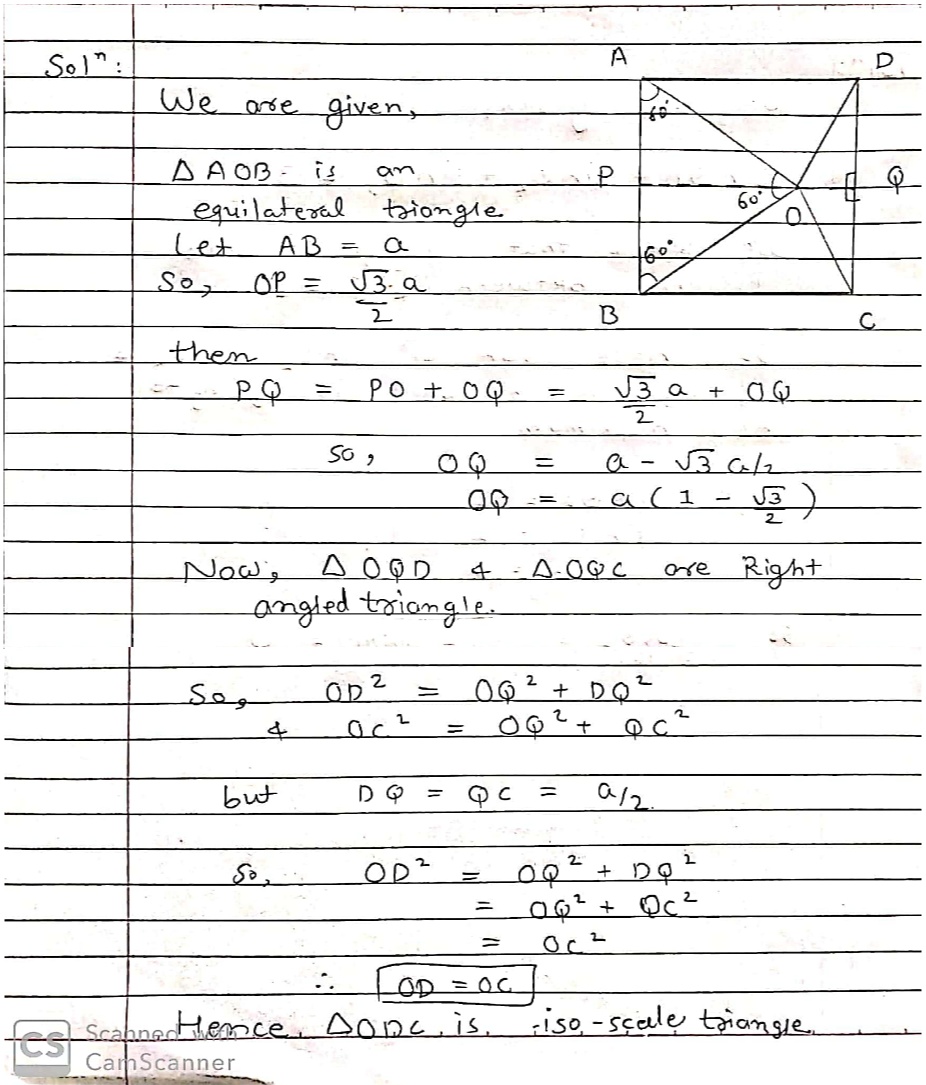
O is a point in the interior of a square ABCD such that OAB is an equilateral triangle. Show that \Delta OCD is an isosecleles triangle.
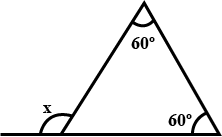
Find the value of the unknown exterior angle x in the following diagram.
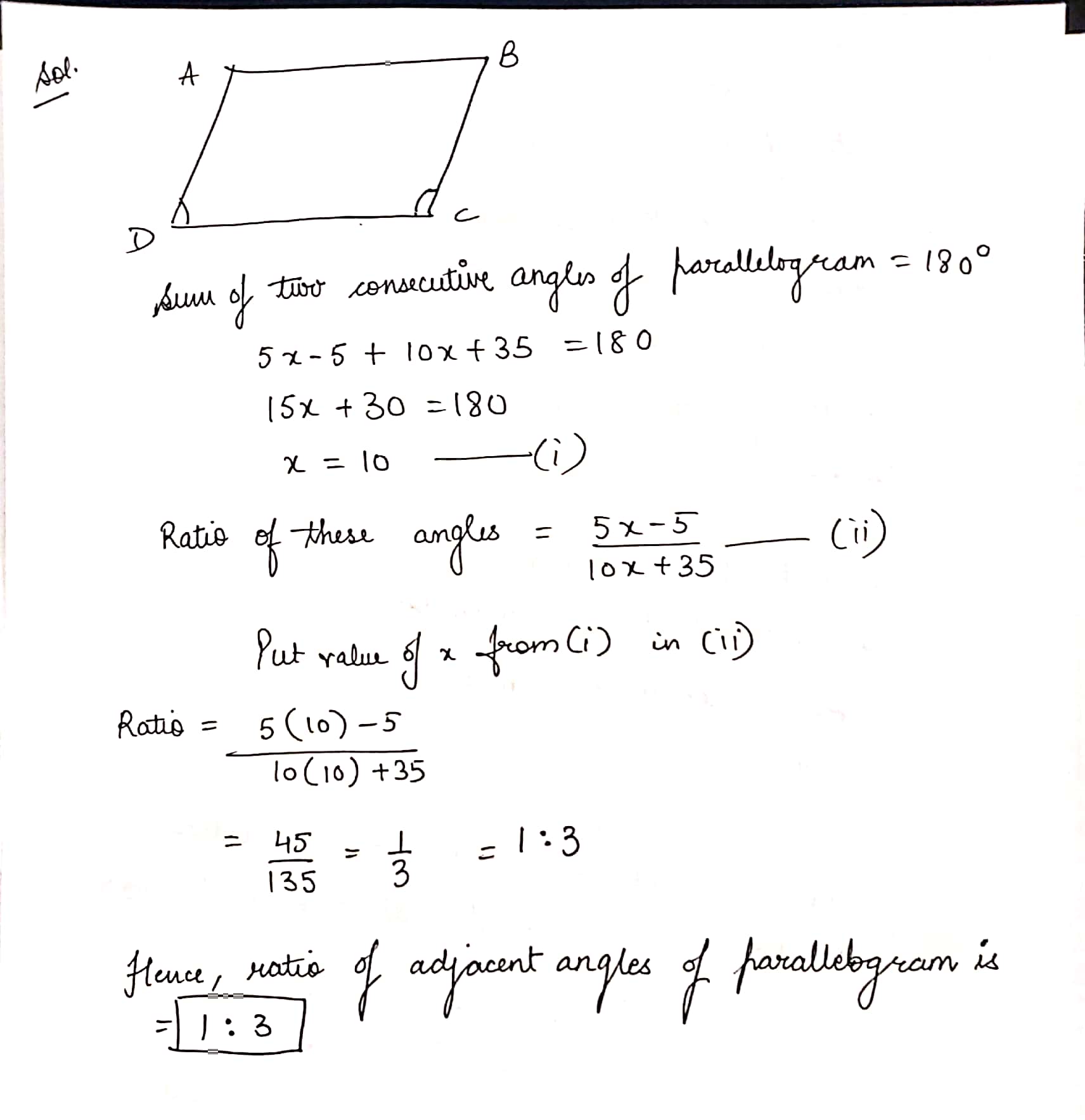
If two adjacent angles of a parallelogram are (5x-5)^{\circ} and (10x+35)^{\circ}, then find the ratio of these angles.
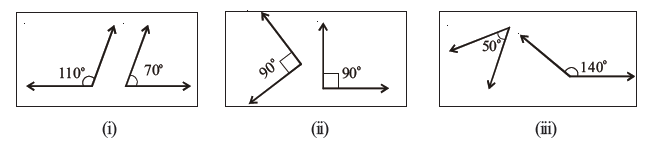
Find the value of the unknown exterior angle x in the following diagrams.

List three situations where reflex angles may be seen.
What value of x makes the lines \bar{AB} and \bar{CD} parallel to each other?

What will be the complement of 59^o?
Find the value of the unknown exterior angle x in the following diagram.
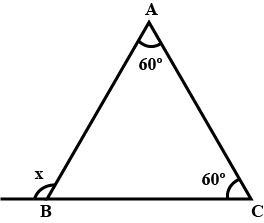
Find the value of the unknown interior angle x in the following figure.
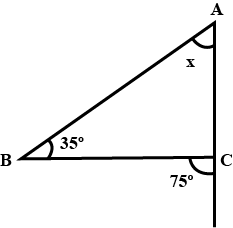
Find the value of unknown x in the following diagram.

Find the value of unknown x in the following diagram.

Find the value of the unknown interior angle x in the following figure.
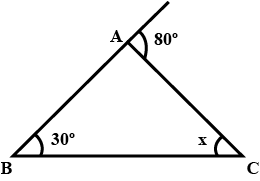
Find the values of the unknown x and y in the following diagram.

Find the value of unknown x in the following diagram.
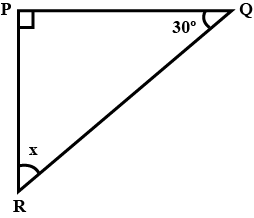
Find the value of the unknown interior angle x in the following figure.
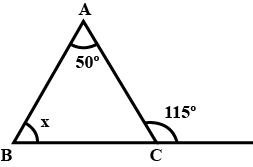
Find the value of unknown x in the following diagram.
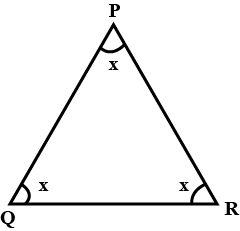
Find the value of unknown x in the following diagram.

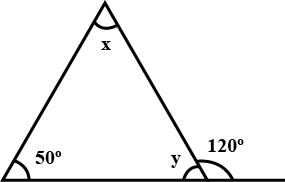
Find the values of the unknown x and y in the following diagram.
Find the angle which is equal to its supplement.
Find the values of the unknown x and y in the following diagram.

In the given figure, \angle 1 and \angle 2 are supplementary angles. If \angle 1 is decreased, what changes should take place in \angle 2 so that both the angles still remain supplementary?
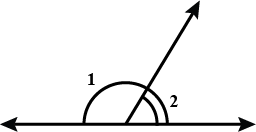
Find the supplement of each of the following angles:
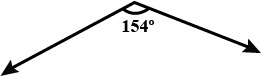
Find the values of the unknown x and y in the following diagram.

Find the supplement of the angle shown in the above figure.
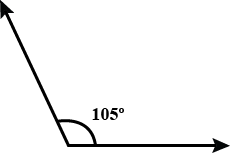
Find the complement of the angle represented in given figure.
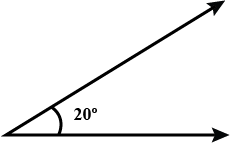
Find the values of the unknown x and y in the following diagram.
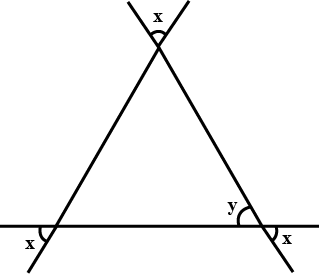
Find the supplement of each of the following angles:
Find the values of the unknown x and y in the following diagram.

Two supplementary angles are in the ratio 4:Find the angles.
Write the supplement of the following angle: 54^{\circ}
Write the complement (in degree) of the following angles: 77^{\circ}
Write the complement (in degree) of the following angles: 20^{\circ}.
Write the supplement of the angle 132^{\circ}
An angle is equal to 8 times its complement. Determine its measure.
Write the complement (in degree) of the following angles: 30^{\circ}.
Can two angles be supplementary if both of them are: (i) acute (ii) obtuse (iii) right?
Write the complement (in degree) of the following angles: 35^{\circ}.
Write the complement (in degrees) of the following angles: 90^{\circ}.
In Fig., l,m and n are parallel lines intersected by traversal p at X,Y and Z respectively. Find \angle 1,\angle 2 and \angle 3.

In FIG., show that AB \parallel EF.
In a \Delta ABC, if \angle A = 55^{\circ}, \angle B = 40^{\circ}, find \angle C. (value only)
How many pairs of adjacent angles are formed when two lines intersect in a point?
In Fig., AOC is a line, find x.

Fill in the blanks in each of the following to make the statement true:
If two parallel lines are intersected by a transversal, then interior angles on the same side of the transversal are _______
How many pairs of adjacent angles, in all, can you name in Fig.
Prove that if the two arms of an angle are perpendicular to the two arms of another angle, then the angles are either equal or supplementary.
In Fig., ACB is a line such that \angle DCA = 5x and \angle DCB = 4x. Find the values of \angle DCA and \angle DCB.

Classify the following angle as acute, obtuse, straight, right, zero and complete angle.
118^o.
Classify the following angle as acute, obtuse, straight, right, zero and complete angle.
145^o.
Fill in the blanks to make the following statements true.
Sum of the angles of a triangle is ______.
Compute the value of x in the following figure:

In a \Delta PQR, If PQ = QR and L, M and N are the mid-points of the sides PQ, QR and RP respectively. Prove that LN = MN.
From figure, write all pairs of parallel lines.

Construct the following angles using set-squares: 75^{\circ}
In Fig., line n is a transversal to lines l and m. Identify the following:
Alternate and corresponding angles in Fig.

Classify the following angle as acute, obtuse, straight, right, zero and complete angle.
165^o.
Can a triangle have: All angles less than 60^{\circ}?
Justify your answer in each case.
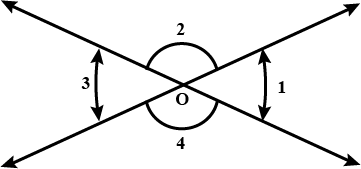
In figure, write down each pair of vertically opposite angles.

Write down each pair of adjacent angles shown in figure.
In figure, name all the pairs of adjacent angles.

In figure, name all the pairs of adjacent angles.
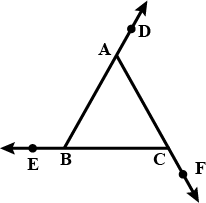
Are the angles 1 and 2 given in adjacent angles?
Classify the following angle as acute, obtuse, straight, right, zero, and complete angle.
89.5^o.
Classify the following angle as acute, obtuse, straight, right, zero and complete angle.
180^o.
Classify the following angle as acute, obtuse, straight, right, zero and complete angle.
75^o.
In figure, write down each linear pair.

One of the angles forming a linear pair is an obtuse angle. What kind of angle is the other?
One of the angles forming a linear pair is an acute angle. What kind of angle is the other?
In figure, A,\ B,\ C are collinear points and \angle DBA=\angle EBA. Name two pairs of supplementary angles.

One of the angles forming a linear pair is a right angle. What can you say about the other angle?
If the complement of an angle is 28^o, then find the supplement of the angle.
Find the complement of the following angle: 72^o.
If two supplementary angles have equal measure, what is the measure of each angle?
In figure, name each linear pair and each pair of vertically opposite angles.

Name the four pairs of supplementary angles shown in figure.

In figure, A, B, C are collinear points . Name two linear pairs.
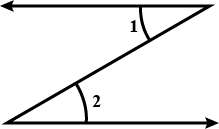
Can two acute angles form a linear pair?
In figure, it being given that \angle 1=65^o, find all other angles.
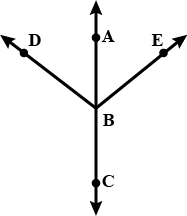
In figure, write all pairs of adjacent angles and all the linear pairs.
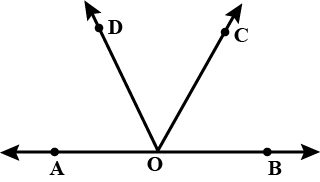
Find the value of x in the following figure.
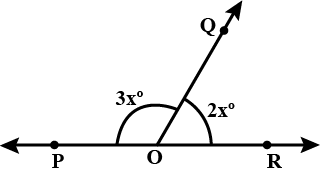
Find the value of x in the following figure.
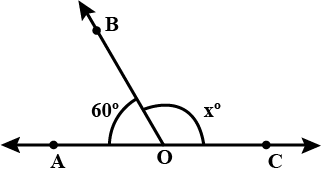
In figure, find \angle x. Further find \angle BOC, \angle COD and \angle AOD.
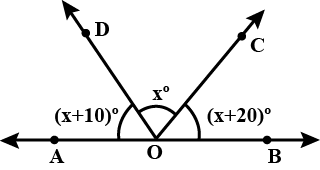
Find the value of x in the following figure.
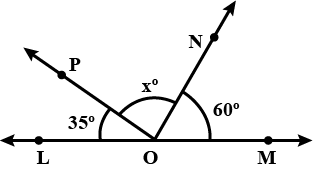
In figure, OA and OB are opposite rays, if x=25^{\circ}, what is the value of y^{\circ} ?
In figure, OA and OB are opposite rays if x=25^o, what is the value of y?
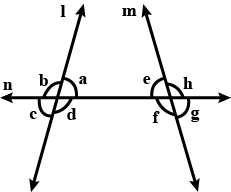
In figure, line n is a transversal to lines l and m. Identify the following alternate and corresponding angles in figure.

In figure, line n is a transversal to lines l and m. Identify the following pairs of interior and exterior angles on the same side of the transversal in figure.
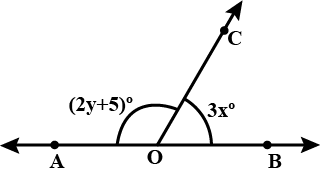
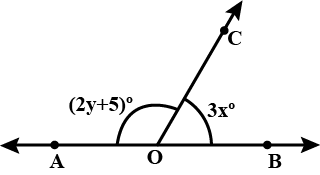
In figure, POS is a line, find x.
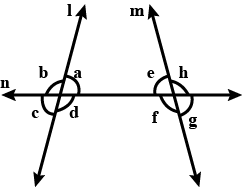
In figure, line l || m and n is a transversal. If \angle 1=40^o, find all the angles and check that all corresponding angles and alternate angles are equal.

In figure, line n is a transversal to lines l and m. Identify the following angles alternate to \angle d and \angle g and angles corresponding to angles \angle f and \angle h in figure.
In figure, AOC is a line, find x.
How many pairs of adjacent angles, in all, can you name in figure?

In figure, line\ XY\ ||\ line \ PQ and we have \angle ALY=(2x-15)^o, \angle LMQ=(x+40)^o, find x.
In figure, line n is a transversal to lines l and m. Identify the following:(a) angle alternate to \angle PQR, (b)angle corresponding to \angle RQF and(c) angle alternate to \angle PQE in figure.
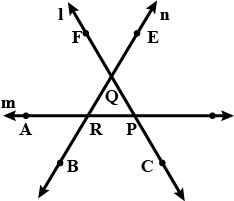
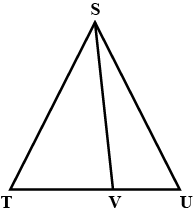
Name the triangle. Also, name the side opposite to \angle B.
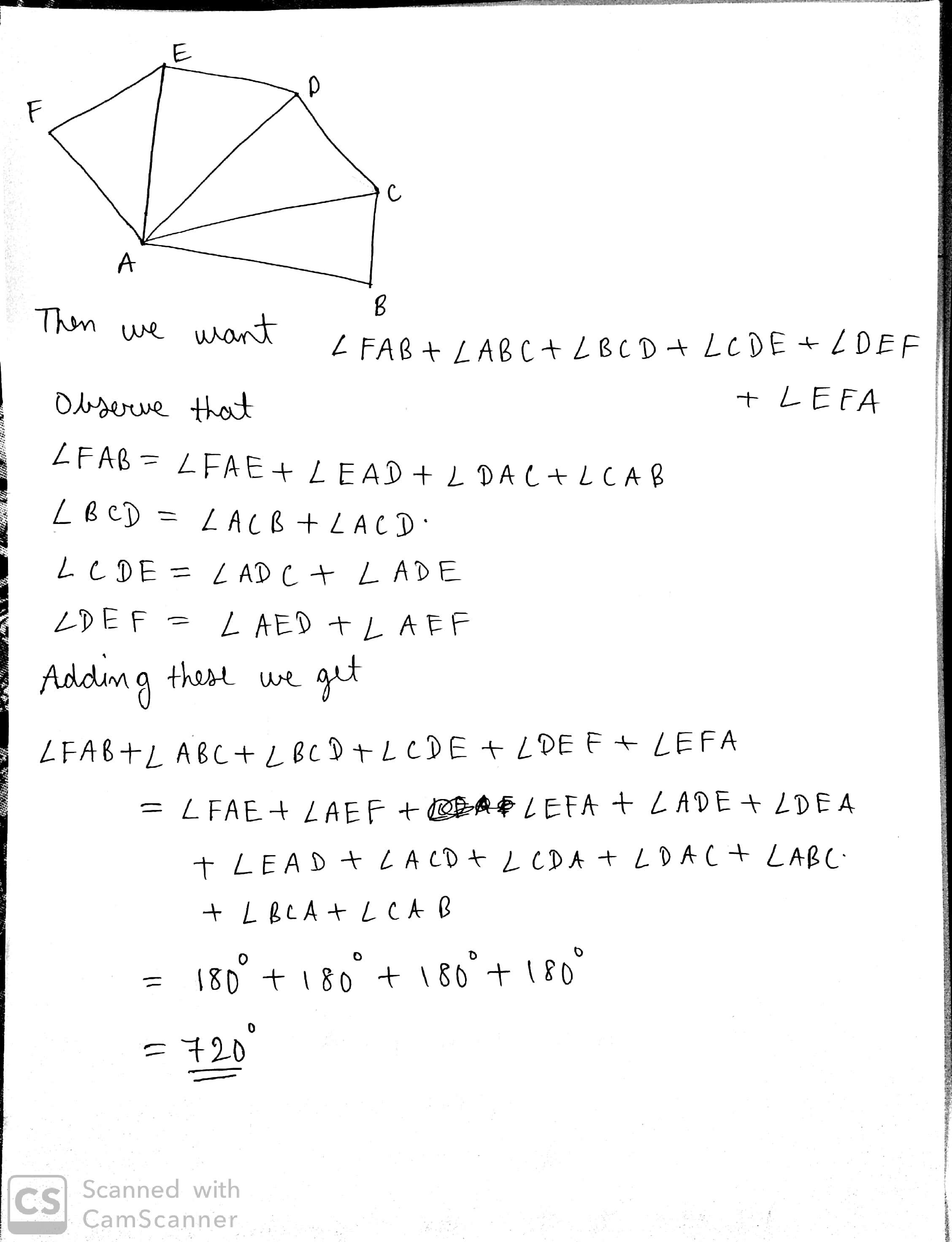
In the six concerned figure, AC, AD and AE are joined. Find \angle FAB+\angle ABC+\angle BCD+\angle CDE+\angle DEF+\angle EFA.

Find the value of x for which the angles {(2x-5)}^{o} and {(x-10)}^{o} are the complementary angles.
Find the supplement of the following angle:
\dfrac{3}{5} of a right angle
Find the measure of an angle that is equal to its supplement.
Find the supplement of the following angle:
{42}^{o}
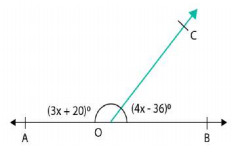
In the figure, what value of x will make AOB, a straight line?
Define the following term:
Obtuse angle
Find the supplement of the following angle:
{124}^{o}
Define the following term:
Supplementary angles
Define the following term:
Reflex angle
Two adjacent angles on a straight line are in the ratio 5:4. Find the measure of each one of these angles.
In \triangle PQR, if \angle P - \angle Q = 42^{\circ} and \angle Q - \angle R = 21^{\circ}, find \angle P, \angle Q and \angle R.
In the given figure, AB and CD are straight lines through the
centre O of a circle. If \angle AOC=80^o and \angle CDE=40^o, find \angle ABC
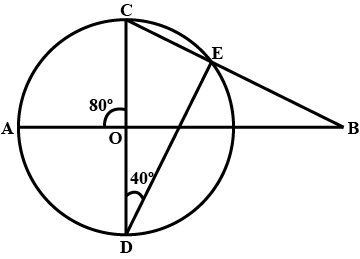
In the given figure, AB and CD are straight lines through the centre O of a circle. If \angle AOC=80^o and \angle CDE=40^o, find \angle DCE

In the given figure, O is the centre of the circle. If \angle PBC=25^o and \angle APB=110^o, find the value of \angle ADB.
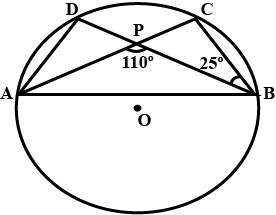
In the given figure, AB\parallel CD. Find the values of x,y and z.

In the given figure, O is the centre of the circle. If \angle ABD=35^o and \angle BAC=70^o, find \angle ACB.
In the figure, ABCD is a square and \triangle EDC is an equilateral triangle. Prove that
(i) AE=BE
(ii) \angle DAE={15}^{o}
In \triangle ABC, if \angle A + \angle B = 108^{\circ} and \angle B + \angle C = 130^{\circ}, find \angle A, \angle B and \angle C.
In \triangle ABC, if \angle A + \angle B = 125^{\circ} and \angle A + \angle C = 113^{\circ}, find \angle A, \angle B and \angle C.
In the adjoining figure, DE is a chord parallel to a diameter AC of the circle with centre O. If \angle CBD=60^o, calculate \angle CDE.

State the type of each of the following angles:
Calculate the value of x in each of the following figures.
In the given figure, O is the centre of a circle in which \angle OAB=20^o and \angle OCB=55^o. Find
\angle BOC

Two angles of a triangle are equal and the third angle is greater than each one of them by 18^{\circ}. Find the angles.
State the type of each of the given angle:
In a \triangle ABC, it is given that \angle A : \angle B : \angle C = 3 : 2 : 1 and CD \perp AC. Find \angle ECD.
Classify the angles whose magnitudes are given below:
30^{o}
State the type of each of the following angles:

In the given figure, O is the centre of a circle in which \angle OAB=20^o and \angle OCB=55^o. Find
\angle AOC

State the type of each of the following angles:
Classify the angles whose magnitudes are given below:
1\dfrac{1}{2} right angles?
Classify the angles whose magnitudes are given below:
128^{o}
Classify the angles whose magnitudes are given below:
(90.5)^{o}
Classify the angles whose magnitudes are given below:
(38.3)^{o}
Classify the angles whose magnitudes are given below:
80^{o}
Classify the angles whose magnitudes are given below:
91^{o}
Classify the angles whose magnitudes are given below:
179^{o}
Classify the angles whose magnitudes are given below:
2/3 right angle?
Classify the angles whose magnitudes are given below:
15^{o}
Classify the angle whose magnitude is given below:
181^{o}
Find the complement of the following angle: 47^\circ.
Find the supplement of the following angle:
123^\circ
Find the complement of the following angle: 73^\circ.
Find the supplement of the following angle:
80^\circ
Find the complement of the following angle: 60^\circ.
Can we have two obtuse angles, whose sum is:
(a) A reflex angle? Why or why not?
(b) A complete angle? Why or why not?
In figure
(a) Name any four angles that appear to be acute angles
(b) Name any two angles that appear to be obtuse angles.
Can we have two acute angles whose sum is
(a) An acute angle? Why or why not?
(b) A right angle? Why or why not?
(c) An obtuse angle? Why or why not?
(d) A straight angle? Why or why not?
(e) A reflex angle? Why or why not?
Number of angles less than {180}^{o} in figure is ____ and their names are _____.
An angle greater than {180}^{o} and less than a complete angle is called ______.
The supplement of a right angles is always ________ angle.
The supplement of an obtuse angle is always ______ angle.
If sum of measures of two angles is 90^{o}, then the angles are ______.
Alternate interior angles are on the _______ side of the transversal.
Alternate interior angles have one common ______.
State True or False:In a pair of complementary angles, each angle cannot be more than 90^{o}.
Two complementary angles differ by 20^o. Find the angles.
In each of the above figure write, if any, (i) each pair of vertically opposite angles, and (ii) each linear pair.
Name the pairs of supplementary angles in the above figure:

Write down each pair of adjacent angles shown in the above figures:
Name the pairs of supplementary angles in the above figure:

In the above figure write, if any, (i) each pair of vertically opposite angles, and (ii) each linear pair.

Two angles are making a linear pair. If one of them is one-third of the other, find the angles.
In Fig. P,Q and R are collinear points and TQ \bot PR,
Name:
Two pair of supplementary angles.
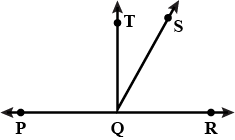
Can two acute angles form a pair of supplementary angles? Give reason support of your answer.
In the figure, P,Q and R are collinear points and TQ \bot PR,
name: A pair of complementary angles.
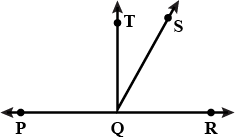
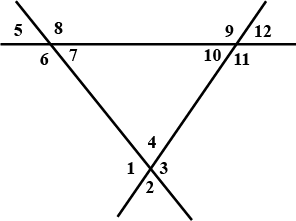
Two lines AB and CD intersect at O Fig. Write all the pairs of adjacent angles by taking angles 1,\ 2,\ 3,\ and 4 only.

Measure (in degree) of two supplementary angles are consecutive odd integers. Find the angles.
What is the type of other angle of a linear pair if one of its angle is obtuse?
What is the type of other angle of a linear pair if one of its angle is acute?
Measures (in degree) of two complementary angles are two consecutive even integers. Find the angles.
If the complementary of an angle is 62^o, then find its supplement.
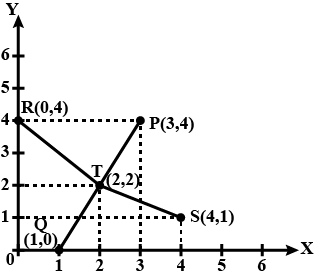
Locate the points P(3, 4),\ Q(1, 0),\ R (0, 4) and S(4, 1) on a graph sheet and write the coordinates of the point of intersection of line segments PQ and RS.
Find complement of the following:
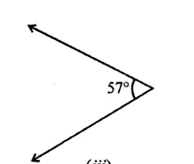
Find the supplement of the following:
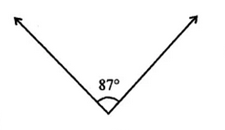
Read the following two statements which are taken as axioms:
(i) If two lines intersect each other, then the vertically opposite angles are not equal.
(ii) If a ray stands on a line, then the sum of two adjacent angles so formed is equal to 180^0.
Is this system of axioms consistent? Justify your answer.
Find the complement of the following:
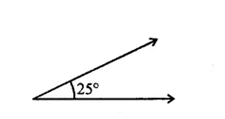
Find the complement of the following:

Find the supplement of the following:

The angles of a triangle are x, y and 40^0 the difference between the two angles x and y is 30^0 Find x and y .
Find the supplement of the following:
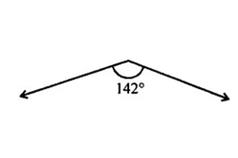
Identify the following pairs of angles as complementary or supplementary: 112^{0},68^{0}
Identify whether the following pairs of angles is complementary: 72^{0},18^{0}.
Find the value of x in the above diagram:
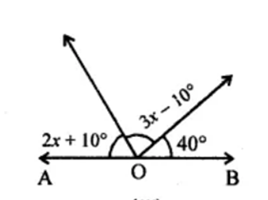
Identify the following pairs of angles as complementary or supplementary: 55^{0},125^{0}
Identify the following pairs of angles as complementary or supplementary: 137^{0},43^{0}
Two complementary angles are (x+4)^{0} and (2x-7)^{0}, find the value of x.
Find the value of x in the above diagram:
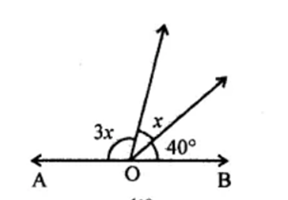
Identify whether the following pairs of angles is complementary: 45^{0},45^{0}.
Two supplementary angles are in ratio of 2:7, find the angles.
Identify whether the following pairs of angles is complementary:34^{0},56^{0}.
Identify each of the given pair of angles as alternate interior angles, co-interior angles or corresponding angles or non of these in the given figure.
(i) \angle 2,\angle 6
(ii) \angle 1,\angle 6
(iii) \angle 3,\angle 5
(iv) \angle 2,\angle 7
(v) \angle 3,\angle 6
(vi) \angle 4,\angle 8

If an angle is half of its complement, find the measure of angles.
Two adjacent angles are in ratio 5:3 and they together form an angle of 128^{0}, find these angles.
Can two right angles be complementary?
Angles of a linear pair are as well as ..
How many degrees are there in an angle which is one-fifth of its complement?
Adjacent angles have a common vertex, a common ______ and no common _______ points.
Two angles forming a linear pair are .
Find the supplementary angle of the following angles:
\frac{1}{2} of 90^{0}
Find the supplementary angle of the following angles:
\frac{3}{7} of 280^{0}
If two angles are complementary, then the sum of their measures is ____.
Supplement of an obtuse angle is .
If two adjacent angles are supplementary, then they form a ..
Write the complement angle of:
\dfrac{1}{6} of 90^{o}
In the following figures, ROPE is parallelograms. Find the measures of angles \mathrm{x}, \mathrm{y} and \mathrm{z} . State the properties you use to find them.
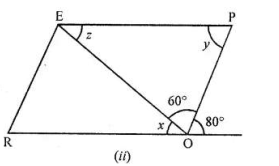
Write the complement angle of: 20^{o}+y^{o}.
Write the complement angle of: \dfrac{1}{2} of 60^{o}.
In the figure, given below name:
Two acute angles

In the figure, given below name:
Three pairs of adjacent angles.
Write the complement angle of:
\dfrac{2}{5} of 70^{o}
Write the complement angle of: \dfrac{1}{5} of 160^{o}.
Write the complement angle of: (x-10)^{o}.
In the figure, given below name:
Two obtuse angles

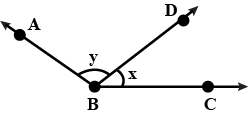
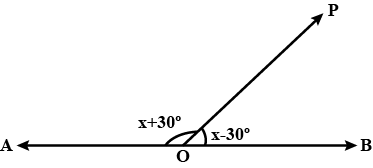
In the given figure, AOB is a straight line. Find the value of x and also answer each of the following:
\angle AOP =.....
Two supplementary angles are in the ratio 7:11. Find the angles
In the given figure, PQR is a straight line. If:
\angle PQS=110^o; find \angle RQS
In the given figure, PQR is a straight line. If:
\angle SQR=75^o; find \angle PQS
In the figure given, name:
Two reflex angles.

The measures of two supplementary angles are (3x+15)^{o} and (2x+5)^{o}. Find x.
Find the angle:
that is equal to its supplement?
Two complementary angles are in the ratio 7:8. Find the angles
Find the angle that is equal to its complement.
In the given diagram, ABC is a straight line
If x=53^o find y
In the given figure, AOB is a straight line. Find the value of x and also answer each of the following:
\angle BOP =.....
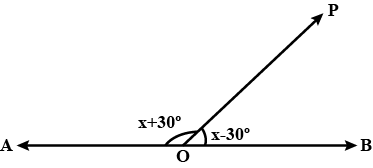
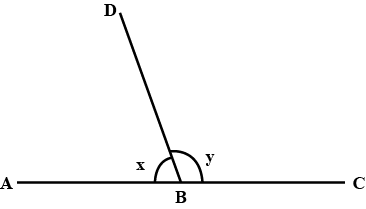
Figure given below shows a pair of adjacent angles AOB and BOC. Find whether or not the exterior arms OA and OC are in the same straight line.
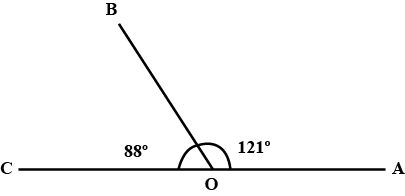
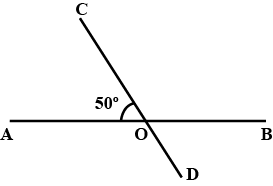
Two straight lines AB and CD intersect each other at a point O and angle AOC = 50^\circ, find:
\angle BOC
Figure given below shows a pair of adjacent anglesAOB and BOC. Find whether or not the exterior arms OA and OC are in the same straight line.
Two straight lines AB and CD intersect each other at a point O and angle AOC = 50^\circ, find:
\angle AOD
For an angle x^{o}, find:
the value of x^{o} if its supplementary angle is three times its complementary angle.
In the given figure, AOB is a straight line. Find the value of x and also answer each of the following:
Which angle is acute?
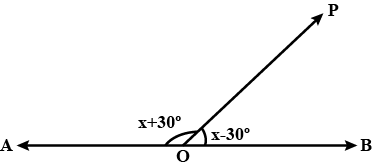
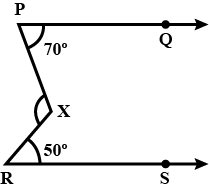
In the given figure, PQR is a straight line. Find x. Then complete the following:
\angle BQP = ________
In the given figure, PQR is a straight line. Find x. Then complete the following:
\angle AQB = ________
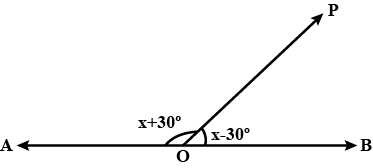
In the given figure, AOB is a straight line. Find the value of x and also answer each of the following:
Which angle is obtuse?
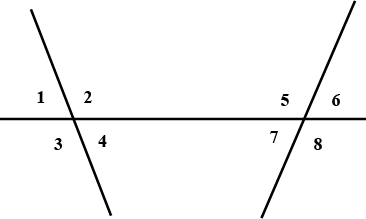
Identify the pair of angles given below by referring the given figure as adjacent angles, vertically opposite angles, interior alternate angles, corresponding angles or exterior alternate angles.\angle 4 and \angle 7
Identify the pair of angles given below by referring the given figure as adjacent angles, vertically opposite angles, interior alternate angles, corresponding angles or exterior alternate angles.
\angle 1 and \angle 8

Identify the pair of angles given below by referring the given figure as adjacent angles, vertically opposite angles, interior alternate angles, corresponding angles or exterior alternate angles.\angle 4 and \angle 11

Identify the pair of angles given below by referring the given figure as adjacent angles, vertically opposite angles, interior alternate angles, corresponding angles or exterior alternate angles.\angle 3 and \angle 5

Identify the pair of angles given below by referring the given figure as adjacent angles, vertically opposite angles, interior alternate angles, corresponding angles or exterior alternate angles.\angle 4 and \angle 8
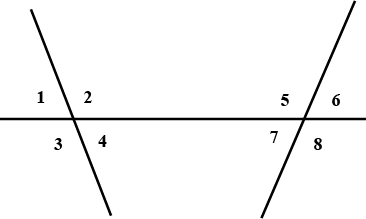
Identify whether the pair of angles given below by referring the given figure as adjacent angles, vertically opposite angles, interior alternate angles, corresponding angles or exterior alternate angles:
\angle 8 and \angle 10
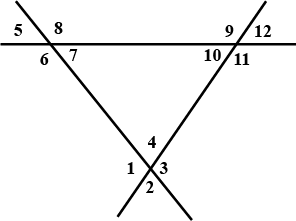
Identify the pair of angles given below by referring the given figure as adjacent angles, vertically opposite angles, interior alternate angles, corresponding angles or exterior alternate angles.\angle 1 and \angle 5

Identify the pair of angles given below by referring the given figure as adjacent angles, vertically opposite angles, interior alternate angles, corresponding angles or exterior alternate angles.\angle 1 and \angle 8
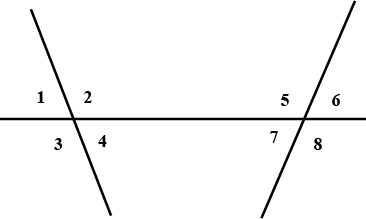
Identify whether the pair of angles given below by referring the given figure as adjacent angles, vertically opposite angles, interior alternate angles, corresponding angles or exterior alternate angles:\angle 2 and \angle 7
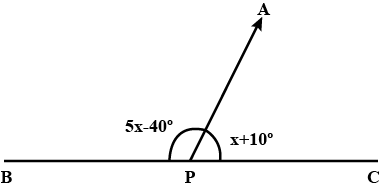
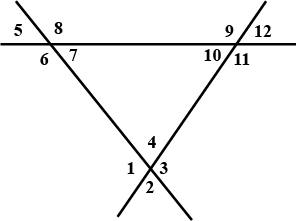
A line segment AP stands at point P of a straight line BC such that \angle APB = 5x - 40^\circ and \angle APC = x + 10^\circ, find the value of x and angle APB.
Identify the pair of angles given below by referring the given figure as adjacent angles, vertically opposite angles, interior alternate angles, corresponding angles or exterior alternate angles.\angle 5 and \angle 7
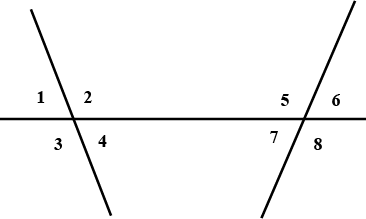
In the given figure, find the following:(i) x (ii) \angle AOB
(iii) \angle BOC
(ii) \angle AOB
(iii) \angle BOC
(iii) \angle BOC
In which of the following figures, are \angle AOB and \angle AOC adjacent angles? Give, in each case, reason for your answer.

In which of the following figures, are \angle AOB and \angle AOC adjacent angles? Give, in each case, reason for your answer.

Find y in the given figure.
Identify the pair of angles given below by referring the given figure as adjacent angles, vertically opposite angles, interior alternate angles, corresponding angles or exterior alternate angles.\angle 2 and \angle 8
In which of the following figures, are \angle AOB and \angle AOC adjacent angles? Give, in each case, reason for your answer.
In which of the following figures, are \angle AOB and \angle AOC adjacent angles? Give, in each case, reason for your answer.

In the adjoining figure, if b^o =a^o +c^o , find b.

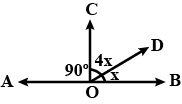
In the given figure, AB is perpendicular to BC at B .
Find:(i) the value of x.
(ii) The complement of angle x.
(ii) The complement of angle x.
Write the complement of: \dfrac{1}{2} of a right angle.
Write the complement of the following angle: a^o
Write the complement of: 21^o \, 17'.
Write the complement of: (30-a)^o .
Write the complement of: \dfrac{ 1}{3} of 180^o .
Write the complement of: (x+5)^o .
Write the complement of: 25^o .
Write the complement of: 90^o .
Write the supplement of:
100^o
Write the supplement of:
1/5 of a right angle
Write the supplement of:
(x+35)^o
Write the supplement of:
0^o
Write the supplement of:
(90+a+b)^o
Write the supplement of:
80^o \quad 49'\quad 25"
Are the following pairs of angles complementary?
37^0 \, 28' and 52^o \, 33'
Write the supplement of:
x^o
Are the following pairs of angles complementary?
10^o and 80^o .
Write the supplement of:
(110-x-2y)^o
In questions 1 and 2, given below, identify the given pairs of angles as corresponding angles, interior alternate angles, exterior alternate angles, adjacent angles, vertically opposite angles or allied angles:
\angle 4 \quad and \quad \angle 7

If 3x+18^o and 2x+25^o are supplementary, find the value of x .
Are the following pairs of angles supplementary?
139^o \quad and \quad 39^o
Are the following pairs of angles supplementary?
26^o 59' \quad and \quad 153^o 1'
Are the following pairs of angles supplementary?
2x^o +65^o \quad and \quad 115^o -2x^o
Are the following pairs of angles complementary?
(x+16)^o and (74-x)^o
In questions 1 and 2, given below, identify the given pairs of angles as corresponding angles, interior alternate angles, exterior alternate angles, adjacent angles, vertically opposite angles or allied angles:
\angle 1 \quad and \quad \angle 4
If two complementary angles are in the ratio 1:5 , find them.
In questions 1 and 2, given below, identify the given pairs of angles as corresponding angles, interior alternate angles, exterior alternate angles, adjacent angles, vertically opposite angles or allied angles:
\angle 3 \quad and \quad \angle 7
Are the following pairs of angles complementary?
54^o and \dfrac{2}{5} of a right angle.
Find the value of 'x' in each of the following diagram.
\angle COB = 90^o
Find the value of 'x' in each of the following diagram.
x + 2x = 180^o
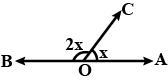
Recognise type of angles in following figures.
Adjacent angles.
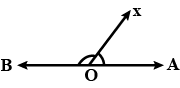
Fill in the blanks with acute, right and straight :
An angle whose measure is greater than that of a right angle is _________
Recognise the type of angles in the following figure.
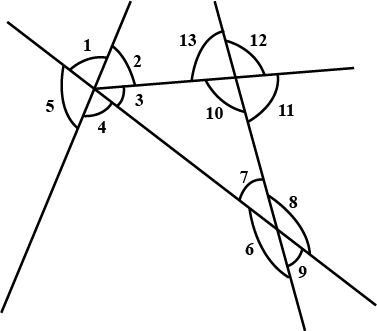
In questions 1 and 2, given below, identify the given pairs of angles as corresponding angles, interior alternate angles, exterior alternate angles, adjacent angles, vertically opposite angles or allied angles:
\angle 11 \quad and \quad \angle 8
It is given that \angle XYZ = 64^{\circ} and XY is produced to point P. Draw a figure from the given information. If ray YQ bisects \angle ZYP, find \angle XYO and reflex \angle QYP =?
In questions 1 and 2, given below, identify the given pairs of angles as corresponding angles, interior alternate angles, exterior alternate angles, adjacent angles, vertically opposite angles or allied angles:
\angle 6 \quad and \quad \angle 7
Two angles which are not adjacent angles, but still supplementary.
Fill in the blanks with acute, right and straight :
An angle whose measure is greater than that of a right angle is ________.
In figure, find the value of x .
If angles of magnitude (2x + 4) and (x - 1) from a linear pair. Find these angles.
Find the magnitude of \angle AOD
Name the adjacent supplementary angles of \angle AOC
Find the value of 'x' in each of the following diagram.
\angle AOB = 180^o
Which pair of angles are supplementary in the following diagram? Are they supplementary rays?
In the adjoining figure the sides BA and CA have been produced such that BA = AD and CA = AE. Prove that DE | | BC (Hint: use the concept of alternative angles).
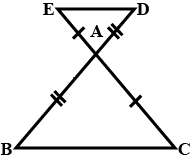
Write the pair of vertically opposite angles.
Suppose two adjacent angles are supplementary. show that one of them is obtuse angle, then the other angle must be acute.
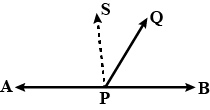
In the given figure, find x and also find \angle POS
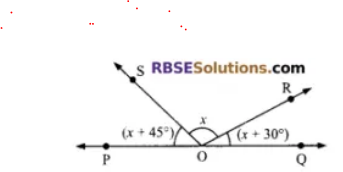
If the angles (3x - 20)^{\circ} and (2x - 40)^{\circ} are complementary angles. Find x .
In the given figure, POS is a line, find the measure of \angle QOR.
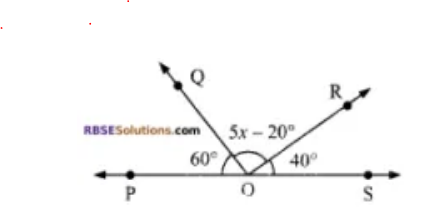
An angle is 25^{\circ} less than its complement. What is it measure?
In the figure, AO and BO are the bisectors of two adjacent angles A and B of a quad.ABCD. Prove that 2 \angle \mathrm{AOB}=\angle \mathrm{C}+\angle \mathrm{D}
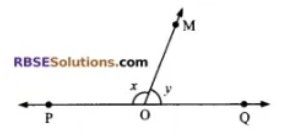
In the figure, \angle POM and \angle QOM from a linear pair. If x - 2y = 30^{\circ}, find x and y .
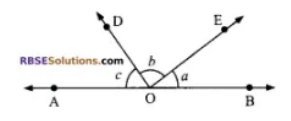
In the figure, a ; b : c = 4 : 3 : If AOB is a straight line, find the value of a, b and c
Evaluate x from figure
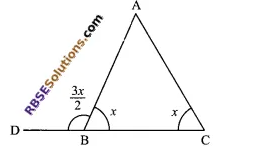
In the fig AB=AC and \angle ACD=120 find \angle A.
In given figure DE||BC and AD:DB=5:4 Find \dfrac { Area(\triangle DEF) }{ Area(\triangle CFB) } .
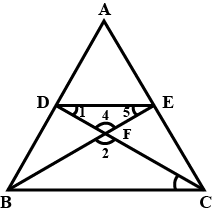
In the given figure,
If \angle AOB=\angle BOC=\angle COD= 38^{\circ},
find reflex angle \angle AOC and reflex angle \angle AOD.
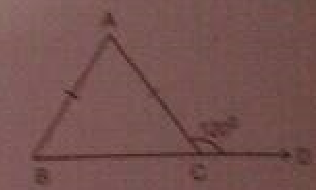
In the diagram given below \angle EDC. The tangent drawn to the circle at C makes an angle of {50}^{o} with AB produced. Find the measure of \angle ACB.
In figure, POQ is a line. Ray OR is perpendicular to line PQ. OS is another ray lying between rays OP and OR. Prove that \angle ROS=\dfrac{1}{2}(\angle QOS -\angle POS).

In fig. 6.57 ,\angle Q>\angle R. PA is the bisector of \angle QPR and PM\bot QR.Prove that \angle APM=\dfrac{1}{2}\left(\angle Q-\angle R\right).
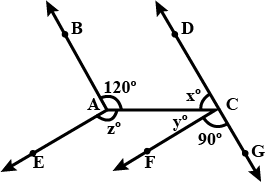
In figure, AB||CD and AE||CF, \angle FCG=90^o and \angle BAC=120^o. Find the values of x, y and z.
Construct the following angles using set-squares: 150^{\circ}
In figure, if array OC stands on line AB such that \angle AOC=\angle COB, then show that \angle AOC={ 90 }^{ \circ }
In Fig., \angle AOF and \angle FOG form a linear pair. If \angle EOB = \angle FOC = 90^{\circ} and \angle DOC =\angle AOB = 30^{\circ}.
Name three pairs of adjacenet angles.

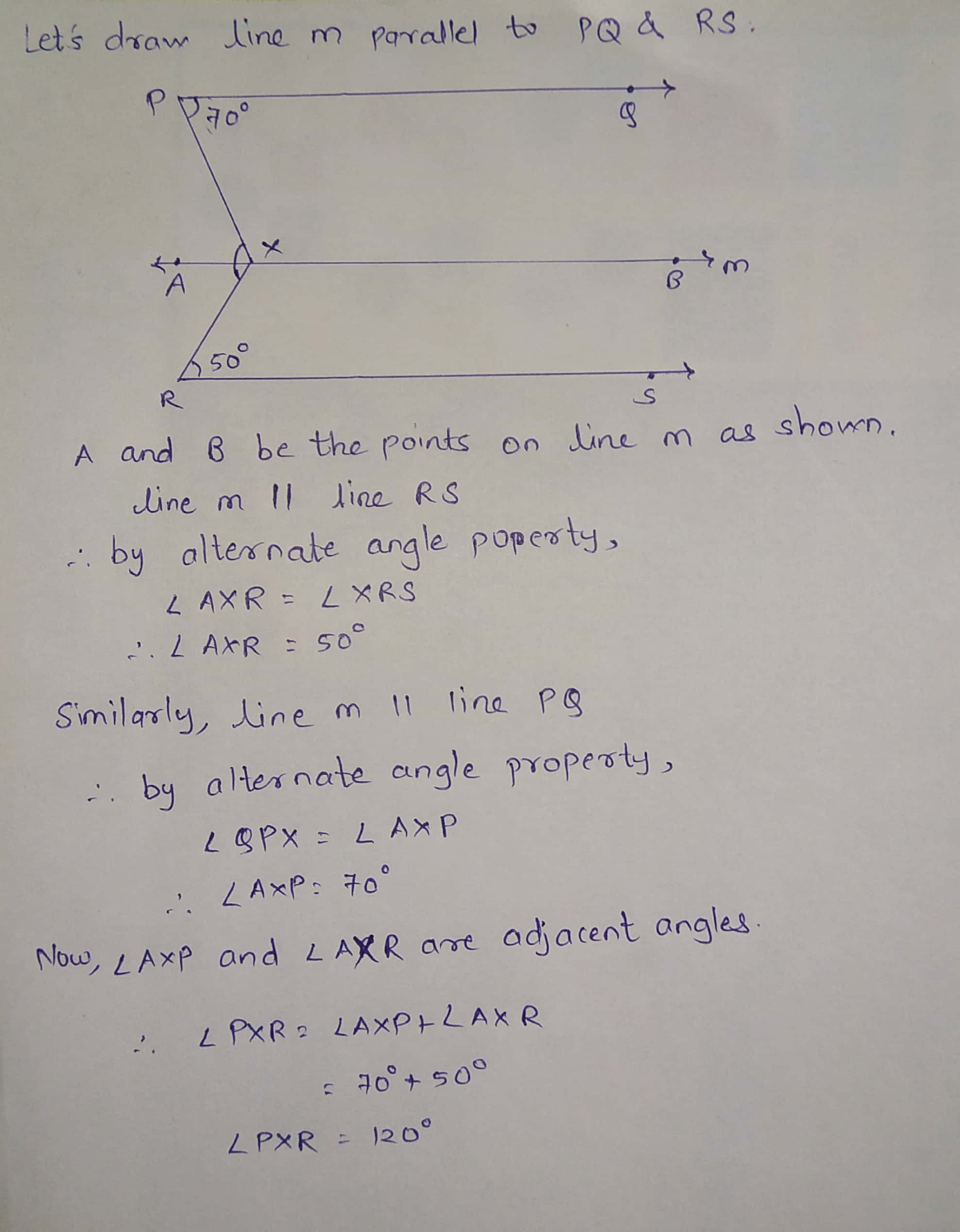
In figure, find out \angle PXR, if PQ || RS.
In the figure, AOB is a straight line. Find the value of x. Here, find \angle AOC,\angle COD and \angle BOD
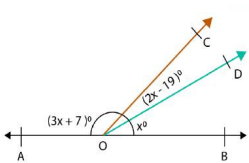
In the given figure, p^\circ =q^\circ =r^\circ , find each angle.
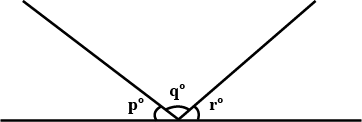
In each of the above figure write, if any, (i) each pair of vertically opposite angles, and (ii) each linear pair.
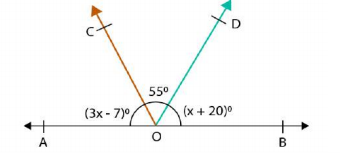
In the figure, AOB is a straight line, Find the value of x. Hence, find \angle AOC and \angle BOD.
In the given figure, PQR is a straight line. Find x. Then complete the following:
\angle AQR = ________
Class 9 Maths Extra Questions
- Areas Of Parallelograms And Triangles Extra Questions
- Circles Extra Questions
- Constructions Extra Questions
- Coordinate Geometry Extra Questions
- Heron'S Formula Extra Questions
- Introduction To Euclid'S Geometry Extra Questions
- Linear Equations In Two Variable Extra Questions
- Lines And Angles Extra Questions
- Number Systems Extra Questions
- Polynomials Extra Questions
- Probability Extra Questions
- Quadrilaterals Extra Questions
- Statistics Extra Questions
- Surface Areas And Volumes Extra Questions
- Triangles Extra Questions