Motion In A Straight Line - Class 11 Medical Physics - Extra Questions
If the relation between acceleration and time for an object is given by a=2t+4t2.
Calculate the position of object from the origin at t=4s (At t=0,v=0,x=0)
Consider a particle initially moving with a velocity of 5ms−1 starts decelerating at a constant rate of 2ms−2. Determine the time at which the particle becomes stationary.
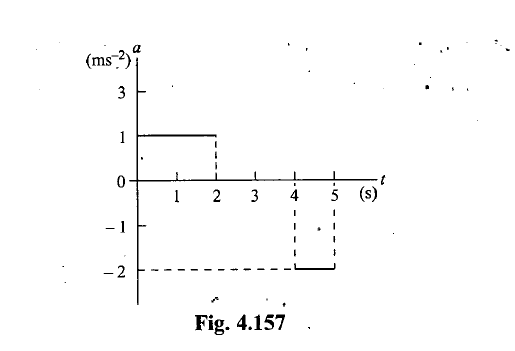
Starting at x=0, a particle moves according to the graph of v vs t shown in Fig. 4.Sketch a graph of the instantaneous acceleration a vs t, indicating numerical values at significant points of the graph.
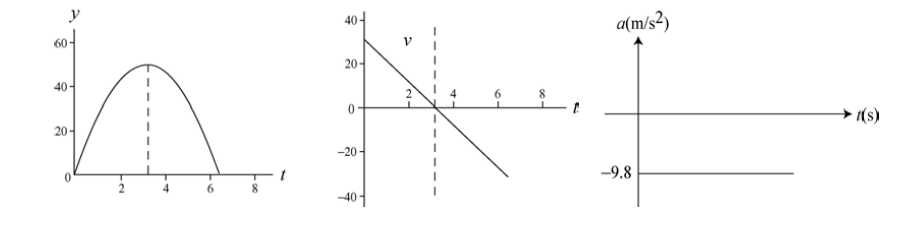
A ball is thrown vertically from ground level to rise to a maximum height of 50m. Sketch graphs of y,v and a versus t for the ball. On the first two graphs, indicate the time at which 50m is reached.
A vehicle is accelerating on a straight road. Its velocity at any instant is 30\ km/h, after 2s, it is 33.6\ km/h and after further 2s, it is 37.2\ km/h. Find the acceleration of vehicle in m/s^{2}. Is the acceleration uniform?
What is the acceleration time graph?
A train of length l=350\:m starts moving rectilinearly with constant acceleration \omega=3.0\cdot10^{-2}\:m/s^2; t=30\:s after the start the locomotive headlight is switched on (event 1), and \tau=60\:s after that event the tail signal light is switched on (event 2) . At what constant velocity V (in m/s) relative to the Earth must a certain reference frame K move for the two events to occur in it at the same point? (round off your answer to the nearest integer)
A car is to be hoisted by elevator to the fourth floor of a parking garage, which is 14 m above the ground. If the elevator can have maximum acceleration of 0.2 \displaystyle m/s^{2} and maximum deceleration of 0.1 \displaystyle m/s^{2} and can reach a maximum speed of 2.5 m/s, the shortest time to make the lift, starting form rest and ending at rest is K. The value of 2K will be
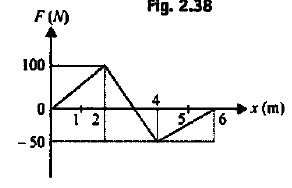
A stationary particle of mass m = 1.5\ kg is acted upon by a variable force. The variation of force with respect to displacement is plotted in fig.
Calculate the velocity acquired by the particle after getting displaced through 6\ m.
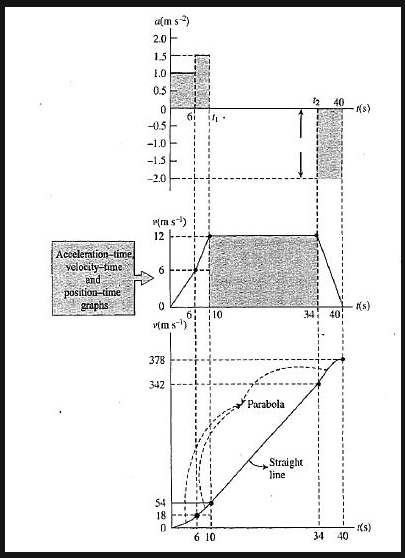
A local train leaves station A; it gains speed at the rate of 1 ms^{-2} for first 6 s and then at the rate of 1.5 ms^{-2} until it has reached the speed of 12 m/s. The train maintains the same speed until it approaches station B; brakes are then applied, giving the train a constant deceleration and bringing it to a stop in 6 s. If the total running time of the train is 40 s. Find (a) the distance between stations A and B. (b) Draw acceleration-time, velocity-time, and the position-time relation of motion.
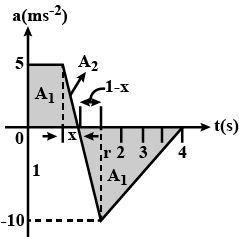
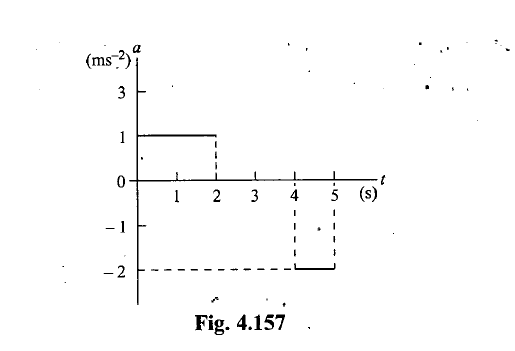
A particle moves along x-axis with an initial speed v_{0} = 5 ms ^{-1}. If its acceleration varies with time as shown in a-t graph in the figure.
a. Find the velocity of the particle at t = 4s.
b. Find the time when the particle starts moving along -x direction.

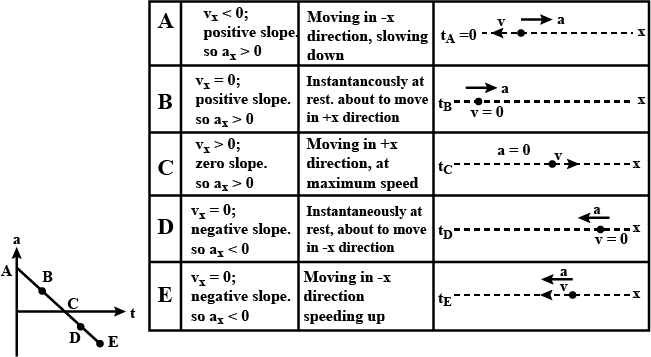
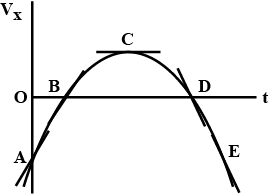
Consider the following v_x = t graph to be parabolic. Plot the acceleration-time graph and analyze the motion of the particle from A to E.
A car starting from rest on a straight road first acceleration with 6 m/s then suddenly decelerates with 3 m/s till it stops if total time of journey is 10 seconds, Then the maximum speed and distance acquired by the car is:
A golf ball is hit off a tee at the edge of a cliff. Its x and y coordinates as functions of time are given by x = 18.0t and y = 4.00t- 4.90t^{2}, where x and y are in meters and t is in seconds. (a) Write a vector expression for the balls position as a function of time, using the unit vectors \hat{i} and \hat{j}. By taking derivatives, obtain expressions for (b) the velocity vector \vec{v} as a function of time and (c) the acceleration vector \vec{a} as a function of time. (d) Next use unit-vector notation to write expressions for the position, the velocity, and the acceleration of the golf ball at t = 3.00 s.
The coordinates of an object moving in the xy plane vary with time according to the equations x = 25.00 \sin \omega t and y = 4.00 - 5.00 \cos\omega t, where v is a constant, x and y are in meters, and t is in seconds. (a) Determine the components of velocity of the object at t = 0. (b) Determine the components of acceleration of the object at t = 0. (c) Write expressions for the position vector, the velocity vector, and the acceleration vector of the object at any time t > 0. (d) Describe the path of the object in an xy plot.
A rocket has total mass M_{i}=360\ kg, including M_{f}=330\ kg of fuel and oxidizer, In interstellar space, it starts from rest at the position x=0, turns on its engine at time t=0, and puts out exhaust with relative speed v_{e}=1\ 500\ m/s at the constant rate k=2.50\ kg/s. The fuel will last for a burn time of T_{b}=M_{f}/k=330\ kg/(2.5\ kg/s)=132\ s.
Graph the acceleration as a function of time.
Class 11 Medical Physics Extra Questions
- Gravitation Extra Questions
- Kinetic Theory Extra Questions
- Laws Of Motion Extra Questions
- Mechanical Properties Of Fluids Extra Questions
- Mechanical Properties Of Solids Extra Questions
- Motion In A Plane Extra Questions
- Motion In A Straight Line Extra Questions
- Oscillations Extra Questions
- Systems Of Particles And Rotational Motion Extra Questions
- Thermal Properties Of Matter Extra Questions
- Units And Measurement Extra Questions
- Waves Extra Questions
- Work, Energy And Power Extra Questions