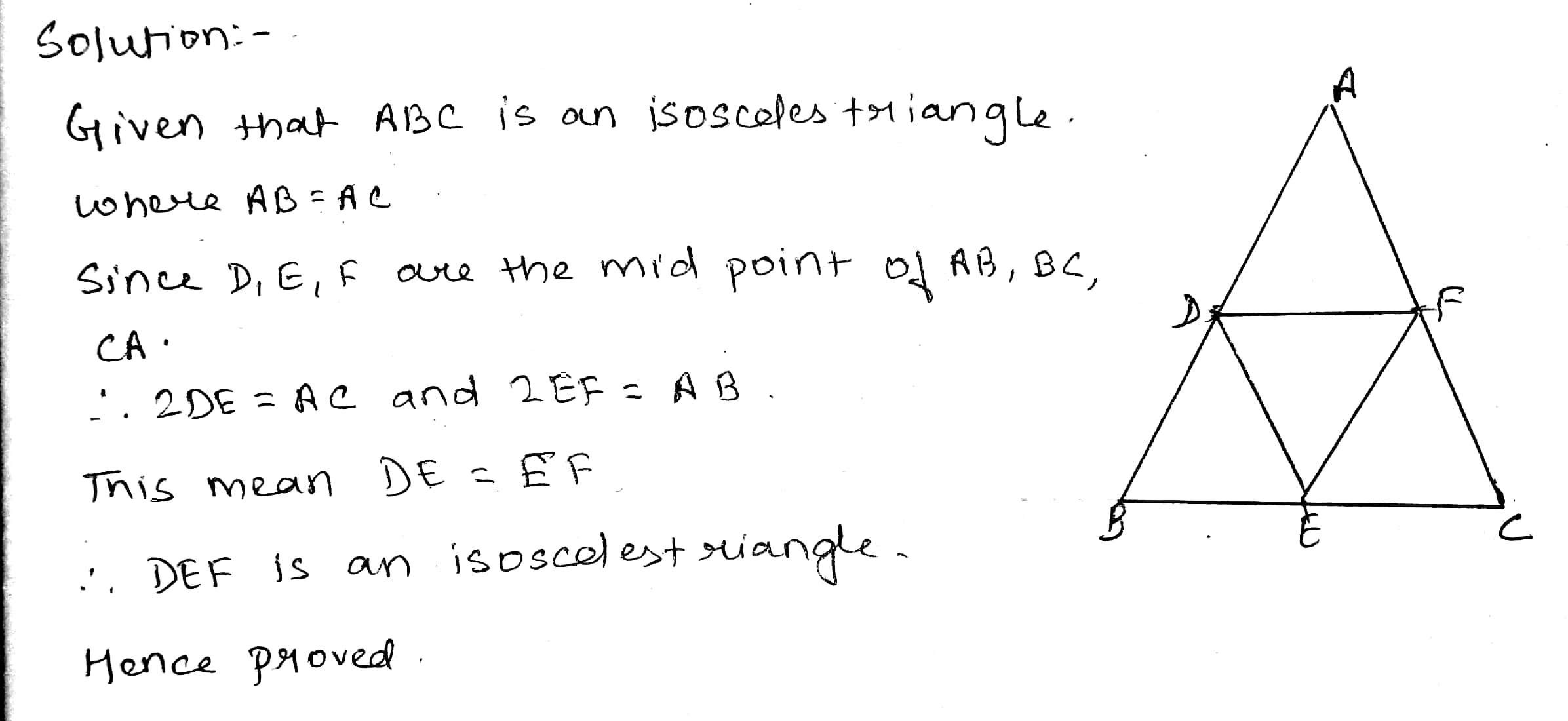Quadrilaterals - Class 9 Maths - Extra Questions
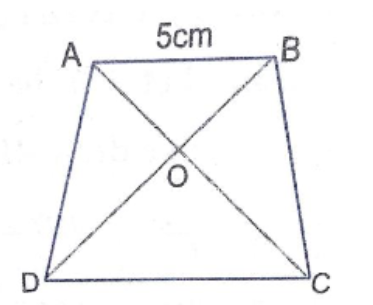
Can a quadrilateral ABCD be a parallelogram for the following condition?(Enter 1 for True and 0 for False)AB=DC=8 cm, AD=4 cm and BC=4.4 cm.
Can a quadrilateral ABCD be a parallelogram for the following condition?(Enter 1 for True and 0 for False)∠A=700 and ∠C=650.
If two adjacent angles of a parallelogram are in the ratio of 2:3 find all the angles of the parallelogram.
The angles of a quadrilateral are of the measures 120o, 90o, 72o and xo, then find x.
Three angles of a quadrilateral are of the measure 130o, 82o, 40o. Find the measure of the fourth angle.
The angles of a quadrilateral are in the ratio 2:3:5:8. Find the smallest angle of the quadrilateral.
In square ABCD, ∠A=120o. If ∠B=∠C=∠D, then find the measures of ∠B.
The measures of two angles of a quadrilateral are 55o each. The third angle is 150o. What is the measure of the fourth angle?
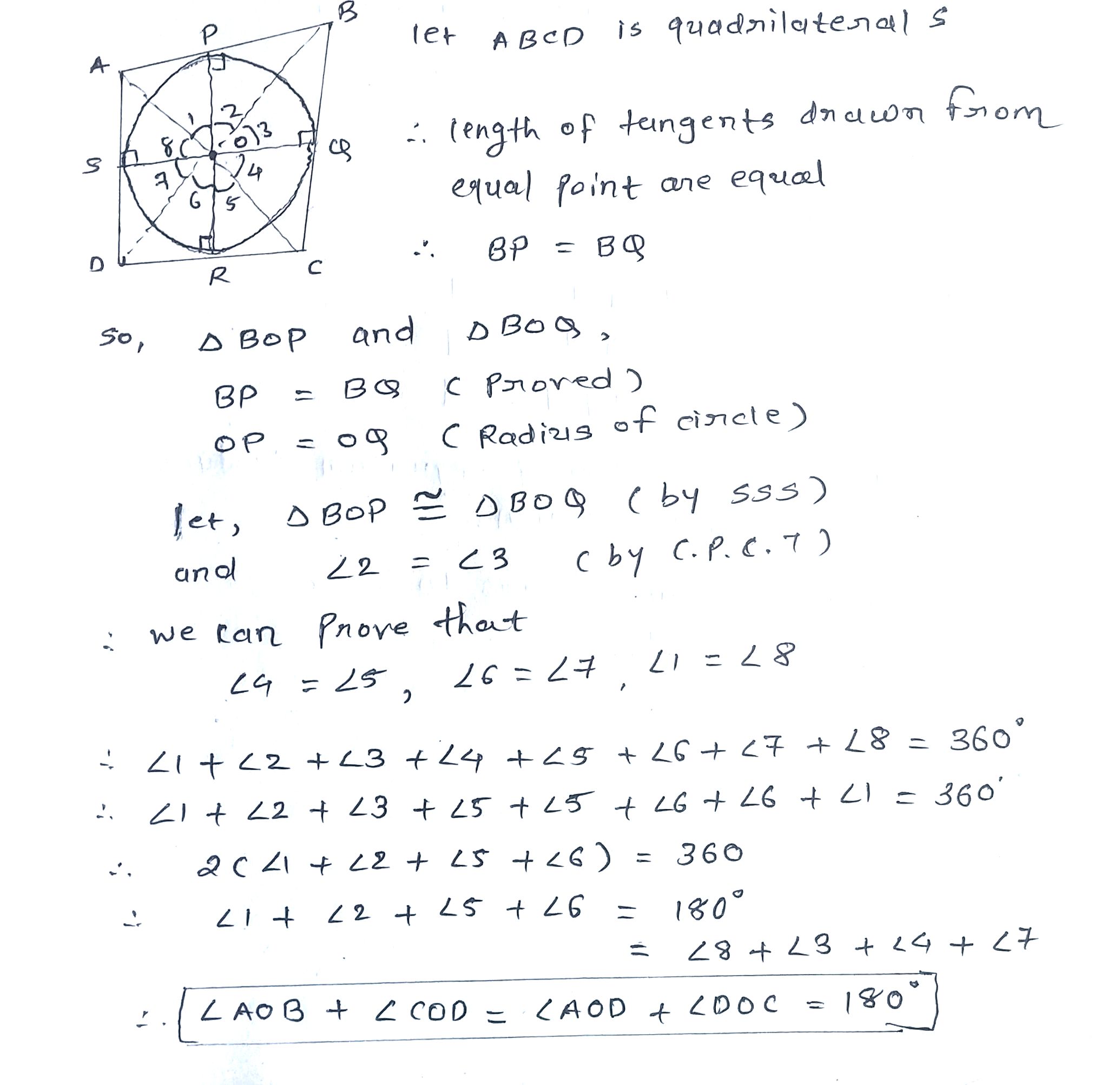
If all the sides of a parallelogram touches a circle, show that the parallelogram is a rhombus.
Examine whether the following points taken in order form a square.
(-1, 2), (1, 0), (3, 2) and (1, 4)
Examine whether the following points taken in order form a rectangle.
(8, 3), (0, -1), (-2, 3) and (6, 7)
Examine whether the following points taken in order form a square.
(0, -1), (2, 1), (0, 3) and (-2, 1)
Examine whether the following points taken in order form a square.
(5, 2), (1, 5), (-2, 1) and (2, -2)
A piece of wire is 60 cm long. What will be the length of each side if the wire is used to form a square?
Three angles of quadrilateral are respectively equal to 110∘,50∘,40∘. Find its fourth angle.
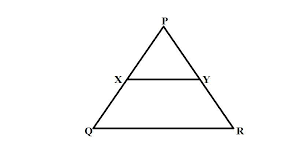
In the given figure, In ΔPQRXY ∥QR, PX:QX=1:3 YR=4.5cm and QR=9cm. Find XY. Further if Ar(ΔPXY)=Acm2, find in terms of A the area of ΔPQR and the area of trapezium XYRQ.
Quadrilateral MORE
MO=6 cm
OR=4.5 cm
∠M=60o
∠O=105o
∠R=105o
A figure is said to be regular if its sides are equal in length and angles are equal in measure. Can you identify the regular quadrilateral?
Fill in the blanks using the correct word given in brackets:
All squares are .......... (similar, congruent).
A quadrant has its all four interior angles equal. What is the measure of each interior angle.
The measure of two adjacent angles of a quadrilateral are 110o and 50o and the other two angles are equal. Find the measure of each angle.
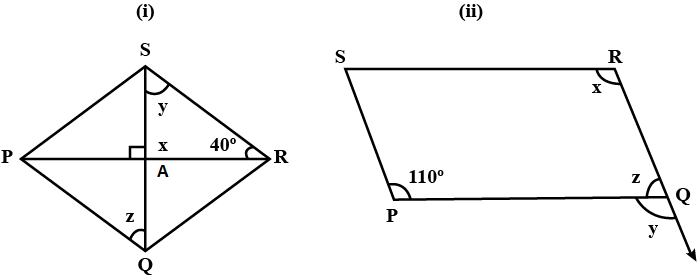
In the given figures, PQRS is a parallelogram .Find the value of x,y and z.
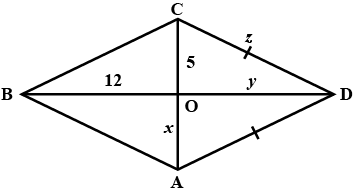
In the given figure, ABCD is a rhombus where BO=12cm and OC=5cm. find the values of x,y and z
The angle of a quadrilateral are respectively 100o,98o,92o. Find the fourth angle.
Show that the point (5,−1,1)(7,−4,7),(1,−6,10)(−1,−3,4) are the vertices of a rhombus.
Angles of a quadrilateral are in the ratio 3:4:4:7 . Find all the angles of the quadrilateral.
Prove that the sum of angles of a quadrilateral is 360o
The sum of two opposite angles of a quadrilateral is 172o. The other two angles of the quadrilateral are equal. Find the equal angles.
In a quadrilateral if opposite vertices are joint it is called_____
One of the angle of quadrilatral has measure \dfrac{2\pi}{9} radians and other three angles are in the ratio 3: 5: 8, Find the measures in radian.
Find the area of trapezium whose sum of parallel sides is 28 cm and height is 10 cm.
Diagonals of a quadrilateral JOSH intersect at P in such a way that ar(JPH) = ar(OPS).Prove that JOSH is a trapezium.
The four angles of a quadrilateral are equal. Find each of them.
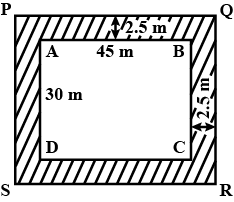
A rectangular park is 45 m long and 30 m wide. A path 2.5 m wide is constructed outside the park. Find the area of the path.
If the measures of internal angles of a quadrilateral are in the ratio of 3:6:4:5. what are the measures of its angle?
{ ABCD } is a parallelogram where { A } ( x , y ) , { B } ( 5,8 ) ,{ C } ( 4,7 ) and { D } ( 2 , - 4 ) . Find
(i) the coordinates of A.
(ii) the equation of the diagonal BD.
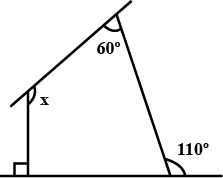
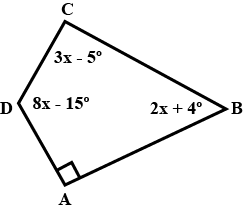
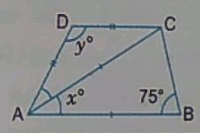
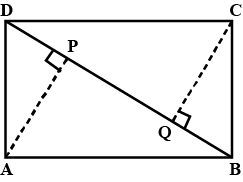
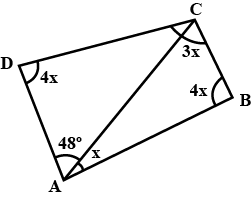
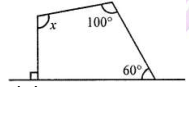
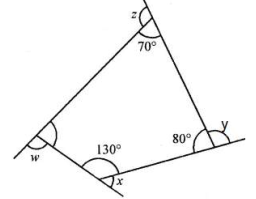
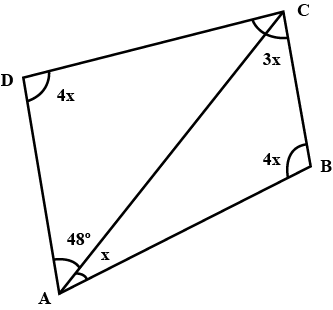
Find the value of x

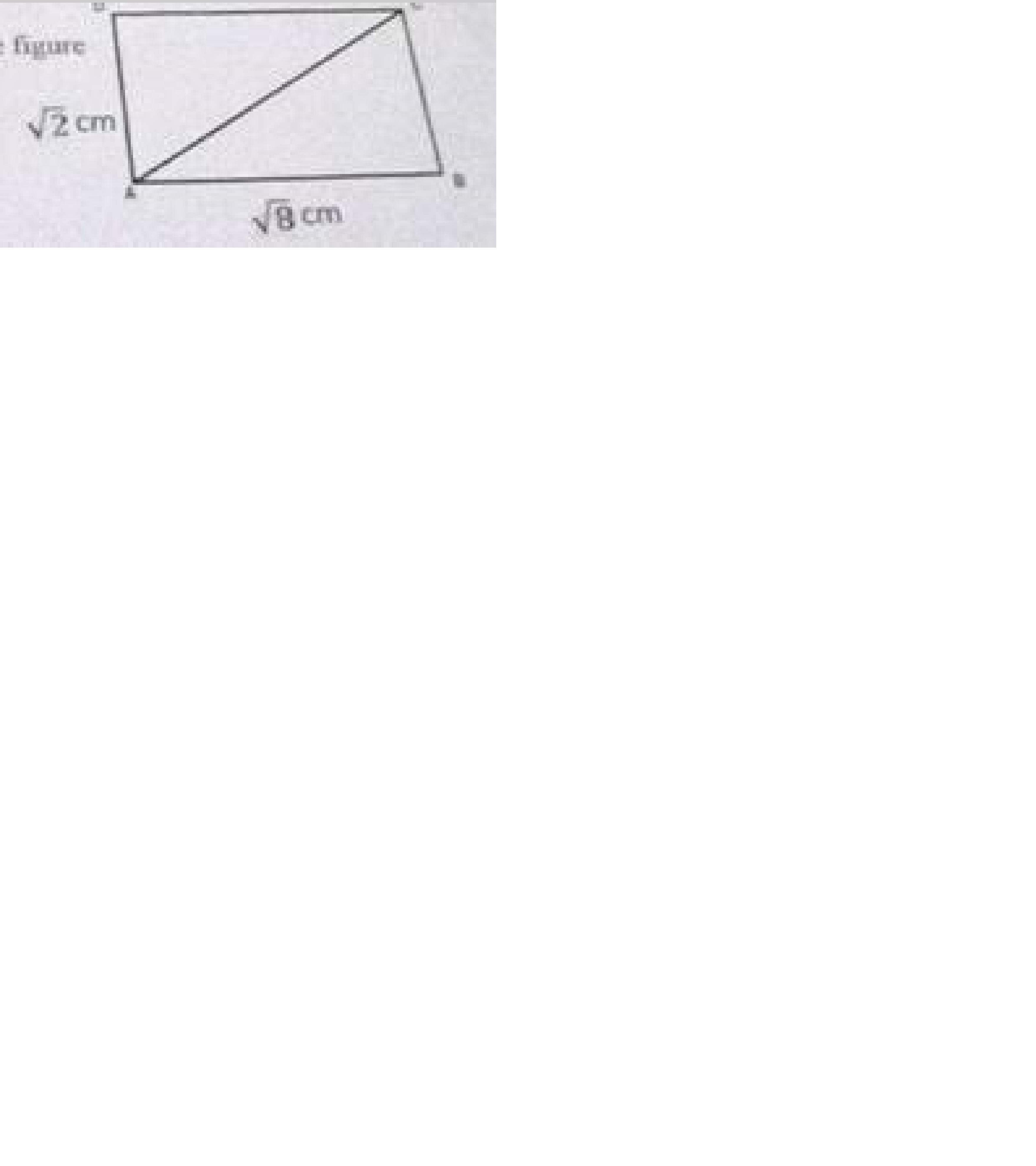
Find the area and perimeter of the rectangle in the figure.
Find the length of a diagonal of a quadrilateral whose area is 195\ m^{2} and the lengths of the perpendicular to the diagonal vertices are 8.5\ m and 11\ m respectively.
Write each properties of trapezium, parallelogram, rectangle, rhombus, square.
If the four angles of a quadrilateral are equal, what will be the measure of each angle ? What is the special name of this figure ?
The ratio of the angles of a quadrilateral is 3 : 5 : 7 : 9. Find the angles.
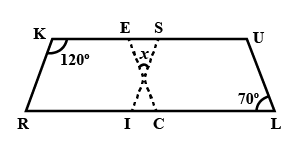
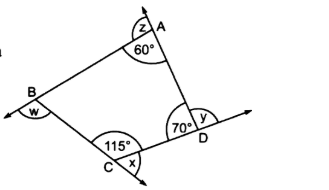
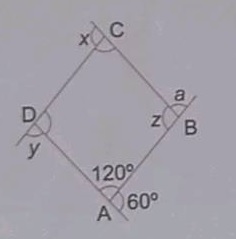
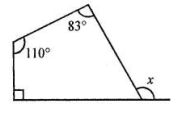
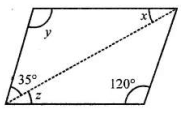
Find x in the picture:

Prove that a cyclic parallelogram is rectangle.
In the figure , 0 is the center of the circle and BA = AC.If\angle ABC = {50^.},find\angle BOC and \angle BDC
The angles of a quadrilateral are in the ratio 2:5:5:6. Find the greatest angle.
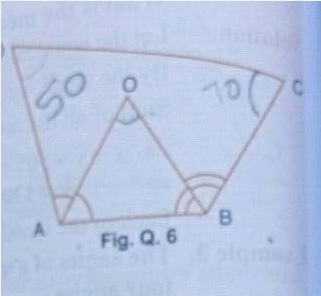
Using information given in the adjacent figure , find the value of x.
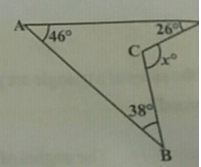
Find the value of a in the following figures.

on a line segment AB = 4 cm, construct a square,
ABCD is a quadrilateral such that diagonal AC bisects the angles \angle A an \angle C. Prove that AB=AD and CB=CD.
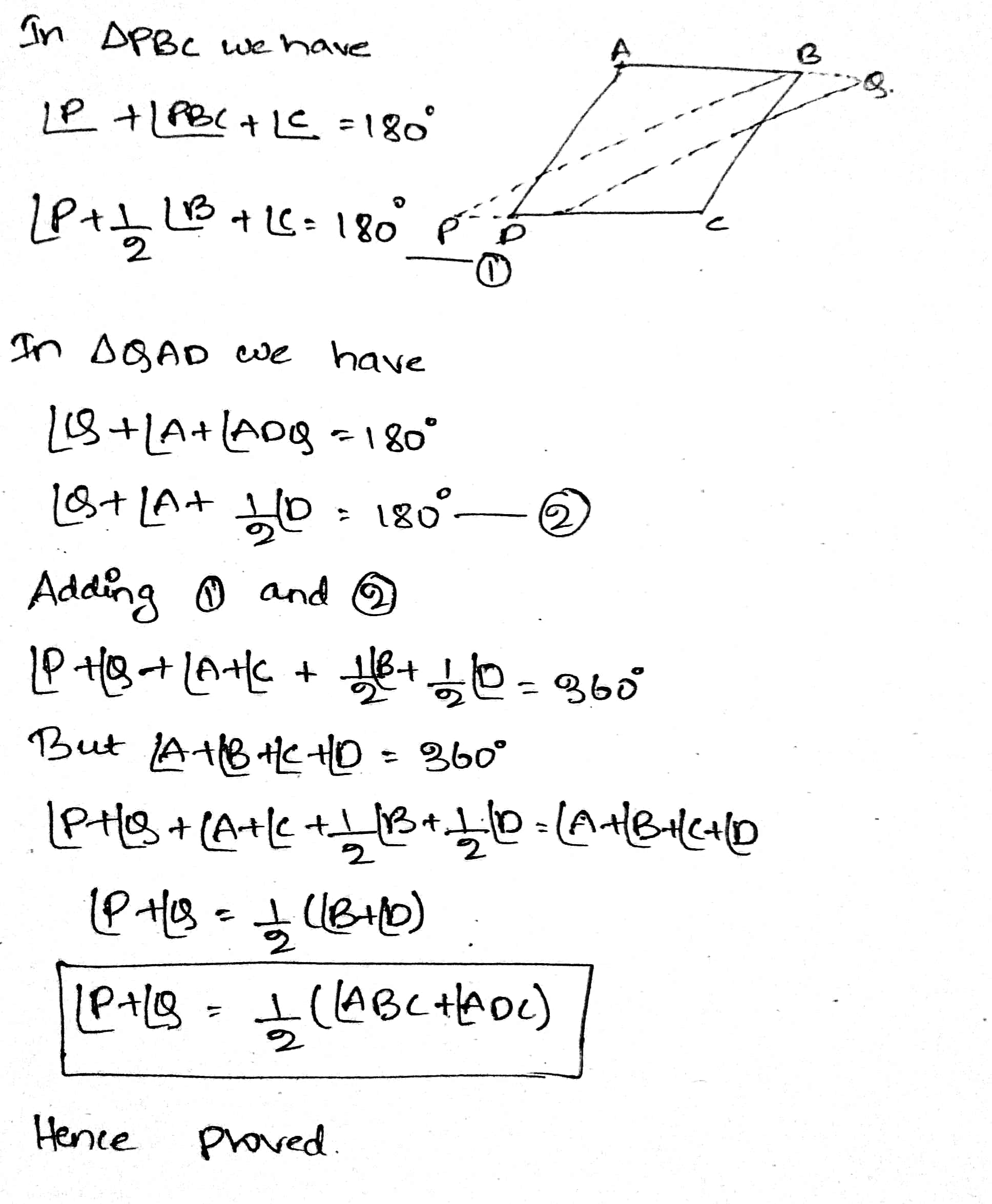
AO and DO are the bisectors of \angle A and \angle D of the quadrilateral ABCD. Prove that \angle AOD=\dfrac {1}{2}(\angle B+\angle C)
Find the value of x in each of the following figures.
What is the name given to a parallelogram whose all sides are equal ?
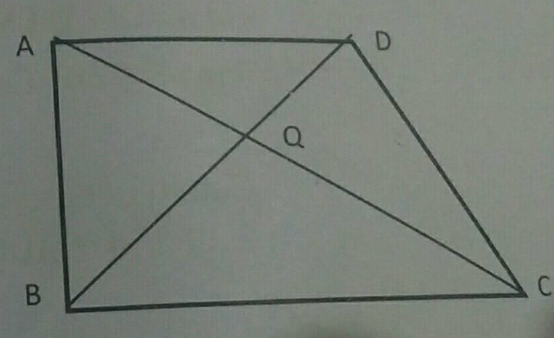
In \Box ABCD, side BC\parallel \ AD. Diagonal AC and diagonal BD Intersect in point Q. If AB=CD .Then show that \Delta ABC is congruent to \Delta DBC
In the following figure,
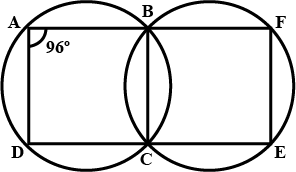
If \angle BAD=96^{o}, find \angle BCD and \angle BFE
In \Box ABCD, side BC < side AD
side BC \parallel side AD and if
side BA \cong side CD
then prove that \angle ABC \cong \angle DCB.
Find area of trapezium whose parallel sides 9cm and 5cm respectively and the distance between these sides is 8cm.
The angles of a qudarilateral are in the ratio 3 : 5 : 9 :Find all the angles of the quadrilateral.
Fill in the blanks:
(i) A quadrilateral with all sides and all angles equal is a .............
(ii) A quadrilateral with four equal sides and no right angles can be called a...................
(iii) A quadrilateral with exactly two sides parallel is a......................
(iv) The diagonals of this quadrilateral are equal but not perpendicular. The quadrilateral is a.............
(v) All rectangles, squares and rhombus are ............,but a trapezium is not.
What is trapezium ?
The angles of quadrilateral rae x,x+20,x+30,x+60 Find x
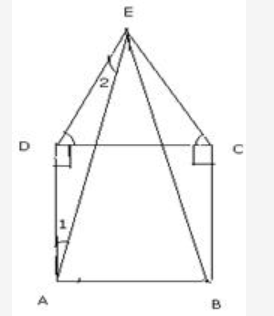
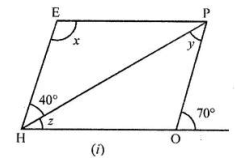
.Use the information given in the following figure to find :
(1) x
(2) \angle B and \angle C
EFGH is a parallelogram with diagonals EG and FH meeting at a point X. Show that ar(\Delta GXF)=ar(\Delta EXH)=ar(\Delta GXH)=ar(\Delta XEF)
The angles of a quadrilateral are in the ratio of 2:3:4:5, then find the angles.
In quadrilateral PQRS.
\angle P:\angle Q:\angle R:\angle S=3:5:9:7. Find all the angles of quadrilateral PQRS.
If a triangle and a parallelogram are on the same base and between the same parallels, then prove that area of triangle is half the area of parallelogram.
Give the difference between a square and a rhombus.
2 angles of quadrilateral are { 70 }^{ o } and { 80 }^{ o } third angle is supplementary of { 70 }^{ o } find the { 4 }^{ th } angle.
Do the angles 120,55,150,35 make a qudrilateral give reasons.
Find the area of the rectangles whose sides are:
3\ cm and 4\ cm
The angles of quadrilateral are x,2x,2x,5x+10 find the value of x
(i) How many pairs of adjacent sides does a quadrilateral have?
(ii) How many pairs of opposite sides does a quadrilateral have?
(iii) How many pairs of adjacent angles does a quadrilateral have?
(iv) How many pairs of opposite angles does a quadrilateral have?
(v) How many diagonals does a quadrilateral have?
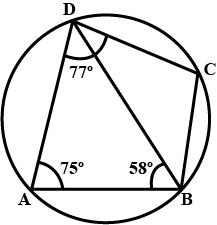
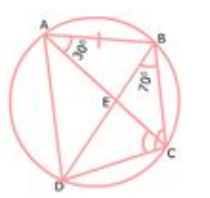
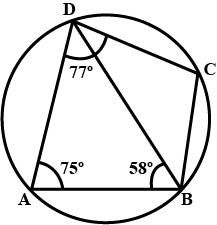
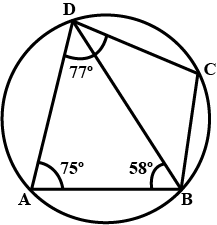
In the figure, given below, ABCD is a cyclic quadrilateral in which \angle BAD=75^{o}; \angle ABD=58^{o} and \angle ADC=77^{o}. Find:
\angle BDC
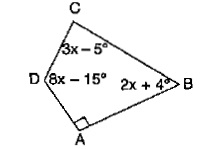
The angles in quadrilateral are 2x,3x-50,4x,5x-10 find x
Show that sum of all sides of a quadrilateral is greater than the sum of the diagonals
ABCD is a quadrilaterial Prove that A B+B C+C D+D A>A C+B D ?
The angle of a quadrilateral are in the ratio 3:5:9:Find all the angles of the quadrilateral.
Angles of a quadrilateral are \left( 4x \right) ^{ \circ },5\left( x+2 \right) ^{ \circ },\left( 7x-20 \right) ^{ \circ } and 6\left( x+3 \right) ^{ \circ }. Find each angle of the quadrilateral.
The diagram shows a regular pentagon and a kite.
Complete the following statement.
The kite has rotational symmetry of order _________.

Find the value of y
Find the area of the rectangles whose sides are:
12\ cm and 21\ cm
Find the area of the rectangles whose sides are:
2\ cm and 3\ cm
In the given figure
b=2a+15^{\circ} and
c=3a+5^{\circ}, find the values of b and c.
The angles of quadrilateral are 3x,4x,5x,6x. Find the value of x.
Angles of a quadrilateral are \left( 4x \right) ^{ \circ },5\left( x+2 \right) ^{ \circ },\left( 7x-20 \right) ^{ \circ } and 6\left( x+3 \right) ^{ \circ }. Find
the value of x.
The sum of the angles of a quadrilateral is_______
A quadrilateral has ...... diagonals.
The tree angles of a quadrilateral are 76^o, 54^o and 108^o . Find the measure of the fourth angle.
Solve the following :
Of the four quadrilaterals - square , rectangle , rhombus and trapezium - one is somewhat different from the others because of its design. Find it and give justifications.
In the given figure, ABCD is a quadrilateral shaped field in which diagonal BD is 36\ m, AL \bot BD and CM \bot BD such that AL=19\ m and CM=11 \ m. Find the area

Solve the following :
The point of intersection of diagonals of a quadrilateral divides one diagonal in the ratio 1 : 2 . Can it be a parallelogram ? Why or why not ?
A quadrilateral has_______ angles.
Prove that the sum of the angles of a quadrilateral is 360^o
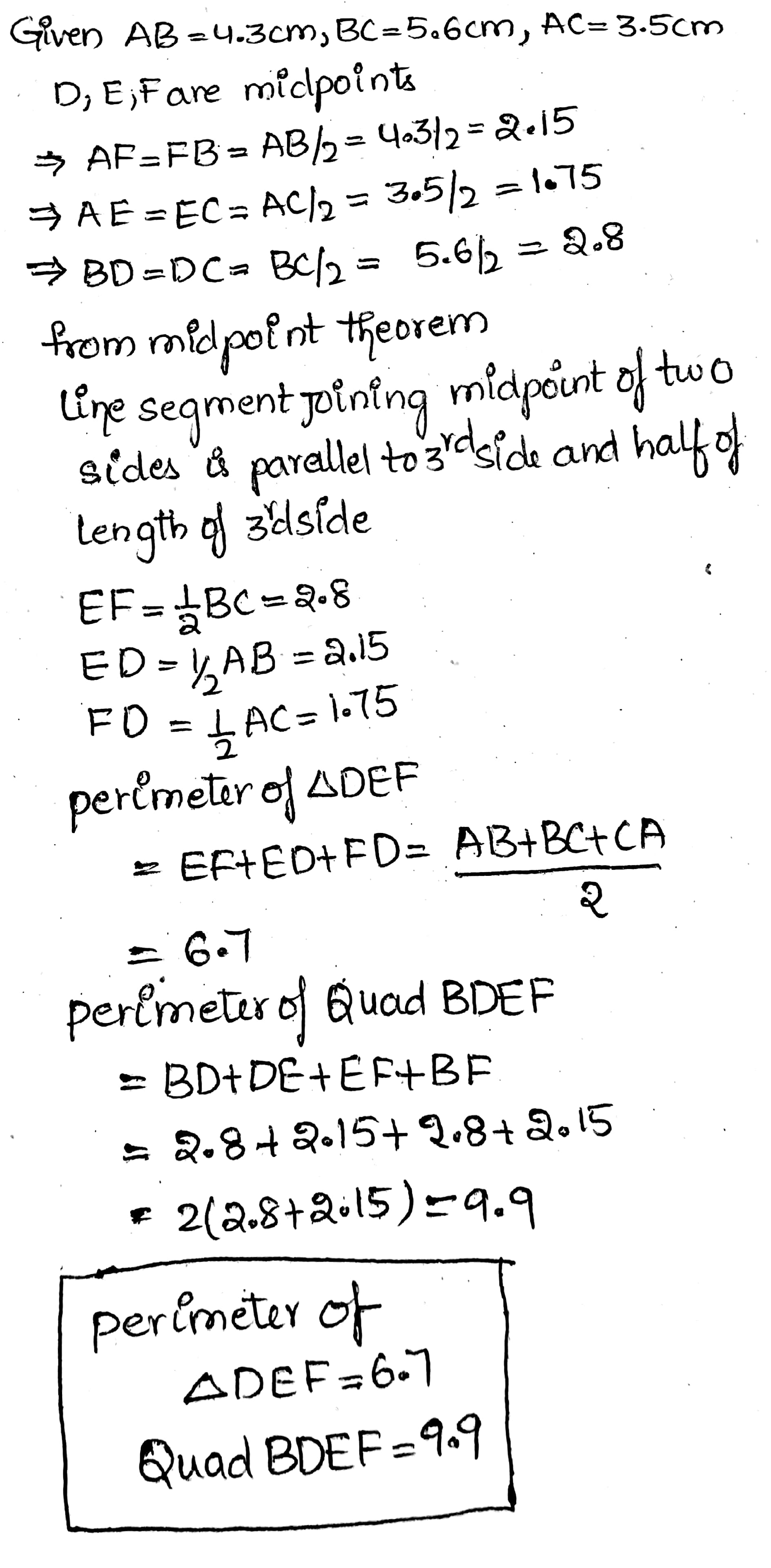
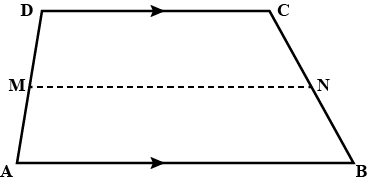
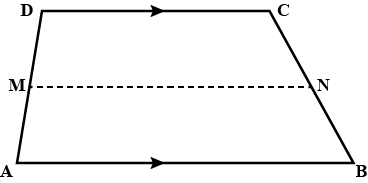
The figure given below, shows a trapezium ABCD. M and N are the mid-points of the non-parallel sides AD and BC respectively. Find:
MN, if AB=11\ cm and DC=8\ cm
The diagonal of a quadrilateral intersect at right angles. Prove that the figure obtained by joining the mid-points of the adjacent sides of the quadrilateral is a rectangle.
Prove that the figure obtained by joining the mid-point of the adjacent sides of a rectangle is a rhombus.
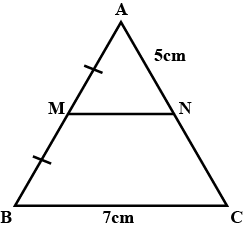
In triangle ABC, M is mid-point of AB and a straight line through M and parallel to BC cuts AC at N. Find the lengths of AN and MN, if BC=7\ cm and AC=5\ cm
Solve the following :
Two sticks each of length 5\,\text{cm} are crossing each other such that they bisect each other. What shape is formed by joining their endpoints? Give reason.
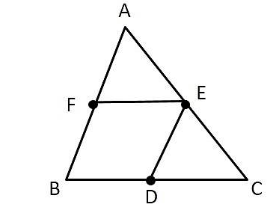
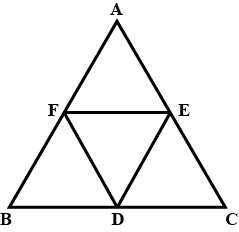
D, E and F are mid-point of the sides BC, CA and AB respectively of a \triangle ABC. Prove that:
(i) FDCE is a parallelogram
(ii) area of \Delta DEF =1 / 4 area of \Delta \mathrm{ABC}
(iii) area of \| \mathrm{gm} FDCE =1 / 2 area of \Delta \mathrm{ABC}
In the quadrilateral ABCD; prove that
AB+BC+CD > DA
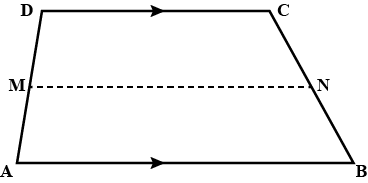
The figure given below, shows a trapezium ABCD. M and N are the mid-points of the non-parallel sides AD and BC respectively. Find:
AB, if DC=20\ cm and MN=27\ cm
The figure given below, shows a trapezium ABCD. M and N are the mid-points of the non-parallel sides AD and BC respectively. Find:
DC, if MN=15\ cm and AB=23\ cm
The following figure shows a trapezium ABCD in which AB\parallel DC. P is the mid-point of AD and PR\parallel AB. Prove that:
PR=\dfrac{1}{2}(AB+CD)
D, E and F are the mid-point of the sides AB, BC and CA respectively of triangle ABC, AE meets DF at O. P and Q are the mid-points of OB and OC respectively. Prove that DPQF is a parallelogram.
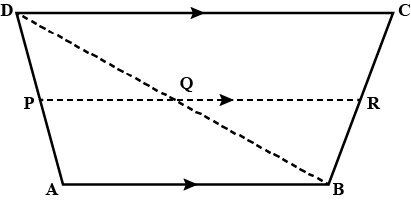
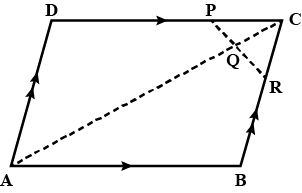
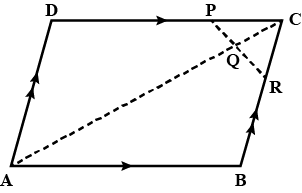
A parallelogram ABCD has P the mid-point of DC and Q a point of AC such that CQ=\dfrac{1}{4}AC. PQ produced meets BC at R
Prove that :
R is the mid-point of BC.
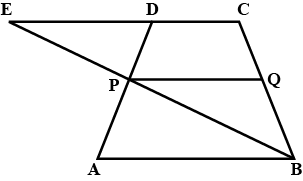
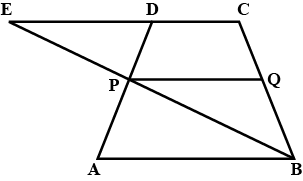
In trapezium ABCD, AB in parallel to DC; P and Q the mid-points of AD and BC respectively. BP produced meets CD produced at point E. Prove that:
Point P bisects BE
In the quadrilateral ABCD; prove that
AB+BC+CD + DA > 2BD
A parallelogram ABCD has P the mid-point of DC and Q a point of AC such that CQ=\dfrac{1}{4}AC. PQ produced meets BC at R
Prove that :
PR=\dfrac{1}{2}DB
In trapezium ABCD, AB in parallel to DC; P and Q the mid-points of AD and BC respectively. BP produced meets CD produced at point E. Prove that:
PQ is parallel to AB.
In a triangle ABC, P is the mid-point of side BC. A line through P and parallel to CA meets AB at point Q; and a line through Q and parallel to BC meets median AP at point R. Prove that:
BC=4QR
In a triangle ABC, P is the mid-point of side BC. A line through P and parallel to CA meets AB at point Q; and a line through Q and parallel to BC meets median AP at point R. Prove that:
AP=2AR
In the quadrilateral ABCD; prove that
AB+BC+CD + DA > 2AC
In quadrilateral ABCD, side AB is the longest and side DC is the shortest. Prove that:
\angle D > \angle B
D and F are mid-points of sides AB and AC of a triangle ABC. A line through F and parallel to AB meets BC at point E.
Prove that BDFE is a parallelogram.
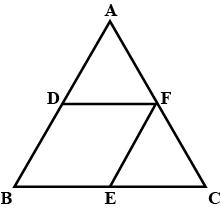
In triangle ABC, and B is obtuse. D and E are mid-point of sides AB and BC respectively and F is a point on side AC such that EF is parallel to AB. Show that BEFD is a parallelogram.
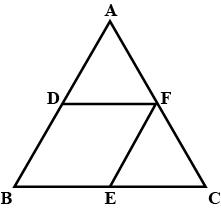
In parallelogram ABCD, E and F are mid-points of the sides AB and CD respectively. The line segments AF and BF meet the line segments ED and EC at points G and H respectively.
Prove that:
GEHF is a parallelogram.

D and F are mid-points of sides AB and AC of a triangle ABC. A line through F and parallel to AB meets BC at point E.
Find AB, if EF=4.8\ cm.
In a triangle ABC, AD is a median and E is mid-point of median AD. A line through B and E meets AC at point F.
Prove that: AC=3AF
In the figure, given below, 2AD=AB, P is mid-point of AB, Q is mid-point of DR and PR\parallel BS. Prove that:
AQ\parallel BS.
The side AC of a triangle ABC is produced to point E so that CE=\dfrac{1}{2}AC.\ D is the mid-point of BC and ED produced meets AB at F. Lines through D and C are drawn parallel to AB which meet AC at point R respectively. Prove that:
3DF=EF
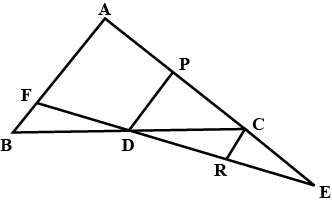
The side AC of a triangle ABC is produced to point E so that CE=\dfrac{1}{2}AC.\ D is the mid-point of BC and ED produced meets AB at F. Lines through D and C are drawn parallel to AB which meet AC at point R respectively. Prove that:
4CR=AB

In parallelogram ABCD, E and F are mid-points of the sides AB and CD respectively. The line segments AF and BF meet the line segments ED and EC at points G and H respectively.
Prove that:
Triangles HEB and FHC are congruent.
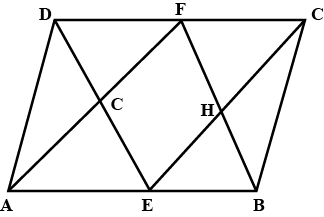
In triangle ABC, M is mid-point of AB, N is mid-point of AC and D is any point in base BC. Use Intercept Theorem to show that MN bisects AD.
Can the angles 110^\circ, 80^\circ, 70^\circ and\ 95^\circ be the angles of a quadrilateral? Why or why not?
If the quadrilateral formed by joining the mid-points of the adjacent sides of quadrilateral ABCD is a rectangle, show that the diagonal AC and BD intersect at right angle.
In triangle ABC; D and E are mid-points of sides AB and AC respectively. Through E, a straight line is drawn parallel to AB to meet BC at F. Prove that BDEF is a parallelogram
If AB=16\ cm, AC=12\ cm and BC=18\ cm, find the perimeter of the parallelogram BDEF.
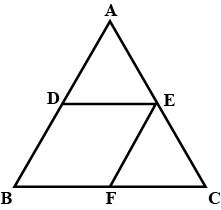
In quadrilateral ABCD , AD = BC and BD = CA prove that
\angle ADB = \angle BCA

In parallelogram ABCD, E is the mid-point of AB and AP is parallel to EC which meets DC at point O and BC produced at P. Prove that:
O is mid-point of AP.
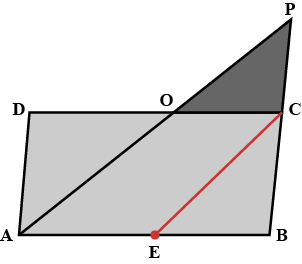
In quadrilateral ABCD , AD = BC and BD = CA prove that
\angle DAB = \angle CBA
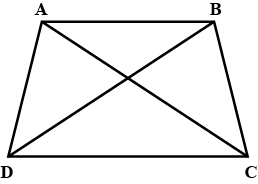
ABCD is a rectangle in which diagonal AC bisects A as well as C. Show that ABCD is a square.
A quadrilateral ABCD will be a parallelogram for the following conditions.
\angle D + \angle B = 180^{0}
If true then enter 1 and if false then enter 0
ABCD is a convex quadrilateral with
AB + BC \leq AC + CD. Prove that AB < AC.
If a quadrilateral ABCD is such that \displaystyle \vec{AB}= b, \vec{AD}= d and \displaystyle \vec{AC}= pb+qd(p+q\geq 1), then show that the area of the quadrilateral is \displaystyle \frac{1}{2}\left ( p+q \right )\left | b\times d \right |.
If all angles of a quadrilateral are congruent, then find their measures.
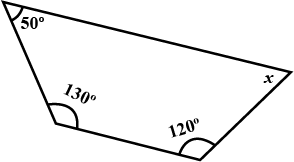
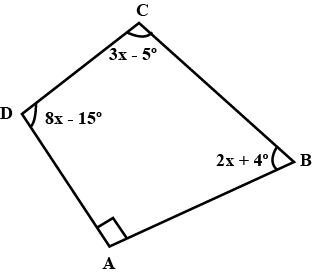
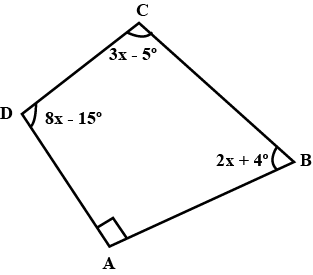
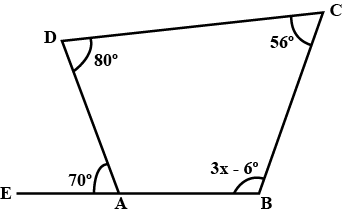
Use the information given in the following figure to find :x (in degrees).
The bisectors of angles B and C of a parallelogram ABCD meet at point O. If the triangle OBC formed is an isosceles right triangle, then ABCD is a rectangle.
If the above statement is true then mention the answer as 1, else mention 0 if false.
State True or False: The figure obtained by joining the mid-points of the adjacent sides of a rectangle is a Trapezium
Construct a rhombus whose diagonals are 47\ cm and 54\ cm.
In rectangle ABCD, the bisectors of angles B and C meet at point O then triangle OBC is an isosceles right triangles.
If the above statement is true then mention answer as 1, else mention 0 if false
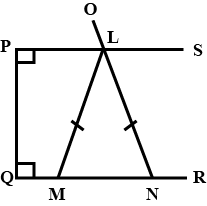
In the fig. given measure of angle \angle PLN=110^o. Then the measure of \angle LMN in degrees is:
ABCD is a trapezium with AB\: // \:DC . A line parallel to AC intersects AB at point M and BC at point N . Prove that : area \: of \: \bigtriangleup ADM \: = \: area \: of \: \bigtriangleup \: ACN.
The diagonal BD of a parallelogram ABCD bisects angles B and D. Prove that ABCD is a rhombus.
Show that the quadrilateral formed by joining the mid-points of the consecutive sides of a rectangle is a rhombus.
The angles of a quadrilateral ABCD are in the ratio $$4 : 9 : 10: 13$$. Find the angles.
In the adjoining figure, ABCD is a trapezium in which AB\;\parallel\;DC\;and\;AD=BC. If P,\,Q,\,R,\,S be respectively the midpoints of BA,\,BD\;and\;CD,\;CA then show that PQRS is a rhombus.
In the given figure, find the values of x and y.

Prove that all four angles of a quadrilateral cannot be obtuse angles.
Prove that all four angles of a quadrilateral cannot be acute angles
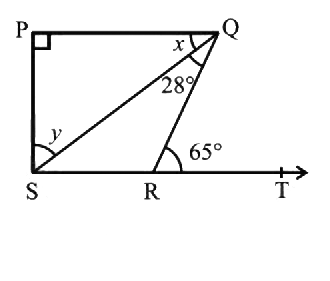
In Fig. 6.43, if PQ \perp PS, PQ\parallel SR,\angle SQR={ 28 }^{ 0} and \angle QRT={ 65 }^{ 0}, then find the values of x and y.
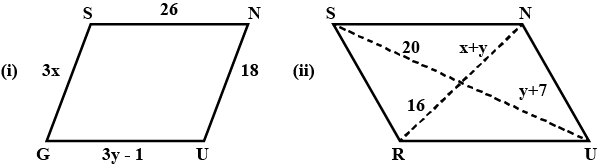
The following figures GUNS and RUNS are parallelograms. Find x and y. (Lengths are in cm)
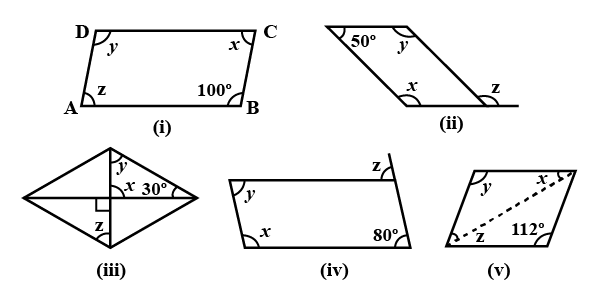
The above figure is a parallelogram. Find the angle measures x, y and z. State the properties you use to find them.

The angles of quadrilateral are in the ratio 3 : 5 : 9 : 13. Find all the angles of the quadrilateral.
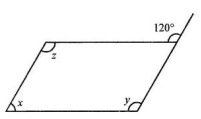
Consider the following parallelograms. Find the values of the unknowns x, y, z
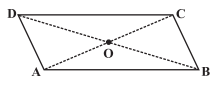
Given a parallelogram ABCD. Complete each statement along with the definition or property used.
(i) AD =(ii) \angle DCB =(iii) OC =(iv) m \angle DAB + m \angle CDA =
Can a quadrilateral ABCD be a parallelogram if
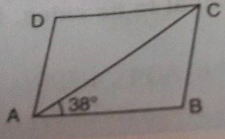
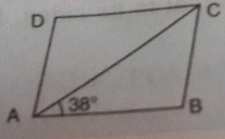
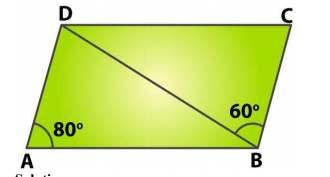
(i) \angle D + \angle B = 180^{\circ}? (ii) AB = DC = 8 cm, AD = 4 cm and BC = 4.4 cm? (iii) \angle A = 70^{\circ} and \angle C = 65^{\circ}?
In the above figure both RISK and CLUE are parallelograms. Find the value of x.
Show that the line segments joining the mid-points of the opposite sides of a quadrilateral bisect each other
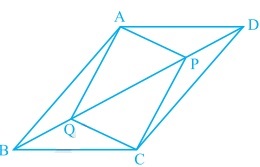
In parallelogram ABCD, two point P and Q are taken on diagonal BD such that DP = BQ. Show that
(i) \triangle APD\cong \triangle CQB
(ii) AP = CQ
(iii) \triangle AQB \cong \triangle CPD
(iv) AQ = CP
(v) APCQ is a parallelogram
ABC is a triangle right angled at C. A line through the mid-point M of hypotenuse AB and parallel to BC intersects AC and D. Show that
(i) D is the mid-point of AC
(ii) MB\perp AC
(iii) CM = MA = \dfrac {1}{2}AB
ABCD is a trapezium in which AB \parallel DC, BD is a diagonal and E is the mid-point of AD. A line is drawn through E parallel to AB, intersecting BC at F (see Fig). Show that F is the mid-point of BC.
Show that the diagonals of a parallelogram divide it into four triangles of equal area.
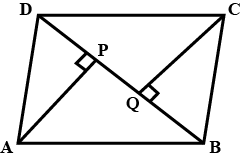
ABCD is a parallelogram and AP and CQ are perpendiculars from vertices A and C on diagonal BD Show that
i) \triangle\ APB \cong \triangle\ CQD
ii) AP = CQ
In a parallelogram ABCD,\ E and F are the mid-points of sides AB and CD respectively (see Fig). Show that the line segments AF and EC trisect the diagonal BD.
If E, F, G and H are respectively the mid-points of the sides of a parallelogram ABCD, show that ar(EFGH) = \dfrac {1}{2} ar (ABCD)
In the given Figure, ABCD is a parallelogram, AE\perp DC and CF \perp AD. If AB = 16\ cm, AE = 8\ cm and CF = 10\ cm, find AD.

Prove that the rhombus with equal diagonals is a square.
In the figure, suppose \angle P and \angle Q are supplementary angles and \angle R = 125^{\circ}. Find the measures of \angle S.

All rectangles are parallelograms, but all parallelograms are not rectangles. Justify this statements
Is parallelogram a rectangle? Can you call a rectangle a parallelogram?
Two angles of a quadrilateral are 70^{\circ} and 130^{\circ} and the other two angles are equal. Find the measure of these two angles.
Complete the following table by writing (YES) if the property holds for the particular Quadrilateral and (NO) if property does not holds.
| Properties | Trapezium | Parallelogram | Rhombus | Rectangle | Square |
| a. Consecutive angles are supplementary |
Complete the following table by writing (YES) if the property holds for the particular Quadrilateral and (NO) if the property does not hold.
| Properties | Trapezium | Parallelogram | Rhombus | Rectangle | Square |
| Diagonals are equal |
Suppose in a rectangle, the diagonals are perpendicular to each other. Prove that it is a square.
Complete the following table by writing (YES) if the property holds for the particular Quadrilateral and (NO) if property does not holds.
| Properties | Trapezium | Parallelogram | Rhombus | Rectangle | Square |
| a. Opposite angles are equal |
Complete the following table by writing (YES) if the property holds for the particular Quadrilateral and (NO) if property does not holds.
| Properties | Trapezium | Parallelogram | Rhombus | Rectangle | Square |
| a. All sides are equal |
Complete the following table by writing (YES) if the property holds for the particular Quadrilateral and (NO) if property does not holds.
| Properties | Trapezium | Parallelogram | Rhombus | Rectangle | Square |
| a. One pair of opposite sides are parallel |
Suppose in a quadrilateral, the diagonals bisect the angles at the vertices. Prove that it is a rhombus.
Complete the following table by writing (YES) if the property holds for the particular Quadrilateral and (NO) if property does not holds.
| Properties | Trapezium | Parallelogram | Rhombus | Rectangle | Square |
| a. Two pairs of opposite sides are parallel |
The midpoint of the side AB of a triangle ABC is D and P is any point on BC. Suppose Q is a point on AC such that ADPQ is a parallelogram. Prove that DQ is parallel to BC.
Complete the following table by writing (YES) if the property holds for the particular Quadrilateral and (NO) if property does not holds.
| Properties | Trapezium | Parallelogram | Rhombus | Rectangle | Square |
| a. Diagonals bisect each other |
ABCD is quadrilateral in which AB\parallel CD. If AD = BC, show that \angle A = \angle B and \angle C = \angle D
Three vertices of a parallelogram \text{ABCD} taken in order are \text{A}\left( 3,6 \right), \text{B}\left( 5,10 \right) and \text{C}\left( 3,2 \right) find :
(i) the coordinates of the fourth vertex \text{D}.
(ii) length of diagonal \text{BD}.
Show that the line segments joining the midpoints of the opposite sides of a quadrilateral and bisect each other
ABCD is a parallelogram AP and CQ are perpendicular drawn from vertices A and C on diagonal BD (see figure) show that
AP = CQ
The four angles of a quadrilateral are in the ratio 1 : 2 : 3 : 4. Find the measure of each angle of the quadrilateral.
Complete the following table by writing (YES) if the property holds for the particular Quadrilateral and (NO) if property does not holds.
| Quadrilateral name | Trapezium | Parallelogram | Rhombus | Rectangle | Square |
| Each angle is a right angle |
Complete the following table by writing (YES) if the property holds for the particular Quadrilateral and (NO) if property does not holds.
| Properties | Trapezium | Parallelogram | Rhombus | Rectangle | Square |
| Diagonals are perpendicular to each other |
The opposite angles of a parallelogram are (3x - 2)^{\circ} and (x + 48)^{\circ}. Find the measure of each angle of the parallelogram.
ABCD is a rectangle AC is diagonal. Find the angles of \triangle ACD. Give reasons
Prove that a line drawn through the mid-point of one side of a triangle parallel to second side bisects the third side.
In the given figure, AED is an isosceles triangle with AE = AD. ABCD is a parallelogram and EGF is a line segment. If \angle DCF = 65^{\circ} and \angle EFB = 100^{\circ}, then the number of diagonals of a regular polygon having its each exterior angle equal to the measure of \angle AEG, is
If the measures of three angles of a quadrilateral are 100^{\circ}, 84^{\circ} and 76^{\circ} then, find the measure of fourth angle.
In \Delta ABC, AD, is a median and E is the mid point of AD. If BE is produced to meet AC in F, show that AF=\dfrac { 1 }{ 3 } AC.
Find the four angles of a cyclic quadrilateral ABCD in which \angle A=(2x-1)^o, \angle B=(y+5)^o \angle C=(2y+15)^o and \angle D =(4x-7)^o.
Find the angles of a rhombus whose diagonal is equal to a side.
The perimeter of a rhombus is equal to 48, and the sum of the lengths of the diagonals is equal to 26. Find the area of the rhombus.
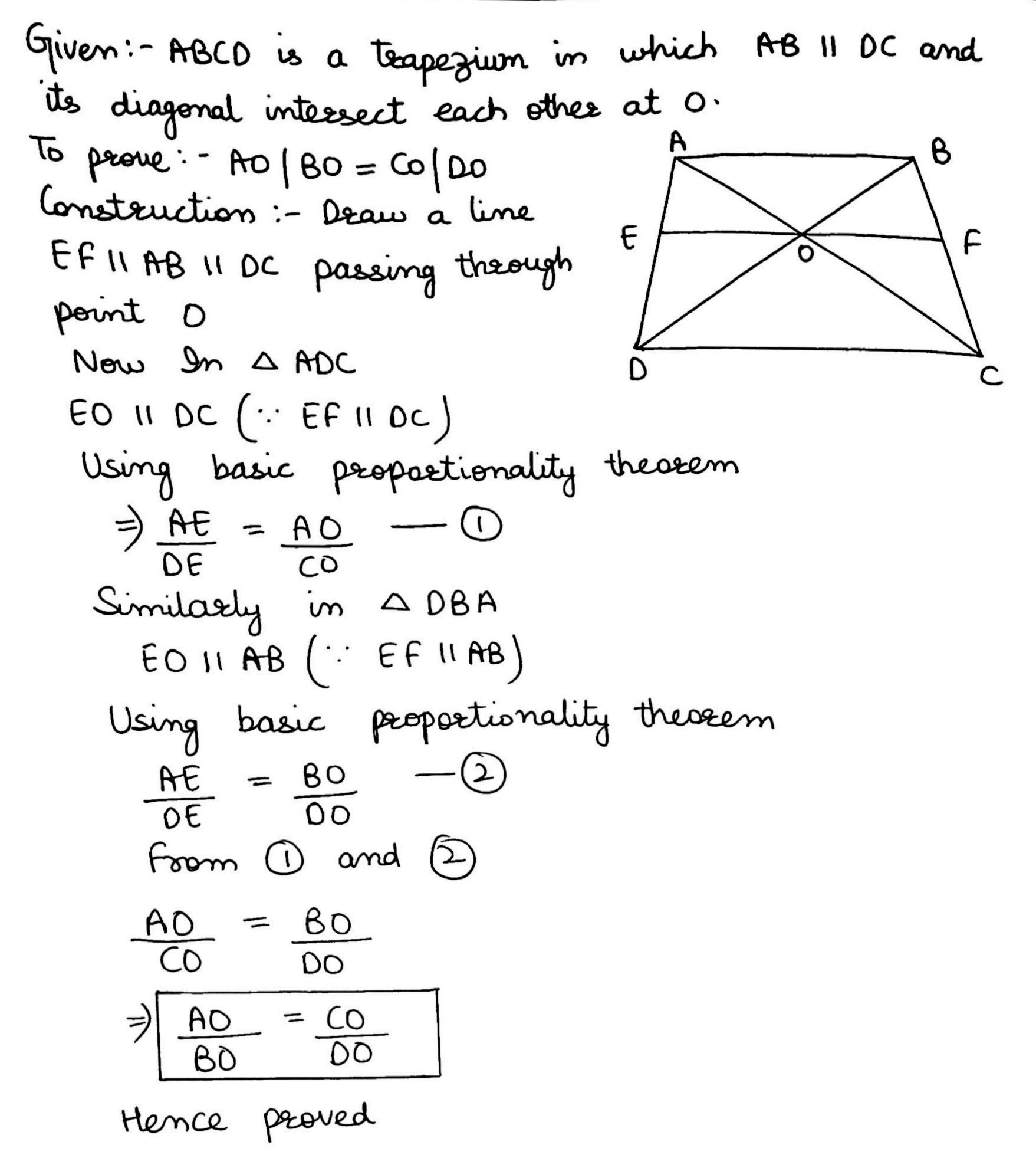
In trapezium ABCD, side AB \parallel side DC, diagonals AC and BD intersect in point O. If AB=20, DC=6, OB=15, then find OD.
If the area of a rectangle is 200 sq.m and the difference of its length and breadth is 10 cm, find its length, breadth and perimeter.
In the adjoining figure, ABCD is a square. A line segment CX cuts AB at X and the diagonal BD at O such that \angle COD = 80^0 and \angle OXA = x^0 . Find the value of x.

State and prove mid point theorem.
In the given figure, AB \parallel DC Prove that(i) \triangle DMU \sim \triangle BMV(ii) DM \times BV = BM \times DU
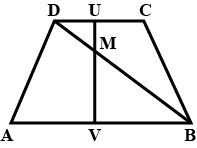
The diagonals of a quadrilateral intersect at right angles. Prove that the figure obtained by joining the mid-points of the adjacent sides of the quadrilateral is a rectangle.
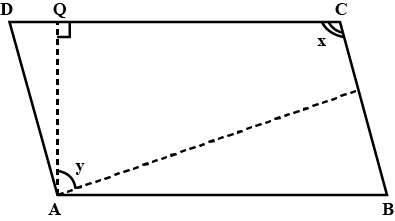
If OC and OD are the bisectors of \angle C and \angle D respectively and \angle A =115^{\circ}, find \angle B.
The following figure shows a trapezium ABCD in which AB is parallel to DC and AD=BC.Prove that :i)\; \;\;\angle DAB = \angle CBAii)\;\; \angle ADC = \angle BCDiii) \;AC=BDiv)\;\; OA=OB and OC=OD
ABCD is a rectangle in which diagonal AC bisects \angle A as well as\angle C. Show that,
(i) ABCD is square
(ii) diagonal BD bisects \angle B as well as \angle D.
(i) ABCD is square
(ii) diagonal BD bisects \angle B as well as \angle D.
Three angles of a quadrilateral are equal to {100^ \circ },{60^ \circ } and {80^ \circ }, respectively. Find its fourth angle.
Three angles of a quadrilateral ABCD are \angle A=70^o,\angle B=50^o and \angle C=82^o. Find \angle D.
In a quadrilateral ABCD, show that AB+BC+CD+DA>AC+BD.
In the adjacent figure the AB and AC of \triangle ABC are produced to point E and D respectively If bisectors BO and CO of \angle CBD and \angle BCD respectively meet at point O, then prove that \angle BOC=90^{o}-\dfrac {1}{2} \angle BAC.

Draw two angles of {90^ \circ } at the ends of a line segment. Extend these rays. Do you get a triangle. Join these 2 rays by another line segment which shape can yo get? What will be the measure of all the angles?
A quadrilateral ABCD is drawn to circumscribe a circle (see Fig). prove that AB+CD=AD+BC.
Angles of a quadrilateral are in the ratio 1: 2: 3: 4. Find all angles. What special name can you give to this quadrilateral and why?
ABCD is a quadrilateral in which AD=BC and \angle DAB=\angle CBA. Prove that \angleABD =\angleBAC.
The perimeter of a square is (4x+20)cm. What will be the length of its diagonal?
Find the measurements of the angles of quadrilateral if they are in ratio of 2:3:4:6
In \squarePQRS, m\angle Q is 10^o less, m\angle R is 20^o less and m\angle S is 30^o less, than m\angle P, then find measure of each angle.
In \Box ABCD, \bar{AB}\parallel\bar{CD}. Prove that AD=BC and AB=CD.
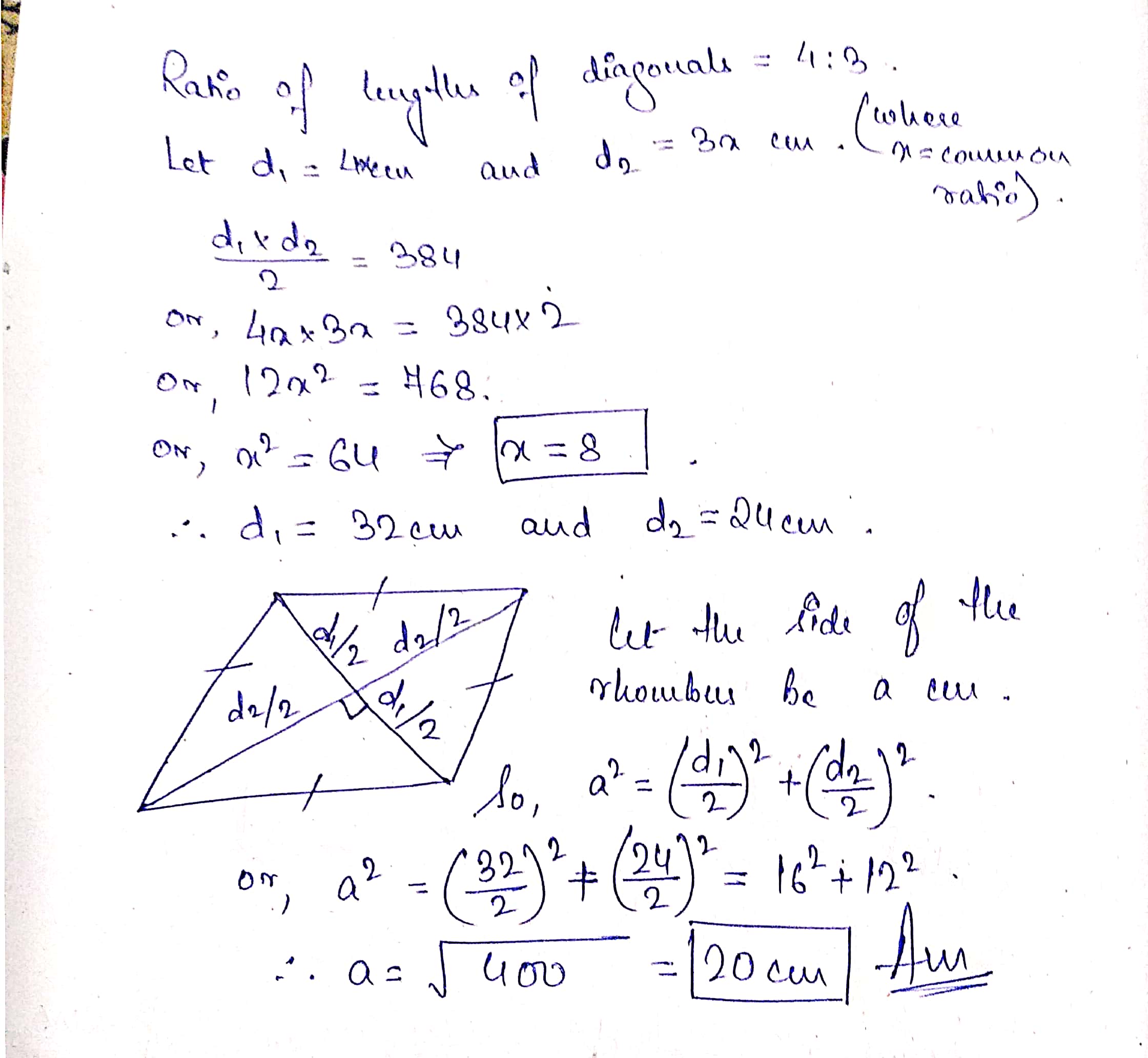
The diagonals of a rhombus ABCD are 6\ cm and 8\ cm. Find the length of a side of the rhombus.
The measure of three angles of a quadrilateral are equal. If measure of each angle is 95^\circ, then find measure of its fourth angle.
In the adjoining figure, D and E are respectively the midpoints of sides AB and AC of \triangle{ABC}. If PQ\parallel BC and CDP and BEQ are straight lines, then prove that ar(\triangle{ABQ})=ar(\triangle{ACP}).
In a quadrilateral ABCD. AO and BO are bisectors of angle A and angle B respectively. Prove that \angleAOB=\dfrac{1}{2}\{\angle C+\angle D\}.
In triangle ABC, XY || AC and divide the triangle into two parts of equal areas. Find the ratio \dfrac{AX}{AB}.
In a \Delta ABC, the internal bisectors of \angle B and \angle C meet at P and the external bisectors of \angle B and \angle C meets at Q. Prove that \angle BPC + \angle BQC = {180^0}.
The interior angles of a quadrilateral are in the ratio 3:5:6:4. Find the angles.
If BC is a diameter of a circle of circle of centre O and OD is perpendicular to the chord \overline { AB } of a circle then CA\equiv________
Two opposite angles A and C of a parallelogram ABCD are (6x-17)^{o} and (x+63)^{o} respectively as shown the figure. Find the measure of angle A.

In figure given, we have X and Y are the midpoints of AB and BC and AX=CY. Show that AB=BC.

Three angles of a quadrilateral are equal. The fourth angle measure {120^ \circ }. What is the measure of each of the equal angles?
In a quadrilateral ABCD, the bisector of \angleC and \angleD intersect at O. Prove that \angleCOD=\dfrac{1}{2}(\angle A+\angle B).
Show that the point A (3,5) B (6,0) C (1,-3) D (-2,2) are the vertex of the square ABCD.
From the given groups of angles which are possible group angles of a quadrilateral:i) 60^{\circ}, 70^{\circ}, 80^{\circ}, and 145^{\circ}ii) 90^{\circ}, 90^{\circ}, 90^{\circ}, and 90^{\circ}
Find the area of a rhombus if its vertices are (3, 0), (4, 5), (-1, 4) and (-2, -1) taken in order.
The sum of two angles of a quadrilateral is 150^{o} and the other angles are in the ratio 2:3. Find the measure of two angles.
In Fig ABCD is a rhombus in which \alpha ABC=56^{o}. Which is the measure of \angle ACD?
In the quadrilateral PQRS, the angles are in the ratio of 3: 4: 5: 6. Find the angles of PQRS. What is the special name given to PQRS?
Prove that each of the four sides of a rhombus is of the same length.
What is parallelogram ?
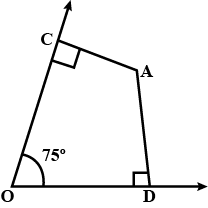
In the adjoining figure, A C \perp O C \text { and } A D \perp O D. Find \angle C A D
Show that the line segment joining the mid points of the opposite sides of a quadrilateral bisect each other.
Show that the points A(1,2),B(5,4),C(3,8) and D(-1,6) are the vertices of a square.
ABCD is a rectangle in which diagonal AC bisects \angle \mathrm { A } as well as \angle \mathrm { C } . Show that
(i) ABCD is a square
(ii) diagonal BD bisects \angle \mathrm { B } as well as \angle \mathrm { D }.
If the diagonals of a quadrilateral bisect each other, then prove that it is a parallelogram.
E and F are points on diagonals AC of a parallelogram ABCD such that AE=CF. Show that BFDE is a parallelogram.
The four angles of a quadrilateral are equal. Draw this quadrilateral in your notebook. Find each of them.
In a quadrilateral, the angles are x^{o},(x+10)^{o},(x+20)^{o},(x+30)^{o}. Find the angles.
Construct Rhombus MATH with \mathrm { AT } = 4 \mathrm { cm } , \angle \mathrm { MAT } = 120 ^ { \circ }
If A , B . C are ( 3,3,3 ) , ( 0,6,3 ) and ( 1,7,7 ) respectively. find D ( x , y , z ) such that ABCD is a square
ABCD is a trapezium is which AB\parallel DC, BD is a diagonal and E is the midpoint of AD. A lie is drawn through E parallel. AB IN intersecting BC at F. show that F is the midpoint of BC
If ABCD is a rohmbus with \angle ABC={ 56 }^{ 0 } Find the measure of \angle ADC
Prove that, the sum of either pair of opposite angles of a cyclic quadrilateral is 180.
In \triangle PQR,point M is on side PQ and point S is on the side PR such that QRSM is what kind of figure?
Find the lengths of diagonals AC and BD. Given AB = 60\ cm and \angle BAD = 60^{\circ}.
In the given figure, ABCD and PQRB are rectangles where Q is the mid point of BD. If QR=5cm, then find the length AB.

AB and CD bisect each other of K.
Prove that AC =BD
The angles of quadrilateral are in the ratio 3:5:9:13. Find all the angles of the quadrilateral.
Show that the line segments joining the mid-points of the opposite sides of a quadrilateral bisect each other.

If one side of a square is represented by 4x-7 and the adjacent side is represented by 3x+5, find the value of x.
The four angles of a quadrilateral are in the ratio 1 : 2 : 3 : 4.Find the measure of each angle of the quadrilateral.
Fill in the blanks
If three acute angles of a quadrilateral measure 70^0 each, then the measure of the fourth angle is ____ .
Prove that the sum of all the angle of a quadrilateral is 360^\circ.
One single of quadrilateral it of { 108 }^{ \circ } and the remaining three angles are equal. Find each of the three equal angles.
In the adjoining figure PQRS is a rectangle. Identify the congruent triangles formed by the diagonals.
The angles of a quadrilateral are in the ratio 2 : 4 : 5 : 7. Find the angles.
Show that the line segments joining the mid-points of the opposite side of a quadrilateral bisect each other.
In the given figure, ABCD is a cyclic quadrilateral , whose diagonals intersect at E such that \angle BAC= 30^{0} , \angle DBC= 70^{0} and AB=BC,Find \angle ACD.
Find the unknown angle.

Show that the four triangles as shown in the adjoining fig. formed by diagonals and sides of rhombus are congruent.
In the given figure, AC is the bisector of \angle A. If AB=AC, AD=CD and \angle ABC=75^{o}, find the value of x and y.
In a trapezium ABCD with AB parallel to CD, the diagonals intersect at P. The area of \triangle A B P is 72\mathrm { cm } ^ { 2 } and of \Delta C D P is 50\mathrm { cm } ^ { 2 } . Find the area of the trapezium.
If A B C D is a parallelogram, A E \perp D C and C F \perp A D . If A B = 16 \mathrm { cm }, A E = 8 cm and C F = 10 \mathrm { cm } , find A D .
In parallelogram A B C D, \angle D = 115 ^ \circ , find the remaining angles .
ABCD is a quadrilateral. AO and BO are the angle bisectors of angles A and B which meet at O. If \angle C=70^{o},\ \angle D=50^{o}, find \angle AOB.
Find the unknown angle.
A photo frame is in the shape of a quadrilateral. With one diagonal longer than the other. Is it a rectangle ? why or why not ?
If the angles of a quadrilateral are in the ratio 1:2:3:4 find the angles
Two angles of a quadrilateral are each of measure 75^o and the other two angles are equal. What is the measure of these two angles ? Name the possible figure so formed.
Construct a parallelogram ABCD such that BC=4.5\ cm,\ BD=4\ cm and AC=5.6\ cm
11 gm MNPQ, MP=3.8 cm , NQ=4.5 cm and angle between MP and NQ is 60^{\circ}.
Prove that the points A(2, 3), B(-2, 2), C(-1, -2) and D(3, -1) are the vertices of a square ABCD.
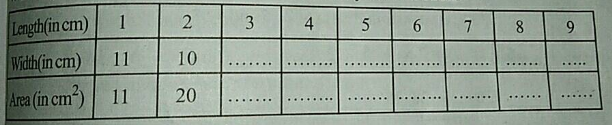
Given a rectangle with a fixed perimeter of 24 meters, if we increase the length by 1\ \text{m}, then the width and area will vary accordingly. Use the following table of values to look at how the width and area vary as the length varies.
What do you observe? Write your observations in your notebooks.
The dimensions of a rectangular room (cubiodal) are l, b and h. What is the area of its four walls?
If D,\ E,\ F are the mid-points of the sides BC,\ CA and AB respectively of \triangle ABC, prove that BDEF is a parallelogram. And also show that ar(\triangle DEF)=\dfrac {1}{4}ar(\triangle ABC).
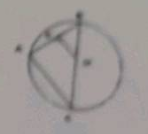
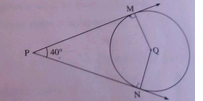
i\\In the figure, Q is the centre of the circle
and PM and PM are tangents to the circle.
If \angle MPN = {40^ \circ }. find \angle MQN.
If three angles of quadrilateral are each equal to 75 ^ { \circ } , the fourth angle is
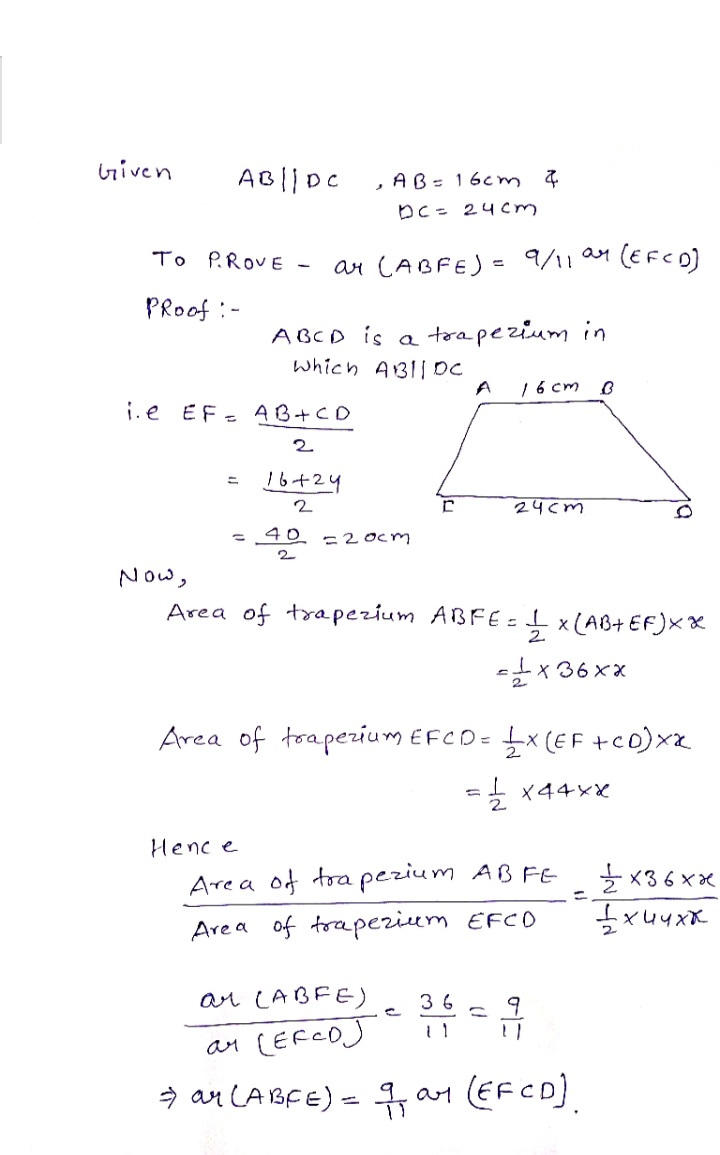
ABCD is a trapezium \overline { A B } \| \overline { C D } If A B = 20 \mathrm { cm }\mathrm { BC } = 8 \mathrm { cm } , \mathrm { CD } = 10 \mathrm { cm } and \mathrm { AD } = 6 \mathrm { cm } . Find the area of \mathrm { ABCD } .
In the adjoining figure , ABCD is a parallelogram. Any line through A cuts DC at a point P and B C produced at Q . Prove that : ar ( \Delta B P C ) = ar (\Delta DPQ).
Diagonals AC and BD of a trapezium ABCD with AB \parallel DC intersect each other at O. Prove that ar ( A O D ) = a r ( B O C )
The angles of quadrilateral are x,80^{\circ},120^{\circ},100^{\circ} find x
Find the value of x in given figure.

Prove that in a parallelogram the opposite angles are equal .
PQRS is a square. Determine \angle SRP.
In the adjoining fig. 10.33 , A B C D is a rhombus.
Find the measure of the following angles, if \angle A C B = 30 ^ { \circ }
(a) \quad \angle B O C
(b) \angle C B O
(c) \quad \angle O A D
(d) \quad \angle A B O
Show that if the diagonals of a quadrilateral are equal and bisect each other at right angle, then it is a square.
Two opposite angles of a parallelogram are (3x-2)^{0} and (50-x)^{0}. Find x
Find the area of the quadrilateral ABCD in which AB= 3 cm, BC= 4 cm,CD= 4 cm , DA=5 cm and AC= 5 cm
The bisectors of \angle B and \angle C of quadrilateral ABCD meet at a point R inside the quadrilateral.If \angle A = 95 ^ { \circ } and \angle D = 111 ^0, find \angle B R C
The angles of a quadrilateral are in the ratio 2:3:4:Find the measure of the smallest and greatest angle.
If the mid points of the sides of a quadrilateral are joined in order, prove that the area of the parallelogram so formed will be half of the area of the given quadrilateral.
If E,F,G and H are respectively the mid-points of he sides of a parallelogram ABCD, show that
ar ( \mathrm { EFGH } ) = \dfrac { 1 } { 2 } ar ( \mathrm { ABCD } )
Diagonal AC of a parallelogram ABCD bisects \angle AShow that:
(i) it bisects \angle C also,
(ii) ABCD is a rhombus
(ii) ABCD is a rhombus
Draw a quadrilateral A B C D. Measure the four angles \angle A , \angle B , \angle C and \angle D. Verify whether their sum is 360 ^ { \circ }.
In a quadrilateral ABCD, \angle A=60^{o},\angle B=105^{o} and \angle D=50^{o}. Find \angle C.
Can all the four angles of a quadrilateral be obtuse (or) acute angles? For reason for your answer.
In given figure, P and Q are points on the sides AB and AC respectively of \triangle ABC such that AP=3.5\ cm,PB=7\ cm,AQ=3\ cm and QC=6\ cm. If PQ=4.5\ cm, find BC.

A field in the form of parallelogram has one of its diagonals 42\ m and the perpendicular distance of this diagonals from either of the outlying vertices in 10\ m\ 8\ dm. Find the area of the field.
A quadrilateral has three actute angles each measuring { 70 }^{ 0 }, what is the measure of the fourth angle?
ABCD is trapezium in which AB \parallel DC, AB=78\ cm, CD = 52\ cm, AD=28\ cm and BC=30\ cm. Find the area of the trapezium.
One side of a parallelogram is \dfrac{3}{4} times its adjacent side. If the perimeter of the parallelogram is 70\ cm, find the sides of the parallelogram .
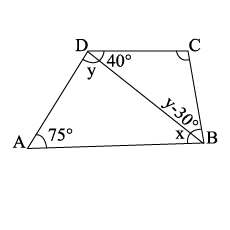
In the given fig. AB||CD,\angle BDC=40^{o}, and \angle BAD=75^{o}. Find x,y,z.
Two angles of a quadrilateral measure 80^{o} each. The other two differ by 20^{o}. What is the measure of each of the other angles.
In a parallelogram if the area, base & corresponding altitude are y^{2}, y-3, y+4. Then find the area of parallelogram in square unit.
In quadrilateral A B C D , \angle A + \angle D = 180 ^ { \circ } . What special name can be given to this quadrilateral?
Prove that the line segments joining the mid points of the opposite sides of any quadrilateral bisect each other.
In a Quadrilateral, the angles are {x^0},{(x + 10)^0},\,{(x + 20)^0},\,{(x + 30)^{0.}} Find the angles.
The ratio of two adjacent sides of a parallelogram is 2:3. Its perimeter is 50\ cm. Find its area if altitude corresponding to large side is 10\ cm.
Prove that the points A(2, 3), B(-2, 2), C(-1, -2) and D(3, -1) are the vertices square ABCD.
ABCD is a parallelogram, AE is perpendicular to DC, If DC=20\ cm, AD=5\ cm and the area of the parallelogram is 40\ cm^{2}. Find DE.
The perimeter of a parallelogram is 156\ cm. If one side measures 36\ cm, find the measure of its adjacent side.
If the diagonals of a quadrilateral bisect each other then quadrilateral is a parallelograms. Prove it
Can the angles 110 ^ { 0 } , 80 ^ { \circ } , 70 ^ { \circ } and 95 ^ { \circ } be the angles of a quadrilateral? Give your reasons.
In the figure, \Box ABCD is a parallelogram. AB=8, BC=12 and \angle B=30^{o}. Find A(\Box ABCD).
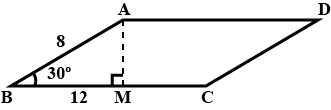
Show that if the diagonal of a quadrilateral interest each other at right angle, then the sum of square of opposite sides are equal;.
ABCD is a cyclic quadrilateral. If \angle{BAC}={50}^{\circ} and \angle{DBC}={60}^{\circ} then find \angle{BCD}
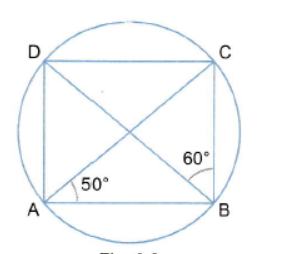
ABCD is a parallelogram and AP and CQ are perpendiculars from vertices A and C on diagonal BD.Show that AP = CQ
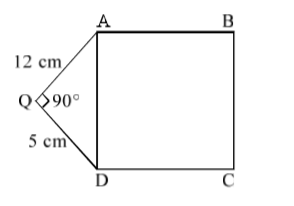
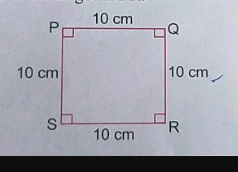
What is the area of the square ABCD shown in the diagram?
If the angles of quadrilateral are in the ratio 3:5:9:13, find the value of smallest angle of quadrilateral.
Angles of a quadrilateral are (4x)^{\circ},\ 5(x + 2)^{\circ},\ (7x - 20)^{\circ} and 6(x + 3)^{\circ}.
(i) Find the value of x (in degrees).
(ii) Each angle of the quadrilateral
ABCD is a parallelogram and AP and CQ are perpendiculars from vertices A and C on diagonal BD.Show that \triangle{APB}\cong \triangle{CQD}
The parallel sides of a trapezium are 28 cm and 21 cm and each of the non-parallel sides is 17 cm. Find the area of the trapezium.
The diagonal of a rhombus are 15cm and 18cm. Find its area and side.
In a quadrilateral ABCD, AB \parallel CD . If \angle A : \angle D = 2:3 and \angle B : \angle C = 7:8 , find the measure of each angle.
Prove that any line parallel to the parallel sides of a trapezium divides the non-parallel sides proportionally.
In the figure ABCD is a rectangle and DEC is an equilateral. Find \angle DAE.
The given fig is drawn using more than one triangle:
\angle D A B + \angle A B C + \angle B C D + \angle C D A

Find x+y+z+w, in the given figure.
The angles of a quadrilateral are (x+10), (2x+5), (2x-20) & (2x-5), then find their measures.
Find the measure of the fourth angle of the quadrilateral if the three interior angles 48^{o},\ 85^{o} and 140^{o}.
Find the four angles of equilateral, if the angles are in the ratios:
5:7:11:13
Find the angle measure x in the following figures.
If D,E,F are midpoint of the sides BC, CA an AB of triangle ABC, then show that BDEF is a parallelogram
Find the four angles of quadrilateral, if the angles are in the ratios:
2:3:4:6
ABCD is equilateral \triangle BEC is rectangle to BD cross ED at 'O' point \angle BOC=?
The angles in Quadrilateral are 105,35,45,x find x
In figure, ABCD is a parallelogram
Prove that \dfrac{DQ}{DP}=\dfrac{AQ}{DC}
In the figure given above, ABCD is a cyclic quadrilateral in which \angle BAD=75^{\circ},\; \angle ABD=58^{\circ} and \angle ADC=77^{\circ}. Find the measure of \angle CBD.
Do 120,45,64,76 form Quadrilateral .give reasons.
Find the measure of angles x,y,z and a in the following parallelograms .
Is it possible to have a quadrilateral whose angles are of measures 105, 165, 55 and 45 . Give reason ?
From the following figure find ,
(i) x
(ii) \angle ABC
(iii) \angle ACD
A B C D is a rhombus in which diagonals A C and B D intersect each other at O . If \angle A C D = 55 ^ { \circ } find \angle B D C
In the figure, given below, ABCD is a cyclic quadrilateral in which \angle BAD=75^{o}; \angle ABD=58^{o} and \angle ADC=77^{o}. Find: 1)\angle CBD 2)\angle BCD
Two adjacent angles of a parallelogram are in the ratio 1:5. Find all the angles of the parallelogram.
Two opposite angles of a parallelogram are {( 3x-2)}^{\circ} and {(50-x)}^{\circ}.find the measure of each angle of the parallelogram.
\ln a \Delta A B C, P and Q are points on sides A B and A C respectively, such that PQ \|\mathrm{BC} . If A P=2.4 \mathrm{cm}, A Q=2 \mathrm{cm}, Q C=3 cm and B C=10\mathrm{cm}, find A B and P Q
Find the area of a square whose perimeter is 160 \mathrm{m} . A floor 3.0 \mathrm{m} long and 2.0 \mathrm{m} wide is to be covered with square shaped tiles of length 10 \mathrm{cm}. Find the cost of flooring it, if the cost of tiles is Rs. 150 per 100 tiles.
In the figure, PQRS is a parallelogram with PQ = 16 cm and QR = 10cm, L is a point on PR such that RL: LP = 2: 3. QL produced meets RS at M and PS produced at N.Find the lengths of PN and RM
The angles of a quadrilateral are in the ratio 3:5:9:13. Find all the angles of the quadrilateral.
A square PQRS is of side 10\, \text{cm} each. Write the length of its diagonal PR.
All the angles of a quadrilateral are equal. What special name is given to this quadrilateral?
In the given fig. XY\parallel BC,\,\,BE\parallel CA\,\,and\,\,FC\parallel AB.
Prove that ar\left( {\Delta ABE} \right) = ar\left( {\Delta ACF} \right).
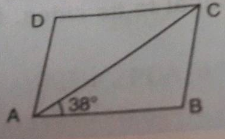
In given figure ABCD is a rhombus. If \angle BAC=38^{o}, find:
\angle DAC
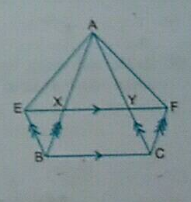
In the adjoining figure, ABC is triangle. Through A, B and C lines are drawn parallel to BC, CA and AB respectively, which forms a \Delta PQR. Show that 2\left( {AB + BC + CA} \right) = PQ + QR + RP.
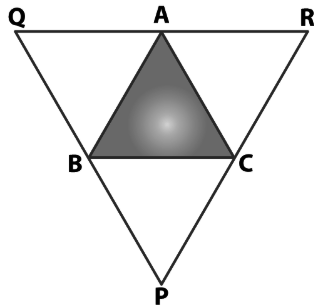
If two sides of a cyclic quadrilateral are parallel, prove that the remaining two sides equal and the diagonals are also equal.
In given figure ABCD is a rhombus. If \angle BAC=38^{o}, find:
\angle ADC
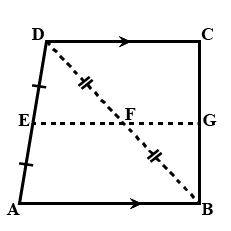
In the quadrilateral (1) given below, AB||DC, E and F are mid point of AD and BD respectively. Prove that G is mid point of BC.
Show that opposite angles of parallelogram are equal.
ABCD is a quadrilateral in which \overline {AB} = \overline {CD} and \overline {AD} = \overline {BC} . Show that it is a parallelogram.
In given figure ABCD is a rhombus. If \angle BAC=38^{o}, find:
\angle ACB
Prove that opposite sides of a quadrilatral circumscribing a ciecle subtend supplementary angle at the centre of the circle.
In the quadrilateral given below, AB||DC, E and F are mid point of of non-parallel sides AD and BC respectively. Calculate EF if AB=6 \text{ cm} and DC=4 \text{ cm}
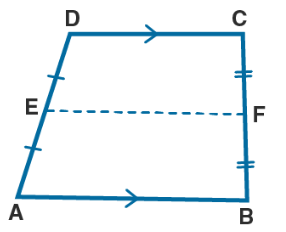
In the quadrilateral (1) given below, AB||DC, E and F are mid point of AD and BD respectively. Prove that
EG=\dfrac{1}{2}(AB+DC)
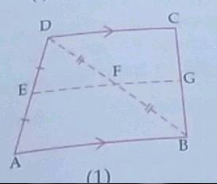
In the quadrilateral given below, AB||DC, E and F are mid point of of non-parallel sides AD and BC respectively. Calculate AB, if DC=8 cm and EF=9 cm.

Show that the diagonals of a parallelogram divide it into four triangles of equal area.
A B C D is a quadrilateral.
Prove that ( A B + B C + C D + D A ) > ( A C + B D )
In parallelogram ABCD,E is the mid-point of AD and F is the mid-point of BC. Prove that BFDE is a parallelogram.
If the points A(1,-2),B(2,3),C(a,2) and D(-4,-3) from a parallelogram. Find the value of 'a' and height of the parallelogram taking AB as base.
Let ABCD be a rectangle and let P,Q,R,S be the mid-points of AB, BC, CD,DA respectively. Prove that PQRS is a rhombus.
The length of a diagonals of square is \sqrt {2}(6+2\sqrt {5})cm. Then finds the length of sides of the square.
The three angles of a quadrilateral are { 80 }^{ \circ }, { 70 }^{ \circ }and { 120 }^{ \circ }. The fourth angle is.
ABCD is a quadrilateral in which P, Q, R, and S are mid-points of the sides AB, BC, CD, and DA. AC is a diagonal. Show that: PQ=SR
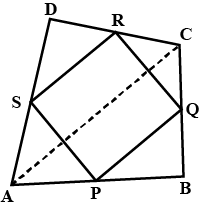
In parallelogram PQRS, \angle Q=\left( 4x-5 \right) ^{ \circ } and \angle S=\left( 3x-10 \right) ^{ \circ }. Calculate : \angle Q and \angle R .
ABCD is a quadrilateral in which P, Q, R, and S are mid-points of the sides AB, BC, CD, and DA. AC is a diagonal. Show that: PQRS is a parallelogram.
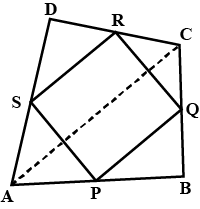
Check whether the points A \left(2,-1\right), B\left(3,4\right), C\left(-2,3\right) and D\left(-3,2\right) represents a Rhombus
E is the mid-point of the median AD of \Delta ABC and BE is produced to meet AC at F .Show that AF\frac{1}{3}AC.
In a right angled \Delta BAC,\angle BAC={ 90 }^{ 0 } segment AD, BE and CF are the medians
Prove that 2\left( { AD }^{ 2 }+{ BE }^{ 2 }+{ CF }^{ 2 } \right) ={ 3BC }^{ 2 }
ABCD is a quadrilateral with \angle{A}={80}^{\circ}, \angle{B}={40}^{\circ}, \angle{C}={140}^{\circ},\angle{D}={100}^{\circ}.Is ABCD is a trapezium?Justify your answer.
In a quadrilateral , the angle are x^{0},(x+10)^{o},(x+20)^o,(x+30)^o.Find the angles
D,E and F are respectively the mid points of the sides AB,BC and CA, respectively of a \Delta ABC. Prove that by joining these mid-points D,E and F the \Delta ABC is divided into four congruent triangle
The angles of the quadrilateral are in the ratio 3:5:9:Find all the angles of the quadrilateral.
If two angles of a quadrilateral are 40^{\circ} and 110^{\circ} and the other two are in the ratio 3:4, find these angles.
The angles of a quadrilateral are 2x,\; 2x+15,\; 4x-12 and 3x+5. Find the value of x and all angles of the quadrilateral.
P,Q and R are the mid points of sides BC,CA, and AB of ABC, and AD. is . the perpendicular from A to BC. prove that P,Q,R and D are concyclic.
In figure, ABCD is a cyclic quadrilateral. If \angleBAC=50^o and \angleDBC=60^o, then find \angleBCD.

The ratio between three angles of a quadrilateral is 7:11:13 respectively, the value of the fourth angle of the quadrilateral is 112^o. what is the difference between the largest and smallest angles of the quadrilateral ?
Show that line segment joining the midpoints of the opposite sides of a quadrilateral bisects each other.
ABCD is a quadrilateral. Is AB + BC + CD + DA > AC + BD?
The diagram shows a quadrilateral on a 1\ cm^{2} grid.
Write down the mathematical name of this quadrilateral.

If following statement is true enter 1 else enter 0.The opposite sides of a quadrilateral are parallel. If one angle of the quadrilateral is 60^{\circ}, then the other angles are 120^{\circ},60^{\circ},120^{\circ} .
Give reasons for the following.
A rectangle can be thought of as a special parallelogram.
Find the missing values:
| S.No. | Base | Height | Area of the parallelogram |
| a. | 20\ cm | 246 cm^2 | |
| b. | 15\ cm | 154.5 cm^2 | |
| c. | 8.4\ cm | 48.72 cm^2 | |
| d. | 15.6\ cm | 16.38 cm^2 |
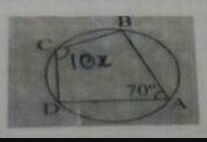
in a given figure a circle passes through points A,B,C and D. \angle BAD=70 ^{\circ} ,find x
ABCD is quadrilateral. Is AB+BC+CD+DA<2(AC+BD)?
Give reasons for the following.
A square can be thought of as a special rectangle.
A quadrilateral has three acute angles each measure {80}^{o}. What is the measure of the fourth angle?
A quadrilateral has all its four angles of the same measure. What is the measure of each?
In a quadrilateral define the following:
Adjacent sides
The angles of a quadrilateral are {110}^{o},{72}^{o},{55}^{o} and {x}^{o}. Find the value of x.
Two angles of a quadrilateral are of the measure {65}^{o} and the other two angles are equal. What is the measure of each of these two angles?
The four angles of a quadrilateral are as 3:5:7:9. Find the angles.
In a quadrailateral define the following:
Diagonals
Three angles of a quadrilateral are equal. The fourth angle is of measure {150}^{o}. What is the measure(in degree) of equal angles?
In a quadrilateral define the following:
Adjacent angles
The three angles of a quadrilateral are respectively equal to {110}^{o},{50}^{o} and {40}^{o}. Find its fourth angle. (in degrees)
The angle between the altitudes of a parallelogram, through the same vertex of an obtuse angle of the parallelogram is 60^{\circ}. Find the angles of the parallelogram.
In the figure, find the measure of \angle MPN.

In a quadrilateral ABCD, the angles A,B,C,D are in the ratio 1:2:4:5. Find the measure of each angle of the quadrilateral.
State the midpoint theorem.
In a quadrilateral, ABCD, CO and DO are the bisectors of \angle C and \angle D respectively. Prove that \angle COD=\cfrac{1}{2}(\angle A+\angle B)
In the figure, ABCD is a trapezium in which AB\parallel DC. If \angle A={55}^{o} and \angle B={70}^{o}, find \angle C and \angle D.
In the adjoining figure OD is perpendicular to the chord AB of a circle with centre O. If BC is a diameter, show that AC\parallel DO and AC=2\times OD

Three angles of a quadrilateral are {75}^{o},{90}^{o} and{75}^{o}. Find the measure of the fourth angle.
In figure, AB || CD. Find the values of x, y, z.

If the sum of the two angles of a quadrilateral is {180}^{o}. What is the sum of the remaining two angles in degree?
In a trapezium ABCD, AB || DC, AB=a\ cm, and DC=b\ cm. If M and N are the midpoint of the nonparallel sides, AD and BC respectively then find the ratio of ar(DCNM) and ar(MNBA).

P, Q, R, S are respectively the midpoints of the sides AB, BC, CD and DA of parallelogram ABCD. Show that PQRS is a parallelogram and also show that ar (||gm PQRS)=\dfrac{1}{2} \times ar(||gm ABCD).
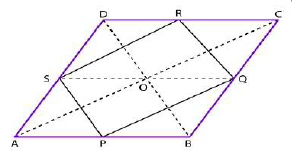
In the adjoining figure, D and E are respectively the mid point of sides AB and AC of \triangle ABC. If PQ ||BC and CDP and BEQ are straight lines then prove that ar(\triangle ABQ)=ar (\triangle ACP).
In the figure, ABCD is a parallelogram in which \angle A={72}^{o}. Calculate \angle B,\angle C and \angle D.
Prove that the sum of all the angles of a quadrilateral is {360}^{o}.
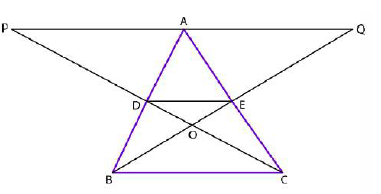
In the figure, ABCD is a parallelogram in which \angle DAB={80}^{o} and \angle DBC={60}^{o}
P,Q,R and S are respectively the midpoints of the sides AB,BC,CD and DA of a quadrilateral ABCD. Show that PQ\parallel SR
Prove that the line segments joining the middle points of the sides of a triangle divide it into four congruent triangles.
In the figure, \text{D, E} and \text{F} are the midpoints of the sides \text{BC, CA} and \text{AB} respectively, of \triangle \text{ABC}. Show that \angle \text{EDF}=\angle \text{A}, \angle \text{DEF}=\angle \text{B} and \angle \text{DFE}=\angle \text{C}
D is the midpoint of BC of \triangle ABC and E is the midpoint of BD. If O is the midpoint of AE, prove that ar(\triangle BOE)=\dfrac{1}{8} ar(\triangle ABC).
In the figure given below, ABCD is a parallelogram. E is a point on AB, CE intersects the diagonal BD at O and EF \parallel BC. If AE : EB = 2 : 3, find
(i) EF : AD
(ii) area of \triangle BEF : area of \triangle ABD
(iii) area of \triangle ABD : area of trapezium AFED
(iv) area of \triangle FEO : area of \triangle OBC.
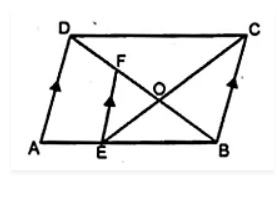
Show that the quadrilateral formed by joining the midpoints of the pairs of adjacent sides of a square is a square.
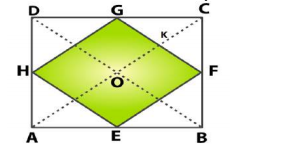
Prove that the line segments joining the midpoints of opposite sides of a quadrilateral bisect each other.
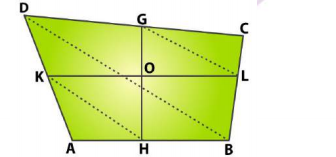
The diagonals of a quadrilateral ABCE are equal. Prove that the quadrilateral formed by joining the midpoints of its sides is a rhombus.
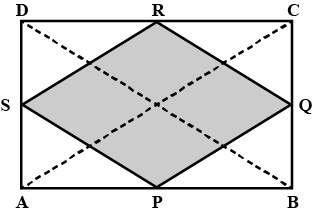
Prove that the lines joining the middle points of opposite sides of a quadrilateral and the line joining the middle points of its diagonals meet in a point and bisect one another.
In Figure ,\dfrac{A O}{O C}=\dfrac{B O}{O D}=\dfrac{1}{2} and A B=5 \ {cm} . Find the value of DC.
The diagonals of a quadrilateral ABCD are perpendicular to each other. Prove that the quadrilateral formed by joining the midpoints of its sides is a rectangle.
Fill in the blanks to make the statements true.
The sum of all _______ of a quadrilateral is 360^{\circ} .
Fill in the blanks to make the statements true.
In quadrilateral HOPE , the pairs of opposite sides are _________
Fill in the blanks to make the statements true.
A quadrilateral in which a pair of opposite sides is parallel is ________ .
Fill in the blanks to make the statements true.
In quadrilateral WXYZ , the pairs of opposite angles are _________.
Fill in the blanks to make the statements true.
If all sides of a quadrilateral are equal , it is a _______ .
Fill in the blanks to make the statements true.
________ is a regular quadrilateral.
Solve the following :
Two sticks each of length 7 \,cm are crossing each other such that they bisect each other at right angles. What shape is formed by joining their end points ? Give reason .
A line l is parallel to line m and a transversal p intersects them at X , Y respectively . Bisectors of interior angles at X and Y intersect at P and Q . Is PXQY a rectangle ? Give reason.
Fill in the blanks to make the statement true.
If the diagonals of a quadrilateral bisect each other , it is a __________.
Fill in the blanks to make the statements true.
If only one diagonal of a quadrilateral bisects the other , then the quadrilateral is known as ________
ABC and BDE are two equilateral triangles such that D is the mid-point of BC. Then
ar(\Delta BDE) = \dfrac{1}{4} ar (\Delta ABC). Write True or False and justify your answer:
In the given figure, ABCD and EFGD are two parallelogram and G is the mid-point of CD. Then ar(\Delta DPC) = \dfrac{1}{2} ar (\parallel EFGD). Write True or False and justify your answer:
Find the angle measure x in the following figures:
Find the angle measure x in the following figures:
In trapezium ABCD, AB \parallel DC and L is the mid-point of BC. Through L, a line PQ \parallel AD has been drawn which meets AB is P and DC produced in Q (Fig. 9.18). Prove that ar (ABCD) = ar(APQD)

Diagonals of a quadrilateral ABCD bisect each other. If \angle A = 35^\circ, determine \angle B.
Can all the angles of a quadrilateral be acute angles ? Give reason for your answer.
Can all the angles of a quadrilateral be right angles ? Give reason for your answer.
Find the angle measure x in the following figures:
What type of quadrilateral do the points A(2 , -2) , B (7 , 3) , C(11 , -1) and D(6 , -6) taken in that order form?
Consider the following parallelograms. Find the values of x, y, z in each.
Consider the following parallelograms. Find the values of x, y, z in each.

Name the quadrilateral whose diagonals are perpendicular bisectors of each other.
In the following figures, HOPE is parallelograms. Find the measures of angles \mathrm{x}, \mathrm{y} and \mathrm{z} . State the properties you use to find them.
Consider the following parallelograms. Find the values of x, y, z in each.
ABCD is a rhombus with P, Q and R as midpoints of AB, BC and CD respectively. Prove that PQ \bot QR.
In the given figure, find x+y+z+w.
Name the quadrilateral whose diagonals bisect each other.
Name the quadrilateral whose diagonals are equal
Consider the following parallelograms. Find the values of x, y, z in each.

In the figure given, ABCD is a kite in which BC = CD, AB =AD. And E,\ F,\ G are midpoints of CD,\ BC and AB. Prove that the line drawn through G and parallel to FE bisects DA.

In the adjoining figure, the lines l,\ m, and n are parallel to each other, and G is mid-point of CD. Calculate: CF if GE=2.3cm

In the adjoining figure, the lines l,\ m, and n are parallel to each other, and G is mid-point of CD. Calculate:BG if AD=6cm
In the given figure, ABCD is a parallelogram. If P and Q are mid-points of sides CD and BC respectively, show that CR=\dfrac14 AC.
In given figure ABCD is a quadrilateral in which P, Q, R and S are mid-points of the sides. AB, BC, CD and DA. AC is a diagonal. Show that: PQ = SR
The diagonal AC and BD of a parallelogram ABCD intersect at O. If P is the midpoint of AD, prove that PO=\dfrac12\ CD
The diagonal AC and BD of a parallelogram ABCD intersect at O. If P is the midpoint of AD, prove that PQ \parallel AB
In the given figure, ABCD is a parallelogram and E is mid-points of AD. DL \parallel EB produced at F. Prove that B is mid-points of AF and EB=LF.
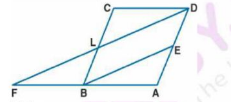
In the figure given ABCD is a parallelogram. E and F are mid-point of the sides AB and CO respectively. The straight lines AF and BF meet the straight lines ED and EC in points G and H respectively. Prove that GEHF is a parallelogram.
In given figure ABCD is a quadrilateral in which P, Q, R and S are mid-points of the sides. AB, BC, CD and DA. AC is a diagonal. Show that: PQRS is a parallelogram

In the given figure, D, E and F are mid points of the sides BC, CA and AB respectively of \Delta \mathrm{ABC} . Prove that BCEF is a trapezium and area of trap. BCEF =3 / 4 area of \Delta \mathrm{ABC} .
In the adjoining figure, the lines l,\ m, and n are parallel to each other, and G is mid-point of CD. Calculate: AB if BC=2.4cm
Find the size of each lettered angle in the above figures.
In the given figure, ABC is an isosceles triangle in which AB = AC. AD bisects exterior angle PAC and CD \, || \, BA. Show that
(i) \angle DAC=\angle BCA
(ii) ABCD is a parallelogram.
(i) \angle DAC=\angle BCA
(ii) ABCD is a parallelogram.
In the figure ( 2 ) given below, A B C is right-angled triangle at A. AGFB is a square on the side A B and B C D E is a square on the hypotenuse BC. If A N \perp ED, prove that:
(i) \Delta \mathbf{B C F} \cong \Delta \mathbf{A B E}
(ii) area of square ABFG = area of rectangle BENM.
Prove that the quadrilateral obtained by joining the mid-points of an isosceles trapezium is a rhombus.
In the figure ( 2) given below, DE is drawn parallel to the diagonal AC of the quadrilateral ABCD to meet BC produced at the point E. Prove that area of quad. \mathrm{ABCD}= area of \Delta \mathrm{ABE}
In the figure ( 2) given below, DE is drawn parallel to the diagonal AC of the quadrilateral ABCD to meet BC produced at the point E. Prove that area of quad. \mathrm{ABCD}= area of \Delta \mathrm{ABE}
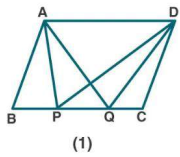
If E,F,G and H are the midpoint of the point of the sides AB,BC,CD and DA respectively of a parallelogram ABCD, prove that area of || gm ABCD.
In the adjoining figure, the lines l,\ m, and n are parallel to each other, and G is mid-point of CD. Calculate: ED if FD=4.4cm

Angles of a quadrilateral are (4x)^o, 5(x+2)^o, (7x-20)^o and 6(x+3)^o. Find
Each angle of the quadrilateral.
In quadrilateral ABCD, side AB is parallel to side DC. If \angle A: \angle D =1:2 and \angle C: \angle B =4:5
Calculate each angle of the quadrilateral.
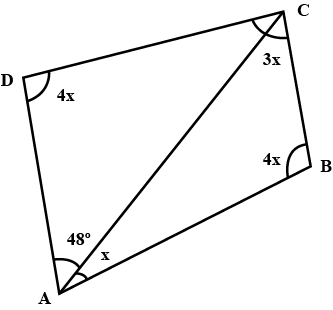
Use the information given in the following figure to find:
\angle B and \angle C
Use the information given in the following figure to find:
x
From the following figure find:
x
Two angles of a quadrilateral are 89^o and 113^o. If the other angles are equal;
find equal angles.
One angle of a quadrilateral is 90^o and all other angles are equal; find each equal angle
If angles of quadrilateral are in the ratio 4:5:3:6; find each angle of the quadrilateral.
Angles of a quadrilateral are (4x)^o, 5(x+2)^o, (7x-20)^o and 6(x+3)^o. Find
The value of x.
Two angles of a quadrilateral are 68^o and 76^o. If other two angles are in the ratio 5:7, find the measure of each of them.
In the given figure
\angle b=2a+15
And \angle c=3a+5; find the value of b and c

Three angles of a quadrilateral are equal. If the fourth angle is 69^o; find the measure of equal angles.
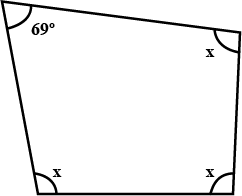
Given: In quadrilateral ABCD; \angle C=40^o, \angle D= \angle C -8^o , \angle A=5(a+2)^o and \angle B=2(2a+7)^o.
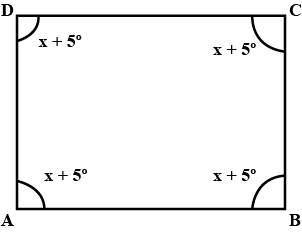
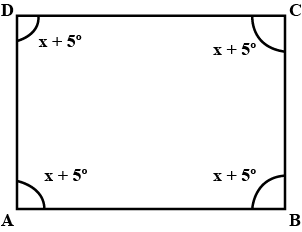
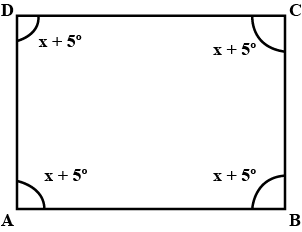
Each angle of a quadrilateral is x+5^o. Find
The value of x
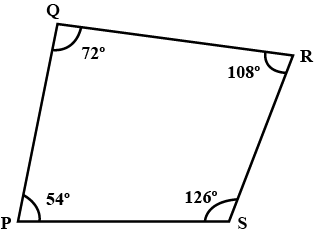
In the quadrilateral PQRS, \angle P: \angle Q: \angle R: \angle S=3:4:6:7,
Calculate to each other. Is PS also parallel to QR?
From the following figure find:
\angle ABC
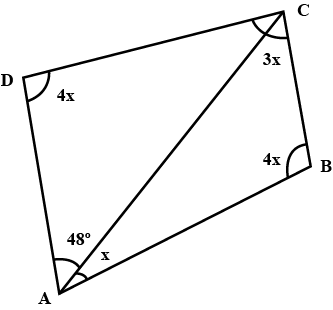
From the following figure find:
\angle ACD
The following figure shows a quadrilateral in which sides AB and DC are parallel. If \angle A:\angle D=4:5, \angle B=(3x-15)^o and \angle C=(4x+20)^o, find each angle of the quadrilateral ABCD.
Two diagonals of an isosceles trapezium are x\ cm and (3x-8) cm. Find the value of x.
Use the information given in the following figure to find the value of x.
Each angle of a quadrilateral is x+5^o. Find
Give the special name of the quadrilateral taken.
In parallelogram ABCD,E is a mid-point of side AB and CE bisect angle BCD. Prove that
Angle DEC is a right angle.
Each angle of a quadrilateral is x+5^o. Find
Each angle of the quadrilateral
In parallelogram ABCD,E is a mid-point of side AB and CE bisect angle BCD. Prove that
DE bisects and \angle ADC and
In the given figure, M is mid-point of AB and DE, whereas N is mid-point of BC and DF. Show that: EF=AC.
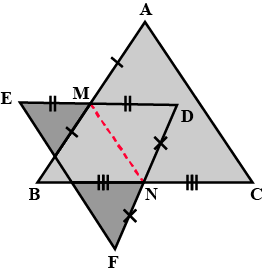
D, E and F are the mid-points of the sides AB, BC and CA of an isosceles triangle DEF is also isoceles.
In parallelogram ABCD,E is a mid-point of side AB and CE bisect angle BCD. Prove that
AE=AD.
In rhombus ABCD:
If \angle A=74^{\circ}; find \angle B and \angle C.
In parallelogram ABCD,\angle A=3 times \angle B. Find all the angles of the parallelogram. In the same parallelogram if AB=5x-7 and CD=3x+1; find the length of CD.
Given: Parallelogram ABCD in which diagonals AC and BD intersect at M. Prove: M is mid-point of LN.
In trapezium ABCD, sides AB and DC are parallel to each other. E is mid-point of AD and F is the mid-point of BC.
Prove that: AB+DC=2EF

In parallelogram ABCD, E is the mid-point of AB and AP is parallel to EC which meets DC at point O and BC produced at P. Prove that: BP=2AD
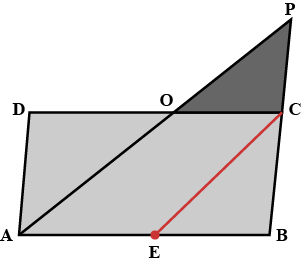
In the following figure, AC || PS || QR || SR.
Prove that:
Area of quadrilateral PQRS=2\times Area of quad ABCD.

In each of the following figures ,ABCD is a parallelogram in each case find the value of x and y.

In the following figure, BD is parallel to CA,E is midpoint of CA and BD=\dfrac{1}{2}CA. Prove that:
Ar.(\triangle ABC)=2\times Ar.(\triangle DBC)

E,F,G and H are the midpoints of the sides of a parallelogram ABCD. SHow that area of quadrilateral EFGH is half of the area of parallelogram ABCD.
State 'true' or 'false'
The diagonals of a quadrilateral bisect each other.
In parallelogram ABCD , AP and AQ are perpendicular from vertex of obtuse angle A as shown .If angles x : y = 2 : 1 ; find the angle of the parallelogram.
In triangle ABC,E and F are the mid-points of sides AB and AC respectively. If BF and CE intersect each other at point O, prove that the triangle OBC and quadrilateral AEOF are equal in area.
E is midpoint of sides AB and F is the midpoint of side DC pf parallelogram ABCD . Prove that AEFD is a parallelogram,
ABCD is a parallegram. E,\ F are the midpoints of BC
and CD respectively. AE,\ AF meet the diagonal BD at
Q and P respectively. Show that P and Q trisect
DB.
Prove that the line segments joining the midpoints of the adjacent sides of a quadrilateral form a parallelogram.
\square \mathrm{ABCD} is a parallelogram, \mathrm{P} and \mathrm{Q} are midpoints of side \mathrm{AB} and \mathrm{DC} respectively, then prove \square \mathrm{APCQ} is a parallelogram.

SL = LR
In a parallelogram ABCD, If \angle A = (3x + 2)^o, \angle B = (2x - 32)^o then find the value of x and then find the measures of \angle C and \angle D.
In the given figure, \DeltaABC is an equilateral triangle. Points F, D and E are the midpoints of sides AB, BC and AC respectively. Show that \Delta FED is an equilateral triangle.
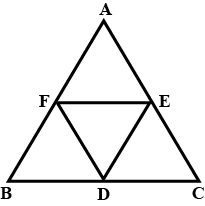
In the given figure, points X, Y, Z are the midpoints of side AB, side BC and side AC of \DeltaABC respectively. AB = 5 cm, AC = 9 cm and BC = 11 cm. Find the length of XY, YZ, XZ.
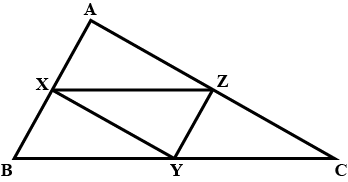
\square ABCD is a parallelogram, ratio of \angle A to \angle B of this parallelogram is 5 : 4. Find the measure of \angle B.
LN = \frac{1}{2} SQ
If the ratio of measures of two adjacent angles of a parallelogram is 1:2, find the measures of all angles of the parallelogram.
In a quadrilateral, \mathrm{IJKL}, IJ\parallel KL, \angle I=108^{\circ}, \angle \mathrm{K}=53^{\circ} \text { then find the measure of } \angle \mathrm{J} \text { and } \angle \mathrm{L}.
ABCD is a quadrilateral in which AD = BC and \angle DAB = \angle CBA . Prove that
(1) \triangle ABD \cong \triangle BAC
(2) BD = AC
(3) \angle ABD = \angle BAC
In quadrilateral ACBD, AC = AD and AB bisects \angle A. Show that \triangle ABC = \triangle ABD. What can you say about BC and BD?
In \square \mathrm{ABCD}, side \mathrm{BC}< side \mathrm{AD} in following figure.side \mathrm{BC} \| side \mathrm{AD} and if side \mathrm{BA} \cong \operatorname{side} \mathrm{CD} then prove that \angle \mathrm{ABC} \cong \angle \mathrm{DCB}

In the adjacent figure, \square \mathrm{ABCD} is a trapezium \mathrm{AB} \| \mathrm{DC} . Points \mathrm{M} and \mathrm{N} are midpoints of diagonal \mathrm{AC} and \mathrm{DB} respectively then prove that \mathrm{MN} \| \mathrm{AB} .

In the adjacent figure, seg \mathrm{AB} \| seg \mathrm{PQ}, seg \mathrm{AB} \cong \operatorname{seg} \mathrm{PQ}, seg \mathrm{AC} \| seg \mathrm{PR}, seg \mathrm{AC} \cong \operatorname{seg} \mathrm{PR} , then prove that, seg \mathrm{BC} \| seg \mathrm{QR} and \operatorname{seg} B C \cong \operatorname{seg} QR.
Give a definition for each of the following terms. Are there other terms that need to be defined first ? What are they, and how might you define them ?
Square:
In \square \mathrm{ABCD}, side \mathrm{BC} \| side \mathrm{AD}, side \mathrm{AB} \cong side \mathrm{DC} If \angle \mathrm{A}=72^{\circ} then find the measure of \angle \mathrm{B}, and \angle \mathrm{D}
AB and CD are respectively the smallest and longest sides of a quadrilateral ABCD. Show that \angle A> \angle C and \angle B > \angle D.
In the given Figure, \mathrm{ABCD} is a trapezium. AB\parallel \mathrm{DC} . Points \mathrm{P} and \mathrm{Q} are midpoints of seg \mathrm{AD} and seg \mathrm{BC} respectively. Then prove that, \mathrm{PQ} \| \mathrm{AB} and \mathrm{PQ}=\dfrac{1}{2}(A B+D C) .

D, E and F are respectively the midpoints of the sides BC, CA and AB of a \triangle ABC. Show that
BDEF is a parallelogram.
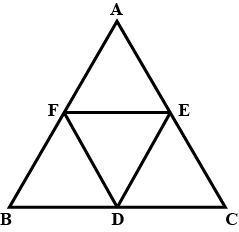
In figure ABC and BDE are two equilateral triangles such thta D is the mid-point of BC.AE intersects BC in F. Prove that
ar(\triangle BDE)=\dfrac {1}{4}ar(\triangle ABC)
ar. (\triangle ABC)=2 ar.(\triangle BEC)
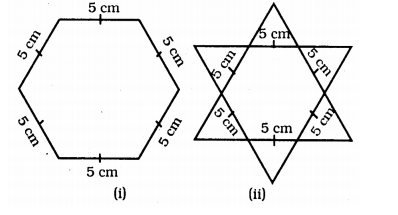
Complete the hexagonal and star shaped Rangolies by filling them with as many equilateral triangles of side 1 cm as you can. Count the number of triangles in each case. Which has more triangles ?
In a triangle ABC, E is the mid-point of median AD. Show that ar.(\triangle BED)=\dfrac{1}{4} ar.(\triangle ABC).
If E, F, G and H are respectively the mid-points of the sides of a parallelogram ABCD, show that

D, E and F are respectively the midpoints of the sides BC, CA and AB of a \triangle ABC. Show that
ar.(\triangle DEF)=\dfrac{1}{4}\ ar.(\triangle ABC)
Which of the following figures lie on the same base and between the same parallels. In such a case, write the common base and the two parallels.
In figure ABC and BDE are two equilateral triangles such thta D is the mid-point of BC.AE intersects BC in F. Prove that
ar(\triangle BDE)=\dfrac {1}{4}ar(\triangle ABC)
ar. (\triangle FED)=\dfrac 18 ar.(\triangle AFC)

In figure ABC and BDE are two equilateral triangles such thta D is the mid-point of BC.AE intersects BC in F. Prove that
ar(\triangle BDE)=\dfrac {1}{4}ar(\triangle ABC)
ar. (\triangle BDE)=\dfrac 12 ar.(\triangle BAE)
In figure ABC and BDE are two equilateral triangles such thta D is the mid-point of BC.AE intersects BC in F. Prove that
ar(\triangle BDE)=\dfrac {1}{4}ar(\triangle ABC)
ar. (\triangle BEF)=ar.(\triangle AFD)

Complete the following:
A quadrilateral has ___ diagonals
P and Q are respectively the mid points of sides AB and BC of a triangle AABC and R is the mid point of AP, show that
ar. (\triangle RQC)=\dfrac 38 ar. (\triangle ABC)
In fig. ABCDE is a pentagon. A line through B parallel to AC meets DC produced at F. Show that
ar.(\triangle ACB)=ar.(\triangle ACF)

A villager Itwaari has a plot of land of the shape of a quadrilateral. The Gram Panchayat of the village decide to take over some portion of his plot from one of the corners to construct a Health Centre. Itwaari agrees to the above proposal with the condition that he should be given equal amount of land in lieu of his land adjoining his plot so as to form a triangular plot.
Explain how this proposal with be implemented.

BACD is a trapezium with AB\parallel DC. A line parallel to AC intersects AB at X and BC at Y. Prove that area (ADx)= area (ACy). [Hint: Join Cx.)

In the figure ABCD is a square . M , N , O & P are the midpoint of sides AB , BC CD and DA respectively . Identify the congruent triangles.
In fig. ABCDE is a pentagon. A line through B parallel to AC meets DC produced at F. Show that
ar.(\triangle EDF)=ar.(ABCDE).

Complete the following:
A quadrilateral has _____ sides.
D, E and F are respectively the midpoints of the sides BC, CA and AB of a \triangle ABC. Show that
ar.(BDEF)=\dfrac{1}{2}\ ar.(\triangle ABC).

P and Q are respectively the mid points of sides AB and BC of a triangle AABC and R is the mid point of AP, show that
ar. (\triangle PRQ)=\dfrac 12 ar. (\triangle ARC)

In any quadrilateral ABCD, \angle A+\angle D={90}^{o}. Prove that {AC}^{2}+{BD}^{2}={AD}^{2}+{BC}^{2}
Fill in the blanks:
All squares are _____.
Let ABCD be a quadrilateral in which AB is the smallest side and CD is a largest side \angle A > \angle C and \angle B > \angle D ( Hint: join AC and BD)
Let AB ,. CD be two segment such that AB | | CD and AD | | BC . Let E be the midpoint of BC and let DE extended meet AB in F. Prove that AB = BF
Suppose ABC is a triangle and D is the midpoint of BC . Assume that the perpendicular from D to AB and AC are of equal length . Prove that ABC is isosceles .

Let ABCD be a quadrilateral in which AD is the largest side and BC is the smallest side. Prove that $$ \angle A < \angle C$$ [Hint: join AC ]
In a triangle ABC, let D be the midpoint of BC. Prove that AB+AC > 2AD (what property of quadrilateral is needed here )
Let ABCD be a quadrilateral and P, Q, R, S be the midpoints of AB, BC, CD, DA respectively prove that PQRS is a parallelogram [ what extra result you need to prove this ] It can be proved if ABCD is a rectangle
Prove that midpoint (C) of hypotaneous in a right-angled triangle AOB is situated at equal distance from vertices 0, \mathrm{A} , and \mathrm{B} of triangle.
Let ABCD be a quadrilateral with diagonals AC and BD. Prove the following statement
AB+BC+CD > AD
Prove that the line segment joining the mid-point of the hypotenuse of a right triangle to the vertex of the right angle is equal to half the hypotenuse.
Show that the line segment joining the mid-points of the consecutive sides of a square is also a square.
In figure, ABCD is a parallelogram P and Q are the mid-points of opposite sides AB and DC of a parallelogram ABCD. Prove that PRQS is a parallelogram.
In the given figure, ABCD is a trapezium in which AB \parallel CD. M and N are mid-points of the diagonal AC and BD respectively. Prove that MN \parallel CD and MN= \dfrac12 (CD- AB).
One angle of a quadrilateral is 108^\circ and the other three angles are equal. Find the value of each of the equal angle.
In a quadrilateral ABCD, \angle A = 3x, \angle B= 5x, \angle C= 20x and \angle D= 8 x. Find the value of x.
Can all the four angles a quadrilateral are obtuse? Give reason for your answer.
The three angles of a quadrilateral measure 56^\circ, 100^\circ and 88^\circ. Find the measure of the fourth angle.
In figure, the diagonal AC of a quadrilateral ABCD bisects the angle A and C. Prove that AB = AD and CB = CD.
State whether the following statement is true or false, give a reason. The angles of a certain quadrilateral are 50^{\circ}, 60^{\circ}, 112^{\circ}, 130^{\circ}
Let ABCD be a unit square. Draw a quadrant of a circle with A as the center and B,\ D as endpoints of the arc. Similarly, draw a quadrant of a circle with B as the center and A,\ C as endpoints of the arc. Inscribe a circle \Gamma touching the arc AC externally, the arc BD internally, and also touching the side AD. Find the radius of the circle \Gamma.
Suppose EFGH is a trapezium in which EF is parallel to HG. Through X, the mid-point of EH, XY is drawn parallel to EF meeting FG at Y. Prove that XY bisects FG.
Suppose X, Y and Z are the midpoints of the sides PQ, QR and RP respectively of a triangle PQR. Prove that XYRZ is a parallelogram.
The base of a pyramid is a rhombus whose diagonals are 6 m and 8 m long. The altitude of the pyramid passes through the point of intersection, of the diagonals of the rhombus and is 1 m long. Find the lateral area of the pyramid.
Prove that if the mid-points of the opposite sides of a quadrilateral are joined, they bisect each other.
In a rectangle ABCD, P, Q, R and S are the midpoints of the sides AB, BC, CD and DA respectively. Find the area of PQRS in terms of area of ABCD.
Prove the converse of the mid-point theorem following the guidelines given below: Consider a triangle ABC with D as the mid-point of AB. Draw DE \parallel BC to intersect AC in E. Let {E}_{1} be the mid-point of AC. Use mid-point theorem to get D{E}_{1} \parallel BC and D{E}_{1} = {BC}/{2}. Conclude E = {E}_{1} and hence E is the mid-point of AC.
Prove that the sum of four angles of a quadrilateral ABCD is 360^{0}.
Ina quadrilateral ABCD, AB=AD and CB=CD. Prove that :(i) AC bisects angle BAD.(ii) AC is perpendicular bisector of BD.
In quadrilateral ABCD \angleB=90^o, \angleC -\angleD=60^o and \angleA -\angleC-\angleD =10^o. Find \angleA, \angleC and \angleD.

In a parallelogram ABCD, \angle A = x ^ { \circ } , \angle B = ( 3 x + 20 ) ^ { \circ } . Find x and \angle C and \angle D
ABCD is a parallelogram and \angle DAB=60^{o}. If the bisects AP and BP of angles A and B respectively, meet at P on CD.Prove that P is the midpoint of CD.
In the adjacent figure, ar(PEA)=ar(PAC) and ar(RPC)=ar(KEA) show that quadrilateral PARK and PACE are trapezium.
ABCD is a rhombus and P, Q, R and S are the mid-points of the sides AB, BC, CD and DA respectively. Show that the quadrilateral PQRS is a rectangle.
In the given figure, ABCD is a trapezium, Diagonals AC and BD interset at Q Prove that the area of \Delta AOD is the same as \Delta BOC
ABCD is a rhombus. If \angle BCA=35^{o}, find \angle ADC.
State the angle sum property of a quadrilateral.
The area of a rectangular plot is 540 { m }^{ 2 }. If its length is 27 m, find its breadth and perimeter.
ABCD is a parallelogram. The circle through A, B and C intersects CD produced at E. If AB=10\ cm, BC=8\ cm, CE=14\ cm . Find AE
Parallelogram ABCD and rectangle ABEF are on the same base and have equal areas. Show that perimeter of the parallelogram is greater than that of rectangle.
In the figure, \Box ABCD is a trapezium, AB\parallel DC. Point P and Q are midpoints of seg\ AD and seg\ BC respectively. Then prove that, PQ\parallel AB and PQ=\dfrac{1}{2}(AB+DC).
If the tangents P A and P B from a point P to the circle with cetre O inclined to each other at the angle of { 110 }^{ }, then find \angle POA.
In a parallelogram, the bisector of any two consecutive angles intersect at right angle, Prove it.
The diagonals of a rectangle ABCD intersect at o. if \angle BOC =70^{\circ}. Find \angle ODA.
PQRS is a parallelogram. PM is the height from P to \overline {SR} and PN is the height from P to \overline {QR} . If SR=12 cm and PM=7.6 cm. Find the area of the parallelogram PQRS.
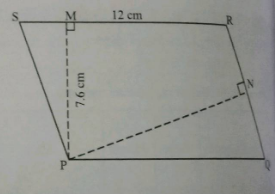
Both the pair of opposite angles of quadrilateral are equal and supplementary. Find the measure of each angle.
If non parallel sides of a trapezium are equal, prove that it is cyclic.
Line segment joining the mid - points M and N of parallel sides AB and DC respectively of a trapezium ABCD is perpendicular to both the sides AB and DC . Prove that AD = BC.
In triangle ABC, P is the mid-point of side BC. A line through P and parallel to CA meets AB at point Q; and a line through Q meets at S. QS parallel to BC meets median AP at point R. prove that : (i) AP = 2AR (ii) BC= 4QR
In the above figure RISK and CLUE are parallelograms, Find the value of x.
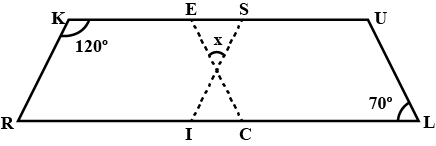
In figure above, the bisectors of \angle P and \angle Q meet at a point O. If \angle R={ 100 }^{ \circ } and \angle S={ 50 }^{ \circ}, find \angle {POQ}.
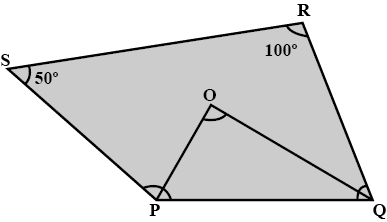
Prove that opposite sides of a quadrilateral circumscribing a circle subtend supplementarily angles at the center of the circle.
In the adjoining figure, D, E and F are mid points of the side BC, CA and AB of \Delta ABC. If AB =4.3 cm , BC =5.6 cm and AC =3.5 cm. Find the perimeter of
(i) \Delta DEF (ii) Quad. BDEF
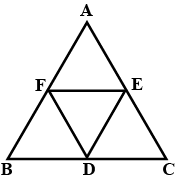
ABCD is a trapezium in which AB || DC, AB=16\ cm and DC=24\ cm. If E and F are respectively the midpoints of AD and BC, prove that ar(ABEF)=9/11\ ar (EFCD).
Image
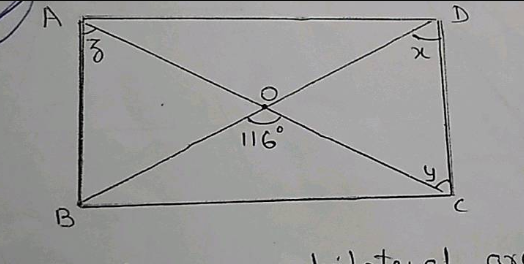
The length of the diagonals of a rhombus is in the ratio 4:3 .If its area is 384 cm^{2}, find its side.
Show that the quadrilateral formed by joining the midpoints of the pairs of adjacent sides of a rhombus is a rectangle.

Bisectors of \angle{B} and \angle{D} of a quadrilateral ABCD meet CD and AB produced at P and Q respectively. Prove that \angle{P} + \angle{Q} = 1/2(\angle{ABC} + \angle{ADC})
P,Q,R and S are respectively the midpoints of the sides AB,BC,CD and DA of a quadrilateral ABCD, Show that PQ\parallel AC and PQ=\cfrac{1}{2}AC
ABCD is a trapezium in which AB \parallel DC and its diagonals intersect each other at O. Using Basic Proportionality theorem, prove that AO/BO = CO/DO.
The midpoints of the sides AB,BC,CD and DA of a quadrilateral ABCD are joined to form a quadrilateral. If AC=BD and AC\bot BD then prove that the quadrilateral formed is a square.

If D,\ E and F mid-points of sides AB,\ BC and CA respectively of an isosceles triangle ABC, prove that \triangle DEF is also isosceles.
The angles of quadrilateral are in the ratio 3 : 4 : 5 : 6 Show that the quadrilateral is a trapezium.
Class 9 Maths Extra Questions
- Areas Of Parallelograms And Triangles Extra Questions
- Circles Extra Questions
- Constructions Extra Questions
- Coordinate Geometry Extra Questions
- Heron'S Formula Extra Questions
- Introduction To Euclid'S Geometry Extra Questions
- Linear Equations In Two Variable Extra Questions
- Lines And Angles Extra Questions
- Number Systems Extra Questions
- Polynomials Extra Questions
- Probability Extra Questions
- Quadrilaterals Extra Questions
- Statistics Extra Questions
- Surface Areas And Volumes Extra Questions
- Triangles Extra Questions
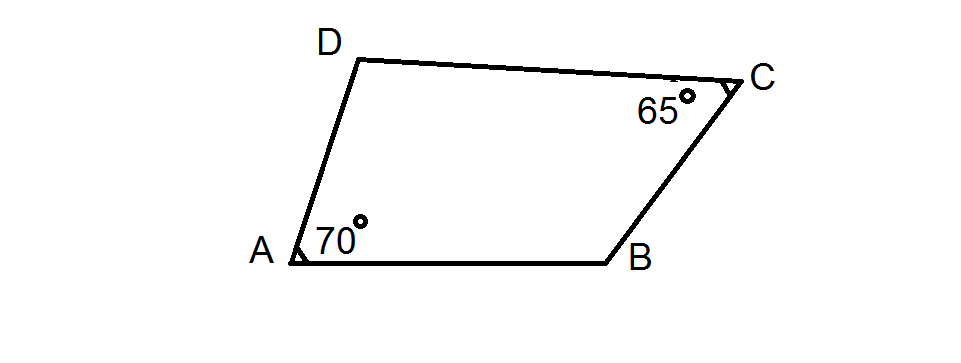
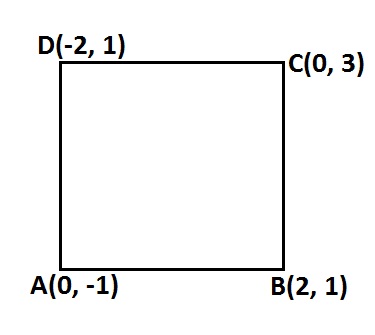

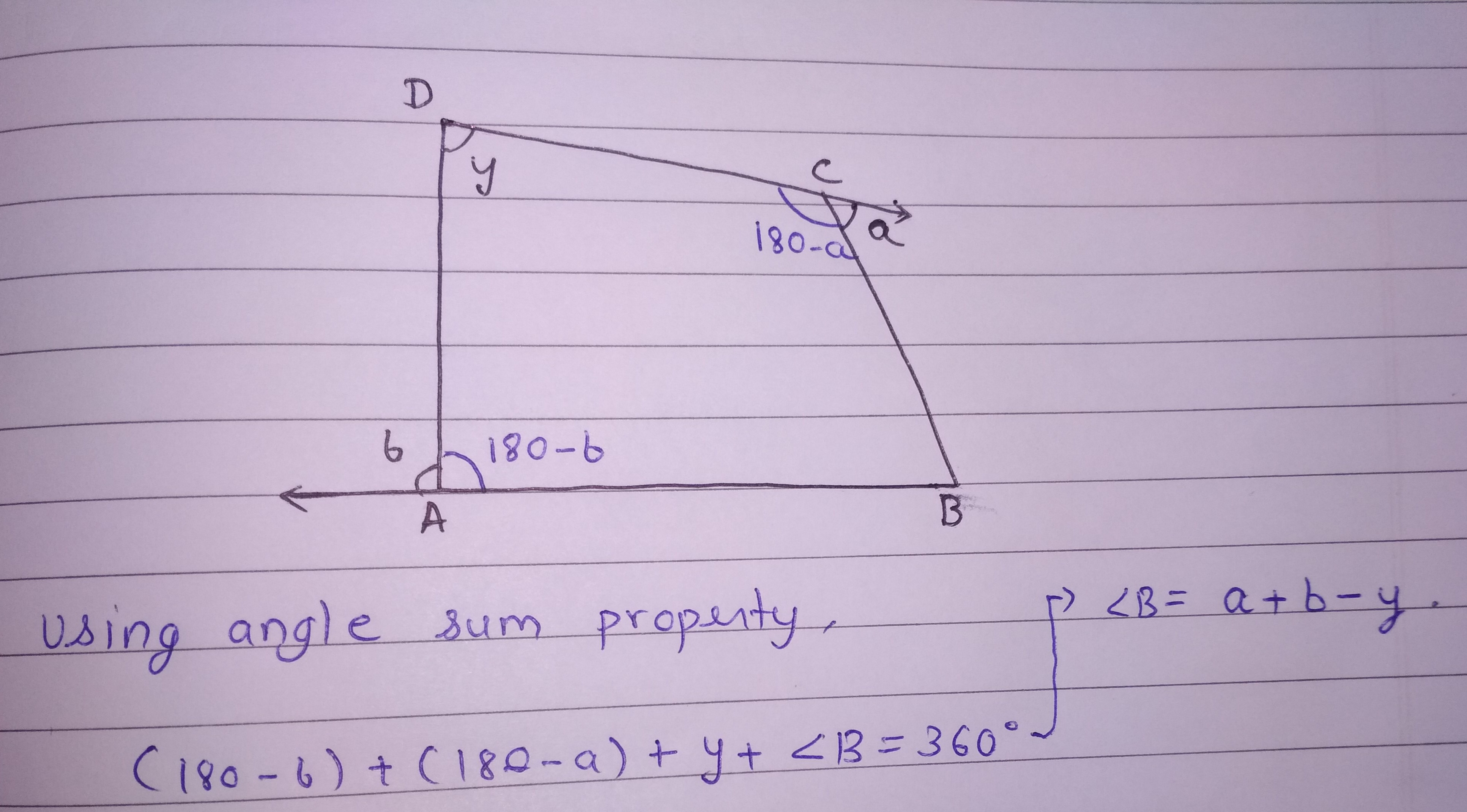



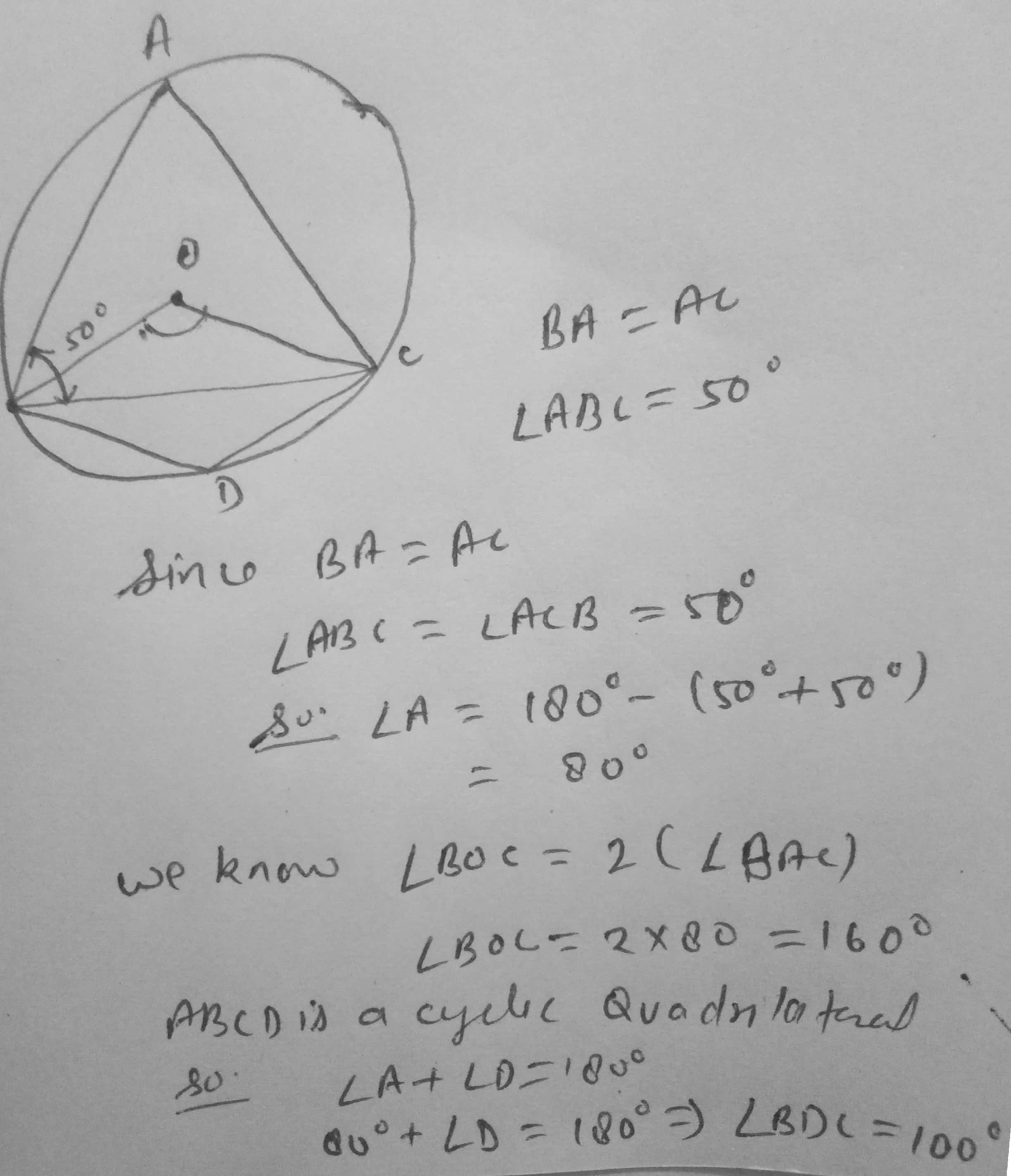

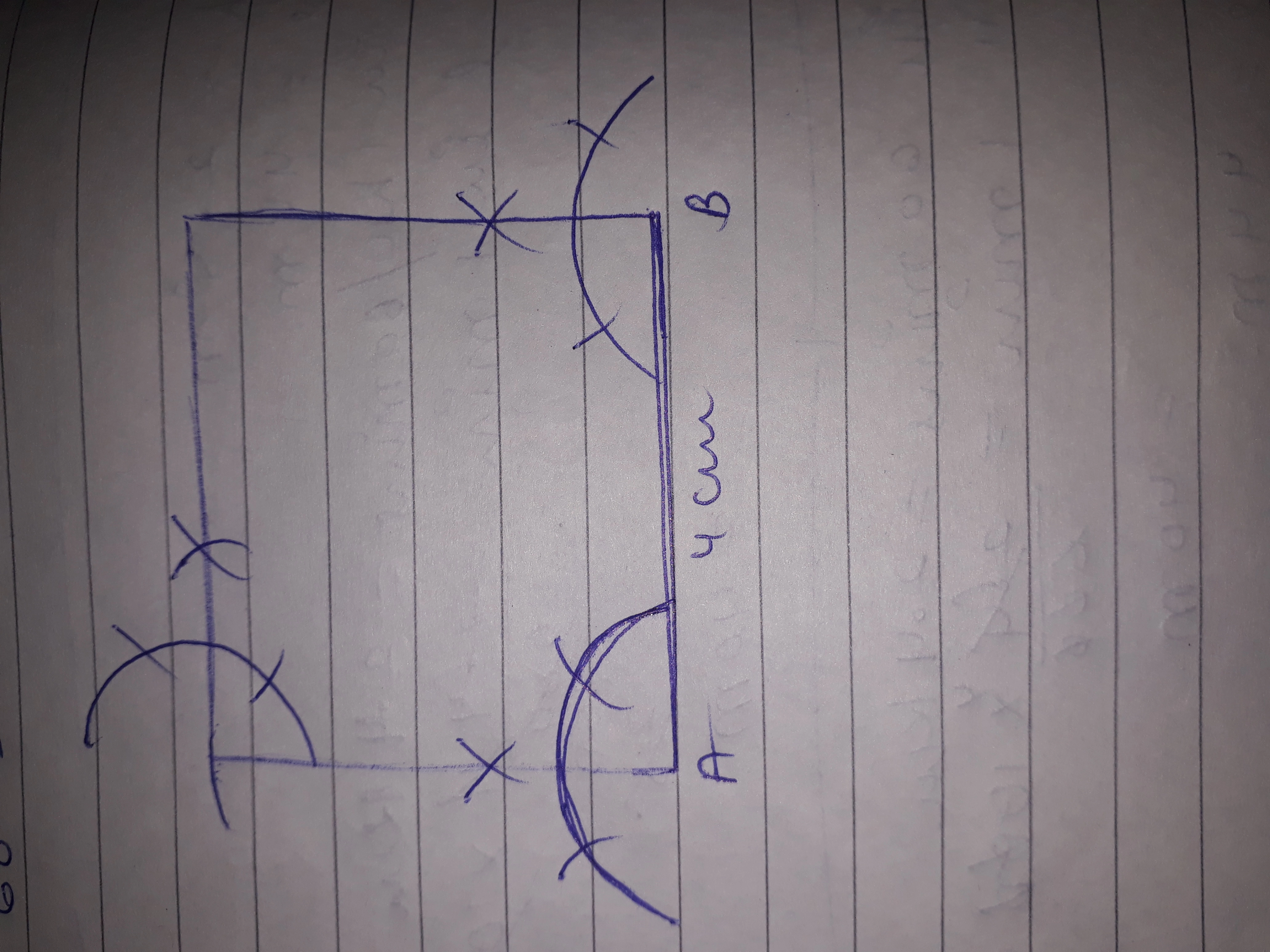

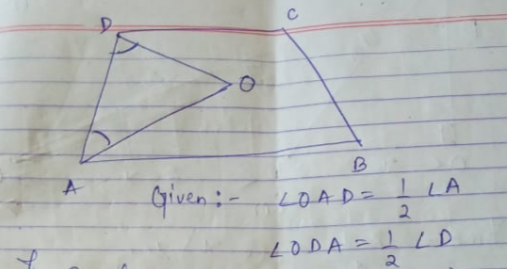


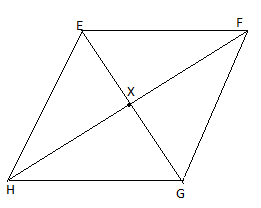

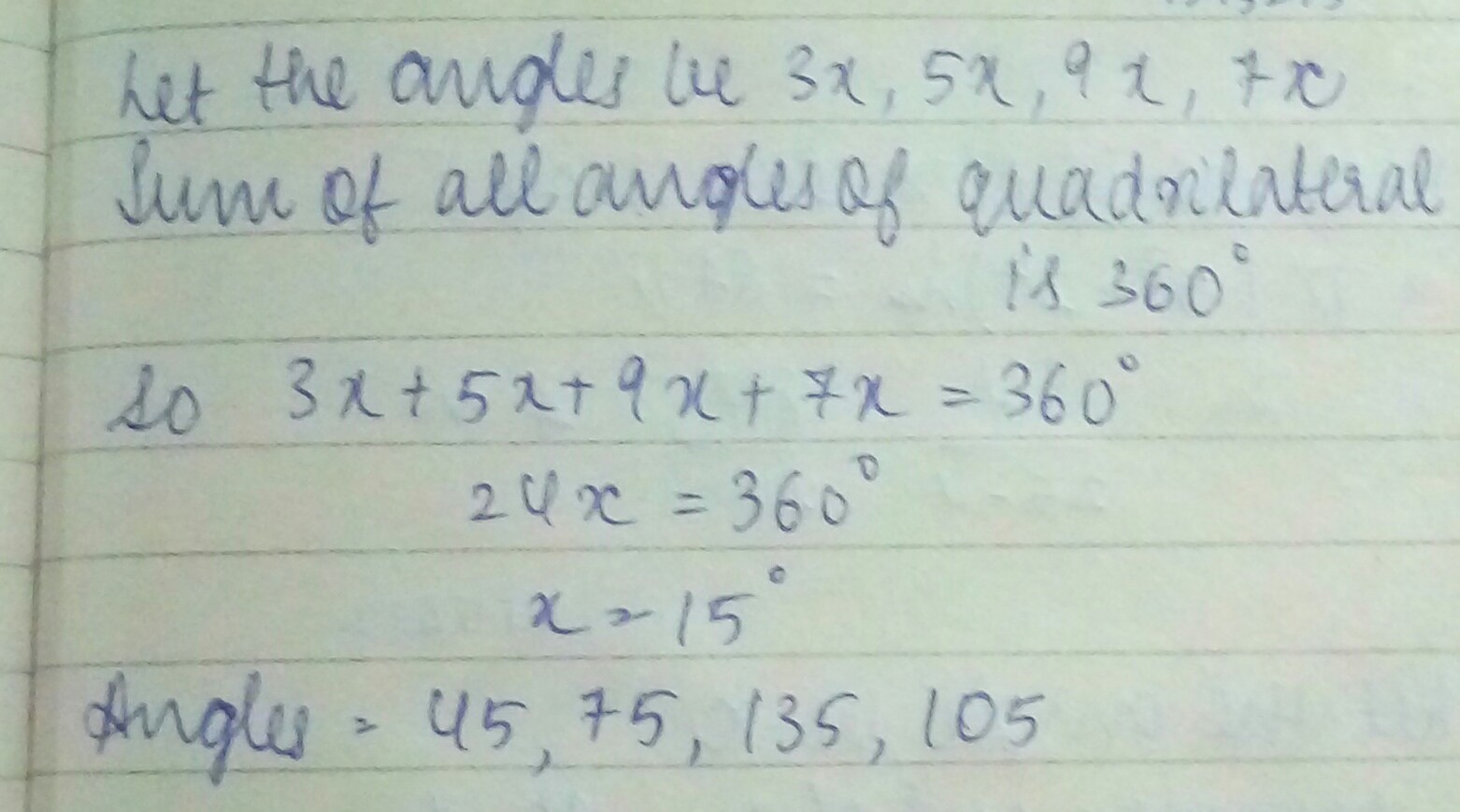
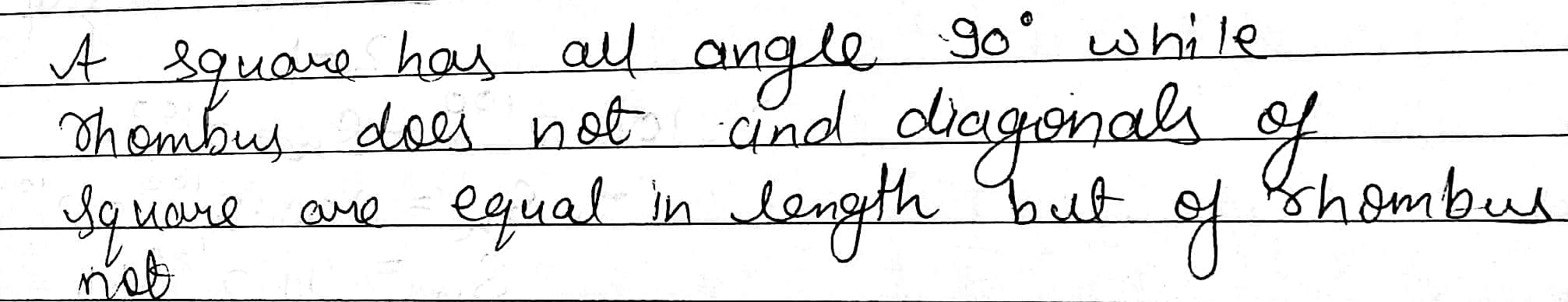
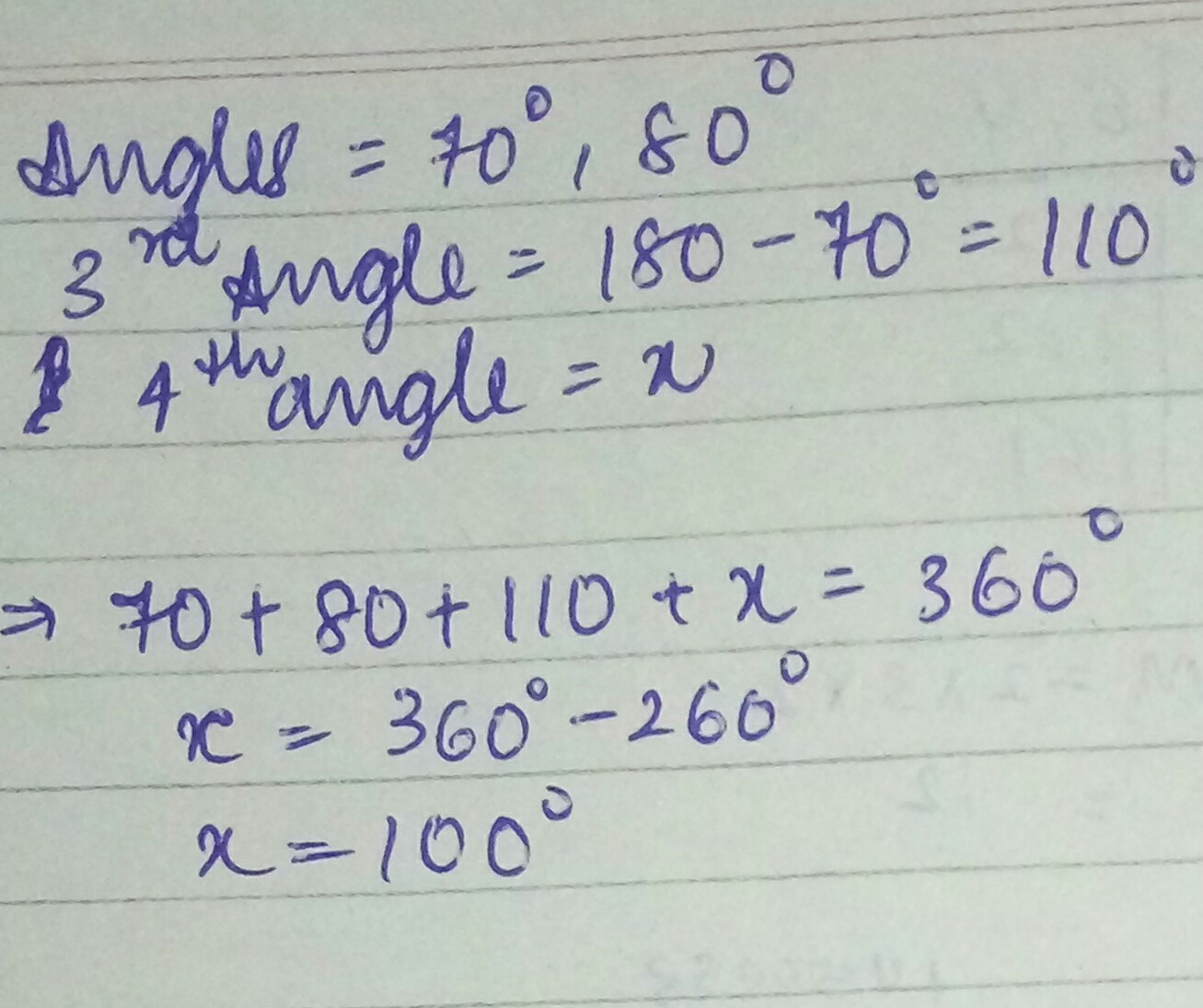
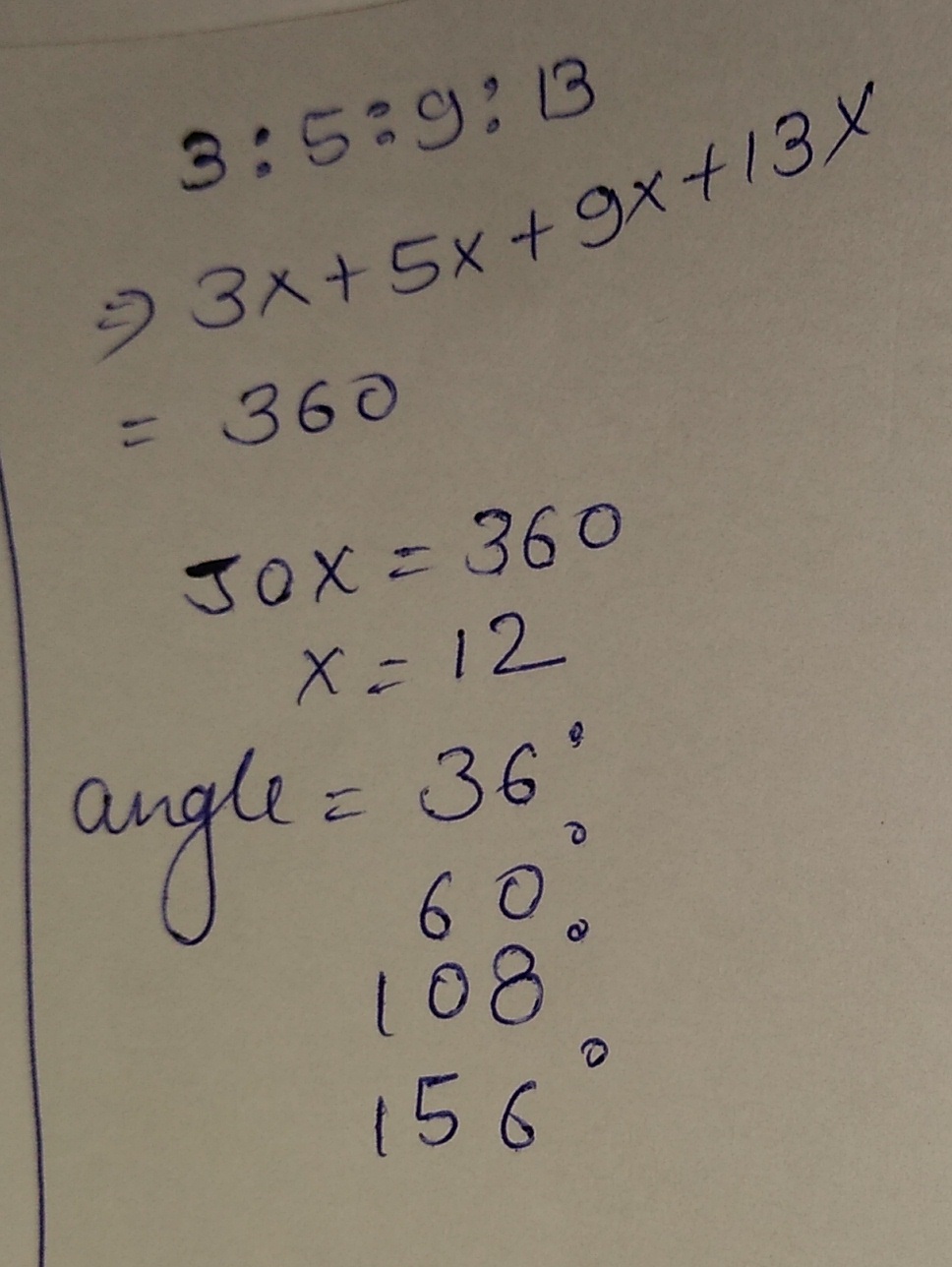

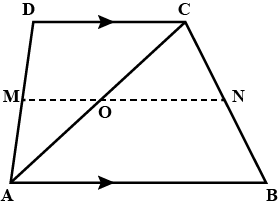


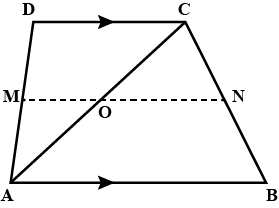
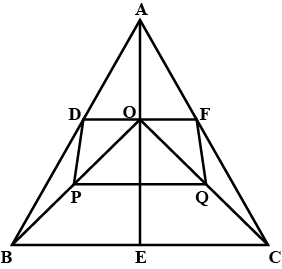

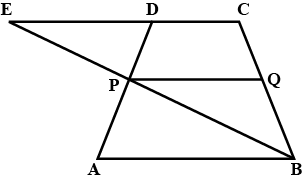


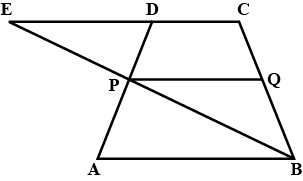
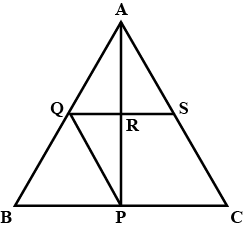
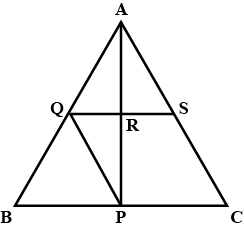

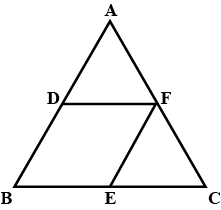
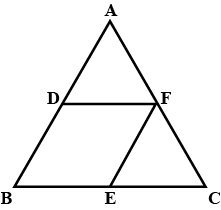
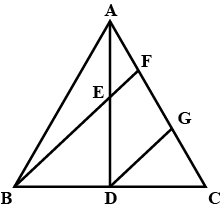
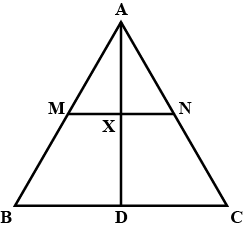
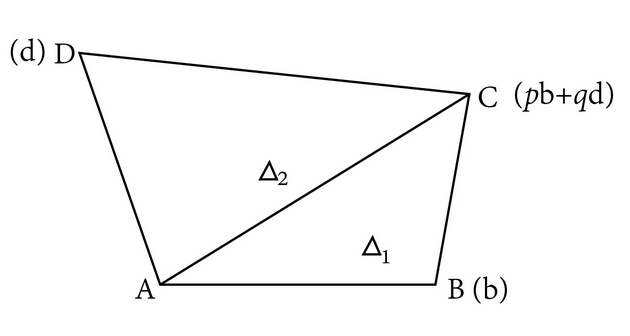
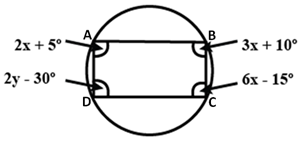

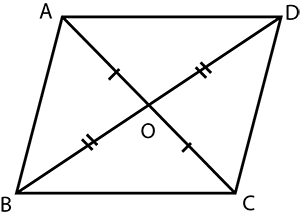
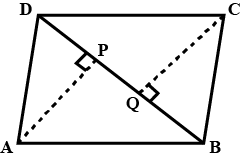

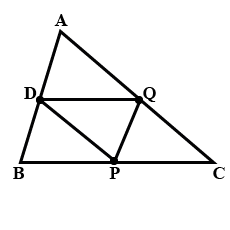

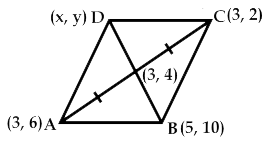

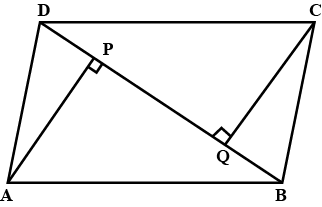


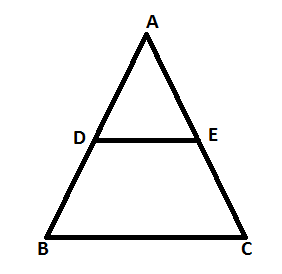

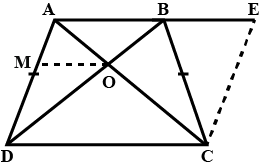
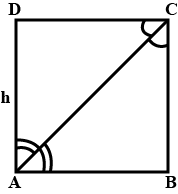



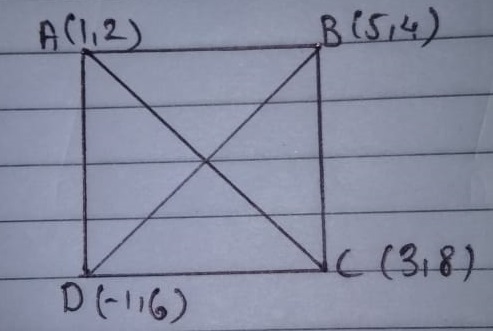


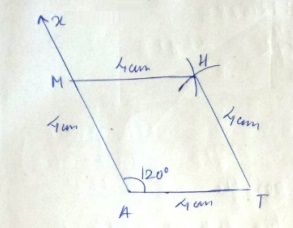
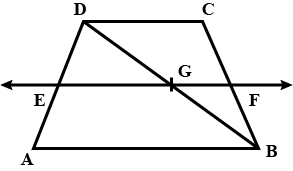

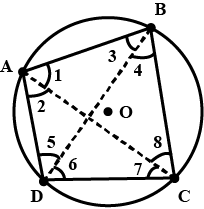

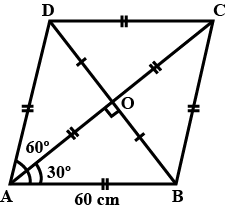
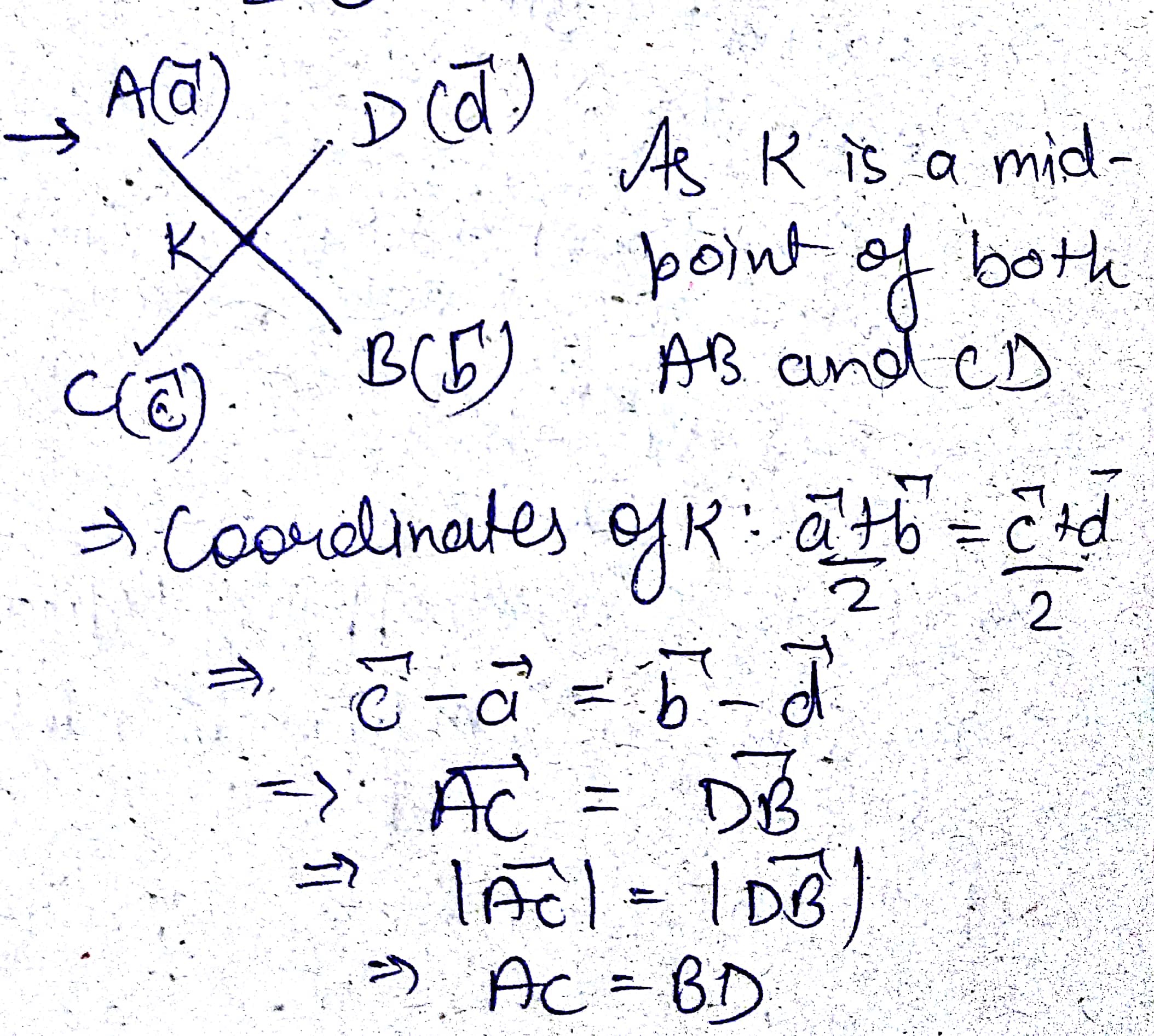

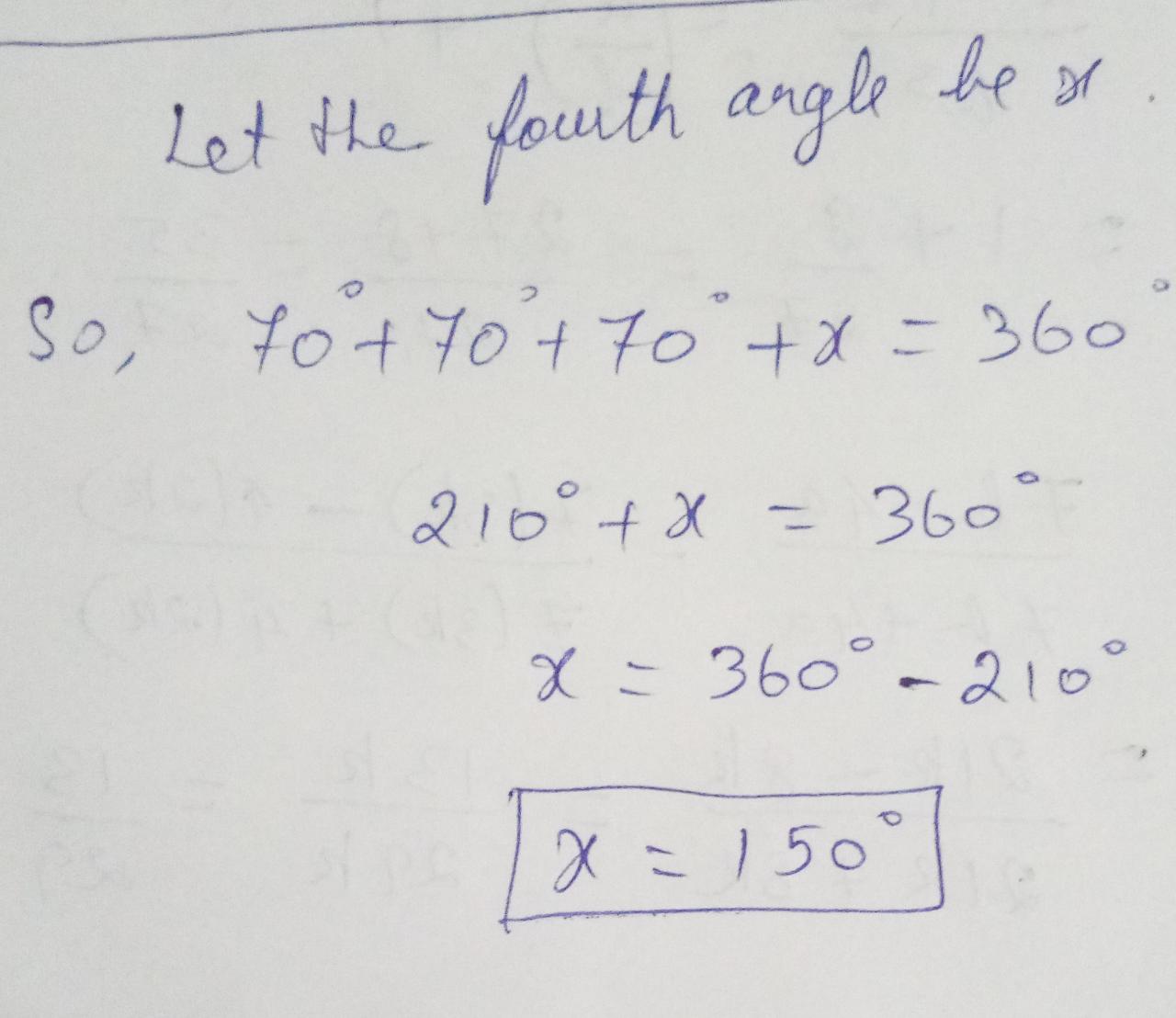



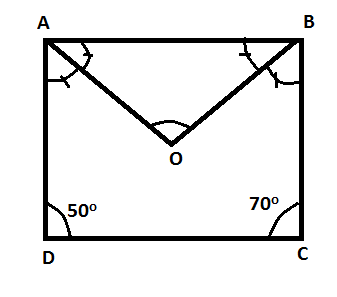
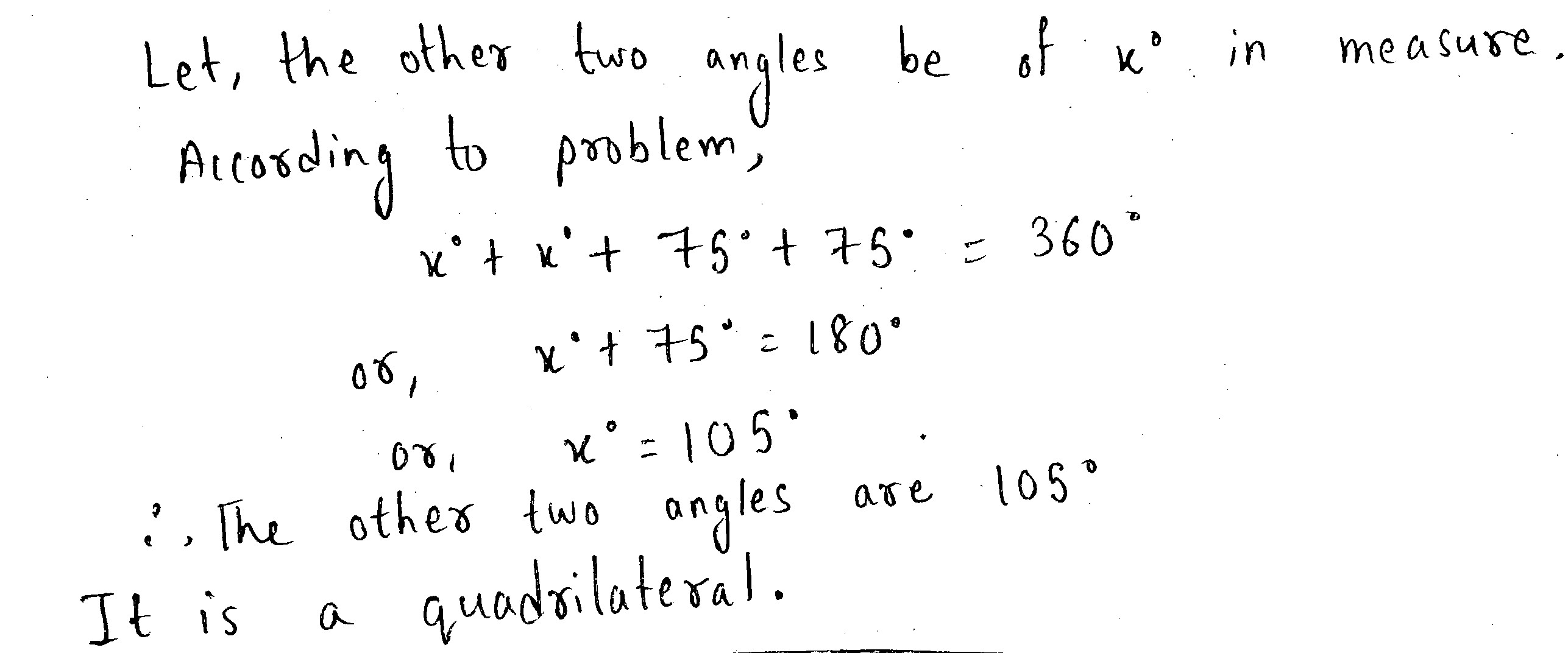
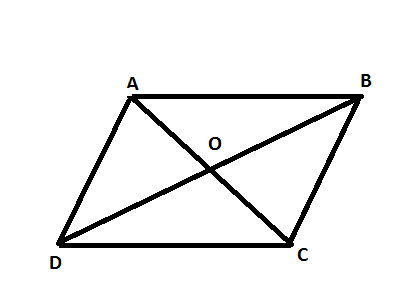

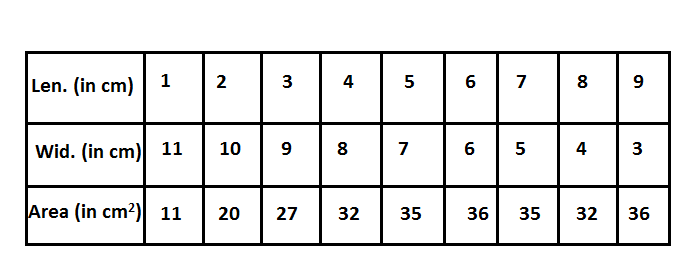

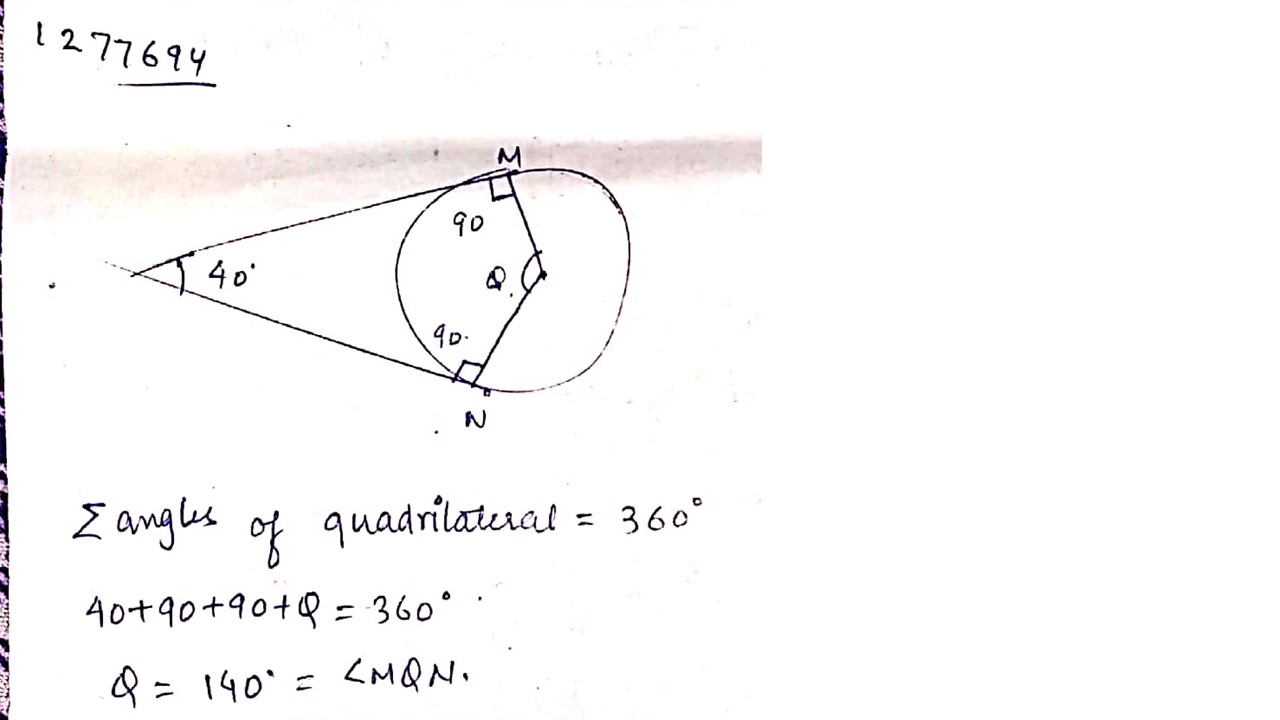
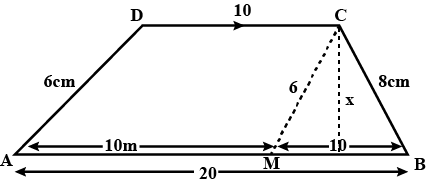
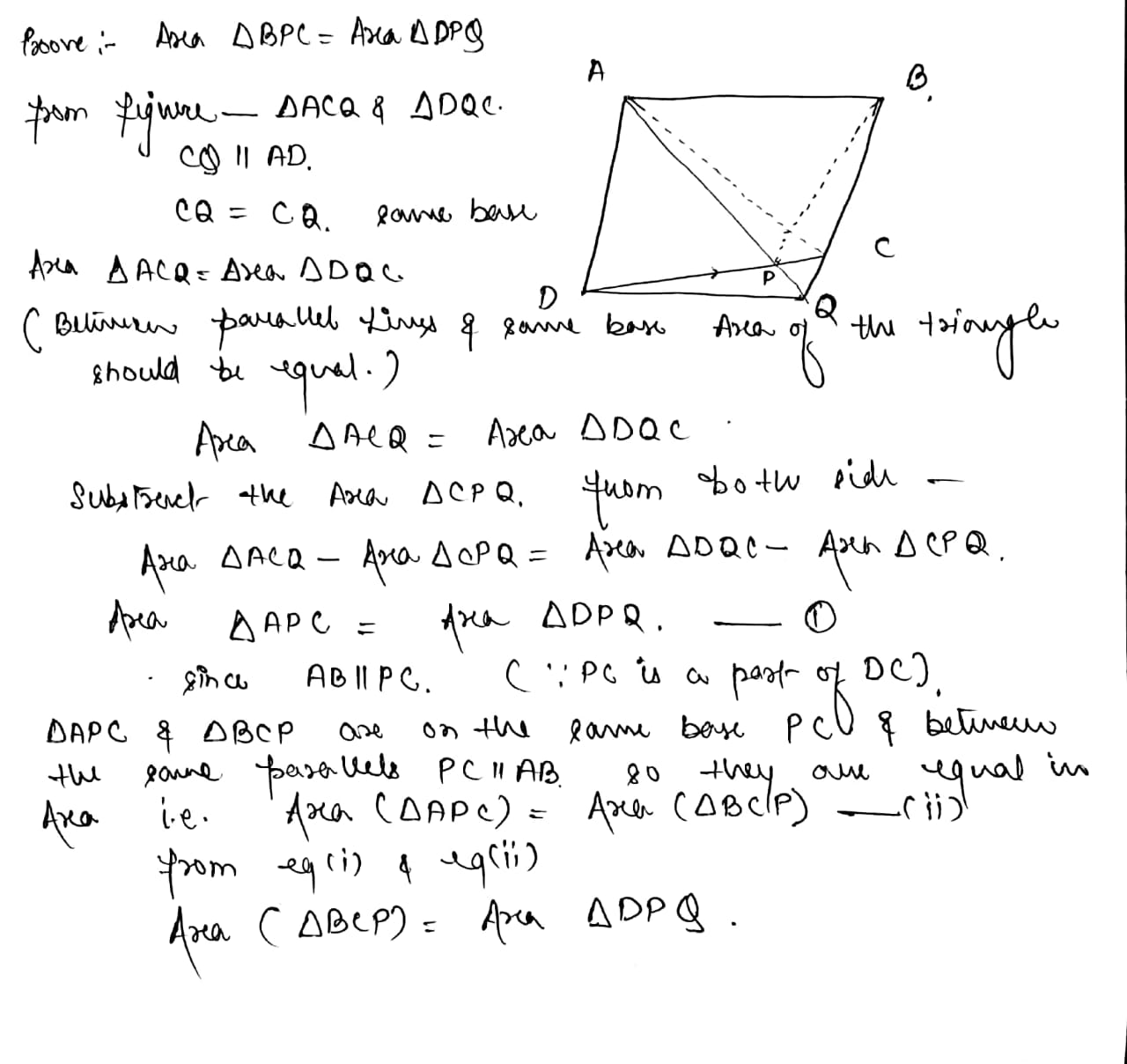

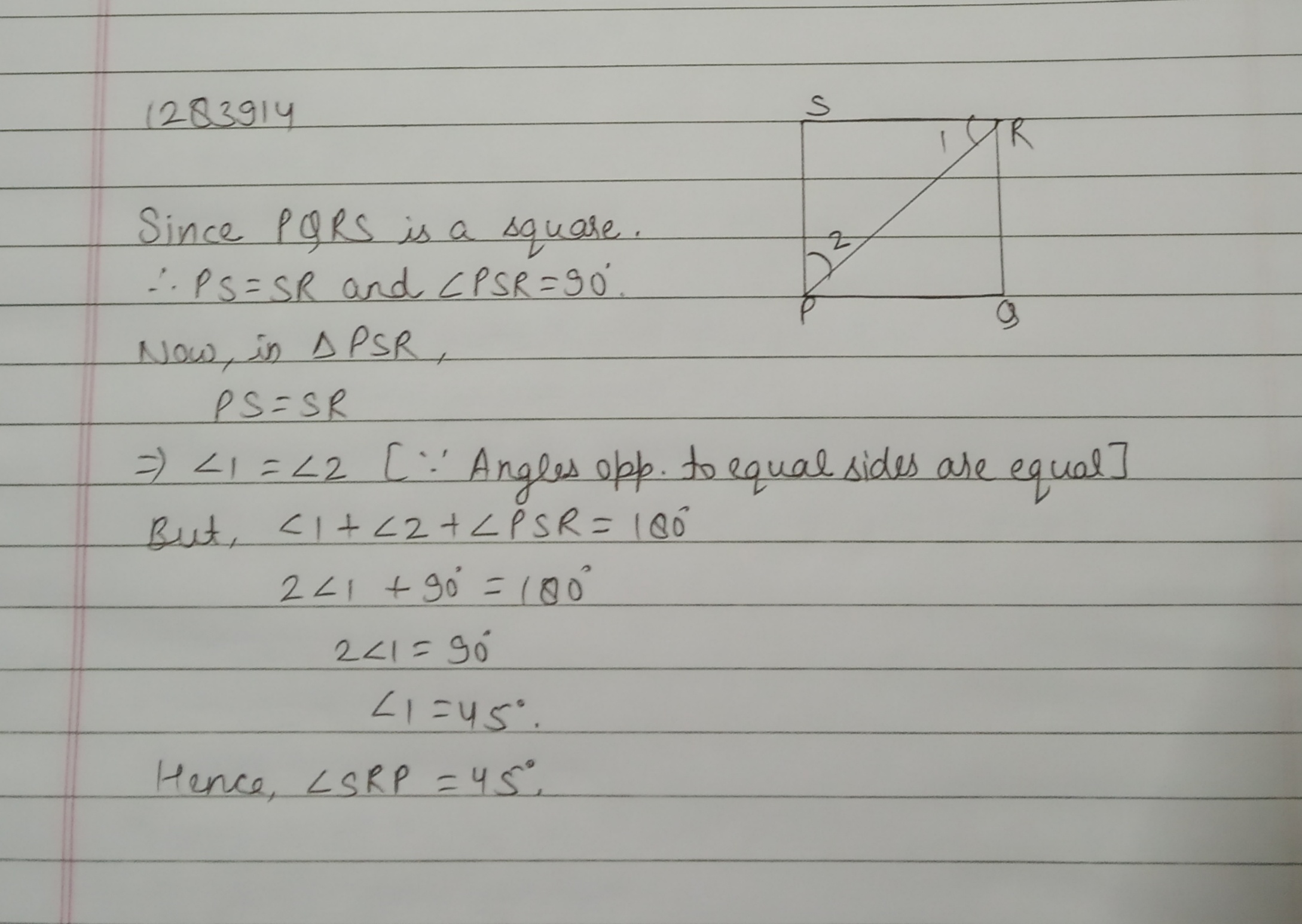
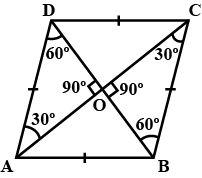
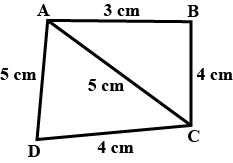

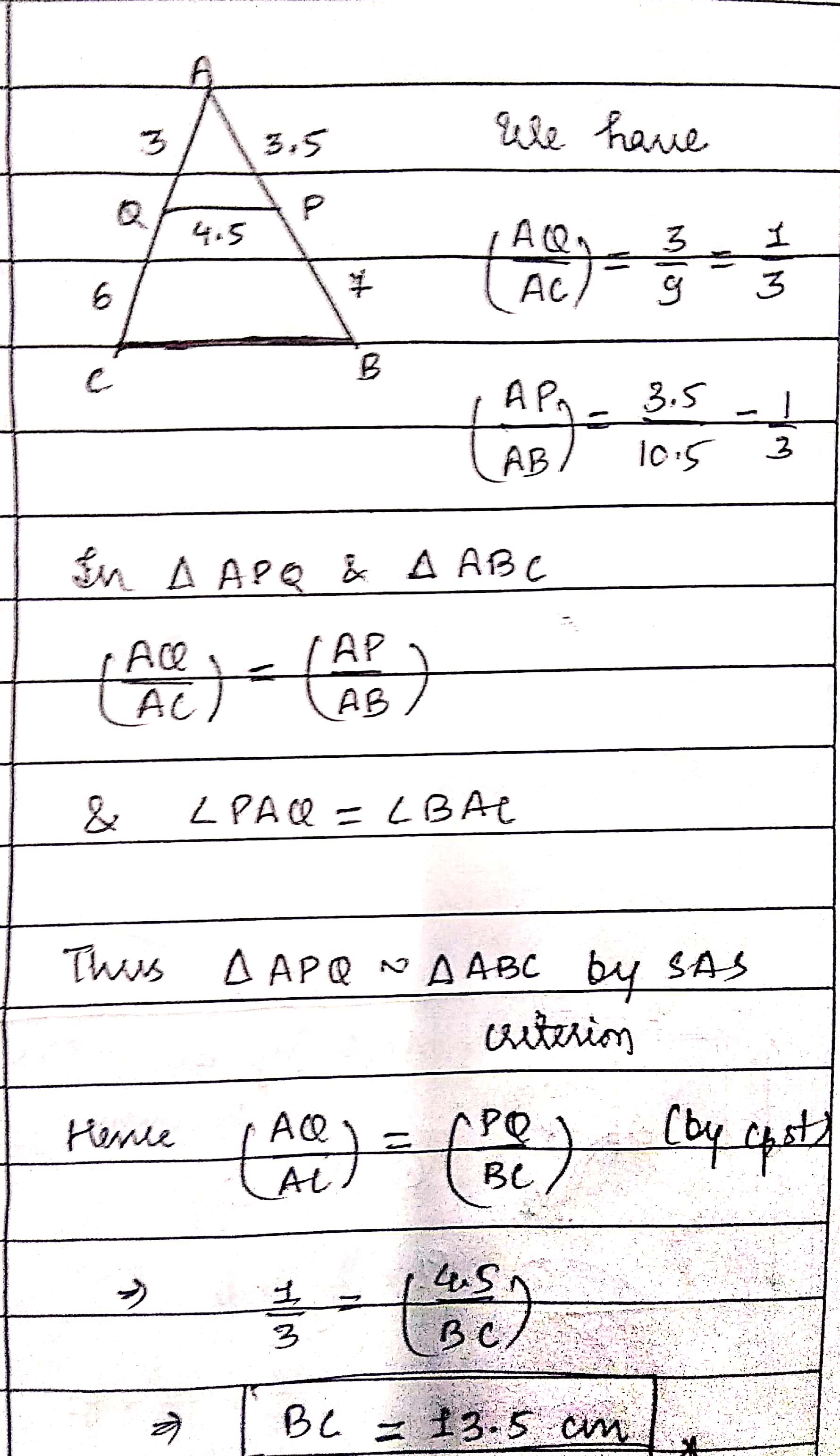
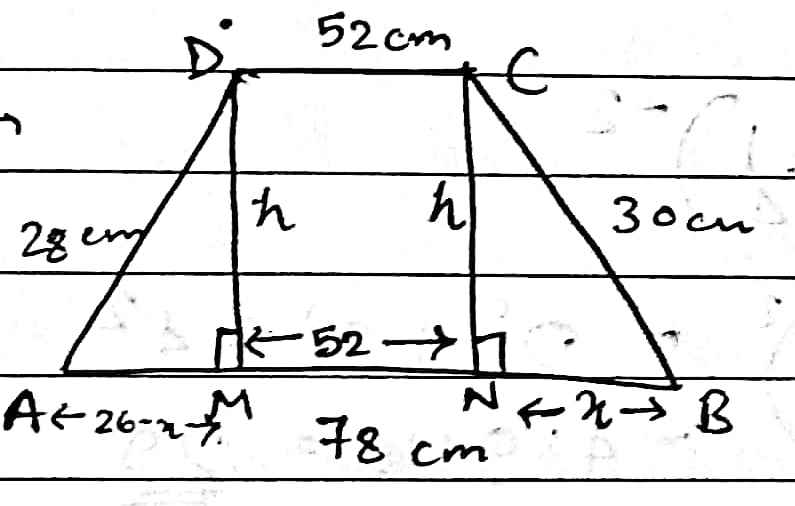
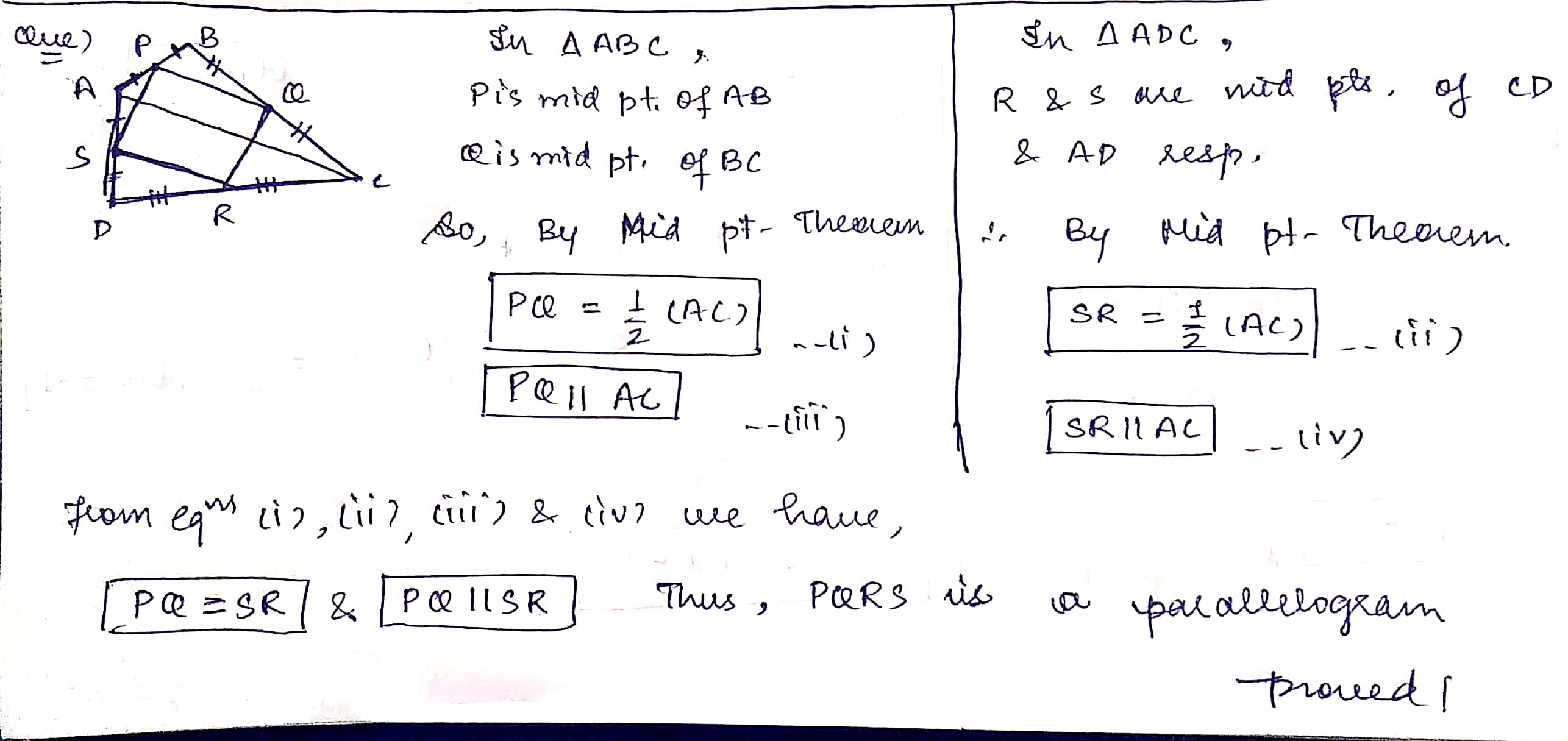




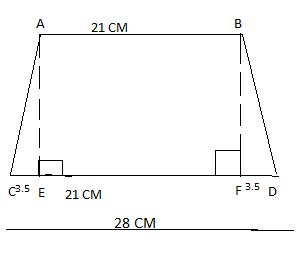
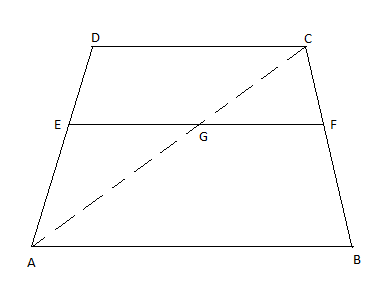
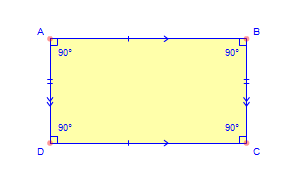
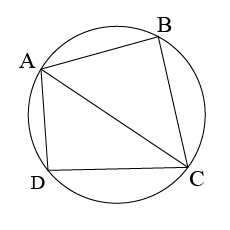




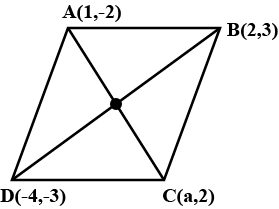

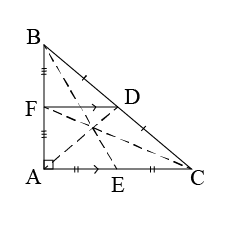
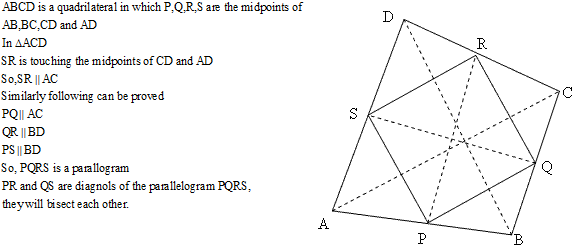
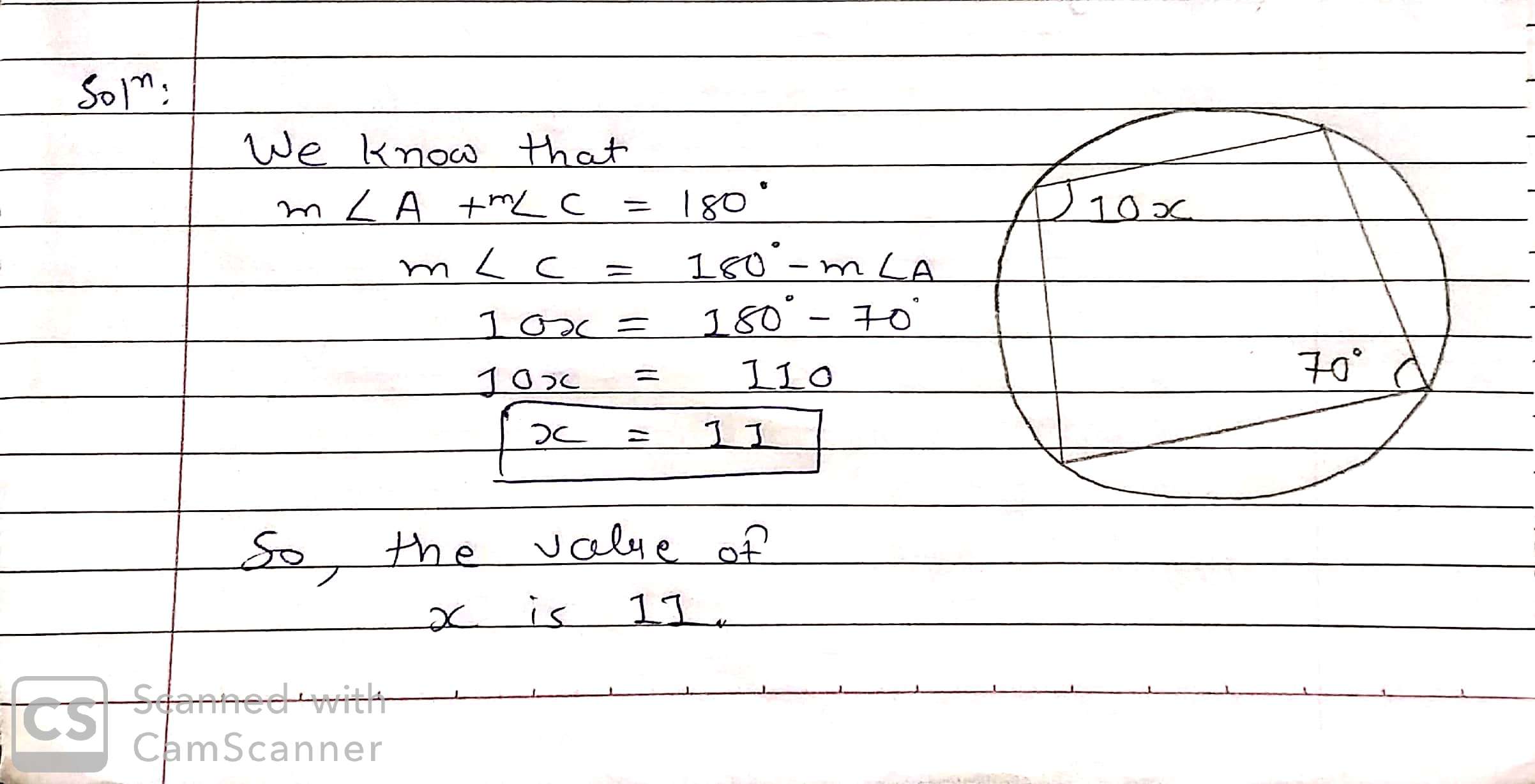
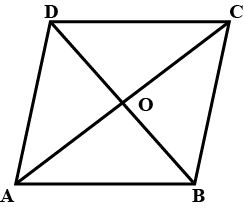


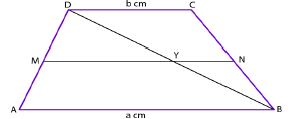


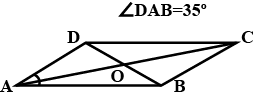
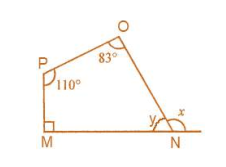

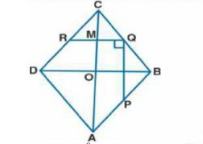


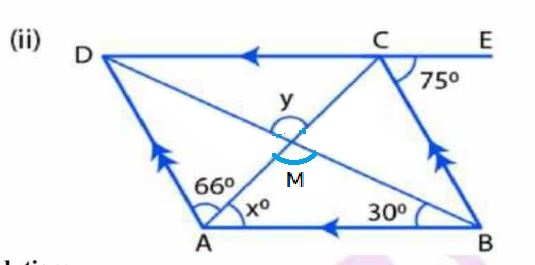
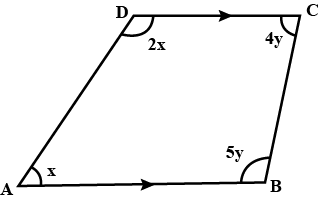

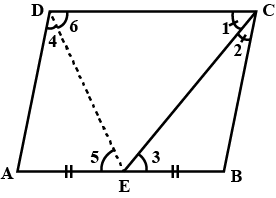

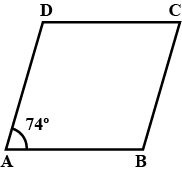
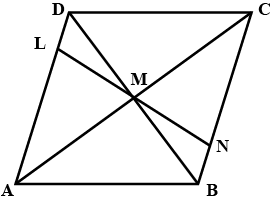

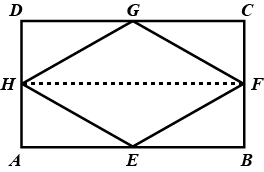
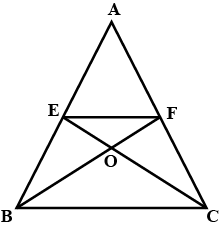



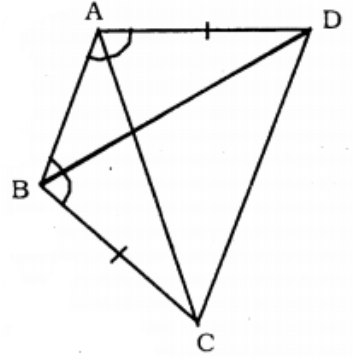
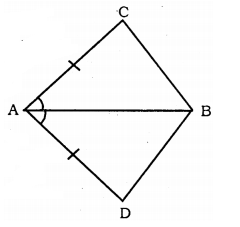
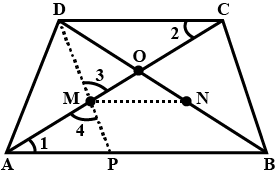


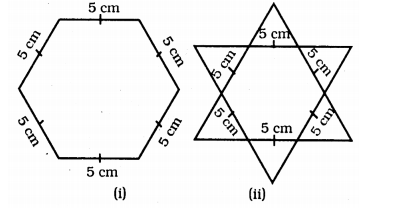
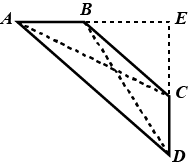


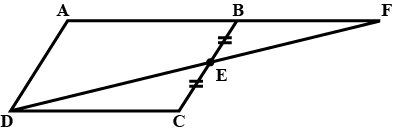

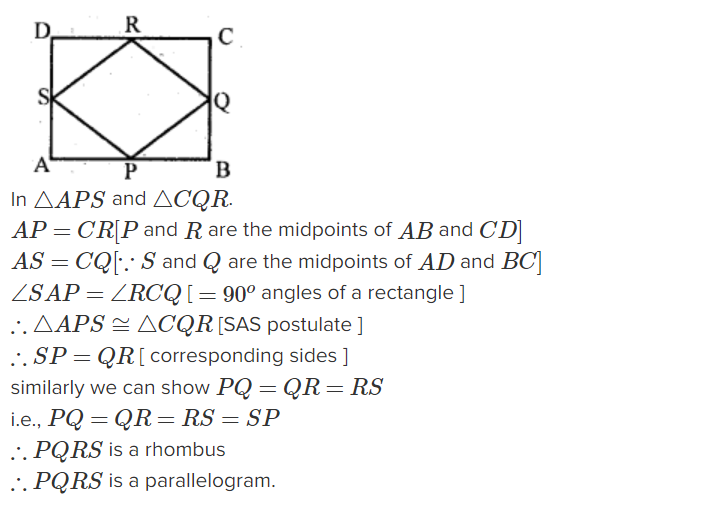


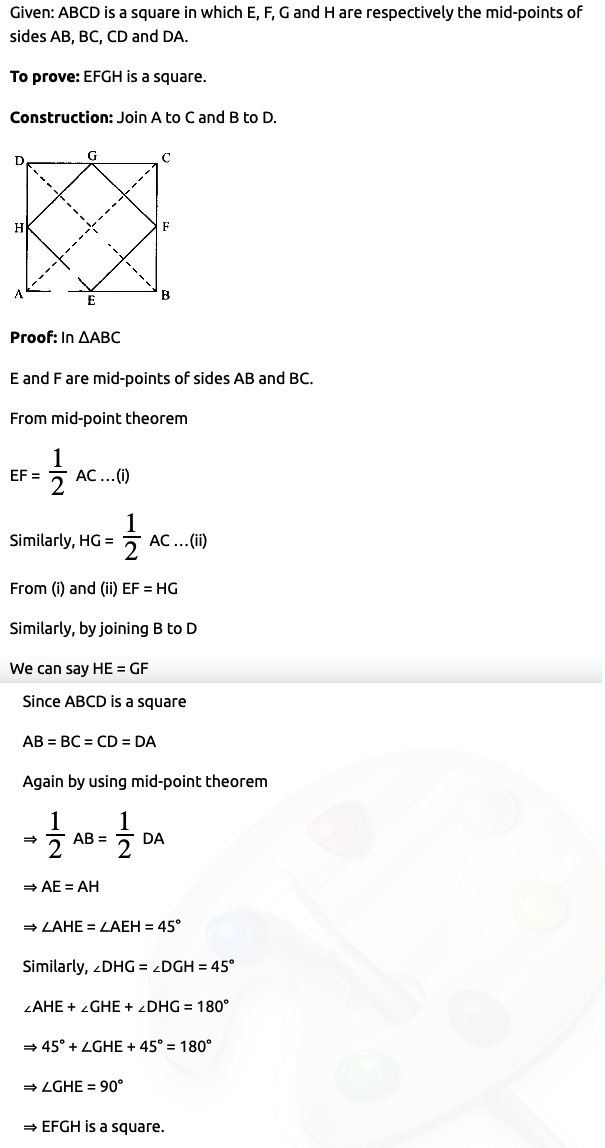
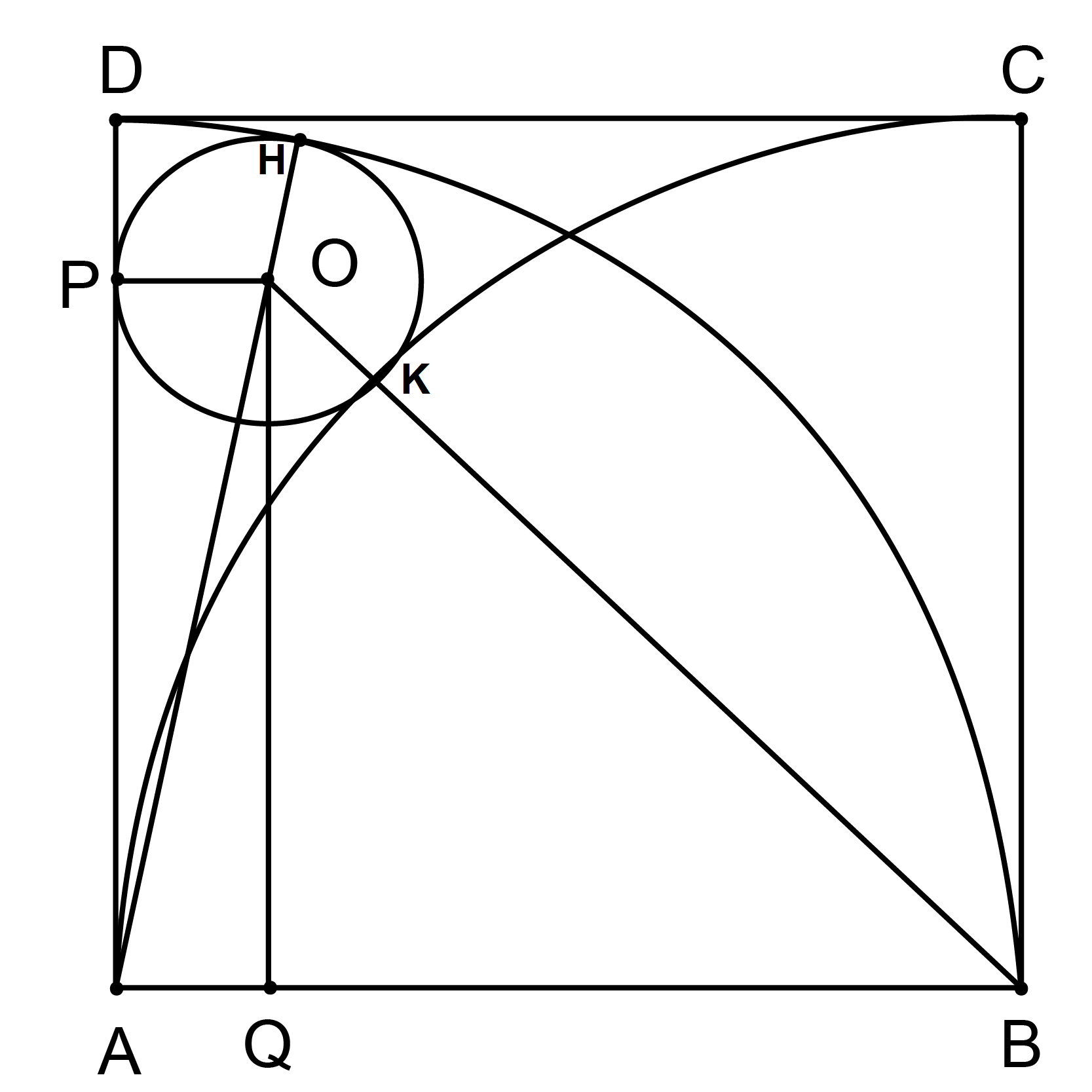
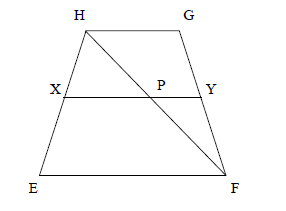
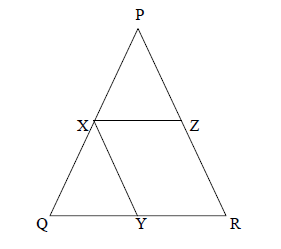




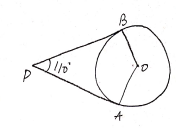
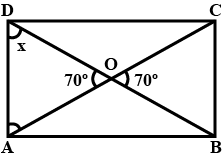


.png)
.png)