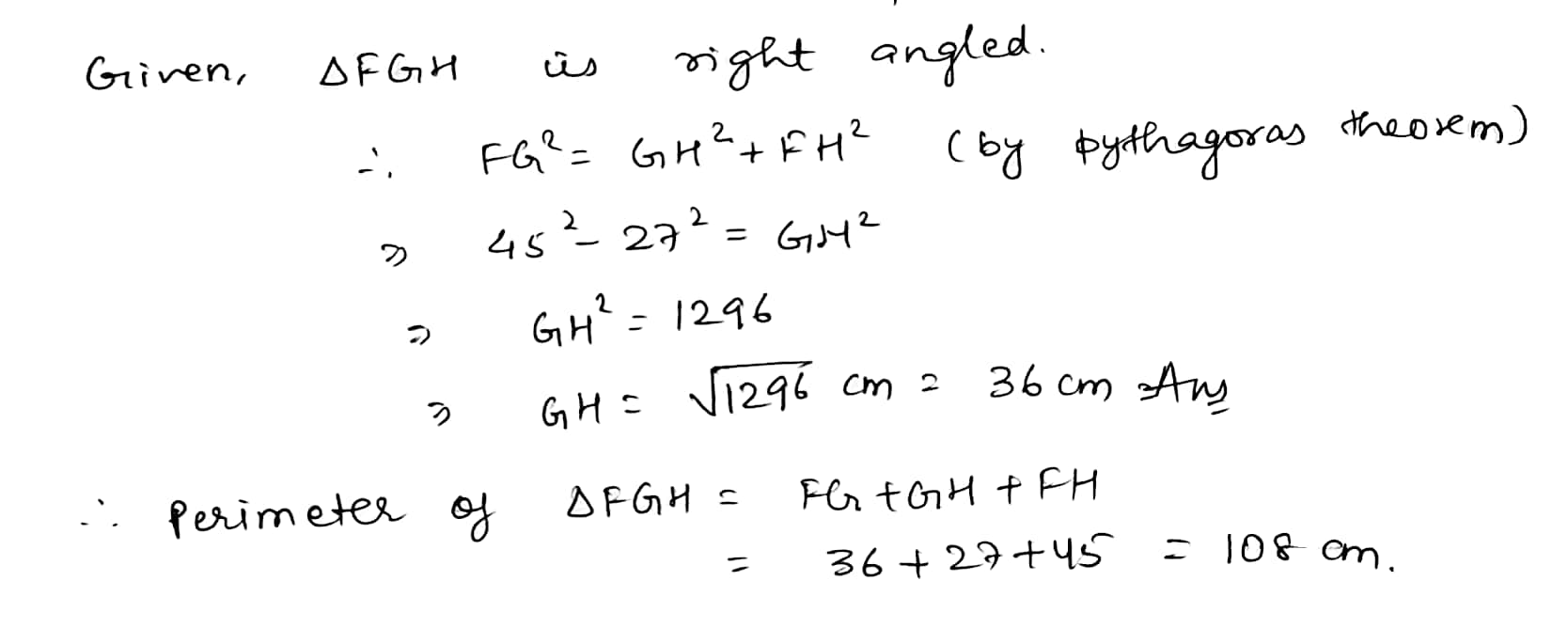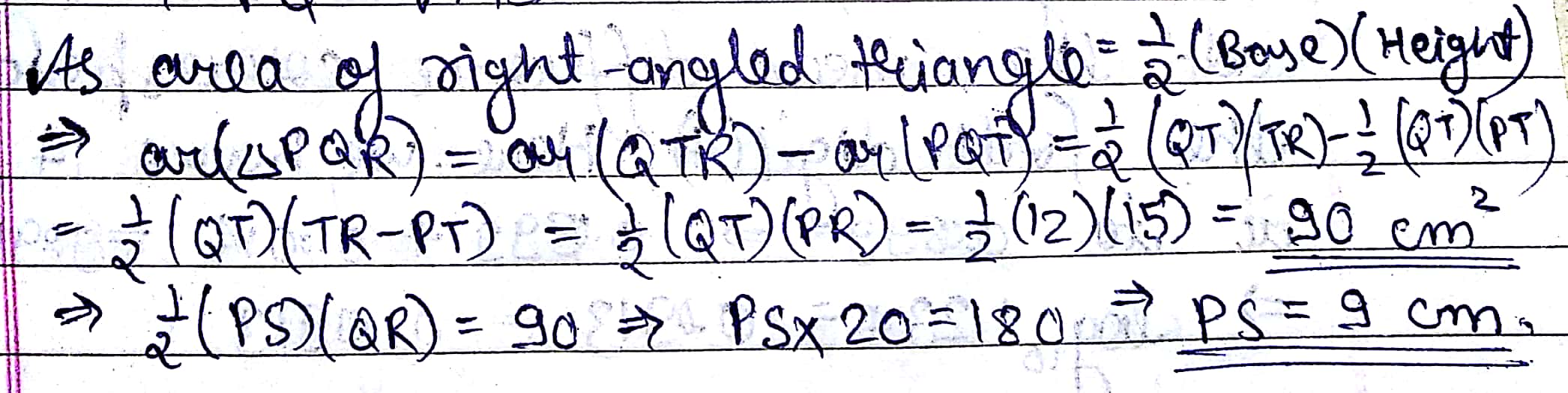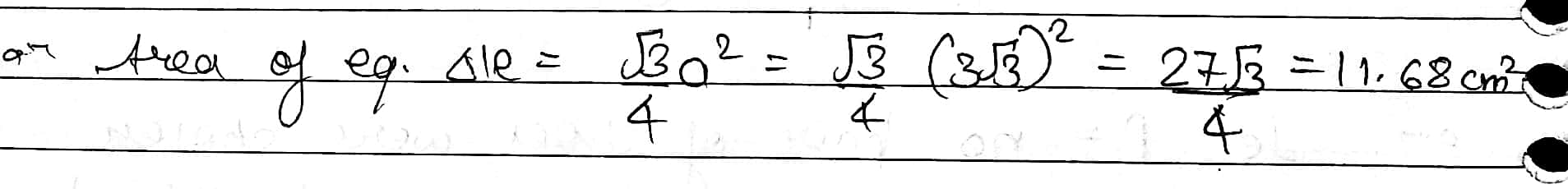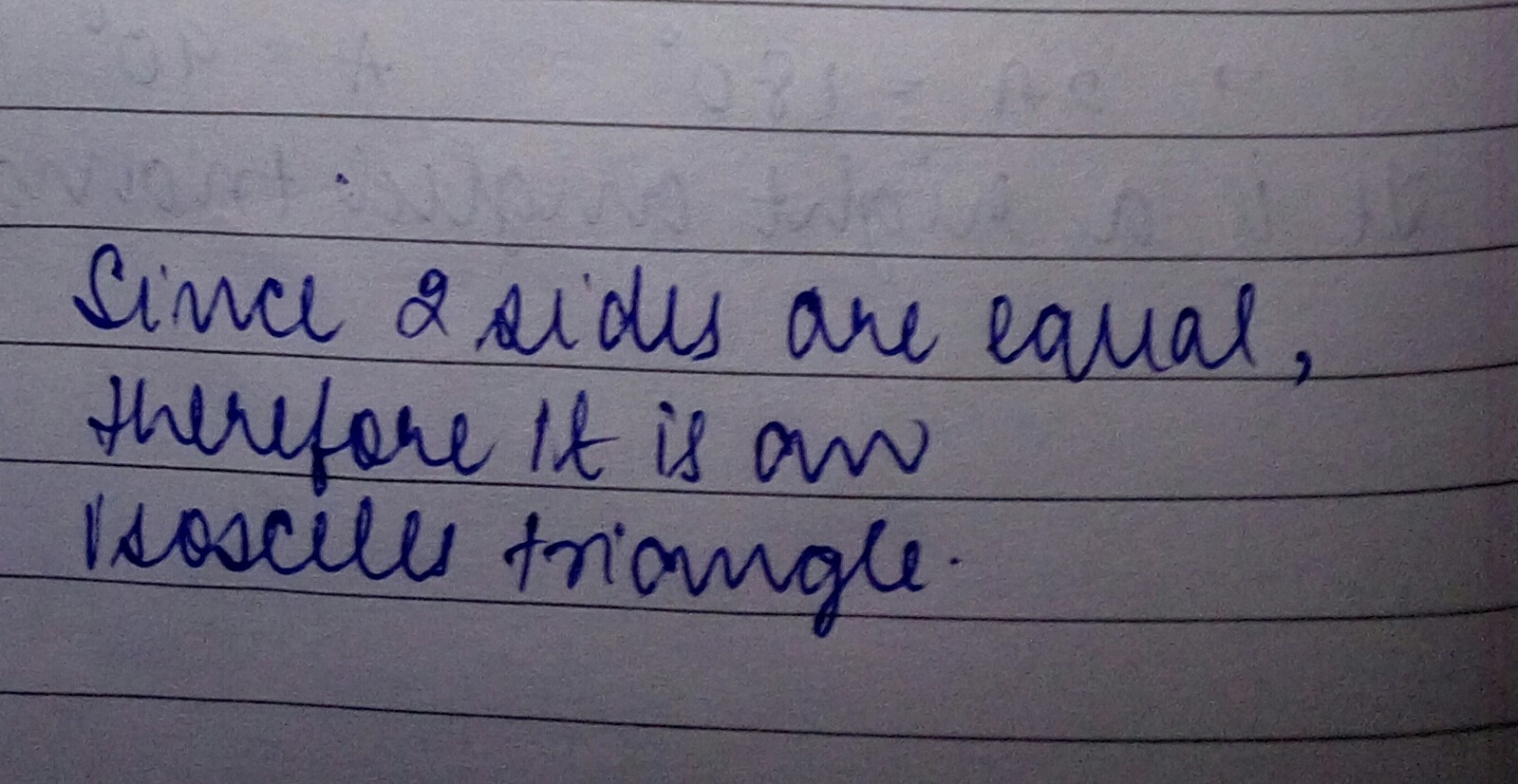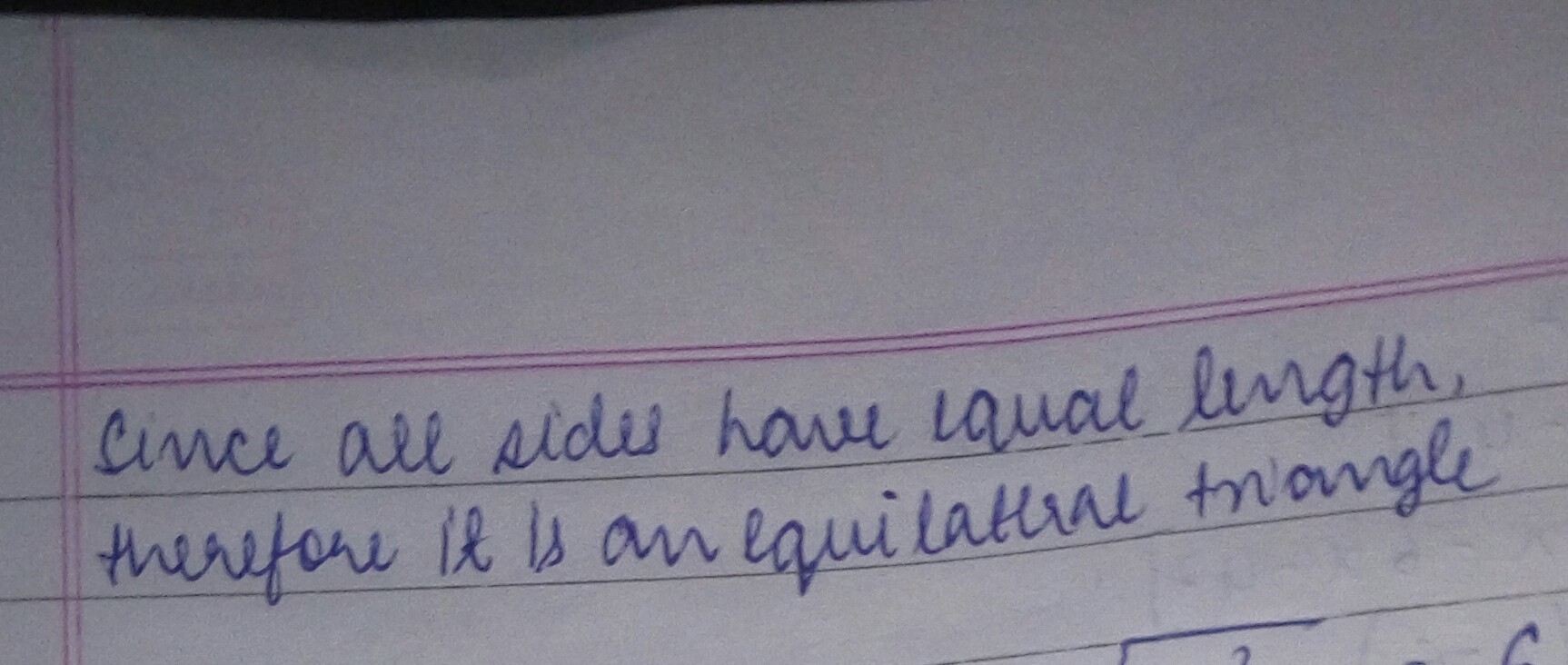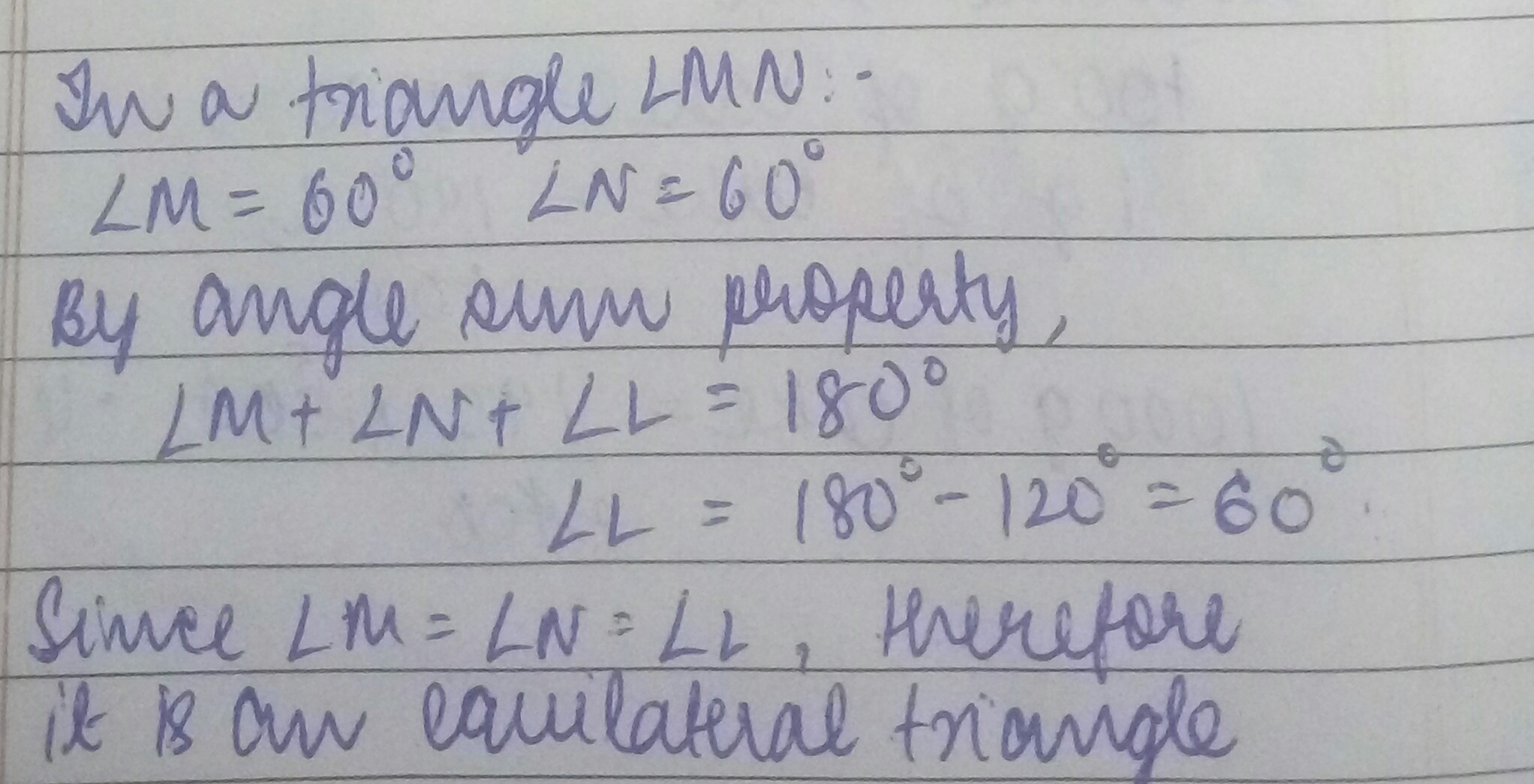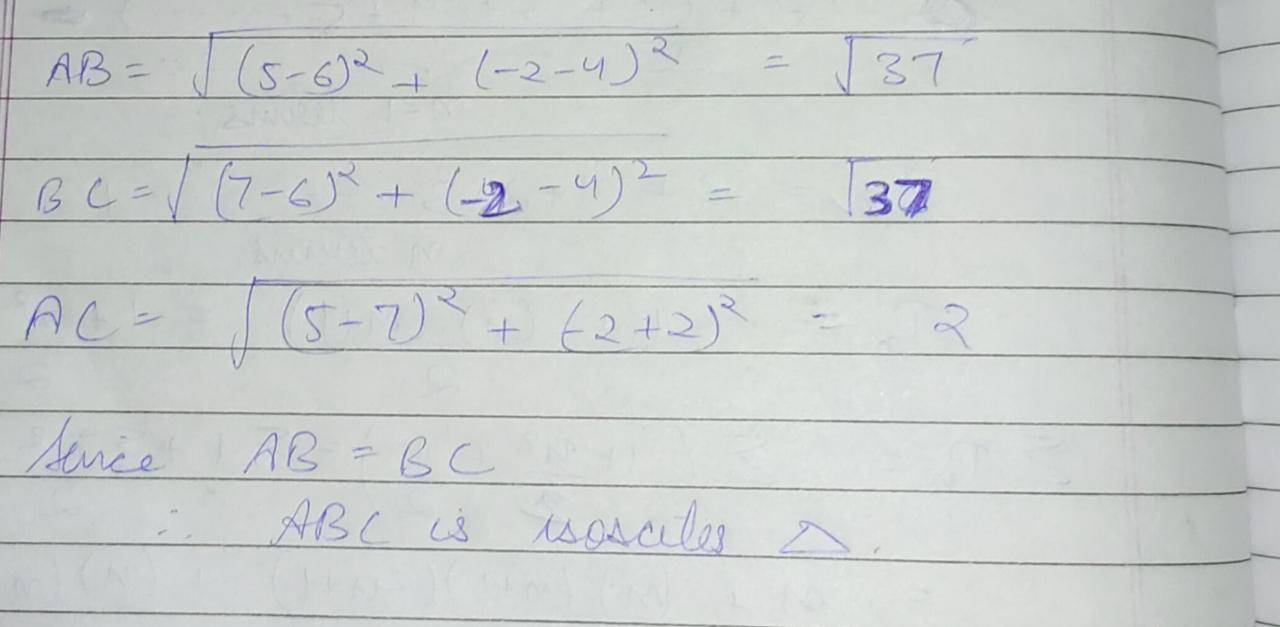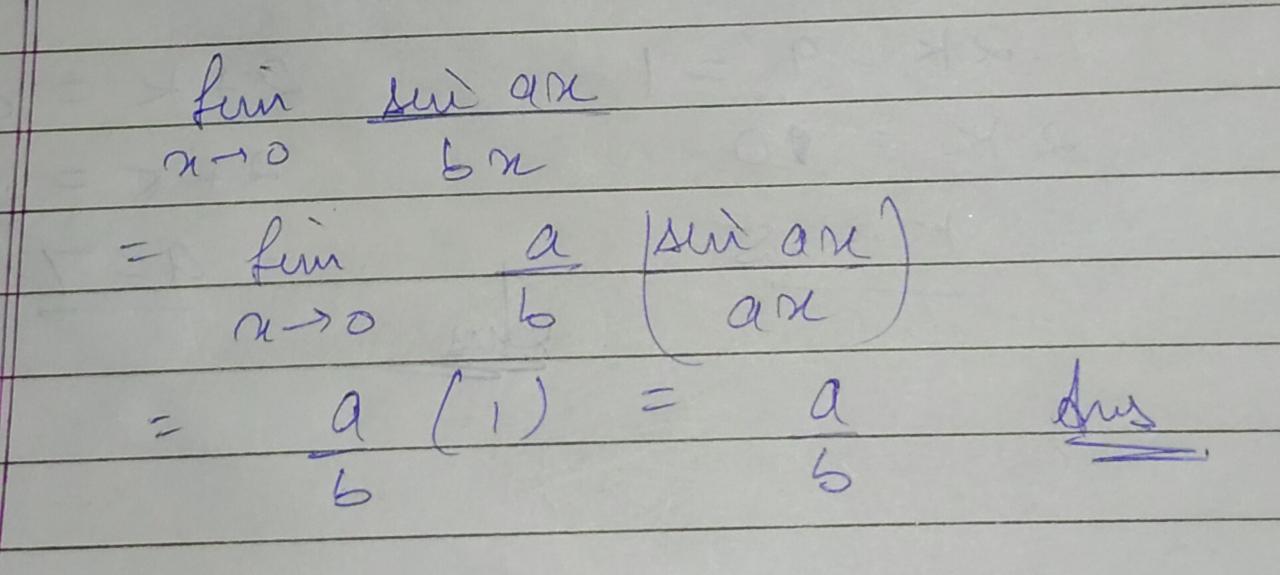The Triangle And Its Properties - Class 7 Maths - Extra Questions
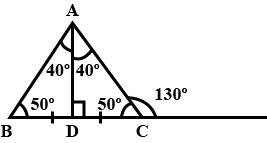
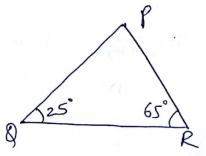
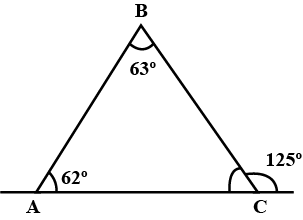
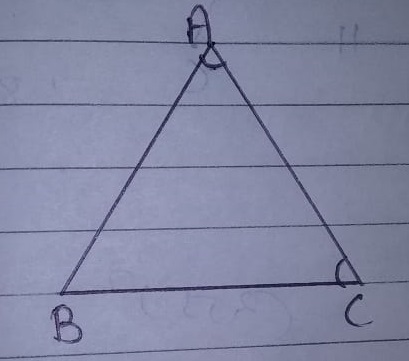
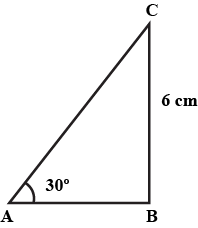
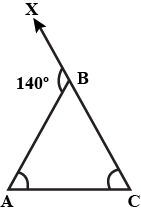
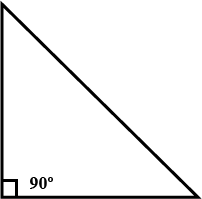
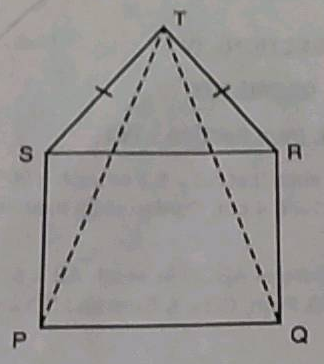
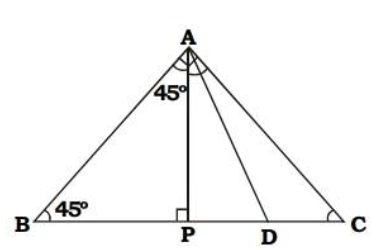
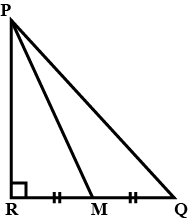
In Fig., there is a triangle. The measures of some angles have been indicated. State whether triangle is acute, right or obtuse.
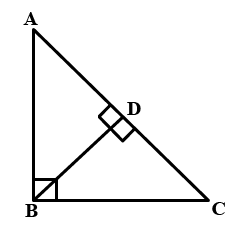
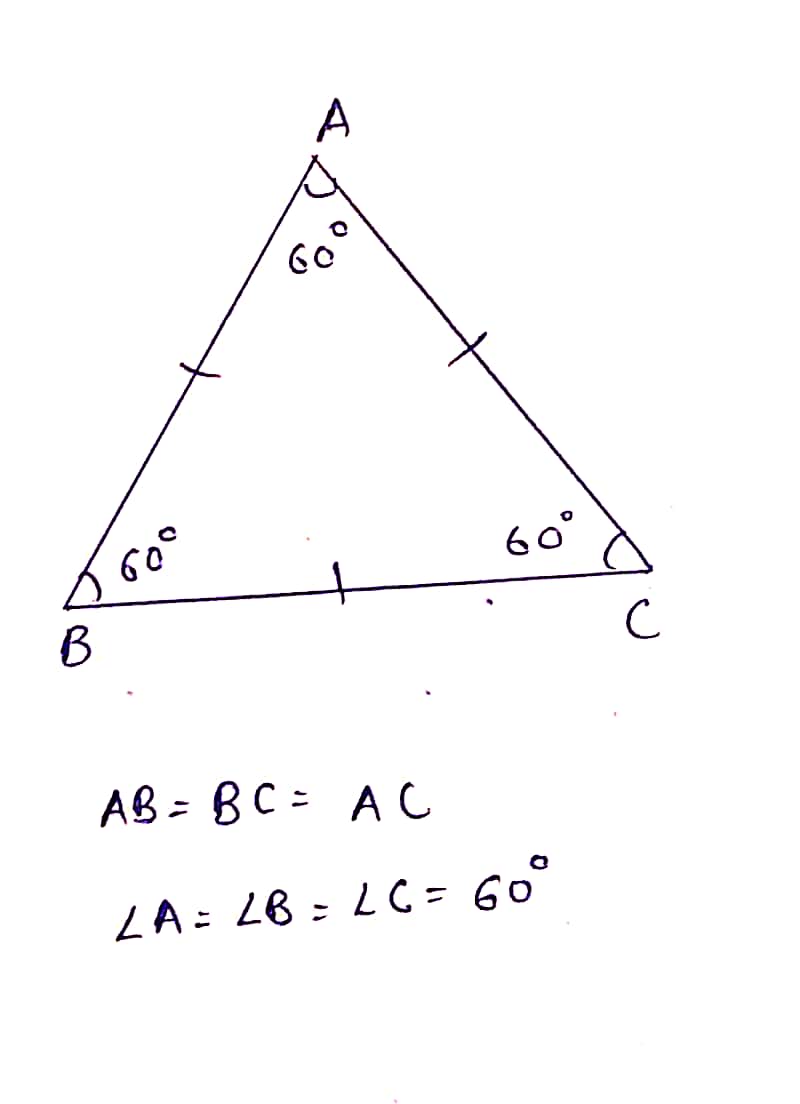
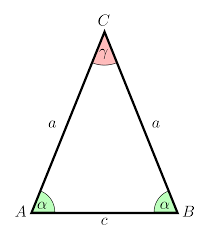
The diagram shows an isosceles triangle.
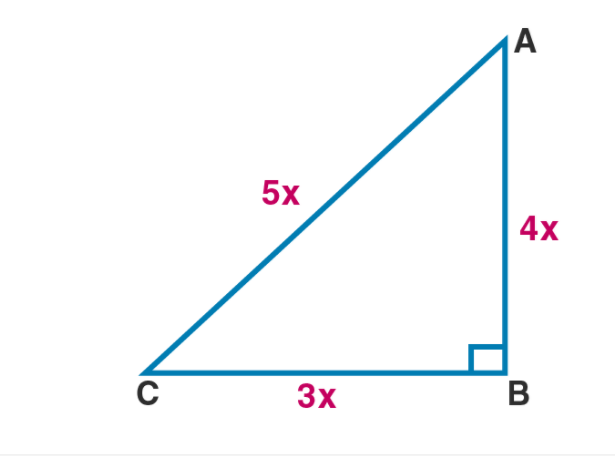
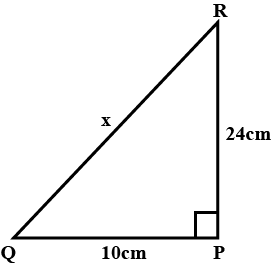
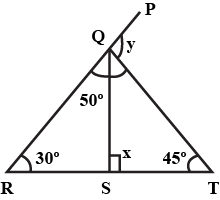
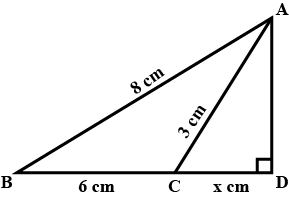
Find the value of x.

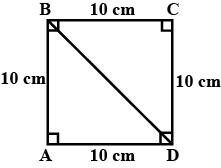
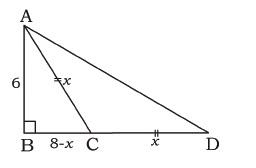
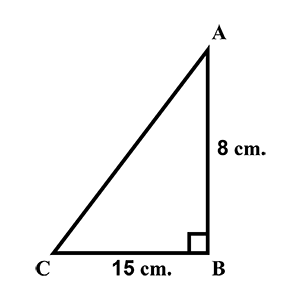
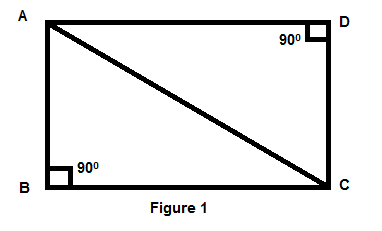
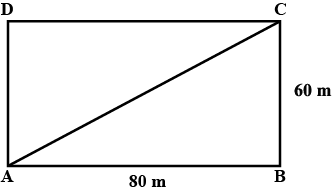
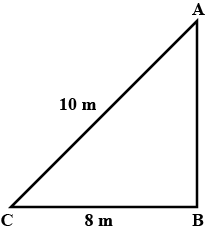
Find the length of the diagonal of a rectangle whose sides are to 10 cm and 8 cm.
Construct the following triangle :
(i) Equilateral ΔXYZ with XY=YZ=XZ=5cm.
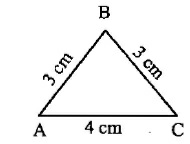
The lengths of sides of a triangle are 3 cm,4 cm and 5 cm. Is the triangle a right angle? Give reason.
Identify the triangles as equilateral, isosceles or scalene.Answer: Isosceles
Mark answer as 1 if true else 0 if false
Mark answer as 1 if true else 0 if false
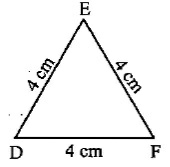
Identify the triangles as equilateral, isosceles or scalene.Answer: Equilateral
Mark answer as 1 if true else 0 if false
Mark answer as 1 if true else 0 if false
Identify the triangles as equilateral, isosceles or scalene.
Answer : Equilateral
Mark answer as 1 if true else 0 if false

Mark answer as 1 if true else 0 if false
Identify the triangles as equilateral, isosceles or scalene.
Answer: Scalene
Mark answer as 1 if true else 0 if false
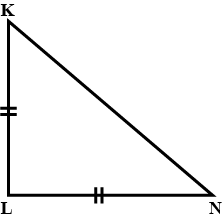
Mark answer as 1 if true else 0 if false
Identify the triangle as equiangular, acute, obtuse or right.
Answer: Equiangular
Mark answer as 1 if true else 0 if false
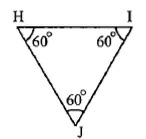
Mark answer as 1 if true else 0 if false
Identify the triangle as equiangular, acute, obtuse or right.
Triangle is obtuse angled.Mark answer as 1 if true and 0 if false.
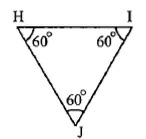
Mark answer as 1 if true and 0 if false.
One angle of an isosceles obtuse-angled triangle is 35∘. Find the measure of its obtuse angle in degrees.
Each angle of equilateral triangle is 600. If true enter 1 else 0.
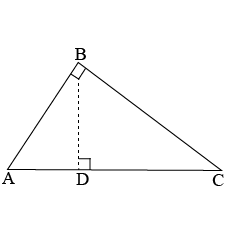
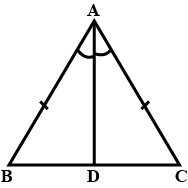
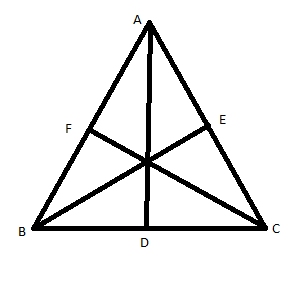
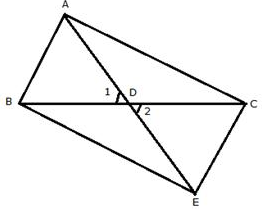
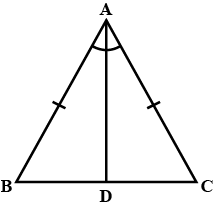
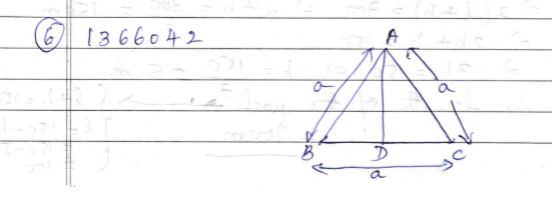
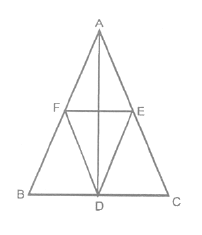
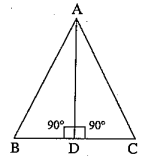
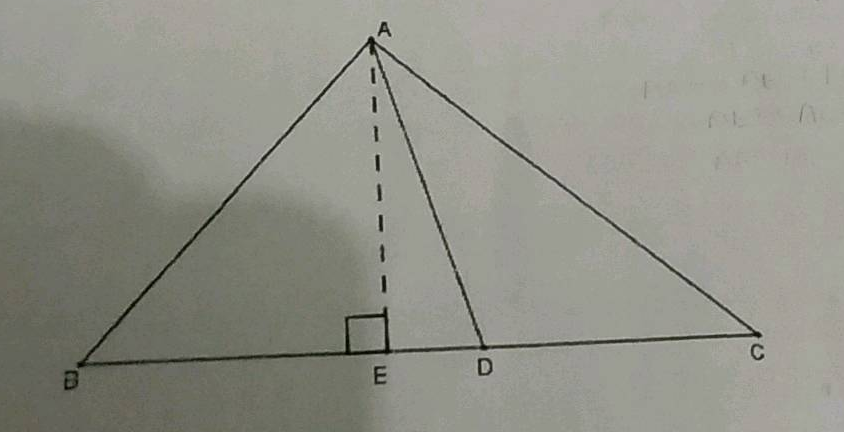
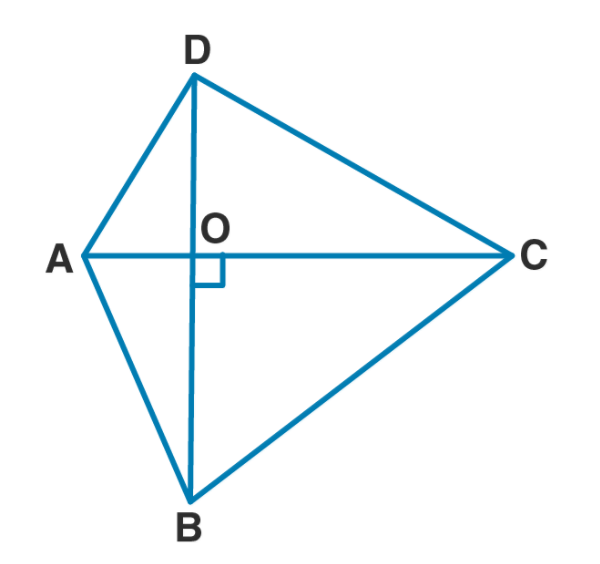
△ABC is an isosceles triangle such that AB = AC Then prove that altitude AD from A on BC bisects BC
Based on the sides, classify the following triangles
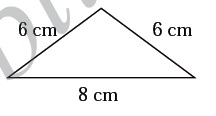
Based on the sides, classify the following triangles
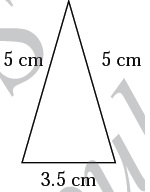
Based on the sides, classify the following triangles
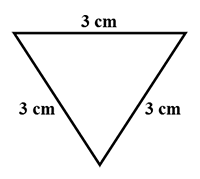
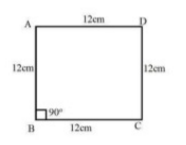
Find the diagonal of a square whose side is 10 cm
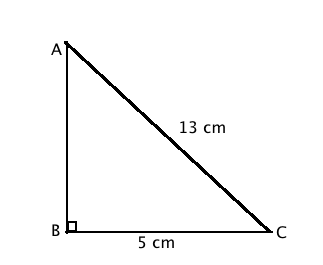
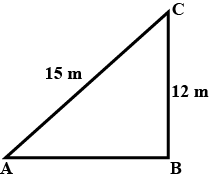
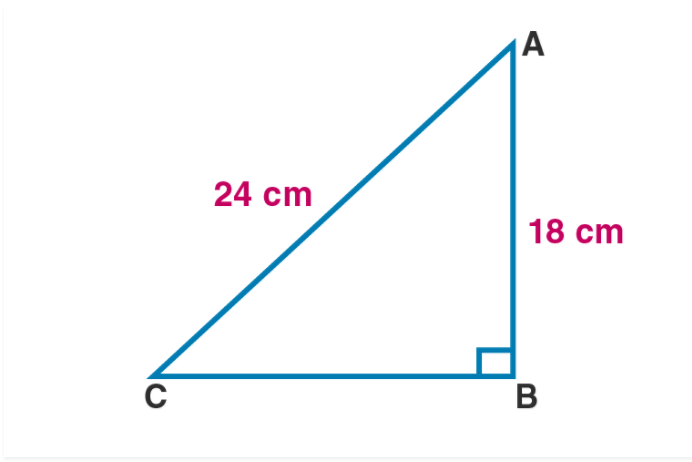
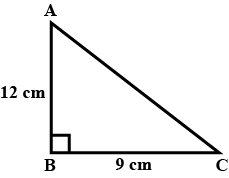
Write the length of the hypotenuse of the following right angled △ABC.

In △ABC, C is a point on BD such that BC:CD=1:2 and △ABC is an equilateral triangle. Prove that AD2=7AC2
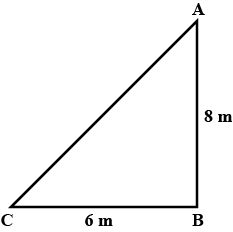
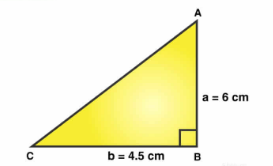
If the sides a triangle are 6 cm,8 cm and 10 cm respectively, determine whether the triangle is right angled triangle or not.
In △ABC, AC2=AB2+BC2, then right angle is at ___________.
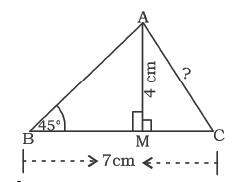
In △ABC, ABC=45o, AM⊥BC, AM=4cm and BC=7cm. Find the length of AC.
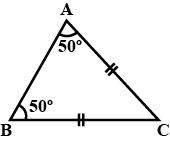
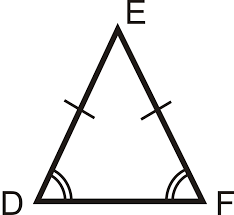
In ΔABC, ∠A=∠B=500. Name the pair of sides which are equal.
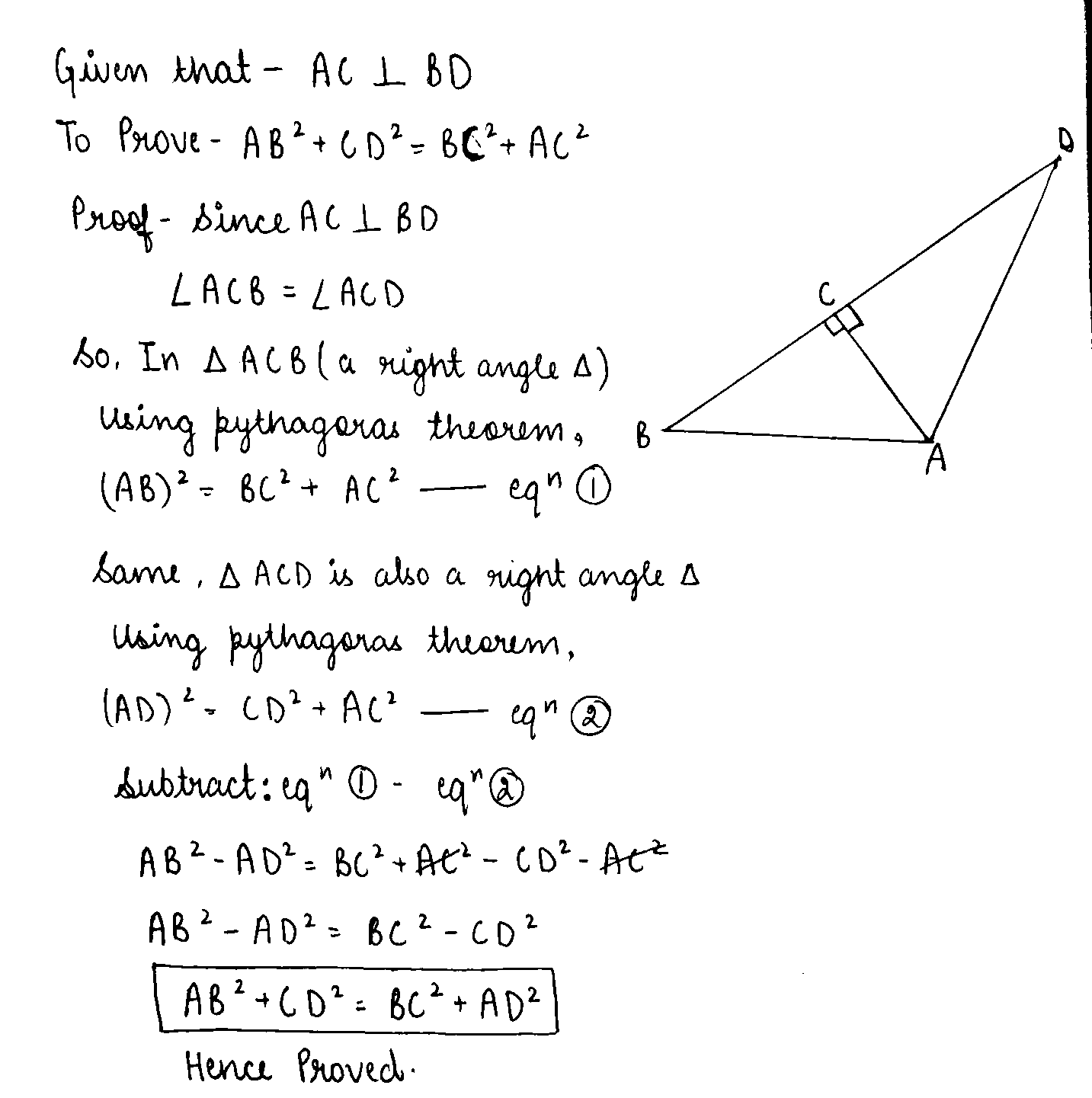
In the given figure, AD⊥BC, Prove that AB2+CD2=BD2+AC2
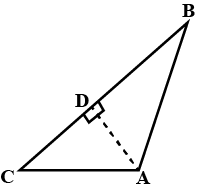
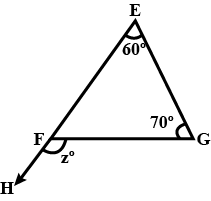
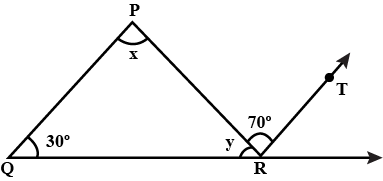
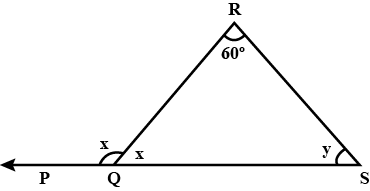
In the given triangles, find ∠z
Check whether the following can be the sides of a right angled triangle:
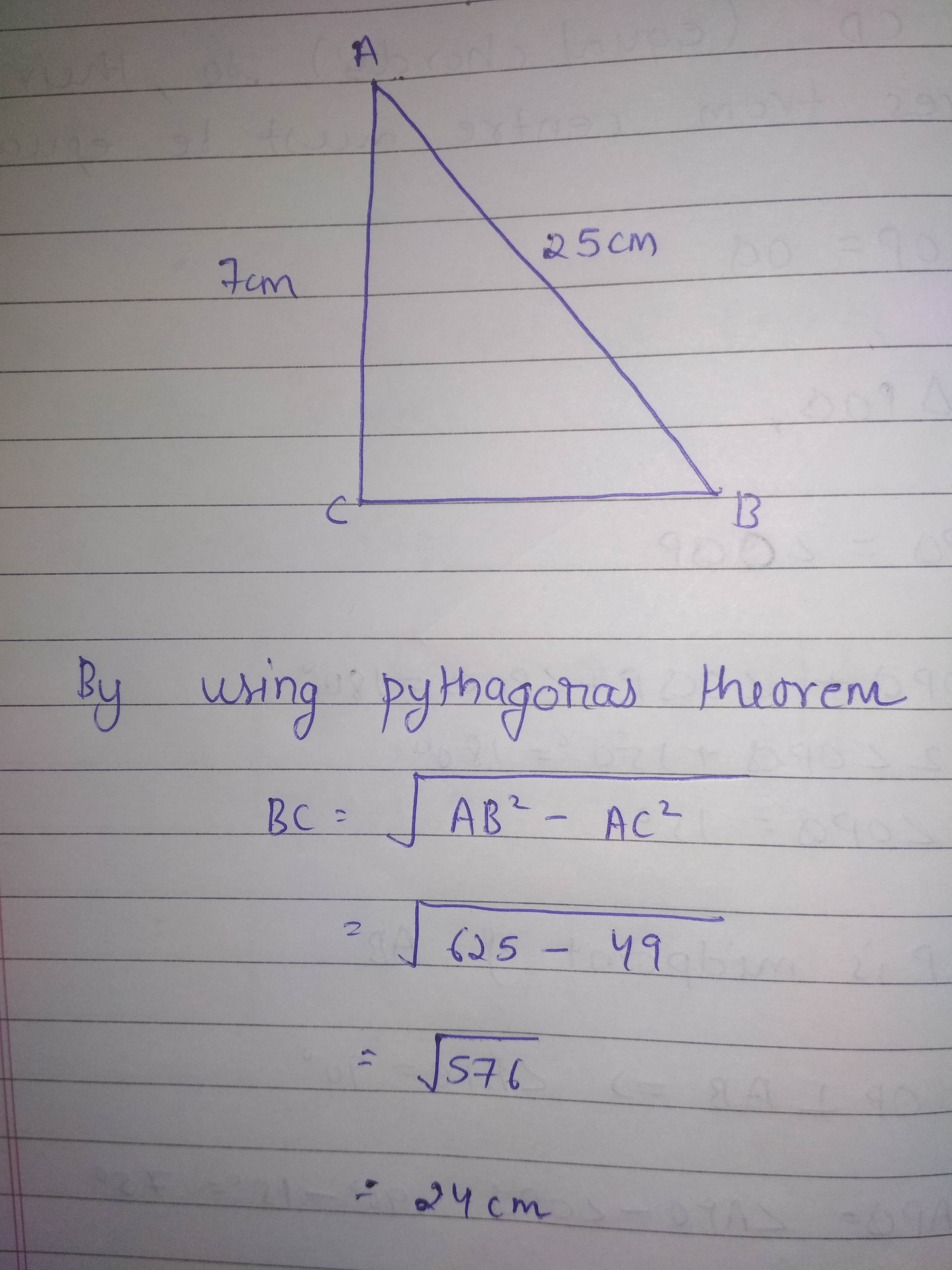
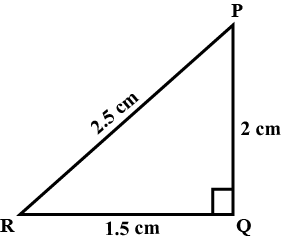
AB=25 cm,BC=24 cm,AC=7 cm.
In △ABC,m∠B=90o. Prove that AC2=AB2+BC2.
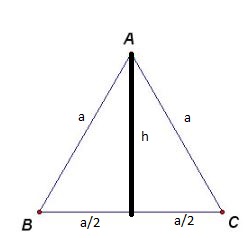
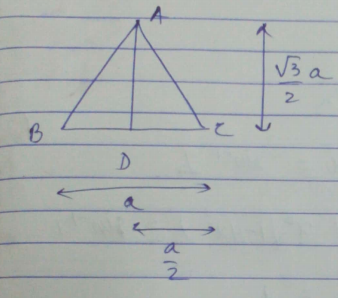
Find the height of an equilateral triangle whose side is 6 units.
Prove that in an equilateral triangle, three time the square of a side is equal to four time the square of its altitude.
Write the pythogorean triplet whose one member is 16.
If a:b:c=13:5:12, then prove that the triangle is right angled at A.
An isosceles triangle has perimeter 30 cm and length of its congruent sides is 12 cm. Find the area of the triangle.
Prove that the cubic equation whose roots are the radii r1,r2,r3 of escribed circles of a triangle is t3−(r+4R)t+s2t−s2r=0
State the Pythagoras theorem.
If the sides of triangle are 3 cm,4 cm and 6 cm long, determine whether the triangle is a right-angled triangle.
State Pythagoras theorem and its converse.
Look at the figure and complete the given statements.
(a) Interior opposite angles corresponding to the exterior angle ∠5 are ______and ____.
(b) Interior adjacent angle corresponding to ∠6 is_______
(c) The exterior angle at the vertex R is _____
(d) The interior opposite angles corresponding to the exterior angle ∠6 are _______ and ____
(e) Interior angle adjacent angle corresponding to the exterior angle at vertex P is_____

An isosceles right angled triangle has area 8cm2. Find the length of its hypotenuse.
if the area of an equalatral Δ is 36√3cm2. Find its hight.
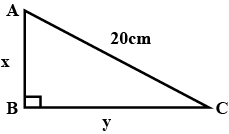
Traingle ABC is right angled at C. If AB is 20 cm and BC is 12 cm, find AC.
Triangles in which all the three sides are of equal length are called ............triangle.
An ..............angle of a triangle is equal to the sum of its two interior opposite angle.
Find a pythagorean triplet whose smallest member is 12.
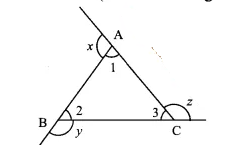
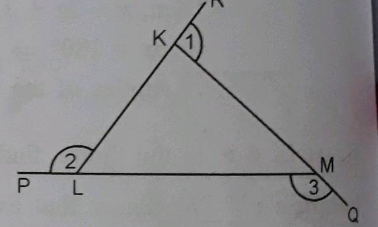
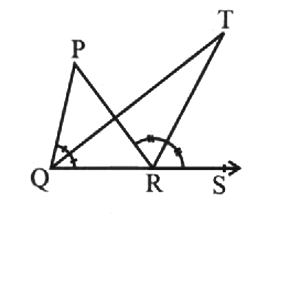
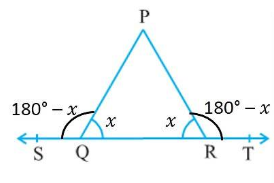
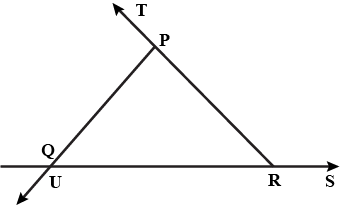
If the sides of △KLM are produced to P,Q and R as shown, find the sum of exterior angles ∠1,∠2 and ∠3.
Calculate the area and the height of an equilateral triangle whose perimeter is 60 cm.
In an isosceles triangle, the vertex angle is 50∘. What are the base angles of the triangle?
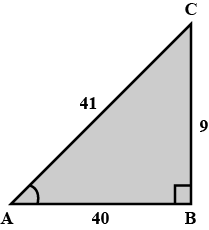
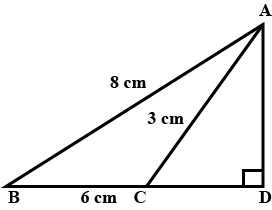
ABC is a triangle right-angled at C . If AB = 25 cm and AC = 7 cm , find BC .
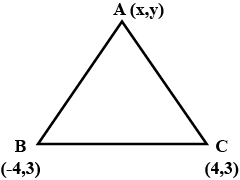
If (-4, 3) and (4, 3) are two vertices of an equilateral triangle, find the co-ordinates of the third vertex.
Show that the points (-1,6) and (-4,9) and (0,7) form an isosceles triangle.
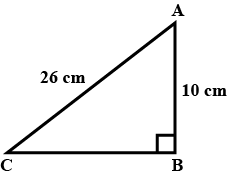
The hypotenuse of a right angled triangle is 26\ cm . If the sum of the other two sides is 34\ cm, find the other two sides.
From the given figure calculate :
\angle ADC
Prove that the bisector of the vertical angle of an isosceles triangle bisects the base at right angles.
Length of the hypotenuse of a right-angled triangle is 61\ cm. Its one side is 11\ cm. Find the length of the other side.
Two verities of an equilateral triangle are (-1,0) and (1,0) and its third vertex lies above the x-axis. The equation of its circumcircle is________
The altitude of a triangle is 7 cm less then its base. If the hypotenuse is 13 cm, Find the other two sides.
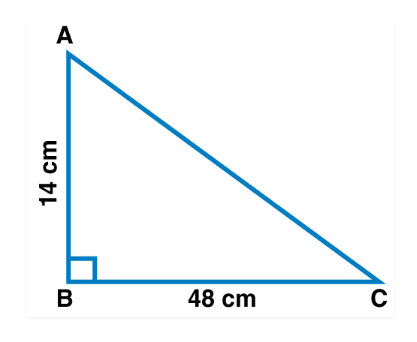
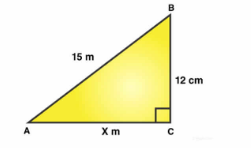
Find the length of the hypotenuse of a right triangle, the other two sides of which measure 9\ cm and 12\ cm.
If a and b are real number between 0 and 1 such that the point (a,1),(1,b) and (0,0) from an equilateral triangle find a and b.
Show that the angles of an equilateral triangle are {60^ \circ } each
Construct an isosceles \Delta A B C such that :
(i) base A B = 4.2 cm , base angle = 30 ^ { \circ }.
In a right triangle, the sides are 12cm,16cm find its hypotenuse.
Determine whether 50\ cm,80\ cm,100\ cm can be the sides of a right triangle or not.
Define Isosceles triangle.
In triangle ABC,AD is perpendicular to BC.Prove that:AB^2-AC^2=BD^2-CD^2
One of the two equal angles of an isosceles triangle measures 55^{\circ} determine the other angles.
Find the area of an equilateral triangle of side 20\ m.
Find the length of hypotenuse if 12 adn 5 are other sides of right angles triangle
Show that the angle of an equilateral triangle are 60^ {o} each.
If the 2 sides of right angles triangle is 7,24 find the hypotenuse.
In an equilateral triangle of side 3\sqrt 3 , find the length of the altitude.
In an equilateral triangle of side 3 \sqrt{3} \mathrm{cm}, find the length of the altitude.
in a right triangle the hypotenuse is the .....side
Write the definition of Pythagoras theorem.
A piece of wire is 36 cm long what will be the be length of each side if we form an equilateral triangle.
The sides of right angles triangle are 63,9 find its hypotenuse .
State Pythagoras theorem.
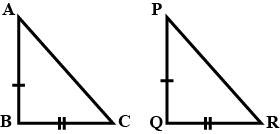
Name the following triangles with regards to sides :

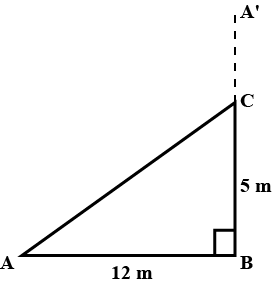
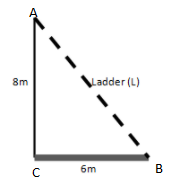
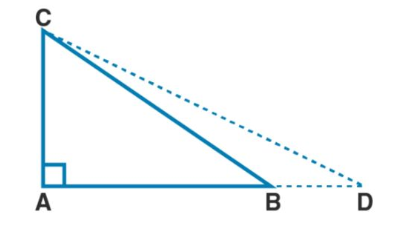
Height of a pole is 8 m. Find the length of rope tied with its top from a point on the ground at a distance of 6 m from its bottom. Solution: Let ABC be the right-angled triangle? Give reason
Jiya walks 6km due east and then 8km due north. How far is she from her starting place ?
The height of a pole is 8\ m. Find the length of rope tied with its top from a point on the ground at a distance of 6\ m from its bottom.
In the given figure, find the length of AD in terms of b and c.
Solve the question :
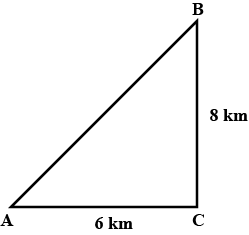
FGH is a right-angled triangle
Calculate the perimeter of the triangle.
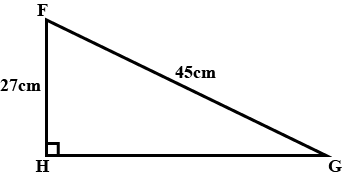
Solve the question :
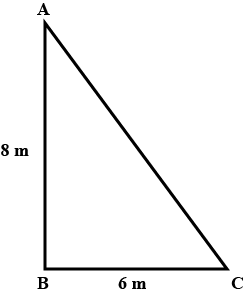
FGH is a right-angled triangle
Calculate the area of the triangle

Classify the following triangles according to sides:
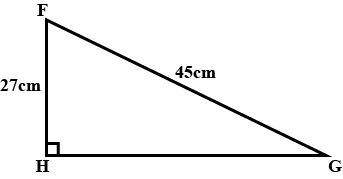
Solve the question :
FGH is a right-angled triangle.
Calculate GH
In a right angled triangle ABC, \angle B = { 90 }^{ \circ }.
If AC = 13\ cm, BC = 5\ cm, find AB
4 PM^{2} = 4 PQ^{2} + QR^{2}
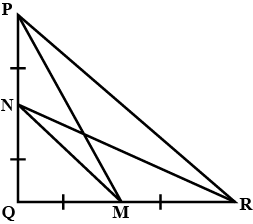
4 RN^{2}= PQ^{2} + 4QR^{2}
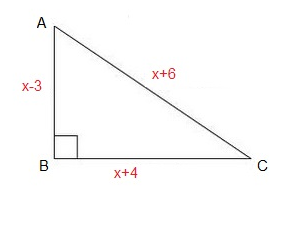
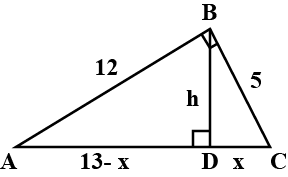
In \Delta ABC, \angle B=90^{\circ}, Find the sides of the triangle, if AB = \left ( x-3 \right )\ cm, BC = \left ( x+4 \right )\ cm and AC = \left ( x+6 \right )\ cm.
In triangle \displaystyle ABC,\angle B = 90^{\circ} and D is the mid-point of side Be. Prove that :
\displaystyle AC^2=AD^2+3CD^2
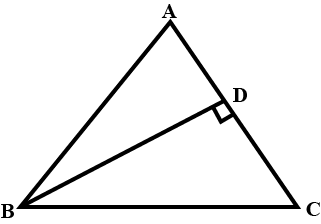
In an isosceles triangle ABC with AB=AC, BD is perpendicular from B to side AC. Prove that BD^{2}-CD^{2}=2CD.AD
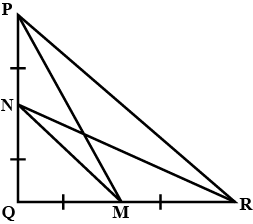
In a triangle ABC, let D be a point on the segment BC such that AB + BD = AC + CD. Suppose that the points B,C, and the centroids of \Delta ABD & \Delta ACD lie on a circle. Prove that AB = AC.
Using converse of Pythagoras theorem, check whether the sides form a right angled triangle 13, 15 and 16?
Using converse of Pythagoras theorem, check whether the sides form a right angled triangle 63, 65 and 16?
In the figure, \angle PQR=\angle PRQ, then prove that \angle PQS=\angle PRT.
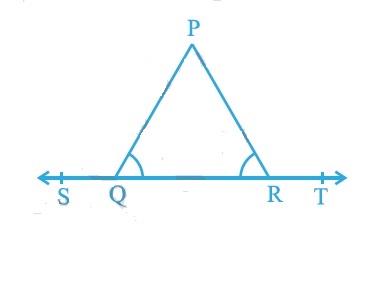
Which side is the hypotenuse for the sides, 48, 73 and 55 in a right angled ? (Apply Converse of Pythagoras theorem).
Check whether the sides form a right angled triangle 35, 12 and 34? (Apply Converse of Pythagoras theorem).
Check whether the sides form a right angled triangle 65, 72 and 97? (Apply Converse of Pythagoras theorem).
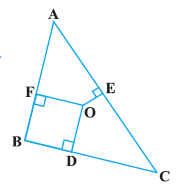
In the above figure, O is a point in the interior of a triangle ABC, OD \bot BC, \,OE \bot AC and OF\bot AB. Show that:
(i) OA^2 + OB^2 + OC^2 -OD^2 -OE^2 -OF^2 = AF^2 + BD^2 + CE^2
(ii) AF^2 + BD^2 + CE^2 = AE^2 + CD^2 + BF^2
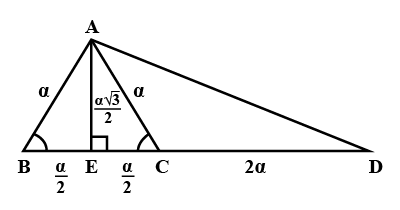
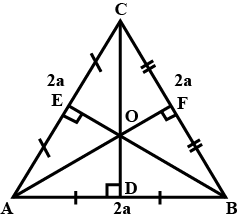
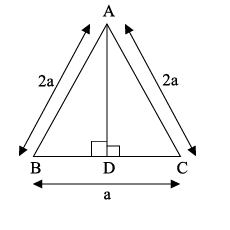
ABC is an equilateral triangle of side 2a. Find each of its altitudes.
In Figure, the side QR of \triangle PQR is produced to a point S. If the bisectors of \angle PQR and \angle PRS meet at point T, then prove that \angle QTR=\dfrac { 1 }{ 2 } \angle QPR.
The altitudes of \Delta ABC, AD, BE and CF are equal. Prove that \Delta ABC is an equilateral triangle.
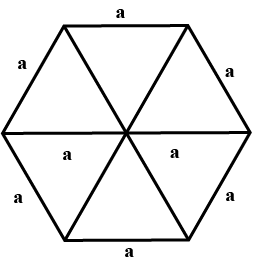
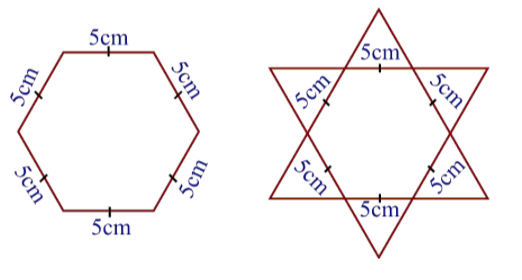
Complete the hexagonal and star shaped Rangolies [see Fig. (i) and (ii)] by filling them with as many equilateral triangles of side 1 cm as you can. Count the number of triangles in each case. Which has more triangles?
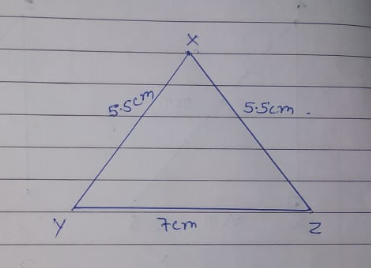
Construct \triangle XYZ with YZ = 7 cm,\, XY = 5.5 cm and XZ =5.5 cm.
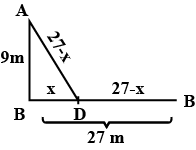
An insect 8 m away from the foot of a lamp post which is 6 m tall, crawls towards it. After moving through a distance, its distance from the top of the lamp post is equal to the distance it has moved. How far is the insect away from the foot of the lamp post? [Bhaskaracharya's Leelavathi]
In a right angled \triangle ABC, \angle B = {90}^{o}, AC = 17 cm and AB = 8 cm, find BC.
The sides of a right angled triangle containing the right angle are 5 cm and 12 cm, find its hypotenuse.
In the rectangle WXYZ, XY + YZ = 17 cm and XZ + YW = 26 cm, calculate the length and breadth of the rectangle.

Find the length of the diagonal of a square of side 12\ cm.
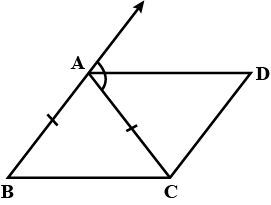
ABC is an isosceles triangle in which AB = AC. AD bisects exterior angle QAC and CD\parallel BA as shown in the figure. Show that \angle DAC = \angle BCA
In an equilateral triangle ABC, the side BC is trisected at point D. Prove that 9{AD}^{2}=7{AB}^{2}. (Hint : Draw AE \perp BC).
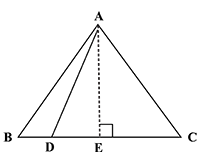
If the bisector of an angle of a triangle bisects the opposite side, prove that the triangle is isosceles.
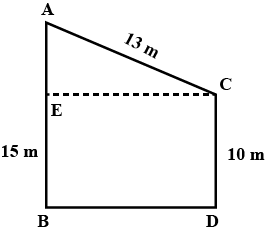
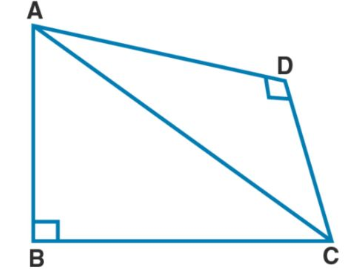
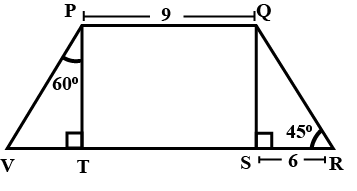
In the following figure, quadrilateral PQRV is a trapezium in which PQ \parallel VR, SR = 6\text{ cm}, PQ = 9\text{ cm}, then find VR.
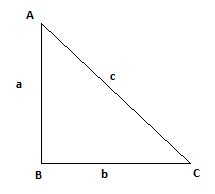
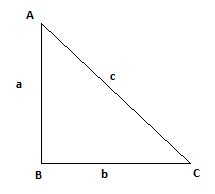
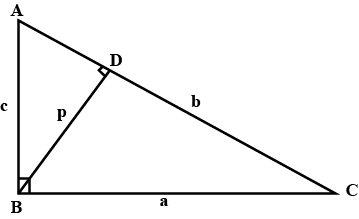
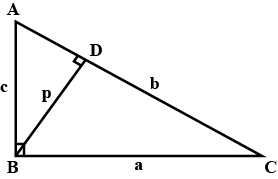
In \triangle ABC, \angle ABC = {90}^{o}, BD \perp AC. If AB = 'c' units, BC = 'a' units, BD = 'p' units, CA = 'b' units.
Prove that \dfrac{1}{{a}^{2}} + \dfrac{1}{{c}^{2}} = \dfrac{1}{{p}^{2}}.
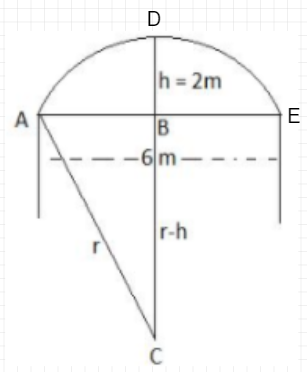
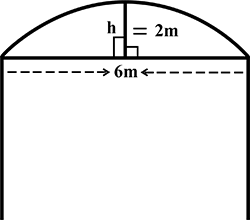
A door of width 6 meter has an arch above it having a height of 2\ m. Find the radius of the arch.
Find the angles of the triangle ABC, given in the figure.
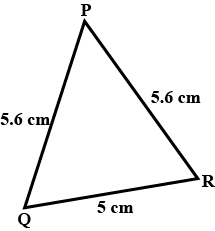
\angle Q and \angle R of a triangle PQR are 25^o and 65^o. Is \triangle PQR a right angled triangle?
Moreover PQ is 4 cm and PR is 3 cm. Find QR.
State Pythagoras theorem.
In the given figure, \dfrac{PK}{KS} = \dfrac{PT}{TR} and \angle PKT = \angle PRS. Prove that \Delta PSR is an isosceles triangle.

PQR is a triangle right angled at P. If PQ = 10\ cm and PR = 24\ cm, find QR.
Are the numbers 12, 5 and 13 form a Pythagorean Triplet?
Show that the following points form an isosceles triangle.
(1, -2), (-5, 1) and (1, 4)
Show that the following points form an isosceles triangle.
(2, 3), (5, 7) and (1, 4)
Show that the following points form an isosceles triangle.
(-1, -3), (2, -1) and (-1, 1)
Show that the following points form an equilateral triangle. (a, 0), (-a, 0) and (0, a\sqrt 3)
Show that the following points form an isosceles triangle.
(1, 3), (-3, -5) and (-3, 0)
Construct \triangle ABC with AB=5 cm,\, BC =5 cm and AC= 5 cm.
Show that the following points form an equilateral triangle. (\sqrt 3, 2), (0, 1) and (0, 3)
In a right angled triangle, the length of perpendicular and base are 8\ cm and 15\ cm respectively, then find its hypotenuse.
If d and e are points on sides AB and AC respectively of a \triangle {abc} such that DE\parallel BC and BD=CE. Prove triangle abc is isosceles.
D, E, and F are, respectively the mid-points of sides BC, CA and AB of an equilateral triangle ABC. Prove that DEF is also an equilateral triangle.
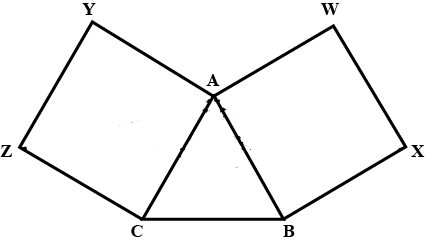
In the given figure, \triangle ABC is an equilateral triangle and \square AWXB and \square AYZC are two squares. The value of \dfrac{1}{10}(\angle ZXA) is:
"An equilateral triangle is a polygon made up of three line segments out of which two line segments are equal to the third one and all its angles are 60^o each." Define the terms used in this definition which you feel necessary. Are there any undefined terms in this? Can you justify that all sides and all angles are equal in a equilateral triangle
Find the length of the altitude of an equilateral triangle with side 6cm.
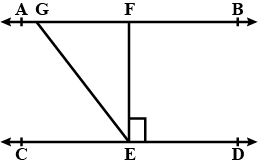
In the fig. If AB || CD, EF \perp CD and \angle GED = 126^0, find \angle AGE , \angle GEF and \angle FGE.
A point D is on the side BC of an equilateral triangle ABC, such that DC =\cfrac { 1 }{ 4 } BC. Prove that { AD }^{ 2 }={ 13CD}^{ 2 }.
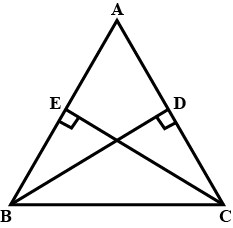
In the given figure, \triangle ABC is an isosceles triangle in which AB-AC. If BD\bot AC and CE\bot AB, prove that BD=CE.
In a quadrilateral ABCD, given that \angle A+ \angle D = 90^{\circ}. Prove that { AC }^{ 2 }+{ BD }^{ 2 }={ AD }^{ 2 }+{ BC}^{ 2 }.
A man goes 15 metres due west and then 8 metres due north. How far is he from starting point?
The sides of certain triangles are given below. Determine which of them are right angled triangles.
(i) a = 7 cm, b = 24 cm and c = 25 cm
(ii) a = 9 cm, b = 16 cm and c = 18 cm
(iii) a = 1.6 cm, b = 3.8 cm and c = 4 cm
(iv) a = 8 cm, b = 10 cm and c = 6 cm
In given figure, \dfrac { PS }{ SQ } =\dfrac { PT }{ TR } and \angle PST=\angle PRQ. Prove that \triangle PQR is an isosceles triangle.

The hypotenuse of a right triangle is 3\sqrt { 5 } cm. If the smaller side is tripled and the larger side is doubled, the new hypotenuse will be 15cm. Find the length of each side.
In given figure equilateral triangles are drawn on the sides of a right triangle. Show that the area of the triangle on the hypotenuse is equal to the sum of the areas of triangles on the other two sides.
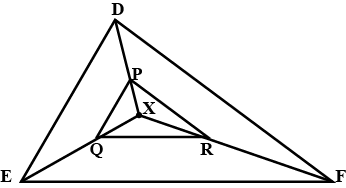
In the figure , X is any point in the interior of triangle . Point x is joined to the vertices of triangle
seg{\rm{ }}PQ\parallel seg{\rm{ }}DE
seg{\rm{ }}PR\parallel seg{\rm{ }}DFproove:seg{\rm{ }}QR\parallel seg{\rm{ }}EF
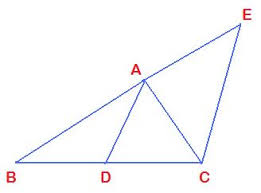
In \triangle ABC, AD is perpendicular to BC. Prove that :{ AB }^{ 2 }+{ CD }^{ 2 }={ AC }^{ 2 }+{ BD }^{ 2 }

Find the altitude of an equilateral triangle if one of its sites measures 10\sqrt 3 cm
In the given figure, \triangle ABC is an isosceles with AB = AC, D and E are points on BC such that BE = CD. show that AD=AE.

Show that the median to the base of an isosceles triangle is perpendicular to base.
If \Delta ABC is an equilateral triangle of side a and D is a point on BC such that BD = \dfrac{1}{3}BC then the prove that AD = \dfrac{{\sqrt 7 a}}{3}
If \cos A + \cos B+ \cos C = \dfrac{3}{2}, then show that the triangle is equilateral.
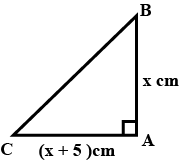
In triangle ABC ; Angle A =90^{0} , side AB = x cm, AC = (x+5) cm and area =150 cm ^{2}. Find the sides of triangle.
Length of diagonals of a rhombus ABCD are 16cm and 12cm. Find the side and perimeter of the rhombus.
In the figure, AD is the internal bisector of \angle A and CE\parallel DA. If CE meets BA produced at E, prove that \Delta CAE is isosceles.
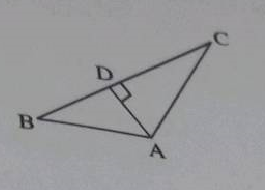
If AD \bot BC, prove that AB^{2}+CD^{2}=BD^{2}+AC^{2}
In triangle ABC, BC is produced to D, A & D are joined. If \angle{BAC}={60}^{o}, \angle{ABC}={45}^{o}. Find \angle{ACD}
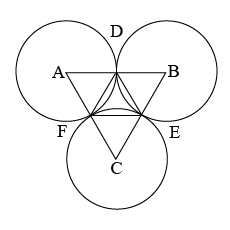
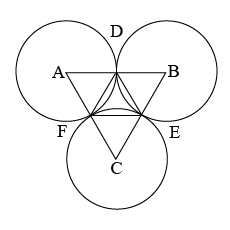
Three congruent circles with centres A, B and C with radius 5\ cm each, touch each other in point D, E, F as shown in figure.
(i) What is the perimeter of \Delta ABC?
(ii) What is the length of side DF of \Delta DEF?
In the following triplet pythagorean ? show working
(18, 79, 82)
A girl goes on a bicycle to East for 15 km, then turns back and comes to the starting point, then goes to west for 13 km and turns back again and stops after 5 km. In which direction is she when she stops? How far is she from the starting point? How much total distance has she covered?
Two isosceles triangles have equal vertical angles and their areas are in the ratio 36 : 25 . Find the ratio of their corresponding heights.
Bisectors of angles B and C of an isosceles triangle intersect each other at O and AB=AC. BO is produced to a point M on side AC. Prove that \angle MOC = \angle ABC.
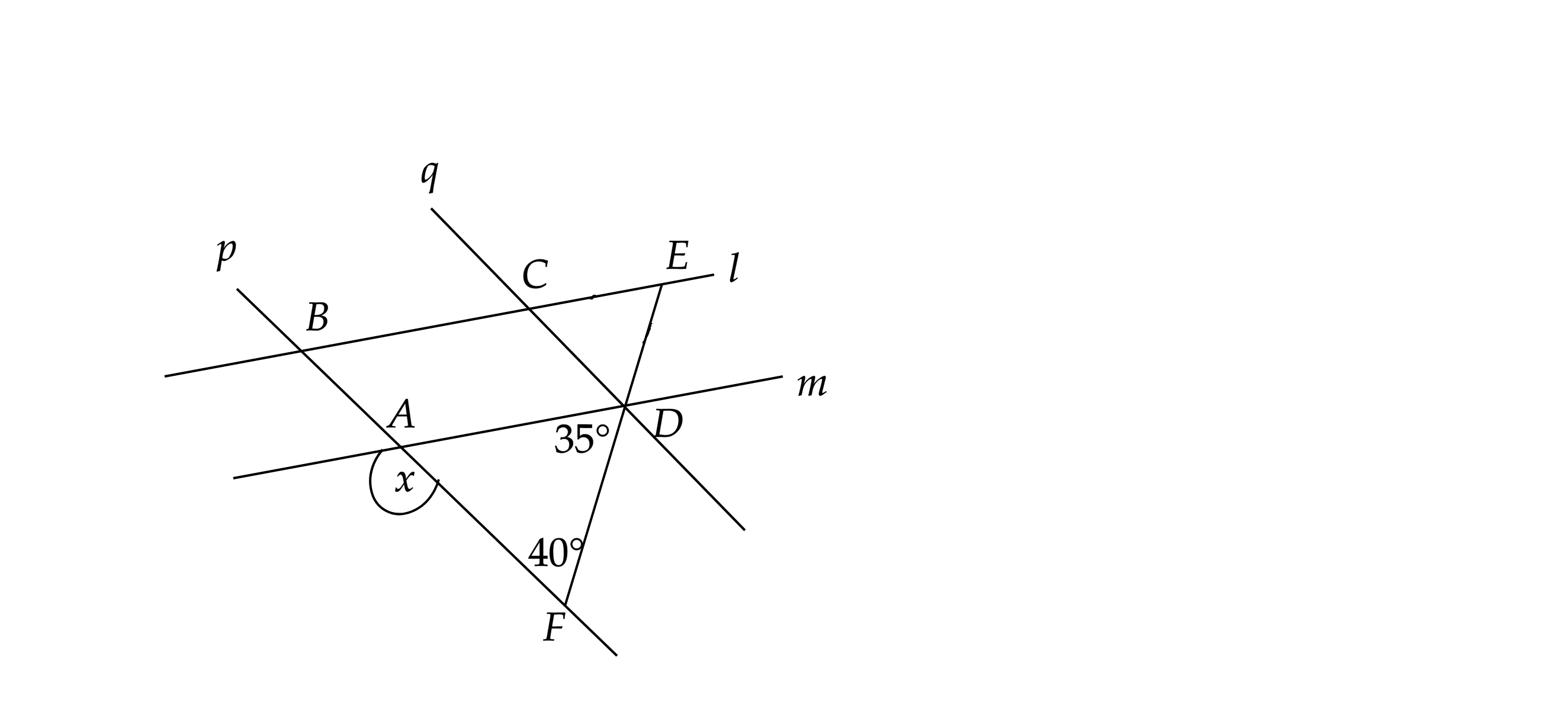
In the figure. Find x , If l\parallel m & p\parallel q .
Prove that the median to the base of an isosceles triangle is perpendicular to the base.
If \DeltaABC is right-angled at B, then show that AB^2+BC^2=AC^2.
In the given figure, PQ || RT. Find the value of {a} + {b}.

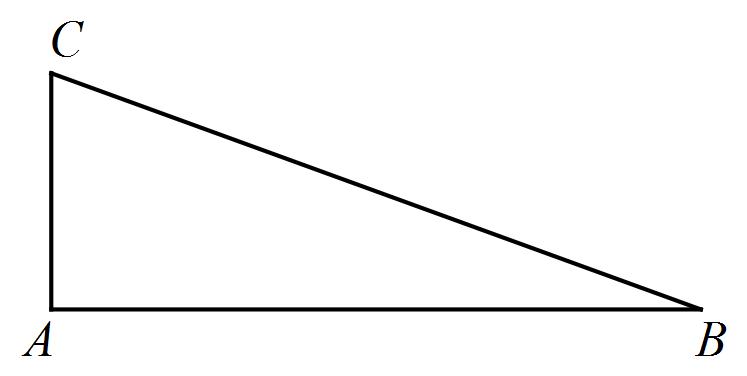
Name the type of the triangle:
\triangle PQR such that PQ = QR = PR = 5\ cm
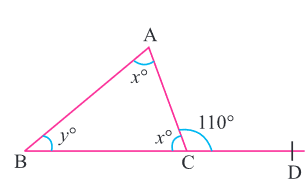
\angle ACD is an exterior angle of \Delta ABC the measure of \angle A and \angle B are equal. If \angle ACD = {140^ \circ }, find the measure of the angles \angle A and \angle B
Name the types of following triangles :
\triangle XYZ with m\angle Y = 90^{\circ} and XY = YZ.
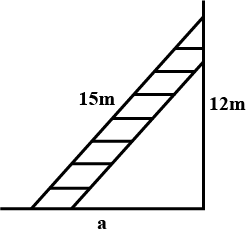
A 15m long ladder reached a window 12m high from the ground on placing it against a wall . Find the distance of the foot of the ladder from the wall .
The hypotenuse of a right triangle is 1 metres more than twice the shortest side. If the third side is 7 metres less than the hypotenuse, find the sides of the triangle.
In a rectangle ABCD, prove that
{AC}^{2}+{BD}^{2}={AB}^{2}+{BC}^{2}+{CD}^{2}+{DA}^{2}
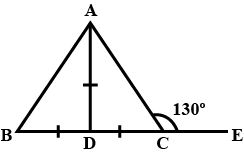
"In the given figure ,\angle BAC is {62^ \circ } says Sita . Do you agree with her?

The length of hypotenuse of a right angled triangle is 15. Find the length of the median of the hypotenuse.
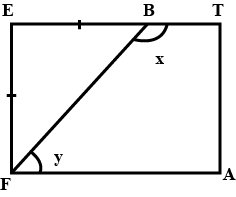
A playground is in the form of a rectangle FATE. Two players are standing at the points F and B. Where EF=EB Find the values of x and y.
In \triangle ABC,\angle B is a right angle. If AB=8 and BC=6. find AC.
In an isosceles triangle, the base is two third of each of other two equal sides. If the perimeter of the triangle is 24 \ m, find the length of all the three sides.
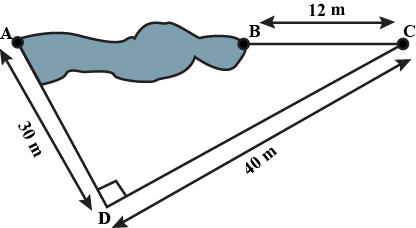
A boat in a circular lakes lies at the center, about 10m away from a bridge lying in 40 m distance across the circular lake. How much distance will boat have to reach to the extreme point of the left side of the bridge?
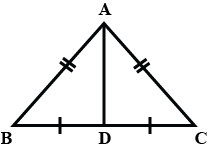
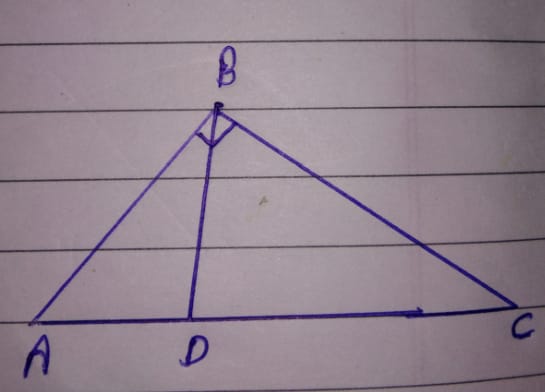
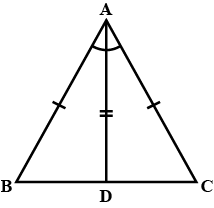
\Delta A B C is an isosceles triangle with A B = A C . AD bisects \angle A . Prove that \angle B = \angle C
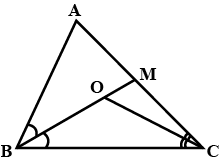
In a \Delta \angle ABC,\,\angle ACB and the bisectors of \angle ABC and \angle ACB at O such that \angle BOC = {120^0}
show that \angle A = \angle B = \angle C = {60^0}
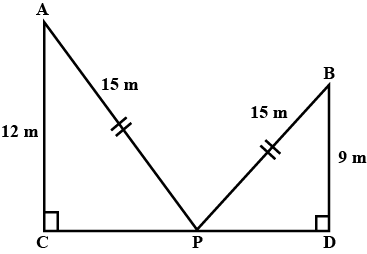
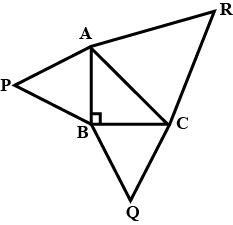
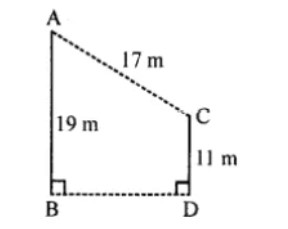
In the given figure, angle ACP = \angle BDP = 90^{o},\ AC=12\ m,\ BD=9\ m and PA=PB=15\ m. Find
(i) CP
(ii) PD
(iii) CD
Show that (m^{2} - 1), (2m), m^{2} + 1 always form a pythagoran triplet.
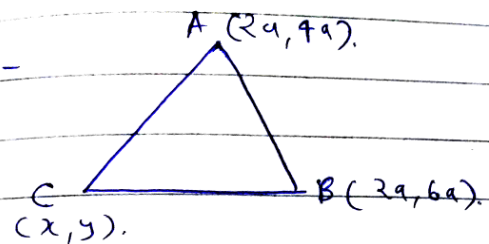
If A (2a,4a) and B (2a,6a) are two vertices of a equilateral triangle ABC then the vertex C is given by
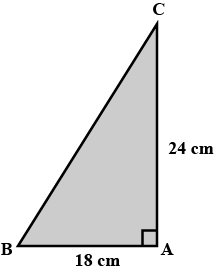
Triangle ABC is right-angled at vertex A. Calculate the length of BC, if AB=18 and AC=24 cm.
In \triangle {ABC}, if AD is the median, then show that {AB}^{2}+{AC}^{2}=2({AD}^{2}+{BD}^{2})
In \Delta PQR, if PR^2 = PQ^2 + QR^2, prove that \angle Q is right angle.
The sides of a triangle are 4 cm, 60 cm, 61 cm. Verify that it is a right angle triangle or not.
Area of a regular hexagon of side a cm is the sum of the 5 equilateral \Delta with side a cm. Is this statement true or false ?
From the adjacent figure find the value of (i) \angle PRS (ii) \angle PTS (iii) \angle STR (iv) \angle PRQ

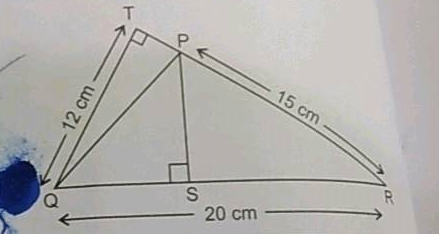
In the figure, find the area of \triangle {PQR} and the height PS.
In an equilateral \triangle ABC, if AD \bot BC then prove that AD^{2}=3\ BD^{2}
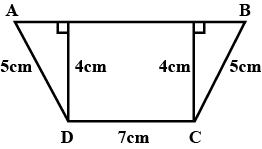
ABCD is a trapezium in which AB\parallel DC, DC=7cm distance between AB and DC is 4cm. Find AB.
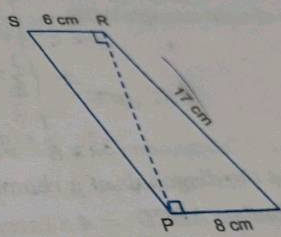
Calculate the area of the quadrilateral PQRS shown in the adjoining figure, given that PQ=8\ cm, RQ=17\ cm, \angle{RPQ}={90}^{o}, RS=6\ cm and \angle {PRS}={90}^{o}.
The equation of two equal sides of an isosceles triangle are 7x-y+3=0 and x+y+3=0 and its third side is passes through the point (1,\ -10) . The equation of the third side is
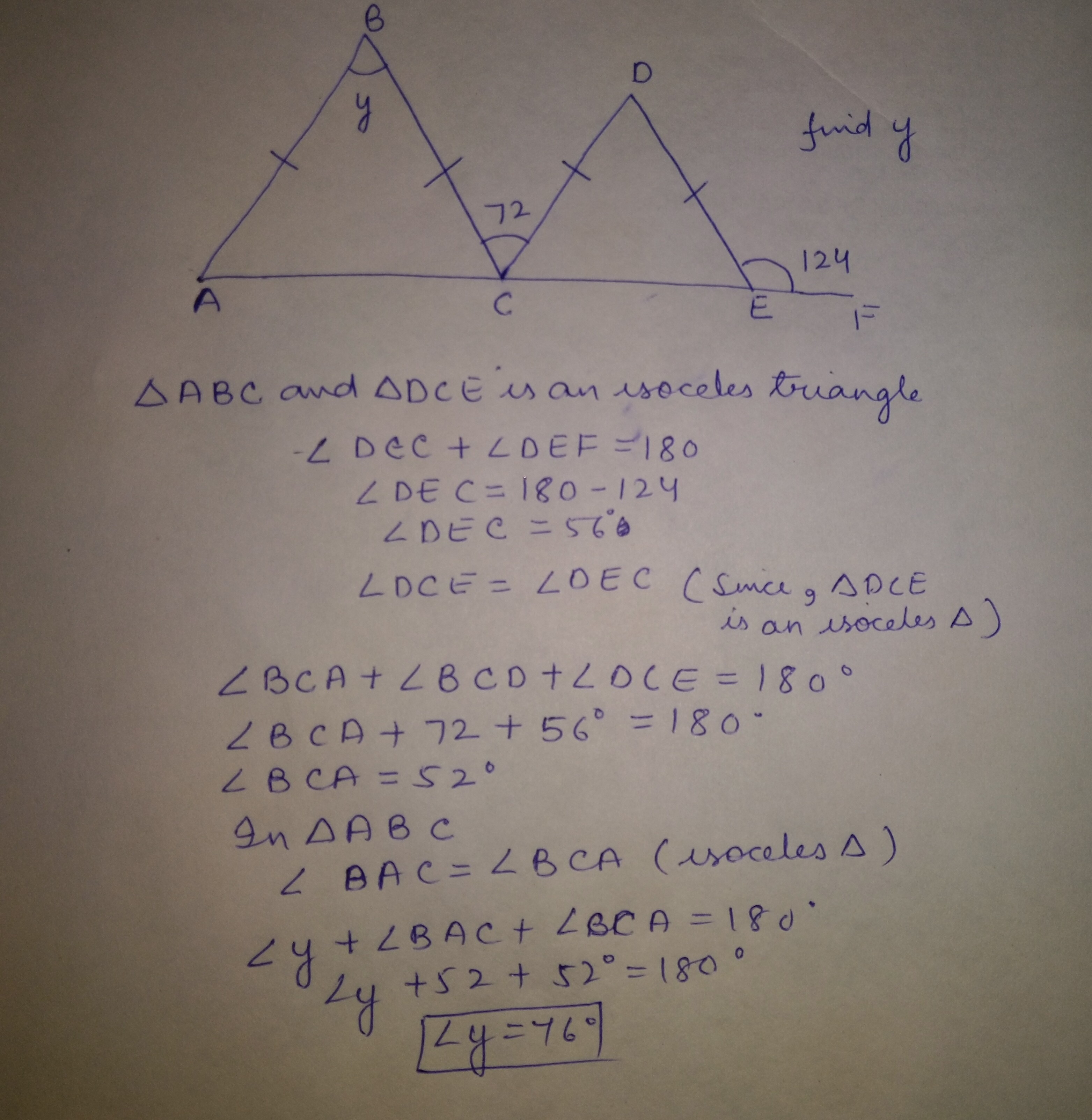
Triangles ABC and CDE are isosceles triangles, ACEF is a straight line. Find the value of y.
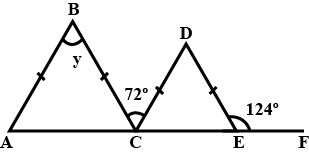
Find the values of \angle a \angle b in the figure given below.
In an equilateral triangle with side 'a', prove that the area of the triangle is \dfrac{\sqrt{3}}{4} a^2.
The sides of a triangle are equal and have equations 2x-y=0,3x+y=0 ,x-3y+10=0, respectively find the equation of three medians of the triangle and verify that they are concurrent
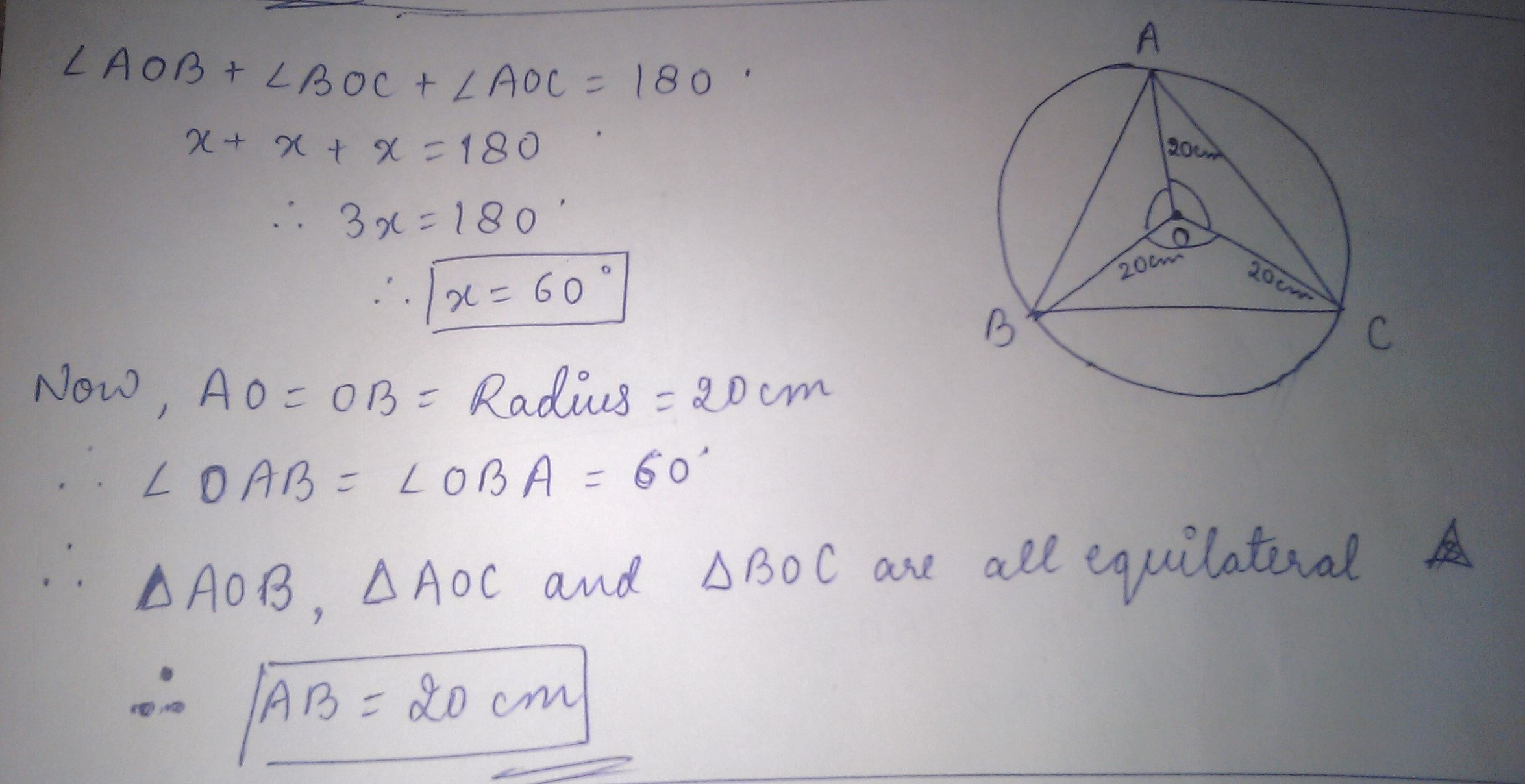
\Delta ABC is a equilateral triangle.Radius=20. Find AB
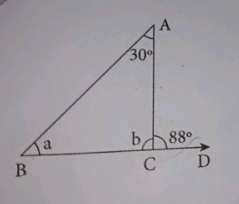
In the given figure, the side QR of \Delta PQR is produced to a point S. If the bisectors of \angle PQR and \angle PRS meets at point T, then prove that \angle QTR=\dfrac{1}{2}\angle QPR

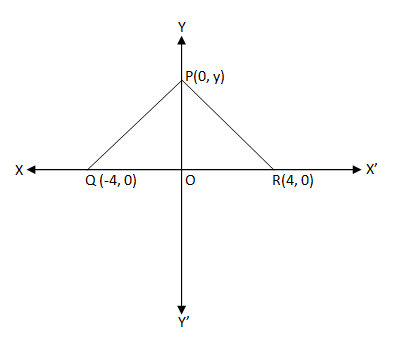
The base QR of an equilateral triangle PQR lies on x-axis. The coordinates of the point Q are (-4,0) and origin is the midpoint of the base. Find the coordinates of the points P and R.
The equation of the base of an equilateral triangle with vertex at (2,-1) is x+y-2=0. Then find its length of sides.
The base of an isosceles triangle is 18 cm and its area is 108 cm. Find its perimeter.
In isosceles \Delta has perimeter 30\ cm and each of the equal sides is 12\ cm. Find the area of the \Delta .
Find the area of the equilateral triangle which has the height is equal to \dfrac{\sqrt{3}}{2}.
The side of an equilateral triangle is 3\sqrt{3} cm. Find the area of the triangle [Take \sqrt{3} = 1.732]
If non-parallel sides of an isosceles trapezium are prolonged, an equilateral triangle ABC with sides of 6 cm would be formed. Knowing that the trapezium is half the height of the triangle, calculate the area of the trapezium.

Prove that the three angles of an equilateral triangle are equal.
Can you prove this that in a right-angled triangle:
\text{Base}^{ 2 }+{ \text{Perpendicular} }^{ 2 }={ \text{Hypotenuse} }^{ 2 }
Find angle x in each figure :
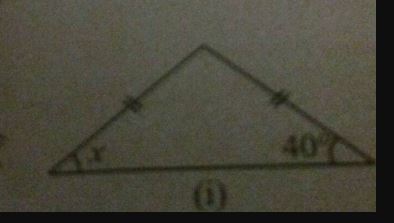
A lawn in a garden is in the shape of an equilateral triangle. If the length of the one side of the equilateral triangle is 75 m, find the of cost of fencing at the rate of Rs. 12 per metre?
An isosceles triangle has perimeter 36 cm and each of its equal sides is 13 cm long . The area of the triangle is
The base of an equilateral triangle with side 2a lies along the y -axis such that the mid-point of the base is at the origin. Find vertices of the triangle.
If the ratio of the altitude and area of a triangle is 3:12, then find the length of the base of triangle.
In an isosceles triangle ABC, one of the equal sides BC and the base AC are in the ratio of 7:5. If the perimeter of the triangle is 532\ cm, find the measures of all the three sides.
PQRS is a square and \Delta TSR is an isosceles triangle with TS=TR. Prove that PT=QT.
Prove that the lines represented by 3x^2-8xy-3y^2=0 and x+2y=3 form the sides of an isosceles right angled triangle.
In an equilateral \triangle BAC, point D is the midpoint of side BC. Prove that 4AD^{2}=3BC^{2}.
Find the perimeter of the following shape:
An equilateral triangle of side 11\ \text{cm}
In the given figure AD divides \angle BAC in the ration 1 : 3 and AD = DB betermine the value of X.

A right triangle ABC with sides 7cm, 24cm and 25cm is revolved about the side 24cm. Find the volume of the solid so obtained.
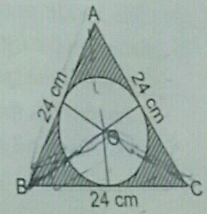
In an equilateral triangle of side 24\ cm, a circle is inscribed touching its sides. Find the area of the remaining portion of the triangle.
Name the following triangle :
XY=7\ cm, YZ=7\ cm, ZX=4\ cm
Do these sides that are given below form a right angle triangle?
2.5cm, 6.5cm, 6cm
In the case of a right-angled triangle, identify the side opposite to the right angle.
Prove that area of an equilateral triangle is equal to \dfrac{\sqrt{3}}{4}{a}^{2}, where a is the side of the triangle.
Prove that in a right angled triangle, square of the hypotenuse is equal to sum of the squares of other two sides.
In the given figure,\angle{PRQ}=\angle{PQR}, then prove that \angle{PQS}=\angle{PRT}
State the kind of triangle in the adjoining figure.

If in a triangle LMN:
\angle M=60^{o}, \angle N=60^{o}, find \angle L
Mention the kind of triangle also.
In a right-angled triangle the hypotenuse is of 13 cm and the difference between the other sides is 7 cm, then find the two sides.
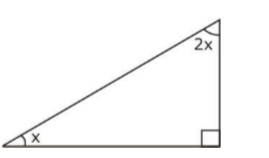
Find the value of x
In the given figure, OO is right-angled.
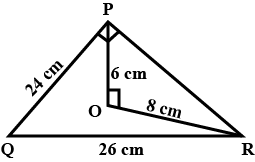
Solve
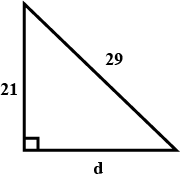
Naman is doing fly-fishing in a stream. The tip of his fishing rod is 1.8 m above the surface of the water and the fly at the end of the string rests on the water 3.6 m away from him and 2.4 m from the point directly under the tip of the rod. Find the length of the string.
Prove that each angle of an equilateral triangle is 60^{\circ}.
The sides of a triangle is given below. Determine if it is a right angled triangle.
1.4 cm, 4.8 cm, 5 cm
The sides AB and AC of \Delta ABC are produced to points E and D respectively. If Bisectors BO and CO of, \angle CBE\,\,and\,\,\angle BCD respectively meet at point O, then prove that \angle BOC = {90^0} - \frac{1}{2}\angle BAC.
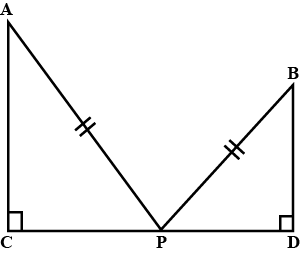
In UABC\ \hat {e}BAC=90^{o}, AB=AC seg APo side BC, B-P-C. D is any points on side BC. Prove that: 2AD^{2}=BD^{2}+CD^{2}
In a triangle, if the square of one side is equal to the sum of the squares of other two sides, then the angle opposite to the first side is a right angle. Prove it
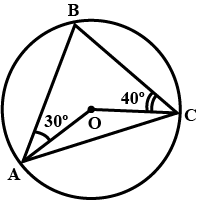
In the given figure, O is the centre of the circle . \angle OAB and \angle OCB are 30^{ \circ } and 40^{ \circ } respectively . Find \angle AOC. Show your steps of working
In \triangle \mathrm{PQR}, \angle \mathrm{PQR}=90^{\circ} if PR=15 and PQ=9cm find QR
If the side of a triangle are in the ratio 3:4:5, prove that it is right -angled triangle.
In an isosceles triangle, angles opposite to equal sides are___ .
Write the following statements:-
in \angle ABC,AB = 6\sqrt 3 cm, AC = 12 cm, BC = 6 cm. Find measure of B.
Explain the following term.
Equilateral triangle.
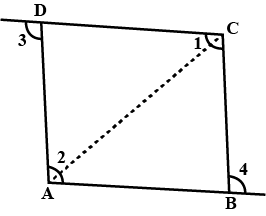
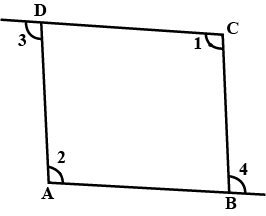
In the given figure, \angle 3 and \angle 4 are exterior angles of quadrilateral ABCD at point D and B respectively, and \angle A = \angle 2,\angle C = \angle 1, prove that \angle 3 + \angle 4 = \angle 1 + \angle 2
Suppose a triangle is equilateral. prove that it is equi-angular.
In \triangle ABC, right angled at B,AC=20\ cm and \tan{\angle ACB=\cfrac{3}{4}}, calculate the measure of AB and BC.
From the given figure, find x.

If AD is perpendicular to BC, then find the length of AB.

In the right-angled \triangle PQR,\angle P={ 90 }^{ \circ }. If l(PQ) = 24 cm and l(PR) = 10 cm, find the length of side QR?
Prove that in a right triangle,the square of the hypotenuse is equal to the sum of the square of the other sides.
Check whether A(5,-2),B(6,4) and C(7,-2) are the vertices of an isosceles triangle.
If the area of an equilateral triangle is 21.2 sq.cm, find the length of its each side.
In the given figure, \angle A = 90^{\circ} and AD \perp BC If BD = 2 cm and CD = 8 cm, find AD.

If two angles of a triangle are completely then what type of triangle will be formed.
Do these sides that are given below form a right angled triangle?
1.5cm, 2cm, 2.5cm
In the case of right angled triangles, identify the side opposite to right angle.
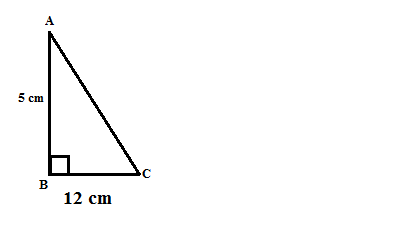
ABC is a triangle, right-angled at C. If AB =25cm and AC =7cm, find BC.
Do the sides 2 cm, 2 cm, 5 cm form a right-angled triangle?
In the case of right-angled triangles, identify the side opposite to the right angle.
A tree is broken at a height of 5m from the ground and its top touches the ground at a distance of 12m from the base of the tree. Find the original height of the tree.
A 15 m long ladder reached a window 12 m high from the ground on placing it against a wall at a distance of a m. Find the distance of the foot of the ladder from the wall.
PQR is a triangle, right angled at P. If PQ =10 cm and PR =24 cm, then find QR.
In a \Delta ABC, \angle ABC = \angle ACB and the bisectors of \angle ABC and \angle ACB intersect at O such that \angle BOC = 120^{\circ}. Show that \angle A = \angle B = \angle C = 60^{\circ}.

Fill in the blank:
In a \Delta ABC if \angle A = \angle C, then AB = .....
Fill in the blanks to make the following statements then the opposite interior angles.
An exterior angle of a triangle is equal to the sum of two ______ opposite angles.
An exterior angle of a triangle is always _______ than either of the interior opposite angles.
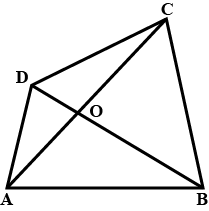
In Fig., A, B, C and D are four points, and no three points are collinear. AC and BD intersect at O. There are eight triangles that you can observe. Name all the triangles.
Explain the following terms:
Isosceles triangle
In Fig., the length (in cm) of each side has been indicated along the side. State for each triangle whether it is scalene, isosceles or equilateral:
Fill in the blanks to make the following statements correct.
The triangle formed by joining the mid-points of the sides of an isosceles triangle is ______
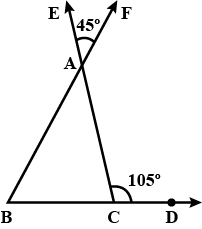
In figure, the sides BC, CA and BA of a \Delta ABC have been produced to D, E and F respectively. If \angle ACD=105^o and \angle EAF=45^o; find all the angles of the \Delta ABC.
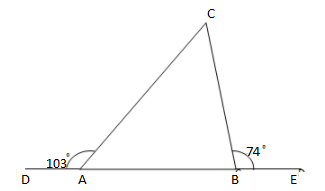
A student when asked to measure two exterior angles of \Delta ABC observed that the exterior angles at A and B are of 103^o and 74^o respectively. Is this possible? Why or why not?
The exterior angles, obtained on producing the base of a triangle both ways are 104^o and 136^o. Find all the angles of the triangle.
The foot of a ladder is 6m away from a wall and its top reaches a window 8m above the ground. If the ladder is shifted in such a way that its foot is 8m away from the wall, then to what height does its top reach?
Explain the following term.
Isosceles triangle.
If cos \theta = 0.6 , show that (5 sin \theta-3 tan \theta) =
The sides of certain triangles are given below. Determine which of them are right triangles.
1.6 cm, 3.8 cm, 4 cm
If sin A = \dfrac{9}{41}, find the values of cos A and tan A.
If 15 cot A = 8,find the values of sin A and secA.
The sides of certain triangles are given below. Determine which of them are right triangles.
(a - 1) cm, 2\sqrt{a} cm, (a + 1) cm
The sides of certain triangles are given below. Determine which of them are right triangles.
9 cm, 16 cm, 18 cm
The sides of certain triangles are given below. Determine which of them are right triangles.
1 cm, 24 cm, 25 cm
A ladder is placed in such a way that its foot is at a distance of 15 m from a wall and its top reaches a window 20 m above the ground. Find the length of the ladder.
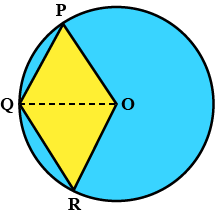
In the given figure, OPQR is a rhombus, three of whose vertices lie on a circle with centre O. If the area of the rhombus is \displaystyle 32\sqrt{3} \ cm^2, find the radius of the circle.
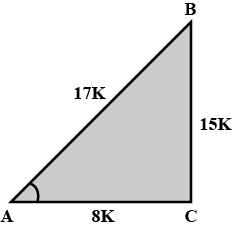
Find the length of altitude AD of an isosceles \Delta ABC in which AB = AC = 2a units and BC = a units.
In the adjoining figure, \triangle ABC is a right-angled at B and \angle A = 30^{\circ}. If BC = 6\ cm, Find (i) AB, (ii) AC.
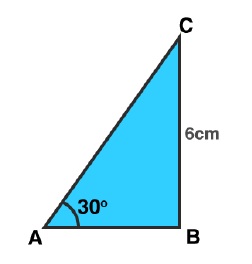
A man goes 80 m due east and then 150 m due north. How far is he from the starting point?
In the adjoining figure, \triangle ABC is a right-angled triangle in which \angle B = 90^{\circ}, \angle A = 30^{\circ} and AC = 20\ cm.
Find (i) BC, (ii) AB.
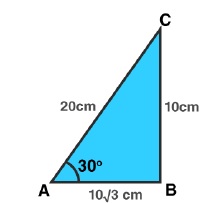
A 13-m-long ladder reaches a window of a building 12 m above the ground. Determine the distance of the foot of the ladder from the building.
A 5.5m long ladder is leaned against a wall. The ladder reaches the wall to a height of 4.4m. Find the distance between the wall and the foot of the ladder.
A man goes 10 m due south and then 24 m due west. How far is he from the starting point?
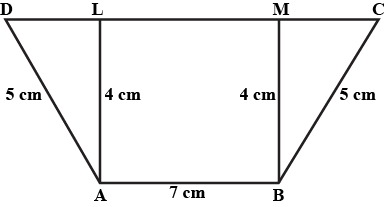
In the adjoining figure, \,DCBA\, is a trapezium where AL\,\,and\,\,BM are perpendicular to DC and DL=MC. It is given that AL=BM=4cm, BC=AD=5cm and AB=LM=7cm. Also AL \,||\, BM. Then find area of trapezium DCBA and also find the length of DC. DCBA is a and
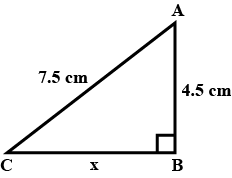
The length of one side of a right triangle is 4.5cm and the length of its hypotenuse is 7.5cm. Find the length of its third side.
Find the length of the hypotenuse of a right triangle, the other two sides of which measures 9\ cm and 12\ cm
The distance between the middle point of BC and the foot of the perpendicular from A is \dfrac{b^2- c^2}{2a}
The two legs of a right triangle are equal and the square of its hypotenuse is 50. Find the length of its third side.
The hypotenuse of a right triangle is 26cm long. If one of the remaining two sides is 10cm long, find the length of the other side.
The bisectors \angle B and \angle C of and isosceles triangle with AB = AC intersect each other at a point O. BO is produced to meet AC at a point M. Prove that \angle MOC = \angle ABC.

A 15m long ladder is placed against a wall to reach a window 12m high. Find the distance of the foot of the ladder from the wall.
The perimeter of a triangle is 28\ cm. One of its sides is 8\ cm. Write all the sides of the possible isosceles triangles with these measurements.
In right \triangle ABC, the lengths of its legs are given as a=6cm and b=4.5cm. Find the length of its hypotenuse.
The perpendicular from A on side B C of a \Delta A B C intersects B C at D such that D B=3 C D (see figure). Prove that 2AB^{2}=2 AC^{2}+BC^{2}

In Figure find thee measures of \angle PON and \angle NPO
In \triangle ABC, if \angle A=\angle C, and exterior angle ABX=140^{o}, then find the angles of the triangle.
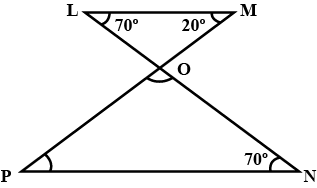
Find the values of x and y Figure
Jayanti takes shortest route to her home by walking diagonally across a rectangular park. The park measures 60 meters\times 80 meters. How much shorter is the route across the park than the route around its edges?
If the sides of a triangle are produced in an order, show that the sum of the exterior angles so formed is 360^{o}
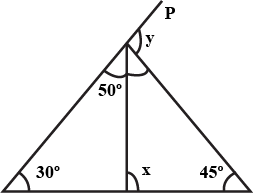
In Figure, find the measures of \angle x and \angle y.
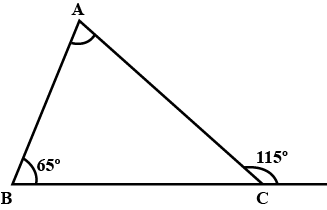
Find the measure of \angle A in Figure
In Figure, QP || RT. Find the value of x and y.
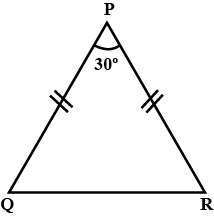
In \triangle PQR of Figure PQ=PR. Find the measures of \angle Q and \angle R.
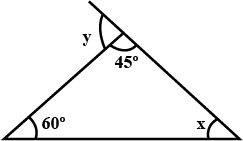
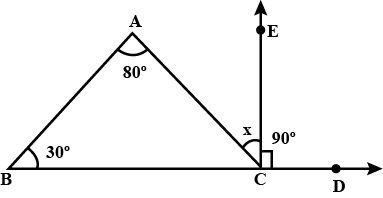
Find the value of x in Figure
Find the length of the side of a square if the length of its diagonal is 10cm.
The sum of an exterior angle of a triangle and its adjacent angle is always ______.
Measures of each of the angles of an equilateral triangle are ______.
In an isosceles triangle, two angles are always ______.
Fill in the blanks to make the statements true.
The sides of a right triangle whose hypotenuse is 17 cm are ______ and ______.
Can a right triangle with sides 6cm, 10cm and 8cm be formed? Give reason.
The dimensions of a rectangular field are 80m and 18m. Find the length of its diagonal.
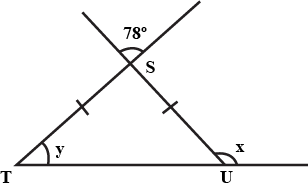
In Fig., if ST=SU, then find the values of x and y.
In Fig. if y is five times x, find the value of z.
Point A and B are on the opposite edges of a pond as shown in Figure. To find the distance between the two points, the surveyor makes a right angled triangle as shown. FInd the distance AB

The foot of a ladder is 6 m away from its wall and its top reaches a window 8 m above the ground,
(a)If the ladder is shifted in such a way that its foot is 8 m away from the wall, to what height does its top reach?
Two poles of 10\ m and 15 \ m stand upright on a plane ground. If the distance between the tops is 13 \ m , find the distance between their feet.
The foot of a ladder is 6 m away from its wall and its top reaches a window 8 m above the ground, (a) Find the length of the ladder.
In Fig.,
(i) \angle TPQ = \angle ______ + \angle ______
(ii) \angle UQR = \angle ______ + \angle ______
(iii) \angle PRS = \angle ______ + \angle ______
Rahul walks 12m north from his house and turns west to walk 35m to reach his friend's house. While returning, he walks diagonally from his friend's house to reach back to his house. What distance did he walk while returning?
Lengths of sides of triangles are given below. Determine which of them are right triangles. In case of a right triangle, write the length of its hypotenuse:13 cm, 12 cm, 5 cm
A vertical tree is broken by the wind at height of 6 meter from its foot and top touches the ground at a distance of 8 meter from the foot of the tree. Calculate the distance between the top of tree before breaking and the point at which tip of the touches the ground, after it breaks.
Area of a triangle PQR right-angled at Q is 60\, cm^2 (Fig). If the smallest side is 8\, cm long, find the length of the other two sides.

In the given figure, AB and CD are two vertical poles of height 19 m and 11 m respectively. If the shortest distance between their tops is 17 m, find how far apart they are?
Lengths of sides of triangles are given below. Determine which of them are right triangles. In case of a right triangle, write the length of its hypotenuse:1.4 cm, 4.8 cm, 5 cm.
In a right triangle ABC, \angle B=90^{\circ}. If AB=14\ cm, BC=48\ cm, find AC.
Lengths of sides of triangles are given below. Determine which of them are right triangles. In case of a right triangle, write the length of its hypotenuse:
(i) 3 cm, 8 cm, 6 cm
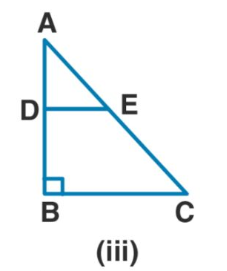
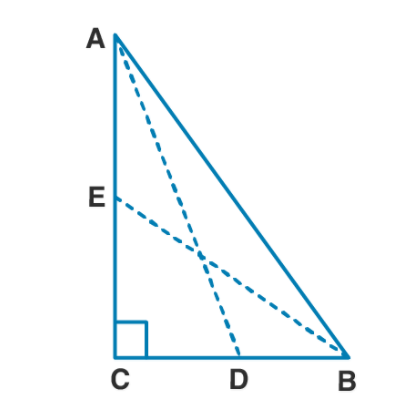
(c) In figure (iii) given below, triangle ABC is right angled at B. Given that AB = 9 cm, AC = 15 cm and D, E are mid-point of the sides AB and AC respectively, calculate
(i) the length of BC
(ii) the area of \Delta ADE.
In a right-angled triangle, if hypotenuse is 20 cm and the ratio of the other two sides is 4:3, find the sides.
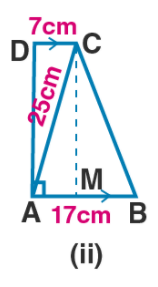
In figure (i) given below, BC = 5 cm,B =90, AB = 5AE, CD = 2AE and AC = ED. Calculate the lengths of EA, CD, AB and AC.

In the figure given above, \angle D = 90°, AB = 16 cm, BC = 12 cm and CA = 6 cm. Find CD.

(b) In figure (ii) given below, PSR = 90, PQ = 10 cm, QS = 6 cm and RQ = 9 cm. Calculate the length of PR.
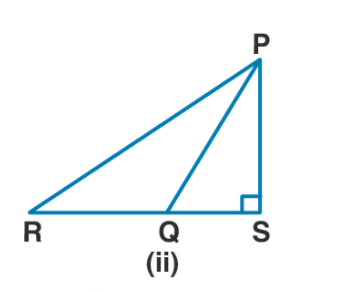
In the figure (ii) given below, ABC is a right triangle right angled at C. If D is mid-point of BC, prove that AB^2= 4AD^2 3AC^2.
A guy attached a wire 24 m long to a vertical pole of height 18 m and has a stake attached to the other end. How far from the base of the pole should the stake be driven so that the wire will be tight?
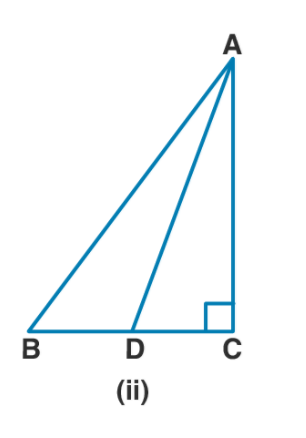
In figure (i) given below, D and E are mid-points of the sides BC and CA respectively of a ABC, right angled at C.
Prove that : (i) 4AD^2=4AC^2 + BC^2
In figure (ii) given below, AB || DC, A = 90, DC = 7 cm, AB = 17 cm and AC = 25 cm. Calculate BC.
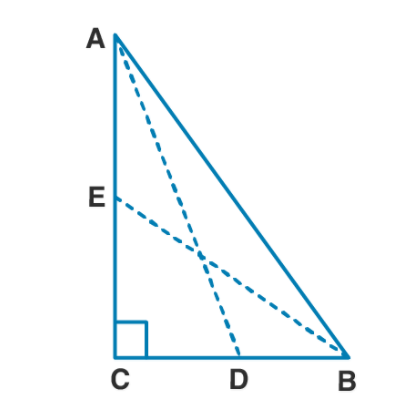
In figure (i) given below, D and E are mid-points of the sides BC and CA respectively of a ABC, right angled at C.
Prove that : (ii) 4BE^2 = 4BC^2+AC^2

In fig. (i) given below, the diagonals AC and BD of a quadrilateral ABCD intersect at O, at right angles.
Prove that AB^2 + CD^2 = AD^2 + BC^2
In a right angled triangle ABC, right angled at C, P and Q are the points on the sides CA and CB respectively which divide these sides in the ration 2:1.
Prove that
(i) 9 AQ^2 = 9 AC^2 + 4 BC^2
(ii) 9 BP^2 = 9 BC^2 + 4 AC^2
(iii) 9 (AQ^2 + BP^2) = 13 AB^2.
In a ABC, A = 90, CA = AB and D is a point on AB produced. Prove that : DC^2 BD^2 = 2ABAD.
In a quadrilateral ABCD, B = 90 = D. Prove that 2 AC^2 BC^2 = AB^2 + AD^2 + DC^2.
A peacock on a pillar of 9 feet height on seeing a snake coming towards its hole situated just below the pillar from a distance of 27 feet away from the pillar will fly to catch it. If both possess the same speed, how far from the pillar they are going to meet?
In the given figure, \Delta PQR is right angled at Q and point S and T trisect side QR. Prove that PT^2 = 3PR^2 + 5PS^2.
In the given figure, D and E are mid-points of the sides BC and CA respectively of a ΔABC, right angled at C.
Prove that : 4(AD^2+BE^2) = 5AB^2
A ladder 10 \ m long just reaches the top of a building 8 \ m high from the ground. Find the distance of the foot of the ladder from the building.

The exterior angles of a triangle obtained by producing, the sides in order are 110^{\circ} , 130^{\circ} and x . Find x .
The exterior angles of a triangle obtained by producing, the sides in order are 110^o,\space 130^o and x. Find x.
Prove that the sum of the exterior angles of a triangle formed by producing the sides of the triangle in order is equal to 4 right angles.
Given : AC is perpendicular to BD.
Prove : AB^2 + CD^2 = BC^2 + AD^2
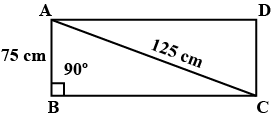
The length of the diagonal of a rectangular playground is 125 \ m and the length of one side is 75\ m. Find the length of the other side.
In \Delta ABC, \angle B=90^{\circ}, Find the sides of the triangle, if AB = x\ cm, BC = \left ( 4x+4 \right )\ cm and AC = \left ( 4x+5 \right )\ cm
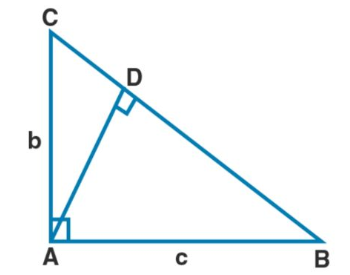
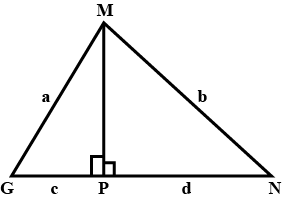
In \triangle MGN, MP \perp GN. If MG = a units, MN = b units, GP = c units and PN = d units.
Prove that \left(a + b\right) \left(a - b\right) = \left(c + d\right) \left(c - d\right).
In a right triangle the lengths of the medians of the acute angles are 9 cm and 13 cm. Find the length of the hypotenuse of the triangle.
In Figure AB=8 cm, BC=6cm, AC=3cm and the \angle ADC=90^{\circ}. Calculate CD.
Show that the following points form an equilateral triangle. (-\sqrt 3, 1), (2 \sqrt 3, -2) and (2 \sqrt 3, 4)
The straight lines 3x + 4y = 5 and 4x - 3y = 15 -intersect at the point A. On these lines, the points B and C are chosen so that AB = AC. Find possible equation of the line BC passing through the point (1, 2).
Two parallel lines - are at a distance 1 apart and a point P between them is at a distance p from one of them. Q and R are chosen on these two parallel, lines such that \Delta PQR is an equilateral triangle. Prove that the length of the side of this equilateral triangle is\sqrt{\dfrac{p^2 \, - \, p \, + \, 1}{3}}
\triangle ABD is a right triangle right-angled at A and AC\bot BD. Show that (i) { AB }^{ 2 } = BC.BD(ii) { AC }^{ 2 } = BC.DC(iii) { AD }^{ 2 } = BD \cdot CD(iv) \dfrac { { AB }^{ 2 } }{ { AC }^{ 2 } } = \dfrac { BD }{ DC }
If in the given \triangle ABC, AB=BD=BC, then find the ratio of \angle ACD : \angle ADC
In this fig. M is the midpoint of Q R, \angle P R Q = 90 ^ { \circ }, then prove that, P Q ^ { 2 } = 4 P M ^ { 2 } - 3 P R ^ { 2 }
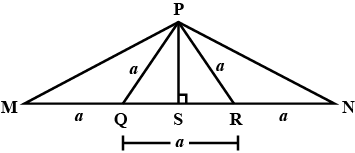
If 5,12,135,12,13 are the length of the side of a triangle. show that the triangle is right angled. Find the length of altitude on the hypotenuse.
From the information given in the figure, prove that PM=PN= \sqrt { 3 } \times a
If (-5,4) and (5,4) are tow vertices of an equilateral triangle, then find coordinates of the third vertex, given that the origin lies in the interior of the triangle.
In the given figure \triangle{ABC} is isosceles with AB=AC.D,E and F are respectively the mid-points of BC,CA and AB.Show that the line segment AD is perpendicular to the line segment EF and is bisected by it.
If A is ( - 1,2,0 ) , B is ( 1,2,3 ) and C is ( 4,2,1 ) , then prove that \Delta A B C is an isosceles right triangle.
In the adjoining figure, A B C is a triangle in which A D is the bisector of \angle A. If A D \perp B C, show that \Delta A B C is isosceles.

Show that the points A(1, 2), B(1, 6), C(1 + 2 \sqrt{3}, 4) are vertices of an equilateral triangle.
Show that difference of any two sides of a triangle is less than to the third side.
Class 7 Maths Extra Questions
- Algebraic Expressions Extra Questions
- Comparing Quantities Extra Questions
- Data Handling Extra Questions
- Exponents And Powers Extra Questions
- Fractions And Decimals Extra Questions
- Integers Extra Questions
- Lines And Angles Extra Questions
- Perimeter And Area Extra Questions
- Practical Geometry Extra Questions
- Rational Numbers Extra Questions
- Simple Equations Extra Questions
- Symmetry Extra Questions
- The Triangle And Its Properties Extra Questions
- Visualising Solid Shapes Extra Questions