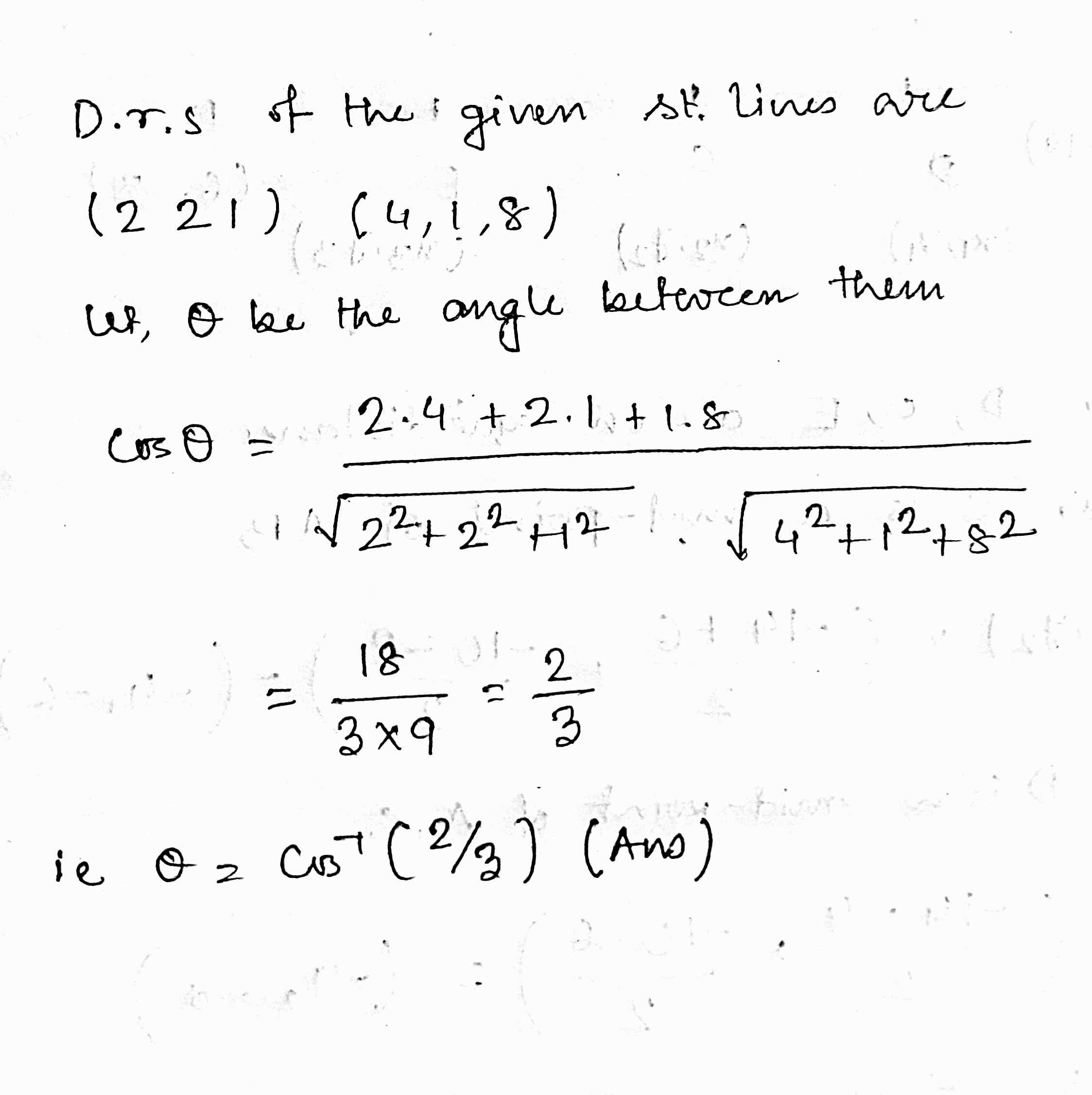Three Dimensional Geometry - Class 12 Engineering Maths - Extra Questions
If the coordinates of the points A,B,C,D be (1,2,3),(4,5,7),(−4,3,−6) and (2,9,2) respectively, then find the angle between the lines AB and CD
Angle between lines whose direction cosine satisfy l+m+n=0,l2+m2−n2=0.
Find the vector equation of the plane passing through the points 4¯i−3¯j−¯k,3¯i+7¯j−10¯k and 2¯i+5¯5−7¯k and find if the point ¯i+2¯j−3¯k lies on the plane.
Find the angle between two lines, one of which has direction ratios 2,2,1 while the other one is obtained by joining the points (3,1,4) and (7,2,12).
Find the angle between the lines y−√3x−5=0 and √3y−x+6=0.
Find the angle between the following pairs of lines:
x−51=2y+6−2=z−31 and x−23=y+14=z−65
Find the angle between the following pairs of lines:
5−x−2=y+31=1−z3 and x3=1−y−2=z+5−1
Find the angle between the following pairs of lines:
x−12=y−23=z−3−3 and x+3−1=y−58=z−14
Find the angle between the following pairs of lines:
→r=3ˆi+ˆj−2ˆk+λ(ˆi+ˆj−2ˆk) and →r=2ˆi−ˆj−56ˆk+μ(3ˆi−5ˆj−4ˆk).
Find the angle between the following pair of lines:
(i) →r=2ˆi−5ˆj+ˆk+λ(3ˆi−2ˆj+6ˆk) and →r=7ˆi−6ˆk+μ(ˆi+2ˆj+2ˆk)(ii) →r=3ˆi+ˆj−2ˆk+λ(ˆi−ˆj−2ˆk) and →r=2ˆi−ˆj−56ˆk+μ(^3i−5ˆj−4ˆk)
Find the angle between the following pair of lines:
(i) x−22=y−15=z+3−3 and x+2−1=y−48=z−54
(ii) x2=y2=z1 and x−54=y−21=z−38
Find the angle between the two straight lines whose direction cosines are given by 2l+2m−n=0 and mn+nl+lm=0.
Measure of angle between x2=y2=z1 and x2=y−1=z−2 is
The angle between the lines x−23=y+1−2=z−2 and x−11=2y+33=z+52 is equal to:
Find the angle between the following pairs of lines:
x+43=y−15=z+34 and x+11=y−41=z−52
Find the angle between the lines →r=(2ˆi−5ˆj+ˆk)+λ(3ˆi+2ˆj+6ˆk) and →r=7ˆi−6ˆj+μ(ˆi+2ˆj+2ˆk).
Find the angle between the lines 2x=3y=−z and 6x=−y=−4z.
Find the angle between the following pair of lines:
x−22=y−15=z+3−3 and x+2−1=y−48=z−54.
Find the angle between the following pair of lines:
x2=y2=z1 and x−54=y−21=z−38.
Find the angle between the lines
x2=y2=z1 and x−54=y−21=z−38
Class 12 Engineering Maths Extra Questions
- Continuity And Differentiability Extra Questions
- Determinants Extra Questions
- Differential Equations Extra Questions
- Integrals Extra Questions
- Inverse Trigonometric Functions Extra Questions
- Relations And Functions Extra Questions
- Three Dimensional Geometry Extra Questions
- Vector Algebra Extra Questions