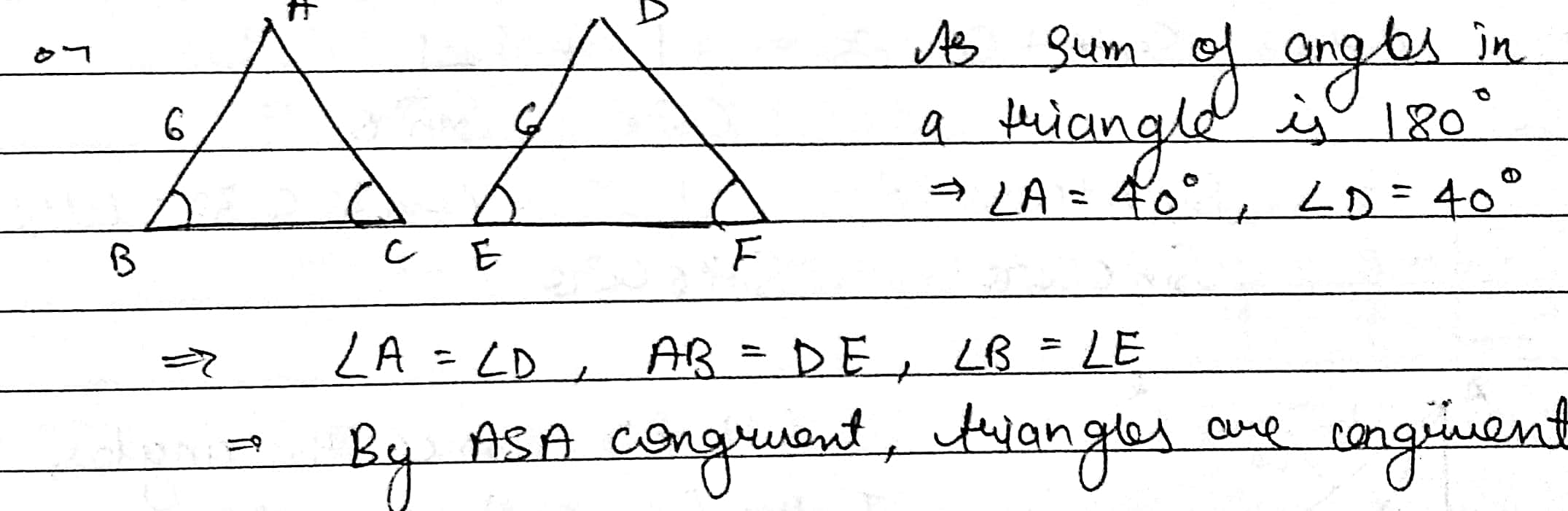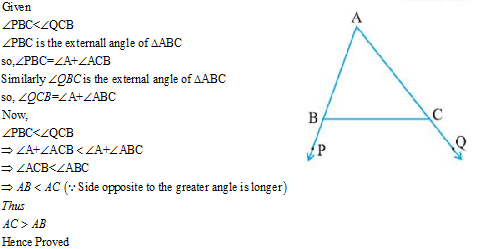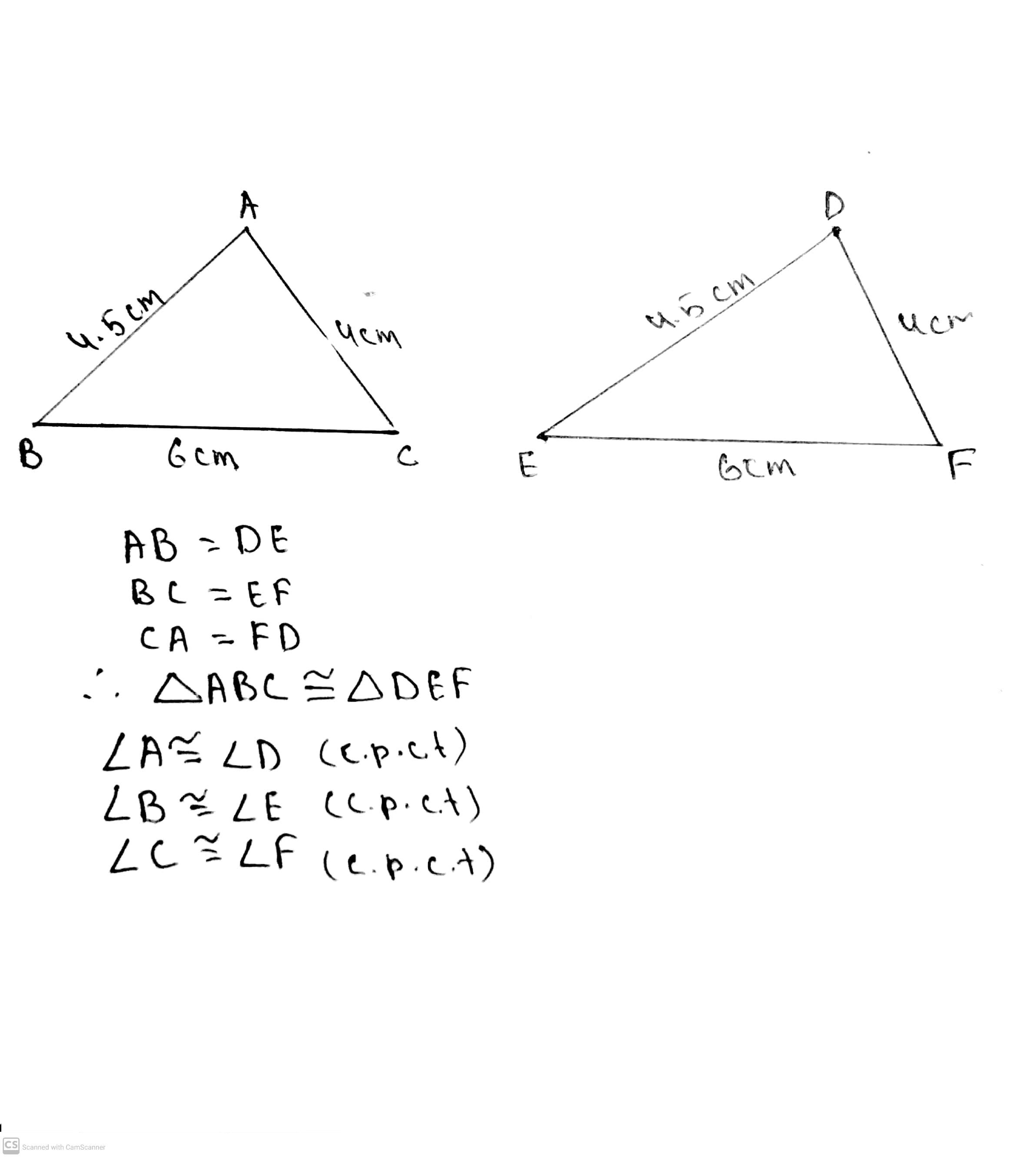Triangles - Class 9 Maths - Extra Questions
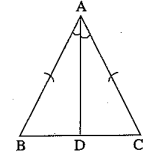
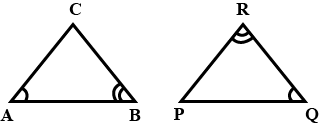
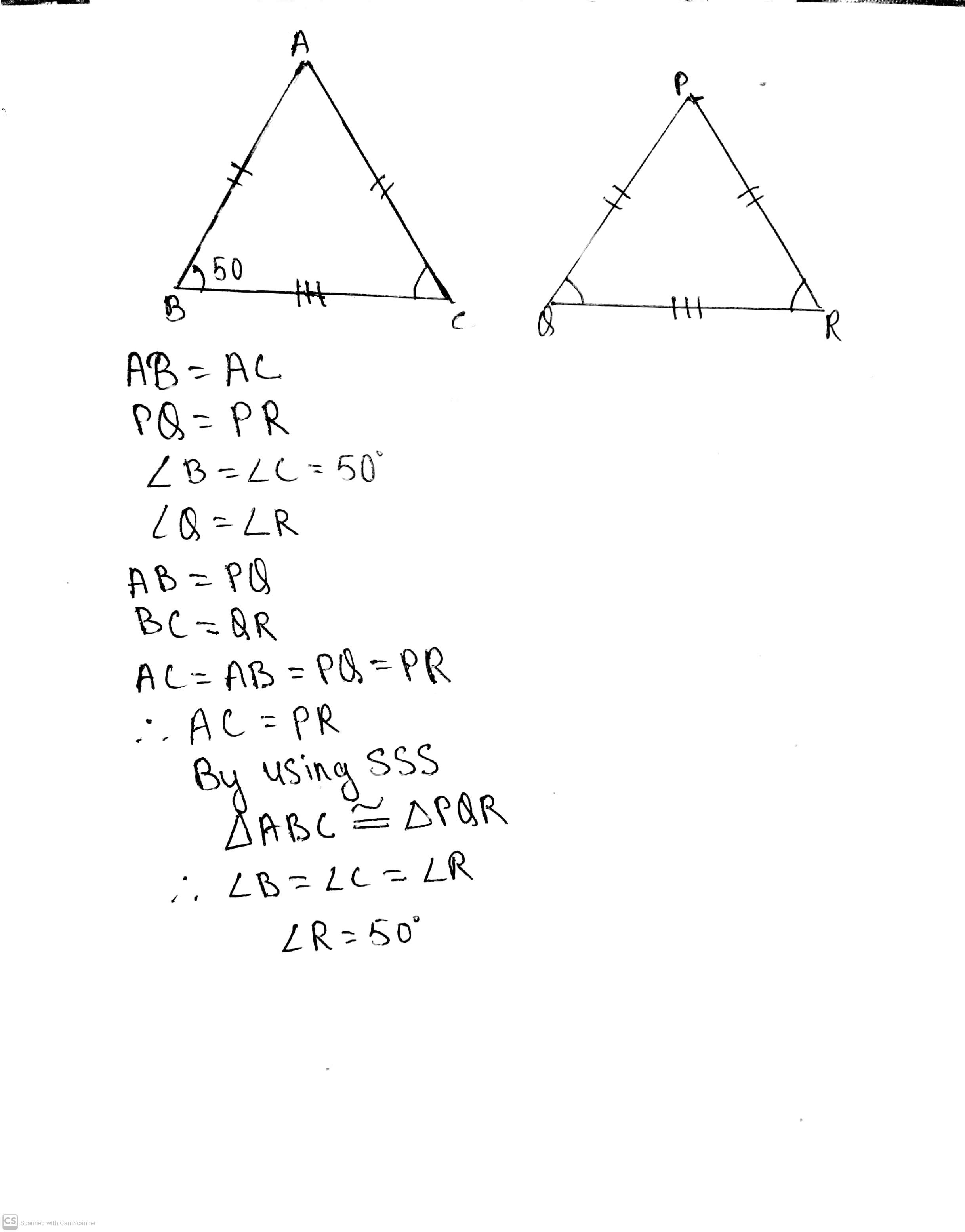
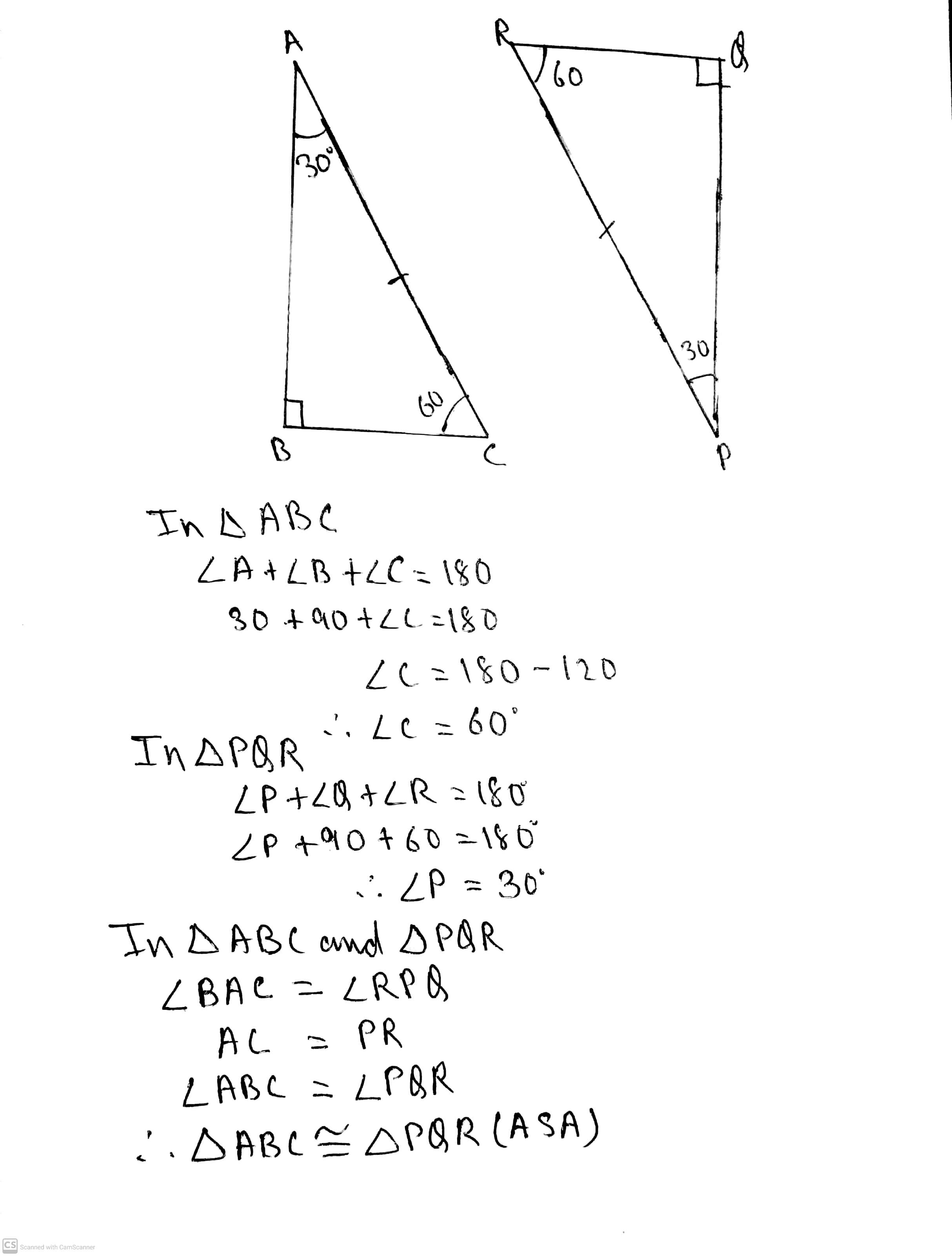
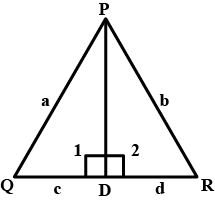
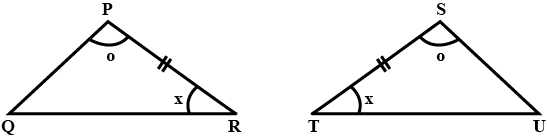
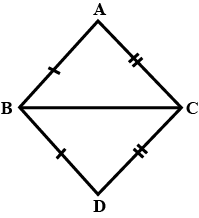
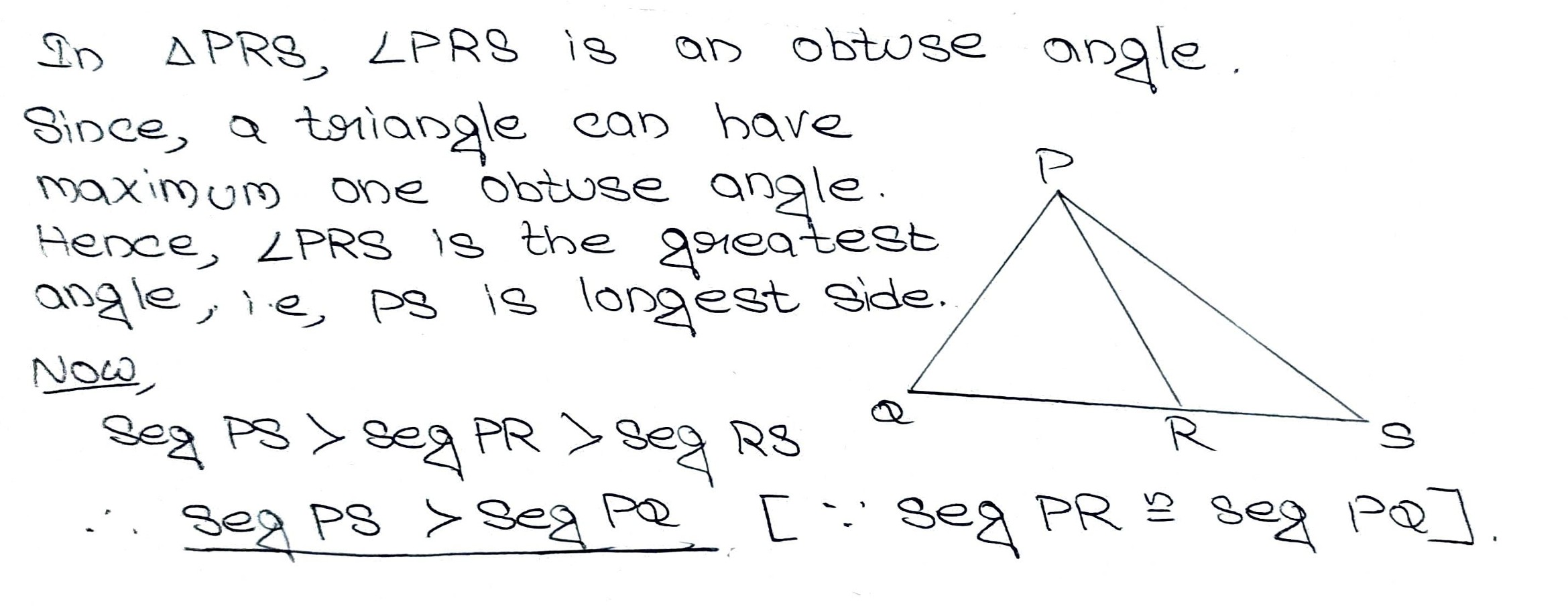
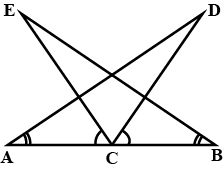
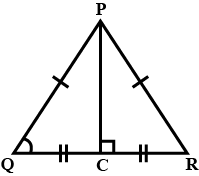
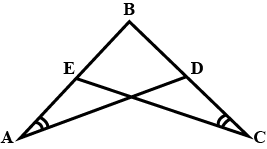
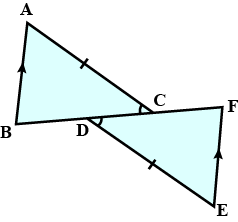
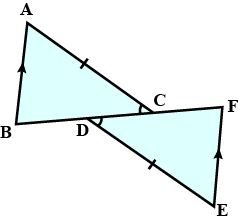
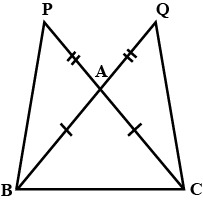
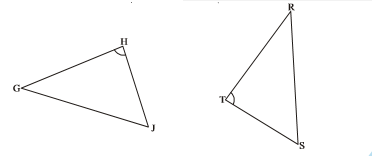
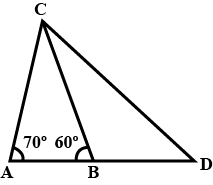
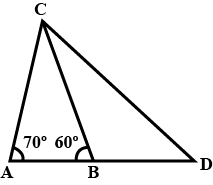
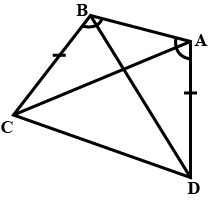
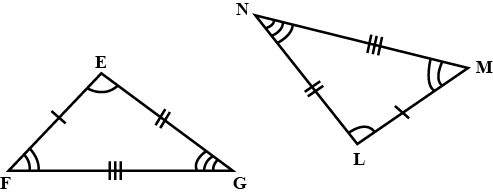
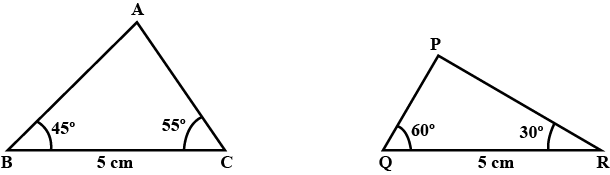
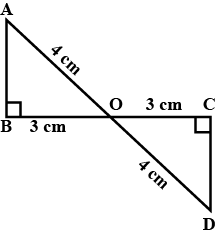
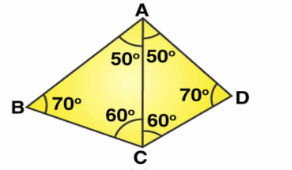
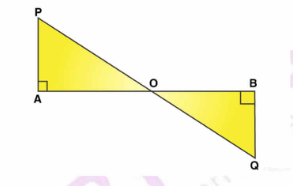
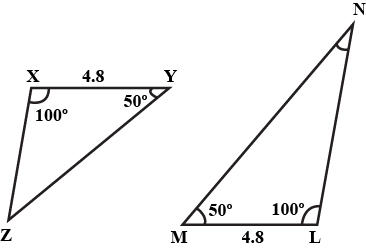
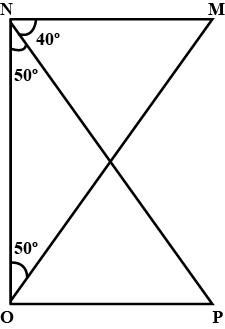
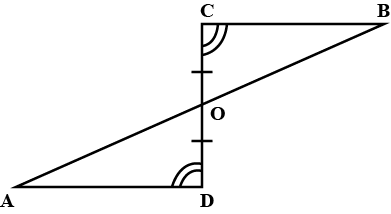
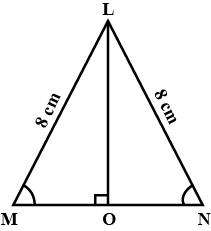
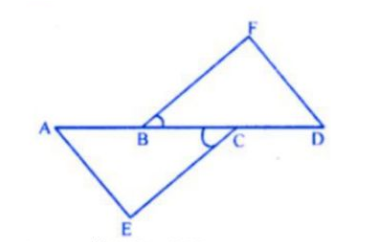
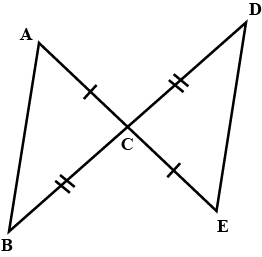
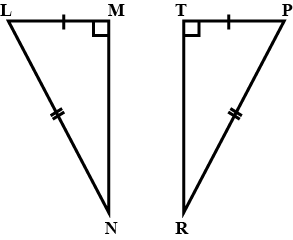
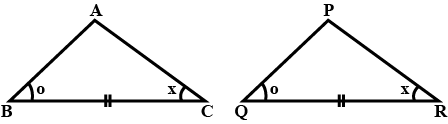
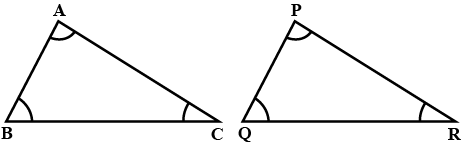
In △ABC and △PQR, BC=QR,∠A=90∘,∠C=∠R=40∘ and ∠Q=50∘.
Above two triangles are congruent by ASA test
If true then enter 1 else if False enter 0.
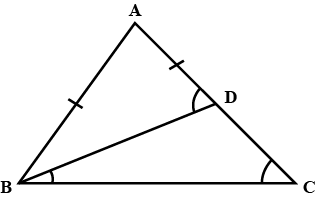
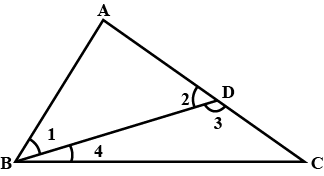
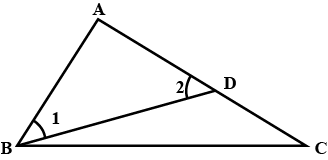
In △ABC and△PQR,AB=PQ,AC=PRandBC=QR, then the two triangles are congruent by which test?
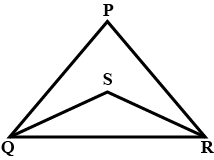
In ΔPQR, seg PQ ≅ seg QR and ∠P=70o, then find the measures of angle Q is:
Without drawing exact triangles, state whether the given pairs of triangle are congruent or not, if congruent use '1' else '0'.
In ΔABCandΔDEF;AB=EF,BC=DFand∠B=∠F
In ΔABC, if AB=76 cm, BC=69 cm and CA=61 cm, then the smallest angle is B.If true then enter 1 and if false then enter 0.
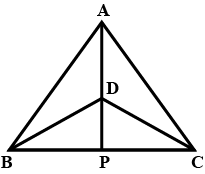
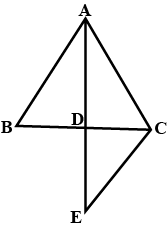
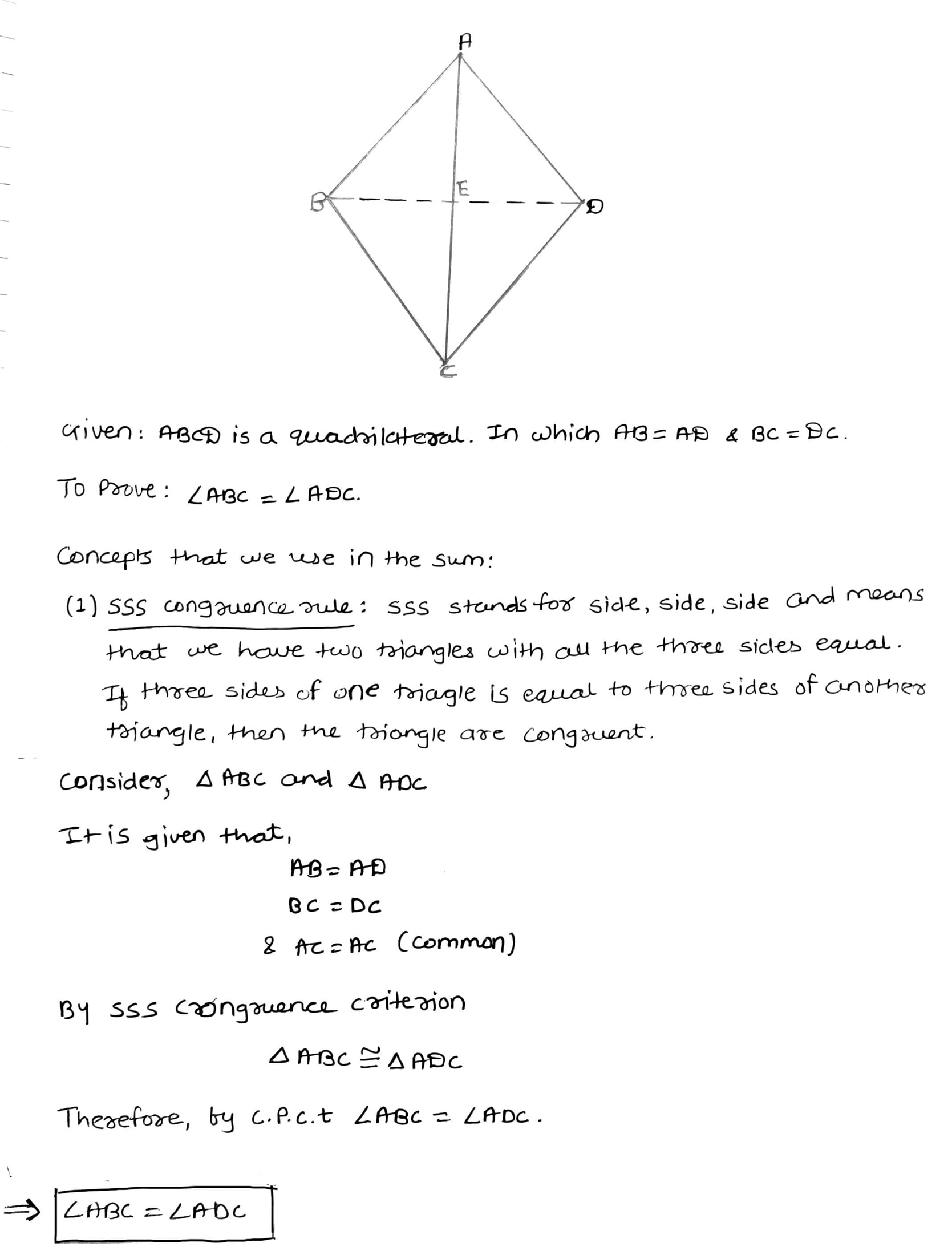
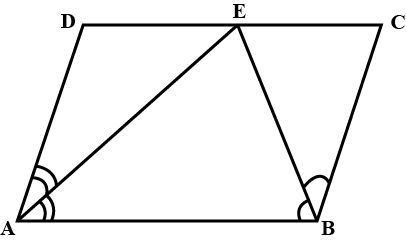
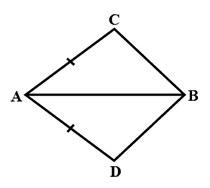
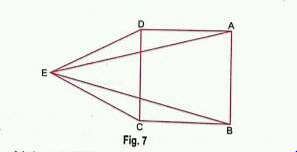
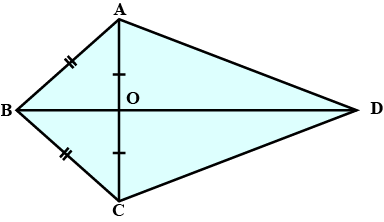
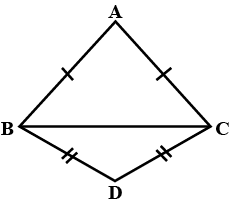
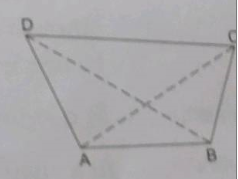
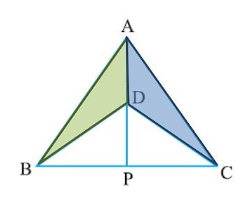
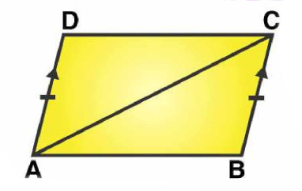
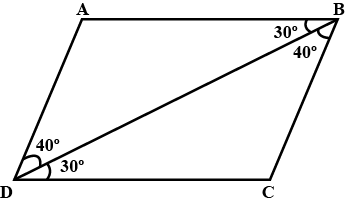
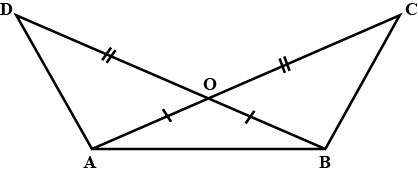
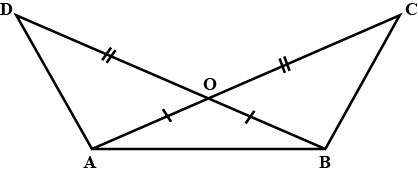
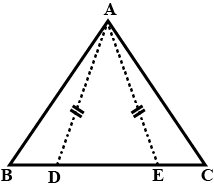
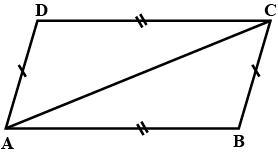
In the alongside diagram, ABCD is parallelogram. Prove that : AB=2BC
Without drawing exact triangles, state, giving reasons, whether the given pairs of triangle are congruent or not :
\Delta PQR \,\,\, and \,\,\, \Delta SQR; PQ=SR\,\, and \,\, \angle PQR = \angle SQR.
Without drawing exact triangles, state, giving reasons, whether the given pairs of triangle are congruent or not :
In \Delta ABC \ and \ \Delta PQR; AB=QR, AC=PR \,\, and \,\, \angle B = \angle R.
If the two sides and the included angle of a triangle are respectively equal to two sides and the included angle of the other triangle, the two triangles are congruent. If true enter 1 else 0.
If \triangle DEF\cong \triangle RPQ, then \angle D=\angle Q.If true enter 1, else 0.
If \triangle PQR\cong \triangle CAB, PQ = CA.If true enter 1, else 0.
If \triangle ABC\cong \triangle PRQ then AB = PQ.If true enter 1, else 0.
State whether the given statement is True(Press 1) or False(Press 0):If the two angles and the included sides of one triangle are respectively equal to the two angles and the included side of the other triangle, then the two triangles are congruent.
Two right triangles are congruent, if hypotenuse and a side of one triangle are respectively equal to the hypotenuse and a side of the other triangle. If true enter 1 else 0.
State true or false:If the two sides of a triangle are unequal, then the larger side has the smaller angle opposite to it.If true enter 1, else 0.
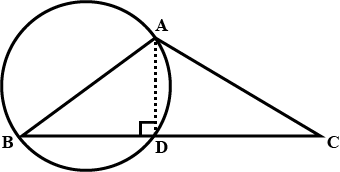
Prove that the circle drawn with any equal side of an isosceles triangle as diameter bisects the base
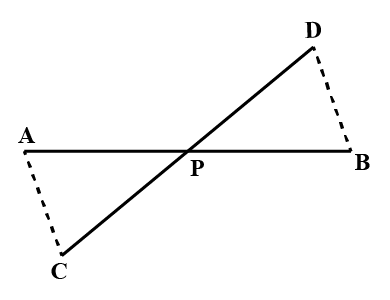
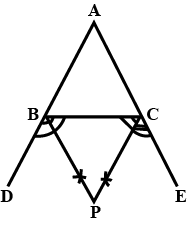
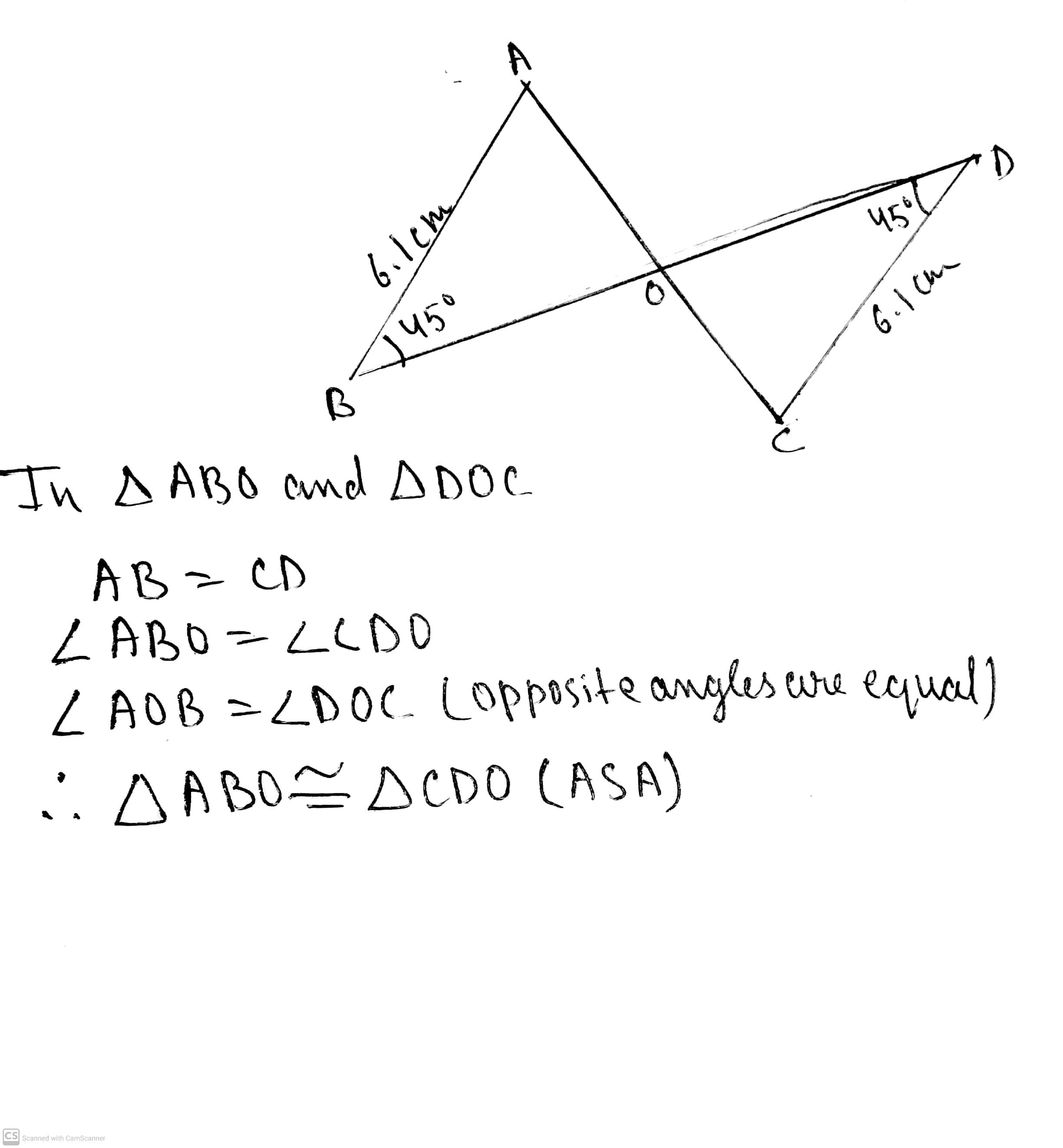
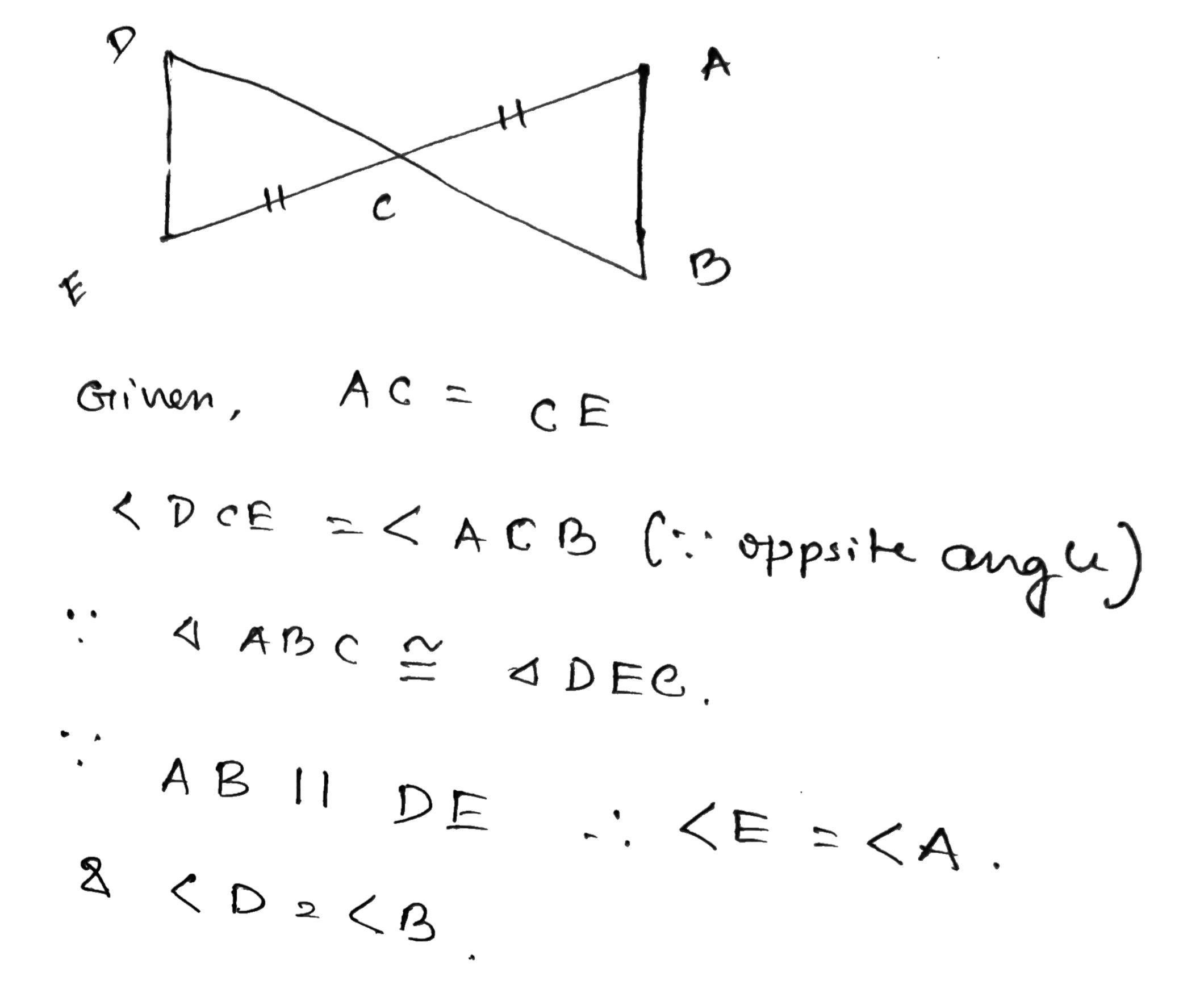
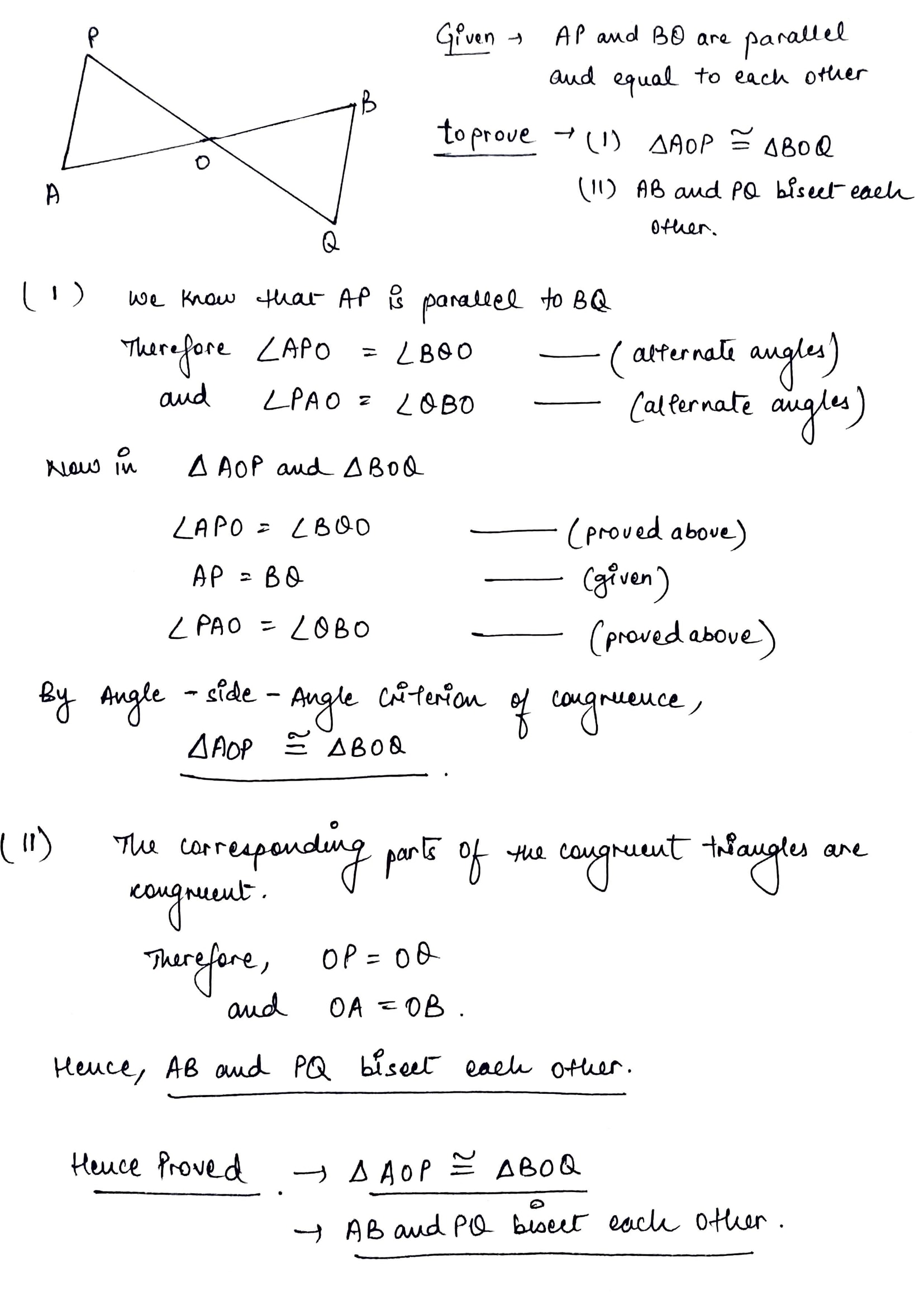
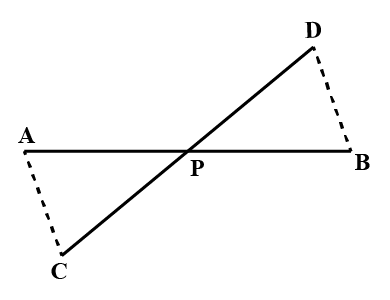
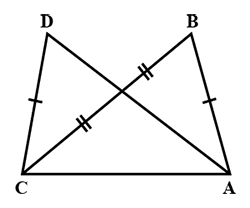
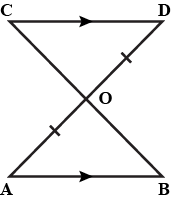
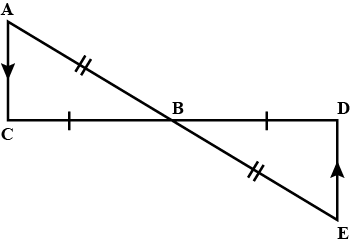
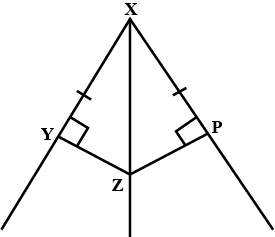
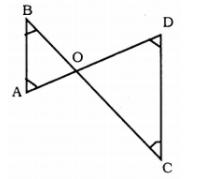
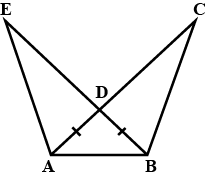
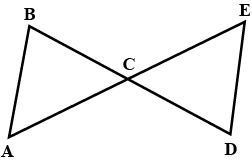
AB is a line segment AX and BY are two equal line segments drawn opposite sides of line AB such that AX ||BY.
If line segments AB and XY intersect each other at point P. Prove that
(a) \Delta APX \cong \Delta BPY (b) line segments AB and XY bisect each other at P.

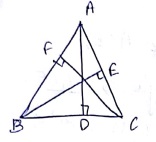
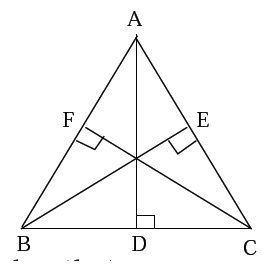
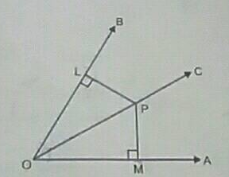
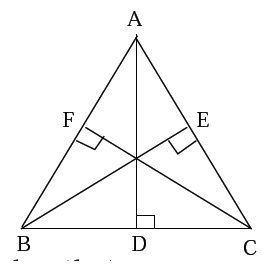
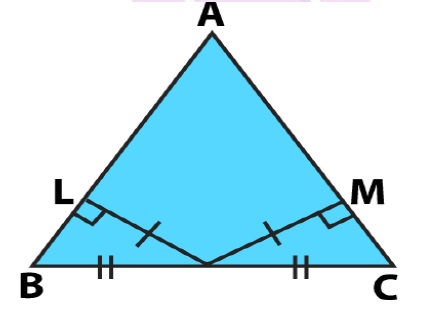
In \Delta ABC, the bisector AX of \angle A intersects BC at X. XL\perp AB and XM \perp AC are drawn. Is XL = XM?
Why or why not?
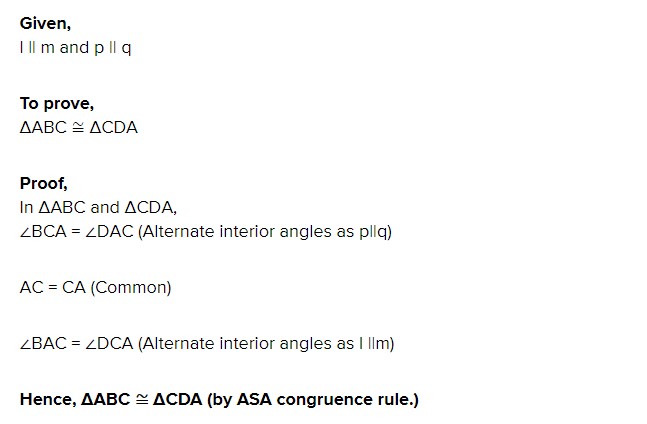
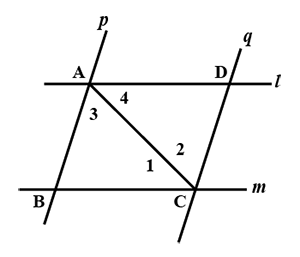
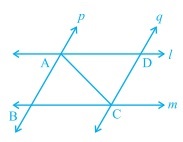
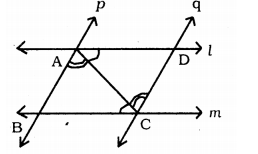
Two parallel lines l and m are intersected by another pair of parallel lines p and q as in the figure. Show that triangles ABC and CDA are congruent.
In the figure AD = BC and BD = CA. Prove that \angle ADB = \angle BCA and \angle DAB = \angle CBA.
In \triangle ABC, AB = BC and \angle B = 64^o. Find \angle C.
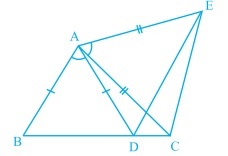
In the figure, C is the mid point of AB, \angle BAD = \angle CBE, \angle ECA= \angle DCB.
Prove that (a) \Delta DAC \cong \Delta EBC (b) DA = EB.
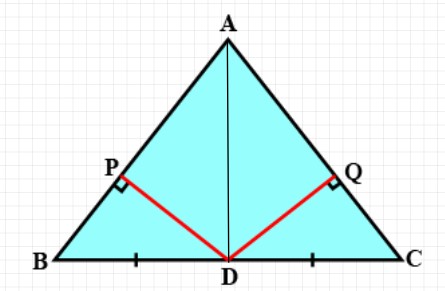
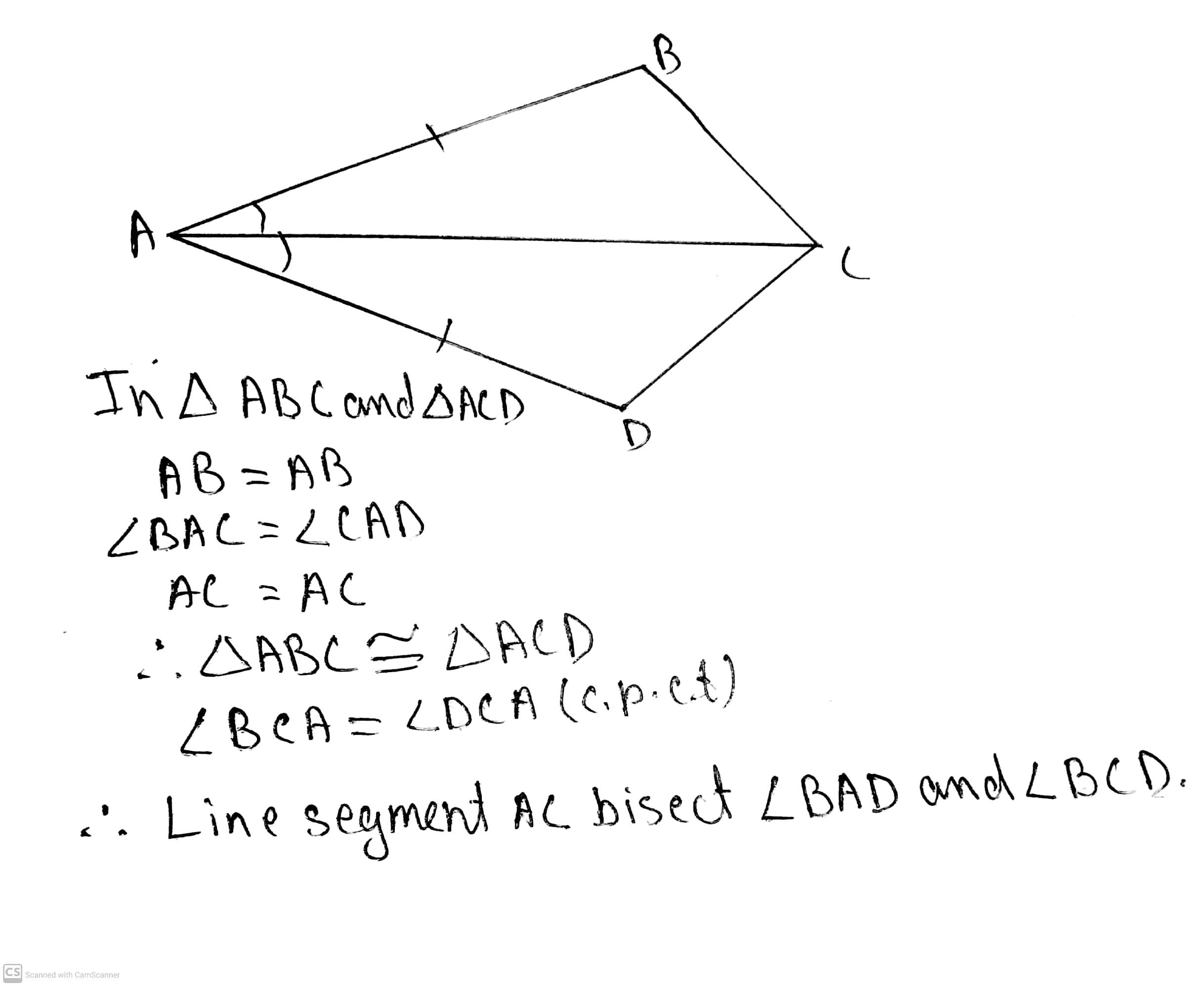
In a quadrilateral ACBC, AC = AD and AB bisect \angle A. Show that \triangle ABC is congruent to \triangle ABD.
In the figure, it is given that AE = AD and BD = CE. Prove that \triangle AEB is congruent \triangle ADC.
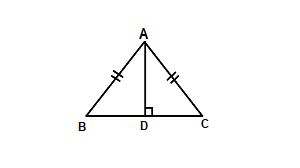
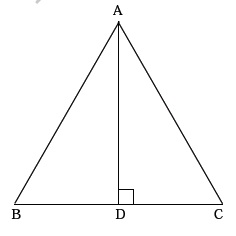
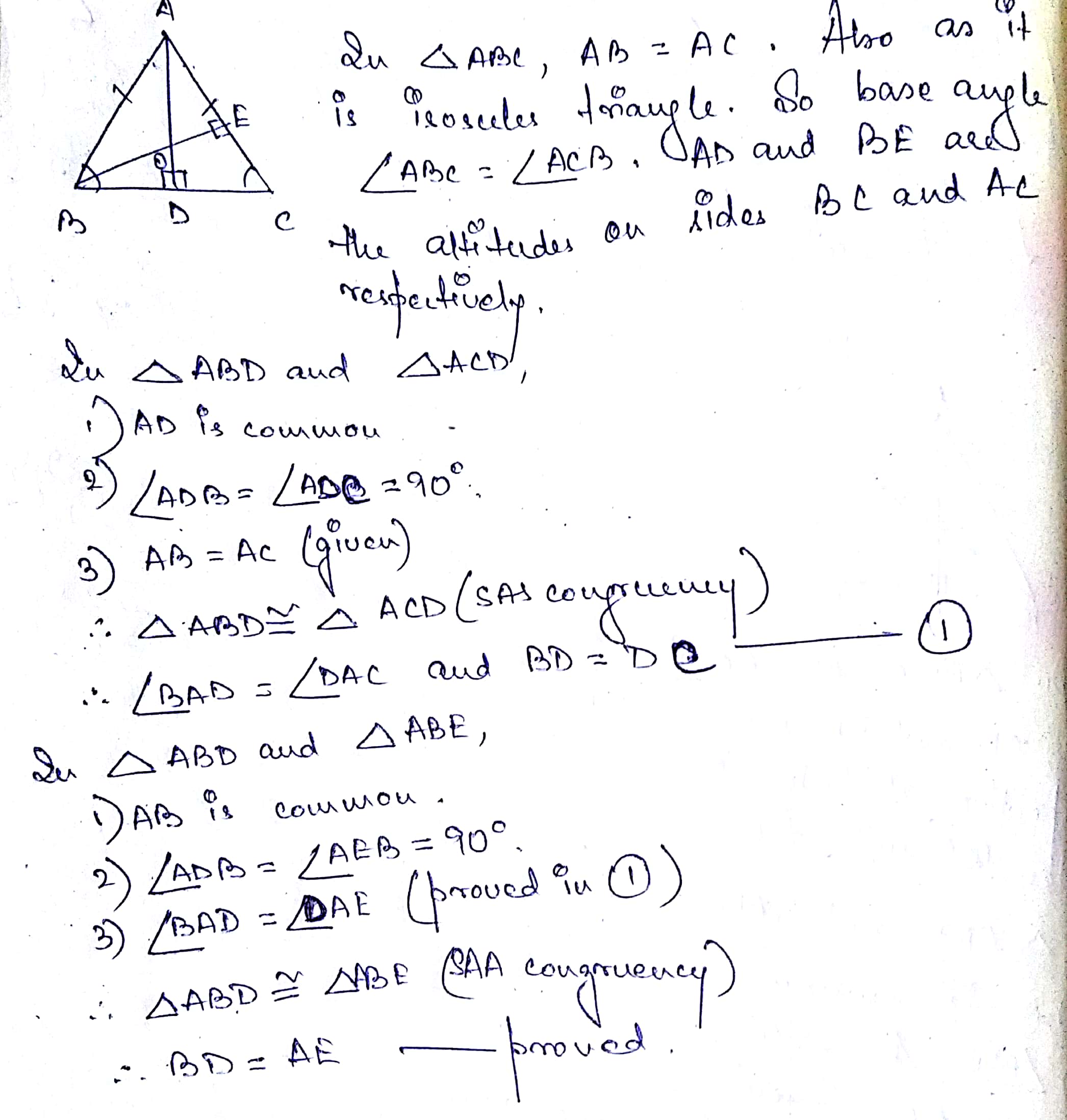
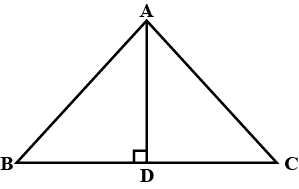
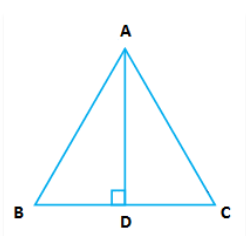
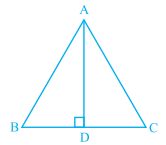
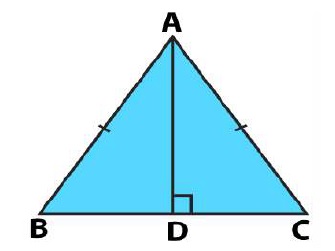
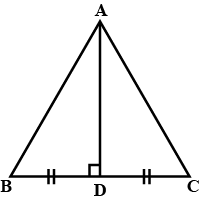
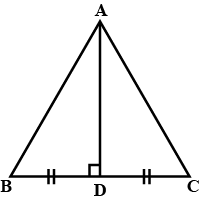
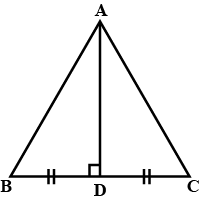
In \triangle ABC, AD is the perpendicular bisector of BC (see given figure). Show that \triangle ABC is an isosceles triangle in which AB = AC
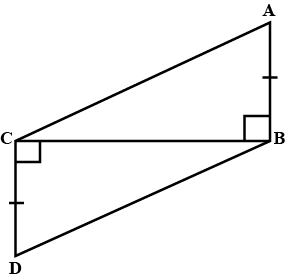
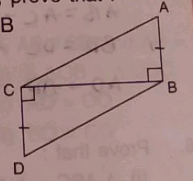
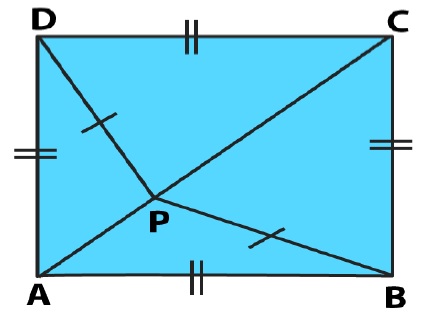
In the given figure, the point P bisects AB and DC. Prove that
\triangle APC\cong \triangle BPD
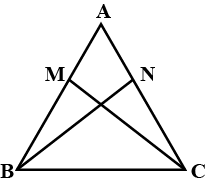
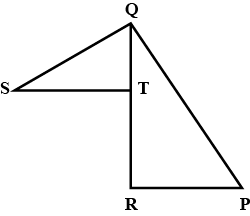
The Indian Navy flight fly in a formation that can be viewed as two triangles with common side. Prove that \triangle SRT \cong \triangle QRT, if T is the midpoint of SQ and SR = RQ.

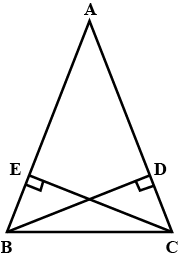
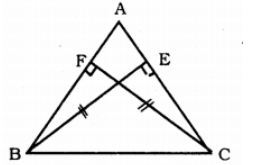
ABC is an isosceles triangle in which altitudes BD and CE are drawn to equal sides AC and AB respectively (see figure). Show that these altitudes are equal
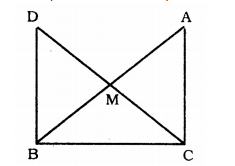
In the figure given below, AB = DC and AC = DB. Is \Delta ABC \cong \Delta DCB.
Complete the congruence statement.
\Delta ABC \equiv ?

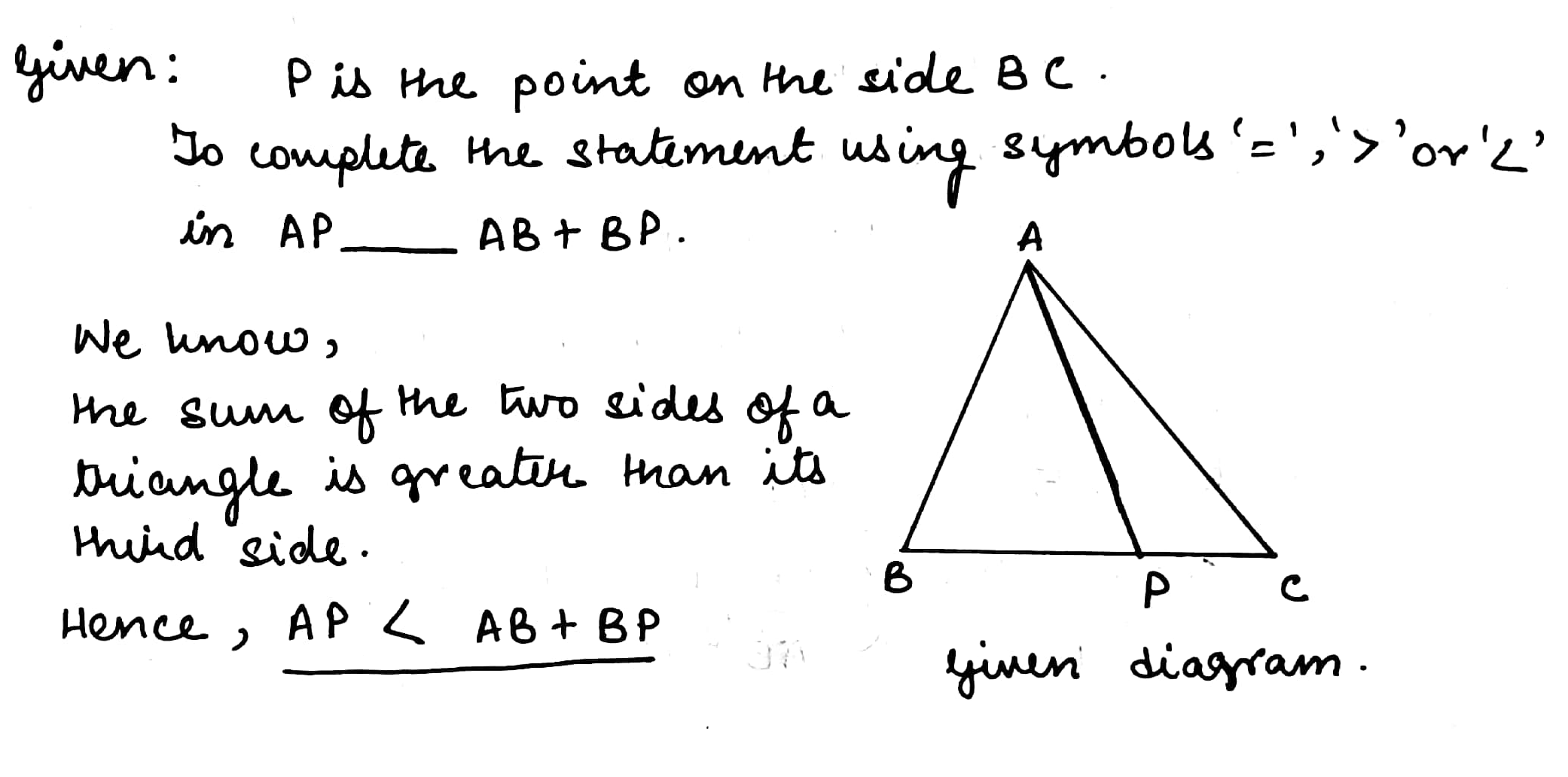
DE = EC show that AB + BC > AD.
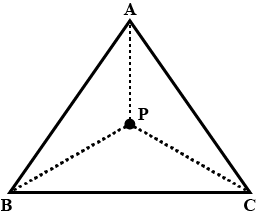
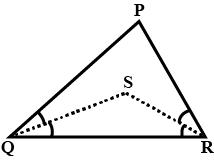
If S is any point in the interior of \Delta PQR, prove that (SQ + SR) < (PQ + PR).
Prove that a median divides a triangle into equal parts
In the adjoining figure \angle{ABD}={132}^{o} & \angle{EAC}={120}^{o}. Prove that AB>AC
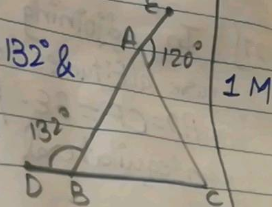
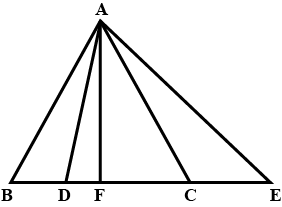
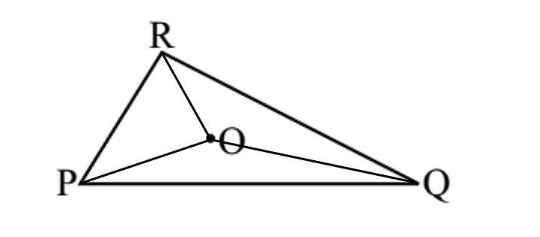
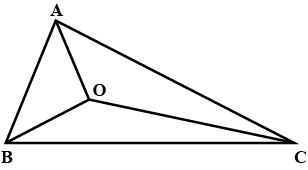
Write down the total number of True statement:Take any point O in the interior of a triangle PQR.Is
(i) OP + OQ > PQ?
(ii) OQ + OR > QR?
(iii) OR + OP > RP?
(i) OP + OQ > PQ?
(ii) OQ + OR > QR?
(iii) OR + OP > RP?
"If two sides and an angle of one triangle are equal to two sides and an angle of another triangle, then the two triangles must be congruent". Is the statement true? Why?
In figure The sides BA and CA have been produced such that BA=AD and CA=AE prove that segment DE\parallel BC
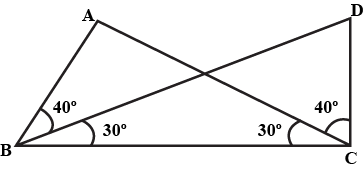
In triangles ABC and PQR, \angle A = \angle Q and \angle B = \angle R. Which side of \Delta PQR should be equal to side AB of \Delta ABC so that the two triangle are congruent? Give reason for your answer.
In fig., PQRS is square and SRT is an equilateral triangle. Prove that
(i) PT = QT (ii) \angle TQR = {15^ \circ }
Give any two real-life examples for congruent shapes.
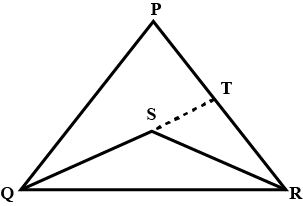
In figure, PR>PO and PS bisect OPR. Prove that \angle{PSR}>\angle{PSO}
If the point p\left( {{a^2},\,a} \right)\, lies in the region. Corresponding in the acute angle between the lines 2y = x\,\,and\,\,4y = x, then find the value of a or the range in which a lies.
In the given fig \triangle PQC & PRC such that QC=CR, PQ=PR. Prove that \triangle PQC\cong\triangle CPR
If the area of two similar triangles are equal, prove that they are congruent.
Give any two real-life examples of congruent shapes.
S and T are respectively the mid points of equal sides PQ and PR of \triangle {PQR}. Show that Qt=RS.
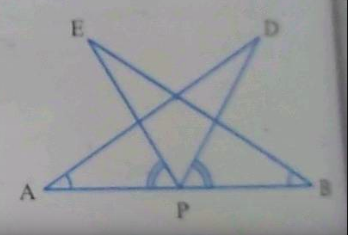
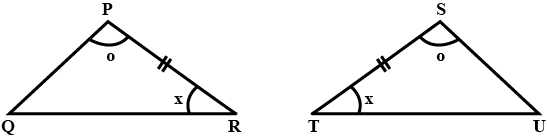
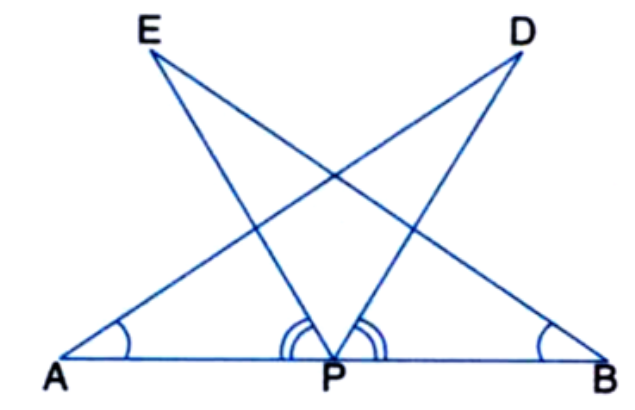
You want to show that \Delta ART \cong \Delta PEN.
If it is given that AT = PN and you are to use ASA criterion, you need to have
i) ?
ii) ?
\DeltaABC and \DeltaDBC are two isosceles triangles on the same base BC and vertices A and D are on the same side of BC. If AD is extended to intersect BC at P, show that \Delta ABP\cong \Delta ACP.

In \triangle PQR, PQ=PR and m \angle P=40^{o} then m \angle Q=.
In a \triangle ABC,BC=a,CA=b and \angle BCA=120^{o},CD is angle bisector of \angle BCA which meets AB on D find the length of CD
In the given figure \Delta ABC \cong \, \Delta ABT, write all the corresponding sides.

In the adjoining figure, PQ=PR and QL=MR. Prove that PL=PM.

Explain, why ?
\Delta A B C = \Delta F E D
In figure ABCD, ABFE and CDEF are parallelograms. Prove that ar(\triangle {ADE})=ar(\triangle{BCF)}
If \bigtriangleup ABC\cong\bigtriangleup FED under\ the\ corresponding \ ABC \leftrightarrow FED. Write all the corresponding congruent part of the triangle.
In the following figure, \angle A = \angle C and AB = BC.
Prove that \Delta ABD \cong \Delta CBE
Prove that in a right angled triangle, hypotenuse is the longest side.
Given: \Delta A \bot AB, CB \bot AB and AC = BD
State 3 points of equal pairs in \Delta ABC & \Delta ABD & Congruence rule.
ABC is a right isosceles triangle right angled at A. Find the value of \angle B and \angle C.
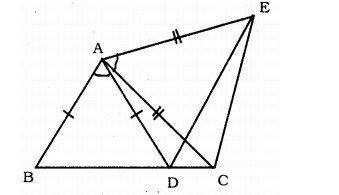
In the given figure, equal sides BA and CA of \Delta ABC are produced to Q and P respectively such that AP = AQ. Prove that PB = QC.
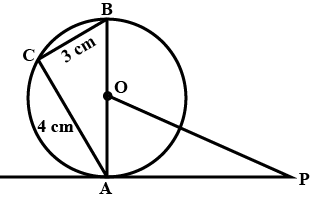
PA is tangent to the circle with centre O. If BC = 3cm, AC = 4 cm and \Delta ACB \sim \Delta PAO, then find OA and \frac{OP}{AP}.
Is it possible to have a triangle with the following sides?2 cm, 3 cm,5 cm
In figure, equal sides have been marked by the same signs.
Can we say \Delta ABC\cong \Delta DBC?
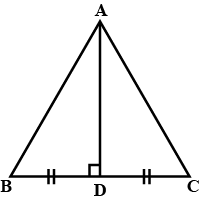
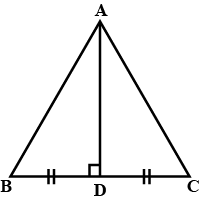
In \triangle ABC, AD is perpendicular bisector of BC (See adjacent figure). Show that \triangle ABC is an isosceles triangle in which AB=AC
In the figure, AP =AQ and BP= BQ.
Prove that AB is the bisector of \angle PAQ and \angle PBQ.
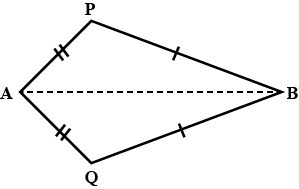
Prove that AB is the bisector of \angle PAQ and \angle PBQ.
In figure, AB=AC and \angle 1=\angle 2. Is \Delta ABD\cong \Delta ACD ?(Give reason)
If \Delta A B C \cong \Delta F E D under the correspondence A B C \leftrightarrow F E D, write all the corresponding congruent parts of the triangles.
If \Delta A B C \cong \triangle N M O , name the congruent sides and angles.
Consider the figure, PQRS is a rectangle. PT and RU are perpendicular from P and R on SQ. Prove that \Delta PTS\cong \Delta QRU
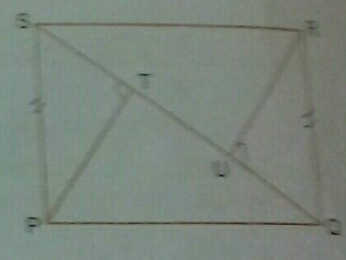
In figure, equal sides have been marked by the same signs.
Is \Delta ABC\cong \Delta DCB?
In the figure, \Delta CDE is an equilateral triangle formed on a side CD of a square ABCD. Show that \Delta ADE \cong \Delta BCE.
In the given figure, prove that:
AC=DB
One of the angles of a \triangle is { 75 }^{ }. If the difference of the other two other is { 35 }^{ }.
Find the largest angle of the \triangle .
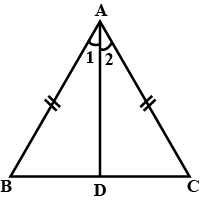
In \triangle{ABC},AB=AC and AD is the perpendicular bisector of BC. Show that \triangle{ADB}\cong\triangle{ADC}
In the given figure, prove that:
\triangle ABC\cong \triangle DCB
If the area of two similar triangles are equal, prove that they are congruent.
I the given figure, we have PQ=SR and PR=SQ. Prove that \triangle PQR \cong \triangle SRQ.
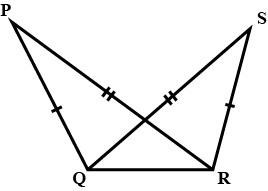
In the givenAB and CD bisect each other at O.State the three pairs of equal parts in two triangles AOC and BOD
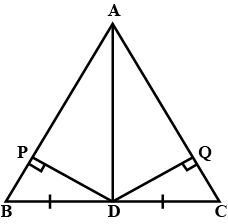
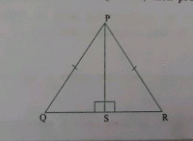
If \Delta PQR is an isosceles triangle such that PQ=PR , then prove that the attitude PS from P on QR bisects QR
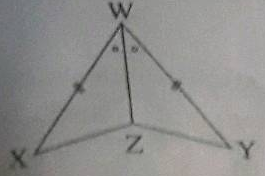
You want to establish \triangle DEF\cong \triangle MNP, using ASA congruence rule. You are given that \angle D=\angle M and \angle F=\angle P. What information is needed to establish the congruence ?
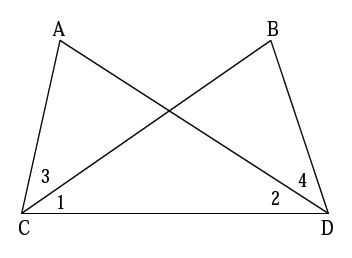
In pair of triangle in the following figure, parts bearing identical marks are congruent. State the test and correspondence if vertices by which triangle in each pair are congurent.
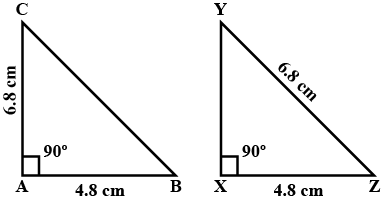
Check whether \triangle{ABC}\cong\triangle{DEF}.Give Reason
In\triangle ABC: AB=6\ cm,\angle B=50^{\circ} and \angle C=90^{\circ}
In \triangle DEF: DE=6\ cm,\angle E=50^{\circ}, \angle F=90^{\circ}.
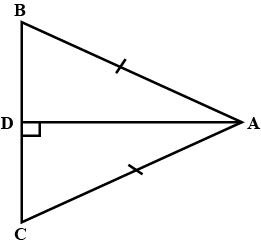
In the given figure, \overline{PL}\bot \overline{OB} and \overline{PM}\bot \overline{OA} such that \overline{PL}=\overline{PM}. Is \triangle PLO\cong \triangle PMO? Give reasons in support of your answer.
Write converse of the theorem "In \triangle ABC, if AB=AC then \angle C=\angle B"
In the given figure, PS is the bisector of \angle QPR of \Delta PQR Prove that \frac{QS}{SR}=\frac{PQ}{PR}

Is it possible to construct a triangle with sides 9 cm, 6 cm and 17 cm ? If not, why ?
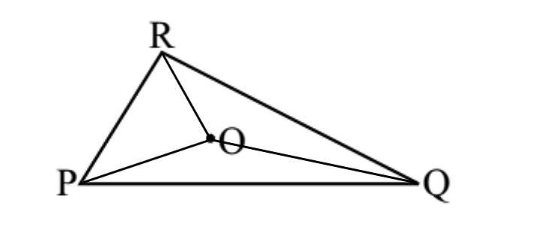
Take any point O in the interior of a \triangle{PQR}.Is OR+OP>RP?
State SSS congruency Rule of traingles.
Take any point O in the interior of a triangle PQR. is
OQ+OR>QR?
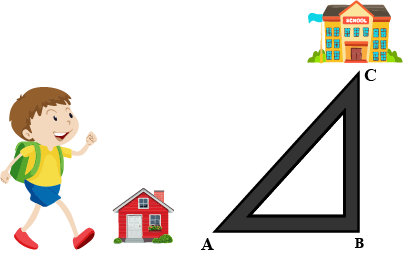
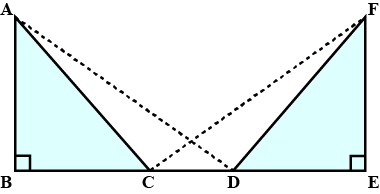
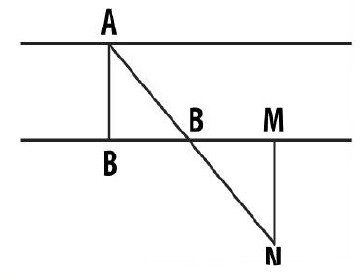
As shown in the figure, Avinash is standing near his house. He can choose from two roads to go to school. Which way is shorter? Explain why.
Give any two real time examples for congruent shapes.
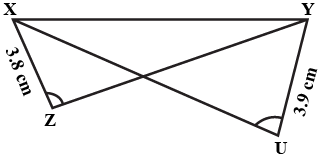
Is \Delta ABC\cong \Delta DEF? Give reasons in support of your answer.

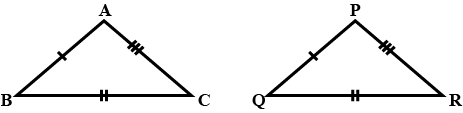
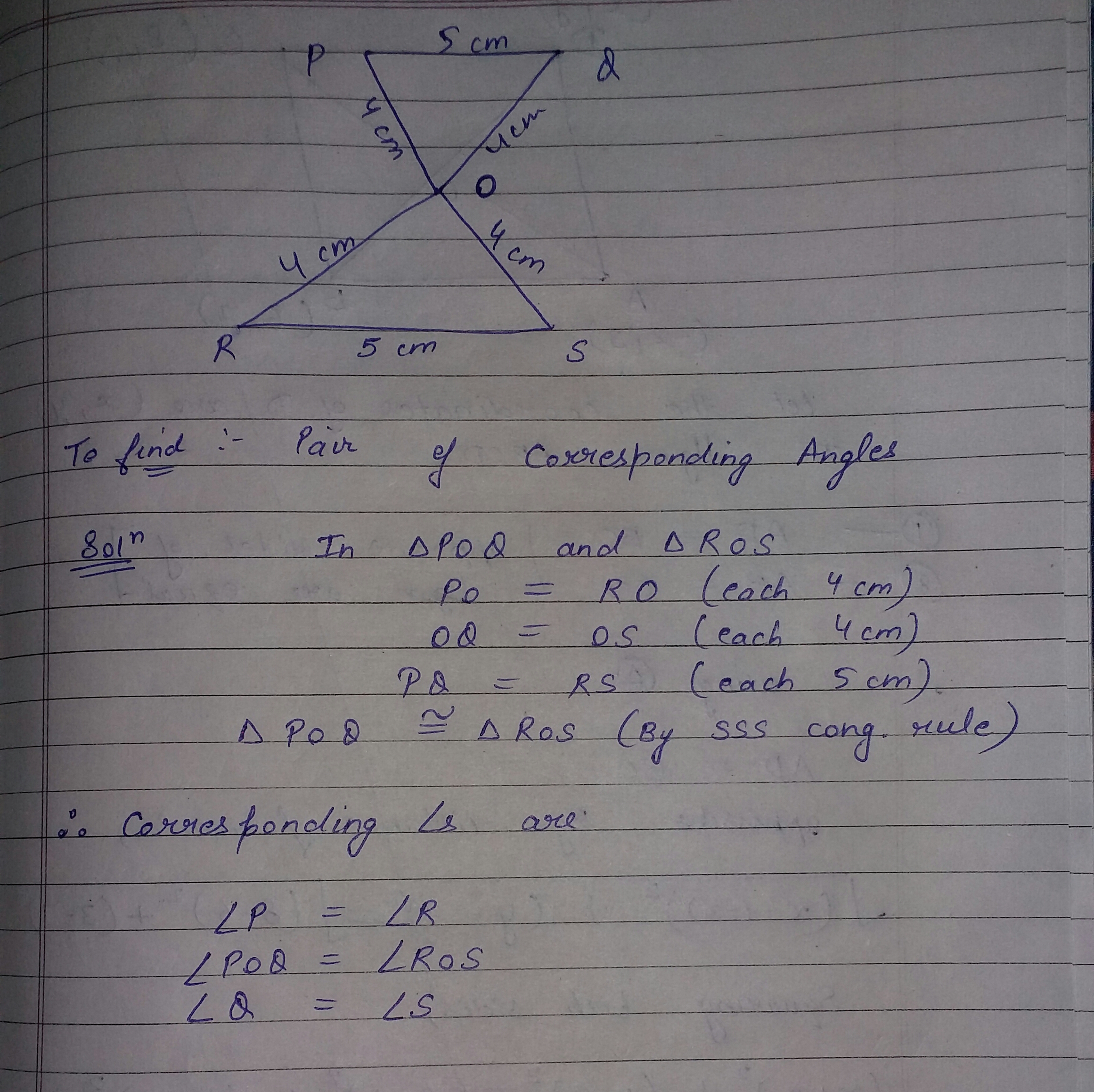
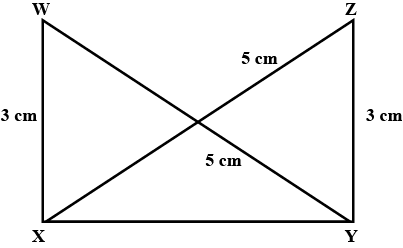
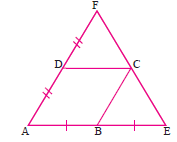
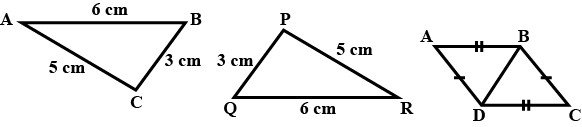
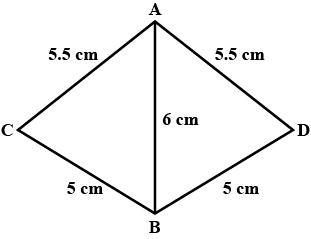
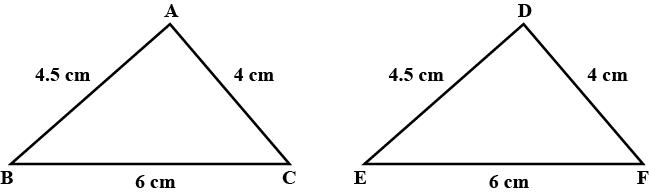
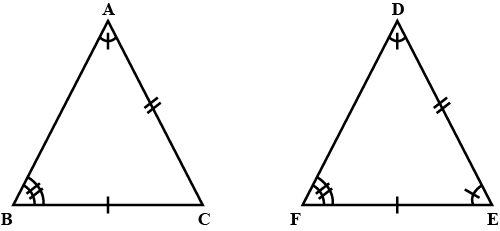
In the following, pairs of triangles of Fig., the lengths of the sides are indicated along the sides. By applying SSS congruence criterion, determine which triangles are congruent. If congruent, write the results in symbolic form.
Without drawing the triangles write all size pairs of equal measures in each of the following pairs of congruent triangles.
\triangle ABC \cong \triangle LMN
In the following, pairs of triangles of Fig., the lengths of the sides are indicated along the sides. By applying SSS congruence criterion, determine which triangles are congruent. If congruent, write the results in symbolic form.
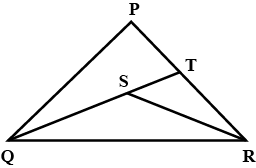
In \triangle PQR and \triangle SQR are both isosceles triangle on a common base QR such that P and S lie on the same side of QR. Are triangles PSQ and PSR congruent ? Which condition do you use ?
In Fig.6.49, it is given that LM = ON and NL = MO
(a) State the three pairs of equal parts in the triangles NOM and MLN.
(b) Is \triangle NOM \cong \triangle MLN. Give reason ?
In the following, pairs of triangles of Fig., the lengths of the sides are indicated along the sides. By applying SSS congruence criterion, determine which triangles are congruent. If congruent, write the results in symbolic form.
In the following, pairs of triangles of Fig. the lengths of the sides are indicated along the sides. By applying SSS congruence criterion, determine which triangles are congruent. If congruent, write the results in symbolic form.
In the following, pairs of triangles of Fig. the lengths of the sides are indicated along the sides. By applying SSS congruence criterion, determine which triangles are congruent. If congruent, write the results in symbolic form.
In the following, pairs of triangles of Fig. the lengths of the sides are indicated along the sides. By applying SSS congruence criterion, determine which triangles are congruent. If congruent, write the results in symbolic form.
Without drawing the triangles write all size pairs of equal measures in each of the following pairs of congruent triangles.
\triangle STU \cong \triangle DEF
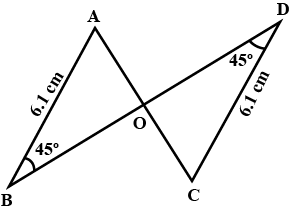
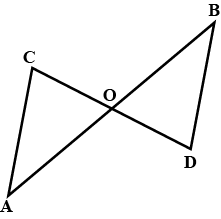
check if the following pair of triangles is congruent? also, state the condition of congruency :
In \Delta ABC and \Delta QRP , AB =QR ,~ \angle B = \angle R and \angle C= \angle P .
In \DeltaDEF, DM and EN are two medians. Prove that 3(DF + EF) > 2(DM + EN).
which of the following pairs of triangle are congruent ? In each case , state the condition of congruency :
In \Delta ABC and \Delta DEF, AB = DE , BE = EF and \angle B = \angle E.
In Fig. which paise of triangles are congruent by SAS congruence criterion (condition)? If congruent, write the congruence of the two triangles in symbolic form.

In Fig. which paise of triangles are congruent by SAS congruence criterion (condition)? If congruent, write the congruence of the two triangles in symbolic form.
In Fig. which paise of triangles are congruent by SAS congruence criterion (condition)? If congruent, write the congruence of the two triangles in symbolic form.
In Fig. which paise of triangles are congruent by SAS congruence criterion (condition)? If congruent, write the congruence of the two triangles in symbolic form.
In \Delta ABC and \Delta DEF \angle B = \angle E= 90^{o}: AC = DF and BC = EF
In Fig. which paise of triangles are congruent by SAS congruence criterion (condition)? If congruent, write the congruence of the two triangles in symbolic form.

In Fig. which paise of triangles are congruent by SAS congruence criterion (condition)? If congruent, write the congruence of the two triangles in symbolic form.

From the following figure: prove that
AB + AC > BC
D is a point in side BC of triangle ABC. If AD > AC show that AB > AC.
From the following figure: prove that
AC > CD
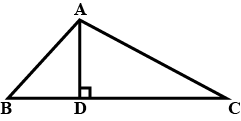
In the following figure, ABC is an equilateral triangle P is any point in AC; prove that:
BP > PA
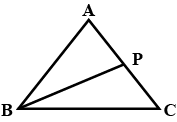
From the following figure: prove that
AB > BD
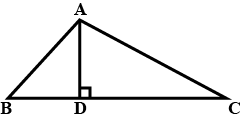
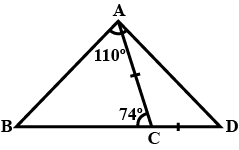
In the following figure:
AC=CD; \angle BAD =1100 and \angle ACB =740
Prove that: BC > CD
Which of the following pairs of triangle are congruent ? In each case , state the condition of congruency :
In \Delta ABC and \Delta PQR , AB =PQ ,AC = PR and BC = QR .
In the following figure, \angle BAC =60^o and \angle ABC=65^o
Prove that:
CF > AF
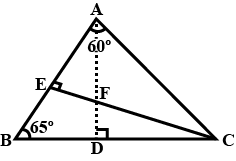
In the following figure, \angle BAC =60^o and \angle ABC=65^o
Prove that:
DC > DF

Which of the following pairs of triangle are congruent ? In each case , state the condition of congruency :
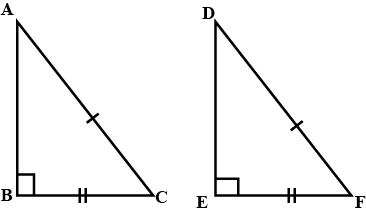
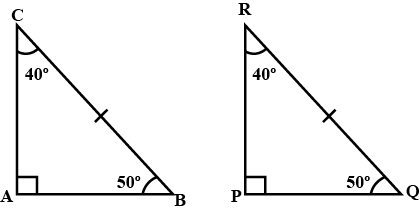
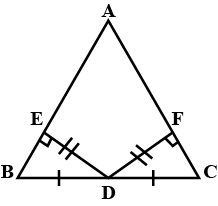
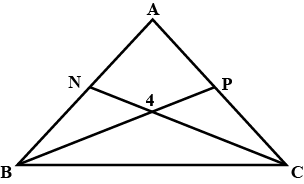
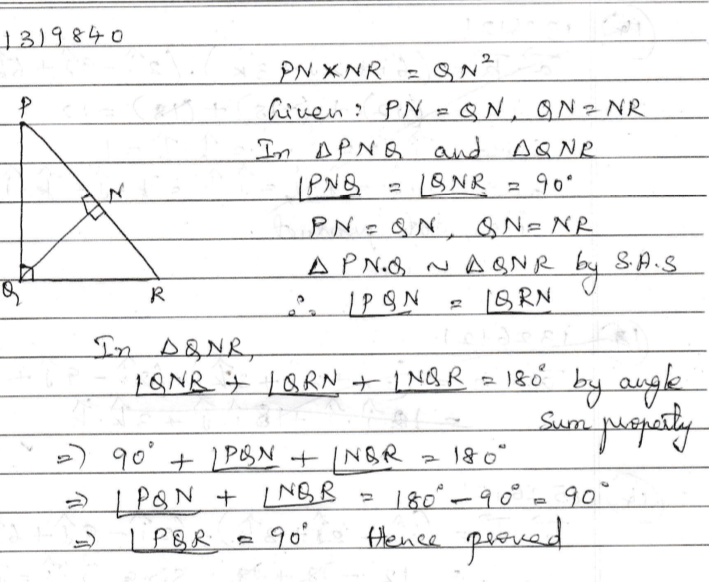
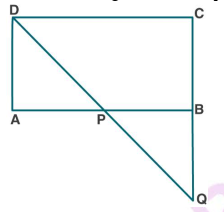
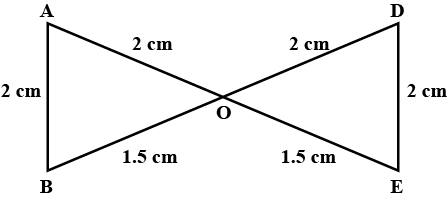
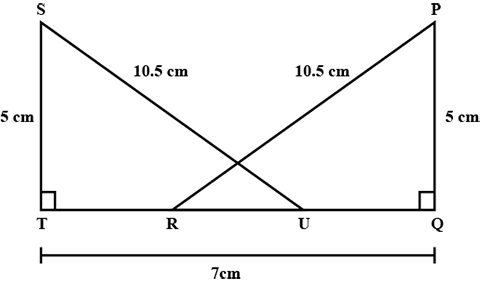
In \Delta ABC and \Delta PQR , BC = QR = \angle A = 90^{o} , \angle C= \angle R = 40 ^{o} and \angle Q = 50^{o}
In the following figure, ABC is an equilateral triangle P is any point in AC; prove that:
BP > PC
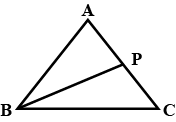
P is any point inside the triangle ABC. Prove that \angle BPC > \angle BAC.
The sides AB and AC of a triangle ABC are produced; and the bisects of the external angles at B and C meet at P. Prove that if AB > AC, then PC > PB.
In quadrilateral ABCD, side AB is the longest and side DC is the shortest. Prove that:
\angle C > \angle A
In a triangle ABC, D is the midpoint of BC; AD is produced up to E so that DE = AD prove that:
\angle DAB = \angle DEC
In the given figure, AB=AC. Prove that:
DP=DQ
In isosceles triangle ABC sides, AB and AC are equal. If point D lies in base BC and point E lies on BC produced (BC being produced to vertex C). prove that:
AC > AD
Prove that the straight line joining the vertex of an isosceles triangle to any point in the base is smaller than either of the equal sides of the triangle.
In a triangle ABC , D is mid - point of BC ; AD is produced upto E so that DE = AD prove that :
\Delta ABD and \Delta ECD are congruent
In a triangle ABC , D is mid - point of BC ; AD is produced upto E so that DE = AD prove that :
AB = EC
In isosceles triangle ABC sides, AB and AC are equal. If point D lies in base BC and point E lies on BC produced (BC being produced vertex C). prove that:
AE > AC
In the given figure AB / FD , AC/GE and BD = CE : prove that :
CF = EG
In isosceles triangle ABC sides, AB and AC are equal. If point D lies in base BC and point E lies on BC produced (BC being produced vertex C). prove that:
AE > AD
Given: ED=EC
Prove : AB + AD > BC
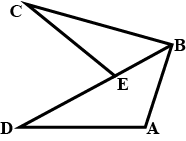
In triangle ABC, AB > AC and D is a point in side BC. Show that: AB > AD.

In the following figure AB = AC and AD is perpendicular to BC . BE bisects angle B and EF is perpendicular to AB
Prove that
ED = EF
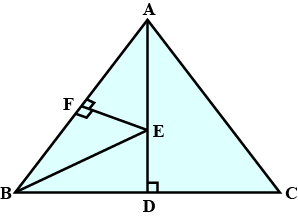
In the given figure, AB=AC. Prove that:
AP=AQ
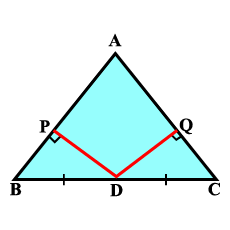
Use the information in the given figure to prove ;
AB = FE
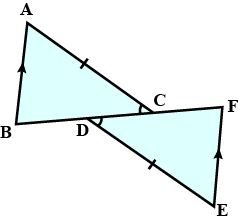
A triangles ABC has \angle B = \angle C
Prove that
The perpendicular from the mid - point of BC to AB and AC are equal'
A triangles ABC has \angle B = \angle C
Prove that
The perpendicular from B and C to the opposite sides are equal .
Use the information in the given figure to prove ;
BD = CF
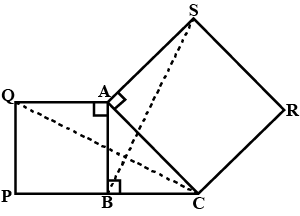
In the figure given below triangles ABC is right-angled at B .\ ABPQ and ACRS are squares. Prove that :
\Delta ACQ and \Delta ASB are congruent
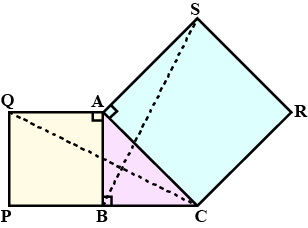
In the figure given below triangles ABC is right - angled at B . ABPQ and ACRS are square . Prove that :
CQ = BS
Use the information in the given figure to prove ;
BC = DF
In triangle ABC ; AB=AC.\ P,Q and R are mid-points of sides AB,AC and BC respectively. Prove that:
PR=QR
In the given figure, AB=AC and \angle DBC=\angle ECB=90^{o}.
BD=CE
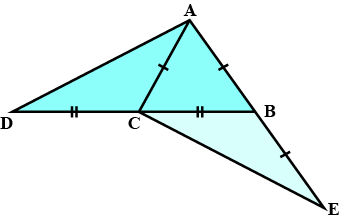
In triangle ABC ; AB=AC.\ P,Q and R are mid-points of sides AB,AC and BC respectively. Prove that:
BQ=CP
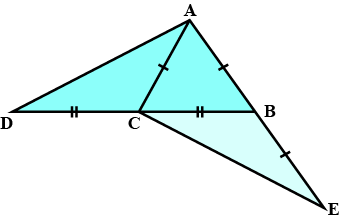
In the given figure, AB=AC and \angle DBC=\angle ECB=90^{o}.
AD=AE
From the following figure, Prove that:
AD=CE
From the following figure, Prove that:
\angle ACD=\angle CBE
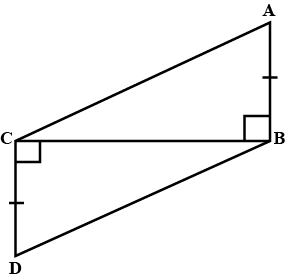
In the following figure AB = EF , BC = DE and \angle B = \angle E = 90^{o}
Prove that AD = FC
In the following figure , OA = OC and AB = BC ,
prove that :
\angle AOB = 90^{o}
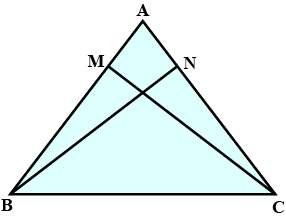
The following figure shows a triangle ABC in which AB = AC . M is a point on AB and N is a point AC such that BM = CN
Prove that
AM = AN
In the adjoining figure If \angle C = \angle F , then AB = ______ and BC _______
In the following figure , OA = OC and AB = BC ,
prove that :
AD = CD
In triangle ABC,D is a points on BC such that Ab=AD=BD=DC. Show that:
\angle ADC:\angle C=4:1
M is a point on side BC of a triangle ABC such that AM is the bisector of \angle BAC. Is it true to say that perimeter of the triangle is greater than 2 AM? If True enter 1, else if False enter 0.
Show that no triangle has two sides each shorter than its corresponding altitude (from the opposite vertex).
Let a, b, c be the three sides of a triangle. Suppose that \dfrac{a}{b}=\dfrac{b}{c}=q. Prove that \dfrac{\sqrt{5}-1}{2}<q<\dfrac{\sqrt{5}+1}{2}
The sides of a triangle have lengths 11, ~15 and k, where k is an integer. For how many values of k is the triangle obtuse?
Show that in any triangle with sides a, b and c, we have (a + b + c)^2 < 4 (ab + bc + ca).
State whether the given statement is true/false:
In the following diagram, AB = AC then which of the following is correct?
AE>AF
AF>AE
In the given figure,\displaystyle BC=CE and \displaystyle \angle 1=\angle 2 Then,
:\displaystyle \Delta GCB \equiv \Delta DCE
If the above statement is true then mention answer as 1, else mention 0 if false
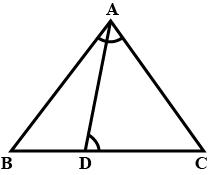
In \triangle ABC, AB=AC and bisector of angle A meets BC at D, then prove that AD is perpendicular to BC.
AB + BC + CD > DA
If the above statement is true, then enter 1 or else enter 0.
The given figure shows \displaystyle PQ=PR and \displaystyle \angle Q=\angle R Prove that \displaystyle \Delta PQS \equiv \Delta PRT
The given figure shows a triangle ABC in which AB = AC. M is a point on AB and N is a point on AC such that BM = CN. Hence, BN=CM.
If the above statement is true, then mention answer as 1, else mention 0 if false.
In triangle ABC, AB > AC and D is a point in side BC.
then, AB > AD.
If the above statement is true then mention answer as 1, else mention 0 if false
AB and PQ bisect each other.
If the above statement is true then mention answer as 1, else mention 0 if false.
In the given triangle ABC, D, E and F are the mid-points of sides BC,CA and AB respectively. Prove that
\displaystyle \dfrac{AB-BC}{2}< AE< \dfrac{AB+BC}{2}.

\displaystyle \dfrac{AB-BC}{2}< AE< \dfrac{AB+BC}{2}.
Show that in an isosceles triangle angles opposite to equal sides are equal
Using triangle inequality theorem check whether the given side lengths a = 3, b = 5 and c = 1 will form a triangle or not.
Using triangle inequality theorem check whether the given side lengths a = 4, b = 5 and c = 8 will form a triangle or not.
In Fig, AC = AE, AB = AD and \angle BAD = \angle EAC. Show that BC = DE
In right angled triangle ABC, right angled at C, M is the mid-point of hypotenuse AB. C is joined to M and produced to a point D such that DM = CM. Point D is joined to point B. Show that:
(i) \triangle AMC\cong \triangle BMD
(ii) \angle DBC is a right angle.
(iii) \triangle DBC\cong \triangle ACB
(iv) CM = \dfrac {1}{2} AB
ABCD is a quadrilateral in which AD = BC and \angle DAB = \angle CBA . Prove that
(i) \triangle ABD \cong\triangle BAC
(ii) BD = AC
(iii) \angle ABD = \angle BAC

In \triangle ABC, AD is the perpendicular bisector of BC. Show that \triangle ABC is an isosceles triangle in which AB = AC.
In an isosceles triangle ABC, with AB = AC, the bisectors of \angle B and \angle C intersect each other at O. Join A to O. Show that :
(i) OB = OC (ii) AO bisects \angle A
l and m are two parallel lines intersected by another pair of parallel lines p and q. Show that \triangle ABC \cong \triangle CDA
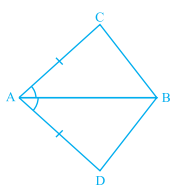
In quadrilateral ACBD, AC = AD and AB bisects \angle A. Show that \triangle ABC \equiv \triangle ABD. What can you say about BC and BD
AB is a line segment and P is its mid-point. D and E are points on the same side of AB such that \angle BAD = \angle ABE and \angle EPA = \angle DPB. Show that
(i) \triangle DAP \cong \triangle EBP
(ii) AD = DE
In fig, ABC is a right triangle right angled at A.BCED, ACFG and ABMN are square on the sides BC, CA and AB respectively. Line segment AX\perp DE meets BC at Y. Show that:
(i) \triangle MBC\cong \triangle ABD
(ii) ar(BYXD) = 2ar(MBC)
(iii) ar(BYXD) = ar (ABMN)
(iv) \triangle FCB\cong \triangle ACE
(v) ar(CYXE) = 2ar (FCB)
(vi) ar(CYXE) = ar(ACFG)
(vii) ar(BCED) = ar(ABMN) + ar(ACFG)
Result (vii) is the famous Theorem of Pythagoras. You shall learn a simpler
proof of this theorem in Class X.
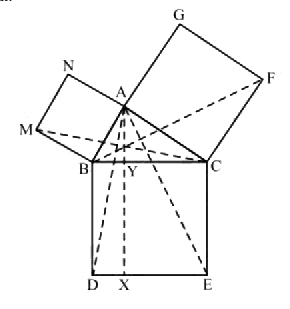
In \triangle ABC and \triangle DEF, AB = DE, AB \parallel DE, BC = EF and BC \parallel EF. Vertices A, B and C are joined to vertices D, E and F respectively. Show that
(i) Quadrilateral ABED is a parallelogram
(ii) Quadrilateral BEFC is a parallelogram
(ii) AD \parallel CF and AD = CF
(iv) Quadrilateral ACFD is a parallelogram
(v) AC = DF
(vi) \triangle ABC \cong \triangle DEF
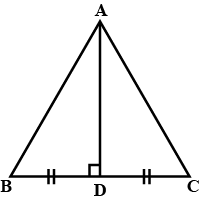
ABC is an isosceles triangle with AB = AC. Draw AP \perp BC. Show that \angle B = \angle C
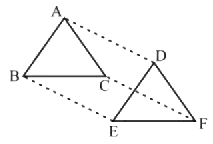
\triangle ABC and \triangle DBC are two isosceles triangles on the same base BC and vertices A and D are on the same side of BC. If AD is extended to intersect BC at P, show that
(i) \triangle ABD \cong \triangle ACD
(ii) \triangle ABP \cong \triangle ACP
(iii) AP bisects \angle A as well as \triangle D.
(iv) AP is the perpendicular bisector of BC.
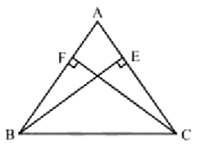
ABC is a triangle in which altitudes BE and CF to sides AC and AB are equal (see Fig.). Show that
(i) \triangle ABE\cong \triangle ACF
(ii) AB = AC, i.e., ABC is an isosceles triangle.
AD is an altitude of an isosceles triangle ABC in which AB = AC. Show that
(i) AD bisects BC (ii) AD bisects \angle A
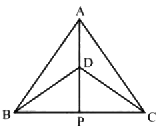
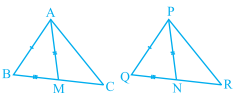
In given figures two sides AB and BC and median AM of one triangle ABC are respectively equal to sides PQ and QR and median PN of \triangle PQR. Show that:
(i) \triangle ABM\cong \triangle PQN
(ii) \triangle ABC \cong \triangle PQR
BE and CF are two equal altitudes of a triangle ABC. Using RHS congruence rule, prove that the triangle ABC is isosceles.
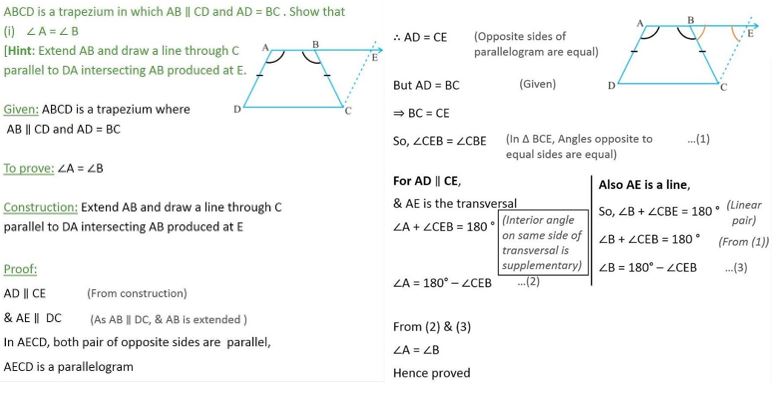
In given figure ABCD is a trapezium in which AB\parallel CD and AD = BC. Show that
(i) \angle A = \angle B
(ii) \angle C = \angle D
(iii) \triangle ABC \cong \triangle BAD
(iv) diagonal AC = diagonal BD
Show that the sum of the three altitudes of a triangle is less than the sum of its three sides.
In \Delta ABC \,and \, \Delta PQR, AB = PQ, BC = QR; CB and RQ are extended to X and Y respectively. \angle ABX= \angle PQY. Prove that \Delta ABC = \Delta PQR.
In the given figure, AB = CF, EF = BD \angle AFE = \angle DBC. Prove that \Delta AFE = \Delta CBD

In the figure, \angle BCD = \angle ADC and \angle ACB = \angle BDA. Prove that AD = BC and \angle A = \angle B.
In a \triangle ABC, AB = AC and \angle A = 50^o. Find \angle B and \angle C.
In a \triangle ABC, we have AB = 4 \ cm, BC = 5.6 \ cm and CA = 7.6 \ cm. Write the angles of the triangle in ascending order of measures.
In the given figure, find the value of x
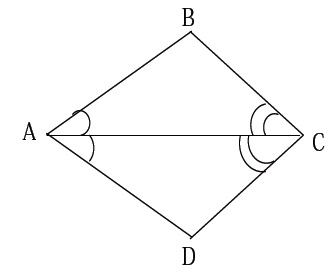
In given figure diagonal AC of a quadrilateral, ABCD bisects the angles \angle A and \angle C. Prove that AB = AD and CB = CD.
In the figure O is the midpoint of AB and CD. Prove that
(i) \triangle AOC \cong \triangle BOD; (ii) AC = BD.

In the figure, find the value of x.
In a triangle ABC, AB = AC and the bisectors of angles B and C intersect at O. Prove that BO = CO and AO is the bisector of angle \angle BAC.
In the figure AB = AC and DB = DC. Prove that \angle ABD = \angle ACD.

In given figure the altitudes AD, BE and CF of triangle ABC are equal. Prove that ABC is an equilateral triangle.
If \triangle ABC \cong \triangle NMO, name the congruent sides and angles.
Prove that the base angles of an isosceles trapezium are equal
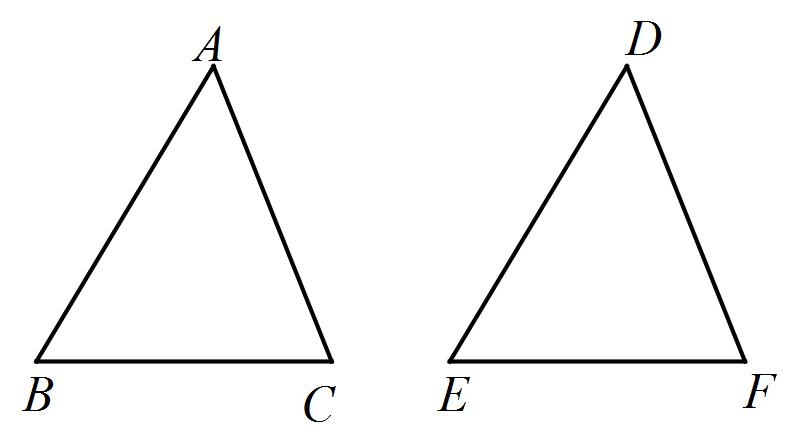
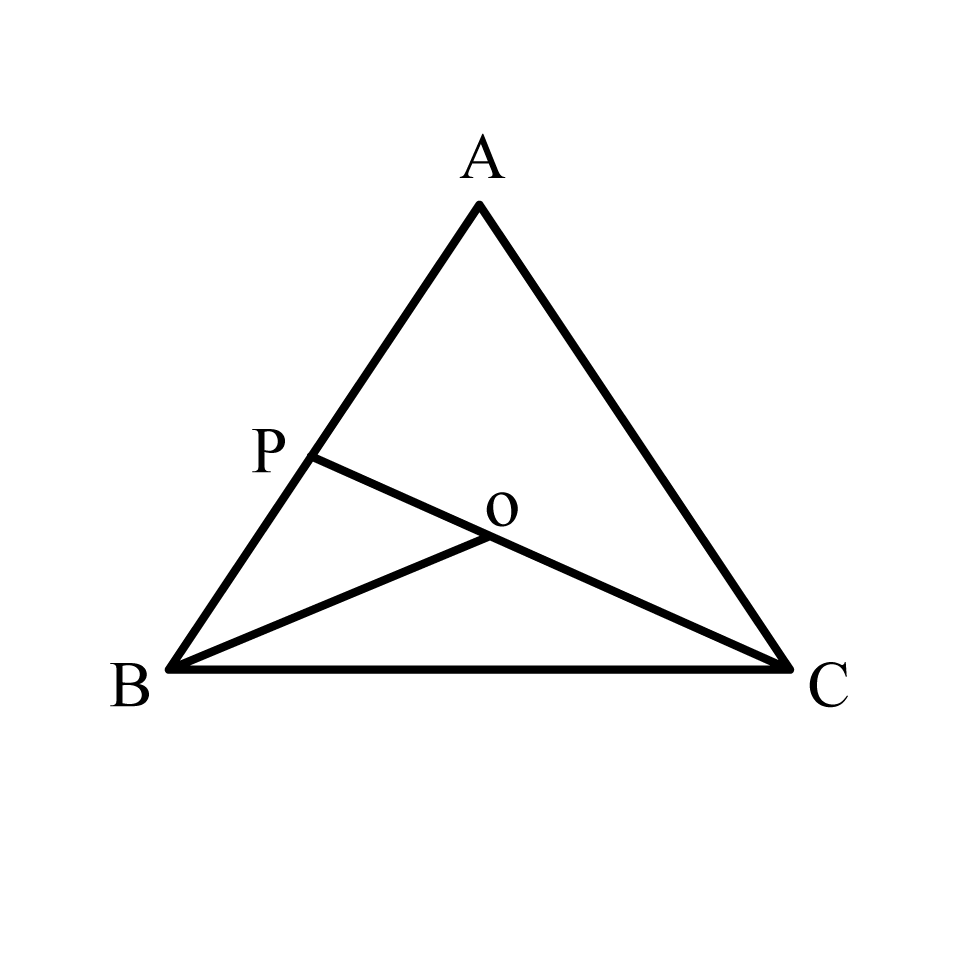
Which minimum measurements do you require to check if the given figures are congruent:
Two rectangles
What are the minimum measurements required for two rhombuses to be congruent?
Suppose ABC is an isosceles triangle such that AB = AC and AD is the altitude from A on BC. Prove that (i) AD bisects \angle A, (ii) AD bisects BC.
State whether the following triangles are congruent or not? Give reasons for your answer
In the figure, it is given that AB = CD and AD = BC. Prove that triangles ADC and CBA are congruent.
State whether the following triangles are congruent or not? Give reasons for your answer.
In the adjoining figure, AB = CD and AD = BC. Show that \angle 1 = \angle 2

\triangle ABC and \triangle DBC are two isosceles triangles on the same base BC (see figure). Show that \angle ABD =\angle ACD
l and m are two parallel lines intersected by another pair of parallel lines p and q. Show that \triangle ABC\cong \triangle CDA

BE and CF are two equal altitudes of a triangle ABC. Using RHS congruence rule, prove that the triangle ABC is isosceles
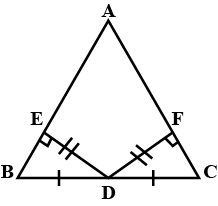
In the adjacent figure \triangle ABC, D is the midpoint of BC. DE\perp AB, DF\perp AC and DE = DF. Show that \triangle BED \cong \triangle CFD
In the adjacent figure \triangle ABC is isosceles as \overline {AB} = \overline {AC}, \overline {BA} and \overline {CA} are produced to Q and P such that \overline {AQ} = \overline {AP}. Show that \overline {PB} = \overline {QC}
In given figure AD is an altitude of an isosceles triangle ABC in which AB = AC.
Show that, AD bisects \angle A
In the adjacent figure ABCD is a square and \triangle APB is an equilateral triangle. Prove that \triangle APD\cong \triangle BPC
ABC is a right angled triangle in which \angle A = 90^{\circ} and AB = AC. Show that \angle B = \angle C.
\triangle ABC is an isosceles triangle in which AB = AC. Show that \angle B = \angle C
Line-segment AB is parallel to another line-segment CD. O is the mid-point of AD.
Show that O is also the midpoint of BC.
If two sides of a triangle measure 4cm and 6cm find all possible measurement (positive Integers) of the third side. How many distinct triangles can be obtained?
In \triangle ABC, the bisector AD of A is perpendicular to side BC. Show that AB = AC and \triangle ABC is isosceles.
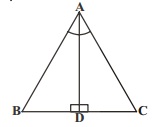
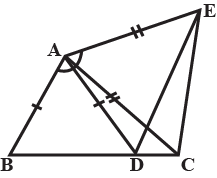
In adjacent figure, PR > PQ and PS bisects \angle QPR. Prove that \angle PSR > \angle PSQ
In the figure, AB\parallel DC and AD\parallel BC Show that \triangle ABC \cong \triangle CDA.

Show that in a right triangle, the hypotenuse is the longest side
In adjacent figure, \angle B < \angle A and \angle C < \angle D. Show that AD < BC

In the given figure, AD is perpendicular to BC and EF \parallel BC, if \angle EAB = \angle FAC, show that triangles ABD and ACD are congruent.
Also, find the values of x and y if AB = 2x + 3, AC = 3y + 1, BD = x and DC = y + 1
In the given figure, AL\parallel DC, E is mid point of BC. Show that \triangle EBL\cong \triangle ECD

In the given figure, AB and CD intersect at 'O', OA = OB and OD = OC. Show that \triangle AOD \cong \triangle BOC.
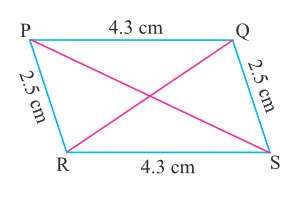
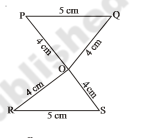
In the figure , PQSR is a parallelogram.
PQ=4.3cm and QR=2.5cm. Is \triangle PQR\equiv \triangle PSR?
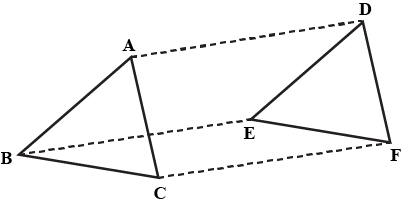
In \triangle^{s}ABC and DEF, AB\parallel DE; BC = EF and BC\parallel EF. Vertices, A, B and C are joined to vertices D, E and F respectively (see figure). Show that
\triangle ABC \cong \triangle DEF
In the figure, ABCD is a parallelogram is produced to E such that AB = BE. AD produced to F such that AD = DF. Show that \triangle FCD \cong \triangle CBE.
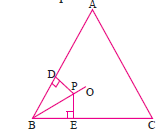
In figure, BO bisects \angle ABC of \triangle ABC. P is any point on BO. Prove that the perpendicular drawn from P to BA and BC are equal.
In given figure D is a point on side BC of \triangle ABC such that AD = AC. Show that AB > AD
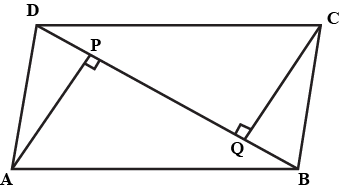
Prove that, if the areas of two similar triangles are equal, then they are congruent.
ABCD is a parallelogram. AP and CQ are perpendicular lines drawn from vertices A and C respectively on diagonal BD (see figure). Show that
\triangle APB\cong \triangle CQD
From the given figures, state whether the given pairs of triangles are congruent by SSS axiom
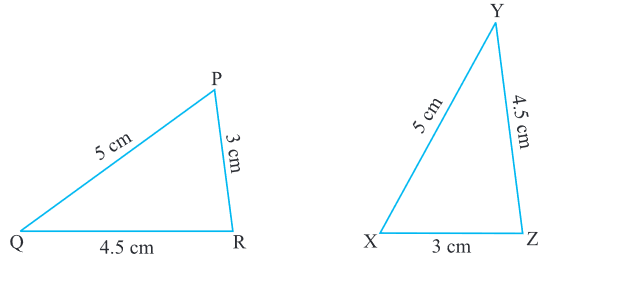
Decide whether the SSS congruence is true with the following figures. Give reasons
What additional information do you need to conclude that the two triangles given here under are congruent using SAS rule?(When\,\, HG = TR )
For the following congruent triangles, find the pairs of corresponding angles.

Decide whether the SSS congruence is true with the following figures. Give reasons
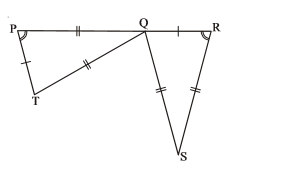
Complete the congruence statement.
\Delta QRS \cong
You want to show that \Delta ART \cong \Delta PEN,
If it is given that \angle T = \angle N and you are to use SAS criterion, you need to have
(a) RT =______(b) PN =______
In Fig. AC = AE, AB = AD and \angle BAD = \angle EAC. Show that BC = DE.
In the given figure, if AC=DC and CB=CE, then prove that AB=DE (State Euclid's A Used
O is a point in the interior of a square ABCD such that OAB is an equilateral triangle show that \triangle OCD is an isosceles triangle
Prove that the lines:
(a+b)x+(a-b)y-2ab=0.......(1)
(a-b)x+(a+b)y-2ab=0.......(2)
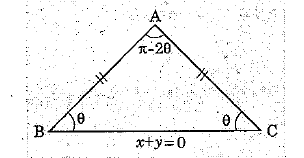
and x+y=0......(3) form an isosceles triangle whose vertical angle is 2\tan ^{ -1 }{ \left( a/b \right) } .
In a right angled triangle, prove that the line segment joining the mid-point of the hypotenuse to the opposite vertex is half the hypotenuse.
Prove that in a \triangle PQR if QR^{2} = PQ^{2} + PR^{2} then \angle P is a right angle.
Prove that the inequality R \geqslant 2r (R and r are the radii of the circumscribed and the inscribed circle) holds true in any triangle, and the equality R = 2r holds only. for a regular triangle.
I and m are two parallel line intersected by another pair of parallel line p and q Fig Show taht \Delta ABC \cong \Delta CDA.

In the adjoining figure, D and E are points on the side BC of \triangle ABC such that BD=EC and AD=AE. Show that \triangle ABD\cong \triangle ACE.
A round balloon of radius r subtends an angle \alpha at the eye of the observer, while the angle of elevation of its centre is \beta. Prove that the height of the centre of the balloon is r \sin\beta\ \text{csc}\dfrac{\alpha}{2}.
Show by diagram any two real-life examples for congruent shapes.
If O is a point within a quadrilateral ABCD, prove that ; OA + OB + OC + OD > AC + BD.
It is to be established by RHS congruence rule that \triangle ABC\cong \triangle RPQ. What additional information is needed, if it is given that \angle B=\angle P=90^o and AB=RP?
In the following figure \angle B < \angle A and \angle C < \angle D, show that AD < BC.
Let x,\ x+1,\ x+2, be the lengths of the three sides of a triangle.
(i) Write down the three inequations in x, each of which represents the given statement.
(ii) List the set of possible values of x which satisfy all the three inequations obtained in your answer to part (i) above, given that x is an integer.
Given that ABCD is a quadrilateral. Is AB+BC+CD+DA<2(AC+BD)?
If AB>AC and length of median from C is 4 units, then the length of median from B can be-
In the given figure, \triangle CDE is an equilateral triangle on a side CD of a square ABCD. Show that \triangle ADE \cong \triangle BCE.
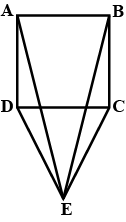
AB is a line segment and P is its mid-point. D and E are points on the same side of AB such that \angle BAD=\angle ABE and \angle EPA=\angle DPB. Show that
i) \triangle DAP\cong \triangle EBP
ii) AD=BE
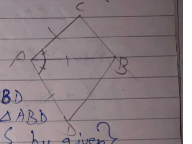
Given :- AC=AD
\angle CAB= \angle BAD
To prove :- \triangle ABC \cong \triangle ABD
Proof :- 9n \triangle ABC & \triangle ABD
AC=AD
\angle CAB= \angle BAD
AB=BA
\triangle ABC \cong \triangle ABD
BC=BD
Prove that sum of any two sides of a triangle is greater than the third side.
In the adjoining figure. AB = AC and BD = DC. Prove that \Delta ADB\, \cong \,\Delta ADC and hence show that
(i) \angle ADB\, = \,\angle ADC\,\,
(ii) \angle BAD\, = \,\angle CAD
The length of two sides of a triangle are 5\ cm and 7\ cm. Between what two measures should the length of the third side fall?
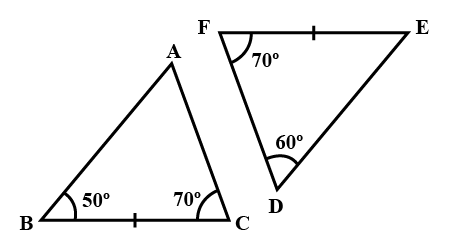
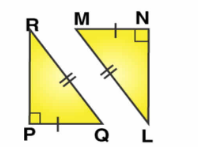
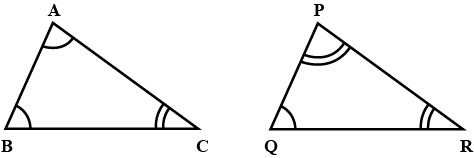
In the example given, a pair of triangles is shown. Equal parts of triangles in pairs are marked with the same signs. Observe the figures and state the test by which the triangles in pair are congruent.
In triangles ABC and PQR, \angle A = \angle Q and \angle B = \angle R. Which side of \Delta PQR should be equal to side BC of \Delta ABC so that the two triangle are congruent? Give reason for your answer.
"If two angles and a side of one triangle are equal to two angles and a side of another triangle, then the two triangles must be congruent". Is the statement true? Why?
Line l is the bisector of an angle \angle{A} and B is any point on l.\,\,BP and BQ are perpendiculars from B to the arms of \angle{A}Show that(i)\triangle{APB}\cong\triangle{AQB}(ii)BP=BQ or B is equidistant from the arms of \angle{A}
S is any point on side QR of a \Delta PQR. Show that : PQ + QR + RP > 2 PS
In \DeltaABC, side AB is produced to D such that BD=BC. If \angle CAB=70^o and \angle CBA=60^o, prove that AD>AC.
AB is a line segment and P is its mid-point. D and E are points on the same side of AB such that \angle BAD =\angle ABE and \angle EPA =\angle DPB. Show that \Delta DAP \cong \Delta EBP.
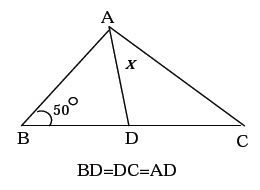
In \DeltaABC, side AB is produced to D such that BD=BC. If \angle A=70^o and \angle B=60^o, prove that AD>CD.
AB is a line segment and P is its mid-point. D and E are points on the same side of AB such that \angle BAD =\angle ABE and \angle EPA =\angle DPB. Show that AD=BE.
In the figure AB=AC,BD=DC,\angle C=40^{o}\angle BDC=160^{o}
Write the measure of all angles of triangle ABD.
D is any point on side AC of a \Delta ABC with AB = AC. Show that CD < BD.
ABCD is a quadrilateral in which AD=BC and \angle DAB=\angle CBA. Prove that BD =AC.
Let ABC be an isosceles triangle with AB=AC and let D, E, F be the mid-point of BC, CA and AB respectively. Show that AD\bot EF and AD is bisected by EF.
AD is an altitude of an isosceles triangle ABC in which AB=AC. show that
(i) AD bisects BC
(ii) AD bisects \angle A
AM is a median of a triangle ABC.
Is AB + BC + CA > 2 AM?
(Consider the sides of triangles \Delta ABM and \Delta ABM.)
In the given figure, \angle B < \angle A and \angle C < \angle D, show that AD < BC.
In Fig., D and E are points on side BC of a \triangle ABC such that BD = CE and AD = AE. Show that \triangle ABD \cong \triangle ACE.
Prove that any two sides of a \Delta are together greater than twice the median drawn to the third side.
In quadrilateral ACBD
AQC=AD and AB bisects \angle{A} (see figure). Show that \triangle{ABC}\cong \triangle{ABD}.
What can you say about BC and BD?
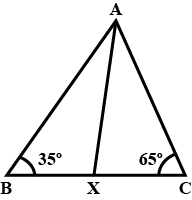
In figure, ABC is a triangle with \angle B = 35^{\circ}, \angle C = 65^{\circ} and bisector of \angle BAC meets BC in X. Arrange AX, BX and CX in descending order.
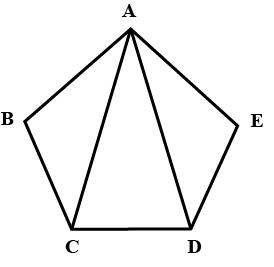
ABCDE is a regular pentagon having all the sides and angles equal. Show that \triangle ABC \cong \triangle AED.
ABC is an isosceles triangle with AB = AC and BD and CE are its two medians. Show that BD = CE.
In the square ABCD show that the two triangles ABC and ADC are congruent to each other
If two angles of a triangle are equal, then prove that sides opposite to them are also equal.
In a triangle ABC median AD is produced to X such that AD+DX. Prove that ABXC is a parallelogram.
State whether the following triangles are congruent or not? Give reasons for your answer.
In the adjoining figure, BA \bot AC, \, DE \bot DF such that BA = DE and BF = EC. Show that \Delta ABC \cong \Delta DEF
ABCD is a quadrilateral
Prove that (AB+BC+CD+DA)>(AC+BD)
In \Delta ABC, AB<AC, PB and PC are the bisectors of \angle B and \angle C. Prove that PB< PC.
l, m and n are three parallel lines intersected by transversals p and q such that l, m and n cut off equal intercepts AB and BC on p. Show that l, m and n cut off equal intercepts DE and EF on q also.

If \DeltaMNO\cong\DeltaQPR, write the six corresponding parts.
In the quadrilateral ABCD,AD=CD and \angle A ={90}^{o}=\angle C, prove that AB=BC.
D is a point on the side BC of a \triangle ABC such that \angle ADC=\angle BAC, then prove that CA^2 = CB \times CD
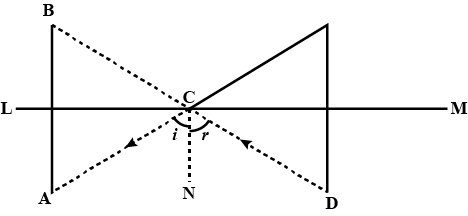
The image of an object placed at a point A before a plane mirror LM is seen at the point B by an observer at D as shown in Fig. Prove that the image is as far behind the mirror as the object is in front of the mirror.
If P is a point in the interior of \triangle ABC then fill in the blanks with > or < or =.
(i) PA+PB.........AB
(ii) PB+PC........BC
(iii) AC.........PA+PC
Prove that the diagonals of a parallelogram bisect each other
In the figure given below , OL is perpendicular to AB and OM is perpendicular to BC such that OL = OM. Is \Delta OLB \cong \Delta OMB?

The lengths of the two sides of a triangle are 6cm and 10cm. Between what two wholes numbers should lie the measure of the third sides?
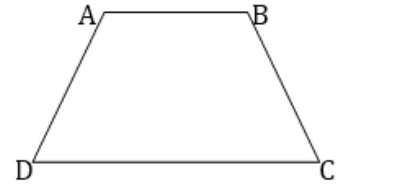
ABCD is a trapzium in which AB||CD and AD=BC. Show that
\angle A=\angle B
ABCD is a trapzium in which AB||CD and AD=BC. Show that \triangle ABC \cong \triangle BAD
ABCD is a trapezium in which AB||CD and AD=BC, then show that \angle C=\angle D.
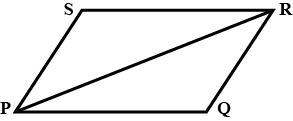
PR is diagonal of a parallelogram PQRS. Show that \Delta PQR \cong \Delta RSP.
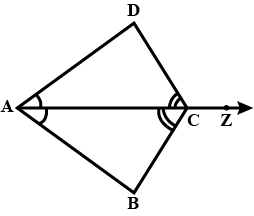
In the figure, ray AZ bisects \angle {DAB} as well as \angle{DCB}
(i) State the three pairs of equal parts in triangles BAC and DAC.
(ii) Is \triangle {BAC}\cong \triangle {DAC}? Give reasons
(iii) Is AB=AD? Justify your answer
(iv) Is CD=CB? Give reasons.
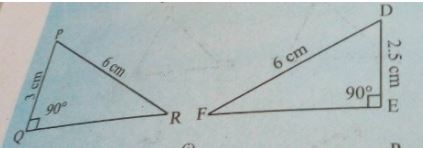
Check whether the following pairs of triangles are congruent. If they are congruent, state the congruence criterion.
By applying SAS congruence rule, you want to establish that \Delta P Q R \cong \Delta F E D. It is given that P Q = F E \text { and } R P = D F. What additional information is needed to establish the congruence ?
ABC is a triangle right angled at C. A line through the mid point M of hypotenuse AB and parallel to BC intersect AC at D .Show that :
CM=MA=\dfrac{1}{2}AB
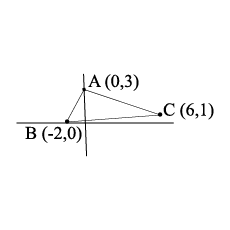
Find number of integral values of \lambda if \left( {\lambda ,\lambda + 1} \right) is an interior point of \Delta ABC, where A=(0,3), B=(-2,0) and C=(6,1).
In the given figure, triangles ABC and DCB are right-angle at A and D respectively and AC=DB. Prove that \Delta ABC \cong \Delta DCB
Express the congruence in the given triangles. Write in symbolic form.
In \Delta XYZ,\ XY=4.2\ cm,\ XZ=6.5\ cm\ \angle Y=90^{o}
In \Delta DEF,\ FE=6.5\ cm,\ FD=4.2\ cm\ \angle D=90^{o}
In the figure AB || DC and AC and PQ intersect each other at the point O. Prove that OA.CQ = OC.AP.
By applying SAS congruence rule, you want to establish that \triangle PQR \equiv \triangle FED. It is given that PQ=FE and RP=DE. What additional information is needed to establish the congruence?
In parallelogram ABCD, two points P and Q are taken on diagonal BD such that DP = BQ (refer to the given figure). Show that :
(i) \triangle APD \cong \triangle CQB
(ii) AP=CQ
(iii) \triangle AQB \cong \triangle CPD
(iv) AQ=CP
(v) APCQ is a parallelogram
Diagonal AC of a parallelogram ABCD bisects \angle A (see Fig. 8.19), show that
(i) it bisects \angle C also (ii) ABCD is a rhombus

In \Delta A B C BE and CF are the two equal altitudes. Prove \Delta A B C is isoceles by R.H.S
In the given figure, AB=DC and BD=CA. Prove that \Delta ABC\cong DCB.
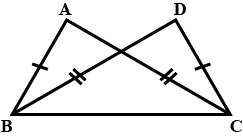
In figure, AB=AC and D is any point on BC produced. Show that AD>AB.
State the correspondence between the sides and angels of the following congruent triangles.
a) \Delta PQR \cong \Delta XYZ
b) \Delta ABC \cong \Delta TUV
In a \Delta A B C , A B = A C . If the bisectors of \angle B and \angle C meet A C and A B at points D and E respectively, show that :
(i) \Delta \mathrm { DBC } \cong \Delta \mathrm { ECB }
(ii) \mathrm { BD } = \mathrm { CE }
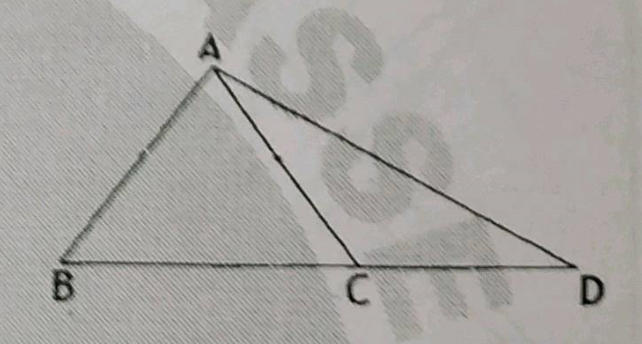
In figure, it is given that AB=CD and AD =BC. Prove that \triangle ADC \cong \triangle CBA.
\triangle EFG \cong \triangle LMN
Write the corresponding vertices, angles and sides of the two triangles.
In the given figure, the triangles are congruent, Find the values of x and y.

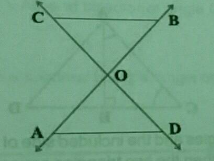
In figure, two lines AB and CD intersect each other at the point O such that BC\parallel DA. Show that O is the mid-point of both the line-segments AB and CD.
Name pair of congruent triangle in the figure.

PSDA is a parallelogram. Points Q and R are takes on PS such that PQ=QR and PA||QB||RC. Prove that area (\Delta PQE)=(\Delta CFD)
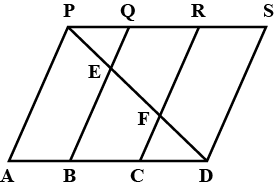
in the adjacent figure , parallelogram ABCD , Show that ABC \cong CDA
Prove that in an isosceles triangle, the angles opposite the equal sides are equal.
\triangle{ABC} and \triangle{DBC} are two isosceles triangles on the same base BC and vertices A and D are on the same side of BC as in figure.If AD is extended to intersect BC at P,Show that \triangle{ABP}\cong\triangle{ACP}

If in two right triangles the hypotenuse and one side of one triangle are equal to the hypotenuse and one side of the other triangle, then prove that two triangles are congruent.
In \triangle{PQR},N is a point on PR such that QN\bot PR. If PN\times NR={QN}^{2}, prove that \angle{PQR}={90}^{\circ}
\triangle{ABC} and \triangle{DBC} are two isosceles triangles on the same base BC and vertices A and D are on the same side of BC as in figure.If AD is extended to intersect BC at P,Show that \triangle{ABD}\cong\triangle{ACD}
ABCD is a square and BD is the diagonal. Find the measure of the area of \triangle ABD.
If area of \triangle{ABC}= area of \triangle{DEF} then prove that \triangle{ABC}\cong\triangle{DEF}
In the given figure AB=CD and AD=BC. Prove that \angle BAC= \angle ACD.
\triangle ABC is a right angled in which LC=90^{o} and CD\bot AB. If BC=a, CA=b, AB=c and CD=p then prove that
cp=ab
Is it possible to have a triangle with the following sides:
3\ cm,\ 4\ cm and 5\ cm
\triangle ABC is a right angled in which LC=90^{o} and CD\bot AB. If BC=a, CA=b, AB=c and CD=p then prove that
\dfrac{1}{p^{2}}=\dfrac{1}{a^{2}}+\dfrac{1}{b^{2}}
By applying ASA congruence rule, it is to be established that \Delta ABC \cong \Delta QRP and it is given that BC=RP. What additional information is needed to establish the congruence?
AB and CD intersect each other at O and O is the midpoint of both AB and CD. Prove that AD=BC.
In the following figure, state the conditions you would use to show that \Delta ABC and \Delta EDC are congruent.
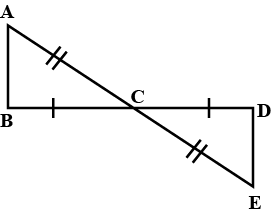
In the given figure, it is given that AB=CD and AD=BC. Prove that \Delta ADC \cong \Delta CBA
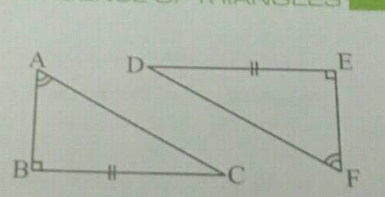
In given figure explain, why \triangle ABC\cong \triangle FED
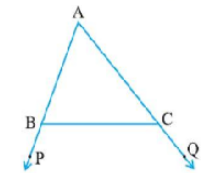
In the adjoining figure, sides AB and AC of \triangle{ABC} are extended to points P and Q respectively.Also, \angle{PBC} < \angle{QCB}. Show that AC>AB.
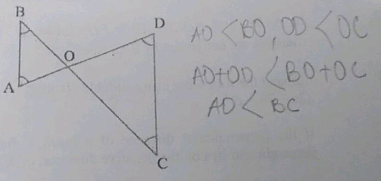
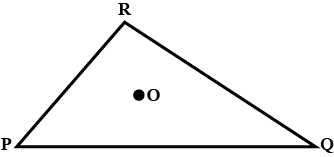
Take any point O in the interior of a triangle PQR. Is OQ+OR>QR?
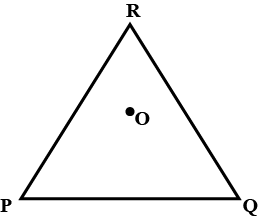
Is it possible to have a triangle with the following sides?
6cm, 3cm, 2cm.
Take any point O in the interior of a \triangle PQR. Is OP+OQ>PQ?
Take any point O in the interior of a triangle PQR. Is OR+OP>RP?
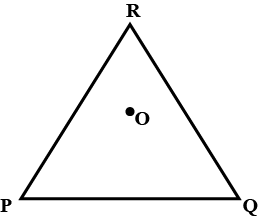
Is it possible to have a triangle with the following sides?
3cm, 6cm, 7cm.
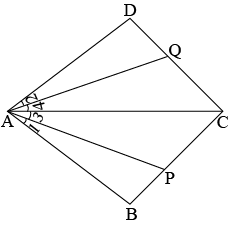
In the given figure, AB=AD,\,\angle 1=\angle 2=\angle 3=\angle 4. Prove that AP=AQ.
Is it possible to have a triangle with the following sides?
1cm, 3cm, 5cm.
In the given figure, AB\parallel CD and O is the midpoint of AD.
Show that (i) \triangle AOB\cong \triangle DOC
(ii) O is the midpoint of BC.
The lengths of the two sides of a triangle are 12 cm and 15 cm. Between what two measures should the length of the third side fall?
In Fig. AD \perp CD and CB \perp CD. If AQ = BP and DP = CQ , prove that \angle DAQ = \angle CBP.
In a rectangle ABCD, prove that \triangle ACB \cong \triangle CAD.
Explain the following term.
Interior of a triangle.
In Fig., there is a triangle. The measures of some angles have been indicated. State whether triangle is acute, right or obtuse.

In Fig., there is a triangle. The measures of some angles have been indicated. State whether triangle is acute, right or obtuse.
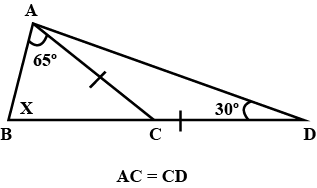
In \Delta ABC, side AB is produced to D so that BD = BC. If \angle B = 60^{\circ} and \angle A = 70^{\circ}, prove that: AD > AC
The angles of a triangle are arranged in ascending order of magnitude. If the difference between two consecutive angles is 10^{\circ}, find the three angles.
In the given figure, it is given that RT = TS, \angle 1 = 2\angle 2 and \angle 4 = 2\angle 3. Prove that \Delta RBT \cong \Delta SAT.
Explain the following term.
Obtuse triangle.
Fill in the blank:
Two squares are congruent if ________.
Fill in the blank.
Two line segments are congruent if ________.
In the following triangle, the lengths of the sides are indicated along sides. By applying SSS condition, determine which are congruent. State the result in symbolic form.
Explain the concept of congruence of figures with the help of certain examples.
In the given figure, AB=DC and BC=AD. Is \DeltaABC\cong\DeltaCDA?

Identify the pairs of triangles are congruent by ASA condition?
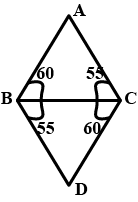
Triangles ABC and PQR are both isosceles triangles with AB=AC and PQ=PR respectively. If AB=PQ and BC=QR, then are the two triangles congruent? Which condition do you use? If \angle B=50^o, what is the measure of \angle R?
In the following pairs of triangles, the lengths of the sides are indicated along sides. By applying SSS condition, determine which are congruent. State the result in symbolic form.
In the given figure, line segments AB and CD bisect each other at O. Prove that, \Delta AOC\cong \Delta BOD.
In the given figure, AB=AD and \angle BAC=\angle DAC. Complete the following, so as to make it true: Line segment AC bisects _______ and _________.
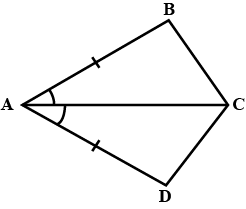
In figure, AD bisects \angleA and AD\perpBC. Is it true to say that BD=DC?
Draw any triangle ABC. Use ASA condition to construct another triangle congruent to it.
Identify the pairs of triangles are congruent by ASA condition?
In figure, AO=OB and \angleA=\angleB. Is \DeltaAOC\cong\DeltaBOD?

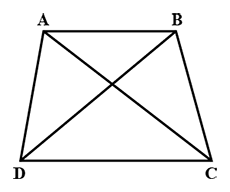
State the three pairs of matching parts you have used to answer.(Is \DeltaABC\cong\DeltaACB)
In figure, AD bisects \angleA and AD\perpBC. State the three pairs of matching parts you have used after proving the triangles congruent.
Can the pairs of triangles be congruent by ASA condition?
Is \DeltaABC\cong\DeltaACB?
Identify the pairs of triangles are congruent by ASA condition?
In figure, AX bisects \angleBAC as well as \angleBDC. State the three facts needed to ensure that \DeltaABD\cong\DeltaACD.
In figure, BD and CE are altitudes of \DeltaABC and BD=CE. Is \DeltaBCD\cong\DeltaCBE?
In the following pairs of right triangles, the measures of some parts are indicated along side. State by the application of RHS congruence condition which are congruent. State the result in symbolic form.
In figure, AO=OB and \angle A=\angle B. State the matching pair you have used for \Delta AOC \cong\Delta BOD, which is not given in the question.
In figure, AO=OB and \angle A=\angle B. Is it true to say that \angle ACO=\angle BDO?
In the following pairs of right triangles, the measures of some parts are indicated along side. State by the application of RHS congruence condition which are congruent. State the result in symbolic form.

In figure, BD and CE are altitudes of \DeltaABC and BD=CE. State the three pairs of matching parts you have used to answer.(Is \DeltaBCD\cong\DeltaCBE).
In parallelogram ABCD, points M and N have been taken on opposite sides AB and CD respectively such that AM=CN. Show that AC and MN bisect each other.

In the figure, ABCD is a quadrilateral and AC is one its AB+BC+CD+DA> 2AC diagonals. Prove that
In the given figure, ABCD is a quadrilateral in which AB=AD and BC=DC. Prove that
AC bisects \angle A and \angle C.
In the adjoining figure, OPQR is a square. A circle drawn with centre O cuts the square in X and Y.
Prove that QX=QY

If O is a point within a quadrilateral ABCD, show that OA+OB+OC+OD> AC+BD
In the given figure, ABCD is a square and \angle PQR={90}^{o}. If PB=QC=DR, prove that AB+BC+CD+DA> AC+BD
In the given figure, ABCD is a quadrilateral in which AB=AD and BC=DC. Prove that
BE=DE
In the given figure, ABCD is a square and \angle PQR={90}^{o}. If PB=QC=DR, prove that AB+BC+CD> DA
\Delta ABC is an isosceles triangle with AB = AC = 13 cm. The length of altitude from A on BC is 5 cm. Find BC.
In the given figure, ABCD is a quadrilateral in which AB=AD and BC=DC. Prove that
\angle ABC=\angle ADC
Given the base a of a triangle, the opposite angle A and the product k^2 of the other sides, solve the triangle and show that there is no such triangle if a < 2k \sin \dfrac{A}{2}.
The line segments joining the midpoints M and N are parallel sides AB and DC respectively of a trapezium ABCD is perpendicular to both the sides AB and DC. Prove that AD = BC.

In the adjoining figure, X and Y are respectively two points on equal sides AB and AC of \triangle ABC such that AX = AY. Prove that CX = BY.
The angles of a triangle are as 1:2:7; prove that the ratio of the greatest side to the least side is \sqrt{5}+1:\sqrt{5}-1.
In \triangle ABC, AB = AC and the bisectors \angle B and \angle C meet at a point O. Prove that BO = CO and the ray AO is the bisector of \angle A.

In \triangle ABC, D is the midpoint of BC. If DL \perp AB and DM \perp AC such that DL = DM, prove that AB = AC.
A square is inscribed in an isosceles right triangle so that the square and the triangle have one angle common. Show that the vertex of the square opposite the vertex of the common angle bisects the hypotenuse.
If the angles of a triangle b in A.P. and the lengths of the greatest and least sides be 24 and 16 feet respectively, find the length of the third side and the angles, given
log 2=.30103, log 3=.4771213,
and L\tan 19^o6'=9.5394287, diff. for 1'=4084.
In the given figure, ABCD is a square and P is a point inside it such that PB = PD. Prove that CPA is a straight line.
In the adjoining figure, explain how one can find the breadth of the river without crossing it.
Show that for any triangle with sides a, b and c, 3(ab + bc + ca) < (a + b +c)^2 < 4(bc + ca + ab). when are the first two expressions equal?
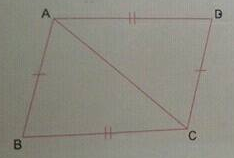
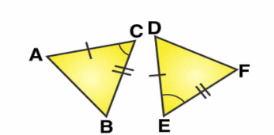
Given is a pair of congruent triangles. State the property of congruence and the name of the congruent triangles.
Given is a pair of congruent triangles. State the property of congruence and the name of the congruent triangles.
Given is a pair of congruent triangles. State the property of congruence and name of the congruent triangles.
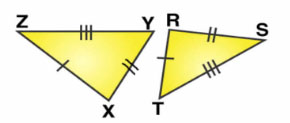
Given is a pair of congruent triangles. State the property of congruence and the name of the congruent triangles.
State the correspondence between the vertices, sides and angles of the following pair of congruent triangles.
\triangle CAB\cong \triangle QRP
State the correspondence between the vertices, sides and angles of the following pair of congruent triangles.
\triangle XZY\cong \triangle QPR
Given is a pair of congruent triangles. State the property of congruence and the name of the congruent triangles.
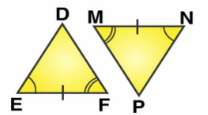
State the correspondence between the vertices, sides and angles of the following pair of congruent triangles.
\triangle MPN\cong \triangle SQR
State the correspondence between the vertices, sides and angles of the following pair of congruent triangles.
\triangle ABC\cong \triangle EFD
In the given figure, PA\bot AB, QB\bot AB and PA=QB. Prove that \triangle OAP=\triangle OBQ. Is OA=OB?
In \triangle ABC, \angle A is acute. BD and CE are perpendicular on AC and AB respectively. Prove that AB\times AE = AC \times AD.
In figure, AD=BC and AD\parallel BC. Is AB=DC? Give reasons in support of your answer.
In the figure, AB=AD and CB=CD. Prove that \triangle ABC\cong \triangle ADC.

In the given figure, triangles ABC and DCB are right-angled at A and D respectively and AC=DB. Prove that \triangle ABC\cong \triangle DCB
In the adjoining figure, AB=AC and BD=DC. Prove that \triangle ADB\cong \triangle ADC and hence show that (i) \angle ADB=\angle ADC={90}^{o}, (ii) \angle BAD=\angle CAD
In figure, PL\bot OA and PM\bot OB such that PL=PM. Is \triangle PLO\cong \triangle PMO?
Give reasons in support of your answer.
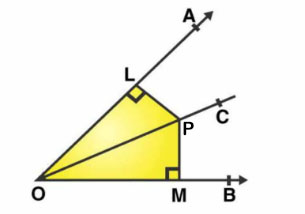
In the given pairs of triangles, applying only the ASA congruence criterion, determine which triangles are congruent. Also, write the congruent triangles in symbolic form.
In the given pair of triangles, applying only the ASA congruence criterion, determine which triangles are congruent. Also, write the congruent triangles in symbolic form.
In given pairs of triangles in the figure, applying only the ASA congruence criterion, determine which triangles are congruent. Also, write the congruent triangles in symbolic form.
In the given pairs of triangles, applying only the ASA congruence criterion, determine which triangles are congruent. Also, write the congruent triangles in symbolic form.
In Fig., \Delta PQR \cong \Delta ______
In the given pairs of triangles, applying only the ASA congruence criterion, determine which triangles are congruent. Also, write the congruent triangles in symbolic form.
If \Delta PQR and \Delta XYZ are congruent under the correspondence QPR \leftrightarrow XYZ, then,
(i) \angle R = ______ (ii) QR = ______
(iii) \angle P = ______ (iv) QP = ______
(v) \angle Q = ______ (vi) RP = ______
In the given pair of triangles, using only the RHS congruence criterion, determine which pairs of triangles are congruent. In case of congruence. write the result in symbolic form
Enter 1 if it is true else enter 0.In Fig., \triangle PQR \cong \triangle XYZ
In Fig., \Delta ______ \cong PQR

In Fig., AB = AD and \angle BAC = \angle DAC.
Then,
(i) \Delta ______ \cong \Delta ABC
(ii) BC = ______
(iii) \angle BCA = ______
(iv) Line segment AC bisects ______ and ______.
In the given pair of triangles, using only the RHS congruence criterion, determine which pairs of triangles are congruent. In case of congruence. write the result in symbolic form:
In the given pair of triangles, applying only the ASA congruence criterion, determine which triangles are congruent. Also, write the congruent triangles in symbolic form.
Without drawing the triangles write all size pairs of equal measures in each of the following pairs of congruent triangles.
\triangle XYZ \cong \triangle MLN
In the following, pairs of triangles of Fig. the lengths of the sides are indicated along the sides. By applying SSS congruence criterion, determine which triangles are congruent. If congruent, write the results in symbolic form.
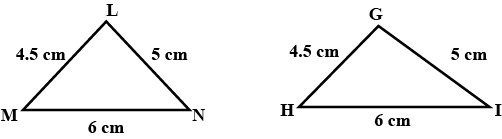
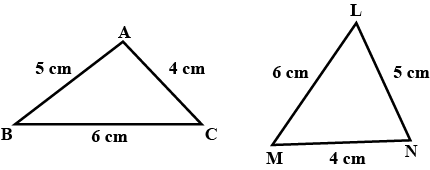
In the following, pairs of triangles of FIg. the lengths of the sides are indicated along the sides. By applying SSS congruence criterion, determine which triangles are congruent. If congruent, write the results in symbolic form.
In the given pairs of triangles, using only the RHS congruence criterion, determine which pairs of triangles are congruent. In case of congruence. write the result in symbolic form:
In Fig. which phase of triangles are congruent by SAS congruence criterion (condition)? If congruent, write the congruence of the two triangles in symbolic form.
In the given pairs of triangles, using only the RHS congruence criterion, determine which pairs of triangles are congruent. In case of congruence. write the result in symbolic form:
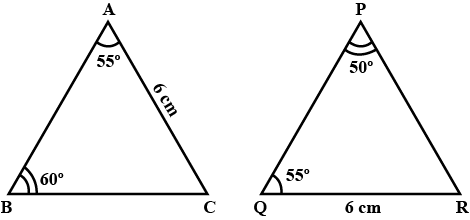
State which of the following pairs of triangles are congruent. If yes, write them in symbolic form (you may draw a rough figure).
\triangle PQR : PQ = 3.5cm, QR = 4.0cm, \angle Q = 60^{o}
\triangle STU : ST = 3.5cm, TU = 4cm, \angle T = 60^{o}
Without drawing the triangles write all size pairs of equal measures in each of the following pairs of congruent triangles.
\triangle YZX \cong \triangle PQR
In the given pair of triangles, using only RHS congruence criterion, determine which pairs of triangles are congruent. In case of congruence. write the result in symbolic form:
In the given pairs of triangles, using only the RHS congruence criterion, determine which pairs of triangles are congruent. In case of congruence. write the result in symbolic form:
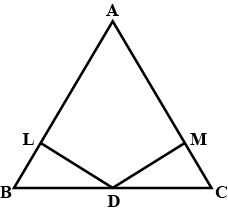
Observe, Figure and state the three pairs of equal parts in triangles ABC and DBC.
(i) Is \triangle ABC \cong \triangle DCB? Why?
(ii)Is AB=DC?Why?
(iii)Is AC=DB?Why?
In Figure, state the three pairs of equal parts in \triangle ABC and \triangle EOD. Is \triangle ABC \cong \triangle EOD ?Why?
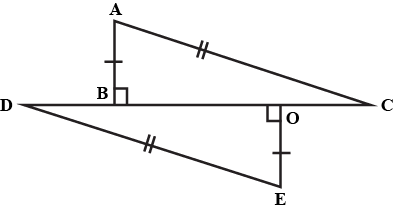
In Figure QS \bot PR, RT \bot PQ and QS=RT.
(i) Is \triangle QSR \cong \triangle RTQ? Give Reasons
(ii) Is ∠ PQR = ∠ PRQ? Give reasons.
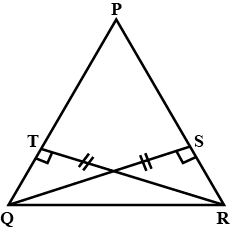
In above figure, \angle 1=\angle 2 and \angle 3 =\angle 4.
(i) Is \triangle ADC \cong \triangle ABC? Why?
(ii)Show that AD=AB and CD=CB.
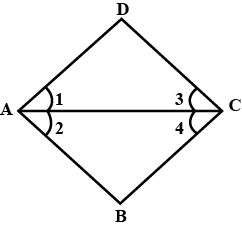
In the figure shown above, DE=IH, EG=FI and \angle E=\angle I. Is \triangle DEF \cong \triangle HIG? If yes, by which congruence criterion?
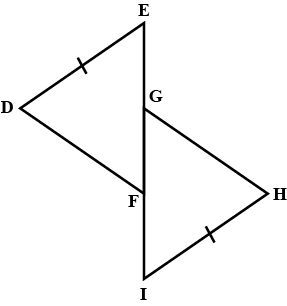
AD is a median of the triangle ABC. Is it true that AB+BC+CA>2AD? Give reason for your answer.
State which of the following pairs of triangles are congruent. If yes, write them in symbolic form (you may draw a rough figure).
\triangle ABC : AB = 4.8cm, \angle A = 90^{o}, AC = 6.8cm
\triangle XYZ : YZ = 6.8cm, \angle X = 90^{o}, ZX = 4.8cm
In Fig. PQ = PS and \angle 1 = \angle 2.
(i) Is \triangle PQR \cong \triangle PSR ? Give Reasons.
(ii) Is QR = SR ? Give reasons.

If two sides and an angle of one triangle are equal to two sides and an angle of another triangle, then the two triangles must be congruent. Is the statement true? Why?
In \Delta PQR , PD QR such that D lies on QR . If PQ = a , PR = b , QD = c and DR = d , prove that (a + b) (a - b) = (c + d)(c - d) .
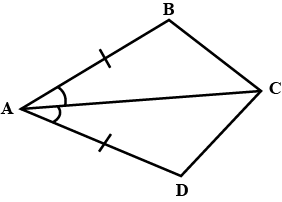
In the given figure, AB=AC, P and Q are points on BA and CA respectively such that AP=AQ. Prove that
\triangle APC\cong \triangle AQB
In the above figure, BA \perp AC, \ ED \perp FD such that BA=DE and BF=EC. Show that \triangle \ ABC \cong \triangle \ DEF.
The longest side of a triangle is 3 times the shortest side and the third side is 2\ cm shorter than the longest side. If the perimeter of the triangle is at least 61\ cm, find the minimum length of the shortest side.
We know that in a triangle, the sum of lengths of any two sides is greater than the length of the third side. Is the sum of any angles of a triangle also greater than the third angle? If no, draw a rough sketch to show such a case.
In the given figure, ABCD is a quadrilateral in which AD=BC and \angle DAB=CBA. Prove that
\triangle ABD \cong \triangle BAC
In the above figure, AD is the bisector of \angle BAC. Prove that AB>BD.
In the adjoining figure, AB=CD, CE=BF and \angle ACE =\angle DBF. Prove that
\triangle ACE\cong \triangle DBF
In the adjoining figure, ABCD is a square P,Qand R are points on the sides AB, BC and CD respectively such that AP=BQ=CR and \angle PQR =90^o. Prove that
PQ=QR

State, whether the pairs of triangles given in the following figures are congruent or not:

In the figure given ABCD is a parallelogram. E and F are mid-point of the sides AB and CO respectively. The straight lines AF and BF meet the straight lines ED and EC in points G and H respectively. Prove that \triangle HEB\cong \triangle HCF

In the adjoining figure, ABCD is a square P,Qand R are points on the sides AB, BC and CD respectively such that AP=BQ=CR and \angle PQR =90^o. Prove that
\angle PRQ = 45^o
In the given figure, PQ || BA and RS\ CA. If BP=RC, prove that :
RS=CQ

In the adjoining figure, ABCD is a square, P,Qand R are points on the sides AB, BC and CD respectively such that AP=BQ=CR and \angle PQR =90^o. Prove that \triangle PBQ \cong \triangle QCR.
In triangle ABC and DEF, \angle A= \angle D, \angle B=\angle E and AB =EF. Will the two triangle be congruent? Give reasons for your answer.
State, whether the pairs of triangles given in the following figures are congruent or not:

State, whether the pairs of triangles given in the following figures are congruent or not:
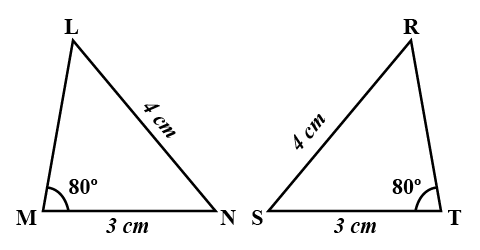
\Delta ABC in which AB = 2\,cm , BC = 3.5 \,cm and \angle C = 80^{\circ} and \Delta DEF in which DE = 2\,cm , DF = 3.5\,cm and \angle D = 80^{\circ}
\mathrm{ABCD} is a rectangle and \mathrm{P} is mid-point of \mathrm{AB} . DP is produced to meet \mathrm{CB} at \mathrm{Q} . Prove that area of rectangle \mathrm{ABCD}= area of \Delta \mathrm{DQC} .
Prove that
\angle ADB = \angle ADC

Prove that
\Delta ABD \cong \Delta ACD
From the given figure, prove that:
AB = ED
In the given figure , prove that
\Delta ACB \cong \Delta ECD
Prove that
\angle A = \angle C

Prove that
\Delta ABD \cong \Delta BDC
In the given figure , prove that
\Delta ABD \cong \Delta ACD
Prove that
\angle ADB = 90^{\circ}
Prove that :
(i) \Delta ABC \cong \Delta ADC
(ii) \angle B = \angle D
(iii) AC bisects angle DCB .
Prove that
\angle B = \angle C
In the given figure , prove that :
\Delta ADB \cong \Delta BCA

In the given figure , prove that :
AD = BC
In the given figure, prove that :
\Delta AOD \cong \Delta BOC
In the given figure , prove that :
\Delta XYZ \cong \Delta XPZ
In the given figure , prove that :
YZ = PZ

The given figures shows a triangle ABC in which AD is perpendicular to side BC and BD = CD. Prove that :
(i) \Delta ABD \cong \Delta ACD
(ii) AB = AC
(iii) \angle B = \angle C
In the given figure , \angle 1 = \angle 2 and AB = AC .
Prove that:
(i) \angle B = \angle C
(ii) BD = DC
(iii) AD is perpendicular to BC
In the given figure , prove that :
\angle YXZ = \angle PXZ
In the given figure , prove that :
\angle ADB = \angle ACB
In the given figure , prove that :
\Delta ABC \cong \Delta DCB
In the given figure AB , = DB and AC = DC
If \angle ABD = 58 ^{o}
\angle DCB = (2x - 4) ^{o}
\angle ACB = y + 15 and
\angle DCB = 63 ^{o} : find the values of x and y

In the following example, a pair of triangles is shown. Equal parts of triangle in each pair are marked with the same sign. Observe the figure and state the test by which triangles in each pair are congruent.
By.............test
\Delta PQR \cong \Delta STU
In the following example, a pair of triangles is shown. Equal parts of triangle in each pair are marked with the same sign. Observe the figure and state the test by which the triangle in each pair are congruent.
By............test
\Delta LMN \cong \Delta PTR
In the given figure, \angle P =\angle R and PQ =RQ. Prove that, \Delta PQT \cong \Delta RQS
Observe the information shown in pair of triangle shown above. State the test by which the two triangles are congruent. Write the remaining congruent parts of the triangle.

In the given alongside figure,AD=AB=AC,BD is parallel to CA and \angle ACB=65^o.
Find the \angle DAC.
As shown in the following figure, in \Delta LMN and \Delta PNM, LM = PN, LN = PM. Write the test which assures the congruence of the two triangles. Write their remaining congruent part.
Observe the information shown in the pair of triangles given below. State the test by which the two triangles are congruent. Write the remaining congruent parts of the triangles.
In the following example, a pair of triangles is shown. Equal parts of the triangle in each pair are marked with the same sign. Observe the figure and state the test by which the triangle in each pair are congruent.
By............test
\Delta XYZ \cong \Delta LMN

In the figure, point D and E are on side B C of \Delta A B C, such that B D=C E and A D=A E
show that \triangle ABD \cong \triangle ACE.
Fill in the blank using the correct word given in brackets:
All squares are _____. (similar, congruent )
Line 1 is the bisector of an angle \angle A and B is any point on l. BP and BQ are perpendiculars from B to the arms of \angle A. Show that :
(i) \triangle APB \cong \triangle AQB
(ii) BP = BQ or B is equidistant from the arms of \angle A.

In the given figure, bisector of \angle \mathrm{BAC} intersects side \mathrm{BC} at point \mathrm{D} . Prove that \mathrm{AB}>\mathrm{BD}
In the given figure, point S is any point on side \mathrm{QR} of \Delta \mathrm{PQR}
Prove that : \mathrm{PQ}+\mathrm{QR}+\mathrm{RP}>2 \mathrm{PS}
In the figure, points D and E are on side BC of \Delta ABC, such that BD = CE and AD = AE.
Show that \Delta \mathrm{ABD} \cong \Delta \mathrm{ACE}
In \Delta \mathrm{PQR}, If \mathrm{PQ}>\mathrm{PR} and bisectors of \angle \mathrm{Q} and \angle \mathrm{R} intersect at \mathrm{S} . Show that \mathrm{SQ}>\mathrm{SR} .
Fill in the blank using the correct word given in brackets:
All circles are ______. (Congruent, similar)
l and m are two parallel lines intersected by another pair of parallel lines p and q. Show that \triangle ABC \cong \triangle CDA.
ABC is a triangle in which altitudes BE and CF to sides AC and AB are equal. Show that
(i) \triangle ABE = \triangle ACF
(ii) AB = AC i.e., \triangle ABC is an isosceles triangle.
In fig \angle B < \angle A and \angle C < \angle D. Show that AD < BC.
In sides AB and AC of \triangle ABC are extended to points P and Q respectively. Also, \angle PBC < \angle QCB. Show that AC > AB.

Two sides AB and BC and median AM of one triangle ABC are respectively equal to sides PQ and QR and median PN of \triangle PQR. Show that :
(i) \triangle ABM \cong \triangle PQN
(ii) \triangle ABC \cong \triangle PQR

ABC and DBC are two isosceles triangles on the same base BC. Show that \angle ABD = \angle ACD.

AB is a line segment and P is its midpoint. D and E are points on the same side of AB such that \angle BAD = \angle ABE and \angle EPA = \angle DPB. Show that
(i) \triangle DAP \cong \triangle EBP
(ii) AD = BE
In right triangle ABC, right angled at C, M is the mid-point of hypotenuse AB. C is joined to M and produced to a point D such that DM = CM. Point D is joined to point B. Show that :
(i) \triangle AMC \cong \triangle BMD
(ii) \angle DBC is a right angle.
(iii) \triangle DBC \cong \triangle ACB
(iv) CM = \frac{1}{2} AB.
In AC = AE, AB = AD and \angle BAD = \angle EAC. Show that BC = DE
ABC is an isosceles triangle with AB = AC. Show that \angle B = \angle C.

BE and CF are two equal altitudes of a triangle ABC. Using RHS congruence rule, prove that the triangle ABC is isosceles.
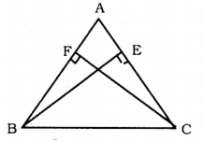
In ( \triangle ABC , AB = BC and | B = 64^{\circ} find | \angle C
In a triangle ABC , AB = AC . Points G on AB and D on AC are such that AE = AD prove that triangles BCD and CBE are congruent
Justify the following statement with reason Difference of any two side is less than the third side
In right triangle the make the statement true
The sum of any two sides of a triangle is ____ than the third side
In \bigtriangleup ABC , AC = AB and the altitude AD bisects BC prove that \bigtriangleup ADC = \bigtriangleup ADB

In Fg. PR > PQ and PS bisects \angle QPR. Prove that \angle PSR > \angle PSQ.
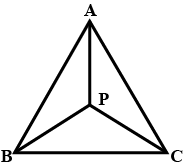
Let ABC be a triangle and P be an interior point , prove that AB + BC + CA < 2 ( PA + PB + PC )
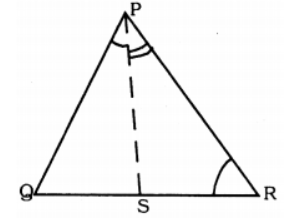
In the adjoining figure If BC = EF then, \angle C = _____ and \angle A = _______
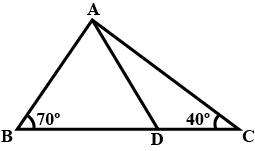
Let AABC be a triangle such that \angle B = 70^{\circ} and \angle C = 40^{\circ} . Suppose D is a point on BC such that AB = AD Prove that Ab > CD
In a \bigtriangleup ABC , AB = AC and | A = 50^{\circ} find \angle B and \angle C
Two triangles ABC and DBC have common base BC. Suppose AB= DC and \angle ABC = \angle BCD , then prove that AC = BD.
In triangles, ABC and PQR, \angle A = \angle Q and \angle B = \angle R then which side of \Delta PQR is equal to side BC of \Delta ABC, so that both triangles congruent? Give reasons for your answer.
If two sides and one angle of a triangle are equal to two sides and the angle of other then both the triangles must congruent to each other. Is this statement true?
Let ABC be a triangle . Drawn a triangle BDC externally on BC such that AB = BD and AC = CD Prove that \bigtriangleup ABC \cong \bigtriangleup DBC
In figure, ADBC is a quadrilateral in which \angle ABC = \angle ABD and BC = BD then show that \Delta ABC \cong \Delta ABD.
In \Delta ABC and \Delta PQR, \angle A = \angle Q and \angle B = \angle R. Then which side of \Delta PQR is equal to side AB of \Delta ABC. So that both triangles become congruent. Give reason for your answer.
If two angles and one side of a triangle are equal to two angles and one side of other triangle then both As must congruent to each other. Is this statement true?
In the adjoining figure If AC = CE and \bigtriangleup ABC \cong \bigtriangleup DEC then \angle D = _____ and \angle A = _______
In figure, Q is a point on side SR of \Delta PSR such that PQ = PR. Prove that PS > PQ.
\Delta ABC and \Delta DBC are two isosceles I triangles on the same base BC and vertices A and D are on the same side of BC (see figure). If AD is extended to intersect BC at P, show that
(i) \Delta ABD \cong \Delta ACD
(ii) \Delta ABP \cong \Delta ACP
(iii) AP bisects \angle A as well as \angle D
(iv) AP is the perpendicular bisector of BC.
AD is the median of any \Delta ABC. Is it true to say that AB + BC + CA > 2AD. Give reason for your answer.
BE and CF are two equal altitudes of \Delta ABC. By using RHS congruency rule, prove that \Delta ABC is an isosceles triangle.
In \Delta ABC, \angle A > \angle B and \angle B > \angle C, then write the smallest side.
In an \Delta ABC with AB = AC, D is any point on the side AC. Show that CD < BD.
In \Delta PQR, S is any point on side QR. Show that PQ + QR + RP > 2PS.
In figure, AD = BD and \angle C = \angle E. Prove that BC = AE.
In figure, O is an interior point of \Delta ABC. Show that: AB + BC + CA < 2(OA + OB +OC).
In any triangle ABC if AB > AC and D is any point on BC then prove that AB > AD.
In the given figure, O is the middle point of both AB and CD. Prove that AC = BD and AC || BD.

In figure, CB = AD and AB = CD. Can we say \angle ABC and \angle ADC are equal? Why?
Prove that the difference of any two sides of a triangle is less than the third side.
In figure, \angle B > \angle A and \angle D > \angle E then show that AE > BD.
In figure, BA \perp AC, DE \perp EF, such that BA = DE and BF = CD, prove that AC = EF.
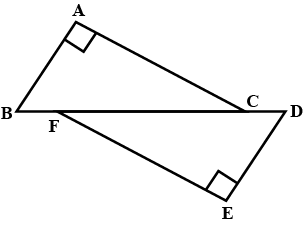
In figure, side AB and AC are produced to point D and E respectively. Bisectors BO and CO of \angle DBC and \angle ECB respectively meet at O. If AB > AC. Prove that OC > OB.

In the given figure, PQRS is a quadrilateral. PQ is its longest side and RS is its shortest side. Prove that: \angle R > \angle P and \angle S > \angle Q.
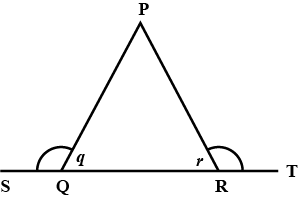
In figure, side QR of \Delta PQR is produced on both sides such that \angle PQS = \angle PRT. Prove that PQ = PR.
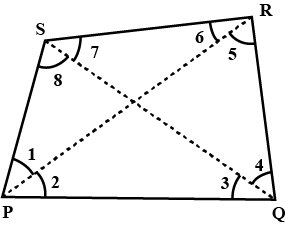
If figure, ABCD is a quadrilateral. Prove that AB + BC + CD + DA > AC + BD.
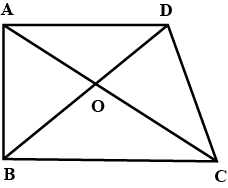
In figure, T is a point on side QR of \Delta PQR and S is a point such that RT = ST. Prove that PQ + PR > QS.
If two sides of a triangle are unequal then the longer side has greater angle opposite to it. Prove it.
In figure, PQR is a triangle and S is any point in its interior, show that SQ + SR < PQ + PR.
For the following congruent triangles, find the pairs of corresponding angles.
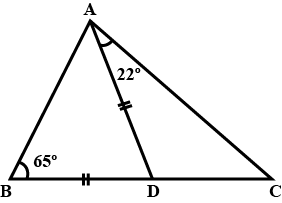
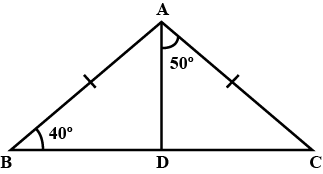
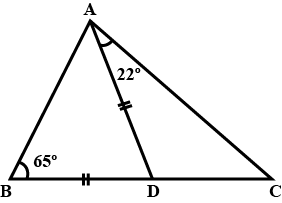
In the adjoining figure, AD=BD \angle{ABD}={65}^{o} & \angle{DAC}={22}^{o}.
Find \angle{ACD}
If a, b, c are sides of a scalene triangle then prove that(a + b + c)^{3} > 27 (a + b - c)(b + c - a)(c + a -b)
In given figures sides AB and BC and median AD of a \triangle ABC are respectively proportional to sides PQ, QR and median PM of \triangle PQR. Show that \triangle ABC \sim \triangle PQR.
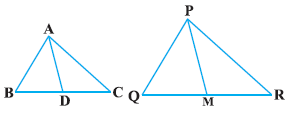
In given figure two sides AB, BC and median AM of one triangle ABC are respectively equal to sides PQ and QR and median PN of \triangle PQR. Show that:
\triangle ABM\cong \triangle PQN
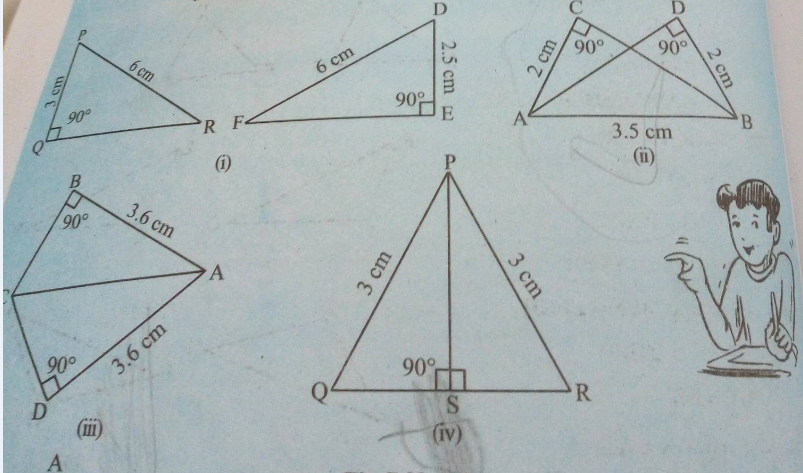
In Fig 7.32, measure of some parts of triangles are given. By applying RHS congruence rule, state which pairs of triangles are congruent. In case of congruent triangles, write the result in symbolic form.
If any point O in the interior of a triangle ABC. Prove that AB + BC + CA > OA + OB + OC.
ABC is an isosceles triangle right angled at B. Equilateral triangles ACD and ABE are constructed on sides AC and AB. Find the ratio between the areas of \triangle{ABE} and \triangle{ACD}.
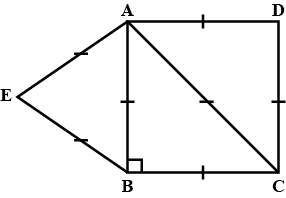
In the adjacent figure ABCD is a square and \Delta A P B is an equilateral triangle. Prove that \Delta A P D \cong \Delta B P C .
(Hint: In \Delta A P D and \Delta B P C \quad \overline { A D } = \overline { B C } , \overline { A P } = \overline { B P } and
\angle P A D = \angle P B C = 90 ^ { \circ } - 60 ^ { \circ } = 30 ^ { \circ } )

O if any point in the interior of a triangle ABC. Prove that AB + AC > OB + OC.
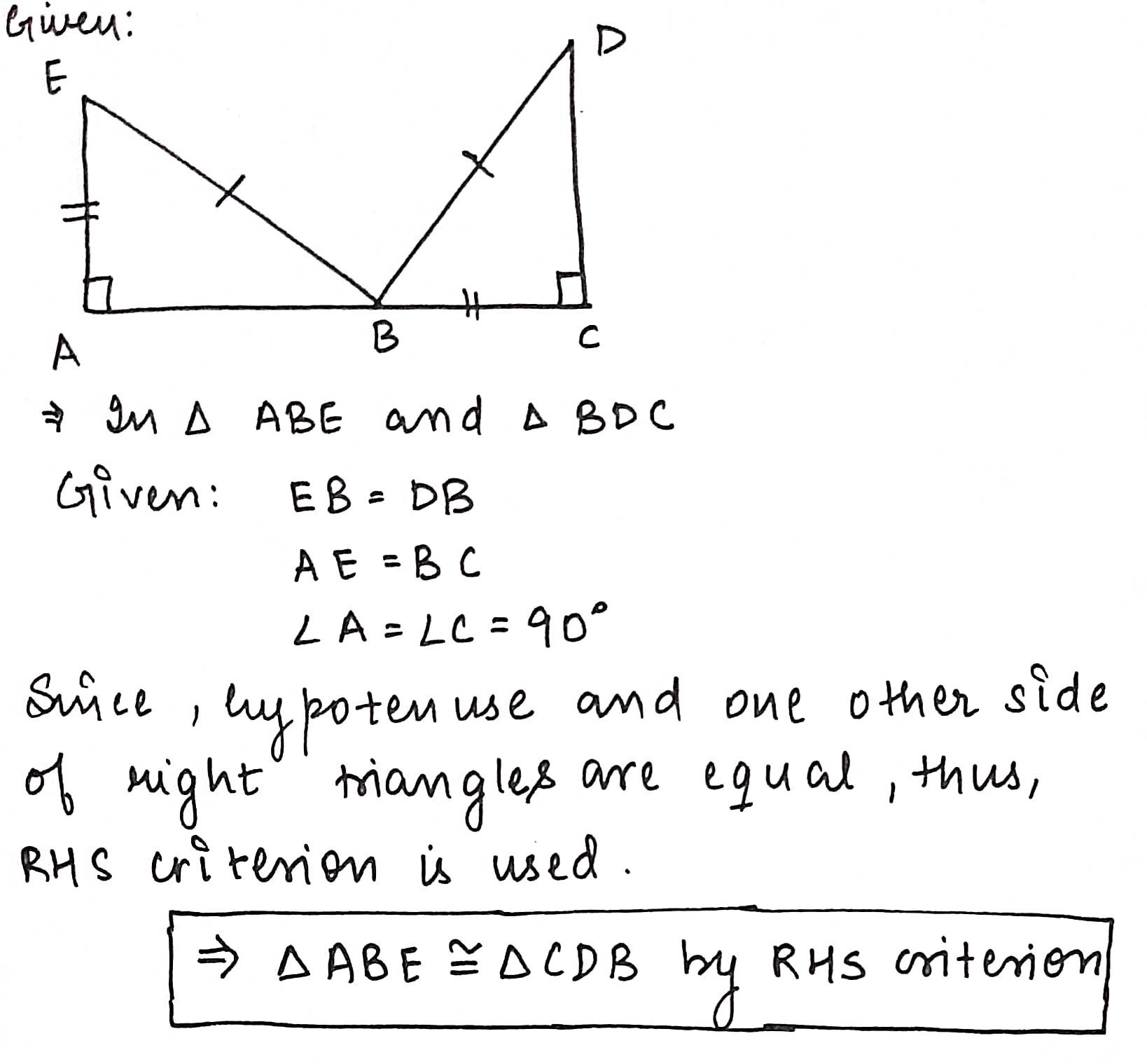
Given : EB = DB
AE = BC
\angle A\, = \,\angle C\, = \,90^\circ
So, \Delta ABE\, \cong \,\Delta CDB
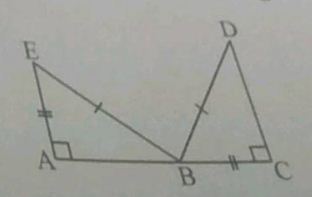
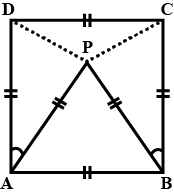
ABC is an isosceles triangle in which AC=BC\ AD and BE are respectively two altitudes of side BC and AC. Prove that AE=BD.
In the given figure, \angle \mathrm { BMN } = \angle \mathrm { CMN } and AN bisects \angle B A C. Prove that \Delta A B M \equiv \Delta A C M.
In the adjacent figure ABCD is a square and \Delta A P B is an equilateral triangle. Prove that \Delta APD \cong \Delta PPC
(Hint : In \Delta APD and \Delta B P C \quad \vec { A D } = \overline { B C } , \overline { A P } = \overline { B P } and \angle P A D = \angle P B C = 90 ^ { \circ } - 60 ^ { \circ } = 30 ^ { \circ } ]

In the figure,prove that \triangle APM \cong \triangle APN if PM=PN, PM\bot AB and PN \bot AC

In \Delta PQR , If PQ>PR and bisectors of \angle Q and \angle R interested at S. Show that SQ>SR.
Find the circumcentre of the triangle with the vertices A (-3,4) , B (3,4) and C (-4,-3). What is the circumradius of the circle?
ABCD is a square. E and F are respectively the mid-point of BC and CD. If R is the mid point of EF prove that
ar(AER)=ar(AFR) .
In right triangle ABC, right angled at C,M is the mid-point of hypotenuse AB. C is joined to M and produced to a point D such that DM = CM. Point D is joined to point B. Show that CM = \dfrac{1}{2}AB
In figure ,if seg\,PR \cong \,seg\,PQ,
show that seg\,PS > \,seg\,PQ.
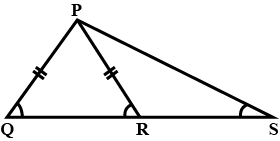
If line segments AB and CD bisect each other
at O, then using SAS congruence rule prove
that \Delta AOC \equiv \Delta BOD .
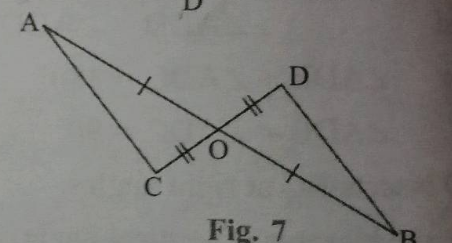
Show that the origin in with in the triangle whose angular points are (2, 1), (3, -2) and (-4, -1).
In the following diagram, AP and BQ are equal and parallel to each other.
Prove that:
(i)\Delta AOP \equiv \Delta BOQ
(ii)AB and PQbisect each other

In a \Delta PQR, if PQ=QR and L, M and N are mid-points of the sides PQ,QR and RP respectively. Prove that LN=MN.
In right triangle ABC, right angled at C, M is the mid-point of hypotenuse AB. C is joined to M and produced to a point D such that DM = CM. Point D is joined to point B (see the given figure). Show that: (i) \triangle{AMC}\cong \triangle{BMD} \left(ii\right) CM = \dfrac{1}{2}AB
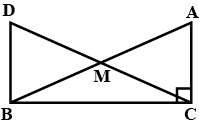
Can you construct a triangle ABC with BC=6cm, \angleB=60^o and AB+AC=5cm? If not give reason.
ABC and D B C are two isosceles triangles on the same base B C (see Fig.). Show that \angle ABD=\angle ACD.
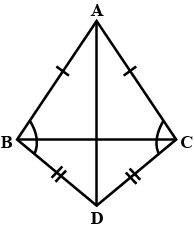
If \Delta ABC \sim \Delta PQR, \ ar( \Delta ABC)=16 \ cm^2 and \ ar(\Delta PQR)=81 \ cm^2, AB=2 \ cm find PQ.
In \Delta ABC,BD \perp AC and CE \perp AB. If BD and CE intersect at O, prove that \angle BOC = 180^{\circ} - A.
In a \Delta ABC, AD bisects \angle A and \angle C > \angle B. Prove that \angle ADB > \angle ADC.
In figure, P is the point on the side BC. Complete the following statement using symbol ' = ' , ' > ' or ' < ' so as to make it true.
AP.....AB+BP.

Class 9 Maths Extra Questions
- Areas Of Parallelograms And Triangles Extra Questions
- Circles Extra Questions
- Constructions Extra Questions
- Coordinate Geometry Extra Questions
- Heron'S Formula Extra Questions
- Introduction To Euclid'S Geometry Extra Questions
- Linear Equations In Two Variable Extra Questions
- Lines And Angles Extra Questions
- Number Systems Extra Questions
- Polynomials Extra Questions
- Probability Extra Questions
- Quadrilaterals Extra Questions
- Statistics Extra Questions
- Surface Areas And Volumes Extra Questions
- Triangles Extra Questions