Alternating Current - Class 12 Engineering Physics - Extra Questions
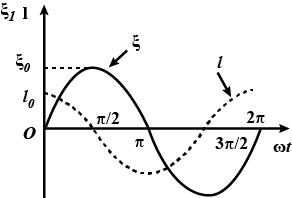
An AC source generating a voltage e=e0sinωt is connected to a capacitor of capacitance C. Find the expression for the current i flowing through it. Plot a graph of e and i versus ωt.
In the double-slit experiment of Fig. 35−10, the electric fields of the waves arriving at point P are given by
E1=(2.00μV/m)sin[(1.26×1015)t]
E2=(2.00μV/m)sin[(1.26×1015)t+39.6 rad]
where time t is in seconds.
In a phasor diagram of the electric fields, what is the angle between the phasors?
What transformation of energy takes place when current is drawn from a cell ?
Write phase difference between current and voltage for an ac circuit, when (I) f=fr (ii) f<fr (iii) f>fr here fr is the resonant frequency.
Add the quantities y1=10sinωt,y2=15sin(ωt+30∘) and y3=5.0sin(ωt−45∘) using the phasor method.
In the double-slit experiment of Fig. 35−10, the electric fields of the waves arriving at point P are given by
E1=(2.00μV/m)sin[(1.26×1015)t]
E2=(2.00μV/m)sin[(1.26×1015)t+39.6rad]
where time t is in seconds.
In a phasor diagram of the electric fields, at what rate would the phasors rotate around the origin?
Find the sum y of the following quantities:
y1=10sinωt and y2=8.0sin(ωt+30∘)
Compare resistance and reactance.
An AC circuit consists of only an inductor of inductance 2H. If the current is represented by a sine wave of amplitude 0.25A and frequency 60Hz, calculate the effective potential difference across the inductor (π=3.142)
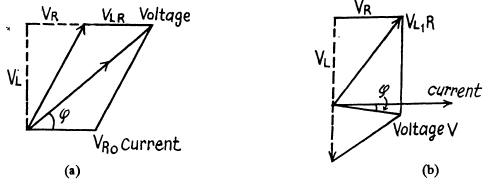
Draw the approximate voltage vector diagrams in the electric circuits shown in Fig.4.33 a,b. The external voltage V is assumed to e alternating harmonically with frequency ω.

A capacitor with capacitance C and coil with active resistance R and inductance L are connected in parallel to a source of sinusoidal voltage voltage of frequency ω. Find the phase difference between the current fed to the circuit and the source voltage.
The power factor of an a.c. circuit is 0.5 what is phase difference between voltage and current.
If a direct current of α unit is superimposed with an alternating current I=βsinωt , then effective value of resulting current is
Match the following column
An electrical device draws 2kW power from AC mains [Voltage 223 V(rms)=√50,000V]. The current differs (lags) in phase by ϕ(tanϕ=−34) as compared to voltage. Find (i) R, (ii) XC−XL, and (iii) IM. Another device has twice the values for R,XC and XL. How are the answers affected?
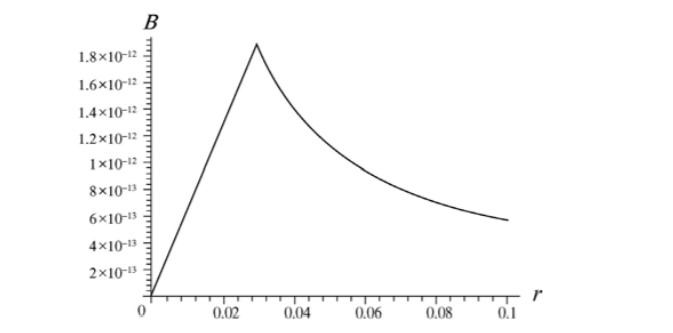
Suppose that a parallel-plate capacitor has circular plates
with radius R=30 mm and plate separation of 5.0 mm.
Suppose also that a sinusoidal potential difference with a maximum value of 150 V and a frequency of 60 Hz is applied across
the plates; that is,
V=(150V)sin[2π(60Hz)t]
(a) Find Bmax(R), the maximum value of the induced magnetic field
that occurs at r=R. (b) Plot Bmax(r) for 0<r<10cm.
The inductive current in the ring varies with time as I=Imsin (ωt−ϕ), where Im=ωϕ0√R2+ω2L2 with tan ϕ=ωL/R.
Additional Problems(81)
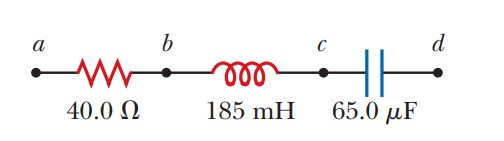
An AC source with Δ=Vrms=120V is connected between points a and d in Figure. At what frequency will it deliver a power of 250W? Explain your answer.
Class 12 Engineering Physics Extra Questions
- Alternating Current Extra Questions
- Atoms Extra Questions
- Current Electricity Extra Questions
- Dual Nature Of Radiation And Matter Extra Questions
- Electric Charges And Fields Extra Questions
- Electromagnetic Induction Extra Questions
- Electromagnetic Waves Extra Questions
- Electrostatic Potential And Capacitance Extra Questions
- Magnetism And Matter Extra Questions
- Moving Charges And Magnetism Extra Questions
- Nuclei Extra Questions
- Ray Optics And Optical Instruments Extra Questions
- Semiconductor Electronics: Materials,Devices And Simple Circuits Extra Questions
- Wave Optics Extra Questions