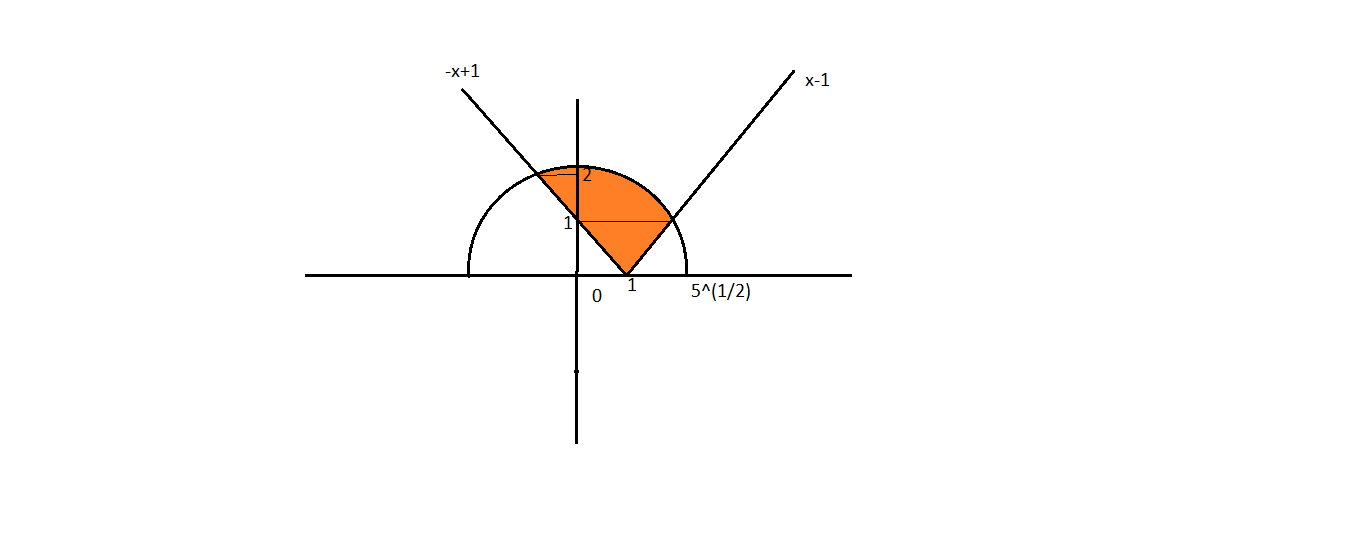Applications Of Integrals - Class 12 Commerce Applied Mathematics - Extra Questions
If y=mx bisects the area enclosed by the lines x=0,y=0,x=32 and the curve y=1+4x−x2 then 6m is equal to
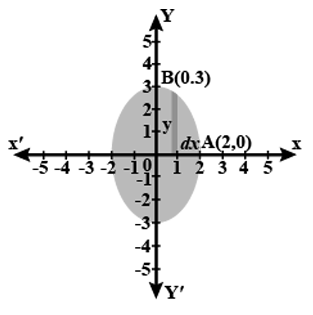
Find the area of the region bounded by the ellipse x24+y29=1.
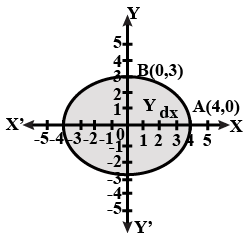
Find the area of the region bounded by the ellipse x216+y29=1.
Find the area of the region bounded by the parabola y=x2 and y=|x|.
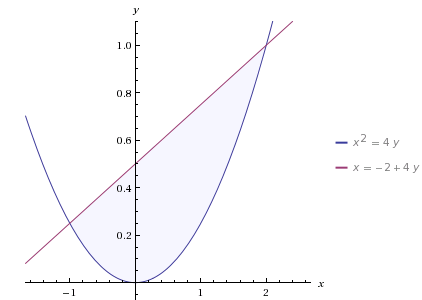
Find the area bounded by the curve x2=4y and the line x=4y−2.
Find the area enclosed between the parabola y2=4ax and the line y=mx.
Find the area of the region bounded by the curves y=x2+2,y=x,x=0 and x=3.
Find the area of the smaller region bounded by the ellipse x2a2+y2b2=1 and the line xa+yb=1
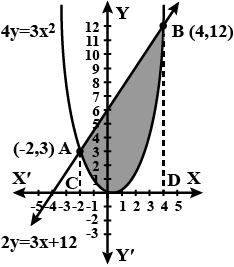
Find the area enclosed by the parabola 4y=3x2 and the line 2y=3x+12
Find the area of the region enclosed by the parabola x2=y and the line y=x+2.
Find the area bounded by curves {(x,y):y≥x2 and y=|x|}
Find the area of the smaller region bounded by the ellipse x29+y24=1 and the line x3+y2=1
Using the method of integration find the area bounded by the curve |x|+|y|=1
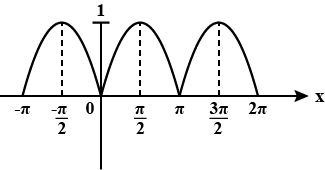
Draw the curve y=sin4x between x=0 and x=2π.
Draw the curve of y=cosx between x=0 and x=2π .
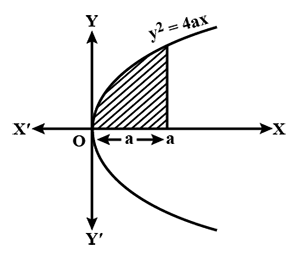
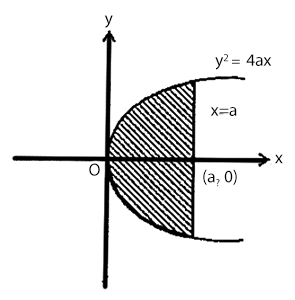
Find the area bounded by the curve y2=4ax, X-axis and the lines x=0 and x=a.
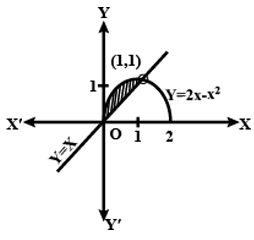
Find the area bounded by the curve y=2x−x2, and the line y=x.
Find the area of the region bounded by the curve y=x2 and the line y=4.
Find the area of the region bounded by the parabola y2=16x and the line x=3.
Find the area bounded by the parabola y2=4x and the straight line y = x. (Draw the figure in answer book)
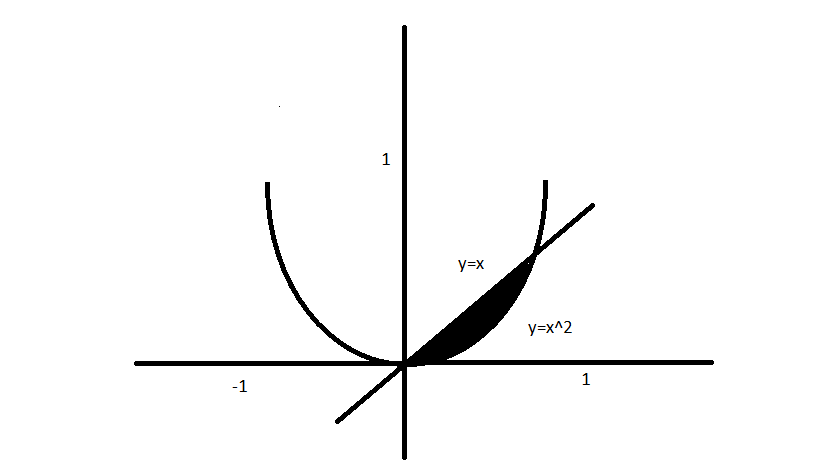
Find the area enclosed between the curves y=x and y=x2 from x=0 and x=1
Find the area lying between the curve y2=4x and the line y=2x.
Find the area of the region bounded by the curve y=x2 and the line y=4.
Find the area of the region bounded by the curve y2=4x and the line x=3.
Find the area enclosed between the parabola 4y=3x2 and the straight line 3x−2y+12=0
Find the area between curve x2=4y and the line x=4y−2.
The area above x-axis, bounded by the line x=4 and the curve y=f(x), where f(x)=x2,0≤x≤1 and f(x)=√x,x≥1, is
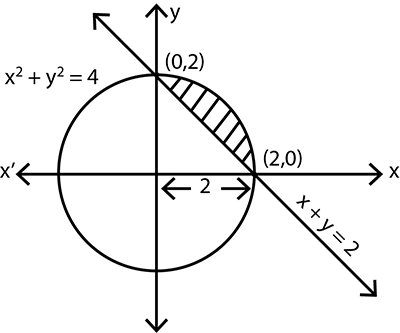
Find the area of the region {(x,y):x2+y2≤4,x+y≥2}.
Sketch the region bounded by the curves y=√5−x2 and y=|x−1| and find its area using intergration.
Using integration, find the area bounded by the curve x2=4y and the line x=4y−2.
Find the area of the region bounded by the parabola y2=4x and the line 2x−y=4.
The area of smaller part bounded by circle x2+y2=a2 and the line x=a√2 is a22(πP−1). Find the value of P.
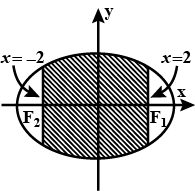
Find the area of the region bounded by the ellipse x29+y25=1 between the two latus rectum.
Find the area of the sector of a circle bounded by the circle x2+y2=16 and the line y=x in the first quadrant.
Find the smaller area enclosed by the circle x^2+y^2=4 and the line x+y = 2.
Find the area of the region bounded by the ellipse \dfrac{x^2}{9} + \dfrac{y^2}{5} = 1 between the two latus rectums.
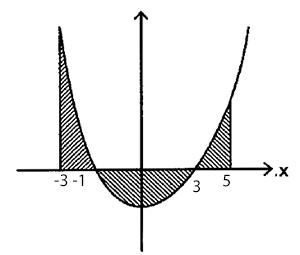
Find the area between the curves y={ x }^{ 2 }-2x-3, x-axis and the lines x=-3 and x=5.
Class 12 Commerce Applied Mathematics Extra Questions
- Application Of Derivatives Extra Questions
- Applications Of Integrals Extra Questions
- Definite Integrals Extra Questions
- Determinants Extra Questions
- Differential Equations Extra Questions
- Indefinite Integrals Extra Questions
- Linear Equations Extra Questions
- Matrices Extra Questions
- Probability Distribution And Its Mean And Variance Extra Questions
- Quantification And Numerical Applications Extra Questions
- Standard Probability Distributions Extra Questions