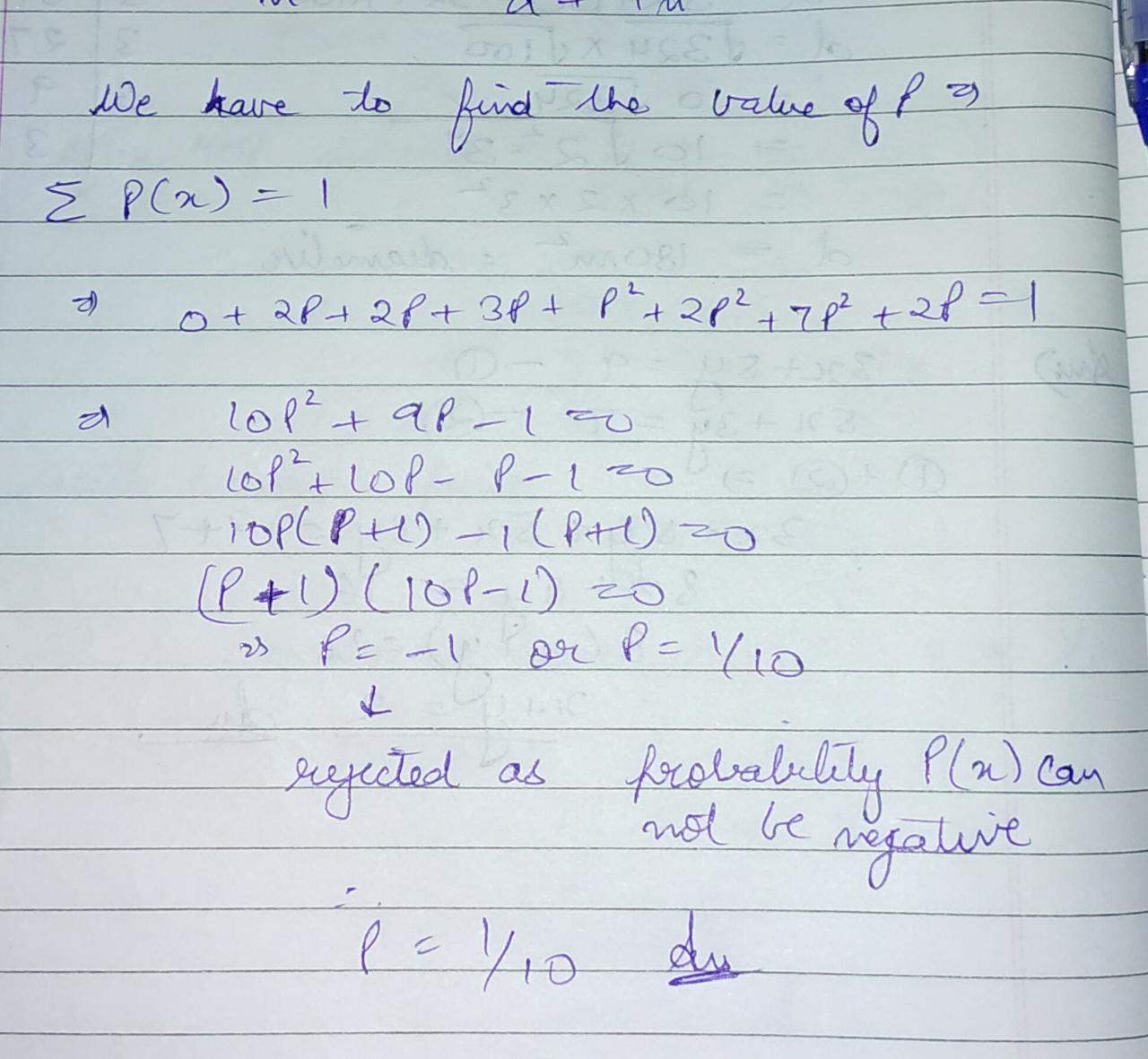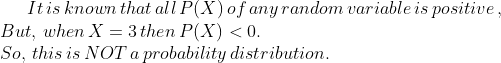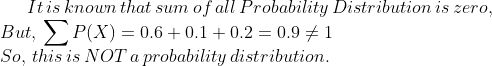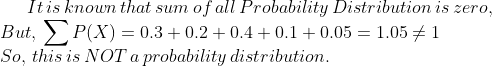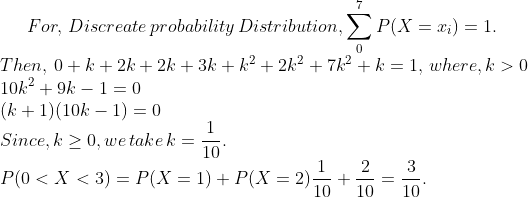Probability Distribution And Its Mean And Variance - Class 12 Commerce Applied Mathematics - Extra Questions

The probability mass function (p.m.f) of $$X$$ is given below:
$$X=x$$$$1$$$$2$$$$3$$
$$P(X=x)$$$$\cfrac{1}{5}$$$$\cfrac{2}{5}$$$$\cfrac{2}{5}$$

We know, $$E({ X }^{ 2 })=\sum { { X }^{ 2 } } P(X)$$
Therefore, $$E({ X }^{ 2 })={ 1 }^{ 2 }\times \cfrac { 1 }{ 5 } +{ 2 }^{ 2 }\times \cfrac { 2 }{ 5 } +{ 3 }^{ 2 }\times \cfrac { 2 }{ 5 } $$
$$=\cfrac { 1 }{ 5 } +\cfrac { 8 }{ 5 } +\cfrac { 18 }{ 5 } =\cfrac { 27 }{ 5 } $$


The mean and variance of a Binomial distribution is $$4$$ and $$3$$ respectively. Find its parameters.

Fact: In binomial distribution, mean $$=np$$ and variance $$=npq$$

Here given $$np=4$$ and $$npq=3$$
So $$q=\dfrac{3}{4}\Rightarrow p=1-p=\dfrac{1}{4}$$
$$np = \cfrac14$$
$$\Rightarrow n\left(\cfrac14\right) = 4$$
$$\Rightarrow n = 16$$


The random variable x has a probability distribution $$P(x)$$ of the following form where $$k$$ is some number:
$$P(x)=\begin{cases}k & if\, x=0\\2k&if\, x=1 \\3k&if\, x=2\\0&otherwise  \end{cases}$$
Determine the value of $$k$$ and $$P(X \le 2).$$

$$\sum _{ x=0 }^{ \infty  }{ P(x)=1 } $$
$$P(1)+P(2)+......=1$$
$$k+2k+3k=1$$
$$k=\dfrac { 1 }{ 6 } $$
$$P(x\le 2)=P(0)+P(1)+P(2)=k+2k+3k=6k=1$$


Find the variance and standard deviation of the random variable $$X$$ whose probability distribution is given below:
$$x$$$$0$$$$1$$$$2$$$$3$$
$$P(X = x)$$$$\dfrac {1}{8}$$$$\dfrac {3}{8}$$$$\dfrac {3}{8}$$$$\dfrac {1}{8}$$

Let mean of $$X$$ be $$\mu$$
$$\therefore \mu=\sum_{x=0}^3 X\times P(X)=\dfrac{12}{8}=\dfrac{3}{2}$$
$$\therefore \text{Var}(X)=\sigma^2=\sum_{x=0}^3 X^2\times P(X)-\mu^2 =\dfrac{24}{8}-\dfrac{9}{4}=\dfrac{3}{4}$$
$$\therefore \text{Standard Deviation}=\sigma=\sqrt{\text{Var}(X)}=\sqrt{\dfrac{3}{4}}=\dfrac{\sqrt{3}}{2}$$
These are the required answers.


The probability that a doctor successfully performs an operation is $$80\%$$. What is the value of $$625$$ times the probability that at least $$3$$ operations out of $$4$$ conducted by him will be successful?

The probability that a doctor successfully perform an operation is 80% = $$\cfrac { 4 }{ 5 } $$
Case(1): All 4 operations are successful
Probability = $${ \left( \cfrac { 4 }{ 5 }  \right)  }^{ 4 }$$
Case(2): One of the 4 operations is unsuccessful
Probability $$=\quad _{ 1 }^{ 4 }{ C }.{ \left( \cfrac { 4 }{ 5 }  \right)  }^{ 3 }\left( \cfrac { 1 }{ 5 }  \right) $$
$$\therefore$$ Total Probability $$=\quad { \left( \cfrac { 4 }{ 5 }  \right)  }^{ 3 }\left( \cfrac { 8 }{ 5 }  \right) $$
$$=\quad { \left( \cfrac { 4 }{ 5 }  \right)  }^{ 3 }\left( \cfrac { 8 }{ 5 }  \right) \\ \cong \quad 81.92%$$


Two cards are drawn simultaneously (or successively without replacement) from a well shuffled pack of $$52$$ cards. Find the Probability that both are king.

No .of favourable outcomes $$4$$
No.of possible out comes $$52$$
Probabilty that it is a king is $$\dfrac{4}{52} * \dfrac{3}{52}=\dfrac{3}{676}$$


Three balls are drawn simultaneously from a bag containing 5 white and 4 red balls. Find the probability distribution of number of red balls drawn.

$$5$$ white     $$4$$ red
$$P(red)=\cfrac { red }{ red+white } =\cfrac { 4 }{ 9 } $$


Find $$k$$ such that the function
$$P(x)=\quad \begin{cases} k\begin{pmatrix} 4 \\ x \end{pmatrix};\quad x=0,1,2,3,4,k>0 \\ 0;\quad otherwise \end{cases}$$ is a probability mass function.

As sum of all probabilities$$=1$$
$$\Rightarrow R({^{4}C_0}+{^{4}C_1}+{^{4}C_2}+{^{4}C_3}+{^{4}C_4})=1$$
$$=R(1+4+6+4+1)=1$$
$$\Rightarrow R(16)=1$$
$$\Rightarrow R=\dfrac{1}{16}$$.

1114475_1195282_ans_70b8f54aa26943aaa796a84af79d034f.jpg


Given $$X \sim B(n, p)$$, If $$E(X)=6, \text{ Var. }(X)=4.2$$, find $$n$$ and $$p$$.

We have,
$$E(x)=6$$
$$np=6$$ 
$$Var.(X)=4.2$$
$$npq=4.2$$

Therefore,
$$\dfrac{npq}{np}=\dfrac{4.2}{6}$$
$$q=0.7$$
$$p=1-q=0.3$$

Therefore,
$$n=\dfrac{6}{p}=\dfrac{6}{0.3}=20$$

Hence, this is the answer.


In a dice game , a player pays a stake of Re $$1$$ for each throw of a die. She receives Rs. $$5$$if the die shows a $$3$$, Rs $$2$$  if the die shows a $$1$$ or $$6$$ , and nothing otherwise . What is the player’s expected profit per throw over a long series of throws ?

 X $$-1$$ $$5$$$$2$$ 
 $$P(X)$$ $$\cfrac{1}{2}\\(either 4,5)$$ $$\cfrac{1}{6}\\(only \quad 3)$$ $$\cfrac{1}{3}\\ (either\quad 1 \quad or\quad 6)$$
From the above table $$\sum P(X)=-\cfrac{1}{2}+\cfrac{5}{6}+\cfrac{2}{3}\\ \quad\quad =Rs1$$
 


A random variable X has the following probability distribution 
X01234
P(X) 0k2k3k1/2
Determine P(0 < X < 3) 

Since, $$P(X)=1$$
$$k+2k+3k+\dfrac{1}{2}=1$$
$$6k=\dfrac{1}{2}$$

$$k=\dfrac{1}{12}$$
Now,
$$P(0<X<3)=P(1)+P(2)$$
$$=k+2k=3k=3\times \dfrac{1}{12}=\dfrac{1}{4}$$


A random variable has the following probability distribution :
Find P

1466792_5829cae709964f5c9e302eed4875f664.png


1306788_1466792_ans_49250299a43c4febb801684109392119.jpeg


Given below is the probability distribution of $$X$$:
$$x$$$$0$$$$1$$$$2$$$$3$$$$4$$
$$P[X=x]$$$$k$$$$2k$$$$4k$$$$2k$$$$k$$
Find:
$$P(X\ge 2), P(X < 3), P(X\le 1)$$.


1303399_1367564_ans_82aa2e6ebe404d169c9253131dbf4a54.jpeg


A purse contains four coins each of which is either a rupee or two rupees coin. Find the expected value of a coin in that purse.

Various possibilities of coins in the purse can be

$$p_{i}$$
$$M_{i}$$
$$p_{i}M_{i}$$
(i) 4 1 rupee coins
$$\displaystyle \frac{1}{16}$$
4
$$\displaystyle \frac{4}{16}$$
(ii) 3 one Rs.+1 two Rs.
$$\displaystyle \frac{4}{16}$$
5
$$\displaystyle \frac{20}{16}$$
(iii) 2 one Rs.+2 two Rs.
$$\displaystyle \frac{6}{16}$$
6
$$\displaystyle \frac{36}{16}$$
(iv) 1 one Rs.+3 two Rs.
$$\displaystyle \frac{4}{16}$$
7
$$\displaystyle \frac{28}{16}$$
(iv) 4 two Rs.
$$\displaystyle \frac{1}{16}$$
8
$$\displaystyle \frac{8}{16}$$



6 / -
Hence expected value is Rs, $$6 / -$$


A pair of dice is thrown $$5$$ times. If getting a doublet is considered as a success, then find the mean and variance of successes.

In a single throw of a pair of dice, probability of getting a doublet$$\displaystyle =\frac{1}{6}$$
considering it to be a success, $$\displaystyle p=\frac{1}{6}$$
$$\therefore $$ $$\displaystyle q=1-\frac{1}{6}=\frac{5}{6}$$
mean$$\displaystyle =5\times \frac{1}{6}=\frac{5}{6},$$ variance$$\displaystyle =5\times \frac{1}{6}.\frac{5}{6}=\frac{25}{36}$$


There are $$100$$ tickets in a raffle (Lottery). There is $$1$$ prize each of Rs. $$1000/-,$$ Rs. $$500/-$$ and Rs. $$200/-.$$ Remaining tickets are blank. Find the expected price of one such ticket.

Expectation$$=\sum p_{i}M_{i}$$ outcome of a ticket can be
$$\displaystyle \begin{matrix}
 & p_{i} & M_{i} & p_{i}M_{i}\\
(i)\: I\: prize & \cfrac{1}{100} & 1000 & 10\\
(ii)\: II\: prize & \cfrac{1}{100} & 500 & 5\\
\ (iii)\: III\: prize & \cfrac{1}{100} & 200 & 2\\
(iv)\: Blank & \cfrac{97}{100} & 0 & 0\\
 &  &  & \sum p_{i}M_{i}=17
\end{matrix}$$
Hence expected price of one such ticket Rs. $$17$$


A pair of dice is thrown $$4$$ times. If getting a total of $$9$$ in a single throw is considered as a success then find the mean and variance of successes.

$$P=$$probability of getting a total of $$\displaystyle 9=\frac{4}{36}=\frac{1}{9}$$
$$\therefore $$ $$\displaystyle 1-\frac{1}{9}=\frac{8}{9}$$
$$\therefore $$ mean$$\displaystyle =np=4\times \frac{1}{9}=\frac{4}{9}$$
variance$$\displaystyle =npq=4\times \frac{1}{9}\times \frac{8}{9}=\frac{32}{81}$$


A fair die is tossed. If $$2, 3$$ or $$5$$ occurs, the player wins that number of rupees, but if $$1, 4$$ or $$6$$ occurs, the player loses that number of rupees. Then find the possible payoffs for the player.

$$A_{i}$$
$$2$$  $$3$$$$5$$
$$1$$
$$4$$
    $$6$$
$$M_{i}$$$$2$$
  $$3$$
$$6$$
$$-1$$
$$-4$$
   $$-6$$
$$P_{i}$$$$1/6$$
  $$1/6$$  
$$1/6$$  
$$1/6$$  
$$1/6$$
   $$1/6$$
Then expected value $$E$$ of the game payoffs for the player
$$\displaystyle =2\left ( \frac{1}{6} \right )+3\left ( \frac{1}{6} \right )+5\left ( \frac{1}{6} \right )-1\left ( \frac{1}{6} \right )-4\left ( \frac{1}{6} \right )-6\left ( \frac{1}{6} \right )=-\left ( \frac{1}{6} \right )$$
Since $$E$$ is negative therefore game is unfavorable to the player.


Find the mean number of heads in three tosses of a fair coin.

Let $$X$$ denote the success of getting heads.
Therefore, the sample space is
$$S = \left\{HHH, HHT, HTH, HTT, THH, THT, TTH, TTT\right\}$$
It can be seen that $$X$$ can take the value of 0, 1, 2 or 3.
$$\therefore P\left(X = 0\right) = P\left(TTT\right)$$
$$= P\left(T\right) \cdot P\left(T\right) \cdot P\left(T\right)$$
$$= \displaystyle\frac{1}{2} \times \displaystyle\frac{1}{2} \times \displaystyle\frac{1}{2}$$
$$= \displaystyle\frac{1}{8}$$
$$\therefore  P\left(X = 1\right) = P\left(HHT\right) + P\left(HTH\right) + P\left(THH\right)$$
$$= \displaystyle\frac{1}{2} \times \displaystyle\frac{1}{2} \times \displaystyle\frac{1}{2} + \displaystyle\frac{1}{2} \times \displaystyle\frac{1}{2} \times \displaystyle\frac{1}{2} + \displaystyle\frac{1}{2} \times \displaystyle\frac{1}{2} \times \displaystyle\frac{1}{2} $$
$$= \displaystyle\frac{3}{8}$$
$$\therefore  P\left(X = 2\right) = P\left(HHT\right) + P\left(HTH\right) + P\left(THH\right)$$
$$= \displaystyle\frac{1}{2} \times \displaystyle\frac{1}{2} \times \displaystyle\frac{1}{2} + \displaystyle\frac{1}{2} \times \displaystyle\frac{1}{2} \times \displaystyle\frac{1}{2} + \displaystyle\frac{1}{2} \times \displaystyle\frac{1}{2} \times \displaystyle\frac{1}{2} $$
$$= \displaystyle\frac{3}{8} $$
$$\therefore   P\left(X =3\right) = P\left(HHH\right)$$
$$= \displaystyle\frac{1}{2} \times \displaystyle\frac{1}{2} \times \displaystyle\frac{1}{2} $$
$$= \displaystyle\frac{1}{8} $$
Therefore, the required probability distribution is as follows.
Mean of $$X  E\left(X\right),  \mu = \sum { { X }_{ i } } P\left( { X }_{ i } \right) $$
$$=0\times \displaystyle\frac { 1 }{ 8 } +1\times \displaystyle\frac { 3 }{ 8 } +2\times \displaystyle\frac { 3 }{ 8 } +3\times \displaystyle\frac { 1 }{ 8 } $$
$$=\displaystyle\frac { 3 }{ 8 } +\displaystyle\frac { 3 }{ 4 } +\displaystyle\frac { 3 }{ 8 } $$
$$=\displaystyle\frac { 3 }{ 2 } $$
$$= 1.5$$


Find the mean and variance for the first $$10$$ multiples of $$3$$

The first 10 multiples of 3 are $$3,6,9,12,15,18,21,24,27,30$$
Here number of observation are $$n=10$$
$$\therefore$$ Mean,$$\displaystyle \bar{X}=\frac{\sum_{i=1}^{10}x_{i}}{10}=\frac{165}{10}=16.5 $$
Now,
$$\displaystyle x_{i}$$
 $$ \displaystyle \left ( X_{i}-\bar{X} \right ) $$ $$ \displaystyle \left ( X_{i}-\bar{X} \right )^{2} $$
3-13.5182.25
6-10.5110.25
9-7.556.25
12-4.520.25
15-1.52.25
181.52.25
214.520.25
247.556.25
2710.5110.25
3013.5182.25


742.5
$$\therefore $$

Variance  $$ \displaystyle \left (\sigma ^{2}  \right

)=\frac{1}{n}\sum_{i=1}^{10}\left ( X_{i}-\bar{X} \right

)^{2}=\frac{1}{10}\times 742.5=74.25 $$


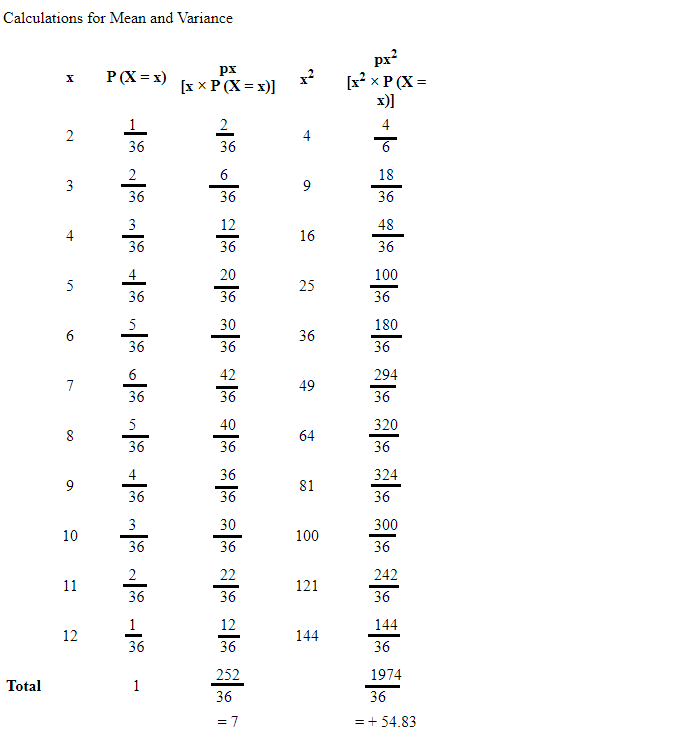
Let $$X$$ denote the sum of the numbers obtained when two fair dice are rolled. Find the variance and standard deviation of $$X$$.

When two fair dice are rolled, $$6 \times 6 = 36$$ observations are obtained.
$$P\left(X = 2\right) = P\left(1, 1\right) = \displaystyle\frac{1}{36}$$
$$P\left( X=3 \right) =P\left( 1,2 \right) +P\left( 2,1 \right) =\displaystyle\frac { 2 }{ 36 } =\displaystyle\frac { 1 }{ 18 } $$
$$P\left( X=4 \right) =P\left( 1,3 \right) +P\left( 2,2 \right) +P\left( 3,1 \right) =\displaystyle\frac { 3 }{ 36 } =\displaystyle\frac { 1 }{ 12 } $$
$$P\left( X=5 \right) =P\left( 1,4 \right) +P\left( 2,3 \right) +P\left( 3,2 \right) +P\left( 4,1 \right) =\displaystyle\frac { 4 }{ 36 } =\displaystyle\frac { 1 }{ 9 } $$
$$P\left( X=6 \right) =P\left( 1,5 \right) +P\left( 2,4 \right) +P\left( 3,3 \right) +P\left( 4,2 \right) +P\left( 5,1 \right) =\displaystyle\frac { 5 }{ 36 } $$
$$P\left( X=7 \right) =P\left( 1,6 \right) +P\left( 2,5 \right) +P\left( 3,4 \right) +P\left( 4,3 \right) +P\left( 5,2 \right) +P\left( 6,1 \right) =\displaystyle\frac { 6 }{ 36 } =\displaystyle\frac { 1 }{ 6 } $$
$$P\left( X=8 \right) =P\left( 2,6 \right) +P\left( 3,5 \right) +P\left( 4,4 \right) +P\left( 5,3 \right) +P\left( 6,2 \right) =\displaystyle\frac { 5 }{ 36 } $$
$$P\left( X=9 \right) =P\left( 3,6 \right) +P\left( 4,5 \right) +P\left( 5,4 \right) +P\left( 6,3 \right) =\displaystyle\frac { 4 }{ 36 } =\displaystyle\frac { 1 }{ 9 } $$
$$P\left( X=10 \right) =P\left( 4,6 \right) +P\left( 5,5 \right) +P\left( 6,4 \right) =\displaystyle\frac { 3 }{ 36 } =\displaystyle\frac { 1 }{ 12 } $$
$$P\left( X=11 \right) =P\left( 5,6 \right) +P\left( 6,5 \right) =\displaystyle\frac { 2 }{ 36 } =\displaystyle\frac { 1 }{ 18 } $$
$$P\left( X=12 \right) =P\left( 6,6 \right) =\displaystyle\frac { 1 }{ 36 } $$
Therefore, the required probability distribution is as follows.
Then, $$E\left( X \right) =\sum { { X }_{ i }\cdot P\left( { X }_{ i } \right)  } $$
$$=2\times \displaystyle\frac { 1 }{ 36 } +3\times \displaystyle\frac { 1 }{ 18 } +4\times \displaystyle\frac { 1 }{ 12 } +5\times \displaystyle\frac { 1 }{ 9 } +6\times \displaystyle\frac { 5 }{ 36 } +7\times \displaystyle\frac { 1 }{ 6 } +8\times \displaystyle\frac { 5 }{ 36 } +9\times \displaystyle\frac { 1 }{ 9 } +10\times \displaystyle\frac { 1 }{ 12 } +11\times \displaystyle\frac { 1 }{ 18 } +12\times \displaystyle\frac { 1 }{ 36 } $$
$$=\displaystyle\frac { 1 }{ 18 } +\displaystyle\frac { 1 }{ 6 } +\displaystyle\frac { 1 }{ 3 } +\displaystyle\frac { 5 }{ 9 } +\displaystyle\frac { 5 }{ 6 } +\displaystyle\frac { 7 }{ 6 } +\displaystyle\frac { 10 }{ 9 } +1+\displaystyle\frac { 5 }{ 6 } +\displaystyle\frac { 11 }{ 18 } +\displaystyle\frac { 1 }{ 3 } $$
$$= 7$$
$$E\left( { X }^{ 2 } \right) =\sum { { X }_{ i }^{ 2 }\cdot P\left( { X }_{ i } \right)  } $$
$$=4\times \displaystyle\frac { 1 }{ 36 } +9\times \displaystyle\frac { 1 }{ 18 } +16\times \displaystyle\frac { 1 }{ 12 } +25\times \displaystyle\frac { 1 }{ 9 } +36\times \displaystyle\frac { 5 }{ 36 } +49\times \displaystyle\frac { 1 }{ 6 } +64\times \displaystyle\frac { 5 }{ 36 } +81\times \displaystyle\frac { 1 }{ 9 } +100\times \displaystyle\frac { 1 }{ 12 } +121\times \displaystyle\frac { 1 }{ 18 } +144\times \displaystyle\frac { 1 }{ 36 } $$
$$=\displaystyle\frac { 1 }{ 9 } +\displaystyle\frac { 1 }{ 2 } +\displaystyle\frac { 4 }{ 3 } +\displaystyle\frac { 25 }{ 9 } +5+\displaystyle\frac { 49 }{ 6 } +\displaystyle\frac { 80 }{ 9 } +9+\displaystyle\frac { 25 }{ 3 } +\displaystyle\frac { 121 }{ 18 } +4$$
$$=\displaystyle\frac { 987 }{ 18 } =\displaystyle\frac { 329 }{ 6 } =54.833$$
Then, $$Var\left( X \right) =E\left( { X }^{ 2 } \right) -{ \left[ E\left( X \right)  \right]  }^{ 2 }$$
$$=54.833-{ \left( 7 \right)  }^{ 2 }$$
$$= 54.833 - 49$$
$$= 5.833$$
$$\therefore $$ Standard deviation $$=\sqrt { Var\left( X \right)  } $$
$$=\sqrt { 5.833 } $$
$$=2.415$$


If $$F\left( x \right) =\dfrac { 1 }{ \pi  } \left( \dfrac { \pi  }{ 2 } +\tan ^{ -1 }{ x }  \right)$$, $$-\infty < x < \infty $$ is a distribution function of a continuous variable $$X$$, then find $$P\left( 0\le x\le 1 \right) $$

Given, $$F\left( x \right) =\dfrac { 1 }{ \pi  } \left( \dfrac { \pi  }{ 2 } +\tan ^{ -1 }{ \left( x \right)  }  \right) $$
Therefore, $$P\left( 0\le x\le 1 \right) =F\left( 1 \right) \sim F\left( 0 \right) $$
$$=\dfrac { 1 }{ \pi  } \left( \dfrac { \pi  }{ 2 } +\tan ^{ -1 }{ \left( 1 \right)  }  \right) -\dfrac { 1 }{ \pi  } \left( \dfrac { \pi  }{ 2 } +\tan ^{ -1 }{ \left( 0 \right)  }  \right) $$
$$=\dfrac { 1 }{ \pi  } \left( \dfrac { \pi  }{ 2 } +\dfrac { \pi  }{ 4 }  \right) -\dfrac { 1 }{ \pi  } \left( \dfrac { \pi  }{ 2 } +0 \right) $$
$$=\dfrac { 1 }{ \pi  } \left( \dfrac { \pi  }{ 2 } +\dfrac { \pi  }{ 4 } -\dfrac { \pi  }{ 2 }  \right) =\dfrac { 1 }{ 4 } $$


Given the p.d.f. (probability density function) of a continuous random variable $$x$$ as
$$f(x) =\begin{cases} \dfrac{x^2}{3}\,\,\,  -1 < x < 2\\\\ 0 \,\,\, \text{otherwise}\end{cases}$$
Determine the c.d.f. (cumulative distribution function) of $$x$$ and hence find $$P(x < 1), P(x \leq -2), P(x > 0), P(1 < x < 2)$$.

c.d.f. of the continuous variable is given by,
$$f(x) = \displaystyle \int_{-1}^{x} \cfrac{y^2}{3} dy$$
$$= \left [ \cfrac{y^3}{9} \right ]^x_{-1}$$
$$=  \cfrac{(x^3 + 1)}{9} , x \in R$$
Consider $$P(x < 1) = f(1) = \cfrac{(1)^3 + 1}{9} = \cfrac{2}{9}$$
$$P(x \leq -2) = 0$$
$$P(x > 0) = 1 - P(x \leq 0)$$
$$= 1 - f(0)$$
$$= 1 - \left( \cfrac{1}{9} \right ) = \cfrac{8}{9}$$
$$P(1 < x < 2) = f(2) - f(1)$$
$$= 1 - \cfrac{2}{9}$$
$$= \cfrac{7}{9}$$


Find the mean and variance of distribution
$$f(x) = \left\{\begin{matrix}3e^{-x} & 0<x<\infty \\ 0 & \text{elsewhere}  \end{matrix}\right.$$

$$f(x)=\begin{cases} 3{ e }^{ -x },0<x<\infty \quad  \\ 0,-\infty <x\le 0 \end{cases}$$
$$Mean = \int _{ -\infty  }^{ \infty  }{xf(x)\quad dx } $$

   $$\mu = $$ $$\int _{ -\infty  }^{ 0 }{ x.0\quad dx\quad +\quad \int _{ 0 }^{ \infty  }{ 3x{ e }^{ -x }\quad dx }  } $$
   $$\mu = $$ $$0\quad - \quad$$ $$3{ \left| x{ e }^{ -x } \right|  }_{ 0 }^{ \infty  }\quad -\quad 3\int _{ 0 }^{ \infty  }{ -{ e }^{ -x }\quad dx } $$
   $$\mu = 0\quad +\quad (-3){ \left| { e }^{ -x } \right|  }_{ 0 }^{ \infty  }$$
   $$\boxed{ \mu = 3 }$$
$$Variance = \int _{ -\infty  }^{ \infty  }{ { (x-\mu ) }^{ 2 }f(x)\quad dx } $$
    $${ \sigma  }^{ 2 } = $$ $$0\quad +\quad \int _{ 0 }^{ \infty  }{ { (x-3) }^{ 2 }.3{ e }^{ -x }\quad dx } $$
    $${ \sigma }^{2} = $$ $$\int _{ 0 }^{ \infty  }{ 3{ x }^{ 2 }{ e }^{ -x }dx\quad +\quad \int _{ 0 }^{ \infty  }{ 27{ e }^{ -x }dx\quad -\quad \int _{ 0 }^{ \infty  }{ 6.3x{ e }^{ -x }dx }  }  } $$   
    $${ \sigma }^{2} = $$ $$6-27 \left| {e}^{-x} \right | _{0}^{\infty} - 6*3$$
    $$\boxed{ { \sigma }^{ 2 } = 15 }$$
   


The following is the p.d.f. (Probability Density Function) of a continuous random variable $$X$$:
$$F(x) = \dfrac {x}{32}, 0 < x < 8; = 0$$, otherwise
Also find its value at $$x = 0.5$$ and $$9$$.

Values of $$F(x)$$ at different values of $$x$$.
At $$x = 0.5$$
$$ F(x) = F(0.5) = \dfrac {(0.5)^{2}}{64} $$.............(integration of F(x))
$$= \dfrac {0.25}{64}$$
$$ = \dfrac {1}{256}$$
For any value of $$x$$ greater than or equal to $$8, F(x) = 1$$
$$\therefore F(9) = 1$$


A random variable $$X$$ has the following probability distribution:
$$X=x$$$$0$$$$1$$$$2$$$$3$$$$4$$$$5$$$$6$$
$$P(X=x)$$$$k$$$$3k$$$$5k$$$$7k$$$$9k$$$$11k$$$$13k$$
(a) Find $$k$$
(b) Find $$P(0< X< 4)$$
(c) Obtain cumulative distribution function (c.d.f) of $$X$$.

(a) $$K+3K+5K+7K+9K+11K+13K=1$$
$$\therefore$$ $$49K=1$$
$$\therefore$$ $$K=\cfrac{1}{49}$$
(b)  $$P(0< x< 4)=P(1)+P(2)+P(3)$$
$$\cfrac { 3 }{ 49 } +\cfrac { 10 }{ 49 } +\cfrac { 21 }{ 49 } =\cfrac { 34 }{ 49 } $$
(c) C.D.F $$=(2x+1)K,0\le x$$


The table gives the probability distribution of a random variable $$X$$.
$$x$$12345
$$P(X=x)$$$$0.2$$$$0.1$$$$0.3$$$$0.3$$$$p$$
(i)  Find $$P$$.
(ii)  Find the mean of $$X$$
(iii)  Find the variance of $$X$$.

$$\textbf{Using the concept of probability distribution}$$
$$\text{The given data is,}$$
 $$x$$                     $$1$$               $$2$$             $$3$$           $$4$$          $$5$$
$$P(X=x)$$    $$0.2$$           $$0.1$$          $$0.3$$        $$0.3$$         $$p$$

$$\text{Now, we know}$$ $$\sum_{i=1}^{5}P(X=i)=1$$
$$\Rightarrow (0.2+0.1+0.3+0.3+p)=1$$
$$\Rightarrow0.9+p=1$$
$$\Rightarrow p=0.1$$

$$\text{Mean of X, M=}$$  $$\dfrac { 1+2+3+4+5 }{ 5 } = 3$$
$$\text{Variance of}$$ $$X=\dfrac { { (1-3) }^{ 2 }+{ (2-3) }^{ 2 }+{ (3-3) }^{ 2 }+{ (4-3) }^{ 2 }+{ (5-3) }^{ 2 } }{ 4 } = \dfrac { 4+1+1+4 }{ 4 } =\dfrac { 5 }{ 2 } $$
$$\textbf{Hence the respective values of p, M and variance are}$$ $$\boldsymbol{0.1, 3}$$ $$\textbf{and}$$ $$\boldsymbol{\dfrac{5}{2}}$$



The range of a random variable $$X$$ is $$\{0, 1, 2\}$$.
Given that $$P(X = 0) = 3C^3, P(X = 1) = 4C - 10C^2, P(X = 2) = 5C - 1$$. Find the value of:
(i) $$C$$
(ii) $$P(X < 1)$$
(iii) $$P(1 < X \leq 2)$$ and $$P(0< X \leq 3)$$.

(i) We must have $$\sum P(X)=1$$
$$\Rightarrow P(X=0)+P(X=1)+P(X=2)=1$$
$$\Rightarrow (3C^3)+(4C-10C^2)+(5C-1)=1$$
$$\Rightarrow 3C^3-10C^2+9C-2=0$$
$$\Rightarrow (C-1)(3C^2-7C+2)=0$$
$$\Rightarrow (C-1)(C-2)(3C-1)=0$$
$$\Rightarrow C=1,2,\dfrac{1}{3}$$
Also $$0\le P(X=0), P(X=1), P(X=2)\le 1$$
So only acceptable value of $$C$$ is $$\dfrac{1}{3}$$

(ii) $$P(X<1)=P(X=0)=3\left(\dfrac{1}{3}\right)^3=\dfrac{1}{9}$$

(iii) $$P(1<X\le 2)=P(X=2)=4C-10C^2=4\cdot \dfrac{1}{3}-10\cdot \dfrac{1}{9}=\dfrac{2}{9}$$
and 
$$P(0<X\le 3)=1-P(X=0)=1-\dfrac{1}{9}=\dfrac{8}{9}$$


X =12345
P(X =)$$\dfrac{1}{2}$$
$$\dfrac{1}{4}$$$$\dfrac{1}{8}$$$$\dfrac{1}{16}$$P
The probability distribution of a random variable X taking values 1, 2, 3, 4, 5 is given
(a) Find the value of P.
(b) Find the mean of X.
(c) Find the variance of X.

$$\sum _{ i=1 }^{ 5 }{ P({ X }=i) } =1$$
$$\Rightarrow P({ X }=1)+P({ X }=2)+P({ X }=3)+P({ X }=4)+P({ X }=5) =1$$
$$\displaystyle \Rightarrow \frac { 1 }{ 2 } +\frac { 1 }{ 4 } +\frac { 1 }{ 8 } +\frac { 1 }{ 16 } +P=1$$
$$\Rightarrow P=1-\cfrac { 1 }{ 16 } $$
$$\Rightarrow P= \cfrac { 15 }{ 16 } $$
Mean of $$X=\cfrac { 1+2+3+4+5 }{ 5 } = 3$$
Variance of $$\displaystyle X=\frac { { (1-3) }^{ 2 }+{ (2-3) }^{ 2 }+{ (3-3) }^{ 2 }+{ (4-3) }^{ 2 }+{ (5-3) }^{ 2 } }{ 4 } = \frac { 4+1+1+4 }{ 4 } =\frac { 5 }{ 2 } $$


The mean and variance of a binomial distribution are $$4$$ and $$3$$ respectively. Fix the distribution and find $$P(X \geq 1)$$.

Fact: In binomial distribution , mean $$=np$$ and variance $$=npq$$
Here given $$np=4$$ and $$npq=3$$
So $$q=\dfrac{3}{4}\Rightarrow p=1-p=\dfrac{1}{4}$$
Thus $$n=16$$
Hence $$P(X\ge 1)=1-P(X=0)=1-^{16}C_0p^0q^{16}=1-\left(\dfrac{3}{4} \right)^{16}$$


Verify $$f(x)=\begin{cases} 30{ x }^{ 4 }{ e }^{ -6{ x }^{ 5 } };\quad \quad x>0 \\ 0;\quad otherwise \end{cases}$$ for p.d.f if $$f(x)$$ is a p.d.f then find $$P(1)$$.

$$\displaystyle p.d.f \text{ of } f(x)=\int_0^\infty30x^4e^{-6x^5}dx$$

Let  $$ 6x^5=t\Rightarrow 30x^4=dt$$, we get

$$\Rightarrow \displaystyle \int_0^\infty e^{-t} dt=[-e^{-t}]_0^\infty=1$$


From a lot of 30 bulbs which include 6 defectives, a sample of 4 bulbs is draw at random with replacement.Find the probability distribution of the number defective bulbs.

We have a lot of $$30$$  bulbs, out of which $$6$$ are defective, so, $$24$$ are non defective.
probability of drawing a defective bulb $$=\dfrac{6}{30}=\dfrac{1}{5}$$.
$$\Rightarrow$$ probability of drawing a non-defective bulb =$$\dfrac{4}{5}$$.
Let $$X$$ be the random variable denoting the drawing of $$4$$ defective bulbs from the lot with replacement.
Then the probability distribution is 
$$X\ :$$             $$0$$                          $$ 1$$                                        $$2$$                                  $$3$$                             $$4$$

$$P(X)\ :$$       $$\left(\dfrac{1}{5}\right)^4$$         $$\left(\dfrac{1}{5}\right)^3\times $$$$\left(\dfrac{4}{5}\right)$$           $$\left(\dfrac{1}{5}\right)^2\times$$$$\left(\dfrac{4}{5}\right)^2$$         $$\left(\dfrac{1}{5}\right)^1\times$$$$\left(\dfrac{4}{5}\right)^3$$            $$\left(\dfrac{4}{5}\right)^4$$    

     or, 

$$X\ :$$             $$0$$                  $$ 1$$                 $$2$$              $$3$$              $$4$$

$$P(X)\ :$$       $$\dfrac{1}{625}$$          $$\dfrac{4}{625}$$          $$\dfrac{16}{625}$$       $$\dfrac{64}{625}$$        $$\dfrac{256}{625}$$   
     



From a lot of $$6$$ items containing $$2$$ defective items, a sample of $$4$$ items are drawn at random. Let the random variable X denote the number of defective items in the sample. If the sample is drawn without replacement, find Variance of X.

We have a lot of $$6$$ items, 2 are defective $$\rightarrow 6-2=4$$ are non-defective.
P (drawing a defective item) = $$\dfrac{2}{6}=\dfrac{1}{3}$$
P (drawing a non-defective item) = 1 - P (drawing a defective item) $$=1-\dfrac{1}{3}=\dfrac{2}{3}$$
Note$$: 4$$ items can be drawn from a lot of 6 items in $$^{6}C_4$$ ways.
Let $$X$$ be the random variable of drawing 2 defective items from the lot.
$$P (X = 0) =$$ P (no defective item in the sample) = $$\dfrac{^{4}C_4}{^{6}C_4}=\dfrac{1}{15}$$
$$P (X = 1) =$$ P (one defective item from the lot) = P(one defective, 3 non-defective items) $$=(\large\frac{^{2}C_1\;^{4}C_3}{^{6}C_4}) = \dfrac{8}{15}$$
$$P (X = 2) =$$ P (two defective items from the lot) = P(2 defective, 2 non-defective bulbs) =$$\large\frac{^{2}C_2\;^{4}C_2}{^{6}C_4} = \dfrac{6}{15}$$
Therefore the required probability distribution is as follows: 
$$X: \space\space\space\space\space\space\space\space\space 0\space\space\space\space 1\space\space\space\space 2$$
$$P(X) :\dfrac{1}{15}\space\dfrac{8}{15}\space\dfrac{6}{15}$$

So Variance of $$X$$ will be $$:E(X^2)-(E(X))^2$$
$$E(X)=\sum_{i=0}^{2}x_iP(X=x_i)$$
$$\rightarrow 0\times \dfrac{1}{15}+1\times\dfrac{8}{15}+2\times\dfrac{6}{15}=\dfrac{20}{15}$$
$$E(X^2)=\sum_{i=0}^{2}x_i^2P(X=x_i)$$
$$\rightarrow 0^2\times\dfrac{1}{15}+1^2\times\dfrac{8}{15}+2^2\times\dfrac{6}{15}=\dfrac{32}{15}$$
Variance of $$X=\dfrac{32}{15}-(\dfrac{20}{15})^2=\dfrac{16}{45}$$


From a lot of $$6$$ items containing $$2$$ defective items, a sample of $$4$$ items are drawn at random. Let the random variable X denote the number of defective items in the sample. If the sample is drawn without replacement, find Mean of X.

We have a lot of $$6$$ items, 2 are defective $$\rightarrow 6-2=4$$ are non-defective.
P (drawing a defective item) = $$\dfrac{2}{6}=\dfrac{1}{3}$$
P (drawing a non-defective item) = 1 - P (drawing a defective item) $$=1-\dfrac{1}{3}=\dfrac{2}{3}$$
Note$$: 4$$ items can be drawn from a lot of 6 items in $$^{6}C_4$$ ways.
Let $$X$$ be the random variable of drawing 2 defective items from the lot.
$$P (X = 0) =$$ P (no defective item in the sample) = $$\dfrac{^{4}C_4}{^{6}C_4}=\dfrac{1}{15}$$
$$P (X = 1) =$$ P (one defective item from the lot) = P(one defective, 3 non-defective items) $$=(\large\frac{^{2}C_1\;^{4}C_3}{^{6}C_4}) = \dfrac{8}{15}$$
$$P (X = 2) =$$ P (two defective items from the lot) = P(2 defective, 2 non-defective bulbs) =$$\large\frac{^{2}C_2\;^{4}C_2}{^{6}C_4} = \dfrac{6}{15}$$
Therefore the required probability distribution is as follows: 
$$X: \space\space\space\space\space\space\space\space\space 0\space\space\space\space 1\space\space\space\space 2$$
$$P(X) :\dfrac{1}{15}\space\dfrac{8}{15}\space\dfrac{6}{15}$$

So Mean of $$X$$ will be$$:\sum_{i=0}^{2}x_iP(X=x_i)$$
$$\rightarrow 0\times \dfrac{1}{15}+1\times\dfrac{8}{15}+2\times\dfrac{6}{15}=\dfrac{4}{3}$$


A random variable $$x$$ has the following probability distribution:
$$x$$$$0$$$$1$$$$2$$$$3$$$$4$$$$5$$$$6$$
$$P(X = x)$$$$k$$$$3k$$$$5k$$$$7k$$$$9k$$$$11k$$$$13k$$
Find $$'k'$$.

$$ x$$$$ 0$$$$1$$ $$2$$ $$3$$$$ 4$$ $$5$$ $$6$$
 $$P(X = x)$$  $$k$$ $$3k$$ $$5k$$ $$7k$$ $$9k$$ $$11k$$ $$13k$$
We know,

$$\displaystyle\sum _{ r=0 }^{ 6 }{ P(X=x) } =1$$

$$\therefore k+3k+5k+7k+9k+11k+13k=1$$

$$49k=1$$

$$k=\cfrac { 1 }{ 49 } $$


The mean and standard deviation of a random variable X are $$10$$ and $$5$$ respectively. Find.
$$E\left(\left(\dfrac{x-10}{5}\right)^2\right)$$.

$$E(X)=10$$

We know that $$E\left(\dfrac{x-10}{5}\right)=E\left(\dfrac{x}{5}-2\right)$$

$$=\dfrac{E(X)}{5}-2$$

$$=\dfrac{10}{5}-2$$

$$=0$$

Standard deviation is $$\sigma=5$$

$$E\left(\left(\dfrac{x-10}{5}\right)^2\right)-\left[E\left(\dfrac{x-10}{5}\right)\right]^2=\sigma^2$$

$$\implies E\left(\left(\dfrac{x-10}{5}\right)^2\right)=25$$


The P.M.F of a r.v. $$X$$ is $$P\left( x \right) = \frac{1}{5}$$ for $$x = 1,2,3, \ldots 14,15;$$ $$P(x)=0$$ otherwise.
Find (i) $$E(X)$$ (ii) $$Var(X)$$

i)E(X)=$$\sum { xP(X=x) } =1*\frac { 1 }{ 5 } +2*\frac { 1 }{ 5 } +3*\frac { 1 }{ 5 } ...+15*\frac { 1 }{ 5 } =\frac { 15*16 }{ 2*5 } =24\\ $$
ii)Var(X)=$$=E({ X }^{ 2 })-{ E(X) }^{ 2 }$$
$$\Rightarrow E({ X }^{ 2 })=\sum { { x }^{ 2 }P(X=x) } ={ 1 }^{ 2 }*\frac { 1 }{ 5 } +2^{ 2 }*\frac { 1 }{ 5 } +{ 3 }^{ 2 }*\frac { 1 }{ 5 } ...+{ 15 }^{ 2 }*\frac { 1 }{ 5 } =\frac { 15*16*31 }{ 6*5 } =31*8=248$$
Var(X)=248-576=-328


The mean and standard deviation of a random variable X are $$10$$ and $$5$$ respectively. Find.
$$E\left(\left(\dfrac{x-10}{5}\right)\right)$$.

$$E(X)=10$$

We know that $$E\left(\dfrac{x-10}{5}\right)=E\left(\dfrac{x}{5}-2\right)$$

$$=\dfrac{E(X)}{5}-2$$

$$=\dfrac{10}{5}-2$$

$$=0$$


The mean and standard deviation of a random variable X are $$10$$ and $$5$$ respectively. Find $$E(X^2)$$

$$Mean=E(X)=10$$

standard deviation$$=\sigma=5$$

We know that

$$\sigma^2=E(X^2)-(E(X))^2$$

$$\implies 5^2=E(X^2)-10^2$$

$$\implies E(X^2)=125$$


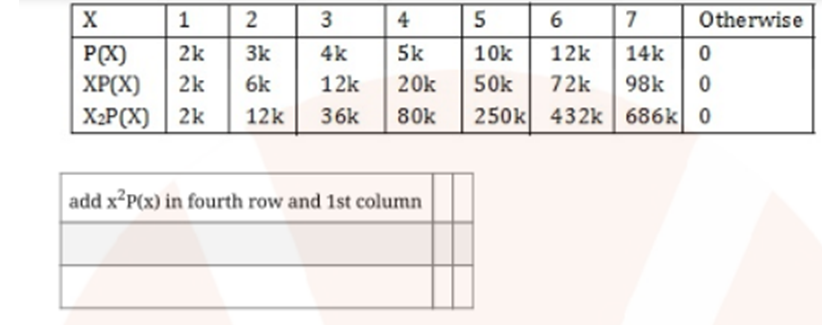
Find the mean of the probability distribution.

Given $$P(X=x)=\begin{cases} K\quad ,\quad x=0\quad or\quad 1 \\ 2Kx\quad ,\quad x=2 \\ K(5-x)\quad ,\quad x=3\quad or\quad 4 \end{cases}$$
Mean
$$=0.P(X=0)+1.P(X=1)+2.P(X=2)+3.P(X=3)+4.P(X=4)\\ \Rightarrow 1.K.1+2.2K.2+3.K(5-3)+4.K(5-4)\\ \Rightarrow 0+K+8K+6K+4K\\ \Rightarrow 19K\\ \mu =19K$$


Find k, the probability distribution of X is as follows?
X1234
P(X)2k3k3k2k

$$\because\sum{X.P(X)}=1$$

$$1(2k)+2(3k)+3(3k)+4(2k)=1$$

$$2k+6k+9k+8k=1$$

$$25k=1$$

$$\therefore k=\dfrac{1}{25}$$


Calculate the expected value and variance of $$x$$, if $$x$$ denotes the number obtained on the uppermost face when a fair die is thrown.

Let $$x$$ denote the number on the uppermost face of the dice.
And the probability of any number on the uppermost face is $$1/6$$
when $$x=1\quad P(1)=1/6$$
          $$x=2\quad P(2)=1/6$$
          $$x=3\quad P(3)=1/6$$
          $$x=4\quad P(4)=1/6$$
          $$x=5\quad P(5)=1/6$$
          $$x=6\quad P(6)=1/6$$
Hence adding all we get mean as $$6/6$$


Events A and B are such that $$P\left( A \right) = \frac{1}{2},P\left( B \right) = \frac{1}{{12}}$$ and $$P\left( {notA\, \text{OR} \, not\,B} \right) = \frac{1}{4}$$ state whether A and B are independent?

P(A'UB')=1/4=1-P(A$$\cap $$B)
P(A$$\cap $$B)=3/4
P(A)*P(B)=1/24 not equals toP(A$$ \cap$$ B)
A and B are not independent


A r.v. $$X$$ has the following probability distribution:
$$X=x$$$$-2$$$$-1$$$$0$$$$1$$$$2$$$$3$$
$$P(x)$$$$0.1$$$$k$$$$0.2$$$$2k$$$$0.3$$$$k$$
Find the value of $$k$$ and calculate mean and variance of $$X$$.

 $$x=x$$$$-2$$$$-1$$ $$0$$ $$1$$ $$2$$ $$3$$ 
 $$P(x)$$$$0.1$$ $$k$$ $$0.2$$ $$2k$$ $$0.3$$ $$k$$ 
Since sum of probabilities $$=1$$
$$0.1+k+0.2+2k+0.3+k=1$$
$$4k=1-0.6$$
$$k=0.1$$
Mean $$=(-2)0.1+(-1)k+(0.2)(o)+1(2k)+2(0.3)+3(k)$$
$$=4k+0.4$$
$$=4(0.1)+0.4$$
$$(\mu)=0.8$$
Variance $$\Rightarrow (6^{2})=\displaystyle\sum_{i=1}^{n}x^{2}(p(x=xi)-\mu i$$
$$6^{2}=4(0.1)+1(k)+0(0.2)+1(2k)+4(0.3)+9k-\mu^{2}$$
$$=12(0.1)+1.6-(0.8)^{2}$$
$$=1.2+1.6-0.64$$
$$6^{2}\Rightarrow 2.16$$


 X 1 2 3 4 5 6
 P(X=x) k 2k 3k 4k 5k 6k
The probability distribution of discrete r.v. $$X$$ is 
Then $$P(X\le 4)$$ = 

Sum of the probabilities is equal to 1

$$\therefore k+2k+3k+4k+5k+6k=1$$

$$21k=1$$

$$k=\dfrac{1}{21}$$

$$P(X\leq 4)$$

$$=P(1)+P(2)+P(3)+P(4)$$

$$=k+2k+3k+4k$$

$$=\dfrac{1}{21}+\dfrac{2}{21}+\dfrac{3}{21}+\dfrac{4}{21}$$

$$=\dfrac{1+2+3+4}{21}$$

$$=\dfrac{10}{21}$$

$$\therefore P(X\leq 4)=0.476$$


Two number are selected at random (without replacement ) from the first six positive integers. Let X denote the larger of the numbers obtained. Find E (X). 

The first 6 positive integers are $$1,2,3,4,5,6$$.
We can select the two positive numbers in $$6\times 5 =30$$ different ways.
Out of this, 2 numbers are selected at random and let X denote the larger of the two numbers.
Since X is the larger of the two numbers, X can assume the value of $$2,3,4,5$$ or $$6$$.
P(X=2) = P(larger number is 2) = {(1,2), (2,1)} = $$\dfrac{2}{30}$$

P(X=3) = P(larger number is 3) = {(1,3), (3,1), (2,3), (3,2)} = $$\dfrac{4}{30}$$

P(X=4) = P(larger number is 4) =  {(1,4), (4,1), (2,4), (4,2), (3,4), (4,3)} = $$\dfrac{6}{30}$$

P(X=5) = P(larger number is 5) = {(1,5), (5,1), (2,5), (5,2), (3,5), (5,3), (4,5), (5,4)} = $$\dfrac{8}{30}$$

P(X=6) = P(larger number is 6) = {(1,6), (6,1), (2,6), (6,2), (3,6), (6,3), (4,6), (6,4), (5,6), (6,5)} = $$\dfrac{10}{30}$$

The expected value of mean can be calculated as :
Mean = $$\Sigma(X_{i}\times P(X_{i})) = $$
$$=2\times \dfrac{2}{30} + 3\times \dfrac{4}{30}+4 \times \dfrac{6}{30} +5\times \dfrac{8}{30}+6\times \dfrac{10}{30} = \dfrac{14}{3}$$


The random variable $$X$$ has probability distribution $$P(X)$$ of the following form.
$$P(X)=\begin{cases} k\quad if\quad X=0 \\ 2k\quad if\quad X=1 \\ 3k\quad if\quad X=2 \\ 0\quad otherwise \end{cases}$$
(a) determine value of $$K$$
(b) Find $$P(X< 2), P(X\le 2), P(X\ge 2)$$

$$(a)$$ As Sum of all probabilities should be 
  $$\Rightarrow k+2k+3k=1 \Rightarrow k=1/6$$
$$(b)$$ $$P(x<2)=p(x=0)+p(x=1)=k+2k=3\left(\dfrac{1}{6}\right)=\dfrac{1}{2}$$
  $$p(x \le 2)=\dfrac{1}{2}+3k=\dfrac{1}{2}+\dfrac{3}{6}=\dfrac{1}{2}+\dfrac{1}{2}=1$$
  $$P(x\ge 2)=3k+0=3\left(\dfrac{1}{6}\right)+0=\dfrac{1}{2}$$


The p. m. f. X- number of major defects randomly selected appliance of a certain type is : 
X-x01234
P(a)0.080.150.450.270.05
Find the expected value and standard deviation of X.

 x 0 12 3 4 
 $$ P(\mu )$$ 0.080.15 0.45 0.27 0.05 
expected value .
$$ E(x) = 0\times 0.008+1\times 0.15+2\times 0.45+3\times 0.27+4\times 0.05$$
$$ = 0.15+0.90+0.81+0.20 $$
$$ E(x) = 2.06 = \mu $$
$$ \rightarrow $$ variance of x.
$$ V(x)= \dfrac{E(x-\mu )2}{\sim }$$
$$ = (0-\mu )^{2}0.08+(1-\mu )^{2}\times 0.15+(2-\mu )^{2}\times 0.45$$
$$ +(3-\mu )^{2}\times 0.27+(4-\mu )^{2}\times 0.05 $$
$$ v(x) = 0.9364$$
$$ \rightarrow $$ standard deviation of x 
$$ SD (x) = \sqrt{V(x)}$$
$$ = \sqrt{0.9364}$$
$$ = 0.9677$$


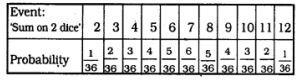
Let a pair of dice be thrown and the random variable $$  X  $$ be the sum of the numbers that appear on the two dice. Find the mean of $$  X  $$ .

$$P(X=2)=P(1,1)=\cfrac{1}{36}$$
$$P(X=3)=P[(1,2),(2,1)]=\cfrac{2}{36}$$
$$P(X=4)=P[)1,3),(2,2),(3,1)]=\cfrac{3}{36}$$
$$P(X=5)=P[(1,4),(2,3),(3,2),(4,1)]=\cfrac{4}{36}$$
$$P(X=6)=P[(1,5),(2,4),(3,3),(4,2),(5,1)]=\cfrac{5}{36}$$
$$P(X=7)=P[(1,6),(2,5),(3,4),(4,3),(5,2),(6,1)]=\cfrac{6}{36}$$
$$P(X=8)=P[(2,6,(3,5),(4,4),(5,3),(6,2)]=\cfrac{5}{36}$$
$$P(X=9)=P[(3,6),(4,5),(5,4),(6,3)]=\cfrac{4}{36}$$
$$P(X=10)=P[(4,6),(5,5),(6,4)]=\cfrac{3}{36}$$
$$P(X=11)=P[(5,6),(6,5)]=\cfrac{2}{36}$$
$$P(X=12)=P[(6,6)]=\cfrac{1}{36}$$
$$X$$$$P=P(X)$$$${p}_{i}{x}_{i}$$
2$$\cfrac { 1 }{ 36 } $$$$\cfrac { 2 }{ 36 } $$
3$$\cfrac { 2 }{ 36 } $$$$\cfrac { 6 }{ 36 } $$
4$$\cfrac { 3 }{ 36 } $$$$\cfrac { 12 }{ 36 } $$
5$$\cfrac { 4 }{ 36 } $$$$\cfrac { 20 }{ 36 } $$
6$$\cfrac { 5 }{ 36 } $$$$\cfrac { 30 }{ 36 } $$
7$$\cfrac { 6 }{ 36 } $$$$\cfrac { 42 }{ 36 } $$
8$$\cfrac { 5 }{ 36 } $$$$\cfrac { 40 }{ 36 } $$
9$$\cfrac { 4 }{ 36 } $$$$\cfrac { 36 }{ 36 } $$
10$$\cfrac { 3 }{ 36 } $$$$\cfrac { 30 }{ 36 } $$
11$$\cfrac { 2 }{ 36 } $$$$\cfrac { 22 }{ 36 } $$
12$$\cfrac { 1 }{ 36 } $$$$\cfrac { 12 }{ 36 } $$
Total      $$1$$                   $$\cfrac { 252 }{ 36 } $$
Mean of $$X==\cfrac { \sum { { p }_{ i }{ x }_{ i } }  }{ \sum { { p }_{ i } }  } =\cfrac { 252 }{ 36\times 1 } =7$$
Mean $$=7$$


12 different objecs are to be distributed among 9 persons. Find the number of ways in which objects can be distributed . (All objects must be distributed and each person should get atleast 1 object)

No. of ways of distributing $$12$$ objects among $$9$$ persons to that each gets atleast one 
$$={ C }_{ 9-1 }^{ 12+9-1 }={ C }_{ 8 }^{ 20 }\\ =\cfrac { 20! }{ 8!\times 12! } =\cfrac { 20\times 19\times 18\times 17\times 16\times 15\times 14\times 13\times 12! }{ 8\times 7\times 6\times 5\times 4\times 3\times 2\times 12! } \\ =19\times 17\times 15\times 13\times 2$$
$$ =125970$$ways


Four red balls are numbered from $$1$$ to $$4$$ and three blue balls are numbered from $$3$$ to $$5$$. If these balls are placed in a bag and a ball is drawn from a bag, what is the probability of choosing a red ball and a blue ball numbered $$5$$?

Given,

4 red balls, 3 blue balls.

Therefore the probability of choosing a red ball $$=\dfrac{1}{4+3}=\dfrac{1}{7}$$

Total of blue balls 3, numbered from 3,4,5.

Therefore the probability of choosing a 5 numbered blue ball $$=\dfrac{1}{1+1+1}=\dfrac{1}{3}$$


The probability distribution of a random variable X is given below
$$X=x_i$$$$P(X=x_i)$$
$$1$$k
$$2$$$$2k$$
$$3$$$$3k$$
$$4$$$$4k$$
$$5$$$$5k$$
Find the value of k and the mean and variance of X.

As we know that
$$\sum{{P}_{i}} = 1$$
$$\therefore k + 2k + 3k + 4k + 5k = 1$$
$$\Rightarrow k = \cfrac{1}{15}$$
Thus the value of $$k$$ is $$\cfrac{1}{15}$$
$$X = {x}_{i}$$ $$P{\left( X = {x}_{i} \right)}$$ $$XP$$ $${X}^{2} P$$ 
$$1$$ $$k$$ $$k$$ $$k$$ 
$$2$$$$2k$$ $$4k$$$$8k$$
$$3$$$$3k$$ $$9k$$$$27k$$
$$4$$$$4k$$$$16k$$$$64k$$
$$5$$$$5k$$$$25k$$$$125k$$
  $$\sum{XP} = 55k = 55 \times \cfrac{1}{15} = 3.66$$$$\sum{{X}^{2} P} = 225 k 225 \times \cfrac{1}{15} = 15$$
Mean of the given data $$\left( \bar{x} \right) = \sum{XP} = 3.66$$
Variance of the given data $$= \sum{{X}^{2}P} - {\bar{x}}^{2} = 15 - {\left( 3.66 \right)}^{2}  = 15 - 13.4 = 1.6$$
Hence the mean and variance of the given data is $$3.66$$ and $$1.6$$ respectively.


Two unbiased dice are thrown together at random. Find the expected value of the total number of points shown up.


Let $$"x"$$ indicate the sum of the points on the two dice

[Since you are required to find the expected value the sum of the scores on the two dice, the variable would represent the sum of the points on the dice]

The sum of the points on the two dice would be

  • $$2$$ if the dice show up $$\{(1,1)\}$$

  • $$3$$ if the dice show up $$\{(1,2), (2,1)\}$$

  • $$4$$ if the dice show up $$\{(1,3), (2,2), (3,1)\}$$

  • $$5$$ if the dice show up $$\{(1,4), (2,3), (3,2), (4,1)\}$$

  • $$6$$ if the dice show up $$\{(1,5), (2,4), (3,3), (4,2), (5,1)\}$$

  • $$7$$ if the dice show up $$\{(1,6), (2,5), (3,4), (4,3), (5,2), (6,1)\}$$

  • $$8$$ if the dice show up $$\{(2,6), (3,5), (4,4), (5,3), (6,2)\}$$

  • $$9$$ if the dice show up $$\{(3,6), (4,5), (5,4), (6,3)\}$$

  • $$10$$ if the dice show up $$\{(4,6), (5,5), (6,4)\}$$

  • $$11$$ if the dice show up $$\{(5,6), (6,5)\}$$

  • $$12$$ if the dice show up $$\{(6,6)\}$$

⇒ The values carried by the variable ("x") would be either $$1, 2, 3, ... , 12$$

⇒ "X" is a discrete random variable with range $$= \{1, 2, 3, ... , 12\}$$

$$"X"$$ represents the random variable and $$P(X = x)$$ represents the probability that the value within the range of the random variable is a specified value of $$"x"$$

In the experiment of tossing $$2$$ dice

Total no. of possible choices

=

$$6 × 6$$


=

$$36$$


$$x$$$$2$$$$3$$$$4$$$$5$$$$6$$$$7$$$$8$$$$9$$$$10$$$$11$$
$$12$$

$$p(X = x)$$
$$\dfrac{1}{36}$$

$$\dfrac{2}{36}$$$$\dfrac{3}{36}$$$$\dfrac{4}{36}$$$$\dfrac{5}{36}$$$$\dfrac{6}{36}$$$$\dfrac{5}{36}$$$$\dfrac{4}{36}$$$$\dfrac{3}{36}$$$$\dfrac{2}{36}$$$$\dfrac{1}{36}$$
$$E(x) = \Sigma px=\dfrac{252}{36}$$  (or)  $$7$$

Detailed calculation for mean and variance table is shown above:

Variance of the sum of the points on the two dice
⇒ var (x)=E (x2) − (E(x))2
⇒ var (x)=Σ px2 − (Σ px)2
=54.83 − (7)2
=54.83 − 49
=5.83
Standard Deviation of the sum of the points on the dice
$$⇒ SD (x)=+ \sqrt {Var (x)}$$
$$=+\sqrt \{5.83\}$$
$$=+2.412$$


1198706_1514408_ans_3add4c3aeb3c446cb7a443a1be67c793.PNG


Two cards are drawn (without replacement) from a well shuffled deck of $$52$$ cards. Find probability distribution and mean of number of cards numbered $$4$$

Total no. of cards in the pack $$= 52$$
The number of cards numbered $$4$$ in the pack of cards $$= 4$$
Let $$X$$ be the discrete random variable denoting the number of cards numbered $$4$$ when two cards are drawn without replacement. 
$$X$$ takes the values $$0, 1, 2$$.
Now,
Case I:- Probability of no $$4$$ being drawn
$$P{\left( X = 0 \right)} = \cfrac{{^{48}{C}_{2}}}{{^{52}{C}_{2}}} = \cfrac{188}{221}$$
Case II:- Probability of one $$4$$ being drawn
$$P{\left( X = 1 \right)} = \cfrac{{^{48}{C}_{1}} \times {^{4}{C}_{1}}}{{^{52}{C}_{2}}} = \cfrac{32}{221}$$
Case III:- Probability of two $$4$$ being drawn
$$P{\left( X = 2 \right)} = \cfrac{{^{4}{C}_{2}}}{{^{52}{C}_{2}}} = \cfrac{1}{221}$$
Therefore, the probability distribution is-
$$X$$ $$0$$ $$1$$ $$2$$ 
$$P{\left( x \right)}$$$$\cfrac{188}{221}$$$$\cfrac{32}{221}$$ $$\cfrac{1}{221}$$ 
Now, mean of probability distribution-
Mean $$= \sum{{x}_{i} {P}_{i}} \\ = 0 \times \cfrac{188}{221} + 1 \times \cfrac{32}{221} + 2 \times \cfrac{1}{221} \\ = \cfrac{0 + 32 + 2}{221} = \cfrac{34}{221}$$


Find the probability distribution of the number of doublets in three throws of a pair of dice 

Let $$X$$ denote the number of doublets. Possible doublets are $$\left(1, 1\right), \left(2, 2\right), \left(3, 3\right), \left(4, 4\right), \left(5, 5\right), \left(6, 6\right)$$.

Since, the dice thrown trice, $$\therefore\, X$$ can take the value $$0, 1, 2$$, or $$3$$.
Probability of getting a doublet $$= p = \dfrac{1}{6}$$ and $$q = 1 – \dfrac{1}{6}=\dfrac{5}{6}$$
$$P\left(0\right)=P\left(no\,doublet\right)=qqq=\dfrac{5}{6}\times\dfrac{5}{6}\times\dfrac{5}{6}=\dfrac{125}{216}$$

$$P\left(1\right)=P\left(one\,doublet\right)=pqq+qpq+qqp$$
$$=\dfrac{1}{6}\times\dfrac{5}{6}\times\dfrac{5}{6}+\dfrac{5}{6}\times\dfrac{1}{6}\times\dfrac{5}{6}+\dfrac{5}{6}\times\dfrac{5}{6}\times\dfrac{1}{6}$$
$$=\dfrac{25}{216}+\dfrac{25}{216}+\dfrac{25}{216}=\dfrac{75}{216}$$

$$P\left(2\right)=P\left(two\,doublet\right)=pqq+ppp+qpp$$
$$=\dfrac{1}{6}\times\dfrac{1}{6}\times\dfrac{5}{6}+\dfrac{1}{6}\times\dfrac{5}{6}\times\dfrac{1}{6}+\dfrac{5}{6}\times\dfrac{5}{6}\times\dfrac{1}{6}$$
$$=\dfrac{5}{216}+\dfrac{5}{216}+\dfrac{5}{216}=\dfrac{15}{216}$$

$$P\left(3\right)=P\left(three\,doublet\right)=ppp=\dfrac{1}{6}\times\dfrac{1}{6}\times\dfrac{1}{6}=\dfrac{1}{216}$$

Mean $$\mu=E\left(X\right)=\displaystyle\sum_{i=0}^{3}{P\left({x}_{i}\right){x}_{i}}$$
$$=0\times\dfrac{125}{216}+1\times\dfrac{75}{216}+2\times\dfrac{15}{216}+3\times\dfrac{1}{216}$$
$$=\dfrac{0+75+30+3}{216}=\dfrac{108}{216}=\dfrac{1}{2}$$


Find expected value of the random variable $$X$$ whose probability mass function is :
$$X=x$$123
$$P(X=x)$$$$\dfrac{1}{5}$$$$\dfrac{2}{5}$$$$\dfrac{2}{5}$$

$$\displaystyle E(x)=\sum x_iP(x_i)$$

     $$=1(\dfrac{1}{5})+2(\dfrac{2}{5})+3(\dfrac{2}{5})$$

     $$=\dfrac{1+4+6}{5}$$

     $$=\dfrac{11}{5}$$

$$\boxed{\therefore E(x)=\dfrac{11}{5}}$$


If $$f(x)=kx,0 < x < 2$$
             $$=0$$, otherwise,

is a probability density function of a random variable $$X$$, then find:
(i) Value of $$k$$,
(ii) $$P(1 < X< 2)$$

$$\because$$ the value of a probability density function over the whole range is equal to $$'1'$$

i) $$\displaystyle \therefore \int^2_0 f(x)dx=\int^2_0 kx dx=1$$

                   $$\Rightarrow K\left[\dfrac{x^2}{2}\right]^2_0=1$$

                   $$\Rightarrow k\left[\dfrac{4}{2}-0\right]=1$$

                   $$\Rightarrow k\times 2=1$$

                   $$\Rightarrow k=\dfrac{1}{2}$$

ii) $$P(1< x<2)$$

                  $$\displaystyle \int^2_1 f(x) dx=\int^2_1\dfrac{1}{2}x dx $$ Putting value of $$k=\dfrac{1}{2}$$

                 $$=\dfrac{1}{2}\left[\dfrac{x^2}{2}\right]^2_1$$

                 $$=\dfrac{1}{2}\left[\dfrac{4}{2}-\dfrac{1}{2}\right]$$

                 $$=\dfrac{1}{2}\times \dfrac{3}{2}=\dfrac{3}{4}$$

$$\therefore \boxed{P(1< x< 2)=\dfrac{3}{4}}$$


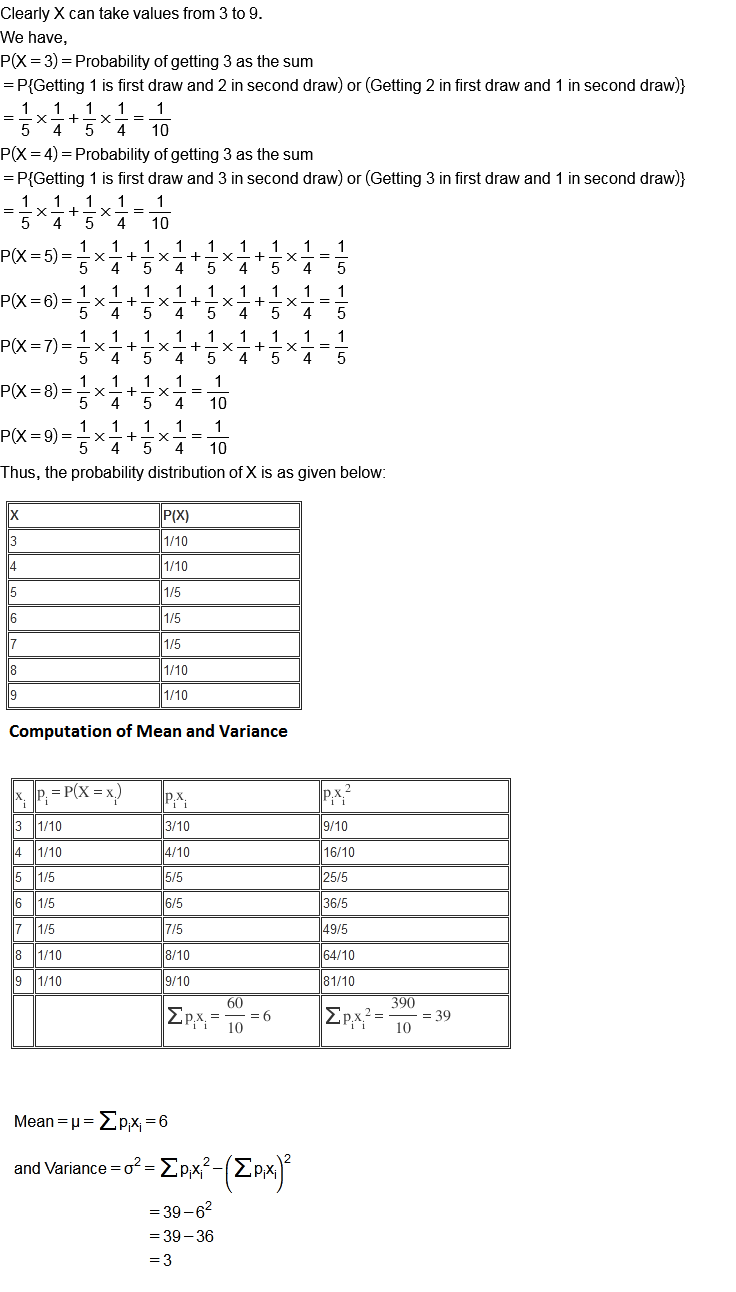
There are 5 cards , numbered 1 to 5, one number of each card . two cards are drawn at random without replacement . Let X denote the sum of the numbers on the two cards drawn.find the mean and variance of X.


1569607_1708260_ans_9344c1864efe43ee9d28a6f089f601b8.png


The probability distribution of $$X$$ is :
    $$ X$$   $$0$$$$1$$$$2$$$$3$$
  $$P(X)$$ $$0.2$$$$ K$$ $$ K $$$$2K$$ 
 
Write the value of $$ K $$ .

We have , 

$$ SP(X) = 1 $$    $$ \Rightarrow \,\, 0.2  + 4K = 1 $$  where $$SP$$ is the sum of probabilities

$$ \Rightarrow \,\, 4K = 0.8  \,\,\, \Rightarrow $$        $$ K = 0.2 $$


Consider the probability distribution of a random variable $$ x $$ :
$$\begin{array}{|l|l|l|l|l|l|}\hline  \mathrm{X}  & 0 & 1 & 2 & 3 & 4 \\\hline  \mathrm{P}(\mathrm{X})  & 0.1 & 0.25 & 0.3 & 0.2 & 0.15 \\\hline\end{array}$$
Calculate
(ii) Variance of $$ \mathrm{X} $$

we have,
$$\begin{array}{|l|l|l|l|l|l|}\hline  \mathrm{X}  & 0 & 1 & 2 & 3 & 4 \\\hline  \mathrm{P}(\mathrm{X})  & 0.1 & 0.25 & 0.3 & 0.2 & 0.15 \\\mathrm{XP(X)}&0&0.25&0.6&0.6&0.60 \\\hline \mathrm{X^2P(X)}&0&0.25&1.2&1.8&2.40 \\\hline\end{array}$$

$$ \operatorname{Var}(X)=E\left(X^{2}\right)-[E(X)]^{2} $$

Where, $$ E(X)=\mu=\sum_{i=1}^{n} x_{i} P_{i}(x i) $$

And $$ E\left(X^{2}\right)=\sum_{i=1}^{n} x_{i}^{2} P\left(x_{i}\right) $$

$$ \therefore E(X)=0+0.25+0.6+0.6+0.60=2.05 $$

$$ E\left(X^{2}\right)=0+0.25+1.2+1.8+2.40=5.65 $$

(ii) $$ \mathrm{V}(\mathrm{X})=1.44475 $$


A biased die is such that $$ \mathrm{P}(4)=\dfrac{1}{10} $$ and other scores being equally likely. The die is tossed twice. If $$ X $$ is the 'number of four seen', find the variance of the random variable $$ \mathbf{X} $$

since, $$ \mathrm{X}= $$ number of four seenOn tossing two die, $$ \mathrm{X}=0,1,2 $$

Also, $$ P_{(4)}=\dfrac{1}{10} $$ and $$ P_{(not 4)}=\dfrac{9}{10} $$
$$ \mathrm{So} $$

$$ P(X=0)=P_{(not 4)} \cdot P_{(not 4)}=\dfrac{9}{10} \cdot \dfrac{9}{10}=\dfrac{81}{100} $$

$$ P(X=1)=P_{(not 4)}, P_{(4)}+P_{(4)} \cdot P_{(not4)}=\dfrac{9}{10} \cdot \dfrac{1}{10}+\dfrac{1}{10} \cdot \dfrac{9}{10}=\dfrac{18}{100} $$

$$ P(X=2)=P_{(4)} \cdot P_{(4)}=\dfrac{1}{10} \cdot \dfrac{1}{10}=\dfrac{1}{100} $$
Thus, we get following table

$$\begin{array}{|l|l|l|l|}\hline \mathrm{X} & 0 & 1 & 2 \\\hline \mathrm{P}(\mathrm{X}) & \dfrac{81}{100} & \dfrac{18}{100} & \dfrac{1}{100} \\\hline \mathrm{XP}(\mathrm{X}) & 0 & 18 / 100 & 2 / 100 \\\hline \mathrm{X}^{2} \mathrm{P}(\mathrm{X}) & 0 & 18 / 100 & 4 / 100 \\\hline\end{array}$$

$$ \therefore \operatorname{Var}(X)=E\left(X^{2}\right)-[E(X)]^{2}=\sum X^{2} P(X)-\left[\sum X P(X)\right]^{2} $$ 

$$ =\left[0+\dfrac{18}{100}+\dfrac{4}{100}\right]-\left[0+\dfrac{18}{100}+\dfrac{2}{100}\right]^{2} $$

$$ =\dfrac{22}{100}-\left(\dfrac{20}{100}\right)^{2}=\dfrac{11}{50}-\dfrac{1}{25} $$
 
$$ =\dfrac{11-2}{50}=\dfrac{9}{50}=\dfrac{18}{100}=0.18 $$


The probability distribution of a random variable X is given below:
$$\begin{array}{|l|l|l|l|l|}\hline \mathrm{X} & 0 & 1 & 2 & 3 \\\hline \mathrm{P}(\mathrm{X}) &  k  & \dfrac{k}{2} & \dfrac{k}{4} & \dfrac{k}{8} \\\hline\end{array}$$
(i) Determine the value of $$ \mathbf{k} $$

We have,
$$\begin{array}{|l|l|l|l|l|}\hline \mathrm{X} & 0 & 1 & 2 & 3 \\\hline \mathrm{P}(\mathrm{X}) &  k  & \dfrac{k}{2} & \dfrac{k}{4} & \dfrac{k}{8} \\\hline\end{array}$$
(i) since, $$\displaystyle \sum_{i=1}^{n} P_{i}=1, i=1,2, \ldots, n $$ and $$ p_{i} \geq 0 $$
$$ \therefore k+\dfrac{k}{2}+\dfrac{k}{4}+\dfrac{k}{8}=1 $$
$$\Rightarrow 8 k+4 k+2 k+k=8$$
$$ k=\dfrac{8}{15} $$


If $$ \mathrm{X} $$ is the number of tails in three tosses of a coin, determine the standard deviation of $$ x $$

Given that, random variable $$ X $$ is the number of tails in three tosses of a coin.

$$ \mathrm{So}, X=0,1,2,3 $$

$$\Rightarrow P(X-x)=^{n} C_{x}(p)^{x} q^{n-x} \Rightarrow P(X=r)=^{n} C_{r}(p)^{r}(q)^{n-r}$$

Where $$ n=3, p=1 / 2, q=1 / 2 $$ and $$ x=0,1,2,3 n=3, p=\dfrac{1}{2}, q=\dfrac{1}{2}, r=0,1,2,3 $$

$$\begin{array}{|l|l|l|l|l|}\hline \mathrm{X} &0&1&2&3 \\\hline \mathrm{P(X)}&\dfrac{1}{8}&\dfrac{3}{8}&\dfrac{3}{8}&\dfrac{1}{8} \\\hline \mathrm{XP(X)}&0&\dfrac{3}{8}&\dfrac{3}{4}&\dfrac{3}{8}\\\hline \mathrm{X^2P(X)}&0&\dfrac{3}{8}&\dfrac{3}{2}&\dfrac{9}{8} \\\hline\end{array}$$

We know that, $$ \operatorname{Var}(X)=E\left(X^{2}\right)-[E(X)]^{2} $$ Eq.... $$ (\mathrm{i}) $$

Where, $$ E\left(X^{2}\right)=\sum_{i=1}^{n} x_{i}^{2} P\left(x_{i}\right) $$ and $$ E(X)=\sum_{i=1}^{n} x_{i} P\left(x_{i}\right) $$

$$ \therefore E\left(X^{2}\right)=\sum_{i=1}^{n} x_{i}^{2} P\left(X_{i}\right)=0+\dfrac{3}{8}+\dfrac{3}{2}+\dfrac{9}{8}=\dfrac{24}{8}=3 $$

And $$ [E(X)]^{2}=\left[\sum_{i=1}^{n} x_{i}^{2} P\left(x_{i}\right)\right]^{2}=\left[0+\dfrac{3}{8}+\dfrac{3}{4}+\dfrac{3}{8}\right]^{2}=\left[\dfrac{12}{8}\right]^{2}=\dfrac{9}{4} $$

$$ \therefore \operatorname{Var}(X)=3-\dfrac{9}{4}=\dfrac{3}{4}[\text { using Eq. }(\mathrm{i})] $$
And standard deviation of $$ X=\sqrt{\operatorname{Var}(X)}=\sqrt{\dfrac{3}{4}}=\dfrac{\sqrt{3}}{2} $$


Consider the probability distribution of a random variable $$ x $$ :
$$\begin{array}{|l|l|l|l|l|l|}\hline  \mathrm{X}  & 0 & 1 & 2 & 3 & 4 \\\hline  \mathrm{P}(\mathrm{X})  & 0.1 & 0.25 & 0.3 & 0.2 & 0.15 \\\hline\end{array}$$
Calculate:
(i) $$ \mathrm{V}\left(\dfrac{\mathrm{X}}{2}\right) $$

we have,
$$\begin{array}{|l|l|l|l|l|l|}\hline  \mathrm{X}  & 0 & 1 & 2 & 3 & 4 \\\hline  \mathrm{P}(\mathrm{X})  & 0.1 & 0.25 & 0.3 & 0.2 & 0.15 \\\mathrm{XP(X)}&0&0.25&0.6&0.6&0.60 \\\hline \mathrm{X^2P(X)}&0&0.25&1.2&1.8&2.40 \\\hline\end{array}$$

$$ \operatorname{Var}(X)=E\left(X^{2}\right)-[E(X)]^{2} $$

Where, $$\displaystyle E(X)=\mu=\sum_{i=1}^{n} x_{i} P_{i}(x i) $$

And $$ E\left(X^{2}\right)=\sum_{i=1}^{n} x_{i}^{2} P\left(x_{i}\right) $$

$$ \therefore E(X)=0+0.25+0.6+0.6+0.60=2.05 $$

$$ E\left(X^{2}\right)=0+0.25+1.2+1.8+2.40=5.65 $$

(i) $$ V\left(\dfrac{X}{2}\right)=\dfrac{1}{4} V(X)=\dfrac{1}{4}\left[5.65-(2.05)^{2}\right] $$

$$ \dfrac{1}{4}[5.65-4.2025]=\dfrac{1}{4} \times 1.4475=0.361875 $$


A discrete random variable X has the probability distribution given as below:
$$\begin{array}{|l|ll|l|l|}\hline \mathrm{X} &0.5 & 1 & 1.5 & 2 \\\hline \mathrm{P(X)}& k & k^2 & 2k^2 & k \\\hline\end{array}$$
(ii) Determine the mean of distribution.

We have,
$$\begin{array}{|l|ll|l|l|}\hline \mathrm{X} &0.5 & 1 & 1.5 & 2 \\\hline \mathrm{P(X)}& k & k^2 & 2k^2 & k \\\hline\end{array}$$
 We know that, $$\displaystyle \sum_{i=1}^{n} P_{i}=1 $$, where $$ P_{i} \geq 0 $$
$$ \Rightarrow P_{1}+P_{2}+P_{3}+P_{4}=1 $$
$$ \Rightarrow k+k^{2}+2 k^{2}+k=1 $$
$$ \Rightarrow 3 k^{2}+2 k-1=0 $$
$$ \Rightarrow 3 k^{2}+3 k-k-1=0 $$
$$ \Rightarrow 3 k(k+1)-1(k+1)=0 $$
$$ \Rightarrow(3 k-1)(k+1)=0 $$
$$ \Rightarrow k=1 / 3 \Rightarrow k=-1 $$
$$ \mathrm{k}=-1 $$ not possible because $$ k $$ is $$ \geq 0 \Rightarrow \mathrm{k}=1 / 3 $$

 Mean of the distribution $$ (\mu)=E(X)=\sum_{i=1}^{n} x_{i} p_{i} $$
$$ =0.5(k)+1\left(k^{2}\right)+1.5\left(2 k^{2}\right)+2 k=4 k^{2}+2.5 k $$
$$ =4 \cdot \dfrac{1}{9}+2.5 \cdot \dfrac{1}{3}\left[\because k=\dfrac{1}{3}\right] $$
$$ =\dfrac{4+7.5}{9}=\dfrac{23}{18} $$


A die is thrown three times. Let $$ x $$ be 'the number of two seen'. Find the expectation of $$ x $$

We have, $$ X= $$ number of two seen

So, on throwing a die three times, we will have $$ X=0,1,2,3 $$

$$ \therefore P(X=0)=P_{(\operatorname{not} 2)} \cdot P_{(\operatorname{not} 2)} \cdot P_{(\operatorname{not} 2)}=\dfrac{5}{6} \cdot \dfrac{5}{6} \cdot \dfrac{5}{6}=\dfrac{125}{216} $$

$$ P(X=1)=P_{(\operatorname{not} 2)} \cdot P_{(\operatorname{not} 2)} \cdot P_{(2)}+P_{(\operatorname{not} 2)} \cdot P_{(2)} \cdot P_{(\operatorname{not} 2)}+P_{(2)} \cdot P_{(\operatorname{not} 2)} \cdot P_{(\operatorname{not} 2)} $$

$$ =\dfrac{5}{6} \cdot \dfrac{5}{6} \cdot \dfrac{1}{6}+\dfrac{5}{6} \cdot \dfrac{1}{6} \cdot \dfrac{5}{6}+\dfrac{1}{6} \cdot \dfrac{5}{6} \cdot \dfrac{5}{6}=\dfrac{25}{36} \cdot \dfrac{3}{6}=\dfrac{25}{72} $$

$$ P(X=2)=P_{(\operatorname{not} 2)} \cdot P_{(2)} \cdot P_{(2)}+P_{(2)} \cdot P_{(2)} \cdot P_{(\operatorname{not} 2)}+P_{(2)} \cdot P_{(\operatorname{not} 2)} \cdot P_{(2)} $$

$$ =\dfrac{5}{6} \cdot \dfrac{1}{6} \cdot \dfrac{1}{6}+\dfrac{1}{6} \cdot \dfrac{1}{6} \cdot \dfrac{5}{6}+\dfrac{1}{6} \cdot \dfrac{5}{6} \cdot \dfrac{1}{6} $$

$$ =\dfrac{1}{36} \cdot\left[\dfrac{15}{6}\right]=\dfrac{15}{216} $$

and $$ P(X=3)=P_{(2)} \cdot P_{(2)} \cdot P_{(2)}=\dfrac{1}{6} \cdot \dfrac{1}{6} \cdot \dfrac{1}{6}=\dfrac{1}{216} $$

We know that, $$ E(X)=\sum X P(X)=0 \cdot \dfrac{125}{216}+1 \cdot \dfrac{25}{216}+3 \cdot \dfrac{1}{216} $$

$$ =\dfrac{75+30+3}{216}=\dfrac{108}{216}=\dfrac{1}{2} $$


Two cards are drawn successively without replacement from a well shuffled deck of cards. Find the mean and standard variation of the random variable X where X is the number of aces.

Let $$E_1 =$$ Event that first ball being red

And $$E_2 =$$ Event that exactly two of three balls being red

$$\therefore P(E_1) = P_R \cdot P_{R} \cdot P_{R} + P_{R} \cdot P_{R} \cdot P_{\bar R} + P_{R} \cdot  P_{\bar R} \cdot P_R + P_{R} \cdot P_{\bar R} \cdot P_{\bar R}$$

$$= \dfrac{5}{8} \cdot \dfrac{4}{7} \cdot \dfrac{3}{6} + \dfrac{5}{8} \cdot \dfrac{4}{7} \cdot \dfrac{3}{6} + \dfrac{5}{8} \cdot \dfrac{3}{7} \cdot \dfrac{4}{6} + \dfrac{5}{8} \cdot \dfrac{3}{7} \cdot \dfrac{2}{6}$$ 

$$\dfrac{60 + 60 + 60 + 30}{336} = \dfrac{210}{336}$$

$$P (E_1 \cap E_2) = P_R \cdot P_{\bar R} \cdot P_R + P_R \cdot P_R \cdot P_{\bar R}$$

$$= \dfrac{5}{8} \cdot \dfrac{3}{7} \cdot \dfrac{4}{6} + \dfrac{5}{8} \cdot \dfrac{4}{7} \cdot \dfrac{3}{6} = \dfrac{120}{336}$$

$$\therefore P(E_2 / E_1) = \dfrac{P(E_1 \cap E_2)}{P(E_1)} = \dfrac{120/336}{210/336} = \dfrac{4}{7}$$



1781158_1802795_ans_010246d7428f49489b3c65a32c68f1c6.PNG


Find the variance of the distribution:Vedantu
$$\begin{array}{|l|l|l|l|l|l|l|}\hline \mathrm{x} & 0 & 1 & 2 & 3 & 4 & 5 \\\hline \mathrm{P}(\mathrm{x}) & \dfrac{1}{6} & \dfrac{5}{18} & \dfrac{2}{9} & \dfrac{1}{6} & \dfrac{1}{9}  &  \dfrac{1}{18}  \\\hline\end{array}$$

We have,
$$ \begin{array}{|l|l|l|l|l|l|l|} \hline  \mathrm{X}  & 0 & 1 & 2 & 3 & 4 & 5 \\\hline  \mathrm{P}(\mathrm{X})  &  \dfrac{1}{6}  &  \dfrac{5}{18}  &  \dfrac{2}{9}  &  \dfrac{1}{6}  &  \dfrac{1}{9}  &  \dfrac{1}{18}  \\\hline  \mathrm{XP}(\mathrm{X})  & 0 &  \dfrac{5}{18}  &  \dfrac{4}{9}  &  \dfrac{1}{2}  &  \dfrac{4}{9}  &  \dfrac{5}{18}  \\\hline  \mathrm{X}^{2} \mathrm{P}(\mathrm{X})  & 0 &  \dfrac{5}{18}  &  \dfrac{8}{9}  &  \dfrac{3}{2}  &  \dfrac{16}{9}  &  \dfrac{25}{18}  \\\hline \end{array}$$
$$ \therefore $$ Variance $$ =E\left(X^{2}\right)-[E(X)]^{2}=\sum X^{2} P(X)-\left[\sum X P(X)\right]^{2} $$
$$ =\left[0+\dfrac{5}{18}+\dfrac{8}{9}+\dfrac{3}{2}+\dfrac{16}{9}+\dfrac{25}{18}\right]-\left[0+\dfrac{5}{18}+\dfrac{4}{9}+\dfrac{1}{2}+\dfrac{4}{9}+\dfrac{5}{18}\right]^{2} $$ 
$$ =\left[\dfrac{5+16+27+32+25}{18}\right]-\left[\dfrac{5+8+9+8+5}{18}\right]^{-2} $$ 
$$ =\dfrac{105}{18}-\dfrac{35 \cdot 35}{18 \cdot 18}=\dfrac{18 \cdot 105-35 \cdot 35}{18 \cdot 18} $$
$$ =\dfrac{35}{18 \cdot 18}[54-35]=\dfrac{19 \cdot 35}{324}=\dfrac{665}{324} $$


Two probability distributions of the discrete random variable $$ X $$ and $$ Y $$ are given below.
$$\begin{array}{|l|l|l|l|l|}\hline \mathrm{X} & 0 & 1 & 2 & 3 \\\hline \mathrm{P}(\mathrm{X}) & \dfrac{1}{5} & \dfrac{2}{5} & \dfrac{1}{5} & \dfrac{1}{5} \\\hline\end{array}$$
$$ \begin{array}{|l|l|l|l|l|}\hline \mathrm{Y} & 0 & 1 & 2 & 3 \\\hline \mathrm{P}(\mathrm{Y}) & \dfrac{1}{5} & \dfrac{3}{10} & \dfrac{2}{5} & \dfrac{1}{5} \\\hline\end{array}$$
Prove that $$ E\left(Y^{2}\right)=2 E(X) $$

$$\begin{array}{|l|l|l|l|l|}\hline \mathrm{X} & 0 & 1 & 2 & 3 \\\hline \mathrm{P}(\mathrm{X}) & \dfrac{1}{5} & \dfrac{2}{5} & \dfrac{1}{5} & \dfrac{1}{5} \\\hline\end{array}$$
$$ \begin{array}{|l|l|l|l|l|}\hline \mathrm{Y} & 0 & 1 & 2 & 3 \\\hline \mathrm{P}(\mathrm{Y}) & \dfrac{1}{5} & \dfrac{3}{10} & \dfrac{2}{5} & \dfrac{1}{5} \\\hline\end{array}$$
since, we have to prove that, $$ E\left(Y^{2}\right)=2 E(X) $$
$$ \therefore E(X)=\sum X P(X) $$
$$ =0 \cdot \dfrac{1}{5}+1 \cdot \dfrac{2}{5}+2 \cdot \dfrac{1}{5}+3 \cdot \dfrac{1}{5}=\dfrac{7}{5} $$
$$ \Rightarrow 2 E(X)=\dfrac{14}{5} \ldots(i) $$
$$ E(Y)^{2}=\sum Y^{2} P(Y) $$
$$ =0 \cdot \dfrac{1}{5}+1 \cdot \dfrac{3}{10}+4 \cdot \dfrac{2}{5}+9 \cdot \dfrac{1}{10} $$
$$ =\dfrac{3}{10}+\dfrac{8}{5}+\dfrac{9}{10}=\dfrac{28}{10}=\dfrac{14}{5} $$
$$ \Rightarrow E(Y)^{2}=\dfrac{14}{5} $$From Eqs. (i) and (ii),
$$ E(Y)^{2}=2 E(X) $$ Hence proved.


The probability distribution of a discrete random variable X is given as under:
Calculate :
(i) The value of A if E(X) = 2.94
(ii) Variance of X.
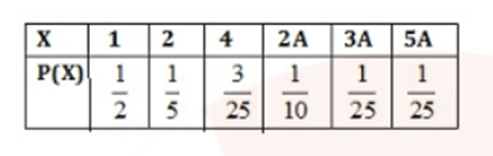
1802225_85738c65452e454ba04e63e77d0bdb5f.PNG

(i) We have, $$\sum XP(X) = \dfrac{1} {2} +  \dfrac{2} {5} +  \dfrac{12} {25} +  \dfrac{2A} {10} +  \dfrac{3A} {25} +  \dfrac{5A} {25}$$

$$ = \dfrac{25 + 20 + 24 + 10A + 6A + 10A} {50} = \dfrac{69 + 26A} {50}$$
Since, $$E(X) = \sum XP(X)$$

$$\Rightarrow 2.94 = \dfrac{69 + 26A} {50}$$

$$\Rightarrow 26 A = 50 \times 2.94 - 69$$

$$\Rightarrow A = \dfrac{147 - 69} {26} = \dfrac{78} {26} = 3$$

(ii) We know that,
$$Var(X) = E(X)^{2} - [E(X)]^{2}$$

$$\sum X^{2}P(X) - [\sum XP(X)]^{2}$$

$$=  \dfrac{1} {2} +  \dfrac{4} {5} +  \dfrac{48} {25} +  \dfrac{4A^{2}} {10} +  \dfrac{9A^{2}} {25} +  \dfrac{25A^{2}} {25} - [E(X)]^{2}$$

$$ = \dfrac{25 + 40 + 96 + 20A^{2} + 18A^{2} + 50A^{2}} {50}  - [E(X)]^{2}$$

$$ = \dfrac{161 + 88 A^{2}} {50} - [E(X)]^{2} = \dfrac{161 + 88 (3)^{2}} {50} - [E(X)]^{2} [\therefore A = 3]$$

$$\dfrac{953} {50} - [2.94]^{2} [\therefore E(X) = 2.94]$$

$$= 19.0600 - 8.6436 = 10.4164$$



Find the probability distribution of the maximum of the two scores obtained when die is thrown twice. Determine also the mean of the distribution

Let X is the random variable score obtained when a die is thrown twice.
$$\begin{array}{l}\therefore \mathrm{x}=1,2,3,4,5,6 \\\text { Here, } S=\{(1,1),(1,2),(2,1),(2,2),(1,3),(2,3),(3,1),(3,2),(3,3), \ldots,(6,6)\}\end{array}$$
$$ P(X=1)=\dfrac{1}{6} \cdot \dfrac{1}{6}=\dfrac{1}{36} $$
$$ P(X=2)=\dfrac{1}{6} \cdot \dfrac{1}{6}+\dfrac{1}{6} \cdot \dfrac{1}{6}+\dfrac{1}{6} \cdot \dfrac{1}{6}=\dfrac{3}{36} $$ 
$$ P(X=3)=\dfrac{1}{6} \cdot \dfrac{1}{6}+\dfrac{1}{6} \cdot \dfrac{1}{6}+\dfrac{1}{6} \cdot \dfrac{1}{6}+\dfrac{1}{6} \cdot \dfrac{1}{6}+\dfrac{1}{6} \cdot \dfrac{1}{6}=\dfrac{5}{36} $$
similarly$$ P(X=4)=\dfrac{7}{36} $$
$$P(X=5)=\dfrac{9}{36}$$
$$ P(X=6)=\dfrac{11}{36} $$
So, the required distribution is
$$\begin{array}{|l|l|l|l|l|l|l|}\hline \mathrm{X} & 1 & 2 & 3 & 4 & 5 & 6 \\\hline  \mathrm{P}(\mathrm{X})  &  1 / 36  &  3 / 36  &  5 / 36  &  7 / 36  &  9 / 36  &  11 / 36  \\\hline\end{array}$$
Also, we know that, Mean $$ \{E(X)\}=\sum X P(X) $$
$$=\dfrac{1}{36}+\dfrac{6}{36}+\dfrac{15}{36}+\dfrac{28}{36}+\dfrac{45}{36}+\dfrac{66}{36}=\dfrac{161}{36}$$


Let X be a discrete random variable whose probability distribution is defined as follows:
$$P(X = x)$$ =$$ \begin{matrix} k(x + 1) & for \space x = 1, 2, 3, 4 \\ 2kx & for \space x = 5, 6, 7 \\ 0 & otherwise \end{matrix}$$
where k is a constant. Calculate
(i) the value of k
(ii) E(x)
(iii) Standard deviation of X.

$$ \begin{matrix} k(x + 1) & for \space x = 1, 2, 3, 4 \\ 2kx & for \space x = 5, 6, 7 \\ 0 & otherwise \end{matrix}$$
Thus, we have following table

(i) Since, $$\sum P_i = 1$$

$$\Rightarrow K (2 + 3 + 4 + 5 + 10 + 12 + 14) = 1 \Rightarrow K = \frac{1} {50}$$
(ii) $$\therefore E(X) = \sum XP (X)$$

$$\therefore E(X) = 2k + 6k + 12k + 20k + 50k + 72k + 98k + 0 = 260k$$

$$= 260 \times \dfrac{1} {50} = \dfrac{26} {5} = 5.2 \left [ \therefore k = \dfrac{1} {50} \right ]$$ .....(i) 

(iii) We know that,
$$Var(X) = [E(X^{2})] -  [E(X)]^{2} = \sum X^{2} P(X) - [ \sum  \left \{XP(X)  \right \} ]^{2}$$

$$\therefore E(X) =  [2k + 6k + 12k + 20k + 50k + 72k + 98k + 0] - [5.2]^{2}$$ [using Eq. (i)]

$$[1498k] - 27.04 = \left[ 1498 \times \dfrac{1} {50}\right ] - 27.04 \left[ \therefore k = \dfrac{1} {50}\right ] $$

$$= 29.96 - 27.04 = 2.92$$

We know that, standard deviation of $$X = \sqrt{Var(X)} = \sqrt{2.92} = 1.7088 = 1.7 $$ (approx)

1781134_1802139_ans_268dbd30a767494b9b486e07c84fdb53.PNG


State which of the following are not the probability distributions of a random variable. Give reasons for your answer.
$$X$$$$0$$$$1$$$$2$$$$3$$$$4$$
$$P(X)$$$$0.1$$$$0.5$$$$0.2$$$$-0.1$$$$0.3$$


1960761_1860091_ans_7dde8e50c00a4db6b4d09b5d50be3188.gif


State which of the following are not the probability distributions of a random variable. Give reasons for your answer.
$$Y$$$$-1$$$$0$$$$1$$
$$P(Y)$$$$0.6$$$$0.1$$$$0.2$$


1960763_1860096_ans_5e438c0e1af946199d3669aaa3249066.gif


Let X be a random variable taking values $$x_1, x_2,....... x_n$$ with probabilities
$$p_1, p_2,......p_n$$ respectively. Then var(X) = __________.

$$Var(X) = (X^{2}) - [E(X)]^{2}$$
$$= \sum_{i=1}^{n} X^{2}P(X) - \left [\sum_{i=1}^{n} XP(X)  \right ]^{2}$$
$$= \sum P_i x_i - \left (\sum P_i x_i  \right )^{2}$$


State which of the following are not the probability distributions of a random variable. Give reasons for your answer.
$$Z$$$$3$$$$3$$$$1$$$$0$$$$-1$$
$$P(Z)$$$$0.3$$$$0.2$$$$0.4$$$$0.1$$$$0.05$$


1960765_1860104_ans_269eb51a350344cf85c1d505f4612124.gif


State which of the following are not the probability distributions of a random variable. Give reasons for your answer.
$$X$$$$0$$$$1$$$$2$$
$$P(X)$$$$0.4$$$$0.4$$$$0.2$$


1959850_1860079_ans_8c7abd86c7eb4f3886b8b106a6e83bf5.gif


Find the probability distribution of $$X$$, the number of heads in a simultaneous toss of two coins.

Possible values of $$X$$ are $$0,1$$ or $$2$$
Now $$P(X=0)=$$P(No head)
$$=$$P(Tail first time and tail second time)
$$=$$P(Tail first time).P(tail in second time)
$$=\cfrac{1}{2}\times {1}{2}=\cfrac{1}{4}$$
$$=$$P(TH or HT)=P(TH)+P(HT)
$$=\cfrac{1}{4}+\cfrac{1}{4}=\cfrac{1}{2}$$
$$P(x=2)=$$P(2 Head)=P(HH)$$=\cfrac{1}{4}$$
Required is distribution
$$X$$012
$$P(X)$$$$\cfrac { 1 }{ 4 } $$$$\cfrac { 1 }{ 2 } $$$$\cfrac { 1 }{ 4 } $$


Complete the following table:
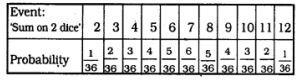
1869364_d4d52d875fa541f39bee8caa5a9f705c.png

Completing the table:
Possibility of throwing 2 dice with $$ \operatorname{six} $$ faces, $$ n(S)=6 \times 6=36 $$
1. Sum on 2 dice will be 3 means (1,2),(2,1)
$$ \therefore \mathrm{n}(\mathrm{A})=2 $$
$$ \therefore P(A) \quad=\dfrac{n(A)}{n(S)}=\dfrac{2}{36} $$
2. Sum on 2 dice, getting 4 means(1,3),(2,2),(3,1)
$$ \therefore n(B)=3 $$
$$ \therefore P(B) \quad=\dfrac{n(B)}{n(S)}=\dfrac{3}{36} $$
3. Sum of 2 dice, getting 5 means (1,4),(2,3),(3,2),(4,1)
$$ \therefore \mathrm{n}(\mathrm{C})=4 $$
$$ \therefore P(C) \quad=\dfrac{n(C)}{n(S)}=\dfrac{4}{36} $$
4. Sum of 2 dice, getting 6 means ( 1, 5), ( 2, 4), (3, 3), (4, 2), (5, 1)
$$ \therefore \mathrm{n}(\mathrm{D})=5 $$
$$ \therefore P(D) \quad=\dfrac{n(D)}{n(S)}=\dfrac{5}{36} $$
5. Sum of 2 dice, getting 7 means ( 1,6 ), (2,5),(3,4),(4,3),(5,2),(6,1) $$ \therefore \mathrm{n}(\mathrm{E})=6 $$
$$ \therefore P(E) \quad=\dfrac{n(E)}{n(S)}=\dfrac{6}{36} $$
6. Sum of 2 dice, getting 9 means (3,6),(4,5),(5,4),(6,3)
$$ \therefore \mathrm{n}(\mathrm{F})=4 $$
$$ \therefore P(F) \quad=\dfrac{n(F)}{n(S)}=\dfrac{4}{36} $$
7. Sum of 2 dice, getting 10 means (4,6),(5,5),(6,4)
$$ \therefore \mathrm{n}(\mathrm{G})=3 $$
$$ \therefore P(G) \quad=\dfrac{n(G)}{n(S)}=\dfrac{3}{36} $$
8. Sum of 2 dice, getting sum 11 means (5,6),(6,5) 
$$ \therefore \mathrm{n}(\mathrm{H})=2 $$
$$ \therefore P(H) \quad=\dfrac{n(H)}{n(S)}=\dfrac{2}{36} $$
$$ \therefore $$ The Table is completed as follows:
1957640_1869364_ans_0d17aecaa6a24a869186b7560f86f27c.png


The random variable X has a probability distribution $$P(X)$$ of the following form, where $$k$$ is some number.
$$P(X = x) = \begin{cases}k,& \text{if} \ x = 0 \\ 2k, & \text{if} \ x=1 \\ 3k, & \text{if} \ x=2 \\ 0, & \text{otherwise} \end{cases}$$ 
Determine the value of $$k$$. 

As Sum of all probabilities should be $$1$$
$$  ⇒k+2k+3k=1⇒k=\dfrac 16$$


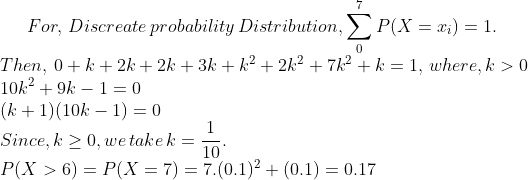
A random variable $$X$$ has the following probability distribution:
$$X$$$$0$$$$1$$$$2$$$$3$$$$4$$$$5$$$$6$$$$7$$
$$P(X)$$$$0$$$$k$$$$2k$$$$2k$$$$3k$$$$k^2$$$$2k^2$$$$7k^2+k$$
Determination
$$P(X>6)$$


1965290_1860171_ans_4a7d44b825584b52b8d4971df38e46c0.gif


A random variable $$X$$ has the following probability distribution:
$$X$$$$0$$$$1$$$$2$$$$3$$$$4$$$$5$$$$6$$$$7$$
$$P(X)$$$$0$$$$k$$$$2k$$$$2k$$$$3k$$$$k^2$$$$2k^2$$$$7k^2+k$$
Determination
$$P(X<3)$$


1965046_1860168_ans_a187032c28be41c7a9cc3d31e4144700.png


A random variable $$X$$ has the following probability distribution:
$$X$$$$0$$$$1$$$$2$$$$3$$$$4$$$$5$$$$6$$$$7$$
$$P(X)$$$$0$$$$k$$$$2k$$$$2k$$$$3k$$$$k^2$$$$2k^2$$$$7k^2+k$$
Determination
$$K$$


1964807_1860164_ans_a9c741db28294f299c26f8c1b22550bd.png


The random variable $$X$$ has a probability distribution $$P(X)$$ of the following from, where $$k$$ is some number:
$$P(X)=\left\{ \begin{matrix} k,\quad if\quad x=0 \\ 2k,\quad if\quad x=1 \\ 3k,\quad if\quad x=2 \\ 0,\quad otherwise \end{matrix} \right .$$
Find $$P(X<2),P(X\le 2),P(X\ge 2)$$


1965314_1860200_ans_b0f3bf83040940d5b4f51b80b9e21aae.gif


A random variable $$X$$ has the following probability distribution:
$$X$$$$0$$$$1$$$$2$$$$3$$$$4$$$$5$$$$6$$$$7$$
$$P(X)$$$$0$$$$k$$$$2k$$$$2k$$$$3k$$$$k^2$$$$2k^2$$$$7k^2+k$$
Determination
$$P(0<X<3)$$


1965293_1860175_ans_d0c4c9f30d0c41e99334012a14c2bb28.gif


A fair coin is tossed until a head or five tails occur. If $$X$$ denotes the number of tosses of the coin, find the mean of $$X$$.

Given $$X$$ denotes the number of coins. Coins is tossed until a head or five tails accur therefere, it is clear if on $$X=1$$, head comes then the process will be stopped and if tail comes then coin will be tossed second time. Clearly it will be repeated again and again till 5 tails come maximum
Then the value of $$X$$ will be $$1,2,3,4,5$$
$$S=\left\{ H,TH,TTH,TTTH\quad or\quad TTTTH,TTTTT \right\} $$
Probability that heat comes in first throw
$$P(X=1)=\cfrac{1}{2}$$
Similarly
$$P(X=2)=P(TH)=P(T)P(H)=\cfrac { 1 }{ 2 } \times \cfrac { 1 }{ 2 } =\cfrac { 1 }{ 4 } $$
$$P(X=3)=P(TTH)=P(T)P(T)P(H)$$
$$=\cfrac { 1 }{ 2 } \times \cfrac { 1 }{ 2 } \times \cfrac { 1 }{ 2 } =\cfrac { 1 }{ 8 } $$
$$P(X=4)=P(TTTH)=P(T)P(T)P(T)P(H)$$
$$=\cfrac { 1 }{ 2 } \times \cfrac { 1 }{ 2 } \times \cfrac { 1 }{ 2 } \times \cfrac { 1 }{ 2 } =\cfrac { 1 }{ 16 } $$
$$P(X=5)=P(TTTTH\cup TTTTT)$$
$$=P(T)P(T)P(T)P(T)P(H)+P(T)P(T)P(T)P(T)P(T)$$
$$=\cfrac { 1 }{ 2 } \times \cfrac { 1 }{ 2 } \times \cfrac { 1 }{ 2 } \times \cfrac { 1 }{ 2 } \times \cfrac { 1 }{ 2 } +\cfrac { 1 }{ 2 } \times \cfrac { 1 }{ 2 } \times \cfrac { 1 }{ 2 } \times \cfrac { 1 }{ 2 } \times \cfrac { 1 }{ 2 } =\cfrac { 1 }{ 32 } +\cfrac { 1 }{ 32 } =\cfrac { 1 }{ 16 } $$
Hence probability distribution will be like this
$$X$$12345
$$P(X)$$$$\cfrac { 1 }{ 2 } $$$$\cfrac { 1 }{ 4 } $$$$\cfrac { 1 }{ 8 } $$$$\cfrac { 1 }{ 16 } $$$$\cfrac { 1 }{ 16 } $$
Mean $$=\sum _{ i=1 }^{ 5 }{ { p }_{ i }{ x }_{ i } } =1\times \cfrac { 1 }{ 2 } +2\times \cfrac { 1 }{ 4 } +3\times \cfrac { 1 }{ 8 } +4\times \cfrac { 1 }{ 16 } +5\times \cfrac { 1 }{ 16 } =\cfrac { 1 }{ 2 } +\cfrac { 1 }{ 2 } +\cfrac { 3 }{ 8 } +\cfrac { 1 }{ 4 } +\cfrac { 5 }{ 16 } $$
$$=\cfrac { 16+16+12+8+5 }{ 32 } =\cfrac { 31 }{ 16 } =1.9$$


A class has 15 students whose ages are 14, 17, 15, 14, 21, 17, 19, 20, 16, 18, 20, 17, 16, 19 and 20 years. One student is selected in such a manner that each has the same chance of being chosen and the age $$X$$ of the selected student is recorded. What is the probability distribution of the random variable $$X$$ ? Find mean, variance and standard deviation of $$X$$.

There are 15 students in the class. Each student has the same chance to be chosen.
Therefore, the probability of each student to be selected is $$\displaystyle\frac{1}{15}$$.
The given information can be compiled in the frequency table as follows.
$$P\left( X=14 \right) =\displaystyle\frac { 2 }{ 15 } , P\left( X=15 \right) =\displaystyle\frac { 1 }{ 15 } , P\left( X=16 \right) =\displaystyle\frac { 2 }{ 15 } , P\left( X=16 \right) =\displaystyle\frac { 3 }{ 15 } , P\left( X=18 \right) =\displaystyle\frac { 1 }{ 15 } , P\left( X=19 \right) = \displaystyle\frac { 2 }{ 15 } , P\left( X=20 \right) =\displaystyle\frac { 3 }{ 15 } , P\left( X=21 \right) =\displaystyle\frac { 1 }{ 15 } $$
Therefore, the probability distribution of random variable $$X$$ is as follows.
Then, mean of $$X = E\left( X \right) $$
$$=\sum { { X }_{ i }P\left( { X }_{ i } \right)  } $$
$$=14\times \displaystyle\frac { 2 }{ 15 } +15\times \displaystyle\frac { 1 }{ 15 } +16\times \displaystyle\frac { 2 }{ 15 } +17\times \displaystyle\frac { 3 }{ 15 } +18\times \displaystyle\frac { 1 }{ 15 } +19\times \displaystyle\frac { 2 }{ 15 } +20\times \displaystyle\frac { 3 }{ 15 } +21\times \displaystyle\frac { 1 }{ 15 } $$
$$=\displaystyle\frac { 1 }{ 15 } \left( 28+15+32+51+18+38+60+21 \right) $$
$$=\displaystyle\frac { 263 }{ 15 } $$
$$=17.53$$
$$E\left( { X }^{ 2 } \right) =\sum { { X }_{ i }^{ 2 }P\left( { X }_{ i } \right)  } $$
$$={ \left( 14 \right)  }^{ 2 }\cdot \displaystyle\frac { 2 }{ 15 } +{ \left( 15 \right)  }^{ 2 }\cdot \displaystyle\frac { 1 }{ 15 } +{ \left( 16 \right)  }^{ 2 }\cdot \displaystyle\frac { 2 }{ 15 } +{ \left( 17 \right)  }^{ 2 }\cdot \displaystyle\frac { 3 }{ 15 } +{ \left( 18 \right)  }^{ 2 }\cdot \displaystyle\frac { 1 }{ 15 } +{ \left( 19 \right)  }^{ 2 }\cdot \displaystyle\frac { 2 }{ 15 } +{ \left( 20 \right)  }^{ 2 }\cdot \displaystyle\frac { 3 }{ 15 } +{ \left( 21 \right)  }^{ 2 }\cdot \displaystyle\frac { 1 }{ 15 } $$
$$=\displaystyle\frac { 1 }{ 15 } \cdot \left( 392+225+512+867+324+722+1200+441 \right) $$
$$=\displaystyle\frac { 4683 }{ 15 } $$
$$=312.2$$
$$\therefore $$ Variance $$\left( X \right) =E\left( { X }^{ 2 } \right) { \left[ E\left( X \right)  \right]  }^{ 2 }$$
$$=312.2-{ \left( \displaystyle\frac { 263 }{ 15 }  \right)  }^{ 2 }$$
$$=312.2-307.4177$$
$$=4.7823$$
$$\approx 4.78$$
Standard deviation $$=\sqrt { Variance\left( X \right)  } $$
$$=\sqrt { 4.78 } $$
$$=2.186$$
$$\approx 2.19$$


In a meeting, 70% of the members favour and 30% oppose a certain proposal. A member is selected at random and we take $$X = 0$$ if he opposed, and $$X = 1$$ if he is in favour. Find $$E\left(X\right)$$ and $$Var\left(X\right)$$.

It is given that $$P\left(X = 0\right) = 30$$% = \displaystyle\frac{30}{100} = 0.3$$
$$P\left(X = 1\right) = 70$$% = \displaystyle\frac{70}{100} = 0.7$$
Therefore, the probability distribution is as follows.
Then, $$E\left( X \right) =\sum { { X }_{ i }P\left( { X }_{ i } \right)  } $$
$$=0\times 0.3+1\times 0.7$$
$$=0.7$$
$$E\left( { X }^{ 2 } \right) =\sum { { X }_{ i }^{ 2 }P\left( { X }_{ i } \right)  } $$
$$={ 0 }^{ 2 }\times 0.3+{ \left( 1 \right)  }^{ 2 }\times 0.7$$
$$=0.7$$
It is known that, $$Var\left( X \right) =E\left( { X }^{ 2 } \right) -{ \left[ E\left( X \right)  \right]  }^{ 2 }$$
$$=0.7-{ \left( 0.7 \right)  }^{ 2 }$$
$$=0.7-0.49$$
$$=0.21$$


A die is thrown, a man $$C$$ gets a prize of Rs. $$5$$ if the die turns up $$1$$ and gets a prize of Rs. $$3$$ if the die turns up $$2,$$ else he gets nothing, A man $$A$$ whose probability of speaking the truth is $$\displaystyle \frac{1}{2}$$ tells $$C$$ that the die has turned up $$1$$ and another man $$B$$ whose probability of speaking the truth is $$\displaystyle \frac{2}{3}$$ tells $$C$$ that the die has turned up $$2.$$ Find the expectation value of $$C.$$

Let $$A$$ and $$B$$ be the events '$$A$$ speaks the truth' and '$$B$$ speaks the truth' respectively, Then $$\displaystyle P\left ( A \right )=\frac{1}{2}$$ and $$P\left ( B \right )=\frac{2}{3}.$$
The experiment consists of three hypothesis
(i) the die turns up $$1$$
(ii) the die turns up $$2$$
(iii) the die turns up $$3, 4, 5$$ or $$6$$
Let these be the events $$E_{1}, E_{2}$$ and $$E_{3}$$ respectively then $$\displaystyle P\left ( E_{1} \right )=P\left ( E_{2} \right )=\frac{1}{6}$$ and $$\displaystyle P\left ( E_{3} \right )=\frac{4}{6}.$$
Let $$E$$ be the event that the statements made by $$A$$ and $$B$$ agree to the same conclusion.
$$\therefore $$ $$\displaystyle P\left ( E/E_{1} \right )=P\left ( A \right ).P\left ( \bar{B} \right )=\frac{1}{2}\times \frac{1}{3}=\frac{1}{6}$$
$$\displaystyle P\left ( E/E_{2} \right )=P\left ( \bar{A} \right ).P\left ( B \right )=\frac{1}{2}\times \frac{2}{3}=\frac{2}{6}$$
$$\displaystyle P\left ( E/E_{3} \right )=P\left ( \bar{A} \right ).P\left ( \bar{B} \right )=\frac{1}{2}\times \frac{1}{3}=\frac{1}{6}$$
$$P\left ( E \right )=P\left ( E_{1} \right )P\left ( E/E_{1} \right )+P\left ( E_{2} \right )P\left ( E/E_{2} \right )+P\left ( E_{3} \right )P\left ( E/E_{3} \right )$$
   $$\displaystyle =\frac{1}{6}.\frac{1}{6}+\frac{1}{6}.\frac{2}{6}+\frac{4}{6}.\frac{1}{6}=\frac{7}{36}$$
Thus $$P\left ( E_{1}/E \right )=\frac{P\left ( E_{1} \right )P\left ( E/E_{1} \right )}{P\left ( E \right )}=\frac{1}{7}$$
$$\displaystyle P\left ( E_{2}/E \right )=\frac{P\left ( E_{2} \right )P\left ( E/E_{2} \right )}{P\left ( E \right )}=\frac{2}{7}$$
$$\displaystyle P\left ( E_{3}/E \right )=\frac{P\left ( E_{3} \right )P\left ( E/E_{3} \right )}{P\left ( E \right )}=\frac{4}{7}$$
$$\therefore $$ expectation of $$\displaystyle C=\frac{1}{7}\times 5+\frac{2}{7}\times 3+0=$$ Rs. $$\displaystyle \frac{11}{7}$$


The numbers are selected at random (without replacement) from the first six positive integers. Let X denote the larger of the two numbers obtained. Find the probability distribution of the random variable X and hence find the means of the distribution.

 Variable (X)
 $$2$$ $$3$$ $$4$$ $$5$$ $$6$$
 Probability P(X)
$$\dfrac{1}{15}$$ $$\dfrac{2}{15}$$ $$\dfrac{3}{15}$$ $$\dfrac{4}{15}$$ $$\dfrac{5}{15}$$
First six numbers are $$1, 2, 3, 4, 5, 6.$$
X is bigger number among two numbers.
If X $$= 2$$ for P(X) $$=$$ probability of event that bigger of the two chosen number is $$2.$$
So, Cases $$= (1, 2)$$
So, $$P(X)=\dfrac{1}{^6C_2}=\dfrac{1}{15}$$---(1)
If X $$= 3 $$
So, favourable cases are $$= (1, 3), (2, 3)$$
$$P(X)=\dfrac{2}{^6C_{2}}=\dfrac{2}{15}$$---(2)
If X $$= 4$$
So, favourable cases are $$= (1, 4), (2, 4), (3, 4)$$
$$P(X)=\dfrac{3}{^6C_{2}}=\dfrac{3}{15}$$---(3)
If X $$= 5$$
So, favourable cases are $$= (1, 5), (2, 5), (3, 5), (4, 5)$$
$$P(X)=\dfrac{4}{^6C_{2}}=\dfrac{4}{15}$$---(4)
If X $$= 6$$
So, favourable cases are $$= (1, 6), (2, 6), (3, 6), (4, 6), (5, 6)$$
$$P(X)=\dfrac{5}{^6C_{2}}=\dfrac{5}{15}$$---(5)
We can put all value of $$P(X),$$
Required mean = $$2.\left ( \dfrac{1}{15} \right )+3.\left ( \dfrac{2}{15} \right )+4.\left ( \dfrac{3}{15} \right )+5.\left ( \dfrac{4}{15} \right )+6.\left ( \dfrac{5}{15} \right )$$
$$=\dfrac{70}{15}\Rightarrow \dfrac{14}{3}$$


Two the numbers are selected at random (without replacement) from first six positive integers. Let X denote the larger of the two numbers obtained. Find the probability distribution of X. Find the mean and variance of this distribution.

The numbers are 1,2,3,4,5,6
Let X be the bigger number among the two number.
i.e., X = 2,3,4,5,6
$$P(x=2)=P(1,2)$$
$$\implies =\dfrac 1{^6C_2}=\dfrac 1{15}$$
$$P(x=3)=P(1,3)(2,3)$$
$$\implies =\dfrac 2{^6C_2}=\dfrac 2{15}$$
$$P(x=4)=P(1,4)(2,4)(3,4)$$
$$\implies =\dfrac 3{^6C_2}=\dfrac 3{15}=\dfrac 15$$
$$P(x=5)=P(1,5)(2,5)(3,5)(4,5)$$
$$\implies =\dfrac 4{^6C_2}=\dfrac 4{15}$$
$$P(x=6)=P(1,6)(2,6)(3,6)(4,6)(5,6)$$
$$\implies =\dfrac 5{^6C_2}=\dfrac 5{15}=\dfrac 13$$
Hence the probability distribution is :

 X 2 3 4 5 6
 p(X=x) $$\dfrac 1{15}$$ $$\dfrac 2{15}$$$$\dfrac 15$$  $$\dfrac 4{15}$$ $$\dfrac 13$$
Mean = $$2\left(\dfrac 1{15}\right)+3\left(\dfrac 2{15}\right)+4\left(\dfrac 15\right)+5\left(\dfrac 4{15}\right)+6\left(\dfrac 13\right)$$
$$\implies =\dfrac 2{15}+\dfrac 65+\dfrac {10}3$$
$$\implies =\dfrac 2{15}+\dfrac {68}{15}\\\implies \dfrac {70}{15}$$
Hence, Mean = $$\dfrac {14}3$$
Using Variance formula,
$$Var(X)=(X-\mu)^2\times P(X=x)\\\implies Var(X)=\left(2-\dfrac {14}3\right)^2\times \dfrac 1{15}+\left(3-\dfrac {14}3\right)^2\times \dfrac 2{15}+\left(4-\dfrac {14}3\right)^2\times \dfrac 1{5}+\left(5-\dfrac {14}3\right)^2\times \dfrac 4{15}+\left(6-\dfrac {14}3\right)^2\times \dfrac 1{3}\\\implies Var(X)=\left(-\dfrac {8}3\right)^2\times \dfrac 1{15}+\left(-\dfrac {5}3\right)^2\times \dfrac 2{15}+\left(-\dfrac {2}3\right)^2\times \dfrac 1{5}+\left(\dfrac {1}3\right)^2\times \dfrac 4{15}+\left(\dfrac {4}3\right)^2\times \dfrac 1{3}\\\implies Var(X)=\dfrac {64}{9\times 15}+\dfrac {50}{9\times 15}+\dfrac {4}{9\times 5}+\dfrac {4}{9\times 15}+\dfrac {16}{9\times 3}\\\implies Var(X)=\dfrac {64+50+12+4+80}{9\times 15}=\dfrac {210}{135}=\dfrac {14}{9}$$
Hence, the variance is $$\dfrac {14}9$$


From a lot of $$15$$ bulbs which include $$5$$ defectives, a sample of $$4$$ bulbs is drawn one by one with replacement. Find the probability distribution of number of defective bulbs. Hence find the mean of the distribution.

Let $$X =$$ number of defective bulbs out of 4 drawn $$= 0, 1, 2, 3, 4$$
Probability of defective bulb = $$\dfrac{5}{15}=\dfrac{1}{3}$$
Probability of non-defective bulb = $$1-\dfrac{1}{3}=\dfrac{2}{3}$$
$$P(X=0)=^4C_0. (\dfrac{2}{3})^4=\dfrac{16}{81}$$
$$P(X=1)=^4C_1. \dfrac{1}{3}. (\dfrac{2}{3})^3=\dfrac{32}{81}$$
Similarly, find the probability for $$x=2,3,4.$$
Probability distribution is shown in the table:
Mean $$=$$ $$\sum xP(x)=\dfrac{108}{81}\Rightarrow \dfrac{4}{3}.$$

562818_505162_ans.png


Three numbers are selected at random (without replacement) from first six positive integers. Let $$X$$ denote the largest of the three numbers obtained. Find the probability distribution of $$X$$. Also, find the mean and variance of the distribution.

 $$x$$ $$p(x)$$$$ xp(x)$$ $$x^2p(x)$$

   
    
    
First six positive integers are $$1, 2, 3, 4, 5, 6.$$
Therefore, Three numbers are selected at random without replacement so, total no. of ways $$^6C_3=20$$.
Let, $$x$$ denote the larger of three numbers.
So, $$x$$ can take values $$3, 4, 5, 6.$$
$$p(x=3)=\dfrac{1}{20}$$

$$p(x=4)=\dfrac{3}{20}$$

$$p(x=5)=\dfrac{6}{20}$$

$$p(x=6)=\dfrac{10}{20}$$
Refer the tabular column:
$$mean = \sum xp(x)$$

$$=\dfrac{3}{20}+\dfrac{12}{20}+\dfrac{30}{20}+\dfrac{60}{20}$$

$$=\dfrac{150}{20}=5.25$$
Variance $$= \sum x^2p(x)-\left ( \sum xp(x) \right )^2$$

$$=\left ( \dfrac{567}{20} \right )-\left ( \dfrac{105}{20} \right )^2$$

$$=28.35-27.56=0.79.$$

565253_493804_ans.png


Let the p.m.f. (probability mass function) of random variable $$x$$ be
$$P(x) = \begin{cases} \left( \dfrac{4}{x} \right ) \left( \dfrac{5}{9}\right)^x \left(\dfrac{4}{x} \right )^{4 - x},\ x = 0, 1, 2, 3, 4\\ 0,\,\,\, \text{otherwise} \end{cases}$$
Find $$E(x)$$ and Var$$(x)$$.

$$P(x) =\left( \dfrac{4}{x} \right ) \left( \dfrac{5}{9} \right )^x \left( \dfrac{4}{x} \right )^{4 - x}$$,         $$ x = 0, 1, 2, 3, 4$$.
$$= 0$$ otherwise
The probability distribution table of the function is
$$x$$           $$0$$          $$1$$          $$2$$           $$3$$           $$4$$


$$P(x)$$


$$\infty$$$$\dfrac{1280}{9}$$$$\dfrac{200}{81}$$$$\dfrac{2000}{6561}$$$$\dfrac{625}{6561}$$
$$x^2$$$$0$$$$1$$$$4$$$$9$$$$16$$

$$P(x). x^2$$    
$$0$$
$$\dfrac{1280}{9}$$

$$\dfrac{800}{81}$$
$$\dfrac{18000}{6561}$$
$$\dfrac{10000}{6561}$$

$$E (x) =\displaystyle \sum_{x =1}^{4} x . P(x)$$
$$= 0 \times \infty  + 1 \times \dfrac{1280}{9} + 2 \times \dfrac{200}{81} + 3 \times \dfrac{2000}{6561} + 4 \times \dfrac{625}{6561}$$
$$= 148.4560$$
Var$$(x)=\displaystyle \sum x^2P(x) - \left(\sum xP(x)\right)^2= \left( 0 + \dfrac{1280}{9} + \dfrac{800}{81} + \dfrac{18000}{6561} + \dfrac{10000}{6561} \right ) - (148.5660)^2$$
$$= 156.366 - (148.4560)^2$$


The time (in minute) for a lab assistant to prepare the equipment for a certain experiment is a random variable $$X$$ taking values between $$25$$ and $$35$$ minutes with p.d.f.
$$f(x) = \begin{cases} \dfrac {1}{10}, 25\leq x \leq 35\\ \\ 0,\,\,\, \text{otherwise} \end{cases}$$.
What is the probability that preparation time exeeds $$33$$ minutes? Also find the c.d.f. of $$X$$.

$$f(x) = \cfrac {1}{10}$$
Required probability $$= P(X > 33)$$
$$= \displaystyle \int _{33}^{\infty} f(x) dx$$
$$= \displaystyle \int _{33}^{35} f(x) dx + \displaystyle \int _{35}^{\infty} f(x) dx$$
$$\displaystyle \int _{33}^{35} f(x)  dx + 0 [\because f(x) = 0$$ when $$x > 35]$$
$$= \displaystyle \int _{33}^{35} \cfrac {1}{10}dx$$.
$$= \cfrac {1}{10} \displaystyle \int _{33}^{35} 1dx$$.
$$= \cfrac {1}{10} [x]_{33}^{35}$$
$$= \cfrac {1}{10} [35 - 33] = \cfrac {1}{5}$$

Let $$F(x)$$ be the c.d.f. of $$X$$.
$$\therefore F(x) = P[X\leq x]$$
$$= \displaystyle \int _{-\infty}^{\infty} f(x) dx$$
$$= \displaystyle \int _{-\infty}^{25} f(x)dx + \displaystyle \int _{25}^{x}f(x) dx$$
$$= 0 + \displaystyle \int _{25}^{x} f(x)  dx [\because f(x) = 0$$, when $$f(x) < 25]$$
$$= \displaystyle \int _{25}^{x} \cfrac {1}{10}dx$$
$$= \cfrac {1}{10}[x]_{25}^{x}$$
$$= \cfrac {1}{10}[x - 25]$$
$$\therefore F(x) = \cfrac {x - 25}{10}$$.


The range of a random variable $$X$$ is $$\left \{0, 1, 2\right \}$$. Given that $$P(X = 0) = 3C^{3}, P(X = 1) = 4C - 10C^{2}, P(X = 2) = 5C - 1$$
i) Find the value of $$C$$.
ii) $$P(X < 1), P(1 < X \leq 2)$$ and $$P(0 < X \leq 3)$$

(i) We must have $$\sum P(X)=1$$
$$\Rightarrow P(X=0)+P(X=1)+P(X=2)=1$$
$$\Rightarrow (3C^3)+(4C-10C^2)+(5C-1)=1$$
$$\Rightarrow 3C^3-10C^2+9C-2=0$$
$$\Rightarrow (C-1)(3C^2-7C+2)=0$$
$$\Rightarrow (C-1)(C-2)(3C-1)=0$$
$$\Rightarrow C=1,2,\dfrac{1}{3}$$
Also $$0\le P(X=0), P(X=1), P(X=2)\le 1$$
So only acceptable value of $$C$$ is $$\dfrac{1}{3}$$.
(ii)$$P(X<1)=P(X=0)=3\left(\dfrac{1}{3}\right)^3=\dfrac{1}{9}$$
(iii)$$P(1<X\le 2)=P(X=2)=4C-10C^2=4\cdot \dfrac{1}{3}-10\cdot \dfrac{1}{9}=\dfrac{2}{9}$$
And (iv)$$P(0<X\le 3)=1-P(X=0)=1-\dfrac{1}{9}=\dfrac{8}{9}$$


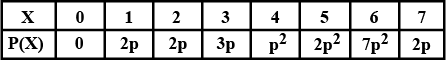
A random variable '$$X$$' has the following probability distribution:
X=x01234567
P(X=x)$$0$$$$k$$$$2k$$$$2k$$$$3k$$$${k}^{2}$$$$2{k}^{2}$$$$7{k}^{2}+k$$
Find:
(i) $$k$$
(ii) The Mean and
(iii) $$P(0< X< 5)$$

Sum of all probabilities must be equal to $$1$$
So we get $$K+2K+2K+3K+{K}^{2}+2{K}^{2}+7{K}^{2}+K=1$$
$$\Rightarrow 10{K}^{2}+9K=1$$
$$\Rightarrow K = -1,\dfrac{1}{10}$$
But probability cannot be negative , therefore the value of $$K=\dfrac{1}{10}$$
The mean is $$1.K+2.2K+3.2K+4.3K+5{K}^{2}+6.2{K}^{2}+7.7{K}^{2}+7K$$
$$= 30K+66{K}^{2}=3+0.66=3.66$$

$$P(0<x<5)=P(x=1)+P(x=2)+P(x=3)+P(x=4)=K+2K+2K+3K=8K=0.8$$


Find the mean and variance for the probability density function : $$f(x)=\begin{cases} \alpha { e }^{ -\alpha x }\,\,\, \text{if}\, x>0 \\ 0\,\,\, \text{otherwise} \end{cases}$$

$$f(x)=\begin{cases} \alpha { e }^{ -\alpha x }\,\,\, \text{if}\, x>0 \\ 0\,\,\, \text{otherwise} \end{cases}$$
$$E(X)=\displaystyle \int _{ -\infty  }^{ \infty  }{ xf(x) } dx=\int _{ 0 }^{ \infty  }{ x(\alpha )\left( { e }^{ -\alpha x } \right) dx } $$
$$=\alpha \displaystyle  \int _{ 0 }^{ \infty  }{ x{ e }^{ -\alpha x } } dx=\cfrac { \alpha (1) }{ { \alpha  }^{ 2 } } =\cfrac { 1 }{ \alpha  } $$
[By Bernoulli's formula $$\displaystyle \int _{ 0 }^{ \infty  }{ { x }^{ n }{ e }^{ -\alpha x } } dx=\cfrac { n }{ { a }^{ n+1 } } $$]
$$E({ X }^{ 2 })=\displaystyle \int _{ 0 }^{ \infty  }{ { x }^{ 2 }\left( \alpha { e }^{ -\alpha x } \right) dx } $$
$$\left[\displaystyle  \int _{ 0 }^{ \infty  }{ { x }^{ n }{ e }^{ -\alpha x } } dx=\cfrac { n }{ { a }^{ n+1 } }  \right] =\alpha \displaystyle \int _{ 0 }^{ \infty  }{ { x }^{ 2 }{ e }^{ -\alpha x } } dx=\cfrac { \alpha (2) }{ { \alpha  }^{ 3 } } =\cfrac { 2 }{ { \alpha  }^{ 2 } } $$
[By Bernoulli's formula]
Mean$$=E(X)=\cfrac { 1 }{ \alpha  } $$
Variance $$=E({ X }^{ 2 })-{ \left[ E(X) \right]  }^{ 2 }=\cfrac { 2 }{ { \alpha  }^{ 2 } } -\cfrac { 1 }{ { \alpha  }^{ 2 } } =\cfrac { 1 }{ { \alpha  }^{ 2 } } $$


A random variable $$X$$ has the following probability distribution.
$$X = x$$$$0$$$$1$$$$2$$$$3$$$$4$$$$5$$$$6$$$$7$$
$$P(X = x)$$$$0$$$$k$$$$2k$$$$2k$$$$3k$$$$k^{2}$$$$2k^{2}$$$$7k^{2} + k$$
Find (i) $$k$$ (ii) The mean and (iii) $$P(0 < X < 5)$$

(i) The total probability should add up to $$1$$.
$$\therefore 0 + k + 2k + 2k + 3k + k^2 + 2k^2 + 7k^2 + k = 1$$
$$\therefore 10k^2 + 9k - 1 = 0$$
$$\therefore 10k^2 + 10k - k - 1 = 0$$
$$\therefore (10k - 1)(k + 1) = 0$$
Since $$k$$ cannot be negative, $$k = \cfrac{1}{10}$$

(ii) Mean $$= \sum x_i P_i$$
$$= 0 \times 0 + 1 \times k + 2 \times 2k + 3 \times 2k + 4 \times 3k + 5 \times k^2 + 6 \times 2k^2 + 7 \times (7k^2 + k)$$
$$= k + 4k + 6k + 12k + 5k^2 + 12k^2 + 49k^2 + 7k $$
$$= 30k + 66k^2$$
$$= 3 + 0.66$$
$$= 3.66$$

(iii) $$P(0 < X < 5) = P(X = 1) + P(X = 2) + P(X = 3) + P(X = 4)$$
$$\therefore P(0 < X < 5) = k + 2k + 2k + 3k = 9k = 0.9$$


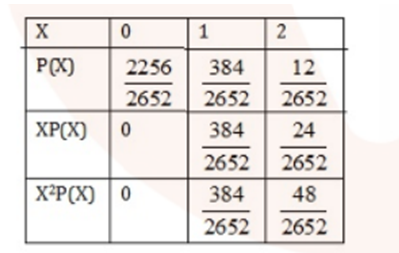
Two cards are drawn with replacement from a well shuffled deck of $$52$$ cards. Find the mean and variance for the number of aces.

Let $$X$$ denote the number of aces drawn in drawing two cards with replacement.
$$\therefore X$$ can take the values $$0, 1$$ and $$2$$.
Let $$P(S) = P$$ (getting aces) $$= \dfrac {4}{52}\Rightarrow P(F)$$
$$= P$$ (not getting aces) $$= \dfrac {48}{52}$$
$$P(X = 0) = P (no\ ace) = P(FF) = P(F)$$
$$P(F) = \dfrac {48}{52}\cdot \dfrac {48}{52} =\dfrac {144}{169}$$
$$P(X = 1) = P $$ (one ace) $$= P(SF\ or\ FS) = P(S) .P(F) + P(F) P(S)$$
$$= 2(PS) . P(F) = 2\times \dfrac {4}{52}\times \dfrac {48}{52} = \dfrac {24}{169}$$
$$P(X - 2) = P$$ (two aces) $$= P(SS) = P(S)$$.
$$P(S) = \dfrac {4}{52}\cdot \dfrac {4}{52} = \dfrac {1}{169}$$
$$X$$$$0$$$$1$$$$2$$
$$P(X = x)$$=$$\dfrac {144}{169}$$$$\dfrac {24}{169}$$$$\dfrac {1}{169}$$
Mean $$= E[X] = \displaystyle \sum_{-\infty}^{\infty} x_{i}p_{i} =(0) \left (\dfrac {144}{169}\right ) + (1)\left (\dfrac {24}{169}\right ) + (2)\left (\dfrac {1}{169}\right )$$
$$= \dfrac {24 + 2}{169} =\dfrac {26}{169} = \dfrac {2}{13}$$
$$E(X^{2}) = \displaystyle \sum_{x = 0}^{2} x_{i}^{2} p_{i} = (0^{2}) \left (\dfrac {144}{169}\right ) + (1^{2})\left (\dfrac {24}{169}\right ) + (2^{2})\left (\dfrac {1}{169}\right )$$ 
$$= \dfrac {24 + 4}{169} = \dfrac {28}{169}$$
Variance $$(X) = E[X^{2}] - (E(X))^{2}$$
$$= \dfrac {28}{169} - \left (\dfrac {2}{13}\right )^{2} = \dfrac {28}{169} - \dfrac {4}{169} = \dfrac {24}{169}$$


If the probability density function of a random variable is given by,
$$f(x) = \left\{\begin{matrix} k(1 - x^2),& 0 < x < 1\\ 0, & \text{elsewhere}\end{matrix} \right.$$ find $$k$$ and the distribution function of the random variable.

(i) Since $$f(x)$$ is a p.d.f. $$\displaystyle \int_{- \infty}^{\infty} f(x) dx = 1$$
$$\Rightarrow \displaystyle \int_0^1 k(1 - x^2) dx  =1 $$
$$\Rightarrow k \left [ x - \dfrac{x^3}{3} \right ]_0^1 = 1$$
$$\Rightarrow k \left( 1 - \dfrac{1}{3}  \right ) = 1 $$
$$\Rightarrow \dfrac{2k}{3} = 1 $$
$$\Rightarrow k = \dfrac{3}{2}$$
(ii) The distribution function $$F(x) = \displaystyle \int_{- \infty}^{x} f(t) dt$$
(a) When $$x \in (-\infty, 0]$$
$$F(x) = \displaystyle \int_{-\infty}^{x} f(t) dt = 0$$
(b) When $$x \varepsilon (0, 1]$$
$$F(x) =\displaystyle  \int_{-\infty}^{x} f(t) dt = \int_{-\infty}^0 f(t) dt + \int_0^x f(t) dt$$
$$ = 0 + \dfrac{3}{2}\displaystyle  \int_0^x (1 - t^2) dt$$
$$F(x) = \dfrac{3}{2} (x - \dfrac{x^3}{3})$$
(c) When $$x \in [1, \infty)$$
$$F(x) = \displaystyle \int_{-\infty}^x f(t) dt$$
$$=\displaystyle  \int_{-\infty}^0 f(t) dt + \int_0^1 f(t) dt + \int_1^x f(t) dt$$
$$= 0 +\displaystyle  \int_0^1 \dfrac{3}{2} (1 - t^2) dt + 0$$
$$= \dfrac{3}{2} \left [ t \dfrac{t^3}{3} \right ]_0^1 = 1$$
$$\therefore F(x) = \left\{\begin{matrix} 0& -\infty < x \leq 0\\ \dfrac{3}{2} \left( x- \dfrac{x^3}{3} \right ),  & 0 < x < 1 \\ 1  & 1 \leq x \infty \end{matrix}\right.$$


A random variable $$X$$ has the following probability mass function.
$$x$$$$0$$$$1$$$$2$$$$3$$$$4$$$$5$$$$6$$
$$P(X = x)$$$$k$$$$3k$$$$5k$$$$7k$$$$9k$$$$11k$$$$13k$$
(a) Find $$k$$.
(b) Evaluate $$P(X < 4), P (X$$ $$\geq$$ $$5)$$ and $$P(3< X$$ $$\leq$$ $$6)$$.
(c) What is the smallest value of $$x$$ for which $$P(X$$ $$\leq$$ $$x) >$$ $$\dfrac{1}{2}$$?

(a) $$P(X = x)$$ is a probability mass function.
$$\displaystyle \sum_{x = 0}^{6} P(X = x) = 1$$
(i.e.,) $$P(X = 0) + P(X = 1) + P(X = 2) + P(X = 3) + P(X = 4) + P(X = 5) + P(X = 6) = 1$$.
$$\Rightarrow k + 3k + 5k + 7k + 9k + 11 k + 13k = 1$$
$$\Rightarrow 49 k = 1 \Rightarrow k = \dfrac{1}{49}$$
(b) $$P(X < 4) = P(X = 0) + P(X = 1) + P(X = 2) + P(X = 3)$$
$$= \dfrac{1}{49} + \dfrac{3}{49} + \dfrac{5}{49} + \dfrac{7}{49} = \dfrac{16}{49}$$
$$P(X \geq 5) = P(X = 5) + P(X = 6) = \dfrac{11}{49} + \dfrac{13}{49} = \dfrac{24}{49}$$
$$P(3 < x \leq 6) = P(X = 4) +P(X = 5) + P(X = 6)$$
$$= \dfrac{9}{49} + \dfrac{11}{49} + \dfrac{13}{49} = \dfrac{33}{49}$$
(c) The minimum value of $$x$$ may be determined by trial and error method.
$$P(X \leq 0) = \dfrac{1}{49} < \dfrac{1}{2} ; P (X \leq 1) = \dfrac{4}{49} < \dfrac{1}{2}$$
$$P(X \leq 2) = \dfrac{9}{49} < \dfrac{1}{2} ; P (X \leq 3) = \dfrac{16}{49} < \dfrac{1}{2}$$
$$P(X \leq 4) = \dfrac{25}{49} > \dfrac{1}{2}$$
$$\therefore$$ the smallest value of $$x$$ for which $$P(X \leq x) > \dfrac{1}{2}$$ is $$4$$.


Given the probability density function (p.d.f) of a continuous random variable $$X$$ as,
$$f(x) = \dfrac {x^{2}}{3}, -1 < x < 2 = 1$$, otherwise
Determine the cumulative distribution function (c.d.f.) of $$X$$ and hence find $$P(X < 1), P(X > 0), P(1 < X < 2)$$

Given the PDF of a continuous random variable $$X.$$
$$\Rightarrow f\left( x \right) =\dfrac { { x }^{ 3 } }{ 3 } ,-1<x<2=1$$
     $$f\left( x \right) =\int _{ -\infty  }^{ \alpha  }{ f\left( x \right) dx } =\int _{ \alpha  }^{ \beta  }{ \dfrac { 1 }{ \beta -\alpha  } dx } =\left[ \dfrac { 1 }{ \beta -\alpha  } x \right] _{ \infty  }^{ x }=\dfrac { x-\alpha  }{ \beta -\alpha  } $$
     $$f\left( x \right) =\int { \dfrac { 1 }{ 2-(-1) } ,-1<x<2}$$       $$=\int { \dfrac{1}{3},-1<x<2}$$
                     $${0,otherwise }$$                                   $${0,otherwise } $$
$$\Rightarrow$$ When, $$P(1<x<2)=\int _{ 1 }^{ 2 }{ \dfrac{1}{3} }\;dx=\left[ \dfrac { 1 }{ 3 } x \right] _{ 0 }^{ 1 }=\dfrac { 1 }{ 3 } $$
     When $$P(x>0)=0$$
     When $$P(1<x<2)=\int _{ 1 }^{ 2 }{ \dfrac{1}{3} }\;dx=\left[ \dfrac { x }{ 3 } x \right] _{ 1 }^{ 2 }=\dfrac { 2 }{ 3 } -\dfrac { 1 }{ 3 } =\dfrac { 1 }{ 3 } $$
Hence, solved.



A die is tossed twice. A success is getting an odd number on a toss. Find the mean and the variance of the probability distribution of the number of successes.

Let $$x$$ be the random variable denoting the number of times an odd number (the number of successes) when a die is tossed twice.

Then $$x$$ takes the values $$0,1,2$$

Let $$P(X=0)$$ be probability of getting no odd number (both times showing even).

$$\therefore P(X=0)=\dfrac{3}{6}\times \dfrac{3}{6}=\dfrac{1}{4}$$

Let $$P(X=1)$$ be probability of getting odd number once.

$$\therefore P(X=1)=2C_1\dfrac{3}{6}\times \dfrac{3}{6}=\dfrac{6}{6}\times \dfrac{3}{6}=\dfrac{1}{2}$$

Let $$P(X=2)$$ be probability of getting odd number twice.

$$\therefore P(X=2)=\dfrac{3}{6}\times \dfrac{3}{6}=\dfrac{1}{4}$$

Thus the probability distribution of $$X$$ is given by
 $$X=x$$ $$x=0$$ $$x=1$$ $$x=2$$
 $$P(X=x)$$ $$\dfrac{1}{4}$$ $$\dfrac{1}{2}$$ $$\dfrac{1}{4}$$
We know that mean $$E(X)=\sum x_iP_i=0\times \dfrac{1}{4}+1\times \dfrac{1}{2}+2\times \dfrac{1}{4}$$

$$\therefore E(X)=0+\dfrac{1}{2}+\dfrac{1}{2}=1$$

Thus mean $$E(X)=1$$.

We know that $$var(X)=E(X^2)-[E(X)]^2$$

$$E(X^2)=\sum x_i^2P_i=0\times \dfrac{1}{4}+1^2\times \dfrac{1}{2}+2^2\times \dfrac{1}{4}$$

$$\therefore E(X^2)=0+\dfrac{1}{2}+4\times \dfrac{1}{4}=\dfrac{3}{2}$$

Thus $$var(X)=\dfrac{3}{2}-[1]^2=\dfrac{3}{2}-1=\dfrac{1}{2}$$

Hence mean is $$1$$ and variance is $$\dfrac{1}{2}$$.



A player tosses $$2$$ fair coins. He wins Rs$$5$$ if $$2$$ heads appear, Rs$$2$$ if head appears and Rs$$1$$ if no head appears. Find his expected winning amount and variance of winning amount.

No. of coin $$=2$$
$$\Rightarrow$$ Sample space $$=2^2=4=[HH,HT,TH,TT]$$
$$\Rightarrow$$ Probability of getting  $$2$$ heads, i.e, $$P_1=\dfrac{1}{4}$$  $$\therefore n_1=Rs. 5$$

     Probability of getting  $$1$$ head, i.e, $$P_1=\dfrac{2}{4}$$  $$\therefore n_2=Rs. 2$$

     Probability of getting  no head, i.e, $$P_3=\dfrac{1}{4}$$  $$\therefore n_3=Rs. 1$$

$$\Rightarrow$$ Expectation $$=n_1p_1+n_2p_2+n_3p_3$$

                          $$=\dfrac{5}{4}+\dfrac{2\times2}{4}+\dfrac{1}{4}$$

                          $$=\dfrac{5+4+1}{4}$$

                          $$=\dfrac{10}{4}$$

                          $$=\dfrac{5}{2}$$
Hence, the answer is $$\dfrac{5}{2}.$$


The following is the c.d.f. of a discrete r.v. X:
X$$-3$$$$-1$$$$0$$$$1$$$$3$$$$5$$$$7$$$$9$$
$$F(x)$$$$0.1$$$$0.3$$$$0.5$$$$0.65$$$$0.75$$$$0.85$$$$0.90$$$$1$$
Find $$P(X\leq 3/X > 0)$$.

$$P\left( X\le 3\right)=P\left( X=-3\right) +P\left( X=-1\right) +P\left( X=0\right)+P\left( X=1\right) +P\left( X=3\right) $$
                    $$=0.1+0.2+0.2+0.5+0.1$$
                    $$=0.75$$
$$\Rightarrow P\left( X>0\right)=P\left( X=1\right) +P\left( X=3\right) +P\left( X=5\right)+P\left( X=7\right) +P\left( X=9\right) $$
                         $$=0.15+0.1+0.1+0.05+0.1$$
                         $$=0.5$$
$$\Rightarrow \dfrac{P\left( X\le 3\right)}{P\left( X>0\right)}=\dfrac{0.75}{0.5}=\dfrac{7.5}{5}=1.5$$
Hence, the answer is $$1.5.$$


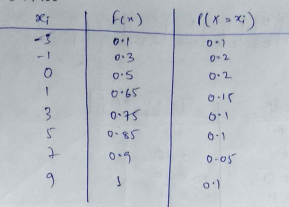
1229829_1347460_ans_88f3d900989944e986e0009c37468389.png


The following is the c.d.f. of a discrete r.v. X:
X$$-3$$$$-1$$$$0$$$$1$$$$3$$$$5$$$$7$$$$9$$
$$F(x)$$$$0.1$$$$0.3$$$$0.5$$$$0.65$$$$0.75$$$$0.85$$$$0.90$$$$1$$
Find $$P(-1 \leq X < 2)$$.

$$P\left( -1 \le X\le 2\right) =P\left( X=-1\right) +P\left( X=0\right) +P\left( X=1\right)$$
                               $$=0.2+0.2+0.15$$
                               $$=0.55$$
Hence, the answer is $$0.55$$


1229235_1347442_ans_0e56af1e276e4b5a8816bf5e38e86f35.png


The following is the c.d.f. of a discrete r.v. X:
X$$-3$$$$-1$$$$0$$$$1$$$$3$$$$5$$$$7$$$$9$$
$$F(x)$$$$0.1$$$$0.3$$$$0.5$$$$0.65$$$$0.75$$$$0.85$$$$0.90$$$$1$$
Find $$P(X \geq 5)$$.

$$P\left( X\ge 5\right)=1-P\left( X<5\right)$$
                    $$=1-0.75$$
                    $$=0.25$$
Hence, the answer is $$0.25.$$



The following is the c.d.f. of a discrete r.v. X:
X$$-3$$$$-1$$$$0$$$$1$$$$3$$$$5$$$$7$$$$9$$
$$F(x)$$$$0.1$$$$0.3$$$$0.5$$$$0.65$$$$0.75$$$$0.85$$$$0.90$$$$1$$
Find the p.m.f. of X.


1379962_1347434_ans_7ca975f3bb364528a1ca0f2a2a8f264f.png


A die is thrown $$120$$ times and getting $$1$$ or $$5$$ is considered a success. Find the  mean and variance of the number of sucess.

Given: dice is thrown $$120$$ times
$$1$$ or $$5$$ is success
$$\therefore\,p=$$ Probability of success $$=\dfrac{2}{6}=\dfrac{1}{3}$$
$$\therefore\,q=$$ Probability of failure =$$1-\dfrac{1}{3}=\dfrac{2}{3}$$
$$\therefore Mean =np$$
$$120\times \dfrac{1}{3}=40$$
$$(\because n=120)$$

$$Variance =npq$$
$$120\times \dfrac{1}{3}\times \dfrac{2}{3}=\dfrac{80}{3}$$


Class 12 Commerce Applied Mathematics Extra Questions