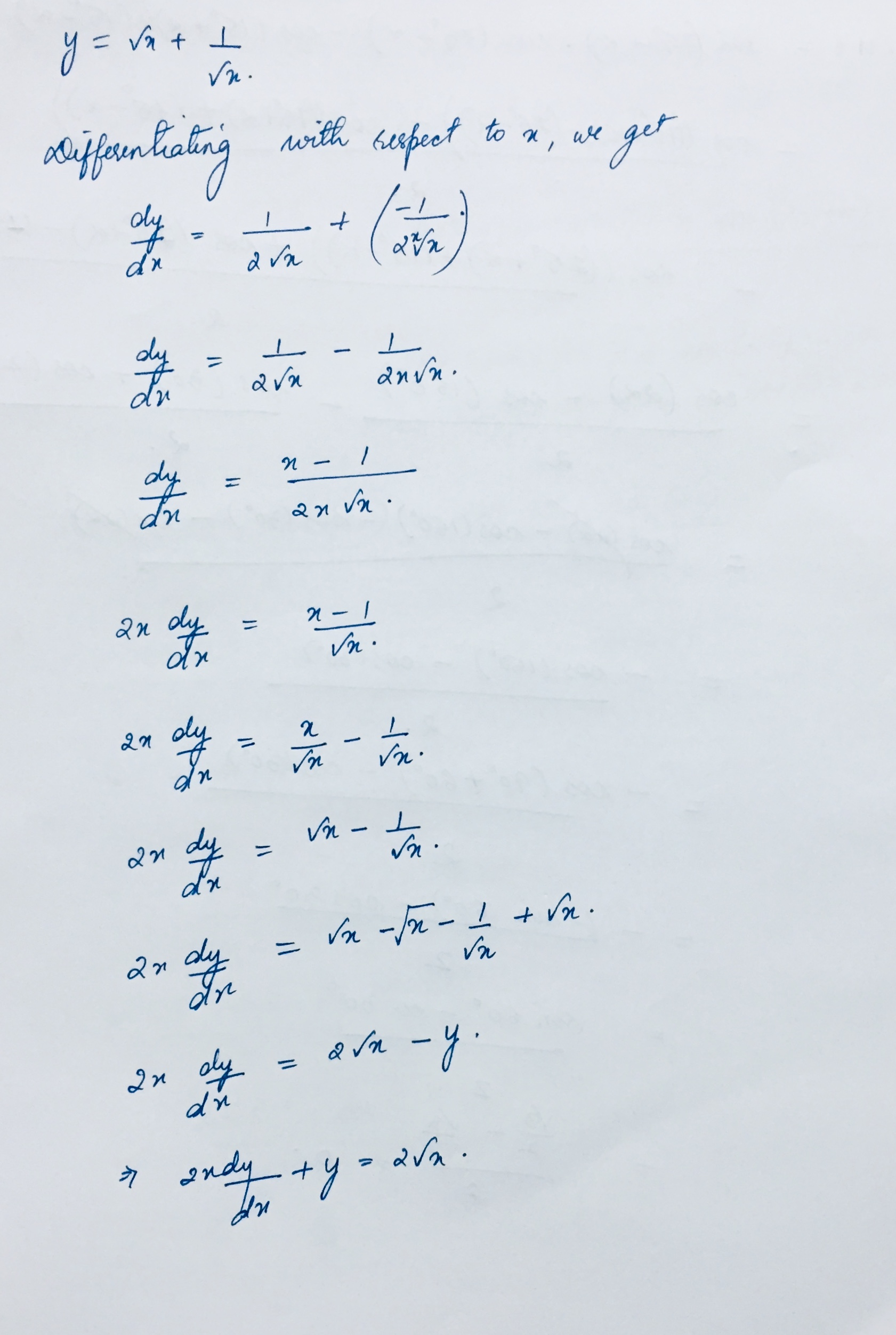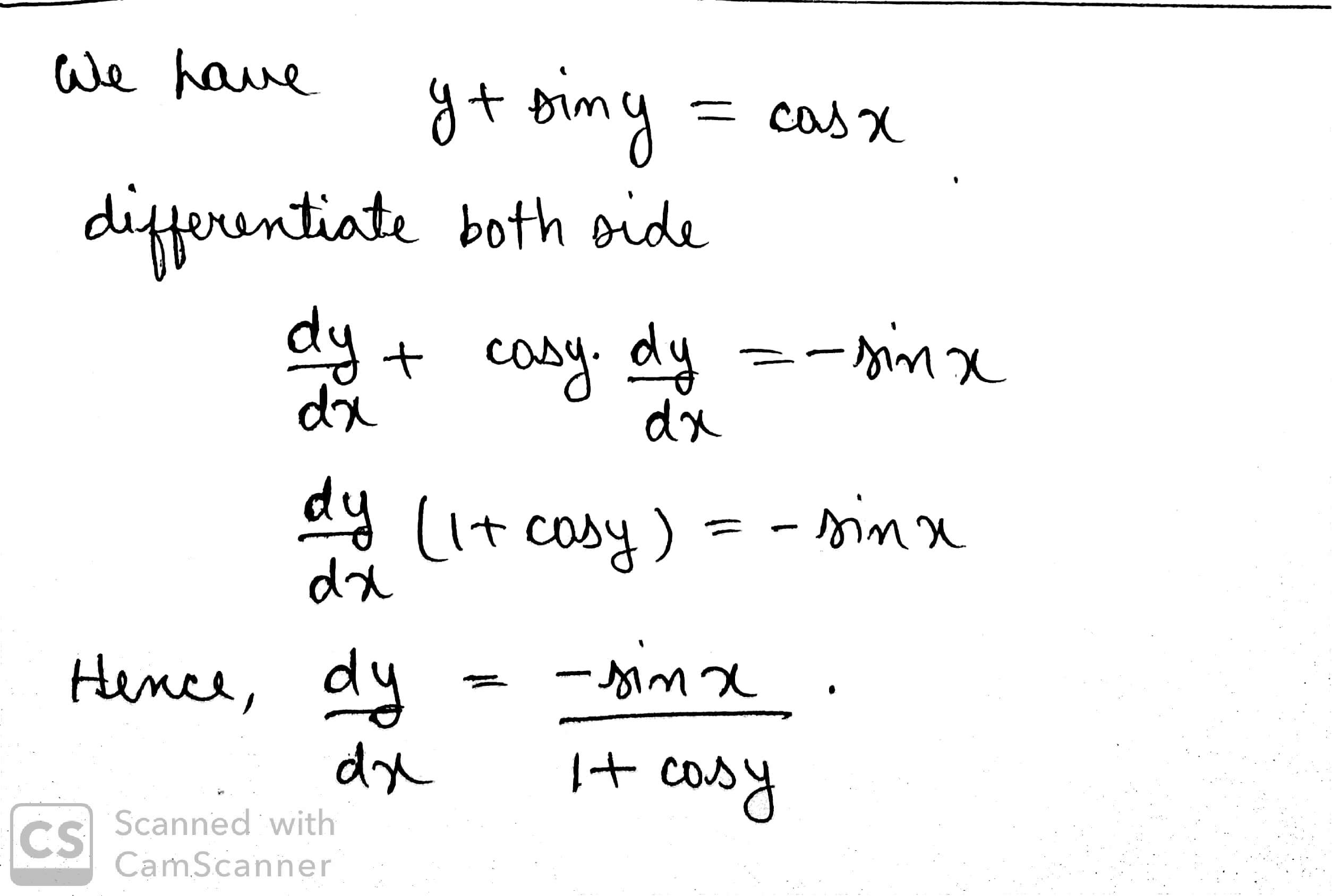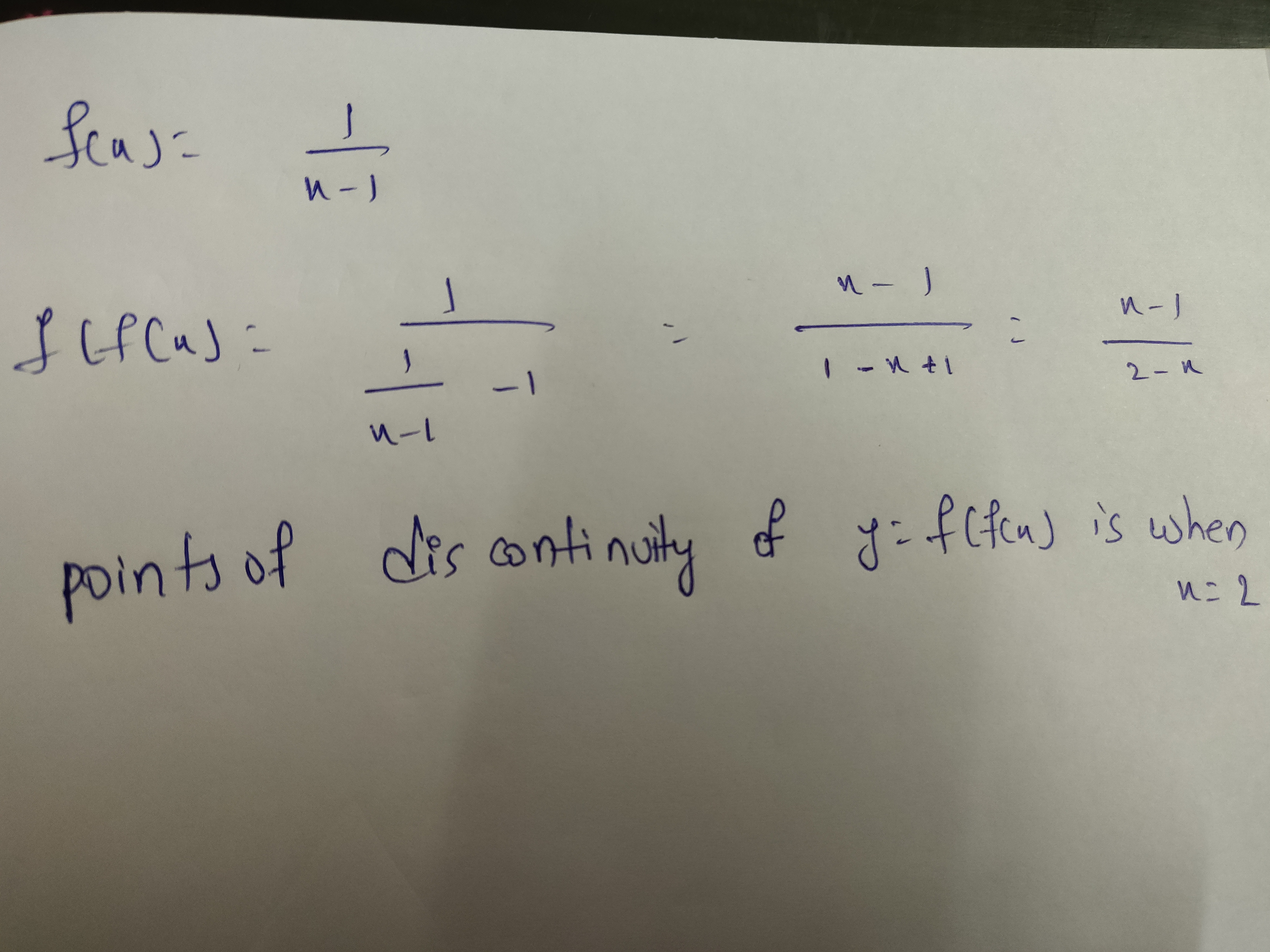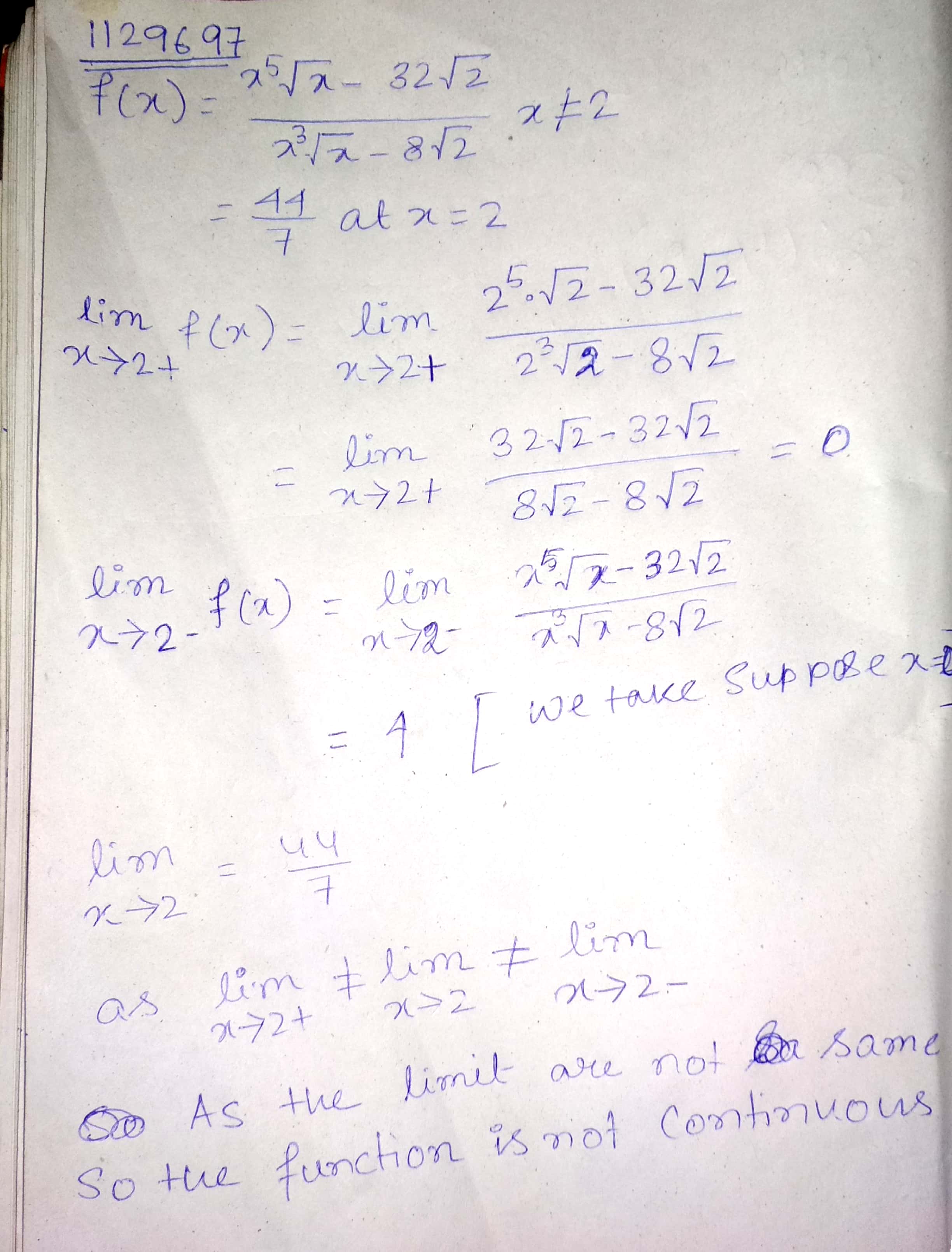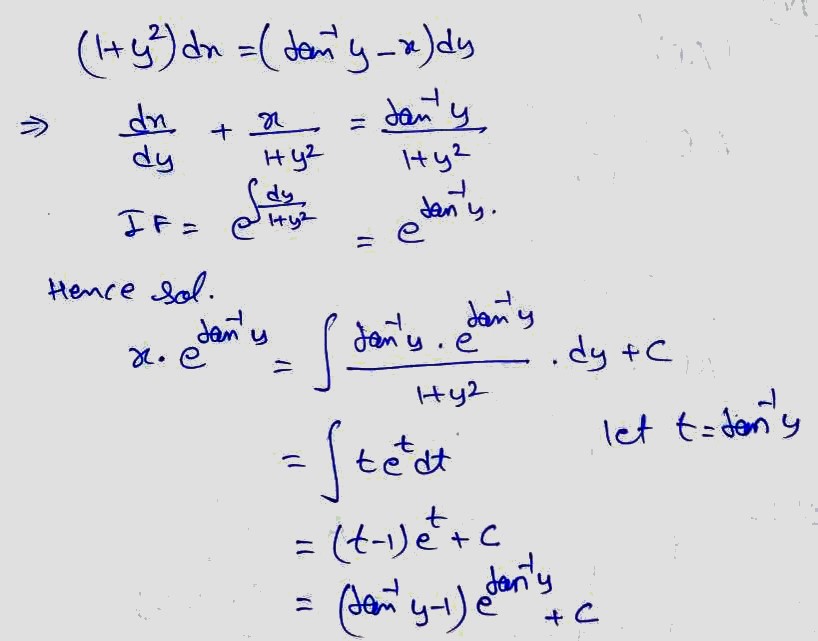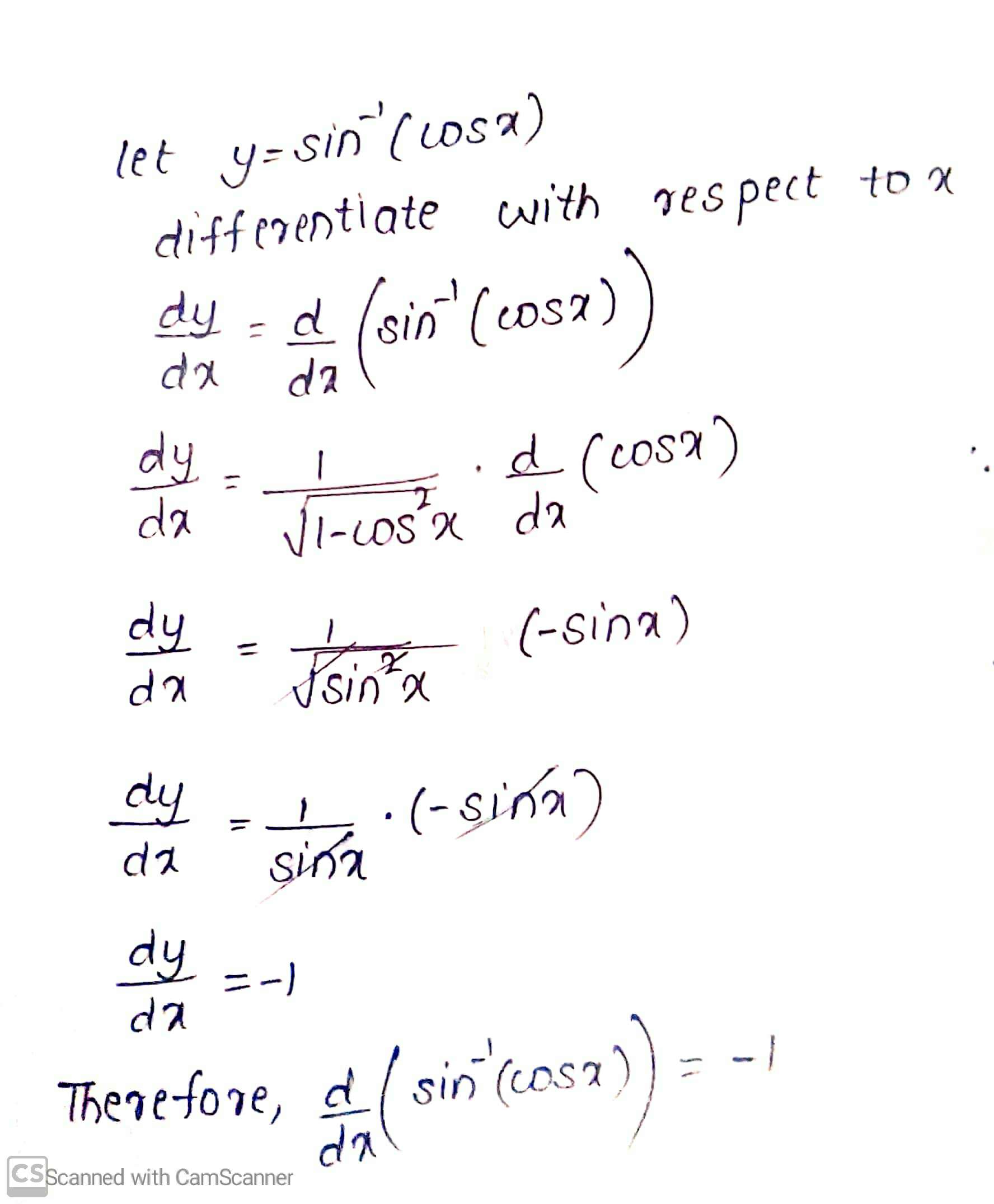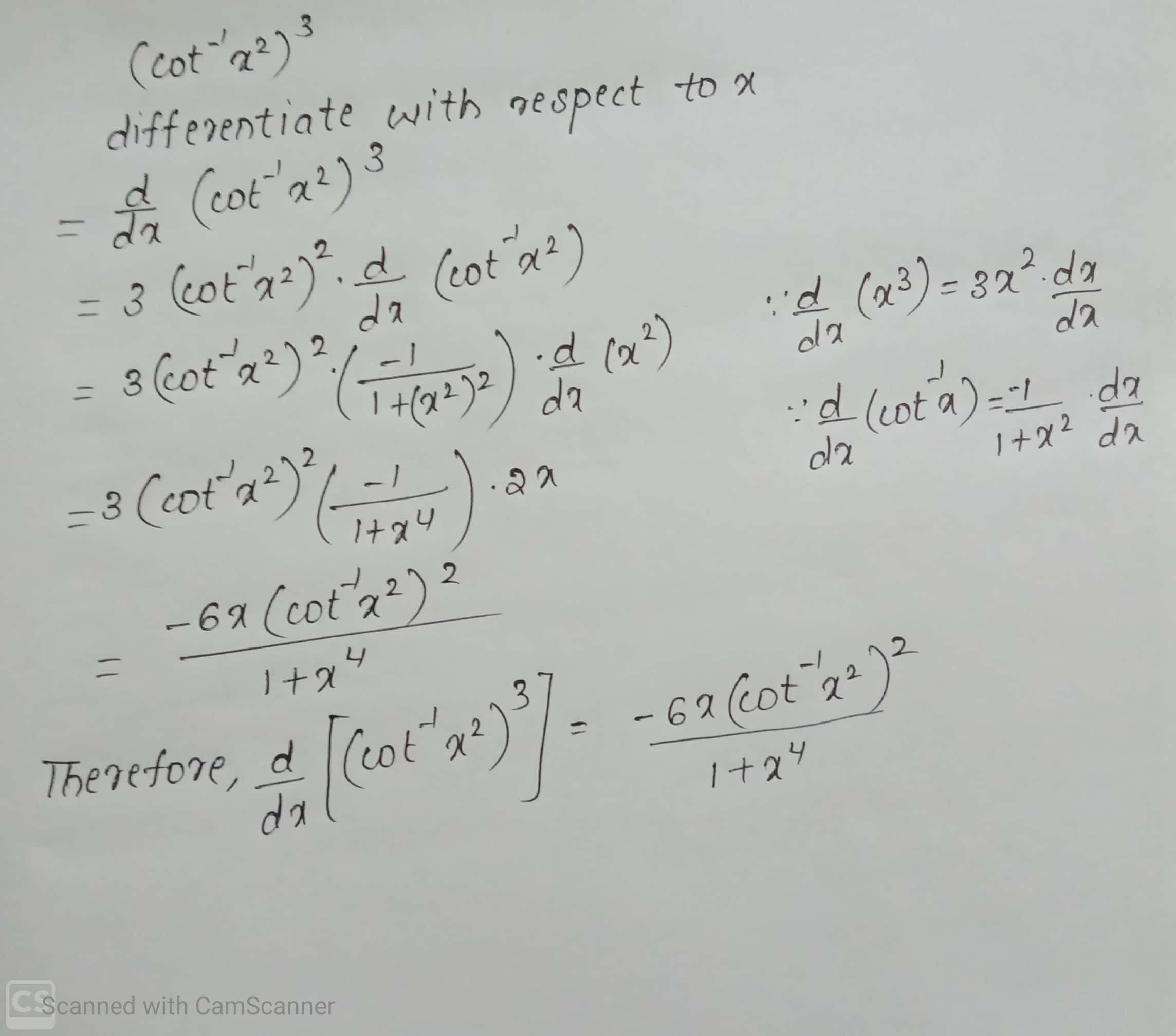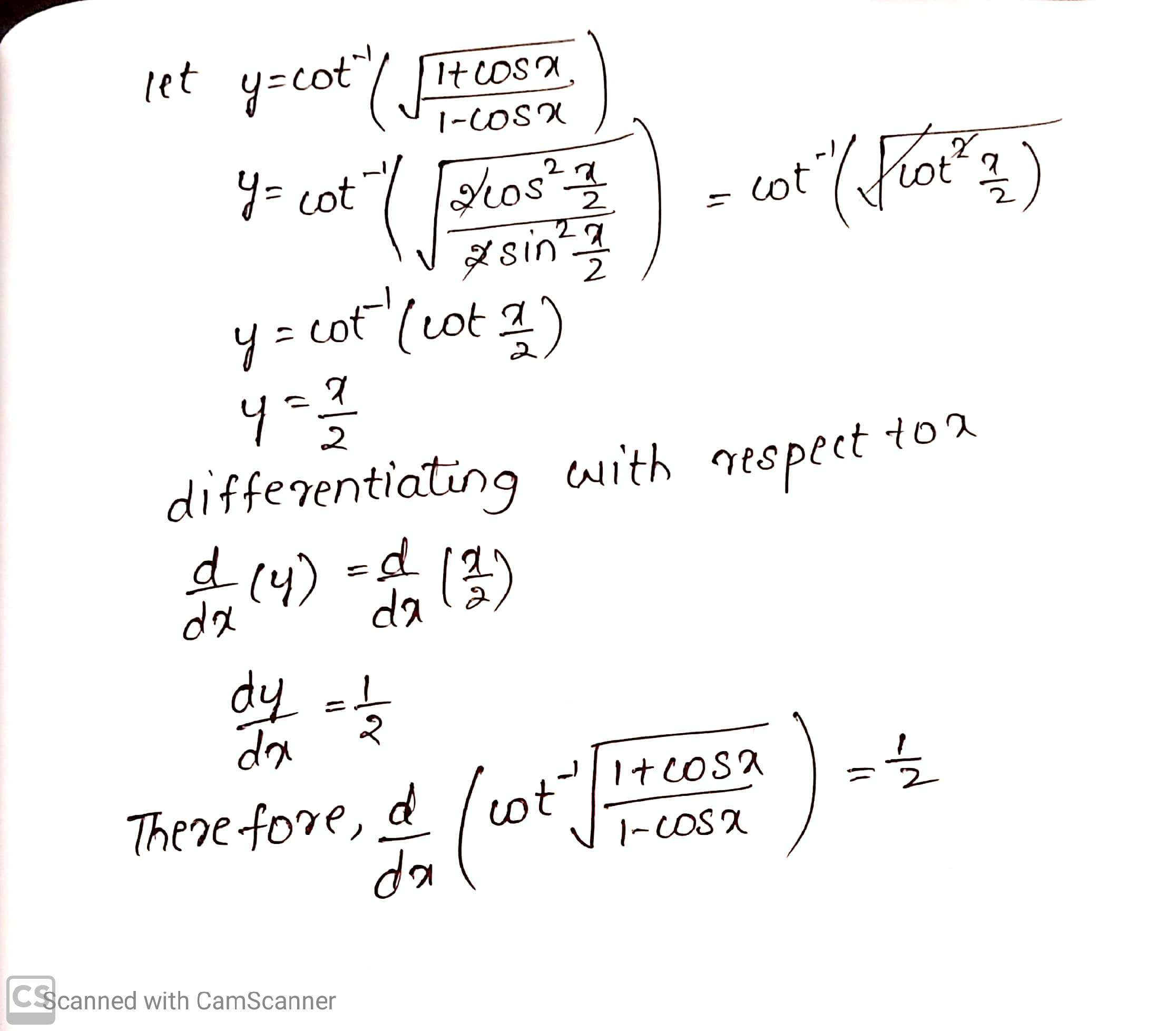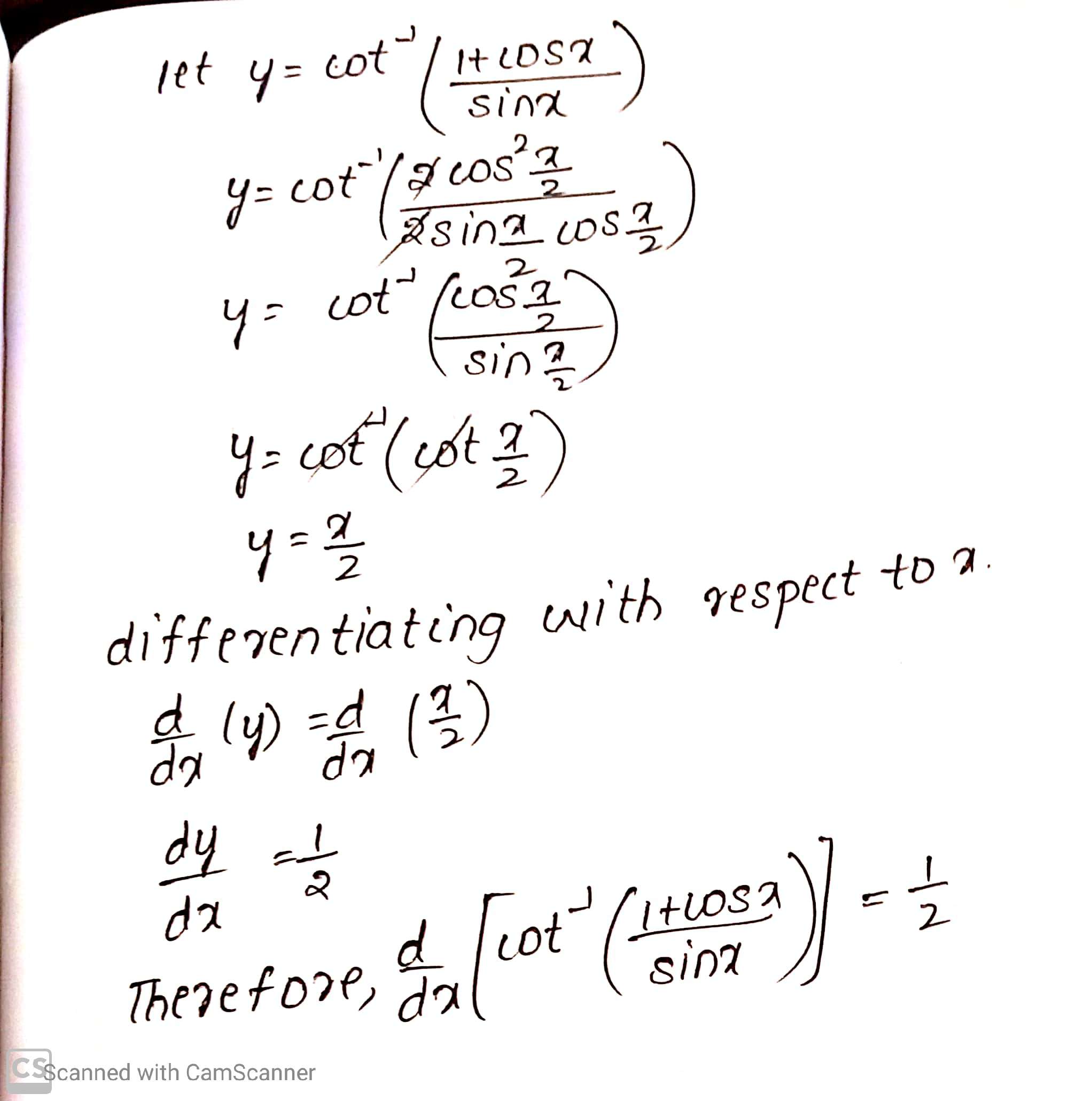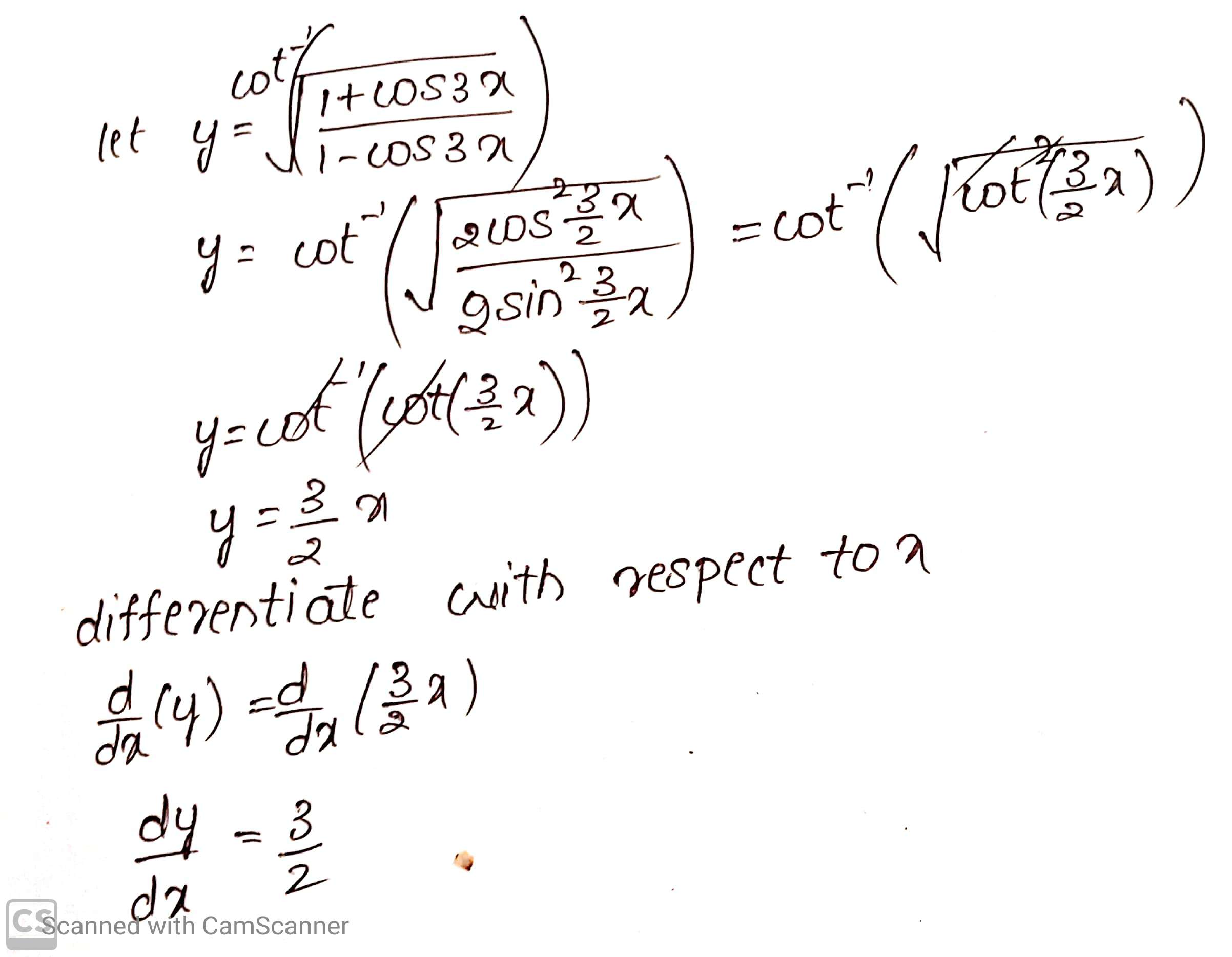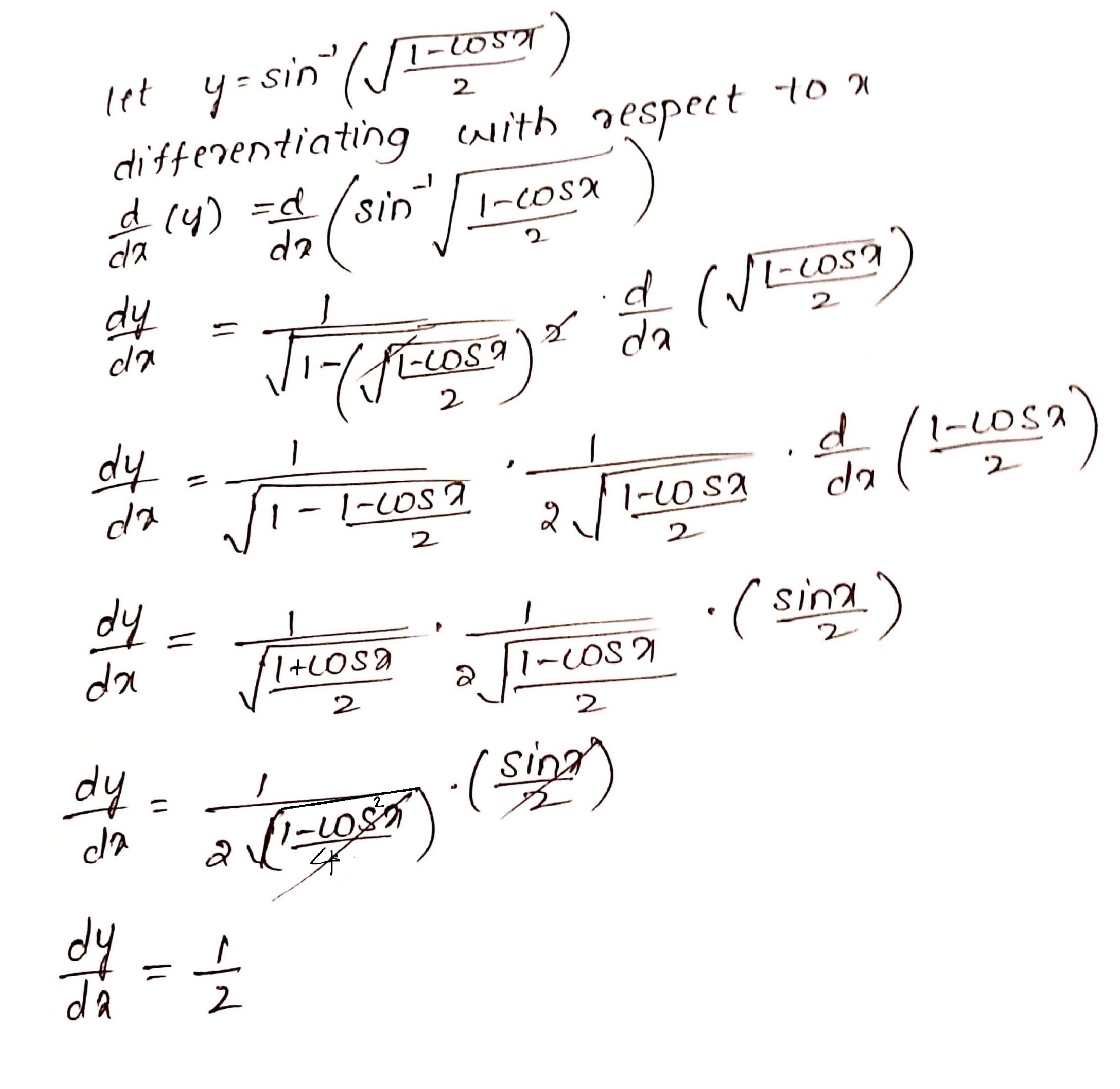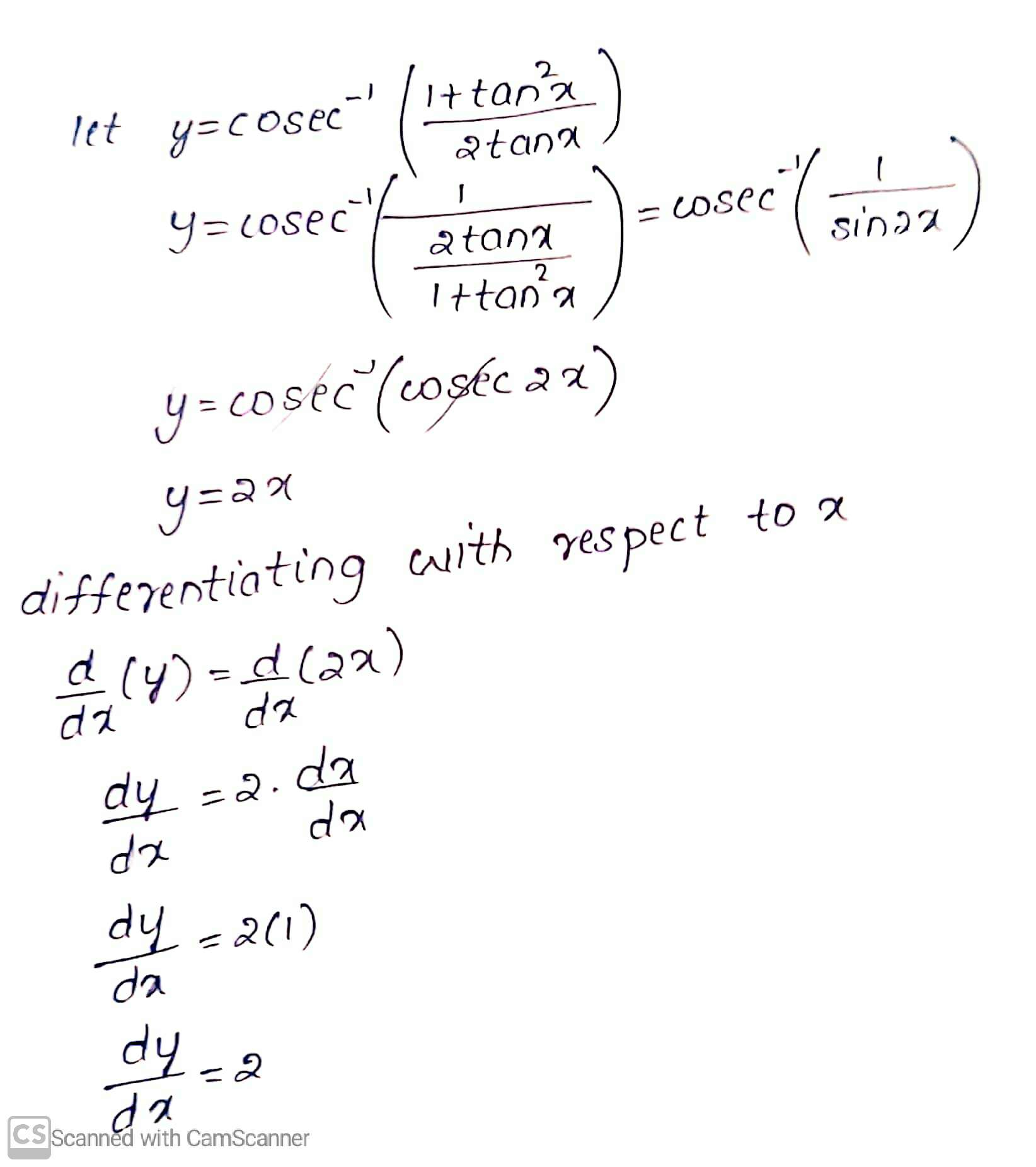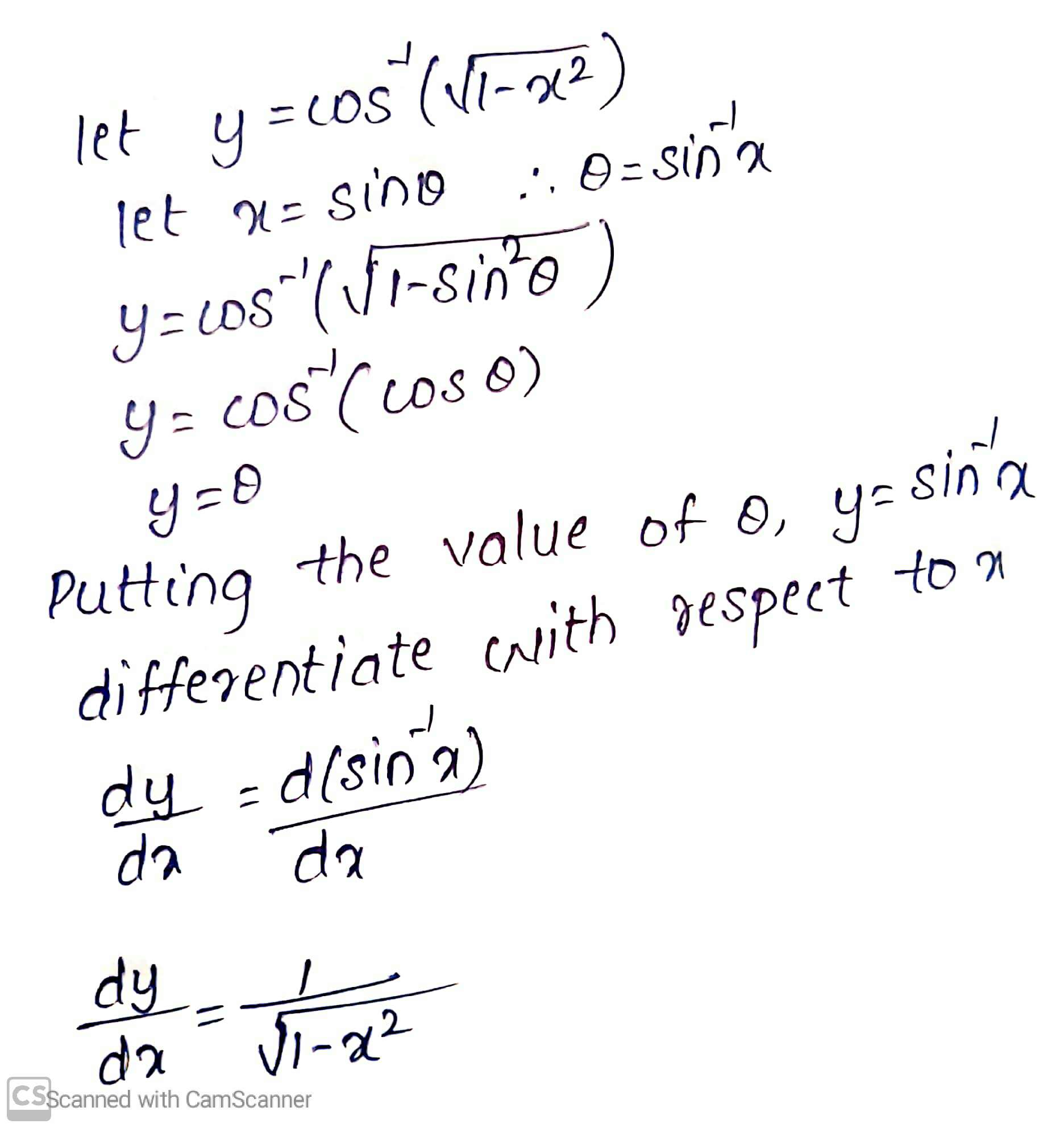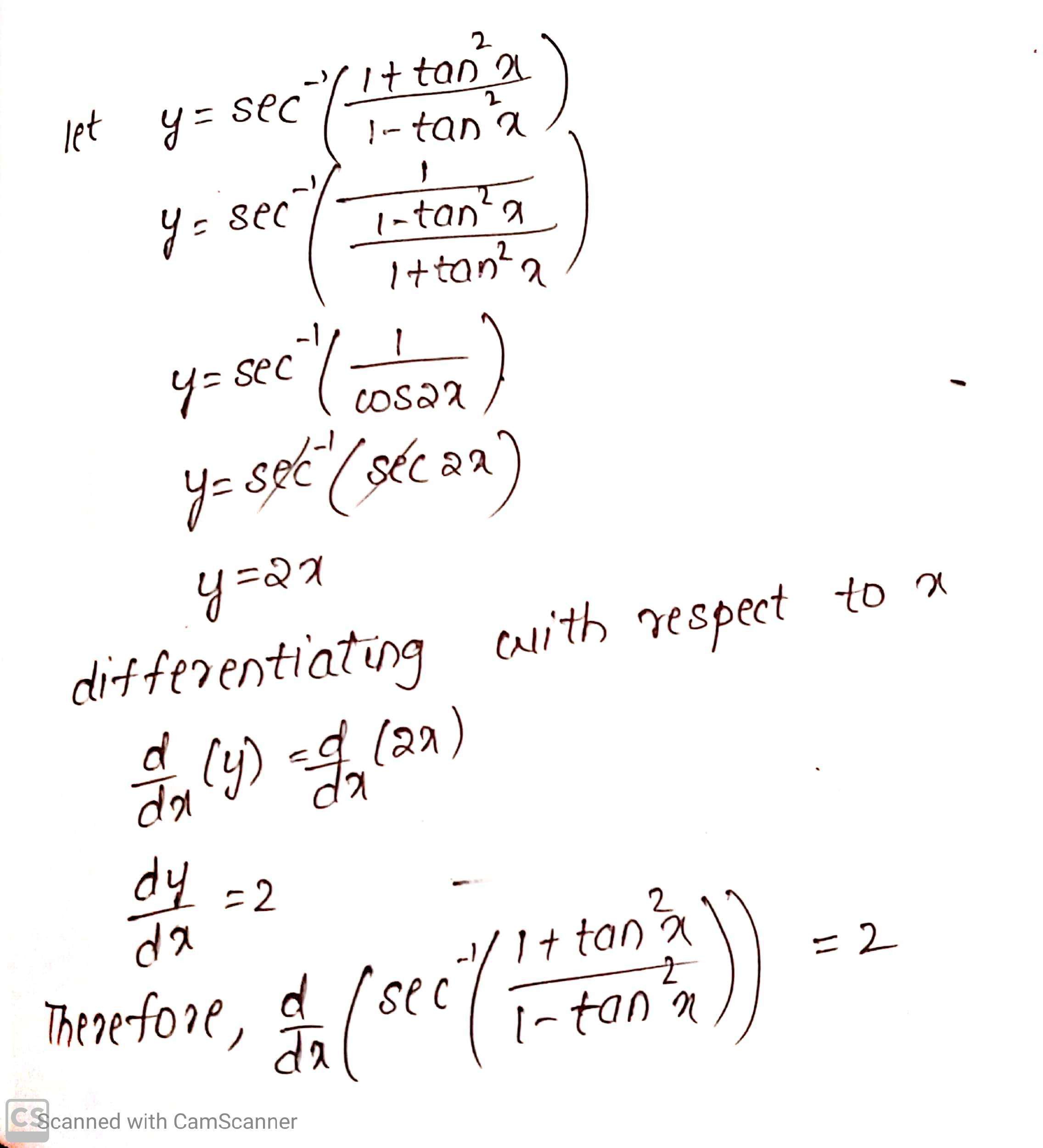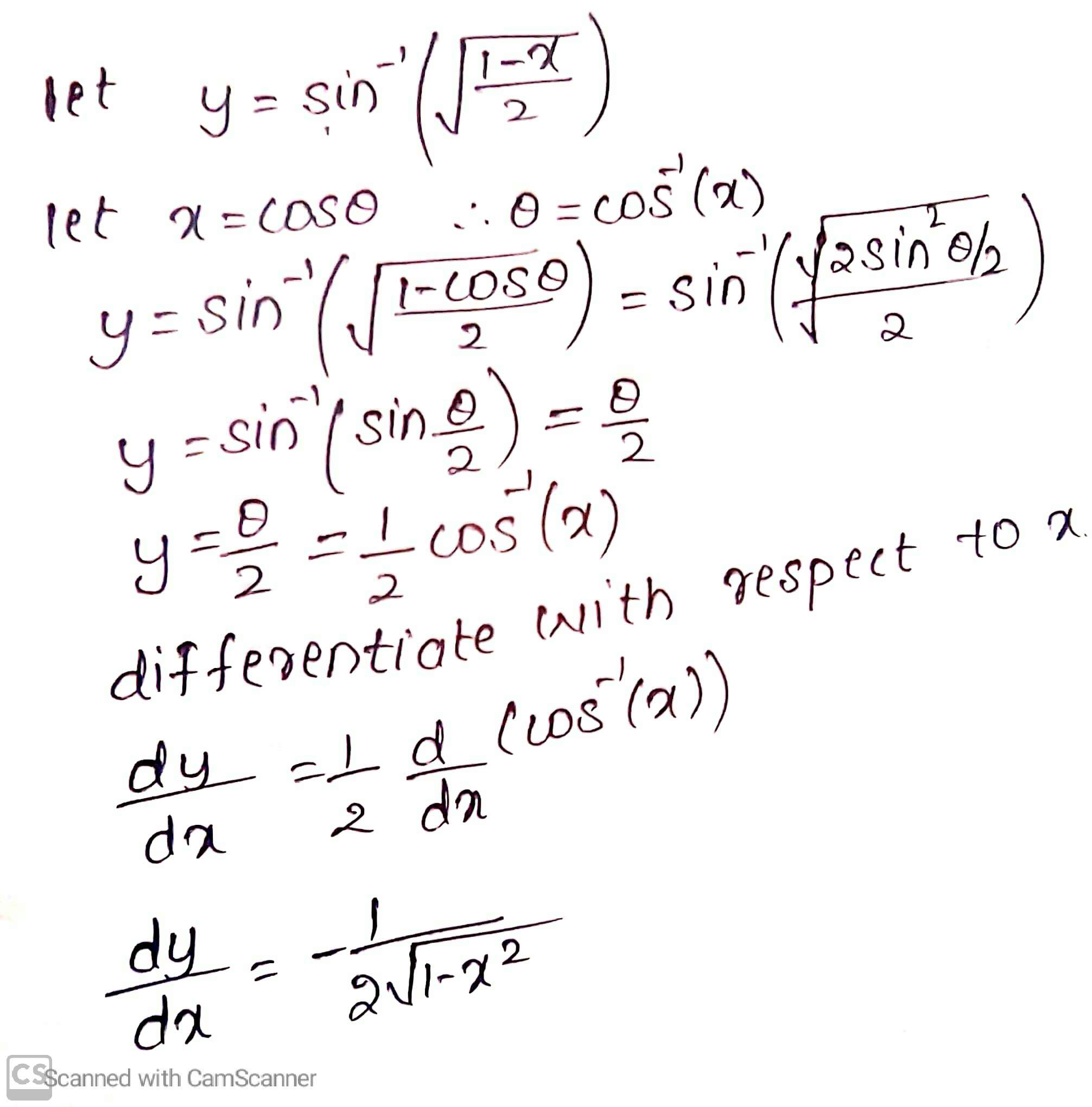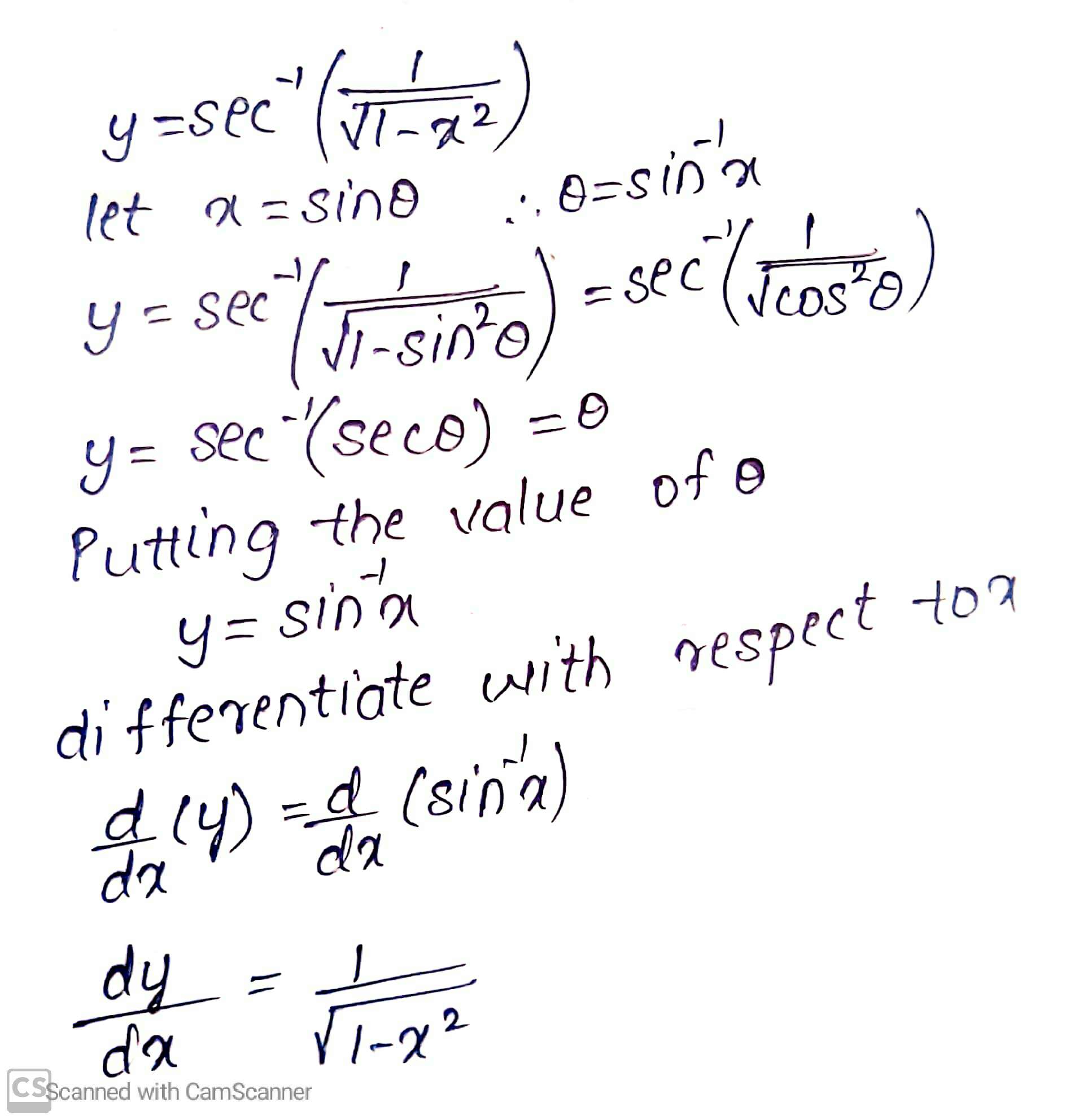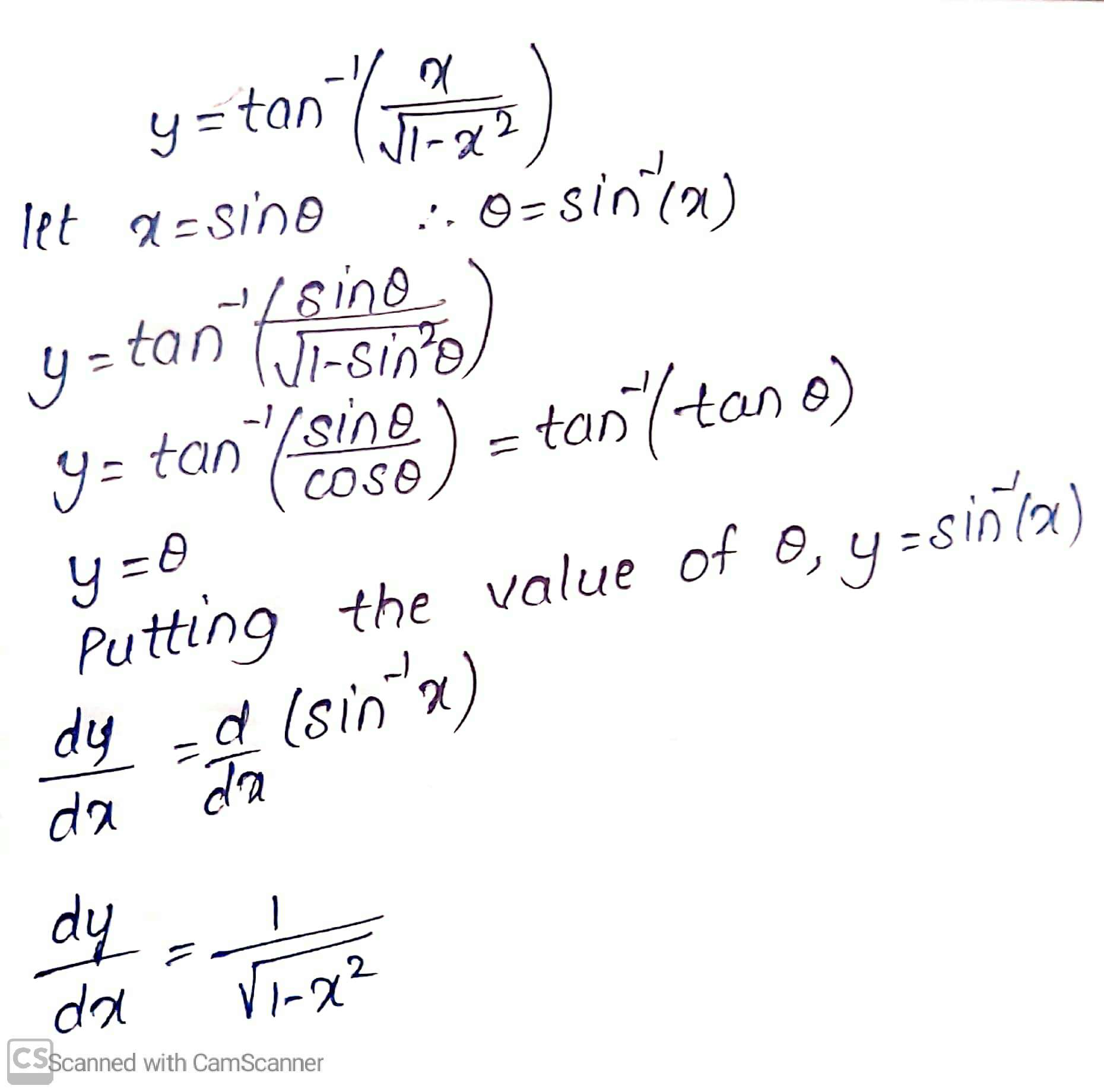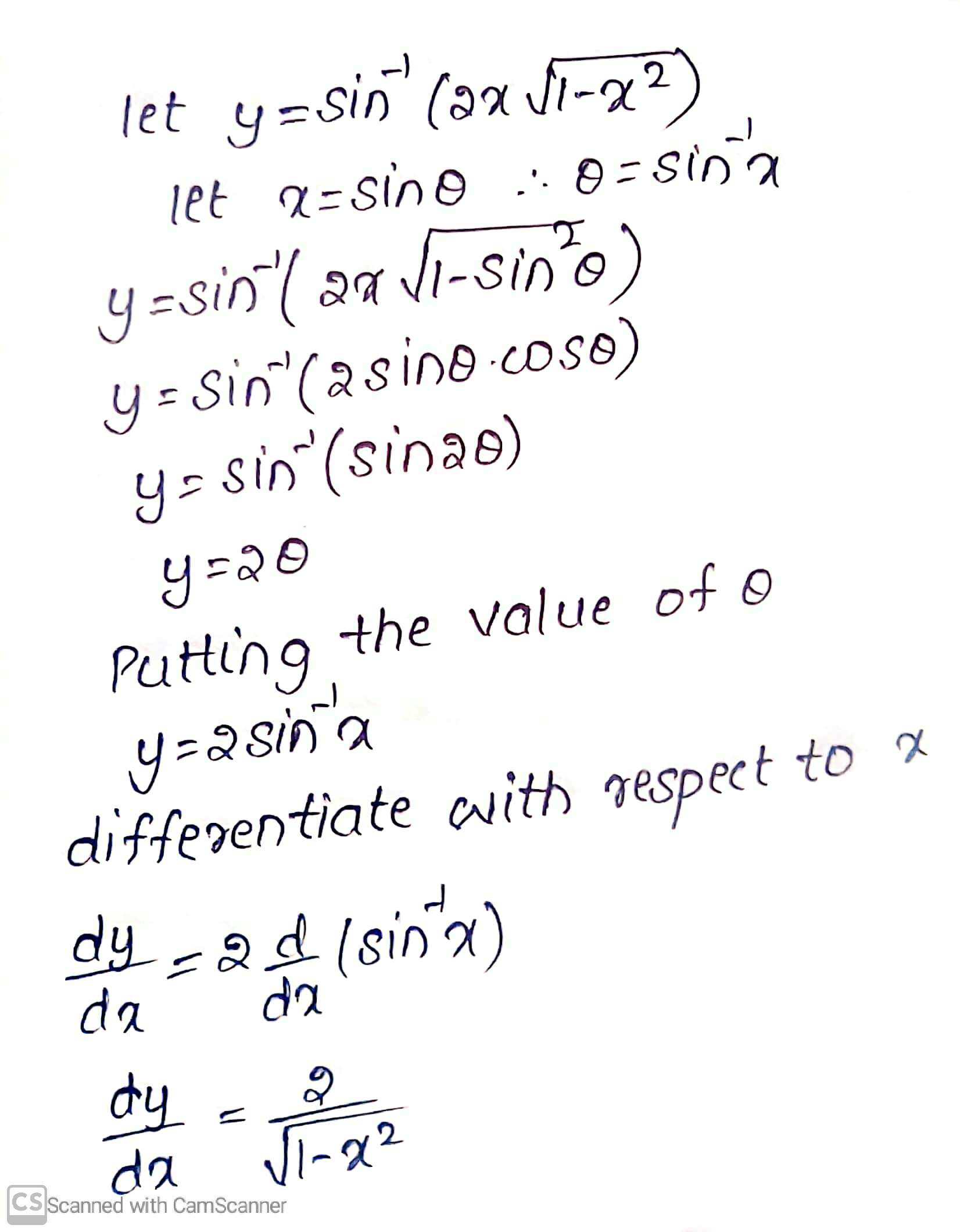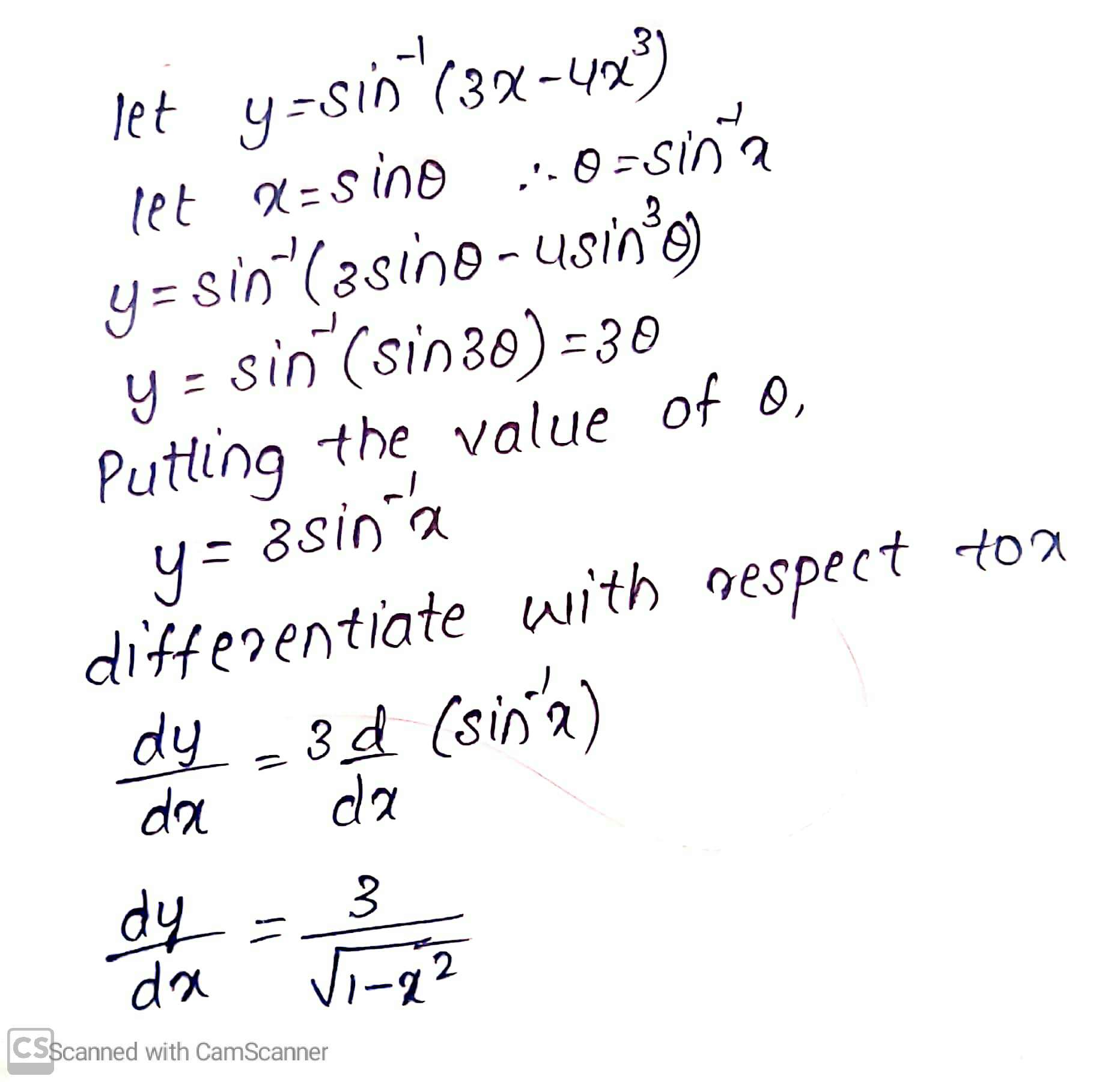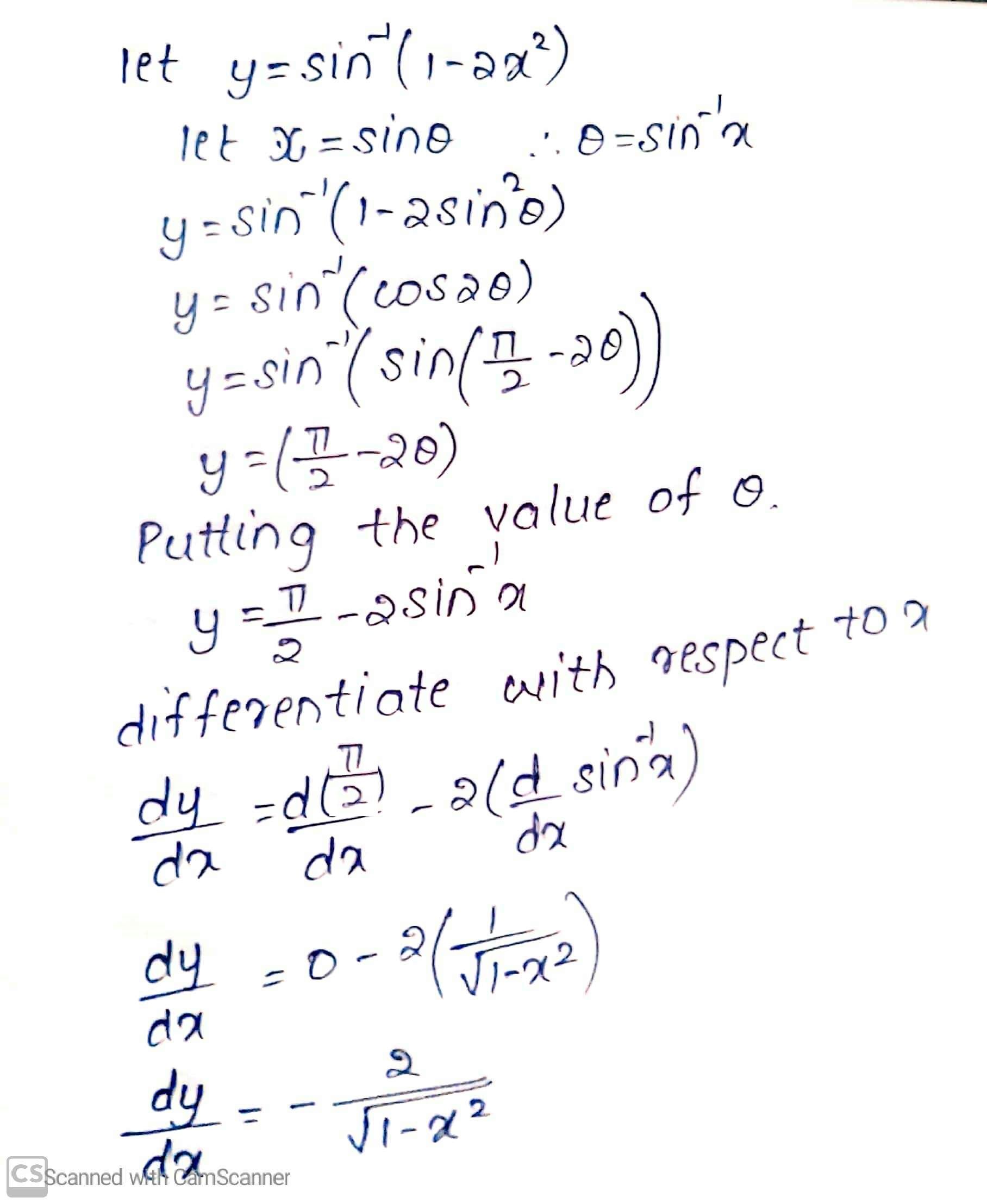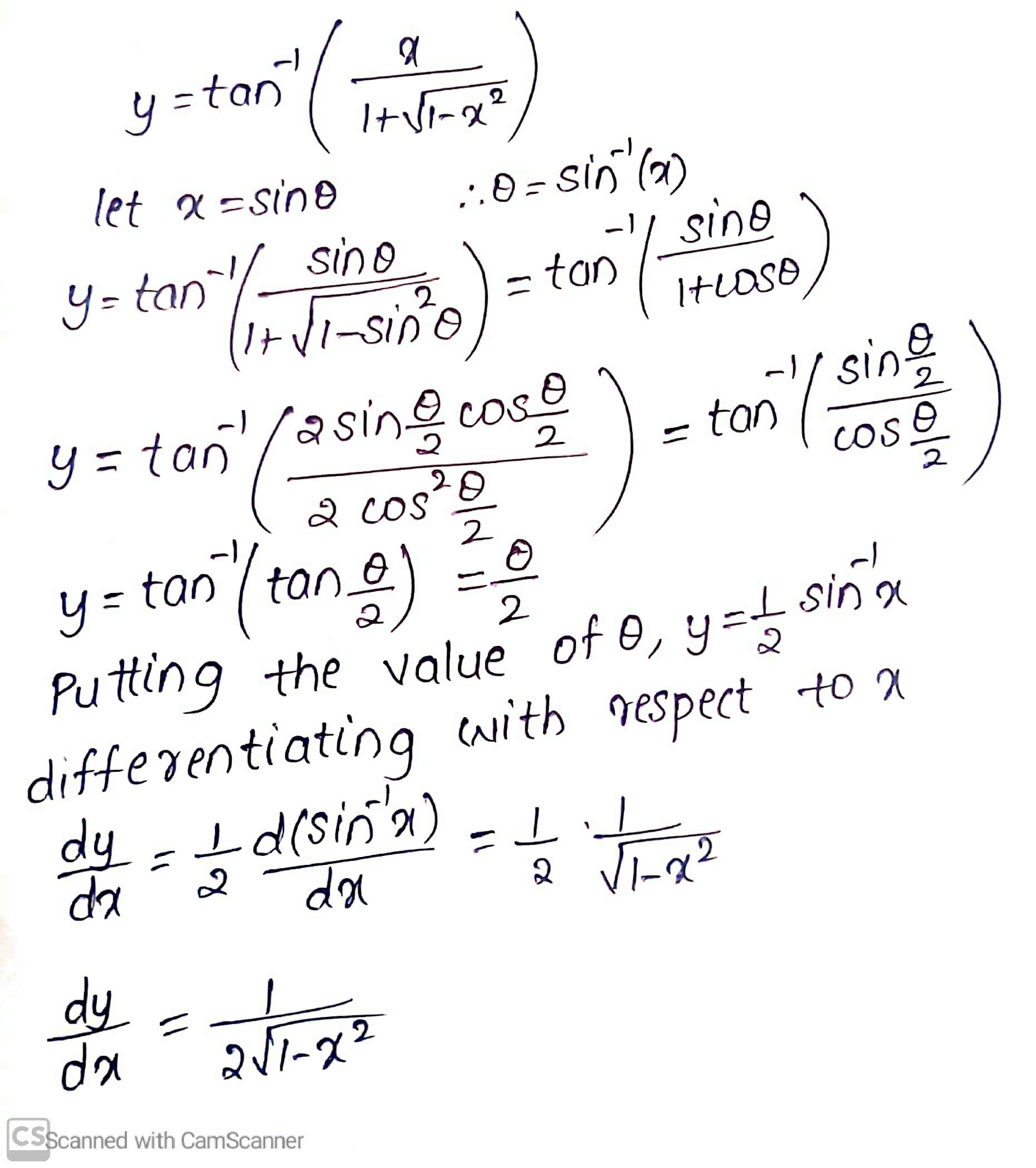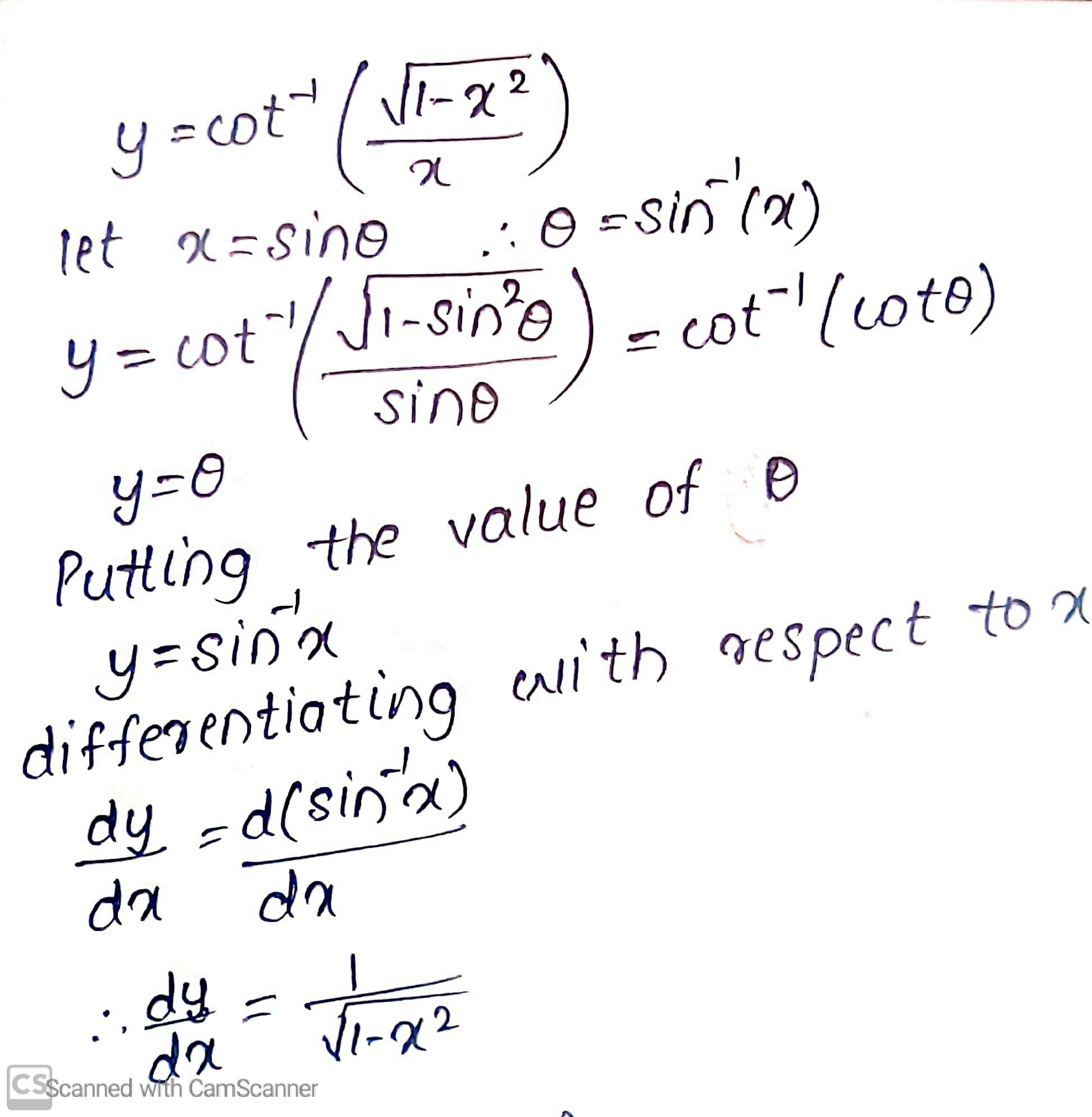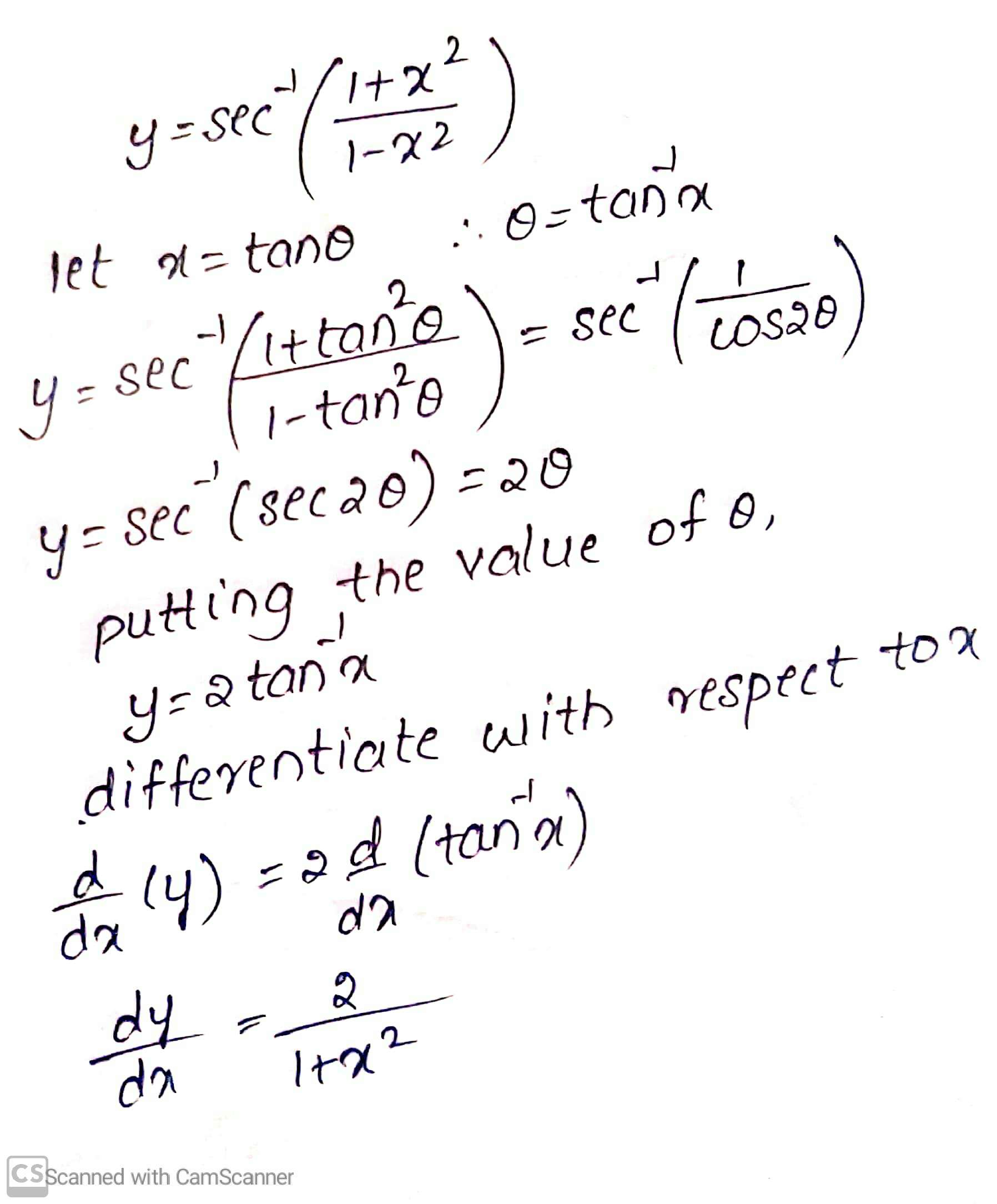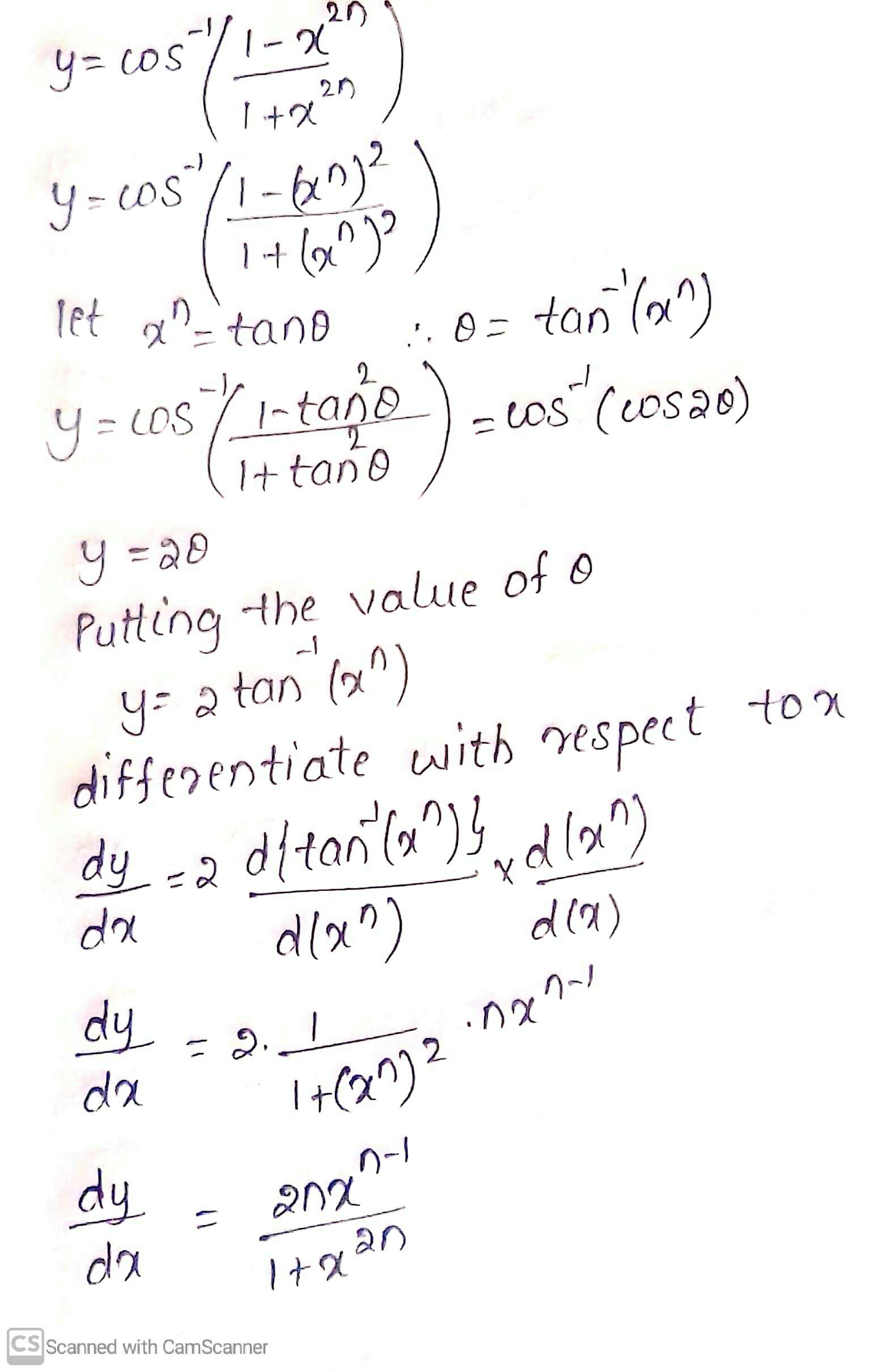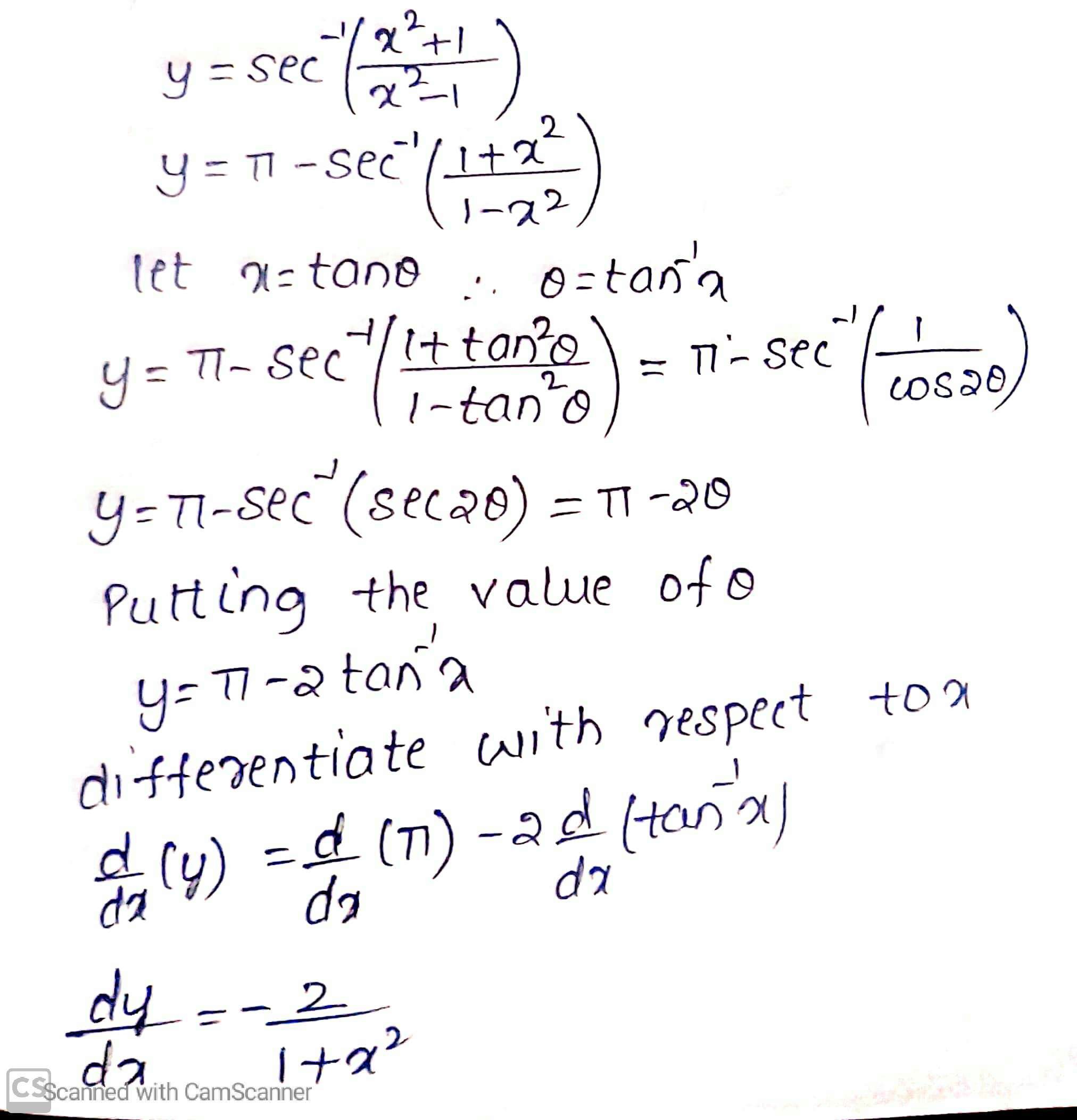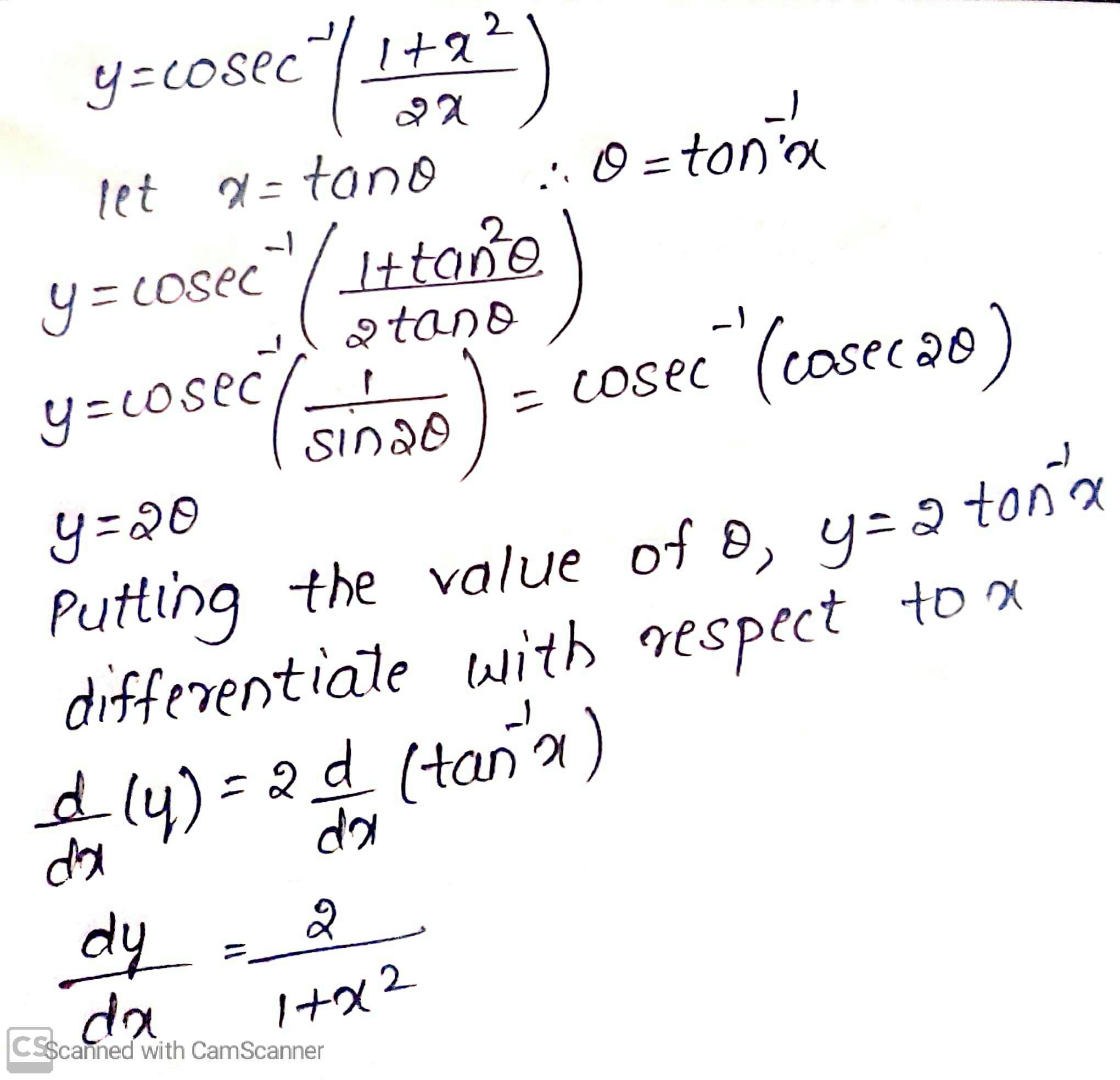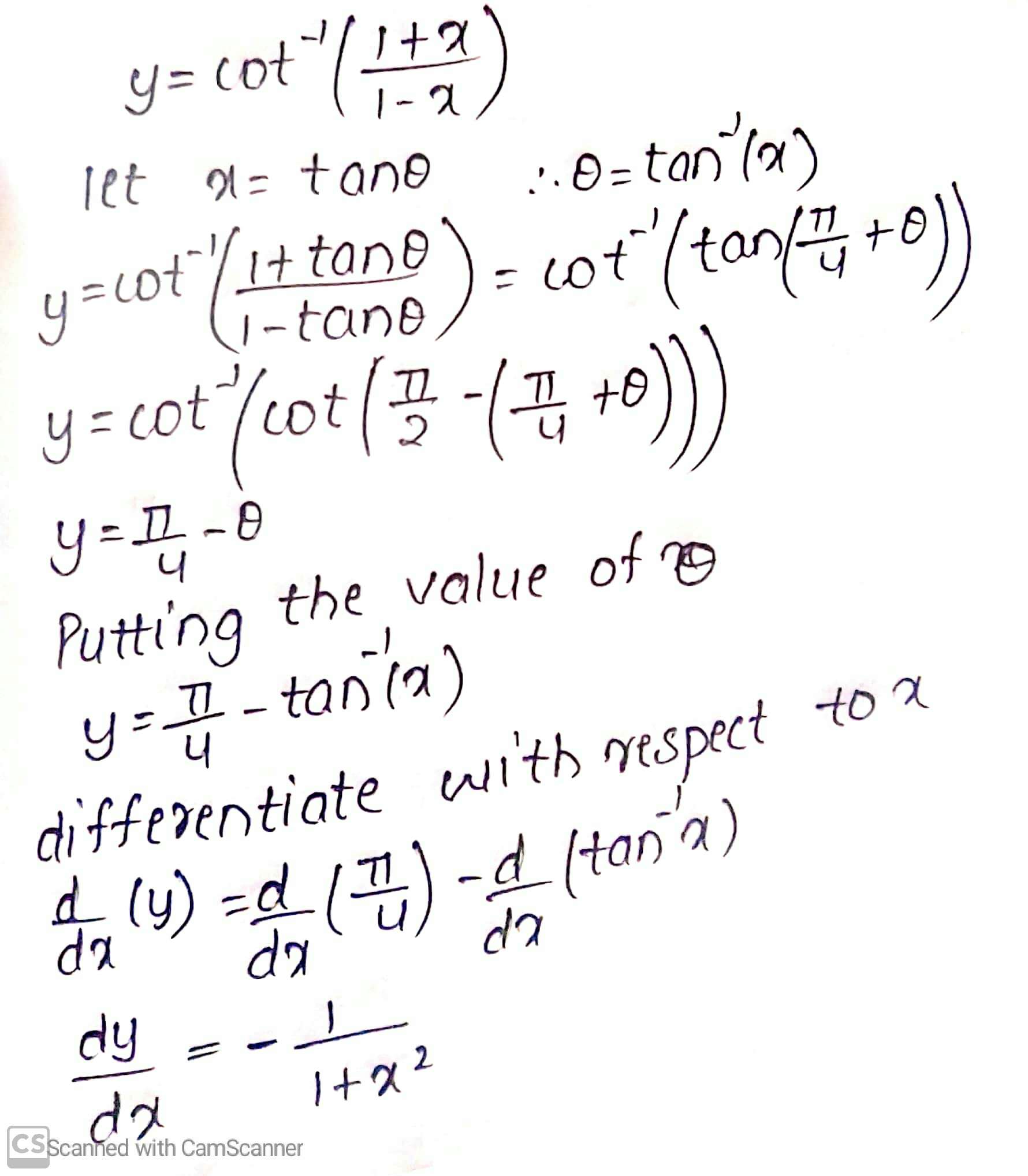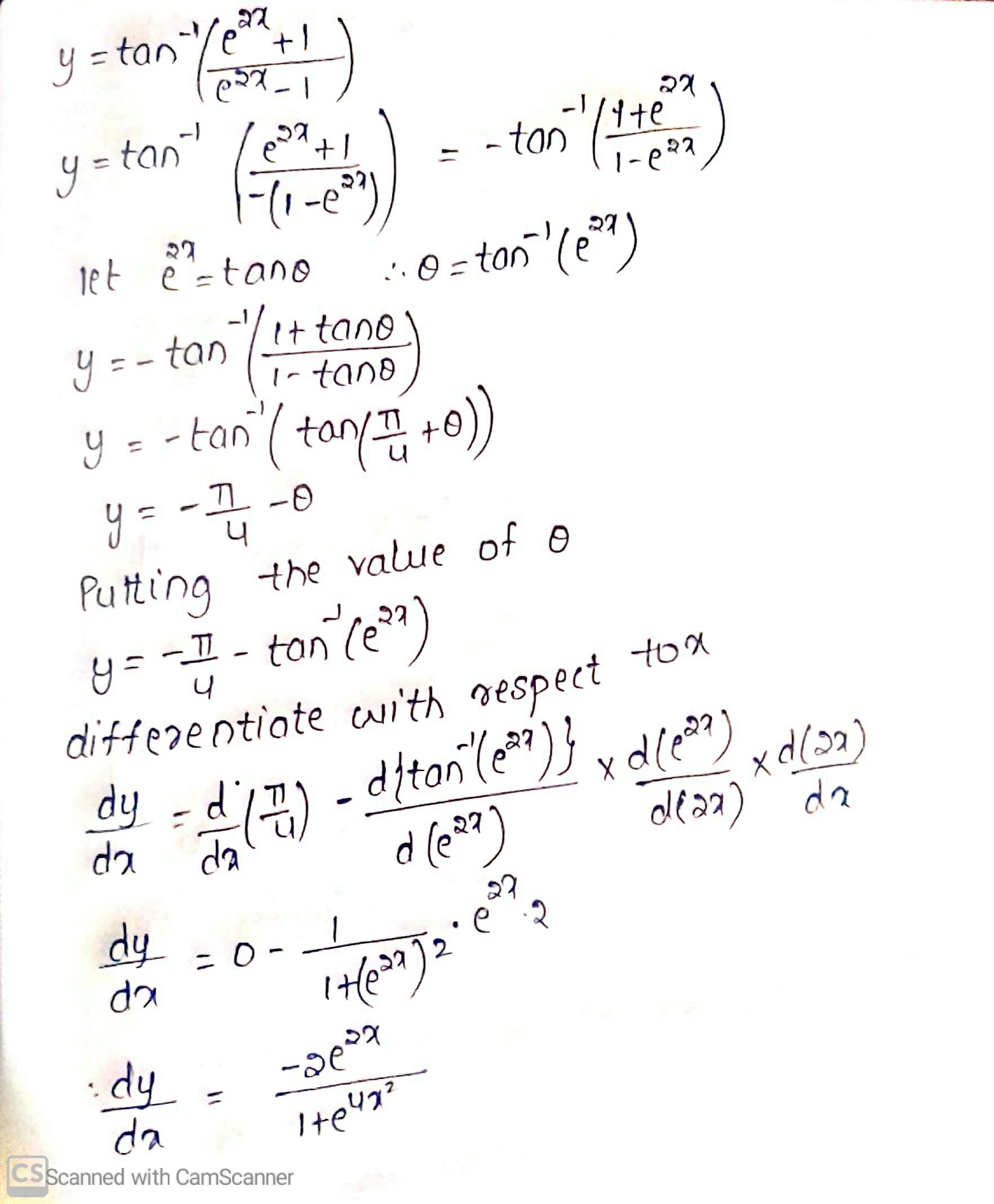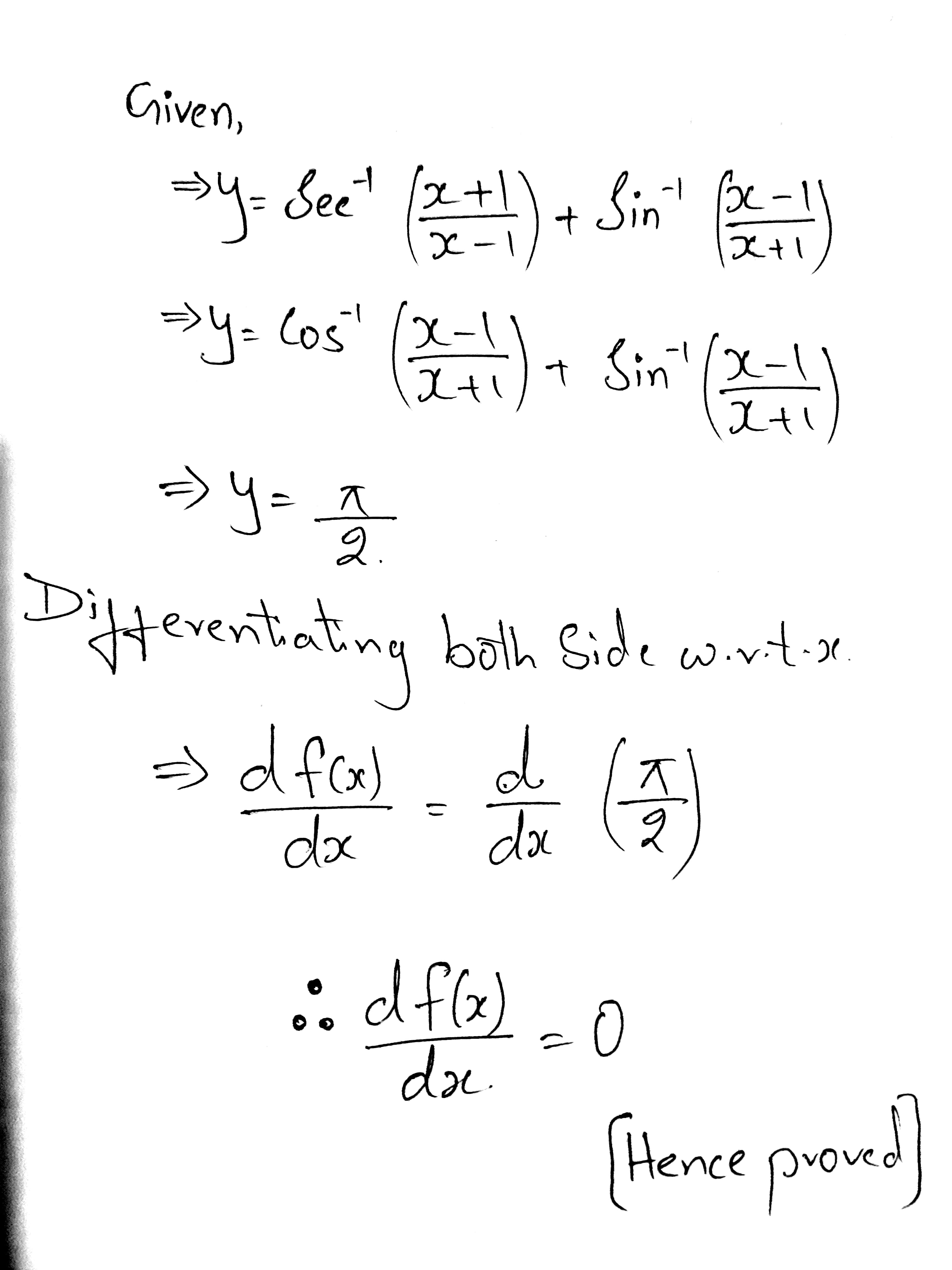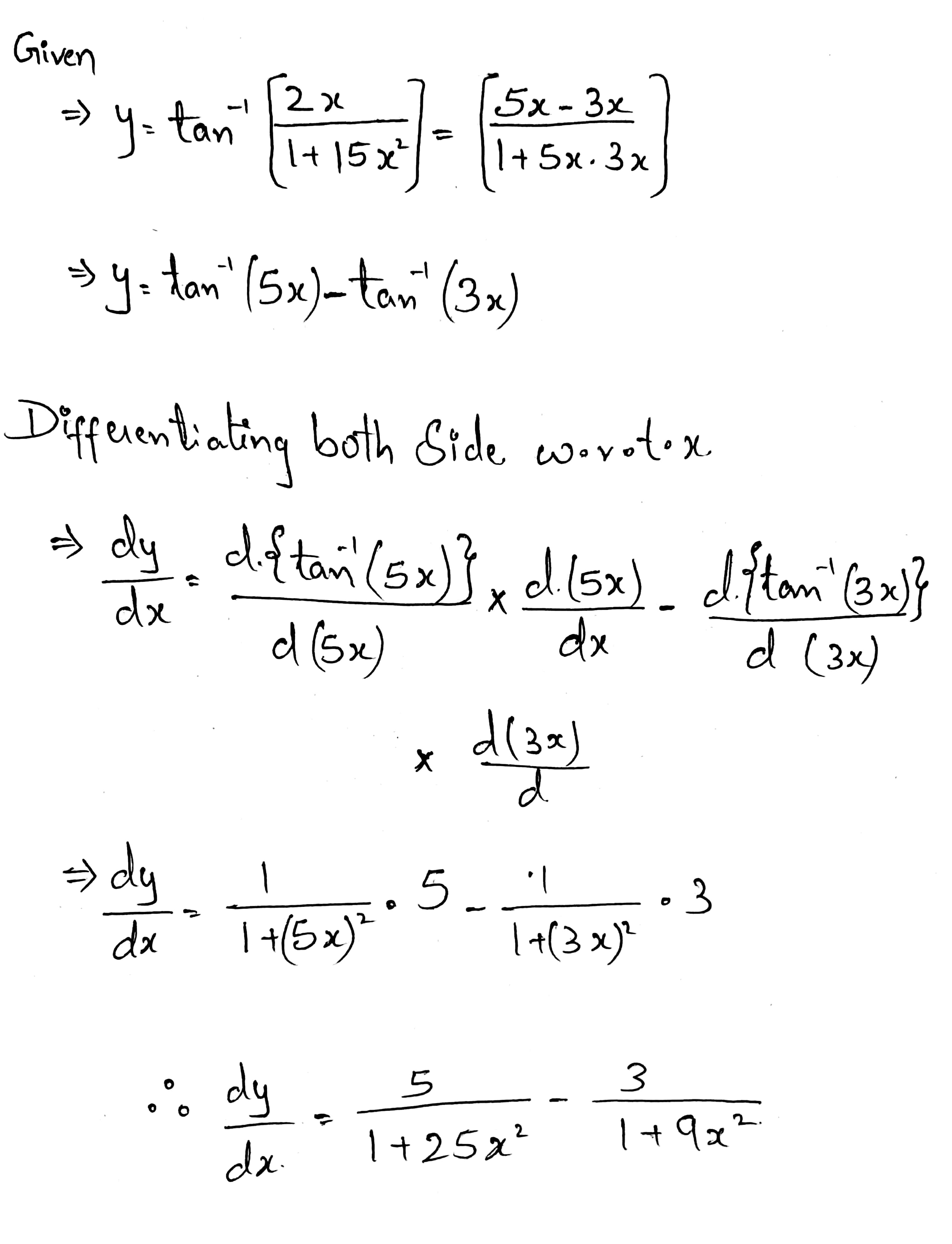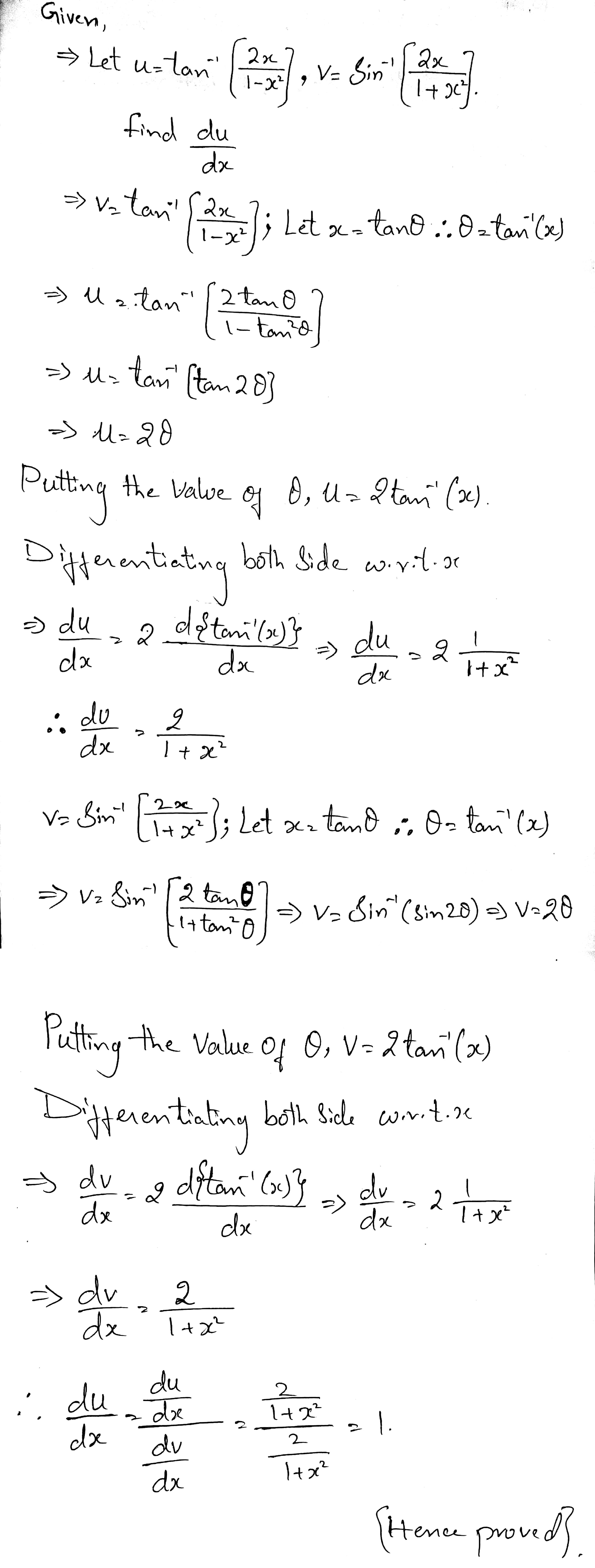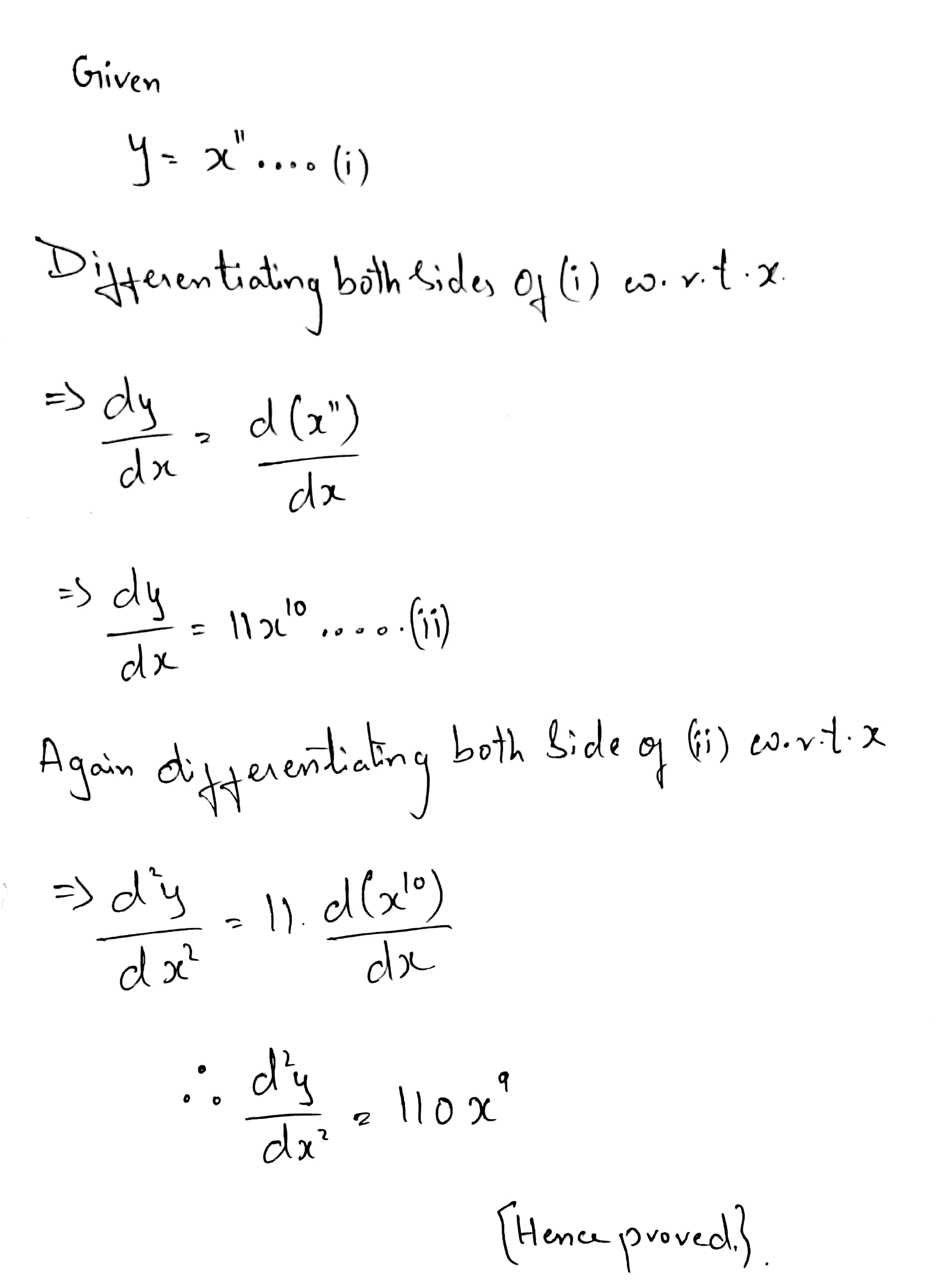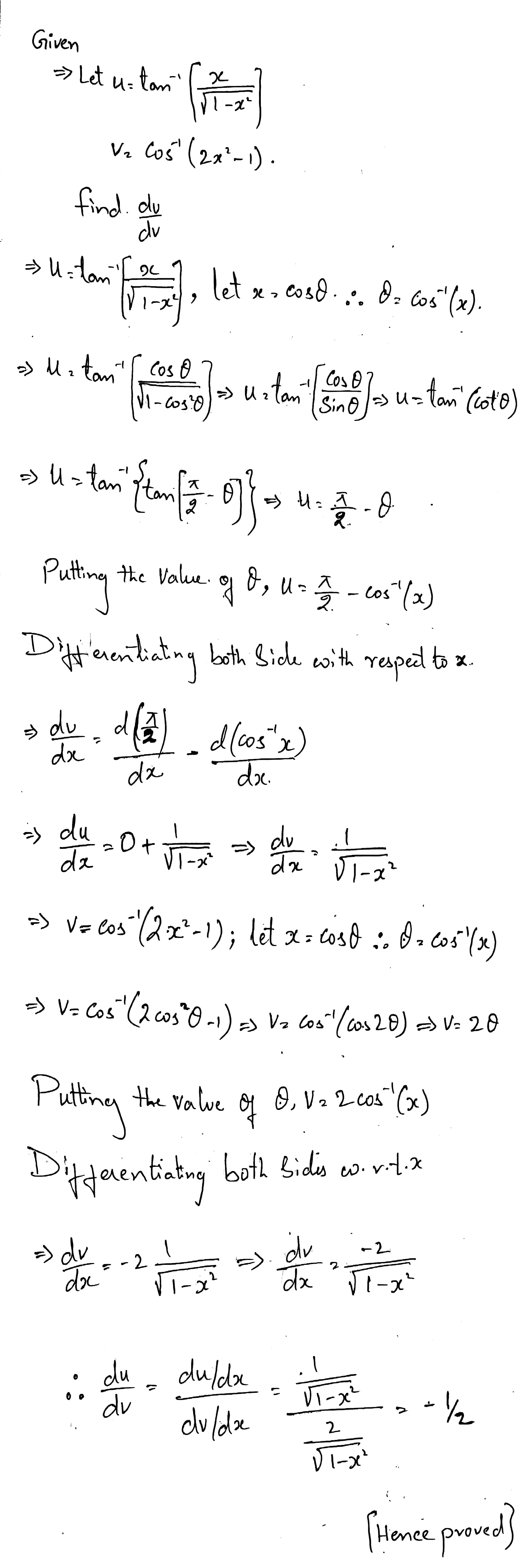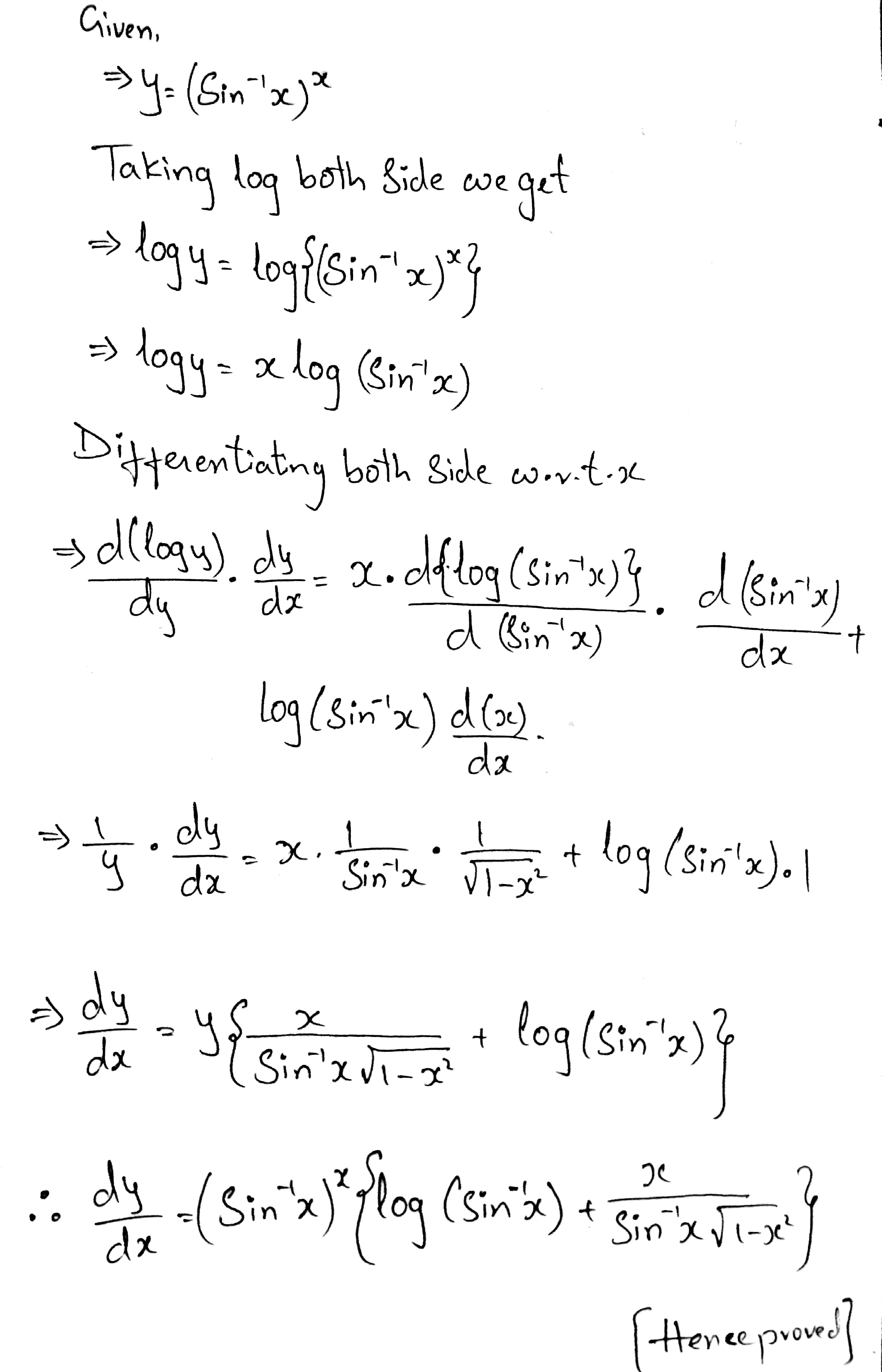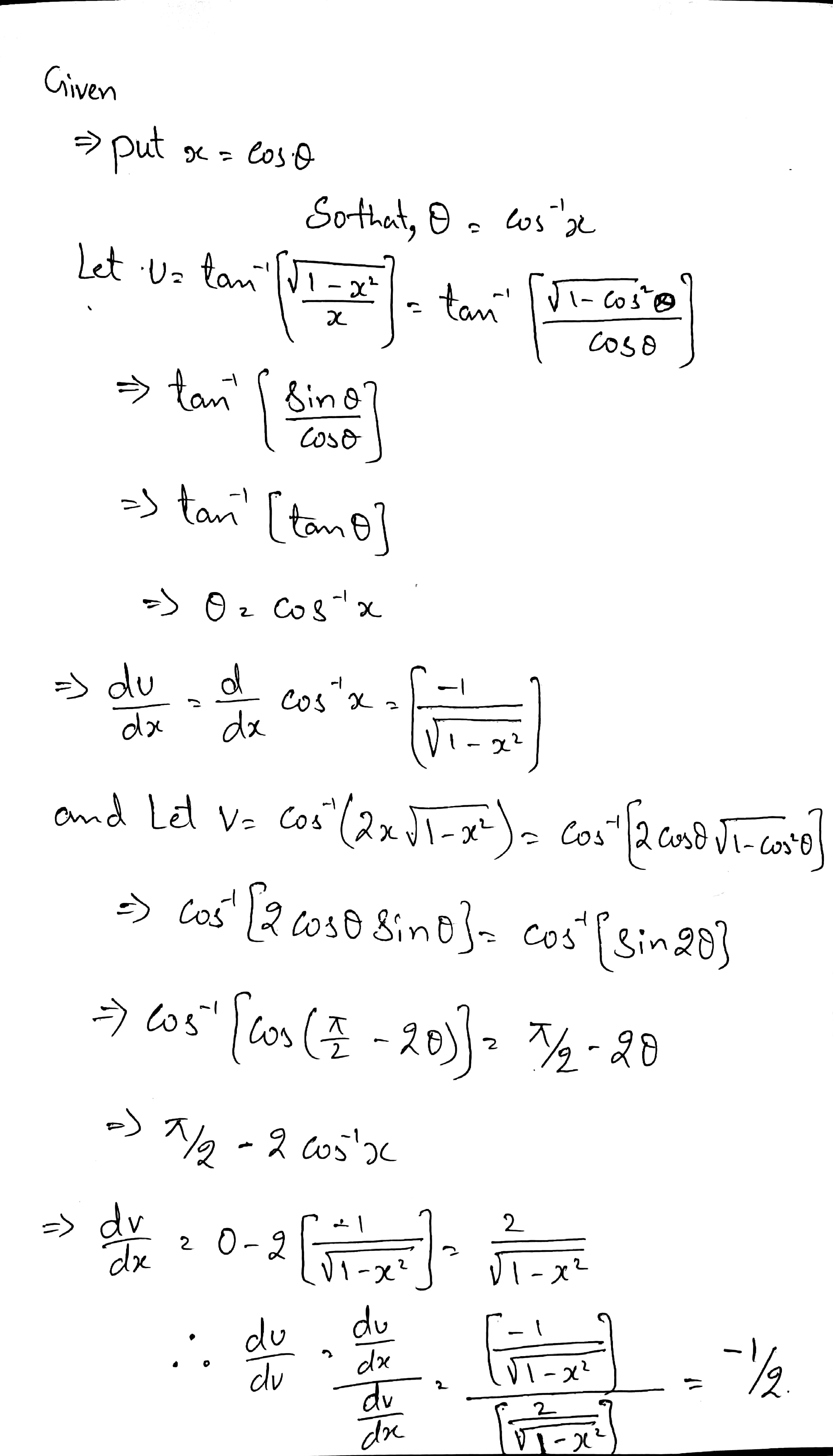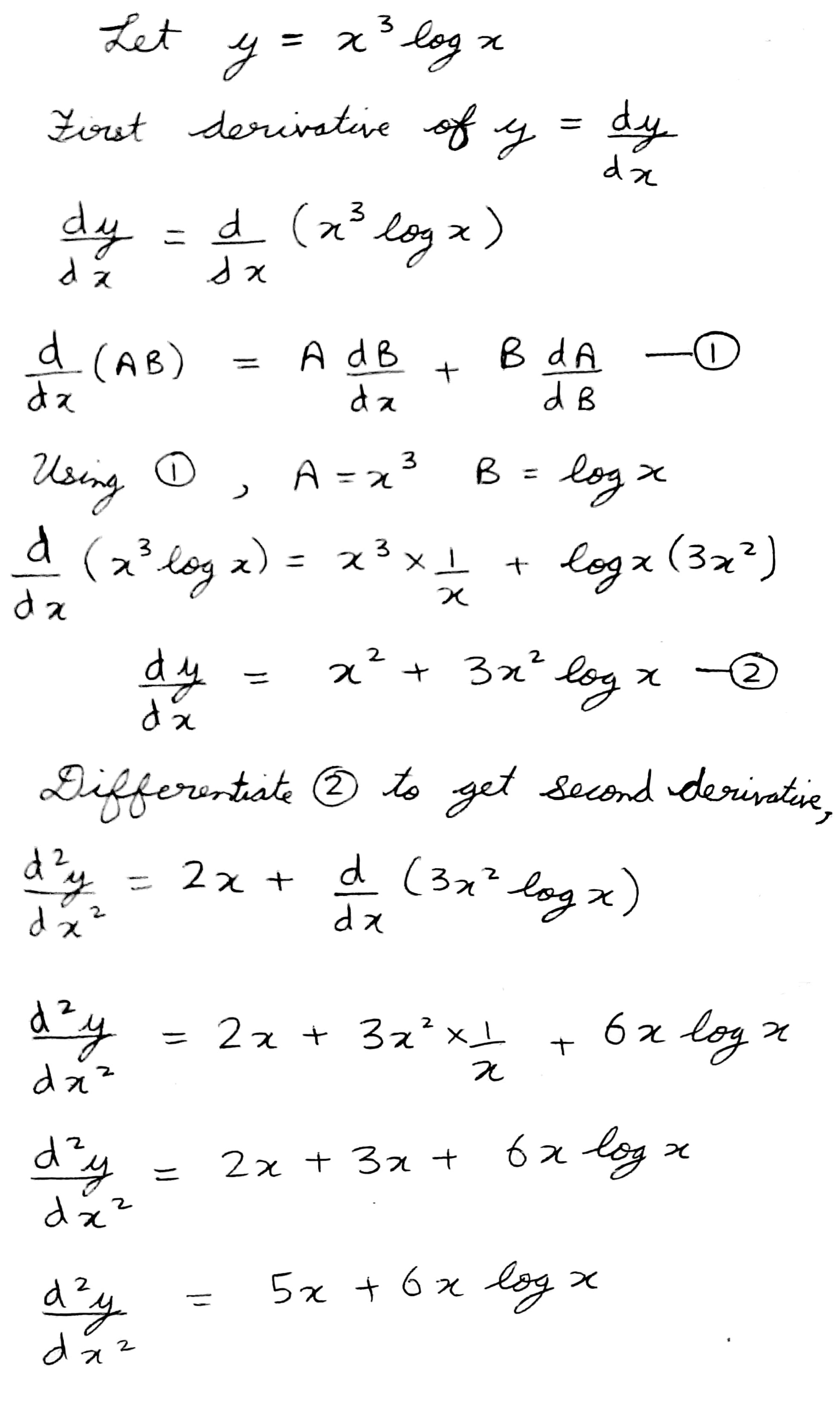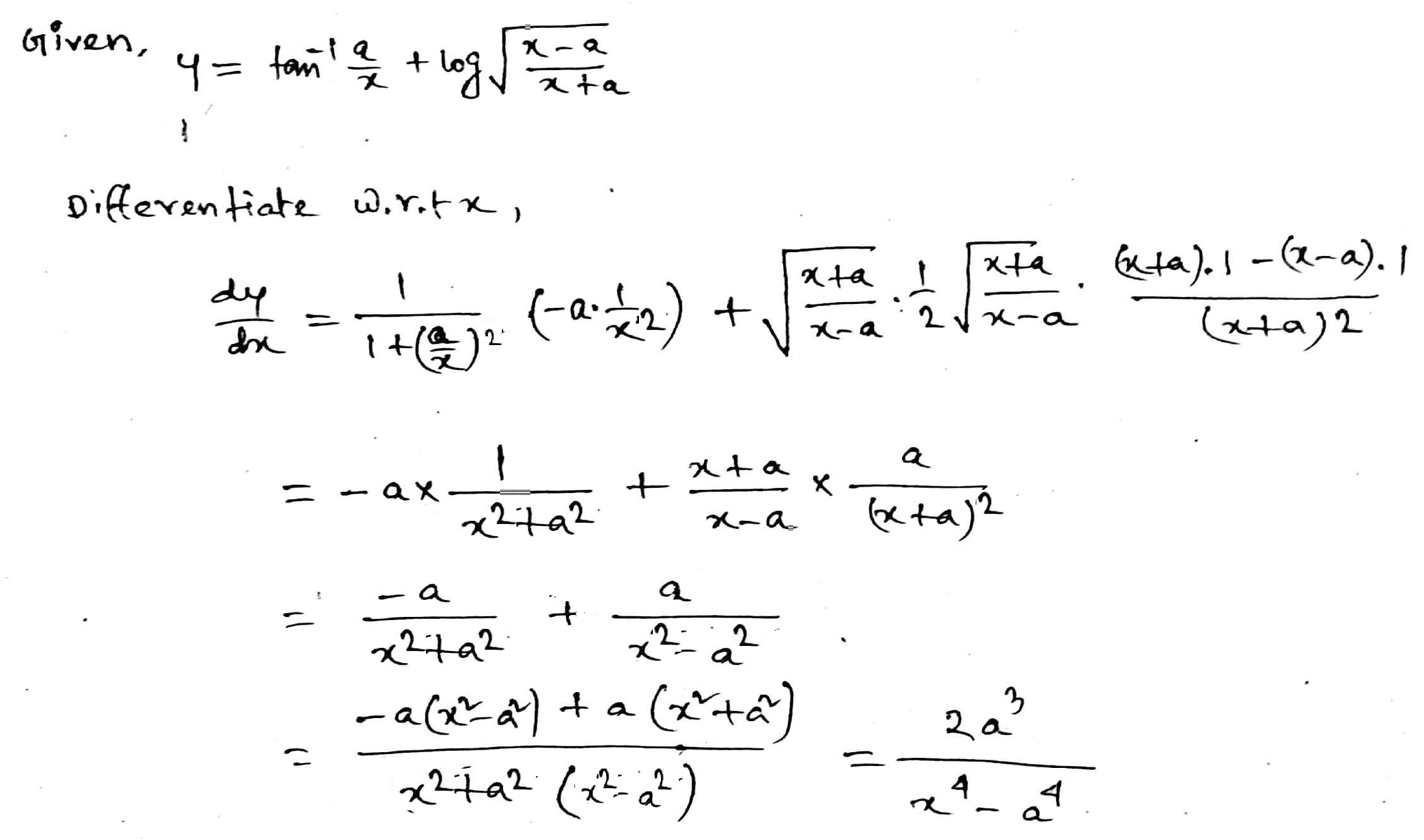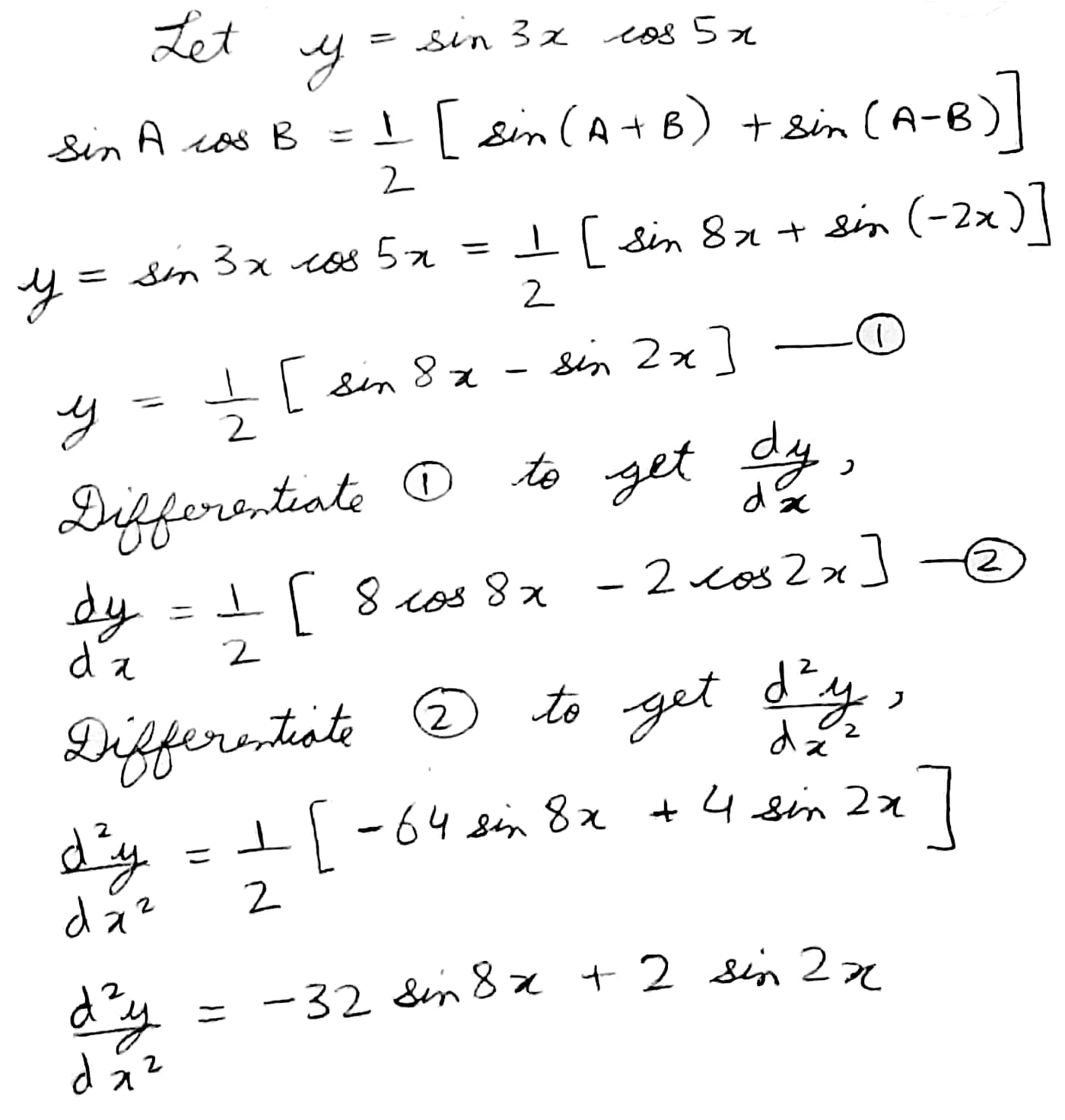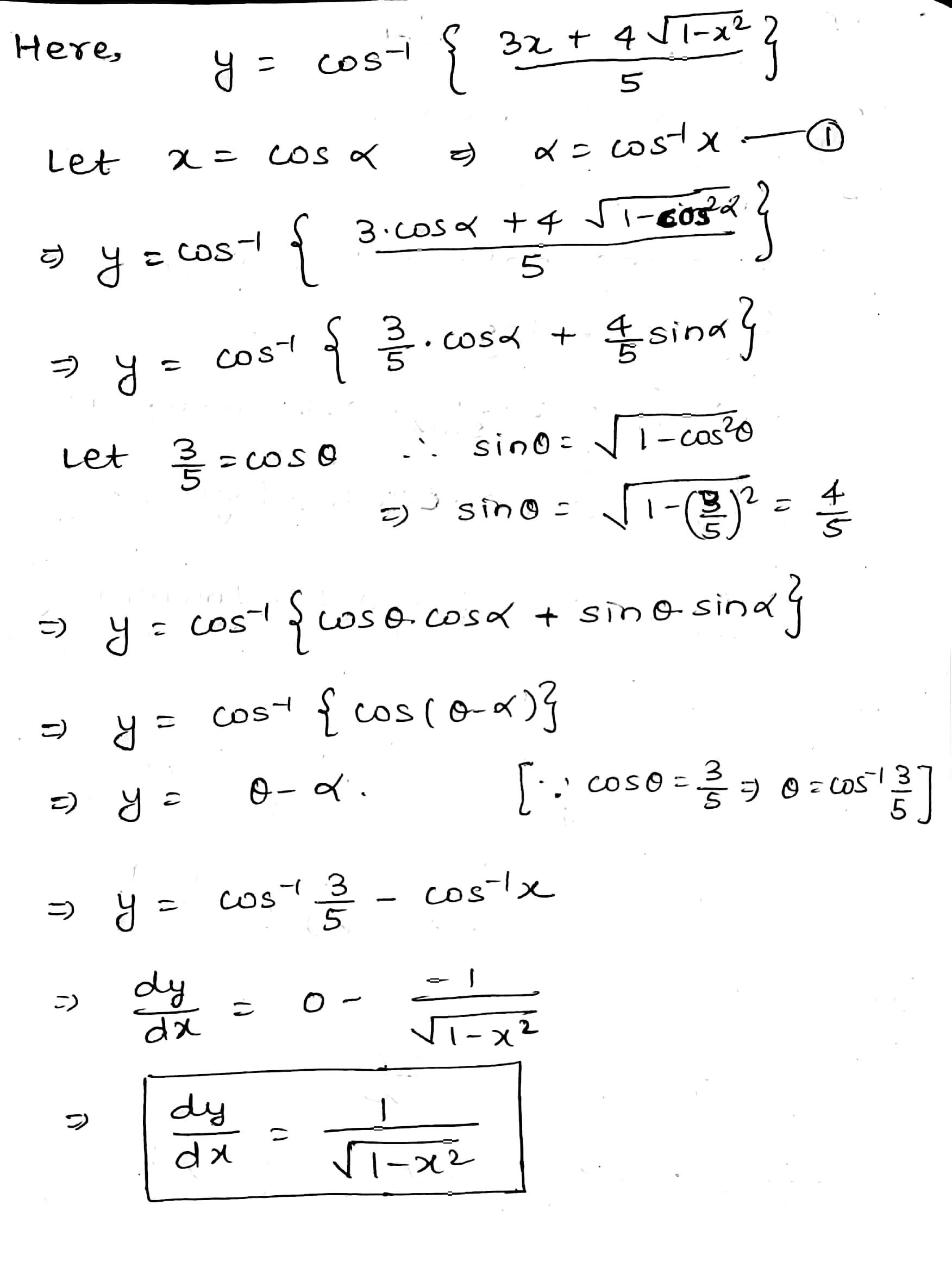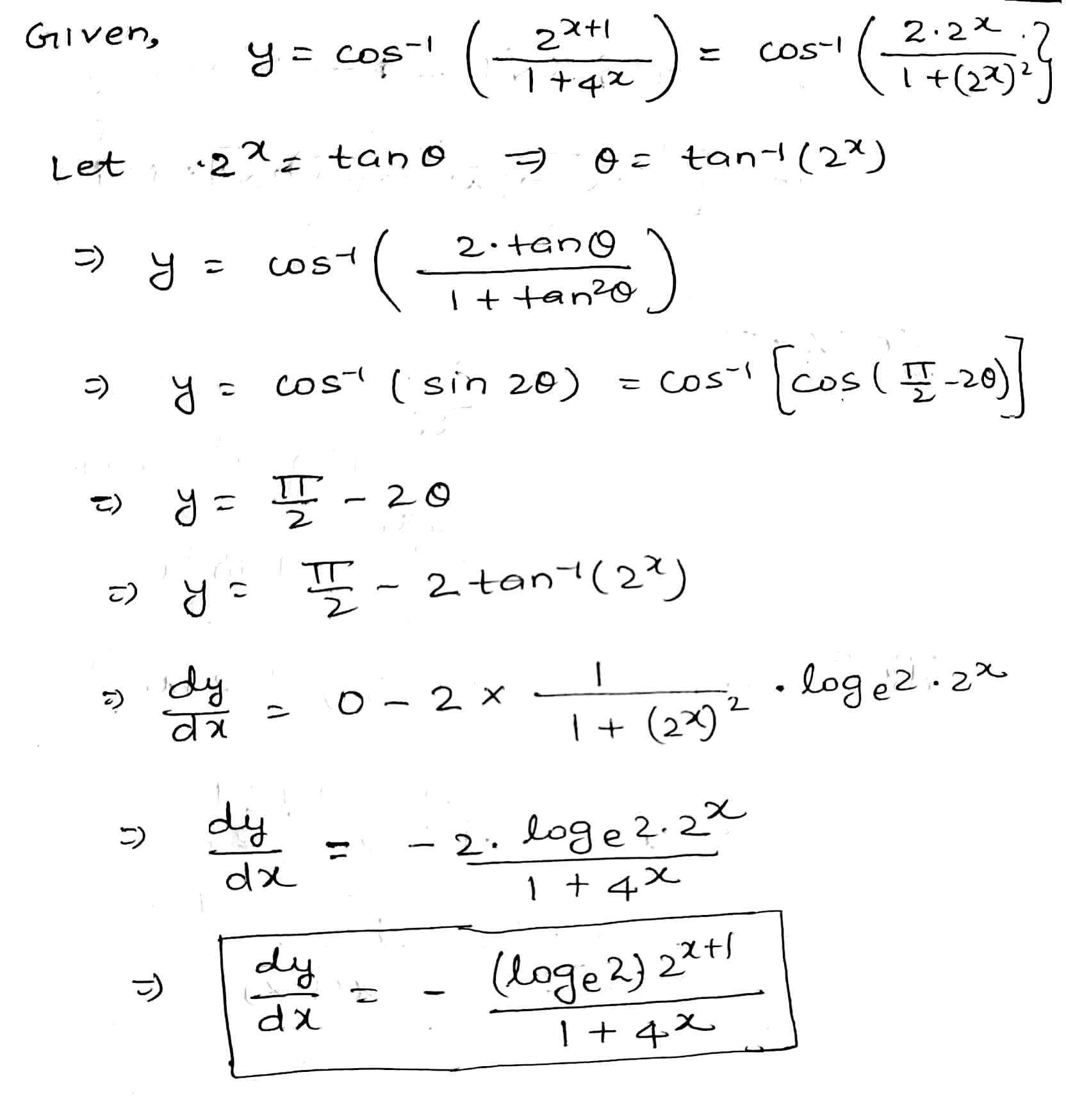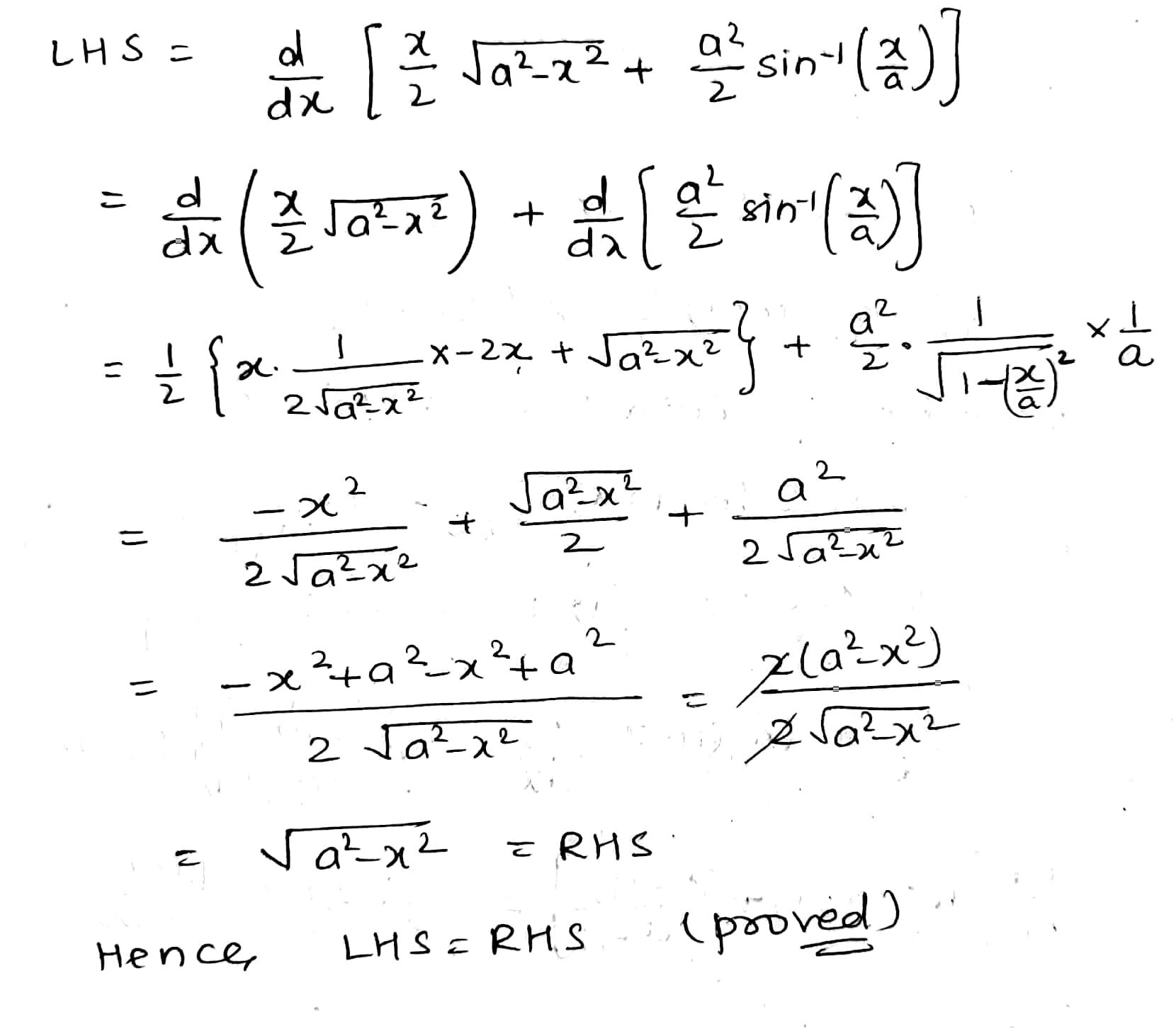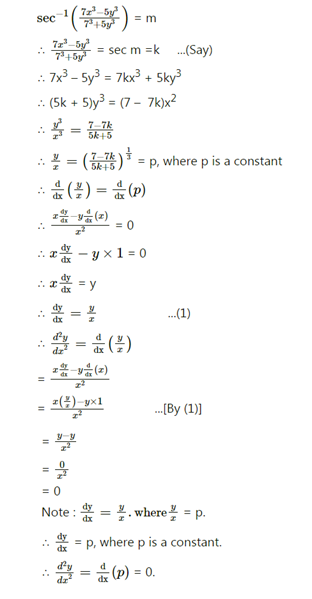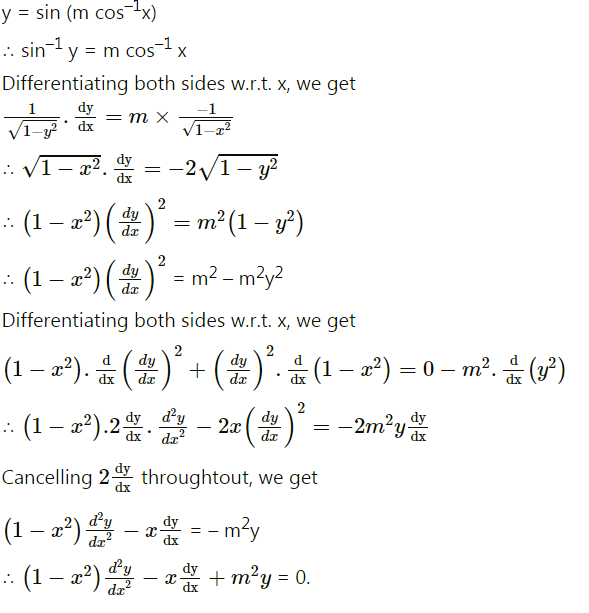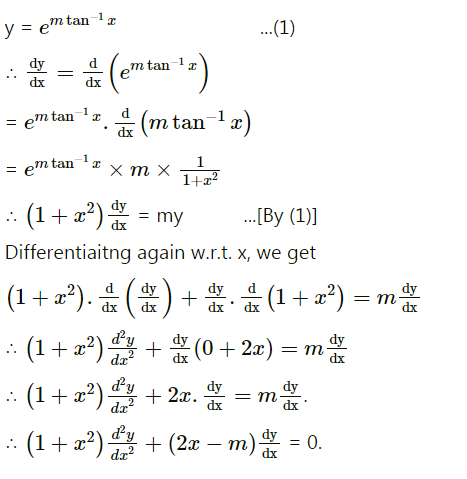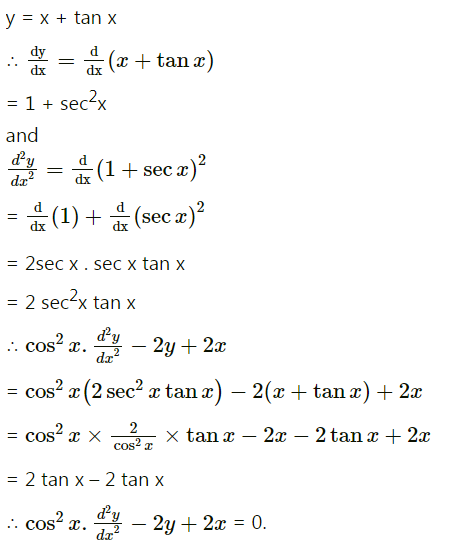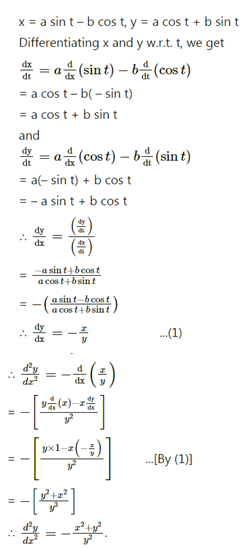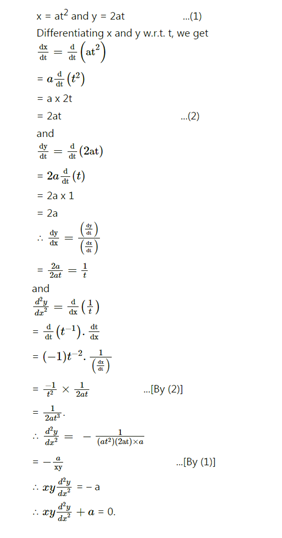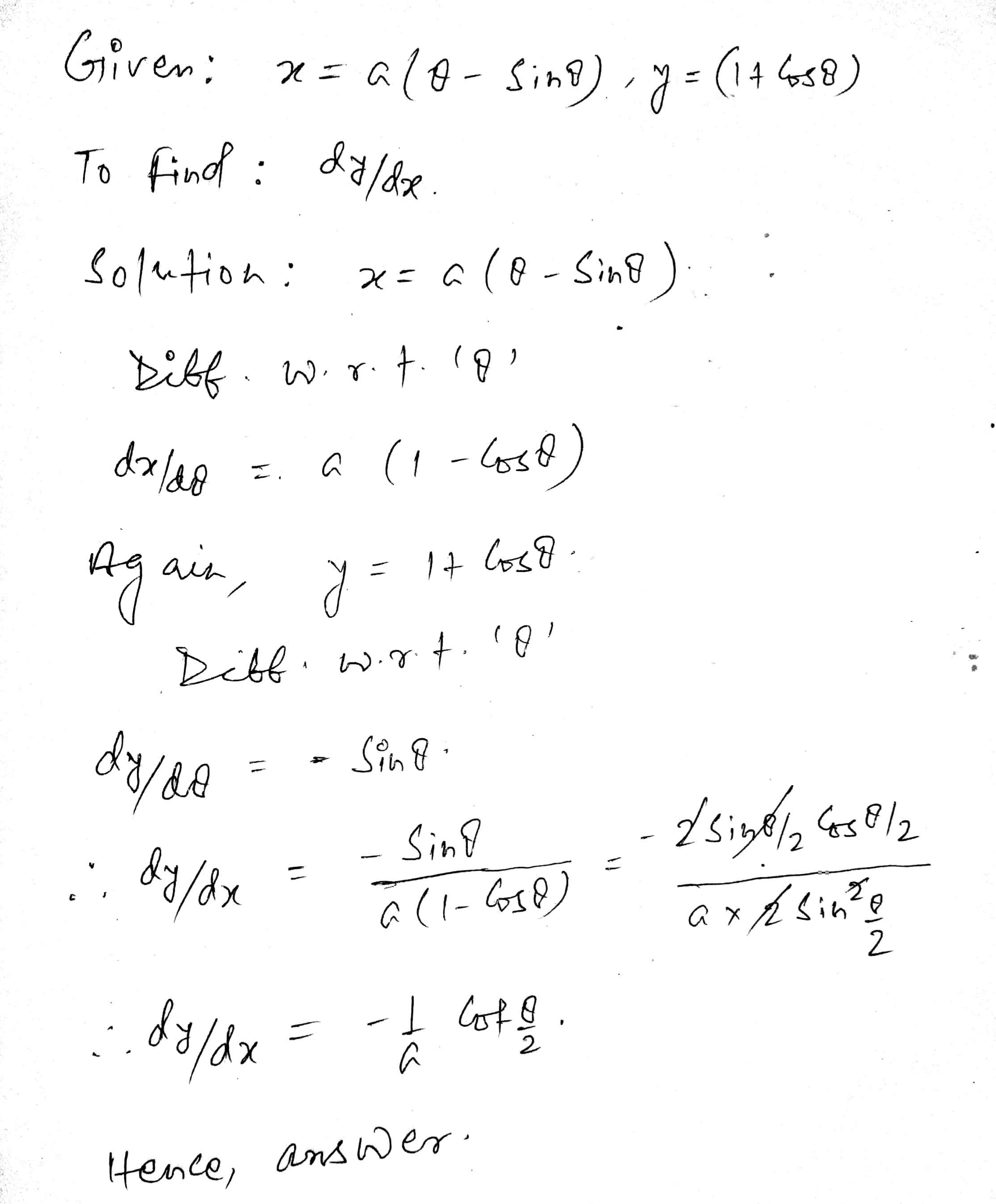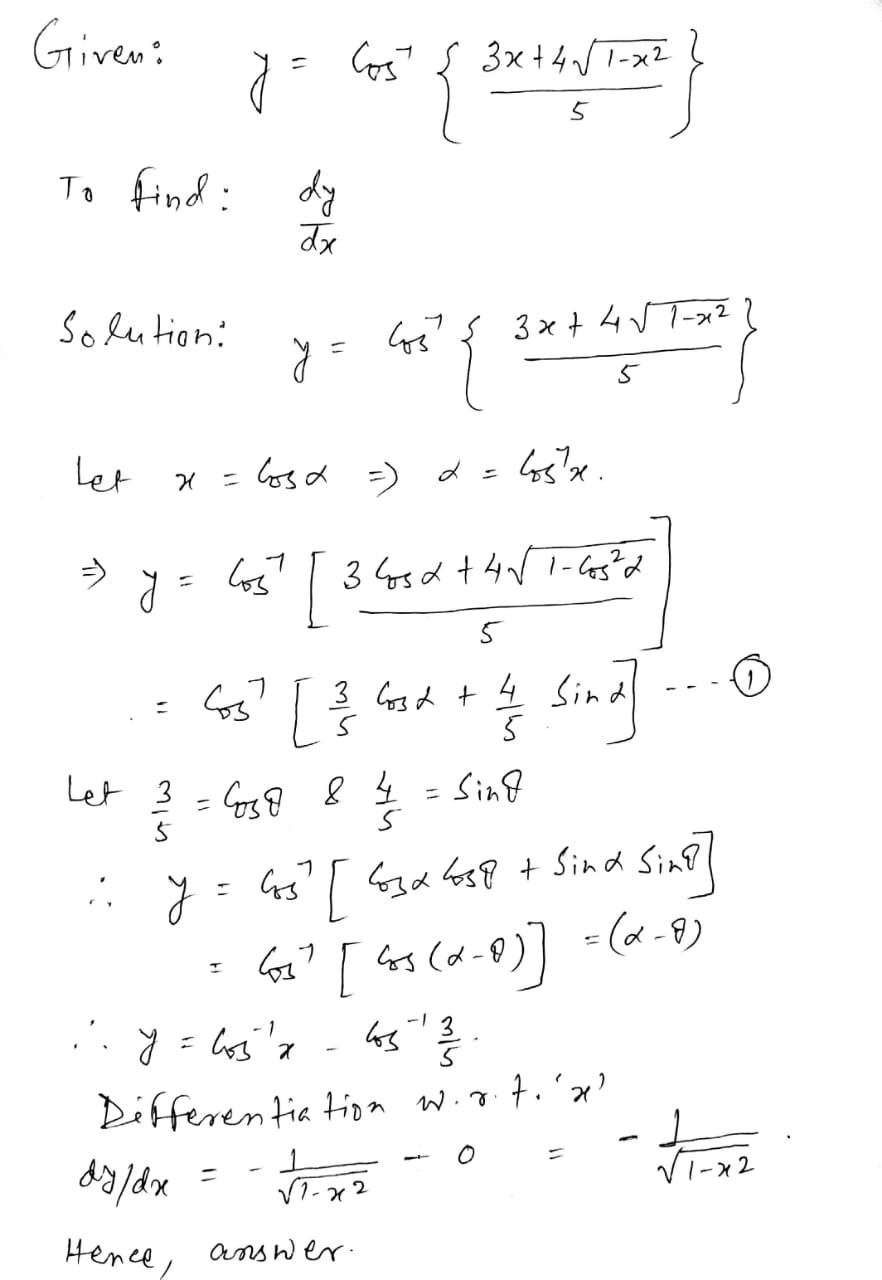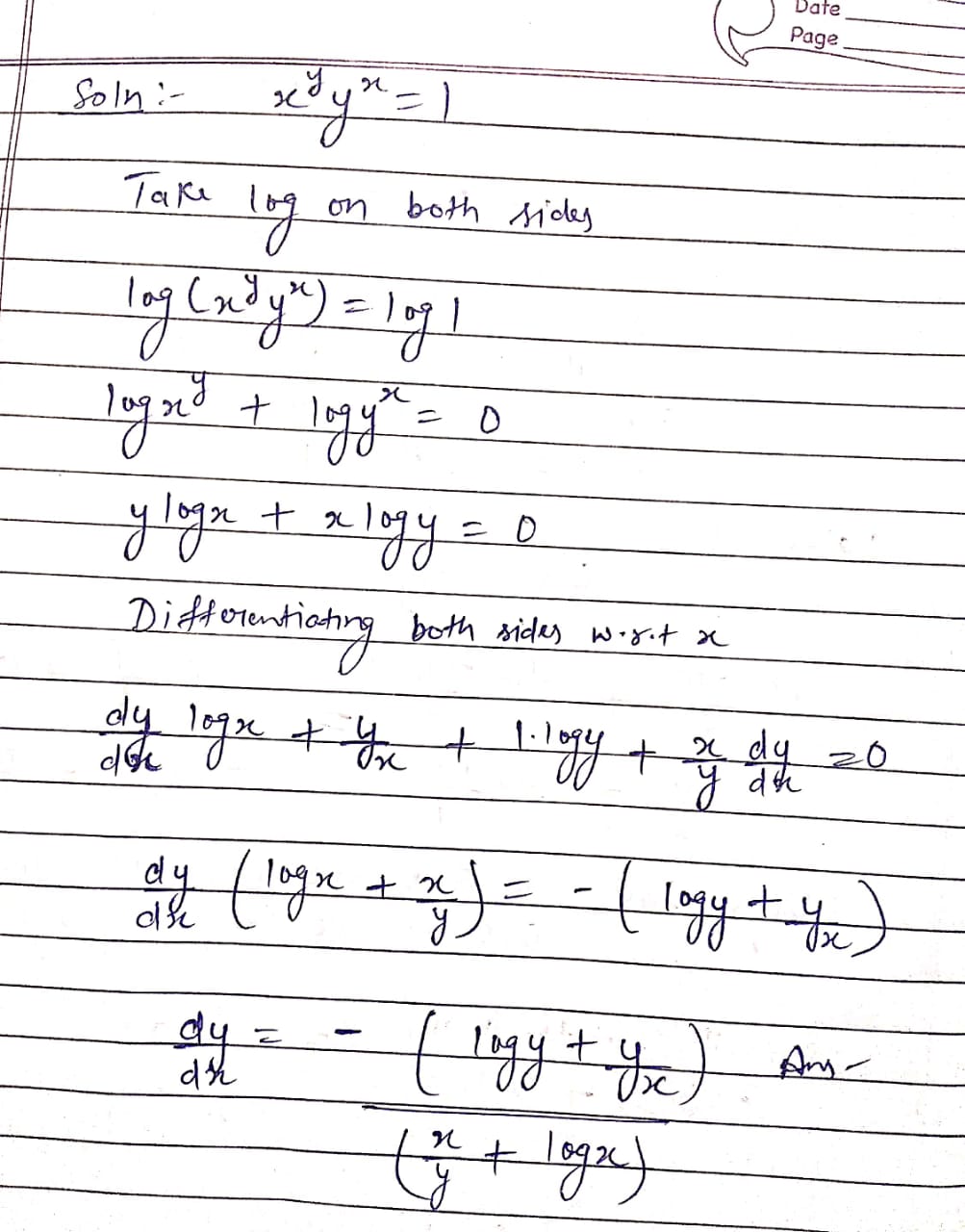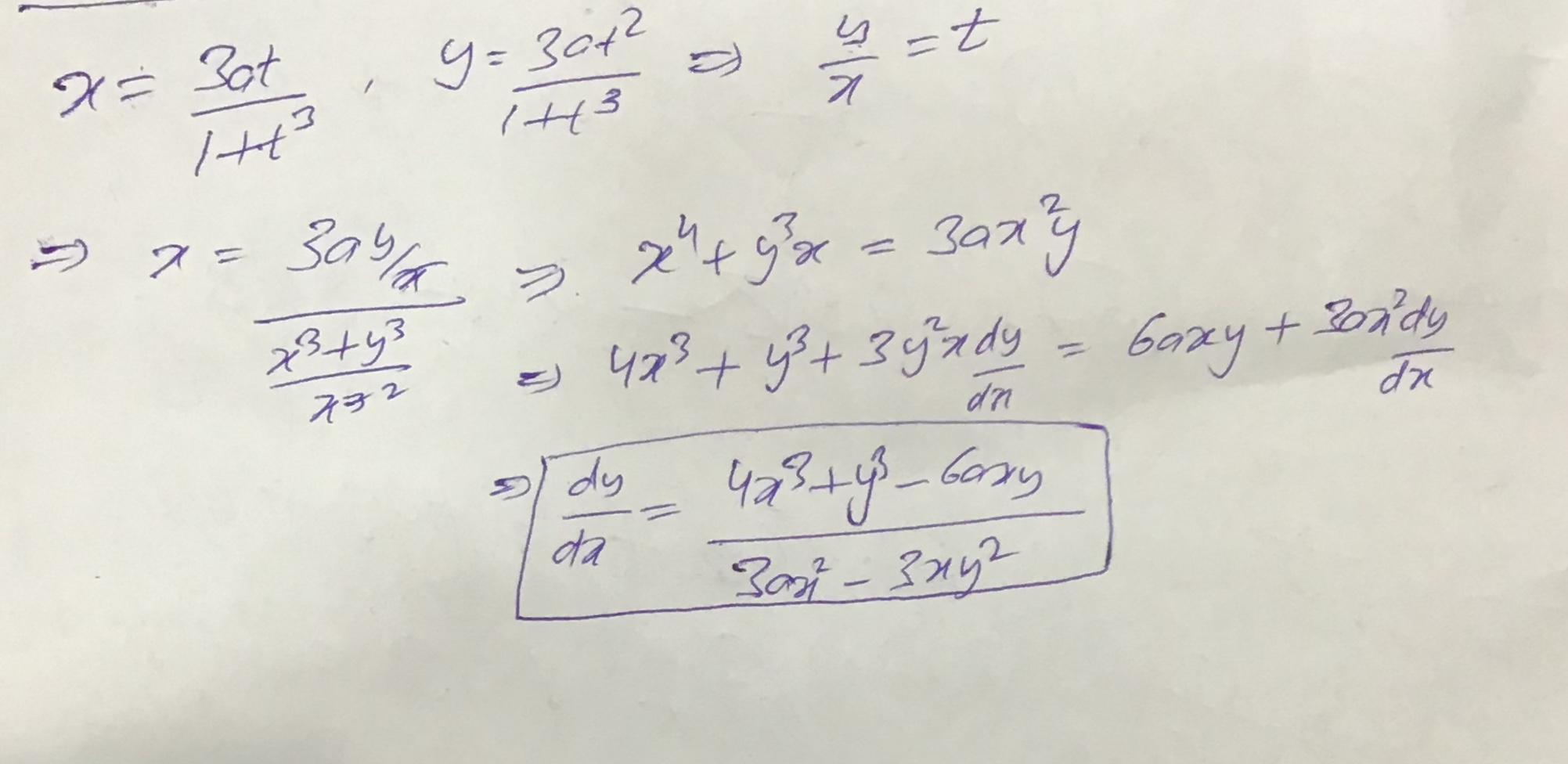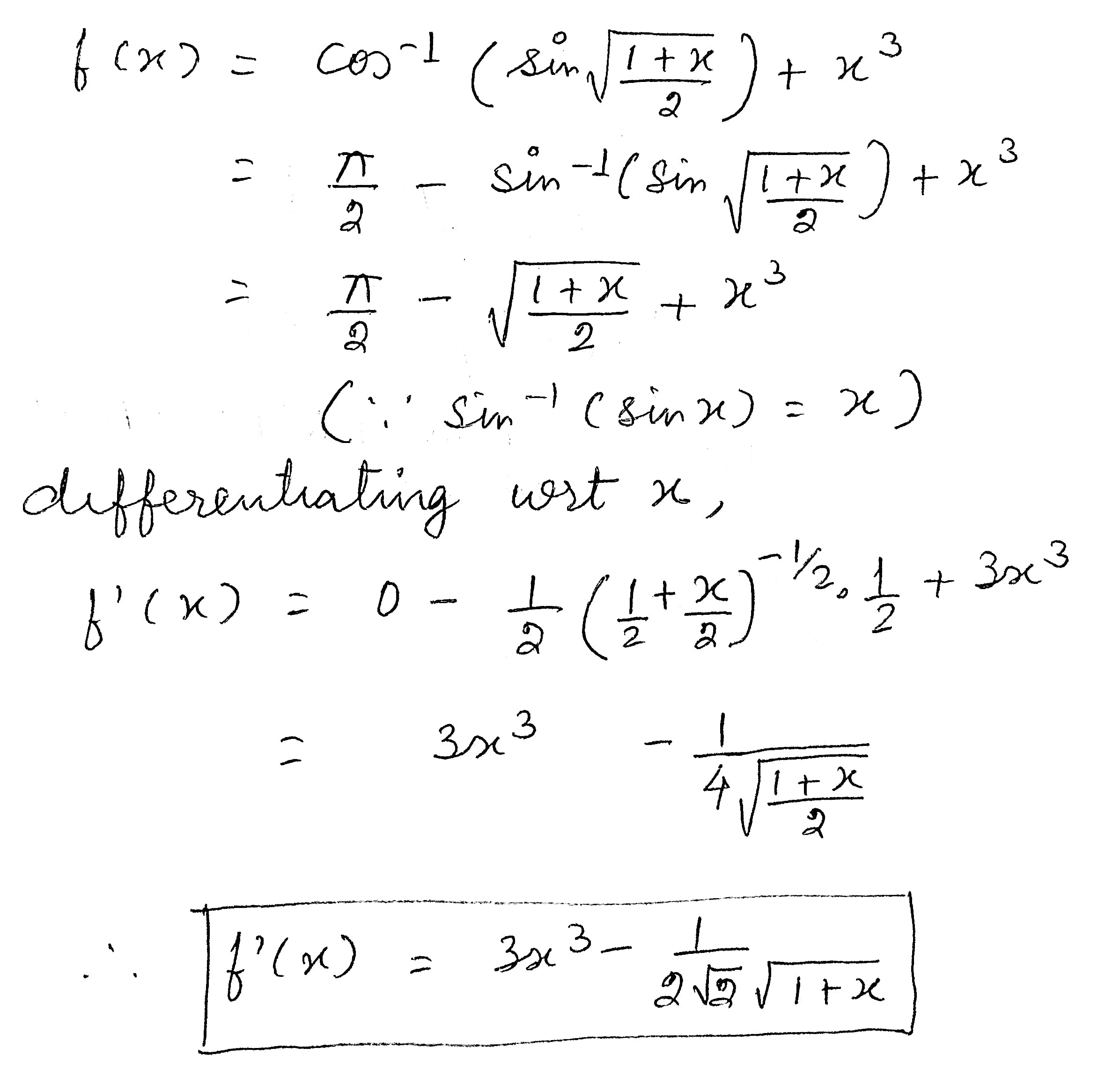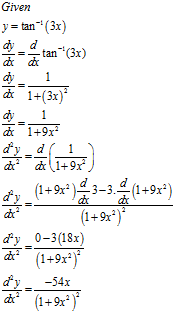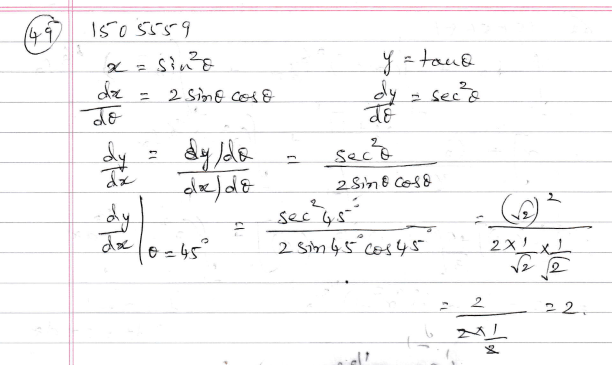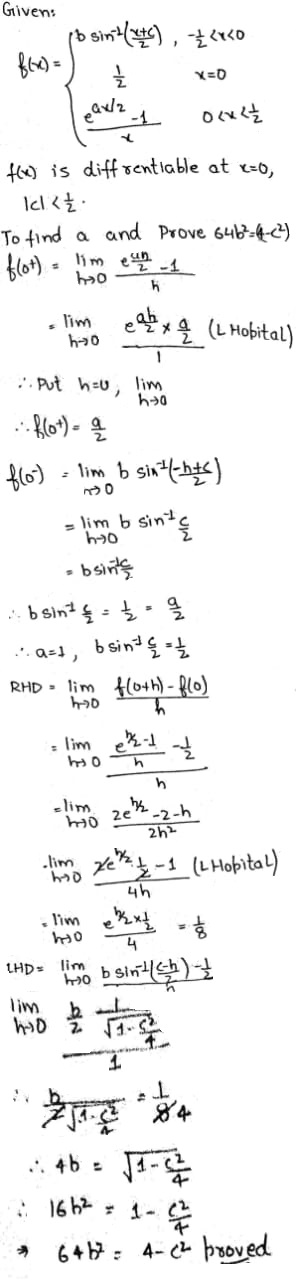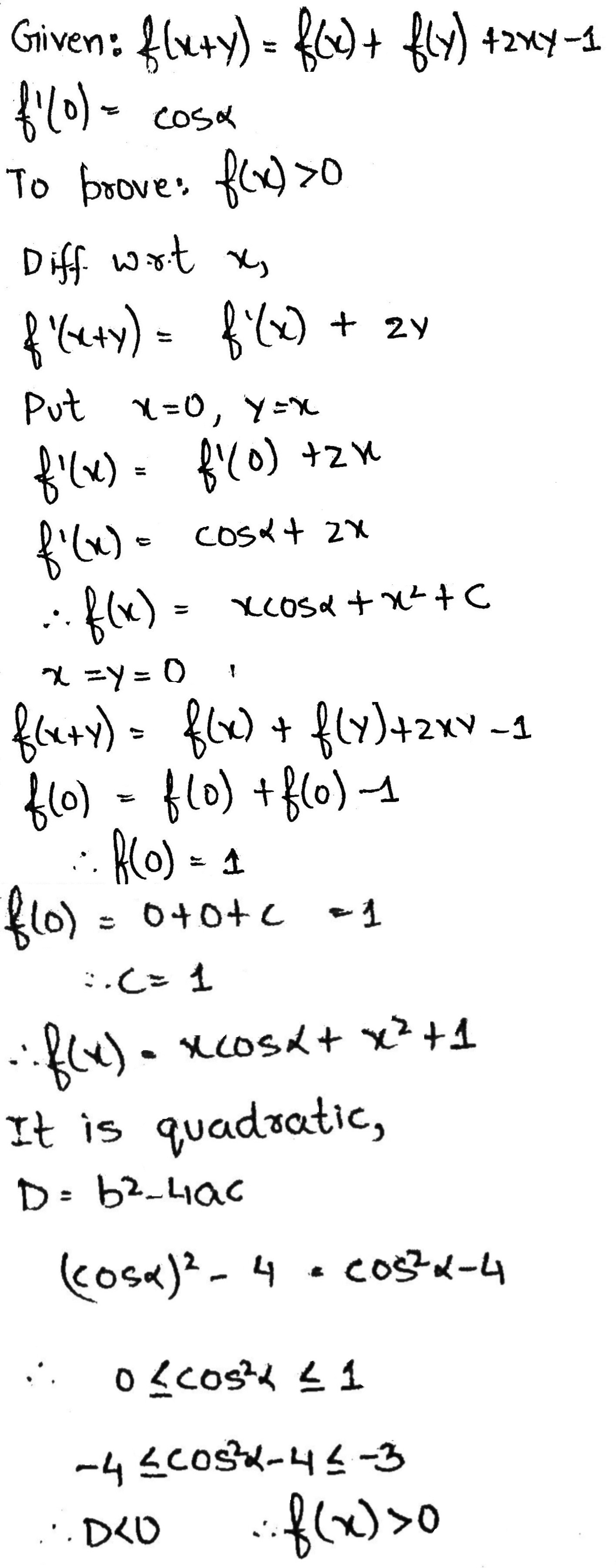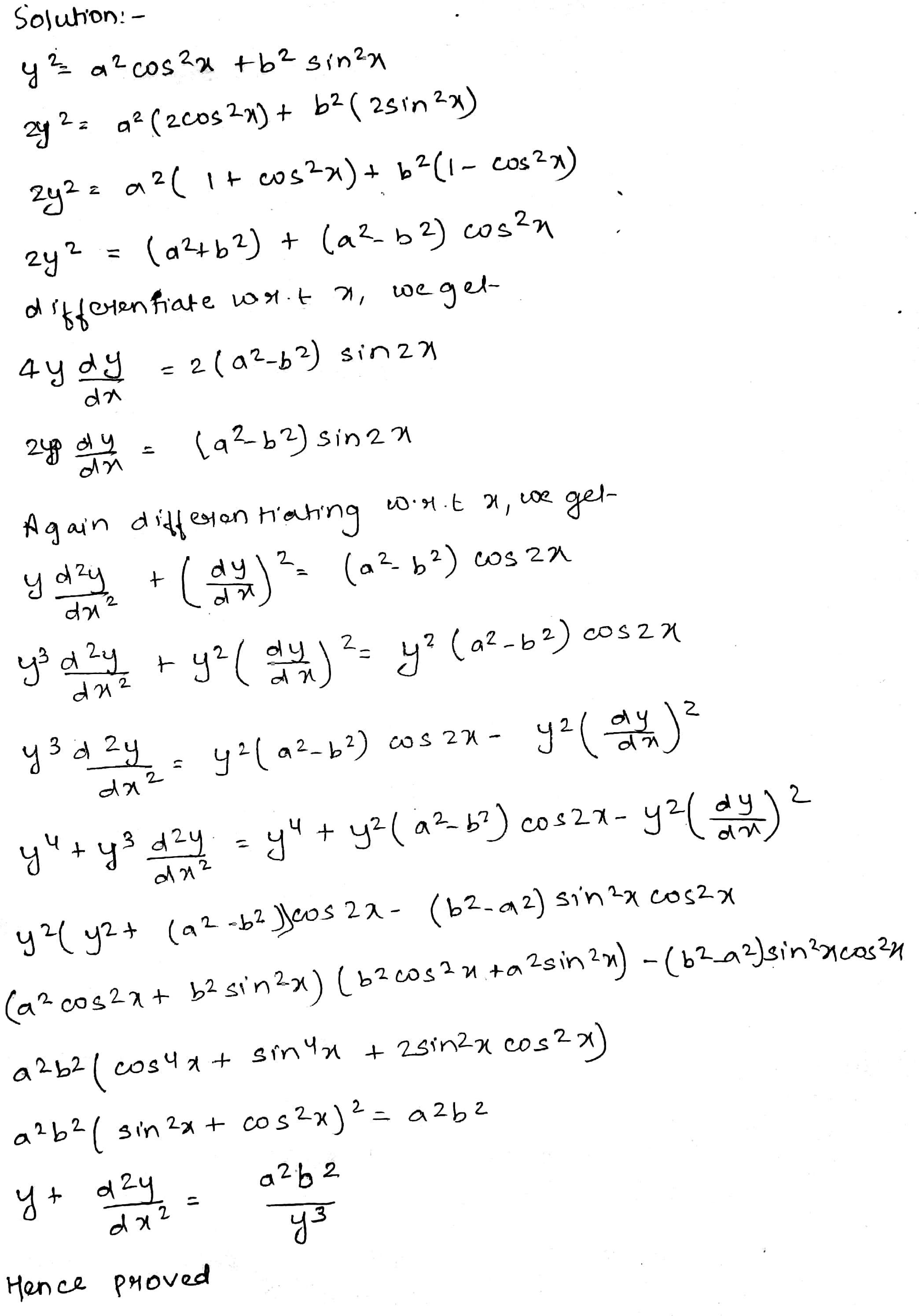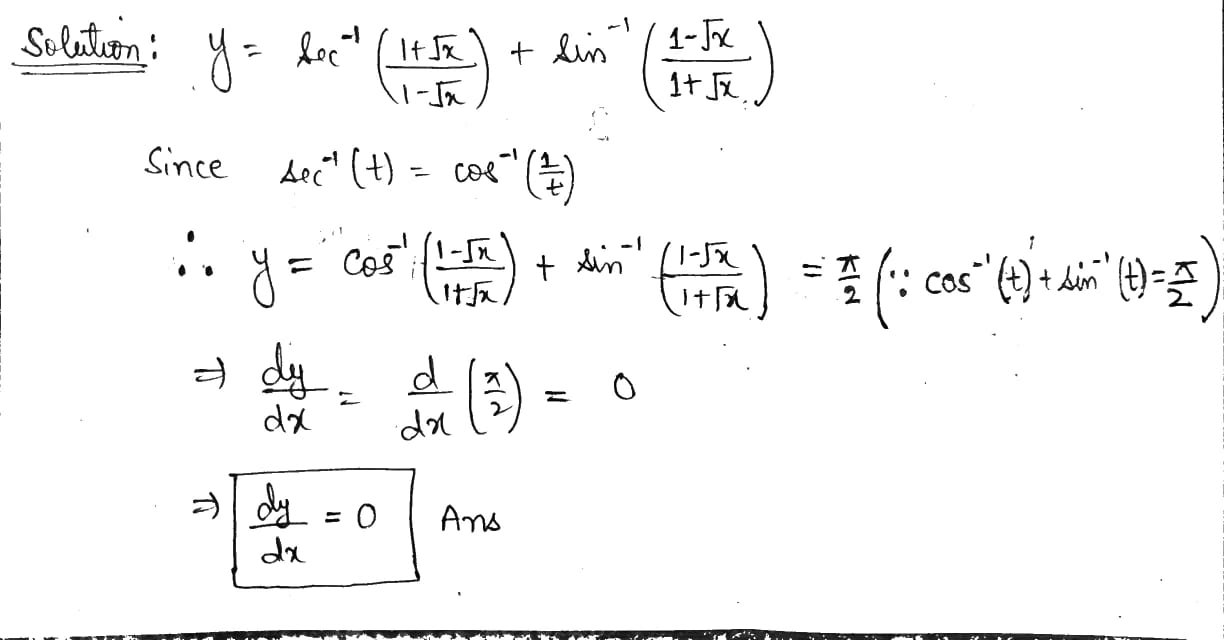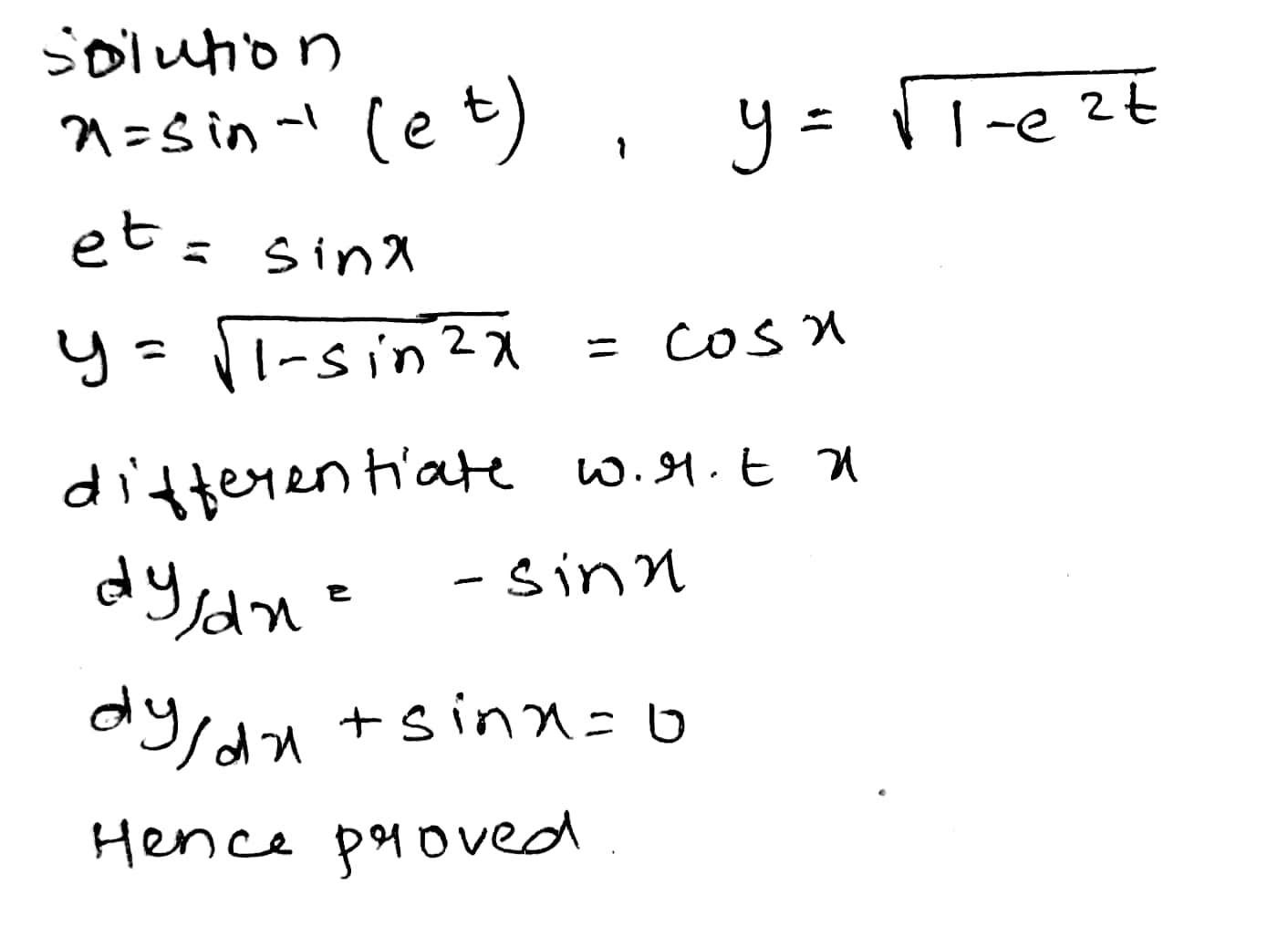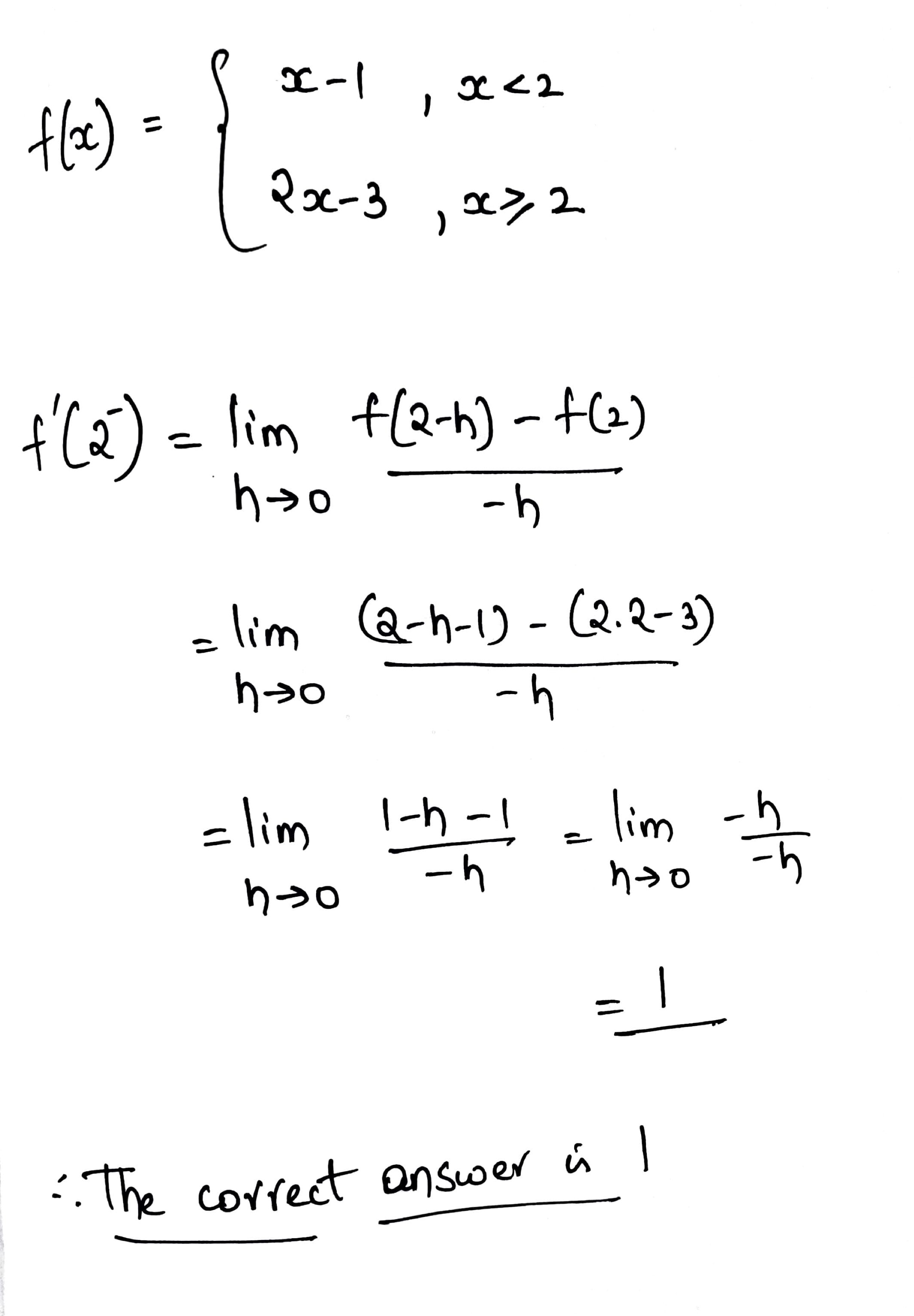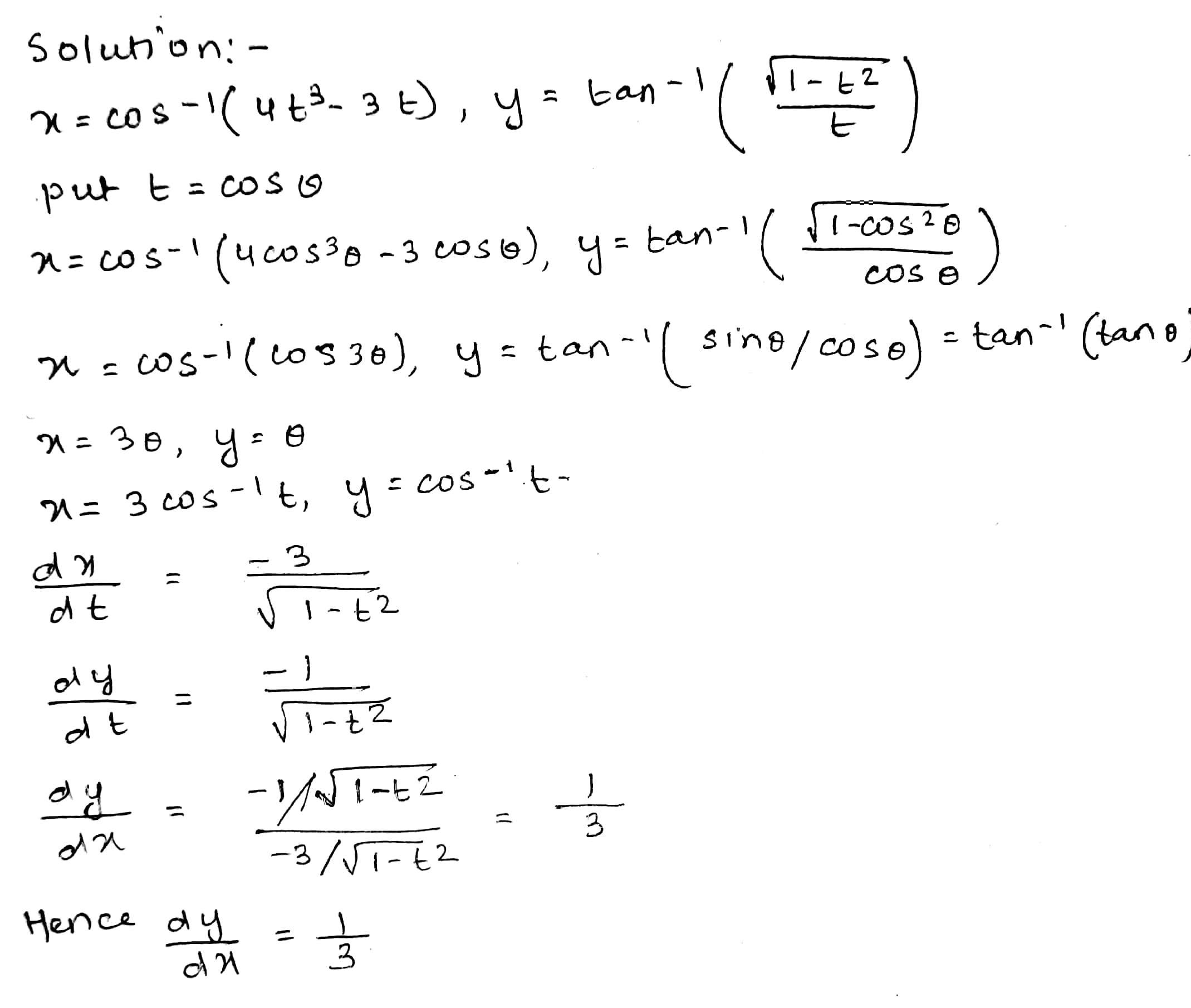Continuity And Differentiability - Class 12 Commerce Maths - Extra Questions
Find dydx, if x2+xy+y2=100.
Differentiate the given function w.r.t. x.
esin−1x
If y=3e2x+2e3x.prove that d2ydx2−5dydx+6y=0.
Differentiate (x+1x)x with respect to x.
Solve lim
Let f (x) = \left\{\begin{matrix} \sin x,& for \ x \geq 0\\ 1 - \cos x, & for \ x < 0\end{matrix}\right. and g(x) = e^x. Then (g of)' (0) is
Differentiate \sin { { h }^{ -1 }\left( \cfrac { x }{ 3 } \right) } with respect to x. Find out the solution of the integration \int { \cfrac { 1 }{ \left( { x }^{ 2 }+9 \right) } dx } Further find out the value of the integral \int { \cfrac { 1 }{ \left( { x }^{ 2 }+49 \right) } dx } ?
Show that \cfrac { d }{ dx } \left( \tan { { h }^{ -1 }x } \right) =\cfrac { 1 }{ 1-{ x }^{ 2 } }
Differentiate the following function with respect to x
\tan { { h }^{ -1 }\left( 3x+1 \right) }
Differentiate the following function w.r.t.x
\sqrt [3] {(2x^{2}-7x-4)^{5}}
Show that \cfrac { d }{ dx } \left( \cos { { h }^{ -1 }x } \right) =\cfrac { 1 }{\sqrt{ { x }^{ 2 }-1 } }
Prove that
\cfrac { d }{ dx } \left( \cot { { h }^{ -1 }x } \right) =\cfrac { -1 }{ \left( { x }^{ 2 }-1 \right) }
Differentiate {X}^{\log{x}}+{(\log{x})}^{x} w.r.t.x.
Find \dfrac{{dy}}{{dx}} if f(x)=\cos 3x.6{e^{6x}}
Find k such that function is continuous at x=0f(x)=\dfrac{x^3-3x}{sinx} for x\neq0 =k for x=0
Differentiate the following function w.r.t.x.
\dfrac{1}{(x^{2}+3^{2})}
Differentiate: f\left( x \right) = {\left[ {\log \left( {7x + 3} \right)} \right]^5} with respect to x.
Differentiate \sec {x^{\tan x}} with respect to x
If x = a\left( {\cos t + t\sin t} \right) and y = a\left( {\sin t - t\cos t} \right), find \dfrac{{{d^2}y}}{{d{x^2}}}
Show that f(x)=|x-2|+|x-3| is not differentiable at x=2.
If \dfrac{x^2}{a^2} + \dfrac{y^2}{b^2} = 1, find \dfrac{dy}{dx}.
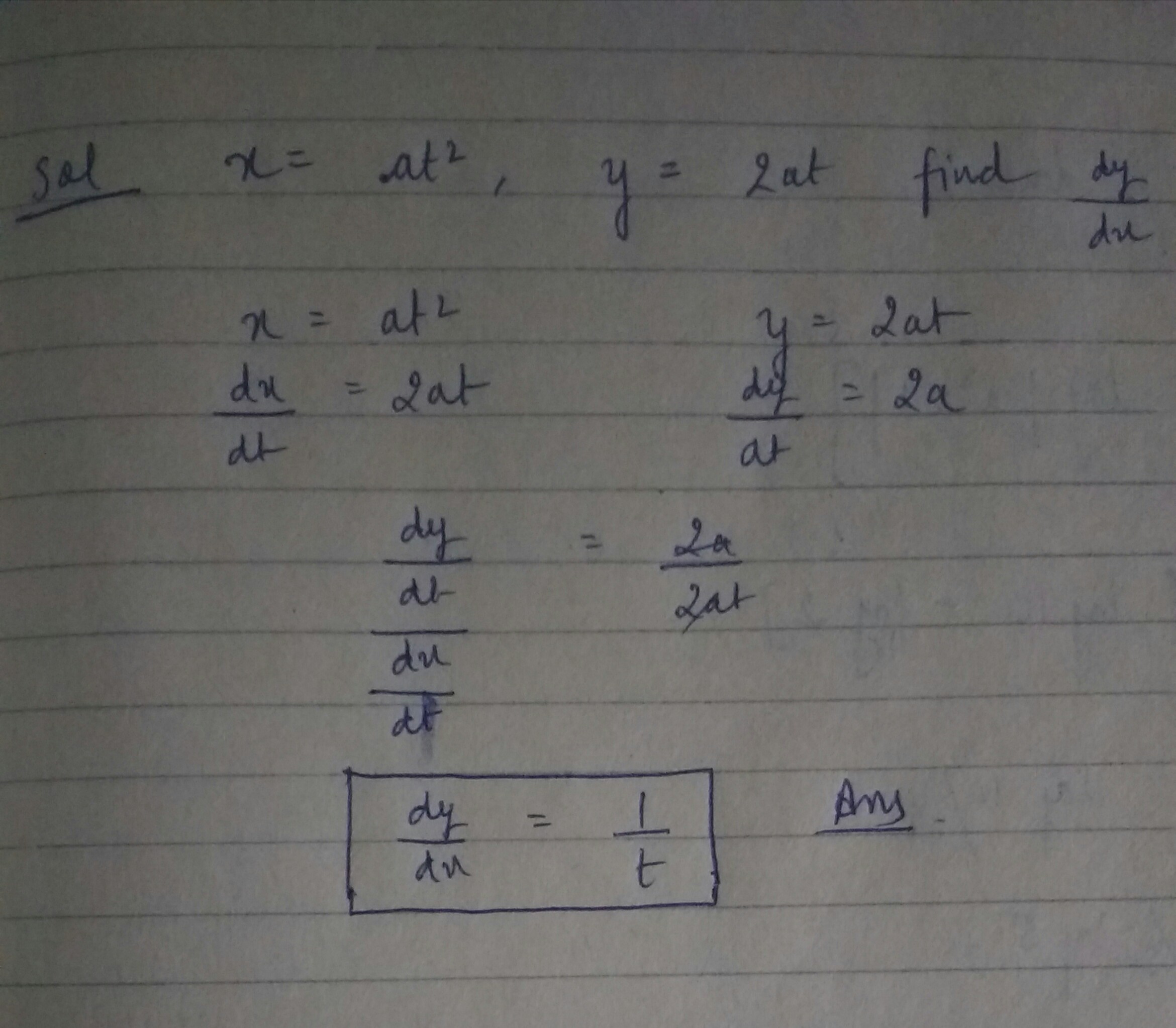
Find \dfrac {d^{2}y}{dx^{2}}, of the following functions: x=at^{2},y=2at.
For a positive constant 'a' find \dfrac{{dy}}{{dx}}, where
y = {a^{t + \frac{1}{t}}},\,and\,x = {\left( {t + \frac{1}{t}} \right)^a}
If x = 2 \,at^2, y = at^4 find \dfrac{dy}{dx}.
Let y=x^2-2x+1 then find the value of y for which \dfrac{dy}{dx}=-2.
Differentiate \dfrac{\tan x}{x}\left(\log\dfrac{e^x}{x^x}\right).
Let y=2^x+x^2+2 then find \dfrac{dy}{dx}.
Let y=x^{10}+10^x+10 then find \dfrac{dy}{dx}
Find \dfrac {dy}{dx} inx = a (\theta - \sin \theta)
y = b (1 - \cos \theta)
y = b (1 - \cos \theta)
Given that {\left( {f\left( x \right)} \right)^{g\left( y \right)}} = {e^{f\left( x \right) - g\left( y \right)}}. Find \dfrac{{d f(x)}}{{d g(x)}}
f ( x ) = \dfrac { 2 x ^ { 2 } - 5 x + 7 } { ( x - 1 ) ( x - 2 ) ( x - 3 ) } is continuous or not?
Differentiate w.r.t. x in \tan^{-1} \left(\dfrac{5x}{1 - 6x^2} \right)
Differentiate { x }^{ { x }^{ 2 } } w.r.t. x
x=a(\cos 2t+2t\ \sin 2t) and y=a(\sin 2t-2t \cos 2t) find second order derivative.
If x=2\cos{\theta}-\cos{2\theta} and y=2\sin{\theta}-\sin{2\theta}, then prove that \cfrac{dy}{dx}=\tan{(\cfrac{3\theta}{2})}
If x = a \left (t - \dfrac {1}{t}\right ), y = a\left (t + \dfrac {1}{t}\right )
Show that: \dfrac {dy}{dx} = \dfrac {x}{y}.
For what values k is the following function continuous at x=-\dfrac{\pi}{6} ?
f\left( x \right) = \left\{ \begin{array}{l}\dfrac{{\sqrt 3 \sin x + \cos x}}{{x + \dfrac{\pi }{6}}}\,,\,x \ne - \dfrac{\pi }{6}\\\,\,\,\,\,\,\,\,\,\,\,\,\,\,k\,\,\,\,\,\,\,\,\,\,\,\,\,\,,x = - \dfrac{\pi }{6}\end{array} \right.
y=x^2+x then Find \dfrac {dy}{dx}.
If x=\dfrac {\sin^{3}t}{\sqrt {\cos 2t}},y=\dfrac {\cos^{3}t}{\sqrt {\cos 2t}}, then find \dfrac {dy}{dx}.
Evaluate : \displaystyle \lim_{x\rightarrow 2}{\dfrac{x^{3}-8}{x^{2}-4}}
Find the derivative of \tan^{-1} \left(\dfrac{\sin x}{1+\cos x}\right) with respect to \tan^{-1}\left(\dfrac{\cos x}{1+\sin x}\right).
Determine the value of 'k' for which the following function is continuous at x = 3 ,\, f (x)= \begin {cases}\dfrac{(x+3)^2-36}{x-3} , & x \neq 3\\ k , & x =3\end {cases}
If y=\sqrt { x } +\dfrac { 1 }{ \sqrt { x } } , show that 2x\dfrac { dy }{ dx } +y=2\sqrt { x } .
y = \sqrt{x} + \frac{1}{\sqrt{x}}
Differentiating with respect to x, we get
\frac{dy}{dx} = \frac{1}{2\sqrt{x}} + (\frac{-1}{2\sqrt[x]{x}})
\frac{dy}{dx} = \frac{1}{2\sqrt{x}} - \frac{1}{2x\sqrt{x}}.
\frac{dy}{dx} = \frac{x-1}{2x\sqrt{x}}
2x\frac{dy}{dx} = \frac{x-1}{\sqrt{x}}
2x\frac{dy}{dx} = \frac{x}{\sqrt{x}} - \frac{1}{\sqrt{x}}.
2x\frac{dy}{dx} =\sqrt{x}-\frac{1}{\sqrt{x}}
2x\frac{dy}{dx} = \sqrt{x}-\sqrt{x}-\frac{1}{\sqrt{x}}+\sqrt{x}
2x\frac{dy}{dx} = 2\sqrt{x}-y
\Rightarrow 2x\frac{dy}{dx} +y = 2\sqrt{x}
If r=at^2, y=2at, then find \dfrac{dy}{dx}.
Sol x=at^{2}, y=2 at find \frac{dy}{dx}
x=at^{2} y=2at
\frac{dx}{dt}=2at \frac{dy}{at}=2a
\frac{\frac{dy}{dt}}{\frac{dx}{dt}} =\frac{2a}{2at}
\frac{dy}{dx}=\frac{1}{t}
Find \dfrac{dy}{dx} :6x-9+8y=0
Find \dfrac {dy}{dx}, if y+\sin{y}=\cos{x}.
Find \dfrac{dy}{dx} :
\sin x +y = 5x-10x^{2}
Differentiate the following functions
{\left[ {{{\left( {\tan \,x} \right)}^{\tan \,x}}} \right]^{\tan \,x}} at x = \frac{\pi }{4}.
Find \dfrac{dy}{dx} :x^{2}+5x+2y^{2}=2
y = 2\tan x Find \dfrac{dy}{dx} at x=\dfrac{\pi}{4}
Find \dfrac{dy}{dx} :2y^{2}+6x=5
Find \dfrac{dy}{dx} if y=\dfrac 1{\sqrt x}
y={ sinx }^{ } find \dfrac{d^2y}{dx^2}
Show that f(x)=\left\{\begin{matrix}12x-13, & if & x\leq 3\\ 2x^2+5, & if & x > 3\end{matrix}\right. is differentiable at x=3. Also, find f'(3).
Define differentiability of a function at a point.
Find whether the following function is differentiable at x=1 and x=2 or not:
f(x)=\left\{\begin{matrix} x, & x\leq 1\\ 2-x, & 1\leq x\leq 2\\ -2+3x-x^2, & x > 2\end{matrix}\right..
Is every differentiable function continuous?
If f(x) is differentiable at x=c, then write the value of \displaystyle\lim_{x\rightarrow c}f(x).
Show that f(x)=\left\{\begin{matrix}12x-13, & if & x\leq 3\\ 2x^2+5, & if & x > 3\end{matrix}\right. is differentiable at x=3. Also, find f'(3).
If \tan (x+y)+ \tan (x-y)=1, then find \dfrac{dy}{dx}.
Is every continuous function differentiable?
If f(x)=|x-2| write whether f'(2) exists or not.
If y=x^x, then find \dfrac{dy}{dx}.
Differentiate with respect to x:
(x^x)
Find \dfrac{dy}{dx}, when
If x=\cos t and y=\sin t, prove that \dfrac {dy}{dx}=\dfrac{1}{\sqrt{3}} at t=\dfrac {2 \pi}{3}
If x=10(t-\sin t),\ y=12(1-\cos t), find \dfrac {dy}{dx}.
If x=\sin t and y=\cos t, then find \dfrac{dy}{dx}.
If x=3\sin {t} ,\ y=3\cos {t} , then find \dfrac {dy}{dx} at t=\dfrac { \pi }{ 3 } .
If x=4t^2,y=6t, then find \dfrac{dy}{dx}.
Find \dfrac {dy}{dx}, when x= {2t} and y={1-t^{2}}.
Find \dfrac{dy}{dx}, when x=4t, y=\dfrac{4}{t}.
Find \dfrac{dy}{dx}, when
x=3at^2 and y=2at.
Differentiate the following w.r.t. x: \operatorname{cosec}^{-1}\left(\dfrac{1}{4 \cos ^{3} 2 x-3 \cos 2 x}\right)
Find the second order derivative of y= x^{20}
Find \dfrac{dy}{dx}, if x=2at^2 and y=at^4.
If x=\sin t and y=\cos t, find \dfrac{dy}{dx}.
If x=e^t \cos t and y=e^{-t} \sin t, find \dfrac{dy}{dx}.
Differentiate the following w.r.t. x: \cot ^{-1}\left(x^{3}\right)
Find \dfrac{dy}{dx}, if x=a\cos \theta, y=b\cos \theta.
Find \dfrac{dy}{dx}, if x=\sin t, y=\cos 2t.
Find derivative of y=x^2+e^x+x^x.
If f(x) is a twice differentiable function such that f(a) = 0, f(b) = 2, f(c) = 1, f(d) = 2, f(e) = 0, where a < b < c < d < e, then the minimum number of zeroes of g(x) = (f'(x))^{2}+f''(x)f(x)) in the interval [a, e] is
If f(x) is a twice differentiable function such that \displaystyle f\left ( a \right )= 0, f\left ( b \right )= 2, f\left ( c \right )=-1, f\left ( d \right )=2 and \displaystyle f\left ( e \right )=0, where \displaystyle a< b< c< d< e, then the minimum number of zeros of \displaystyle g(x)={f'(x)}^{2}+f"(x)\cdot f\left ( x \right ) in the interval [a,c] is
Let \displaystyle f\left ( x \right )=\frac{x^{3}+x^{2}-16x+20}{\left ( x-2 \right )^{2}}if x\neq 2,=k,ifx=2. If \displaystyle f\left ( x \right ) is continuous for all x,then k=b \times7 Find b
If \displaystyle y=\frac{(x+1)^{2}\sqrt{x-1}}{(x+4)^{2}e^{x}}, then show that \displaystyle \frac{dy}{dx}=\frac{x+1^{2}\sqrt{(x-1)}}{(x+4)e^{x}}\left [ \frac{5x-3}{2(x^{2}-1)}+\frac{x+6}{x+4} \right ]
If f(x) and g(x) both are discontinuous at any point, then show that their composition may be differentiable at that point.
Solve \displaystyle \lim_{x\rightarrow \pi /2}\frac{\sin x-\left ( \sin x \right )^{\sin x}}{1-\sin x+\log \sin x}
Differentiate the function w.r.t. x.
\displaystyle x^{x \cos x} + \frac{x^2 + 1}{x^2 - 1}
Differentiate the function w.r.t. x.
(\log x)^x + x^{\log x}
Differentiate the function w.r.t. x.
\displaystyle \left( x + \frac{1}{x} \right)^x + x^{\left( 1 + \tfrac{1}{x} \right) }
Differentiate the function w.r.t. x.
(\log x)^{\cos x}
Differentiate the function w.r.t. x.
\displaystyle x^{\sin x} + (\sin x)^{\cos x}
Differentiate the given function w.r.t. x:
(\sin x - \cos x)^{(\sin x - \cos x)}, \displaystyle{\frac{\pi}{4} < x < \frac{3\pi}{4}}
Differentiate the given function w.r.t. x:
x^{x^{2} - 3} + (x - 3)^{x^{2}}, for x > 3
If x and y are connected parametrically by the given equation, then without eliminating the parameter, find \displaystyle \frac{dy}{dx}
x = 2at^2, y = at^4
Discuss the continuity of the following function :
f(x)=\sin { x } +\cos { x } .
If x=\sqrt { { a }^{ \sin ^{ -1 }{ t } } } ,\quad y=\sqrt { { a }^{ \cos ^{ -1 }{ t } } } , show that \cfrac{dy}{dx}=-\cfrac{y}{x}
If u, v and w are functions of x, then show that
\cfrac { d }{ dx } \left( u,v,w \right) =\cfrac { du }{ dx } v.w+u.\cfrac { dv }{ dx } .w+u.v\cfrac { dw }{ dx }
in two ways- first by repeated application of product rule, second by logarithmic differentiation.
If x and y are connected parametrically by the given equation, then without eliminating the parameter, find \displaystyle \frac{dy}{dx} .x = a(\cos \theta + \theta \sin \theta) and y = a(\sin \theta - \theta \cos \theta)
Differentiate w.r.t x.
\sin^{-1} \left (\dfrac {a\cos x + b\sin x}{\sqrt {a^{2} + b^{2}}}\right )
If x = a\left (t - \dfrac {1}{t}\right ), y = a\left (t + \dfrac {1}{t}\right ), then show that \dfrac {dy}{dx} = \dfrac {x}{y}
If x = at^{2}, y = 2at, then find \dfrac {dy}{dx}.
Find \cfrac { dy }{ dx } where { x }^{ y }={ y }^{ x };x>0,y>0.
If x^{y} = e^{x - y}, then show that \dfrac {dy}{dx} = \dfrac {\log x}{(1 + \log x)^{2}}
Show that the function f(x) = \left\{\begin{matrix} 3 - x, & if &x < 1 \\ 2, & if & x = 1\\ 1 + x, & if & x > 1\end{matrix}\right. is continuous at x = 1
If x=a \sin^3\theta and y=a \cos^3\theta, then find the value of \displaystyle\frac{dy}{dx}.
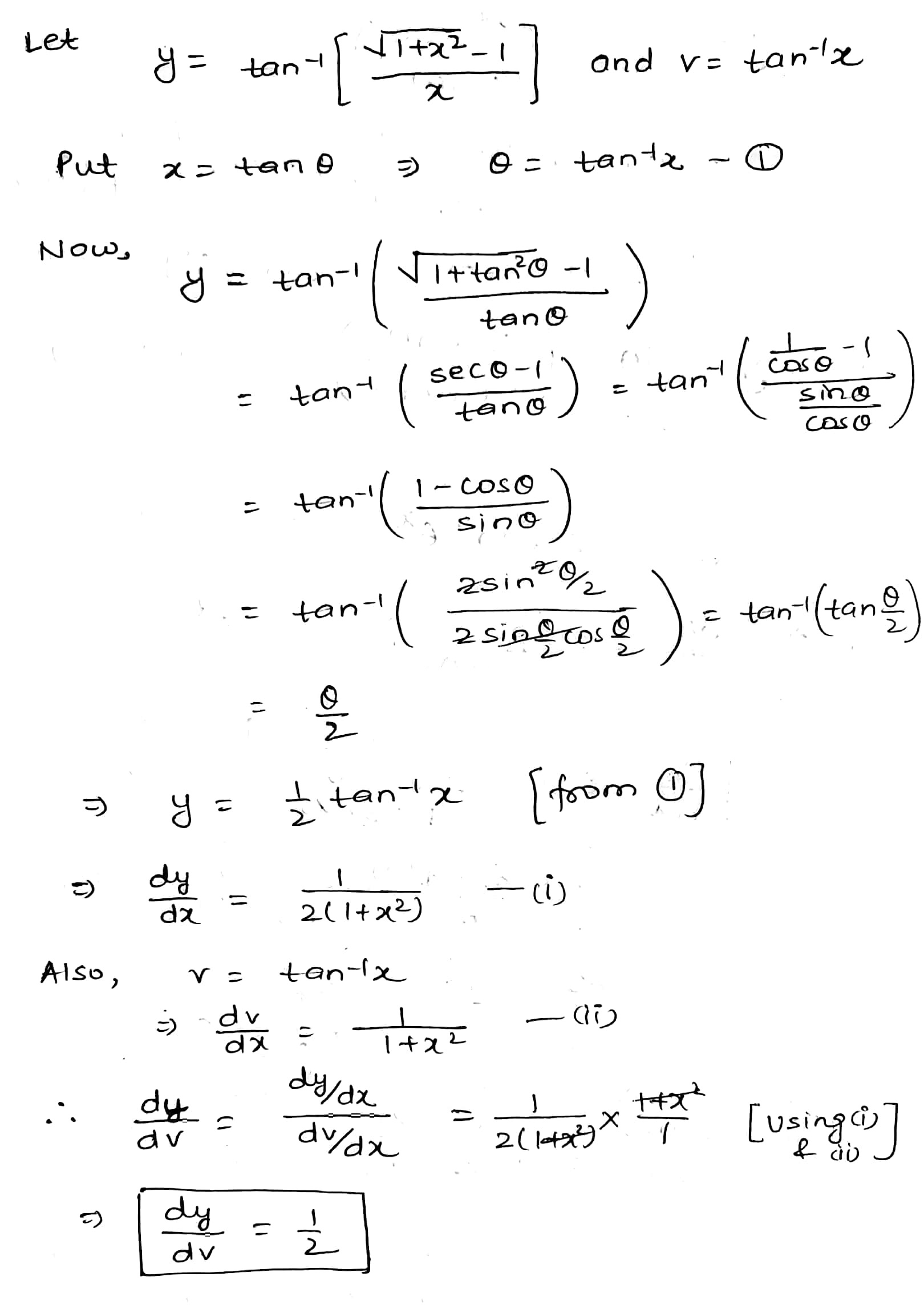
Find the differential coefficient of \tan ^{ -1 }{ x }
If y = x^x, find \dfrac{dy}{dx}.
Determine the value of 'k' for which the following function is continuous at x = 3:
f(x) = \left\{\begin{matrix} \dfrac{(x + 3)^2 - 36}{x - 3}& , x \neq 3 \\ k & , x = 3\end{matrix}\right.
If a curve is represented parametrically by the equations.
x=\sin\left(t+\dfrac{7\pi}{12}\right)+\sin\left(t-\dfrac{\pi}{12}\right)+\sin\left(t+\dfrac{3\pi}{12}\right), y = \cos\left(t+\dfrac{7\pi}{12}\right)+\cos\left(t-\dfrac{\pi}{12}\right)+\cos\left(t+\dfrac{3\pi}{12}\right) then find the value of \dfrac{d}{dt}\left(\dfrac{x}{y}-\dfrac{y}{x}\right) at t = \dfrac{\pi}{8}
If y= \sec x + \tan x, then prove that \displaystyle \frac{d^2y}{dx^2} = \frac{\cos x}{(1 - \sin x)^2}
Suppose f is continuous f(0)=0, f(1)=1, f'(x)> 0 and \displaystyle\int_{0}^{1}f(x)dx=\frac{1}{3}. Find the value of the definite integral \displaystyle\int_{0}^{1}f^{-1}(y)dy.
Differentiate sec^{-1}x w.r.to x by first principle.
Differentiate the function \tan^{-1} \dfrac{2x}{1 - x^{2}} w.r.to \cos^{-1}(\dfrac{1 - x^{2}}{1 + x^{2}})
Differentiate \tan ^{ -1 }{ \left( \dfrac { x }{ \sqrt { 1-{ x }^{ 2 } } } \right) } w.r.t \sin ^{ -1 }{ \left( 2x\sqrt { 1-{ x }^{ 2 } } \right) }.
Differentiate the following function with respect to x
\cos { { h }^{ -1 }(4+3x) }
Differentiate the following function with respect to x:
\sin { { h }^{ -1 }\left( \sqrt { x } \right) }
Differentiate the following \displaystyle\frac{e^x}{\sin x}.
If x=a(2\theta -\sin 2\theta) and y=a(1-\cos 2\theta), find \displaystyle\frac{dy}{dx} when \theta =\displaystyle\frac{\pi}{3}.
Evaluate: y={ \left( \sin { x } \right) }^{ \tan { x } }+{ \left( \cos { x } \right) }^{ \sec { x } }
For x = \sqrt { { a }^{ \tan ^{ -1 }{ t } } } ,y=\sqrt { { a }^{ \cot ^{ -1 }{ t } } } ,t\in R, find \cfrac { dy }{ dx } .
Let f(x) be differentiable function in \left[ \left. -1,\infty \right)\right. and f(0)=1 such that \lim\limits_{t \to x+1}\large{\frac{t^2f(x+1)-(x+1)^2 f(t)}{f(t)-f(x+1)}}=1. Find the value of \lim\limits_{x \to 1}\large{\frac{\ln \left(f(x)\right)- \ln 2}{x-1}}
Find \dfrac{dy}{dx}, when
i) x=at^2 and y=2at
ii) x=a(\theta+\sin \theta) and y=a(1-\cos \theta).
If x = \sin t, y = \cos 2t then prove that \dfrac {dy}{dx} = -4\sin t.
Find the derivative with respect to x of the function:
(\log_{\cos x}\sin x)(\log_{\sin x} \cos x)^{-1}+\sin ^{-1}\dfrac{2x}{1+x^2} at x=\dfrac{\pi}{4}.
Differentiate: \sqrt {e^{\sqrt {x}}},x>0
Find \dfrac{dy}{dx}, if y = x^{\sin \, x} + (\sin \, x)^{\cos \, x}
If y = {\cos ^{ - 1}}\left\{ {\dfrac{{2x - 3\sqrt {1 - {x^2}} }}{{\sqrt {13} }}} \right\}. Find \dfrac{{dy}}{{dx}}.
If y = {\left( {{{\cot }^{ - 1}}x} \right)^2}, then show that \left( {1 + {x^2}} \right)^2{{{d^2}y} \over {d{x^2}}} + 2x\left( {1 + {x^2}} \right){{dy} \over {dx}} = 2.
If Y=\cot ^{ -1 }{ \left[ \dfrac { \sqrt { 1+\sin { x } } +\sqrt { 1-\sin { x } } }{ \sqrt { 1+\sin { x } } -\sqrt { 1-\sin { x } } } \right] } . Prove that \dfrac {dy}{dx} is independent of x.
Find the derivative of \tan {\sqrt {x}} w.r.t. x., using first principle
If {y^x} = {e^{y - x}} then prove that \frac{{dy}}{{dx}} = \frac{{{{\left( {1 + \log y} \right)}^2}}}{{\log y}}.
Differentiate w.r.t. x :f(x) = \sqrt {\sin \left( {\cos x} \right)}
Solve : y = \sin^{-1} \left(\dfrac{1 - x^2}{1 + x^2} \right) , 0 < x < 1
If x=a(\cos t+log \tan \dfrac{t}{2}) and y=a\sin t, then find \dfrac{d^2y}{dx^2} at t=\dfrac{\pi}{3}.
If x=a\sec^3\theta, y=a\tan^3\theta find \dfrac{dy}{dx} at \theta =\dfrac{\pi}{4}.
Differentiate y=\left[ \sin ^{ -1 }{ \left( x-\dfrac { 4{ x }^{ 3 } }{ 27 } \right) } \right] w.r.t x
Find \dfrac{dy}{dx} when y=\dfrac{1+\sin 2x-\cos 2x}{1+\sin 2x+\cos 2x}.
Find \dfrac{{dy}}{{dx}}:
x = \dfrac{{{e^t} + {e^{ - t}}}}{2}\,and\,y = \dfrac{{{e^t} - {e^{ - t}}}}{2}
If x = a{\cos ^3}\theta and y = a{\sin ^3}\theta , then find the value of \,\dfrac{{{d^2}y}}{{d{x^{2\,\,}}}} at \theta = \dfrac{n}{6}
f(x)=x cosx
Find f'(\pi)
Formula: cos(A+B)= cosA cosB- sinA sinB.
Find \dfrac{dy}{dx} for 2x^2+5xy+3y^2=1.
If f(x)=\begin{cases} \dfrac { { 2 }^{ x+2 }-16 }{ { 4 }^{ x }-16 }\ ,\ if x\neq 2 \\ k \quad \quad \quad \quad, \ if x = 2 \end{cases} is continuous at x=2, find k
Let y=e^x.\sin(ax^2+bx+c) then find \dfrac{dy}{dx}.
If x=3\sin \theta-\sin 3\theta \\ y=3 \cos \theta-\cos \theta
Find \dfrac {dy}{dx} at x=\dfrac {\pi}{3}
Find \frac{dy}{dx}=sin^{-1}x
Diffrentiate w.r.t x
\tan^{-1}x
Find the derivative w.r.t to x i)x^y
Find the derivative of x^x - 2^{\sin x} w.r.t. x
Find d=\dfrac{dy}{dx} if x=\dfrac{\sin^3t}{\sqrt{\cos 2t}}
y=\dfrac{\cos^3t}{\sqrt{\cos 2t}}
If the function f(x)=\dfrac {(4^{\sin x}-1)^{2}}{x\log (1+2x)} for x \neq 0 is continuous at x=0, Find f(0)
Let y=3x^2+5x find \dfrac{dy}{dx}
If y is a differentiable function of u and u is a differentiable function of x, then prove that \dfrac { dy }{ dx } =\dfrac { dy }{ du } .\dfrac { du }{ dx } .
Given f\left( x \right) = \dfrac{1}{{x - 1}} . Find the points of discontinuity of the composite function y =[ f\left\{ {f\left( x \right)} \right\}]
f(x)=\dfrac{1}{x-1}
f(f(x))=\dfrac{1}{\dfrac{1}{x-1}-1}=\dfrac{x-1}{1-x+1}=\dfrac{x-1}{2-x}
Points of discontinuity of y=f(f(x)) is when x=2.
Show that f(x)=\left\{\begin{matrix} \dfrac{\cos ax-\cos bx}{x^2} & if & x\neq 0\\ \dfrac{1}{2}\left(b^2-a^2\right) & if & x=0\end{matrix}\right. where a and b are real constants, is continuous at 0.
Let f(x) = 1 + 4x - x^{2}, \forall x\epsilon R
g(x) = \left\{\begin{matrix} max.&\left \{f(t); x \leq t\leq (x + 1); 0\leq x < 3\right \} \\ min. & \left \{(x + 3); (3\leq x \leq 5\right \}\end{matrix}\right.
Discuss the continuity and differentiability of g(x) for all x\epsilon [0, 5].
If x=a\sin 2t(1+\cos 2t) and y=b2t(1-\cos 2t), find the values of \dfrac{dy}{dx} at t=\dfrac{\pi}{4} and t=\dfrac{\pi}{3}.
Differentiate x\ \cos^{-1} x.
Discuss the continuity of f(x)
= \dfrac {x^{5}\sqrt {x} - 32\sqrt {2}}{x^{3}\sqrt {x} - 8\sqrt {2}} x\neq 2
= \dfrac {44}{7}, at x = 2.
Verify Rolle's Theorem for the function f\left( x \right) = {x^2} + 2x - 8,x \in \left[ { - 4,2} \right]
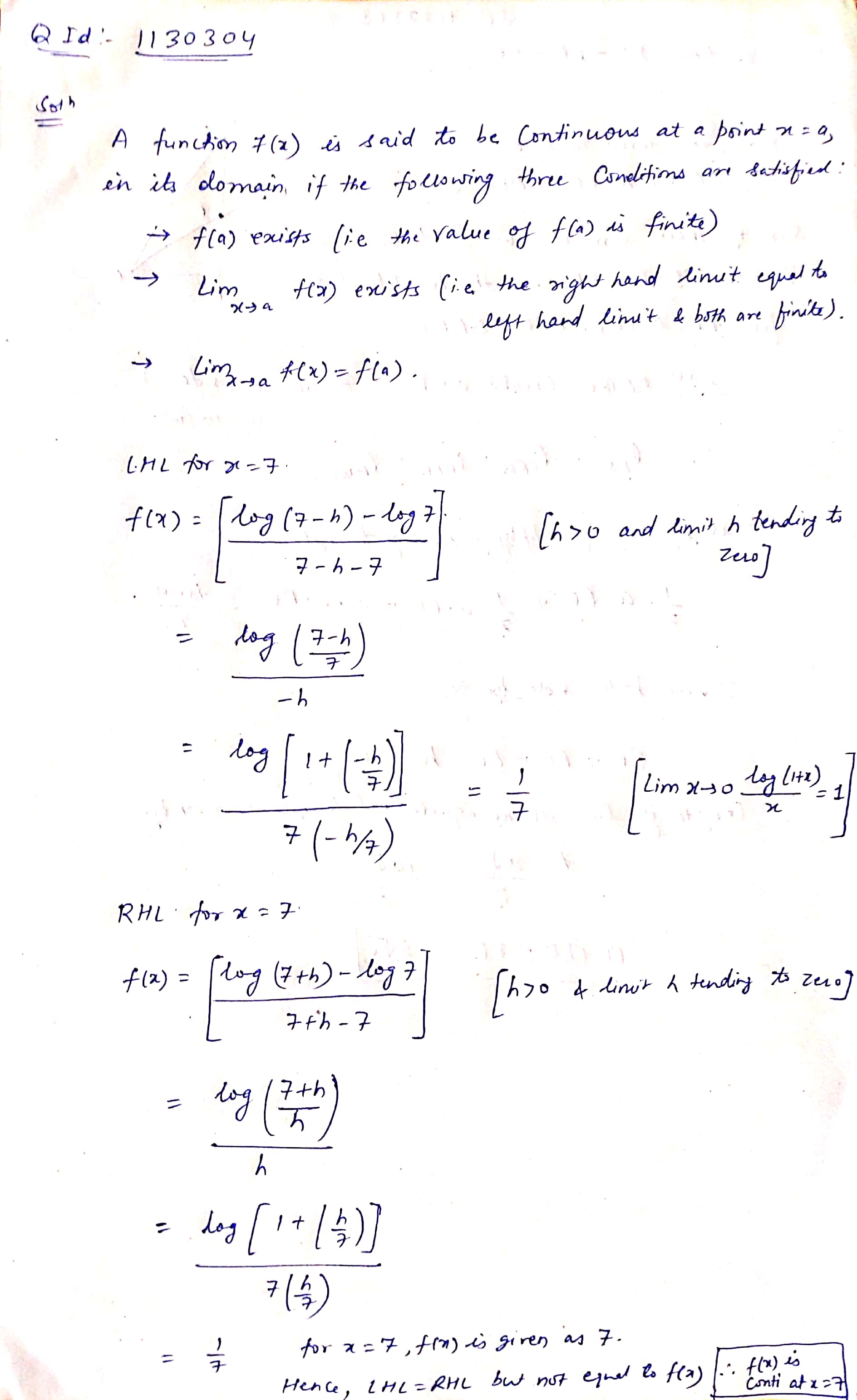
Prove that given function is not continuousf(x) = \left.\begin{matrix} \dfrac {\log x - \log 7}{x - 7},& for\ x\neq 7\\ = 7, & for\ x = 7\end{matrix}\right\} at x = 7.
A function 7(x) is said to be continuous at a point x=0, in its domain if the following three conditions are satisfied:
\rightarrow f(a) exists (i.e., the value of f(a) is finite)
\rightarrow \displaystyle\lim_{x\rightarrow a}f(x) exists (i.e. the right hand limit equal to left hand limit & both are finite).
\rightarrow \displaystyle\lim_{x\rightarrow a}f(x)=f(a)
LHL for x=7
f(x)=\left[\dfrac{log(7-h)-log 7}{7-h-7}\right] [h > 0 and limit h tending to zero]
=\dfrac{log\left(\dfrac{7-h}{7}\right)}{-h}
=\dfrac{log\left[1+\left(-\dfrac{h}{7}\right)\right]}{7\left(-\dfrac{h}{7}\right)}=\dfrac{1}{7} \left[\displaystyle\lim_{x\rightarrow 0}\dfrac{log(1+x)}{x}=1\right]
RHL for x=7
f(x)=\left[\dfrac{log(7+h)-log 7}{7+h-7}\right] [h > 0 & limit h tending to zero]
=\dfrac{log\left(\dfrac{7+h}{h}\right)}{h}
=\dfrac{log\left[1+\displaystyle\left(\dfrac{h}{7}\right)\right]}{7\left(\dfrac{h}{7}\right)}
=\dfrac{1}{7}
for x=7, f(x) is given as 7
Hence, LHL=RHL but not equal to f(x)
\therefore f(x) is conti at x=7.
Use Rolle's theorem to prove that equation ax^{2}+bx=\dfrac{a}{3}+\dfrac{b}{2} has a root between 0 and 1.
If y = {x^x},{\text{ then prove that }}{{{d^2}y} \over {d{x^2}}} - {1 \over y}{\left( {{{dy} \over {dx}}} \right)^2} - {y \over x} = 0.
If {\left( {{x^2} + {y^2}} \right)^2} = xy, find \frac{{dy}}{{dx}}.
If x=asin2t(1+cos2t) and y=bcos2t(1-cos2t), then find the values of \frac { dy }{ dx } at t=\frac { \pi }{ 4 } and t=\frac { \pi }{ 3 } .
Differentiate \tan^{-1}\left(\dfrac{3a^2x-x^3}{a^3-3ax^2}\right), -\dfrac{1}{\sqrt{3}}<\dfrac{x}{a}<\dfrac{1}{\sqrt{3}}
Differentiate { \left( x+\dfrac { 1 }{ x } \right) }^{ x } w.r.t x.
Solve :
y = {x^{{x^{{x^{....\infty }}}}}} ,then \frac{{dy}}{{dx}}
If y = ( \log x ) ^ { x } - x ^ { \log x } then find \dfrac { d y } { d x }.
If y=(\sin x)^{\log x} then find \dfrac{dy}{dx}.
Find \dfrac{dy}{dx} of the function (\cos x)^y=(\cos y)^x w.r.t.x.
If x^2 + 2xy + y^2 = 42, then find \dfrac{dy}{dx}
If y = x ^ { x }, find \frac { d y } { d x }.
f(x)\begin{matrix} =2,\quad for\quad x<1 \\ =ax+b,\quad for\quad 1\le x<3 \\ =3,\quad for\quad x\ge 3 \end{matrix} is continuous at x=1 and x=3, find a and b.
f(x)\begin{matrix} =\cfrac { { x }^{ 2 }-16 }{ x-4 } +a\quad for\quad x<4 \\ =2,\quad for\quad x=4 \\ =3{ x }^{ 2 }+4x+b,\quad for\quad x>4 \end{matrix} is continuous at x=4, find a and b.
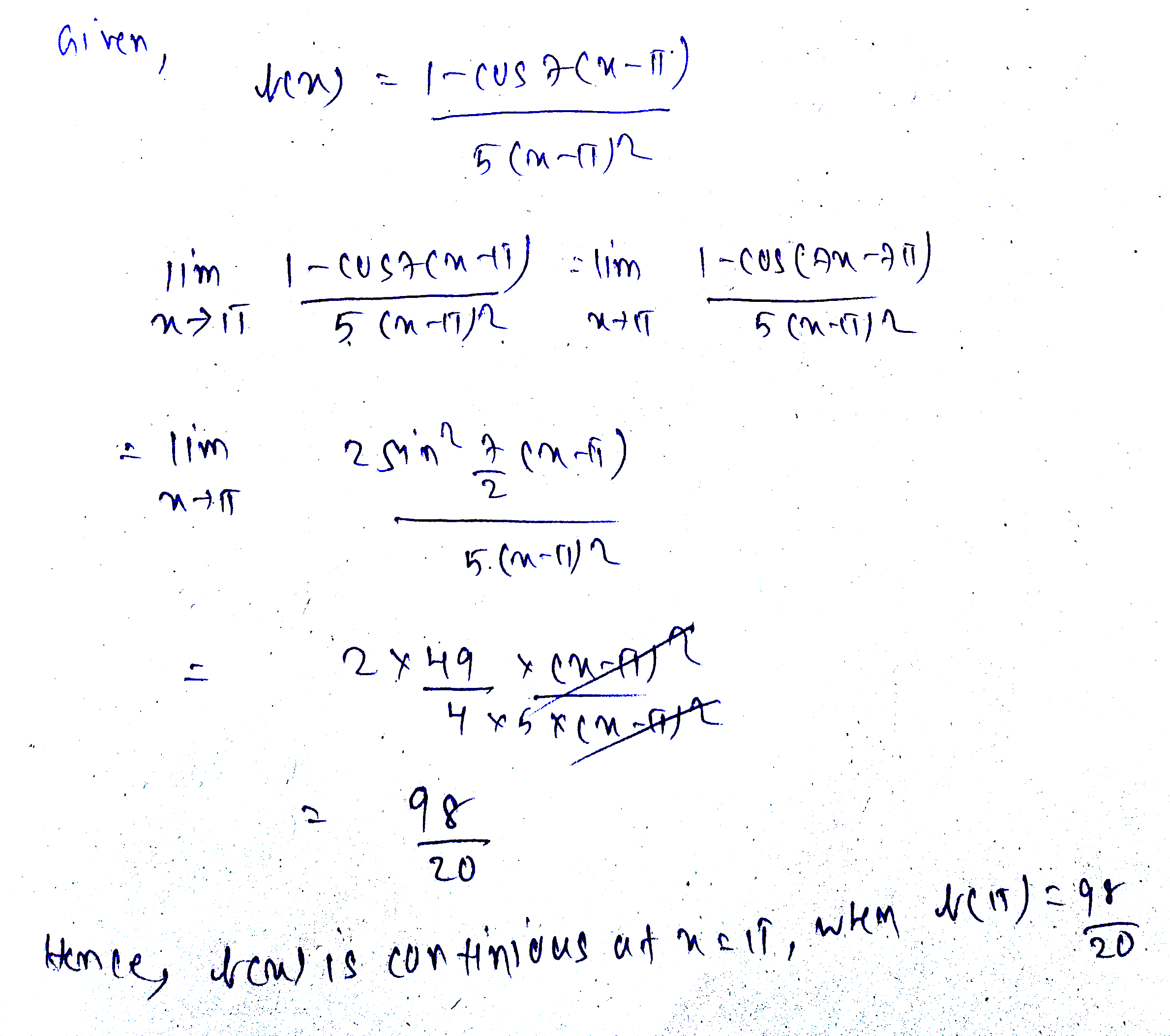
Extend the definition of the following by continuity f(x)=\cfrac{1-\cos{7(x-\pi)}}{5{(x-\pi)}^{2}} at the point x=\pi.
Check whether the function |x| is continuous at x=0.
Verify that y = ae^{-x} is a solution of
\dfrac{d^2y}{dx^2}=\dfrac{1}{y}\left(\dfrac{dy}{dx}\right)^2
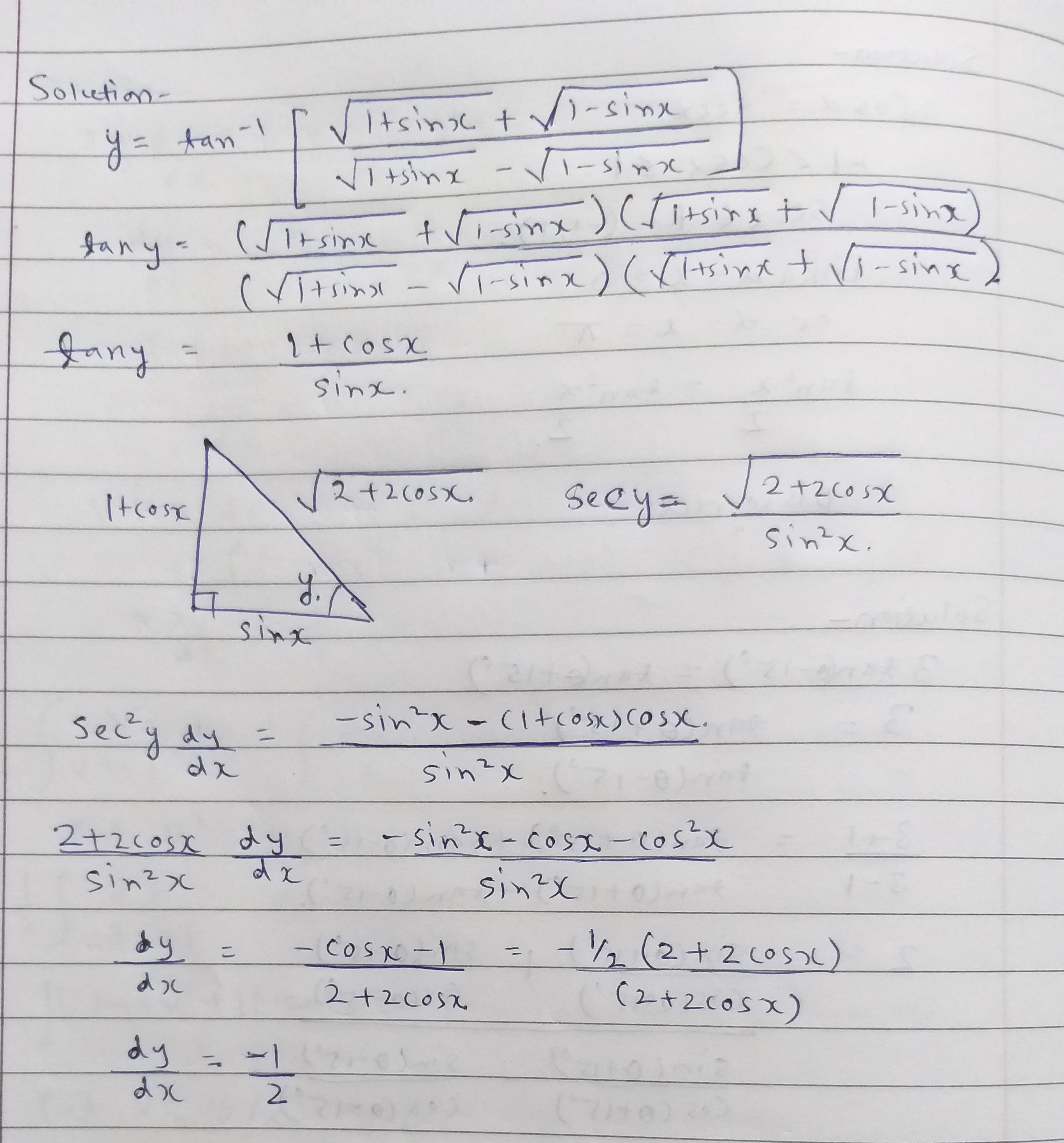
Find \dfrac{{dy}}{{dx}}\ if\,\,\,y = \,\,\,\,\,{\tan ^{ - 1}}\left[ {\dfrac{{\sqrt {1 + \sin x} + \sqrt {1 - \sin x} }}{{\sqrt {1 + \sin x} - \sqrt {1 - \sin x} }}} \right]
R.E.F image
Solution -
y = tan^{-1} [\frac{\sqrt{1+sin\,x}+\sqrt{1-sin\,x}}{\sqrt{1+sin\,x}-\sqrt{1-sin\,x}}]
tan\,y = \frac{(\sqrt{1+sin\,x}+\sqrt{1-sin\,x})(\sqrt{1+sin\,x}+\sqrt{1-sin\,x})}{\sqrt{1+sin\,x}-\sqrt{1-sin\,x}(\sqrt{1+sin\,x}+\sqrt{1-sin\,x})}
tan\,y = \frac{1+cos\,x}{sin\,x}
sec\,y = \frac{\sqrt{2+2\,cos\,x}}{sin^{2}x}
sec^{2}\frac{dy}{dx} = \frac{-sin^{2}x-(1+cos\,x)cos\,x}{sin^{2}x}
\frac{2+2cos\,x}{sin^{2}x} \frac{dy}{dx} = \frac{-sin^{2}x-cos\,x-cos^{2}x}{sin^{2}x}
\frac{dy}{dx} = \frac{-cosx-1}{2+2cos\,x} = \frac{-1/2(2+2\,cos\,x)}{(2+2\,cos\,x)}
\frac{dy}{dx} = \frac{-1}{2}
If x ^ { 2 } + y ^ { 2 } = 1 - \frac { 1 } { t } and x ^ { 4 } + y ^ { 4 } = t ^ { 2 } + \frac { 1 } { t ^ { 2 } }. Show that \frac { d y } { d x } = \frac { 1 } { x ^ { 3 } y }.
If x = a\left( {\theta - \sin \theta } \right)\,and\,y = a\left( {1 + \cos \theta } \right) then prove that \frac{{dy}}{{dx}} = - \cot \left( {\frac{\theta }{2}} \right).
\frac { d ^ { 2 } } { d x } \left( \tan ^ { - 1 } \left( \frac { \sqrt { 1 + x } - \sqrt { 1 - x } } { \sqrt { 1 + x } + \sqrt { 1 - x } } \right) \right) = \dots \dots \dfrac {x}{p \sqrt {({1-x^2})^3}} ( \left| x \right| > 1) then p =
If f ( x ) = \dfrac { 4 ^ { x } - 2 ^ { x + 1 } + 1 } { 1 - \cos x } , \text { for } x \neq 0 is continuous at x = 0, find f(0)
Find \dfrac {dy}{dx }, if x=a(1+\cos\theta) ,y=a(\theta+\sin\theta)
Let f(x+y)=f(x)+f(y) for all x,\ y\in R. If f(x) is continue at x=0 show that f(x) is continuous at all x
Differentiate the following w.r.t. x :
\cos^{-1}\left (\dfrac{3\cos x - 4\sin x}{5}\right)
Find the derivative of (\sin x) ^{\cos^{-1}x} with respect to x.
If y=\sin^{-1}x then find y".
Find the values of a and b so that the function f(x)=\left\{\begin{matrix} x^2+3x+a, & if & x\leq 1\\ bx+2, & if & x > 1\end{matrix}\right. is differentiable at each x\in R.
f(x)=\left\{\begin{matrix} x^2+3x+a, & if & x\leq 1\\ bx+2, & if & x > 1\end{matrix}\right.
f(x) is differentiable
\Rightarrow \displaystyle\underset{x\rightarrow 1^-}{lt}f^1(x)=\underset{x\rightarrow 1^+}{lt}f^1(x)
\Rightarrow \underset{x\rightarrow 1^-}{lt}(2x+3)=b
\therefore b=2+3=5
\therefore f(x) is continuous as it is differentiable
\Rightarrow \underset{x\rightarrow 1^-}{lt}f(x)=\underset{x\rightarrow 1^+}{lt}f(x)
\Rightarrow \underset{x\rightarrow 1^-}{lt}(x^2+3x+a)=\underset{x\rightarrow 1^+}{lt}(bx+2)
\Rightarrow 1+3+a=\underset{x\rightarrow 1^+}{lt}(5x+2)
\therefore a=7-4=3.
Let y=\sin^{-1}(\cos x) then find \dfrac{dy}{dx}.
If x=\left(t+\dfrac{1}{t}\right)^{a},\ y=a^{t+\tfrac{1}{t}}, then find \dfrac{dy}{dx}.
Find f'(1) for f(x)=e^{5x+3}.
Find the derivative of f(x)=2x^{2}+3x-5 at x=-1 Also prove that f'(0)+3f'(-1)=0
If y=\dfrac{x\sin^{-1}{x}}{\sqrt{1-x^{2}}}, find \dfrac{dy}{dx}
If x= a \cos ^3 \theta,\, y= a \sin ^3 \theta, then find \dfrac{{{d^2}y}}{{d{x^2}}} at \theta = \dfrac {\pi}{4}.
If ax+by^{2}=\cos y, then find \dfrac{dy}{dx}.
Prove that : \dfrac{d{(x^x)} }{{dx}}= {x^x}(1 + \log x)
The equation x=\dfrac{2a\theta }{1+\theta ^{2}},y=\dfrac{a(1-\theta ^{2})}{1+\theta ^{2}} (where 'a' is a constant) is the parametric equation of the curve.Find \dfrac{dy}{dx}
If x=\cos \theta and y=\tan \theta find \dfrac{dy}{dx}
Differentiate \tan ^ { - 1 } \left( \dfrac { 2 ^ { x + 1 } } { 1 - 4 ^ { x } } \right) w.r.t. x.
If { ax }^{ 2 }+2xy+{ by }^{ 2 } =0 then find \dfrac { dy }{ dx } .
If f(x) is continuous at f\left( x \right) =\begin{matrix} \dfrac { 1-\cos { kx } }{ { x }^{ 2 } } , \\ =\dfrac { 1 }{ 2 } , \end{matrix}\begin{matrix} for\ x\neq 0 \\ for\ x=0 \end{matrix}
Find k.
If \cos{x}=\cfrac{t}{\sqrt{1+t^2}} and \sin{y}=\cfrac{1}{\sqrt{1+t^2}} then prove that \cfrac{dy}{dx}=1
Find \dfrac{dy}{dx} :
3x^{2}+tanx+secx=y+6
y=\sin x find \dfrac{d^2y}{dx^2}
Let y=\log \dfrac{1+x}{1-x} then find \dfrac{dy}{dx}.
Find
\dfrac { dy}{ dx } , If x=4t and y=\dfrac { 4 }{ t }
Find \dfrac{dy}{dx} at t=\dfrac{\pi}{4}If x=cost and y=sin t
If {x^y} = {y^x}, then find \dfrac {dy}{dx}.
x^{y}=y^{x}.
Taking log on both sides
y \log x= x \log y.
Differentiating both the sides by uv rule
y.\dfrac{1}{x}+logx.\dfrac{dy}{dx}=x.\dfrac{1}{y}\dfrac{dy}{dx}+\log y
\Rightarrow \dfrac{y}{x}-logy =\dfrac{x}{y}.\dfrac{dy}{dx}-logx\dfrac{dy}{dx}
\Rightarrow \dfrac{y-xlogy}{x}=\dfrac{dy}{dx}(\dfrac{x-ylogx}{y})
\Rightarrow \dfrac{dy}{dx}=\dfrac{\dfrac{y-xlogy}{x}}{\dfrac{x-ylogx}{y}}
\Rightarrow \dfrac{dy}{dx}=\dfrac{y}{x}(\dfrac{y-xlogy}{x-ylogx}).
If x=\dfrac { a }{ 1+{ t }^{ 3 } } ,y=\dfrac { at }{ 1+{ t }^{ 3 } } then show that \dfrac { dy }{ dx } at t=1 is \dfrac{1}{p} then p =
If f\left( x \right) is continuous at x=0 where f\left( x \right) = {{\left( {{e^{3x}} - 1} \right)\sin x} \over {x\log \left( {1 + x} \right)}} for x \ne 0 find f\left( 0 \right)
Differentiate x=y+\dfrac{1}{y+\dfrac{1}{y+\dfrac{1}{y+\dfrac{1}{y+\dfrac{1}{y+...\infty}}}}} w.r.t x
If x=a \cos \theta +b\sin\theta , y=a \sin \theta -b \cos \theta , then show that { y }^{ 2 }\dfrac { d^{ 2 }{ y } }{ { dx }^{ 2 } } -x\dfrac { dy }{ dx } +y=0.
If \displaystyle \int{\dfrac{\sin{x}}{\sin{\left(x-\alpha\right)}}dx}=Ax+B\log{\sin{\left(x-\alpha\right)}}+c then find the value of \left(A,B\right)
If x ^ { y } = a ^ { x } , prove that \dfrac { d y } { d x } =\dfrac {\log a(\log x-1)}{(\log x)^2}.
x^{y}=a^{x}\Rightarrow y log x= x log a
y=\frac{x}{log x}log a
\frac{dy}{dx}= log a \left \{ \frac{log x-x.\frac{1}{x}}{(log x)^{2}} \right \}= log a\left \{ \frac{log x-1}{(log x)^{2}} \right \}
If { x }^{ 2 }=c^{ x-y }, then find \dfrac{ dy }{ dx }
Differentiate y={ 3 }^{ x },(x>0) w.r.t x
The parametric equations of a curve are x = 2t - \sin 2t, y = 5t + \cos 2t, for 0\leq t \leq \dfrac {1}{2}\pi. At the point P on the curve, the gradient of the curve is 2.
Show that the value of the parameter at P satisfies the equation 2 \sin 2t - 4 \cos 2t = 1.
Solve the differential equation
(tan^{-1}y-x)dy=(1+y^{2})dx
Show that f(x)=|x-3| is continuous but not differentiable at x=3.
Show that the function f(x)=\left\{\begin{matrix} x^m\sin\left(\dfrac{1}{x}\right), & x\neq 0\\ 0, & x=0\end{matrix}\right. is continuous but not differentiable at x=0, if 0 < m < 1.
If f(x)=\left\{\begin{matrix} ax^2-b & , & if & |x| <1 \\ \dfrac{1}{|x|} & , & if & |x| \geq 1\end{matrix}\right. is differentiable at x=1, find a, b.
Find the values of a and b, if the function f(x) defined by f(x)=\left\{\begin{matrix} x^2+3x+a, & x\leq 1\\ bx+2, & x >1\end{matrix}\right. is differentiable at x=1.
Find the values of a and b so that the function f(x)=\left\{\begin{matrix} x^2+3x+a, & if & x\leq 1\\ bx+2, & if & x > 1\end{matrix}\right. is differentiable at each x\in R.
Find \dfrac{dy}{dx} in xy=c^{2}.
Show that the function f(x)=\left\{\begin{matrix} x^m\sin\left(\dfrac{1}{x}\right), & x\neq 0\\ 0 &, x=0\end{matrix}\right. is differentiable at x=0, if m > 1.
Show that the function f(x)=\left\{\begin{matrix} |2x-3|[x], & x \geq 1\\ \sin\left(\dfrac{\pi x}{2}\right), & x < 1\end{matrix}\right. is continuous but not differentiable at x=1.
Show that the function f(x)=\left\{\begin{matrix} x^m\sin\left(\dfrac{1}{x}\right), & x\neq 0\\ 0, & x=0\end{matrix}\right. is neither continuous nor differentiable, if m\leq 0.
Show that the function f defined as follows
f(x)=\left\{\begin{matrix} 3x^2-2, & 0 < x\leq 1\\ 2x^2-x, & 1 < x\leq 2\\ 5x-4, & x > 2 \end{matrix}\right.
is continuous but not differentiable at x=2.
Examine the differentiability of the function f defined by f(x)=\left\{\begin{matrix} 2x+3, & if & -3\leq x < -2\\ x+1, & if & -2\leq x < 0\\ x+2, & if & 0\leq x\leq 1\end{matrix}\right..
Find whether the following function is differentiable at x=1 or not:
f(x)=\left\{\begin{matrix} x, & x\leq 1\\ 2-x, & 1\leq x\leq 2\\ -2+3x-x^2, & x > 2\end{matrix}\right..
Find \dfrac{dy}{dx},, if x=at^{2} and y=2\ at.
Discuss the continuity and differentiability of f(x)=e^{|x|}.
Find whether the following function is differentiable at x=1,2:
f(x)=\left\{\begin{matrix} x, & x\leq 1\\ 2-x, & 1\leq x\leq 2\\ -2+3x-x^2, & x > 2\end{matrix}\right..
Differentiate the following functions with respect to x
(\log x)^{\log x}
Discuss the continuity and differentiability of f(x)=|\log |x||.
Consider the given function.
f(x)=|\log |x||
Since, it is an absolute function. So, it a continuous function.
Let see the graph of the given function,
From the graph, it is clear that the function f(x) is not differentiable at x=-1 and x=1 but continuous for all x.
Write an example of a function which is everywhere continuous but fails to be differentiable exactly at five points.
Differentiate w.r.t. x:y=e^x +10^x+x^x
If x=\sin 3t and y=5t^2, find \dfrac{dy}{dx}.
Find \dfrac {dy}{dx}, if x= {3at} and y={3at^{2}}.
Find \dfrac{dy}{dx}, when x=e^t-3 and y=e^t+5.
Find \dfrac{dy}{dx}, if x=\sin 3t and y=4t^3.
Find \dfrac{dy}{dx}, if x=a\left( \theta +\sin { \theta } \right) and y=a\left( 1-\cos { \theta } \right) .
Find \dfrac{dy}{dx}, when
x=a \cos {\theta} and y=b \sin {\theta}
x^{x^{2}-3}+(x-3)^{x^2}
Find \dfrac {dy}{dx}, if x=b \sin^{2} {\theta} and y=a \cos^{2} {\theta}.
Find \dfrac{dy}{dx}, when
If x=e^t\sin t and y=e^{-t}
Differentiate the following function with respect to x.
\dfrac{x^n}{\sin x}.
Let S be the set of points where the function, f(x)=|2-|x-3||,x\epsilon R, is not differentiable.
Then \displaystyle \sum_{x\epsilon S}\,f(f(x)) is equal to ______.
f(x)= \begin{cases} \,\,\,\,\,\,1-x\,\,\,\,\,\,x<1 \\ \,\,\,\,\,\,x- 1\,\,\,\,\,\,\,1\le x< 3\\ { \begin{matrix} \,\,\,\,5-x \,\,\,3\le x< 5\\x-5\,\,x\ge 5 \end{matrix} } \end{cases}
Given f(x)=|2-|x-3||So here f(x) is not differentiable
at x=1,3,5
S=\{1,3,5\}
S=\{1,3,5\}
\displaystyle \therefore \sum_{x\in s}f(x)=f(f(1))+f(f(3))+f(f(5))
=f(0)+f(2)+f(0)
=1+1+1
=3
Let f defined on [0,1] be twice differentiable such that \left| f''(x) \right| \le 1 for all x\in [0,1]. If f(0)=f(1), then show that \left| f'(x) \right| <1 for all x\in [0,1]
Differentiate w.r.t. x:y=x^n+n^x +x^x +n^n
Show that a constant function is always differentiable.
Differentiate each of the following w.r.t.x:
\cos^{-1}2x
Differentiate each of the following w.r.t.x:
\tan^{-1}(\cos x)
Differentiate each of the following w.r.t.x:
\sin^{-1}(\cos x)
Differentiate each of the following w.r.t.x:
\tan^{-1}x^{2}
Differentiate each of the following w.r.t.x:
\sec^{-1}\sqrt{x}
Differentiate each of the following w.r.t.x:
(1+x^{2})\tan^{-1}x
Differentiate each of the following w.r.t.x:
(\cot^{-1}x^{2})^{3}
Differentiate each of the following w.r.t.x:
\cot^{-1}(e^{x})
Differentiate each of the following w.r.t.x:
\cot^{-1}x^{3}
Differentiate each of the following w.r.t.x:
\sin^{-1}\dfrac{x}{a}
Differentiate the following w.r.t.x:
\cot^{-1}\left(\sqrt{\dfrac{1+\cos x}{1-\cos x}}\right)
Differentiate the following w.r.t.x:
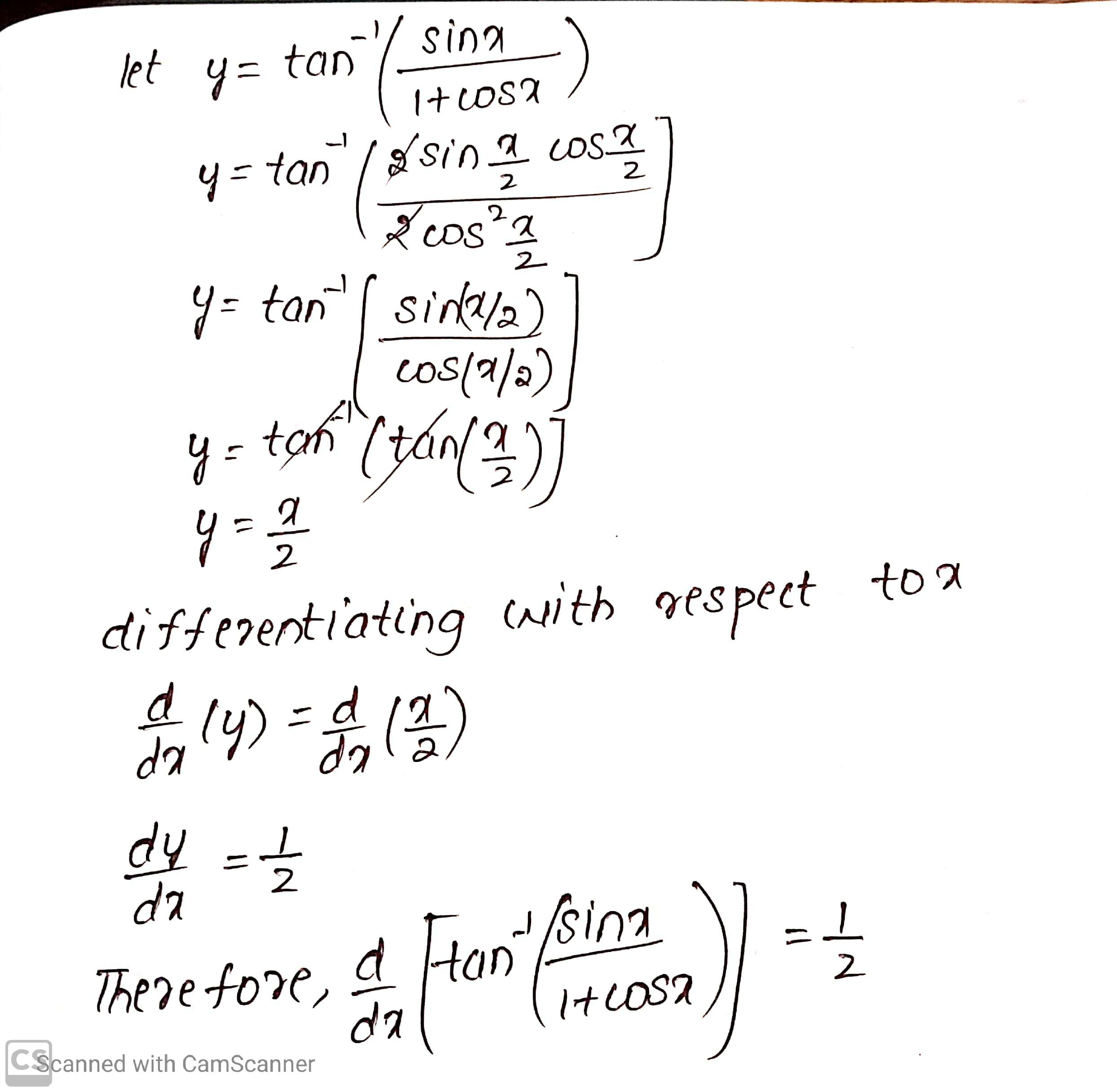
\tan^{-1}\left(\dfrac{\sin x}{1+\cos x}\right)
Differentiate each of the following w.r.t.x:
\tan(\sin^{-1}x)
Differentiate the following w.r.t.x:
\cot^{-1}\left(\dfrac{1+\cos x}{\sin x}\right)
Differentiate each of the following w.r.t.x:
\tan^{-1}(\cos\sqrt{x})
Differentiate each of the following w.r.t.x:
\sqrt{\sin^{-1}x^{2}}
Differentiate each of the following w.r.t.x:
\cot^{-1}\left(\sqrt{\dfrac{1+\cos 3x}{1-\cos 3x}}\right)
Differentiate the following w.r.t.x:
\cot^{-1}\left(\dfrac{\cos x-\sin x}{\cos x+\sin x}\right)
Differentiate each of the following w.r.t.x:
\sin^{-1}\left\{\sqrt{\dfrac{1-\cos x}{2}}\right\}
Differentiate the following w.r.t.x:
\tan^{-1}\left(\dfrac{\cos x+\sin x}{\cos x-\sin x}\right)
Differentiate the following w.r.t.x:
\tan^{-1}(\cot x)+\cot^{-1}(\tan x)
Differentiate the following w.r.t.x:
\csc^{-1}\left(\dfrac{1+\tan^{2}x}{2\tan x}\right)
Differentiate the following w.r.t.x:
e^{-\sin 2x}
Differentiate the following w.r.t.x:
\cos^{-1}(\sqrt{1-x^{2}})
Differentiate the following w.r.t.x:
\cos^{-1}\left\{\sqrt{\dfrac{1+x}{2}}\right\}
Differentiate each of the following w.r.t.x:
\sin^{-1}\left\{\sqrt{1-x^{2}}\right\}
Differentiate the following w.r.t.x:
\sin^{-1}\left(\dfrac{1-\tan^{2}x}{1+\tan^{2}x}\right)
Differentiate the following w.r.t.x:
\sec^{-1}\left(\dfrac{1+\tan^{2}x}{1-\tan^{2}x}\right)
Differentiate the following w.r.t.x:
\sin^{-1}\left(\sqrt{\dfrac{{1-x}}{2}}\right)
Differentiate each of the following w.r.t.x:
\cot^{-1}(\text cosec x+\cot x)
Differentiate the following w.r.t.x:
\sin^{-1}\left\{\dfrac{1}{\sqrt{1+x^{2}}}\right\}
Differentiate the following w.r.t.x:
\sec^{-1}\left(\dfrac{1}{\sqrt{1-x^{2}}}\right)
Differentiate the following w.r.t.x:
\tan^{-1}\left(\dfrac{x}{\sqrt{1-x^{2}}}\right)
Differentiate the following w.r.t.x:
\sin^{-1}\left\{2x\sqrt{1-x^{2}}\right\}
Differentiate the following w.r.t.x:
\sin^{-1}(3x-4x^{3})
Differentiate the following w.r.t.x:
\sin^{-1}(1-2x^{2})
Differentiate the following w.r.t.x:
\tan^{-1}\left(\dfrac{x}{1+\sqrt{1-x^{2}}}\right)
Differentiate the following w.r.t.x:
\cot^{-1}\left(\dfrac{\sqrt{1-x^{2}}}{x}\right)
Differentiate the following w.r.t.x:
\sec^{-1}\left(\dfrac{1}{1-2x^{2}}\right)
Differentiate the following w.r.t.x:
\tan^{-1}\left(\dfrac{1+x}{1-x}\right)
Differentiate the following w.r.t.x:
\tan^{-1}\left\{\dfrac{\sqrt{1+a^{2}x^{2}}-1}{ax}\right\}
Differentiate the following w.r.t.x:
\sin^{-1}\left\{2ax\sqrt{1-a^{2}x^{2}}\right\}
Differentiate the following w.r.t.x:
\sin^{-1}\left(\dfrac{1}{\sqrt{1+x^{2}}}\right)
Differentiate the following w.r.t.x:
\sec^{-1}\left(\dfrac{1+x^{2}}{1-x^{2}}\right)
Differentiate the following w.r.t.x:
\cos^{-1}\left(\dfrac{1-x^{2n}}{1+x^{2n}}\right)
Differentiate the following w.r.t.x:
\tan^{-}\left\{\dfrac{x}{\sqrt{a^{2}-x^{2}}}\right)
Differentiate the following w.r.t.x:
\tan^{-1}\left(\dfrac{3x-x^{3}}{1-3x^{2}}\right)
Differentiate the following w.r.t.x:
\sec^{-1}\left(\dfrac{x^{2}+1}{x^{2}-1}\right)
Differentiate the following w.r.t.x:
\text cosec^{-1}\left(\dfrac{1+x^{2}}{2x}\right)
Differentiate the following w.r.t.x:
\cot^{-1}\left(\dfrac{1+x}{1-x}\right)
If y=\sin^{-1}\left(\dfrac{2x}{1+x^{2}}\right)+\sec^{-1}\left(\dfrac{1+x^{2}}{1-x^{2}}\right), show that \dfrac{dy}{dx}=\dfrac{4}{(1+x^{2})}
Differentiate the following w.r.t.x:
\tan^{-1}\left(\dfrac{e^{2x}+1}{e^{2x}-1}\right)
Differentiate the following w.r.t.x:
\tan^{-1}\left(\dfrac{5x}{1-6x^{2}}\right)
Differentiate the following w.r.t.x:
\tan^{-1}\left(\dfrac{3-2x}{1+6x}\right)
Differentiate the following w.r.t.x:
\tan^{-1}\left(\dfrac{\sqrt{a}+\sqrt{x}}{1-\sqrt{ax}}\right)
If y=\sec^{-1}\left(\dfrac{x+1}{x-1}\right)+\sin^{-1}\left(\dfrac{x-1}{x+1}\right), show that \dfrac{dy}{dx}=0.
If y=\tan^{-1}\left(\dfrac{ax-b}{bx-a}\right), prove that \dfrac{dy}{dx}=\dfrac{1}{(1+x^{2})}
Differentiate \sin^{-1}\left(\dfrac{2^{x+1}}{1+4^{x}}\right) w.r.t.x.
Differentiate the following w.r.t.x:
\sin^{-1}\left\{\dfrac{x^{2}}{\sqrt{x^{4}+a^{4}}}\right\}
Differentiate each of the following w.r.t.x:
\tan^{-1}\left(\dfrac{2x}{1+15x^{2}}\right)
Differentiate \tan^{-1}\left(\dfrac{2x}{1-x^2}\right) with respect to \sin^{-1}\left(\dfrac{2x}{1+x^2}\right)
Differentiate \cos^{-1}\left(\dfrac{1-x^2}{1+x^2}\right) with respect to \tan^{-1}\left(\dfrac{3x-x^3}{1-3x^2}\right).
Find the second derivation of:
x^{11}
Differentiate \tan^{-1}\left(\dfrac{x}{\sqrt{1-x^2}}\right) with respect to \cos^{-1}(2x^2-1)
Find \dfrac {dy}{dx}, when y=\dfrac {x\cos^{-1}x}{\sqrt {1-x^2}}.
Find \dfrac {dy}{dx}, when y=(\sin^{-1}x)^{x}.
If y=\dfrac {\sin ^{-1}x}{\sqrt {1-x^2}}, prove that (1-x^2)\dfrac {dy}{dx}=(xy+1)
Differentiate \tan^{-1}\left(\dfrac{\sqrt{1-x^2}}{x}\right) w.r.t. \cos^{-1}(2x\sqrt{1-x^2}), when x\neq 0.
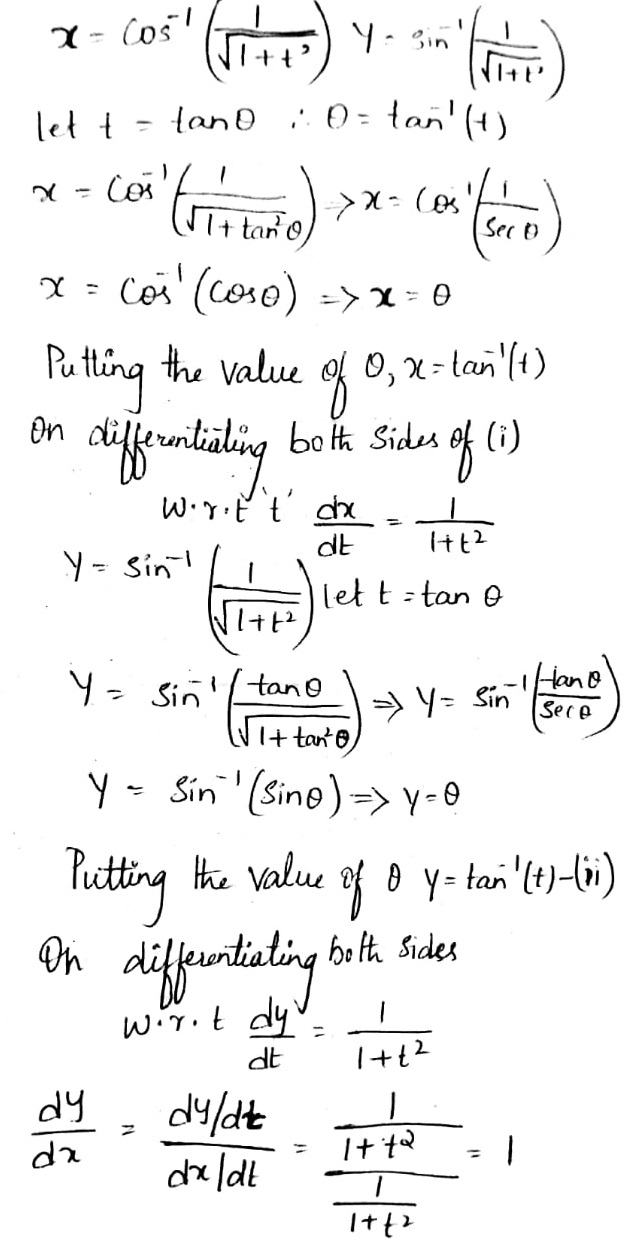
Find \dfrac{dy}{dx}, when x=\cos^{-1}\dfrac{1}{\sqrt{1+t^2}}, y=\sin^{-1}\dfrac{t}{\sqrt{1+t^2}}.
Find \dfrac {dy}{dx}, when y=x^{(\cos^{-1}x)}.
Find the second derivation of:
x^{3}\log x
If y=\tan^{-1}\dfrac {a}{x}+\log \sqrt {\dfrac {x-a}{x+a}}, prove that \dfrac {dy}{dx}=\dfrac {2a^3}{(x^4 - a^4)}
Find the second derivation of:
\tan x
Find the second derivation of:
5^{x}
Find the second derivation of \sin\ 3x\cos 5x.
Find the second derivation of:
e^{2x}\cos 3x
Find the second derivative of e^{3x}\sin 4x.
Fill in the blanks
Let f(x)=x\left | x \right |. The set of points Where f(x) is twice differentiable is ...
We have Clearly f'' (x) exists at every point except except x=0 Thus f(x) is twice differentiable on R-{0}
If f(x)=\cos ^{-1} \dfrac{1}{\sqrt{13}}(2 \cos x-3 \sin x)
+\sin ^{-1} \dfrac{1}{\sqrt{13}} \times(2 \cos x+3 \sin x) \text { w.r.t. } \sqrt{1+x^{2}}
then find \dfrac { d f(x)} {d x} at x=3 / 4
If x \in\left(0, \dfrac{\pi}{2}\right), then show that \dfrac{d}{d x} \cos ^{-1}\left\{\dfrac{7}{2}(1+\cos 2 x)+\sqrt{\left(\sin ^{2} x-48 \cos ^{2} x\right)} \sin x\right\}
=1+\dfrac{7 \sin x}{\sqrt{\sin ^{2} x-48 \cos ^{2} x}}
A function is represented parametrically by the equations x = \dfrac{1 + t}{t^3} ; y = \dfrac{3}{2t^2} + \dfrac{2}{t}, then the value of \left|{\dfrac{dy}{dx} - x{\left(\dfrac{dy}{dx}\right)^3}}\right| is.
The derivative of \sec^{-1} \left ( \frac{1} {2x^{2} - 1} \right ), then f^{'}(x) at x = e is ________________. (IIT-JEE, 1985)
\operatorname{Let} g(x) be differentiable on R and \int_{\sin t}^{1} x^{2} g(x) d x\\=(1-\sin t), where t \in\left(0, \dfrac{\pi}{2}\right) . Then the value of g\left(\dfrac{1}{\sqrt{2}}\right)is
Let u(x) and v(x) are differentiable function such that \dfrac{u(x)}{v(x)}=7 . If \dfrac{u^{\prime}(x)}{v^{\prime}(x)}=p and \left(\dfrac{u(x)}{v(x)}\right)^{\prime}=q, then \dfrac{p+q}{p-q} has the value equal to \cdots
Let F(x) = f(x) g(x) h(x) for all real x, where f(x), g(x) and h(x) are differentiable functions. At some point x_0, F'(x_0) = 21F(x_0), f'(x_0) = 4f(x_0), g'(x_0) = -7g(x_0) and h'(x_0) = kh(x_0). Then k= _______________. (IIT-JEE, 1997)
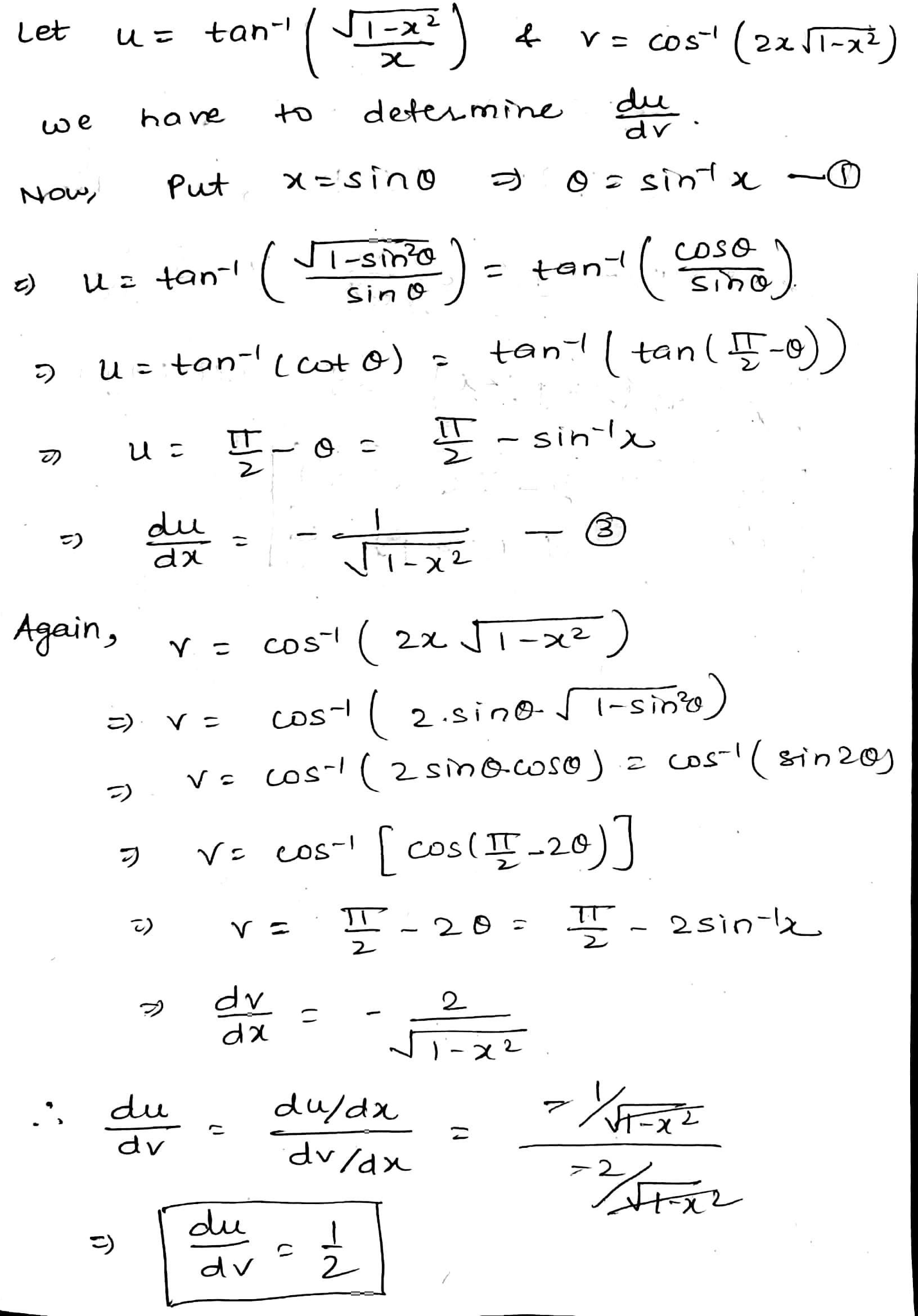
Differentiate \tan ^{-1} \left ( \dfrac{\sqrt{1 - x^{2}}}{x} \right ) with respect to \cos ^{-1} (2x \sqrt{1 - x^{2}}), when x \neq 0.
Differentiate \tan ^{-1} \dfrac{x}{\sqrt{1 - x^{2}}} with respect to \sin ^{-1} (2x \sqrt{1 - x^{2}})
If y = \cos ^{-1} \left \{ \dfrac{3x + 4\sqrt{1 - x^{2}}}{5} \right \} then find \dfrac{dy}{dx}
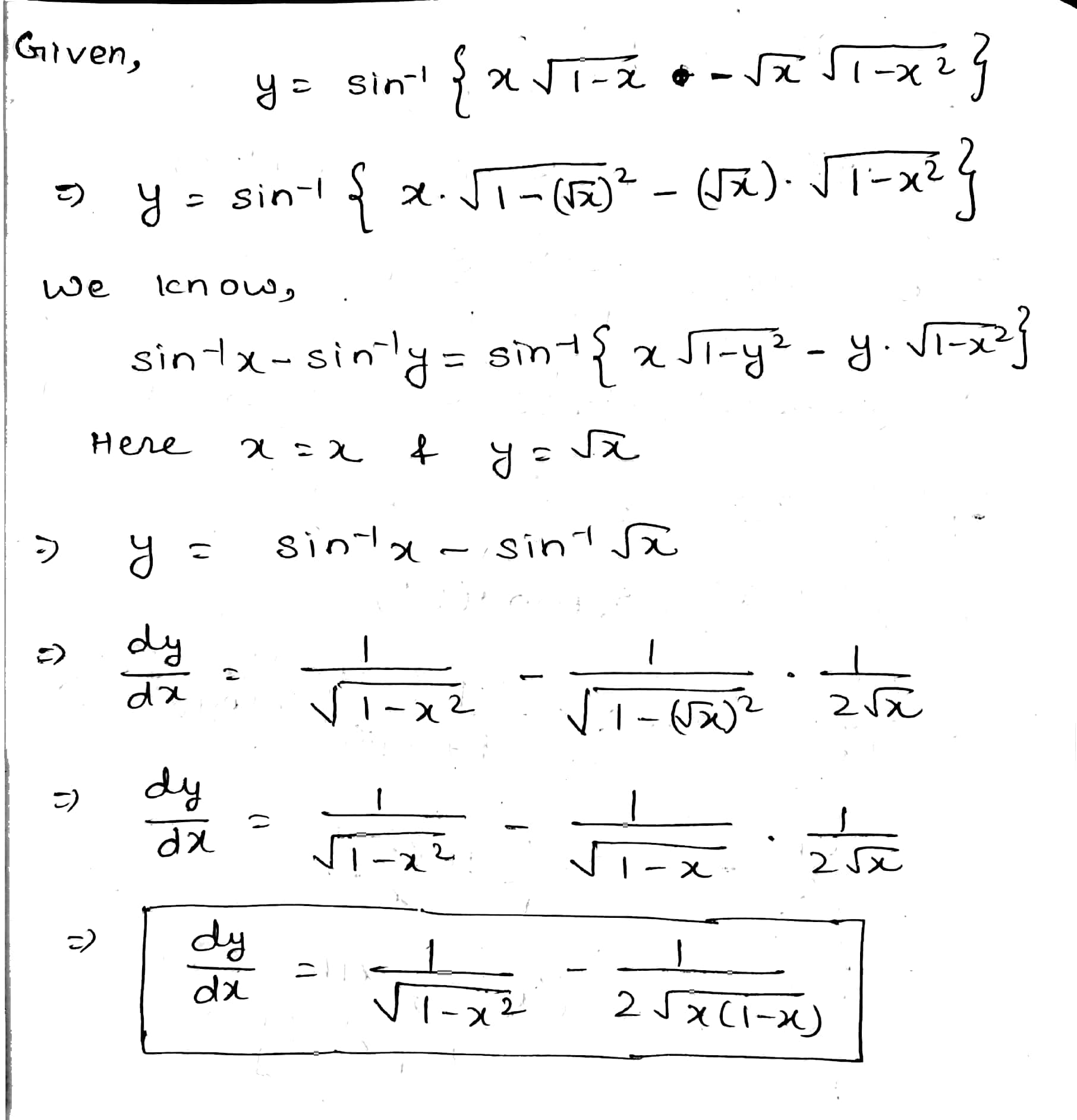
Find \dfrac{dy}{dx} if y = \sin ^{-1} \left [ x\sqrt{1 - x} - \sqrt{x} \sqrt{1 - x^{2}} \right ]
Differentiate the following function with respect to x
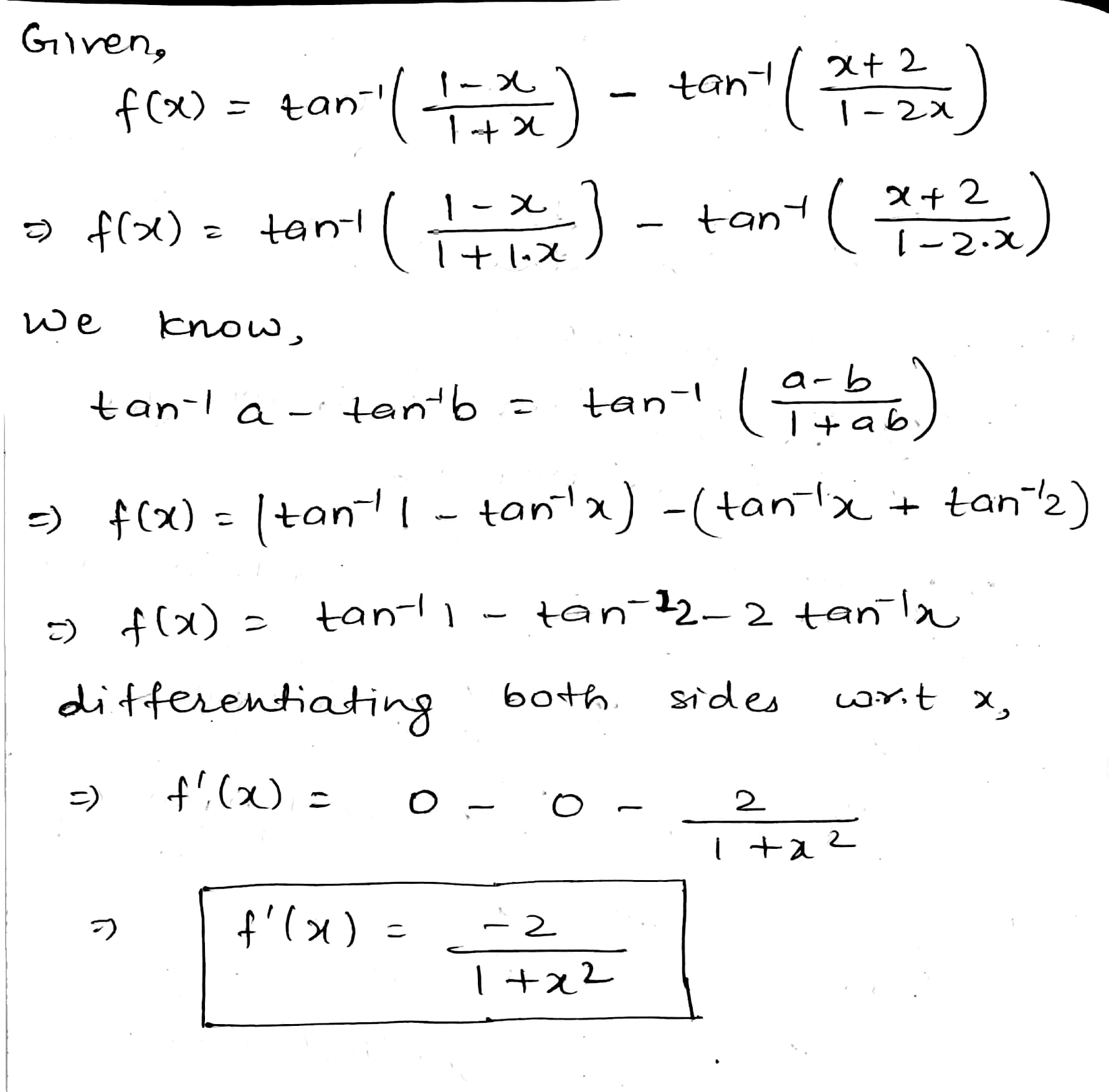
f(x) = \tan ^{-1} \left ( \dfrac{1 - x}{1 + z} \right )- \tan ^{-1} \left ( \dfrac{x + 2}{1 - 2x} \right ).
If y = \cos ^{-1} \left ( \dfrac{2^{x+1}}{1 + 4^{x}} \right ), then find \dfrac{dy}{dx}
Differentiate the following with respect to :
\tan ^{-1} \left ( \dfrac{\sqrt{1 + x} - \sqrt{1 - x}}{\sqrt{1 + x} + \sqrt{1 - x}} \right )
Differentiate \tan ^{-1} \left [ \dfrac{\sqrt{1 + x^{2}} - 1}{x} \right ] with respect to x.
Prove that : \dfrac{d}{dx} \left [ \dfrac{x}{2}\sqrt{a^{2} - x^{2}} + \dfrac{a^{2}}{2} \sin ^{-1} \left ( \dfrac{x}{a} \right ) \right ] = \sqrt{a^{2} - x^{2}}
Differentiate the following w.r.t. x: \tan ^{-1}(\log x)
If f(x) be a differentiable function such that f'(x) = g(x) , g"(x) exists, |f(x)| < 1 and (f(0))^2 + (g(0))^2 = 9. Prove that there is a point c where c \in (-3, 3) such that g(c). g"(c) < 0
Differentiate with respect to x:
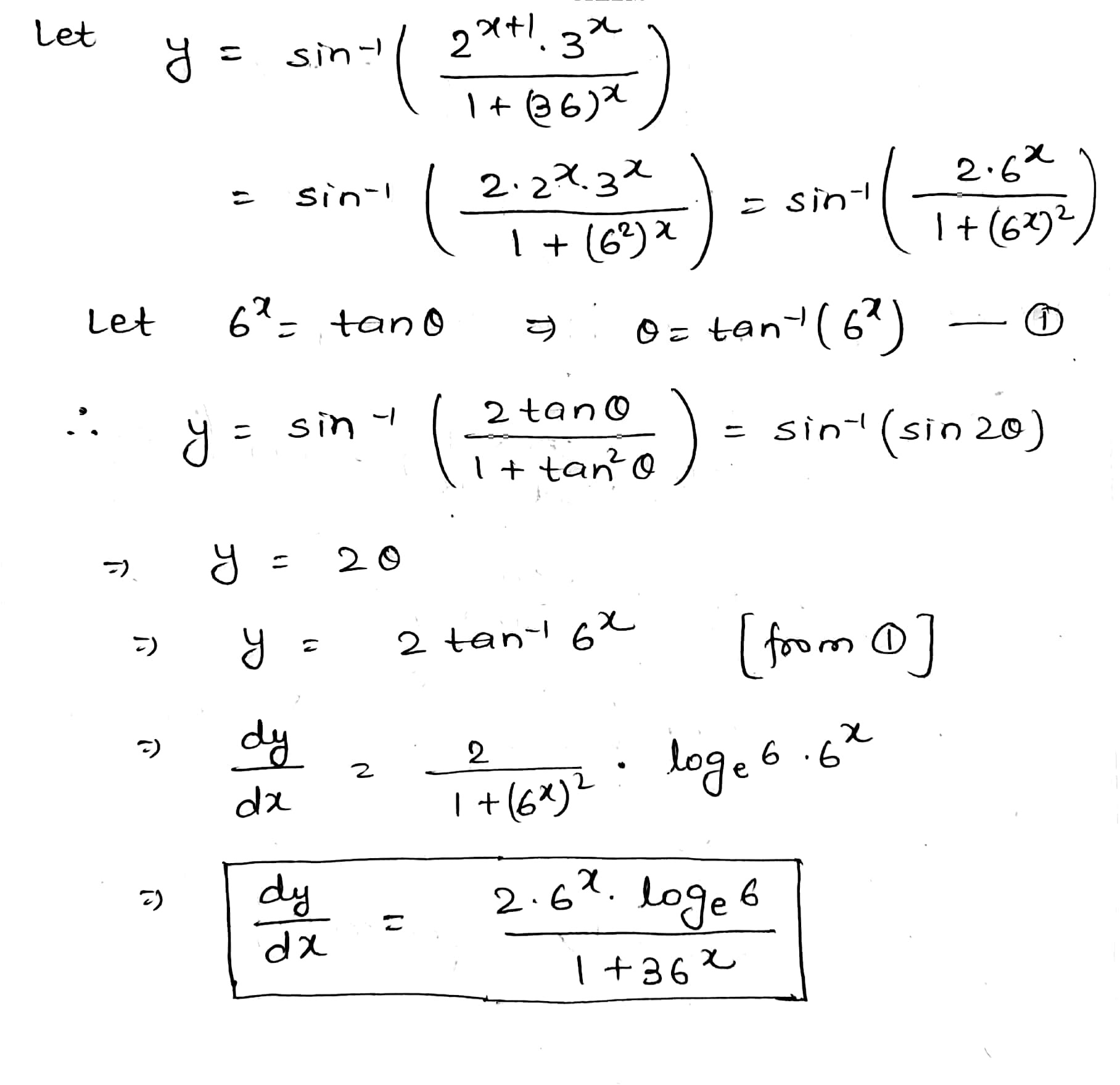
\sin ^{-1} \left ( \dfrac{2^{x + 1}. 3^x}{1 + (36)^{x}} \right )
Using derivative, prove that: \sec ^{-1} x+\operatorname{cosec}^{-1} x=\dfrac{\pi}{2} \quad \ldots [for \left.|x| \geq 1\right]
Differentiate \tan ^{-1} \left ( \dfrac{\sqrt{1 + x^{2}} - 1}{x} \right ) with respect to \tan ^{-1} x, when x \neq 0.
Using derivative, prove that: \tan ^{-1} x+\cot ^{-1} x=\dfrac{\pi}{2}
Differentiate the following w.r.t. x: \sin ^{-1}\left(\sqrt{\dfrac{1+x^{2}}{2}}\right)
Differentiate the following w.r.t. x: \operatorname{cosec}^{-1}\left(e^{-x}\right)
Differentiate the following w.r.t. \mathrm{x}: \operatorname{cosec}^{-1}\left[\dfrac{1}{\cos \left(5^{x}\right)}\right]
Differentiate the following w.r.t. x: \cos ^{-1}\left(\sqrt{\dfrac{1+\cos x}{2}}\right)
Differentiate the following w.r.t. x: \cot ^{-1}\left[\cot \left(e^{x^{2}}\right)\right]
Differentiate the following w.r.t. x: \tan ^{-1}(\sqrt{x})
Differentiate the following w.r.t. x: \cos ^{-1}\left(1-x^{2}\right)
Differentiate the following w.r.t. x: \cos ^{-1}\left(\dfrac{\sqrt{1-\cos \left(x^{2}\right)}}{2}\right)
Differentiate the following w.r.t. x: \sin ^{-1}\left(x^{\frac{3}{2}}\right)
Differentiate the following w.r.t. x: \cot ^{-1}\left(4^{x}\right)
Find the second order derivatives of the following :
2x^{5} - 4x^{3} - \frac{2} {x^{2}} - 9
Differentiate the following w.r.t. x: \tan ^{-1}\left[\dfrac{1-\tan \left(\dfrac{x}{2}\right)}{1+\tan \left(\dfrac{x}{2}\right)}\right]
Differentiate the following w.r.t x : \sin \left [ 2 \tan^{-1} \left( \sqrt{\frac{1 - x}{1 + x}} \right ) \right ]
Differentiate the following w.r.t x : \tan^{-1} \left( \frac{ \sqrt{x}(3 - x)}{1 - 3x} \right )
Differentiate the following w.r.t x : \tan^{-1} \left[ \sqrt{ \frac{ \sqrt{1 + x^2} + x}{\sqrt{1 + x^2} - x}} \right ]
Differentiate the following w.r.t x : \cos^{-1} \left( \frac{ \sqrt{1 + x} - \sqrt{1 - x}}{2} \right )
Differentiate the following w.r.t. x: \tan ^{-1}\left(\sqrt{\dfrac{1+\cos x}{1-\cos x}}\right)
Find second order derivatives of the following :
e^{2x} \cdot \tan x
Find the second order derivatives of the following:
\log \left(\log x \right)
Find the second order derivatives of the following :
x^{3} \cdot \log x
Find the second order derivatives of the following :
x^{x}
Differentiate the following w.r.t. x: \cot ^{-1}\left(\dfrac{\sin 3 x}{1+\cos 3 x}\right)
Differentiate the following w.r.t. x: \tan ^{-1}\left(\dfrac{\cos 7 x}{1+\sin 7 x}\right)
Find the second order derivatives of the following :
e^{4x} \cdot \cos 5x
Differentiate the following w.r.t. x: \tan ^{-1}(\operatorname{cosec} x+\cot x)
Differentiate the following w.r.t. x: \tan ^{-1}\left[\dfrac{1+\cos \left(\dfrac{x}{3}\right)}{\sin \left(\dfrac{x}{3}\right)}\right]
Differentiate the following w.r.t. x: \cos ^{-1}\left(\dfrac{\sqrt{3} \cos x-\sin x}{2}\right)
Differentiate the following w.r.t. x: \operatorname{cosec}^{-1}\left[\dfrac{10}{6 \sin \left(2^{x}\right)-8 \cos \left(2^{x}\right)}\right]
Differentiate the following w.r.t. x: \sin ^{-1}\left(\dfrac{4 \sin x+5 \cos x}{\sqrt{41}}\right)
Differentiate the following w.r.t. \mathrm{x}: \cos ^{-1}\left[\dfrac{3 \cos \left(e^{x}\right)+2 \sin \left(e^{x}\right)}{\sqrt{13}}\right]
Differentiate the following w.r.t. x: \sin ^{-1}\left(\dfrac{1-x^{2}}{1+x^{2}}\right)
Differentiate the following w.r.t. x: \cot ^{-1}\left[\dfrac{\sqrt{1+\sin \left(\dfrac{4x}{3}\right)}+\sqrt{1-\sin \left(\dfrac{4 x}{3}\right)}}{\sqrt{1+\sin \left(\dfrac{4 x}{3}\right)}-\sqrt{1-\sin \left(\dfrac{4 x}{3}\right)}}\right]
Differentiate the following w.r.t. x: \sin ^{-1}\left(\dfrac{\cos \sqrt{x}+\sin \sqrt{x}}{\sqrt{2}}\right)
Differentiate the following w.r.t. x: \cos ^{-1}\left(\dfrac{3 \cos 3 x-4 \sin 3 x}{5}\right)
Differentiate the following w.r.t. x: \cos ^{-1}\left(\dfrac{1-x^{2}}{1+x^{2}}\right)
Differentiate the following w.r.t. x: \tan ^{-1}\left(\dfrac{2 x}{1-x^{2}}\right)
Differentiate the following w.r.t. x: \sin ^{-1}\left(2 x \sqrt{1-x^{2}}\right)
Differentiate the following w.r.t. x: \sin ^{-1}\left(\dfrac{1-25 x^{2}}{1+25 x^{2}}\right)
Differentiate the following w.r.t. x: \sin ^{-1}\left(\dfrac{4^{x+\frac{1}{2}}}{1+2^{4 x}}\right)
Differentiate the following w.r.t. x: \cos ^{-1} \dfrac{\left(1-9^{x}\right)}{\left(1+9^{x}\right)}
Differentiate the following w.r.t. x: \cot ^{-1}\left(\dfrac{1-\sqrt{x}}{1+\sqrt{x}}\right)
Differentiate the following w.r.t. x: \sin ^{-1}\left(\dfrac{1-x^{3}}{1+x^{3}}\right)
Differentiate the following w.r.t. x: \tan ^{-1}\left(\dfrac{2 x^{\tfrac{5}{2}}}{1-x^{5}}\right)
Differentiate the following w.r.t. x: \cos ^{-1}\left(\dfrac{e^{x}-e^{-x}}{e^{x}+e^{-x}}\right)
Find \dfrac{d^{2}y} {dx^{2}} of the following :
x = a \left(\theta - \sin \theta\right), y = a \left(1 - \cos \theta\right)
Differentiate the following w.r.t. x: \cos ^{-1}\left(3 x-4 x^{3}\right)
Differentiate the following w.r.t. x: \tan ^{-1}\left[\dfrac{2^{x}+2}{1-3\left(4^{x}\right)}\right]
Differentiate the following w.r.t. x: \tan ^{-1}\left(\dfrac{2^{x}}{1+2^{2 x+1}}\right)
Differentiate the following w.r.t. \mathrm{x}: \cot ^{-1}\left(\dfrac{a^{2}-6 x^{2}}{5 a x}\right)
Differentiate the following w.r.t. x: \tan ^{-1}\left(\dfrac{2 \sqrt{x}}{1+3 x}\right)
Differentiate the following w.r.t. \mathrm{x}: \tan ^{-1}\left(\dfrac{a+b \tan x}{b-a \tan x}\right)
Differentiate the following w.r.t. x: \cot ^{-1}\left(\dfrac{1+35 x^{2}}{2 x}\right)
Find \dfrac{d^{2}y} {dx^{2}} of the following:
x = 2at^{2}, y= 4at
Differentiate the following w.r.t. x: \tan ^{-1}\left(\dfrac{5-x}{6 x^{2}-5 x-3}\right)
Differentiate the following w.r.t. x: \tan ^{-1}\left(\dfrac{8 x}{1-15 x^{2}}\right)
Differentiate the following w.r.t. x: \cot ^{-1}\left(\dfrac{4-x-2 x^{2}}{3 x+2}\right)
If sec^{-1} \left(\frac{7x^{3} - 5y^{3}} {7^{3} + 5y^{3}} \right) = m, show \frac{d^{2}y} {dx^{2}} = 0.
If y= \sin \left(m \cos^{-1}x \right), then show that \left(1 - x^{2}\right) \frac{d^{2}y} {dx^{2}} - x\frac{dy} {dx} + m^{2}y = 0
If x = \cos t, y = e^{mt}, show that \left(1 - x^{2} \right) \frac{d^{2}y} {dx^{2}} - x \frac{dy} {dx} - m^{2}y = 0
If y = e^{m \tan^{-1} x}, show that \left(a + x^{2} \right) \frac{d^{2}y} {dx^{2}} + \left(2x - m\right) \frac{dy} {dx} = 0
If x^{2} + 6xy + y^{2} = 10, show that \frac{d^{2}} {dx^{2}} = \frac{80} {\left(3x + y \right)^{3}}
Find \frac{d^{2}y} {dx^{2}} of the following:
x = a \cos \theta, y = b \sin \theta at \theta = \frac{\pi} {4}
Find \frac{d^{2}y} {dx^{2}} of the following :
x = \sin \theta, y = \sin^{3} \theta at \theta = \frac{\pi} {2}
If y = x + \tan x, show that \cos^{2}x \cdot \frac{d^{2}y} {dx^{2}} - 2y + 2x = 0
If x = a \sin t - b \cos t, y = a \cos t + b \sin t, show that \frac{d^{2}y} {dx^{2}} = - \frac{x^{3} + y^{2}} {y^{3}}
If x = at^{2} and y = 2at, then show that xy \frac{d^{2}y} {dx^{2}} + a = 0
Differentiate w.r.t .x.
(\sin x)^{x} + \sin ^{-1} \sqrt{x}
Differentiate \tan ^{-1}\left(\dfrac{\cos x}{1+\sin x}\right) w . r . t . \sec ^{-1} x
Differentiable the functions given in Exercises 1 to 11 w.r.t .x.
y = \sqrt{\dfrac{(x - 1)(x -2)}{(x - 3)(x - 4) (x - 5)}}
Differentiable \space the \space following \space wrt\space x: y = \dfrac{\cos x}{\log x}
If a and y connected parametrically by the equation given in Exercise 1 to 10 , without eliminating the parameter ,find \dfrac{dy}{dx}
x = 2 at^{2} , y = at^{4}
Differentiate the following w.r.t. x:
e^{\sin^{-1}} x
Differentiate the following w.r.t. x:
y = \dfrac{e^{x}}{\sin x}
Differentiate the following wrt x:
\sin ( \tan^{-1}e^{-x})
Differentiate w.r.t .x.
y = x^{x \cos x} + \dfrac{x^{2}+1}{x^{2}- 1}
Differentiate \tan ^{-1}\left(\dfrac{\sqrt{1+x^{2}}-1}{x}\right) w \cdot r \cdot t \tan ^{-1}\left(\dfrac{2 x \sqrt{1-x^{2}}}{1-2 x^{2}}\right)
If a and y connected parametrically by the equation given in Exercise 1 to 10 , without eliminating the parameter ,find \dfrac{dy}{dx}
Undefined control sequence /cfrac
If a and y connected parametrically by the equation given in Exercise 1 to 10 , without eliminating the parameter ,find \dfrac{dy}{dx}
x = a \sec \theta , y = b \tan \theta
If a and y connected parametrically by the equation given in Exercise 1 to 10 , without eliminating the parameter ,find \dfrac{dy}{dx}
x = \cos \theta - \cos 2 \theta y = \sin \theta - \sin 2 \theta
If a and y connected parametrically by the equation given in Exercise 1 to 10 , without eliminating the parameter ,find \dfrac{dy}{dx}
x = a \cos \theta , y = b \cos \theta
If a and y connected parametrically by the equation given in Exercise 1 to 10 , without eliminating the parameter ,find \dfrac{dy}{dx}
x = \sin t , y \cos 2t
If a and y connected parametrically by the equation given in Exercise 1 to 10 , without eliminating the parameter ,find \dfrac{dy}{dx}
x = a (\theta - \sin \theta), y = ( 1 + \cos \theta)
If a and y connected parametrically by the equation given in Exercise 1 to 10 , without eliminating the parameter ,find \dfrac{dy}{dx}
x = 4t , y = \dfrac{4}{t}
If a and y connected parametrically by the equation given in Exercise 1 to 10 , without eliminating the parameter ,find \dfrac{dy}{dx}
x = a (\cos t + \log \tan \dfrac{t}{2}) , y = a \sin t
If a and y connected parametrically by the equation given in Exercise 1 to 10 , without eliminating the parameter ,find \dfrac{dy}{dx}
x = a ( \cos \theta + \sin \theta) , y = a ( \sin \theta - \theta \cos \theta )
Find the second order derivatives of the function x^{3} \log x
Find the second order derivatives of the function given in Exercise 1 to 10 .
\sin (\log x)
Find the second order derivatives of the function given function:
x^{2} + 3x + 2
Find the second order derivatives of the function given below:
\tan^{-1} x
Find the second order derivatives of the function given in Exercise 1 to 10 .
x . \cos x
Find the second order derivatives of the function given in Exercise 1 to 10 .
e^{6x}cos 3 x
Find the second order derivatives of the function given below.
e^{x} \sin 5x
If y = \cos^{-1} x find \dfrac {dy}{dx}
Find the second order derivatives of the function given in Exercise 1 to 10 .
\log (\log x)
Find the second order derivatives of the function given below:
\log x
If y = \cos^{-1} \left \{ \dfrac{3x +4\sqrt{1 - x^{2}}}{5} \right \} Find \dfrac{dy}{dx}
Differentiate
If y = \sin^{-1} \left [ \dfrac{1 - \sqrt{x}}{1 +\sqrt{x}} \right ] + \cos^{-1}\left [ \dfrac{1 + \sqrt{x}}{1 - \sqrt{x}} \right ] , find \dfrac{dy}{dx}
Differentiate sin^{-1}\left ( \dfrac{t}{\sqrt{1 + t^{2}}} \right ) w .r.t.x
\cos^{-1}\left ( \dfrac{1}{\sqrt{1 + t^{2}}} \right )
Show that following functions are differentiable for every value of x:
Constant function, f(x)=c, where c is a constant
Differentiate w.r.t.x the function in Exercise 1 to 11 .
\sin^{-1} (x\sqrt{x}) , 0 \leq x \leq 1
If f : [-5 , 5] \rightarrow R is differentiable function if f (x) does not vanish anywhere , then prove that f (-5) \neq f (5).
Show that following functions are differentiable for every value of x:
Identify functions, f(x)=x
Find \dfrac{dy}{dx} if y = 12 ( 1 - \cos t) ; x = 10 ( t - \sin t)
If y = 500 e ^{7x} + 600^{-7x} show that \dfrac{d^{2}y}{dx^{2}} = 49 y
Show that following functions are differentiable for every value of x:
f(x)=e^x
Examine the function f(x)=\begin{cases} |x-3|;\ x \ge 1 \\ \dfrac{x^2}{4}-\dfrac{3x}{2}+\dfrac{13}{4};\ x < 1 \end{cases} for continuity at x=1 and 3
Examine the function f(x)=\begin{cases} \dfrac{\tan x}{\sin x};\ x \ne 0 \\ 1;\ x=0 \end{cases} for continuity at x=0
Examine the function fix) for continuity at x=0. If f(x)=\begin{cases} \dfrac{e^{1/x}-1}{e^{-1/x}+1},\ x \ne 0 \\ 1,\ \ x=0 \end{cases}
Examine the function for differentiability at
x=0; f(x)=\begin{cases} \begin{matrix} x \tan^{-1} x; & x \ne 0 \end{matrix} \\ \begin{matrix} 0; & x=0 \end{matrix} \\ \begin{matrix} & \end{matrix} \end{cases}
Test the continuity of the function f(x)=\dfrac{|3x-4|}{3x-4} at x=\dfrac{4}{3}
If function f(x)=\begin{cases} \begin{matrix} \dfrac{\sin (m+1x+ \sin x)}{x} & x < 0 \end{matrix} \\ \begin{matrix} \dfrac{1}{2}; & x=0 \end{matrix} \\ \begin{matrix} \dfrac{x^{3/2}+1}{2}; & x < 0 \end{matrix} \end{cases} is continuous at x=0, then find m
Find the derivative of following functions w.r.t. x:
\sin^{-1} \left\{ 2x \sqrt{1-x^2}\right\}, -\dfrac{1}{2} < x < \dfrac{1}{2}
Find the derivative of following functions w.r.t. x:
\cos^{-1} \left( \dfrac{1-x^2}{1+x^2} \right), x \in (0,\infty)
Find the derivative of following functions w.r.t. x:
\sin^{-1} (3x-4x^3), x \in \left( \dfrac{-1}{2},\dfrac{1}{2} \right)
Find the derivative of following functions w.r.t. x:
\tan^{-1} \left( \dfrac{a+x}{1-ax}\right) (Hint : Put x= \tan \theta, a= \tan \alpha)
Find the derivative of following functions w.r.t. x:
\cos^{-1} \left( \dfrac{1-x^2}{1+x^2} \right), x \in (0,1)
Find the derivative of following functions w.r.t. x:
\cos^{-1} (2x)+2 \cos^{-1} (\sqrt{1-4x^2}) (Hint : Put 2x= \cos \theta)
Find the derivative of following functions w.r.t. x:
\sin^{-1} \left( \dfrac{1+x^2}{1-x^2}\right)+\cos^{-1}\left( \dfrac{1+x^2}{1-x^2}\right) [Hint: \sin^{-1} \theta+\cos^{-1}\theta=\dfrac{\pi}{2}]
Find the derivative of following functions w.r.t. x:
\cos^{-1} \left( \sqrt{\dfrac{1+x}{2}} \right) (Hint x= \cos \theta)
Find the derivative of following functions w.r.t. x:
\cos^{-1} (4x^3-3x), x \in \left( \dfrac{1}{2} , 1 \right)
Find the derivative of following functions w.r.t. x:
sec^{-1} \left( \dfrac{1}{2x^2-1} \right), x \in \left(0, \dfrac{1}{2}\right)
Find the derivative of following functions w.r.t. x:
\tan^{-1} \left( \dfrac{2^x+1}{1-4^x}\right) (Hint : Put 2^x= \tan \theta, )
If y=a \sin x+ b \cos x, then prove that:
\dfrac{d^2y}{dx^2}+y=0
Find \dfrac{d^2y}{dx^2}, when :
y=x^3+ \tan x
Find \dfrac{d^2y}{dx^2}, when :
y=x^2+3x+2
Find the derivative of following functions w.r.t. x:
\sin \left\{ 2 \tan^{-1} \left( \sqrt{\dfrac{1-x}{1+x}}\right) \right\} (Hint : Put x= \cos \theta)
Find \dfrac{dy}{dx} of following functions:
y \sqrt{1-x^2}=\sin^{-1}x
Find \dfrac{d^2y}{dx^2}, when :
y=x \cos x
Find \dfrac{d^2y}{dx^2}, when :
y=e^{-x} \cos x
Find the derivative of following functions w.r.t. x:
\cos^{-1} (\sqrt{1+x^2+x}) (Hint : Put x= \cos \theta)
Find \dfrac{d^2y}{dx^2}, when :
y=a \sin x- b \cos x
Find \dfrac{d^2y}{dx^2}, when :
y=2 \sin x+3 \cos x
If y=(\sin^{-1}x)^2, then prove that:
(1-x^2) \dfrac{d^2 y}{dx^2}-x \dfrac{dy}{dx}-2=0
Differentiate given functions w.r.t. x:
\sin^{-1} x+ \sin^{-1} \sqrt{1-x^2}
If y=\sec x+ \tan x, then prove that:
\dfrac{d^2 y}{dx^2}=\dfrac{\cos x}{(1- \sin x)^2}
Differentiate given functions w.r.t. x:
\dfrac{\cos^{-1} \dfrac{x}{2}}{\sqrt{2x+7}}, -2 < x < 2
Find whether function is increasing or decreasing in given domain f(x) = tan^{-1} X - X, X \epsilon R
Solve the following differential equationx+y=\sin^{-1} \left(\dfrac {dy}{dx}\right)
Solve:
\sin^{-1}\left[\dfrac{dy}{dx}+\dfrac{2}{x}y\right]=x
Match the columns
Derivatives of function
If x = \cos t (3 - 2\cos^{2}t) and y =\sin t (3 - 2\sin^{2} t), find the value of \dfrac {dy}{dx} at t = \dfrac {\pi}{4}
If x and y are differentiable functions of t, then show that
\dfrac {dy}{dx} = \dfrac {\dfrac {dy}{dt}}{\dfrac {dx}{dt}} if \dfrac {dx}{dt}\neq 0
Differentiate the function w.r.t. x.
\displaystyle (x \cos x)^x + (x \sin x)^{\tfrac{1}{x}}
Prove that the difference of the infinite continued fractions \displaystyle\frac{1}{a+}\frac{1}{b+}\frac{1}{c+}..., \frac{1}{b+}\frac{1}{a+}\frac{1}{c+}....., is equal to \displaystyle\frac{a-b}{1+ab}.
Show that \left(\displaystyle\frac{1}{a+}\frac{1}{b+}\frac{1}{c+}....\right)\left(c+\displaystyle\frac{1}{b+}\frac{1}{a+}\frac{1}{c_1}....\right)=\displaystyle \frac{1+bc}{1+ab}.
Solve:
If x^yy^x=1,Find \dfrac{dy}{dx}
When x \leq 2, f(x) = 2x +3 here f(x) is a polynomial function, therefore it is continuous on R, in particular it is continuous when x\leq2
When x> 2, f(x) = 2x -3 here f(x) is a polynomial function, therefore it is continuous on R, in particular it is continuous when x>2 at x=2
x = \cfrac{{{{\sin }^3}t}}{{\sqrt {\cos 2t} }},\,y = \cfrac{{{{\cos }^3}t}}{{\sqrt {\cos 2t} }} then find \dfrac{dy}{dx}
Differentiate the following function w.r.t. x :{\cos}^{-1}{(1-2{\sin}^{2}{x})}
If x=4t^{2}+5,y=6t^{2}+7t+3, find \dfrac{d^{2}y}{dx^{2}}.
If y=(\sin x)^{x}+x^{x} then find \dfrac{dy}{dx}
Find \dfrac{dy}{dx}, where x=\dfrac{3at}{1+t^3}, y=\dfrac{3at^2}{1+t^3}
x=\dfrac{3at}{1+t^{3}}, y=\dfrac{3at^{2}}{1+t^{3}}\Rightarrow \dfrac{y}{x}=t
\Rightarrow x=\dfrac{3ay/x}{\dfrac{x^{3}+y^{3}}{x+2}}\Rightarrow x^{4}+y^{3}x= 3ax^{2}y
\Rightarrow 4x^{3}+y^{3}+3y^{2}x\dfrac{dy}{dx}= 6axy+3ax^{2}\dfrac{dy}{dx}
\Rightarrow \dfrac{dy}{dx}=\dfrac{4x^{3}+y^{3}-6axy}{3ax^{2}-3xy^{2}}
log\left(\dfrac{dy}{dx}\right)=3x+4y; y=0 when x=0.
Find \frac { d y } { d x } for
If x = \dfrac { \sin ^ { 3 } t } { \sqrt { \cos 2 t } } , y = \dfrac { \cos ^ { 3 } t } { \sqrt { \cos 2 t } } then find \dfrac { d y } { d x }
If for x\epsilon \left( 0,\frac { 1 }{ 4 } \right) , the derivative of { tan }^{ -1 }\left( \frac { 6x\sqrt { x } }{ 1-9{ x }^{ 3 } } \right) is \sqrt { x } \cdot g\left( x \right) , then g(x)= \dfrac {1-kx^3}{(1+9x^3)^2} then k =
if y={ b\tan }^{ -1 }\left( \frac { x }{ a } +{ \tan }^{ -1 }\left( \frac { y }{ x } \right) \right) ,\quad find\quad \frac { dy }{ dx }
if x\sqrt { 1+y } +y\sqrt { 1+x } =0,-1<x<1,x\neq y then prove that \frac { dy }{ dx } =-\frac { 1 }{ { \left( 1+x \right) }^{ 2 } }
Find:
\dfrac { dy }{ dx } =sin(x+y)+cos(x+y)
Find the deivative of f (x)={ cos }^{ 1 }\left( sin\sqrt { \dfrac { 1+x }{ 2 } } \quad \right) +{ x }^{ 3 }
If x^{y}+y^{x}=a^{b}, then show that \dfrac{dy}{dx}=-\left (\dfrac{yx^{y-1}+y^{x}\log y}{x^{y}\log x+xy^{x-1}}\right)
If y=\tan^{-1}(3x), then find \dfrac{d^{2}y}{dx^{2}}.
Given
y=tan^{-1}(3x)
\frac{dy}{dx}=\frac{d}{dx}tan^{-1}(3x)
\frac{dy}{dx}=\frac{1}{1+(3x)^{2}}
\frac{dy}{dx}=\frac{1}{1+9x^{2}}
\frac{d^{2}y}{dx^{2}}=\frac{d}{dx}(\frac{1}{(1+9x^{2})})
\frac{d^{2}y}{dx^{2}}=\frac{(1+9x^{2})\frac{d}{dx}3-3\frac{d}{dx}(1+9x^{2})}{(1+9x^{2})^{2}}
\frac{d^{2}y}{dx^{2}}=\frac{0-3(18x)}{(1+9x^{2})^{2}}
\frac{d^{2}y}{dx^{2}}=\frac{-54x}{(1+9x^{2})^{2}}
If x=\sin^{2}\theta ,y=\tan \theta , then find \dfrac{dy}{dx} at \theta ={45}^{\circ}.
If x=a\left( \cos { t } +\log { \tan { \dfrac { t }{ 2 } } } \right) ,y=a\sin { t } , then show that \dfrac { dy }{ dx } =\tan { t } .
Differentiate the following functions with respect to x
\sqrt {\dfrac {1+\sin x}{1-\sin x}}
If \cos^{-1}\left (\dfrac {x^2 - y^2}{x^2 + y^2}\right)=\tan^{-1}\ a, prove that \dfrac {dy}{dx}=\dfrac {y}{x}.
If y=x \sin y, prove that \dfrac {dy}{dx}= \dfrac{\sin y}{(1-x \cos y)}.
Differentiate the following function from first principle:
\sin^{-1} (2x+3)
f(x) = \left\{\begin{matrix} x^2 + ax + 1,& x \ \text{is rational} \\ ax^2+2x+b, & x \ \text{is irrational} \end{matrix}\right.
A function f(x) defined as is continuous at x=1 and 2 then the values of a and b
If f\left(\dfrac{x+y}{3}\right)=\dfrac{2+f(x)+f(y)}{3} for all real x and y and f^{\prime}(2)=2, then determine y=f(x)
f(x) = \left\{\begin{matrix} b\sin^{-1} \left(\dfrac{c+x}{2}\right), & -\dfrac{1}{2} < x < 0 \\ \dfrac{1}{2}, & x = 0 \\ \dfrac{e^{ax/2}-1}{x}, & 0 < x < \dfrac{1}{2} \end{matrix}\right.if \left | C \right |\leq \dfrac{1}{2} and f(x) is a differentiable function at x=0 given Find the value of a and prove that 64 b^{2}=4-c^{2}
Let f(x+y)=f(x)+f(y)+2 x y-1 for all real x and y and f(x) be differentiable function.
If f^{\prime}(0)=\cos \alpha, then prove that f(x)>0 \forall x \in R
If y^{2} = a^{2} cos^{2} x + b^{2} sin^{2} x, show that y + \dfrac{d^{2}}{dx^{2}} = \dfrac{a^{2}b^{2}}{y^{3}}
Differentiate the following w.r.t. x: x^{\tan ^{-1} x}
If y=\sec^{-1}\Bigg(\dfrac{1+\sqrt{x}}{1-\sqrt{x}}\Bigg)+\sin^{-1}\Bigg(\dfrac{1-\sqrt{x}}{1+\sqrt{x}}\Bigg), then \dfrac{dy}{dx} is equal to ___________.
If \sin ^{-1}\left(\dfrac{x^{5}-y^{5}}{x^{5}+y^{5}}\right)=\dfrac{\pi}{6}, show that \dfrac{d y}{d x}=\dfrac{x^{4}}{3 y^{4}}
Let the function ln (f(x)) is defined where f(x) exists for x \le 2 and k is fixed positive real number. Prove that if \dfrac{d}{dx} (x\ f (x)) \ge -k\ f(x), then f(x) \ge Ax^{k-1} where A is independent of x.
If x=\sin ^{-1}\left(e^{t}\right), y=\sqrt{1-e^{2 t}}, show that \sin x+\dfrac{d y}{d x}=0
If f(x)=\begin{cases} x-1;x<2 \\ 2x-3; x \ge 2 \end{cases} then find f^{'}(2-0)
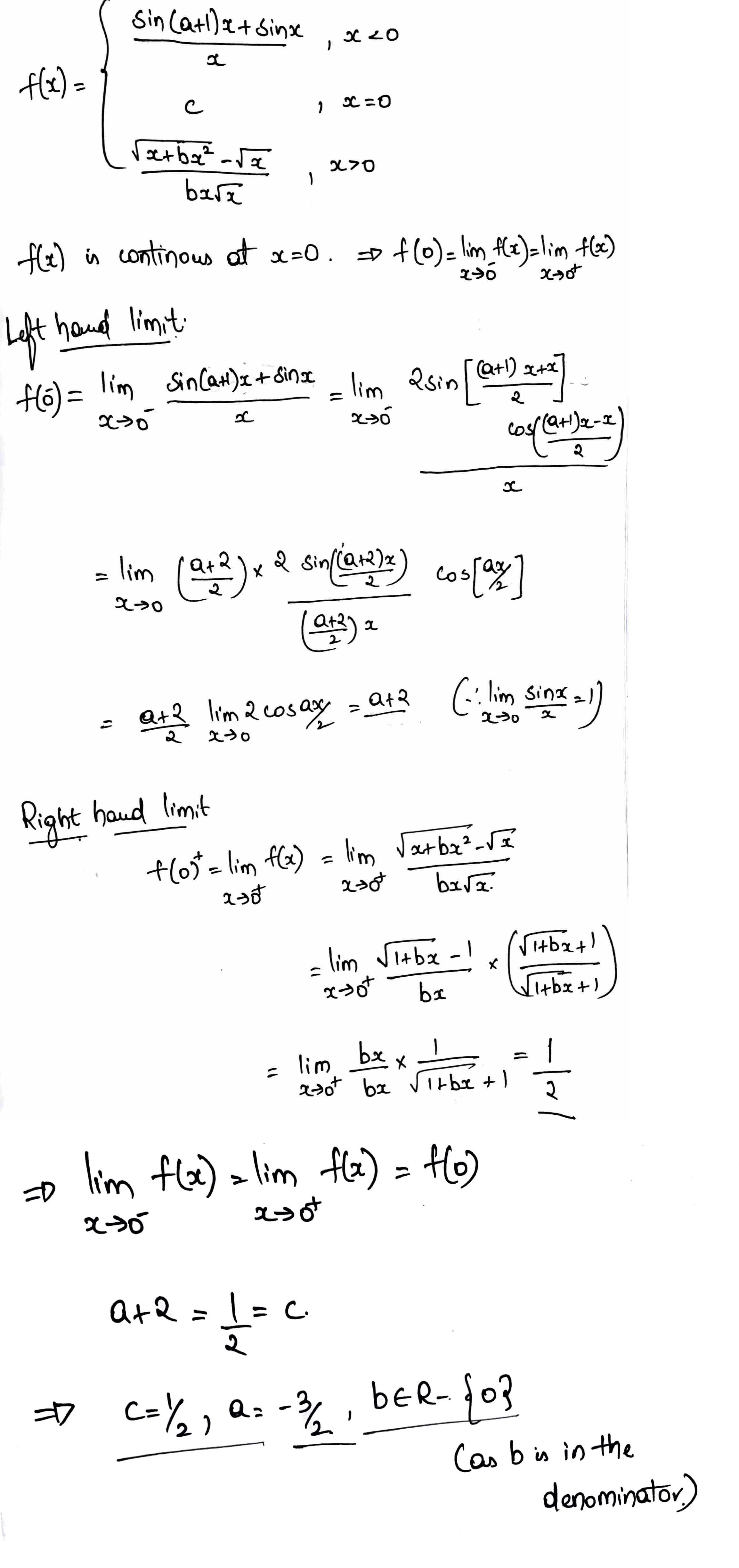
Find a,b,c if the function f(x)=\begin{cases} \begin{matrix} \dfrac{\sin (a+1)x+\sin x}{x} & x< 0 \end{matrix} \\ \begin{matrix} c, & x=0 \end{matrix} \\ \begin{matrix} \dfrac{\sqrt{x+bx^2}-\sqrt x}{bx \sqrt x} & x > 0 \end{matrix} \end{cases} is continuous at x=0
Differentiate \sin ^{-1}\left(\dfrac{2 x}{1+x^{2}}\right) w . r . t . \cos ^{-1}\left(\dfrac{1-x^{2}}{1+x^{2}}\right)
Find \dfrac{d y}{d x}, if : x=\cos ^{-1}\left(\dfrac{2 t}{1+t^{2}}\right), y=\sec ^{-1}\left(\sqrt{1+t^{2}}\right)
Find \dfrac{\mathrm{dy}}{\mathrm{dx}}, if : x=\cos ^{-1}\left(4 t^{3}-3 t\right), y=\tan ^{-1}\left(\dfrac{\sqrt{1-t^{2}}}{t}\right)
Differentiate \tan ^{-1}\left(\dfrac{x}{\sqrt{1-x^{2}}}\right) w . r . t . \sec ^{-1}\left(\dfrac{1}{2 x^{2}-1}\right)
Class 12 Commerce Maths Extra Questions
- Application Of Derivatives Extra Questions
- Application Of Integrals Extra Questions
- Continuity And Differentiability Extra Questions
- Determinants Extra Questions
- Differential Equations Extra Questions
- Integrals Extra Questions
- Inverse Trigonometric Functions Extra Questions
- Linear Programming Extra Questions
- Matrices Extra Questions
- Probability Extra Questions
- Relations And Functions Extra Questions
- Three Dimensional Geometry Extra Questions
- Vector Algebra Extra Questions