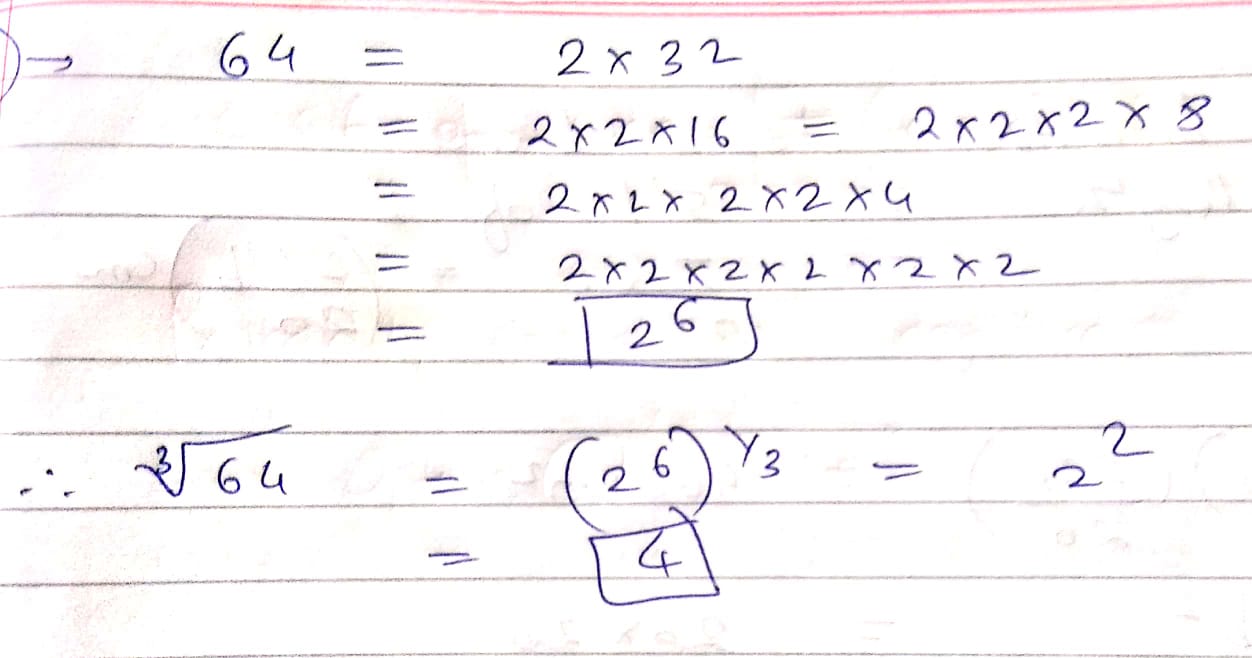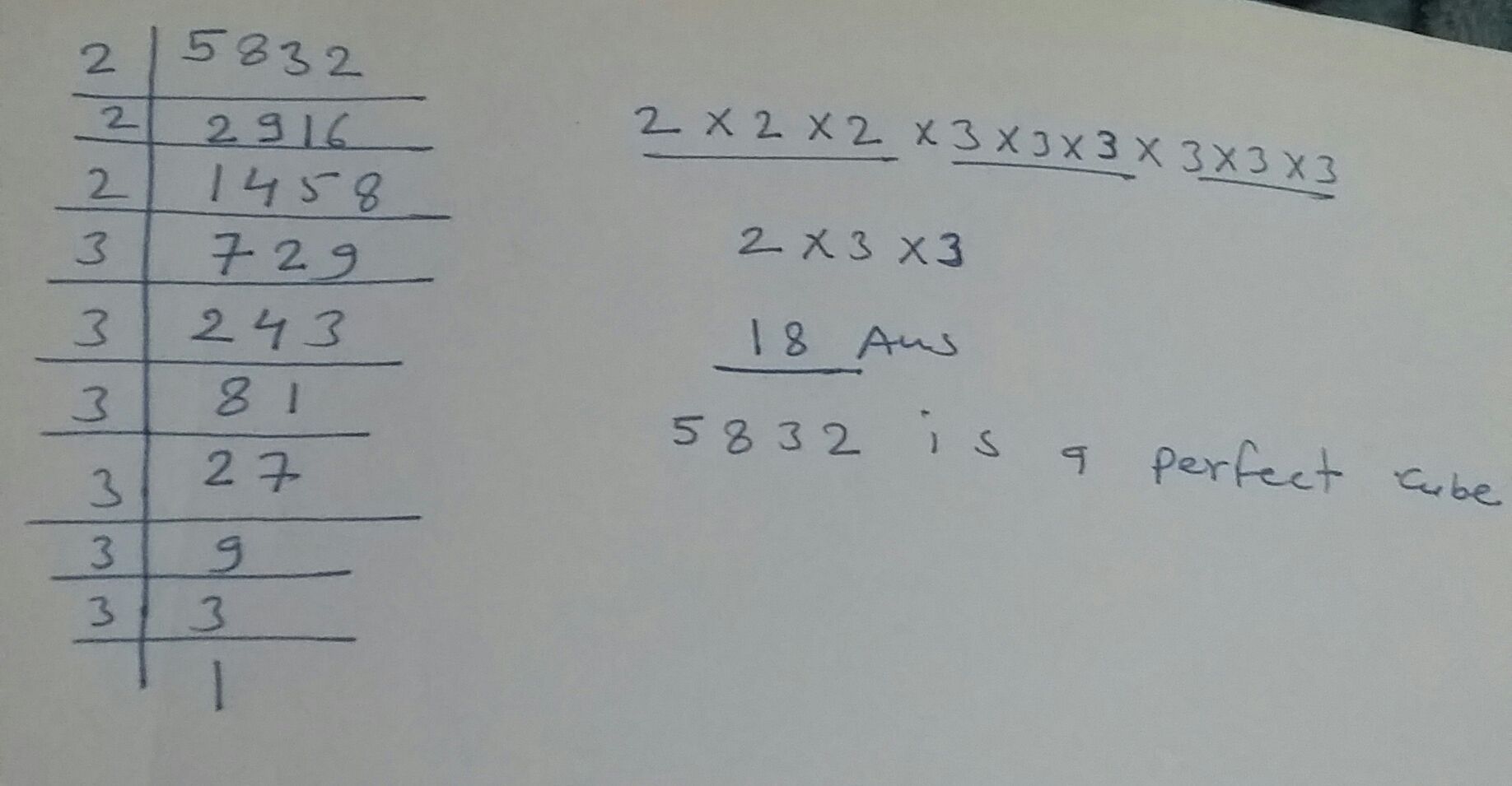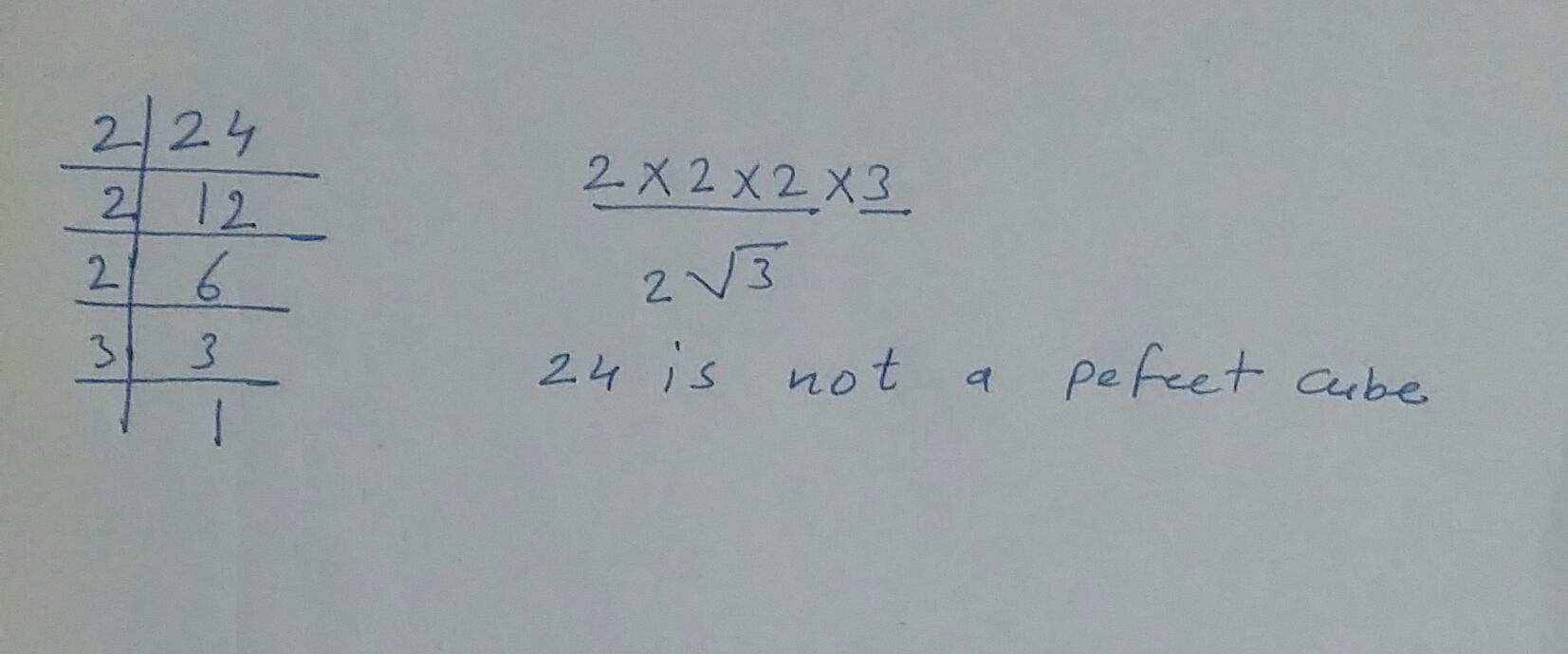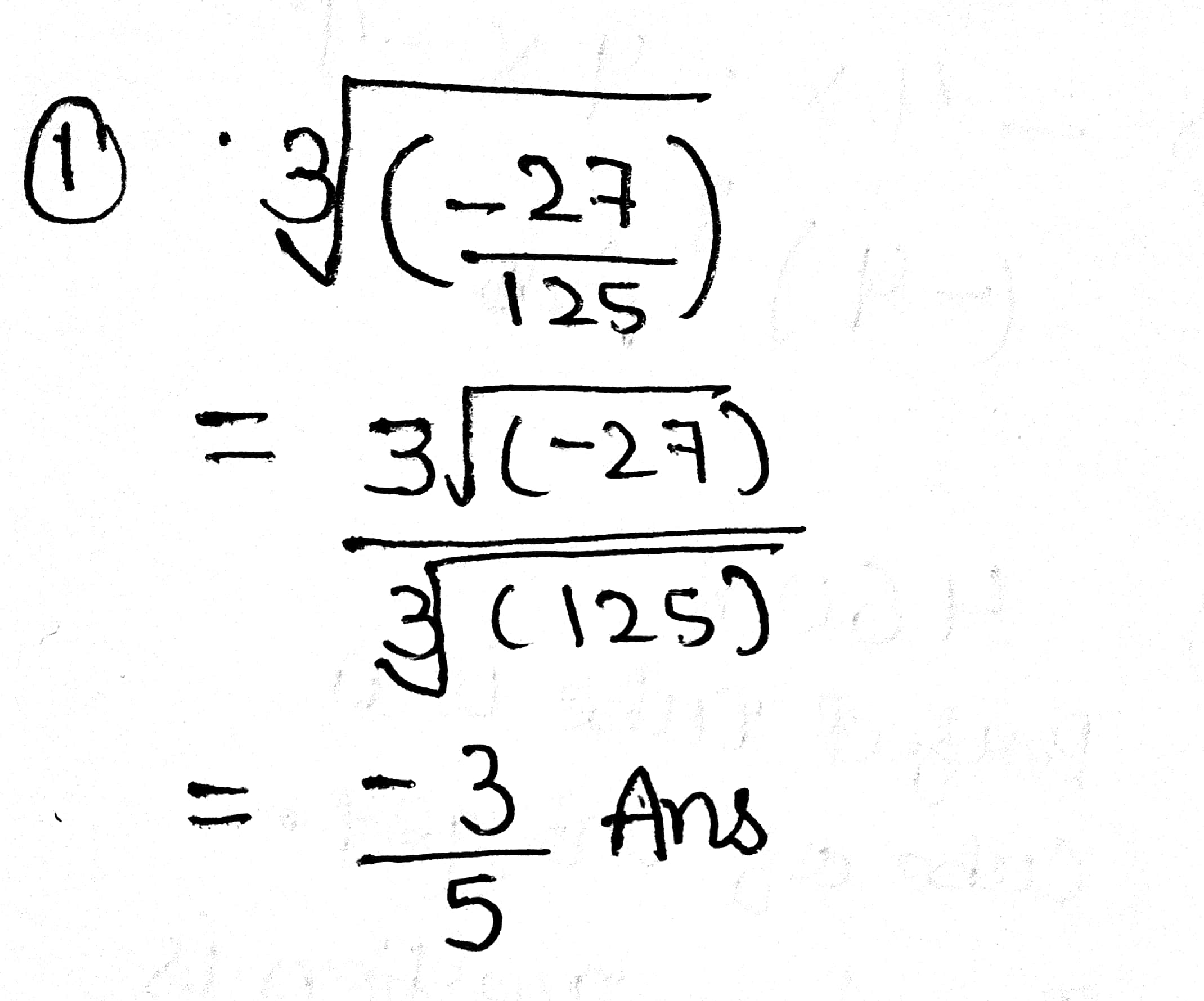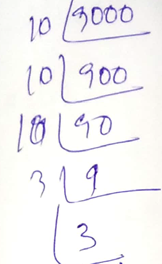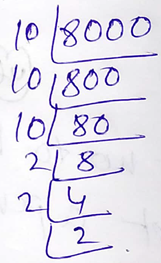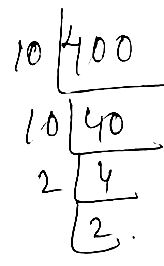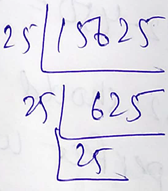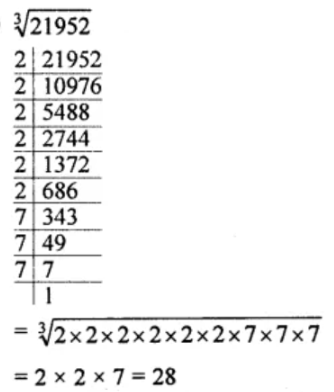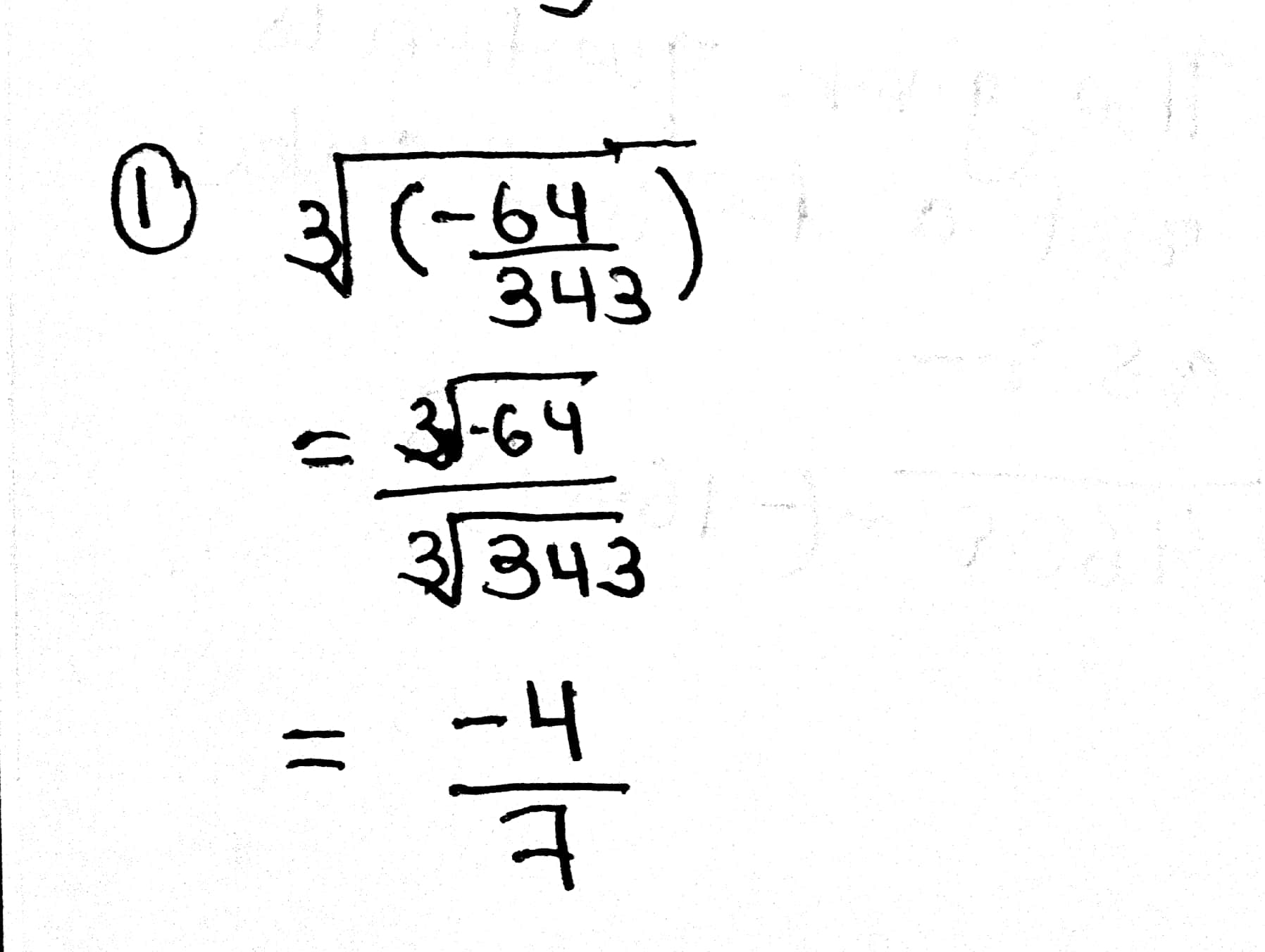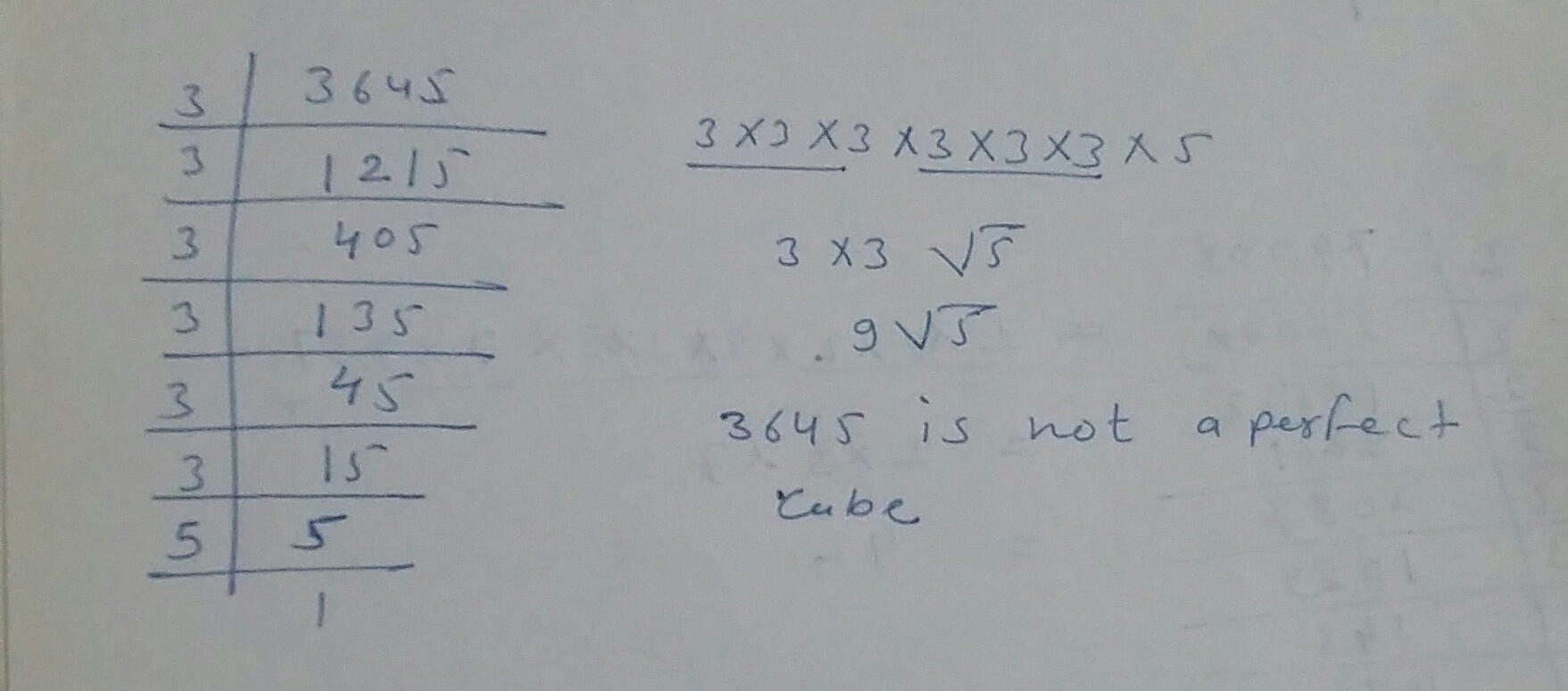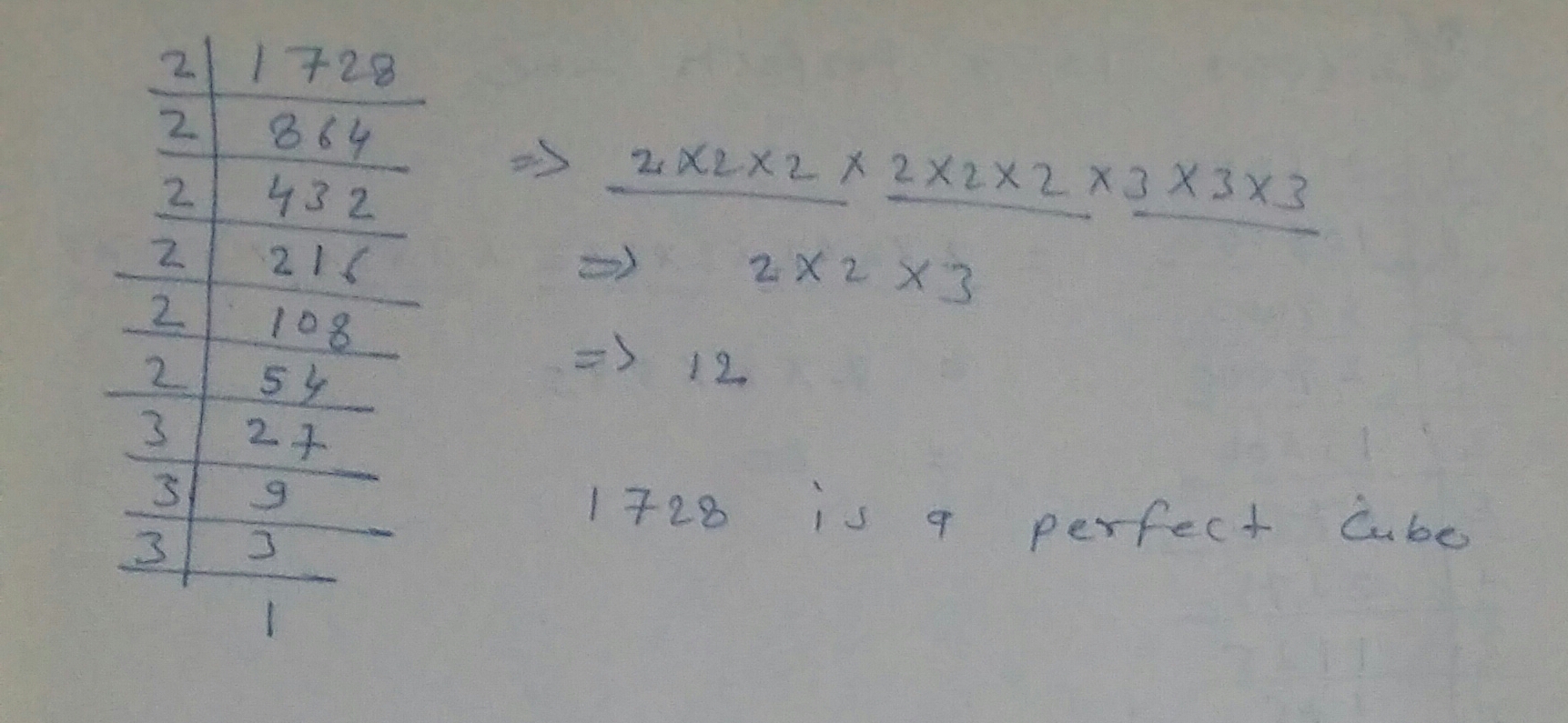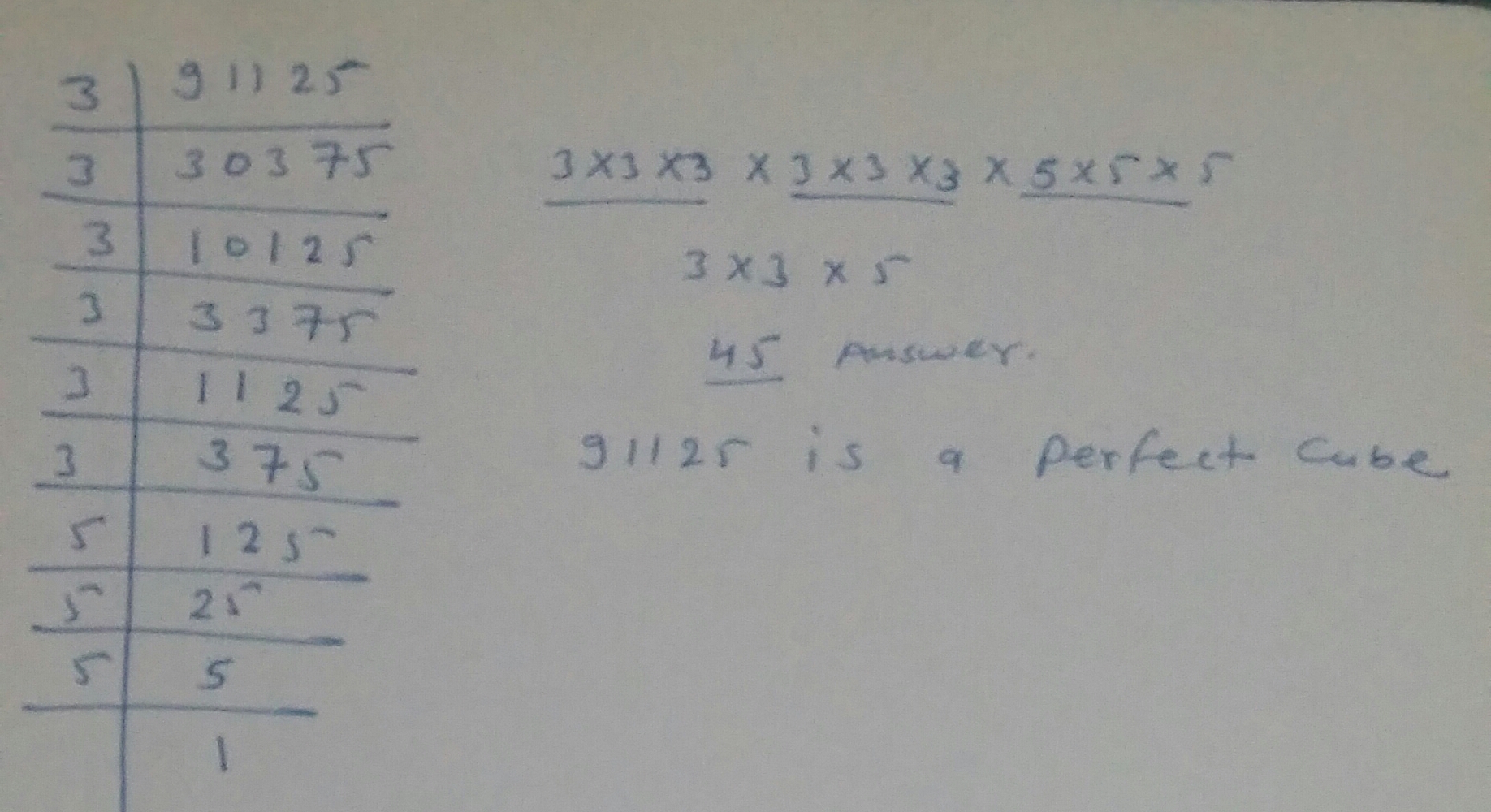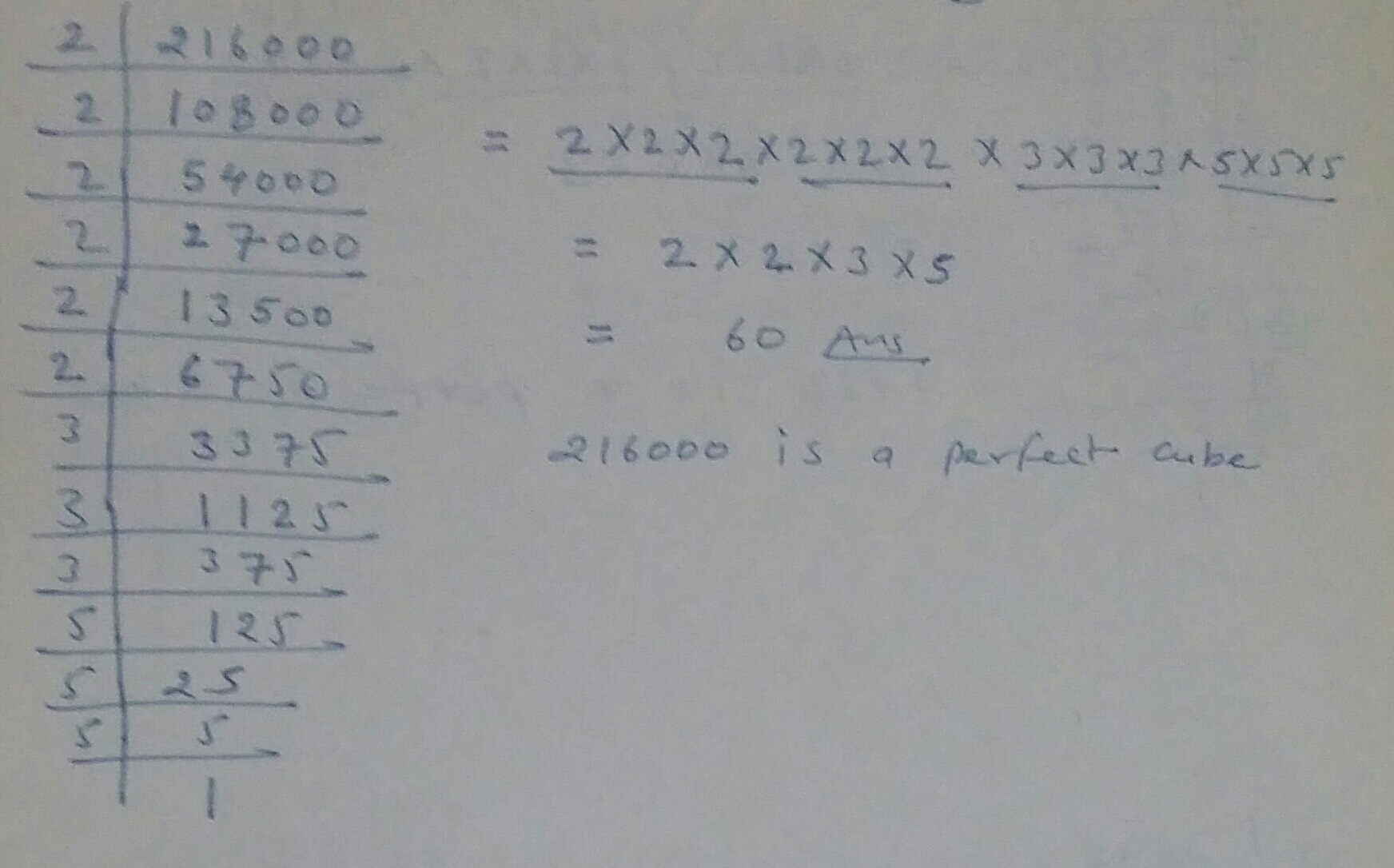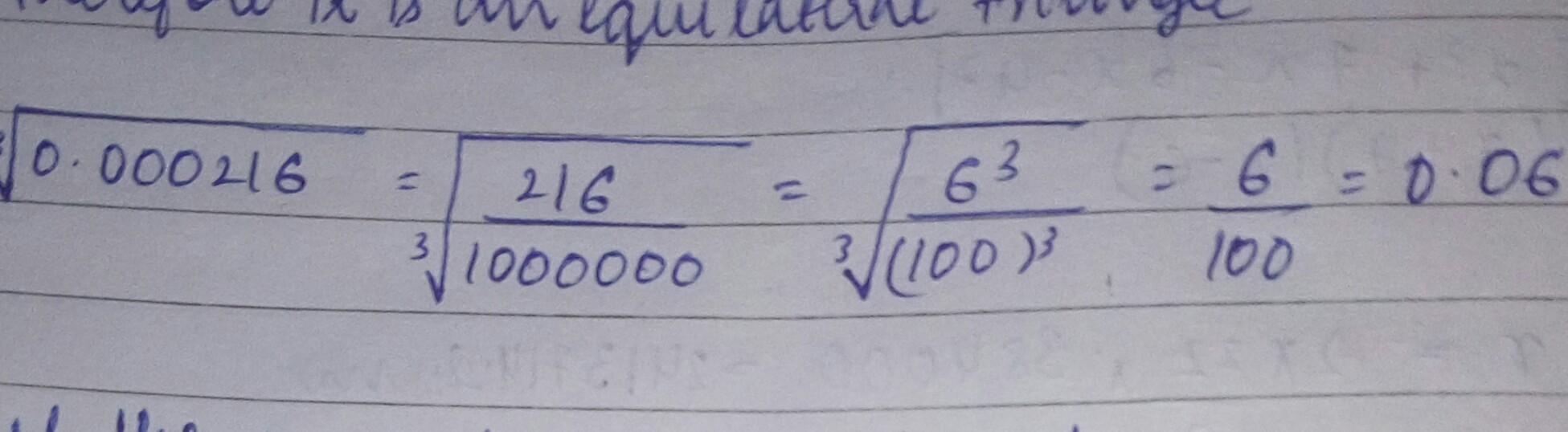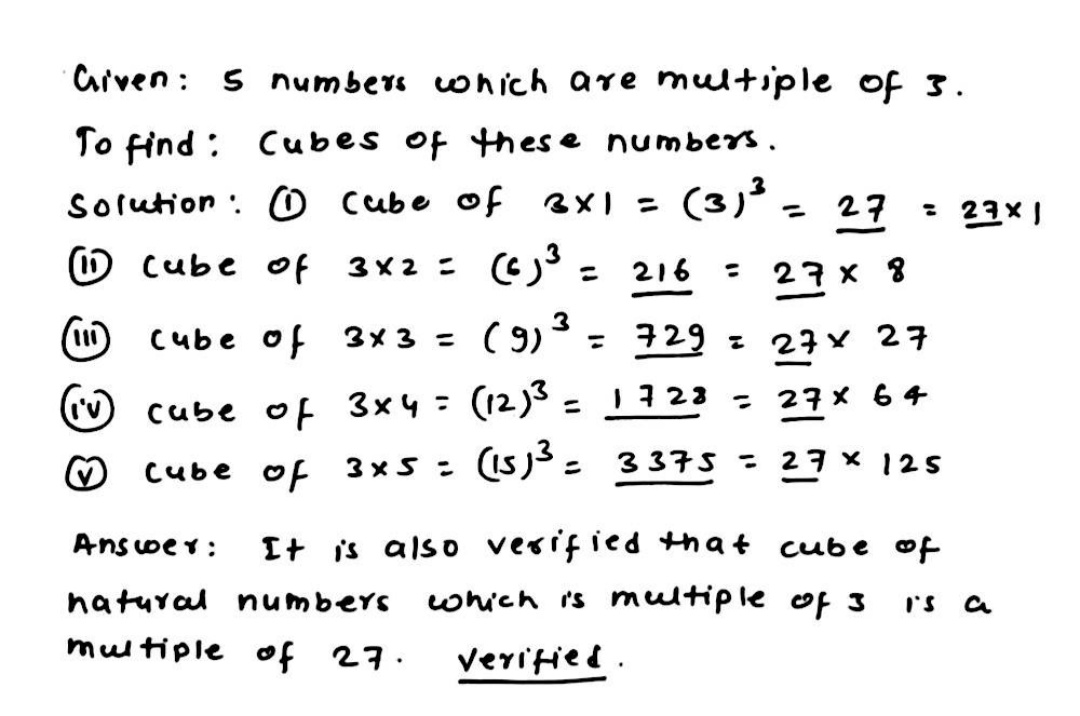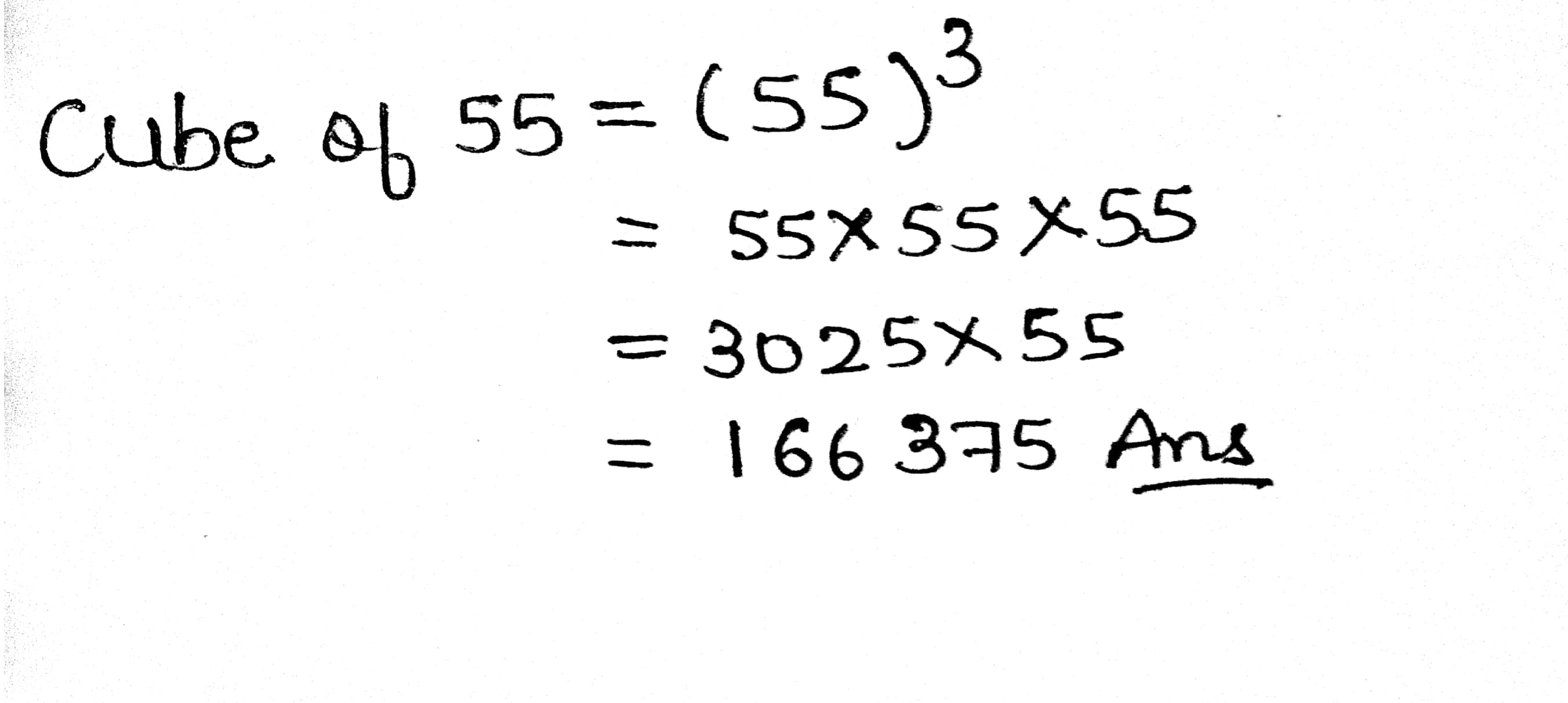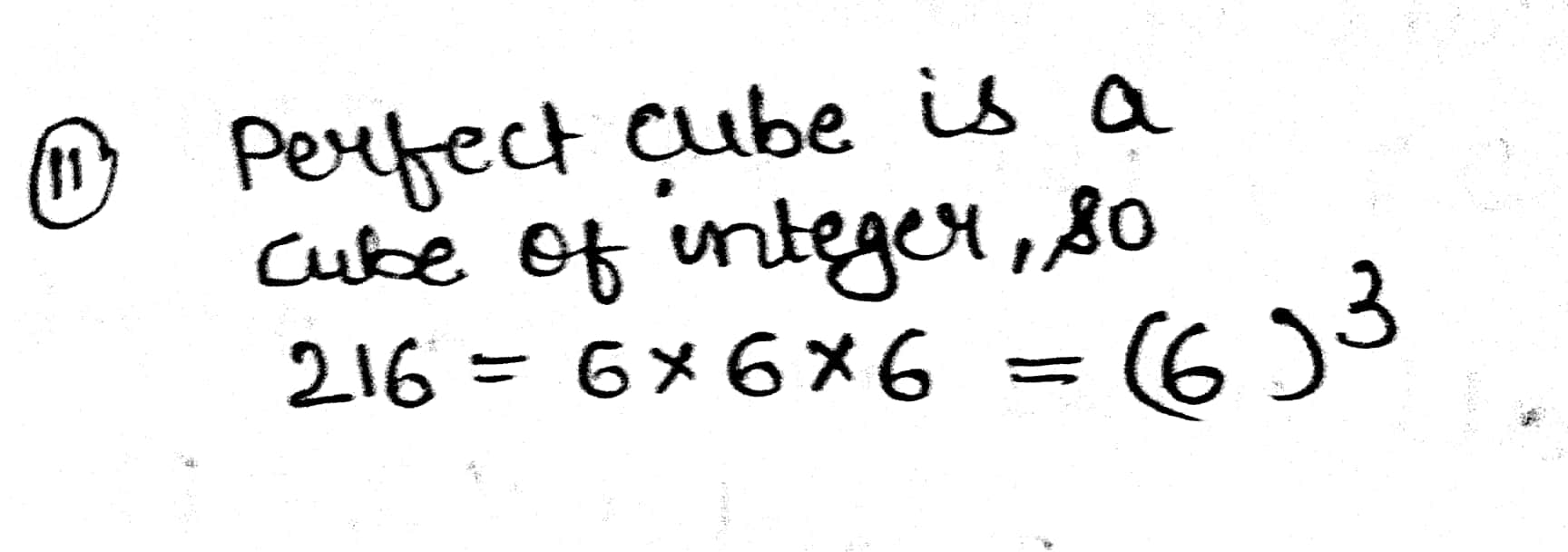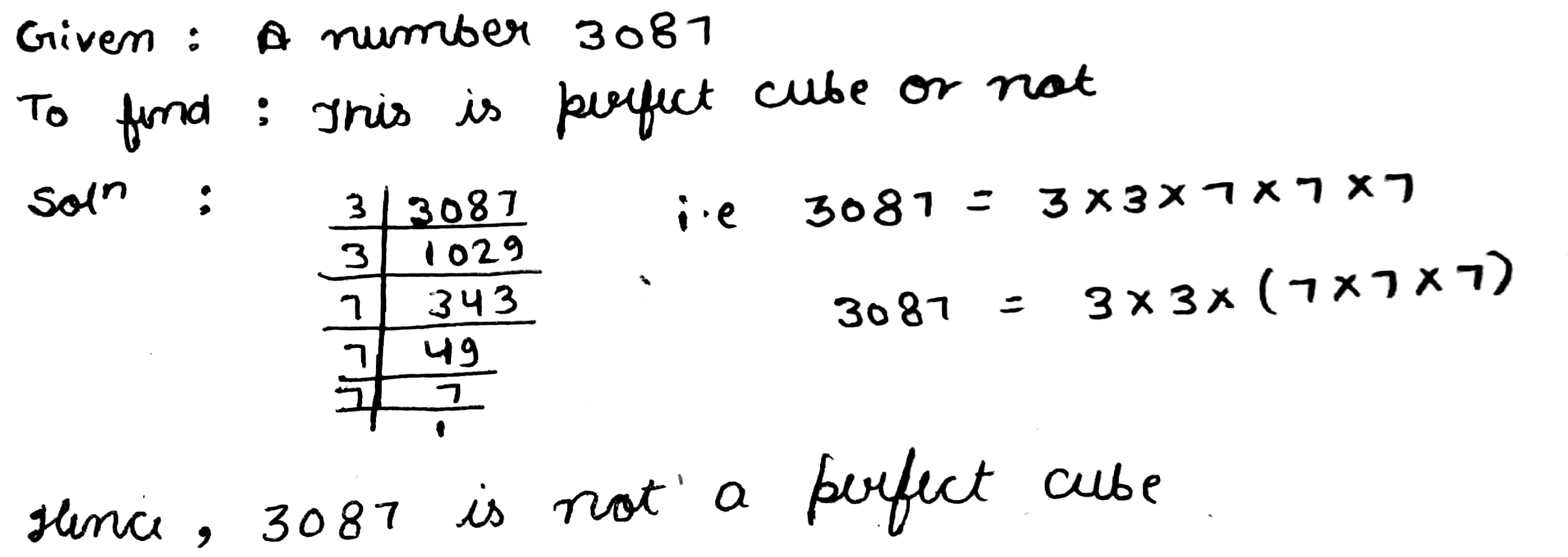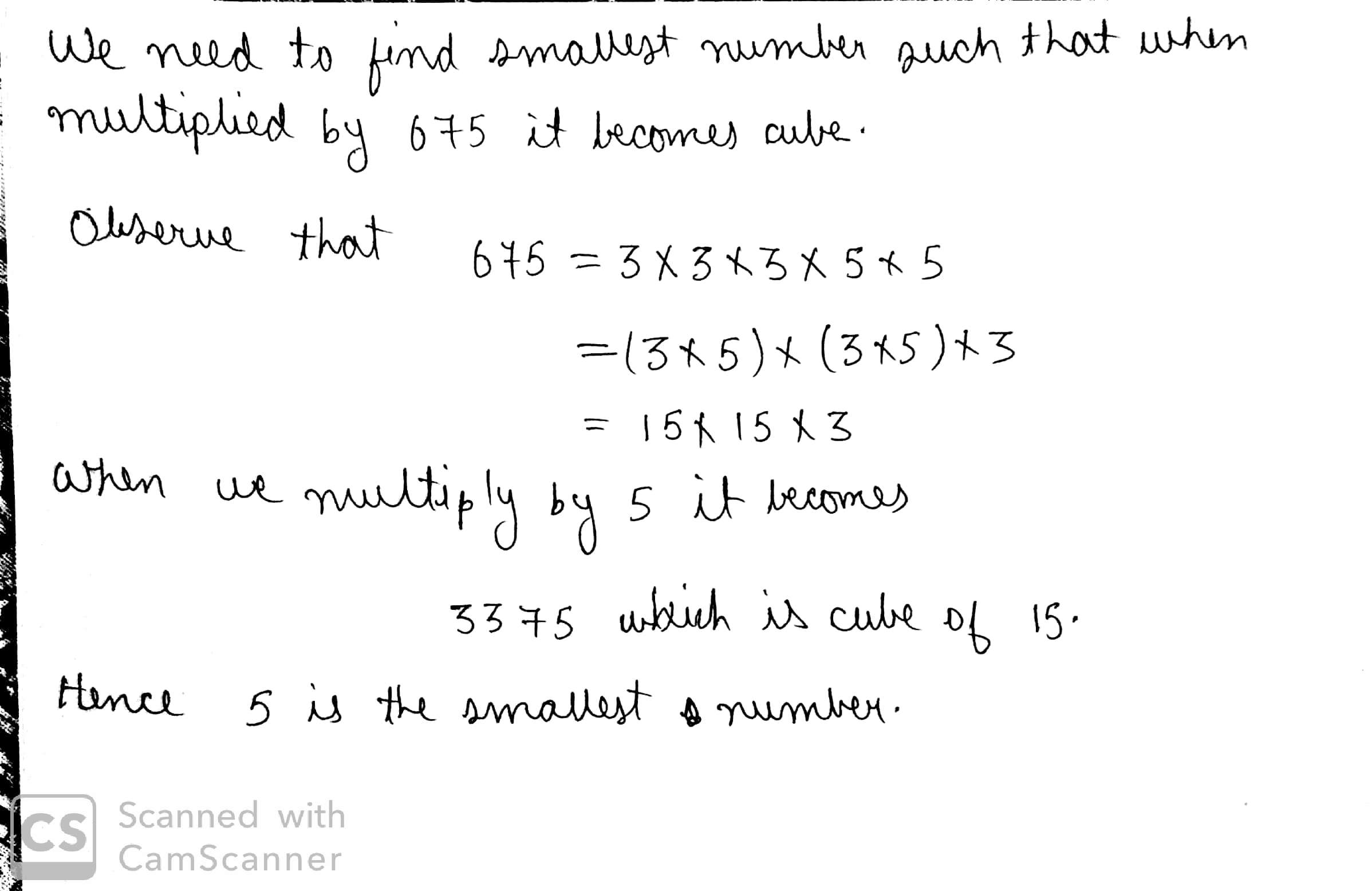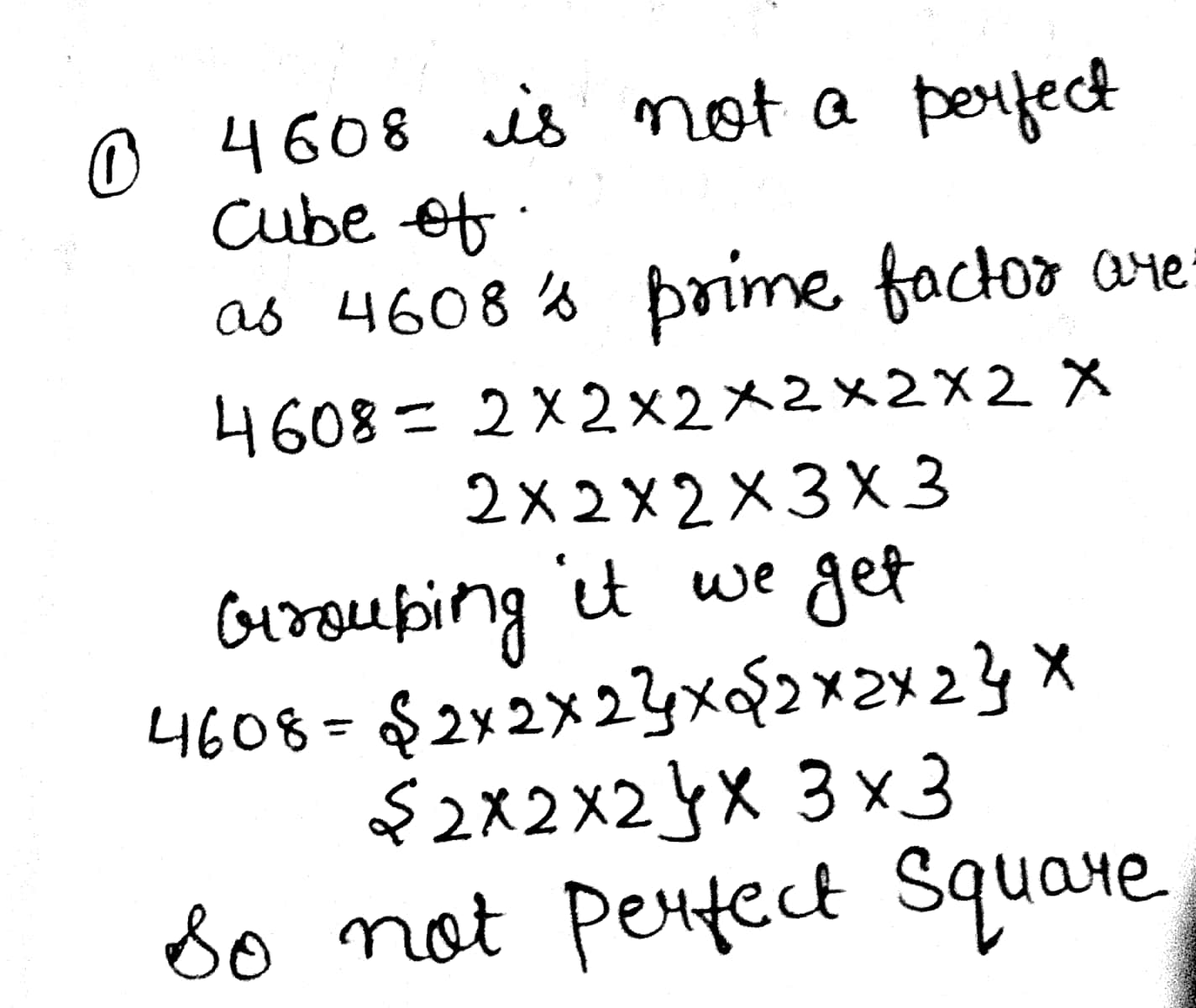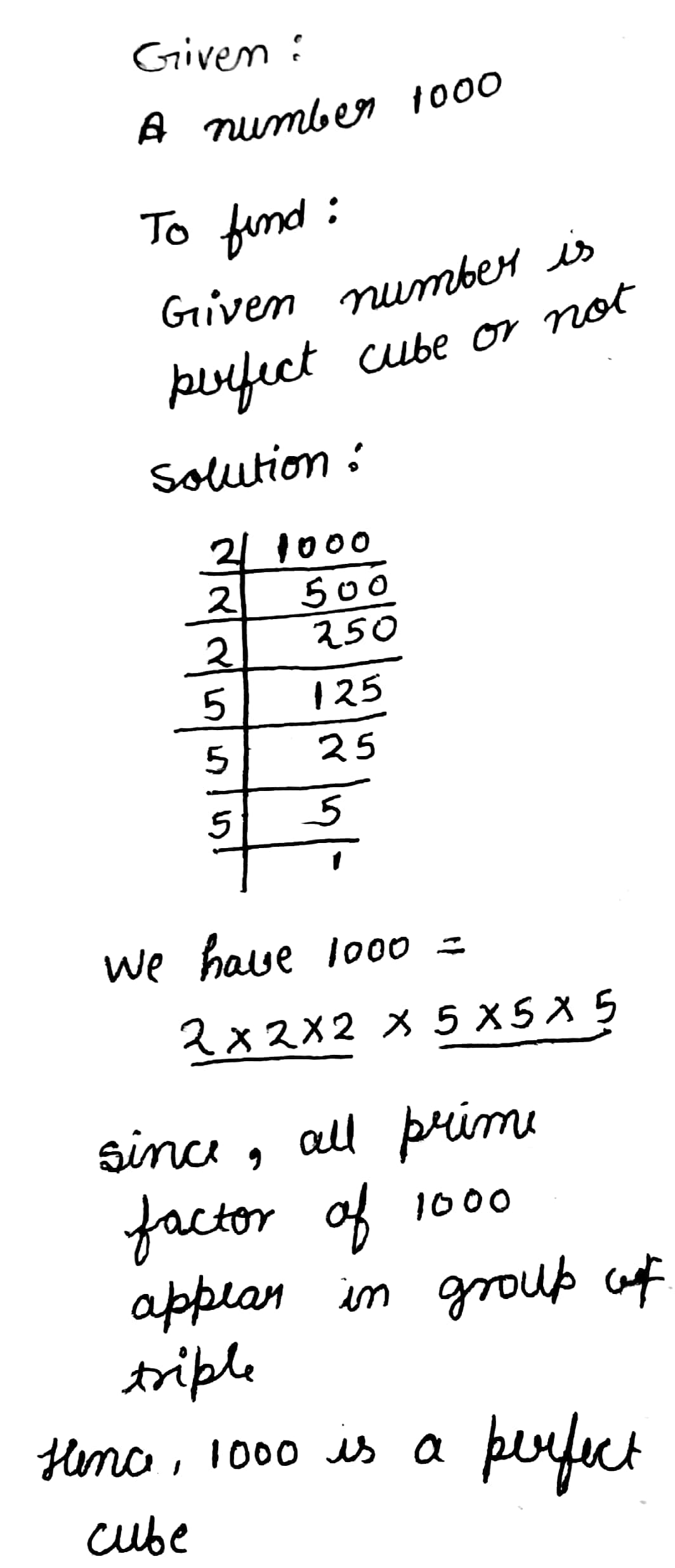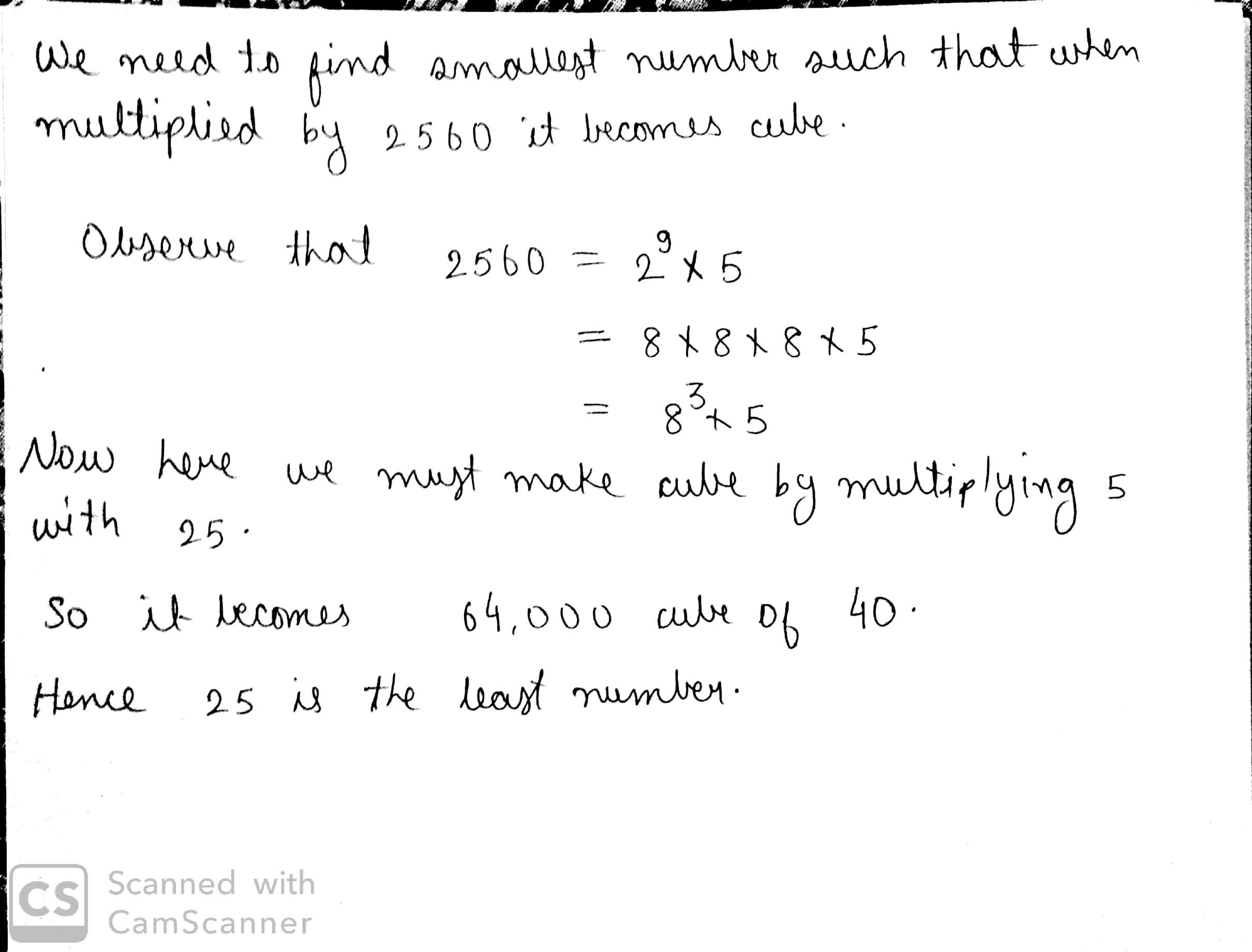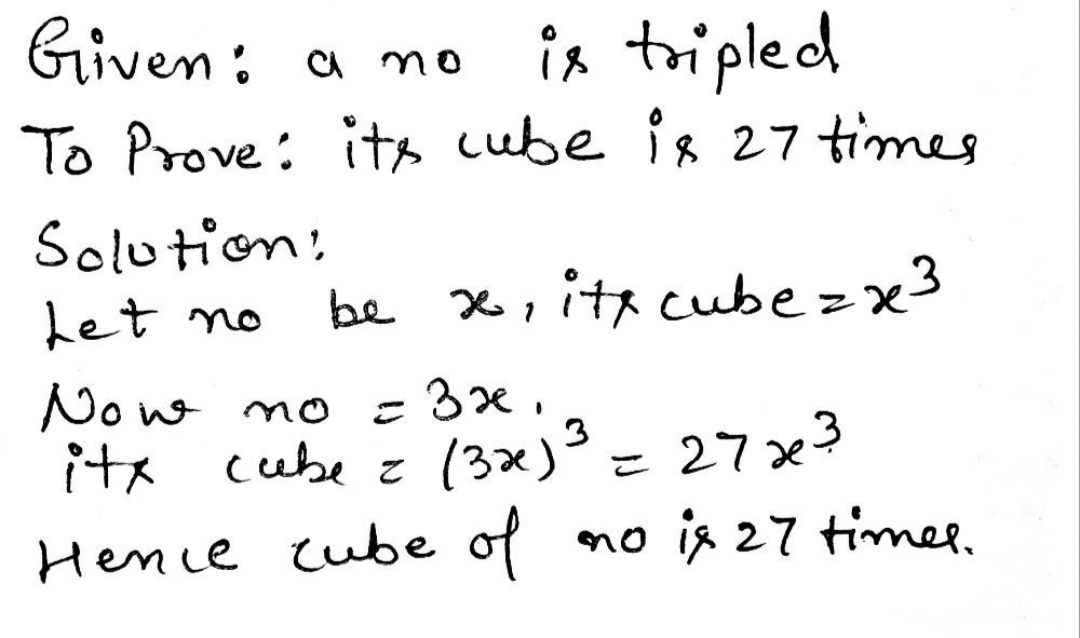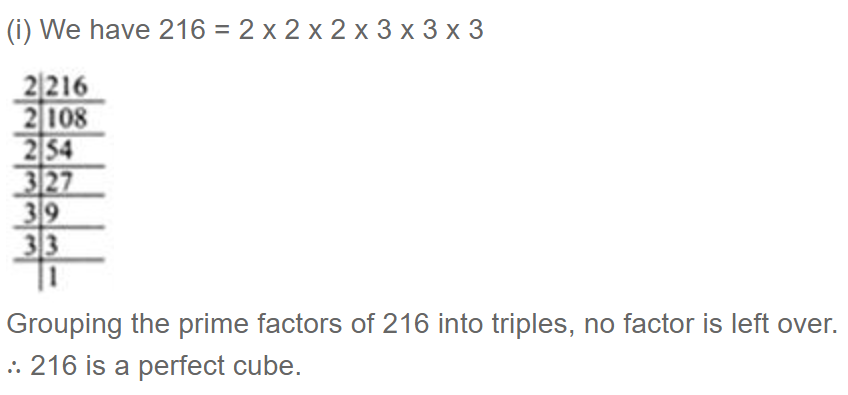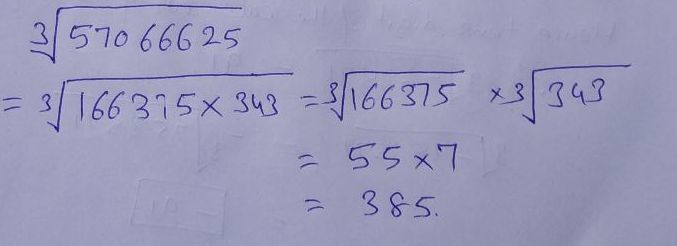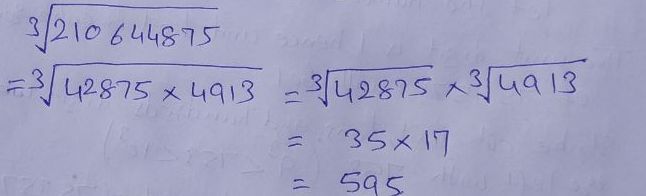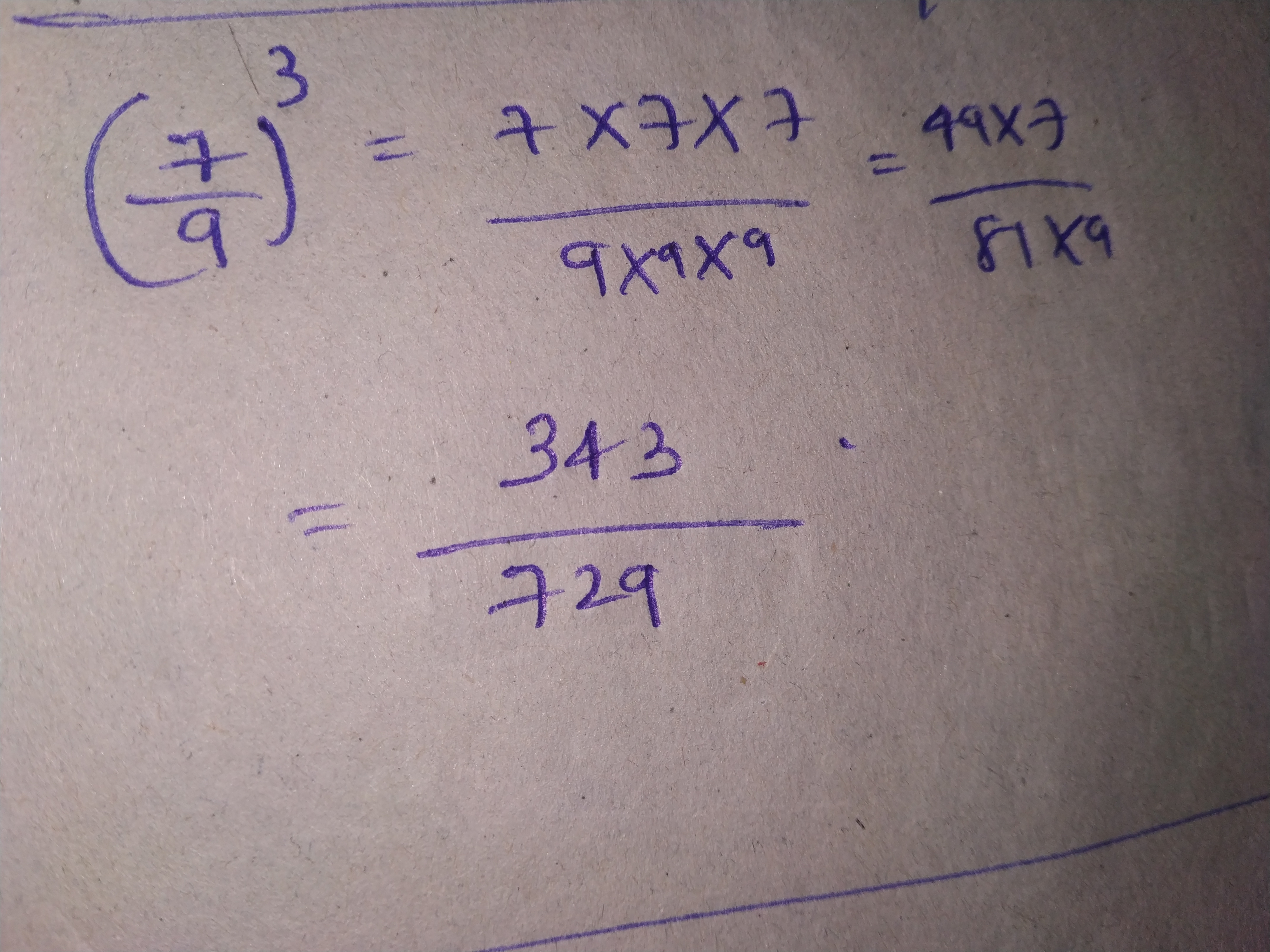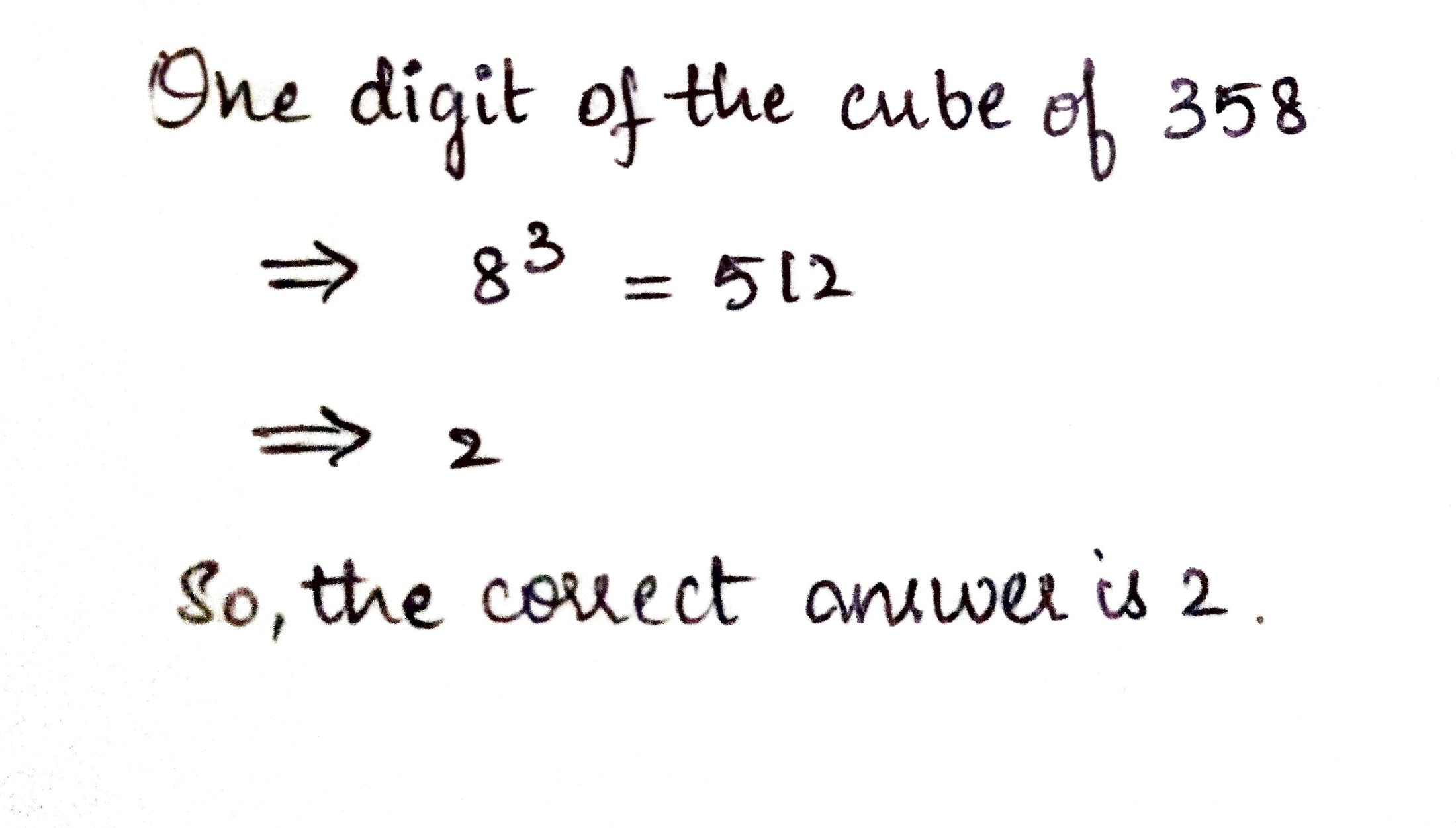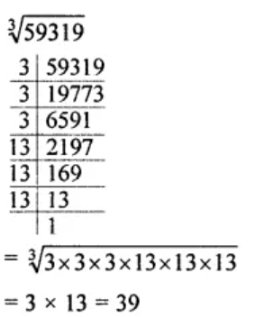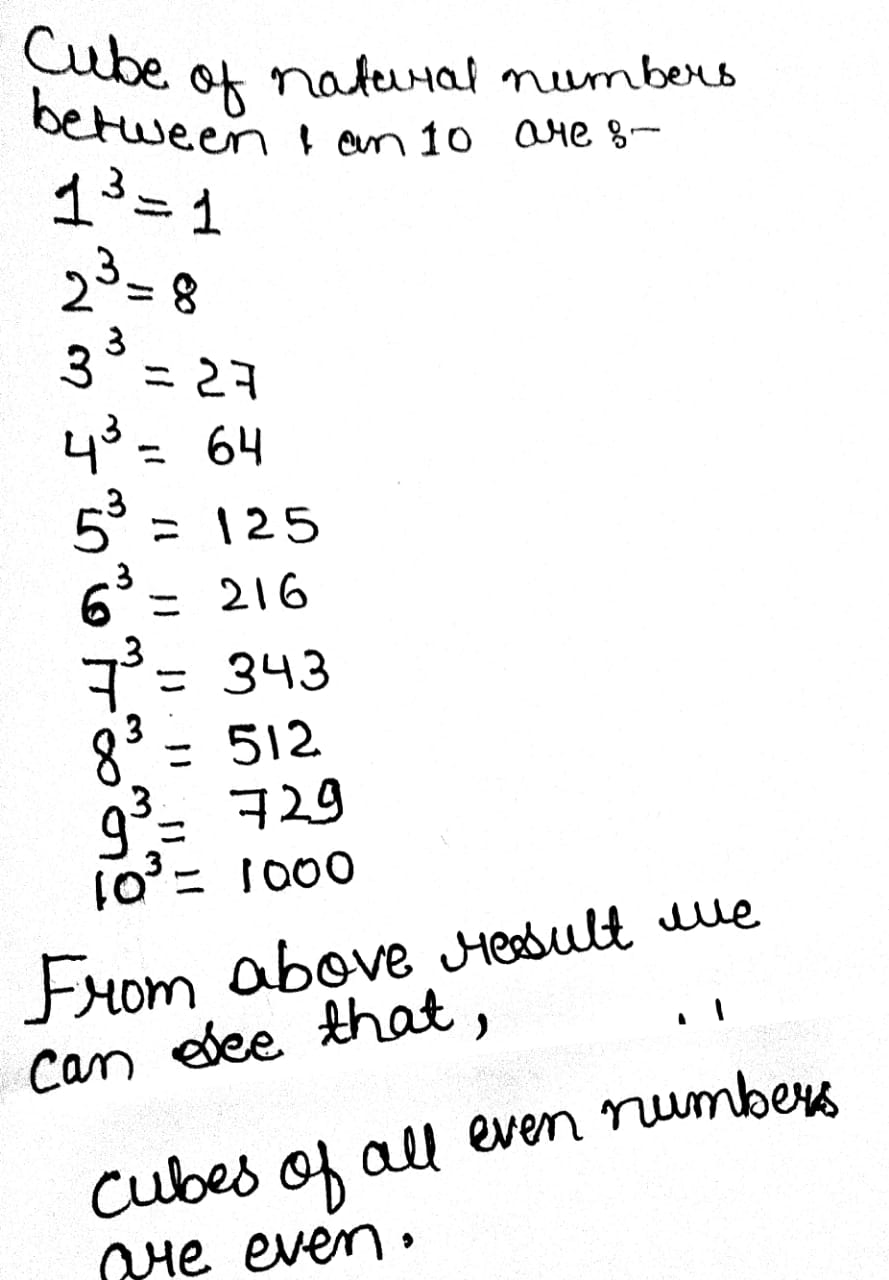Class 8 Maths - Cubes And Cube Roots

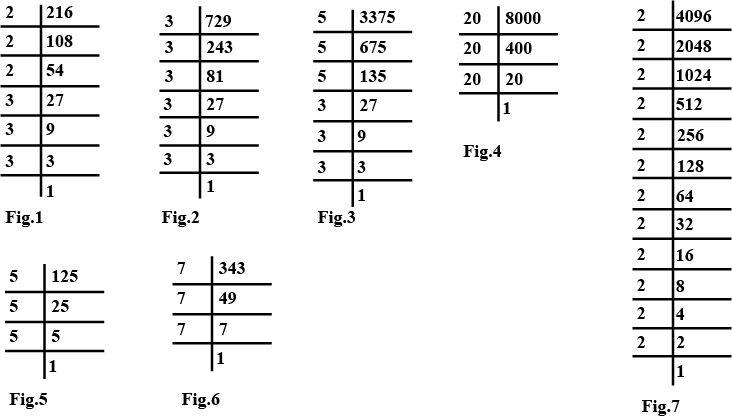
Find the value of: $$\displaystyle \sqrt[3]{729}$$.

On prime factorising, we get,

$${729}=\underline{3\times 3\times 3}\times \underline{3\times 3\times 3}=3^3 \times 3^3 =9^3$$.

Then, value of $$\sqrt[3]{729}$$ is:

$$\sqrt[3]{729}=\sqrt[3]{9^3}= 9$$.

Therefore, $$9$$ is the required solution.

Cube root of $$185193$$ is:

Given number is $$185193$$.

On prime factorising, we get,

$$185193 = 57\times57\times57=57^3$$.

Then, cube root of $$185193$$ is:

$$\sqrt[3]{185193}=\sqrt[3]{57^3}= 57$$.

Hence, the cube root of $$185193$$ is $$57$$.

Find the cube root of the following numbers by prime factorization:
$$157464$$

$$\sqrt[3]{157464}$$
$$= \sqrt[3]{2 \times 2 \times 2 \times 3 \times 3 \times 3 \times 3 \times 3 \times 3 \times 3 \times 3 \times}$$
$$= 2 \times 3 \times 3 \times 3 = 54$$
Thus, the cube root of $$157464$$ is $$54.$$
1760546_1806857_ans_a039534a5b054e77b0dbb2bc36a9be41.png

1760546_1806857_ans_a039534a5b054e77b0dbb2bc36a9be41.png

Fill in the blanks to make the statements true.
Ones digit in the cube of 38 is ______.

Unit digit of $$38 = 8$$

Cube of $$8 = 512$$

$$\therefore$$ Ones digit in the cube of $$38$$ is $$ 2$$

Find the cube root of each of the following numbers by prime factorization method.
$$64$$

1311593_1462133_ans_e37bcba1c92743c988323cbdf7a53383.jpeg

Find the cube root of the following numbers by prime factorization:
$$32768$$

$$\sqrt[3]{32768}$$
$$= \sqrt[3]{2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2}$$
$$= 2 \times 2 \times 2 \times 2 \times 2 = 32$$
Thus, the cube root of $$32768$$ is $$32.$$

Find the cube root of $$9a{ b }^{ 2 }+\left( { b }^{ 2 }+24{ a }^{ 2 } \right) \sqrt { { b }^{ 2 }-3a^{ 2 } } $$.

$$9ab^2+(b^2+24a^2)\sqrt{b^2-3a^2}$$

$$=(b^2-3a^2)\sqrt{b^2-3a^2}+27\sqrt{b^2-3a^2}+27a^3+9ab^2-27a^3$$

$$=(3a)^3+(\sqrt{b^2-3a^2})^3+3\times3a(\sqrt{b^2-3a^2})^2+3\times(3a)^2\times\sqrt{b^2-3a^2}$$

$$=(3a+\sqrt{b^2-3a^2})^3$$

Hence, cube root is $$3a+\sqrt{b^2-3a^2}$$

Find the cube of following decimal numbers:
(a) $$0.7$$
(b) $$0.09$$
(c) $$1.1$$

We have,

$$(a)\ \ 0.7$$

$$(0.7)^3=0.7\times 0.7\times 0.7=0.343$$

$$(b)\ \ 0.09$$

$$(0.09)^3=0.09\times 0.09\times 0.09=0.000729$$

$$(c)\ \ 1.1$$

$$(1.1)^3=1.1\times 1.1\times 1.1=1.331$$

Hence, this is the answer.

Are the following number perfect cubes?If yes,then find the number whose cube is the given numbers:$$5832$$

1146614_1191246_ans_246705d73eab4b3295227fcceae9375e.jpg

Are the following number perfect cubes?If yes,then find the number whose cube is the given numbers:$$24$$ ?

1146599_1191241_ans_5633b8e34d594d1e8baf1fd2b7c3168b.jpg

Find the cube of $$-12$$.

Cube of $$-12$$ is:

$$(-12)^3=(-12) \times (-12) \times (-12)$$

$$=(-12) \times 144$$

$$=-1728$$.

Hence, the cube of $$-12$$ is $$-1728$$.

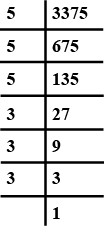
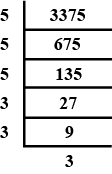
Find the cube roots of the number $$3048625$$ using the fact that
$$3048625=3375\times 729$$.

1504677_1672101_ans_598be53b42064e4ea84d0e36372d8ed1.jpg

Evaluate: $$(1.2)^{3}$$

To evaluate the cube of $$(1.2)$$ i.e $$(1.2)^3$$:

We need to multiply the given number three times i.e. $$1.2\ \times\ 1.2\ \times\ 1.2 \ =\ {1.728}$$

Now, we have to convert the given number into fraction we get,

$$\dfrac{1728}{1000}=\dfrac{216}{125}$$

$$\therefore$$, the cube of $$1.2$$ is $${1.728}$$ or $$\dfrac{216}{125}$$

Evaluate: $$(21)^{3}$$

To evaluate the cube of $$21$$ i.e $$21^3$$:

We need to multiply the given number three times i.e.

$$21\ \times\ 21\ \times\ 21 \ =\ {9261}$$

$$\therefore$$, the cube of $$21$$ is $${9261}$$

Evaluate: $$(15)^{3}$$

To evaluate $$15^3$$ i.e cube of $$15$$:

We need to multiply the given number three times that is

$$15\ \times\ 15\ \times\ 15 \ =\ {3375}$$

$$\therefore$$, the cube of $$15$$ is $${3375}$$

Evaluate the cube root of :
$$\displaystyle \sqrt [ 3 ]{ \left (\dfrac{-27}{125} \right ) } $$

1540025_425518_ans_b5b0e041766f41b190478163eb05347c.jpg

Find the cube root of
$$135\sqrt { 3 } -87\sqrt { 6 } $$

$$153\sqrt3-87\sqrt6$$

$$=81\sqrt3+54\sqrt3-81\sqrt6-6\sqrt6$$

$$=(3\sqrt3)^3+(-\sqrt6)^3+3\times3\sqrt3\times(-\sqrt6)(3\sqrt3-\sqrt6)$$

$$=(3\sqrt3-\sqrt6)^3$$

Hence Cube root is $$3\sqrt3-\sqrt6$$

Find the cube root of
$$38+17\sqrt { 5 } $$

$$38+17\sqrt5$$

$$=8+30+5\sqrt5+12\sqrt5$$

$$=(\sqrt5)^3+2^3+3\times2\times(\sqrt5)^2+3\times2^2\times\sqrt5$$

$$=(\sqrt5+2)^3$$

Hence, cube root of $$38+17\sqrt5$$ is $$\sqrt5 +2$$

Find the given number is a perfect cube or not.
$$540$$

$$\sqrt[3]{540}$$

$$=\sqrt[3]{{2}\times {{3}}\times 5\times 19}$$

Hence, the given number is not a perfect cube.

Find the number is perfect cube or not?
$$620$$

We have,

$$\sqrt[3]{620}=\sqrt[3]{2\times 2\times 5\times 31}$$

This is not perfect cube.

Hence, this is the answer.

Find the cube root of each of the following cube numbers through estimation.
$$148877$$

$$148877$$

$${ 50 }^{ 3 }=1,25,000\quad \quad { 55 }^{ 3 }=166375$$

$${ 53 }^{ 3 }=148877$$

$$\Rightarrow \sqrt [ 3 ]{ 148877 } =53$$

Find the cubes of:
$$-30$$

$$ -30 = (-30)^3 = -30 \times -30 \times -30 $$

$$ = -(30 \times 30 \times 30) = -27000 $$

Evaluate $$\sqrt[3]{64}$$

The prime factors of $$64 = 2\times2\times2\times2\times2\times2$$

Now by grouping into three we get, $$(2\times2\times2)\times(2\times2\times2)$$

$$\therefore$$ $$\sqrt[3]{((2)^{3}\times(2)^{3})} =(2\times2)=4$$

$$\therefore$$ $$\sqrt[3]{64}$$$$=4$$

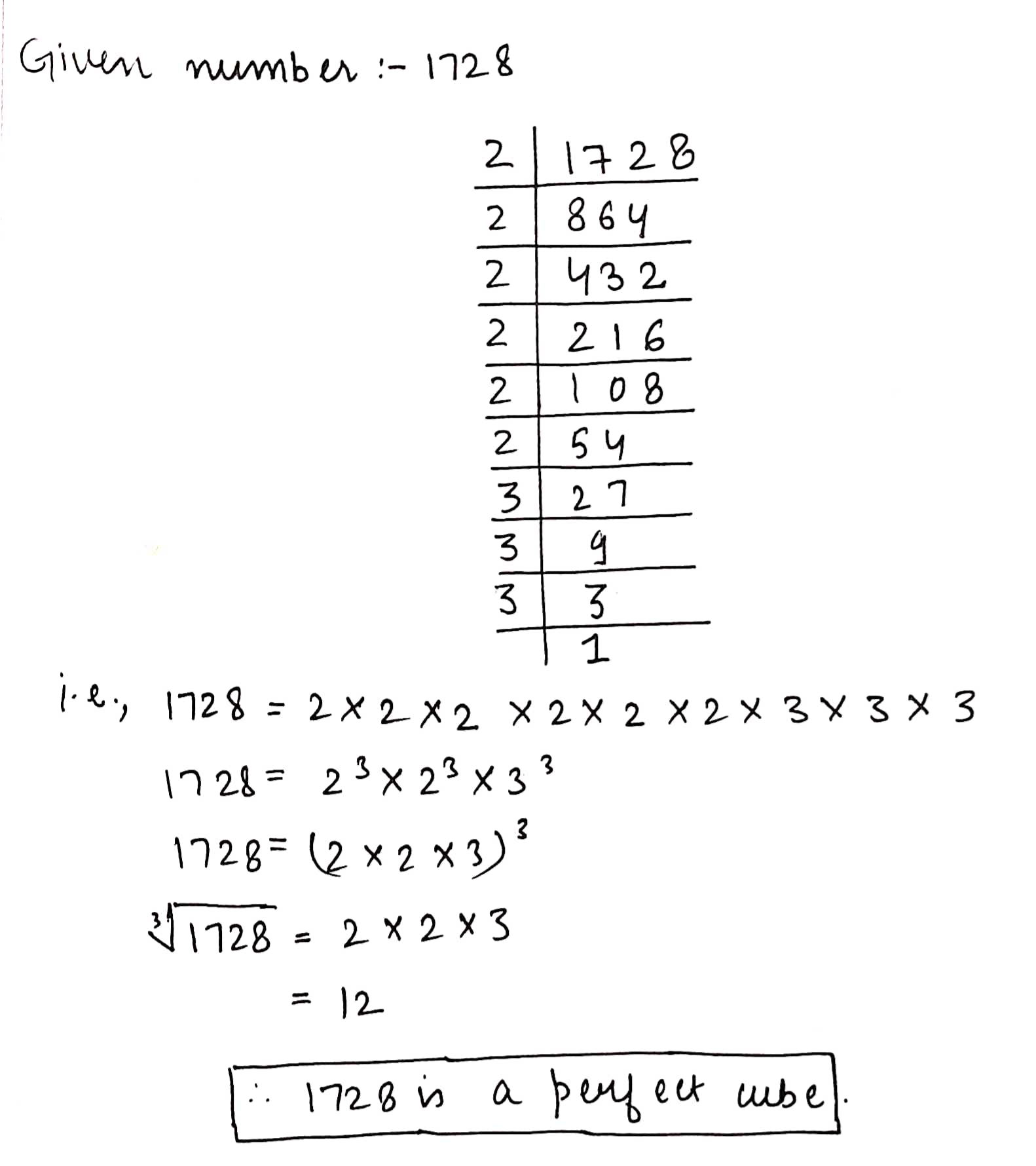
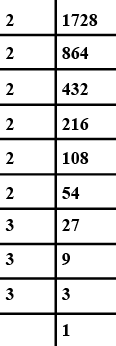
Which of the following numbers are not perfect cubes?
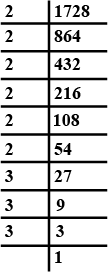
$$1728$$

2039788_1671849_ans_202d2765baeb4da8ba9b32105b29e671.jpg

Find the cube root of the given number through estimation:
$$1512$$

$$1512=2\times 2\times 2\times 3\times 3\times 3\times 7\\ \therefore \sqrt [ 3 ]{ 1512 } =2\times 3\times \sqrt [ 3 ]{ 7 } \\ =6\times \sqrt [ 3 ]{ 7 } \\ =6\times 1.913$$

$$=11.48$$ (approx.)

Evaluate: $$\sqrt[3] {8\times 17\times 17\times 17}$$.

On prime factorising, we get,

$$8=\underline{2 \times 2\times 2}$$.

Then,

$$\sqrt[3] {8\times 17\times 17\times 17}$$
$$=\sqrt[3] {\underline{2\times 2\times 2}\times \underline{17\times 17\times 17}}$$
$$=2\times 17$$
$$=34$$.

Therefore, $$34$$ is the solution.

The natural number whose cube is equal to itself is ____ .

Consider the number $$1$$.

Then, cube of $$1$$, i.e. $$ \displaystyle 1^{3}=1.$$

That is, $$1$$ is a natural number whose cube is $$1$$ itself.

Therefore, the natural number whose cube is equal to itself is $$1$$.

Evaluate :
(i) (1.2)$$ \displaystyle ^{3}$$
(ii) (3.5)$$ \displaystyle ^{3}$$
(iii) (0.8)$$ \displaystyle ^{3}$$
(iv) (0.5)$$ \displaystyle ^{3}$$

$$1.2\times 1.2\times 1.2=1.728$$

$$3.5\times 3.5\times 3.5=42.875$$

$$0.8\times 0.8\times 0.8=0.512$$

$$0.5\times 0.5\times 0.5=0.125$$

If $$a+b+c=0$$ then prove that $$a^3+b^3+c^3=3abc$$.

Given $$a+b+c=0$$

or, $$a+b=-c$$.......(1).

Now cubing both sides we get,

$$(a+b)^3=-c^3$$

or, $$a^3+b^3+3ab(a+b)=-c^3$$

or, $$a^3+b^3-3abc=-c^3$$ [ Using (1)]

or, $$a^3+b^3+c^3=3abc$$.

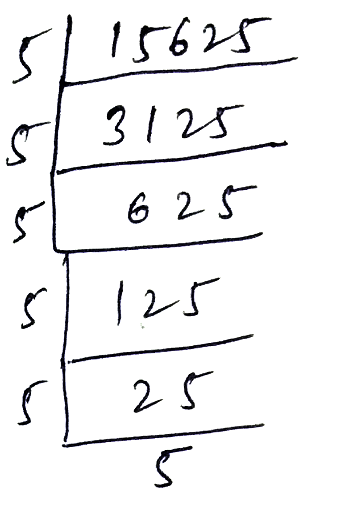
Find the cube root of $$15625$$ by prime factorization method.

$$15625=5\times 5\times 5\times 5\times 5\times 5=5^{6}$$

$$\therefore \sqrt[3]{15625}=(5^{6})^{\frac{1}{3}}=5^{2}=25$$

1042385_1038008_ans_a23f3ed665994b86b7a66ea4ab740b44.png

Find the smallest number by which $$243$$ must be multiplied to obtain a perfect cube.

Prime factorizing $$243$$, we get,

$$243=3\times 3\times 3\times 3\times 3=3^5$$.

We know, a perfect cube has multiples of $$3$$ as powers of prime factors.
Here, number of $$3$$'s is $$5$$.

So we need to multiply another $$3$$ in the factorization to make $$243$$ a perfect cube.

Hence, the smallest number by which $$243$$ must be multiplied to obtain a perfect cube is $$3$$.

Find the smallest number by which $$72$$ must be multiplied to obtain a perfect cube.

Prime factorising $$72$$, we get,

$$72= 2\times2\times2\times3\times3$$ $$ = 2^{3}\times3^2$$.

We know, a perfect cube has multiples of $$3$$ as powers of prime factors.

Here, number of $$2$$'s is $$3$$ and number of $$3$$'s is $$2$$.

So we need to multiply another $$3$$ in the factorization to make $$72$$ a perfect cube.

Hence, the smallest number by which $$72$$ must be multiplied to obtain a perfect cube is $$3$$.

Find the smallest number by which $$675$$ must be multiplied to obtain a perfect cube.

Prime factorising $$675$$, we get,

$$675=5\times 5\times 3\times 3\times 3$$

$$ = 3^{3}\times5^2$$.

We know, a perfect cube has multiples of $$3$$ as powers of prime factors.

Here, number of $$3$$'s is $$3$$ and number of $$5$$'s is $$2$$.

So we need to multiply another $$5$$ in the factorization to make $$675$$ a perfect cube.

Hence, the smallest number by which $$675$$ must be multiplied to obtain a perfect cube is $$5$$.

Find the smallest number by which the following number must be divided to obtain a perfect cube:
$$128$$

Prime factorising $$128$$, we get,

$$128= 2 \times 2\times 2\times 2 \times 2 \times 2 \times 2 =2^7$$.

We know, a perfect cube has multiples of $$3$$ as powers of prime factors.

Here, number of $$2$$'s is $$7$$.

So we need to divide the factorization by $$2$$ to make $$128$$ a perfect cube.

Hence, the smallest number by which $$128$$ must be divided to obtain a perfect cube is $$2$$.

Find the cubic root of the following number by prime factorisation method:
$$13824$$

On prime factorising, we get,

$$13824 = 2\times2\times2\times2\times2\times2\times2\times2\times2\times3\times3\times3$$

$$= 2^3\times2^3\times2^3\times3^3$$.

Then, cube root of $$13824$$ is:

$$\sqrt[3]{13824}=\sqrt[3]{2^3\times 2^3\times2^3 \times 3^3}= 2\times2\times2\times3=24$$.

Therefore, $$24$$ is the required solution.

Find the smallest number by which $$256$$ must be multiplied to obtain a perfect cube.

Prime factorizing $$256$$, we get,

$$256 = 2\times2\times2\times2\times2\times2\times2\times2=2^8$$.

We know, a perfect cube has multiples of $$3$$ as powers of prime factors.
Here, number of $$2$$'s is $$8$$.

So we need to multiply another $$2$$ in the factorization to make $$256$$ a perfect cube.

Hence, the smallest number by which $$256$$ must be multiplied to obtain a perfect cube is $$2$$.

Find the cube roots of $$1728$$ by prime factorization:

On prime factorising, we get,

$$1728 = (2 \times 2 \times 2) \times (2 \times 2 \times 2) \times (3 \times 3 \times 3)$$

$$= 2^3 \times 2^3 \times 3^3$$.

Then, cube root of $$1728$$ is:

$$\sqrt[3]{1728}=\sqrt[3]{2^3 \times 2^3 \times3^3}= 2\times 2\times 3=12$$.

Therefore, $$12$$ is the solution.

Find the cube of $$133$$.

Cube of the number $$133$$:

$$(133)^{ 3 } =133\times133\times133 = 2352637$$.

Evaluate: $$\sqrt [3]{8\times 125}$$.

On prime factorisation of the numbers individually, we get,

$$ 8=\underline { 2 \times 2 \times 2 }=2^3$$.

$$125=\underline { 5 \times 5 \times 5 }=5^3$$.

Therefore,

$$ \displaystyle \sqrt [ 3 ]{ 8 \times 125 } = \displaystyle \sqrt[3]{2^3 \times 5^3}=2 \times 5=10$$.

Thus, $$10$$ is the solution.

Find the cube root of $$474552$$:

On prime factorising, we get,

$$\Rightarrow {474552}={\underline{2 \times 2 \times 2}\times \underline{3 \times 3 \times 3}\times \underline {13 \times 13 \times 13}}$$

$$= 2^3 \times 3^3 \times 13^3$$.

Then, cube root of $$474552$$ is:

$$\sqrt[3]{474552}=\sqrt[3]{3^3 \times 2^3 \times 13^3}= 2\times 3\times 13=78$$.

Therefore, $$78$$ is the solution.

By what smallest number $$3645$$ be multiplied so that the product becomes a perfect cube?

Prime factorising $$3645$$, we get,

$$ 3645 = 5 \times 3 \times 3 \times 3 \times 3 \times 3 \times 3 $$

$$= 5^1 \times 3 ^6$$.

We know, a perfect cube has multiples of $$3$$ as powers of prime factors.

Here, number of $$5$$'s is $$1$$ and number of $$3$$'s is $$6$$.

So we need to multiply another $$5^2$$ to the factorization to make $$3645$$ a perfect cube.

Hence, the smallest number by which $$3645$$ must be multiplied to obtain a perfect cube is $$5^2=25$$.

Find the negative of cube root of $$-2744000$$.

We need to find the cube root of $$-2744000$$.

Since the number contains a negative sign, its cube root will also be negative (only for odd roots).

Prime factorising, we get,

$$ {274400}= {\underline{2\times2\times2}\times \underline{2\times2\times2}\times \underline {5\times5\times5} \times \underline {7\times7\times7}}$$.

Since we need to find the cube root we group them in 3.

$$ \sqrt[3]{274400}=\sqrt[3]{\underline{2\times2\times2}\times \underline{2\times2\times2}\times \underline {5\times5\times5} \times \underline {7\times7\times7}}$$.

From each group, take single number and multiply them.

Therefore, we get,

$$ \sqrt[3]{274400}$$ $$=2\times2\times5\times7=140$$.

Since, the given cube root $$ \sqrt[3]{-274400}$$ contains a minus sign, the answer will also contain a minus sign.

Therefore, $$ \sqrt[3]{-274400}$$ $$=-140$$.

Then, negative of $$-140$$ is $$140$$.

Find the smallest number by which $$1323$$ must be multiplied so that the product is a perfect cube.

Prime factorising $$1323$$, we get,

$$1323 = 3 \times 3 \times 3 \times 7 \times 7 $$

$$= 3 ^3 \times 7 ^2 $$.

We know, a perfect cube has multiples of $$3$$ as powers of prime factors.

Here, number of $$3$$'s is $$3$$ and number of $$7$$'s is $$2$$.

So we need to multiply another $$7$$ in the factorization to make $$1323$$ a perfect cube.

Hence, the smallest number by which $$1323$$ must be multiplied to obtain a perfect cube is $$7$$.

Show that $$\sqrt [3]{125\times 64}=\sqrt [3]{125}\times \sqrt [3]{64}$$

$$\Rightarrow \sqrt [3]{125\times 64}=\sqrt [3]{125}\times \sqrt [3]{64}$$
$$\Rightarrow \sqrt[3]{(5)^3\times (4)^3}=\sqrt[3]{(5)^3}\times \sqrt[3]{(4)^3}$$
$$\Rightarrow 5\times 4=5\times 4$$
$$\Rightarrow 20=20$$
$$\Rightarrow 20-20=0$$

Find the value of $$\displaystyle (28)^{3}-(78)^{3}+(50)^{3}$$

Let $$a = 28, b = - 78, c = 50$$
Then $$a + b + c = 28 - 78 + 50 = 0$$
Using, If $$(a+b+c)=0$$ ,then $$a^3+b^3+c^3=3abc$$
$$\therefore a^{3}+b^{3}+c^{3}=3abc$$
So $$\displaystyle (28)^{3}+ (-78)^{3}+(50)^{3}=3\times 28\times (-78)\times 50=-327600$$

Show that $$\sqrt [3]{216\times (-343)}=\sqrt [3]{216}\times \sqrt [3]{-343}$$

$$\sqrt [3]{216\times (-343)}=\sqrt [3]{216}\times \sqrt [3]{-343}$$
$$\sqrt[3]{\left (6 \right )^3\times\left (-7 \right )^3 }=\sqrt[3]{\left (6 \right )^3}\times \sqrt[3]{\left (-7 \right )^3}$$
$$6\times -7=6\times -7$$
$$-42=-42$$
$$-42+42=0$$

Evaluate: $$\sqrt [ 3 ]{ 27\times 64 } $$.

On prime factorisation of the numbers individually, we get,

$$27=\underline {3 \times 3 \times 3 }=3^3$$.

$$64=\underline { 2 \times 2 \times 2 } \times \underline { 2 \times 2 \times 2 }=4^3$$.

Therefore,

$$\sqrt[3]{27\times 64}$$ $$=\sqrt[3]{3^3\times 4^3}=3\times4=12$$.

Hence, $$12$$ is the solution.

Evaluate $$(96)^3$$

$$ (96)^3 = (100-4)^3$$
$$= ^3C_0 (100)^3 - ^3C_1 (100)^2(4) + ^3C_2 (100)(4)^2 - ^3C_3 (4)^3$$
$$= (100)^3 - 3(100)^2 (4) + 3(100)(4)^2 - (4)^3$$
$$= 1000000 - 120000 + 4800 - 64$$

$$= 884736$$

Evaluate $$\sqrt [ 3 ]{ 125\times 64 } $$.

On prime factorising, we get,

$$125=5 \times 5\times 5$$ $$= 5^3$$

$$64=2\times2\times2\times2\times2\times2= 4 \times 4\times 4$$ $$= 4^3$$.

Then,

$$\sqrt[3]{125 \times 64}=\sqrt[3]{5^3\times4^3}= 5\times4=20$$.

Therefore, $$20$$ is the solution.

Find the cube root of the following number by prime factorisation method
175616

$$ \displaystyle 175616=2^{3}\times 2^{3}\times 2^{3}\times 7^{3} $$
$$\displaystyle \Rightarrow $$$$ \displaystyle \sqrt[3]{175616}=2\times 2\times 2\times 7=56 $$

Find the cube root of the following number by the prime factorization method.
91125

Sum of the number $$91125 = 18$$ that means the number is divisible by $$3$$. Also, the number ends with $$5$$ therefore, it is also divisible by $$5$$.

Thus,

$$ \displaystyle 91125= 5\times 5\times 5\times 3\times 3\times 3\times 3\times 3\times 3$$

$$ = 5^{3}\times 3^{3}\times 3^{3} $$

$$ \displaystyle \Rightarrow \sqrt[3]{91125}=5\times 3\times 3=45 $$

Evaluate the following:$$\sqrt [3] { 512\times 729 }$$

$$\sqrt[3]{{512 \times 729}}$$

$$ = \sqrt[3]{{8 \times 8 \times 8 \times 9 \times 9 \times 9}}$$

$$=8 \times 9$$

$$=72$$

Find the negative of cube root of $$-512$$.

On prime factorising, we get,

$$512=\underline{2\times 2\times 2}\times \underline{2\times 2\times 2}\times \underline {2\times2\times2}$$

$$=2^3\times 2^3\times 2^3=8^3$$.

Then, $$-512$$ $$=(-8)^3$$.

Therefore, cube root of $${-512}$$,

i.e. $$\sqrt[3]{-512}=\sqrt[3]{(-8)^3}= -8$$.

Then, negative of cube root of $${-512}$$ means negative of $$-8=8$$.

Hence, $$8$$ is the solution.

Find the cube root of: $$8$$

On prime factorising, we get,

$$8=2 \times 2\times 2$$ $$= 2^3 $$.

Then, cube root of $$8$$ is:

$$\sqrt[3]{8}=\sqrt[3]{2^3}= 2$$.

Therefore, $$2$$ is the solution.

Find $$(7)^3=$$?

$$\displaystyle { \left( 7 \right) }^{ 3 }$$

$$=7\times 7\times 7$$

$$=343$$

The value of $$(21)^3$$ is?

The value of $$(21)^3$$:

$$(21)^3 = 21 \times 21 \times 21$$

$$=9261$$.

Find $$(12)^3=$$?

$$\displaystyle { \left( 12 \right) }^{ 3 }$$

$$=12\times 12\times 12$$

$$=144\times 12$$

$$=1728$$.

Hence, $$\displaystyle { \left( 12 \right) }^{ 3 }$$ $$=1728$$.

Using the method of successive subtraction of numbers $$1, 7, 19, 37, 61, 91, 127, 169, 217, 271, 331, 397,..........$$
Examine if the following number is a perfect cube or not
792

792-1=791, 791-7=784, 784-19= 765, 765-37=728, 728-61=667, 667-91=576, 576-127=449, 449-169=280,80-217=63.
The next number to be subtracted is 331 which is greater than 63.

The remainder got is not zero.

$$\displaystyle \\ \therefore $$ 792 is not a perfect cube.

Find $$(100)^3$$.

The value of $$(100)^3$$:

$$\displaystyle { \left( 100 \right) }^{ 3 }$$

$$=100\times 100\times 100$$

$$=1000000$$.

Multiply $$137592$$ by the smallest number so that the product is a perfect cube.

Prime factorising $$137592$$, we get,

$$\displaystyle 137592=2\times 2\times 2\times 3\times 3\times 3 \times 7\times 7\times 13$$

$$= 2 ^3 \times 3 ^3 \times 7^2 \times 13$$.

We know, a perfect cube has multiples of $$3$$ as powers of prime factors.

Here, number of $$2$$'s is $$3$$, number of $$3$$'s is $$3$$, number of $$7$$'s is $$2$$ and number of $$13$$'s is $$1$$.

So we need to multiply another $$7$$ and $$13^2$$ to the factorization to make $$137592$$ a perfect cube.

Hence, the smallest number by which $$137592$$ must be multiplied to obtain a perfect cube is $$7\times 13^2=1183$$.

Find the value of $$(302)^3$$?

The value of $$(302)^3$$:

$$\displaystyle { \left( 302 \right) }^{ 3 }$$

$$=302\times 302\times 302$$

$$=27543608$$.

Write cubes of $$5$$ natural numbers which are multiples of $$3$$ and verify the following:
'The cube of natural number, which is a multiple of $$3$$ is a multiple of $$27$$.

$$\displaystyle { \left( 3 \right) }^{ 3 }=3\times 3\times 3=27$$
$$\displaystyle { \left( 6 \right) }^{ 3 }=6\times 6\times 6=216$$
$$\displaystyle { \left( 9 \right) }^{ 3 }=9\times 9\times 9=729$$
$$\displaystyle { \left( 12 \right) }^{ 3 }=12\times 12\times 12=1728$$
$$\displaystyle { \left( 15 \right) }^{ 3 }=15\times 15\times 15=3375$$
Verification:
$$\displaystyle { \left( 3 \right) }^{ 3 }=27=27\times 1$$
$$\displaystyle { \left( 6 \right) }^{ 3 }=216=27\times 8$$
$$\displaystyle { \left( 9 \right) }^{ 3 }=729=27\times 27$$
$$\displaystyle { \left( 12 \right) }^{ 3 }=1729=27\times 64$$
$$\displaystyle { \left( 15 \right) }^{ 3 }=3375=27\times 125$$
$$\displaystyle \therefore $$ 'The cube of natural number, which is a multiple of 3 is a multiple of 27'.

Find the cube root of $$74088$$.

On prime factorising, we get,

$$74088 = 2\times2\times2\times3\times3\times3\times7\times7\times7$$

$$= 2^3\times3^3\times7^3$$.

Then, cube root of $$74088$$ is:

$$\sqrt[3]{74088}=\sqrt[3]{2^3 \times 3^3 \times 7^3}= 2\times3\times7=42$$.

Therefore, $$42$$ is the required cube root.

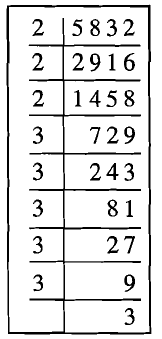
Find the cube root of a given number through estimation: $$5832$$.

Step $$I$$: Form groups of $$3$$ starting from right most digit of $$5832$$, i.e. $$\displaystyle \overline { 5 }\: \overline { 832 } $$.

Then, the two groups are $$5$$ and $$832.$$

Here, $$5$$ has $$1$$ digit and $$832$$ has $$3$$ digit.

Step $$II$$: Take $$832$$.

Digit in unit place $$= 2$$.

Therefore, we take one's place of required cube root as $$8$$ ....[Since, $$8^3=512$$].

Step $$III$$: Now, take the other group $$5$$.

We know, $$\displaystyle {1}^{ 3 }=1 $$ and $${2 }^{ 3 }=8$$.

Here, the smallest number among $$1$$ and $$2$$ is $$1$$.

Therefore, we take $$1$$ as ten's place.

$$\displaystyle \therefore \quad \sqrt[3] { 5832 } =18$$.

By the method of finding unit digit and tens digit of cube root, find the cube root of $$-68921.$$

Cube root of $$-68921$$ is that,

$$\begin{array}{l} \sqrt [ 3 ]{ { -68921 } } \\ ={ \left( { { { \left( { -41 } \right) }^{ 3 } } } \right) ^{ ^{ \frac { 1 }{ 3 } } } } \\ =-41 \end{array}$$

State true or false.
(i) Cube of any odd number is even.
(ii) A perfect cube does not end with two zeros.
(iii) If square of a number ends with $$5$$, then its cube ends with $$25$$.
(iv) There is no perfect cube which ends with $$8$$.
(v) The cube of a two digit number may be a three digit number.
(vi) The cube of a two digit number may have seven or more digits.
(vii) The cube of a single digit number may be a single digit number.

(i) Cube of any odd number is even.

FALSE: Odd multiplied by odd is always odd

(ii) A perfect cube does not end with two zeros.

TRUE: A perfect cube will end with odd number of zeroes

(iii) If square of a number ends with $$5$$, then its cube ends with $$25$$.

TRUE: $$5$$ multiplied by $$5$$ any number of times always gives $$5$$ at units place

(iv) There is no perfect cube which ends with $$8$$.

False: $$ 2^{3} =8 $$

(v) The cube of a two digit number may be a three digit number.

FALSE: The smallest two digit number is $$10$$ and $$10^{3} $$ $$=1000$$ is a three digit number

(vi) The cube of a two digit number may have seven or more digits.

FALSE: $$99$$ is the largest $$2$$ digit number; $$ 99^{3} $$ $$=989901$$ is a $$6$$ digit number

(vii) The cube of a single digit number may be a single digit number.

TRUE: $$ 2^{3} = 8 $$ is a single digit number.

Looking at the pattern, fill in the gaps in the following
$$2$$ $$3$$ $$4$$ $$-5$$ $$8$$
$$2^3 = 8$$ $$3^3 = ......$$ $$.... = 64$$ $$..... = .....$$ $$6^3 = .....$$ $$..... = ....$$ $$.... = -729$$

$${ 2 }^{ 3 }=8$$

$${ 3 }^{ 3 }=27$$

$${ 4 }^{ 3 }=64$$

$${ \left( -5 \right) }^{ 3 }=-125$$

$${ { 6 }^{ 3 }= }216$$

$${ 8 }^{ 3 }=512$$

$${ \left( -9 \right) }^{ 3 }=729$$

How many perfect cubes you can find from $$1$$ to $$100$$? How many from $$-100$$ to $$100$$?

Let us find the perfect cubes between $$1$$ to $$100$$.

We know that, $$1^3=1, 2^3=8, 3^3=27, 4^3=64$$ and the rest numbers i.e. $$4,5,6,....$$ have their cubes greater than $$100$$.

So, only these four numbers have their cubes between $$1$$ to $$100$$.

Thus, there are $$4$$ perfect cubes from $$1$$ to $$100$$.

Now, let's find the cubes between $$-100$$ to $$0$$

We know, $$0^3=0, (-1)^3=-1, (-2)^3=-8, (-3)^3=-27, (-4)^3=-64$$ and the rest numbers have their cubes less than $$-100$$

So, only these $$5$$ numbers have their cubes between $$-100$$ to $$0$$ and $$4$$ perfect cubes are there from $$1$$ to $$100$$.

Thus, there are $$9$$ perfect cubes from $$-100$$ to $$100$$.

Find the cube root by prime factorisation: $$46656$$

Writing the prime factors of $$46656$$ we get: $$46656=$$$$2\times 2\times 2\times 2\times 2\times 2\times 9\times 9\times 9$$

Taking one prime number from each of the triplets we get:

$$\sqrt [ 3 ]{ 46656 } =2\times 2\times 9=36$$

Find the cube root by prime factorization $$10648$$

Writing the prime factors of 1$$10648$$ we get:

$$10648=$$ $$2\times 2\times 2\times 11\times 11\times 11$$

Taking one prime number from each of the triplets of prime numbers we get $$\sqrt [ 3 ]{ 10648 } =11\times 2=22$$

What is the least positive integer k for which 15k is the cube of a number?

Prime factor of 15=$$5\times 3$$

To make a perfect cube we make the pair of three in the prime factor.

$$\therefore $$Prime factor is multiply by=$$5\times 5\times 3\times 3=225$$

Find the cube root by prime factorisation $$15625$$

Writing the prime factors of $$15625$$ we get: $$15625=$$$$5\times 5\times 5\times 5\times 5\times 5$$

Taking one prime number from each of the triplets we get:

$$\sqrt [ 3 ]{ 15625 } =5\times 5=25$$

Find the cube of $$6$$.

We have $$6^3 = 6 \times 6 \times 6 = 36 \times 6 = 216.$$

What is the least positive integer with which you have to multiply $$243$$ to get a perfect cube?

Let us factorise 243. We observe that
$$243 = 3 \times (81) = 3 \times 3 \times 3 \times 3 \times 3.$$
If we multiply $$243$$ by $$3$$, we see that
$$243 \times 3 = 3 \times 3 \times 3 \times 3 \times 3 \times 3 = 9 \times 9 \times 9$$,
and we get a perfect cube. The answer is therefore $$3$$.

Find the cube root of
$$99-70\sqrt { 2 } $$

Given term: $$99-70\sqrt2$$

This can be written as

$$=27+72-16\sqrt2-54\sqrt2$$

$$=3^3+(-2\sqrt2)^3+3\times3^2\times(-2\sqrt2)+3\times3\times(-2\sqrt2)^2$$

$$=3^3-(2\sqrt2)^3-3\times3^2\times(2\sqrt2)+3\times3\times(-2\sqrt2)^2$$ $$[(a-b)^3 = a^3 - b^3 - 3a^2b+3ab^2]$$

$$=(3-2\sqrt2)^3$$

Hence, cube root of $$(99-70\sqrt2)$$ is $$(3-2\sqrt2)$$

$$Evaluate\,\sqrt[3]{{0.000343}} + \sqrt[3]{{0.729}} + \sqrt[3]{{1.331}}$$

$$\root 3 \of {0.000343} + \root 3 \of {0.729} + \root 3 \of {1.331} $$

$$ = \root 3 \of {\dfrac{{343}}{{1000000}}} + \root 3 \of {\dfrac{{729}}{{1000}}} + \root 3 \of {\dfrac{{1331}}{{1000}}} $$

$$ = \dfrac{7}{{100}} + \dfrac{9}{{10}} + \dfrac{{11}}{{10}}$$

$$ = \dfrac{{7 + 90 + 110}}{{100}} = \dfrac{{207}}{{100}} = 2.07$$

Find the cube root of a given number through estimation: $$2197$$.

Step $$I$$: Form groups of $$3$$ starting from right most digit of $$2197$$, i.e. $$\displaystyle \overline { 2 }\: \overline {197 } $$.

Then, the two groups are $$2$$ and $$197.$$

Here, $$2$$ has $$1$$ digit and $$197$$ has $$3$$ digit.

Step $$II$$: Take $$197$$.

Digit in unit place $$= 7$$.

Therefore, we take one's place of required cube root as $$3$$ ....[Since, $$3^3=27$$].

Step $$III$$: Now, take the other group $$2$$.

We know, $$\displaystyle { 1}^{ 3 }=1$$ and $${ 2 }^{ 3 }=8$$.

Here, the smallest number among $$1$$ and $$2$$ is $$1$$.

Therefore, we take $$1$$ as ten's place.

$$\displaystyle \therefore \quad \sqrt[3] {2197} =13$$.

Factorise $$8{x^3} + 27{y^3} + 36{x^3}y + 54x{y^2}$$

Given that:

$$8x^3+27y^3+36x^2y+54xy^2$$

$$=(2x)^3+(3y)^3+3(2x)^2(3y)+3(2x)(3y)^2$$

Since we know $$(a + b)^3 = a^3 + b^3 + 3a^2b + 3ab^2$$

Therefore,

$$=(2x + 3y)^3$$

$$=(2x+3y)(2x+3y)(2x+3y)$$

Hence , factorization of given expression is $$(2x + 3y) , (2x + 3y)$$ and $$(2x + 3y)$$.

Find the cube roots of $$64$$

$$64$$ can be written as $$4\times 4\times 4$$

$$\Rightarrow 64=4^3$$

$$\Rightarrow (64)^{1/3}=4$$

Therefore cube root of $$64$$ is $$4$$

Which of the following numbers are perfect cubes? In case of perfect cube find the number with cube is the given number.
$$21952$$

We have,

$$\displaystyle \sqrt[3]{21952}=\sqrt[3]{2\times2\times2\times2\times2\times2\times7\times7\times7}$$

This is perfect cube.

Hence, this is the answer.

Find the cube of the following:
$$0.4$$.

$$0.4^3=4^3*0.1^3=64*.001=.064$$

Cube of $$\left(2-\dfrac{1}{3}\right)$$ is

$${ (2-\cfrac { 1 }{ 3 } ) }^{ 3 }$$

$${ (a-b) }^{ 3 }={ a }^{ 3 }-{ b }^{ 3 }-3{ a }^{ 2 }b+3a{ b }^{ 2 }$$

$$ { (2-\cfrac { 1 }{ 3 } ) }^{ 3 }=8-\cfrac { 1 }{ 27 } -4+\cfrac { 2 }{ 3 }$$

$$=4-\cfrac { 1 }{ 27 }+\cfrac { 2 }{ 3 }$$

$$=\cfrac {4\times 27-1+2\times 9 }{ 27 }$$

$$=\cfrac {108-1+18}{ 27 }$$

$$ =\cfrac { 125 }{ 27 } $$

Find that the number is a perfect cube or not?
$$588$$

$$\sqrt[3]{588}$$

$$=\sqrt[3]{{{2}^{4}}\times 43}$$

Hence, the given number is not a perfect cube.

Among the following, the correct statement is
i) $$(a + b)^3 = a^3 + 3a^2b + 3ab^2 + b^3$$
ii) $$(a - b)^3 = a^3 - 3a^2b + 3ab^2 - b^3$$

i) $$(a + b)^3 = a^3 + 3a^2b + 3ab^2 + b^3$$

ii) $$(a - b)^3 = a^3 - 3a^2b + 3ab^2 - b^3$$

These are direct formulas for finding the cube of numbers.

So both of the formulas are true.

$$\sqrt[3]{{8 \times 125}}$$

$$\sqrt[3]{8 \times 125}$$

$$= \sqrt[3]{{2}^{3} \times {5}^{3}}$$

$$= 2 \times 5 = 10$$

Find following is a perfect cubes or not ?
$$9000$$

$${ 10 }^{ 3 }\times { 3 }^{ 2 }$$

$$ \therefore $$ Not a perfect cube.

1157999_1090546_ans_39fa3e41af4847dab45701d037b2eea1.png

Find $$8000$$ is a perfect cubes or not ?

$$={ 20 }^{ 3 }=8000$$

$$\therefore$$ Perfect cube.

1157795_1090543_ans_a338663d7b1446c1ade29e626dc2afd6.png

Find if the given number is a perfect cube or not?
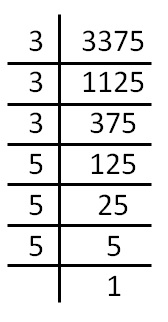
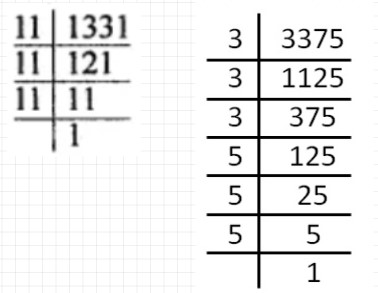
$$3375$$

$$3375=3^3\times 5^3$$

$$\because$$ both the factors $$3$$ and $$5$$ occur in triplet

$$\therefore 3375$$ is a perfect cube.

1156862_1090542_ans_1da6c0c4c9804ff69a71682a2594d119.jpg

Find it is a perfect cubes or not ?
$$400$$

$$\therefore$$ Not a perfect cube.
1155753_1090541_ans_a654acbc94d347bea6b488c4b3122d1d.png

Find the given number is a perfect cube or not.
$$13824$$

$$\sqrt[3]{13824}$$

$$=\sqrt[3]{{{2}^{9}}\times {{3}^{3}}}$$

$$={{2}^{3}}\times 3$$

$$=24.$$

Hence, the given number is a perfect cube.

Find following is a perfect cubes or not ?
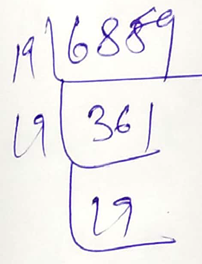
$$6859$$

$${ 19 }^{ 3 }=6859$$

$$\therefore$$ Perfect cube.

1157651_1090547_ans_a10da01166824483a2d13b5821ada374.png

State whether $$10648$$ is a perfect cube or not?

On prime factorization of given number,

$$10648=2\times2\times2\times11\times11\times11$$

$$={{2}^{3}}\times {{11}^{3}}$$

$$=22^3$$

Hence, the given number is a perfect cube of $$22$$.

By multiplying an integer convert number to a perfect cube,Find the number?
$$2025$$

On factoring the given number, we get

$$2025={{5}^{2}}\times {{9}^{2}}$$

Hence, It should be multiplied by $$45$$ to make the given number a perfect cube.

Find it is a perfect cubes or not ?
15625

$$={ 25 }^{ 3 }=15625$$

$$\therefore$$ Perfect cube.

1157797_1090545_ans_6e483740282f4da39f1f77dd47075d53.png

If the square of a number ends with $$5$$, then its cube ends with $$25$$. True or false? Give reason.

True.

The square of a number ends with $$5$$ if and only the number itself ends with $$5$$ so cube of the number will always ends with $$25.$$

For example: $$5^2=25,5^3=125.$$

Find which of the following are perfect cubes?
(i) $$243$$ (ii) $$588$$ (iii) $$1331$$ (iv) $$24000$$ (v) $$1728$$ (vi) $$1938$$

$$243=7^{3}$$

$$588=7^{2}\times 2^{2}\times 3$$

$$1331=11^{3}$$

$$24000=3\times 2^{3}\times 10^{3}$$

$$1728=3^{3}\times 4^{3}=(12)^{3}$$

$$1938=3\times 2\times 323$$

$$\therefore$$ The numbers which are perfect cubes are $$\Rightarrow 243, 1331, 1728$$

1409093_1097277_ans_5a0b6583956b4b5e9ae6672a35333189.png

Test whether the numbers $$243, 516, 729, 8000$$ are perfect cubes or not

$$243$$ is a perfect cube as it is a cube of $$7$$

$$516$$ is not a perfect cube as it is not a cube of any natural number.

$$729$$ is a perfect cube as it is a cube cube of $$9$$

$$8000$$ is a perfect cube as it is a cube cube of $$20$$

So, $$243, 729, $$and $$8000 $$are perfect cubes but $$516$$ is not a perfect cube.

Is $$51123$$ a perfect cube?

1183729_1154250_ans_292ad6c960dd41dba7270137433742d1.jpg

Find the Cube Using Identity (i) $$105$$ (ii) $$27$$ (iii) $$45$$

$$i)105^3=105\times 105\times 105=11,57,625$$

$$ii)27^3=27\times 27\times 27=19,683$$

$$i)45^3=45\times 45\times 45=91,125$$

Solve $$12x^{7}+x^{7}+x$$ for if $$x=7$$?

According to given question,

$$12x^7+x^7+x$$

$$\implies 13x^7+x$$

At, $$x=7$$, this becomes,

$$13(7)^7+7$$

$$\implies 13(823,543)+7$$

$$\implies 10706059+7$$

$$\implies 10706066$$

Which of the following numbers are not perfect cubes?
$$216$$

6 is perfect cubes of 216

Evaluate $$\sqrt [3]{343}$$.

$$\sqrt [3]{343}$$

$$\sqrt [3]{7\times 7 \times 7}=7$$

Find the value of $$64^{\frac{1}{3}}$$.

4*4*4=64

therefore required answer in 4

Solve $$(-10)^{3}+(7)^{3}+(3)^{3}$$

According to the problem,

$${\left( { - 10} \right)^3} + {\left( 7 \right)^3} + {\left( 3 \right)^3}$$

$$ = - 1000 + 343 + 27$$

$$ = - 630$$

Classify as linear, cubic or quadratic.
$$y+y^3+y^2$$.

The given equation

$$y+y^3+y^2$$

the highest degree of polynomial is 3

Therefore it is a cubic equation.

Find the cube of the number $$8$$.

Cube of $$8$$ is:

$$(8)^3=8\times 8\times 8$$

$$=512$$.

Hence, the cube of $$8$$ is $$512$$.

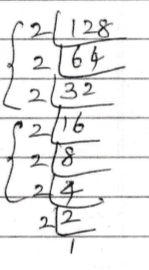
Is $$128$$ a perfect cube?Find its cube root.

$$1252418$$

$$\therefore$$ $$128=(2\times 2\times 2)\times (2\times 2\times 2)\times 2$$

$$\sqrt [ 3 ]{ 128 } ={ \left( { 2 }^{ 3 }\times { 2 }^{ 3 }\times { 2 }^{ } \right) }^{ 1/3 }={ 2 }^{ 3/3 }\times { 2 }^{ 2/3 }\times { 2 }^{ 1/3 }=2\times 2\times \sqrt [ 3 ]{ 2 } =4\sqrt [ 3 ]{ 2 } $$

$$\therefore$$ $$128$$ is not a perfect cube

Its cube root is $$4\sqrt [ 3 ]{ 2 } $$

1343180_1252418_ans_f67c4abca8b7494d84510f1f06aedd8b.PNG

Find the cube root of each of the following cube numbers through estimation.
$$59319$$

We know that,

$${ 35 }^{ 3 }=42875\quad \quad \quad { 40 }^{ 3 }=64000$$

Since,

$$35<\sqrt [ 3 ]{ 59319 } <40$$

$${ 39 }^{ 3 }=59319$$

$$\Rightarrow \sqrt [ 3 ]{ 59319 } =39$$

Hence, this is the answer.

Evaluate:$${\left(103\right)}^{3}$$

According to the equation,
$$ (a +b)^3 = a^3 +b^3+ 3ab (a+b) $$

we get,
$$ (103)^3 $$$$= (100 +3)^3 $$

By using the formula,
$$ = 100^3 +3^3 +3(100)(3)(100 +3) $$
$$ = 1000000 +27 +900 (103 ) $$

On further calculation,
$$ = 1000000 +27 +92700 $$
$$ (103)^3 = 1092727 $$

Evaluate $${ 46 }^{ 3 }+{ 34 }^{ 3 }$$

We have,

$${{46}^{3}}+{{34}^{3}}$$

Then,

$$ {{46}^{3}}+{{34}^{3}} $$

$$ \Rightarrow {{\left( 50-4 \right)}^{3}}+{{\left( 40-6 \right)}^{3}} $$

$$ \Rightarrow {{50}^{3}}-{{4}^{3}}-3\times 50\times 4\left( 50-4 \right)+{{40}^{3}}-{{6}^{3}}-3\times 40\times 6\left( 40-6 \right) $$

$$ \Rightarrow 625000-64-30000+2400+64000-216-28800+4320 $$

$$ \Rightarrow 636640 $$

Hence, this is the answer.

Find the cube root of each of the following numbers:
$$-250047$$

$$250047$$

$$=9\times 9\times 9\times 7\times 7\times 7$$

$$\therefore$$ $$\sqrt [ 3 ]{ 250047 } =9\times 7=63$$

$$\therefore$$ $$\sqrt [ 3 ]{ -\left( 250047 \right) } =-63$$

Hence, this is the answer.

Calculate cube root:
$$8000$$

$$ \sqrt[3]{8000} = \sqrt[3]{2 \times 2 \times 2 \times 10^{3}} = \sqrt[3]{2^{3} \times 10^{3}} = \sqrt[3]{20^{3}}$$

$$ \therefore \sqrt[3]{8000} = 20 $$

1126276_1295097_ans_157cb3bd786344b5bc09dc3df7fe1d4d.JPG

Find the unit's digit of the cube of $$5022$$.

Unit's digit in the cube of $$5022$$ would be equal to the unit's digit of the cube of the digit at unit's place of that number.

$$\therefore $$ for the number $$5022$$ the digit at unit's place is $$2$$.

$$\Rightarrow$$ cube of $$2 = 2^3 = 8$$

Therefore, Cube of $$2 $$ has the digit at unit's place = $$8$$

Hence, the Unit's digit in the cube of $$5022$$ would be equal to $$8$$.

Which of the following numbers are not perfect cubes?
$$ 1000$$

10 is perfect cube of 1000

Evaluate $$\sqrt[3] {(-1331) \times 3375}$$

$$\sqrt[3]{(-1331) \times (3375)}$$ $$= \sqrt[3]{-1331} \times \sqrt[3]{3375}$$

$$= -\sqrt[3]{1331} \times \sqrt[3]{3375}$$

$$= -\sqrt[3]{11 \times 11 \times 11} \times \sqrt[3]{3 \times 3 \times 3 \times 5 \times 5 \times 5}$$

$$= -11 \times 3 \times 5 $$

$$= -11 \times 15$$

$$= -165$$

$$\therefore \sqrt[3]{(-1331) \times (3375)} = -165$$
1760566_1806867_ans_475ca28c05ba41678fe1725ba5eba1de.jpg

Find the cube root of the following numbers by prime factorization:
$$35937$$

$$\sqrt[3]{35937}$$
$$= \sqrt[3]{3 \times 3 \times 3 \times 11 \times 11 \times 11}$$
$$= 3 \times 11 = 33$$
Thus, the cube root of $$35937$$ is $$33.$$

Find the cubes of the following number:
$$-13$$

Cube of $$-13 = (-13)^3$$
$$= (-13) \times (-13) \times (-13)$$
$$= -2197$$

Solved:
$$\sqrt [ 3 ]{ 64 } $$

$$\sqrt[3]{64}=\sqrt[3]{4^{3}}\\(4^3)^{1/3}=4$$

Find the cubes of the following number:
$$3 \dfrac{1}{5}$$

Cube of $$3 \dfrac{1}{5}$$
$$= (16 / 5)^3 = (16 \times 16 \times 16) / (5 \times 5 \times 5)$$
$$= 4096 / 125$$
$$= 32 \dfrac{96}{125}$$

Find the cubes of the following number:
$$-5 \dfrac{1}{7}$$

Cube of $$-5 \dfrac{1}{7}$$
$$= (-36 / 7)^3 = (-36 \times -36 \times -36) / (7 \times 7 \times 7)$$
$$= -46656 / 49$$
$$= -136 \dfrac{8}{343}$$

Find the cube of :
$$54$$

$$(54)^3 = 54 \times 54 \times 54 = 157464 $$

Find the cube of :
$$31$$

$$(31)^3 = 31 \times 31 \times 31 = 29791 $$

Find the cubes of :
$$1.6$$

$$ 1.6 = (1.6)^3 = \left ( \dfrac{16}{10} \right )^3 = \dfrac{16 \times 16 \times 16}{10 \times 10 \times 10} $$
(Splitting the terms)
$$ = \dfrac{4096}{1000} = 4.096 $$

Find the side of a cube whose volume is $$4096 \,m^3.$$

Given,
Volume of a cube $$= 4096 \,m^3$$
We know that,
Thus, the side of the cube is $$16$$ m.
1760654_1806914_ans_fcb69e7b9ef34f3ea63b7c471f40ca8e.png

Find the cube root of the following numbers by prime factorisation:
$$21952$$

Thus, the cube root of $$21952$$ is $$28.$$
1760623_1806897_ans_beffeb15dd04402289c113c919958daf.png

Find the cube of :
$$42$$

$$(42)^3 = 42 \times 42 \times 42 = 74088 $$

Find the cube of the following number:
$$-3 \dfrac{4}{9}$$

Cube of $$-3 \dfrac{4}{9}$$ $$= -31 / 9$$ is
$$= (-31 / 9) \times (-31 / 9) \times (-31 / 9)$$
$$= -29791 / 729$$
$$= -40 \dfrac{631}{729}$$

Find the cube of the following number:
$$-17$$

Cube of $$-17 = (-17) \times (-17) \times (-17)$$
$$= -4913$$

Find the value of:
$$7^3$$

$$7^3$$
It can be written
$$= 7 \times 7\times 7$$
$$= 343$$

Find the cube of :
$$11$$

$$(11)^3 = 11 \times 11 \times 11 = 1331 $$

Find the cubes of:
$$-3$$

$$ -3 = (-3)^3 = -3 \times -3 \times -3 $$
$$ = - (3 \times 3 \times 3) = -27 $$

Find the cubes of:
$$-25$$

$$ -25 = (-25)^3 = -25 \times -25 \times -25 $$
$$ = - (25 \times 25 \times 25) = - 15625 $$

Find the cubes of :
$$0.12$$

$$ 0.12 = (0.12)^3 = \left ( \dfrac{12}{100} \right )^3 $$
$$ = \dfrac{12 \times 12 \times 12}{100 \times 100 \times 100} $$

$$ = \dfrac{1728}{1000000} = 0.001728 $$

Find the cubes of:
$$-50$$

$$ -50 = (-50)^3 = -50 \times -50 \times -50 $$

$$ = -(50 \times 50 \times 50) = -125000 $$

Find the cubes of:
$$-7$$

$$ -7 = (-7)^3 = -7 \times -7 \times -7 $$
$$ = -(7 \times 7 \times 7) = -343 $$

Find the cube of :
$$0.02$$

Cube of $$ 0.02 = (0.02)^3 = \left ( \dfrac{2}{100} \right )^3 $$
$$ = \dfrac{2 \times 2 \times 2}{100 \times 100 \times 100} $$

$$ = \dfrac{8}{1000000}$$

$$ = 0.000008 $$

Find the cube of $$-18$$

$$ (-18)^3 = -18 \times -18 \times -18 $$

$$ = - (18 \times 18 \times 18) $$

$$= - 5832 $$

Find the cube of :
$$0.8$$

$$ 0.8 = (0.8)^3 = \left ( \dfrac{8}{10} \right )^3 = \dfrac{8 \times 8 \times 8}{10 \times 10 \times 10} $$

$$ = \dfrac{512}{1000} = 0.512 $$

Find the cubic root of the following number by prime factorisation method:
$$15625$$

On prime factorising, we get,

$$15625 = 5\times5\times5\times5\times5\times5$$

$$= 5^3\times5^3=25^3$$.

Then, cube root of $$15625$$ is:

$$\sqrt[3]{15625}=\sqrt[3]{25^3}= 25$$.

Therefore, $$25$$ is the required solution.

Find the cubic root of the following number by prime factorisation method:
$$10648$$

On prime factorising, we get,

$$10648 = 2\times2\times2\times11\times11\times11$$

$$= 2^3\times11^3$$.

Then, cube root of $$10648$$ is:

$$\sqrt[3]{10648}=\sqrt[3]{2^3 \times 11^3}= 2\times11=22$$.

Therefore, $$22$$ is the required solution.

Find the smallest number by which a given number must be divided to obtain a perfect cube:
$$192$$

Prime factorising $$192$$, we get,

$$192 = 2\times2\times2\times2\times2\times2\times3$$

$$= 2^6 \times 3^1$$.

We know, a perfect cube has multiples of $$3$$ as powers of prime factors.

Here, number of $$2$$'s is $$6$$ and number of $$3$$'s is $$1$$.

So we need to divide $$3$$ from the factorization to make $$192$$ a perfect cube.

Hence, the smallest number by which $$192$$ must be divided to obtain a perfect cube is $$3$$.

Find the cube root of $$46656$$ by prime factorisation method.

On prime factorising, we get,

$$46656=2\times 2\times2\times2\times2\times2\times3\times3\times3\times3\times3\times3$$

$$= 2^3\times2^3\times3^3\times3^3$$.

Then, cube root of $$46656$$ is:

$$\sqrt[3]{46656}=\sqrt[3]{2^3 \times 2^3 \times 3^3 \times 3^3}= 2\times2\times3\times3=36$$.

Therefore, $$36$$ is the required solution.

Find the smallest number by which a given number must be divided to obtain a perfect cube:
$$704$$

Prime factorising $$704$$, we get,

$$704 = 2\times2\times2\times2\times2\times2\times11$$

$$= 2^6 \times 11 ^1 $$.

We know, a perfect cube has multiples of $$3$$ as powers of prime factors.

Here, number of $$2$$'s is $$6$$ and number of $$11$$'s is $$1$$.

So we need to divide $$11$$ from the factorization to make $$704$$ a perfect cube.

Hence, the smallest number by which $$704$$ must be divided to obtain a perfect cube is $$11$$.

Find the smallest number by which a given number must be divided to obtain a perfect cube:
$$135$$

Prime factorising $$135$$, we get,

$$135 = 5\times3\times3\times3$$ $$ = 3^3 \times 5^1$$.

We know, a perfect cube has multiples of $$3$$ as powers of prime factors.

Here, number of $$3$$'s is $$3$$ and number of $$5$$'s is $$1$$.

So we need to divide the factorization by $$5$$ to make $$135$$ a perfect cube.

Hence, the smallest number by which $$135$$ must be divided to obtain a perfect cube is $$5$$.

You are told that $$1,331$$ is a perfect cube. Can you guess without factorisation what is its cube root?

The given number is $$1331$$.

Step $$I$$: Form groups of three starting from the rightmost digit of $$1331$$. In this case one group i.e., $$331$$, has three digits whereas other group has only one digit i.e, $$1$$.

Step $$II$$: Take $$331$$, the digit $$1$$ is at its one's place. We know that $$1$$ comes at the unit's place of a number only when its cube root ends in $$1$$. So, we take the one's place of the required cube root as $$1$$.

Step $$III$$: Take the other group, i.e., $$1$$. $$ 1^3 = 1$$, and $$2^3 = 8$$, so, $$1$$ lies between $$1$$ and $$8$$. The smaller number among $$1$$ and $$2$$ is $$1$$. The one's place of $$1$$ is $$1$$ itself. Take $$1$$ as ten's place of the cube root of $$1331$$.

Hence, $$\sqrt[3]{1331} = 11$$.

Find the cubic root of the following number by prime factorisation method:
$$27000$$

On prime factorising, we get,

$$27000 = 2\times2\times2\times5\times5\times5\times3\times3\times3$$

$$= 2^3\times5^3\times3^3$$.

Then, cube root of $$27000$$ is:

$$\sqrt[3]{27000}=\sqrt[3]{3^3 \times 5^3 \times 2^3}= 3\times5\times2=30$$.

Therefore, $$30$$ is the required solution.

Find the smallest number by which the given number must be divided to obtain a perfect cube:
$$81$$

The given number is $$81$$

Prime factorizing $$81$$, we get,

$$ 81= 3 \times 3\times 3\times 3 =3^4$$.

We know, a perfect cube has multiples of $$3$$ as powers of prime factors.

Here, number of $$3$$'s is $$4$$.

So we need to divide the factorization by $$3$$ to make $$81$$ a perfect cube.

Hence, the smallest number by which $$81$$ must be divided to obtain a perfect cube is $$3$$.

What is the smallest number by which $$243$$ should be multiplied to get a perfect cube?

$$ 243 = \underline { 3\times 3\times 3 }

\times \underline { 3\times 3 } $$
The prime factor $$ 3 $$ does not appear in a group of three the second time.

So, $$ 243 $$ is not a perfect cube.

To make it perfect cube we need one more $$ 3 $$.
Hence, the smallest number by which $$ 243 $$ must be multiplied so that the product is a perfect cube is $$ 3 $$.

Simplify: $$\displaystyle \frac{\sqrt[3]{512}-\sqrt[3]{216}}{\sqrt[3]{125}-\sqrt[3]{64}}$$.

On prime factorisation of the numbers individually, we get,

$$512=\underline { 2\times2\times2 } \times \underline { 2\times2\times2 } \times \underline { 2\times 2\times2 }$$

$$\Rightarrow \sqrt [ 3 ]{ 512 } =2\times2\times2=8$$.

$$ 216=\underline { 2 \times 2 \times 2 } \times \underline { 3 \times 3 \times 3 }$$

$$ \Rightarrow \sqrt [ 3 ]{ 216 } =2\times3=6 $$.

$$125=\underline { 5 \times 5 \times 5 }$$

$$ \Rightarrow \sqrt [ 3 ]{ 125 } =5$$.

$$64=\underline { 2 \times 2 \times 2 } \times \underline { 2 \times 2 \times 2 }$$

$$ \Rightarrow \sqrt [ 3 ]{ 64 } =2 \times 2=4$$.

Therefore,

$$ \displaystyle\frac { \sqrt [ 3 ]{ 512 } -\sqrt [ 3 ]{ 216 } }{ \sqrt [ 3 ]{ 125 } -\sqrt [ 3 ]{ 64 } } =\displaystyle\frac { 8-6 }{ 5-4 } =\displaystyle\frac { 2 }{ 1 } =2$$.

Thus, $$2$$ is the solution.

Find the cube root of $$5832$$ by prime factorisation method.

On prime factorising, we get,

$$5832=(2 \times 2\times 2)\times (3\times3\times3)\times(3\times3\times3)$$

$$= 2^3 \times 3^3 \times 3^3$$.

Then, cube root of $$5832$$ is:

$$\sqrt[3]{5832}=\sqrt[3]{2^3 \times 3^3 \times 3^3}= 2\times 3\times 3 = 18$$.

Therefore, $$18$$ is the solution.

What is the smallest number by which $$18522$$ must be divided so that the quotient is a perfect cube?

On Prime factorization of $$18522$$, we get,

$$18522 = 2 \times 3 \times 3 \times 3 \times 7 \times 7 \times 7$$

$$= 2^1 \times 3^3 \times 7 ^3 $$.

We know, a perfect cube should have each prime factor in power of $$3$$.

Here, number of $$2$$'s is in power of $$1$$, number of $$3$$'s is in power of $$3$$ and number of $$7$$'s is in power of $$3$$.

So, we need to remove $$2$$ from the factors to make $$18522$$, a perfect cube.

Hence, the smallest number by which $$18522$$ must be divided to obtain a perfect cube is $$2$$.

What is the smallest number by which 675 must be multiplied so that the product is a perfect cube?

Grouping the factor in triplets of equal factors we get,

$$675 = (3 \times 3 \times 3) \times 5 \times 5$$

We find that $$3$$ occurs as a prime factor of $$675$$ thrice but $$5$$ occurs as a prime factor only twice.

Thus, if we multiply $$675$$ by $$5$$, 5 will also occur as a prime factor thrice and the product will be $$3 \times 3 \times 3 \times 5 \times 5 \times 5$$ which is a perfect cube.

Hence, we must multiply $$675$$ by $$5$$ so that the product becomes a perfect cube.

What is the smallest number by which $$675$$ must be multiplied so that the product is a perfect cube?

Prime factorising $$675$$, we get,

$$675=5\times 5\times 3\times 3\times 3$$ $$ = 3^{3}\times5^2$$.

We know, a perfect cube has multiples of $$3$$ as powers of prime factors.

Here, number of $$3$$'s is $$3$$ and number of $$5$$'s is $$2$$.

So we need to multiply another $$5$$ in the factorization to make $$675$$ a perfect cube.

Hence, the smallest number by which $$675$$ must be multiplied to obtain a perfect cube is $$5$$.

Find the smallest number by which $$68600$$ must be multiplied to get a perfect cube.

Prime factorising $$68600$$, we get,

$$68600=2\times 2\times 2\times 5\times 5\times 7\times 7\times 7$$

$$= 2 ^3 \times 5 ^2 \times 7^3$$.

We know, a perfect cube has multiples of $$3$$ as powers of prime factors.

Here, number of $$2$$'s is $$3$$, number of $$5$$'s is $$2$$ and number of $$7$$'s is $$3$$.

So we need to multiply another $$5$$ in the factorization to make $$68600$$ a perfect cube.

Hence, the smallest number by which $$68600$$ must be multiplied to obtain a perfect cube is $$5$$.

Evaluate
(i) $$\displaystyle (1005)^{3}$$ (ii) $$\displaystyle (997)^{3}$$

(i) $$\displaystyle (1005)^{3}$$ = $$\displaystyle (1000+5)^{3}$$
Using, $$(a+b)^3=a^3+b^3+3ab(a+b)$$
$$= \displaystyle (1000)^{3}+(5)^{3} + 3 (1000) (5) (1000 + 5)$$
$$= 1000000000 + 125 + 15000 (1000 + 5)$$
$$= 1000000000 + 125 + 15000000 + 75000$$
$$= 1015075125$$
(ii) $$\displaystyle (997)^{3}=(1000-3)^{3}$$
Using, $$(a-b)^3=a^3-b^3-3ab(a-b)$$
$$= \displaystyle (1000)^{3}-(3)^{3}-3\times 1000\times 3\times (1000-3)$$
$$= 1000000000 - 27 - 9000 x (1000 - 3)$$
$$= 1000000000 - 27 - 9000000 + 27000$$
$$= 991026973$$

You are told that 1,331 is a perfect cube. Can you guess without factorisation what is its cube root?Similarly, guess the cube roots of 4913, 12167, 32768

1. 1331

Let us divide $$1331$$ into groups of three digits starting from the right. So $$1331$$ has two groups, one is $$331$$ and another is $$1.$$

For first group $$331,$$ digit $$1$$ is at one's place. $$1$$ comes at the unit's place of a number only when its cube root ends with $$1.$$ So unit's place of the required cube root is $$1.$$
For another group, i.e. $$1,\, 1^3=1$$ and $$2^3=8.$$ So $$1$$ lies between $$0$$ and $$8.$$ The smaller number among $$1$$ and $$2$$ is $$1.$$ So the one's place of $$1$$ is $$1$$ and ten's place of cube root $$1331$$ is $$1$$
Hence $$\sqrt[3]{1331}=11$$

2. $$4913$$

Let us divide $$4913$$ into groups of three-digit starting from the right. So $$4913$$ has two groups one is $$913$$ and another is $$4.$$

For first group $$913,$$ the digit $$3$$ is at unit's place. $$3$$ comes at the unit's place of a number only when its cube root ends in $$7.$$ So unit's place of the required cube root is $$7.$$
For another group, i.e. $$4,$$ we know that $$1^3=1$$ and $$2^3=8.$$ We know that $$4$$ lies between $$1$$ and $$8.$$ The smaller number among $$1$$ and $$2$$ is $$1.$$ So the one's place of $$1$$ is $$1$$$ and ten's place of cube root $$4913$$ is $$1$$
Hence $$\sqrt[3]{4913}=17$$

3. $$12167$$

Let us divide $$12167$$ into groups of three-digit starting from the right. So $$12167$$ has two groups one is $$167$$ and another is $$12.$$

For first group $$167,$$ the digit $$7$$ is at unit's place. $$7$$ comes at a unit place of a number only when its cube root ends in $$3.$$ So unit's place of the required cube root is $$3.$$
For another group, i.e. $$12,$$ we know that $$2^3=8$$ and $$3^3=27.$$ We find that $$12$$ lies between $$8$$ and $$27.$$ The smaller number among $$2$$ and $$3$$ is $$2.$$ So the one's place of $$2$$ is $$2$$ itself and ten's place of cube root $$12167$$ is $$2$$
Hence $$\sqrt[3]{12167}=23$$

4. $$32768$$

Let us divide $$32768$$ into groups of three-digit starting from the right. So $$32768$$ has two groups one is $$768$$ and another is $$32.$$

For first group $$768,$$ the digit $$8$$ is at unit's place. $$8$$ comes at the unit place of a number only when its cube root ends in $$2.$$ So unit's place of the required cube root is $$2.$$
For another group, i.e. $$32,\, 3^3=27$$ and $$4^3=64.$$ So $$32$$ lies between $$27$$ and $$64.$$ The smaller number among $$3$$ and $$4$$ is $$4.$$ So the ten's place of cube root $$32768$$ is $$3$$
Hence $$\sqrt[3]{32768}=32$$

Find the smallest number by which $$72$$ must be multiplied to obtain a perfect cube.

$$ \displaystyle 72=2\times 2\times2\times 3\times 3 $$
Number of $$2$$s is $$3$$ and that of $$3$$s is $$2$$

So, $$72$$ needs to be multiplied by $$3$$ to become a perfect cube.

Find the smallest number by which $$256$$ must be multiplied to obtain a perfect cube.

By prime factorization, $$256$$ can be written as $$2^3 \times 2^3 \times 2^2$$ by making $$2$$ into groups of $$3.$$

In the third group, one $$2$$ is missing.

$$256 = 2^3 \times 2^3 \times 2^2 $$

So, $$512 = 2^3 \times 2^3 \times 2^2 \times 2$$

Hence, number $$2$$ must be smallest number that must be multiplied to make it a perfect cube.

Find the smallest number which should be multiplied to $$2916$$ to get a perfect cube.

Prime factorising $$2916$$, we get,

$$ 2916 = 2 \times 2 \times 3 \times 3 \times 3 \times 3 \times 3 \times 3$$

$$= 2^2 \times 3^6$$.

We know, a perfect cube has multiples of $$3$$ as powers of prime factors.

Here, number of $$2$$'s is $$2$$ and number of $$3$$'s is $$6$$.

So we need to multiply another $$2$$ in the factorization to make $$2916$$ a perfect cube.

Hence, the smallest number by which $$2916$$ must be multiplied to obtain a perfect cube is $$2$$.

Find the smallest number which should be multiplied to $$36125$$ to get a perfect cube.

Prime factorising $$36125$$, we get,

$$ 36125= 5 \times 5 \times 5 \times 17 \times 17$$

$$= 5 ^3 \times 17 ^2$$.

We know, a perfect cube has multiples of $$3$$ as powers of prime factors.

Here, number of $$5$$'s is $$3$$ and number of $$17$$'s is $$2$$.

So we need to multiply another $$17$$ to the factorization to make $$36125$$ a perfect cube.

Hence, the smallest number by which $$36125$$ must be multiplied to obtain a perfect cube is $$17$$.

Find the smallest number which should be multiplied to $$432$$ to get a perfect cube.

Prime factorising $$432$$, we get,

$$ 432 = 2 \times 2 \times 2 \times 2 \times 3 \times 3 \times 3$$

$$= 2 ^4 \times 3 ^3 $$.

We know, a perfect cube has multiples of $$3$$ as powers of prime factors.

Here, number of $$2$$'s is $$4$$ and number of $$3$$'s is $$3$$.

So we need to multiply another $$2^2$$ in the factorization to make $$432$$ a perfect cube.

Hence, the smallest number by which $$432$$ must be multiplied to obtain a perfect cube is $$2^2=4$$.

Find the smallest number which should be multiplied to $$100$$ to get a perfect cube.

Prime factorising $$100$$, we get,

$$ 100 = 2 \times 2 \times 5 \times 5$$

$$= 2 ^2 \times 5 ^2 $$.

We know, a perfect cube has multiples of $$3$$ as powers of prime factors.

Here, number of $$2$$'s is $$2$$ and number of $$5$$'s is $$2$$.

So we need to multiply another $$2$$ and $$5$$ in the factorization to make $$100$$ a perfect cube.

Hence, the smallest number by which $$100$$ must be multiplied to obtain a perfect cube is $$2\times 5 = 10$$.

Find the smallest number which should be multiplied to $$10976$$ to get a perfect cube.

Prime factorising $$10976$$, we get,

$$10976 = 2 \times 2 \times 2 \times 2 \times 2 \times 7 \times 7 \times 7$$

$$= 2 ^5 \times 7^3$$.

We know, a perfect cube has multiples of $$3$$ as powers of prime factors.

Here, number of $$2$$'s is $$5$$ and number of $$7$$'s is $$3$$.

So we need to multiply another $$2$$ to the factorization to make $$10976$$ a perfect cube.

Hence, the smallest number by which $$10976$$ must be multiplied to obtain a perfect cube is $$2$$.

Find the smallest number which should be multiplied to $$1323$$ to get a perfect cube.

We can see that;

$$1323=\underline{3\times 3\times 3}\times \underline{7\times 7}$$

Therefore $$7$$ should be multiplied to $$1323$$ to give a perfect cube.

$$\Rightarrow 1323\times 7=9261\ is\ a\ perfect\ cube$$

Find the smallest number which should be multiplied to $$10584$$ to get a perfect cube.

Prime factorising $$10584$$, we get,

$$10584 = 2 \times 2 \times 2 \times 3 \times 3 \times 3 \times 7 \times7$$

$$= 2 ^3 \times 3 ^3 \times 7^2$$.

We know, a perfect cube has multiples of $$3$$ as powers of prime factors.

Here, number of $$2$$'s is $$3$$, number of $$3$$'s is $$3$$ and number of $$7$$'s is $$2$$.

So we need to multiply another $$7$$ to the factorization to make $$10584$$ a perfect cube.

Hence, the smallest number by which $$10584$$ must be multiplied to obtain a perfect cube is $$7$$.

Find the smallest number which should be multiplied to $$15625$$ to get a perfect cube.

Prime factorising $$15625$$, we get,

$$ 15625 = 5 \times 5 \times 5 \times 5 \times 5 \times 5$$ $$= 5^6$$.

We know, a perfect cube has multiples of $$3$$ as powers of prime factors.

Here, number of $$5$$'s is $$6$$.

That is, the given number is a perfect cube.

So we need to multiply only $$1$$ in the factorization to make $$15625$$ a perfect cube.

Hence, the smallest number by which $$15625$$ must be multiplied to obtain a perfect cube is $$1$$.

Evaluate :
(i) (8)$$ \displaystyle ^{3}$$
(ii) (15)$$ \displaystyle ^{3}$$
(iii) (21)$$ \displaystyle ^{3}$$
(iv) (60)$$ \displaystyle ^{3}$$

(i)$$8\times 8\times 8=512$$

(ii)$$15\times 15\times 15=3375$$

(iii)$$21\times 21\times 21=9261$$

(iv)$$60\times 60\times 60=216000$$

Evaluate the cube root of :
$$\displaystyle \sqrt [ 3 ]{ \left ( \dfrac{-64}{343} \right )} $$

1540028_425520_ans_82e1bfd0f9ab4525baa696a80a03f91b.jpg

Find cube root of $$1331$$

$$\textbf{Step 1: Find the cube root.}$$

$$\text{To find the cube root of 1331.}$$

$$\text{Prime factorising 1331, we get,}$$

$$\mathrm{1331=11\times11\times11}$$

$$\mathrm{\therefore\sqrt[3]{1331}=\sqrt[3]{11\times11\times11}}$$

$$\mathrm{=11}$$

$$\textbf{Hence, the required cube root is 11.}$$

Find the smallest number by which $$2808$$ must be multiplied so that the product is a perfect cube.

By prime factorising $$2808$$, we get,

$$ 2808= 2 \times 2 \times 2 \times 3 \times 3 \times 3 \times 13$$

$$= 2 ^3 \times 3 ^3 \times 13^1$$.

We know, a perfect cube has multiples of $$3$$ as powers of prime factors.

Here, number of $$2$$'s is $$3$$, number of $$3$$'s is $$3$$ and number of $$13$$'s is $$1$$.

So we need to multiply another $$13^2$$ to the factorization to make $$2808$$ a perfect cube.

Hence, the smallest number by which $$2808$$ must be multiplied to obtain a perfect cube is $$13^2=169$$.

Find the smallest number by which $$33275$$ must be multiplied so that the product is a perfect cube.

On prime factorization of $$33275$$, we get,

$$ 33275 = 5 \times 5 \times 11 \times 11 \times 11$$

$$= 5 ^2 \times 11 ^3$$

A perfect cube has factors with exponents $$3.$$

Here, number of $$5$$'s is $$2$$ and number of $$11$$'s is $$3$$.

So, we need to multiply another $$5$$ to the number $$33275$$ to make it a perfect cube.

Hence, the smallest number by which $$33275$$ must be multiplied to obtain a perfect cube is $$5.$$

What is the smallest number by which $$392$$ must be multiplied so that the product is a perfect cube?

Prime factorising $$392$$, we get,

$$\displaystyle 392=2\times 2\times 2\times 7\times 7$$

$$= 2 ^3 \times 7 ^2$$.

We know, a perfect cube has multiples of $$3$$ as powers of prime factors.

Here, number of $$2$$'s is $$3$$ and number of $$7$$'s is $$2$$.

So we need to multiply another $$7$$ to the factorization to make $$392$$ a perfect cube.

Hence, the smallest number by which $$392$$ must be multiplied to obtain a perfect cube is $$7$$.

Is the following number a perfect cube or not? If it is perfect cube then answer $$1$$, otherwise answer $$0$$.
$$106480$$

Prime factorising, we get,

$$\displaystyle 106480=2\times 2\times 2\times 2\times 5\times 11\times 11\times 11$$

$$\displaystyle =\left( { 2 }^{ 3 }\times 2\times 5\times { 11 }^{ 3 } \right) ={ \left( 2\times 11 \right) }^{ 3 }\times 2\times 5$$

We know, a perfect cube has multiples of $$3$$ as powers of prime factors.

In the above factorisation, $$2$$ and $$5$$ does not have their powers as multiple of $$3$$.

Therefore, $$106480$$ is not a perfect cube.

Hence, $$0$$ is the answer.

Find the cube root of $$8000$$.

On prime factorising, we get,

$$8000=\underline{2\times 2\times 2}\times \underline{2\times2\times2} \times \underline{5\times5\times5}$$ $$=2^3\times 2^3 \times 5^3=20^3$$.

Therefore, cube root of $${8000}$$ is:

$$\sqrt[3]{8000}=\sqrt[3]{(20)^3}= 20$$.

Therefore, $$20$$ is the required solution.

What is the smallest number by which $$8640$$ must be divided so that the quotient is a perfect cube?

$$\displaystyle 8640=2\times 2\times 2\times 2\times 2\times 2\times 3\times 3\times 3\times 5$$
$$5$$ occurs as a prime number only once.
Hence, $$5$$ is the smallest number by which $$8640$$ must be divided, so that the quotient is a perfect cube.

Divide the number $$26244$$ by the smallest number so that the quotient is a perfect cube.

$$26244 = 3\times 3\times 3\times 3\times 3\times 3\times 3\times 3 \times 2\times 2$$

$$\displaystyle 2\times 2\times 3\times 3=36$$ is the smallest number by which $$26244$$ must be divided so that the quotient is a perfect cube.

Find the cube root of $$2744$$.

By prime factorisation, we get,

$$\displaystyle 2744=2\times 2\times 2\times 7\times 7\times 7=2^3\times7^3=14^3$$.

Then, cube root of $$2744$$ is:
$$\displaystyle \sqrt [ 3 ]{ 2744 } =\sqrt [ 3 ]{ 14^3 } $$ $$=14$$.

Hence, $$14$$ is the required solution.

Write cubes of all natural numbers between 1 and 20 and verify the following statement. If the statement is true then answer is $$1$$ if not then the answer is $$0$$

Statement: Cubes of all even natural numbers are even.

$$1 ^3 = 1 $$

$$2^3=8 $$

$$3^3=27$$

$$4^3=64$$

$$5^3=125 $$ so on.

Assume a general even number to be of the form $$2n $$ where $$n $$ is a whole number.

This is true for all even numbers, by definition are divisible by 2.

Cube of a general even number= $$2n \times 2n \times 2n =8n $$

This number $$8n$$ is again divisible by 2

So cube of an even natural number is even.

Find the cube root of $$512$$

$$\sqrt [3]{512} = (512)^{\dfrac {1}{3}}$$
$$= ((2\times 2\times 2)\times (2\times 2\times 2)\times (2\times 2\times 2)^{\dfrac {1}{3}}$$
$$= (2^{3}\times 2^{3} \times 2^{3})^{\dfrac {1}{3}}$$
$$= (2^{9})^{\dfrac {1}{3}} = 2^{3}$$
$$\sqrt [3]{512} = 8$$.

Write cubes of $$5$$ natural numbers which are of the form $$\displaystyle 3n+1$$ (e.g. 4,7,10,....) and verify the following:
'The cube of a natural number of the form $$\displaystyle 3n+1$$ is a natural number of the same form'.

The 5 natural number which are of the form $$\displaystyle 3n+1$$ (e.g 4,7,10,....) are as follows:
$$\displaystyle 3\times 1+1=3+1=4$$
$$\displaystyle 3\times 2+1=6+1=7$$
$$\displaystyle 3\times 3+1=9+1=10$$
$$\displaystyle 3\times 4+1=12+1=13$$
$$\displaystyle 3\times 5+1=15+1=16$$
The cubes of 5 natural numbers which are of the form $$\displaystyle 3n+1$$ (e.g. 4,7,10,....) are as follows:
$$\displaystyle { \left( 4 \right) }^{ 3 }=4\times 4\times 4=64$$
$$\displaystyle { \left( 7 \right) }^{ 3 }=7\times 7\times 7=343$$
$$\displaystyle { \left( 10 \right) }^{ 3 }=10\times 10\times 10=1000$$
$$\displaystyle { \left( 13 \right) }^{ 3 }=13\times 13\times 13=2197$$
$$\displaystyle { \left( 16 \right) }^{ 3 }=16\times 16\times 16=4096$$
Verification:
$$\displaystyle 64=3\times 21+1$$
$$\displaystyle 343=3\times 114+1$$
$$\displaystyle 1000=3\times 333+1$$
$$\displaystyle 2197=3\times 732+1$$
$$\displaystyle 4096=3\times 1365+1$$
$$\displaystyle \therefore $$ 'The cube of a natural number of the form $$\displaystyle 3n+1$$ is a natural number of the same form'

Find the cube root of 125.

On prime factorising, we get,

$$125 = 5\times 5\times 5= 5^3$$

Then, cube root of $$125$$ is:

$$\sqrt[3]{125}=\sqrt[3]{5^3 }=5$$

Therefore, $$5$$ is the required solution.

Find cube root of the following numbers by prime factorisation method.
(i) $$64$$
(ii) $$512$$
(iii) $$10648$$
(iv) $$27000$$
(v) $$15625$$
(vi) $$13824$$
(vii) $$110592$$
(viii) $$46656$$
(ix) $$175616$$
(x) $$91125$$

(i) $$64 = 2\times 2\times 2\times 2\times 2\times 2 = $$ $$ 2^{3}\times 2^{3} = 4^{3} $$
$$ \sqrt[3]{64} = 4 $$

(ii) $$512 = 2\times 2\times 2\times 2\times 2\times 2\times 2\times 2\times 2\times 2\times 2\times 2 $$
$$=$$ $$ 2^{3}\times 2^{3}\times 2^{3} = 8^{3} $$
$$ \sqrt[3]{512} = 8 $$

(iii) $$10648 =$$ $$ 2^{3}\times 11^{3} = 22^{3} $$
$$ \sqrt[3]{10648} = 22 $$

(iv) $$27000 =$$ $$ 2^{3}\times 3^{3}\times 5^{3} $$$$= 30^{3} $$
$$ \sqrt[3]{27000} = 30 $$

(v) $$15625 =$$ $$ 5^{3}\times 5^{3} = 25^{3} $$
$$ \sqrt[3]{15625} =25 $$

(vi) $$13824 =$$ $$ 2^{3}\times 2^{3}\times 2^{3}\times 3^{3} = 24^{3} $$
$$ \sqrt[3]{13824} = 24 $$

(vii) $$110592 =$$ $$ 2^{3}\times 2^{3}\times 2^{3}\times 2^{3}\times 3^{3} $$$$ = 48^{3} $$
$$ \sqrt[3]{110592} = 48 $$

(viii) $$46656 =$$ $$ 2^{3}\times 2^{3}\times 3^{3}\times 3^{3} $$ $$ =36^{3} $$
$$ \sqrt[3]{46656} = 36 $$

(ix) $$175616 =$$ $$ 2^{3}\times 2^{3}\times 2^{3}\times 7^{3} $$$$= 56^{3} $$
$$ \sqrt[3]{175616} = 56 $$

(x) $$91125 =$$ $$5^{3}\times 3^{3}\times 3^{3}$$ $$= 45^{3} $$
$$ \sqrt[3]{91125} = 45 $$

You are told that $$1331$$ is a perfect cube. Can you guess without factorisation what is its cube root? Similarly, guess the cube root of $$4913, 12167, 32768$$.

The given number is $$1331$$.

Let us form groups of three digits starting from rightmost digit.

Therefore, the two groups are $$\underline {1}$$ and $$\underline {331}$$.

Consider the group : $$331$$

We take the unit's place of required cube root as $$1$$

Consider the group : $$1$$

The unit's place of 1 is 1 itself. So, we take $$1$$ as ten's place of cube root of $$1331$$ as $$1$$.

Thus, $$\sqrt [3] {1331} = 11$$.

Similarly,

$$4913 \Rightarrow \underline 4$$ and $$\underline {913}$$

$$\therefore \sqrt [3]{4913} = 17$$

$$12167 \Rightarrow \underline {12}$$ and $$\underline {167}$$

$$\therefore \sqrt [3]{12167} = 23$$

$$32768 \Rightarrow \underline {32}$$ and $$\underline {768}$$

$$\therefore \sqrt [3]{32768} = 32$$

Which of the following are not perfect cubes?
(i) 216
(ii) 128
(iii) 1000
(iv) 100
(v) 46656

(i) $$216 = 2\times 2\times2 \times 3\times 3\times 3 =$$ $$ 2^{3}\times 3^{3} = 6^3$$
So, $$216$$ is a perfect cube.

(ii) $$128 =2\times 2\times 2\times 2\times 2\times 2\times 2$$
$$=$$ $$ 2^{3} \times 2^{3} \times 2 = 6^3\times2$$
So, $$128$$ is not a perfect cube.

(iii) $$1000 = 2\times 2\times 2\times 5\times 5 \times 5 = 10^3$$
So, $$1000$$ is a perfect cube.

(iv) $$100 = 2\times 2\times 5\times 5 = 10^2$$
So, $$100$$ is not a perfect cube

(v) $$46656 = 2\times 2\times 2\times 2\times 2\times 2\times 3\times 3\times 3\times 3\times 3\times 3 = 36^3$$
So, $$46656$$ is a perfect cube.

How many perfect cubes are there from $$1$$ to $$500$$? How many are perfect squares among these cubes?

Let us find the perfect cubes between $$1$$ to $$500$$.

We know that, $$1^3=1, 2^3=8, 3^3=27, 4^3=64, 5^3=125, 6^3=216, 7^3=343$$ and $$8^3=512$$

This implies, taking number $$\geq 8$$ gives us cubes $$\geq 500$$. So, the rest numbers i.e. $$8,9,10,....$$ have their cubes greater than $$500$$.

So, only these $$7$$ numbers have their cubes between $$1$$ to $$500$$.

Thus, there are $$7$$ perfect cubes from $$1$$ to $$500$$.

Of these $$64$$ is the only perfect square $$\because 64 = 4^3 = 8^2$$.

Find the smallest square no. which is divisible by each of the numbers $$8,15 $$ and $$20$$.

$$8= 2 \times 2 \times 2$$
$$15 = 3 \times 5$$
$$20 = 2 \times 2 \times 5$$
$$L.C.M = 2 \times 2 \times 2 \times 3 \times 5 = 120$$
Required no. $$= 120 \times 3 \times 5 = 1800$$

If $$18^{3}=5832$$ then $$3\sqrt{0.005832}=?$$

$$3\sqrt{\dfrac{01005832}{1000000}}$$

$$= 3\sqrt{\dfrac{18\times 18\times 18}{100 \times 100\times 100}}$$

$$=\dfrac{18}{100} = .18$$

Find the smallest no. by which each of the following no. must be divided to obtain a perfect cube.
(i) $$81$$
(ii) $$128$$
(iii) $$135$$
(iv) $$192$$
(v) $$704$$

(i) $$81 = 3\times 3\times 3\times 3 =$$ $$ 3^{3} \times 3 $$
Rquired no. $$= 3$$

(ii) $$128 =$$ $$ 2^{3} \times 2^{3} \times 2 $$
Required no. $$=2$$

(iii) $$135 =$$ $$ 3^{3} \times 5 $$
Required no. $$= 5$$

(iv) $$192 =$$ $$ 2^{3} \times 2^{3} \times 3 $$
Required no.$$ = 3$$

(v) $$704 =$$ $$ 2^{3} \times 2^{3} \times 11 $$
Required no. $$= 11$$

Find the cubes of the first five odd natural numbers and the cube of the first five even natural numbers. What can we say about the parity of the odd cubes and even cubes?

Odd cubes
$$1^3 = 1$$
$$3^3 = 9$$
$$5^3 = 125$$
$$7^3 = 343 $$
$$9 ^3 = 729$$
Even cubes
$$2^3 = 8$$
$$4^3 = 64$$
$$6^3 = 216$$
$$8^3 = 512$$
$$10^3 = 1000$$
Parity: Cubes of odd numbers is always odd.
Cubes of even numbers is always even.

Write an equivalent exponential form for radical expression.
$$\sqrt [ 3 ]{ 13 } $$

Given, $$\sqrt [ 3 ]{ 13 } $$
Exponential form of given radial expression is $${13}^{1/3}$$.

What is a smallest number by which $$2560$$ is to be multiplied so that the product is a perfect cube?

The given number ends with $$0$$ therefore it is divisible by $$2$$ and $$5$$.

Thus,

$$2560=\underline {2\times 2\times 2} \times \underline {2\times 2\times 2} \times \underline {2\times 2\times 2} \times 5$$

From the factors, we can say that only $$5$$ is a factor that is not occurring three times or in the multiple of it to make it a perfect cube.

So to make it a perfect cube, the above number should be multiplied with $$5\times5=25.$$

So, $$25$$ is the required answer.

If a cube has side-length $$10$$ cm, what is its volume?

Let $$s$$ cm be the side of a cube

Then we have $$V = s^3 = 10 \times 10 \times 10 = 1000 cm^3$$,

What is the smallest number by which $$1600$$ is to be divided, so that the quotient is a perfect cube?

Prime factorising $$1600$$, we get,

$$1600 = 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 5 \times 5$$

$$= 2^6 \times 5 ^2 $$.

We know, a perfect cube has multiples of $$3$$ as powers of prime factors.

Here, number of $$2$$'s is $$6$$ and number of $$5$$'s is $$2$$.

So we need to divide with $$5^2$$ from the factorization to make $$1600$$ a perfect cube.

Hence, the smallest number by which $$1600$$ must be divided to obtain a perfect cube is $$5^2=25$$.

Show that $$6$$ is not a perfect cube.

We observe that $$1 < 6 < 8$$ so that $$1^3 < 6 < 2^3$$. Since no integer exists between $$1$$ and $$2$$, $$6$$ cannot be written as a product of three equal integers. Hence $$6$$ is not a perfect cube.

Find the cube root of $$103823$$.

Here the units digit of $$103823$$ is $$3$$. If $$n^3 = 103823$$, then the units digit of $$n$$ must be $$7$$.
Let us split $$103823$$ as $$103$$ and $$823$$. We observe that $$4^3 = 64 < 103 < 125 = 5^3$$. Hence
$$40^3 = 64000 < 103823 < 125000 = 50^3$$.
Hence $$n$$ must lie between $$40$$ and $$50$$. Since the units digit of $$n$$ is $$7$$, the only such number is $$47$$.

What are the digits in the unit's place of the cubes of $$1, 2, 3, 4, 5, 6, 7, 8, 9, 10$$? Is it possible to say that a number is not a perfect cube by looking at the digit in unit's place of the given number, just like we did for squares?

To determine the digits at unit's place of the cubes of given numbers, first we need to find their cubes.

$$1^3=1,$$ $$2^3=8,$$ $$3^3=27,$$ $$4^3=64,$$ $$5^3=125,$$ $$6^3=216,$$ $$7^3=343,$$ $$8^3=512,$$ $$9^3=729,$$ $$10^3=1000$$

The digits at unit's place are $$1,8,7,4,5,6,3,2,9,0$$

Let's arrange them in order, we get $$0,1,2,3,4,5,6,7,8,9$$ as digits at unit's place.

Each digit occurs at the end of some cube. Hence one cannot conclude that some number is not a cube by looking at the last digit.

Find the cubes of $$10$$, $$30$$, $$100$$, $$1000$$. What can we say about the zeros at the end?

Let us first find the cubes and then identify the pattern

$$10^3=1000,$$

$$30^3=900\times 30=27000,$$

$$100=10000\times 100=1000000,$$

$$1000=1000000\times 1000=1000000000$$

Now, number of zeros in $$10^3$$ is $$3$$, in $$30^3$$ is $$3$$, in $$100^3$$ is $$6$$, in $$1000^3$$ is $$9$$.

So, the number of zeros at the end is always a multiple of $$3$$.

Find the cube root of $$-17576$$ using factorization.

Let us first find the cube root of $$17576$$. As earlier, we have
$$17576 = 2 \times (8788) = 2 \times 2 \times (4394) = 2 \times 2 \times 2 \times (2197)$$
$$= 2 \times 2 \times 2 \times 13 \times (169)$$
$$= 2\times 2 \times 2 \times 13 \times 13 \times 13$$
$$= (2 \times 13) \times (2 \times 13) \times (2 \times 13)$$
$$= 26 \times 26 \times 26$$.
This shows that $$- 17576 = (-26) \times (-26) \times (-26)$$. Thus $$\sqrt[3]{-17576} = -26$$.

What is the cube of $$20$$?

Again $$(20)^3 = 20 \times 20 \times 20 = (400) \times 20 = 8000$$
We have studied about a solid called cube. It is a solid having equal length, breadth and height. If $$l$$ is the side-length of a cube, then its volume $$V = l^3$$ cubic units.

Find the cube root of $$216$$ by factorisation.

Observe
$$216 = 2 \times (108) = 2 \times 2 \times (54) = 2 \times 2 \times 2 \times 27 = 2 \times 2 \times 2 \times 3 \times 3 \times 3$$
$$=(2 \times 3) \times (2 \times 3) \times (2 \times 3)$$
$$= 6 \times 6 \times 6$$
Hence $$\sqrt[3]{216} = 6$$

Find the smallest number that should be multiplied with $$7803$$ so that the product becomes a perfect cube.

By prime factorising $$7803$$,

we get, $$ 7803 = 3 \times 3 \times 3 \times 17 \times 17$$

$$= 3^3 \times 17 ^2 $$

We know, a perfect cube has multiples of $$3$$ as powers of prime factors.

Here, power of $$3$$ is $$3$$ but power of $$17$$ is $$2.$$

So we need to multiply another $$17$$ to the factorization to make $$7803$$ a perfect cube.

Hence, the smallest number by which $$7803$$ must be multiplied to obtain a perfect cube is $$17$$.

Find the cube of $$16$$.

Cube of $$16$$ is:

$${ 16 }^{ 3 }={ 16 }\times { 16 }\times { 16 }=4096$$.

Therefore, the cube of $$16$$ is $$4096$$.

Find the cube of $$21$$.

The cube of $$21$$ = $${ 21 }^{ 3 }={ 21 }\times { 21 }\times { 21 }=9261$$

Test whether the given number is perfect cube or not: $$243$$

$$243=3\times 3\times 3\times 3\times 3$$
$$243={ 3 }^{ 5 }$$

$$\therefore $$ It is not a perfect cube.

Test whether the given number is perfect cube or not: $$2700$$

$$2700=10\times 270$$

$$2700=10\times 10\times 27$$

$$2700=10\times 10\times 3\times 3\times 3$$

$$2700=10\times 10\times 3^3$$

$$\therefore$$ $$2700$$ is not a perfect cube.

Find the cube of $$8$$.

Hint : Here ,we will first multiply the number by itself. The number will then again be multiplied by the original number. The product will be our final answer.

Step 1: Explanation
A cube of a number is a number multiplied by itself 3 times. Hence we just need to find the product of three numbers 8, 8, 8.

Therefore, $$8^3= 8\times8\times8=512$$

So the required cube of 8 is 512.

Find the smallest number by which $$8640$$ must be divided so that the quotient is a perfect cube.

$$\therefore 8640=\underline {2\times 2\times 2} \times \underline {2\times 2\times 2} \times 5\times \underline {3\times 3\times 3} \\ $$

$$\therefore$$ $$5$$ is the smallest number by which $$8640$$ must be divided, so that the quotient is a perfect cube.

Find the cube root of the given number through estimation:
$$3375$$

For calculating the cube root of $$3375$$, this number has to be separated in two group, i.e. $$3$$ and $$375$$.

considering the group $$375$$:

$$375$$ ends with $$5$$ and we know that if digit $$5$$ is at the end of any perfect cube number, then its cube root will have $$5$$ at its unit place only. Therefore, the digits at the units place of the required cube root is taken as $$5$$.

Now consider the other group $$3$$:

We know that the $${ 1 }^{ 3 }=1$$ and $${ 2}^{ 3 }=8$$

Also, $$1<3<8$$

So, $$1$$ will be taken at the tens place. So, the required cube root of $$3375$$ is $$15$$.

$$\sqrt [ 3 ]{ 3375 } =15$$

Find the smallest number by which $$8788$$ must be multiplied to obtain a perfect cube.

Prime factorising $$8788$$, we get,

$$ 8788 = 2 \times 2 \times 13 \times 13 \times 13$$

$$= 2 ^ 2 \times 13 ^ 3$$.

We know, a perfect cube has multiples of $$3$$ as powers of prime factors.

Here, number of $$2$$'s is $$2$$ and number of $$13$$'s is $$3$$.

So we need to multiply another $$2$$ to the factorization to make $$8788$$ a perfect cube.

Hence, the smallest number by which $$8788$$ must be multiplied to obtain a perfect cube is $$2$$.

Find the cube of $$30$$.

Cube of $$30$$ is:

$$(30)^3=30 \times 30 \times 30$$

$$=27000$$.

Therefore, cube of $$30$$ is $$27000$$.

Find the smallest number by which each of the following number must be multiplied to obtain a perfect cube.
(i) $$243$$ (ii) $$256$$ (iii) $$72$$ (iv) $$675$$ (v) $$100$$

(i) $$243 = 3^5$$

$$\therefore 243$$ can be made a perfect cube by multiplying it by $$3$$

(ii) $$256 = 2^8$$

$$\therefore 256$$ can be made a perfect cube by multiplying it by $$2$$

(iii) $$72 = 3^2 \times2^3$$

$$\therefore 72$$ can be made a perfect cube by multiplying it by $$3$$

(iv) $$675 = 5^2 \times3^3$$

$$\therefore 675$$ can be made a perfect cube by multiplying it by $$5$$

(v) $$100 = 2^2 \times5^2$$

$$\therefore 100$$ can be made a perfect cube by multiplying it by $$2\times5=10$$

Which of the following numbers are not perfect cubes?
(i) $$128$$ (ii) $$100$$ (iii) $$64$$ (iv) $$125$$ (v) $$72$$ (vi) $$625$$

Factorizing each number

(i) $$128 = 2^7 $$ $$-$$ $$\text{Not a perfect cube}$$

(ii) $$100 = 2^2 \times5^2$$ $$-$$ $$\text{Not a perfect cube}$$

(iii) $$64 = 2^6$$ $$-$$ $$\text{A perfect cube}$$

(iv) $$125 = 5^3$$ $$-$$ $$\text{A perfect cube}$$

(v) $$72 = 2^3\times3^2 $$ $$-$$ $$\text{Not a perfect cube}$$

(vi) $$625 = 5^4$$ $$-$$ $$\text{Not a perfect cube}$$

Therefore, $$ 128,100,72,625$$ are not perfect cubes

Find the value of the following:
(i) $$15^{3}$$ (ii) $$(-4)^{3}$$ (iii) $$(1.2)^{3}$$ (iv) $$\left (\dfrac {-3}{4}\right )^{3}$$

(i) $$15^{3} = 15\times 15\times 15 = 3375$$
(ii) $$(-4)^{3} = (-4)\times (-4)\times (-4) = -64$$
(iii) $$(1.2)^{3} = 1.2\times 1.2\times 1.2 = 1.728$$
(iv) $$\left (\dfrac {-3}{4}\right )^{3} = \dfrac {(-3)\times (-3) \times (-3)}{4\times 4\times 4} = \dfrac {-27}{64}$$
Observe the question (ii) Here $$(-4)^{3} = -64$$.

Find the smallest number by which each of the following number must be divided to obtain a perfect cube:
(i) $$81$$ (ii) $$128$$ (iii) $$135$$ (iv) $$192$$ (v) $$704$$ (vi) $$625$$

We know, a perfect cube has multiples of $$3$$ as powers of prime factors.

On prime factorising, we get:

(i) $$81 = 3^4$$.

$$\therefore 81$$ can be made a perfect cube by dividing it by $$3$$.

(ii) $$128 = 2^7$$.

$$\therefore 128$$ can be made a perfect cube by dividing it by $$2$$.

(iii) $$135 = 3^3 \times5$$.

$$\therefore 135$$ can be made a perfect cube by dividing it by $$5$$.

(iv) $$192 = 2^6 \times3$$.

$$\therefore 192$$ can be made a perfect cube by dividing it by $$3$$.

(v) $$704 = 2^6 \times11$$.

$$\therefore 704$$ can be made a perfect cube by dividing it by $$11$$.

(vi) $$625 = 5^4$$.

$$\therefore 625$$ can be made a perfect cube by dividing it by $$5$$.

Is $$243$$ a perfect cube? If not, find the smallest number by which $$243$$ must be multiplied to get a perfect cube.

$$243 = \underbrace {3\times 3\times 3}\times 3\times 3$$
In the above Factorization, $$3\times 3$$ remains after grouping the $$3's$$ in triplets. $$\therefore 23$$ is not a perfect cube.
To make it a perfect cube we multiply it by $$3$$.
$$243\times 3 = \underbrace {3\times 3\times 3} \times \underbrace {3\times 3\times 3}$$
$$729 = 3^{3} \times 3^{3} = (3\times 3)^{3}$$
$$729 = 9^{3}$$ which is a perfect cube.
$$\therefore 729$$ is a perfect cube.

Find the cube root of $$27\times 64$$.

Resolving $$27$$ and $$64$$ into prime factors, we get
$$\sqrt [3]{27} = (3\times 3\times 3)^{\dfrac {1}{3}} = (3^{3})^{\dfrac {1}{3}}$$

$$\sqrt [3]{27} = 3$$
$$\sqrt [3]{64} = (2\times 2\times 2\times 2\times 2\times 2)^{\dfrac {1}{3}}$$

$$= (2^{6})^{\dfrac {1}{3}} = 2^{2} = 4$$

$$\sqrt [3]{63} = 4$$

Now, $$\sqrt [3]{27\times 64} = \sqrt [3]{27}\times \sqrt [3]{64}$$

$$= 3\times 4$$

$$\therefore \sqrt [3]{27\times 64} $$ is the cube root of $$12$$

Find the cube root of each of the following numbers by prime Factorization method:
(i) $$729$$ (ii) $$343$$ (iii) $$512$$ (iv) $$0.064$$ (v) $$0.216$$ (vi) $$5\dfrac{23}{64}$$ (vii) $$-1.331$$ (viii) $$-27000$$

(i) $$729 = 3^6$$

$$ \therefore \sqrt[3]{729}=\sqrt[3]{3^6}=3^2=9$$

(ii) $$343 = 7^3$$

$$ \therefore \sqrt[3]{343}=\sqrt[3]{7^3}=7$$

(iii) $$512= 8^3$$

$$ \therefore \sqrt[3]{512}=\sqrt[3]{8^3}=8$$

(iv) $$0.064 = 2^3 \times (0.001)^3$$

$$ \therefore \sqrt[3]{0.064}=\sqrt[3]{2^3 \times (0.1)^3}=0.2$$

(v) $$0.216=2^3 \times 3^3 \times 0.1^3$$

$$ \therefore \sqrt[3]{0.216}=\sqrt[3]{2^3 \times 3^3\times 0.1^3}=0.6$$

(vi) $$5\cfrac{23}{64} = \cfrac{343}{64} = \cfrac{7^3}{2^6}$$

$$ \therefore \sqrt[3]{\cfrac{343}{64}}=\sqrt[3]{\cfrac{7^3}{2^6}}=\dfrac {7}{8}$$

(vii) $$-1.331 = -1^3 \times 11^3$$

$$ \therefore \sqrt[3]{-1331}=\sqrt[3]{-1^3\times 11^3}=-11$$

(viii) $$-27000 = -1^3\times 3^3\times 2^3\times 5^3$$

$$ \therefore \sqrt[3]{-27000}=\sqrt[3]{-1^3\times 3^3\times 2^3\times 5^3}=-30$$

Is $$64$$ a perfect cube?

$$64 = \underbrace {2\times 2\times 2} \times \underbrace {2\times 2\times 2}$$
$$= 2^{3}\times 2^{3} = (2\times 2)^{3} = 4^{3}$$
$$\therefore 64$$ is a perfect cube.

Check whether the following are perfect cubes?
(i) $$400$$ (ii) $$216$$ (iii) $$729$$ (iv) $$250$$ (v) $$1000$$ (vi) $$900$$

Factorizing each number

(i) $$400 = 2^4 \times 5^2$$ - $$\text{Not a perfect cube}$$

(ii) $$216 = 2^3 \times 3^3$$ - $$\text{A perfect cube}$$

(iii) $$729 = 3^6$$ - $$\text{A perfect cube}$$

(iv) $$250 = 5^3\times 2$$ - $$\text{Not a perfect cube}$$

(v) $$1000 = 10^3 $$ - $$\text{A perfect cube}$$

(vi) $$900 = 3^2 \times 2^2 \times 5^2$$ - $$\text{Not a perfect cube}$$

Therefore, $$216,729,1000 \text{ are perfect cubes}$$.

Find the cube root of $$\dfrac {125}{216}$$

Resolving $$125$$ and $$216$$ into prime factors, we get
$$125 = \underbrace {5\times 5\times 5}$$
$$\sqrt [3]{125} = 5$$
$$216 = \underbrace {2\times 2\times 2} \times \underbrace {3\times 3\times 3}$$
$$\therefore \sqrt [3]{216} = 2\times 3$$
$$\therefore \sqrt [3]{216} = 6$$
$$\therefore \sqrt [3]{\dfrac {125}{216}} = \dfrac {5}{6}$$.

$$512$$

Step $$I$$: Form groups of $$3$$ starting from right most digit of $$512$$, i.e. $$\displaystyle \overline { 512 } $$.

Then, the only group is $$512$$.

Here, $$512$$ has $$3$$ digit.

Step $$II$$: Take $$512$$.

Digit in unit place $$= 2$$.

Therefore, we take one's place of required cube root as $$8$$ ....[Since, $$8^3=512$$].

$$\displaystyle \therefore \quad \sqrt[3] {512 } =8$$.

Is $$250$$ a perfect cube? If not, then by which smallest natural number should $$250$$ be divided so that the quotient is a perfect cube?

Prime factorising, $$250 = 2\times \underbrace {5\times 5\times 5}$$.

We know, a perfect cube has multiples of $$3$$ as powers of prime factors.

The prime factor $$2$$ does not appear in triplet form.

Therefore, $$250$$ is not a perfect cube.

Since in the factorization, $$2$$ appears only one time, we must divide the number $$250$$ by $$2$$, then the quotient is a perfect cube.

$$\therefore 250\div 2 = 125$$

$$= 5\times 5\times 5 = 5^{3}$$, which is a perfect cube.

$$\therefore$$ The smallest number by which $$250$$ should be divided to make it a perfect cube is $$2$$.

Find the factors of $$(a + b + c)^{3} - (b + c - a)^{3} - (c + a - b)^{3} - (a + b - c)^{3}$$.

Given,

$$a+b+c=0$$

or, $$a+b=-c$$

cubing both sides we get,

$$(a+b)^3=-c^3$$

or, $$a^3+b^3+3ab(a+b)=-c^3$$

or, $$a^3+b^3-3abc=-c^3$$ [Since $$a+b=-c$$]

or, $$a^3+b^3+c^3=3abc$$.......(1).

Now

$$(a + b - c)^3 + (c + a - b)^3 + (b + c - a)^3$$

$$=( -2 c)^3 + ( -2 b)^3 + (- 2a)^3$$ [Since $$a+b+c=0\Rightarrow a+b=-c, b+c=-a,c+a=-b$$]

$$=-8(a^3+b^3+c^3)$$

$$=-24abc$$ [Using (1)]

Use Euclid's division lemma to show that the cube of any positive integer is of the form $$9m,9m+1$$ or $$ 9m+8$$

According to Euclid's Division Lemma,

$$a = bq + r$$

Let $$a$$ be any positive integer , then it is of the form $$3q , 3q + 1, 3q +2$$

Here are the following cases ----

CASE I : When $$a = 3q$$

$$a = 3q$$

$$a^3 = (3q)^3$$

$$a^3 = 27q^3$$

$$a^3 = 9(3q^3) = 9m$$, where $$m = 3q^3$$

CASE II : When $$a = 3q + 1$$

$$a = 3q + 1$$

$$a^3 = (3q + 1)^3$$

$$a^3 = 27q^3 + 27q^2 + 9q + 1$$

$$a^3 = 9m + 1$$ where $$m = q(3q^2 + 3q + 1)$$

CASE III : When $$a = 3q + 2$$

$$a = 3q + 2$$

$$a^3 = (3q + 2)^3$$

$$a^3 = 27q^3 + 54q^2 + 36q + 8$$

$$a^3 = 9m + 8$$

where $$m = q(3q^2 + 6q + 4)$$

Therefore, $$a^3$$ is either of the form $$9m, 9m + 1 \ or \ 9m + 8$$

Write ones digit of the cube of each of the following numbers:
$$231$$
$$358$$
$$419$$
$$725$$
$$854$$
$$987$$
$$752$$
$$893$$

ones digit of cube of $$231\Rightarrow{1}^{3}=1$$

ones digit of cube of $$358\Rightarrow { 8 }^{ 3 }=512\Rightarrow 2$$

ones digit of cube of $$419\Rightarrow { 9 }^{ 3 }=729\Rightarrow 9$$

ones digit of cube of $$725\Rightarrow { 5 }^{ 3 }=125\Rightarrow 5$$

ones digit of cube of $$854\Rightarrow { 4 }^{ 3 }=64\Rightarrow 4$$

ones digit of cube of $$ 987\Rightarrow { 7 }^{ 3 }=343\Rightarrow 3$$

ones digit of cube of $$752\Rightarrow { 2 }^{ 3 }=8\Rightarrow 8$$

ones digit of cube of $$893\Rightarrow { 3 }^{ 3 }=27\Rightarrow 7$$

$$3375$$

Step $$I$$: Form groups of $$3$$ starting from right most digit of $$3375$$, i.e. $$\displaystyle \overline { 3 }\: \overline { 375 } $$.

Then, the two groups are $$3$$ and $$375.$$

Here, $$3$$ has $$1$$ digit and $$375$$ has $$3$$ digit.

Step $$II$$: Take $$375$$.

Digit in unit place $$= 5$$.

Therefore, we take one's place of required cube root as $$5$$ ....[Since, $$5^3=125$$].

Step $$III$$: Now, take the other group $$3$$.

We know, $$\displaystyle {1}^{ 3 }=1 $$ and $${2 }^{ 3 }=8$$.

Here, the smallest number among $$1$$ and $$2$$ is $$1$$.

Therefore, we take $$1$$ as ten's place.

$$\displaystyle \therefore \quad \sqrt[3] { 3375 } =15$$.

Find the value of $$4^3$$

$$\textbf{Step-1:}$$ $$\textbf{Finding}$$ $${4^3}$$

$$\textbf{Step-2:}$$ $${4^3 = }$$ $${4} \times {4} \times {4 = 64}$$

$$\textbf{Hence, }$$ $${4^3 = 64}$$

$${x}^{3}+7{x}^{2}+x=$$?
If $$x=7$$

For $$x=7$$,

$${x}^{3}+7{x}^{2}+x$$

$$=7^3+7^3+7$$

$$=2.343+7$$

$$=693$$.

Find the cube of $$-1$$.

Hint: For Odd powers, cube of negative number is negative. For even powers cube of negative number is positive.

Explanation:

Cube of -1 = $$(-1)^3$$ = (-1).(-1).(-1) = -1

We can also see that since it is an odd power, cube of negative number has to negative.

$$\sqrt[3]{5832}\times \sqrt[3]{15625}=\sqrt[3]{(18)^3\times ()^3}$$.

$$\sqrt [ 3 ]{ 5832 } \times \sqrt [ 3 ]{ 15625 } =\sqrt [ 3 ]{ { (18) }^{ 3 }{ (x) }^{ 3 } } \\ \sqrt [ 3 ]{ { 3 }^{ 6 }\times { 2 }^{ 3 } } \times \sqrt [ 3 ]{ { (25) }^{ 3 } } =18\times x\\ 18\times 25=18\times x\\ x=25$$

Find $$\left(\dfrac{2}{3}\right)^{3}$$.

$$\left(\dfrac{2}{3}\right)^3=?$$

$$\left(\dfrac{2}{3}\right)^3=\dfrac{2^3}{3^3}$$

$$=\dfrac{2\times 2\times 2}{3\times 3\times 3}$$

$$=\dfrac{8}{27}$$

$$\therefore \left(\dfrac{2}{3}\right)^3=\dfrac{8}{27}$$

Write the ones digit of the cube of each of the following numbers
A) $$231$$
B) $$358$$
C) $$491$$
D) $$854$$
E) $$987$$
F) $$752$$

Multiply one's digit

$$(231)^{3}=231\times 231\times 231$$

$$\Rightarrow $$ One's digit $$=1\times 1\times 1=1$$

$$(358)^{2}=8\times 8\times 8=512$$

$$\Rightarrow $$ One's digit $$=2$$

$$(491)^{3}=1\times 1\times 1=1$$

$$(854)^{3}=4\times 4\times 4=64$$

$$\Rightarrow $$ One's digit $$=4$$

$$(987)^{3}=7\times 7\times 7=343$$

$$\Rightarrow $$ One's digit $$=3$$

$$(752)^{3}=2\times 2\times 2=8$$

If $$7 ^ { 3 } = 343$$ then $$ \sqrt [3]{ 343 } = ?$$

$$\begin{array}{l} We\, have \\ { 7^{ 3 } }=343 \\ Now \\ \sqrt [ 3 ]{ { 343 } } \\ =\sqrt [ 3 ]{ { { { \left( 7 \right) }^{ 3 } } } } \\ =7 \\ which\, is\, \, the\, required\, answer. \end{array}$$

Find the cube root of $$-17576$$ using factorisation.

Let us first find the cube root of $$ 17576$$. as earlier, we have
$$17576=2\times (8788)=2\times 2\times (4394)= 2\times 2 \times 2\times (2197)$$
$$= 2\times 2 \times 2\times 13\times (169)$$
$$= 2\times 2 \times 2\times 13\times 13 \times 13$$
$$= (2\times 13) \times (2\times 13)\times (2 \times 13)$$
$$= 26\times 26\times 26.$$
This shows that $$-17567=(-26)\times (-26)\times (-26).$$
Thus $$-\sqrt[3]{17576}=-26.$$

Simplify :
$$\sqrt [4]{81} - 8\sqrt [3]{216} + 15\sqrt [3]{32} + \sqrt {225}$$.

$$\sqrt[4]{{81}} - 8\sqrt[3]{{216}} + 15\sqrt[5]{{32}} + \sqrt {225} $$

$$ = {\left( {{3^4}} \right)^{\frac{1}{4}}} - 8{\left( {{6^3}} \right)^{\frac{1}{3}}} + 15{\left( {{2^5}} \right)^{\frac{1}{5}}} + 15$$

$$ = 3 - 8 \times 6 + 15 \times 2 + 15$$

$$= 3 - 48 + 30 + 15$$

$$ = 48 - 48$$

$$ = 0$$

Evaluate $$\sqrt[3]{{0.000343}} + \sqrt[3]{{0.729}} + \sqrt[3]{{1.331}}$$

$$\sqrt [ 3 ]{ 0.000343 } +\sqrt [ 3 ]{ 0.729 } +\sqrt [ 3 ]{ 1.331 } \\ \sqrt [ 3 ]{ \cfrac { 343 }{ { 10 }^{ 6 } } } +\sqrt [ 3 ]{ \cfrac { 729 }{ 1000 } } +\sqrt [ 3 ]{ \cfrac { 1331 }{ 1000 } } \\ \cfrac { 7 }{ { 10 }^{ 2 } } +\cfrac { 9 }{ 10 } +\cfrac { 11 }{ 10 } \\ =0.077+0.9+1.1\\ =2.07$$

Express $$15^3$$ as the sum of consecutive odd numbers.

Let the three numbers be $$\left( x - 1 \right), x$$ and $$\left( x + 1 \right)$$.

Given, the sum of three consecutive odd numbers is $${15}^{3}$$.

$$\Rightarrow \; {15}^{3} = 3375$$.

$$\therefore \; \left( x - 1 \right) + x + \left( x + 1 \right) = 3375$$

$$\Rightarrow$$ $$ 3x = 3375$$

$$\Rightarrow$$ $$x = 1125$$.

Therefore,

$${1}^{st}$$ number $$= x - 1 = 1125 - 1 = 1124$$.

$${2}^{nd} $$ number $$ = x = 1125$$.

$${3}^{rd}$$ number $$ = x + 1 = 1125 + 1 = 1126$$.

Hence, the three consecutive numbers are $$1124, 1125, 1126$$.

Then, $$1124+1125+1126=15^3$$.

Simplify : $$\dfrac{\sqrt[3]{8} - \sqrt[3]{125}}{1 - 5\sqrt{2}}$$

Given:$$\dfrac{\sqrt[3]{8}-\sqrt[3]{125}}{1-5\sqrt 2}=\dfrac{\sqrt[3]{2^3}-\sqrt[3]{5^3}}{1-5\sqrt 2}$$

$$=\dfrac{2-5}{1-5\sqrt 2}=\dfrac{3}{5\sqrt 2-1}$$

Multiplying and dividing by $$5\sqrt 2+1$$

$$=\dfrac{3}{5\sqrt 2-1}\times \dfrac{5\sqrt 2+1}{5\sqrt 2+1}$$

$$=\dfrac{3(5\sqrt 2+1)}{51}$$

If $$2a + 3b + c = 0$$ then show that:
$$8{a^3} + 27{b^3} + {c^3} = 18abc$$

We have,

$$2a+3b+c=0$$

$$2a+3b=-c$$ …….. (1)

On taking cube both sides, we get

$$ {{\left( 2a+3b \right)}^{3}}={{\left( -c \right)}^{3}} $$

$$ 8{{a}^{3}}+27{{b}^{3}}+3\times 2a\times 3b\left( 2a+3b \right)=-{{c}^{3}} $$

$$ 8{{a}^{3}}+27{{b}^{3}}+18ab\left( 2a+3b \right)=-{{c}^{3}} $$

From equation (1),

$$ 8{{a}^{3}}+27{{b}^{3}}+18ab\left( -c \right)=-{{c}^{3}} $$

$$ 8{{a}^{3}}+27{{b}^{3}}-18abc=-{{c}^{3}} $$

$$ 8{{a}^{3}}+27{{b}^{3}}+{{c}^{3}}=18abc $$

Hence, proved.

The radius of a nuleus of mass number $$'A'$$ is given by $$R=1.3\ \times 10^{-16}\times A^{1/3}$$. Find the order of magnetude of radius for a nucles with $$A=216$$.

It is given that, $$A=216$$

$$R=1.3\times 10^{-16}\times A^{\frac{1}{3}}$$

$$R=1.3\times 10^{-16}\times (216)^{\frac{1}{3}}$$

$$R=1.3\times 10^{-16}\times 6$$

$$R=7.8\times 10^{-16}$$

Find the cube of the following:
$$2.5$$.

$$2.5^3=25^3*0.1^3=15625*0.001=15.625$$

Factorize:
$$125x^3+27y^3+8z^3-90xyz$$

$$125{ x }^{ 3 }+27{ y }^{ 3 }+{ 8z }^{ 3 }-90xyz\\ { (5x) }^{ 3 }+{ (3y) }^{ 3 }+{ (2z) }^{ 3 }-5x.3y.2z\times 3\\ { a }^{ 3 }+{ b }^{ 3 }+{ c }^{ 3 }-3abc=(a+b+c)({ a }^{ 2 }+{ b }^{ 2 }+{ c }^{ 2 }-ab-bc-ca)\\ (5x+3y+2z)(25{ x }^{ 2 }+9{ y }^{ 2 }+4{ z }^{ 2 }-15xy-6yz-10xz)$$

Find the cube root of $$5832$$.

We need to find the cube root of $$5832$$.

The primes factorization of $$5832$$ is as follows

$$5832=2\times 2\times 2\times 3\times 3\times 3\times 3\times 3\times 3$$

Thus cube root of $$5832=2\times 3\times 3=18$$

Hence $$\sqrt[3]{5832}=18$$

Find the cube root of the following by prime factorization method
$$5832$$ $$and$$ $$21952$$$$ ?$$

In Cube root by prime factorization we make group of 3 same prime factor.

The$$ \sqrt[3]{5832} $$ $$is$$

$$5832$$ = $$3×3×3×3×3×3×2×2×2$$

$$=3×3×2$$
$$=18 $$

The $$ \sqrt[3]{21952}$$ $$ is,$$

$$21952$$= $$2×2×2×2×2×2×7×7×7$$

$$=2×2×7$$

$$=28 $$

Is $$53240$$ a perfect cube? If not then by which smallest natural numbers should be divided so that the quotient is a perfect cube?

Prime factorising, $$53240=5\times 2^3\times 11^3$$.

We know, a perfect cube has multiples of $$3$$ as powers of prime factors.

The prime factor $$5$$ does not appear in triplet form.

Therefore, $$53240$$ is not a perfect cube.

Since in the factorization, $$5$$ appears only one time, we must divide the number $$53240$$ by $$5$$, then the quotient is a perfect cube.

$$\therefore 53240\div 5 = 10648$$

$$= 22\times 22\times 22 = 22^{3}$$, which is a perfect cube.

$$\therefore$$ The smallest number by which $$53240$$ should be divided to make it a perfect cube is $$5$$.

Are the following number perfect cubes?If yes,then find the number whose cube is the given numbers:$$3645$$

1146639_1191256_ans_65585edafa0b4883a90aafeda6a70792.jpg

Is $$392$$ a perfect cube? If not find the smallest natural number by which $$392$$ must be multiplied, so that the product is a perfect cube.

Prime factorising $$392$$, we get,

$$392= 2 \times 2 \times 2 \times 7 \times 7$$

$$= 2 ^3 \times 7 ^2 $$.

We know, a perfect cube has multiples of $$3$$ as powers of prime factors.

Here, number of $$2$$'s is $$3$$ and number of $$7$$'s is $$2$$.

Therefore, $$392$$ is clearly not a perfect cube.

Here, we need to multiply another $$7$$ to the factorization to make $$392$$ a perfect cube.

Hence, the smallest number by which $$392$$ must be multiplied to obtain a perfect cube is $$7$$.

Find the smallest number which when multiplied with $$53240$$ will make the product a perfect cube.

Here the prime factors if the given number

$$53240=2\times 2\times 2\times 5\times 11\times 11\times 11$$

As we know that a cube of a number consists of product of three similar numbers.

in the above factors we find that there is a triplet of $$2$$ and $$11$$, but not of $$5$$.

To make a triplet for $$5$$ we need two more $$5$$

$$=5\times 5$$

$$=25$$

So, the required number is $$25.$$

$$\Rightarrow 53240\times 25=1331000$$

$$1331000$$ is perfect cube of $$110.$$

Are the following number perfect cubes?If yes,then find the number whose cube is the given numbers:$$1728$$

1146649_1191258_ans_788a3b10ad124784855395cf4e37cb35.jpg

Are the following number perfect cubes?If yes,then find the number whose cube is the given numbers:$$175616$$

1146624_1191249_ans_2cdf5a4dd7b24ac781b971ab6a13bce9.jpg

Are the following number perfect cubes?If yes,then find the number whose cube is the given numbers:$$91125$$

1146629_1191252_ans_c3f1c7b2a5f24a1b87c8e79e95fca6a6.jpg

Find the cube root of the following numbers by prime fractorisaiton method.
$$343$$
$$4096$$
$$5832$$
$$125000$$

Finding the cube root of each of the given number by representing them as a product of their prime factors:

$$i)\ 343=7\times 7\times 7\\$$

$$=7^3\\$$

Hence, $$ \sqrt [3]{343}=7$$

$$ii)\ 4096=2\times 2\times 2\times 2\times 2\times 2\times 2\times 2\times 2\times 2\times 2\times 2\\$$

$$=2^{12}\\$$

$$={2}^{4^3}\\$$

Hence, $$ \sqrt[3]{4096}=2^4=16$$

$$iii)\ 5832=3\times 3\times 2\times 3\times 3\times 2\times 3\times 3\times 2\\$$

$$=(3^2\times 2)^3\\$$

Hence, $$ \sqrt[3]{5832}=3^2\times 2=18$$

$$iv)\ 125000=2\times 2\times 2\times 5\times 5\times 5\times 5\times 5\times 5\\$$

$$=2^3\times 5^3\times 5^3\\$$

$$=(2\times 5)^3\times 5^3\\$$

$$=(5\times 10)^3\\$$

Hence, $$ \sqrt[3]{125000}=5\times 10=50$$

$${ (3\times x) }^{ 3 }=1728$$.

What is the value of x.

$$(3 \times x)^3 = 1728$$

factorizing $$1728$$, we get :-

$$(3 \times x)^3 = 3 \times 3 \times 3\times 4 \times 4 \times 4$$

$$\Rightarrow (3 \times x)^3 = (3 \times 4)^3$$

$$\Rightarrow $$ comparing RHS & LHS we get $$x = 4$$

Evaluate $$\sqrt{6+}\sqrt [ 3 ]{ 27 } $$

$$\sqrt{6}+\sqrt [ 3 ]{ 27 } $$

$$=\sqrt{6}+3$$

$$=\sqrt{2}.\sqrt{3}+3$$

$$=\left( 1.414\right) \left( 1.712\right)+3$$

$$=2.449+3$$

$$=5.449.$$

Hence, the answer is $$5.449.$$

Find the value of:
$$9^{3}$$

$$ \Rightarrow { 9^{ 3 } }=? \\ \Rightarrow 9\times 9\times 9=729 \\ $$

Are the following number perfect cubes?If yes,then find the number whose cube is the given numbers:$$74088$$

1146655_1191261_ans_65635324b0d74fadad5fc3c31155d173.jpg

Are the following number perfect cubes?If yes,then find the number whose cube is the given numbers:$$216000$$

1146659_1191266_ans_64a65f560e7d4691a0919824215f9725.jpg

$${\left( { - 5} \right)^a} = - 125$$

Given $$(-5)^{9}= -125$$

$$\Rightarrow (-5)^{9} = (-5)^{3}$$

$$\Rightarrow a=3$$

Find the value of
$${x^3} + {\dfrac {1} {x^3}}$$ if $$x + \dfrac { 1} {x} = 2$$

$$\Rightarrow$$ $${x}^{3}+\cfrac{1}{{x}^{3}}$$

$$={ \left( x+\cfrac { 1 }{ x } \right) }^{ 3 }-3x.\cfrac { 1 }{ x } { \left( x+\cfrac { 1 }{ x } \right) }={ \left( 2 \right) }^{ 3 }-3\times 2=8-6=2$$

Solve : $$\sqrt[3]{1.331} + \sqrt[3]{0.027} + \sqrt[3]{0.008}$$

$$\sqrt [ 3 ]{ 1.331 } +\sqrt [ 3 ]{ 0.027 } +\sqrt [ 3 ]{ 0.008 } \\ = 1.1\quad +\quad 0.3\quad +\quad 0.2\\=1.6$$

Find the value of the following.
$$5^{3}$$

$$ 5^{3} = 5\times 5\times 5 = 25\times 5 = 125 $$

What is the least number by which $$13720$$ must be divide so that the quotient is a perfect cube ?

The prime factorisation of $$13720$$ yields

$$13720=2\times 2\times 2\times 7\times 7\times 7\times 5$$

$$13720=2^3\times 7^2\times 5$$

In order to be a perfect cube we hae to divide the given number with $$5$$ then,

$$\cfrac{13720}{5}=\cfrac{2^3\times 7^3\times 5}{5}=(2\times 7)^3=2744$$

Hence $$5$$ is the least number by which $$13720$$ must be divided so that the quotient ia a perfect square.

Find the cube root of each of the following cube numbers through estimation.
$$85184$$

We know that

$${ 45 }^{ 3 }=91,125$$

$$\therefore$$ $$\sqrt [ 3 ]{ 85184 } <45$$

$${ 44 }^{ 3 }=85184\Rightarrow \sqrt [ 3 ]{ 85184 } =44$$

Hence, this is the answer.

Find the cube root of the number by prime factorization method: 54872.

Given number is $$54872$$.

To find out: The cube root of the number, using prime factorization method.

The prime factors of $$54872$$ are $$2$$ and $$19$$.

$$54872$$ can be represented as the product of its prime factors as:

$$ 54872=2\times 2\times 2\times 19\times 19\times 19 $$

$$\Rightarrow 54872=38\times 38\times 38 $$

$$\Rightarrow 54872={{38}^{3}} $$

$$\therefore \ $$ Cube root of $$54872=38$$.

Hence, $$38$$ is the required answer.

Factorise: $$27{a^3} + \dfrac{1}{{64{b^3}}} + \dfrac{{27{a^2}}}{{4b}} + \dfrac{{9a}}{{16b^2}}$$.

Consider the given expression

$$27a^3+\dfrac{1}{64b^3}+\dfrac{27a^2}{4b}+\dfrac{9a}{16b^2}$$ ………..$$(1)$$

We know that $$(x+y)^3=x^3+y^3+3x^2y+3xy^2+y^3$$ …………$$(2)$$

Thus,

$$27a^3+\dfrac{1}{64.b^3}+\dfrac{27.a^2}{4b}+\dfrac{9a}{16.b^2}$$

$$=(3a)^3+\dfrac{1}{(4)^3, b^3}+3(3a)^2.\left(\dfrac{1}{4b}\right)+3(3a)\left(\dfrac{1}{4b}\right)^2$$ ……….$$(3)$$

Comparing the above two equations $$(2)$$ and $$(3)$$, we have

$$x=3a; y=\dfrac{1}{4b}$$

Thus equation $$(1)$$ becomes.

$$27a^3+\dfrac{1}{64b^3}+\dfrac{27.a^2}{4b}+\dfrac{9a}{16.b^2}=\left[3a+\dfrac{1}{4b}\right]^3$$.

1196693_1394471_ans_f13d0e8afc594b9abc8e26982dece3ba.jpg

Find the smallest number by which the given number must be multiplied to obtain a perfect cube:- 8192

We have,

$$8192$$

But we know that,

$$ 8192=2\times 64\times64= 2\times 2^6 \times{{2}^{6}} $$

$$ \Rightarrow 8192={{2}^{12}}\times 2 $$

As 8192 is not a perfect cube, then, $$2^2$$ is the smallest such number that can be multiplied to 8192 to make a perfect cube.

$$\therefore, 8192\times 2^2 = 2^{13}\times 2^2 = 2^{15}$$ which is the perfect cube of $$2^5$$.

Find the cube root of each of the following cube numbers through estimation.
$$19683$$

$${ 25 }^{ 3 }=15625\quad \quad \quad { 30 }^{ 3 }=27000$$

$$\therefore$$ $$25<\sqrt [ 3 ]{ 19683 } <30$$

$${ 26 }^{ 3 }=17576\quad :\quad \sqrt [ 3 ]{ 19683 } >26$$

$${ 27 }^{ 3 }=19683$$

$$\therefore$$ $$\sqrt [ 3 ]{ 19683 } =27$$

Find the following
$$\sqrt [3]{0.000216}$$

$$\sqrt{0.000216}=\sqrt[3]{\dfrac{216}{1000000}}=\sqrt[3]{\dfrac{6^3}{(100)^3}}=\dfrac{6}{100}=0.06$$.
1189548_1355020_ans_2a2feb607af443acb8dbfbeabcf6e482.jpg

Find the cube root of each of the following numbers:
$$5^{1182}$$

We have,

$${ 5 }^{ 1182 }=\sqrt [ 3 ]{ { 5 }^{ 1182 } } ={ \left( { 5 }^{ 1182 } \right) }^{ 1/3 }={ 5 }^{ \frac { 1182 }{ 3 } }={ 5 }^{ 394 }$$

Hence, this is the answer.

Find the cube of the number $$100$$.

Cube of $$100$$ is:

$$(100)^3=100\times 100 \times 100$$

$$=100 \times 10000$$

$$=1000000$$.

Hence, the cube of $$100$$ is $$1000000$$.

Write the cubes of $$5$$ natural numbers which are multiples of $$3$$ and verify the followings:
The cube of a natural which is a multiple of $$3$$ is a multiple of $$27$$'

1868100_1671763_ans_4d97f00aaa3543e2a42ad20d22d7a789.png

Find the cube of the following number:
$$55$$

Cube of $$55=55^3$$

$$=55\times 55\times 55$$

$$=3025\times 55$$

$$=166375$$

1540032_1671753_ans_4de77956d1394cff95cd43d07cc0ed3e.jpg

Find the cube of the following number $$12$$.

Cube of $$12$$ is,

$$12^3$$ $$=12\times 12\times 12$$

$$=12\times 144$$

$$=1728$$.

Therefore, cube of $$12$$ is $$1728$$.

Find $$\dfrac { \sqrt [ 3 ]{ 729 } +\sqrt [ 3 ]{ 343 } }{ \sqrt [ 3 ]{ 512 } } $$

$$\dfrac { \sqrt [ 3 ]{ 729 } +\sqrt [ 3 ]{ 343 } }{ \sqrt [ 3 ]{ 512 } } $$

$$=\dfrac{{\left({9}^{3}\right)}^{\frac{1}{3}}+{\left({7}^{3}\right)}^{\frac{1}{3}}}{{\left({8}^{3}\right)}^{\frac{1}{3}}}$$

$$=\dfrac{9+7}{8}=\dfrac{16}{8}=2$$

Find the cube of the number $$40$$.

Cube of $$40$$ is:

$$(40)^3=40\times 40 \times 40$$

$$=40 \times 1600$$

$$=64000$$.

Hence, the cube of $$40$$ is $$64000$$.

Find the cubes of the following number $$7$$.

Cube of $$7$$ is:

$$7^3=7 \times 7 \times 7=343$$.

Therefore, the cube of $$7$$ is $$343$$.

Show that cube of any positive integer is of the form $$4 m , 4 m + 1$$ or $$4 m +3$$ , for some integer $$m$$ .

By euclids division Lemma

$$a=bq+r$$

Here $$a$$ is any positive integer & $$b=4$$

& $$0\le r < 4\quad r=0, 1, 2, 3$$

$$a=4\ q+r.....(1)$$

Put $$r=0$$ in equation $$(1)$$

$$a=4\ q$$

Cubing on both sides

$$(a)^3 =(4\ q)^3$$

$$(a)^3=64\ q^3=4(16\ q^3)$$

$$\boxed {a^3 =4\ m}$$ where $$m= 16\ q^3$$

Put $$=1$$ in equation $$(1)$$

$$a=4\ q+1$$ cubing both side

$$a^3=(4\ q+1)^3$$

we know

$$(a+b)^3=a^3+b^3+3a^3 b+3a\ b^2$$

so apply

$$a^3=(4\ q)^3+(1)^3+3(4\ q)^2\times 1+3(4\ q)\times (1)^2$$

$$a^3=64\ q^3+1+3\times 16\ q^2+12\ q$$

$$a^3=64\ q^3+1+48\ q^2+12\ q$$

$$a^3=4(16\ q^3+12\ q^3+3\ q)+1$$

$$\boxed {a^3=4\ m+1}$$

where $$m=16\ q^3+12\ q^2 + 3\ q$$

again put $$r=2$$ in equation $$(1)$$

$$a=4\ q+2$$

cube both sides

$$a^3=(4\ q+2)^3$$

$$a^3=(4\ q)^3+(2)^3+3\times (4\ q)^2\times 2+3\times 4\ q(2)$$

$$a^3=64\ q^3+8+3\times 16\ q^2\times 2+3\times 4\ q\times 4$$

$$a^3=64\ q^3+8+96\ q^2+48\ q$$

$$a^3=4(16\ q^3+2+24\ q^3+12\ q)$$

$$\boxed {a^3 =4\ m}$$

$$m=16\ q^3+2+24\ q^3+12\ q$$

Put $$r=3$$ in equation $$(1)$$

$$a=4\ q+3$$

again cube

$$a^3=(4\ q+3)^3$$

$$a^3=(64\ q^3+27+144\ q^2+108\ q)+3$$

$$a^3=(64\ q^3+24+144\ q^2+108\ q)+3$$

$$a^3=4\ m+3$$

when $$m=16\ q^3+6+36\ q^2+27$$

hence $$a^3$$ is of the for

$$4\ m,\ 4\ m+1, 4\ m+3$$

Which is the smallest number that must be multiplied to $$77175$$ to make it a perfect cube?

The prime factors of $$77175$$ are
$$ = 3 \times 3 \times 5 \times 5 \times 7 \times 7 \times 7 $$
$$ = (7 \times 7 \times 7) \times 3 \times 3 \times 5 \times 5 $$
Clearly, $$ 77175$$ must be multiplied by
$$ 3 \times 5 = 15 $$
1779616_1506648_ans_a91bd818be9943109fe97d7b7747a7c9.png

1779616_1506648_ans_a91bd818be9943109fe97d7b7747a7c9.png

Which of the following are perfect cubes?
$$216$$

1540041_1671774_ans_50592b174b6348aaacdaee14388d16b6.jpeg

Which of the following are perfect cubes?
$$64$$

1540039_1671773_ans_ce4cba7f82d944f89508f1859e7004c3.jpeg

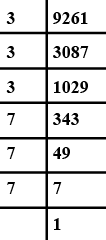
Check whether the following number is perfect cube or not.
$$3087$$

1869230_1671779_ans_0adc1d5be18247fcbc4004f5caddaaa6.jpg

What is the smallest number by which the following numbers must be multiplied, so that the products are perfect cubes?
$$675$$

1522684_1671788_ans_f842d3efab73482b9ed944dac4dda3d4.jpg

Check whether the following number is perfect cube or not.
$$106480$$

1869233_1671781_ans_ebb357504e9d47ca8e09c531e0ff209a.jpg

Check whether the following number is perfect cube or not.
$$456533$$

1869258_1671783_ans_81835fbf2b0e4712b6d9db0418df50f9.jpg

Check whether the following number is perfect cube or not.
$$166375$$

1869256_1671782_ans_f7961290d900431e8cc0d1f9be76b43b.jpg

Which of the following are perfect cubes?
$$4608$$

1540047_1671780_ans_8afa1ac28bcf4f0bacd781974a4504ee.jpeg

Write the cubes of $$5$$ natural numbers of which are multiples of $$7$$ and verify the following:
The cube of a multiple of $$7$$ is a multiple of $$7^{3}$$.

1869011_1671772_ans_8787e8fa88094cd587bdbf7ac7b2ac20.jpg

Check whether the following number is perfect cube or not.
$$1000$$

1869188_1671777_ans_e0ef783440f44e4188f682de4d408850.jpg

What is the smallest number by which the following numbers must be multiplied, so that the products are perfect cubes?
$$7803$$

1522680_1671791_ans_efee40718df348c8979cb176b69a26ed.jpg

What is the smallest number by which the following numbers must be multiplied, so that the products are perfect cubes?
$$107811$$

Prime factorising $$107811$$, we get,

$$ 107811 = 3 \times 3 \times 3 \times 3 \times 11 \times 11 \times 11$$

$$= 3 ^4 \times 11 ^3$$.

We know, a perfect cube has multiples of $$3$$ as powers of prime factors.

Here, number of $$3$$'s is $$4$$ and number of $$11$$'s is $$3$$.

So we need to multiply another $$3^2$$ to the factorization to make $$107811$$ a perfect cube.

Hence, the smallest number by which $$107811$$ must be multiplied to obtain a perfect cube is $$3^2=9$$.

By which smallest number must the following number be divided so that the quotient is a perfect cube?
$$7803$$

Prime factorising $$7803$$, we get,

$$ 7803 = 3 \times 3 \times 3 \times 17 \times 17$$

$$= 3^3 \times 17 ^2 $$.

We know that for a perfect cube prime factors occur in the multiple of $$3$$.

Here, number of $$3$$'s is $$3$$ and number of $$17$$'s is $$2$$.

So we need to divide $$17^2$$ from the factorization to make $$7803$$ a perfect cube.

Hence, the smallest number by which $$7803$$ must be divided to obtain a perfect cube is $$17^2=289$$.

What is the smallest number by which the following numbers must be multiplied, so that the products are perfect cubes?
$$35721$$

Prime factorising $$35721$$, we get,

$$ 35721= 3 \times 3 \times 3 \times 3 \times 3 \times 3 \times 7 \times 7$$

$$= 3 ^6 \times 7 ^2 $$.

We know, a perfect cube has multiples of $$3$$ as powers of prime factors.

Here, number of $$3$$'s is $$6$$ and number of $$7$$'s is $$2$$.

So we need to multiply another $$7$$ to the factorization to make $$35721$$ a perfect cube.

Hence, the smallest number by which $$35721$$ must be multiplied to obtain a perfect cube is $$7$$.

What is the smallest number by which the following numbers must be multiplied, so that the products are perfect cubes?
$$2560$$

1522681_1671790_ans_248ea59acfeb4a02a35b105bdc281e48.jpg

By which smallest number must the following number be divided so that the quotient is a perfect cube?
$$108711$$

1869706_1671810_ans_33f4b9dcd0c045e584e8f172dbf3d652.jpg

By which smallest number must the following numbers be divided so that the quotient is a perfect cube?
$$8788$$

1523213_1671807_ans_4e844b58cb4644ba94afef88f7a6e847.jpg

By which smallest number must the following number be divided so that the quotient is a perfect cube?
$$8640$$

Prime factorising $$8640$$, we get,

$$8640 = 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 5 \times 3 \times 3 \times 3$$

$$= 2^6 \times 5 ^1 \times 3^3 $$.

We know, a perfect cube has multiples of $$3$$ as powers of prime factors.

Here, number of $$2$$'s is $$6$$, number of $$5$$'s is $$1$$ and number of $$3$$'s is $$3$$.

So we need to divide $$5$$ from the factorization to make $$8640$$ a perfect cube.

Hence, the smallest number by which $$8640$$ must be divided to obtain a perfect cube is $$5$$.

By which smallest number must the following numbers be divided so that the quotient is a perfect cube?
$$675$$

Prime factorising $$675$$, we get,

$$675=5\times 5\times 3\times 3\times 3$$ $$ = 3^{3}\times5^2$$.

We know, a perfect cube has multiples of $$3$$ as powers of prime factors.

Here, number of $$3$$'s is $$3$$ and number of $$5$$'s is $$2$$.

So we need to divide $$5^2$$ from the factorization to make $$675$$ a perfect cube.

Hence, the smallest number by which $$675$$ must be divided to obtain a perfect cube is $$5^2=25$$.

What is the smallest number by which the following numbers must be multiplied, so that the products are perfect cubes?
$$1323$$

We need to find the smallest number such that when multiplied by $$1323$$ it becomes a cube.

$$1323=3\times3\times3\times 7\times7$$

$$=3^3\times 7^2$$

For a number to be a perfect cube all its prime factors must have their exponents as multiple of $$3$$.

So, here exponent of prime factor $$3$$ is $$3$$ which is a multiple of $$3$$ but an exponent of prime factor $$7$$ is $$2$$ so, to make it a multiple of $$3$$ we have to multiply $$1323$$ by $$7.$$

Hence, the number will become $$9261$$ which is a perfect cube of $$21.$$

By which smallest number must the following number be divided so that the quotient is a perfect cube?
$$243000$$

On prime factorization of $$2,43,000$$ we get,

$$ 243000 = 2 \times 2 \times 2 \times 5 \times 5 \times 5 \times 3 \times 3 \times 3 \times 3 \times 3$$

$$= 2^3 \times 5 ^3 \times 3^5$$

We know, a perfect cube has multiples of $$3$$ as powers of prime factors.

Here, exponent of $$2$$ is $$3,$$ exponent of $$5$$ is $$3$$ and exponent of $$3$$ is $$5.$$

So we need to divide $$3^2$$ that is $$9$$ from the number $$243000$$ to make a perfect cube.

Check whether the following number is a perfect cube or not.
$$64$$

1874339_1671845_ans_e05f62e1da2340f1b4c2a4fef6b805dc.jpg

Show that:
$$\sqrt[3]{64\times 729}=\sqrt[3]{64}\times \sqrt[3]{729}$$

$$\text{LHS}$$ = $$\sqrt[3]{64 \times 729}=\sqrt[3]{4 \times 4 \times 4 \times 9 \times 9 \times 9}=\sqrt[3]{\{4 \times 4 \times 4\} \times\{9 \times 9 \times 9\}}=4 \times 9=36$$

$$\mathrm{RHS}=\sqrt[3]{64} \times \sqrt[3]{729}=\sqrt[3]{4 \times 4 \times 4} \times \sqrt[3]{9 \times 9 \times 9}=4 \times 9=36$$

Because $$\text{LHS}$$ is equal to $$\text{RHS}$$, the equation is true.

Find the cube of $$-11$$.

Cube of $$-11$$ is:

$$(-11)^3=(-11) \times (-11) \times (-11)$$

$$=(-11) \times 121$$

$$=-1331$$.

Hence, the cube of $$-11$$ is $$-1331$$.

Prove that if a number is tripled then its cube is $$27$$ times the cube of the given number.

1869717_1671816_ans_c5f6f9c1f41740cdb6dcdbee9d0e5a3f.png

Find the cube of $$-21$$.

Cube of $$-21$$ is:

$$(-21)^3=(-21) \times (-21) \times (-21)$$

$$=(-21) \times 441$$

$$=-9261$$.

Hence, the cube of $$-21$$ is $$-9261$$.

Show that:
$$\sqrt[3]{27}\times \sqrt[3]{64}=\sqrt[3]{27\times 64}$$

$$\mathrm{LHS}=\sqrt[3]{27} \times \sqrt[3]{64}=\sqrt[3]{3 \times 3 \times 3} \times \sqrt[3]{4 \times 4 \times 4}=3 \times 4=12$$

$$\mathrm{RHS}=$$ $$\sqrt[3]{27 \times 64}=\sqrt[3]{3 \times 3 \times 3 \times 4 \times 4 \times 4}=\sqrt[3]{\{3 \times 3 \times 3\} \times\{4 \times 4 \times 4\}}=3 \times 4=12$$

Because $$\text{LHS}$$ is equal to $$\text{RHS}$$, the equation is true.

Which of the following numbers are not perfect cubes?
$$216$$

1934712_1671847_ans_35f3eaeee06c43e5bccdd36a67785743.PNG

By which smallest number must the following numbers be divided so that the quotient is a perfect cube?
$$35721$$

Prime factorising $$35721$$, we get,

$$ 35721= 3 \times 3 \times 3 \times 3 \times 3 \times 3 \times 7 \times 7$$

$$= 3 ^6 \times 7 ^2 $$.

We know, a perfect cube has multiples of $$3$$ as powers of prime factors.

Here, number of $$3$$'s is $$6$$ and number of $$7$$'s is $$2$$.

So we need to divide $$7^2$$ from the factorization to make $$35721$$ a perfect cube.

Hence, the smallest number by which $$35721$$ must be divided to obtain a perfect cube is $$7^2=49$$.

Evaluate: $$(3.5)^{3}$$

To evaluate: The cube of $$3.5$$

That is, $$(3.5)^{3}$$

For this, we need to multiply the given number three times i.e.

$$3.5\ \times\ 3.5\ \times\ 3.5 \ =\ {42.875}$$

Hence, $$(3.5)^3={42.875}$$.

Fill in the blanks:
$$\sqrt[3]{480}=\sqrt[3]{3}\times 2\times \sqrt[3]{\text{___}}$$.

Let the unknown number be $$t$$.

We know, prime factorisation of $$480$$:

$$480=3 \times 2 \times 2 \times 2 \times 2 \times 2 \times 5$$.

That is, $$480=3 \times 8 \times 20$$.

Then,

$$\sqrt[3]{480}=\sqrt[3]{3}\times 2\times \sqrt[3]{t}$$

$$\Rightarrow \sqrt[3]{20\times 3\times 8}=\sqrt[3]{3}\times 2\times \sqrt[3]{t}$$

$$=\sqrt[3]{3}\times \sqrt[3]{8}\times \sqrt[3]{20}=\sqrt[3]{3} \times 2\times \sqrt[3]{t}$$

$$\Rightarrow \sqrt[3]{3}\times 2\times \sqrt[3]{20}=\sqrt[3]{3}\times 2\times \sqrt[3]{t}$$

$$\therefore $$ On comparing, $${t=20}$$.

Fill in the blanks:
$$\sqrt[3]{1728}=4\times $$_____.

Given, $$\sqrt[3]{1728}$$ $$=4 \times$$ _____.

Prime factorising, we get,

$$1728= 2\times2\times2\times2\times2\times2\times3\times3\times3$$.

Then,

$$\sqrt[3]{1728}$$

$$=\sqrt[3]{\underline{2\times2\times2}\times \underline{2\times2\times2} \times \underline{3\times3\times3}}$$.

$$= 2\times 2\times 3$$

$$= 4\times 3$$.

On comparing,

$$3$$ is the answer.

Find the cube roots of the numbers $$57066625$$ using the fact that
$$57066625=166375\times 343$$.

1504646_1672129_ans_4df373488a93448b8df7934b74abd25e.jpg

Fill in the blanks:
$$\sqrt[3]{\text{_____}}=\sqrt[3]{4}\times \sqrt[3]{5}\times \sqrt[3]{6}$$

Let the unknown value be $$t$$.

Then,

$$\sqrt [ 3]{ t } =\sqrt [3 ]{ 4 } \times \sqrt [ 3 ]{ 5 } \times \sqrt [ 3 ]{ 6 } $$

$$\sqrt [ 3 ]{ t } =\sqrt [ 3 ]{ 120 } $$

$$\therefore {t=120}$$.

Find the cube roots of the numbers $$20346417$$ using the fact that
$$20346417=9261\times 2197$$.

1504678_1672102_ans_5423811c2130401eaf3d454421d2b0a0.jpg

Fill in the blanks:
$$\sqrt[3]{\text{___}}=\sqrt[3]{7}\times \sqrt[3]{8}$$.

Let the unknown number be $$t$$.

Then,

$$\sqrt [ 3]{t } =\sqrt [ 3 ]{ 7 } \times \sqrt [3 ]{ 8 } $$

$$\Rightarrow \sqrt [ 3 ]{ t } =\sqrt [ 3]{ 56 } $$

$$\therefore {t=56}$$

Find the cube roots of the numbers $$ 210644875$$ using the fact that
$$210644875=42875\times 4913$$.

1504680_1672103_ans_884512d2c8dc48a9bcbd776bc495f80e.jpg

Express each of the following as a rational number of the from $$ \dfrac{p}{q}$$:$$ \left ( \dfrac{7}{9} \right )^3 $$

1516280_1676218_ans_339806ece01849cab1f5a725b78f1a01.jpg

Fill in the blanks:
$$\sqrt[3]{125\times 27}=3\times $$ ______.

Given, $$\sqrt[3]{125\times 27}$$ $$=3 \times$$ _____.

Prime factorising, we get,

$$125= 5\times5\times5$$

and $$27= 3\times3\times3$$.

Then,

$$\sqrt[3]{125\times 27}$$

$$= \sqrt[3]{125}\times \sqrt[3]{27}$$.

$$= 5\times 3$$.
$$= 3\times 5$$.

Hence, $$5$$ is the answer.

Evaluate: $$(8)^{3}$$

To evaluate the cube of $$8$$:

We need to multiply the given number three times, that is,

$$8\ \times\ 8\ \times\ 8 \ =\ {512}$$

$$\therefore$$, the cube of $$8$$ is $${512}$$

Evaluate: $$\left(\cfrac{10}{11}\right)^{3}$$

To evaluate the cube of $$\cfrac{10}{11}$$ i.e $$\left(\cfrac{10}{11}\right)^{3}$$

We need to multiply the given number three times i.e. $$\cfrac{10}{11} \times\cfrac{10}{11} \times\cfrac{10}{11} \ =\ {\cfrac{1000}{1331}}$$

$$\therefore$$, the cube of $$\cfrac{10}{11}$$ is $$\cfrac{1000}{1331}$$

Evaluate: $$(60)^{3}$$

To evaluate the cube of $$60$$ i.e $$60^3$$:

We need to multiply the given number three times i.e.

$$60\ \times\ 60\ \times\ 60 \ =\ {216000}$$

$$\therefore$$, the cube of $$60$$ is $${216000}$$

Evaluate: $$\left(\cfrac4{7}\right)^{3}$$

To evaluate the cube of $$\cfrac4{7}$$ i.e $$\left(\cfrac4{7}\right)^{3}$$:

We need to multiply the given number three times i.e. $$\left(\cfrac4{7}\right) \times\ \left(\cfrac4{7}\right)\times \left(\cfrac4{7}\right) \ =\ {\left(\cfrac{64}{343}\right)}$$

$$\therefore$$, the cube of $$\left(\cfrac4{7}\right)$$ is $$\left(\cfrac{64}{343}\right)$$

Evaluate: $$(0.05)^{3}$$

To evaluate the cube of $$0.05$$ i.e $$(0.05)^{3}$$:

We need to multiply the given number three times i.e. $$0.05\ \times\ 0.05\ \times\ 0.05 \ =\ {0.000125}$$

Now, we have to convert the given number into fraction we get,

$$\dfrac{125}{1000000}=\dfrac{1}{8000}$$

$$\therefore$$, the cube of $$0.05$$ is $${0.000125}$$ or $$\cfrac{1}{8000}$$

Evaluate: $$(0.8)^{3}$$

To evaluate the cube of $$0.8$$ i.e $$(0.8)^{3}$$:

We need to multiply the given number three times i.e. $$0.8\ \times\ 0.8\ \times\ 0.8 \ =\ {0.512}$$

Now, we have to convert the given number into fraction we get,$$\dfrac{512}{1000}=\dfrac{64}{125}$$

$$\therefore$$, the cube of $$0.8$$ is $${0.512}$$ or $$\dfrac{64}{125}$$

Is $$8000$$ a perfect cube? In case of perfect cube, find the number whose cube it is.

A perfect cube can be expressed as a product of three same numbers

Now by resolving the given number into prime factors we get,

$$8000\ =\ 2\ \times\ 2\ \times\ 2\ \times\ 2\ \times\ 2\ \times\ 2\ \times\ 5\ \times\ 5\ \times\ 5$$

As we can group the above factors into three groups of cubes i.e. $$2^{3}$$, $$2^{3}$$, $$5^{3}$$

So, we express it as, $$2^{3}\ \times\ 2^{3}\ \times\ 5^{3}\ =\ 20^{3}$$

$$\therefore$$ $$8000$$ is a perfect cube.

Evaluate: $$\left(\cfrac{1}{15}\right)^{3}$$

To evaluate the cube of $$\cfrac1{15}$$ i.e $$\left(\cfrac{1}{15}\right)^{3}$$:

We need to multiply the given number three times i.e. $$\cfrac1{15}\times\cfrac1{15} \times\cfrac1{15} \ =\ {\cfrac1{3375}}$$

$$\therefore$$, the cube of $$\cfrac1{15}$$ is $${\cfrac1{3375}}$$

Is $$3375$$ a perfect cube? In case of perfect cube, find the number whose cube it is.

A perfect cube can be expressed as a product of three same numbers.

We can see that the SUM of the digits(i.e, 18) of the given number is divisible by 3 and also it is ending with 5 therefore it is divisible by 5 also.

Now by resolving the given number into prime factors we get,

$$3375\ = 3\times 5 \times 225 = 3\times 5\times 15\times 15 = 3\times 3 \times 3 \times 5 \times 5 \times 5$$

As we can group the above factors into groups of three i.e. $$3^{3}$$, $$5^{3}$$

We express it as, $$3^{3}\ \times\ 5^{3}=15^3$$

$$\therefore$$ $$3375$$ is a perfect cube of $$15$$.

Is $$125$$ a perfect cube? In case of perfect cube, find the number whose cube it is.

A perfect cube can be expressed as a product of three same numbers

Now by resolving the given number into prime factors we get,

$$125\ =\ 5\ \times\ 5\ \times\ 5$$

So, It is a perfect cube and can be expressed as $$5^{3}$$

$$\therefore$$ $$125$$ is a perfect cube.

Evaluate: $$\left(\cfrac{13}{15}\right)^{3}$$

To evaluate the cube of $$\cfrac{13}{15}$$ i.e $$\left(\cfrac{13}{15}\right)^{3}$$:

We need to multiply the given number three times i.e. $$\cfrac{13}{15}\times\cfrac{13}{15} \times\cfrac{13}{15} \ =\ {\cfrac{2197}{3375}}$$

$$\therefore$$, the cube of $$\cfrac{13}{15}$$ is $${\cfrac{2197}{3375}}$$

Is $$5324$$ a perfect cube? In case of perfect cube, find the number whose cube it is.

A perfect cube can be expressed as a product of three same numbers

Now by resolving the given number into prime factors we get,

$$5324\ =\ 2\ \times\ 2\ \times\ 11\ \times\ 11\ \times\ 11$$

Since, the given number does not have cubical value i.e we cannot group its factors into pair of three same numbers.

$$\therefore$$ $$5324$$ is not a perfect cube.

Is $$9261$$ a perfect cube? In case of perfect cube, find the number whose cube it is.

A perfect cube can be expressed as a product of three same numbers

Now by resolving the given number into prime factors we get,

$$9261\ =\ 3\ \times\ 3\ \times\ 3\ \times\ 7\ \times\ 7\ \times\ 7$$

As we can group the above factors into three groups of cubes i.e. $$3^{3}$$, $$7^{3}$$

So, we express it as, $$3^{3}\ \times\ 7^{3}=21^3$$

$$\therefore$$ $$9261$$ is a perfect cube.

Is $$243$$ a perfect cube? In case of perfect cube, find the number whose cube it is.

A perfect cube can be expressed as a product of three same numbers

Now by resolving the given number into prime factors we get,

$$243\ =\ 3\ \times\ 3\ \times\ 3\ \times\ 3\ \times\ 3$$

Since the given number has more than three factors i.e its prime factors cannot be grouped into pairs of three same numbers.

$$\therefore$$ $$243$$ is not a perfect cube.

Is $$343$$ a perfect cube? In case of perfect cube, find the number whose cube it is.

A perfect cube can be expressed as a product of three same numbers.

Now by resolving the given number into prime factors we get,

$$343\ =\ 7\ \times\ 7\ \times\ 7$$

So, It is a cube and can be expressed as $$7^{3}$$

$$\therefore$$ $$343$$ is a perfect cube.

Evaluate $$\sqrt[3]{343}$$

The prime factors of $$343 = 7\times7\times7$$

Now by grouping into three we get, $$(7\times7\times7)$$

$$\therefore$$ $$\sqrt[3]{(7)^{3}}=7\\$$

$$\therefore$$ $$\sqrt[3]{343}=7$$

Evaluate $$\sqrt[3]{1728}$$

The prime factors of $$1728 = 2\times2\times2\times2\times2\times2\times3\times3\times3$$

Now by grouping into three we get, $$(2\times2\times2)\times(2\times2\times2)\times(3\times3\times3)$$

$$\therefore$$ $$\sqrt[3]{((2)^{3}\times(2)^{3}\times(3)^{3})} =(2\times2\times3)=12\\$$

$$\therefore$$$$\sqrt[3]{1728}=12$$

Evaluate $$\sqrt[3]{9261}$$

The prime factors of $$9261 = 3\times3\times3\times7\times7\times7$$

Now by grouping into three we get, $$(3\times3\times3)\times(7\times7\times7)$$

$$\therefore$$ $$\sqrt[3]{((3)^{3}\times(7)^{3})} =(3\times7)=21$$

$$\therefore$$ $$\sqrt[3]{9261}=21$$

Evaluate $$\sqrt[3]{8000}$$

The prime factors of $$8000 = 2\times2\times2\times2\times2\times2\times5\times5\times5$$

Now by grouping into three we get, $$(2\times2\times2)\times(2\times2\times2)\times(5\times5\times5)$$

$$\therefore$$ $$\sqrt[3]{((2)^{3}\times(2)^{3}\times(5)^{3})} =(2\times2\times5)=20$$

$$\therefore$$$$\sqrt[3]{8000}=20$$

Evaluate $$\sqrt[3]{3375}$$

The prime factors of $$3375 = 3\times3\times3\times5\times5\times5$$

Now by grouping into three we get, $$(3\times3\times3)\times(5\times5\times5)$$

$$\Rightarrow \sqrt[3]{((3)^{3}\times(5)^{3})} =(3\times5)\\\ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ =15\\$$

$$\therefore$$ $$\sqrt[3]{3375}=15$$

Evaluate $$\sqrt[3]{4096}$$

The prime factors of $$4096 = 2\times2\times2\times2\times2\times2\times2\times2\times2\times2\times2\times2$$

Now by grouping into three we get, $$(2\times2\times2)\times(2\times2\times2)\times(2\times2\times2)\times(2\times2\times2)$$

$$\therefore$$ $$\sqrt[3]{((2)^{3}\times(2)^{3}\times(2)^{3}\times(2)^{3})} =(2\times2\times2\times2)=16\\$$

$$\therefore$$$$\sqrt[3]{4096}=16$$

Evaluate $$\sqrt[3]{729}$$

The prime factors of $$729 = 3\times3\times3\times3\times3\times3$$

Now by grouping into three we get, $$(3\times3\times3)\times(3\times3\times3)$$

$$\therefore$$ $$\sqrt[3]{((3)^{3}\times(3)^{3})} =(3\times3)=9$$

$$\therefore$$ $$\sqrt[3]{729}=9$$

Evaluate:
$$ (1.5)^3 $$

The above question can be written as, $$ (1.5) \times ( 1.5) \times (1.5) $$

First we find the product, $$ (15 ) \times (15) = 225 $$

$$ \therefore 15\times 15\times 15= (225) \times (15) = 3375 $$

Total number of decimal places in the given product $$ =3 $$

So, the product must contain $$3$$ places of decimals

$$ \therefore (1.5)^3 = 3.375 $$

Evaluate:
$$ ( 0.05)^3 $$

The above question can be written as, $$ (0.05) \times ( 0.05) \times (0.05) $$

First we find the product, $$ (5 ) \times (5) = 25 $$

$$\therefore 5\times 5\times 5 = (25) \times (5) = 125 $$

Total number of decimal places in the given product $$ =6 $$

So, the product must contain $$6$$ places of decimals

$$ \therefore (0.05)^3 = 0.000125 $$

Fill in the blanks to make the statements true.
There are ________ perfect cubes between 1 and 1000.

Sr. No.	Number	Working	Cube
1	2	$$2\times 2\times 2$$	8
2	3	$$3\times 3\times 3$$	27
3	4	$$4\times 4\times 4$$	64
4	5	$$5\times 5\times 5$$	125
5	6	$$6\times 6\times 6$$	216
6	7	$$7\times 7\times 7$$	343
7	8	$$8\times 8\times 8$$	512
8	9	$$9\times 9\times 9$$	729

There are $$\underline{8}$$ perfect cubes between 1 and 1000.

Evaluate:
$$ ( 0.3)^3 $$

The above question can be written as, $$ (0.3) \times ( 0.3) \times (0.3) $$

First we find the product, $$ (3 ) \times (3) = 9 $$

$$ \therefore 3\times 3\times 3= (9) \times (3) = 27 $$

Total number of decimal places in the given product$$ =3 $$

So, the product must contain $$3$$ places of decimals

$$ \therefore (0.3)^3 = 0.027 $$

Fill in the blanks to make the statements true.
The cube of 100 will have _______ zeroes.

The cube of 100 will have $$\underline{6}$$ zeroes.
$$(100)^3 = 100 \times 100 \times 100 = 1000000$$

Fill in the blanks to make the statements true.
The least number by which 72 be divided to make it a perfect cube is _________.

The least number by which 72 be divided to make it a perfect cube is $$\underline{9}$$.
Factors of 72 = $$ 2 \times 2 \times 2 \times 3 \times 3$$
By grouping the factors in triplets of equal factors,
$$72 = 2 \times 2 \times 2 \times 3 \times 3$$
Here, if we divide 72 by $$3 \times 3$$
The quotient would be $$2 \times 2 \times 2 $$, that is a perfect cube.
Now,
So, the least number by which 72 be divided to make it, a perfect cube.
1649380_1792018_ans_17ec275a1b91475db7163a2c343ca874.png

1649380_1792018_ans_17ec275a1b91475db7163a2c343ca874.png

Fill in the blanks to make the statements true.
The least number by which 72 be multiplied to make it a perfect cube is __________.

The least number by which 72 be multiplied to make it a perfect cube is $$\underline{3}$$.
Factors of 72 = $$ 2 \times 2 \times 2 \times 3 \times 3$$
By grouping the factors in triplets of equal factors,
$$72 = 2 \times 2 \times 2 \times 3 \times 3$$
Here, 3 = without a pair
So, we have to multiply the number by 3
1649373_1792015_ans_6240263254fa497bad9b1a285b9a6b09.png

1649373_1792015_ans_6240263254fa497bad9b1a285b9a6b09.png

Fill in the blanks to make the statements true.
Cube of a number ending in $$7$$ will end in the digit _________.

Cube of a number ending in $$7$$ will end with the digit $$\underline{3}$$.
Cube of a number ending in $$ 7 = 7 \times 7 \times 7 = 343$$

Write cubes of first three multiples of 3.

first three multiples of 3 are 3, 6 and 9
Cube of $$3 = 3 \times 3 \times 3 = 27$$
Cube of $$6 = 6 \times 6 \times 6 = 216$$
Cube of $$9 = 9 \times 9 \times 9 = 729$$

Find the product of the cube of $$(-2)$$ and the square of $$(+4)$$.

Since, cube of $$(-2)=(-2)^3=-8$$
And square of $$(+4)=(+4)^2=16$$
Therefore, the product $$=(-2)^3 \times (4)^2=(-8)\times 16=-128$$

$$\Big \{\Big[5^2 + (12^2)^{\frac{1}{2}}\Big]\Big \}^3$$

As per question,
$$\Big \{\Big[5^2 + (12^2)^{\frac{1}{2}}\Big]\Big \}^3 = \{25 + 12\}^3 = (37)^3 = 50653$$

Is 9720 a perfect cube? If not, find the smallest number by which it should be divided to get a perfect cube.

Difference of two perfect cubes isIf the cube root of the smaller of the two numbers is 3, find the cube root of the larger number.

Given, Difference of two perfect cubes is 189.
The cube root of the smaller of the two numbers is 3.
So, cube of smaller number = $$(3)^3 = 27$$
Suppose the cube root of the larger number = z
Then, cube of the larger number = $$z^3$$
As per question,
$$z^3 - 27 = 189$$
or $$z^3 =189 + 27$$
or $$z^3 = 216$$
or $$z = 6$$
So, the cube root of the larger number = 6

Find the smallest number by which the following number must be divided to obtain a perfect cube:
$$1536$$

We have,
$$1536 = 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 3$$
After grouping the prime factors in triplets, it’s seen that one factor 3 is left without grouping.
$$1536 = (2 \times 2 \times 2) \times (2 \times 2 \times 2) \times (2 \times 2 \times 2) \times 3$$
So, in order to make it a perfect cube, it must be divided by 3.
Thus, the smallest number by which 1536 must be divided to obtain a perfect cube is 3.

Using prime factorisation, find the cube roots of
a) 512
b) 2197

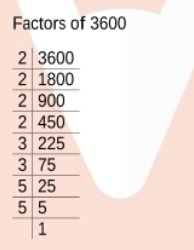
By what smallest number should 3600 be multiplied so that the quotient is a perfect cube? Also find the cube root of the quotient.

$$3600 = \underline{2 \times 2 \times 2} \times 3 \times 3 \times 5 \times 5$$
As we observe, 2, 3 and 5 do not have a pair to make a cube. So, 3600 is not a perfect cube.
To make 3600 a perfect cube, we have to multiply 3600 by
$$2 \times 2 \times 3 \times 5 = 60$$
1653240_1792399_ans_16c51477e3334a8ca9c1dac7ab7371a0.PNG

1653240_1792399_ans_16c51477e3334a8ca9c1dac7ab7371a0.PNG

Find the smallest number by which the following number must be multiplied to obtain a perfect cube:
$$3072$$

Given number is $$3072$$.

It can be represented as the product of its prime factors as:
$$3072 = 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 3$$
After grouping the prime factors in triplets, we get:
$$3072 = (2 \times 2 \times 2 \times 2) \times (2 \times 2 \times 2) \times (2 \times 2 \times 2) \times 2 \times 3$$

We can see that factors $$2 \times 3$$ are left ungrouped.
So, in order to complete them in a group of $$3’s$$ we need the factors $$2 \times 2 \times 3 \times 3$$ to be multiplied.
That is, the factors needed are $$2 \times 2 \times 3 \times 3$$

Their product $$ = 36$$

Hence, the smallest number which should be multiplied to $$3072$$ in order to make it a perfect cube is $$36$$.

Find the smallest number by which each of the following numbers must be multiplied to obtain a perfect cube:
$$19652$$

We have,
$$19652 = 2 \times 2 \times 17 \times 17 \times 17$$
After grouping the prime factors in triplets, it’s seen that factors $$2 \times 2$$ are left ungrouped in 3’s.
$$19652 = 2 \times 2 \times (17 \times 17 \times 17)$$
So, in order to complete it in a triplet one more 2 is needed.
Thus, the smallest number which should be multiplied to 19652 in order to make it a perfect cube is 2.

Using prime factorization, find which of the following are perfect cubes.
a) $$128$$
b) $$343$$
c) $$729$$
d) $$1331$$

a) Factors of $$128,$$

$$128 = \underline{2\times 2 \times 2}\times \underline{2 \times 2 \times 2}\times 2$$

As grouping in triplets, $$2$$ is not grouped.

So, $$128$$ is not a perfect cube.

b) Factors $$343,$$

$$343 = \underline{7\times 7 \times 7}$$

As grouping in triplets, all the numbers are grouped.

So, $$343$$ is a perfect cube.

c) Factors of $$729,$$

$$729 = \underline{3\times 3 \times 3}\times \underline{3\times 3 \times 3}$$

As grouping in triplets, all the numbers are grouped.

So, $$729$$ is a perfect cube.

d) Factors of $$1331,$$

$$1331 = 11 \times 11 \times 11$$

As grouping in triplets, all the numbers are grouped.

So, $$1331$$ is a perfect cube.

1650290_1792332_ans_25f277065eda44759f7618014beb1211.png

Find the smallest number by which the following number must be divided to obtain a perfect cube:
$$28672$$

We have,
$$28672 = 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 7$$
After grouping the prime factors in triplets, it’s seen that one factor 7 is left without grouping.
$$28672 = (2 \times 2 \times 2) \times (2 \times 2 \times 2) \times (2 \times 2 \times 2) \times (2 \times 2 \times 2) \times 7$$
So, it must be divided by 7 in order to get a perfect cube.
Thus, the required smallest number is 7.

Find the smallest number by which the following number must be divided to obtain a perfect cube:
$$10985$$

We have,
$$10985 = 5 \times 13 \times 13 \times 13$$
After grouping the prime factors in triplet, it’s seen that one factor 5 is left without grouping.
$$10985 = 5 \times (13 \times 13 \times 13)$$
So, it must be divided by 5 in order to get a perfect cube.
Thus, the required smallest number is 5.

Write the one's digit of the cube of the following number:
$$419$$

We know that,
The cube of number having $$1, 4, 5, 6$$ or $$9$$ in unit place will end in $$1, 4, 5, 6$$ or $$9$$
And, if the numbers have:
$$2$$ in unit place, then it’s cube ends in $$8$$
$$8$$ in unit place, then it’s cube ends in $$2$$
$$3$$ in unit place then it’s cube ends in $$7$$
$$7$$ in unit place then it’s cube ends in $$3$$
$$0$$ in unit place then it’s cube ends in $$0.$$
So now,
Unit digit of number $$419$$ is $$9,$$ hence its cube will end in $$9.$$

Write the one's digit of the cube of the following number:
$$987$$

Write the one's digit of the cube of the following number:
$$231$$

Which of the following are cubes of even natural numbers or odd natural numbers:
(i) $$125$$
(ii) $$512$$
(iii) $$1000$$
(iv) $$2197$$
(v) $$4096$$
(vi) $$6859$$

We know that,
The cube of an even number is even and the cube of an odd number is odd.
Hence,
$$125, 2197, 6859$$ are cubes of an odd number and $$512, 1000, 4096$$ are cubes of an even number.

Write the one's digit of the cube of the following number:
$$854$$

Write the one's digit of the cube of the following number:
$$358$$

1960922_1806833_ans_e0e61faad1e84717937e1aad15fab34f.jpg

Find the smallest number by which the following number must be divided to obtain a perfect cube:
$$13718$$

$$13718 = 2 \times 19 \times 19 \times 19$$
After grouping the prime factors in triplets, it’s seen that one factor 2 is left without grouping.
$$13718 = 2 \times (19 \times 19 \times 19)$$
So, it must be divided by $$2$$ in order to get a perfect cube.
Thus, the required smallest number is $$2.$$

Write the one's digit of the cube of the following number:
$$725$$

Find the cube root of the following numbers by prime factorization:
$$42875$$

$$\displaystyle \sqrt[3]{42875}$$
$$= \sqrt[3]{5 \times 5 \times 5 \times 7 \times 7 \times 7}$$
$$= 3 \times 7 = 35$$
Thus, the cube root of $$42875$$ is $$35.$$

Write the one's digit of the cube of the following number:
$$752$$

Find the cube root of the following numbers by prime factorization:
$$262144$$

$$\sqrt[3]{262144}$$
$$= \sqrt[3]{2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2}$$
$$= 2 \times 2 \times 2 \times 2 \times 2 \times 2 = 64$$
Thus, the cube root of $$262144$$ is $$64.$$

Find the cube root of the following numbers by prime factorization:
$$21952$$

$$\sqrt[3]{21952}$$
$$= \sqrt[3]{2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 7 \times 7 \times 7}$$
$$= 2 \times 2 \times 7 = 28$$
Thus, the cube root of $$21952$$ is $$28.$$

Find the cube root of the following numbers by prime factorization:
$$373248$$

$$\sqrt[3]{373248}$$
$$= \sqrt[3]{2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 3 \times 3 \times 3 \times 3 \times 3 \times 3}$$
$$= 2 \times 2 \times 2 \times 3 \times 3 = 72$$
Thus, the cube root of $$373248$$ is $$72.$$

Multiply $$6561$$ by the smallest number so that product is a perfect cube. Also, find the cube root of the product.

Performing prime factorization of $$6561,$$
we get
$$6561 = 3 \times 3 \times 3 \times 3 \times 3 \times 3 \times 3 \times 3$$
$$6561 = (3 \times 3 \times 3) \times (3 \times 3 \times 3) \times 3 \times 3$$
After grouping of the equal factors in 3’s, it’s seen that $$3 \times 3$$ is left ungrouped in 3’s.
In order to complete it in triplet, we should multiply it by 3.
Hence, required smallest number $$= 3$$
and cube root of the product $$= 3 \times 3 \times 3 = 27$$
1760578_1806881_ans_9714aa23767d40d4975cf0202e3c6481.png

1760578_1806881_ans_9714aa23767d40d4975cf0202e3c6481.png

Find the cube of :
$$7$$

Hint : A cube number is a result when a number has been multiplied by itself twice.

Step 1: To find the cube of 7

The cube of 7 is $$7^{3}$$

$$ \Rightarrow7 \times 7 \times 7 \\$$

$$\Rightarrow343$$

Therefore, required answer is $$343$$.

Find the cube root of the following numbers by prime factorisation:
$$59319$$

Thus, the cube root of $$59319$$ is $$39.$$
1760620_1806896_ans_b39dab9de35d410eb4243c7408c2f4ea.png

Find the cube root of the following numbers by prime factorization:
$$12167$$

Given: $$12167$$

To find = $$\sqrt[3]{12167}$$
$$= \sqrt[3]{23 \times 23 \times 23}$$
$$= (23^3)^\dfrac{1}{3} = 23^{\dfrac{1}{3} \times 3}$$
$$= 23^1 = 23$$
Thus, the cube root of $$12167$$ is $$23.$$

Write the one's digit of the cube of the following number:
$$893$$

We know that,
The cube of number having $$1, 4, 5, 6$$ or $$9$$ in unit place will end in $$1, 4, 5, 6$$ or $$9$$
And, if the numbers have:
$$2$$ in unit place, then it’s cube ends in $$8$$
$$8$$ in unit place, then it’s cube ends in $$2$$
$$3$$ in unit place then it’s cube ends in $$7$$
$$7$$ in unit place then it’s cube ends in $$3$$
$$0$$ in unit place then it’s cube ends in $$0.$$
So now,
Unit digit of numbers $$893$$ is $$3,$$ hence its cube will end in $$7.$$

Find the cube roots of:
$$ 9261 $$

$$ 9261 = \sqrt[3]{9261} = (3 \times 3 \times 3) \times (7 \times 7 \times 7) $$
$$ = 3 \times 7 = 21 $$
1780353_1837712_ans_1bfa8515f0db4ad2b7153b76afcd1e18.png

Find the cube-roots of:
$$ 64 \times 729$$

$$ 64 \times 729 = \sqrt[3]{64 \times 729} = \sqrt[3]{4 \times 4 \times 4 \times 9 \times 9 \times 9} = 4 \times 9 = 36 $$

Find the cube roots of:
$$ 4096$$

$$ 4096 = \sqrt[3]{4096} = (2 \times 2 \times 2) \times (2 \times 2 \times 2) \times (2 \times 2 \times 2) \times (2 \times 2 \times 2) $$
$$ = 2 \times 2 \times 2 \times 2 = 16 $$
1780354_1837713_ans_b67027911fbb46b98ec5aa5378574138.png

1780354_1837713_ans_b67027911fbb46b98ec5aa5378574138.png

Find the cube roots of:
$$729$$

$$ 729 = \sqrt[3]{729} = (3 \times 3 \times 3) \times (3 \times 3 \times 3) $$
$$ = 3 \times 3 = 9 $$
1779660_1837382_ans_14dbed9c3aaa4d3a9dbde11cbd21d3ce.png

Find that the number is a perfect cube or not ?
$$1331$$

$$1331$$
Taking L.C.M
$$ \because \, 1331 = 11 \times 11 \times 11 = (11)^3 $$
$$ \therefore \, 1331 $$ is a perfect cube.

1779623_1837217_ans_87aaea3bfc8d47f0924bf87d0ef9a526.png

1779623_1837217_ans_87aaea3bfc8d47f0924bf87d0ef9a526.png

Find that the number is a perfect cube or not ?
$$1938$$

$$1938$$
Taking L.C.M.
$$ 1938 = 2 \times 3 \times 17 \times 19 $$
$$ 1938 $$ is not a perfect cube.
1779629_1837239_ans_d86ee55717974aa6918be1dc5bb09ac4.png

Find the cube roots of:
$$1728$$

$$ 1728 = \sqrt[3]{1728} = (2 \times 2 \times 2) \times (2 \times 2 \times 2) \times (3 \times 3 \times 3) = 2 \times 2 \times 3 = 12 $$
1779664_1837383_ans_2322b81f4eff4063967948f4f8c99a36.png

1779664_1837383_ans_2322b81f4eff4063967948f4f8c99a36.png

Find the cube roots of:
$$ 3375 $$

$$ 3375 = \sqrt[3]{3375} = (5 \times 5 \times 5) \times (3 \times 3 \times 3) $$
$$ = 5 \times 3 = 15 $$
1780358_1837716_ans_c6d81a7417ba433b8e3a466d8e4672c4.png

Find that the number is a perfect cube or not ?
$$1728$$

$$1728$$
Taking L.C.M.
$$ \because \, 1728 = 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 3 \times 3 \times 3 $$
$$ = (2)^3 \times (2)^3 \times (3)^3 $$
$$ \therefore \, 1728 $$ is a perfect cube.

1779625_1837237_ans_07614f9db5c14b4dbf797b8473835e8e.png

1779625_1837237_ans_07614f9db5c14b4dbf797b8473835e8e.png

Find that the number is a perfect cube or not ?
$$24000$$

$$24000$$

$$ \because \, 24000 = 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 3 \times 5 \times 5 \times 5 $$

$$ = (2)^3 \times (2)^3 \times (5)^3 \times 3 $$

$$ \therefore \, 24000$$ is not a perfect cube.

What is the cube of 8?

$$\textbf{Step 1 : Write Cube as multiply by itself three times}$$

$$\text{To find the value of 8 cube, determine the value of}$$ $$ 8 \times 8 \times 8 $$
$$8 \times 8 \times 8= $$ $$64 \times 8 $$
$$ = 512$$

$$\textbf{Hence, } \boldsymbol{8^3=512} $$

Which of the following are cubes of ?
(i) An even number
(ii) An odd number
$$ 216 , 729 , 3375 , 8000 , 125 , 343 , 4096 $$ and $$ 9261$$

(Refer Fig.1) $$ 216 = 2 \times 2 \times 2 \times 3 \times 3 \times 3 $$
$$ = (2)^3 \times (3)^3 = (6)^3 $$

(Refer Fig.2) $$ 729 = 3 \times 3 \times 3 \times 3 \times 3 \times 3 $$
$$ = (3)^3 \times (3)^3 = (9)^3 $$

(Refer Fig.3) $$ 3375 = 5 \times 5 \times 5 \times 3 \times 3 \times 3 $$
$$ = (5)^3 \times (3)^3 = (15)^3 $$

(Refer Fig.4) $$ 8000 = 20 \times 20 \times 20 = (20)^3 $$

(Refer Fig.5) $$125 = 5 \times 5 \times 5 = (5)^3 $$

(Refer Fig.6) $$ 343 = 7 \times 7 \times 7 = (7)^3 $$

(Refer Fig.7) $$ 4096 = 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2 $$
$$ = (2)^3 \times (2)^3 \times (2)^3 \times (2)^3 $$
$$ = (16)^3 $$

(i) Cubes of an even number are $$ 216 , 8000 , 4096 $$
(ii) Cubes of an odd number are $$ 729 , 3375 , 125 , 343 , 9261 $$
1780466_1837964_ans_3b74d73753be47f59e579d2f0f03c63a.png

1780466_1837964_ans_3b74d73753be47f59e579d2f0f03c63a.png

Find the smallest number by which $$ 8768$$ must be divided so that the quotient is a perfect cube.

The prime factor of $$8768$$ are
$$ = 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 137 $$
$$ = (2 \times 2 \times 2) \times (2 \times 2 \times 2) \times 137 $$
Clearly , $$ 8768 $$ must be divided by $$137$$
1780469_1837979_ans_98b2d2043e7d492db897e3d80915c1a1.png

1780469_1837979_ans_98b2d2043e7d492db897e3d80915c1a1.png

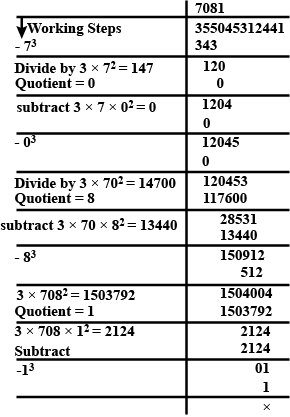
Find the cube root of $$355045312441$$ perfect cube number, by division method.

1956501_1875610_ans_8581af025dfa40c1bc8711ef7f5f8b17.png

Find the cube root by prime factorization.
$$3375$$

$$3375 = 5 \times 5 \times 5 \times 3 \times 3 \times 3 = 15 \times 15 \times 15 $$
$$ \sqrt[3]{3375} = 15 $$
1842748_1870156_ans_abda0b07338946b590ab3d165127fa33.png

Find the cube-roots of:
$$ 3375 \times 512 $$

$$ 3375 \times 512 = \sqrt[3]{3375 \times 512} $$
$$ = \sqrt[3]{15 \times 15 \times 15 \times 8 \times 8 \times 8} $$
$$ = 15 \times 8 = 120 $$

Find the cube-roots of:
$$ 729 \times 8000$$

$$ 729 \times 8000 = \sqrt[3]{729 \times 8000} = \sqrt[3]{9 \times 9 \times 9 \times 20 \times 20 \times 20} $$
$$ = 9 \times 20 = 180$$

Find the cube-roots of:
$$ -125 \times 1000$$

$$ -125 \times 1000 $$
$$( -125 \times 1000)^{1/3} = \sqrt[3]{-125 \times 100} $$
$$ = \sqrt[3]{- (5 \times 5 \times 5) \times (10 \times 10 \times 10)} $$
$$ = -5 \times 10 = -50 $$

Find the cube root of the following number by prime factorization method:
$$729$$

$$\therefore 729=3\times 3\times 3\times 3\times 3\times 3\\ \therefore \sqrt [ 3 ]{ 729 } =3\times 3=9$$

$$\therefore$$ cube root of $$729=3\times3=9$$

Find the cube root of the following number by prime factorization method:
$$1331$$

$$\therefore 1331=11\times 11\times 11\\ \therefore \sqrt [ 3 ]{ 1331 } =11$$

$$\therefore$$ cube root of $$1331$$ is $$11$$.

Find the cube root of $$4096.$$

On prime factorising, we get,

$$4096=\underline{2\times 2\times 2}\times \underline{2\times 2\times 2}\times \underline{2\times 2\times 2}\times \underline{2\times 2\times 2}$$

$$=2^3\times 2^3\times 2^3\times 2^3$$.

Then, cube root of $${4096}$$ is:

$$\sqrt[3]{4096}=\sqrt[3]{2^3 \times 2^3 \times 2^3 \times 2^3}= 2\times2\times2\times2=16$$.

Therefore, $$16$$ is the required solution.

Test whether the given number is perfect cube or not: $$516$$

Doing a prime factorization, we get

$$516= 3\times 43 \times 2 \times 2$$

$$\therefore 516 $$ is not a perfect cube.

Test whether the given number is perfect cube or not: $$729$$

$$\therefore 729=\underline {3\times 3\times 3} \times \underline {3\times 3\times 3}\\ \therefore \sqrt [ 3 ]{ 729 } =3\times 3=9$$

$$\therefore$$ $$729$$ is a perfect cube.

Test whether the given number is perfect cube or not: $$8000$$

$$8000=\underline {2\times 2\times 2} \times \underline {10\times 10\times 10} \\ \therefore \sqrt [ 3 ]{ 8000 } =2\times 10=20$$

$$\therefore$$ $$8000$$ is a perfect cube

Find the cube root of the given number through estimation: $$5832$$

For calculating the cube root of $$5832$$, this number has to be separated in two group, i.e. $$3$$ and $$832$$.

considering the group $$832$$:

$$832$$ ends with $$2$$ and we know that if digit $$2$$ is at the end of any perfect cube number, then its cube root will have $$8$$ at its unit place only. Therefore, the digits at the units place of the required cube root is taken as $$8$$.

Now consider the other group $$3$$:

We know that the $${ 1 }^{ 3 }=1$$ and $${ 2}^{ 3 }=8$$

Also, $$1<5<8$$

So, 1 will be taken at the tens place. So, the required cube root of 5832 is 18.

$$\sqrt [ 3 ]{ 5832} =18$$

Write the cubes of all natural numbers between $$1$$ and $$10$$ and verify the following statements:
Cubes of all even natural numbers are even.

1540037_1671759_ans_834b0f5e4f8d400b8ff04db67b597e74.jpeg

Find the cube root of the given number through estimation:$$2197$$

For calculating the cube root of $$2197$$, this number has to be separated in two group, i.e. $$2$$ and $$197$$.

Considering the group $$197$$:

$$197$$ ends with $$7$$ and we know that if digit $$7$$ is at the end of any perfect cube number, then its cube root will have $$3$$ at its unit place only. Therefore, the digits at the units place of the required cube root is taken as $$3$$.

Now consider the other group $$2$$:

We know that the $${ 1 }^{ 3 }=1$$ and $${ 2}^{ 3 }=8$$

Also, $$1^3<2<2^3$$

So, $$1$$ will be taken at the tens place. So, the required cube root of $$2197$$ is $$13$$.

$$\sqrt [ 3 ]{ 2197 } =13$$

for all cuboids with square base and given volume prove that cube has minimum surface area.

You are told that $$1,331$$ is a perfect cube. Can you guess without factorization what is its cube root? Similarly, guess the cube roots of $$4913,12167,32768$$.

(i) Separating the given number (1331) into two groups:

$$1331\rightarrow 1$$ and $$331$$

$$\because$$ 331 ends in 1

$$\therefore$$ Unit's digit of the cube root $$=1$$

$$\because1^3=1$$ and $$\sqrt[1]{1}$$

$$\therefore$$ Ten's digit of the cube root $$=1$$

$$\therefore\sqrt[3]{1331}=11$$

(ii) Separating the given number (4913) into two groups:

$$4913\rightarrow 4$$ and $$931$$

Unit's digit:

$$\because$$ Unit's digit in 913 is 3

$$\therefore$$ Unit's digit of the cube root $$=7$$

$$\because7^3=343$$ which end in 3

Ten's digit:

$$\because 1^3=1,\ 2^3=8$$

and $$1<4<8$$

i.e $$1^3<4<2^3$$

$$\therefore$$ Ten's digit of the cube root is 1

$$\therefore\sqrt[3]{4913}=17$$

(iii) Separating the given number (12167) into two groups:

$$12167\rightarrow 12$$ and $$167$$

Unit's digit:

$$\because$$ 167 is ending in 7 and cube of a number ending in 3 ends in 7

$$\therefore$$ Unit's digit of the cube root $$=3$$

Ten's digit:

$$\because 2^3=8,\ 3^3=27$$

and $$8<12<27$$

i.e $$2^3<12<3^2$$

$$\therefore$$ Ten's digit of the cube root is 2

$$\therefore\sqrt[3]{121367}=23$$

(iv) Separating the given number (32768) into two groups:

$$32768\rightarrow 32$$ and $$786$$

Unit's digit:

768 will guess the unit's digit in the cube root.

$$\because$$ 768 ends in 8

$$\therefore$$ Unit's digit of the cube root $$=2$$

Ten's digit:

$$\because 3^3=27,\ 4^3=64$$

and $$27<32<64$$

i.e $$3^3<32<4^3$$

$$\therefore$$ Ten's digit of the cube root is 3

$$\therefore\sqrt[3]{32768}=32$$

By taking three different values of $$n$$ verify the truth of the following statements:
If $$n$$ is even, then $$n^{3}$$ is also even.

2039791_1671855_ans_b1955be5d3534fa586580152b2e08556.jpg

By taking three different values of $$n$$ verify the truth of the following statements:
If $$n$$ is odd, then $$n^{3}$$ is also odd.

2039792_1671856_ans_2d1d649b7b6846f99be5bc545fe90ffd.jpg

$$571787$$ is a perfect cube.
Find the cube root of the following number.

On pairing, we get, $$\underline{571}$$ $$\underline{787}$$.

Unit's digit of the given number is $$7$$.

Least perfect cube with unit digit $$7$$ is $$27$$

and $$27=3^3\implies \sqrt[3]{27}=3$$.
So, unit's digit of cube root of $$571787$$ is $$3$$.

Now, striking out the last three digits of the given number, the number left is $$571$$.

The largest number whose cube is less than $$571$$ is $$8$$....(Since, $$8^3=512$$ and $$9^3=729$$).

So, ten's digit of the cube root is $$8$$.

Therefore, the cube root of $$571787$$ is $$83$$.

By taking three different values of $$n$$ verify the truth of the following statements:
If a natural number $$n$$ is of the form $$3p+2$$ then $$n^{3}$$ also a number of the same type.

2039794_1671859_ans_9a87078ec4964acb9cd2d4f6466b90af.jpg

$$2$$	$$3$$	$$4$$	$$-5$$	__	$$8$$	__
$$2^3 = 8$$	$$3^3 = ......$$	$$.... = 64$$	$$..... = .....$$	$$6^3 = .....$$	$$..... = ....$$	$$.... = -729$$

Cubes And Cube Roots - Class 8 Maths - Extra Questions

Find the value of: $$\displaystyle \sqrt[3]{729}$$.

Cube root of $$185193$$ is:

Find the cube root of the following numbers by prime factorization:$$157464$$

Fill in the blanks to make the statements true.Ones digit in the cube of 38 is ______.

Find the cube root of each of the following numbers by prime factorization method.$$64$$

Find the cube root of the following numbers by prime factorization:$$32768$$

Find the cube root of $$9a{ b }^{ 2 }+\left( { b }^{ 2 }+24{ a }^{ 2 } \right) \sqrt { { b }^{ 2 }-3a^{ 2 } } $$.

Find the cube of following decimal numbers:(a) $$0.7$$(b) $$0.09$$(c) $$1.1$$

Are the following number perfect cubes?If yes,then find the number whose cube is the given numbers:$$5832$$

Are the following number perfect cubes?If yes,then find the number whose cube is the given numbers:$$24$$ ?

Find the cube of $$-12$$.

Find the cube roots of the number $$3048625$$ using the fact that$$3048625=3375\times 729$$.

Evaluate: $$(1.2)^{3}$$

Evaluate: $$(21)^{3}$$

Evaluate: $$(15)^{3}$$

Evaluate the cube root of :$$\displaystyle \sqrt [ 3 ]{ \left (\dfrac{-27}{125} \right ) } $$

Find the cube root of$$135\sqrt { 3 } -87\sqrt { 6 } $$

Find the cube root of$$38+17\sqrt { 5 } $$

Find the given number is a perfect cube or not.$$540$$

Find the number is perfect cube or not? $$620$$

Find the cube root of each of the following cube numbers through estimation.$$148877$$

Find the cubes of:$$-30$$

Evaluate $$\sqrt[3]{64}$$

Which of the following numbers are not perfect cubes?$$1728$$

Find the cube root of the given number through estimation:$$1512$$

Evaluate: $$\sqrt[3] {8\times 17\times 17\times 17}$$.

The natural number whose cube is equal to itself is ____ .

Evaluate :(i) (1.2)$$ \displaystyle ^{3}$$(ii) (3.5)$$ \displaystyle ^{3}$$(iii) (0.8)$$ \displaystyle ^{3}$$(iv) (0.5)$$ \displaystyle ^{3}$$

If $$a+b+c=0$$ then prove that $$a^3+b^3+c^3=3abc$$.

Find the cube root of $$15625$$ by prime factorization method.

Find the smallest number by which $$243$$ must be multiplied to obtain a perfect cube.

Find the smallest number by which $$72$$ must be multiplied to obtain a perfect cube.

Find the smallest number by which $$675$$ must be multiplied to obtain a perfect cube.

Find the smallest number by which the following number must be divided to obtain a perfect cube:$$128$$

Find the cubic root of the following number by prime factorisation method:$$13824$$

Find the smallest number by which $$256$$ must be multiplied to obtain a perfect cube.

Find the cube roots of $$1728$$ by prime factorization:

Find the cube of $$133$$.

Evaluate: $$\sqrt [3]{8\times 125}$$.

Find the cube root of $$474552$$:

By what smallest number $$3645$$ be multiplied so that the product becomes a perfect cube?

Find the negative of cube root of $$-2744000$$.

Find the smallest number by which $$1323$$ must be multiplied so that the product is a perfect cube.

Show that $$\sqrt [3]{125\times 64}=\sqrt [3]{125}\times \sqrt [3]{64}$$

Find the value of $$\displaystyle (28)^{3}-(78)^{3}+(50)^{3}$$

Show that $$\sqrt [3]{216\times (-343)}=\sqrt [3]{216}\times \sqrt [3]{-343}$$

Evaluate: $$\sqrt [ 3 ]{ 27\times 64 } $$.

Evaluate $$(96)^3$$

Evaluate $$\sqrt [ 3 ]{ 125\times 64 } $$.

Find the cube root of the following number by prime factorisation method 175616

Find the cube root of the following number by the prime factorization method. 91125

Evaluate the following:$$\sqrt [3] { 512\times 729 }$$

Find the negative of cube root of $$-512$$.

Find the cube root of: $$8$$

Find $$(7)^3=$$?

The value of $$(21)^3$$ is?

Find $$(12)^3=$$?

Using the method of successive subtraction of numbers $$1, 7, 19, 37, 61, 91, 127, 169, 217, 271, 331, 397,..........$$Examine if the following number is a perfect cube or not792

Find $$(100)^3$$.

Multiply $$137592$$ by the smallest number so that the product is a perfect cube.

Find the value of $$(302)^3$$?

Write cubes of $$5$$ natural numbers which are multiples of $$3$$ and verify the following:'The cube of natural number, which is a multiple of $$3$$ is a multiple of $$27$$.

Find the cube root of $$74088$$.

Find the cube root of a given number through estimation: $$5832$$.

By the method of finding unit digit and tens digit of cube root, find the cube root of $$-68921.$$

Looking at the pattern, fill in the gaps in the following$$2$$$$3$$$$4$$$$-5$$ __$$8$$ __$$2^3 = 8$$$$3^3 = ......$$$$.... = 64$$$$..... = .....$$$$6^3 = .....$$$$..... = ....$$$$.... = -729$$

How many perfect cubes you can find from $$1$$ to $$100$$? How many from $$-100$$ to $$100$$?

Find the cube root by prime factorisation: $$46656$$

Find the cube root by prime factorization $$10648$$

What is the least positive integer k for which 15k is the cube of a number?

Find the cube root by prime factorisation $$15625$$

Find the cube of $$6$$.

What is the least positive integer with which you have to multiply $$243$$ to get a perfect cube?

Find the cube root of$$99-70\sqrt { 2 } $$

$$Evaluate\,\sqrt[3]{{0.000343}} + \sqrt[3]{{0.729}} + \sqrt[3]{{1.331}}$$

Find the cube root of a given number through estimation: $$2197$$.

Factorise $$8{x^3} + 27{y^3} + 36{x^3}y + 54x{y^2}$$

Find the cube roots of $$64$$

Which of the following numbers are perfect cubes? In case of perfect cube find the number with cube is the given number.$$21952$$

Find the cube root of the following numbers by prime factorization:
$$157464$$

Fill in the blanks to make the statements true.
Ones digit in the cube of 38 is ______.

Find the cube root of each of the following numbers by prime factorization method.
$$64$$

Find the cube root of the following numbers by prime factorization:
$$32768$$

Find the cube of following decimal numbers:
(a) $$0.7$$
(b) $$0.09$$
(c) $$1.1$$

Find the cube roots of the number $$3048625$$ using the fact that
$$3048625=3375\times 729$$.

Evaluate the cube root of :
$$\displaystyle \sqrt [ 3 ]{ \left (\dfrac{-27}{125} \right ) } $$

Find the cube root of
$$135\sqrt { 3 } -87\sqrt { 6 } $$

Find the cube root of
$$38+17\sqrt { 5 } $$

Find the given number is a perfect cube or not.
$$540$$

Find the number is perfect cube or not?
$$620$$

Find the cube root of each of the following cube numbers through estimation.
$$148877$$

Find the cubes of:
$$-30$$

Which of the following numbers are not perfect cubes?
$$1728$$

Find the cube root of the given number through estimation:
$$1512$$

Evaluate :
(i) (1.2)$$ \displaystyle ^{3}$$
(ii) (3.5)$$ \displaystyle ^{3}$$
(iii) (0.8)$$ \displaystyle ^{3}$$
(iv) (0.5)$$ \displaystyle ^{3}$$

Find the smallest number by which the following number must be divided to obtain a perfect cube:
$$128$$

Find the cubic root of the following number by prime factorisation method:
$$13824$$

Find the cube root of the following number by prime factorisation method
175616

Find the cube root of the following number by the prime factorization method.
91125

Using the method of successive subtraction of numbers $$1, 7, 19, 37, 61, 91, 127, 169, 217, 271, 331, 397,..........$$
Examine if the following number is a perfect cube or not
792

Write cubes of $$5$$ natural numbers which are multiples of $$3$$ and verify the following:
'The cube of natural number, which is a multiple of $$3$$ is a multiple of $$27$$.

Looking at the pattern, fill in the gaps in the following
$$2$$ $$3$$ $$4$$ $$-5$$ $$8$$
$$2^3 = 8$$ $$3^3 = ......$$ $$.... = 64$$ $$..... = .....$$ $$6^3 = .....$$ $$..... = ....$$ $$.... = -729$$

Find the cube root of
$$99-70\sqrt { 2 } $$

Which of the following numbers are perfect cubes? In case of perfect cube find the number with cube is the given number.
$$21952$$

Find the cube of the following:
$$0.4$$.

Find that the number is a perfect cube or not?
$$588$$

Among the following, the correct statement is
i) $$(a + b)^3 = a^3 + 3a^2b + 3ab^2 + b^3$$
ii) $$(a - b)^3 = a^3 - 3a^2b + 3ab^2 - b^3$$

Find following is a perfect cubes or not ?
$$9000$$

Find if the given number is a perfect cube or not?
$$3375$$

Find it is a perfect cubes or not ?
$$400$$

Find the given number is a perfect cube or not.
$$13824$$

Find following is a perfect cubes or not ?
$$6859$$

By multiplying an integer convert number to a perfect cube,Find the number?
$$2025$$

Find it is a perfect cubes or not ?
15625

Find which of the following are perfect cubes?
(i) $$243$$ (ii) $$588$$ (iii) $$1331$$ (iv) $$24000$$ (v) $$1728$$ (vi) $$1938$$

Which of the following numbers are not perfect cubes?
$$216$$

Classify as linear, cubic or quadratic.
$$y+y^3+y^2$$.

Find the cube root of each of the following cube numbers through estimation.
$$59319$$

Find the cube root of each of the following numbers:
$$-250047$$

Calculate cube root:
$$8000$$

Which of the following numbers are not perfect cubes?
$$ 1000$$

Find the cube root of the following numbers by prime factorization:
$$35937$$

Find the cubes of the following number:
$$-13$$

Solved:
$$\sqrt [ 3 ]{ 64 } $$

Find the cubes of the following number:
$$3 \dfrac{1}{5}$$

Find the cubes of the following number:
$$-5 \dfrac{1}{7}$$

Find the cube of :
$$54$$

Find the cube of :
$$31$$

Find the cubes of :
$$1.6$$

Find the cube root of the following numbers by prime factorisation:
$$21952$$

Find the cube of :
$$42$$

Find the cube of the following number:
$$-3 \dfrac{4}{9}$$

Find the cube of the following number:
$$-17$$

Find the value of:
$$7^3$$

Find the cube of :
$$11$$

Find the cubes of:
$$-3$$

Find the cubes of:
$$-25$$

Find the cubes of :
$$0.12$$

Find the cubes of:
$$-50$$

Find the cubes of:
$$-7$$

Find the cube of :
$$0.02$$

Find the cube of :
$$0.8$$

Find the cubic root of the following number by prime factorisation method:
$$15625$$

Find the cubic root of the following number by prime factorisation method:
$$10648$$

Find the smallest number by which a given number must be divided to obtain a perfect cube:
$$192$$

Find the smallest number by which a given number must be divided to obtain a perfect cube:
$$704$$

Find the smallest number by which a given number must be divided to obtain a perfect cube:
$$135$$

Find the cubic root of the following number by prime factorisation method:
$$27000$$

Find the smallest number by which the given number must be divided to obtain a perfect cube:
$$81$$