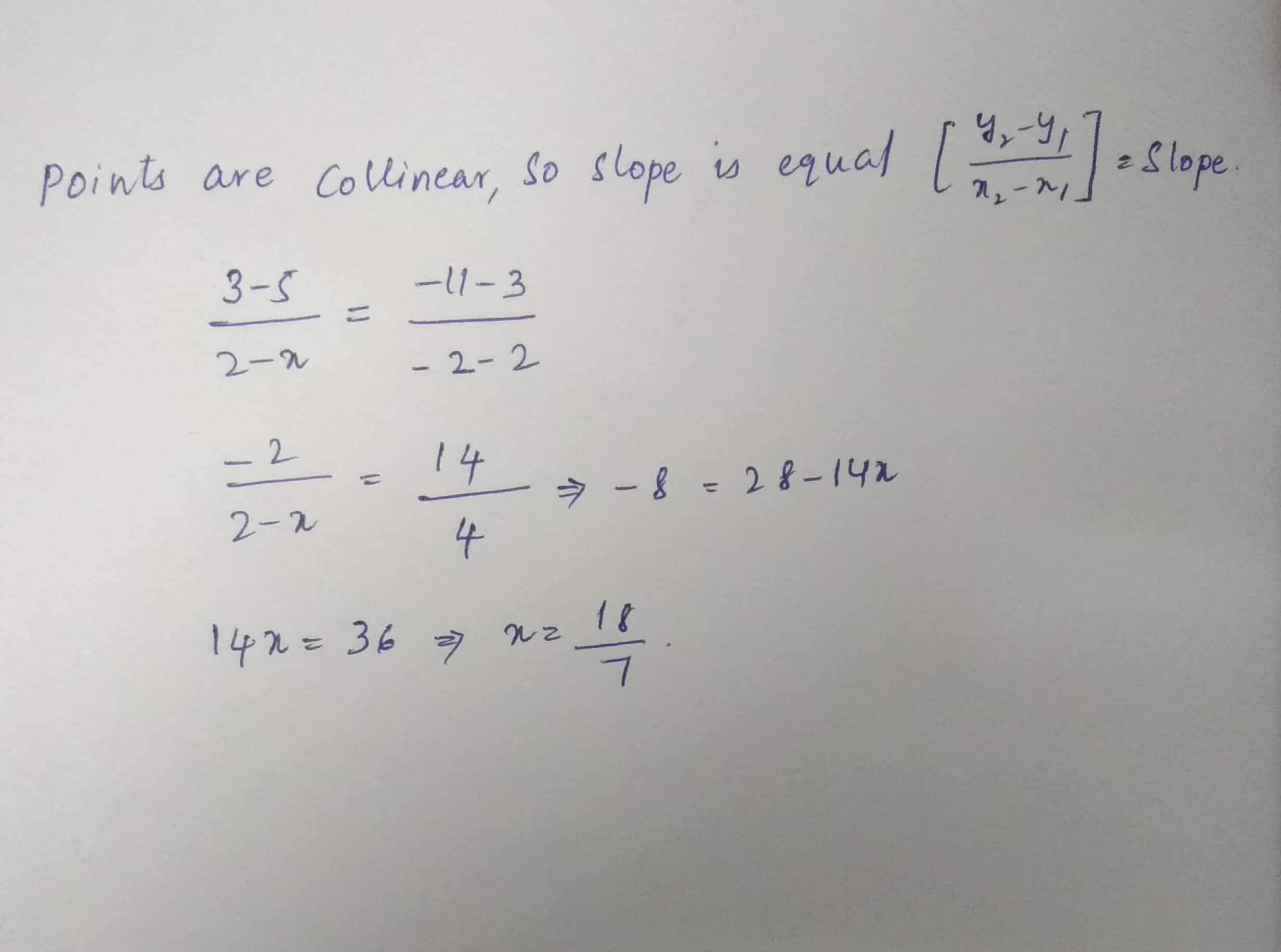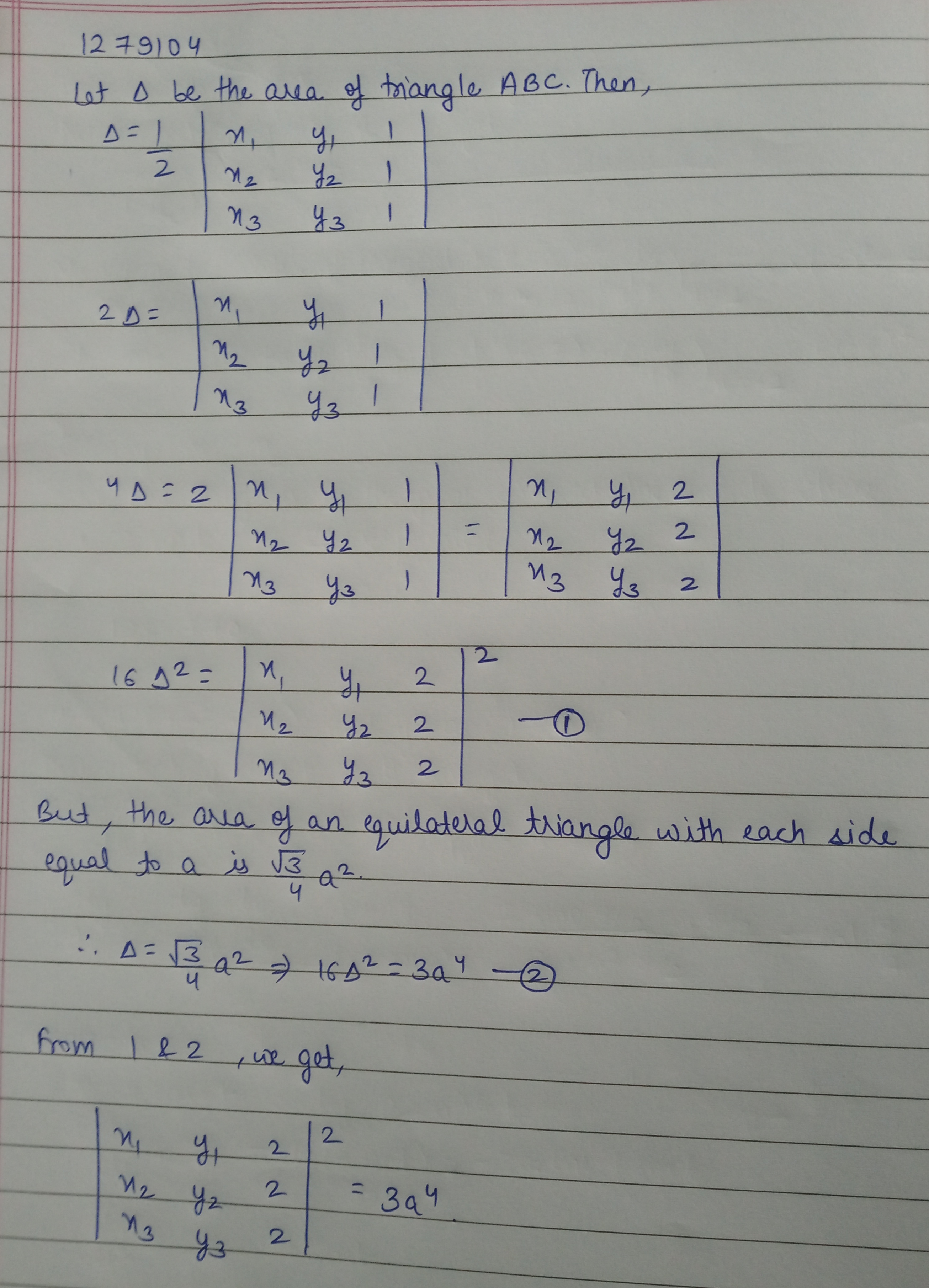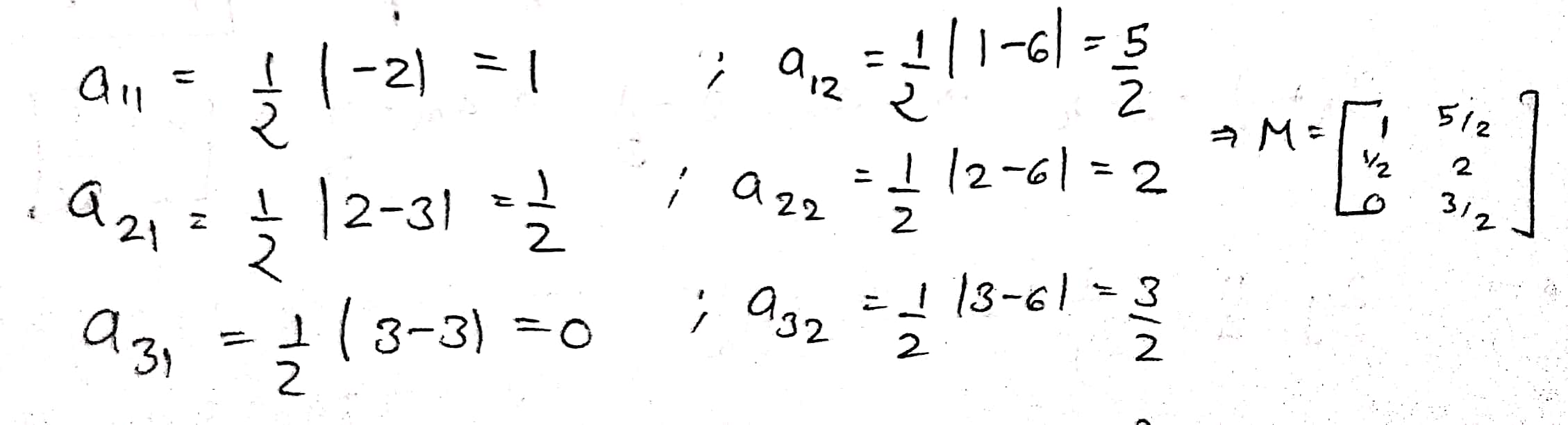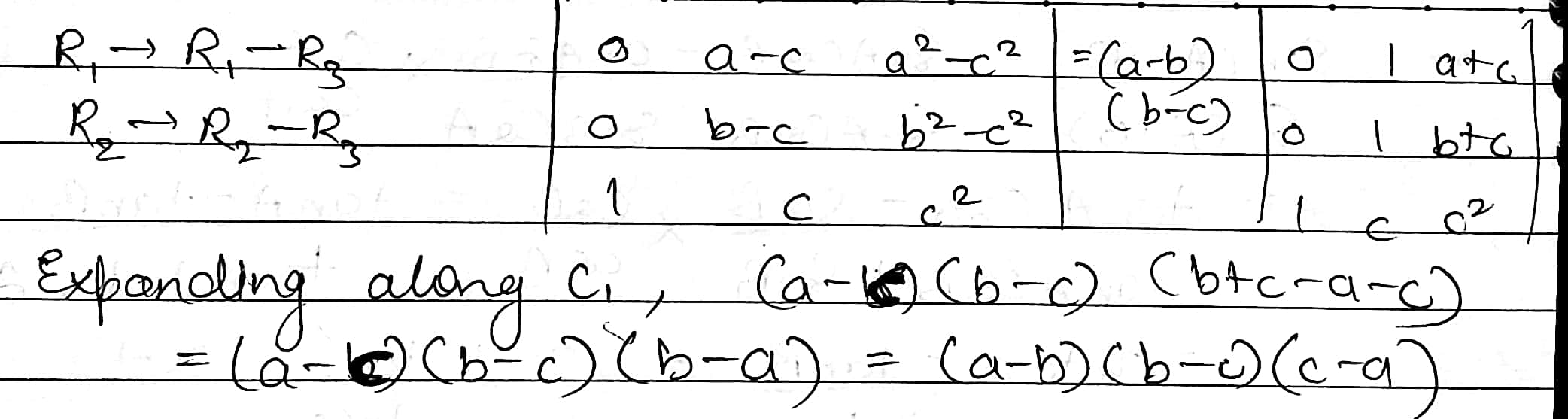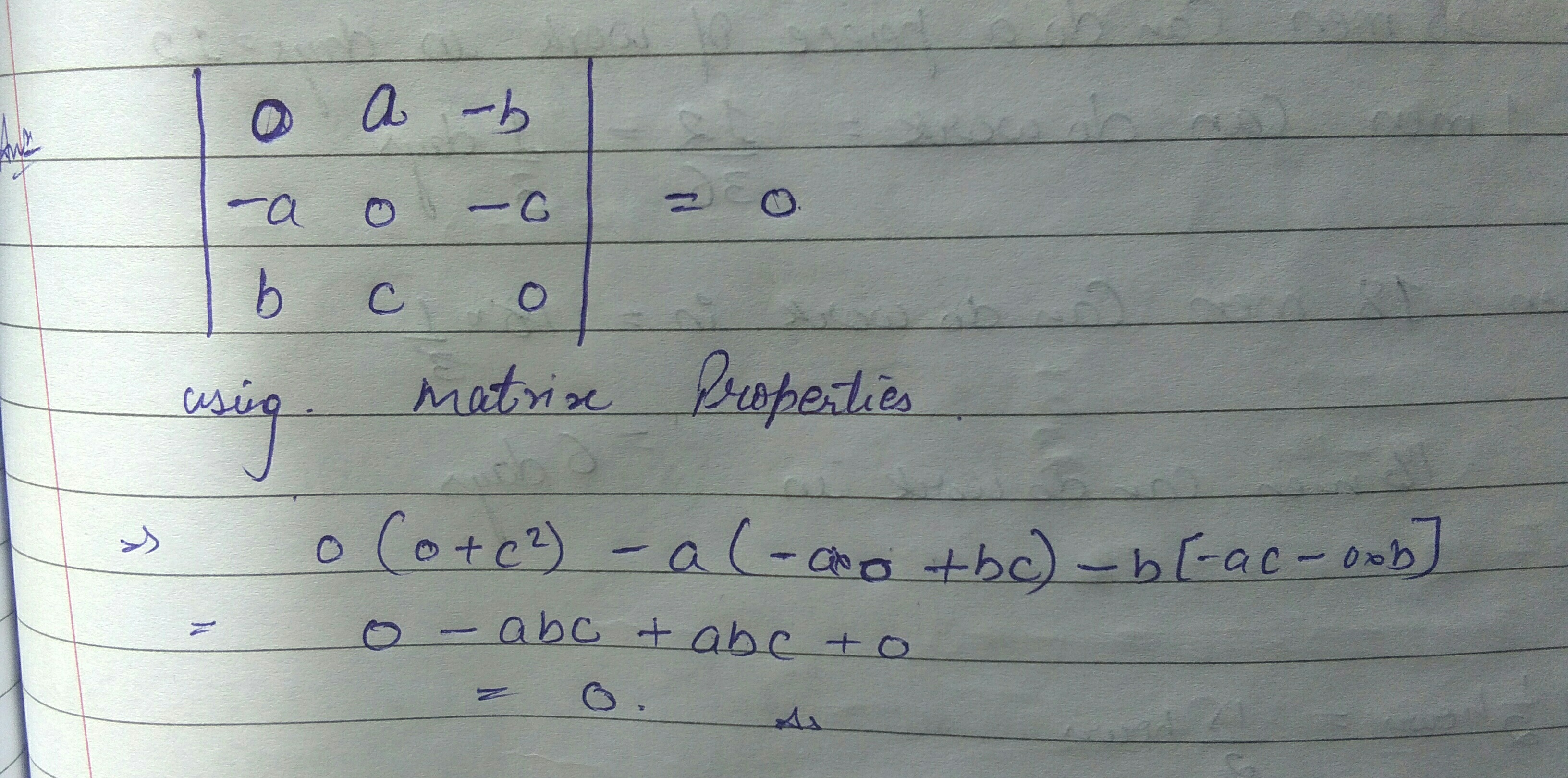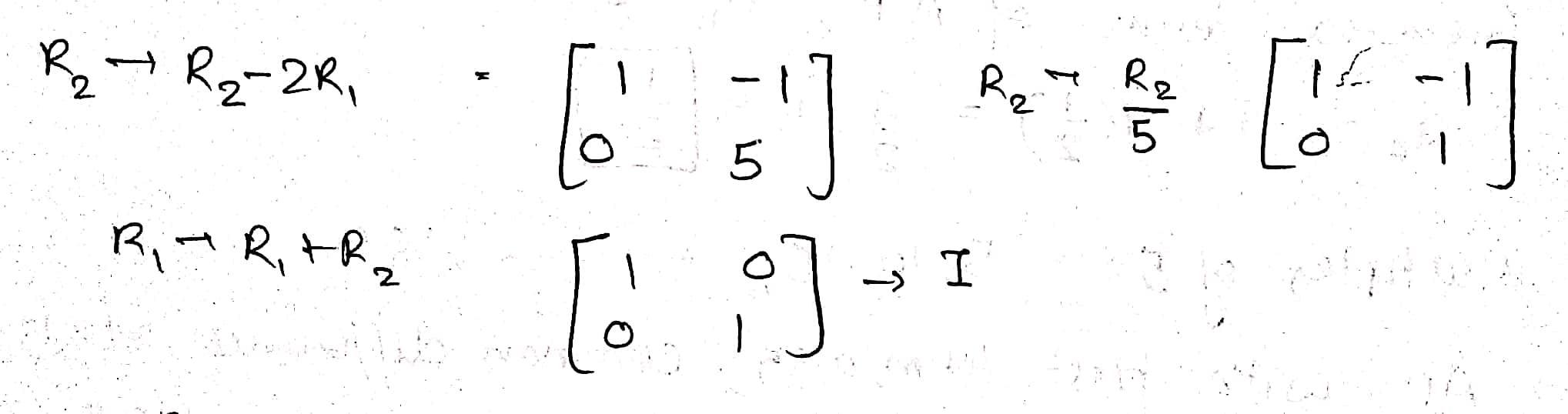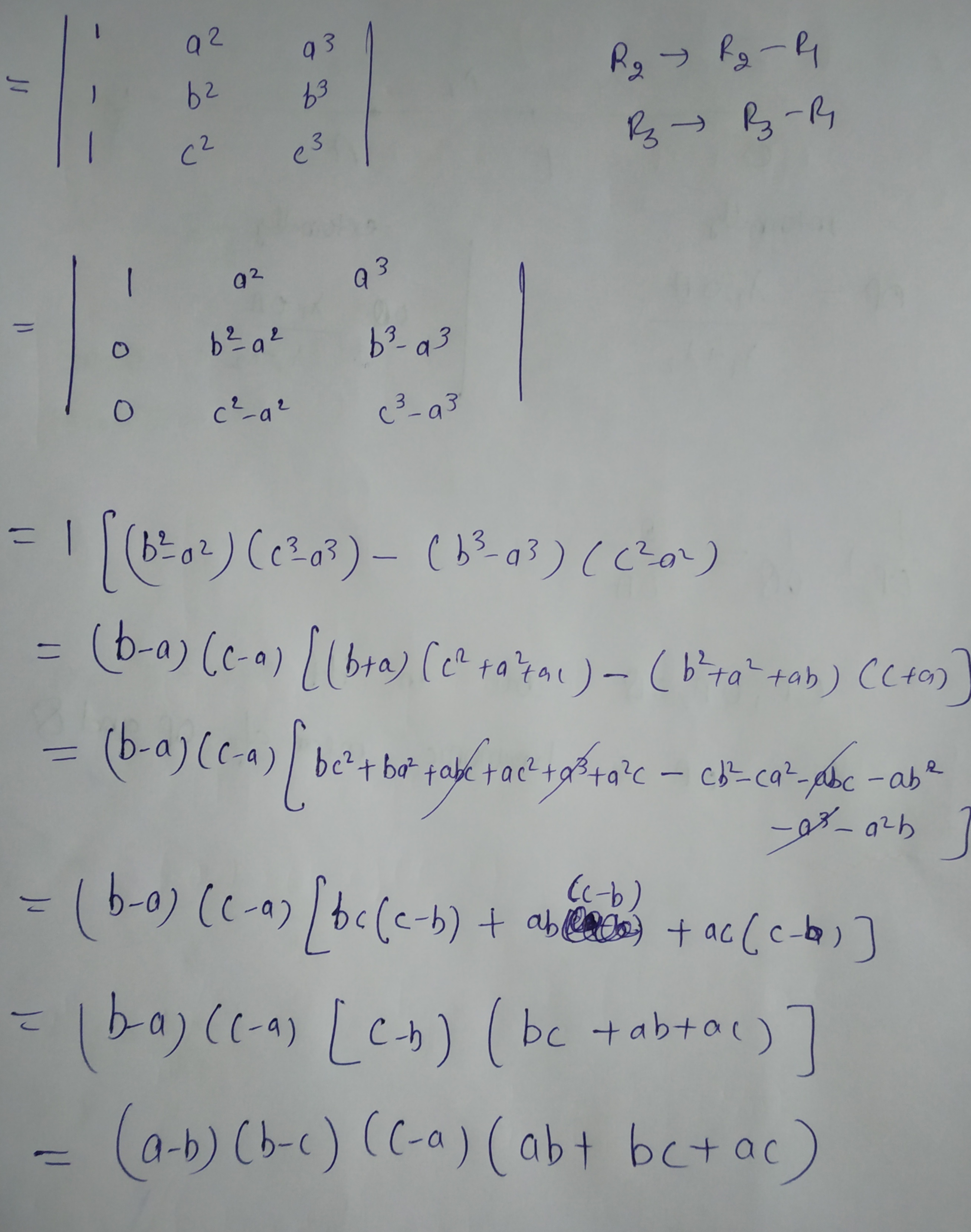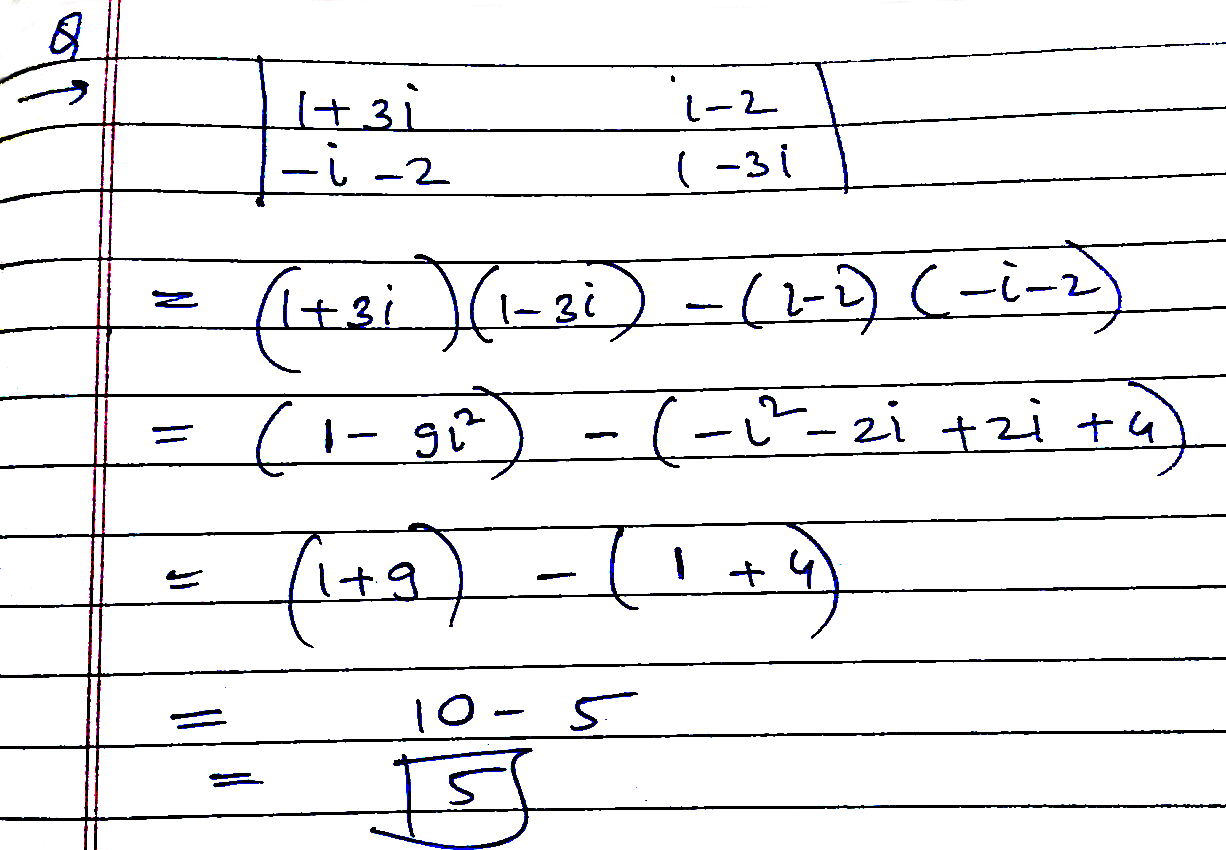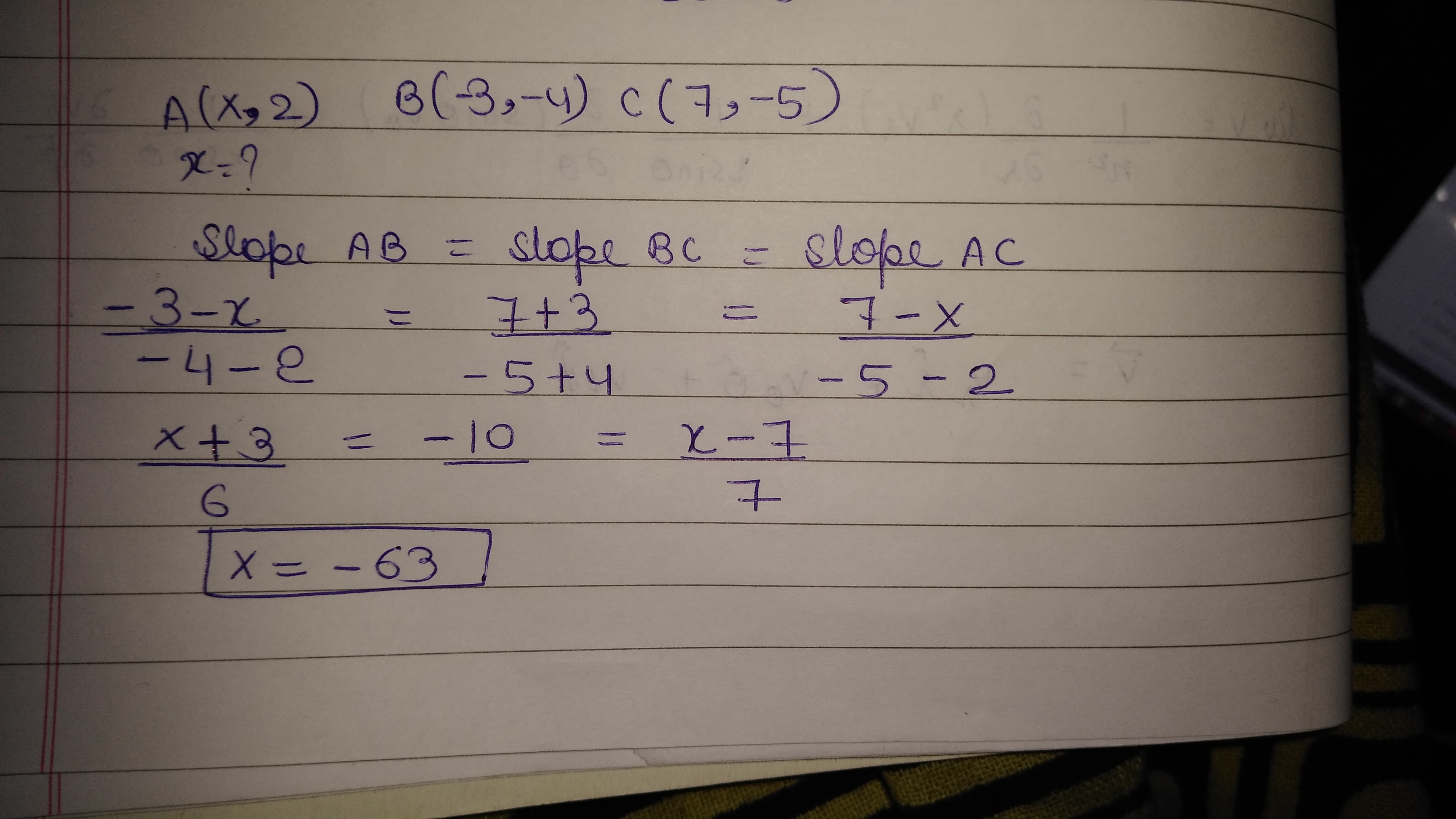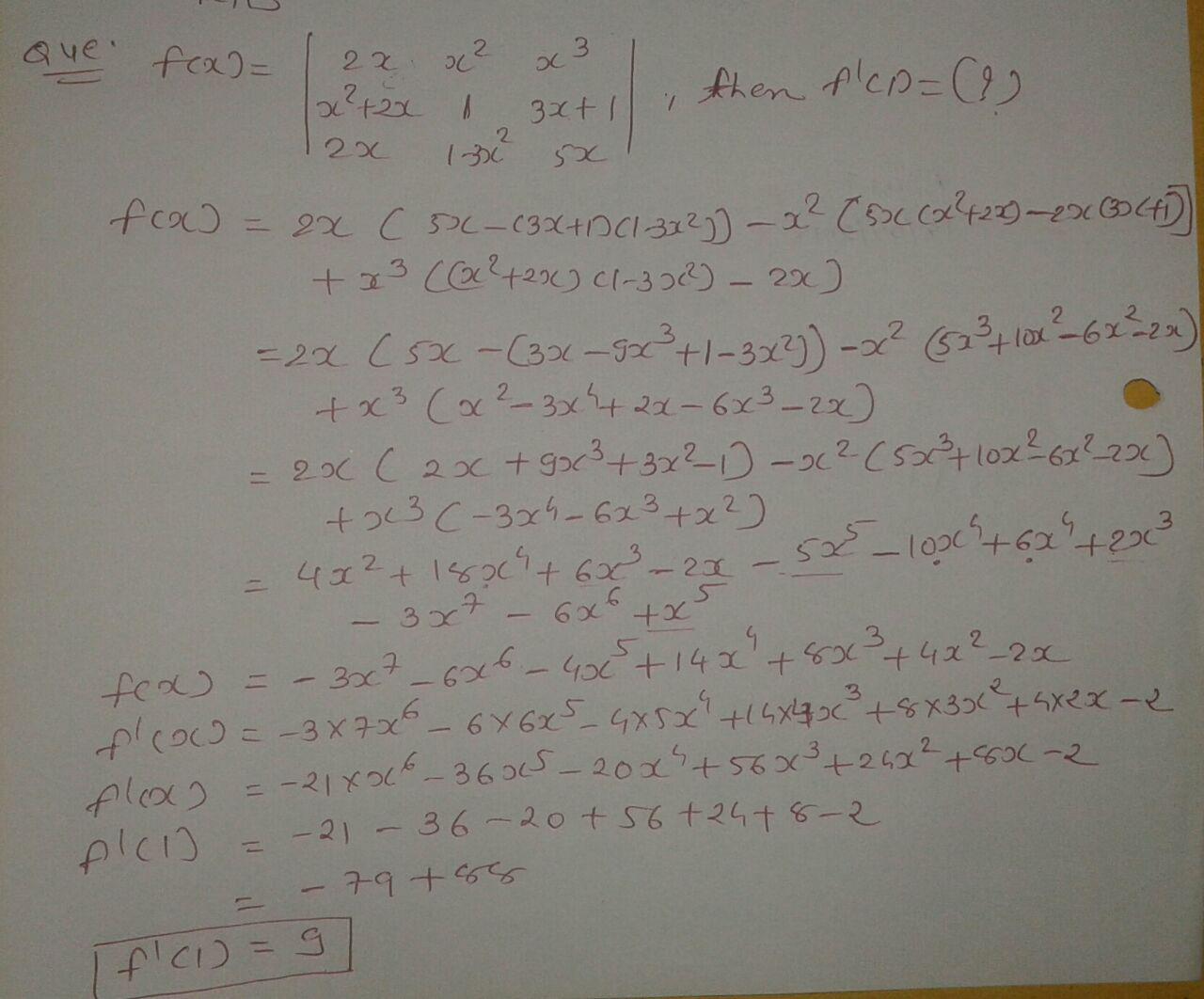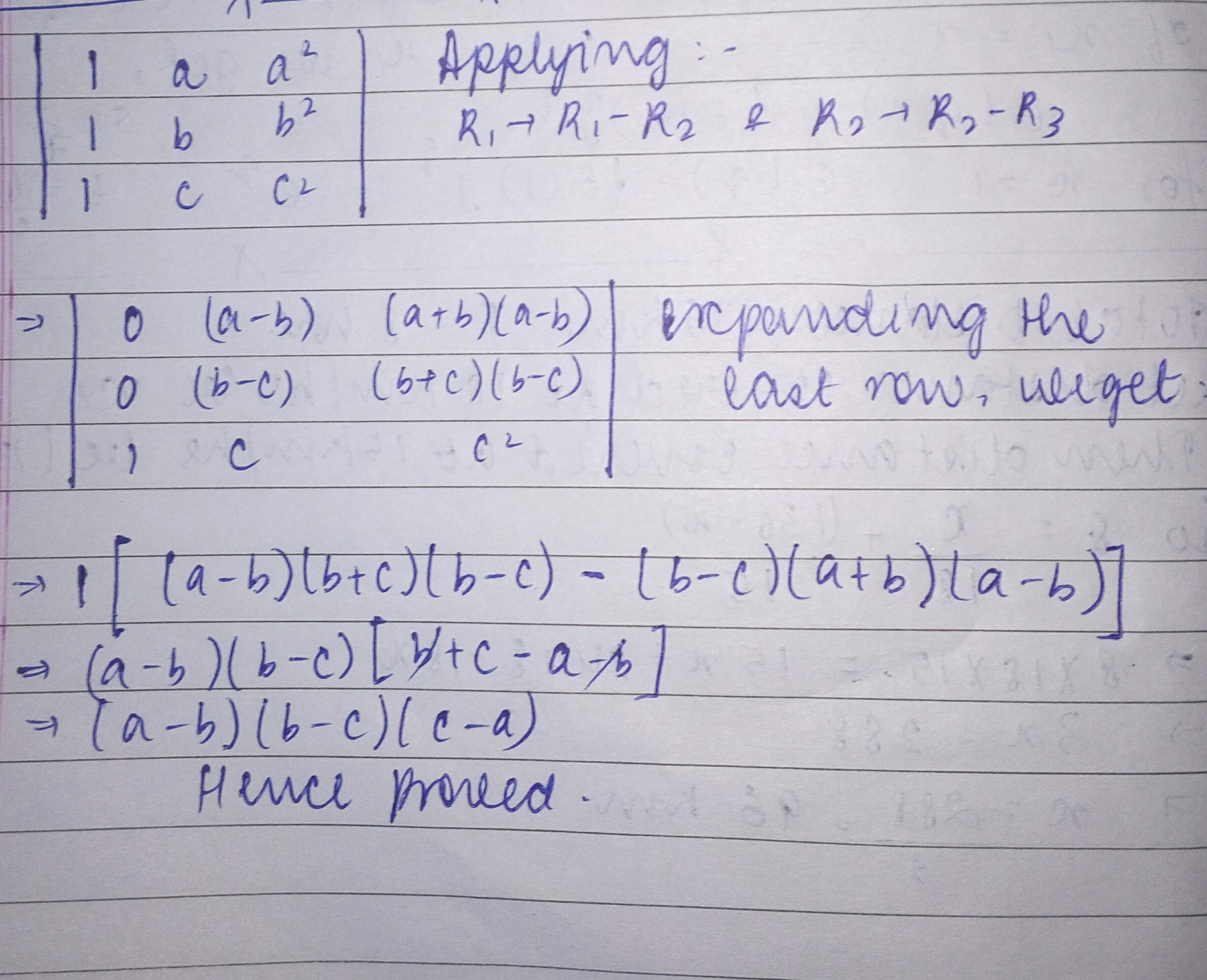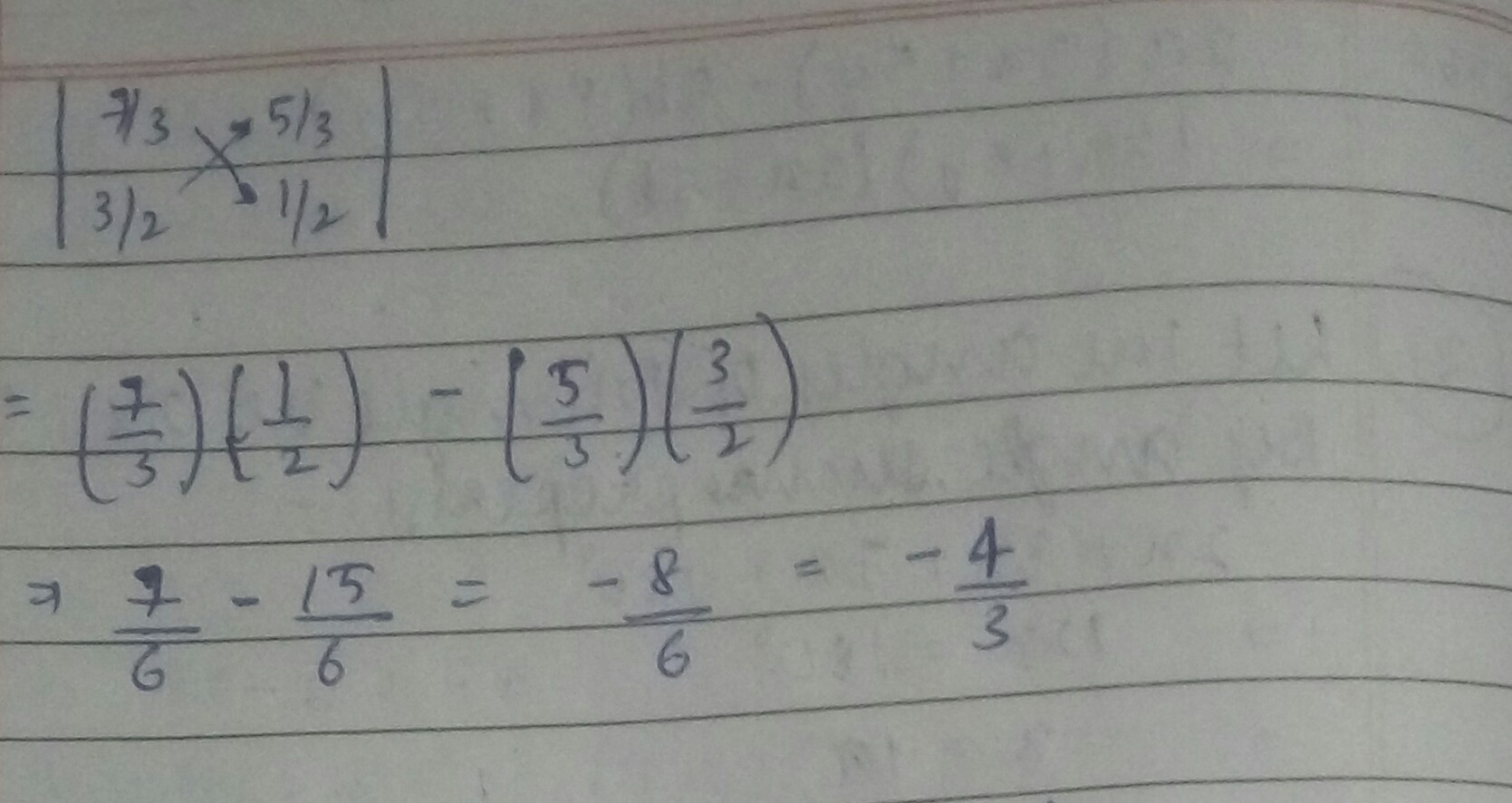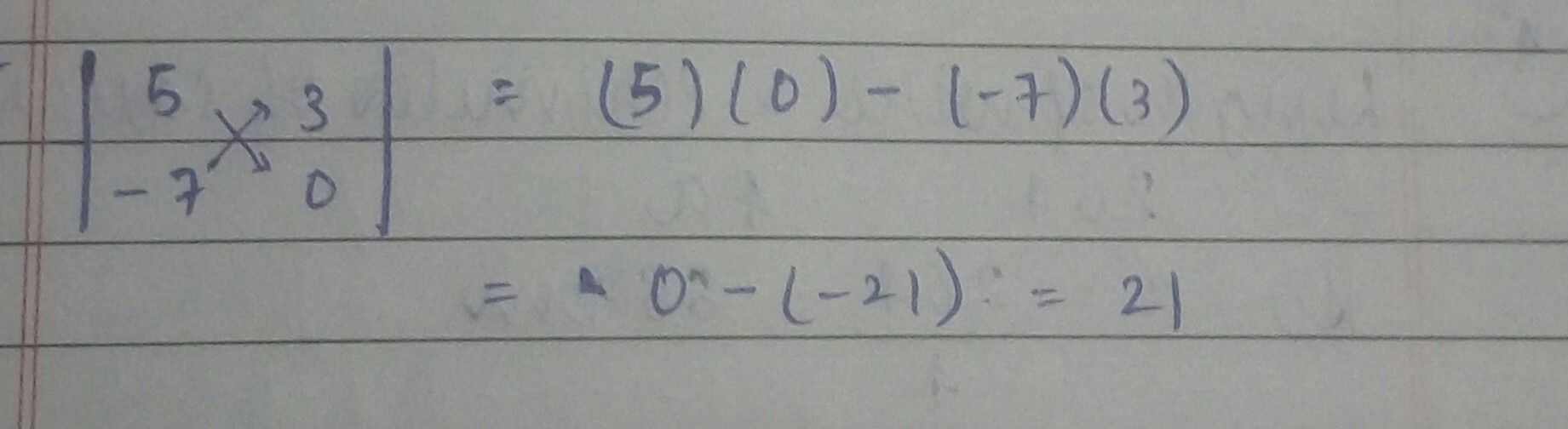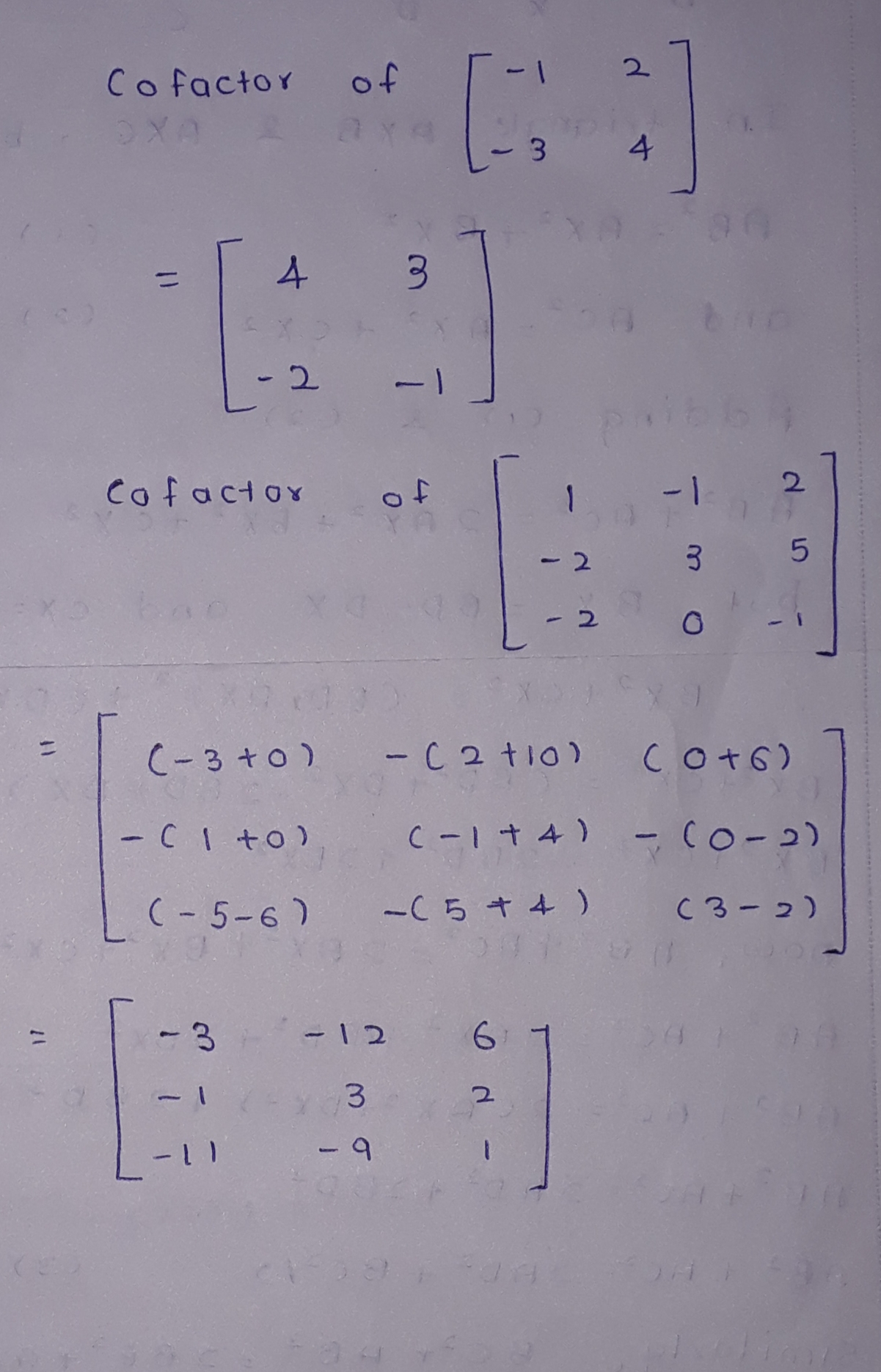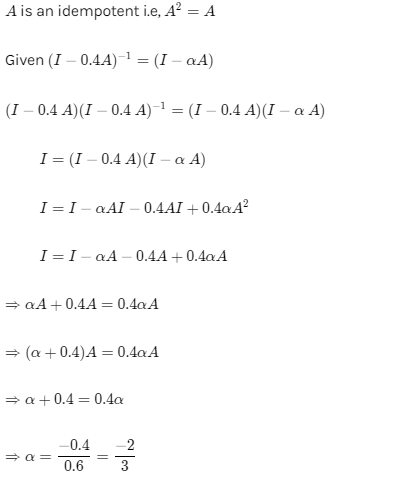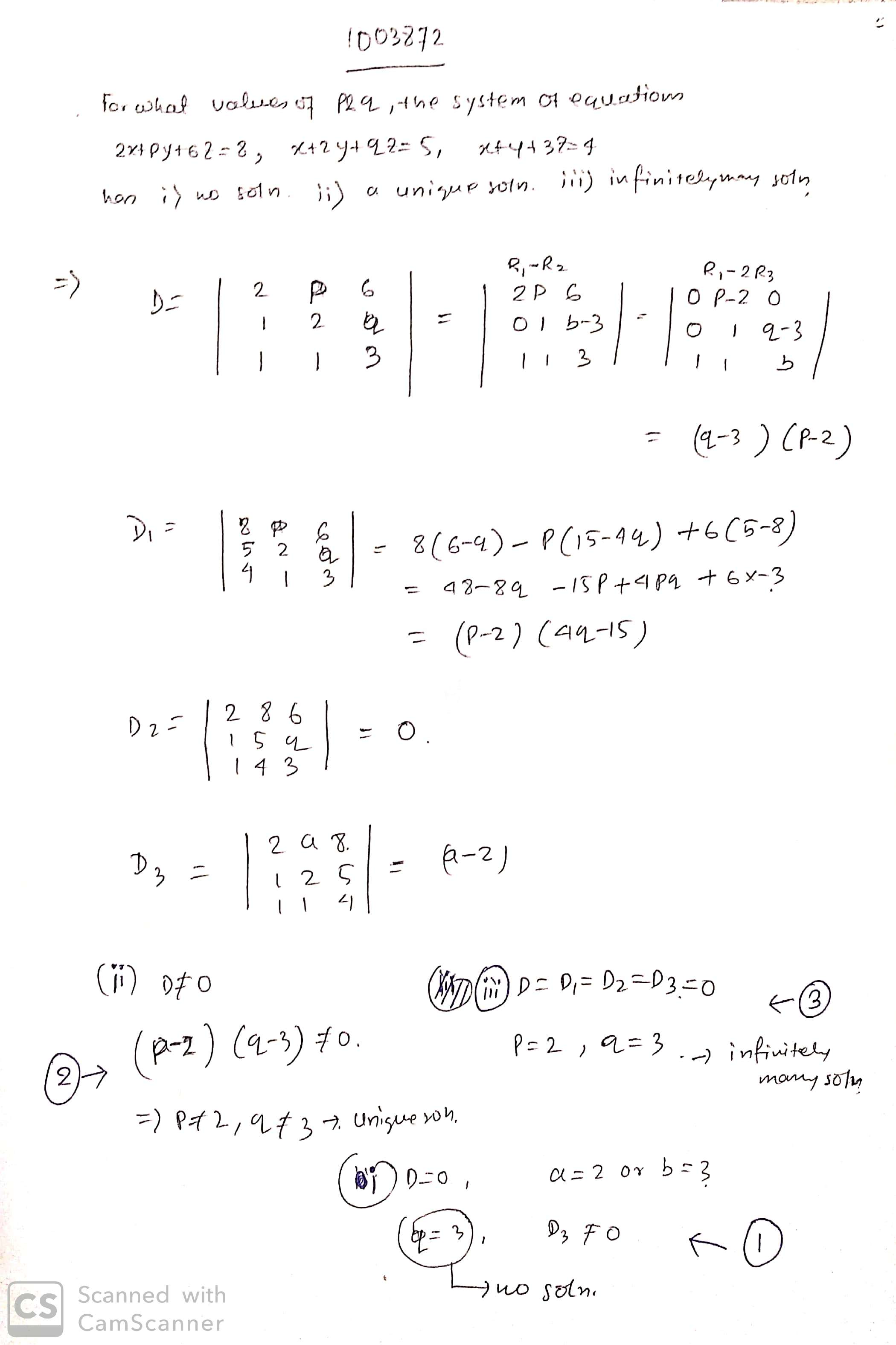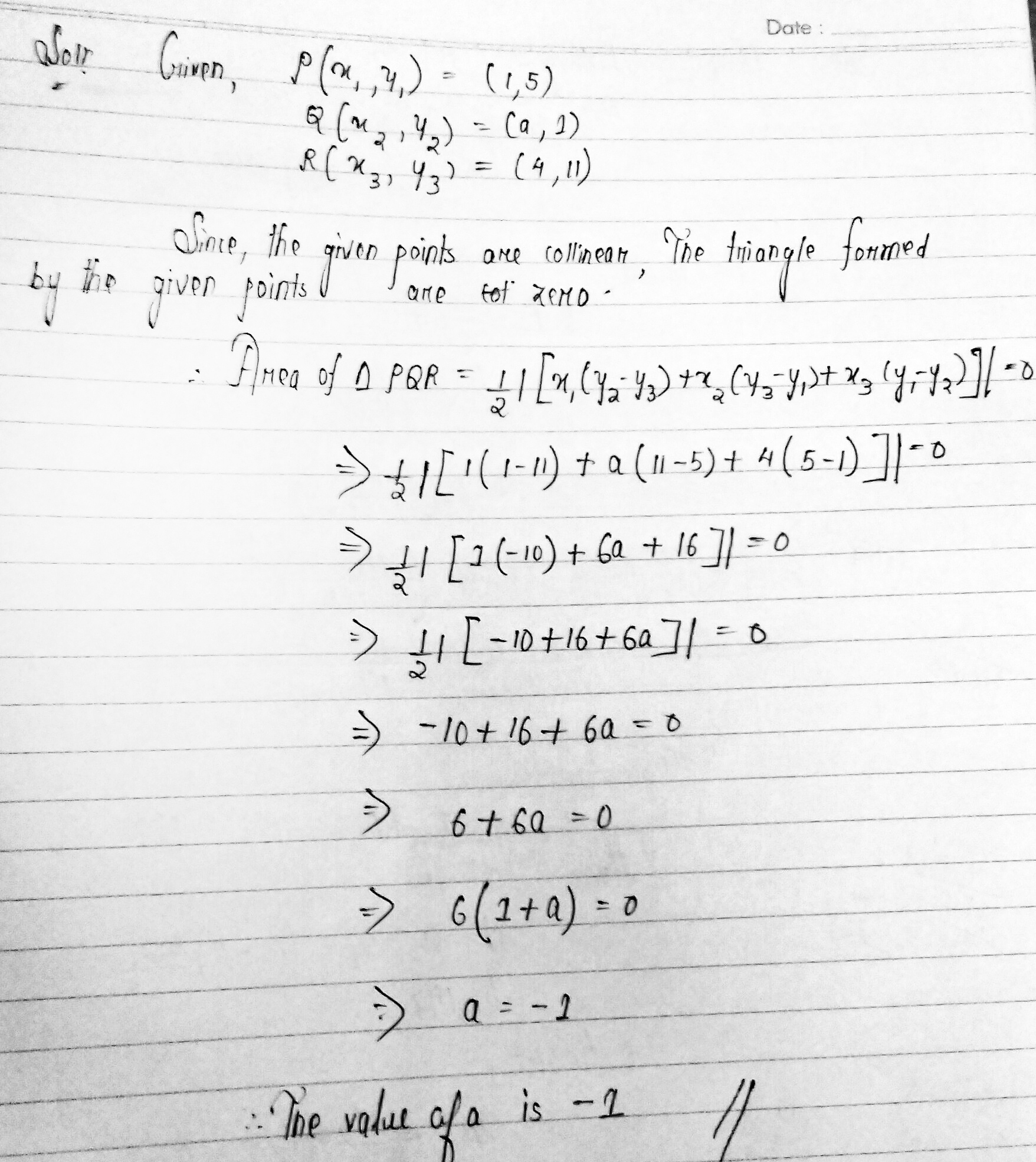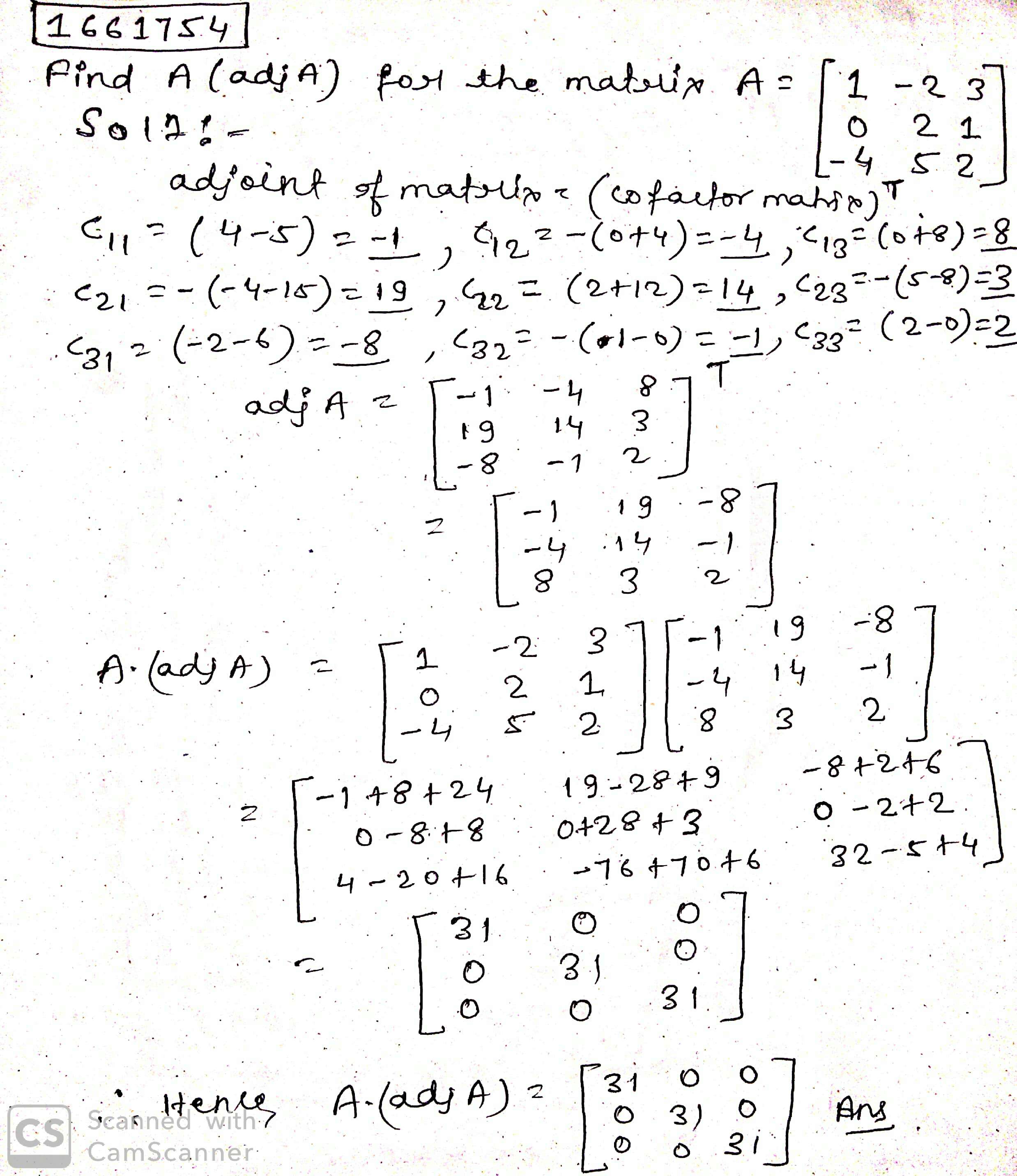Determinants - Class 12 Commerce Maths - Extra Questions
If A is 2×2 matrix, detA=4 then find the product of det(3A) and det(A−1)
Write the value of the determinant [pp+1p−1p]
when p=1342
Using the properties of determinants, find the value of
|0a−b−a0−cbc0|
Evaluate the determinants
|24−5−1|
If A=[1002345−6x] and detA=45; then find x.
Find adjoint of matrix \ \ \ A=\begin{bmatrix} 1 & 2 \\ 3 & 4 \end{bmatrix}
Show that the points A(2,3),B(4,0) and C(6,-3) are collinear.
Write the value of \triangle = \begin{vmatrix}x+y&y+z&z+x\\z&x&y\\-3&-3&-3\end{vmatrix}.
The area of a triangle is zero, then the three points are said to be ______ points.
Find the value of the determinant:
\begin{vmatrix}5 & -2\\ -3 & 1\end{vmatrix}
If for any 2 \times 2 square matrix A, A (adj A) = \begin{bmatrix} 8& 0\\ 0 & 8\end{bmatrix}, them write the value of det[A].
Find a value of x if \begin{vmatrix}x& 2 \\18&x\end{vmatrix} = \begin{vmatrix}6& 2 \\18&6\end{vmatrix}
\begin{vmatrix} 1 & 1 & 1 \\ a & b & c \\ { a }^{ 2 } & { b }^{ 3 } & { c }^{ 3 } \end{vmatrix}
Prove that : = (a - b) (b - c) (c - a) (a + b + c).
Find the values of x for which \begin{vmatrix}3&x\\x&1\end{vmatrix}=\begin{vmatrix}3&2\\4&1\end{vmatrix}.
Evaluate the following deteminants :
i)\begin{vmatrix} x& -7\\ x &5x+1 \end{vmatrix}
ii)\begin{vmatrix} \cos 15^{\circ} & \sin 15^{\circ}\\ \sin 75^{\circ} & \cos 75^{\circ} \end{vmatrix}
If 3^{n} is a factor of the determinant
\left| \begin{matrix} 1 & 1 & 1 \\ _{ }^{ n }{ { C }_{ 1 } } & _{ }^{ n+3 }{ { C }_{ 1 } } & ^{ n+6 }{ { C }_{ 2 } } \\ ^{ n }{ { C }_{ 2 } } & ^{ n+3 }{ { C }_{ 2 } } & ^{ n+6 }{ { C }_{ 2 } } \end{matrix} \right| ,
then the maximum value of n is ____.
Find the determinant in following case:
A=\left [ \begin{array}{c}5\hspace{0.5cm}{20}\\0\hspace{0.5cm}{2}\end{array} \right ]
Find if the points (0, 8/3), (1/3) , and (82, 30) are collinear.
Prove the following :
\left| \begin{matrix} y+z & z & y \\ z & z+x & x \\ y & x & x+y \end{matrix} \right| =4xyz
Prove that the points (a , b), (c , d) and (a - c, b - d) are collinear if ad = bc. Also show that the straight line passing through these points passes through origin.
Show that
\begin{vmatrix} \sin 10^{\circ}& -\cos 10^{\circ}\\ \sin 80^{\circ}&\cos 80^{\circ} \end{vmatrix}=1
Find the value of K, for which the given points are collinear:
\begin{matrix} A \\ \left( 7,-2 \right) \end{matrix}\begin{matrix} B \\ \left( 5,1 \right) \end{matrix}\begin{matrix} C \\ \left( 3,K \right) \end{matrix}
In each of the following find the value of k, for which the points are collinear..
(ii) (8, 1), (k, -4), (2, -5)
Let A=\begin{bmatrix}5&8\\8&13\end{bmatrix} then show that A satisfies the equation x^2-18x+1=0.
In each of the following find the value of k, for which the points are collinear.(i) (7, -2), (5, 1), (3, k)
Find the value of x if the points (x,5),(2,3) and (-2,-11) are collinear.
Points are collinear , so slope is equal \displaystyle \left [\frac{y_2 - y_1}{x_2 - x_1}\right] = slope.
\displaystyle \frac{3 -5}{2-x} = \frac{-11-3}{-2-2}
\displaystyle \frac{-2}{2-x} = \frac{14}{4} \Rightarrow -8 = 28 - 14x
\displaystyle 14x = 36 \Rightarrow x = \frac{18}{7}
If the minor of three-one element (i.e M_{31}) in the determinant \left| \begin{matrix} 0 & 1 & \sec { \alpha } \\ \tan { \alpha } & -\sec { \alpha } & \tan { \alpha } \\ 1 & 0 & 1 \end{matrix} \right| is 1 then find the value of \alpha. (0 \le \alpha \le \pi)
Show that \triangleABC is an isosceles triangle, if the determinant\begin{vmatrix} 1 & 1 & 1 \\ 1+\cos A & 1+\cos B & 1+\cos C \\ \cos^2A +\cos A & \cos^2B+\cos B & \cos^2C+\cos C\end{vmatrix}=0
Using properties of determinants, prove the following\begin{vmatrix} 1+a^2-b^2 & 2ab & -2b \\ 2ab & 1-a^2+b^2 & 2a \\ 2b & -2a & 1-a^2-b^2 \end{vmatrix}=(1+a^2+b^2)^3.
Solve:\begin{vmatrix} 0& -3 &x \\ x+1& 3 &1 \\ 4& 1 & 5\end{vmatrix} =0
If A = \left| {\begin{array}{*{20}{c}} 1&{ - 1} \\ { - 1}&1 \end{array}} \right|, then show that {A^2} = 2A\,\,\& \,\,{A^3} = 4A
Find \begin{vmatrix}4&6&2\\3&0&9\\7&1&5\end{vmatrix}
Show that the point P (a,b+c), Q(b,c+a) and R(c,a+b) are collinear.
Find the adjoint of \begin{vmatrix} 1 & -1 & 2 \\ 3 & 0 & -2 \\ 1 & 0 & 3 \end{vmatrix}
Find the value of following determinant.
\begin{vmatrix} \dfrac{7}{3} & \dfrac{5}{3}\\ \dfrac{3}{2} & \dfrac{1}{2}\end{vmatrix}.
If A=\left[ \begin{matrix} 1 & 1 \\ 2 & 1 \end{matrix} \right], find |A|
If A is a square matrix of order 3 such |A|=5, then find the value of |adjA| ?
If three points (x_1 , y_1) , (x_2 , y_2 ) and (x_3 , y_3 ) lie on the same line, prove that
\dfrac {y_2 - y_3}{x_2 x_3} + \dfrac {y_3 - y_1}{x_3 x_1}+ \dfrac {y_1 - y_2}{x_1 x_2} = 0
Evaluate \begin{vmatrix} a & b & c \\ -1 & 1 & -1 \\ 1 & -1 & 1 \end{vmatrix}.
Find the values of the following determinants
\begin{vmatrix} 2i & -3i \\ { i }^{ 3 } & -2{ i }^{ 5 } \end{vmatrix} where i=\sqrt{-1}.
\begin{vmatrix} 2i & -3i\\ i^3 & -2i^5\end{vmatrix}
=(2i\times 2i^5)-(i^3\times -3i)
=-4i^6-(3i^2)
=4+3
=7.
A = \begin{bmatrix} 2&4 \\ 6&8 \end{bmatrix} find det(A)
Compute the following determinant :
\begin{vmatrix} { a }^{ 2 } & ab \\ ab & { b }^{ 2 } \end{vmatrix}
\begin{vmatrix} { a }^{ 2 } & ab \\ ab & { b }^{ 2 } \end{vmatrix}
Let A = \begin{vmatrix} { a }^{ 2 } & ab \\ ab & { b }^{ 2 } \end{vmatrix}
\because determinant of a matrix \begin{vmatrix} { a }_{11} & a_{12} \\ a_{21} & { a_{22} } \end{vmatrix} is
given by a_{n} a_{22}-a_{21}a_{12}
\Rightarrow |A| = a^{2}b^{2}-(ab)(ab)
= a^{2}b^{2}-a^{2}b^{2} = 0
\therefore |A| = 0
Show that \left| \begin{matrix} 1 & 1 & 1 \\ a & b & c \\ { a }^{ 2 } & b^{ 2 } & c^{ 2 } \end{matrix} \right| =(a-b)(b-c)(c-a).
Compute the following determinant :
\begin{vmatrix} 3 & 5 \\ -2 & 6 \end{vmatrix}
\begin{vmatrix} 3 & 5 \\ -2 & 6 \end{vmatrix}
Let A = \begin{vmatrix} 3 & 5 \\ -2 & 6 \end{vmatrix} [\because \,determinate = a_{4}a_{22}-a_{12}a_{21}]
|A| = determinant = 3\times 6-5\times (-2)
= 18+10 = 28
Find value of the determinant
A = \begin{vmatrix}3 & 2\\ 5 & 7\end{vmatrix}
Prove that \left| \begin{matrix} 1 & \omega & { \omega }^{ 2 } \\ \omega & { \omega }^{ 2 } & 1 \\ { \omega }^{ 2 } & 1 & { \omega } \end{matrix} \right| =0
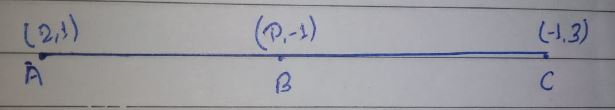
For what value of p are the points (2,1),(p,-1) and (-1,3) collinear.
^m{A_C}{ = ^m}{A_B}
\Rightarrow \frac{{3 - 1}}{{ - 1 - 2}} = \frac{{ - 1 - 1}}{{P - 2}}
\Rightarrow \frac{{ - 2}}{3} = \frac{{ - 2}}{{P - 2}}
\Rightarrow 3 = P - 2
\therefore P = 5
Hence the value of P=5.
Using determinants show that points A(a,b+c),B(b,c+a) and C(c,a+b) are col-linear.
Prove that the adjoint of a symmetric matrix is also a symmetric matrix.
Find deteminant \begin{bmatrix} 1 & 0 \\ 0 & 2 \end{bmatrix}
Prove that: 2\left| \begin{matrix} 8 & -5 \\ -2 & 6 \end{matrix} \right|=\left| \begin{matrix} 14 & -2 \\ -4 & 6 \end{matrix} \right|
2\begin{vmatrix} 8 & -5\\ -2 & 6\end{vmatrix}
=2[8\times 6-(-5\times -2)]
=2[48-(10)]
=2[48-10]
=2\times 38
=76
\begin{vmatrix} 14 & -2\\ -4 & 6\end{vmatrix}
=14\times 6-(-2\times -4)
=84-(8)
=76
Hence, proved.
In the diagram on a lunar eilpse, if the positions od sun,Earth and moon are shown by (-4,6), (k,-2) and (5,-6) respectively, then find the value of k.
If the points (3,-2), (x,2) and (8,8) are collinear find 10x using determinant.
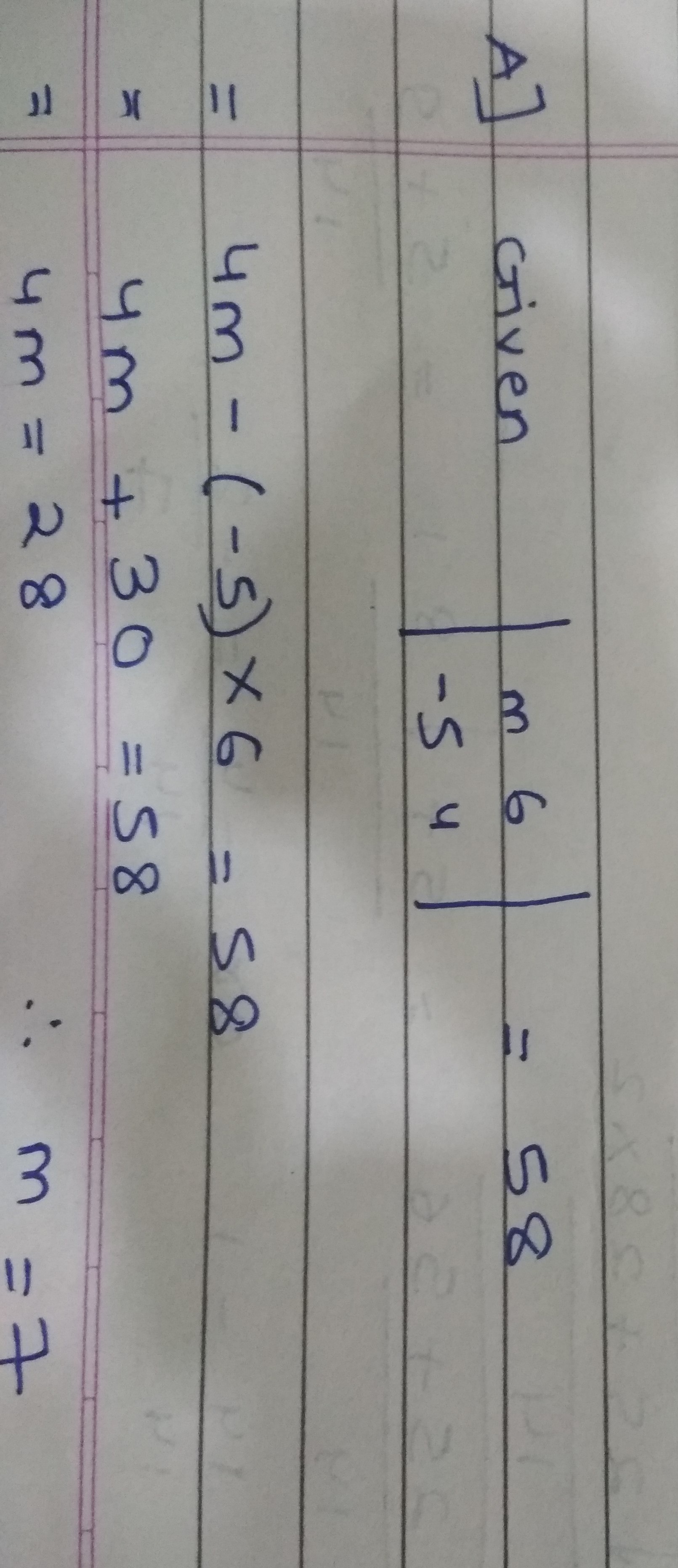
If the value of determinant \left| \begin{matrix} m & 6 \\ -5 & 4 \end{matrix} \right| is 58, then find the value of m.
A] Given \left| \begin{matrix} m & 6 \\ -5 & 4 \end{matrix} \right| is 58,
=4m-(-5)\times 6 = 58
=4m+30 = 58
=4m = 28 \therefore m = 7
If A(x_{1},y_{1}),B(x_{2},y_{2}),C(x_{3},y_{3}) are vertices of an equilateral triangle whose each side is equal to a, then prove that \begin{vmatrix} x_{1} & y_{1} & 2 \\ x_{2} & y_{2} & 2 \\ x_{3} & y_{3} & 2 \end{vmatrix}=3a^4.
Find the value of k if det \begin{vmatrix} K+3 & 1 & 2 \\ 3 & -2 & 1 \\ -4 & -3 & 3 \end{vmatrix}=0
Using the method of slope, show that the following points are collinear : ( i ) A \left( 4,8\right ) , B \left( 5,12 \right) , C \left( 9,28 \right) (ii) A \left( 16 , - 18 \right) , B \left( 3 , - 6 \right) , C \left( - 10,6\right )
(i) Slope of AB=\dfrac{12-8}{5-4}=\dfrac{4}{1}=4
Slope of BC=\dfrac{28-12}{9-5}=\dfrac{16}{4}=4
Hence AB||BC, but B is a point of intersect.
Hence A, B, C are collinear.
(ii) Slope of AB=\dfrac{-6+18}{3-16}=\dfrac{12}{-13}=-\dfrac{12}{13}
Slope of BC=\dfrac{6+6}{-10-3}=\dfrac{12}{-13}=-\dfrac{12}{13}
Slope of AB= slope of BC
Hence A, B, C are collinear.
Show that the points P(-2,3,5), Q(1,2,3) and R(7,0,-1) are collinear.
Show that the points P(-2, 3, 5), Q(1, 2, 3) and R(7, 0, -1) are collinear.
Solve:
\left[ \begin{matrix} 1 & 4 & 2 \\ 2 & -1 & 4 \\ -3 & 7 & 6 \end{matrix} \right]
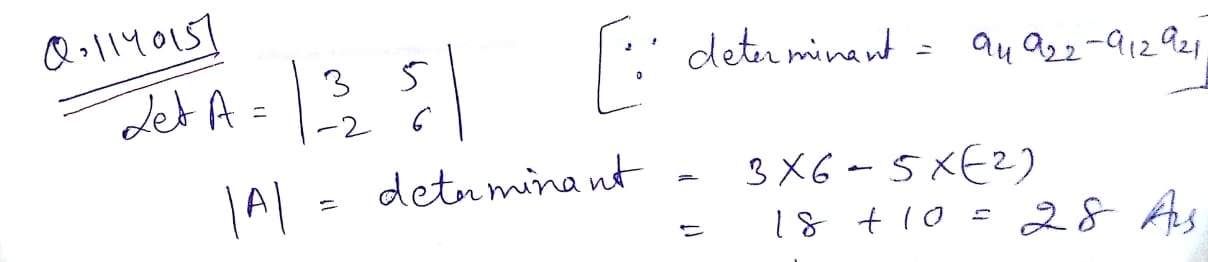
If A= \left[ {\matrix{10 & 0 \cr 4 & 10\cr } } \right], then find \left| A \right|.
Construct a 3\times 2 matrix whose elements are given by a_{1}=\dfrac{1}{2}|i-3j|.
a_{11}=\dfrac{1}{2}|-2|=1; a_{12}=\dfrac{1}{2}|1-6|=\dfrac{5}{2}
a_{21}=\dfrac{1}{2}|2-3|=\dfrac{1}{2}; a_{22}=\dfrac{1}{2}|2-6|=2
a_{31}=\dfrac{1}{2}|3-3|=0; a_{32}=\dfrac{1}{2}|3-6|=\dfrac{3}{2}
\Rightarrow M=\begin{bmatrix} 1 & 5/2\\ 1/2 & 2\\ 0 & 3/2\end{bmatrix}.
Write the value of the determinant \left| \begin{array}{cc}{3} & {-1} \\ {2} & {1}\end{array}\right|
\left| \begin{matrix} 1 & a & { a }^{ 2 } \\ 1 & b & { b }^{ 2 } \\ 1 & c & { c }^{ 2 } \end{matrix} \right| =(a-b)(b-c)(c-a)
R_1\rightarrow R_1 - R_3
R_2\rightarrow R_2 - R_3
\begin{vmatrix} 0 & a-c & a^2-c^2\\ 0 & b-c & b^2-c^2\\ 1 & c & c^2\end{vmatrix} =(a-b)(b-c) \begin{vmatrix} 0 & 1 & a+c\\ 0 & 1 & b+c\\ 1 & c & 0^2\end{vmatrix}
Expanding along C_1, (a-c)(b-c)(b+c-a-c)
=(a-c)(b-c)(b-a)=(a-b)(b-c)(c-a).
Solve:
\left| \begin{matrix} 0 & a & -b \\ -a & 0 & -c \\ b & c & 0 \end{matrix} \right| =0
\left| \begin{matrix} 0 & a & -b \\ -a & 0 & -c \\ b & c & 0 \end{matrix} \right| =0
using matrix propertis
\Rightarrow o(o+c^{2})-a(-ao+bc)-b[-ac-o\times b]
o=-abc+abc+o
=0
Find the value of x for which |_{ x }^{ 3 }\quad _{ 1 }^{ x }|=|_{ 8 }^{ 3 }\quad _{ 1 }^{ 2 }|.
If the points (-3,6), (-9,a) and (0,15) are collinear, then find a.
Convert \begin{bmatrix} 1 & -1 \\ 2 & 3 \end{bmatrix} into an identify matrix by suitable row transformations.
R_2\rightarrow R_2-2R_1 \begin{bmatrix} 1 & -1\\ 0 & 5\end{bmatrix} R_2\rightarrow \dfrac{R_2}{5} \begin{bmatrix} 1 & -1\\ 0 & 1\end{bmatrix}
R_1\rightarrow R_1+R_2 \begin{bmatrix} 1 & 0\\ 0 & 1\end{bmatrix} \rightarrow I.
If A = \left| \begin{array} { c c } { 2 } & { 3 } \\ { - 1 } & { 2 } \end{array} \right| Find A.
Evaluate \Delta = \begin{vmatrix} \cos 2\alpha & \sin 2\alpha \\-\sin 3\alpha & \cos 3\alpha \end{vmatrix}
The value of \begin{vmatrix} \cos15^{\circ} & \sin15^{\circ} \\ \sin75^{\circ} & \cos75^{\circ} \end{vmatrix} is
Find the value of x, if \begin{vmatrix} x+2 & x \\ x-4 & x+3 \end{vmatrix}=\begin{vmatrix} 4 & 2 \\ -2 & 5 \end{vmatrix}
Evaluate:\begin{vmatrix} \cos30^{\circ} & \sin30^{\circ} \\ \sin105^{\circ} & \cos105^{\circ} \end{vmatrix}
Evaluate the determinant :
\begin{vmatrix} \cos\theta & -\sin\theta \\ \sin\theta & \cos\theta \end{vmatrix}
Find the determinant of the matrix A
A=\begin{bmatrix} -1 & 4 \\ 2 & 3 \end{bmatrix}
Show that the points A(-7, 4, -2), B(-2, 1, 0) and C(3, -2, 2) are collinear.
Find the coordinates of a point A, where AB is diameter of a circle whose centre is (2, -3) and B is the point (1, 4).
Evaluate the following determinants :
\begin{vmatrix} \cos15^{\circ} & \sin15^{\circ} \\ \sin75^{\circ} & \cos75^{\circ} \end{vmatrix}
Find the values of x, if \begin{vmatrix} 2 & 3 \\ 4 & 5 \end{vmatrix}= \begin{vmatrix} x & 3 \\ 2x & 5 \end{vmatrix}
Are the following pairs of sets equal? Give reasons.
A=\{x : x is a letter of the word "WOLF"\},
B=\{x : x is a letter of the word "FOLLOW"\}.
The value of the determinant \begin{vmatrix} \cos30^{\circ} & \sin60^{\circ} \\ \sin30^{\circ} & \cos60^{\circ} \end{vmatrix} is
Evaluate \begin{bmatrix} 14 & 9 \\ -8 & -7 \end{bmatrix}.
Find the adjoint of the following matrice:
\begin{bmatrix} a & b \\ c & d \end{bmatrix}
The value of the determinant \begin{vmatrix} \cos15^{\circ} & \sin15^{\circ} \\ \sin75^{\circ} & \cos75^{\circ} \end{vmatrix} is______
If { e }^{ i\theta }=\cos { \theta } +i\sin { \theta } , find the value of -\begin{vmatrix} 1 & { e }^{ i\pi /3 } & { e }^{ i\pi /4 } \\ { e }^{ -i\pi /3 } & 1 & { e }^{ i2\pi /3 } \\ { e }^{ -i\pi /4 } & { e }^{ -i2\pi /3 } & 1 \end{vmatrix}\quad-{2}^{\frac{1}{2}}
Evaluate \begin{vmatrix} \cos { \alpha } \cos { \beta } & \cos { \alpha } \sin { \beta } & -\sin { \alpha } \\ -\sin { \beta } & \cos { \beta } & 0 \\ \sin { \alpha } \cos { \beta } & \sin { \alpha } \sin { \beta } & \cos { \alpha } \end{vmatrix}
If A,B and C are the angles of non-right angles triangle ABC, then find the value of \begin{vmatrix} \tan { A } & 1 & 1 \\ 1 & \tan { B } & 1 \\ 1 & 1 & \tan { C } \end{vmatrix}
If the value of \begin{vmatrix} 1 & 2 & 4 \\ -1 & 3 & 0 \\ 4 & 1 & 0 \end{vmatrix} is k, then find \dfrac{-k}{13}.
The absolute value of the determinant \begin{vmatrix} -1 & 2 & 1 \\ 3+2\sqrt { 2 } & 2+2\sqrt { 2 } & 1 \\ 3-2\sqrt { 2 } & 2-2\sqrt { 2 } & 1 \end{vmatrix} is \displaystyle\frac { k }{ \sqrt { 2 } } then k=
If \begin{vmatrix} x & x+y & x+y+z \\ 2x & 3x+2y & 4x+3y+2z \\ 3x & 6x=3y & 10x+6y+3z \end{vmatrix}=64, then the real value of x is .........
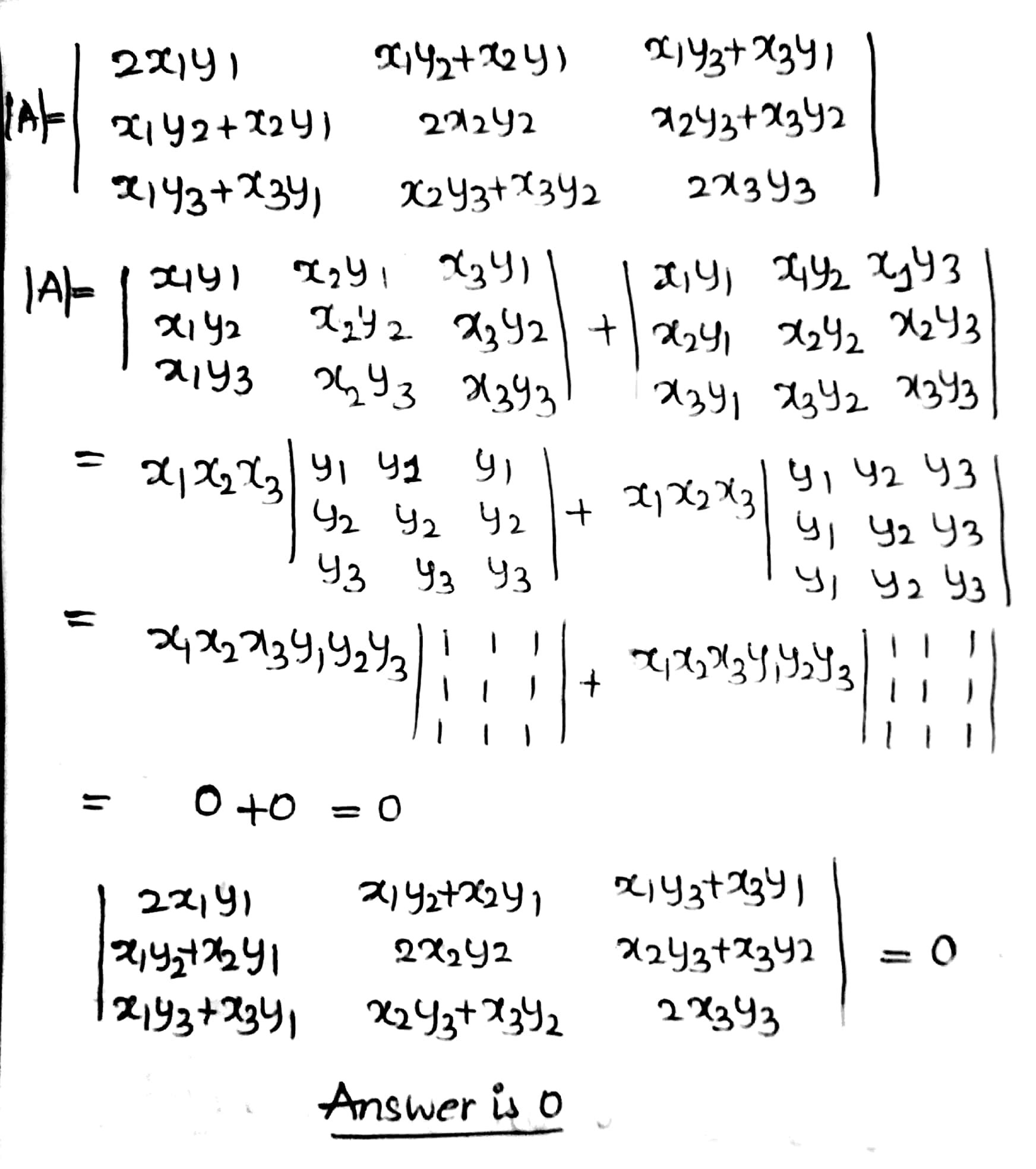
The value of \begin{vmatrix} 2{ x }_{ 1 }{ y }_{ 1 } & { x }_{ 1 }{ y }_{ 2 }+{ x }_{ 2 }{ y }_{ 1 } & { x }_{ 1 }{ y }_{ 3 }+{ x }_{ 3 }{ y }_{ 1 } \\ { x }_{ 1 }{ y }_{ 2 }+{ x }_{ 2 }{ y }_{ 1 } & 2{ x }_{ 2 }{ y }_{ 2 } & { x }_{ 2 }{ y }_{ 3 }+{ x }_{ 3 }{ y }_{ 2 } \\ { x }_{ 1 }{ y }_{ 3 }+{ x }_{ 3 }{ y }_{ 1 } & { x }_{ 2 }{ y }_{ 3 }+{ x }_{ 3 }{ y }_{ 2 } & 2{ x }_{ 3 }{ y }_{ 3 } \end{vmatrix} is ........
The constant k is such that the following system of equations posses a non-trivial(i.e., not all zero) solution over the set of rationals Q
x+ky+3z=0,\quad 3x+ky-2z=0\quad 2x+3y-4z=0
Then \displaystyle \frac{2k}{11} is equal to
If A + B + C = \pi, then \begin{vmatrix} sin (A + B + C)& sin B & cos C\\ - sin B & 0 & tan A\\ cos (A + B) &- tan A &0 \end{vmatrix} = .............
If (1,2), \displaystyle \left(\frac{1}{2} , 3 \right) and (0, k) are collinear points, find the value of k.
State whether True or False(-1, 8), (9, -2), (3,4) are collinear points.
Let
f\left ( x \right )=\begin{vmatrix} 2\cot x & -1 & 0\\ 1 & \cot x & -1\\ 0 & 1 & 2\cot x \end{vmatrix}
then
Let f\left ( \theta \right )=\begin{vmatrix} \cos ^{2}\theta & \cos \theta \sin \theta & -\sin \theta \\ \cos \theta \sin \theta & \sin ^{2}\theta & \cos \theta \\ \sin \theta & -\cos \theta & 0 \end{vmatrix}
find f\left ( \pi /3 \right ).
A_{3 \times 3} is a matrix such that |A|=a, \:B = (adj \:A) such that |B|= b. Find the value of \dfrac{(ab^2 + a^2b + 1)S}{25} where \displaystyle \frac{1}{2}S=\frac{a}{b}+\frac{a^2}{b^3}+\frac{a^3}{b^5} + \:......... \:up \:to \:\infty , and a = 3.
Find the value of x for which the points (x, -1), (2,1) and (4, 5) are collinear.
Find the values of x, if
(i)\begin{vmatrix} 2 & 4 \\ 5 & 1 \end{vmatrix}=\begin{vmatrix} 2x & 4 \\ 6 & x \end{vmatrix} (ii)\begin{vmatrix} 2 & 3 \\ 4 & 5 \end{vmatrix}=\begin{vmatrix} x & 3 \\ 2x & 5 \end{vmatrix}
Using the properties of determinants, show that:
(i)\begin{vmatrix} a-b-c & 2a & 2a \\ 2b & b-c-a & 2b \\ 2c & 2c & c-a-b \end{vmatrix}=(a+b+{ c) }^{ 2 }
(ii)\begin{vmatrix} x+y+2z & x & y \\ z & y+z+2x & y \\ z & x & z+x+2y \end{vmatrix}=2(x+y+{ z) }^{ 3 }
Using the properties of determinants, show that:
(i)\begin{vmatrix} x+4 & 2x & 2x \\ 2x & x+4 & 2x \\ 2x & 2x & x+4 \end{vmatrix}=(5x+4)(4-{ x) }^{ 2 }
(ii)\begin{vmatrix} y+k & y & y \\ y & y+k & y \\ y & y & y+k \end{vmatrix}={ k }^{ 2 }(3y+k)
Find the value of determinant.
(i) \begin{vmatrix} \cos\theta & -\sin\theta \\ \sin\theta & \cos\theta \end{vmatrix}
(ii) \begin{vmatrix} { x }^{ 2 }-x+1 & x-1 \\ x+1 & x+1 \end{vmatrix}
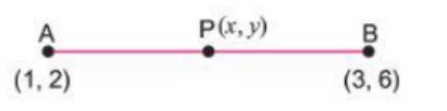
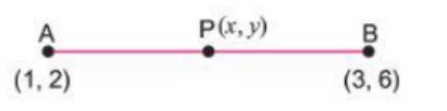
(i) Find equation of line joining (1, 2) and (3, 6) using determinants
(ii) Find equation of line joining (3, 1) and (9, 3) using determinants.
Find co-factors of the matrix,A=\begin{bmatrix} 1 & -1 & 2 \\ 0 & 2 & -3 \\ 3 & -2 & 4 \end{bmatrix}
Write minors and cofactors of the elements of following determinants
(i)\begin{vmatrix} 2 & -4 \\ 0 & 3 \end{vmatrix}
(ii)\begin{vmatrix} a & c \\ b & d \end{vmatrix}
Find the Minors and Cofactors of the elements of the following determinants:(i)\begin{vmatrix} 1 & 0 & 0 \\ 0 & 1 & 0 \\ 0 & 0 & 1 \end{vmatrix}
(ii)\begin{vmatrix} 1 & 0 & 4 \\ 3 & 5 & -2 \\ 0 & 1 & 2 \end{vmatrix}
Find the adjoint of matrix \ \ \ \ A= \begin{bmatrix} 1 & 1 & 2 \\ 2 & 3 & 5 \\ 2 & 0 & 1 \end{bmatrix}
Find co-factors of the matrix,A= \begin{bmatrix} 1 & 0 & 0 \\ 0 & \cos\alpha & \sin\alpha \\ 0 & \sin\alpha & -\cos\alpha \end{bmatrix}
Using properties of determinants, prove that:
\begin{vmatrix} \alpha & { \alpha }^{ 2 } & \beta +\gamma \\ \beta & { \beta }^{ 2 } & \gamma +\alpha \\ \gamma & { \gamma }^{ 2 } & \alpha +\beta \end{vmatrix} =(\alpha- \beta)(\beta- \gamma)(\gamma-\alpha)(\alpha +\beta +\gamma )
Evaluate \begin{vmatrix} \cos\alpha \cos\beta & \cos\alpha \sin\beta & -\sin\alpha \\ -\sin\beta & \cos\beta & 0 \\ \sin\alpha \cos\beta & \sin\alpha \sin\beta & \cos\alpha \end{vmatrix}
If true Enter '1' else '0'.\begin{vmatrix} x & y & x+y \\ y & x+y & x \\ x+y & x & y \end{vmatrix}=-2(x+y)(x^2+y^2-xy)
If true Enter '1' else '0'.
Evaluate \begin{vmatrix} 1 & x & y \\ 1 & x+y & y \\ 1 & x & x+y \end{vmatrix}
Find cofactors of A=\begin{bmatrix} 2 & 1 & 3 \\ 4 & -1 & 0 \\ -7 & 2 & 1 \end{bmatrix}
If A_{ij} is the cofactor of the element a_{ij} of the determinant \begin{bmatrix}2&-3&5\\6&0&4\\1&5&-7 \end{bmatrix} then write the value of a_{32}.A_{32}.
If \triangle = \begin{vmatrix} 5 & 3 & 8 \\ 2 & 0 & 1 \\ 1 & 2 & 3 \end{vmatrix}, white the cofactor of the element a_{32}.
If \Delta = \begin{vmatrix} 5& 3 & 8\\ 2 & 0 & 1\\ 1 & 2 & 3\end{vmatrix}, write the cofactor of the element a_{32}
If \Delta = \begin{vmatrix}1 & 2 & 3\\ 2 & 0 & 1\\ 5 & 3 & 8\end{vmatrix}, write the minor of the elements a_{22}
If \Delta = \begin{vmatrix} 5 & 3 & 8\\ 2 & 0 &1 \\ 1 & 2 & 3\end{vmatrix}, write the minor of the element a_{23}.
Find the relation between x and y if the points A(x, y), B(-5, 7) and C(-4, 5) are collinear.
For how many real values of 'm' the points A (m + 1, 1), B (2m + 1, 3) and C (2m + 2, 2m) are collinear.
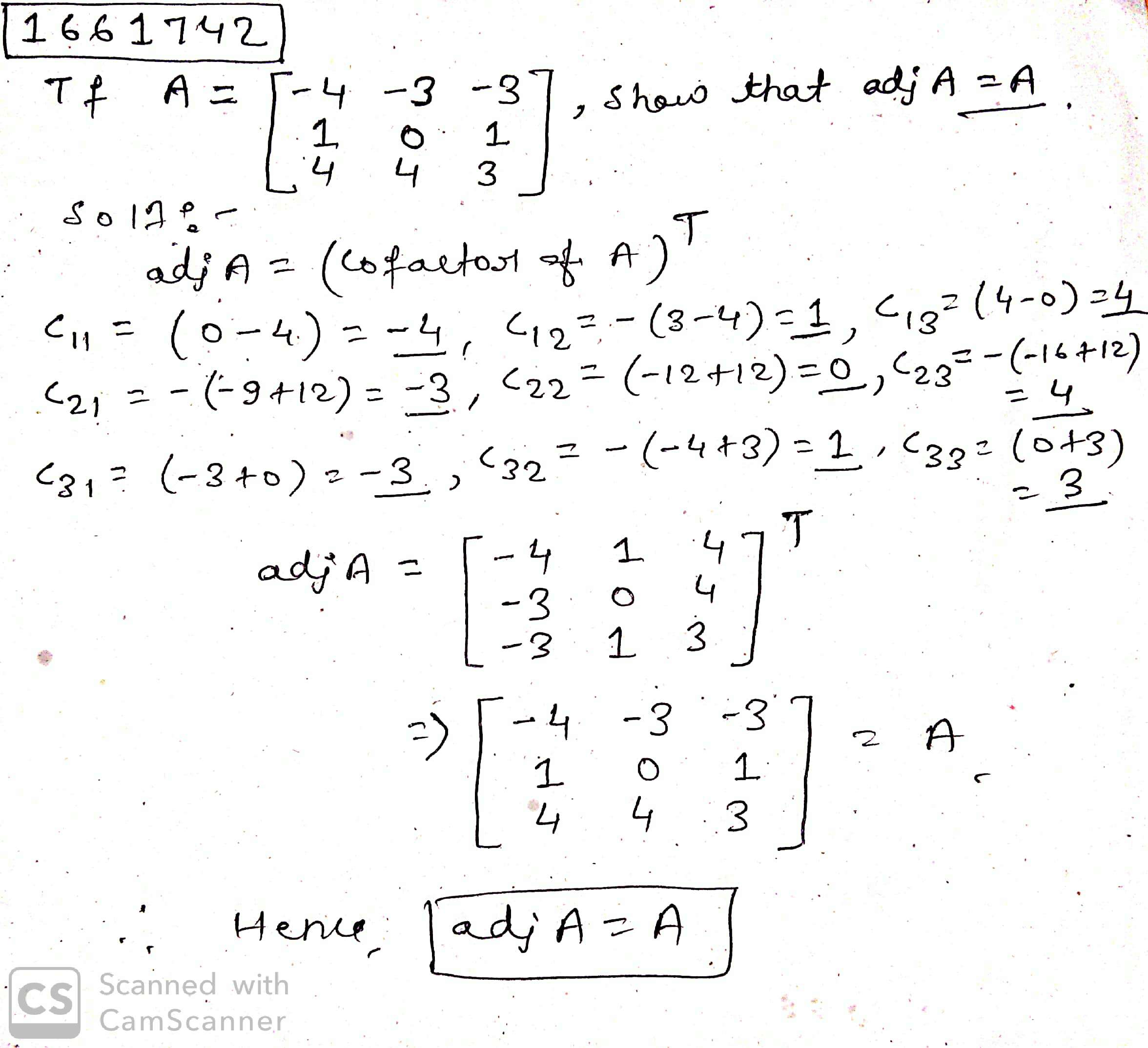
Show that the adjoint of A = \begin{bmatrix}-4 & -3 & -3\\ 1 & 0 & 1\\ 4 & 4 & 3\end{bmatrix} is A itself
Find the value of k if A (4, 11), B (2, 5), C (6, k) are collinear points.
Find the value of a for which the following points A (a, 3), B(2, 1) and C(5, a) are collinear. Hence find the equation of the line.
A, B, and C are three collinear points. The coordinates of A and B are (3, 4) and (7, 7) respectively and AC =10 units. Find sum of co-ordinates of C.
A (3,4) and B(7,7) and C(x,y) or C'(x,y) are collinear points.
AB = √[(7-3)²+(7-4)²] = 5
AC = 10, given.
Slope of AC = slope of AB = (7-4)/(7-3) = 3/4
=> (y-4)/(x-3) = 3/4 --- (1)
=> 4 y - 3 x = 7 --- (2)
AC² = 10² = (y - 4)² + (x - 3)²
= [ 3/4 * (x - 3) ]² + (x-3)²
= (x-3)² * 25/16
=> x - 3 = + 8
=> x = +11 or -5
=> y = (7+3x)/4
= 10 or -2
C = (11, 10) and C' = (-5, -2)
Using determinants show that points A (a, b + c), B(b, c + a) and C (c, a + b) are collinear.
find the value of k for which the points (k,-1)(2,1) and (4,5) are collinear.
Show that the points A(-3,3), B(0,0), C(3,-3) are collinear.
If A=\begin{bmatrix}1&2\\3&4\end{bmatrix}, find |2A|.
Find the equation of line joining (1, 2) and (3, 6) using determinants.
Calculate the values of the determinants:
\begin{vmatrix}a & h & g\\ h& b & f\\ g & f & c\end{vmatrix}.
For what values of m will the expression {y}^{2}+2xy+2x+my-3 be capable of resolution into two rational factors?
Calculate the values of the determinants:
\begin{vmatrix}1 & 1 & 1\\ 1& 1 + x & 1\\ 1 & 1 & 1 + y\end{vmatrix}.
Calculate the values of the determinants:
\begin{vmatrix}1 & z & -y\\ -z& 1 & x\\ y & -x & 1\end{vmatrix}.
Solve for \lambda if \begin{vmatrix} a^2+\lambda & ab & ac\\ ab & b^2+\lambda & bc\\ ac & bc & c^2+\lambda\end{vmatrix}=0.
Calculate the values of the determinants:
\begin{vmatrix}b + c & a & a\\ b& c + a & b\\ c & c & a + b\end{vmatrix}.
Prove that \begin{vmatrix} 1+a & 1 & 1\\ 1& 1+b & 1\\ 1& 1& 1+c\end{vmatrix} =abc\displaystyle (1+\frac{1}{a}+\frac{1}{b}+\frac{1}{c}).
Find the area of \triangle PQR whose vertices are P(2,1),Q(3,4) and R(5,2).
Find the equation of the line joining A(1,3) and B(0,0) using determine and find k of D(k,0) is a point such that area of \triangle ABD is 3 sq. units.
If points \left( a,0 \right) ,\left( 0,b \right) and \left( x,y \right) are collinear, prove that \cfrac { x }{ a } +\cfrac { y }{ b } =1.
If the points A(-2, 1), B(a, b) and C(4, -1) are collinear and a-b=1, find the values of a and b.
Find the ratio in which the line segment joining the points P(x, 2) divides the line segment joining the points A (12, 5) and B(4,-3). Also find the value of x.
Evaluate :
\begin{vmatrix} 2& 3 & -5\\ 7 &1 & -2\\ -3 & 4 &1 \end{vmatrix}
If three points \left( { x }_{ 1 },{ y }_{ 1 } \right) ,\left( { x }_{ 2 },{ y }_{ 2 } \right) ,\left( { x }_{ 3 },{ y }_{ 3 } \right) lie on the same line, prove that
\cfrac { { y }_{ 2 }-{ y }_{ 3 } }{ { x }_{ 2 }{ x }_{ 3 } } +\cfrac { { y }_{ 3 }-{ y }_{ 1 } }{ { x }_{ 3 }{ x }_{ 1 } } +\cfrac { { y }_{ 1 }-{ y }_{ 2 } }{ { x }_{ 1 }{ x }_{ 2 } } =0
Show that the following set of points are collinear.
(2,5), (4,6) and (8,8)
If a\ne b\ne c, prove that the points \left( a,{ a }^{ 2 } \right) ,\left( b,{ b }^{ 2 } \right) ,\left( c,{ c }^{ 2 } \right) can never be collinear.
Find the value of k if points (k,3),(6,-2) and (-3,4) are collinear.
For what value of a the point (a,1),(1,-1) and (11,4) are collinear?
For what the value of a (a,1)\quad (1,-1)\quad and\quad (11,4) are collinear.
Slope of AB=Slop of BC
\cfrac { -1-1 }{ 1-a } =\cfrac { 4+1 }{ 11-1 } \\ \cfrac { -2 }{ 1-a } =\cfrac { 5 }{ 10 } \\ 1-a=-4\\ a=5\quad
For this value, the points will be collinear.
Evaluate :
\Delta=\begin{vmatrix} \cos \alpha \cos \beta& \cos \alpha \sin \beta & -\sin \alpha\\ -\sin \beta &\cos \beta & 0\\ \sin \alpha \cos \beta & \sin \alpha \sin \beta &\cos \alpha \end{vmatrix}
Prove that the points \left( ab, \right) ,\left( { a }_{ 1 },{ b }_{ 1 } \right) and \left( a-{ a }_{ 1 },b-{ b }_{ 2 } \right) are collinear if a{ b }_{ 1 }={ a }_{ 1 }b
Prove that the points (a,0),(0,b) and (1,1) are collinear if \left ( \cfrac { 1 }{ a } +\cfrac { 1 }{ b } =1 \right )
Prove that the points (2,3), (-4,-6) and 1,3/2) do not form a triangle.
Find the value of \lambda if the following equations are consistent
x + y - 3 = 0
(1 + \lambda) x + (2 + \lambda) y - 8 = 0
x - (1 + \lambda) y + (2 + \lambda) = 0
Find cofactors of the elements of the matrix A = \begin{bmatrix} -1 & 2 \\ -3 & 4 \end{bmatrix}
Find the value of k, if the points A(8,1),B(3,-4) and C(2,k) are collinear.
If the points A(-1,-4),B(b,c) and C(5,-1) are collinear and 2b+c=4, find the values of b and c.
Find the value(s) of k for which the points (3k-1,k-2),(k,k-7) and (k-1,-k-2) are collinear.
Find the value of k, if the points A(7,-2),B(5,1) and C(3,2k) are collinear.
Let a > 0,\ d > 0. Find the value of the determinant
\left| \begin{matrix} \dfrac { 1 }{ a } & \dfrac { 1 }{ a\left( a+d \right) } & \dfrac { 1 }{ \left( a+d \right) \left( a+2d \right) } \\ \dfrac { 1 }{ \left( a+d \right) } & \dfrac { 1 }{ \left( a+d \right) \left( a+2d \right) } & \dfrac { 1 }{ \left( a+2d \right) \left( a+3d \right) } \\ \dfrac { 1 }{ \left( a+2d \right) } & \dfrac { 1 }{ \left( a+2d \right) \left( a+3d \right) } & \dfrac { 1 }{ \left( a+3d \right) \left( a+4d \right) } \end{matrix} \right|
Prove the following :
\left| \begin{matrix} ax & by & cz \\ { x }^{ 2 } & { y }^{ 2 } & { z }^{ 2 } \\ 1 & 1 & 1 \end{matrix} \right| =\left| \begin{matrix} a & b & c \\ x & y & z \\ yz & zx & xy \end{matrix} \right|
Without expanding prove that the determinant \left | \begin{array}{111} sinA & CosA & sin(A+\theta) \\ sin B & cos B & sin(B+\theta) \\ sin C & CosC & sin (c+\theta) \\ \end {array} \right | =0
A, B , C are the points (a, p), (b,q) and (c,r) respectively such that a, b, c are in A. P. and p, q ,r in G.P. If the points are collinear then prove that p = q = r
If x,y,z \in R then find Determinant\left( {\matrix{ {{{({2^x} + {2^{ - x}})}^2}} & {{{({2^x} - {2^{ - x}})}^2}} & 1 \cr {{{({3^x} + {3^{ - x}})}^2}} & {{{({3^x} - {3^{ - x}})}^2}} & 1 \cr {{{({4^x} + {4^{ - x}})}^2}} & {{{({4^x} - {4^{ - x}})}^2}} & 1 \cr } } \right)
If A = diag (a, b, c) = \begin{bmatrix} a & 0 & 0 \\[0.3em] 0 & b & 0 \\[0.3em] 0 & 0 & c \end{bmatrix} such that abc \neq 0
then A^{-1} = diag (\frac{1}{a}, \frac{1}{b}, \frac{1}{c}) = \begin{bmatrix} \frac{1}{a} & 0 & 0 \\[0.3em] 0 & \frac{1}{b} & 0 \\[0.3em] 0 & 0 & \frac{1}{c} \end{bmatrix}
If where r = 1,2,3 be the co-ordinates of the points A, B, C respectively, then prove the following:
The equation of internal bisector of angel A is
b \, \begin{vmatrix} x & y & 1\\ x_1 & y_1 & 1\\ x_2 & y_2 & 1 \end{vmatrix} \, + \, c \, \begin{vmatrix} x & y & 1\\ x_1 & y_1 & 1\\ x_3 & y_3 & 1 \end{vmatrix} \, = \, 0
where b = AC and c = AB.
If A is a matrix of order 3 \times 3 then find \left| adj\ A \right| where \left| A \right| =2
Find a if \begin{vmatrix} i & -2i & -1\\ 3i & i^{3} & -2\\ 1& -3 & -i\end{vmatrix} = ai, where i =\sqrt{-1}
Find the non-zero roots of the equation,
\Delta = \begin{vmatrix} a & b & ax + b \\ b & c & bx + c \\ ax + b & bx + c & c\end{vmatrix} = 0
Solve: \begin{vmatrix} 3x+4 & x+2 & 2x+3 \\ 4x+5 & 2x+3 & 3x+4 \\ 10x+17 & 3x+5 & 5x+8 \end{vmatrix}=0. The value of the determinant at x=-1is:
By using properties of determinants prove that \begin{vmatrix} (y+z)^{2}&xy& zx\\ xy&(x+z)^{2} & yz\\ xz & yz & (x+y)^{2}\end{vmatrix} is divisible by (x+ y + z)^{n}. Find n .
Find n if: \begin{vmatrix} 1+a^{ 2 }-b^{ 2 } & 2ab & -2b \\ 2ab & 1-a^{ 2 }+b^{ 2 } & 2a \\ 2b & -2a & 1-a^2-b^2 \end{vmatrix} = (1 + a^2 + b^2)^n
\begin{vmatrix} 1+a & 1 & 1 \\ 1 & 1+b & 1 \\ 1 & 1 & 1+c \end{vmatrix}=abc\left(1+ \dfrac { 1 }{ a } +\dfrac { 1 }{ b } +\dfrac { 1 }{ c } \right)
Evaluate:
\begin {vmatrix} x^{2} -x +1 & x-1\\ x+1 & x+1\end{vmatrix}
Prove that \begin{vmatrix} \left( \beta +\gamma -\alpha -\delta \right) ^{ 4 } & \left( \beta +\gamma -\alpha -\delta \right) ^{ 2 } & 1 \\ \left( \gamma +\alpha -\beta -\delta \right) ^{ 4 } & \left( \gamma +\alpha -\beta -\delta \right) ^{ 2 } & 1 \\ \left( \alpha +\beta -\gamma -\delta \right) ^{ 4 } & \left( \alpha +\beta -\gamma -\delta \right) ^{ 2 } & 1 \end{vmatrix}=-64\left( \alpha -\beta \right) \left( \alpha -\gamma \right) \left( \alpha -\delta \right) \left( \beta -\gamma \right) \left( \beta -\delta \right) \left( \gamma -\delta \right)
Minor m_{33} of the determinant \begin{vmatrix} 2 & 3 & 5 \\ 2 & -1 & 8 \\ 1 & 2 & 4 \end{vmatrix} is
Prove \begin{vmatrix} x+4 & 2x & 2x\\ 2x & x+4 & 2x \\ 2x & 2x & x+4\end{vmatrix}=(5x+4)(4-x)^2.
Find the adjoint of matrix A =\begin{bmatrix}2&0&1\\3&1&2\\-1&1&2\end{bmatrix}
Find the adjoint of { \left( \dfrac { { 7 }^{ -4 } }{ { 4 }^{ -2 } } \right) }^{ \dfrac { 1 }{ 4 } }\begin{bmatrix} 1 & -1 & 2 \\ 3 & 0 & -2 \\ 1 & 0 & 3 \end{bmatrix}
If x, y, z are non-zero real numbers and \begin{vmatrix} 1+x & 1 & 1 \\ 1+y & 1+2y & 1 \\ 1+z & 1+z & 1+3z \end{vmatrix}=0 then -\left( \dfrac { 1 }{ x } +\dfrac { 1 }{ y } +\dfrac { 1 }{ z } \right) is equal to________
Find x if \begin{bmatrix} 1 & 0 & 0 \\ 2 & 3 & 4 \\ 5 & -6 & x \end{bmatrix}=45
Find the adjoint of \begin{bmatrix} 1 & -1 & 2 \\ 3 & 0 & -2 \\ 1 & 0 & 3 \end{bmatrix}
Prove that \begin{vmatrix}ax & by & cz\\x^{2}& y^{2}& z^{2}\\ 1&1&1 \end{vmatrix} = \begin{vmatrix}a&b&c\\x&y&z\\yz&xz&xy \end{vmatrix}
Solve for x:\begin{vmatrix}1&5&2\\2&6&4\\3&7&x\end{vmatrix}=0
Prove that \triangle =\begin{vmatrix} a & b & a\alpha +b \\ b & c & b\alpha +c \\ a\alpha +b & b\alpha +c & 0 \end{vmatrix}=0 if a,b,c are in G.P.
Show that:\left| \begin{matrix} 1 & { a }^{ 2 } & { a }^{ 3 } \\ 1 & { b }^{ 2 } & { b }^{ 3 } \\ 1 & { c }^{ 2 } & { c }^{ 3 } \end{matrix} \right| =(a-b)(b-c)(c-a)(ab+bc+ca)
show that \left| \begin{matrix} 1 & a & { a }^{ 2 } \\ 1 & b & b^{ 2 } \\ 1 & c & c^{ 2 } \end{matrix} \right| =\left( a-b \right) \left( b-c \right) \left( c-a \right)
Find the solution set of \left| {\begin{array}{*{20}{c}}x&5&9\\{16}&{3x\, + \,8}&{36}\\3&1&7\end{array}} \right|\, = \,0.
Solve:\left| {\begin{array}{*{20}{c}}1&1&1\\1&{1 + \sin \theta }&1\\{1 + \cos \theta }&1&1\end{array}} \right|
Solve:
\begin{vmatrix} 1 & 1 & 2 \\ 1 & -2 & 3 \\ 1 & (x+1) & x \end{vmatrix}=0
Find the area of quadrilateral ABCD, the co ordinates of whose vertices are A(-3,2), B(5,4), C(7,-6), D(-5,-4)
Prove that, \left| \begin{matrix} x+\lambda & 2x & 2x \\ 2x & x+\lambda & 2x \\ 2x & 2x & x+\lambda \end{matrix} \right| =(5x+\lambda)( \lambda -x)^{2}.
Show that \begin{vmatrix} b+c & c+a & a+b \\ a+b & b+c & c+a \\ a & b & c \end{vmatrix} = a^3 + b^3 +c^3 - 3abc
If a \ne p, b \ne q and c \neq r \begin{vmatrix} p & b & c \\ a & q & c \\ a & b & r \end{vmatrix}= 0 then, find the value of :-
\dfrac{p}{p-a} + \dfrac{q}{q-b} + \dfrac{r}{r-c} = ?
It should have some corrections in the answer
Find the value of following determinant.
\begin{vmatrix} -1 & 7\\ 2 & 4\end{vmatrix}.
Find the value of the following determinant.
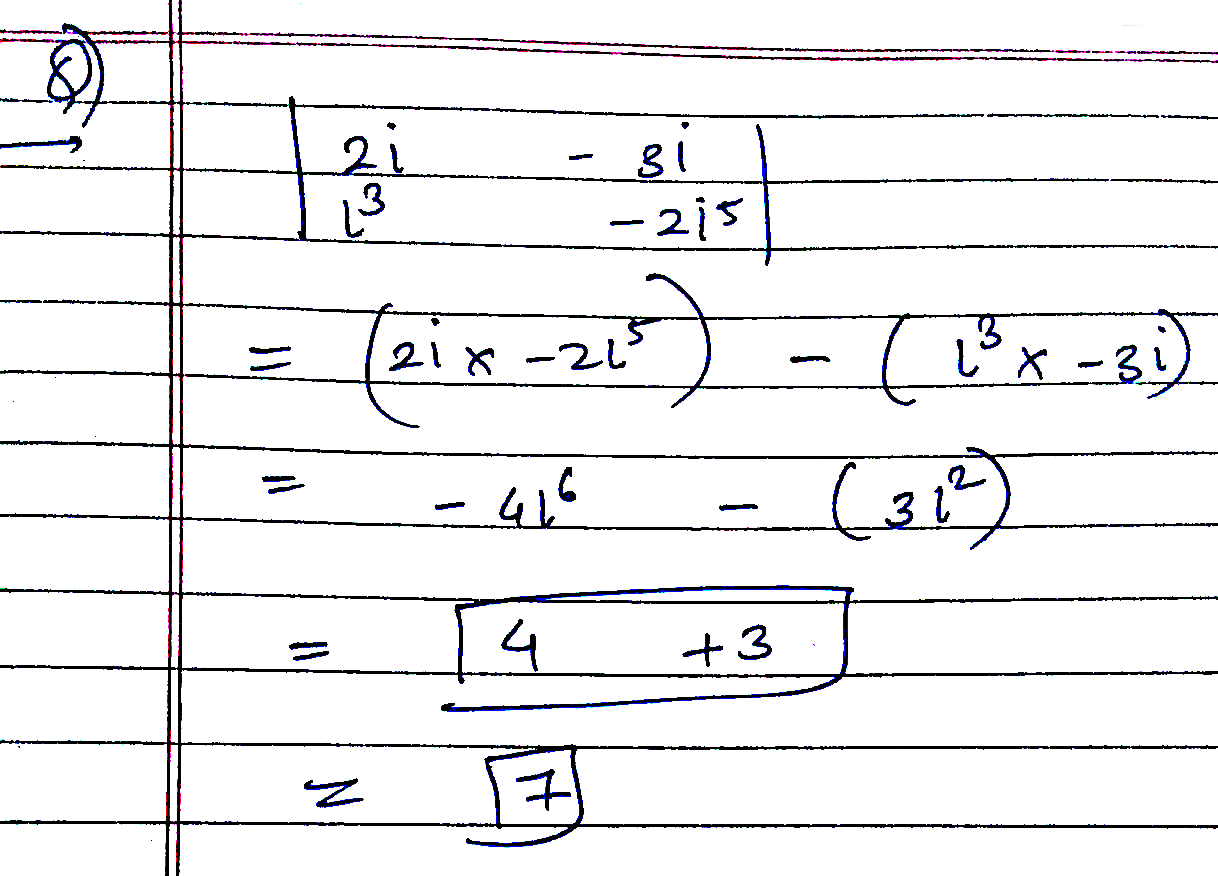
\begin{vmatrix} 2i & -3i \\ { i }^{ 3 } & -2{ i }^{ 3 } \end{vmatrix}, where i=\sqrt {-1}.
If f\left( x \right) = a + bx + c{x^2} and \alpha ,\beta ,\gamma are the roots of the equation {x^3} = 1, then \left| {\begin{array}{*{20}{c}}a & b & c\\b & c & a\\c & a & b\end{array}} \right| is equal to
If A is a skew-symmetric matrix of order 3 then find \left| A \right|
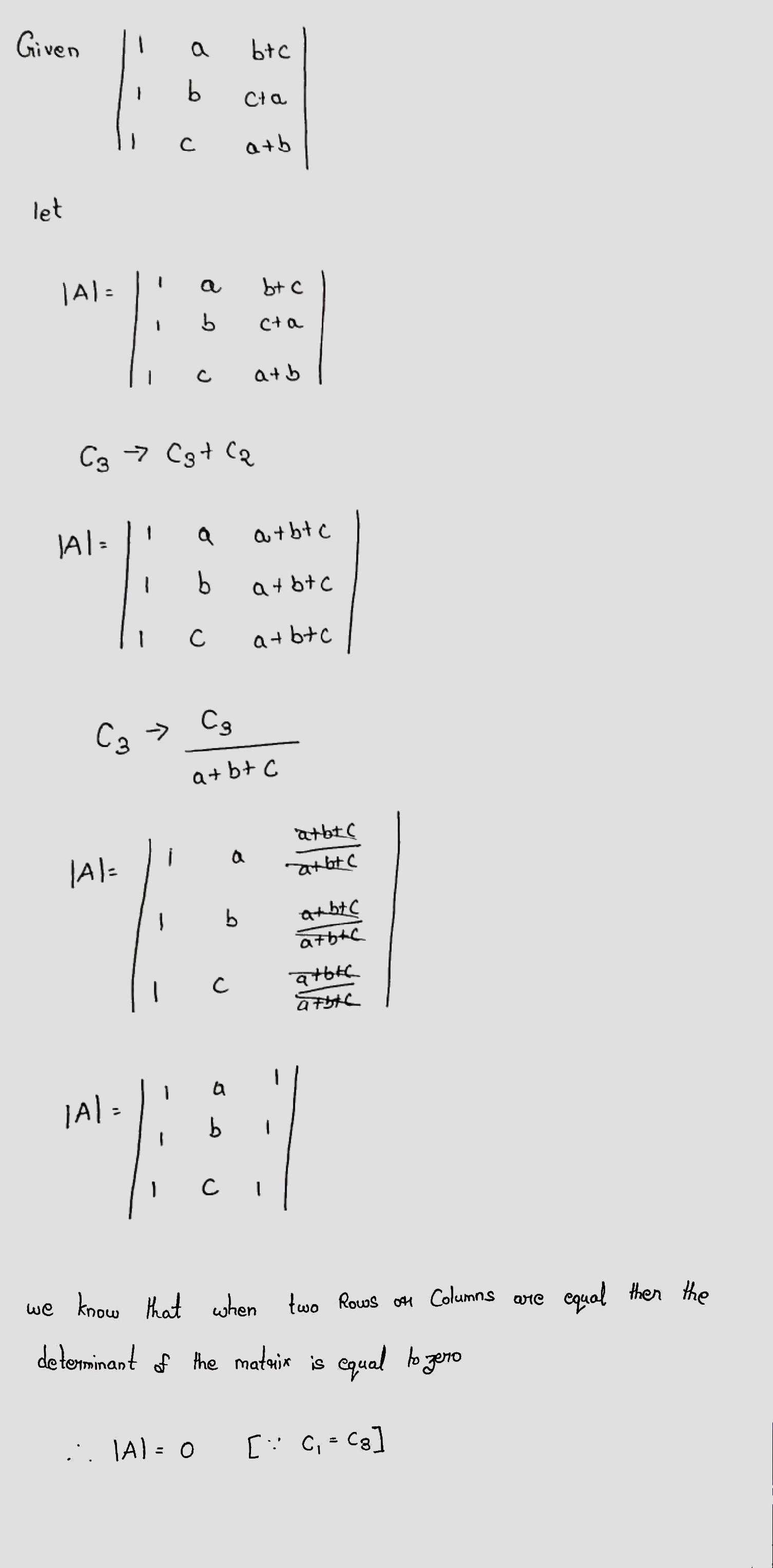
Evaluate \left| \begin{matrix} 1 & a & b+c \\ 1 & b & c+a \\ 1 & c & a+b \end{matrix} \right| without direct expansion.
Find the value of the determinant: \begin{vmatrix} \sqrt{2} & 2\sqrt{3}\\ 3\sqrt{2} & \sqrt{3}\end{vmatrix}.
\begin{vmatrix}1 & 2 & 3\\ 0 & 2 & 4\\ 0 & 0 & 5\end{vmatrix}.
If \vec { a } =\tilde { i } +\tilde { j } +\tilde { k } ,\vec { b } =\tilde { i } -\tilde { j } +\tilde { k } ,\vec { c } =\tilde { i } +2\tilde { j } -\tilde { k } , then the value of \left| \begin{matrix} \overrightarrow { a } .\overrightarrow { a } & \overrightarrow { a } .\overrightarrow { b } & \overrightarrow { a } .\overrightarrow { c } \\ \overrightarrow { b } .\overrightarrow { a } & \overrightarrow { b } .\overrightarrow { b } & \overrightarrow { b } .\overrightarrow { c } \\ \overrightarrow { c } .\overrightarrow { a } & \overrightarrow { c } .\overrightarrow { b } & \overrightarrow { c } .\overrightarrow { c } \end{matrix} \right| =
Prove that \left| \begin{matrix} a-b-c & 2a & 2a \\ 2b & b-c-a & 2b \\ 2c & 2c & c-a-b \end{matrix} \right| =(a+b+c)^3
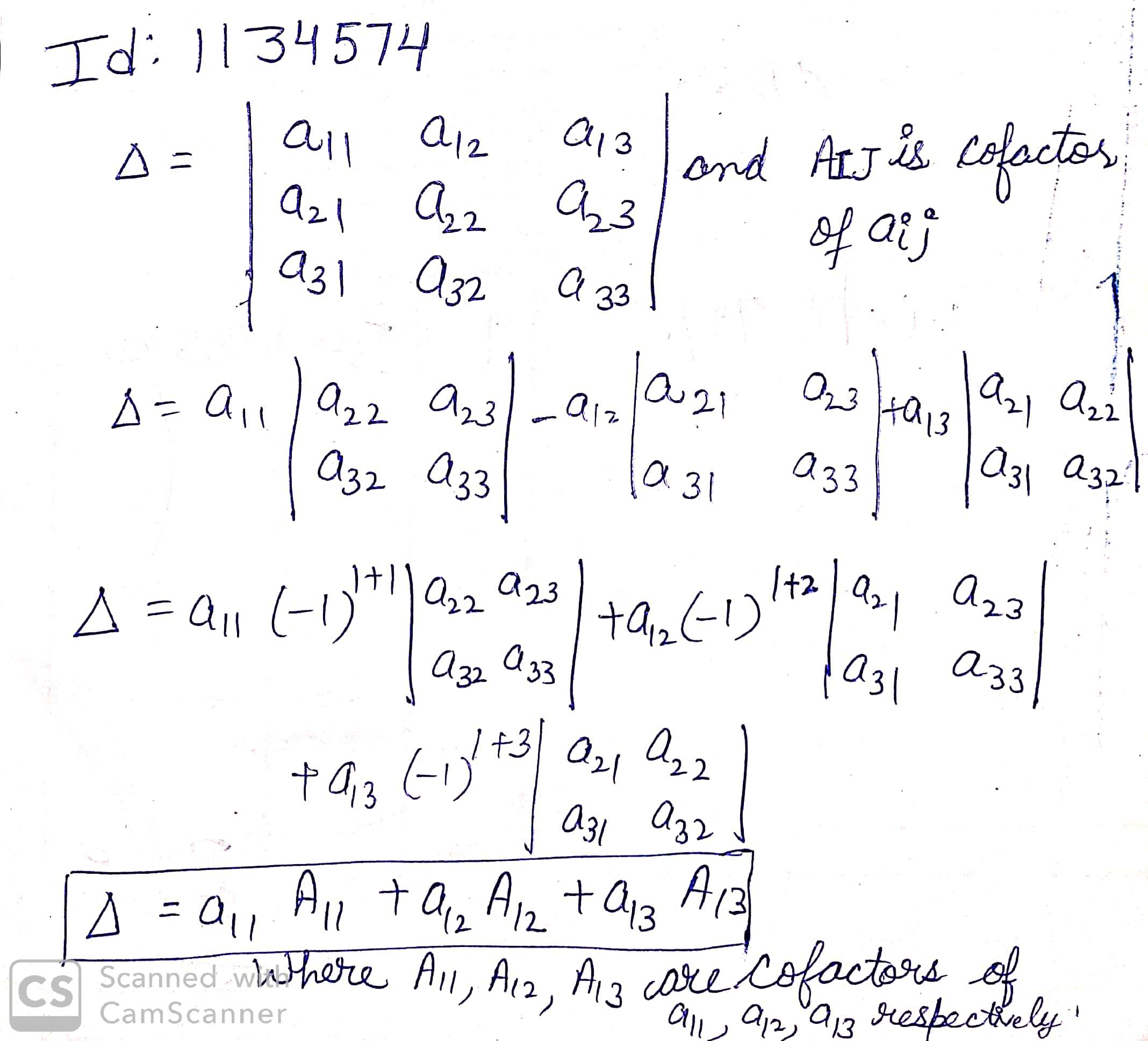
If \Delta=\left| \begin{matrix} { a }_{ 11 } & { a }_{ 12 } & { a }_{ 13 } \\ { a }_{ 21 } & { a }_{ 22 } & { a }_{ 23 } \\ { a }_{ 31 } & { a }_{ 32 } & { a }_{ 33 } \end{matrix} \right| and A_{IJ} is cofactors of a_{ij} then the value of \Delta is given by
\left| \begin{matrix} 1 & w & { w }^{ 2 } \\ w & { w }^{ 2 } & 1 \\ { w }^{ 2 } & w & 1 \end{matrix} \right| Where w is a complex cube root of unity.
Find the value of k if points \left (k,3\right),\left (6,-2\right)\ and \left (-3,4\right) are collinear.
(k,3),(6,-2),(-3,4) are
collinear
here x_1 = k, y_1 = 3
x_2 = 6, y_2 = -2
x_3 = -3,y_3 = 4
So, as given that the points are collinear, then,
x_1(y_2-y_3)+x_2(y_3-y_1)+x_3(y_1-y_2) = 0
k(-2-4)+6(4-3)+3(3+2) = 0
k(-6)+6(1)+(-3)\times 5 = 0
-6k+6-15 = 0
-6k -9 = 0
k = \dfrac{9}{6} = \dfrac{3}{2}
Find the value of \begin{vmatrix} 53 & 106 & 159 \\ 52 & 65 & 91 \\ 102 & 153 & 221 \end{vmatrix}
Solve \left| \begin{matrix} x & -4 & -4 \\ 3 & 2 & 1 \\ -2 & 4 & 1 \end{matrix} \right| =0
Find the value of K if the point A (2,3) ,B (4,K) and C( 6,-3) are collinear?
Find the values of the following determinants
\begin{vmatrix} 1+3i & i-2 \\ { -i-2 } & 1-3{ i } \end{vmatrix}
where i=\sqrt{-1}.
\begin{vmatrix} 1+3i & i-2\\ -i-2 & 1-3i\end{vmatrix}
=(1+3i)(1-3i)-(2-2)(-i-2)
=(1-9i^2)-(-1^2-2i+2i+4)
=(1+9)-(1+4)
=10-5
=5.
If \left| \begin{matrix} a & b & a\alpha +b \\ b & c & b\alpha +c \\ a\alpha +b & b\alpha +c & 0 \end{matrix} \right| =0. Prove that a,b,c are in G.P. or \alpha is a root of ax^{2}+2bx+c=0
Without expanding , find the value of \begin{vmatrix} a+b & 2a+b & 3a+b \\ 2a+b & 3a+b & 4a+b \\ 4a+b & 5a+b & 6a+b \end{vmatrix}
Find the roots of equation \left| {\begin{array}{*{20}{c}}1 & 4 & {20}\\1 & { - 2} & 5\\1 & {2x} & {5{x^2}}\end{array}} \right| = 0.
Solve
\left| {\begin{array}{*{20}{c}}1&1&{ - 1}\\6&4&{ - 5}\\{ - 4}&{ - 2}&3\end{array}} \right|
Verify whether the points (1,5),(2,3),and(-2,-1) are collinear or not.
Solve D = \left| \begin{array} { c c c } { 1 } & { - 2 } & { 1 } \\ { 2 } & { 1 } & { - 1 } \\ { 1 } & { 3 } & { 1 } \end{array} \right]
\left| \begin{matrix} 0 \\ -a \\ b \end{matrix}\begin{matrix} a \\ 0 \\ c \end{matrix}\begin{matrix} -b \\ -c \\ 0 \end{matrix} \right| =0
Prove that points (2,-2),(-3,8),(-1,4) are collinear.
If the points A\ (x,2),B\ (-3,-4),C\ (7,-5) are collinear, then find the value of x.
A(X,2) B(-3,-4) C(7,-5)
x= ?
slope AB = slope BC = slope AC
\frac{-3-x}{-4-2} = \frac{7+3}{-5+4} = \frac{7-x}{-5-2}
\frac{x+3}{6} = -10 = \frac{x-7}{7}
x = -63
Evaluate :
\left| { \begin{array} { *{ 20 }{ c } }3 & { -1 } & { -2 } \\ 0 & 0 & { -1 } \\ 3 & { -5 } & 0 \end{array} } \right|
Find minor & cofactors of elements '6', '5', '0' & '4' of the determinant \begin{vmatrix} 2 & 1 & 3 \\ 6 & 5 & 7 \\ 3 & 0 & 4 \end{vmatrix}
Solve:\left| \begin{matrix} 1 & xy & xy(x+y) \\ 1 & yz & yz(y+z) \\ 1 & zx & zx(2+x) \end{matrix} \right|
\left| {\begin{array}{*{20}{c}}2&1&1\\1&{ - 2}&{ - 3}\\3&2&4\end{array}} \right| = ?
Find x if:\left| \begin{matrix} 2 & 1 & x+1 \\ -1 & 3 & -4 \\ 0 & -5 & 3 \end{matrix} \right| = 1
Show that:
\left| \begin{array} { c c c } { a ^ { 2 } } & { b ^ { 2 } } & { c ^ { 2 } } \\ { a } & { b } & { c } \\ { 1 } & { 1 } & { 1 } \end{array} \right| = - ( a - b ) ( b - c ) ( c - a )
If \Delta=\begin{vmatrix} 1 & \sin{\theta} & 1\\ -\sin{\theta} & 1 & \sin{\theta} \\ -1 & -\sin{\theta} & 1 \end{vmatrix} then prove that 2\le \Delta\le 4
Expand:\begin{vmatrix} 3 & 2 & 5\\ 9 & -1 & 4 \\ 2 & 3 & -5 \end{vmatrix}
Expand:\begin{vmatrix} 1 & 2 & 3\\ 4 & 6 & 2 \\ 5 & 9 & 4 \end{vmatrix}
Prove that
\begin{vmatrix} bc & a & { a }^{ 2 } \\ ca & b & { b }^{ 2 } \\ ab & c & { c }^{ 2 } \end{vmatrix}=\begin{vmatrix} 1 & { a }^{ 2 } & { a }^{ 3 } \\ 1 & { b }^{ 2 } & { b }^{ 3 } \\ 1 & { c }^{ 2 } & { c }^{ 3 } \end{vmatrix}
\begin{vmatrix} bc & a & a^2\\ ca & b & b^2\\ ab & c & c^2\end{vmatrix}
on multiply and divide abc
\dfrac{abc}{abc}\begin{vmatrix} bc & a & a^2\\ ca & b & b^2\\ ab & c & c^2\end{vmatrix}
R_1, R_2, R_3
\dfrac{1}{abc}\begin{vmatrix} abc & a^2 & a^3\\ abc & b^2 & b^3\\ abc & c^2 & c^3\end{vmatrix}
Common from C_1 to abc
\dfrac{abc}{abc}\begin{vmatrix} 1 & a^2 & a^3\\ 1 & b^2 & b^3\\ 1 & c^2 & c^3\end{vmatrix}
\begin{vmatrix} 1 & a^2 & a^3\\ 1 & b^2 & b^3\\ 1 & c^2 & c^3\end{vmatrix}.
R.H.S.
Find the value of k so that point colinear(7,-2)(5,1)(3,k).
Solve
\left| \begin{matrix} 4 \\ 3 \end{matrix}\begin{matrix} x+1 \\ x \end{matrix} \right| =5
If for any 2 x 2 square matrix A, A(adj A) = \left[ \begin{array}{l}8\,\,0\\0\,\,8\end{array} \right],then write the value of \left| A \right| .
Find the value of the determinant \begin{vmatrix} -1 & 2 & 1\\ 3+2\sqrt{2} & 2+2\sqrt{2} & 1 \\ 3-2\sqrt{2} & 2-2\sqrt{2} & 1 \end{vmatrix}
Show that \begin{vmatrix} a & \quad a+b & \quad a+b+c\quad \\ 2a & 3a+2b & 4a+3b+2c \\ 3a\quad & 6a+3b & 10a+6b+3c \end{vmatrix}={ a }^{ 3 }
L.H.S = \begin{vmatrix} a & \quad a+b & \quad a+b+c\quad \\ 2a & 3a+2b & 4a+3b+2c \\ 3a\quad & 6a+3b & 10a+6b+3c \end{vmatrix}
R_{2}\rightarrow R_{2}-2R_{1}, R_{3}\rightarrow R_{3}-2R_{1}
= \begin{vmatrix} a & \quad a+b & \quad a+b+c\quad \\ 0 & a & 2a+b \\ 0\quad & 3a & 7a+3b \end{vmatrix}
Expanding along first column
= a[a(7a+3b)-3a(2a+b)]-0+0
= a[7a^{2}+3ab-6a^{2}-3ab]
= a(a)^{2} = a^{3} = R.H.S.
Prove that \begin{vmatrix} {a}^{2}+1 & ab & ac\\ ab & {b}^{2}+1 & bc \\ ac & bc & {c}^{2}+1 \end{vmatrix}=1+{a}^{2}+{b}^{2}+{c}^{2}
Solve for x\begin{vmatrix} 4x & 6x+2 & 8x+1\\ 6x+2 & 9x+3 & 12x \\ 8x+1 & 12x & 16x+2 \end{vmatrix}=0
Given that matrix A=\left[ \begin{matrix} x & 3 & 2 \\ 1 & y & 4 \\ 2 & 2 & z \end{matrix} \right]. If xyz=2013 and 8x+4y+3z=2012 then A(adj A) is equal to
If f(x)=\left| \begin{matrix} 2x & { x }^{ 2 } & { x }^{ 3 } \\ { x }^{ 2 }+2x & 1 & 3x+1 \\ 2x & 1-3{ x }^{ 2 } & 5x \end{matrix} \right| , then find f'(1)
Que. f(x)=\left| \begin{matrix} 2x & { x }^{ 2 } & { x }^{ 3 } \\ { x }^{ 2 }+2x & 1 & 3x+1 \\ 2x & 1-3{ x }^{ 2 } & 5x \end{matrix} \right| , then f'(1)
f(x) = 2x(5x-(3x+1)(1-3x^{2}))-x^{2}(5x(x^{2}+2x)-2x(3x+1))+x^{3}((x^{2}+2x)(1-3x^{2})-2x)
= 2x(5x-(3x-9x^{3}+1-3x^{2}))-x^{2}(5x^{3}+10x^{2}-6x^{2}-2x)+x^{3}(x^{2}-3x^{4}+2x-6x^{3}-2x)
= 2x(2x+9x^{3}+3x^{2}-1)-x^{2}(5x^{3}+10x^{2}-6^{2}-2x)+x^{3}(-3x^{4}-6x^{3}+x^{2})
= 4x^{2}+18x^{4}+6x^{3}-2x-5x^{5}-10x^{4}+6x^{4}+2x^{3}-3x^{7}-6x^{6}+x^{5}
f(x) = -3x^{7}-6x^{6}-4x^{5}+14x^{4}+8x^{3}+4x^{2}-2x
f^{1}(x) = -3\times 7x^{6}-6\times 6x^{5}-4\times 5x^{4}+14\times 4x^{3}+8\times 3x^{2}+4\times 2x-2
f^{1}(x) = -21\times x^{6}-36x^{5}-20x^{4}+56x^{3}+24x^{2}+8x-2
f^{1}(1) = -21-36-20+56+24+8-2
= -79+88
f^{1}(1) = 9
Show that the points A(1,2,7),\ B(2,6,3) and C(3,10,-1) are collinear.
(k,k ), (2,3) and (4,-1) are collimear. So find the value of k.
Solve the matrix
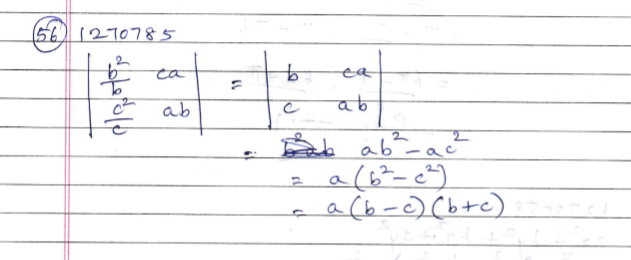
\left| {\begin{array}{*{20}{c}}{\frac{1}{b}{b^2}} & {ca}\\{\frac{1}{c}{c^2}} & {ab}\end{array}} \right|
Prove that point (1,1),(-2,7) and (3,-3) are collinear.
If the points ( a,0),(0,b)and (3,2) are col linear, prove that \dfrac { 2 }{ b } +\dfrac { 3 }{ a } =1
Evaluate \left| \begin{matrix} x & x^{ 2 } & x^{ 2 } \\ y & y^{ 2 } & y^{ 2 } \\ z & z^{ 2 } & z^{ 3 } \end{matrix} \right|
Prove that.\left| \begin{matrix} 1 & a & a^{2} \\ 1 & b & b^{2} \\ 1 & c & c^{2} \end{matrix} \right| (a-b)(b-c)(c-a)
Prove that :
\begin{vmatrix} 0 & a & -b \\ -a & 0 & -c \\ b & c & 0 \end{vmatrix}=0.
Find the value of the following determinants.
\begin{vmatrix} \dfrac { 7 }{ 3 } & \dfrac { 5 }{ 3 } \\ \dfrac { 3 }{ 2 } & \dfrac { 1 }{ 2 } \end{vmatrix}
\begin{vmatrix} \dfrac { 7 }{ 3 } & \dfrac { 5 }{ 3 } \\ \dfrac { 3 }{ 2 } & \dfrac { 1 }{ 2 } \end{vmatrix}
= (\frac{7}{3})(\frac{1}{2}) - (\frac{5}{3}) (\frac{3}{2})
\Rightarrow \frac{7}{6} - \frac{15}{6} = \frac{-8}{6} = \frac{-4}{3}
Given \begin{bmatrix} 2 & 1 \\ -3 & 4 \end{bmatrix} X=\begin{bmatrix} 7 \\ 6 \end{bmatrix}. Write :
the order of the matrix X
the matrix X.
If A=\begin{bmatrix} \cos { \theta } & \sin { \theta } \\ -\sin { \theta } & \cos { \theta } \end{bmatrix} the A^{n}=
Find the value of \lambda for which the points (6,-1,2),(8,-7,\lambda) and (5,2,4) are collinear.
Set B cuts AC in the ratio l:1
{ x }_{ 2 }=\cfrac { l{ x }_{ 3 }+{ x }_{ 1 } }{ l+1 }
8=\cfrac { l\left( 5 \right) +1\left( 6 \right) }{ l+1 }
5l+6=8l+8
3l+2=0
l=\cfrac { -2 }{ 3 }
\therefore B cuts Ac in the ratio \cfrac { -2 }{ 3 } :1
{ z }_{ 2 }=\cfrac { l{ z }_{ 3 }+{ z }_{ 1 } }{ l+1 }
\lambda =\cfrac { \left( \cfrac { -2 }{ 3 } \right) \left( 4 \right) +\left( 2 \right) }{ \cfrac { -2 }{ 3 } +1 }
=\cfrac { \cfrac { -8 }{ 3 } +2 }{ \cfrac { 1 }{ 3 } } =\cfrac { \cfrac { -2 }{ 3 } }{ \cfrac { 1 }{ 3 } } =-2
\therefore \lambda =-2
Find the value of the following determinants.
\begin{vmatrix} -1 & 7 \\ 2 & 4 \end{vmatrix}
\begin{vmatrix} -1 & 7 \\ 2 & 4 \end{vmatrix}
\Rightarrow (-1) (4) - (2) (7) \Rightarrow -4 - 14
\Rightarrow -18
Find the value of the following determinants.
\begin{vmatrix} 5 & 3 \\ -7 & 0 \end{vmatrix}
\begin{vmatrix} 5 & 3 \\ -7 & 0 \end{vmatrix}
(5)(0) - (-7) (3)
= 0-(-21) = 21
If A=[aij] is a matrix of order 2x2 such that |A|=15 and cij represents the co factor of aij then find { a }_{ 21 }{ c }_{ 21 }+{ a }_{ 22 }{ c }_{ 22 }.
A square matrix \mathrm { B } of order 3 , has | B | = 7 , find | B adjB |
A. square matrix A of order 3 , has | A | = 5 , find | A adja|
Find equation of line joining (1,2) and (3,6) using determinants.
\textbf{Step 1: Find the equation using suitable condition.}
\text{Let, $$P(x, y)$$ be the general point on the line joining $$A(1,2)$$ and $$B(3,6)$$}
\text{We know, area of a triangle with vertices }(x_1,y_1),(x_2,y_2)\text{ and }(x_3,y_3)\text{ is given by,}
\Rightarrow\text{Area }=\dfrac{1}{2}\left|\begin{array}{lll}x_1 & y_1 & 1 \\x_2 & y_2 & 1 \\x_3 & y_3 & 1\end{array}\right|
\text{From the figure, it is obvious that the area of }\triangle \text{APB } \text{is zero.}
\Rightarrow\dfrac{1}{2}\left|\begin{array}{lll}x & y & 1 \\1 & 2 & 1 \\3 & 6 & 1\end{array}\right|=0
\Rightarrow\left|\begin{array}{lll}x & y & 1 \\1 & 2 & 1 \\3 & 6 & 1\end{array}\right|=0
\Rightarrow x(2-6)-y(1-3)+1(6-6)=0
\Rightarrow-4 x+2 y=0
\Rightarrow4 x-2 y=0
\Rightarrow2 x- y=0
\textbf{Hence, the required equation of the line is : }\mathbf{2x-y=0.}
Prove that \begin {vmatrix} a^2 & bc & ac + c^2 \\ a^2 + ab & b^2 & ac \\ ab & b^2 + bc & c^2 \end {vmatrix} = 4a^2b^2c^2
Prove that \begin{vmatrix} a & a & a \\ a & b & b \\ a & b & c \end{vmatrix}=a(b-c)(a-b), Hence find the value of \begin{vmatrix} 3 & 3 & 3 \\ 3 & 5 & 5 \\ 3 & 5 & 7 \end{vmatrix}.
$$\begin{vmatrix} a & a & a\\ a & b & b\\ a & b & c \end{vmatrix} = a(b-c)(a-b)$$
Solving LHS we get
$$\begin{vmatrix} a & a & a\\ a & b & b\\ a & b & c \end{vmatrix}$$
\Rightarrow a(bc-b)^2-a(ac-ab)+a(ab-ab)
\Rightarrow ab[c-b]-a^2[c-b]+0
\Rightarrow a(c-b)(b-a)
\Rightarrow a (b-c)(a-b)=RHS
now
$$\begin{vmatrix} 3 & 3 & 3 \\ 3 & 5 & 5\\ 3 & 5 & 7 \end{vmatrix}$$
= 3 (5-7) (3-5) = 3(-2) (-2)
=12
A point P\left(2,-1\right) is equidistant from points \left(a,7\right) and \left(-3,a\right). Find a.
\sqrt{(2-(-3))^2+(-1-a)^2}=\sqrt{(2-a)^2+(-1-7)^2}
Squaring both sides
(2+3)^2+(1+a)^2=(2-a)^2+(-8)^2
\Rightarrow 25+1+a^2+2a=4+a^2-4a+64
\Rightarrow 6a=42
\Rightarrow a=7.
Prove that : \begin {vmatrix} a & c & a + c \\ a + b & b & a \\ b & b + c & c \end {vmatrix} = 4abc
Prove that:
\begin{vmatrix} a+b+2c & a & b \\ c & b+c+2a & b \\ c & a & c+a+2b \end{vmatrix}=2{ \left( a+b+c \right) }^{ 3 }
Fill in the blanks with correct number
\left| \begin{matrix} 3 \\ 4 \end{matrix}\begin{matrix} 2 \\ 5 \end{matrix} \right| =3\times \Box -\Box \times 4=\Box -8=\Box
Using properties of determinant solve :-
\left| \begin{matrix} 1 & a & { a }^{ 2 }-bc \\ 1 & b & { b }^{ 2 }-ac \\ 1 & c & { c }^{ 2 }-ab \end{matrix} \right| =
\begin{vmatrix} 1 & a & a^2-bc\\ 1 & b & b^2-ac\\ 1 & c & c^2-ab\end{vmatrix}
R_1\rightarrow R_1-R_2; R_2\rightarrow R_2-R_3
\begin{vmatrix} 0 & a-b & (a^2-b^2)+c(a-b)\\ 0 & b-c & (b^2-c^2)+a(b-c)\\ 1 & c & c^2-ab\end{vmatrix}
Expanding the determinant
1[(a-b)[(b-c)(a+b+c)]-(b-c)[(a-b)(a+b+c)]]
\Rightarrow 0.
Find the values of x, if
\begin{vmatrix} 2x & 5 \\ 8 & x \end{vmatrix}=\begin{vmatrix} 6 & 5 \\ 8 & 3 \end{vmatrix}
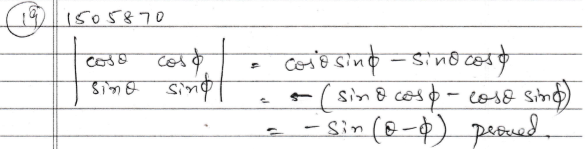
Prove that \left| \begin{matrix} cos\theta & cos\phi \\ sin\theta & sin\phi \end{matrix} \right| =-sin\left( \theta -\phi \right)
Find the cofactors of the elements of the following matrices :
(i) \begin{bmatrix} -1 & 2 \\ -3 & 4 \end{bmatrix}
(ii) \left[ \begin{matrix} 1 & -1 & 2 \\ -2 & 3 & 5 \\ -2 & 0 & -1 \end{matrix} \right]
Find the minor of \begin{bmatrix} 2 & 7 & 3 \\ -4 & 3 & -1 \\ 0 & -3 & 7 \end{bmatrix}.
Prove that \left| \begin{matrix} \sin\alpha & \cos\alpha & \cos(a+\delta ) \\ \sin\beta & \cos\beta & cos(\beta +\delta ) \\ \sin\gamma & \cos\gamma & \cos(\gamma +\delta ) \end{matrix} \right| =0.
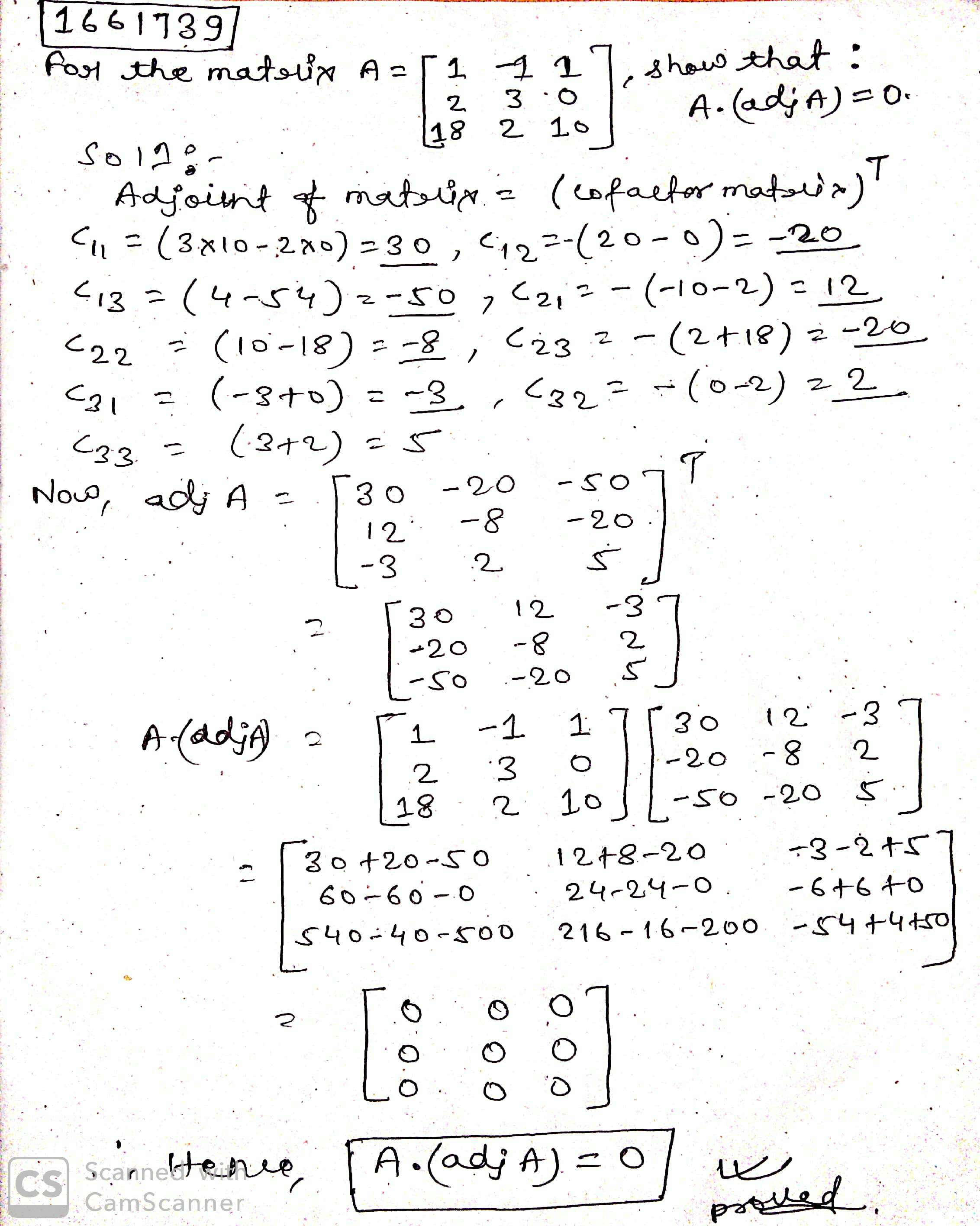
For the matrix A = \begin{bmatrix} 1 & -1 & 1 \\ 2 & 3 & 0 \\ 18 & 2 & 10 \end{bmatrix}, show that A (adj A) = 0 .
Find the values of x, if \begin{vmatrix} 3 & x \\ x & 1 \end{vmatrix}= \begin{vmatrix} 3 & 2 \\ 4 & 1 \end{vmatrix}
Solve: \begin{bmatrix} 1 & 5 & -2 \\ 3x & 2 & 4 \\ 5 & -1 & 0 \end{bmatrix}=0
Solve:
\begin{bmatrix} 1 & 5 & -2 \\ 3x & 2 & 4 \\ 5 & -1 & 0 \end{bmatrix}=0
If A = \begin{bmatrix} -4 & -3 & -3 \\ 1 & 0 & 1 \\ 4 & 4 & 3 \end{bmatrix}, show that adj A = A .
Find the values of x, if
\begin{vmatrix} 4 & x+1 \\ x+2 & 2 \end{vmatrix}= \begin{vmatrix} 4 & 4 \\ 5 & 2 \end{vmatrix}
Find the adjoint of the following matrice:
\begin{bmatrix} \cos\,\alpha & \sin\,\alpha \\ \sin\,\alpha & \cos\,\alpha \end{bmatrix}
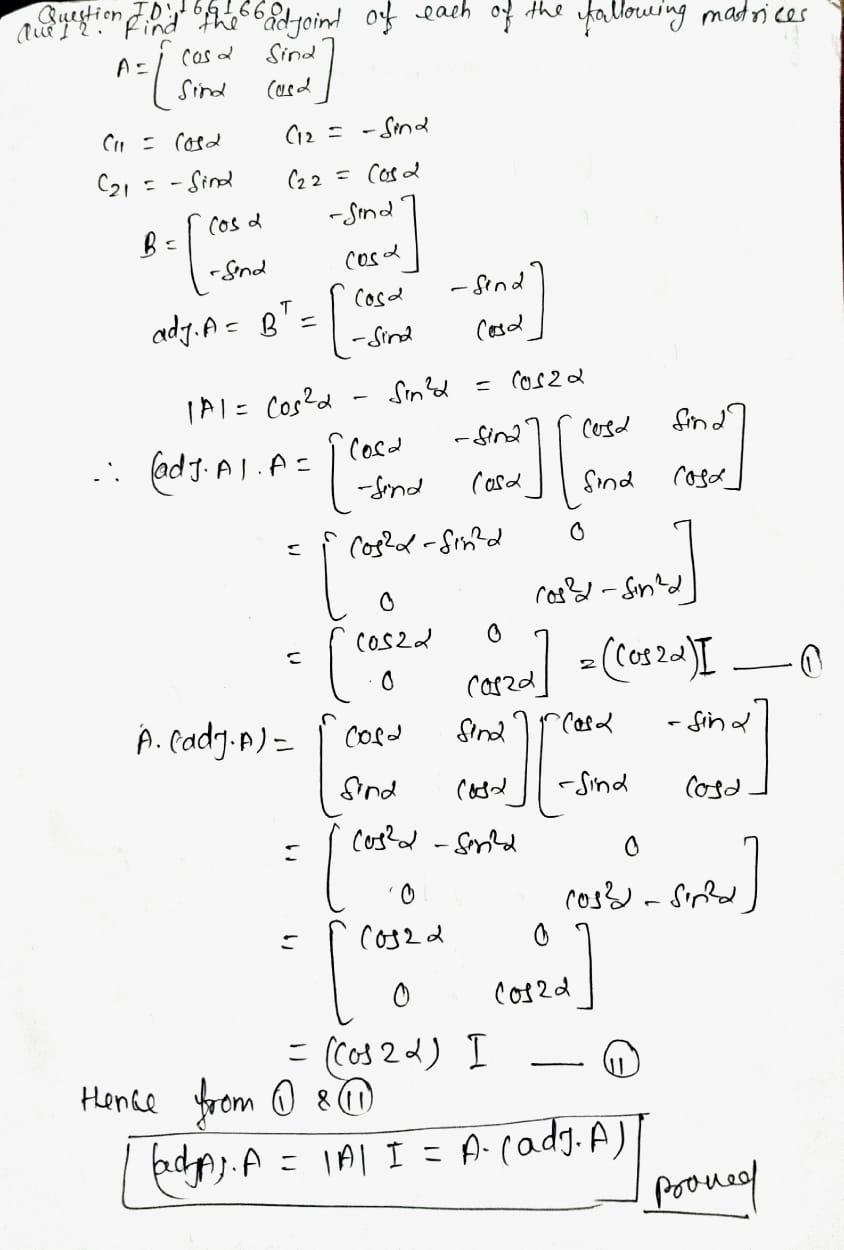
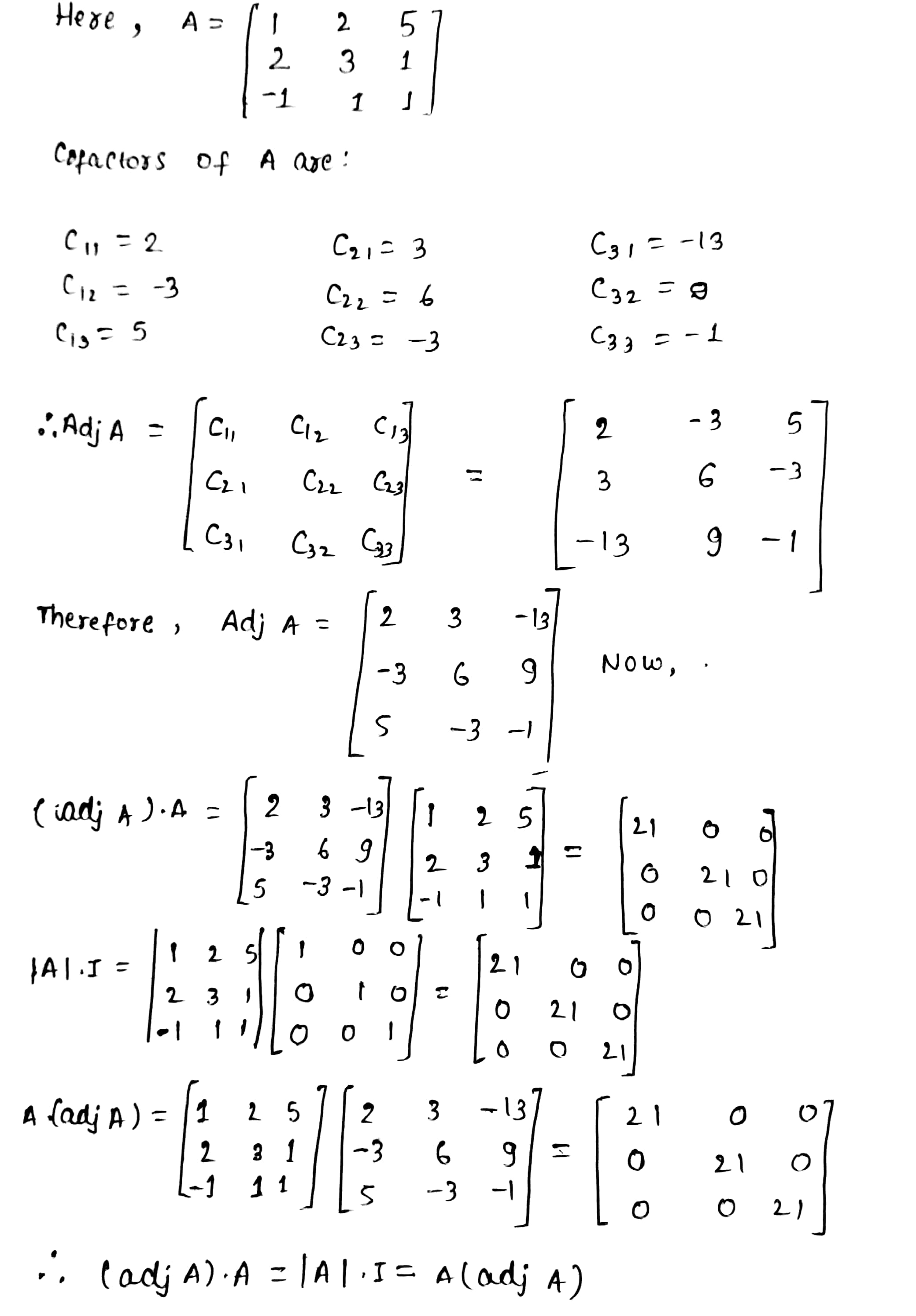
Verify that (adj A ) A = |A| I = A (adj A) for the above matrice.
If A = \begin{bmatrix} -1 & -2 & -2 \\ 2 & 1 & -2 \\ 2 & -2 & 1 \end{bmatrix},show that adj A = 3A^{T}.
Solve: \begin{vmatrix} x+2 & 1 \\ -1 & x\end{vmatrix}=0
If A is a square matrix of order n, prove that \left | A\: adj \: A \right |= \left | A \right |^{n}.
Find the values of x, if
\begin{vmatrix} 2x & 5 \\ 8 & x \end{vmatrix}=\begin{vmatrix} 6 & 5 \\ 8 & 3 \end{vmatrix}
Evaluate the following determinant :
\begin{vmatrix} 1 & -3 & 2 \\ 4 & -1 & 2 \\ 3 & 5 & 2 \end{vmatrix}
Evaluate the following determinant : =\begin{vmatrix} 67 & 19 & 21 \\ 39 & 13 & 14 \\ 81 & 24 & 26 \end{vmatrix}
Find the integral value of x, if \begin{vmatrix} x^{2} & x & 1\\ 0 & 2 & 1 \\ 3 & 1 & 4 \end{vmatrix}= 28
Evaluate the following determinant :
\begin{vmatrix} 1 & 3 & 5 \\ 2 & 6 & 10 \\ 31 & 11 & 38 \end{vmatrix}
Evaluate the following :
\begin{vmatrix} x+\lambda & x & x \\ x & x+\lambda & x \\ x & x & x+\lambda \end{vmatrix}
Evaluate the following : \begin{vmatrix} x+\lambda & x & x \\ x & x+\lambda & x \\ x & x & x+\lambda \end{vmatrix}
Evaluate the following determinant :
\begin{vmatrix} a & h & g \\ h & b & f \\ g & f & c \end{vmatrix}
If a,b,c are real numbers such that \begin{vmatrix} b+c & c+a & a+b \\ c+a & a+b & b+c \\ a+b & b+c & c+a \end{vmatrix}= 0, then show that either a+b+c=0 or, a=b=c.
Evaluate the following :
\begin{vmatrix} 1 & a & bc \\ 1 & b & ca \\ 1 & c & ab \end{vmatrix}
Evaluate the following determinant :
\begin{vmatrix} 15 & 11 & 7 \\ 11 & 17 & 14 \\ 10 & 16 & 13 \end{vmatrix}
Evaluate the following determinant :
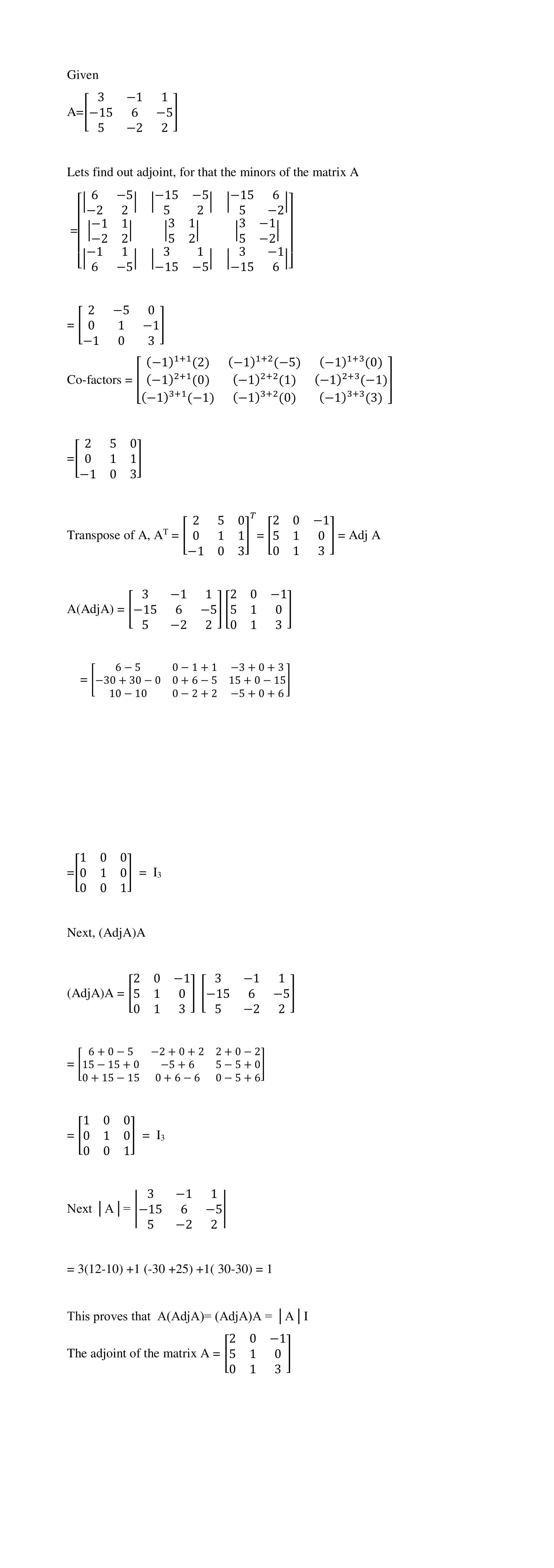
Find the adjoint of the given matrix and verify in each case that A \cdot (adj A) = (adj A)\cdot A = |A| \cdot I.
\begin{bmatrix} 3& -1 & 1\\ -15 & 6 & -5 \\ 5 & -2 & 2\end{bmatrix}
If a, b, c are p^{th}, q^{th} and r^{th} terms respectively of a G.P, then prove that
\begin{vmatrix}\log a& p & 1 \\ \log b& q & 1\\ \log c &r & 1 \end{vmatrix} =0
Find the cofactors of all the elements of \begin{bmatrix}1&-2 \\ 4 & 3 \end{bmatrix}
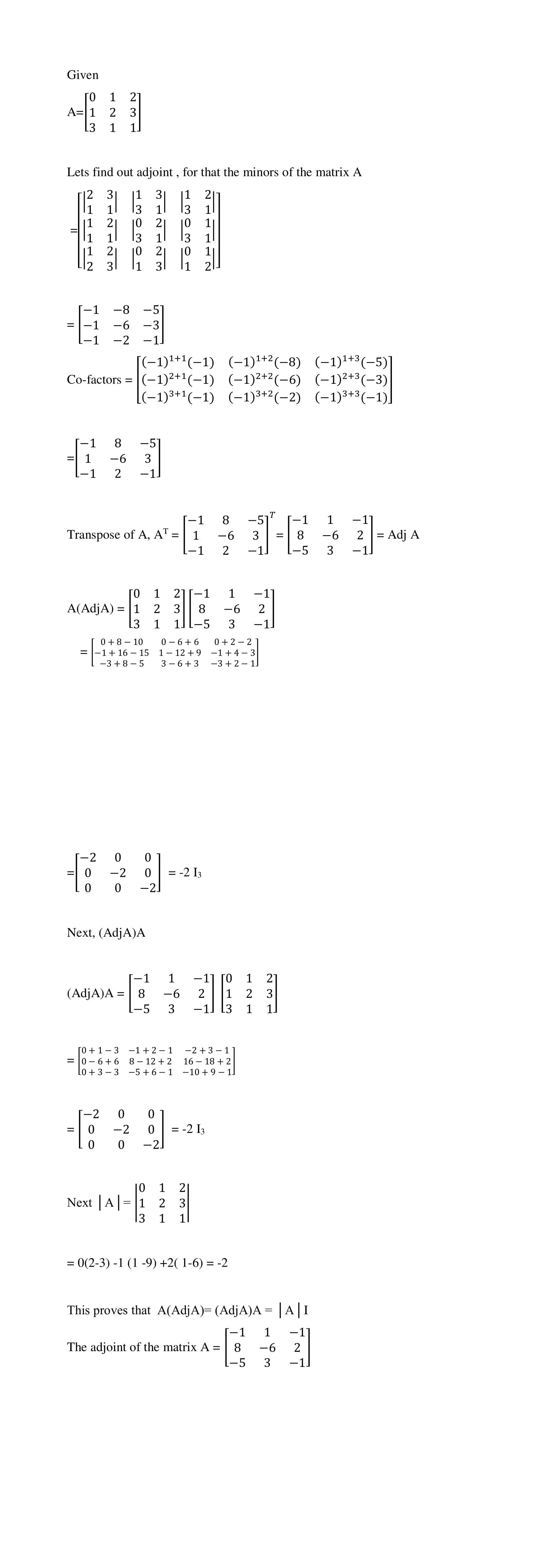
Find the adjoint of the given matrix and verify in each case that A \cdot (adj A) = (adj A)\cdot A = |A| \cdot I.
\begin{bmatrix} 0& 1 & 2\\ 1 & 2 & 3 \\ 3 & 1 & 1\end{bmatrix}
Find the adjoint of the given matrix and verify in each case that A \cdot (adj A) = (adj A)\cdot A = |A| \cdot I.
\begin{bmatrix} 2& 3\\ 5 & 9\end{bmatrix}
Find the adjoint of the given matrix and verify in each case that A \cdot (adj A) = (adj A)\cdot A = |A| \cdot I.
\begin{bmatrix} \cos \alpha& \sin \alpha\\ \sin \alpha & \cos \alpha\end{bmatrix}
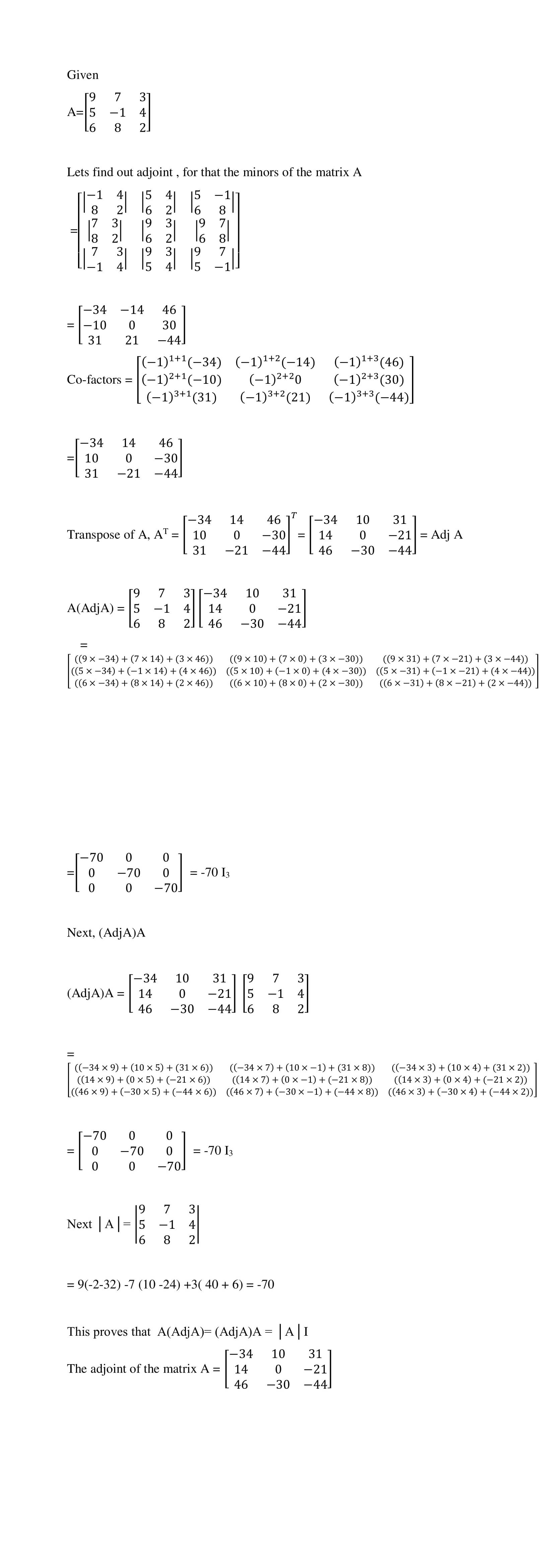
Find the adjoint of the given matrix and verify in each case that A \cdot (adj A) = (adj A)\cdot A = |A| \cdot I.
\begin{bmatrix} 9& 7 & 3\\ 5 & -1 & 4 \\ 6 & 8 & 2\end{bmatrix}
If A and B are square matrices each of order 3 and |A|= 5, \, |B| = 3, then the value of |3AB| is ______
Find the adjoint of the given matrix and verify in each case that A \cdot (adj A) = (adj A)\cdot A = |A| \cdot I.
\begin{bmatrix} 1& -1 & 2\\ 3 & 1 & -2 \\ 1 & 0 & 3\end{bmatrix}
Find the adjoint of the given matrix and verify in each case that A \cdot (adj A) = (adj A)\cdot A = |A| \cdot I.
\begin{bmatrix} 3& -5\\ -1 & 2\end{bmatrix}
Let A be a square matrix of order 3, write the value of |2A|, where |A|=4.
Evaluate \begin{vmatrix} \sqrt { 6 } & \sqrt { 5 } \\ \sqrt { 20 } & \sqrt { 24 } \end{vmatrix}.
If A=\begin{vmatrix} 3 & 4 \\ 1 & 2 \end{vmatrix}, find the value of 3|A|.
Find the adjoint of the given matrix and verify in each case that A \cdot (adj A) = (adj A)\cdot A = |A| \cdot I.
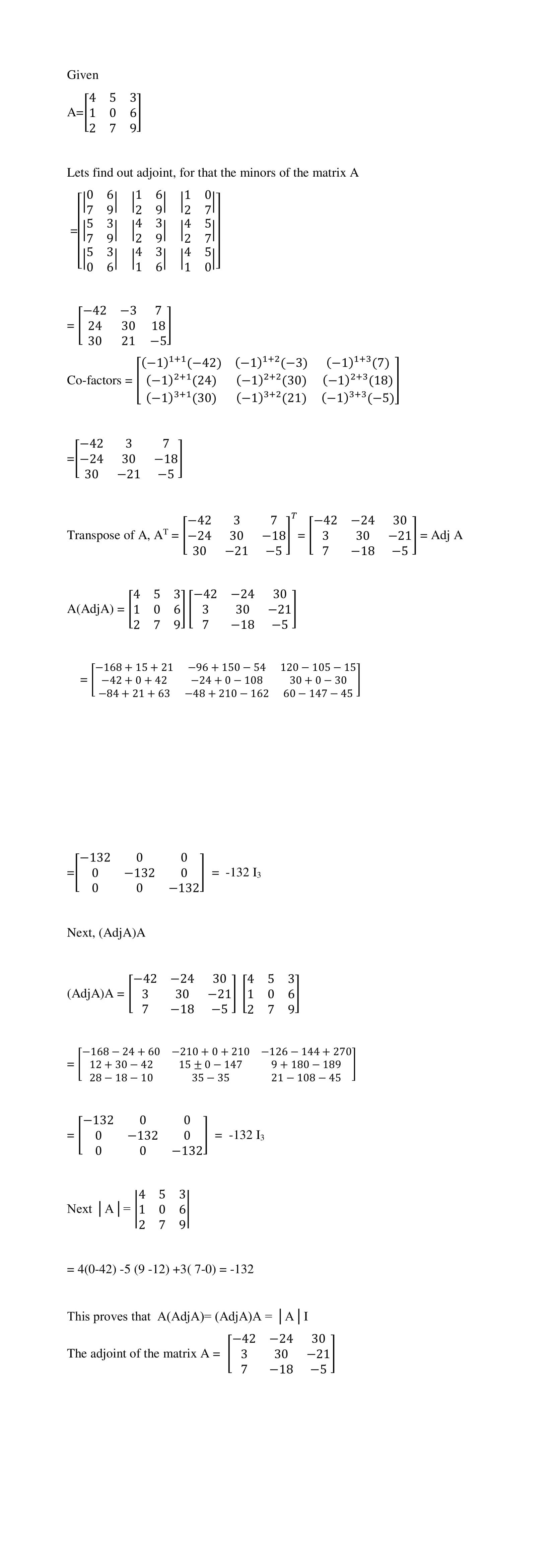
\begin{bmatrix} 4& 5 & 3\\ 1 & 0 & 6 \\ 2 & 7 & 9\end{bmatrix}
If A is a 2\times 2 matrix such that |A|\neq 0 and |A|=5, write the value of |4A|.
If A is a 3\times 3 matrix such that |A|\neq 0 and |3A|=k|A|, then write the value of k.
Evaluate \begin{vmatrix} \sin { { 60 }^{ o } } & \cos { { 60 }^{ o } } \\ -\sin { { 30 }^{ o } } & \cos { { 30 }^{ o } } \end{vmatrix}.
Find the adjoint of the given matrix and verify in each case that A \cdot (adj A) = (adj A)\cdot A = |A| \cdot I.
\begin{bmatrix} \cos \alpha& -\sin \alpha & 0\\ \sin \alpha & \cos \alpha & 0 \\ 0 & 0 & 1\end{bmatrix}
Evaluate \begin{vmatrix} 2\cos { \theta } & -2\sin { \theta } \\ \sin { \theta } & \cos { \theta } \end{vmatrix}.
Evaluate \begin{vmatrix} \cos { \alpha } & -\sin { \alpha } \\ \sin { \alpha } & \cos { \alpha } \end{vmatrix}.
Evaluate \begin{vmatrix} \cos { { 65 }^{ o } } & \sin { { 65 }^{ o } } \\ \sin { 25^{ o } } & \cos { 25^{ o } } \end{vmatrix}.
Evaluate \begin{vmatrix} \cos { { 15 }^{ o } } & \sin { { 15 }^{ o } } \\ \sin { 75^{ o } } & \cos { 75^{ o } } \end{vmatrix}.
Evaluate \begin{bmatrix} \sqrt { 3 } & \sqrt { 5 } \\ -\sqrt { 5 } & 3\sqrt { 3 } \end{bmatrix}.
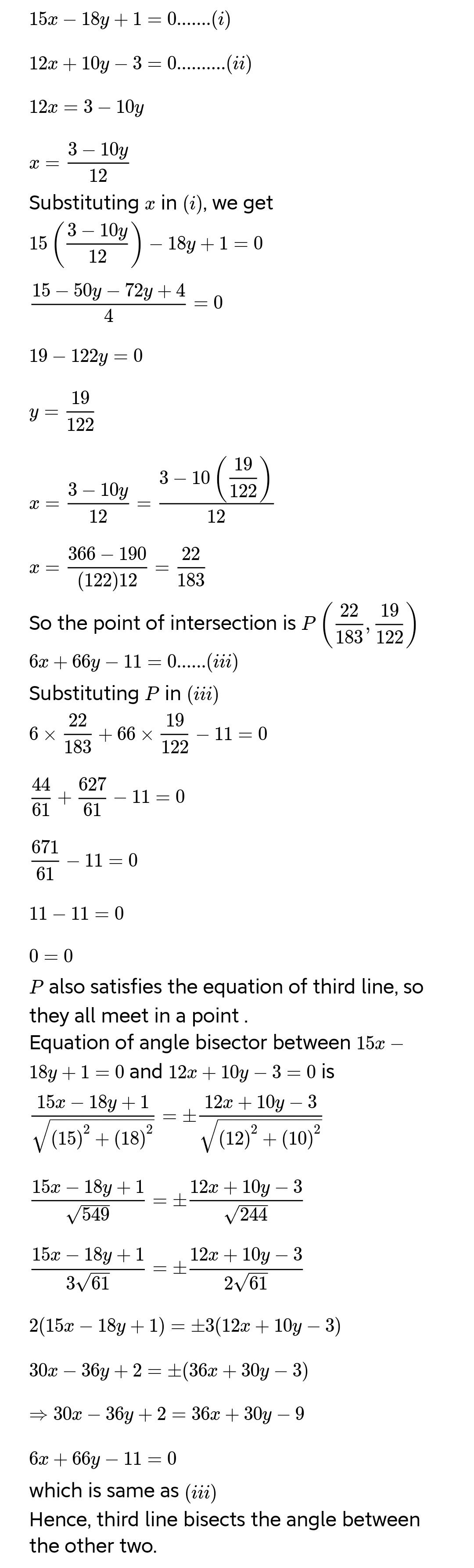
Prove that the three straight lines whose equations are
15x - 18y + 1 = 0, 12x + 10y -3 = 0, and 6x + 66y - 11 = 0
all meet in a point.
Show also that the third line bisects the angle between the other two.
Prove that the following sets of three lines meet in a point.
2x - 3y = 7, 3x - 4y = 13, and 8x - 11y = 33.
If \begin{vmatrix} { \left( \beta +\gamma -\alpha -\delta \right) }^{ 4 } & { \left( \beta +\gamma -\alpha -\delta \right) }^{ 2 } & 1 \\ { \left( \gamma +\alpha -\beta -\delta \right) }^{ 4 } & { \left( \gamma +\alpha -\beta -\delta \right) }^{ 2 } & 1 \\ { \left( \alpha +\beta -\gamma -\delta \right) }^{ 4 } & { \left( \alpha +\beta -\gamma -\delta \right) }^{ 2 } & 1 \end{vmatrix}=k\left( \alpha -\beta \right) \left( \alpha -\gamma \right) \left( \alpha -\delta \right) \left( \beta -\gamma \right) \left( \beta -\delta \right) \left( \gamma -\delta \right) \quad then the value of {(k)}^{1/2} is
Given A=\begin{vmatrix} a & b & 2c \\ d & e & 2f \\ l & m & 2n \end{vmatrix};B=\begin{vmatrix} f & 2d & e \\ 2n & 4l & 2m \\ c & 2a & b \end{vmatrix}, then the value of \dfrac{B}{A} is ________.
Find the minor of the element of second row and third column ( a 23 ) in the following determinant:
\left|\begin{array}{ccc}2 & -3 & 5 \\ 6 & 0 & 4 \\ 1 & 5 & -7\end{array}\right|
If A is an invertible metrix of order 3 and |A| = 5, then find |adj \ A|.
If \Delta=\left|\begin{array}{lll}5 & 3 & 8 \\2 & 0 & 1 \\1 & 2 & 3\end{array}\right| \text { , then write the minor of the element } a_{23}
What positive value of x makes the following pair of determinants equal?
\left|\begin{array}{cc}2 x & 3 \\ 5 & x\end{array}\right|,\left|\begin{array}{cc}16 & 3 \\ 5 & 2\end{array}\right|
Find the cofactor of a_{12} in the following:
\left|\begin{array}{ccc}1 & -3 & 5 \\ 6 & 0 & 4 \\ 1 & 5 & -7\end{array}\right|
\begin{aligned}& \\&\text { Evaluate: }\left|\begin{array}{ll}\cos 15^{\circ} & \sin 15^{\circ} \\\sin 75^{\circ} & \cos 75^{\circ}\end{array}\right|\end{aligned}
If A is idempotent matrix satisfying ( I - 0.4 A)^{-1} = I - \alpha A where I is the unit matrix of the same order as that of A then find the value of | 9 \alpha | .
Find the equation of line joining (1,2) and (3,6) using determinants.
\textbf{Step 1: Find the equation using suitable condition.}
\text{Let, $$P(x, y)$$ be the general point on the line joining $$A(1,2)$$ and $$B(3,6)$$}
\text{We know, area of a triangle with vertices }(x_1,y_1),(x_2,y_2)\text{ and }(x_3,y_3)\text{ is given by,}
\Rightarrow\text{Area }=\dfrac{1}{2}\left|\begin{array}{lll}x_1 & y_1 & 1 \\x_2 & y_2 & 1 \\x_3 & y_3 & 1\end{array}\right|
\text{From the figure, it is obvious that the area of }\triangle \text{APB } \text{is zero.}
\Rightarrow\dfrac{1}{2}\left|\begin{array}{lll}x & y & 1 \\1 & 2 & 1 \\3 & 6 & 1\end{array}\right|=0
\Rightarrow\left|\begin{array}{lll}x & y & 1 \\1 & 2 & 1 \\3 & 6 & 1\end{array}\right|=0
\Rightarrow x(2-6)-y(1-3)+1(6-6)=0
\Rightarrow-4 x+2 y=0
\Rightarrow4 x-2 y=0
\Rightarrow2 x- y=0
\textbf{Hence, the required equation of the line is : }\mathbf{2x-y=0.}
Find the value of \left|\begin{array}{cccc}\sin A & -\sin B \\\cos A & \cos B\end{array}\right| \text { where } A=53^{\circ}, B=37^{\circ}
If A = \begin{bmatrix} \alpha & 0 & 0 \\ 0 & \alpha & 0 \\ 0 & 0 & \alpha \end{bmatrix}, find the value of |adj \ A|.
If \Delta= \left| \begin{matrix} 0 & b-a & c-a \\ a-b & 0 & c-b \\ a-c & b-c & 0 \end{matrix} \right| then show that \Delta is equal to zero.
Write the adjoint of the following matrix:
\Big[\begin{matrix} 2 & -1 \\ 4 & 3 \end{matrix}\Big]
If \left|\begin{array}{ll}x & x \\1 & x\end{array}\right|=\left|\begin{array}{ll}3 & 4 \\1 & 2\end{array}\right| , then write the positive value of x
Find the equation of the line joining A(1,3) and B(0,0) using determinants and find k if D(k, 0) is a point such that the area of \Delta A B D is 3 sq units.
The determinant \Delta = \left| \begin{matrix} \sqrt {23} + \sqrt {3} & \sqrt {5} & \sqrt {5} \\ \sqrt {15} + \sqrt {46} & 5 & \sqrt {10} \\ 3 +\sqrt {115} & \sqrt {15} & 5 \end{matrix} \right| is equal to ...............
Show that if the determinant \Delta = \left| \begin{matrix} 3 & -2 & sin\quad 3\quad \theta \\ -7 & 8& cos \quad 2 \theta \\ -11 & 14 & 2 \end{matrix} \right| = 0 then Sin \theta = 0 or \frac {1}{2}
Write the value of \left|\begin{array}{cc}\sin 20^{\circ} & -\cos 20^{\circ} \\ \sin 70^{\circ} & \cos 70^{\circ}\end{array}\right|
Evaluate :
\left| \begin{matrix} y+z & z & y \\ z & z+x & x \\ y & x & x+y \end{matrix} \right| =\quad 4xyz
If A is a matrix or order 3 \times 3 then number of minors in determinant of A are__________
Fill in the blank.If A is matrix of order 3 \times 3 then |3A| is equal to_________
If x+y+z = 0 prove that \left| \begin{matrix} xa & yb & zc \\ yc & za & xb \\ zb & xc & ya \end{matrix} \right| \quad =\quad xyz\quad \left| \begin{matrix} a & b & c \\ c & a & b \\ b & c & a \end{matrix} \right|
Evaluate :
\left| \begin{matrix} 3x & -x+y & -x+z \\ x-y & 3y & z-y \\ x-z & y-z & 3z \end{matrix} \right|
If A +B +C = 0 then prove that \left| \begin{matrix} 1 & cos\quad C & cos\quad B \\ cos\quad C & 1 & cos\quad A \\ cos\quad B\quad & cos\quad A\quad & 1 \end{matrix} \right| =0
If Cos 2 \theta = 0 then \left| \begin{matrix} 0 & cos \theta &sin \theta \\ cos \theta & sin \theta & 0 \\ sin \theta & 0 & cos \theta \end{matrix} \right|^2 = _________
If a+ b +c \neq 0 and \left| \begin{matrix} a & b & c \\ b & c & a \\ c & a & b \end{matrix} \right| = 0 then prove that a = b = c .
Find the value of the following determinant
\begin{vmatrix} 5 & {-2}\\{-3} & 1\end{vmatrix}.
Find the value of the following determinant
\begin{vmatrix} 3 & {-1}\\{1} & 4 \end{vmatrix}.
Find the adjoint of the matrix A = \begin{bmatrix} 1 & 2\\ 3 & -5\end{bmatrix} and verify the result A(adj \,A) = (adj \,A) = A |A| \cdot I.
Find the adjoint of the following matrix.
\begin{bmatrix} 1& -1 & 2 \\ -2 & 3 & 5 \\ -2 & 0 & -1 \end{bmatrix}
The sum if the products of element of any row with the co-factors of corresponding elements is equal to __________
Find the adjoint of the following matrix.
\begin{bmatrix} 2 & -3 \\ 3 & 5 \end{bmatrix}
Evaluate the determinations:
\begin{vmatrix} \cos { \theta } & -\sin { \theta } \\ \sin { \theta } & \cos { \theta } \end{vmatrix}
If A=\begin{bmatrix} 1 & 1 & -2 \\ 2 & 1 & -3 \\ 5 & 4 & -9 \end{bmatrix} find \left| A \right|
Find the values of determinant.
\begin{vmatrix} -1&7 \\ 2&4 \end{vmatrix}
Evaluate the determinations:
\begin{vmatrix} 2 & 4 \\ -5 & -1 \end{vmatrix}
If A=\begin{bmatrix} 1 & 2 \\ 4 & 2 \end{bmatrix}, then show that \left| 2A \right| =4\left| A \right|
Find the values of following determinant.
\begin{vmatrix} 5&3 \\ -7&0 \end{vmatrix}
Evaluate the determinations:
\begin{vmatrix} { x }^{ 2 }-x+1 & x-1 \\ x+1 & x+1 \end{vmatrix}
there are n points in a plane of which m points are collinear. how many triangles will be formed by joining three points?
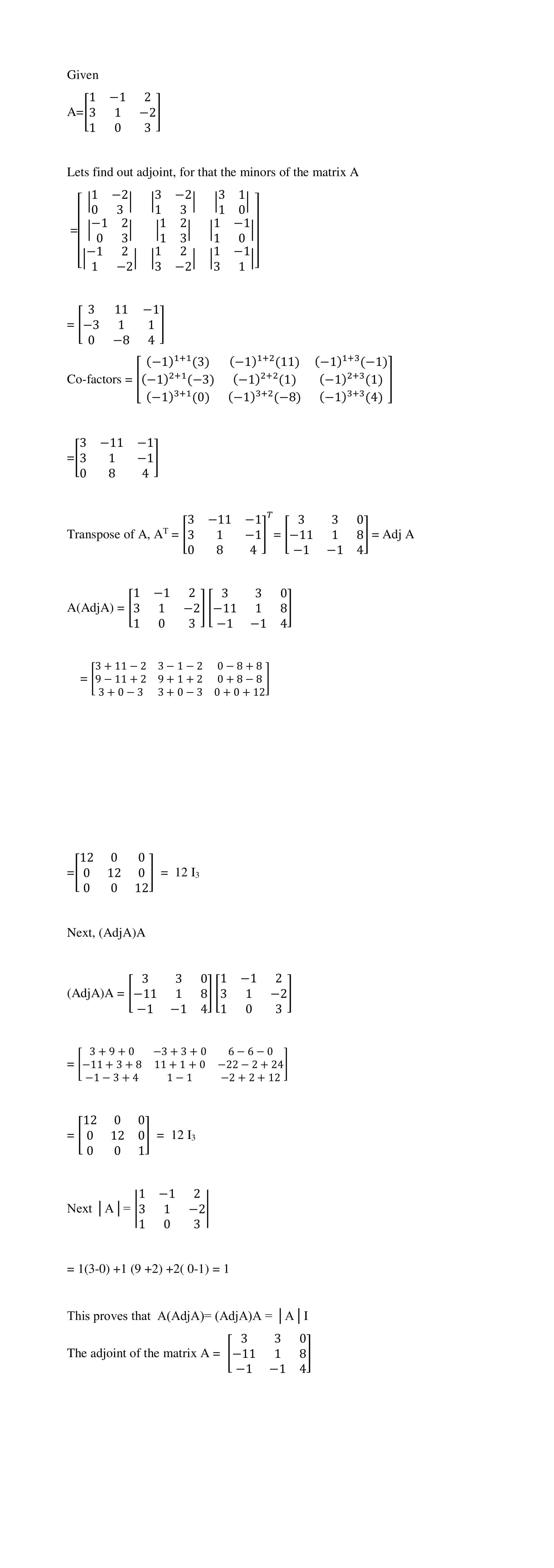
If matrix A=\begin{bmatrix} 1 & -1 & 2 \\ 3 & 0 & -2 \\ 1 & 0 & 3 \end{bmatrix} then find adj.A also prove that A(adj A)= |A| I_3= (adj A)A
Find the minors of elements of second row of determinant \begin{vmatrix} 2 & 3 & 4\\ 3 & 6 & 5\\ 1 & 8 & 9 \end{vmatrix}.
If A be a square matrix of order 3\times 3 then find \left| kA \right|
\left| KA \right| ={ k }^{ n }\left| A \right| when n is the order
For which value of k, det \begin{vmatrix} k & 2\\ 4 & -3 \end{vmatrix} will be zero?
Evaluate
\begin{vmatrix} \sin { 30 } & \cos { 30 } \\ -\sin { 60 } & \cos { 60 } \end{vmatrix}
Find adjoint of matrix :
\begin{vmatrix} 1 & -1 & 2 \\ 2 & 3 & 5 \\ -2 & 0 & 1 \end{vmatrix}
A = \begin{bmatrix}1 & \tan x\\ -\tan x & 1\end{bmatrix} and f(x) is defined as f(x) = det. (A^T A^{-1}) then find the value of \underset{\text{n times}}{\underbrace{f(f(f(f.......f(x))))}} is (n \geq 2).
If A is a square matrix of order 3, then find |(A - A^T)^{2011}|.
Match the statements in Column I with statements in column II
Prove that \begin{vmatrix} bc & bc'+b'c & b'c'\\ ca & ca'+c'a & c'a'\\ ab & ab'+a'b & a'b' \end{vmatrix}=(ab'-a'b)(bc-b'c)(ca'-c'a)
Let
\Delta _{1}=\begin{vmatrix} 1 & \cos \alpha & \cos \beta \\ \cos \alpha & 1 & \cos \gamma \\ \cos \beta & \cos \gamma & 1 \end{vmatrix}
and \Delta _{2}=\begin{vmatrix} 0 & \cos \alpha & \cos \beta \\ \cos \alpha & 0 & \cos \gamma \\ \cos \beta & \cos \gamma & 0 \end{vmatrix} .
If \Delta _{1}=\Delta _{2}, find \sin ^{2}\alpha +\sin ^{2}\beta +\sin ^{2}\gamma
\Delta _{1}=\begin{vmatrix} 1 & \cos \alpha & \cos \beta \\ \cos \alpha & 1 & \cos \gamma \\ \cos \beta & \cos \gamma & 1 \end{vmatrix}
and \Delta _{2}=\begin{vmatrix} 0 & \cos \alpha & \cos \beta \\ \cos \alpha & 0 & \cos \gamma \\ \cos \beta & \cos \gamma & 0 \end{vmatrix} .
If \Delta _{1}=\Delta _{2}, find \sin ^{2}\alpha +\sin ^{2}\beta +\sin ^{2}\gamma
Prove that \begin{vmatrix} bc-a^2 & ca-b^2 & ab-c^2\\ -bc+ca+ab & bc-ca+ab & bc+ca-ab\\ (a+b)(a+c) & (b+c)(b+a) & (c+a)(c+b) \end{vmatrix}=3.(b-c)(c-a)(a-b)(a+b+c)(ab+bc+ca)
If ax_1^2+by_1^2+cz_1^2=ax_2^2+by_2^2+cz_2^2=ax_3^2+by_3^2+cz_3^2=d and ax_2x_3+by_2y_3+cz_2z_3=ax_3x_1+by_3y_1+cz_3z_1=ax_1x_2+by_1y_2+cz_1z_2=f, then prove that \begin{vmatrix} x_1 & y_1 & z_1\\ x_2 & y_2 & z_2\\ x_3 & y_3 & z_3 \end{vmatrix}=(d-f)\left [\frac {d+2f}{abc}\right ]^{1/2}(a, b, c\neq 0)
Are the three points A(2,3),B(5,6) and C(0,2) collinear?
Find the maximum value of \begin{vmatrix} 1& 1 & 1\\ 1 & 1 +\sin \theta & 1\\ 1 & 1 & 1 + \cos \theta\end{vmatrix}
If a^2+b^2+c^2=1 then is the value of the determinant \begin{vmatrix} a^2+(b^2+c^2)cos\theta & ba(1-cos\theta) & ca(1-cos\theta)\\ ab(1-cos\theta) & b^2(c^2+a^2)cos\theta & cb(1-cos\theta)\\ ac(1-cos\theta) & bc(1-cos\theta) & c^2+(a^2+b^2)cos\theta \end{vmatrix} independent of a,b,c?
If yes enter 1 else enter 0.
A line of slope 2 passes through the point A(1,3).
a) Check whether B(3,7) is a point on this line.
b) Write down the equation of this line.
c) Find the coordinates of a point C on the line such that BC = 2AB.
If \begin{vmatrix}x+1&x-1\\x-3&x+2\end{vmatrix}=\begin{vmatrix}4&-1\\1&3\end{vmatrix}, then write the value of x.
Find determinent\left( {\matrix{ 7 & 1 & { - 6} \cr { - 6} & { - 4} & {13} \cr 2 & 5 & { - 8} \cr } } \right)
Find the value of the determinant:\begin{vmatrix}\cos(\theta+\phi)&-\sin (\theta+\phi)&\cos 2\phi\\ \sin \theta& \cos \theta &\sin \phi\\ -\cos \theta &\sin \theta &\cos \phi\end{vmatrix}
For what values of p and q, the system of equations
2x + py + 6z = 8, x + 2y + qz = 5, x + y + 3z = 4
has (i) no solution (ii) a unique solution (iii) infinitely many solutions.
Solve:
\begin{vmatrix} 1+\sin ^2 \theta & \cos ^2\theta & 4\sin 4\theta \\ \sin ^2 \theta &1+\cos ^2 \theta & 4\sin 4\theta \\ \sin ^2 \theta & \cos ^2 \theta & 1+4\sin 4\theta \end{vmatrix} =0; where, 0<\theta <\large{\cfrac{\pi}{2}}.
The straight lines \displaystyle \imath_1, \imath_2 \, and \, \imath_3 are parallel and lie in the same plane. A total of m points are taken on the line \displaystyle \imath_1, n points on \displaystyle \imath_2, and k points on \displaystyle \imath_3. How many triangles are there whose vertices are at these points?
Write minors and cofactors of the elements of the following determinants:
(i) \begin{vmatrix} 2 & -4 \\ 0 & 3 \end{vmatrix}
(ii) \begin{vmatrix} a & c \\ b & d \end{vmatrix}
(iii) \begin{vmatrix} 1 & 0 & 0 \\ 0 & 1 & 0 \\ 0 & 0 & 1\end{vmatrix}
(iv) \begin{vmatrix} 1 & 0 & 4 \\ 3 & 5 & -1 \\ 0 & 1 & 2 \end{vmatrix}
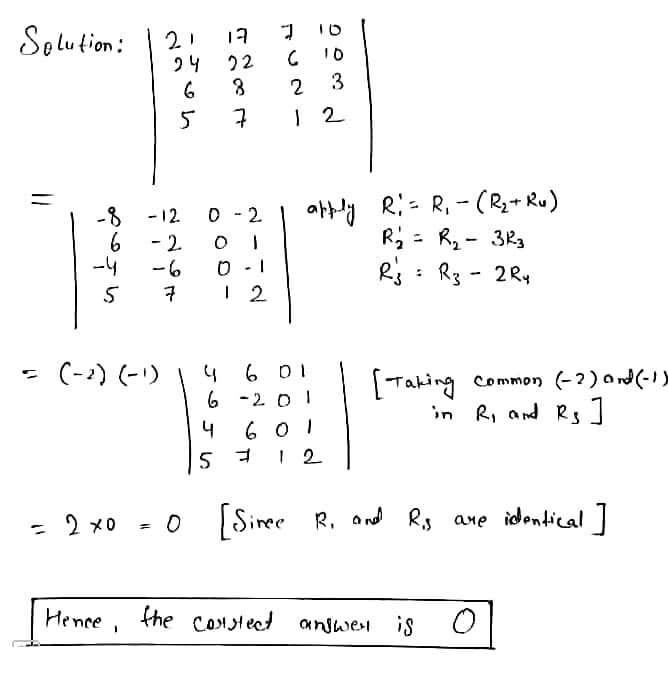
Evaluate the determinants: \left | \begin{array}{111} 21 & 17 & 7 & 10 \\ 24 & 22 & 6 & 10 \\ 6 & 8 & 2 & 3 \\ 5 & 7 & 1 & 2 \\ \end {array} \right |
ax + by + cz = k
a^2x + b^2y + c^2z = k^2
a^3x + b^3y + c^3z = k^3
Solve by Crammer's rule
Using properties of determinants, find the value of n in the following.
\left | \begin{array}{111} 3x & -x+y & -x+z \\ x-y & 3y & z-y \\ x-z & y-z & 3z \\ \end {array} \right | = n( x+y + z)(xy + yz + zx)
The value of determinant \begin{vmatrix} 2 & 7 & 65\\ 3 & 8 & 75 \\ 5 & 9 & 86\end{vmatrix} equals .
\left| \begin{matrix} { a }^{ 2 }+1 & ab & ac \\ ab & { b }^{ 2 }+1 & bc \\ ca & cb & { c }^{ 2 }+1 \end{matrix} \right| =1+{ a }^{ 2 }+{ b }^{ 2 }+{ c }^{ 2 }
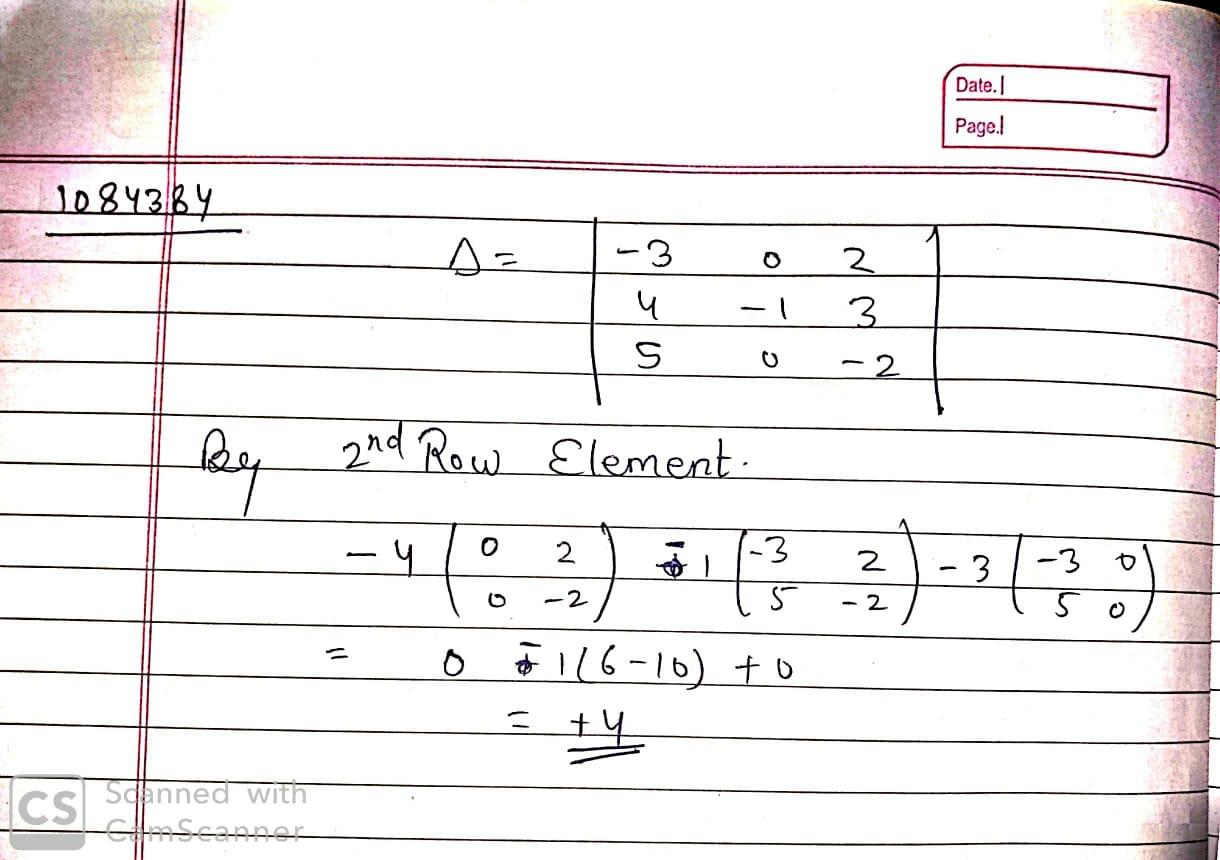
Using cofactor elements of 2nd row, find the value of determinant, \Delta =\begin{vmatrix} -3 & 0 & 2\\ 4 & -1 & 3\\ 5 & 0 & -2\end{vmatrix}.
Show that the points are collinear (-5, 1) (5, 5) (10, 7)
Find the value of determinant \begin{vmatrix} 5 & -3 \\ -7 & -4 \end{vmatrix}
Find the relation between x and y, if the points x,y i.e (1,2) and (7,0) are collinears.
If A =\begin{bmatrix} 1 & -1 & 2 \\ 3 & 0 & -2 \\ 1 & 0 & 3 \end{bmatrix}, then verify that A(adjA)=(adjA)A= I \left| A \right|.
Find the value of a if the points P ( 1,5 ) , Q ( a , 1 ) and R ( 4,11 ) are collinear .
Find the largest value of a third order determinant whose elements are 1 or -1
Find the cofactor matrix of the determinant \begin{vmatrix} 1 & 2 & 3\\ -4 & 3 & 6 \\ 2 & -7 & 9 \end{vmatrix}
If the points (x_{1},\ y_{1}),\ (x_{2},\ y_{2}) and (x_{3},\ y_{3}) are collinear, show that \displaystyle \sum { \left( \frac { { y }_{ 1 }-{ y }_{ 2 } }{ { x }_{ 1 }{ x }_{ 2 } } \right) } =0, i.e
\dfrac {y_{1}-y_{2}}{x_{1}x_{2}}+\dfrac {y_{2}-y_{3}}{x_{2}x_{3}}+\dfrac {y_{3}-y_{1}}{x_{3}x_{1}}=0
If A = \left[ {\begin{array}{*{20}{c}}1 & { - 2} & 3\\4 & 0 & { - 1}\\{ - 3} & 1 & 5\end{array}} \right], then {\left( {adj\,A} \right)} is equal to
Find the largest value of a third order determinant whose elements are 0 or 1
adj (adj A) = |A|^{n - 2} A
Prove that \begin{vmatrix} { yz-x }^{ 2 } & { zx-y }^{ 2 } & xy-z^{ 2 } \\ { zx-y }^{ 2 } & { xy }-z^{ 2 } & { yz-x }^{ 2 } \\ { xy-z }^{ 2 } & { yz-x }^{ 2 } & { zx-y }^{ 2 } \end{vmatrix} is divisible by (x+y+z) and hence find the quotient
If A=\begin{pmatrix} 2 & 3 & 4 \\ 0 & -2 & 1 \\ 3 & -1 & 2 \end{pmatrix},B=\begin{pmatrix} 2 & 0 & -3 \\ 4 & 0 & -1 \\ 3 & 4 & 5 \end{pmatrix} and C=\begin{pmatrix} 5 & 6 & 7 \\ -1 & 2 & 3 \\ 4 & -5 & 4 \end{pmatrix} Prove that A(BC) and (AB)C.
Using properties of determinant, prove that \begin{vmatrix} b+c & a-b & a \\ c+a & b-c & b \\ a+b & c-a & c \end{vmatrix}=3abc-{ a }^{ 3 }-{ b }^{ 3 }-{ c }^{ 3 }
Compute the adjoint of the following matrice:
\begin{bmatrix} 2 & -1 & 3 \\ 4 & 2 & 5 \\ 0 & 4 & -1 \end{bmatrix}
Verify that (adj A)A = |A|I = A(adj A) for the above matrice.
Compute the adjoint of the following matrice:
\begin{bmatrix} 1 & 2 & 2 \\ 2 & 1 & 2 \\ 2 & 2 & 1 \end{bmatrix}
Verify that (adj A)A = |A|I = A(adj A) for the above matrice.
Compute the adjoint of the following matrice:
\begin{bmatrix} 1 & 2 & 5 \\ 2 & 3 & 1 \\ -1 & 1 & 1 \end{bmatrix}
Verify that (adj A)A = |A|I = A(adj A) for the above matrice.
Find the adjoint of the following matrice:
\begin{bmatrix} -3 & 5 \\ 2 & 4 \end{bmatrix}
Verify that (adj A ) A = |A| I = A (adj A) for the above matrice.
Find the adjoint of the following matrice:
\begin{bmatrix} 1 & \tan\,\alpha /2 \\ -\tan\,\alpha /2 & 1 \end{bmatrix}
Verify that (adj A ) A = |A| I = A (adj A) for the above matrice.
Verify that (adj A ) A = |A| I = A (adj A) for the above matrice.
Find A (adj A) for the matrix A = \begin{bmatrix} 1 & -2 & 3 \\ 0 & 2 & 1 \\ -4 & 5 & 2 \end{bmatrix}.
Find the adjoint of the matrix A= \begin{bmatrix} -1 & -2 & -2 \\ 2 & 1 & -2 \\ 2 & -2 & 1 \end{bmatrix} and hence show that A(adj\: A)= \left | A \right |I_{3}.
Compute the adjoint of the following matrice:
\begin{bmatrix} 2 & 0 & -1 \\ 5 & 1 & 0 \\ 1 & 1 & 3 \end{bmatrix}
Verify that (adj A)A = |A|I = A(adj A) for the above matrice.
\left| \begin{matrix} 0 & xyz & x-z \\ y-x & 0 & y-z \\ z-x & z-y & 0 \end{matrix} \right| =_________
Class 12 Commerce Maths Extra Questions
- Application Of Derivatives Extra Questions
- Application Of Integrals Extra Questions
- Continuity And Differentiability Extra Questions
- Determinants Extra Questions
- Differential Equations Extra Questions
- Integrals Extra Questions
- Inverse Trigonometric Functions Extra Questions
- Linear Programming Extra Questions
- Matrices Extra Questions
- Probability Extra Questions
- Relations And Functions Extra Questions
- Three Dimensional Geometry Extra Questions
- Vector Algebra Extra Questions