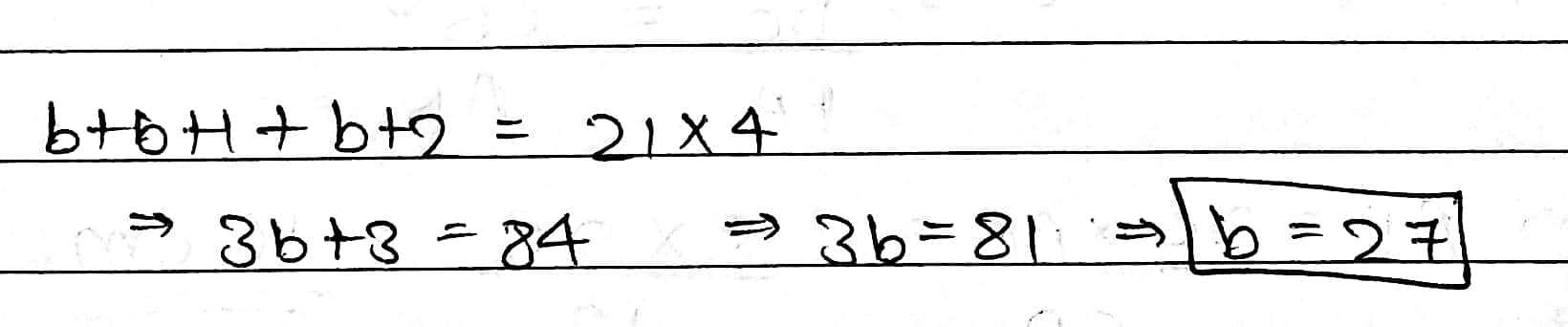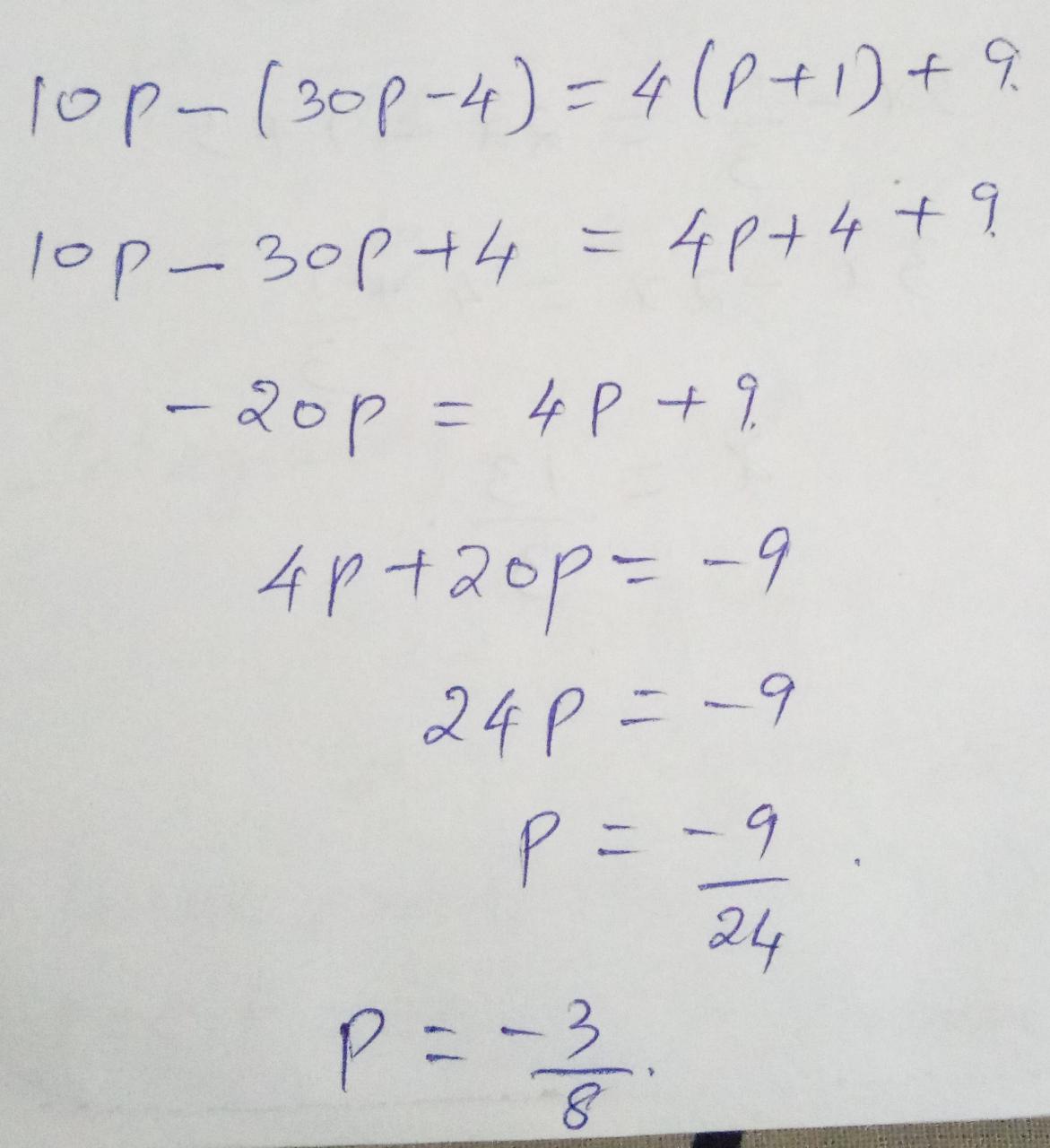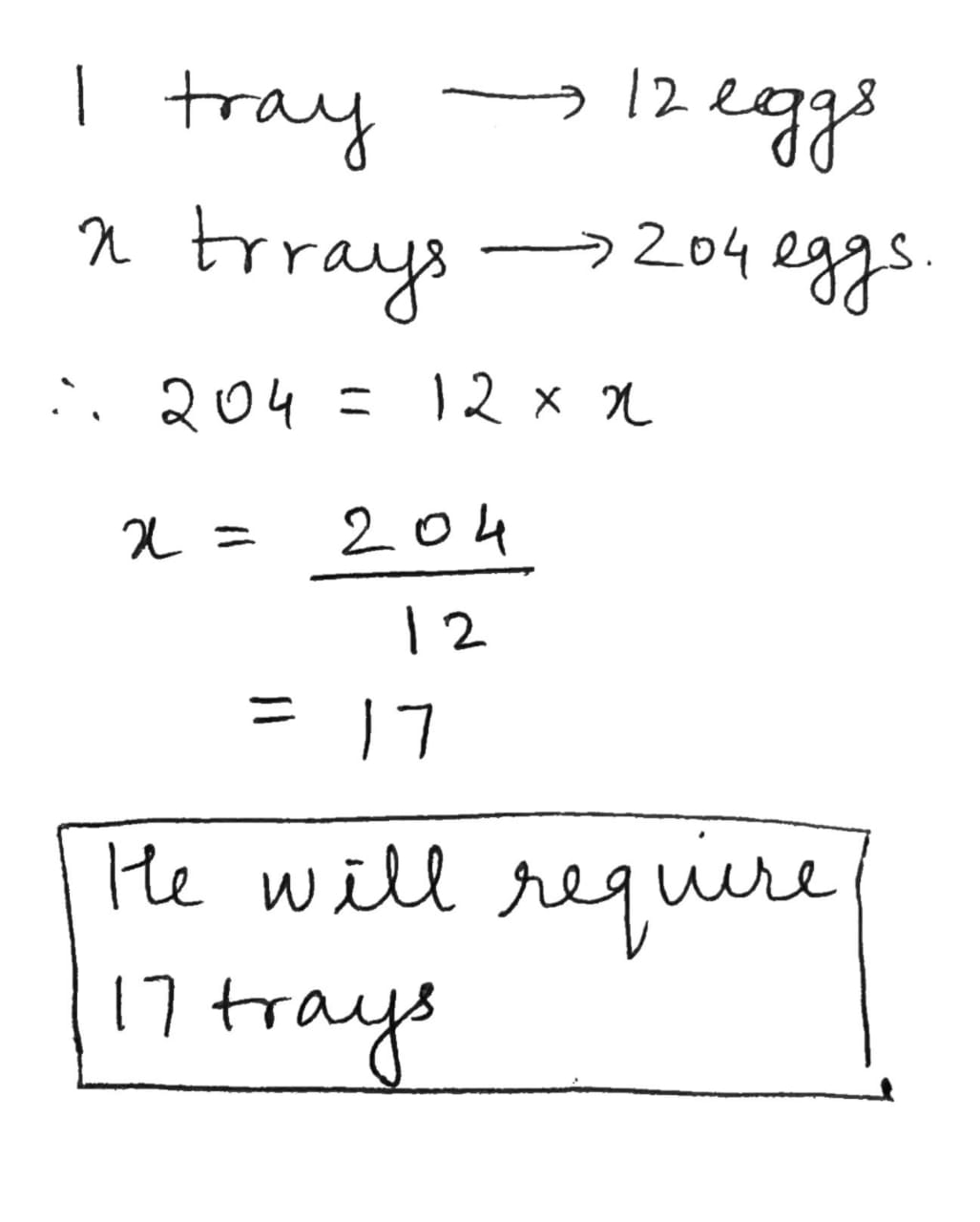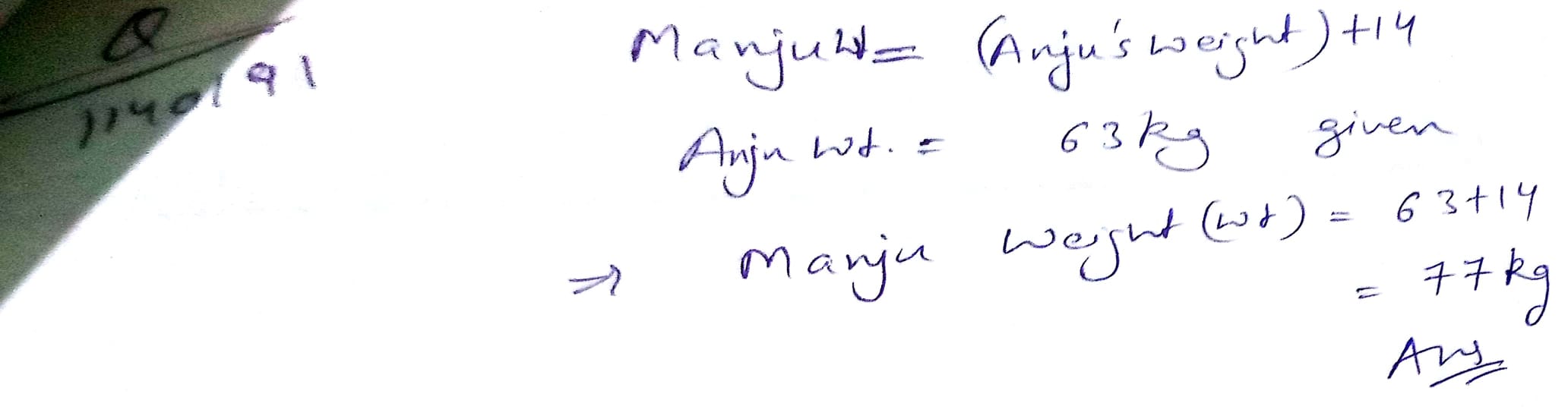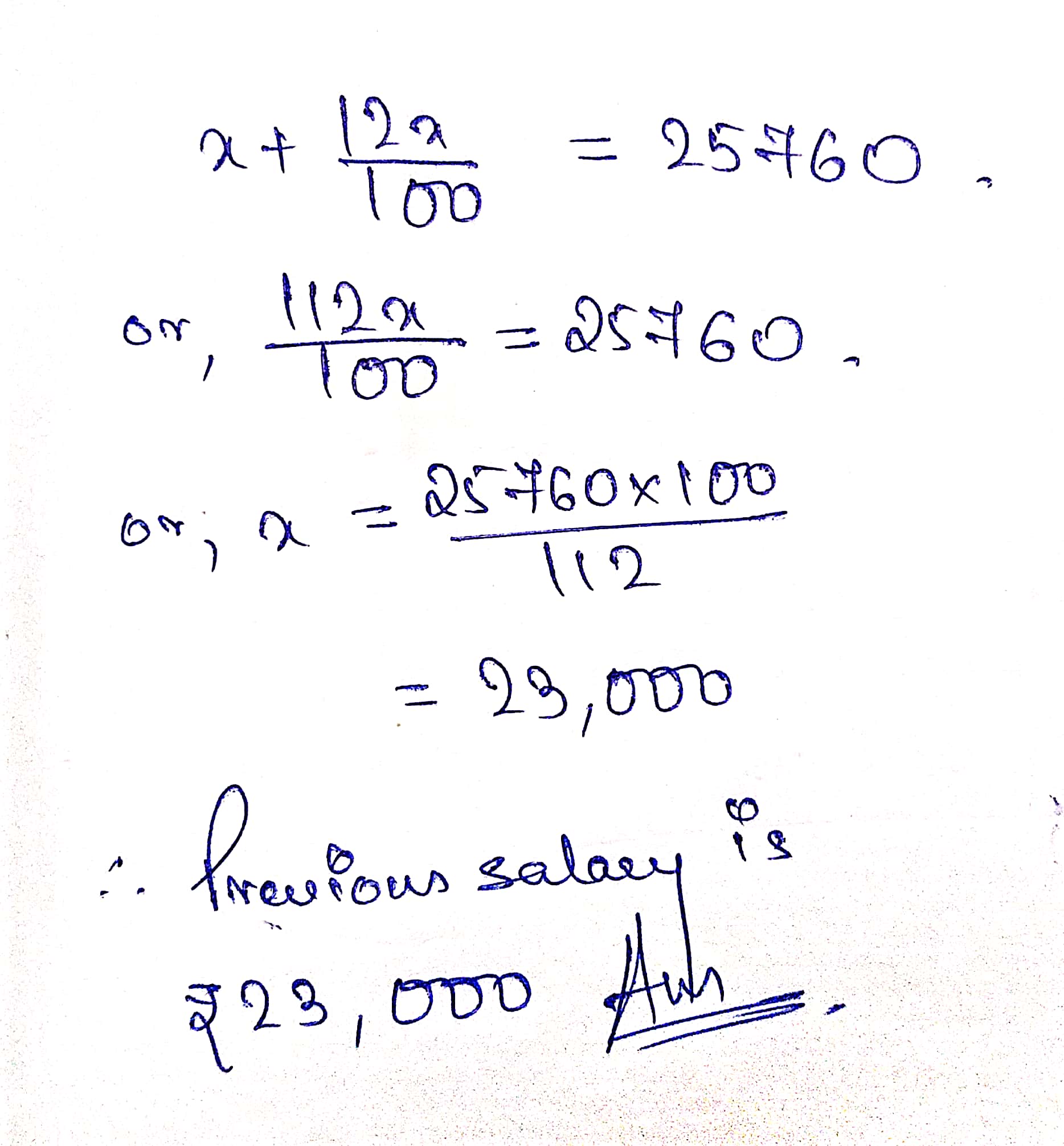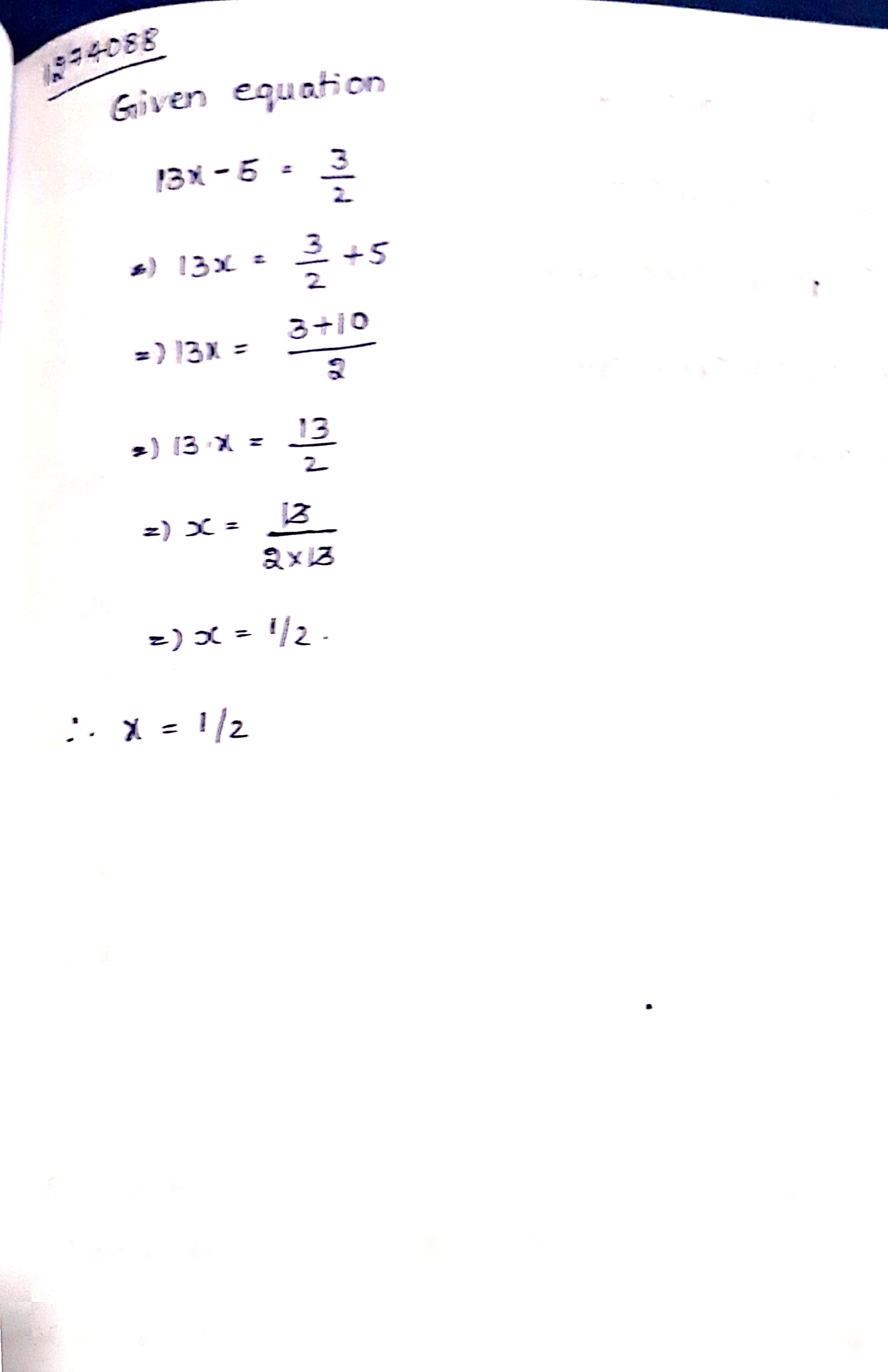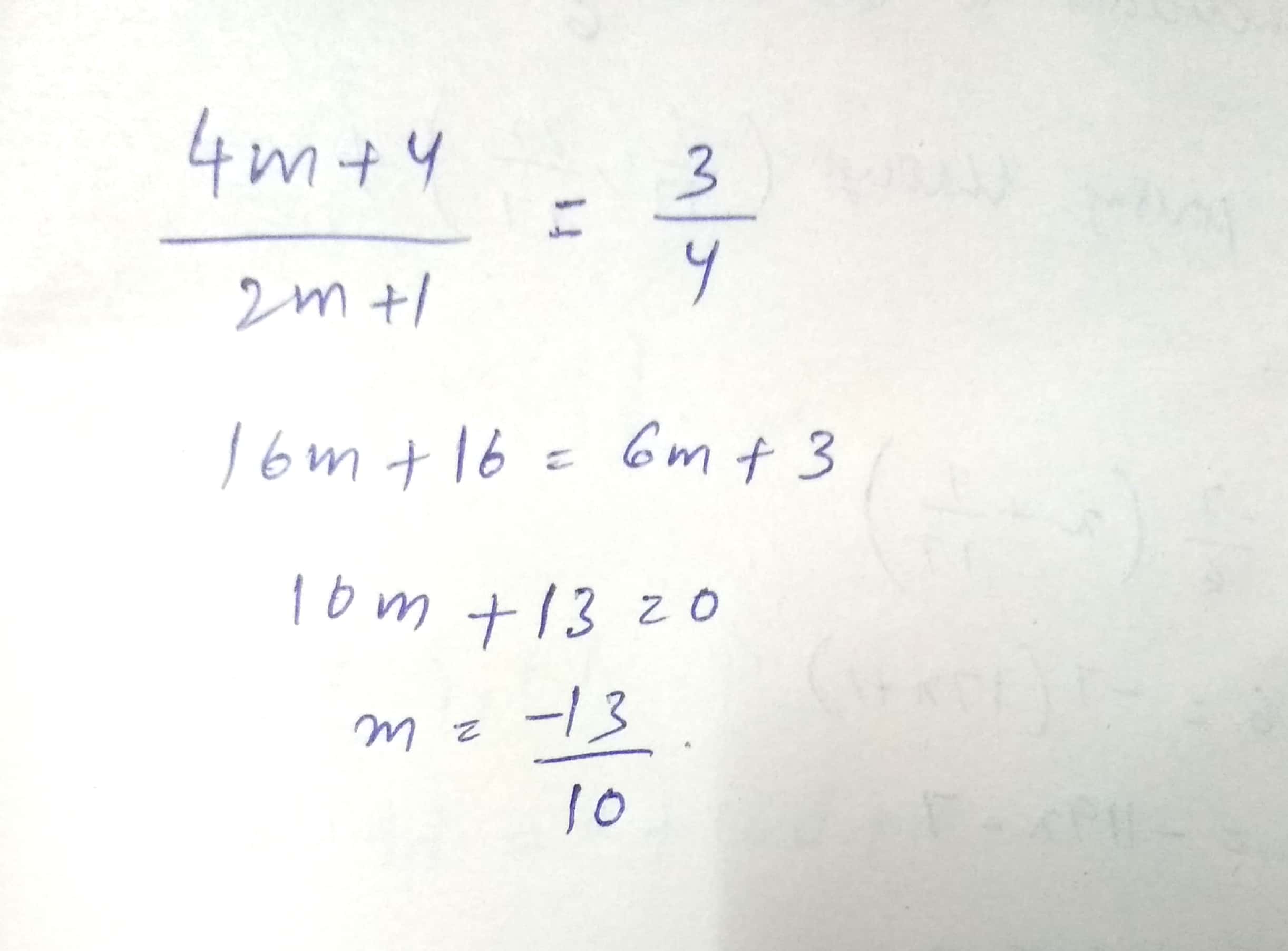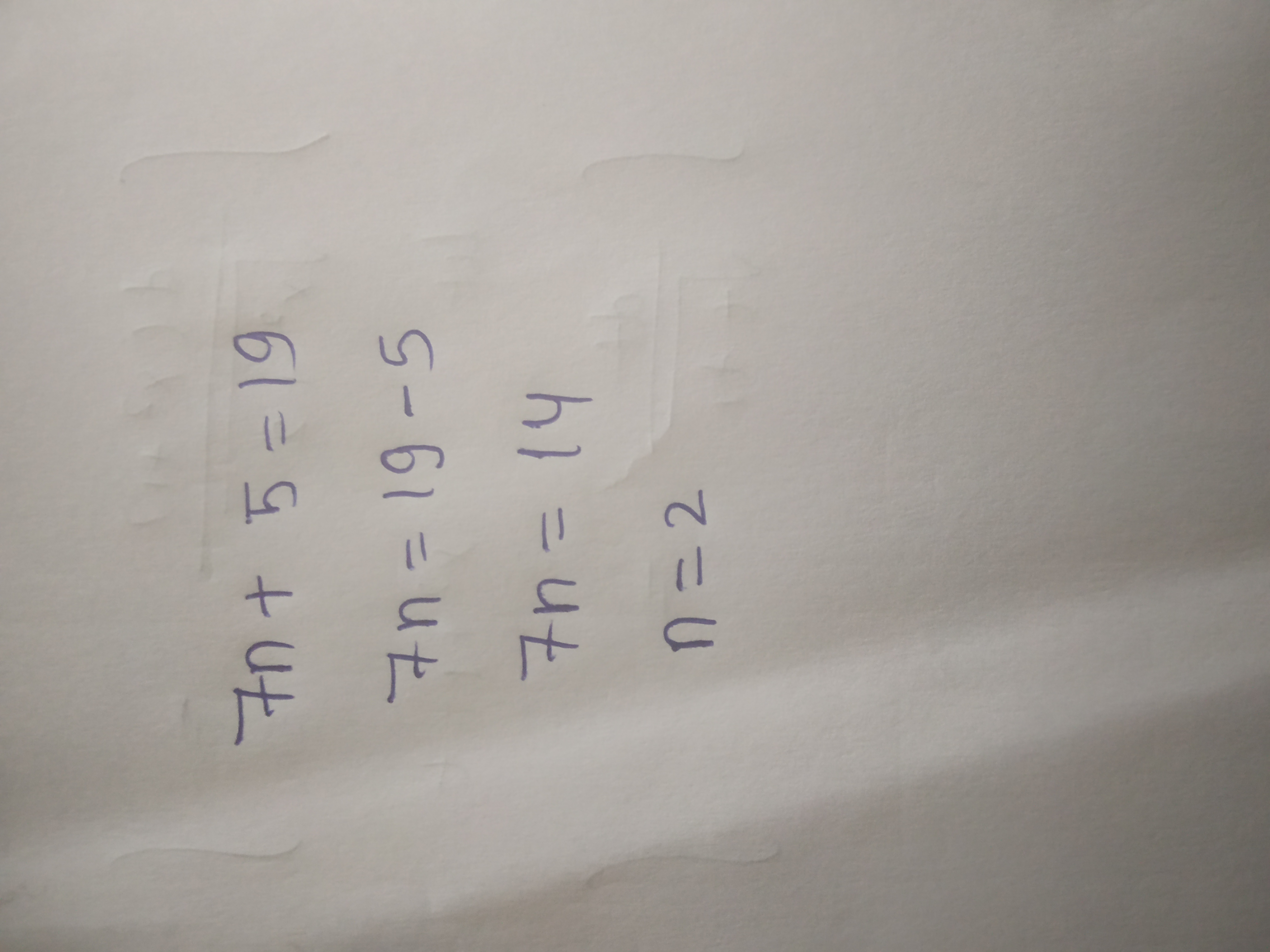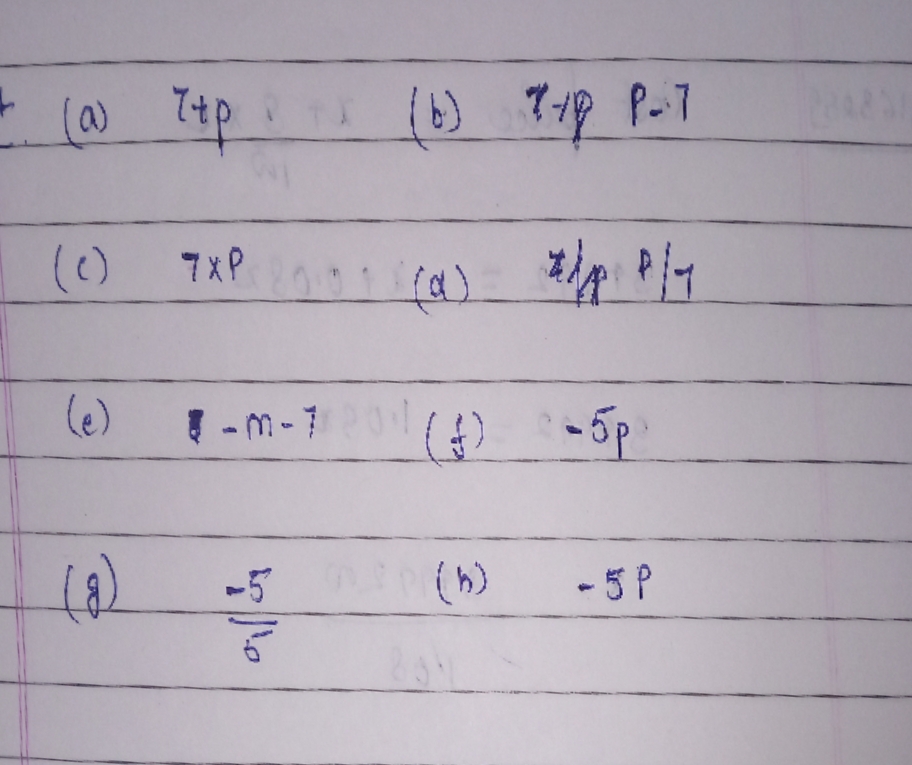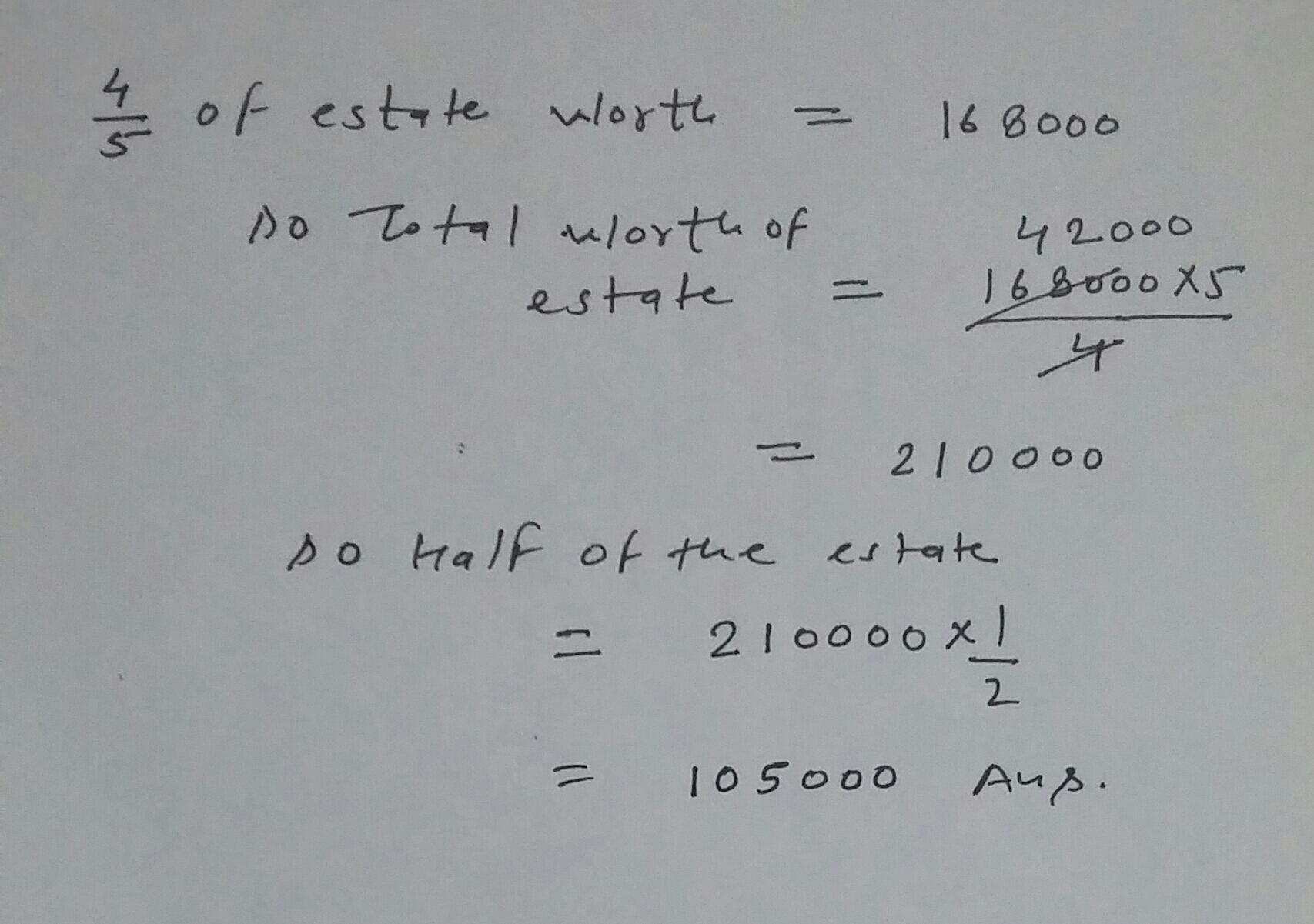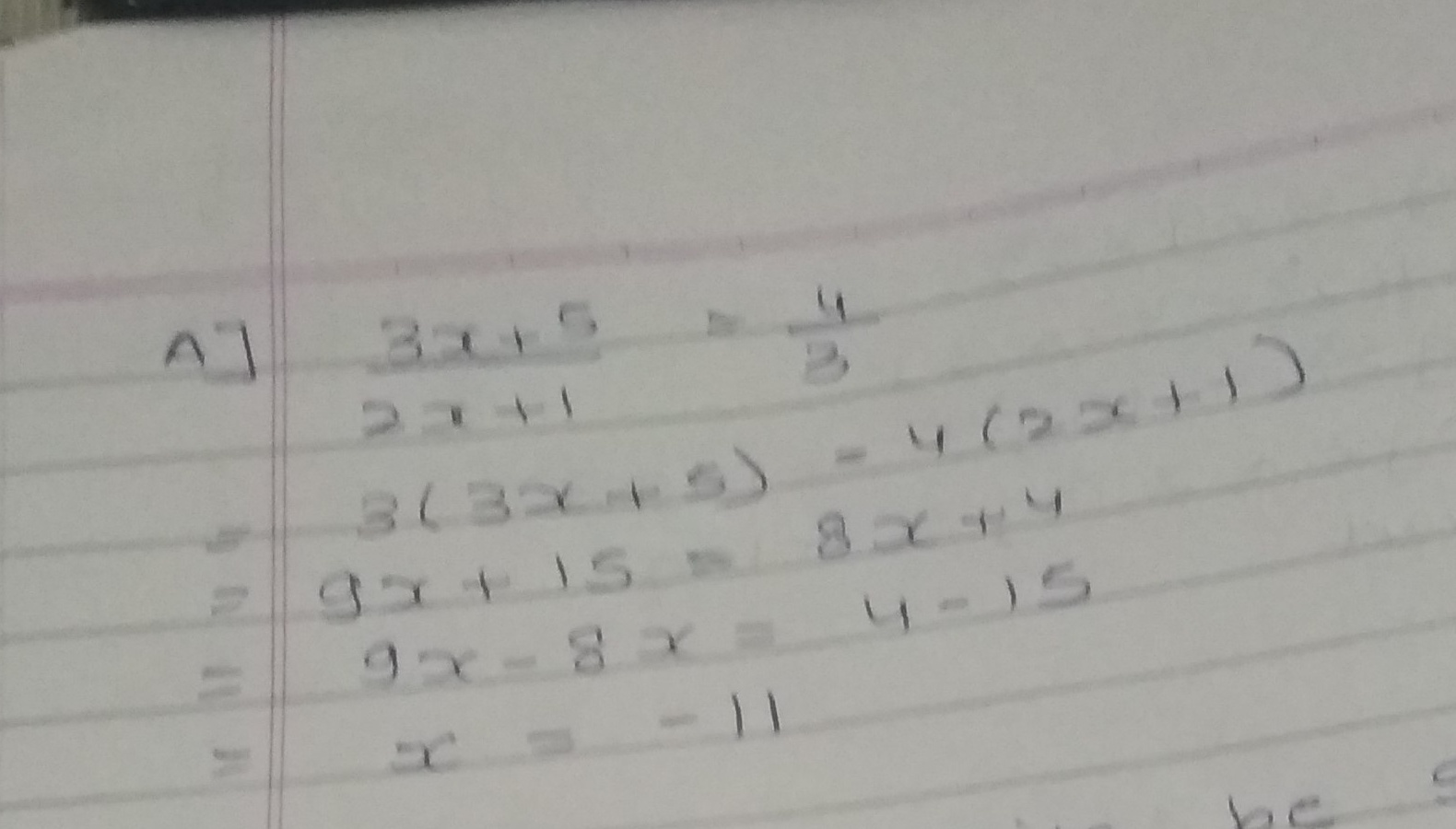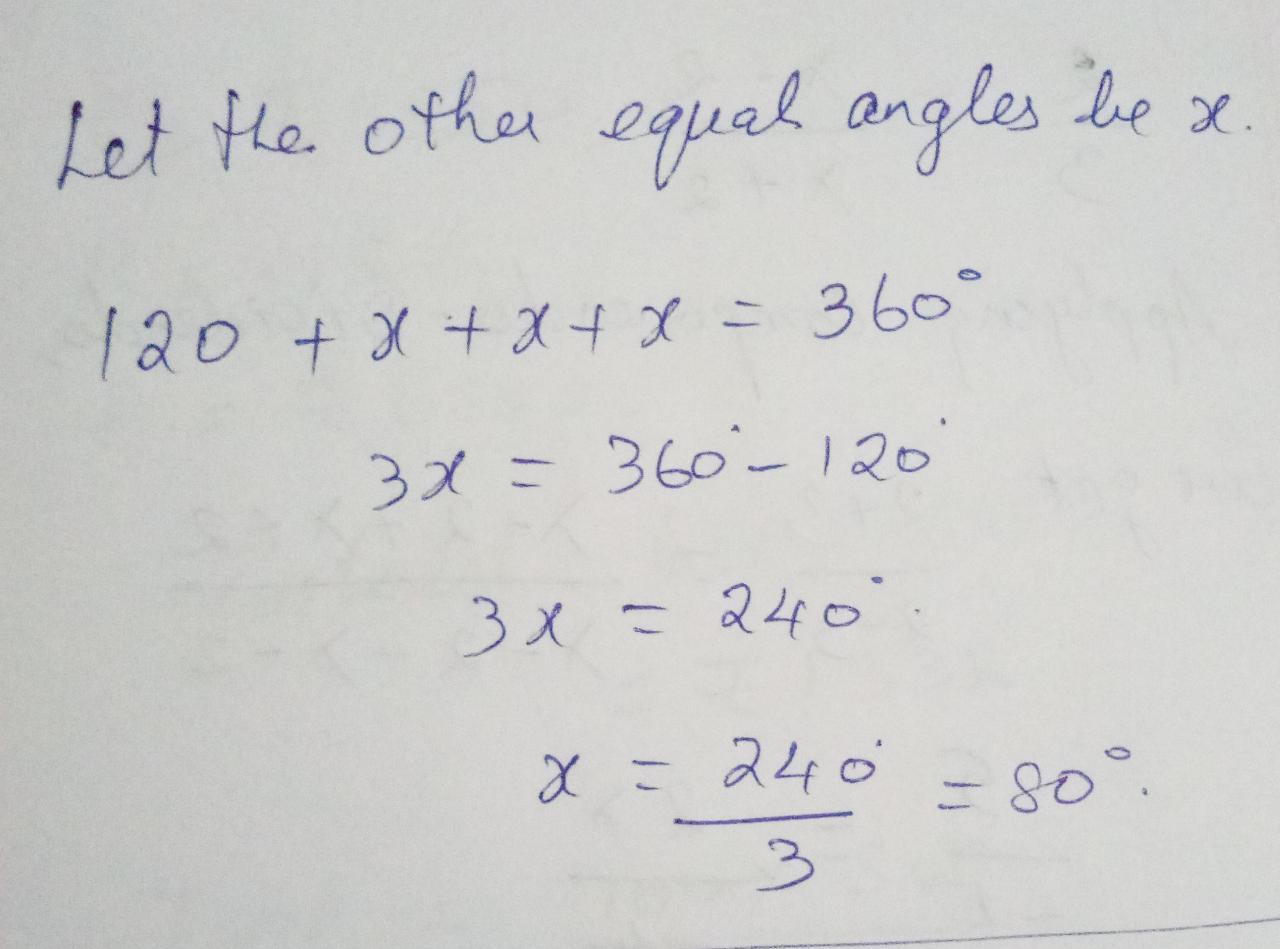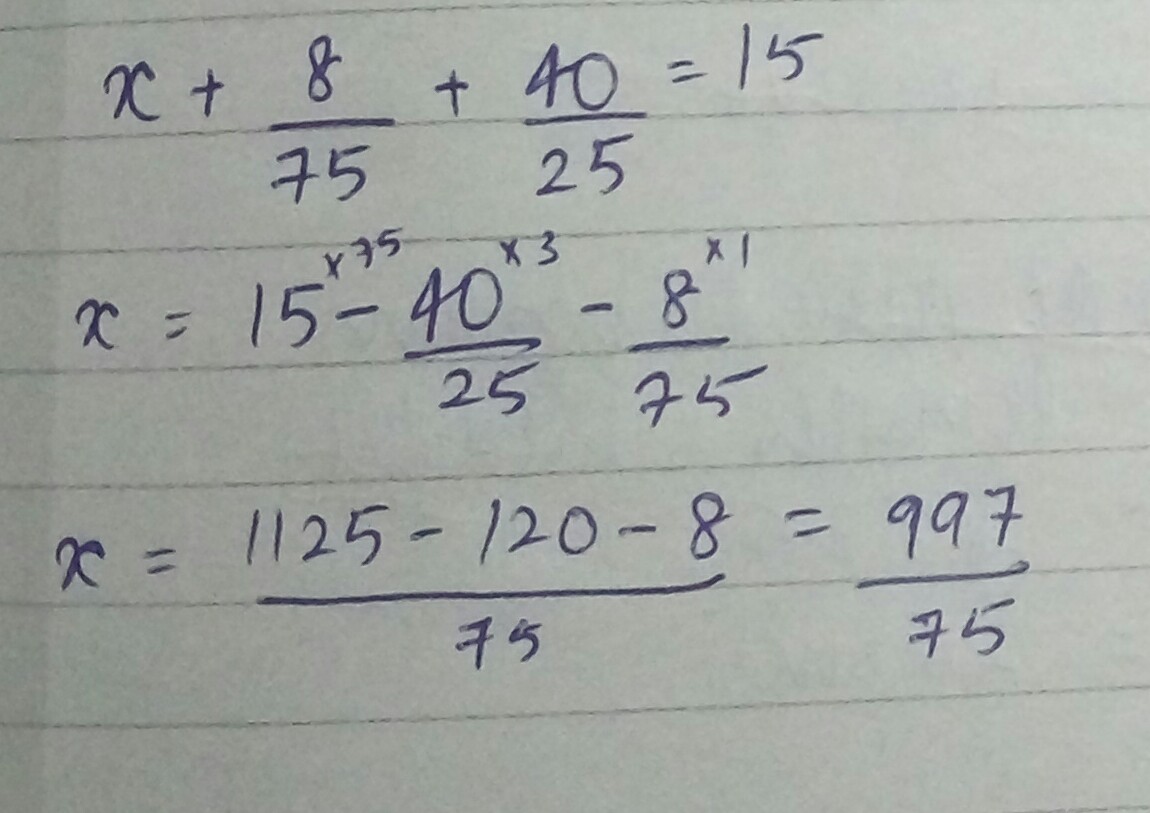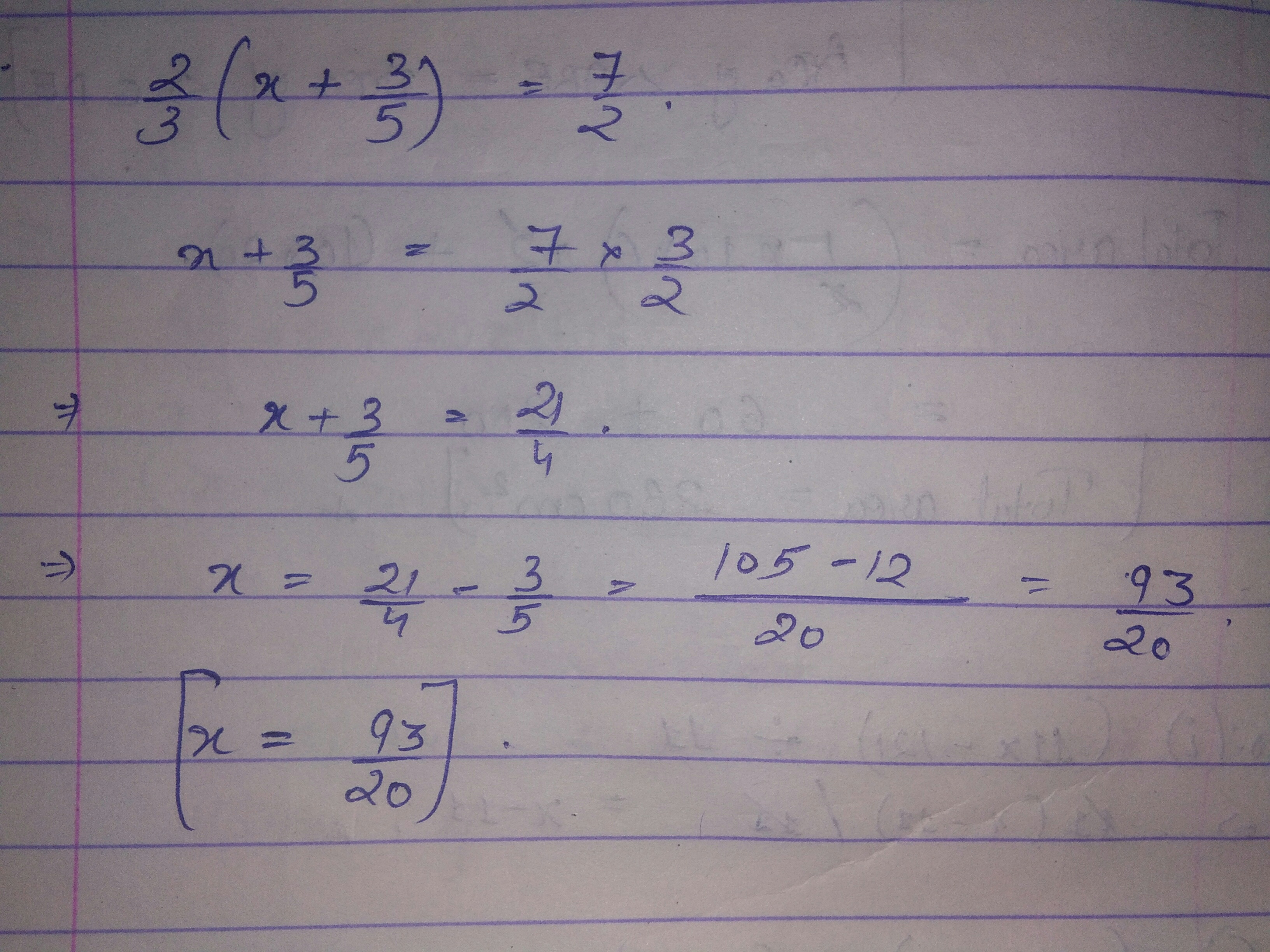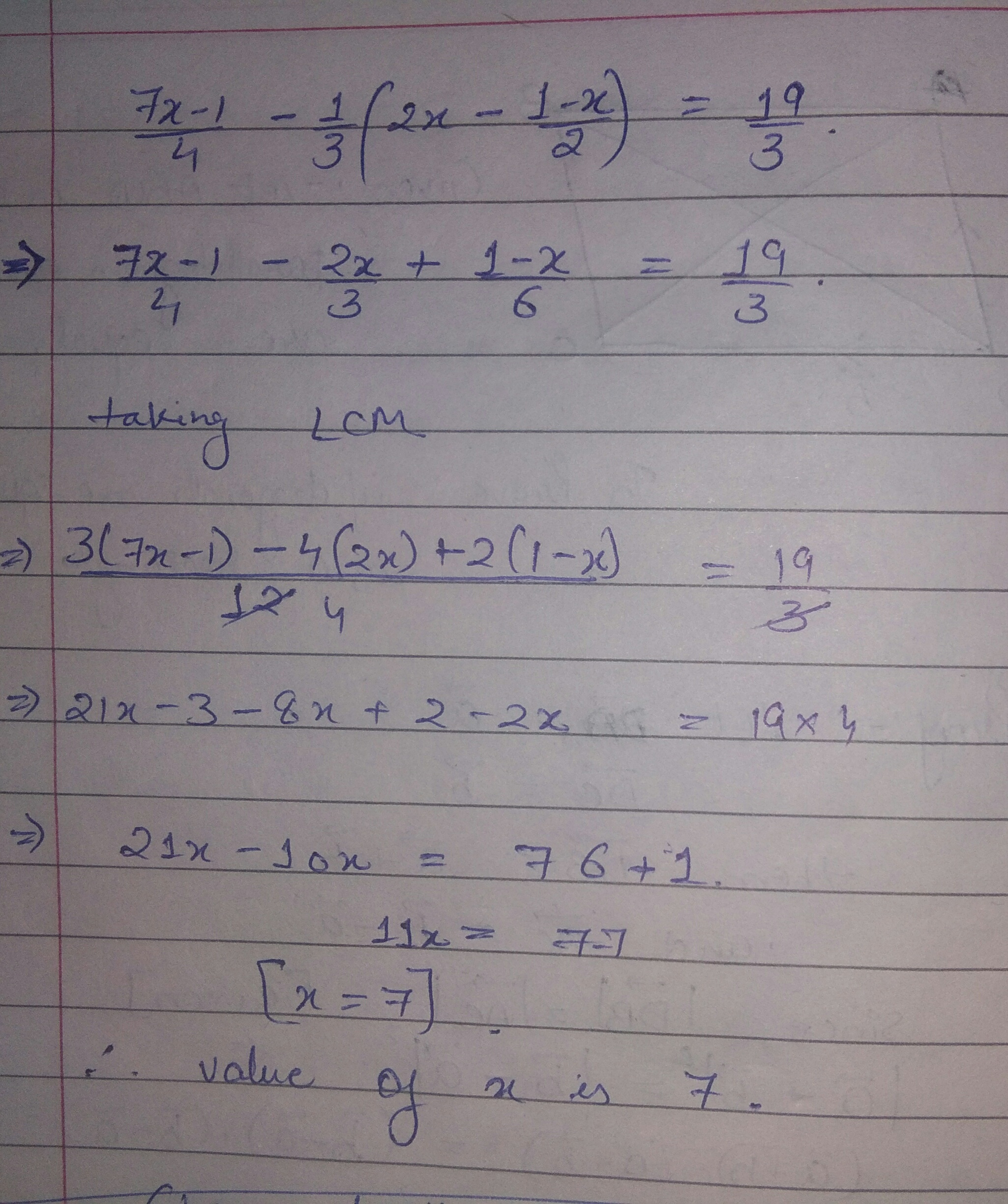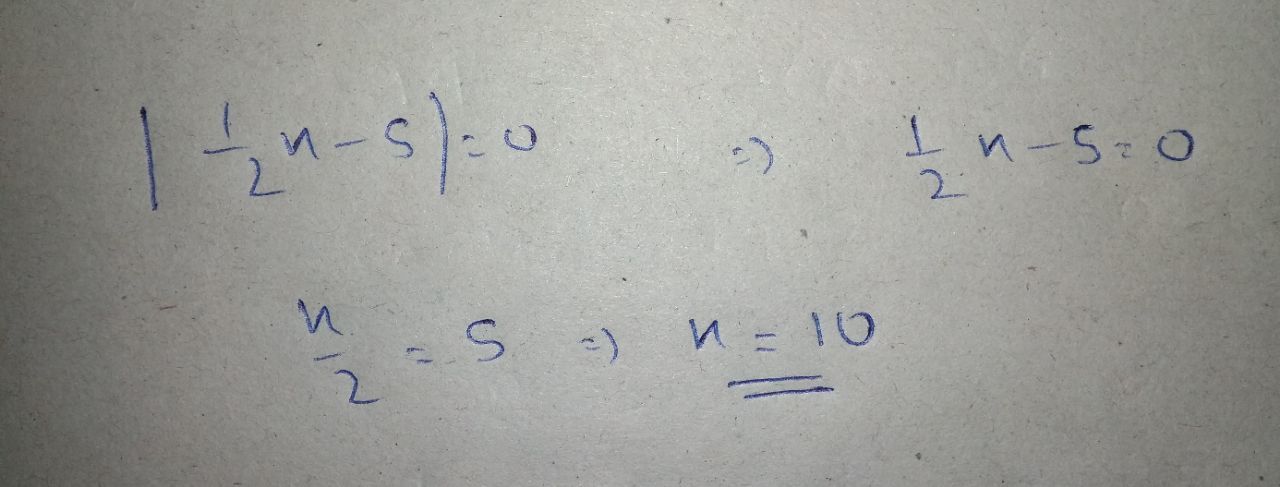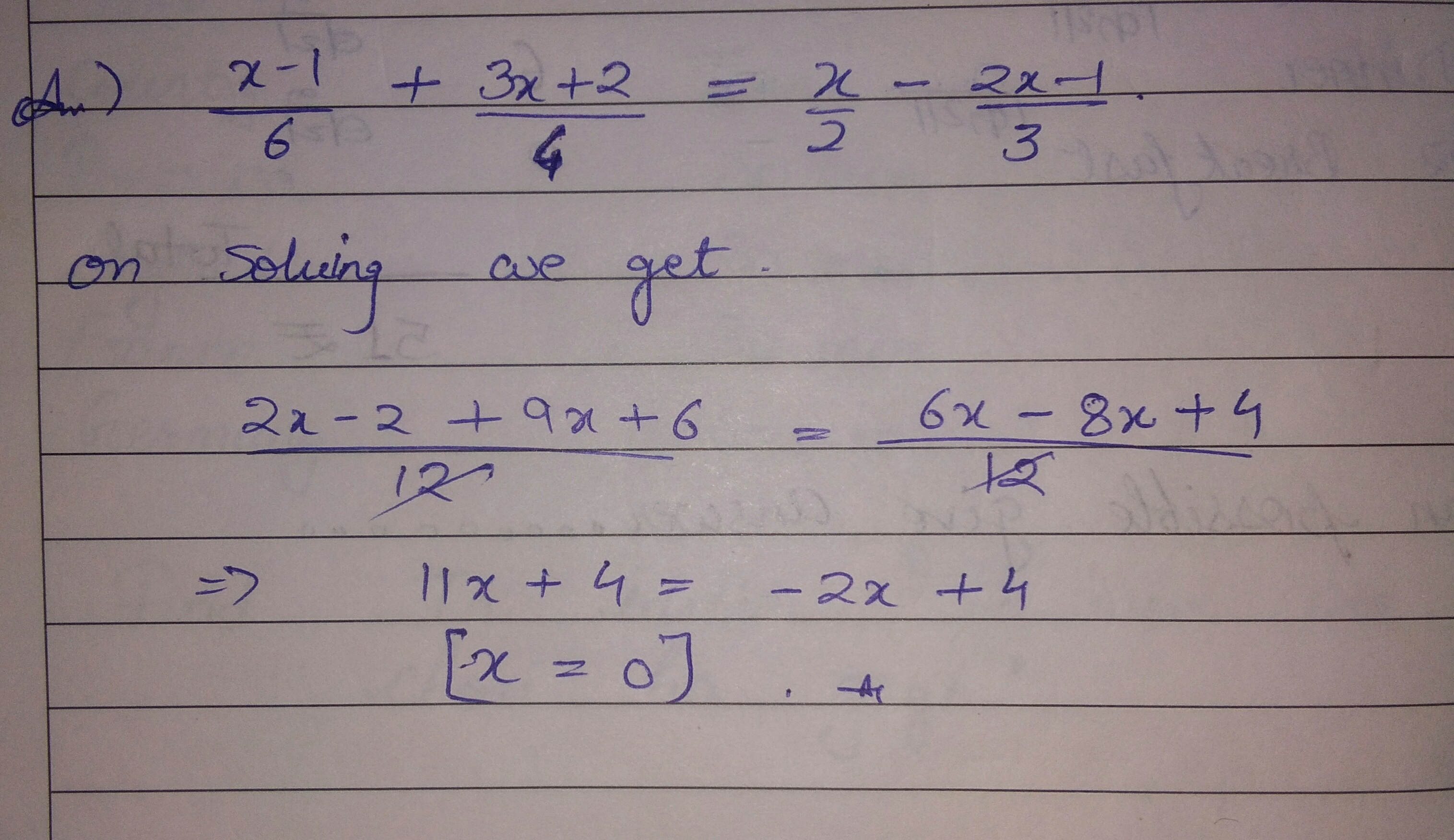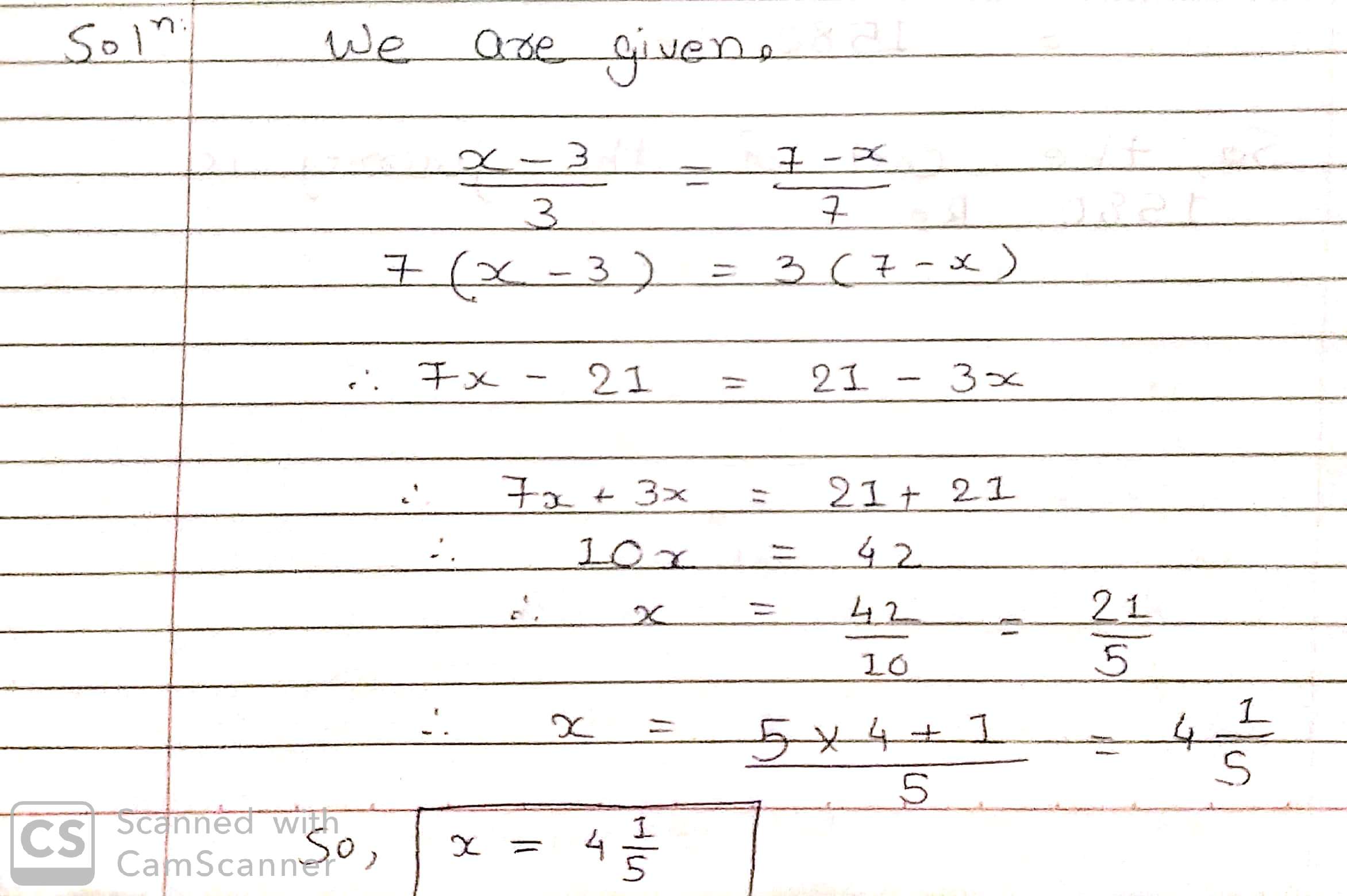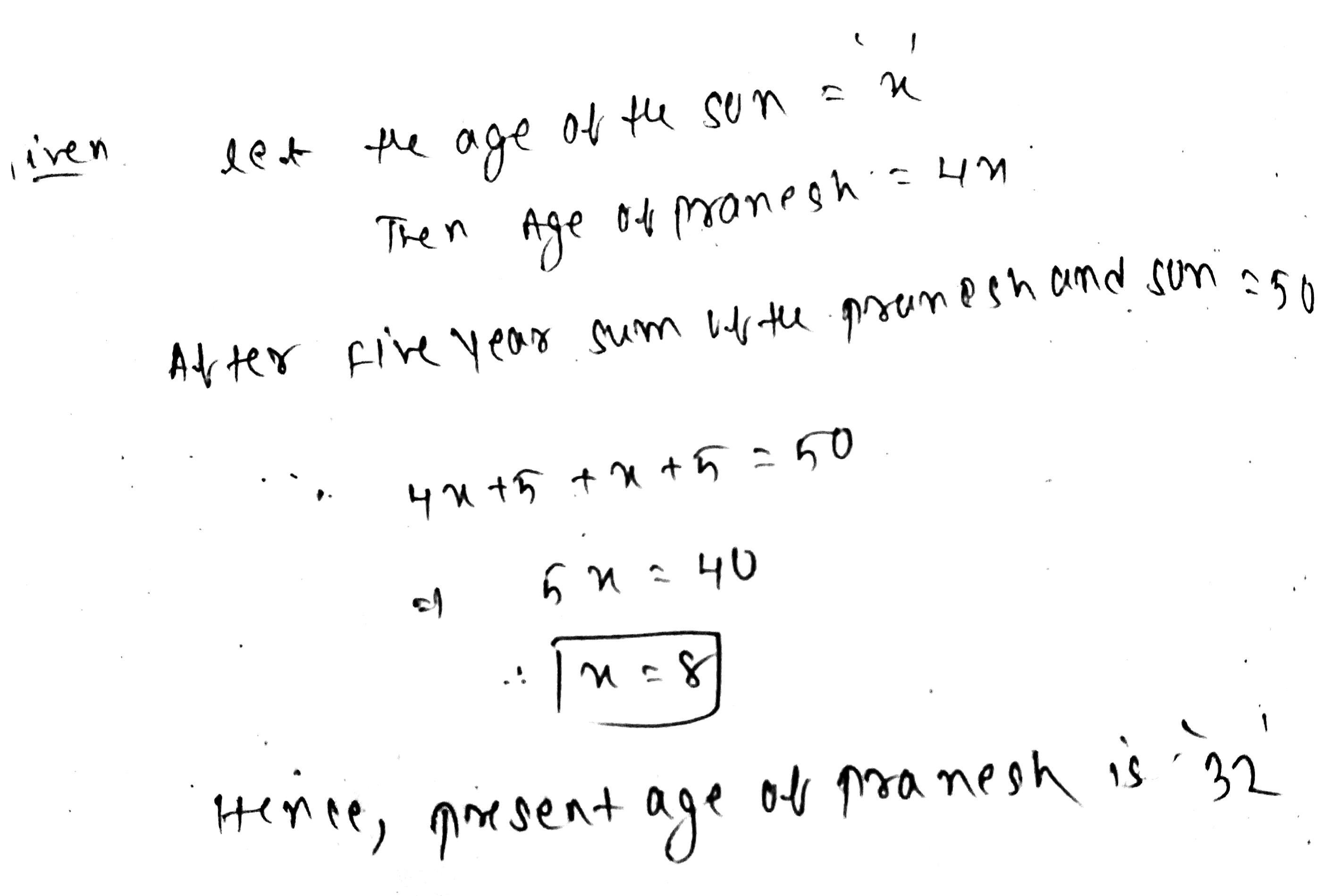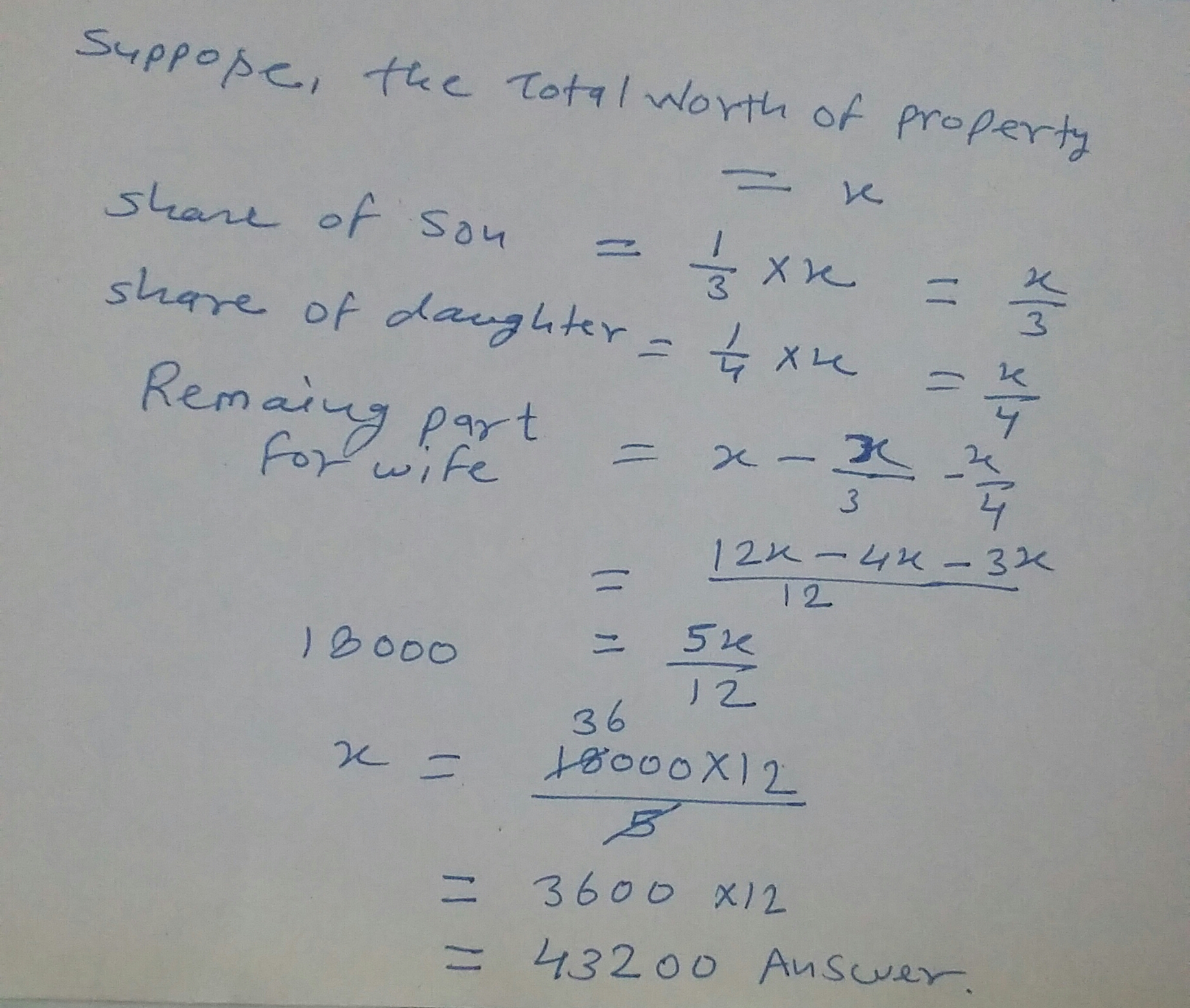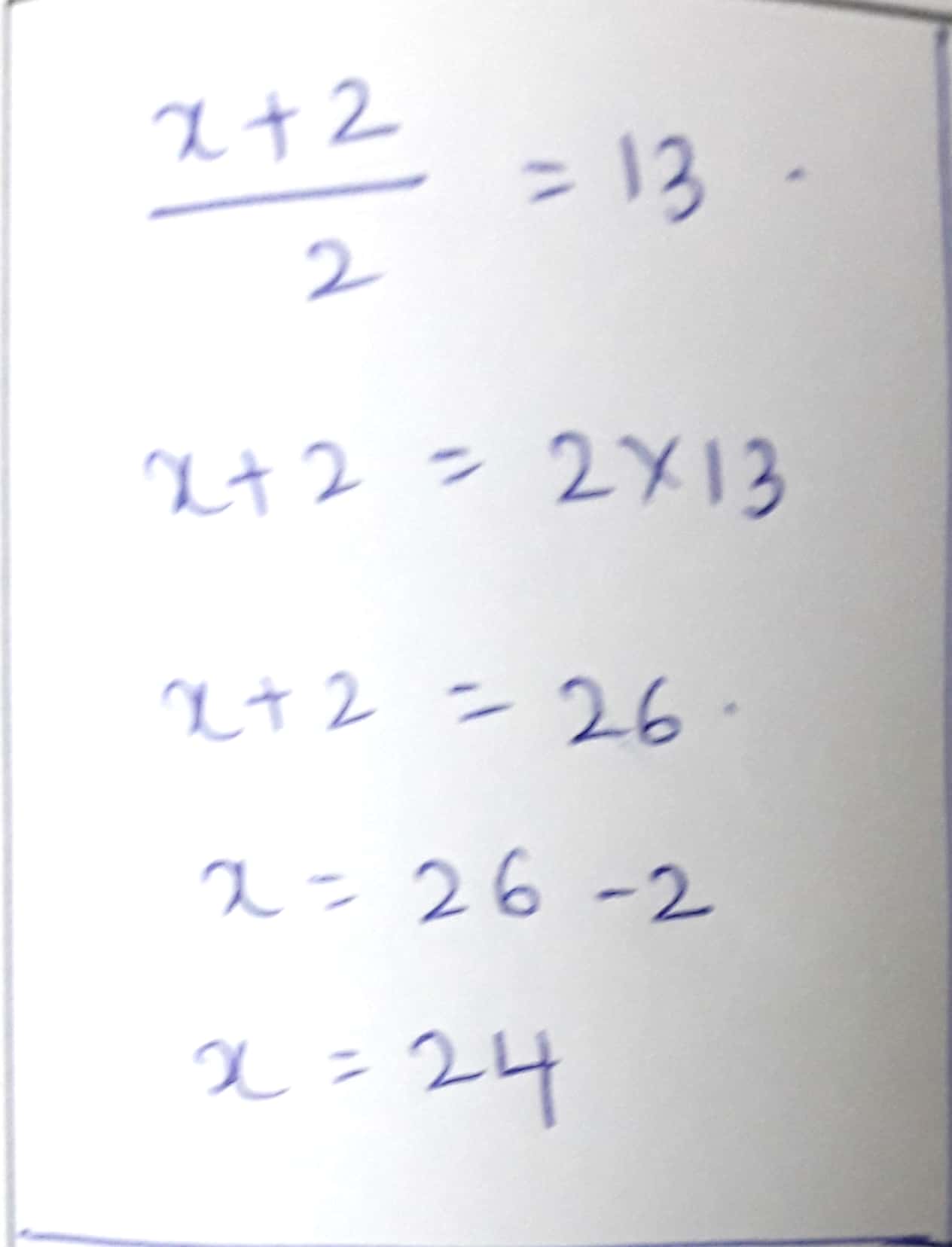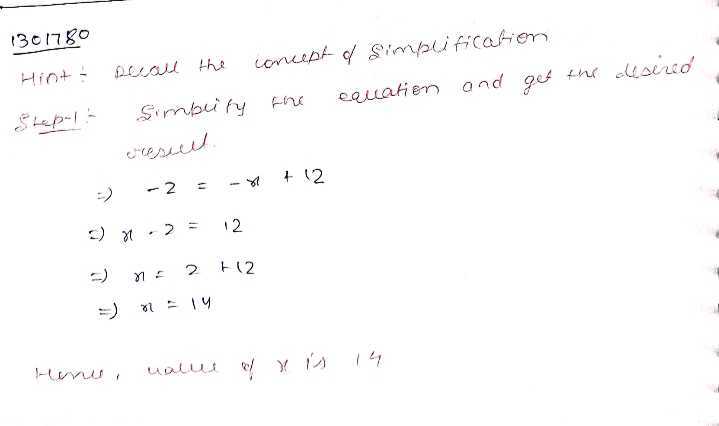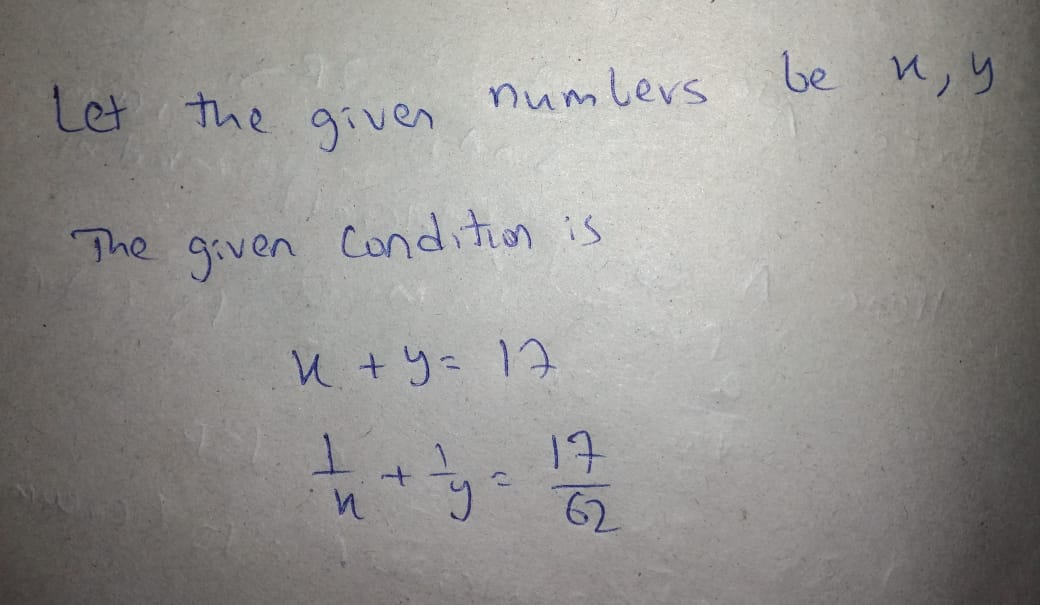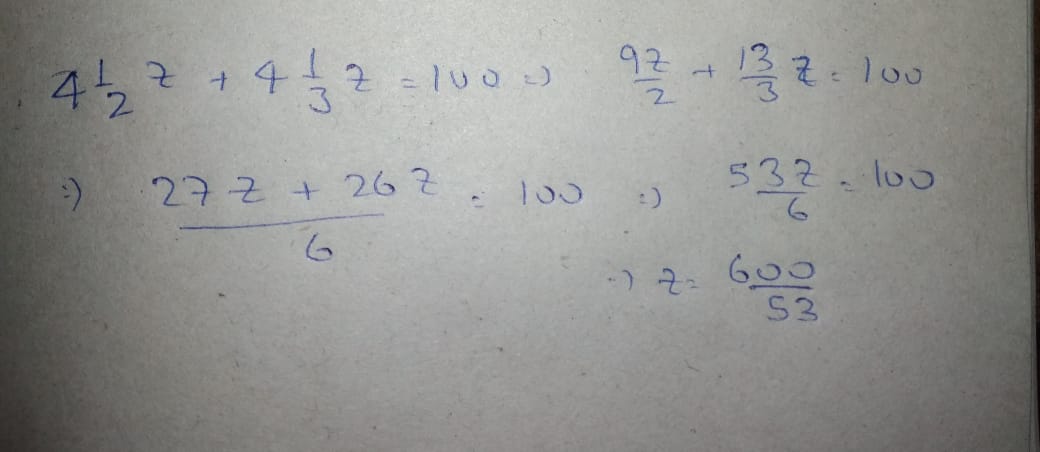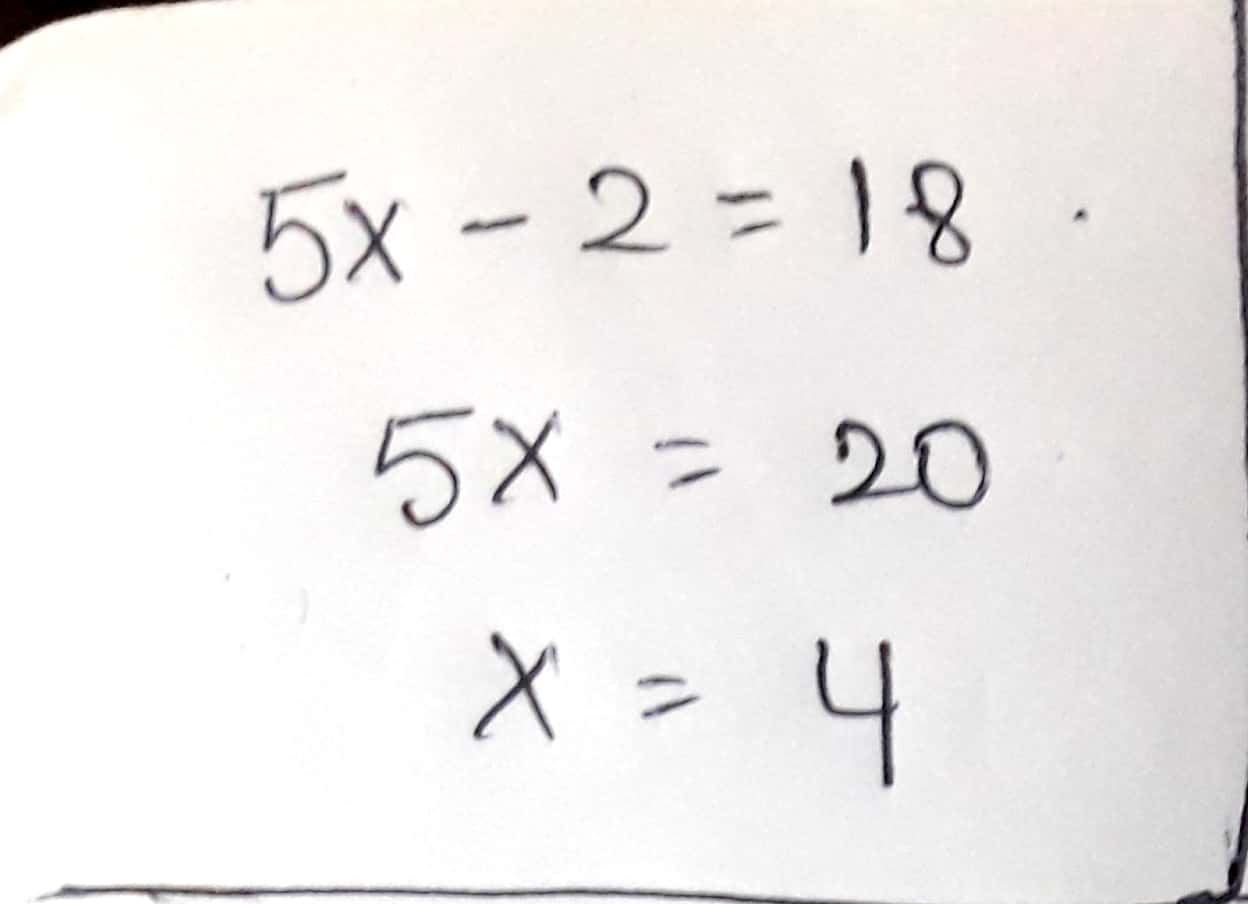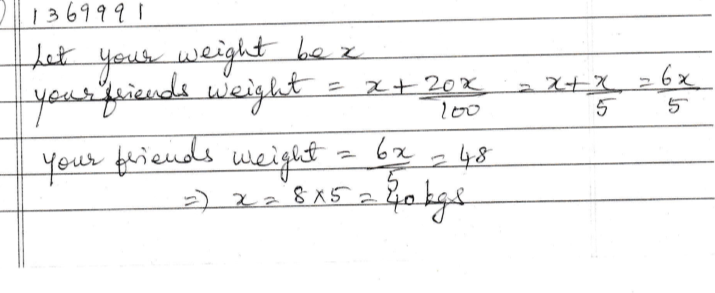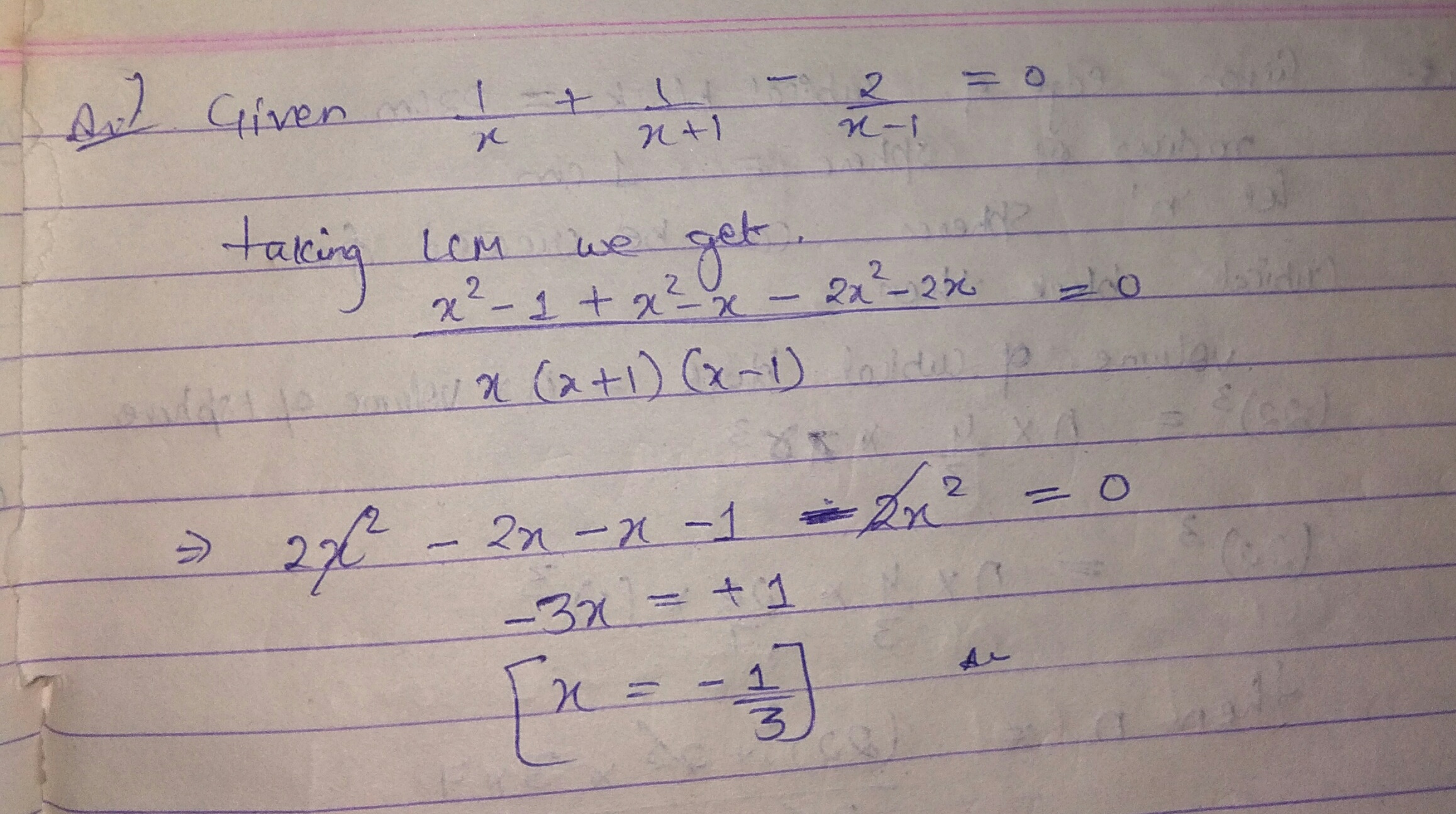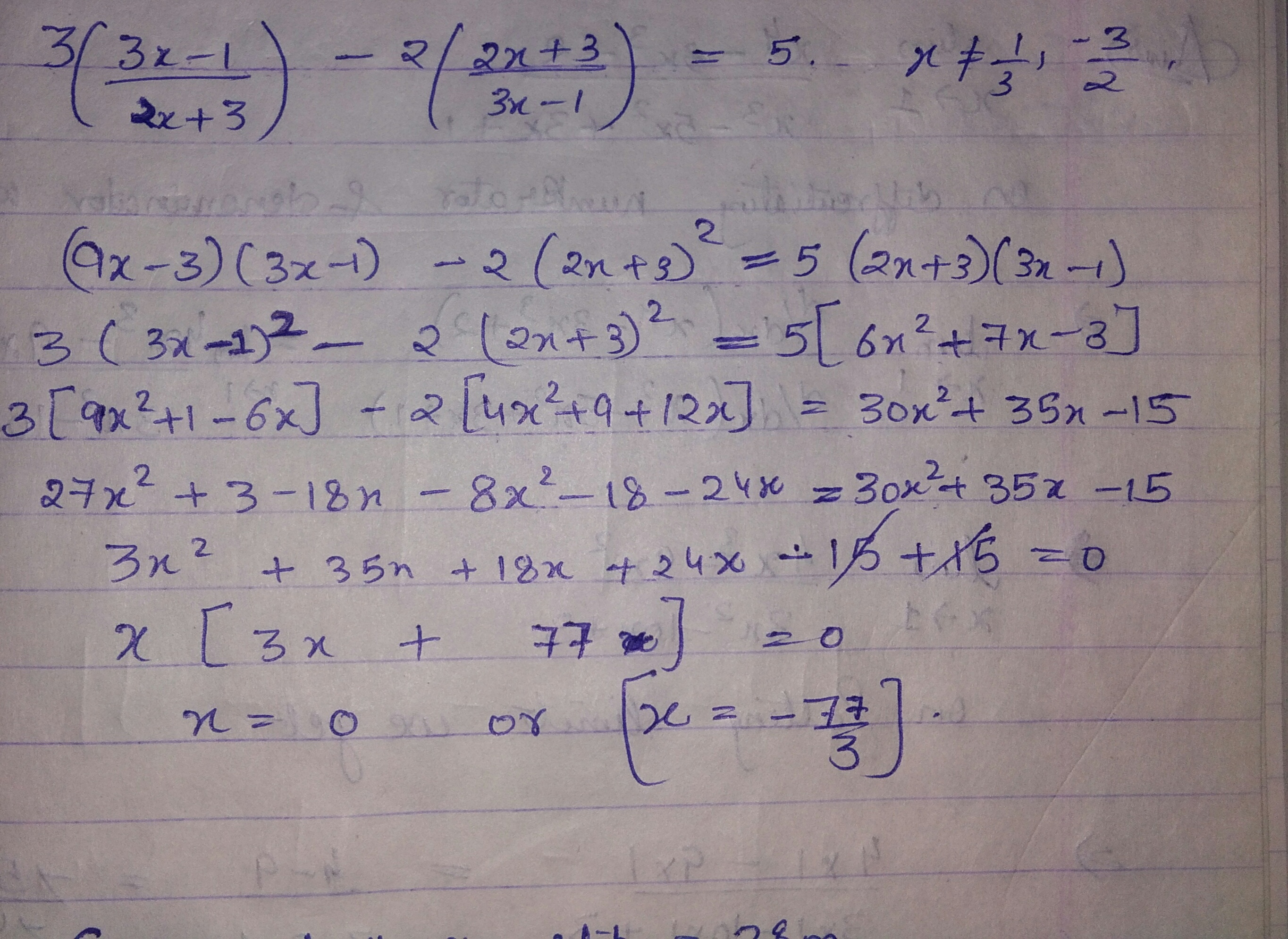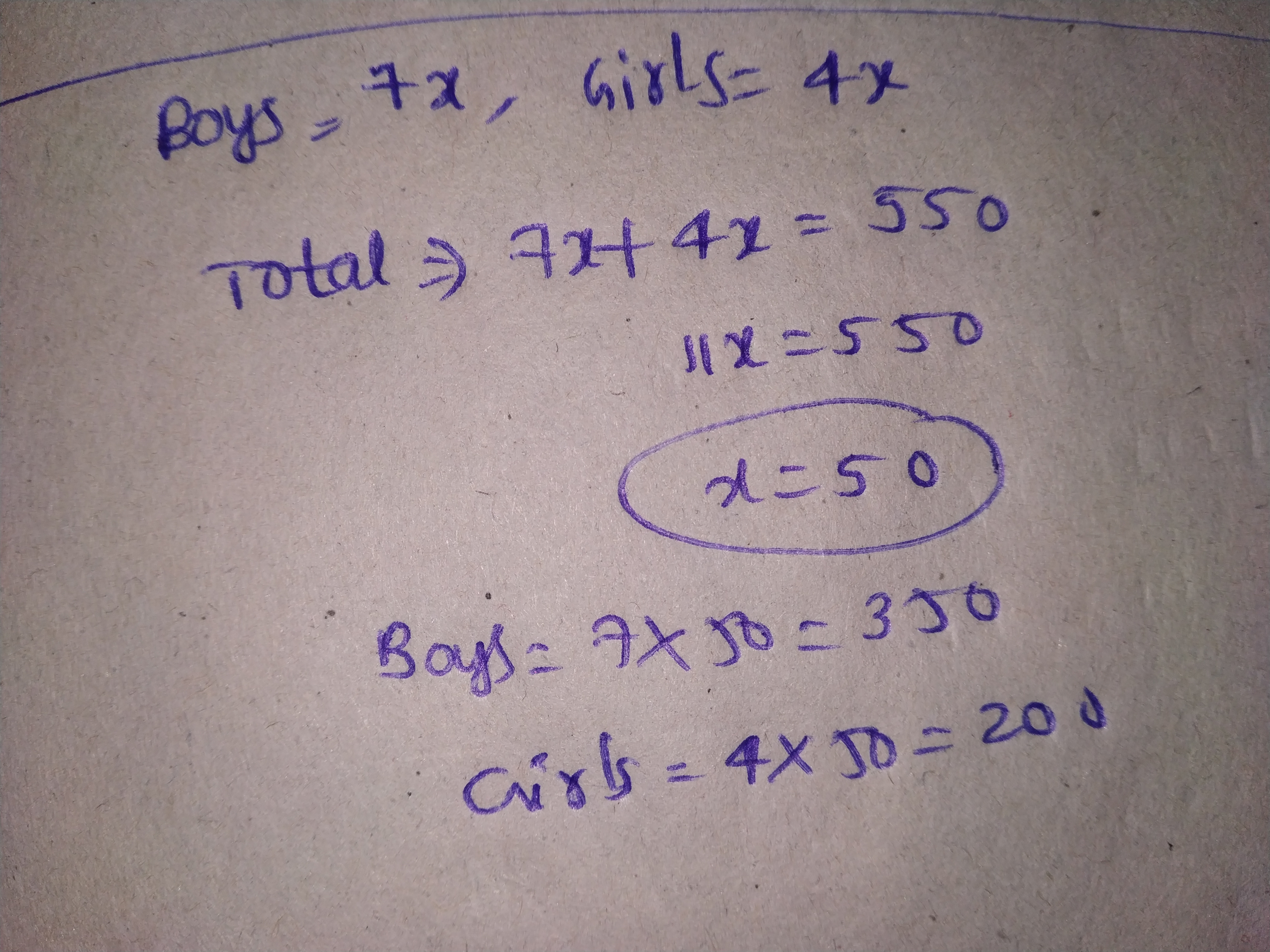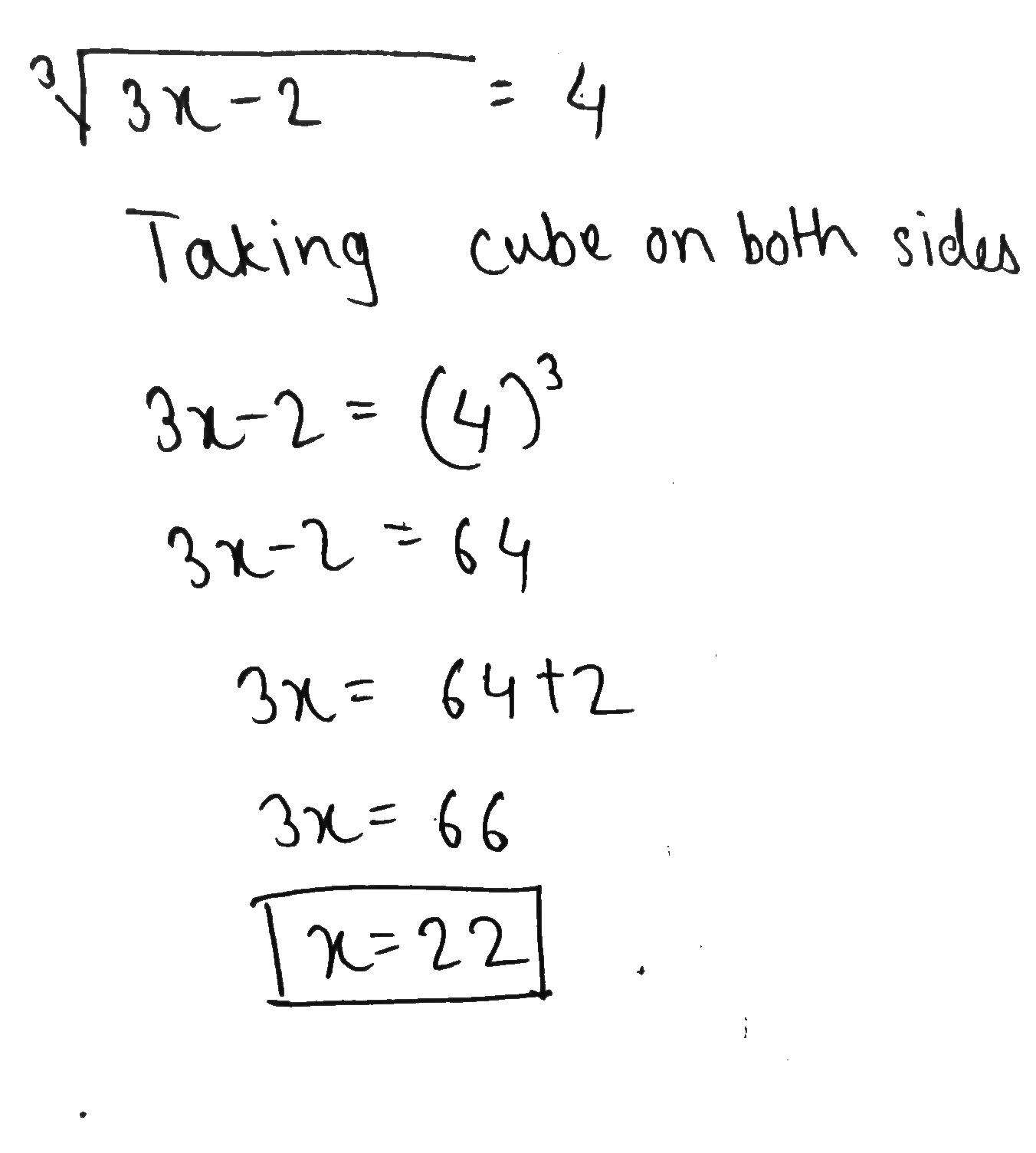Linear Equations In One Variable - Class 8 Maths - Extra Questions
The sum of an integer and twice the next integer is 41. Find the two integers.
Solve the following equations:
(a) 7x−4=10 (b) 96+x=300 (c) 4x+3=2x+11
Solve: 7y+1=29
Solve: 7n+49=497
Solve:
13x2−8x=0
Solve the following equation
b+(b+1)+(b+2)4=21
Three consecutive numbers add up to 48. What are the numbers ?
Dakarai thinks of a number. He divides it by 3 then subtracts 7. The answer is 4. What number did Dakarai think of.
Find the value of p
10p−(30p−4)=4(p+1)+9
Find the value of λ 23=λ−2λ+2
I have a total of Rs 300 in coins of denomination Rs.1,Rs.2 and Rs.5. The number of Rs.2 coins is 3 times the number of Rs.5 coins. The total number of coins is 160. How many coins of each denomination are with me
A man walks 20km, then travels a certain distance by train and then by bus as far as twice by the train. If the whole journey is of 70km, how far did he travel by train?
The cost of 535kg of salt is Rs.50310. What is the cost of 1kg salt?
A labourer is engaged for 20 days on the condition that he will revive Rs. 280 for each day he works and will be fined Rs. 60 for each day he is absent. If he receives Rs. 2540 in all, for how many days did he remain absent?
The product of two numbers is 9. If one of them is 337, then find the other number .
Solve the following equations by systematic method.
a4−3=8
Solve the following equations by systematic method.
5x−6=9
A shopkeeper has 204 eggs. He puts them in egg trays. Each tray has the capacity of 12 eggs.a) How many trays will he need?
9 years later, a girl will be 3 times an old as she was 9 years ago. How old is she now?
Fourteen added to thrice a whole number gives 56. Find the number
The teacher tells the class that the highest marks obtained by a student in her class is twice the lowest marks plus 7. The highest mark is 87. What is the lowest mark?
In a school, the monthly fee collection of 350 students in INR 3,85,000. What will be the collection fee of 58 students for a month?
How many pieces of tape 347cm long can be cut from a tape, which is 1 metre 75 cm?
Find x, if:
14!+36!=x8
Solve 7+2(a+1)−3a=5a
Solve: x3−x4=112.
Solve x+33−x−22=1. Hence find p if 1x+p=1
Manju's weight is 14 more than Anju's weight. If Anju's weight is 63 kg, find Manju's weight.
Fifteen years from now Ravi's age will be four times his present age. What is Ravi's present age?
Due to increase in salary of Ajit singh by 12 % new salary becomes Rs. 25760 . Find his previous salary .
Solve the following equations:
2x−33x+2=−23
In an election there was only two candidates. The winner polled 53% votes and won by 9600 votes. Find the total number of votes polled.
If x+20=80; find x
Solve the following equation
13x−5=32
What number is that of which the third part exceeds the fifth part by 4?
A teacher shares sweets among 8 students so that they get 6 each. How many sweets would they each have got, had there been 12 students?
The 2 consecutive integers has their sum as 67 find the numbers
The sum of 3 consecutive terms is 69 find the numbers
Set up equations and solve them to find the unknown numbers in the following cases:
When I subtracted 11 from twice a number, the result was 15.
Express the given linear equations in the form ax+by+c=0 and indicate the values of a,b and c in given case:
4=3x.
The product of two rational numbers is 15. If one of the numbers is −10, find the other.
Set up equations and solve them to find the unknown numbers in the following case:
Add 4 to eight times a number you get 60.
The sum of three prime numbers is 100. If one of them exceeds another by 36, then find larger number.
If the sum of two consecutive odd integers is 20, find the numbers
Frame and solve the equations for the following statements:
Sum of three consecutive numbers is 90. Find the numbers
Frame and solve the equations for the following statements:
Half of a certain number added to its one third gives 15. Find the number
solve:
43 +8x=14
A packet of soup makes 9 bowls of servings. How many 34 bowls of servings are possible ?
If four fifths of a number is greater than three fourths of the number by 4. Find the number.
Find a number which when multiplied by 7 and then reduced by 3 is equal to 53.
The measures of the angle of a triangle are in the ratio 2:3:4. Find the smallest angle.
The perimeter of an equilateral triangle is 16.5 cm. Find the length of its side. (in cm)
The difference between two numbers isIf one-third of the smaller number is greater than one-seventh of the larger number by 4 then what is the value of greater number?
Sum of two numbers is 95. If one exceeds the other number by 15, find the numbers.
Solve: x4−x+105+434=x−1−x−23
Solve 10=z+3 for the value of z.
The sum of two numbers is 45 and their ratio is 7:8. Find the numbers.
The sum of the page numbers on the facing pages of a book is 373. What are the page numbers?
(Hint: Let the page numbers of open pages are x and x+1)
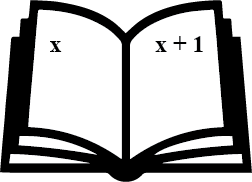
If 4 is added to a number and the sum is multiplied by 3, the result is 30. Find the number.
The product of two numbers is 223. One of the numbers is 8. Find the other.
Solve 2√xa+3√ax=ba+6ab.
Solve the following equations by transposing the terms 2a−3=5
Solve the following equations by transposing the terms
5(x+4)=35
Solve the following equations by transposing the terms
2t−5=3
Solve the following equations by transposing the terms
−3x=15
Solve the following equations by transposing the terms
14=27−x.
Solve the following equations by transposing the terms
2+y=7
Solve the following equations by transposing the terms 10−q=6
Solve the following equations.
32x+1=3x+2+√1−6.3x+32(x+1)
Solve the following equations.
(a−2) √x+4=1.
Solve the following equation:
(49)√x=(2.25)√x−4
If x+2x−2=23. Find x ?
The perimeter of a rectangular field is 35 km. If the length of the field is twice its width then find the area of the rectangle in m2
By What number 35 be multiplied to get 56?
Solve for 't'.16=4+3(t+2)
Find the net weight in grams of the contents of a breakfast cereal package if the gross weight is 1kg 200g and the packaging weighs 15 g.
Solve the equation: 4m+42m+1−34
During the launch of a new book, the publisher gave away 30 copies of the book as complimentary copies of the VIPs who were present at the launch and sold the remaining at a cost of Rs500 each. If the total collection on that day was Rs7,35,000, how many books were given out on that day as complimentary copies?
1200 soldiers in a fort had enough food for 28 days. After 4 days, some soldiers were sent to another fort and thus the food lasted for 32 more days. How many soldiers left the fort?
If the cost of 14 m of cloth isRs.18990, find the cost of 6 m of cloth.
Solve: 3n+7=25
Solve the following
x−14+x−23=416
(i) 23x=4 then x=.............. (ii) 57x=15 then x=................
verify using transposition method: 3(n−4)=30
Find the value of m: 8m−192=12
The ratio of boys and girls in a school is 8:3. If the total number of girls be 375, find the number of boys in the school.
x−20100x=13500
The teacher said, I have through of a number. If you add 7 to 3 times the number, you get 31. What number have I through of ? can your answer the teachers questions?
Solve the following equation:
i) 4=5(p−2)
ii) 4+5(p−1)=34
Solve:
(i) 3x+2=0
(ii) y−2=0
x−35−2=2x5 find he value of x.
Solve :
9x+72−(x−x−27)=−36
Solve: x−513=313.
20 labour can dig a pond in 12 days. How many days will it take 16 laborers to dig the same pond?
The sides of a triangle are in the ratio 3:2:4. If the perimeter of the triangle is 27 cm, find the length of each sides.
Solve: x3−x4=2
Solve: 15x+16x=12
Find n if 7n+5=19
Solve: p−8=23
Find the value of x

Solve: \dfrac{1}{3x} - \dfrac{3}{7x} = 8
The product of two numbers is 900. If one of the number is 90. Find the other?
If 8 tins holds 42 \dfrac{2}{3} \ l of oil. How many liters can 1 such tin hold?
A cart is running at an average speed of 3.6 km/hr. How much time will it take to go 380 metres?
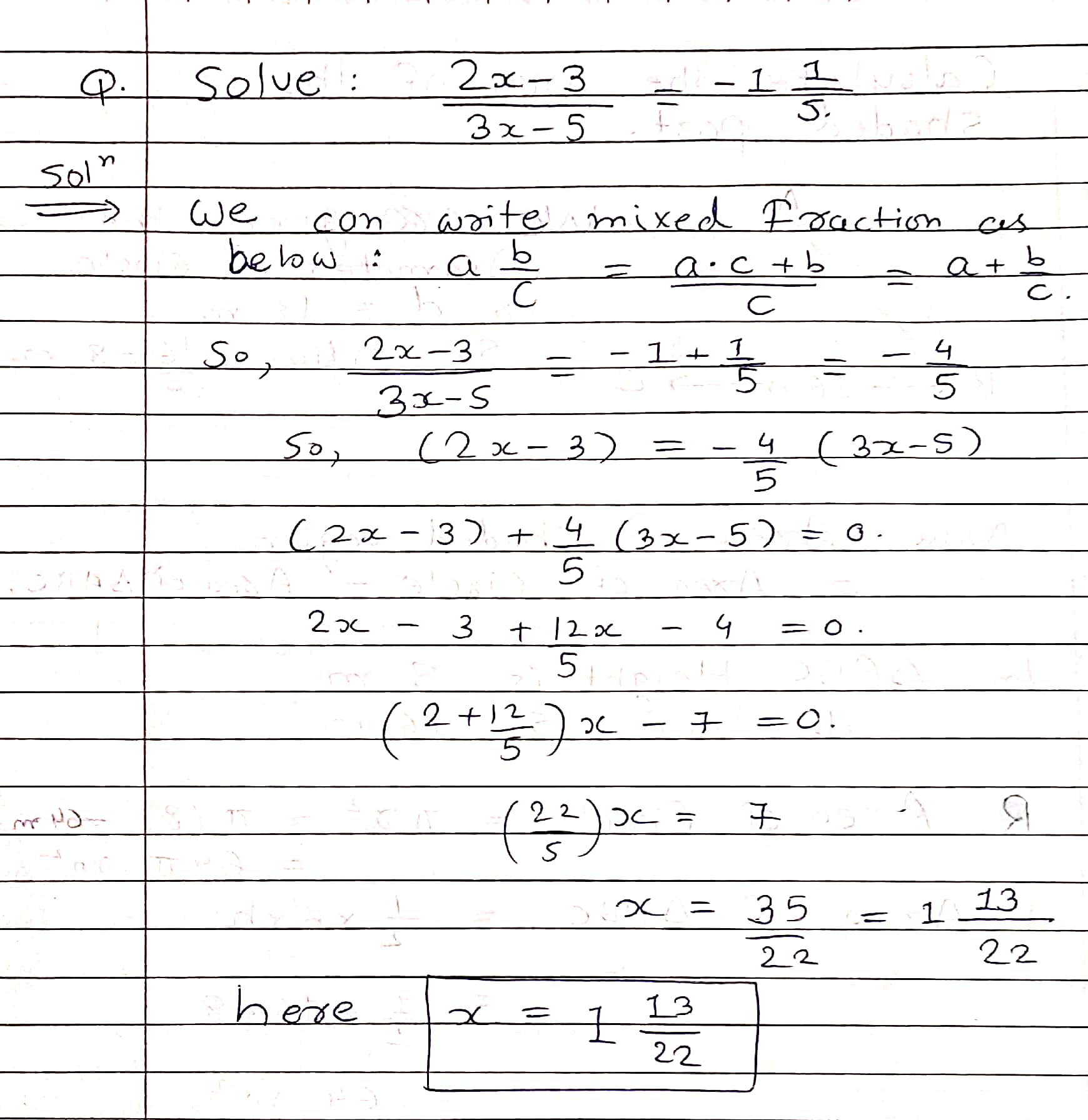
\dfrac{2x-3}{3x-5}=-1\dfrac{1}{5}.
Find 2y+\cfrac { 5 }{ 2 } =\cfrac { 37 }{ 2 }
Which number should be multiplied by \frac{{ - 3}}{5} to get \frac{{ - 1}}{5}?
One-third of a number is eight less than the number. What is the number?
\dfrac{x-5}{2}-\dfrac{x-3}{5}=\dfrac{1}{2}
If \cfrac{2}{3} is added to a number and the sum is multiplied by 2, the answer is \cfrac{16}{3}. What is the number?
Sum of three consecutive integers is '18'. Find the numbers.
Solve:
- x+3 + \left( { - 19} \right) + 15 + \left( { - 10} \right)
Irfan says that he has 7 marbles more then five times the marbles Parmit has, Irfan has 37 marbles. How many marbles does Parmit have?
A piece of wire 12\ \text{m} long is cut into two pieces, one piece is 4.29\ \text{m} long. What is the length of the other piece?
Find x :
\dfrac{1}{2}(x+5)-\dfrac{1}{3}(x-2)=4
The sum of three consecutive number is 39. Find the number.
A number consists of two digits whose sum is 13. If 27 is subtracted from the number , the digits are reversed. Find the number.
Solve:(x-2)(x+1)=(x-1)(x+3)
Find four consecutive numbers whose sum is 74
Solve:-
(4 - x)(\sqrt x + 3)
A number plus itself, plus twice itself, added to 4 times itself equals -104. What is the number?
Solve the following equations:
(a) x-5=7 (b) 3x+18=48 (c) 2x=48 (d) 5(x-1)+3=23
Solve the following equations:
\text {} \dfrac { 8 x - 3 } { 3 x } = 2\\
\text {} \dfrac { 9 x } { 7 - 6 x } = 15\\
\text {} \dfrac { 3 y + 4 } { 2 - 6 y } = \dfrac { - 2 } { 5 }\\
\text {} \dfrac { 7 y + 4 } { y + 2 } = \dfrac { - 4 } { 3 }\\
Give expressions for the following cases.
(a) 7 added to p
(b) 7 subtracted fromp
(c) p multiply by 7
(d) p divide by 7
(e) 7 subtracted from -m
(f) -p multiply by 5
(g) -5 divided by 5
(h) p multiply by -5
Solve the following:
(a) 5x+8=23 (b) 2y-4=-8 (c) 2(t+1)+!5=3 (d) -y+46=32 (e) 2x+3=3
The weight of 72 books is 9kg. What is the weight of 40 such books?
x=2+\dfrac{1}{2+\dfrac{1}{2+....\infty }}, then x is equals
If 5 is substracted 8 times from a number the remainder is 4 . What is the number ?
The product of \dfrac {-7}{12}, \dfrac {-4}{9} & \dfrac {6}{14} is equal to the sum of \dfrac {8}{10} and a number. Find the number.
Solve the following equations:
(1) x-4=3 ,
(2) 2a + 4= 0,
(3) 17 p-2=49 (4) 2m +7 =9
(5) 5(x-3)=3(x+2) (6) 13x-5=\dfrac{3}{2}
(5) 5(x-3)=3(x+2)
Solve the following equations :
3x + 2(x+2) = 20(2x-5)
Find the value of p :-
3p - 7 = 0
Set up equations and solve them to find the unknown numbers in the following cases :
(a) Add 4 to eight times a number ; you get 60 .
(b) One - fifth of a number minus 4 gives 3 .
(c) If I take three -fourths of a number and add 3 to it . I get 21 .
(d) When I substracted 11 from twice a number , the result was 15 .
(e) Munna substracts thrice the number of notebooks he has from 50 , he finds the result to be 8 .
(f) Ibenhal thinks of a number . If she adds 19 to it and divides the sum by 5 , she will get 8 .
If the sum of four consecutive integers is 266, find the integers.
If \dfrac{4}{5} of estate be worth Rs.168000, find the value of half of the estate.
Solve:
\dfrac{x+3}{7}-\dfrac{2(x-4)}{3}=1
If \dfrac{3x+5}{2x+1}=\dfrac{4}{3}, find the value of x
10 boys can dig a pitch in 12 hours. How long will 8 boys take to do it?
A quadrilateral has one angle equal to 120 ^ { \circ } and its other three angles are equal. The measure of each of the equal angles is .....................
Solve: 3n + 7 = 1
solve
(7\times 20)-8z = 0
solve
2x+1=5
Product of a number with 5 subtracted from 70 is 30. Find the number .
12 times a number divided by 10 is 6. Find the number.
solve
x\ (21-7)+7\times 2 = 70
Solve for x : \cfrac{{\sqrt {4x + 1} + \sqrt {x + 3} }}{{\sqrt {4x + 1} - \sqrt {x + 3} }} = \cfrac{4}{1}
Rohan thought of a number, doubled it and subtract 25 from it. The result was 49. Find the number.
Solve:
x+\dfrac{8}{75}+\dfrac{40}{25}=15
sum of x + 2y\,\,{\rm{and}}\,\,3x - y is
A local bus is carrying 40 passengers, some with 50 paisa tickets and the remaining with Rs\ 1.50 tickets. If the total money collected from the passengers is Rs\ 32, then find the number of passengers with 50 paisa tickets.
Solve the following equations.
(a) \dfrac{2x}{3}+\dfrac{5}{2}=4
The sum of two consecutive multiplies of 3 is 69. Find them.
Solve for x:
\frac { 8 x } { 10 } =15
Find the value of x for the following
(1)\dfrac { 2 }{ 5 } =\dfrac { 8 }{ x }
(2)\dfrac { 12 }{ x } =\dfrac { 11 }{ 8 }
(3)\dfrac { x }{ 40 } =\dfrac { 11 }{ 8 }
(1)\dfrac { 2 }{ 5 } =\dfrac { 8 }{ x }
(2)\dfrac { 12 }{ x } =\dfrac { 11 }{ 8 }
(3)\dfrac { x }{ 40 } =\dfrac { 11 }{ 8 }
\dfrac{2}{3}\left ( x+\dfrac{3}{5} \right )=\dfrac{7}{2}
Solve for x:2x-\dfrac{1}{2}=3
Solve for x:\dfrac{7x-1}{4}-\dfrac{1}{3}\left ( 2x-\dfrac{1-x}{2} \right )=\dfrac{19}{3}
Find the number when tripled and added to 2 gives 8
Solved:
x+8=11
Solve:
n+5=19
A swarm of 62 bees flies in a garden. If 3 bees land on each flower, 8 bees are left with no flowers. Find the number of flowers in the garden.
Solve the following equation :
\displaystyle \dfrac{x + 4}{2} + \dfrac{4x}{3} = 9
Solve for m: \dfrac{3m}{3}-1=1
Solve:
\dfrac{x-1}{3}-1=\dfrac{x-2}{4}
Prove that:
2x-3=7
Solve:
\dfrac{1}{10}-\dfrac{7}{x}=35
Find the value of x, if \left|\dfrac{1}{2}x-5\right|=0
The sum of two consecutive numbers is 35 find their product.
Solve: x+2\dfrac{1}{3}=5
Find the value of x, if \dfrac 8x=4.
A number is subtracted from 72. The new number is divided by 6 to give 10. Find the number.
Nine added to thrice a whole number gives 45. Find the number.
Simplify : 3x= 24
Solve:
2x-5\left[7-(x-6)+3x\right]-28=39
Sum of three consecutive number is 39. Find the numbers.
Solve:
\dfrac {x+2}{x-2}=\dfrac {7}{3}
If \left(\dfrac{8}{15}\right)^{3}-\left(\dfrac{1}{3}\right)^{3}-\left(\dfrac{1}{5}\right)^{3}=\dfrac{x}{75}, Find x.
2(x -1) = x + 2
Factorize : x^{2}-17x+72
Solve: \dfrac 1x+4=7
Solve: \frac { x - 1 } { 6 } + \frac { 3 x + 2 } { 4 } = \frac { x } { 2 } - \frac { 2 x - 1 } { 3 }
Solve for x ;5x - 2 = 8
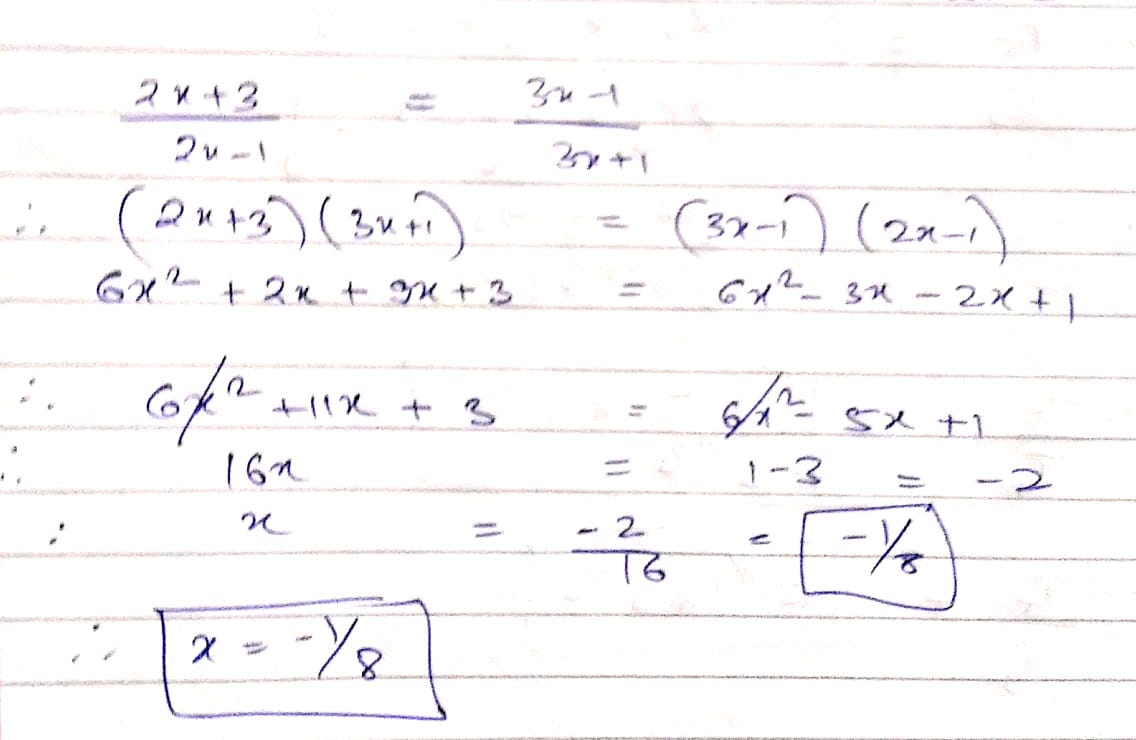
The solution of \frac{2x+3}{2x-1}=\frac{3x-1}{3x+1} is
Solve \frac{x-3}{3}=\frac{7-x}{7}
Solve \dfrac{45-x}{6}=7
Solve : 14-y-8=13
Sum of 3 consecutive numbers is 33. Find those numbers.
Complete the last column of the table:
| Sr. No. | Equation | Value | Say, whether the Equation is satisfied. (Yes / No) |
| (i) | x + 3 = 0 | x = 2 | |
| (ii) | x + 3 = 0 | x = 0 | |
| (iii) | x + 3 = 0 | x = -3 | |
| (iv) | x -7 = 1 | x = 7 | |
| (v) | x -7 = 1 | x=8 | |
| (vi) | 5x=25 | x=0 | |
| (vii) | 5x=25 | x=5 | |
| (viii) | 5x=25 | x=-5 | |
| (ix) | \dfrac{m}{3} = 2 | m=-6 | |
| (x) | \dfrac{m}{3}=2 | m=0 | |
| (xi) | \dfrac{m}{3}=2 | m=6 |
Verify that x=6 is a root of the equation 2x-4=8
The sum of two numbers is 184. If one-third of the one exceeds one-seventh of the other by 8, find the smaller number.
The sum of 2 consecutive numbers are 35 find those numbers
The sum of 3 consecutive numbers is 36 find those numbers
Solve4a-3=-27
\sqrt{2}x+\sqrt{3}x=0
Solve : 3-5(y-2)=-18
Solve:7A-16=9A
Solve the following equation: 7m+\dfrac{19}{2} =13
Solve the following equation 3(n-5)=21
Solve the following equation 34-5(p-1)=4
Write equations for the following statements:
If you take away 6 from 6 times y, you get 60.
Write equations for the following statements:
2 subtract from y is 8
Write the following equations in statement form:
\dfrac{3m}{5} =6
In an examination, a candidate had to answer 25 questions each requiring a single answer 'True' or 'False'. It was marked by giving 2 marks for each correct answer and -1 for each incorrect answer.
Use your formula to find out how many of his answer were correct, if he answered all the questions and scored a total of 2 marks.
A vessel is filled with liquid 3 parts of which are water and 5 parts syrup How much of the mixture must be drown off and replaced with water so that the mixture may be half water and half syrup?
A bag contains 50 p, 25 p and 10 p coins in the ratio 5 : 9 : 4 amounting to Rs.206. Find the number of 50p coins.
In a bundle of 154 shirts, the number of white shirts is 3 less than the number of red shirts, but 5 more than the green shirts. Then how many red shirts are there?
A rabbit hutch consists of some hares and some doves. The hutch contains 35 heads and 98 feet. Find the number of doves in the hutch
Two numbers are in the ratio 5:3. If they differ by 18, what are the numbers?
The perimeter of a rectangular swimming pool is 154\ m. Its length is 2\ m more than twice its breadth. What are the length and the breadth of the pool?
Three consecutive integers add up to 51. What are these integers
Three consecutive integers are such that when they are taken in increasing order and multiplied by 2,\ 3 and 4 respectively, they add upto 74. Find these numbers.
If you subtract \dfrac {1}{2} from a number and multiply the result by \dfrac {1}{2}, you get \dfrac {1}{8}. What is the number?
A rational number is such that when you multiply it by \dfrac {5}{2} and add \dfrac {2}{3} to the product, you get \dfrac {-7}{12}. What is the number?
Solve the following equation and check your results:
3x = 2x + 18
The organisers of an essay competition decide that winner of the competition gets a prize of Rs\ 100 and a participant who does not win gets Rs\ 25 for his participation. The total prize money distributed is Rs\ 3000. Find number of winners, if total number of participants is 63.
The number of boys and girls in a class are in ratio 7:5. The number of boys is 8 more than the number of girls. What is the total class strength?
Baichung's father is 26 years younger than Baichung's grandfather and 29 years older than Baichung. The sum of the ages of all the three is 135 years. What is the age of each of each one of them?
Lakshmi is a cashier in a bank. She has currency notes of denominations Rs. 100, Rs. 50 and Rs. 10 respectively. The ratio of number of these notes is 2 : 3 : 5. The total cash with lakshmi is Rs. 4,00,000. How many notes of each denomination does she have?
Solve the following equation and check your results:
5t - 3 = 3t - 5
Solve the equation: 5x + 9 = 5 + 3x
Solve the following equation and check your result:
8x + 4 = 3 (x - 1) + 7
Solve the following linear equation:
3(t - 3) = 5(2t + 1)
Solve the following equation and check your results:
3m = 5m - \dfrac {8}{5}
Solve the following equation and check your result:
x = \dfrac {4}{5} (x + 10)
Solve the following equation and check your results:
2y + \dfrac {5}{3} = \dfrac {26}{3} - y
Solve the equation 4z + 3 = 6 + 2z and check your results.
Solve linear equation:
x + 7 - \dfrac {8x}{3} = \dfrac {17}{6} - \dfrac {5x}{2}
Solve linear equation: \dfrac {3t - 2}{4} - \dfrac {2t + 3}{3} = \dfrac {2}{3} - t
Solve the following equation:
\dfrac {3y + 4}{2 - 6y} = \dfrac {-2}{5}
Solve the following equation:
\dfrac {9x}{7 - 6x} = 15
Simplify and solve the following linear equation:
3(5z - 7) - 2 (9z - 11) = 4(8z - 13) - 17
Solve the following equation:
\dfrac {8x - 3}{3x} = 2
The diagonal of a rectangular field is 16 metres more than the shorter side. If the longer side is 14 metres more than the shorter side, then find the lengths of the sides of the field.
The ages of Hari and Harry are in ratio 5:7. Four years from now the ratio of their ages will be 3:4. Find their present ages.
The denominator of a rational number is greater than its numerator by 8. If numerator is increased by 17 and denominator is decreased by 1, the number obtained is \dfrac {3}{2}. Find rational number.
Simplify and solve the linear equation: 0.25 (4f - 3) = 0.05 (10f - 9)
Solve the following equation: \dfrac {z}{z + 15} = \dfrac {4}{9}
The value of x that satisfies the equation \dfrac{2}{3}(5x+7)=8x is
Solve the equation 5(-3x-2)-(x+1)=-4(3x+5)+13
Find four consecutive even integers so that the sum of the first two added to twice the sum of the last two is equal to 742.
The sum of three consecutive even integers is equal to 84. Find the least number.
The sum of two numbers is 20. The larger number is four less than twice the smaller number. What are the two numbers?
Find three consecutive integers whose sum is equal to 366.
Find two consecutive integers whose sum is equal 129.
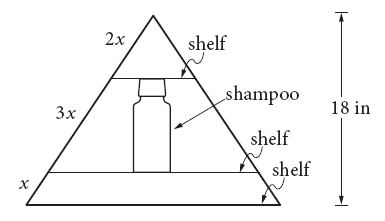
Jim has a triangular shelf system that attaches to his showerhead. The total height of the system is 18 inches, and there are three parallel shelves as shown above. What is the maximum height, in inches, of a shampoo bottle that can stand upright on the middle shelf?
If h hours and 30 minutes is equal to 450 minutes, what is the value of h?
At least how much money should a man have so that he may be left with not less than Rs.600 after giving half of his money to his wife and one-eight to each of his two sons?
The length of a rectangle is 5\ cm less than twice its breadth. If the length is decreased by 5\ cm and breadth is increased by 2 \ cm, the perimeter of the resulting rectangle will be 74\ cm. Find the length and breadth of the original rectangle.
Sristi's salary is 4 times Azar's salary. If together they earn Rs. 3,750 a month, find their individual salaries.
A saleman's commission is 5\% on all sales upto Rs. 10,000 and 4\% on all sales exceeding this. He remits Rs. 31,100 to his parent company after deducting his commission. Find the total sales.
The length of a rectangular field is twice its breadth. If the perimeter of the field is 288 \ \mathrm{m}. Find the dimensions of the field.
The sum of three consecutive even numbers is 336. Find them.
If 5 is subtracted from three times a number, the result is 16. Find the number.
Two friends A and B start a joint business with a capital 60,000. If $$A's share is twice that of B$$, how much have each invested?
In the figure, AB is a straight line. Find x.

Find two numbers such that one of them exceeds the other by 9 and their sum is 81
Find the number whose sixth part exceeds its eighth part by 3.
The sum of three consecutive multiples of 7 is 777. Find these multiples.
(Hint: Three consecutive multiples of 7 are 'x','x+7', 'x+14')
The cost of 7\dfrac{2}{3} meters of cloth is Rs. 12 \dfrac{3}{4}. Find the cost per metre.
What number should -\dfrac{33}{16} be divided by to get -\dfrac{11}{4}?
The distance around a rectangular field is 400 metres. The length of the field is 26 meters more than the breadth. Calculate the length and breadth of the field?
Which is the number when 40 is subtracted gives one-third of the original number?
If \dfrac{2}{5} of a number exceeds \dfrac{1}{7} of the same number by 36. Find the number.
Venay bought a prizza and cut it into three pieces. When he weighed the first piece he found that it was 7g lighter than the second piece and 4g heavier than the third piece. If the whole pizza weighed 300g. How much did each of the three pieces weigh?
Two equal sides of a triangle are each 5 meters less than twice the third side. If the perimeter of the triangle is 55 meters, find the length of its sides?
Solve the following equations:
9\cfrac{1}{4}=y-1\cfrac{1}{3}
Solve the following equations:
\cfrac{z}{2}+\cfrac{z}{3}-\cfrac{z}{6}=8
Solve the following equations:
\cfrac{2p}{3}-\cfrac{p}{5}=11\cfrac{2}{3}
Find three consecutive numbers such that if they are divided by 10,17,26 respectively, the sum of their quotients will be 10.
Solve the following equations:
\cfrac{8p-5}{7p+1}=\cfrac{-2}{4}
A sum Rs.500 is in the form of denominations of Rs.5 and Rs.10. If the total number of notes is 90 find the number of notes of each denomination.
(Hint: let the number of 5 rupee notes be 'x', then number of 10 rupee notes =90-x)
Solve the following equations:
\cfrac{x}{2}-\cfrac{4}{5}+\cfrac{x}{5}+\cfrac{3x}{10}=\cfrac{1}{5}
The difference between two positive integers is 36. The quotient when one integer is divided by other is 4. Find the integers.
In class of 40 pupils the number of girls is three-fifths of the number of boys. Find the number of boys in the class.
Ration is available for 100 students in a hostel for 40 days. How long will it be last, if 20 more students join in the hostel after 4 days?
The present age of Vijaya's mother is four times the present age of Vijaya. After 6 years the sum of their ages will be 62 years. Find their present ages.
Find the two consecutive positive odd integers whose sum is 32.
Solve \cfrac{7}{4}-p=11
The product of two numbers is 1296. If one number is 16 times the other, find the two numbers?
The largest of 50 measurements is 3.84 kg. If the range is 0.46 kg, find the smallest measurement.
Four times a number reduced by 5 equals 19. Find the number.
Sum of two numbers is 29 and one number exceeds another by 5. Find the numbers.
A rational number is such that when we multiply it by \dfrac {5}{2} and add \dfrac {2}{3} to the product we get \dfrac {-7}{12}. What is the number?
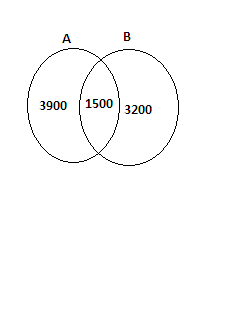
The population of town isOut of these 5400 persons read newspaper A and 4700 read newspaper B. 1500 persons read both the newspapers. Find the number of persons who do not read either of the two papers.
Two trains leave a railway station at the same time. The first train travels due west and the second train due north. The first train travels 5 km/hr faster than the second train. If after two hours, they are 50 km apart, find the average speed of each train.
Solve the following equations.
\displaystyle \frac{\sqrt{x}}{\sqrt{1 \, + \, x}} \, + \, \sqrt{\frac{1 \, + \, x}{x}} \, = \, \frac{5}{2}.
Arun is now half as old as his father. Twelve years ago the fathers age was three times as old as Arun. Find their present ages
One third of one half of one fifth of a number is 15. Find the number
What is the simplest way for a person who has only guineas to pay 10s. 6d. to another who has only half-crowns?
What is the smallest number of florins that must be given to discharge a debt of pounds 6s. 6d., if the change is to be paid in half-crowns only?
Laxmi's father is 49 years old. He is 4 years older than three time Laxmi's age. What is Laxmi's age?
Separate 178 into two parts, so that the first part is 8 less than twice the second part. Find out the two parts.
To a certain number 6 is added. The sum is multiplied by 6 and the product is divided by 13, then 7 is subtracted from the quotient. If the number obtained after these operations is 5, then the original number is
Solve the following equations, \displaystyle \sqrt{x \, + \, 8 \, + \, 2 \sqrt{x \, + \, 7}} \, + \, \sqrt{x \, + \, 1 \, - \, \sqrt{x \, + \, 7}} \, = \, 4.
Sharma family went out for a picnic and took three different types of sandwiches with them. Vegetable, grilled and cheese sandwiches were made in a ratio of 5 to 7 to 8. If a total of 120 sandwiches were made, how many grilled sandwiches were made?
The lengths of the sides of a triangle are proportional to the numbers 5, 12, and The largest side of the triangle exceeds the smallest side by 1.6 m. Find the perimeter and the area of the triangle.
If x satisfies | x \, - \, 1 | \, + \,|x \, - \, 2| \, + \,|x \, - \, 3|\,\geq \,6 then prove that all numbers x which satisfy the above relation are given by x \,\leq \,0 or x \,\geq \,4
Sachin scored twice as many runs as Rahul. Together, their runs fell two short of a double century. How many runs did each one score ?
Solve the following equations and check the solution \dfrac{30}{x-5}=5
Write down the information in the form of algebraic expression and simplify. there is a rectangular farm with length 2{ a }^{ 2 }+3{ b }^{ 2 } metre and breadth { a }^{ 2 }+{ b }^{ 2 } metre. The farmer used a square shaped plot of the farm to build a house. The side of the plot was { a }^{ 2 }-{ b }^{ 2 } metre. What is the area of the remaining part of the farm
Solve 2x+2=10
The sum of three consecutive odd numbers is 111. Find the middle number .
If (-2, 3) and (2, -2) are two solutions of a equation, find equation.
If \dfrac{3}{5} is added to twice the reciprocal of a number, the result is 1. Find the number.
Half of a herd of deer are grazing in the filed and three fourth of the remaining are playing nearby, The rest 9 are drinking water from the pond. Find the number of deer in the herd.
A shopkeeper sells mangoes in two types of boxes, one small and one large. A large box contains as many mangoes as 8 small boxes plus 4 loose mangoes If a large box has 100 mangoes, find the number of mangoes, a small box has
Five times a number when added with 14 gives 49. Find the number.
Ankitesh and his friend Jimmy together sold tickets for a charity show for Rs\ 3800. If Ankitesh sold 1\dfrac{3}{8} times as much as Jimmy sold, how much did each of them sell the tickets for?
If a labourer earn Rs. 672 per week, how much will he earn in 18 days?
The sum of three coseccutive multiples of 7 is 777. Find these multiples
Find x 296+x=-148
Solve the equation:
\dfrac{z}{3}=\dfrac{8}{6}
Find 'x'. 3x+8=5x+2.
Solve the following:
The teacher tells the class that the highest marks obtained by a student in her class is twice the lowest marks plus 7. The highest score is 87. What is the lowest score?
If 8 meters cloth cost Rs.250, find the cost of 5.8 meters of the same cloth.
Population of Sundarnagar was 234339 in the year 1991. In the year 2001 it was found to be increased by 52303. What was the population of the city in 2001
Kanchan spends \dfrac{1}{2} of her money in one shop. she spends \dfrac{1}{3} of what was left with her on rickshaw fair. At the end she has Rs.20 with her. How much did she have in the beginning
In an exam, A got 10\% marks less than B. B got 25\% more than C, C got 20\% less than D. If A got 360 marks of 500, what percent did D get
Neha went to a 'sale' to purchase some pants and skirts. When her friend asked her how many of each she had bought, she answered: "The number of skirts is two less than twice the number of pants purchased". Find the number of skirts is four less than four times the number of pants purchased. Help her friend to find how many pants and skirts Neha bought.
Find the value of k, if x = -1 and y = 2 is a solution of the equation 4x - ky = 10.
Solve the following linear equations.1) 2(3 - 2x) = 13
2) \dfrac{x}{2} = 5 + \dfrac{x}{3}
3) 7(x - 2) =2( 2x - 4)
4) 3x - \dfrac{1}{3} = 2\left( {x - \dfrac{1}{2}} \right) + 5
If the wheel of a carriage 500 cm in circumference takes \frac{1}{2} second more to complete one revolution, the rate of the carriage per hour will be reduced by 3 km/h. How fast is the carriage travelling ?
14\dfrac { 2 }{ 3 } % of a number is 176, find the number.
A fraction becomes \dfrac{4}{5} if 1 is added to both numerator and denominator of how ever s is subtracted from both numerator and denominator , the fraction becomes \dfrac{1}{2}. what is the fraction?
The perimeter of an isosceles triangle is 90m. if the length of the two equal sides is \dfrac { 3 }{ 4 } of the length of unequal side, find the dimensions of the triangle?
Find the value of p from \dfrac{2}{x} +\dfrac{3p}{45}=1, when \dfrac{x-5}{5} +\dfrac{x-3}{2}=1
The sum of two consecutive numbers is 53. Find the numbers
Ibenhal thinks of a number. If she adds 19 to it and divides the sum by 5, she will get 8.
Ram purchased a pen for Rs18.50, a book for Rs70.25 and geometry box for Rs55.95 How much money did Ram spend in all
The sum of a natural number and its reciprocal is \dfrac{5}{2} , then the number is __
Set up equations and solve them to find the unknown numbers in the following cases:
Munna subtracts thrice the number of notebooks he has from 50, he finds the result to be 8.
The organisers of an essay competition decide that a winner in the competition gets a prize of Rs 100 and a participant who does not win gets a prize of Rs 25. The total prize money distributed is Rs 3,000. Find the number of winners, if the total number of participants is 63.
Solve \dfrac {2x - (7 - 5x)}{9x - (3 + 4x)} = \dfrac {7}{6}
The length and width of a rectangle are consecutive odd integers and the perimeter is 168\ cm. Find the length and width of the rectangular.
Evaluate x = a + 4\sqrt 5 then, \sqrt x - \dfrac{1}{{\sqrt x }} = ?
A car travels 195\ km in 3 hours.
How long will take to travel 520\ km?
The cost of 4m of a particular quality of cloth is Rs 240. Tabulate the cost of 2m,7m and 9m cloth of the same type.
If 8 pens cost Rs.\ 356, what is the cost of 14 pens?
The three sides of a triangle are consecutive integers and their perimeter is 72\ cm. Find the measure of each side of the triangle.
Twice the number is 24 greater than its half. Find the number.
Solve for x in terms of a and f:\dfrac{a}{x - f} + \dfrac{f}{x - a} = 2 , \, x \neq o.
Solve the equation (y-1)=-y+5 and represent the solution on:
The cartesian plane
Solve it
3x+3y=1 \ \ (i)
3x+5y=2 \ \ (ii)
x=?\ y=?
Solve the equation (y-1)=-y+5 and represent the solution on:
the number line
The numerator of a fraction is 6 less then the denomiator. If 3 is added to the numerator, the fraction becomes equal to \dfrac {2}{3}. Find the original fraction.
Three bags contain 64.2\ kg of sugar. The second bag contain \dfrac {4}{5} of the contents of the first and third contains 45 \dfrac {1}{2}% of what there is in the second bag. How much sugar is there in each bag?
A workforce of 420 men with a contractor can finish a certain piece of work in 9 months. How many extra men mist be employ to complete the job in 7 months?
\begin{array} { l } { \dfrac { 1 } { 3 }^{rd} \text { share of Anwar's property is equal to Rs. } 15000 \text { , find the value of } \dfrac { 2 } { 5 } th\text { part of } } \\ { \text { property? } } \end{array}
Present age of Pranesh is four times the age of his son. Five years ago, the sum of their ages was 50 years. What is the present age of Pranesh?
If the weight of 12 sheets of thick paper is 40 grams, how many sheets of the same paper would weigh 16\dfrac{2}{3} kilogram?
The sum of 2 consecutive numbers is 45 find those numbers.
Alka spends 90\% of the money that she receives every month, and save Rs. 120. How much money does she receives monthly?
If y=7, find the value of y^3-3(y-2).
If 19=353\times 32.63\left[ \dfrac { 1 }{ 302.5 } -\dfrac { \left( m+1 \right) }{ m } \dfrac { 1 }{ 643 } \right] then find the value of m.
Deeepak is twice as old as his brother Vikas. If the difference of their ages be 11 years, find their present ages.
find the value of:-
200y - 51 = 49
In the year 2010 in the village there were 4000 people who were literate. Every year the number of literate people increases byHow many people will be literate in the year 2020?
Hari Babu left one third of his property to his son,one -fourth to his daughter and the remainder to his wife. If his wife share is Rs.18000,what was the worth of his total property?
A man spent 72\% of his salary and remaining were his savings. If he saved Rs\ 7,200 per month, find his month income.
By what number should \left[\left(\dfrac{-5}{2}\right)\right]^{3} be multiplied to get \left(\dfrac{-2}{5}\right)^{5}?
20\% of dolls produced in a factory were defecting , 25\% of the remaining were damaged, if 4800 dolls were left over , what was the original number of dolls?
In a 3-digit number, the hundreds digit is twice the tens digit while the units digit is thrice the tens digits. Also , the sum of its digits is 18. Find the number.
Archana bought some kilograms of wheat. She requires 12\ kg per month and she got enough wheat milled for 3 months. After that , she had 14\ kg left. How much wheat had Archana bought altogether ?
Mrs. Ghosh bought 1 kg of grapes and served each member of her family an equal amount. How many people did she serve it to if each person got \dfrac 14 of a kg?
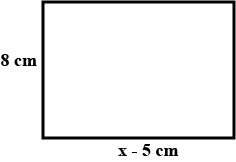
If the perimeter is 40\ \text{cm}, make an equation, and find x.
Oranges are to be transferred from larger boxes to smaller boxes. When a larger box is emptied, the oranges from it fill 3 smaller boxes and still 7 oranges are left. If the number of oranges in a small box are taken to be x, then what is the number of oranges in the larger box
Solve the following equations by systematic method.
n-\dfrac{3}{5}=8
Solve for x\dfrac { \sqrt { 4x+1 } +\sqrt { x+3 } }{ \sqrt { 4x+1 } -\sqrt { x+3 } } =\dfrac { 4 }{ 1 }
Solve the following equations by systematic method.
-7a=84
The sum of five consecutive odd numbers is 85 . Find the numbers.
Add 7 to 3 times of a number, you get 34. Find the number.
Solve for $$x:2 \left(\dfrac{2x + 3}{x - 3}\right) - 25 \left(\dfrac{x - 3}{2x + 3}\right) = 5$$.
2 \left(\dfrac{2x + 3}{x - 3}\right) - 25 \left(\dfrac{x - 3}{2x + 3}\right) = 5$$.
The difference between the square of two consecutive numbers is 31. Find the numbers.
Solve:
\dfrac {x+2}{2}=13
A bird flies 1 kilometer in one minute. Can you express the distance covered by the bird in terms of its flying time in minutes? [use t for flying time in minutes]
Radha takes some flowers in a basket and visit three temples one by one. At each temple, she offers one half of the flowers from the basket. If she is left with 3 flowers at the end, find the number of flowers she had in the beginning.
Purse contains Rs. 250 in the domination of Rs. 10 and Rs. 50. If the no. of Rs. 10 notes is one more than that of 50 notes.
The sum of two consecutive odd integers is 44. Find the two integers.
The sum of two consecutive integers is 39. Find the two integers.
Find the value of x : - 2 = - x + 12
Find three consecutive even numbers whose sum is 66.
Find the distance covered by the wheel of a car in 500 revolutions if the diameter of the wheel is 77 cm.
If sum of two number is 17 and sum of their reciprocal is \dfrac{17}{62} write the representation of given situation
If 11 is subtracted from 4 times a number, the result is 89. Find the number.
Solve the following riddle:
I am a number, Tell my identity!
Take me seven times over, And add a fifty!
To reach a triple century, You still need forty!
The difference between 2 no. is6 times the smaller number plus the smaller number is 77.
Solve:
\dfrac { 2 } { 3 } \left( x + \dfrac { 3 } { 5 } \right) = \dfrac { 7 } { 2 }
A chemist needs to strengten as 15% alcoholic solution of one of 32% alcoholic. How much pure alcohol should be added to 800\ mL of 15% solution?
The sum of ages of Priyanka and Deepika is 34 years. Priyanka is elder to Deepika by 6 years. Then find Priyanka's present age.
A train 320\ m long is travelling at a uniform speed of 66\ km/hr. If it passes a platform is 42 seconds, find the length of the platform .
4\dfrac{1}{2} z + 4\dfrac{1}{3} z = 100 find z.
Find the value of x is \dfrac{1-x}{6}+\dfrac{2x}{3}-\dfrac{1-\dfrac{3}{7x}}{4}=2\dfrac{1}{6}
In a garage, there are a total of 40 cars and motorbikes. The total number of tyres of these 40 vehicles is 140 , Find the number of cars.
solve x: \dfrac { 1 }{ a+b+x } =\dfrac { 1 }{ a } +\dfrac { 1 }{ b } +\dfrac { 1 }{ x }
Six years ago a father was seven times as old as his son.After six years he will be three times as old as his son.Determine their present ages.
The average of 3 angles of a quadrilateral which are in the ratio of 2:4:5 is 77^{o}. Find all the angles of the quadrilateral.
A photograph of a bacteria enlarged 50,000 times attains a length of 5 cm as shown in the diagram. What is the actual length of the bacteria? If the photograph is enlarged 20,000 times only, what would be its enlarged length in cm?
A natural number, when increased by 12, becomes equal to 160 times its reciprocal. Find the number.
Solve the following equations
5x-2=18
Sameer changed \text{Rs.}\ 200 into \text{Rs.}\ 5, \text{Rs.}\ 20 and \text{Rs.}\ 50 notes. If the number of \text{Rs.}\ 5 notes was double the number of \text{Rs.}\ 20 notes, and the \text{Rs.}\ 50 notes were one-fifth the \text{Rs.}\ 20 notes, how many notes of each denomination did he get?
Some tickets of Rs.200 and some of Rs.100, of a drama in a theater were sold. The number of tickets of Rs.200 sold was 20 more than the number of tickets of Rs.100. The total amount received by the theatre by sale of tickets was Rs.37000. Find the number of Rs.100 tickets sold.
A man buys 12.60 kg of one type of rice for Rs 30 per kg and 15 kg of another type of rice for Rs 67.90 per kg. If he mixes them and makes packets, each containing 3,450 g of rice, how many such packets of rice are there and what is the cost of each packet ?
If \dfrac {1}{2} is subtracted from a number, and the difference is multiplied by 4, the result obtained is 5. Find the number.
When Raju multiplies a certain number by 17 and adds 40 to the product, he gets 225.Find the number?
Solve:\dfrac { x+2 }{ 6 } -\left( \dfrac { 11-x }{ 3 } -\dfrac { 1 }{ 2 } \right) =\dfrac { 3x-4 }{ 12 }
The price of 2 tables and 3 chairs is Rs.340. A table costs Rs.20 more than a chair. What is the price of a table ?
Find your weight if your friend's weight is 20% more than you and your friend's weight is 48\ kg.
By what number should { (15) }^{ -1 } be multiplied so that the product may be equal to { (-5) }^{ -1 }?
Solve:\dfrac{2x-3}{2}-\dfrac{x+1}{3}=\dfrac{3x-8}{4}
Solve:5x-3=3x+5
Solve:3x+\dfrac{1}{2}=\dfrac{3}{8}+\dfrac{x}{4}
A father is now three times as old as his son. Five years back, he was four times as old as his son. The present age of the son(in years) is
Two numbers are in the ratio 5:3. If their difference is 18 then find the numbers.
Solve :- 8x+3 = 27 +2x
Sum of two integers is 36. If one of the number be the half of the other then find the numbers.
Solve:
\dfrac{3a-2}{7}-\dfrac{a-2}{4}=2
Solve and check the solution in the following equation.
1. x+7=9
2.\dfrac{x}{4}=25
3.9y=-135
4.15-x=4
5.3(x-3)=15
6.7y+3=9
The digit of tens place of a 2 digit is 3 times the digit at a unit place of the digit are reversed, the new number will be 36 less the original number. Find the original number.
2x-3=55
The sum of 3 consecutive numbers is 36 find the numbers.
If the speed of a car is increased by 10 km per hr, it takes 18 minutes less to cover a distance of 36 km. find the speed of the car.
Solve each of the following system of equations in R.
x+\dfrac { 1 }{ 3 } >\dfrac { 8 }{ 3 }
How are the points (-1,-2), (-2, 3) and (1, 4) situated with respect to the circle {x}^{2}+{y}^{2}-2x–5y+3=0
Solve each of the following of equations in R.
\dfrac { 3x-4 }{ 2 } \le \dfrac { 5 }{ 12 }
The sum of two angles is 90 degrees. The angles are in the ratio 2:3. Find the measure of each angle.
In a circket match,Sachin makes his score thrice the Sehwags's score. Both of them together make a total score of 200 runs.
A number is 25 more than its \left(\dfrac{5}{6}\right)^{th} part. Find the number.
If the weight of the boy holding flower pot is 47.5kgs Find the weight of the cat

Solve:
\dfrac { 1 }{ x } +\dfrac { 1 }{ x+1 } -\dfrac { 2 }{ x-1 } =0
In \triangle PQR if \angle P = 45^{\circ } , and \angle Q = 75^{\circ} , then the measure of \angle R = is ..............
2 . The exponential form of 225 is ...........
3 . The algebraic expression whit three terms is called .......
4 . 65 % in the simple interest from is ......
5 . the area of parallelogram is 320 sq. c m and base is 4 0 c m , its height is ..........
Mr. Grover is three times as old as his son and the sum of their ages is 48. How old is each?
Andy has twice as many marbles as pandy, and sandy has half as many has Andy and pandy put together. If Andy has 75 marbles more than Sandy. How many does each of them have
solve for x : 3\left( \dfrac { 3x-1 }{ 2x+3 } \right) -2\left( \dfrac { 2x+3 }{ 3x-1 } \right) =5;x\neq \dfrac { 1 }{ 3 } ,-\dfrac { 3 }{ 2 }
It the cinema hall, 500 tickets was sold. The total sale was Rs. 12,The tickets were of denomination of Rs. 15 and Rs.How many of each denomination were sold
An odd number of stones are placed at equal intervals of 10 meters in a row on the field.All stones are be gathered at the place of the stone are be gathered at the place of the stone in the middle.If a man can carry only one stone at a time and starts from one of the end stones,he has to cover 3km for the work.How many stones were there?
The sum of three consecutive natural numbers isFind the numbers.
Six more than four times a number is four less than five times the number. Find the number.
The sum of the squares of two consecutive odd numbers is 394. Find the numbers.
Solve 3x-14=4
Ranjana's mother gave her Rs. 245 for buying New Year cards. If she got some 10 -rupee cards, \dfrac { 2 } { 3 } as many 5 rupee cards, and \dfrac { 1 } { 5 } as many 15 -rupee cards, how many of each kind did she buy?
Shalini's weight is 3500 g greater than her younger brother. The weight of her brother is 35 kg. Find shalin's weight.
Solve 12q-5=25
\frac{x}{2.8}=\frac{1}{1.4}
\displaystyle \frac{x+6}{4}-\frac{5x-4}{8}+\frac{x-3}{5}=0
4w=30
c-2=-5
The temperature at 07:00 is -3^{\circ}C. This temperature is 11^{\circ}C higher than the temperature at 01:00. Find the temperature at 01: 00.
Teacher tells the class that the highest score obtained by a student are 9 more than thrice the lowest score. If the highest score isWhat is the lowest score?
Express the given linear equation in the form ax + by + c = 0 and indicate the values of a, b and c in given case:
y - 5 = 0.
The boys and the girls in a school are in the ratio of 7:4. If the total strength of the school is 550, then find the number of boys and girls.
Sum of two numbers is 81. One is twice the other.
If smaller number is x, the other number is _______.
The sum of two numbers is 60 and their difference is 30.
The difference of numbers in term of x is ______.
Find the value of x in the following.
\sqrt[3]{3x-2}=4.
Solve for x: \sqrt{x + 1} - \sqrt{x - 1} = 1
Sum of two numbers is 81. One is twice the other.
The solution of the equation is _______.
On simplification \dfrac{3x+3}{3} = __________ .
In a test Abha gets twice the marks as that of Palak. Two times Abha's marks and three times Palak's marks make 280.
Marks obtained by Abha are ______.
Sum of the digits of a two-digit number is 11. The given number is less than the number obtained by interchanging the digits by 9. Find the number.
What number divided by 520 gives the same quotient as 85 divided by 0.625?
Solve: \dfrac{3x-8}{2x}=1
In a bag, there are 5 and 2 rupee coins. If they are equal in number and their worth is Rs 70, then
There are _______ 5 rupee coins and _______ 2 rupee coins.
Solve: \frac{5(1-x)+3(1+x)}{1-2x}=8.
Sum of two numbers is 81. One is twice the other.
The numbers are ______ and ______.
For what value of c, the linear equation 2x+ cy = 8 has equal values of x and y for its solution.
The sum of two consecutive multiples of 10 is 210. Find the smaller multiple..
Two numbers are in the ratio 5 : 6 . If 8 is subtracted from each of the numbers , the ratio becomes 4 : 5 find the numbers.
Fill the blanks to make the statement true:
After 18 years, Swarnim will be 4 times as old as he is now. His present age is ________.
Fill the blanks to make the statement true:
The share of A when Rs. 25 are divided between A and B so that A get Rs 8 more than B is ________.
Fill the blanks to make the statement true:
When a number is divided by 8, the result is -3. The number is _______.
Orange are to be transferred from larger boxes into smaller boxes. When a large box is emptied, the oranges from it fill two smaller boxes and still, 10 oranges remain outside, if the number of oranges in a small box are taken to be x, what is the number of oranges in the larger box?
If the length of the sides of a triangle is in the ratio 3:4:5 and its perimeter is 48 cm, find its sides.
The length of a rectangle is 5 \,cm less than twice its breadth . If the length is decreased by 3 \,cm and breadth increased by 2 \,cm , the perimeter of the resulting rectangle is 72 \,cm . Find the area of the original rectangle.
The differences between an integer x and (-9) is 6. Find all possible values of x.
A rectangle is 10 \,cm long and 8 \,cm wide . When each side of the rectangle is increased by x \,cm , its perimeter is doubled . Find the equation in x and hence find the area of the new rectangle.
Solve the following equation:
\dfrac{(2x - 3)}{(2x - 1)} = \dfrac{(3x - 1)}{(3x + 1)}
What is the condition for an equation of the form ax^2 + bx + c = 0 to become a linear equation.
In a competitive examination , 1 mark is awarded for each correct answer while 1/2 marks is deducted for every wrong answer . jayanti answered 120 question and got 90 marks . How many question did she answer correctly Rs .
A box contains x\ 50 paise coins, 2x\ one-rupee coins and 5x two-rupee coins. If the total value of these coins is Rs. 25 what is the value of x?
A family is using Liquefied petroleum gas (LPG) of weight 14.2 kg for consumption. (full weight 29.5 kg includes the empty cylinders tare weight of 15.3 kg.). If it is use with constant rate then it last for 24 days. Then the new cylinder is replaced
Find the equation relating the quantity of gas in the cylinder to the days
In a study of bat migration habits, 240 male bats and 160 female bats have been tagged. If 100 more female bats are tagged, how many more male bats must be tagged so that \displaystyle \frac{3}{5} of the total number of bats in the study are male?
The sum of 3 consecutive multiples of 8 is 888. Find the multiples
If f(x)=4x^3-6x^2\cos 2a+3x\cdot \sin 2a\cdot \sin 6a+\sqrt {\log(2a-a^2)}, then show that f'\left (\dfrac {1}{2}\right ) > 0
According to a plan, a team of woodcutters had to cut 216 m^3 of wood in several days. The. first three days, the team fulfilled the daily assignment, and then it cut 8 m^3 of wood over and above the plan every day. Therefore, a day before the planned date they cut 232 m^3 of wood. How many cubic metres of wood a day did the team have to cut according to the plan?
Some ticket of 200 and some of 100, of a drama in theatre were sold. The number of tickets of 200 sold was 20 more than the number of tickets of The total amount received by the theatre by sale of tickets was Find the number of 100 tickets sold.
In a scout camp, there is a food provision for 300 cadets for 42 days. If 50 more persons join the camp for have days will the provision last?
The teacher tells the class that the highest marks obtained by a student in her class is twice the lowest marks plus 7. The highest score is 87. What is the lowest score?
Rs\ 6400 were divided equally among x persons. Had this money been divided equally among (x+14) persons, each would have got Rs\ 28 less. Find the value of x
Class 8 Maths Extra Questions
- Algebraic Expressions And Identities Extra Questions
- Comparing Quantities Extra Questions
- Cubes And Cube Roots Extra Questions
- Data Handling Extra Questions
- Direct And Inverse Proportions Extra Questions
- Exponents And Powers Extra Questions
- Factorisation Extra Questions
- Introduction To Graphs Extra Questions
- Linear Equations In One Variable Extra Questions
- Mensuration Extra Questions
- Playing With Numbers Extra Questions
- Practical Geometry Extra Questions
- Rational Numbers Extra Questions
- Squares And Square Roots Extra Questions
- Understanding Quadrilaterals Extra Questions
- Visualising Solid Shapes Extra Questions