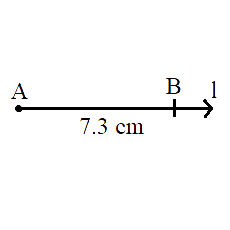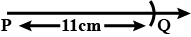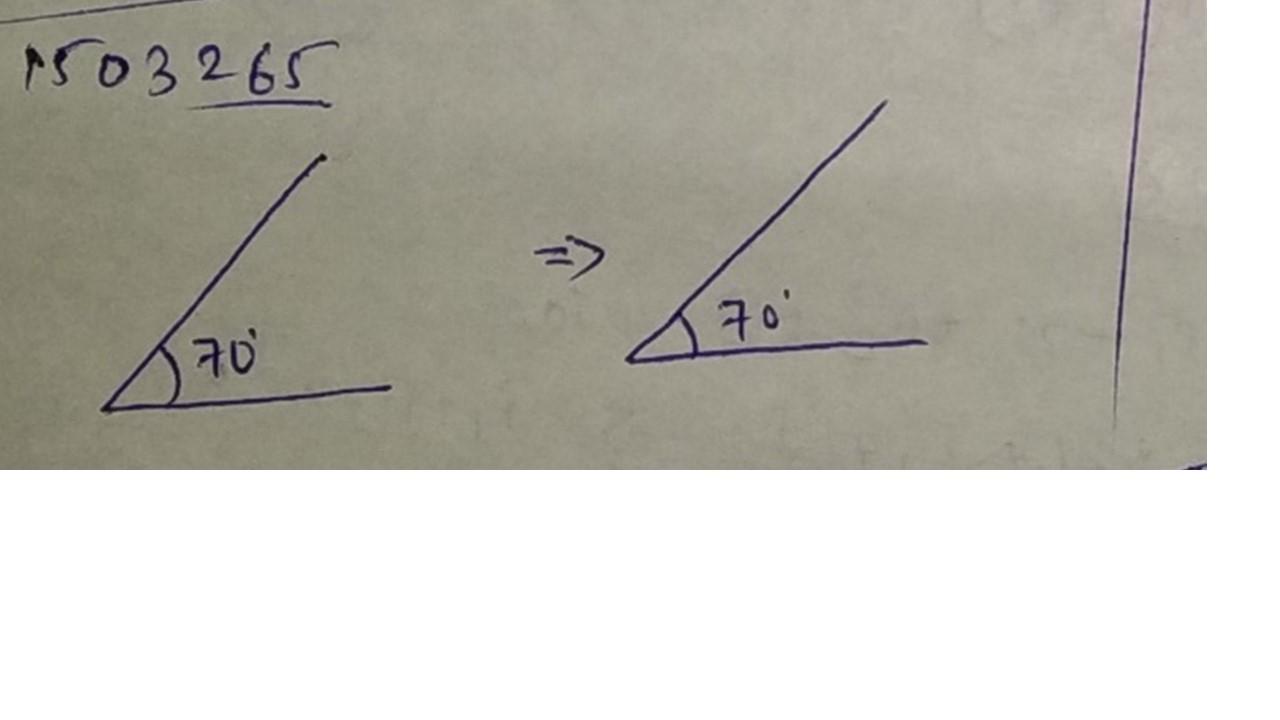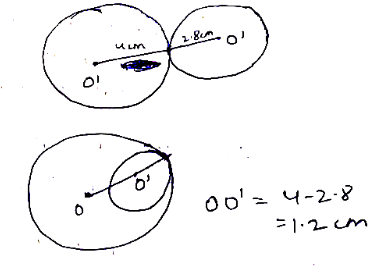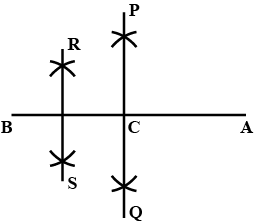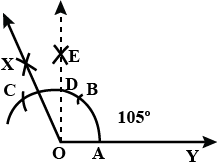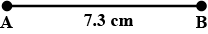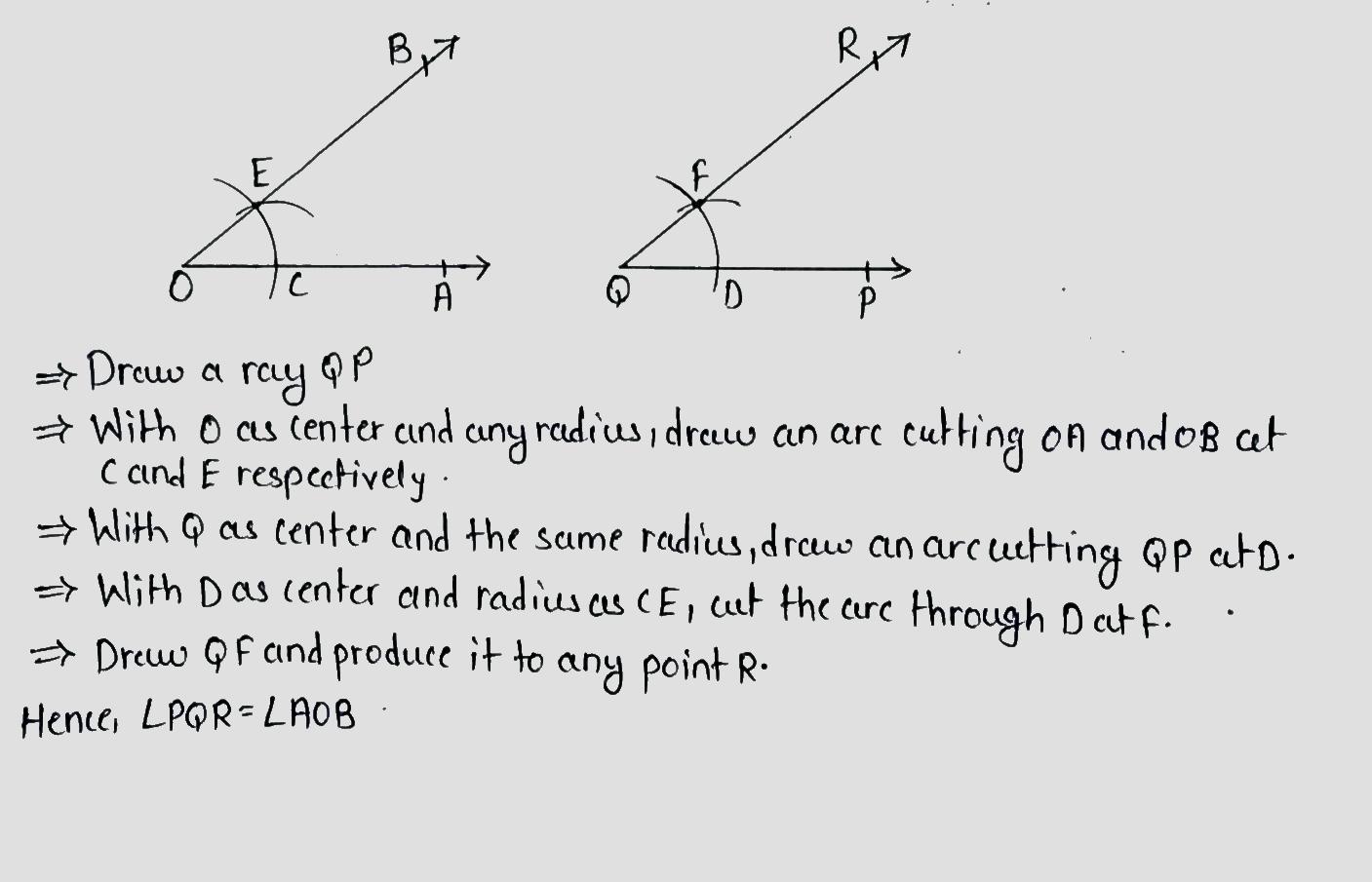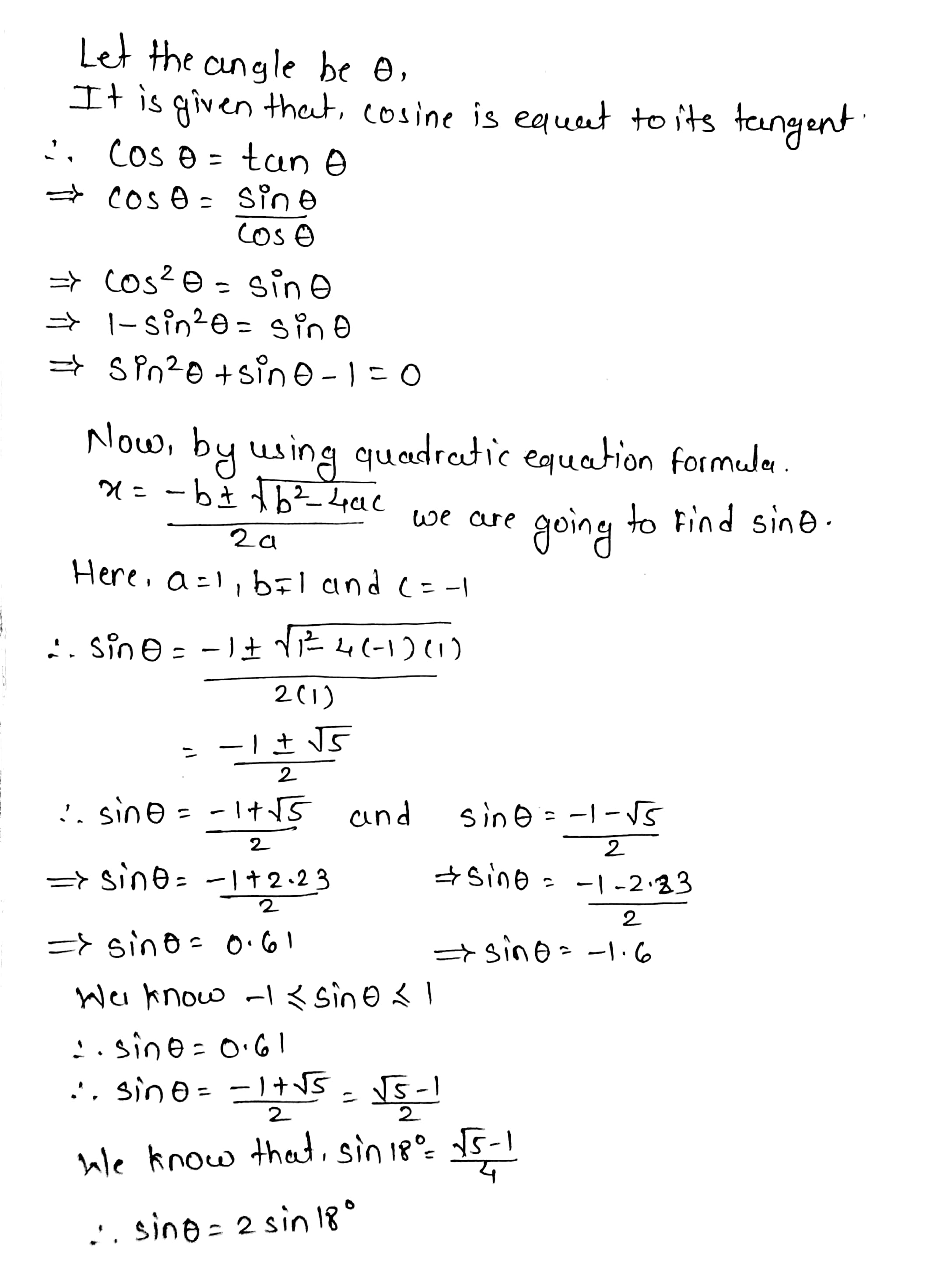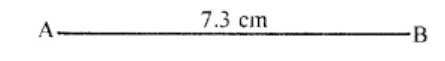Practical Geometry - Class 6 Maths - Extra Questions
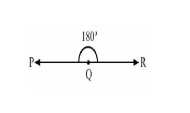
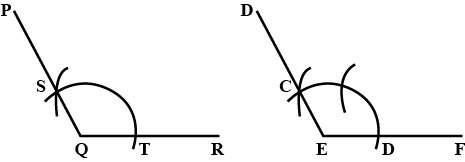
Given a line segment SR of 2.5 cm. Construct a line segment PT perpendicular to SR.
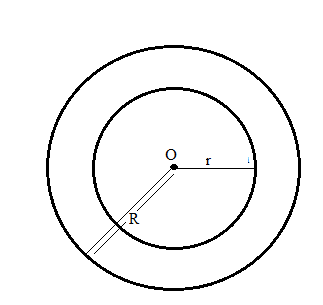
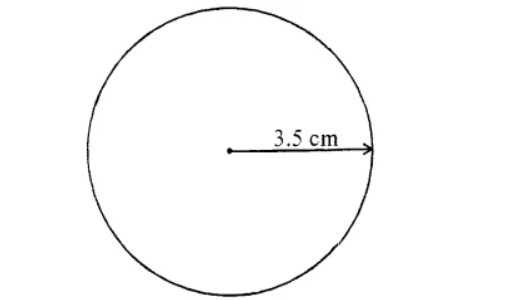
Draw concentric circles having radii 3.5 cm and 5.5 cm . Find out the width of circular ring.
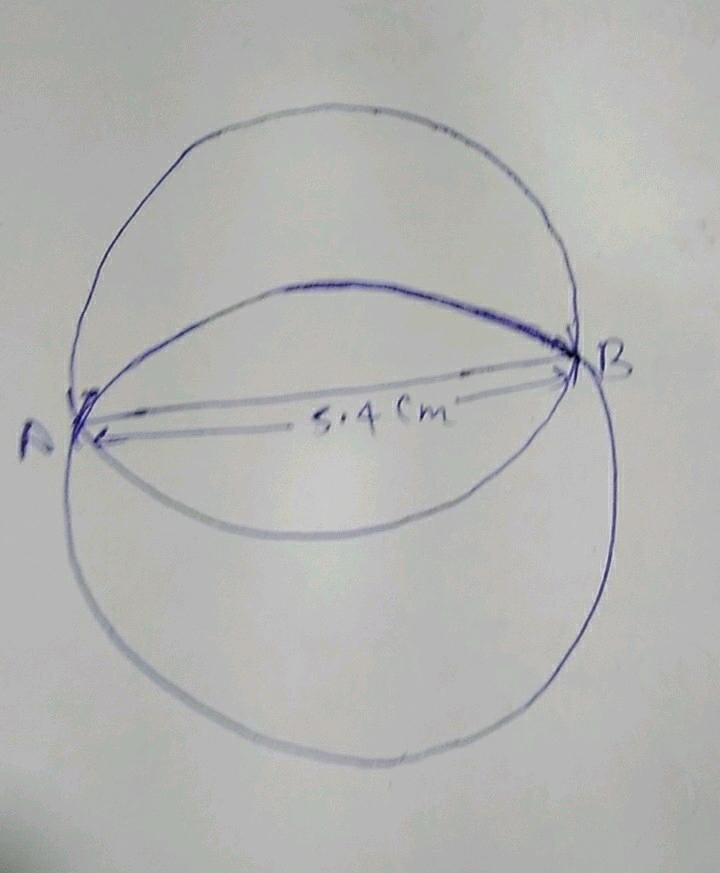
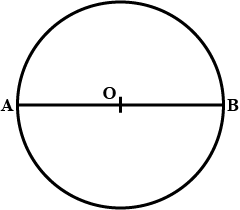
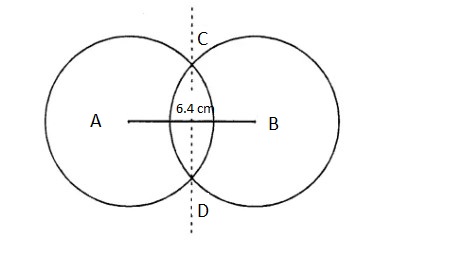
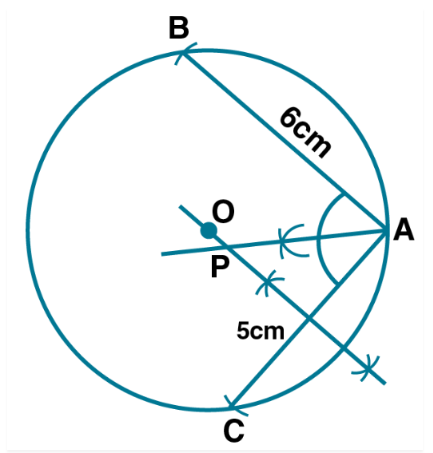
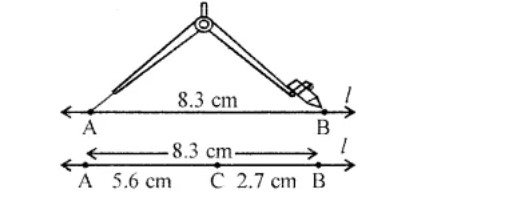
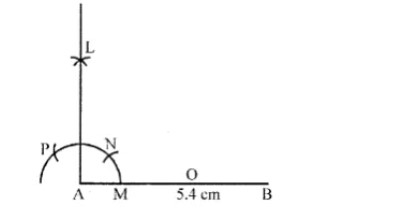
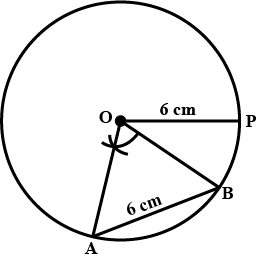
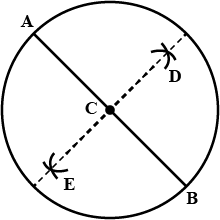
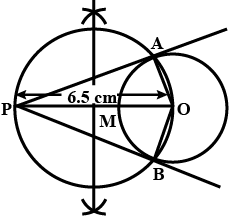
Draw two circles passing through A, B where AB = 5.4 cm
Draw concentric circle for the radii measuring 5.3 cm and 7 cm. Find out the width of circular ring.
Draw concentric circle for the radii measuring 5 cm and 6.5 cm. Find out the width of circular ring.
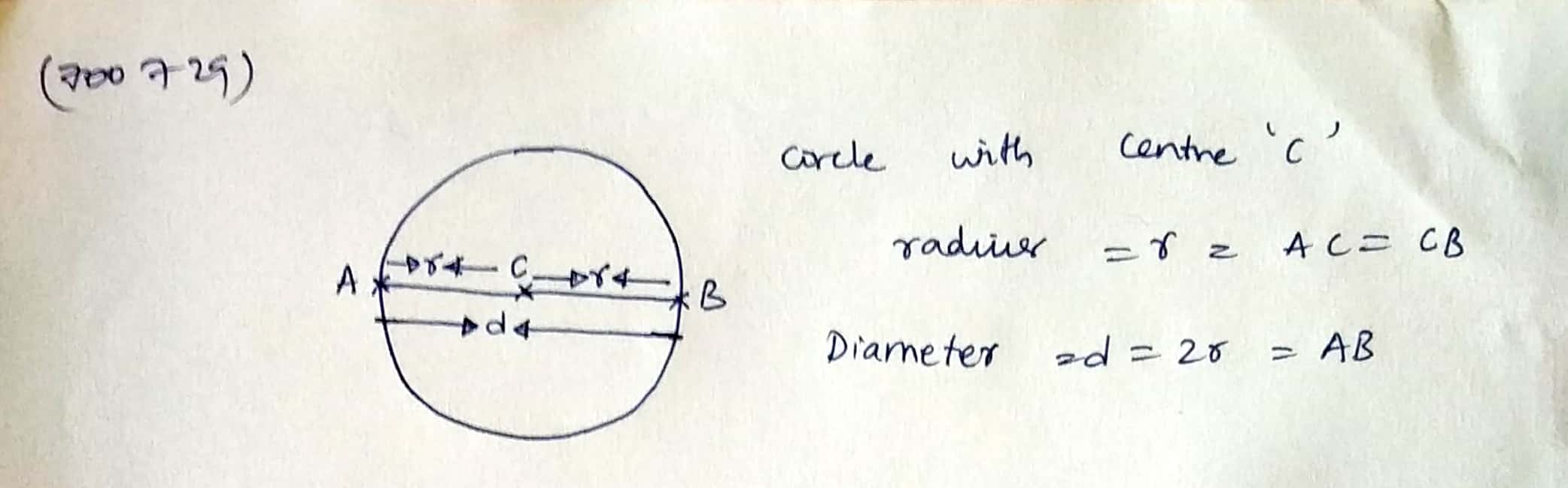
Draw a circle and name its centre, a radius, a diameter and arc.
Draw a circle 4.5 cm radius & divide the circle in 8 equal parts.
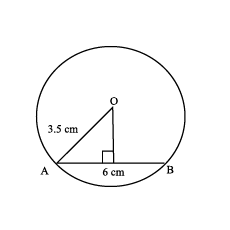
Draw a circle of radius 3.5 cm and construct a chord of length 6 cm in it. Measure and write the distance between the centre and the chord.
Draw a line segment of length 7.2 cm and divide it in the ratio 5:3. Measure the two parts.
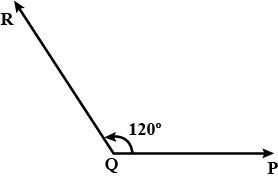
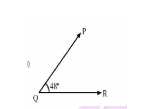
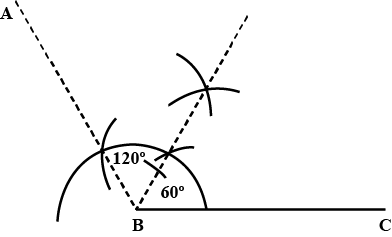
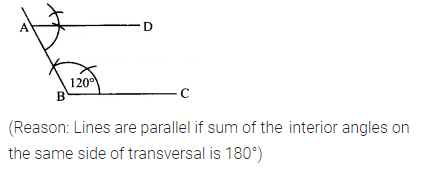
Draw an ∠PQR of 120o.
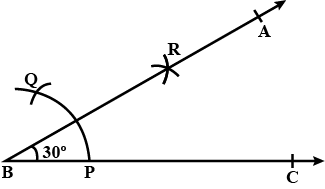
Draw an ∠ABC of 300.
Draw a line segment of length 7.3 cm using a ruler.
Sketch proper figure and answer the questions :
If A-B-C and ℓ(AC)=11,ℓ(BC)=6.5thenℓ(AB) = ?
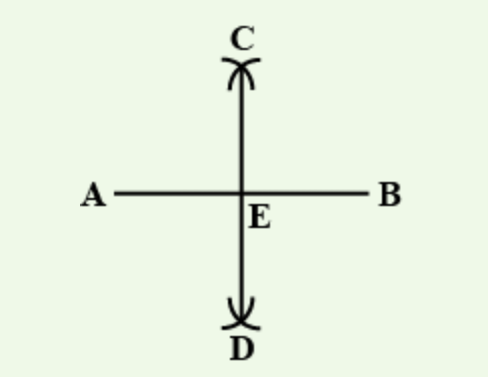
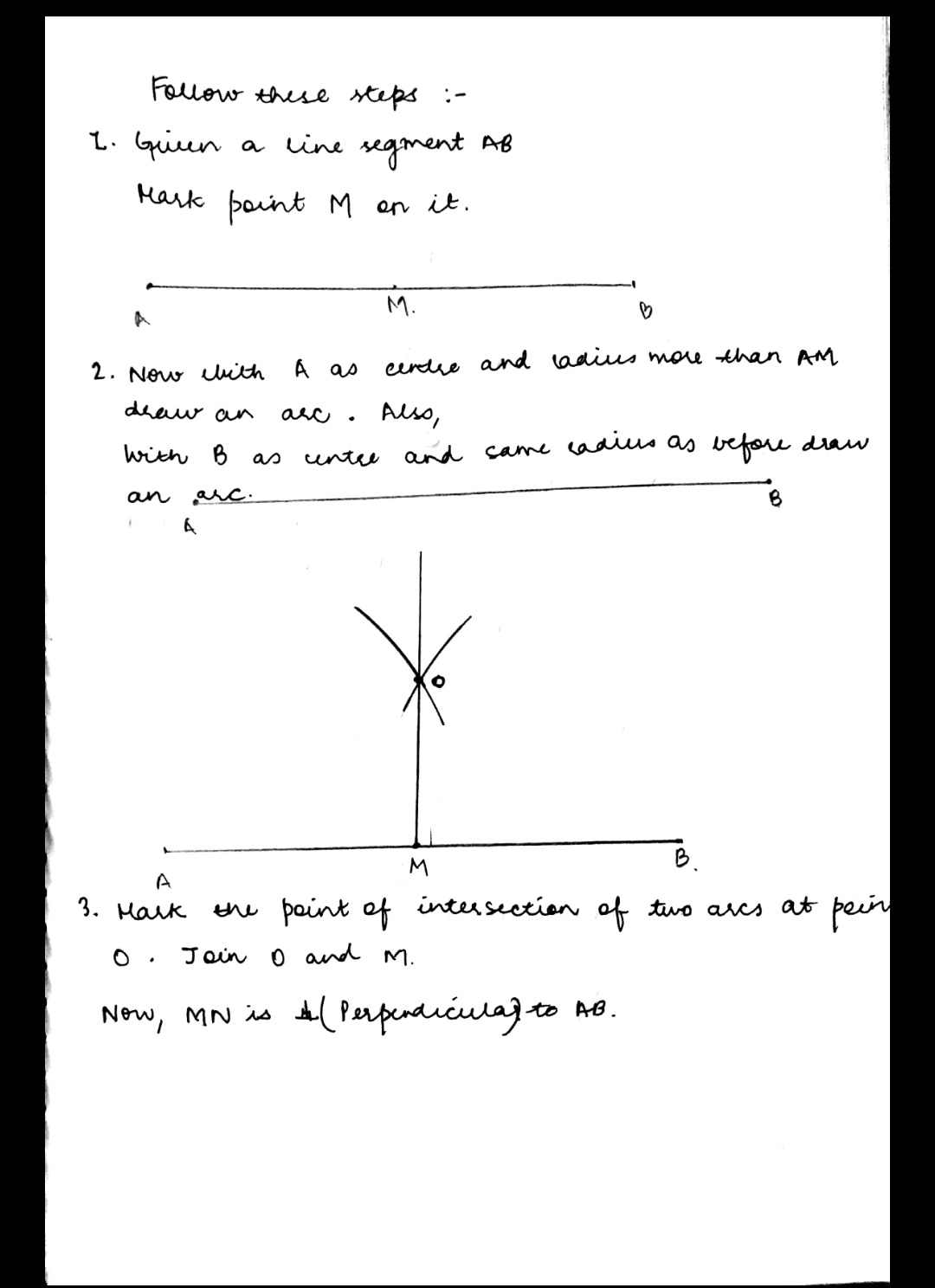
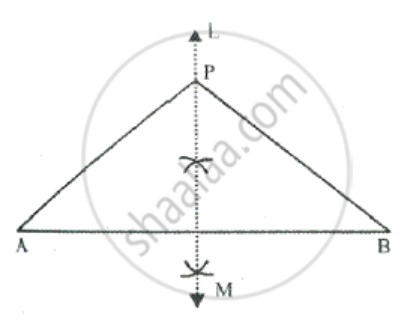
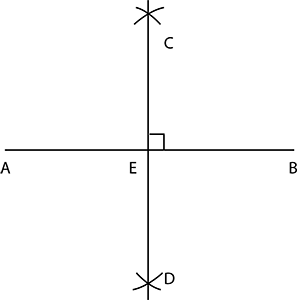
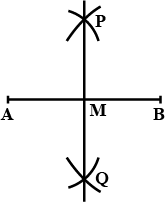
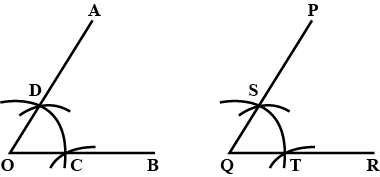
Draw any line segments ¯AB. Mark any point M on it. Through M, draw a perpendicular to ¯AB.
(use ruler and compasses)
Draw a line segment PQ=11 cm.
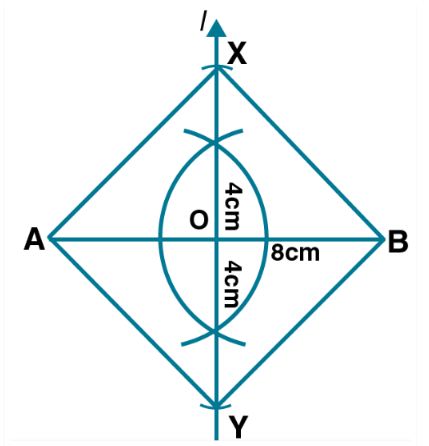
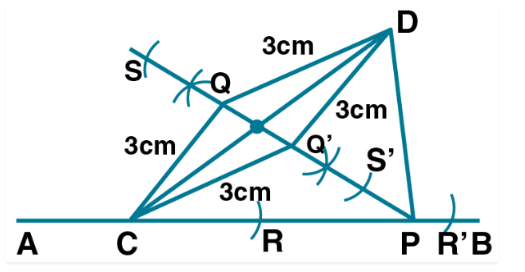
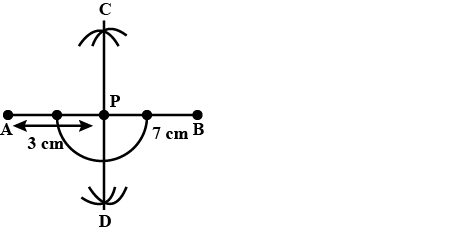
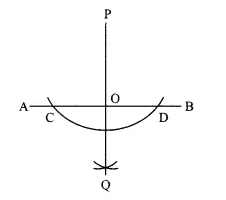
Draw a line AB=6 cm. Draw the locus of all the points which are equidistant from A and B.
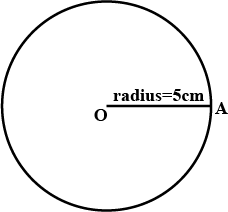
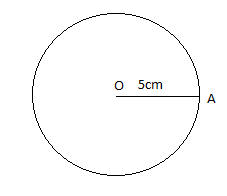
Draw a circle using a compass with the radius 5 cm.
Draw a circle and mark-
(a) its centre (b) a radius (c) a segment (d) its largest chord (e) a sector (f) an arc
Draw a line segment PQ=8cm. Take a point R on it such that l(PR):l(RQ)=3:2 find PR and RQ.
Three equal circles of radius 1 cm touches each other. What is smallest radius of circle touching all three.
Use protector to draw the angle of the following measure and bisect the angle 90o.
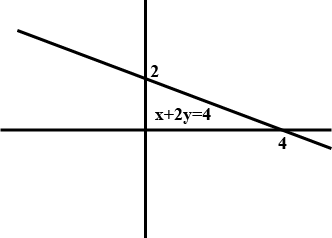
Draw the graph of x+2y=4
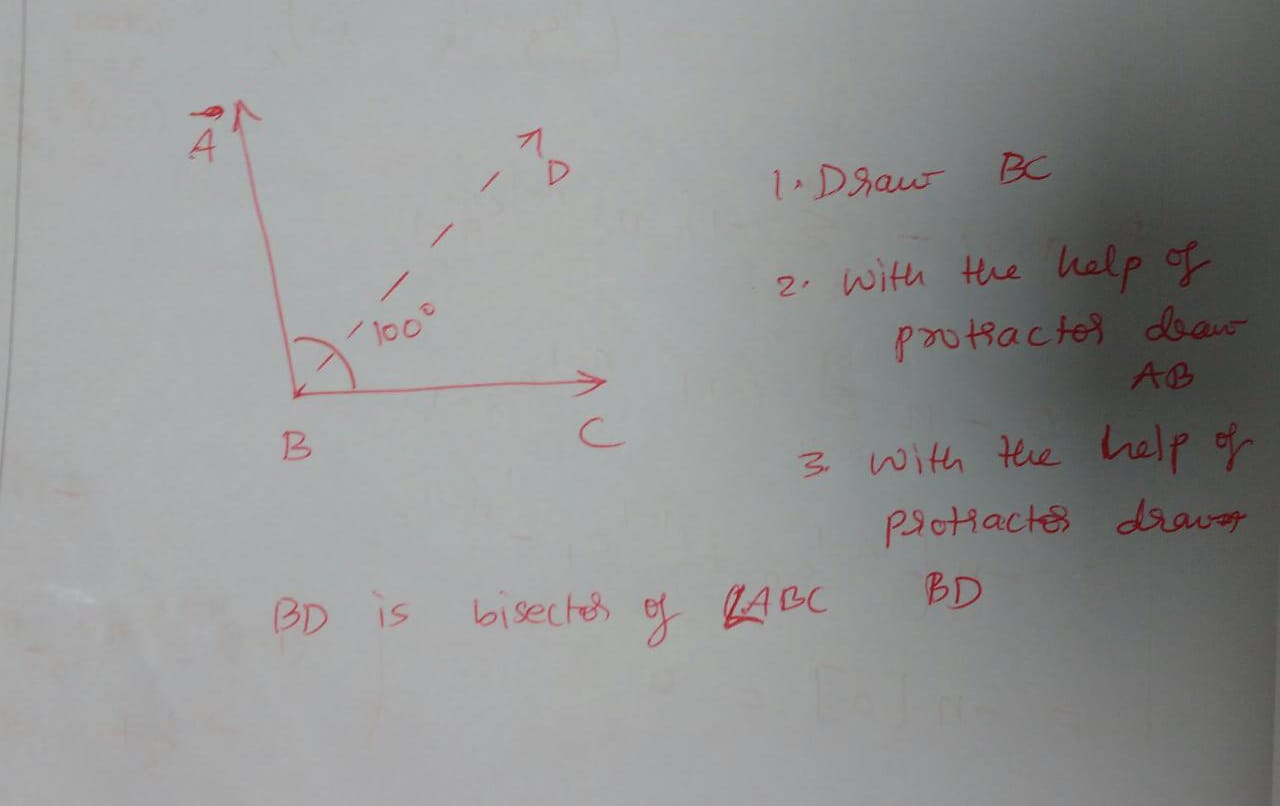
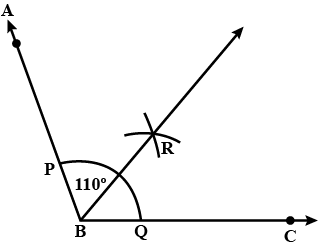
Draw a ∠ABC of measure 1000 . Bisect it.
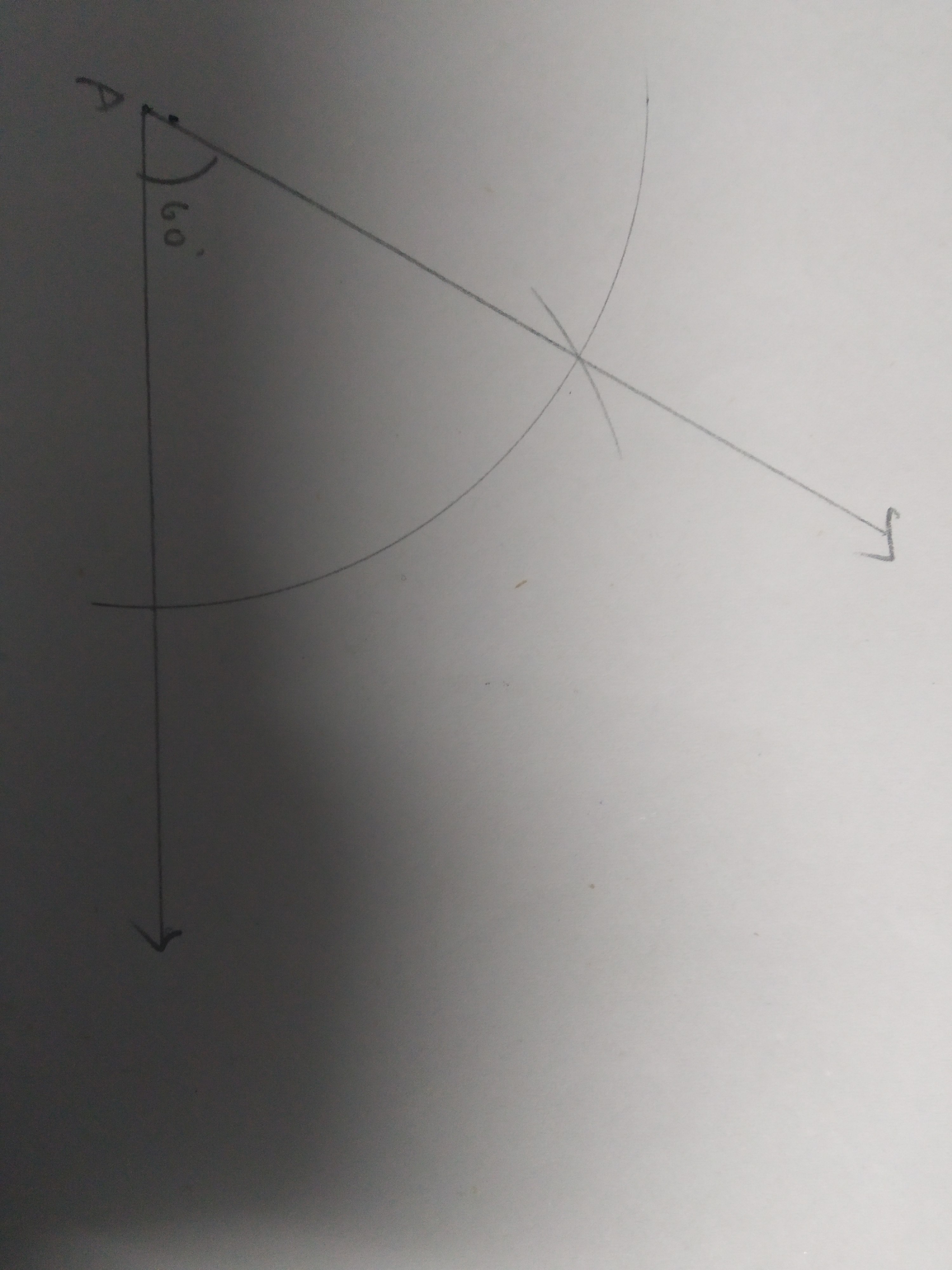
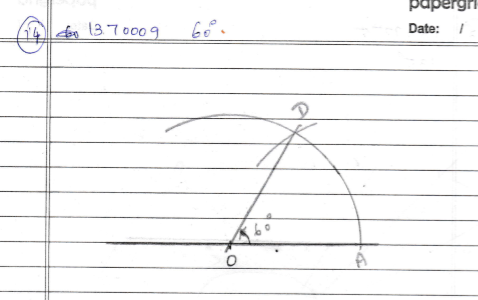
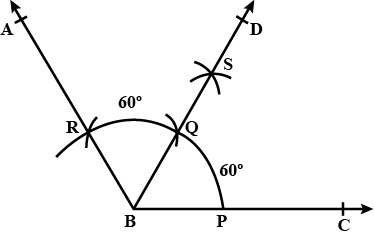
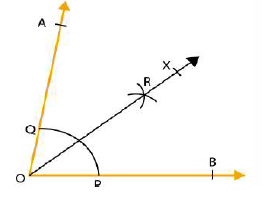
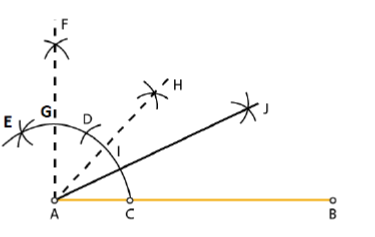
Draw an angle of 60∘ and name it ∠A. Using compasses and ruler.
Draw an angle of 70o. Make a copy of it using only a straight edge and compasses.
Draw ¯AB of length 7.3 cm and find its axis of symmetry.
Draw a line segment of length 4.9 cm and divide it in the ratio 3:4 , measure the two parts.
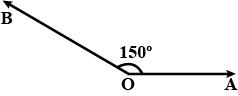
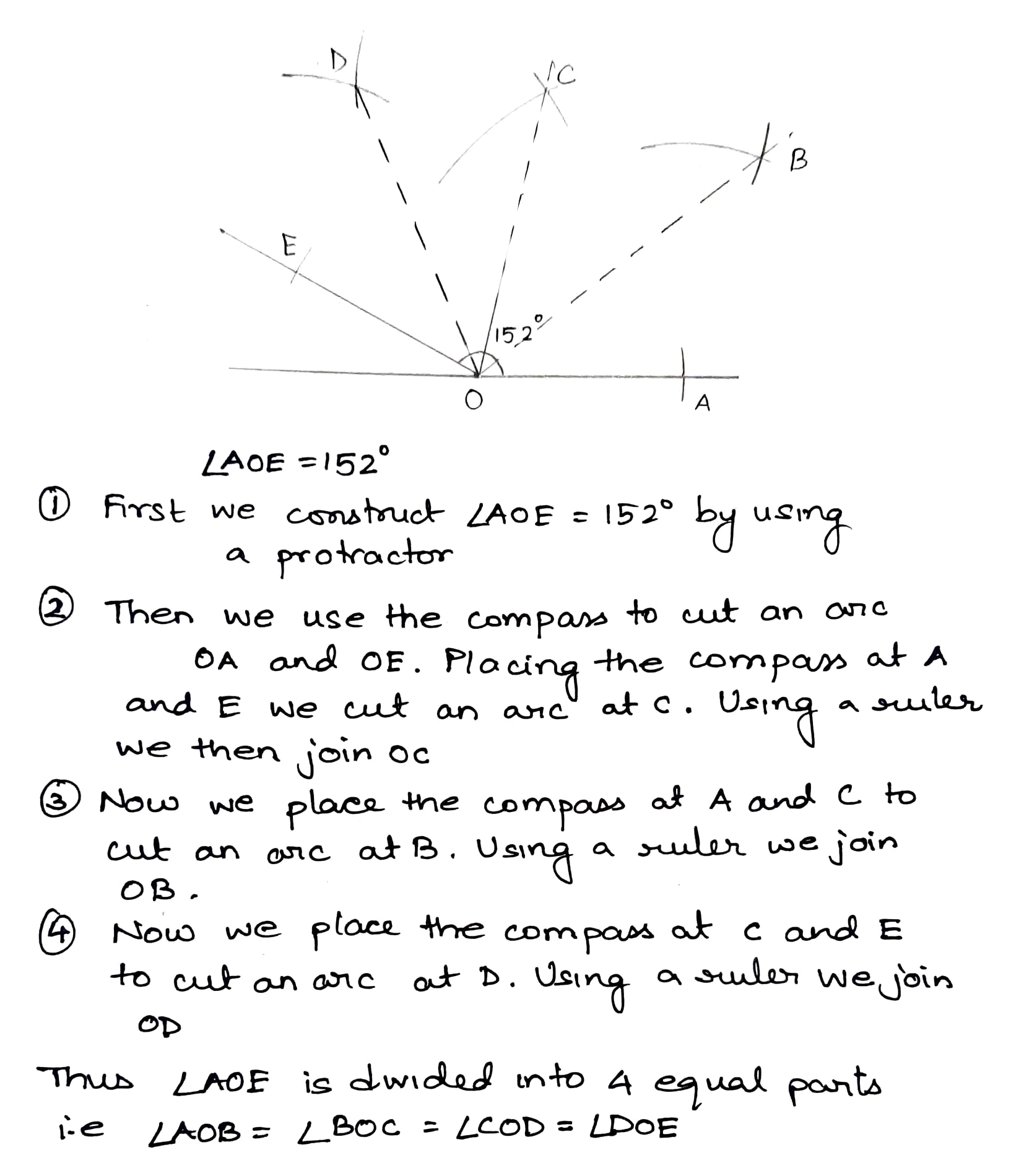
Draw an angle of measure 153∘ using protractor.
Construct with ruler and compasses, angle of the following measure:
120o
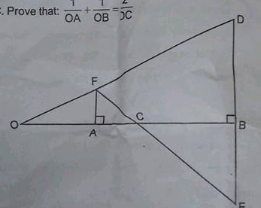
In the figure,OB is the perpndicular bisector of the line segment DE, FA⊥OB and E intersects OB at the point C. Prove that 1OA+1OB=2OC
Draw a line segment PQ=4 cm. Draw a perpendicular bisector to PQ
Explain how to use a pair of compasses to construct 6712o.
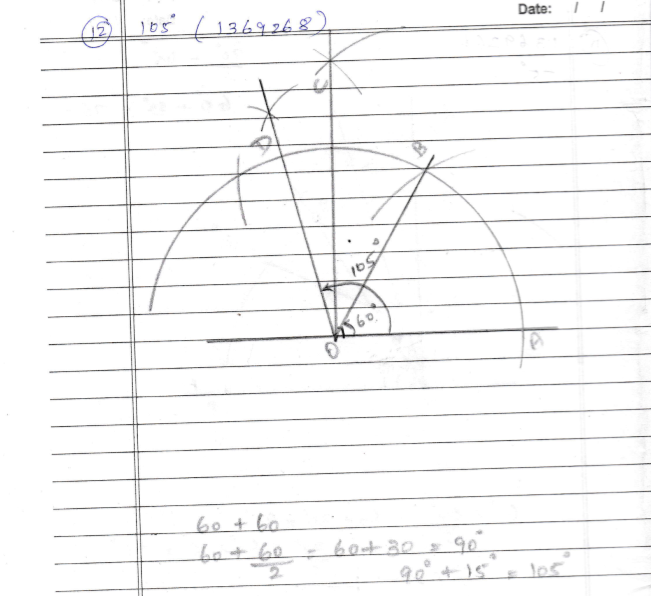
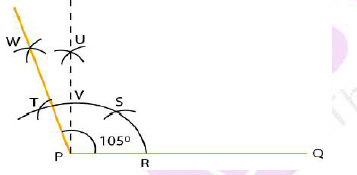
Explain how to construct 105o using a pair of compasses.
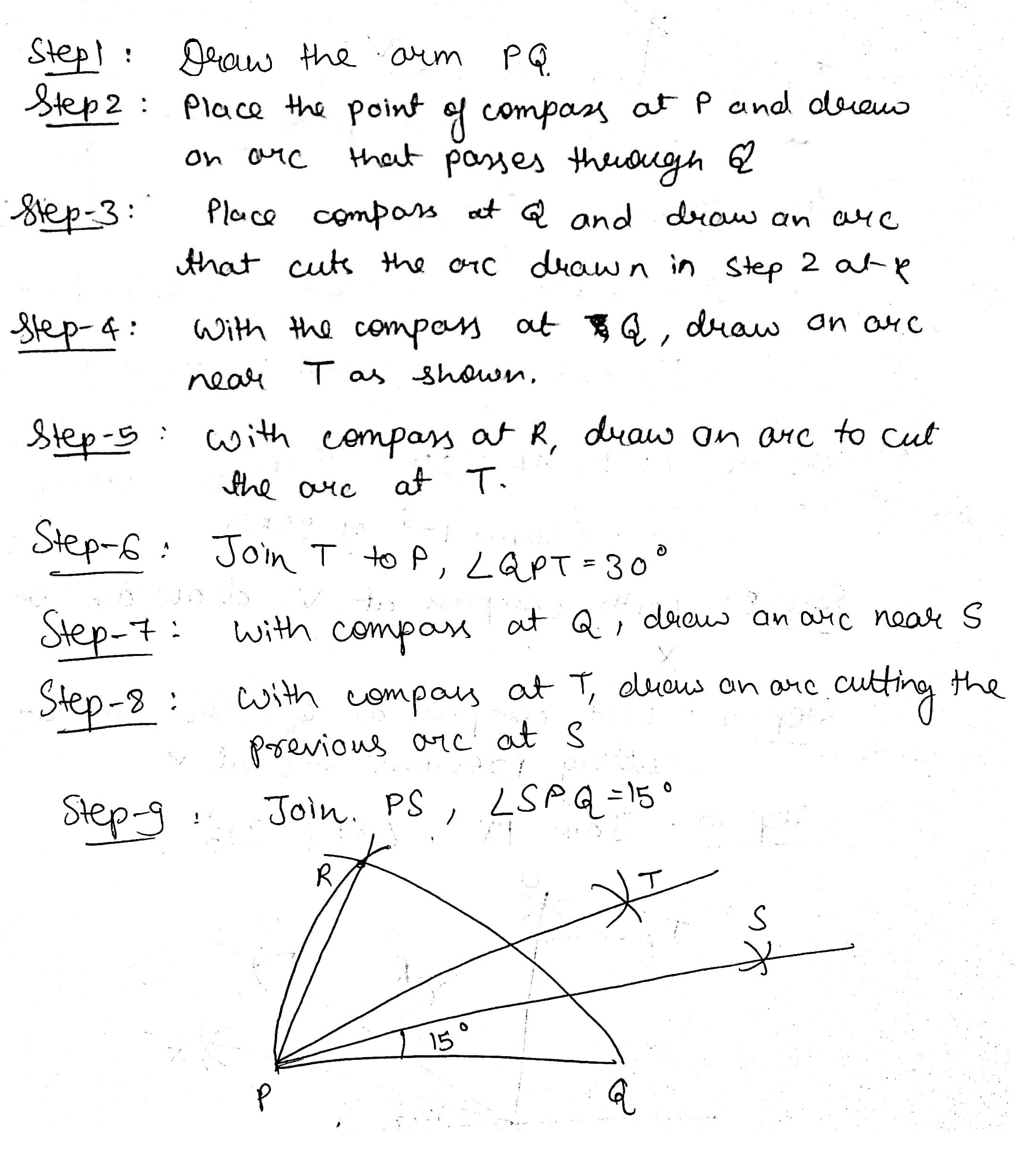
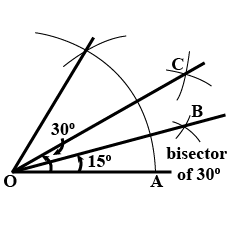
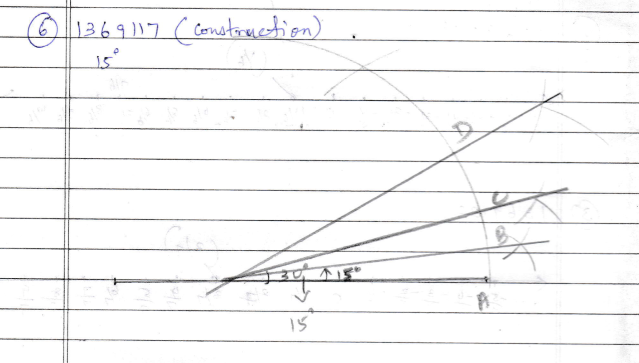
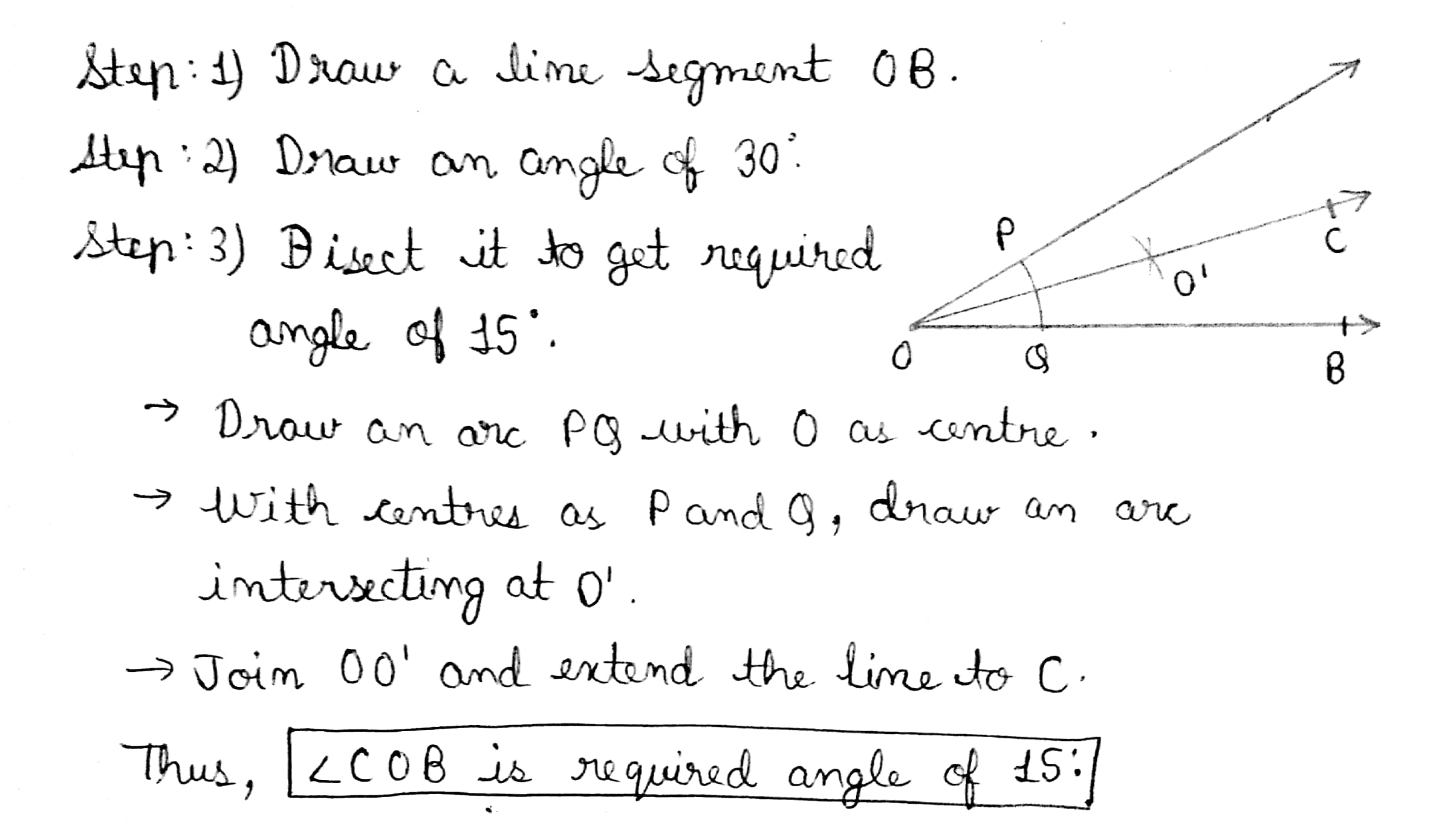
Explain how to construct 15o using a pair of compasses.
If AB = 3 cm then draw 3 AB
Explain how to construct 2212o using a pair of compasses.
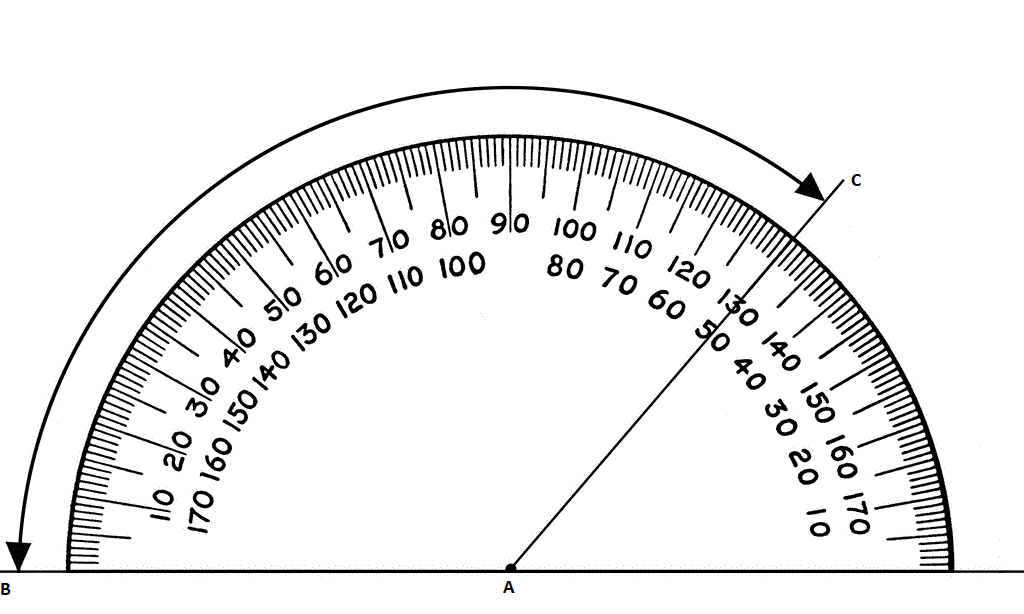
Explain how to draw an obtuse angle using protractor.
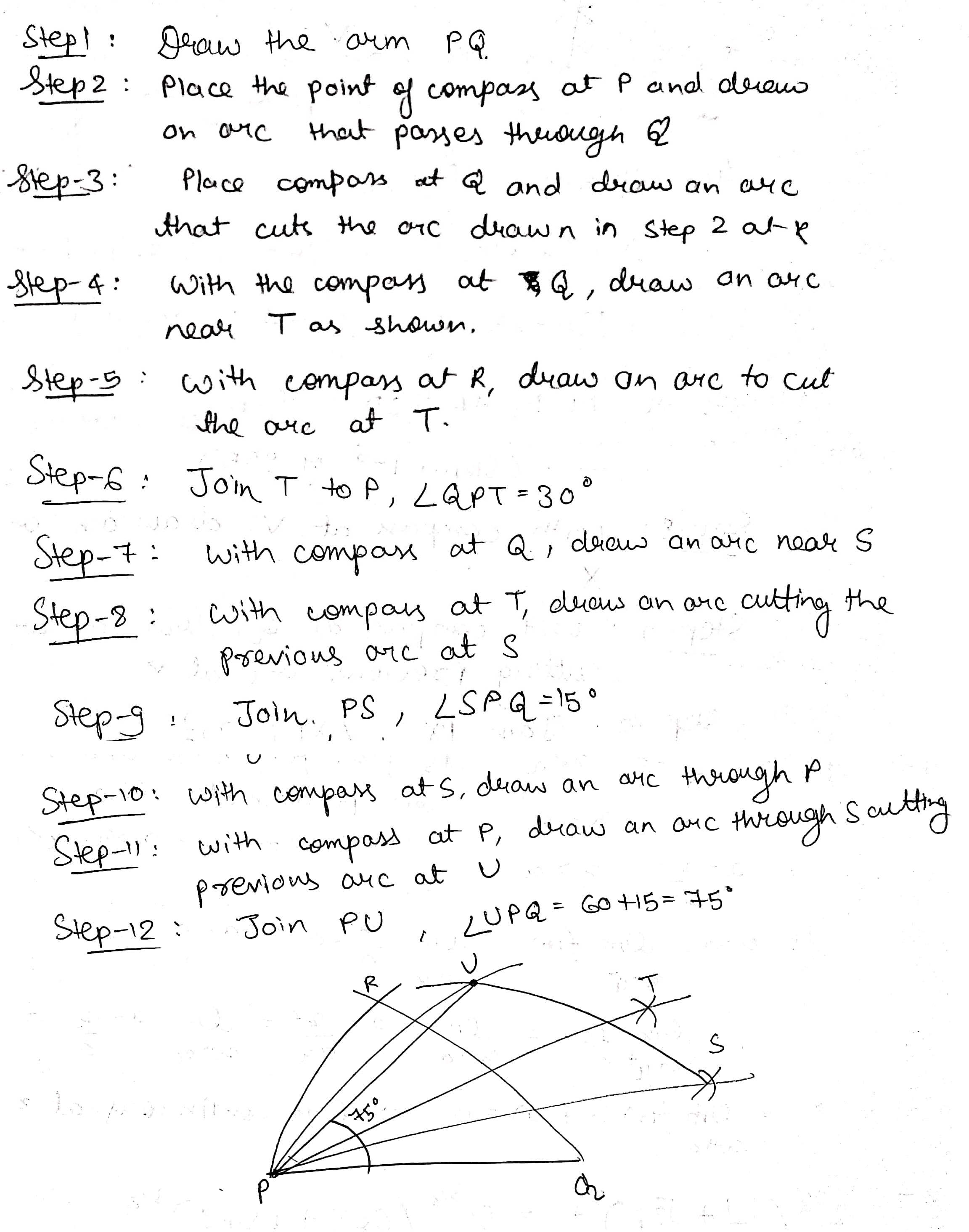
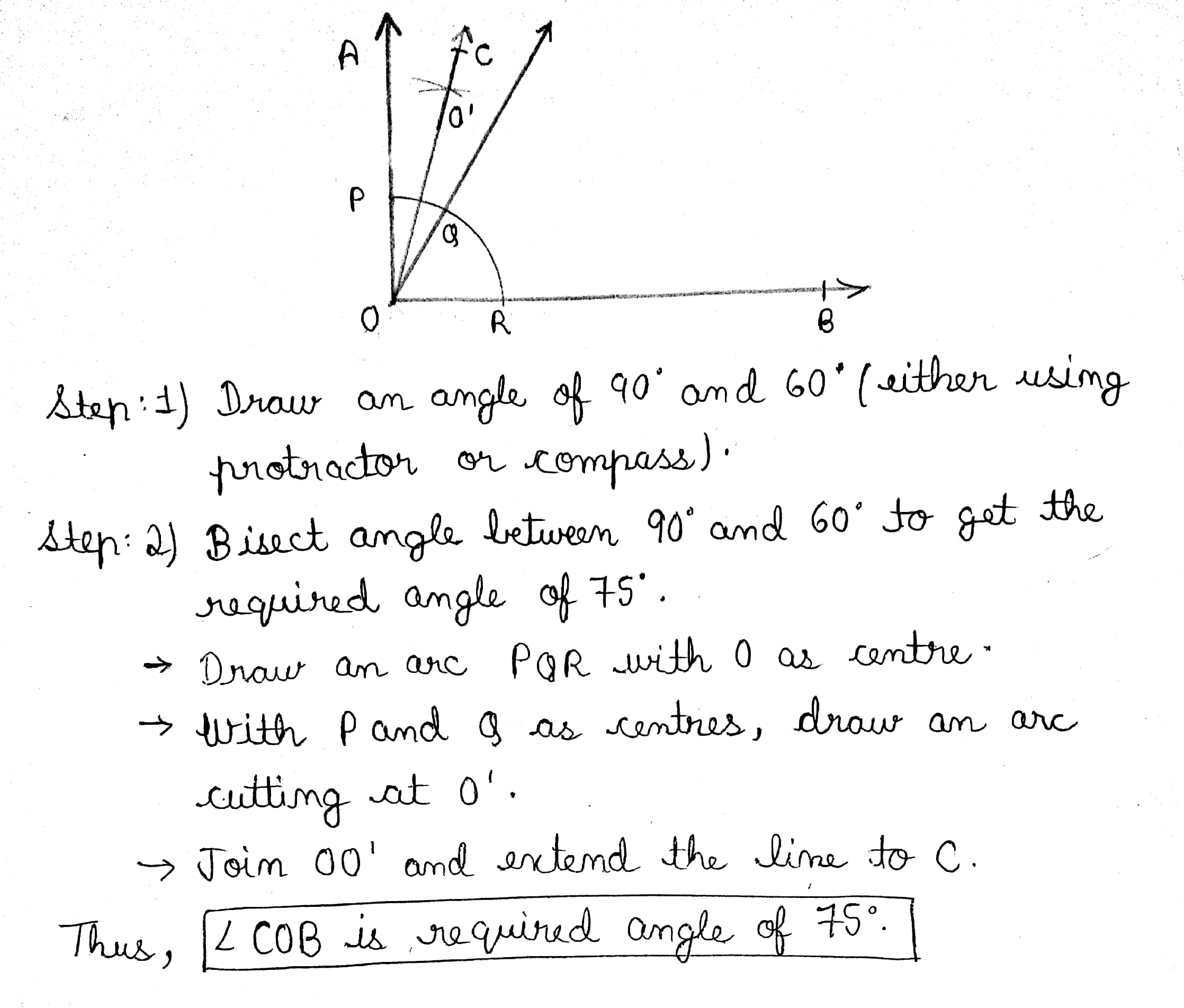
Explain how to construct 75o using a pair of compasses.
Explain how to draw an acute angle using protractor.
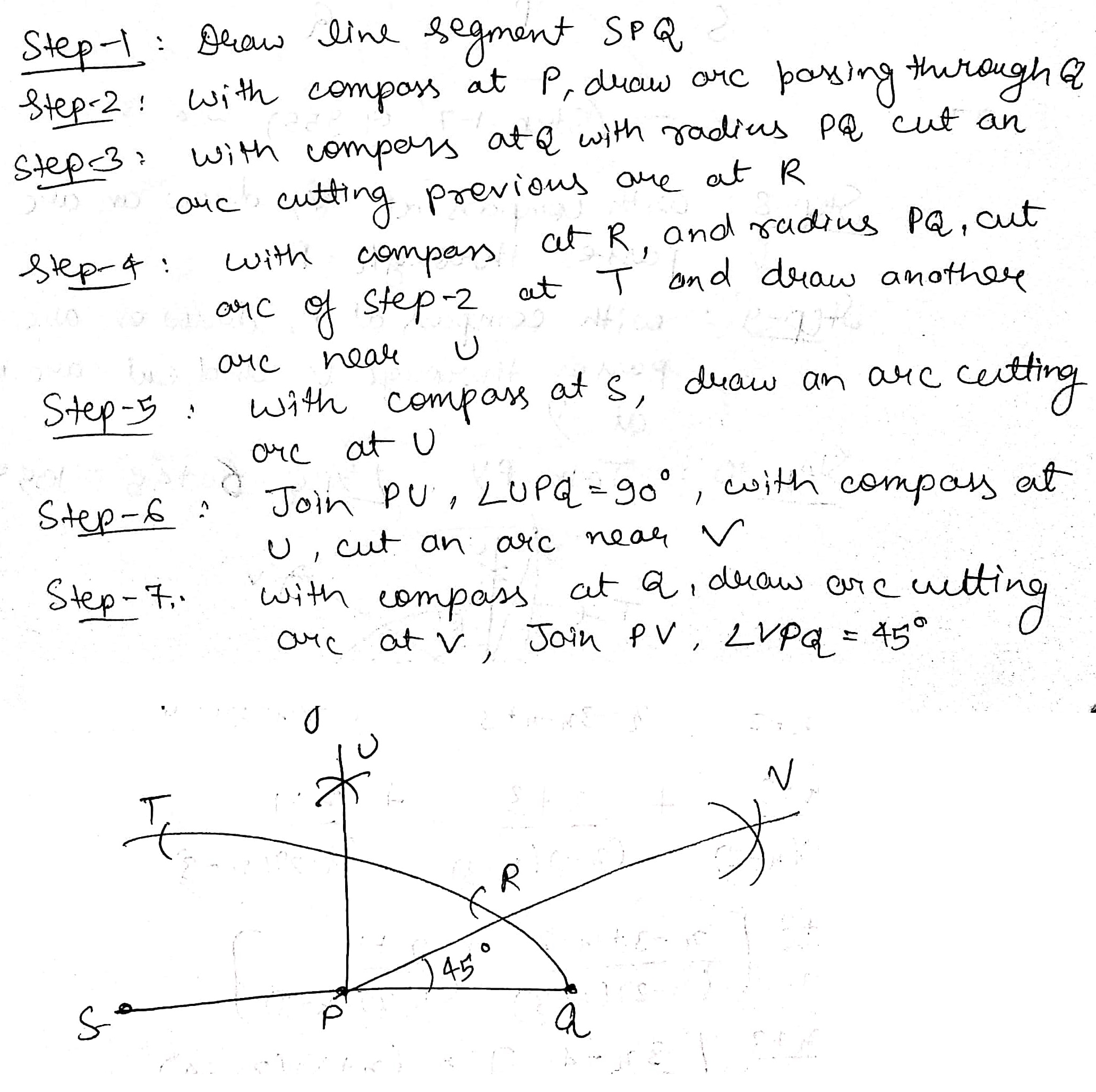
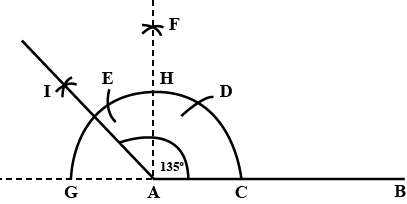
Explain how to construct 135o using a pair of compasses.
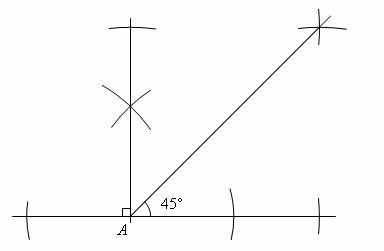
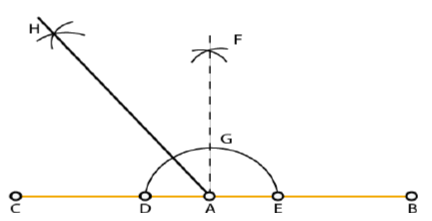
Explain how to use a pair of compasses to construct 45o.
Draw the following angles using compasses.
165∘
Construct an angle 150∘ using protractor and scale.
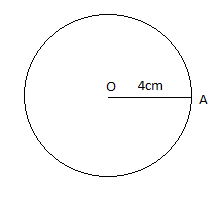
Draw a circle of radius 3.2cm.
Construct an angle of 1300 using protractor.
Draw an angle of 70∘. Make a copy of it using only a straight edge and compasses.
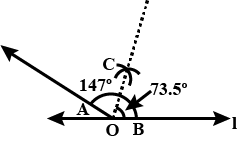
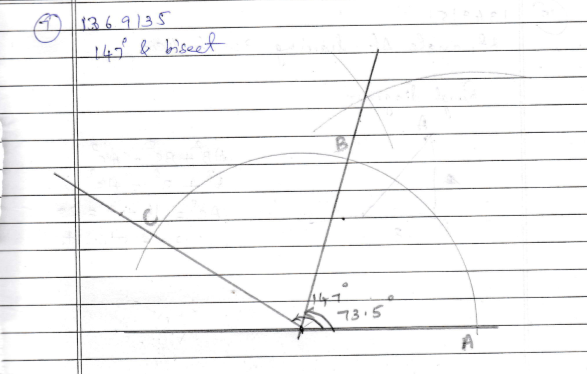
Draw an angle of measure 147o and construct its bisector.
With the same centre O, draw two circles of radii 4cm and 2.5cm.
In the space, draw a circle with diameter 7cm. On your diagram, draw a chord.
Why it better to use a divider and a ruler than a ruler only, while measuring the length of a line segment?
In the space, draw a circle with diameter 7cm.
Use your protector to measure each of the angles marked in the following figures:
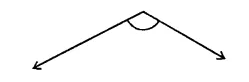
Use your protector to measure the reflex angles marked in the following figures:
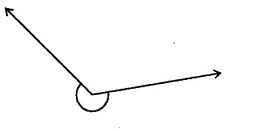
Use your protector to measure the reflex angles marked in the following figures:
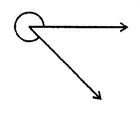
The common properties in the two set-squares of a geometry box are that they have a ________ angle and they are of the shape of a ______ .
Draw rough diagrams of two angles such that they have
Two point in common.
Use your protector to measure each of the angles marked in the following figures:

Use your protector to measure each of the angles marked in the following figures:
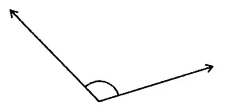
Draw an angle of 80∘ using a protractor and divide it into four equal parts, using ruler and compasses. Check your construction by measurement.
Draw an angle of 80∘ using a protractor and divide it into four equal parts, using ruler and compasses. Check your construction by measurement
Draw a line segment of length 6.5 cm and divide it into four equal parts, using ruler and compasses..
Draw a circle of radius 6cm using ruler and compasses. Draw on of its diameters. Draw the perpendicular bisector of this diameter. Does this perpendicular bisector contain another diameter of the circle
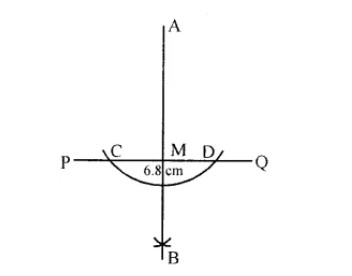
Draw a line segment of length 7cm. Draw its perpendicular bisector, using ruler and compasses.
Enter 1 if it's true else enter 0.A divider is used to compare lengths of the line segment.
Enter 1 if it's true else enter 0.A ruler is used to draw the line and to measure their length.
Enter 1 if its true else enter 0A protractor is used to draw and measure angles.
Enter 1 if its true else enter 0The set squares are two triangular pieces having angles of 30°, 60°, 90° and 45°, 45°, 90°.
Enter 1 if it's true else enter 0.A compass is used to draw circles or arcs of circles.
Draw a line segment of length 6 cm. Construct its perpendicular bisector. Measure the two parts of the line segment.
Enter 1 if its true else enter 0To bisect a line segment of length 7 cm, the opening of the compass should be more than 3.5 cm.
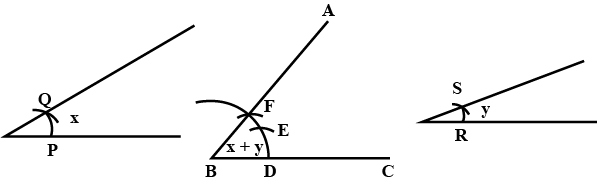
Given below are the angles x and y.
Without measuring these angles, construct:
\angle ABC=x+y
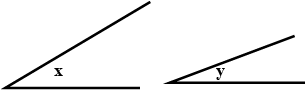
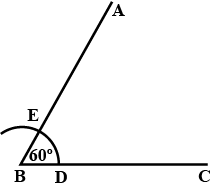
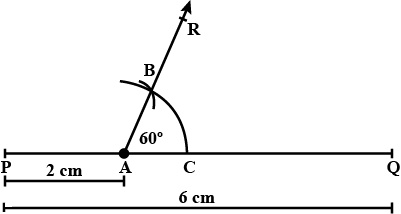
Draw a line segment BC=4cm. construct angle ABC=60^o.
Draw a circle with diameter : 6\ \text{cm}
Measure the length of the radius of the circle drawn.
What is the locus of a points at a distance of 2cm from a fixed line.
Draw rough diagrams of two angles such that they have Three points in common
What is the locus of a point at a distance of 3cm from a fixed point.
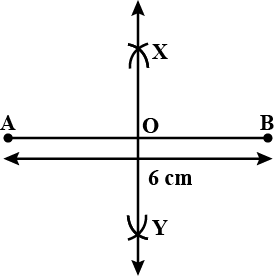
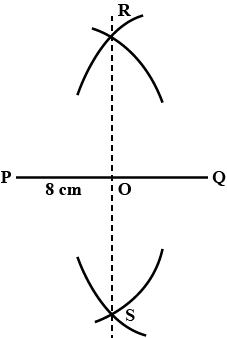
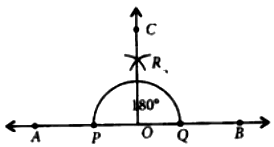
Draw a line segment OP of 5.2 cm. Construct a line perpendicular to OP.
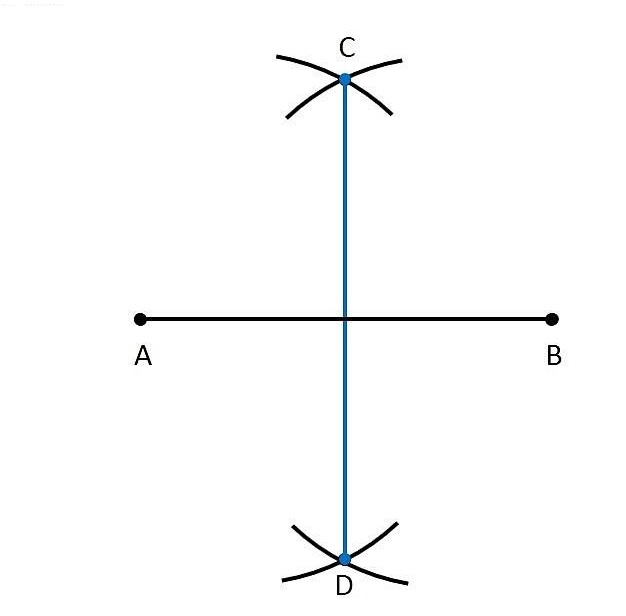
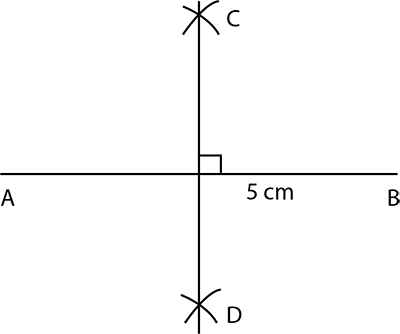
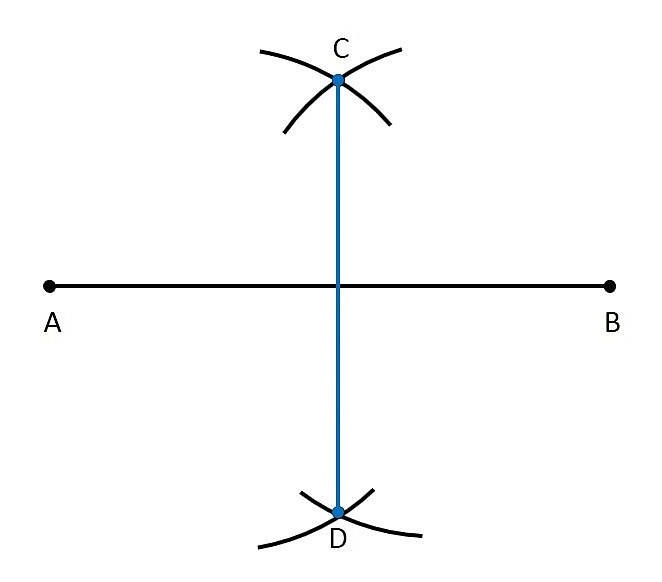
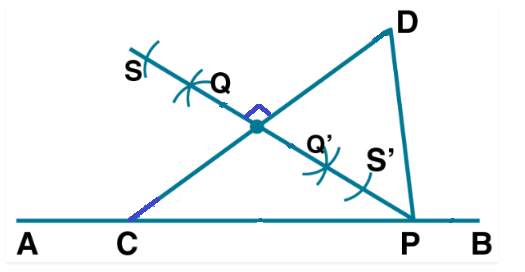
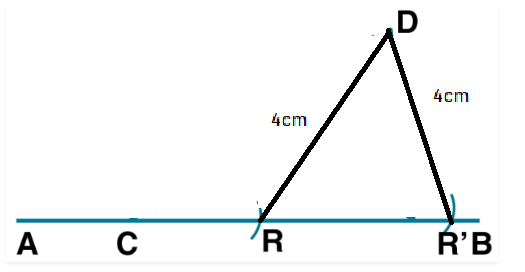
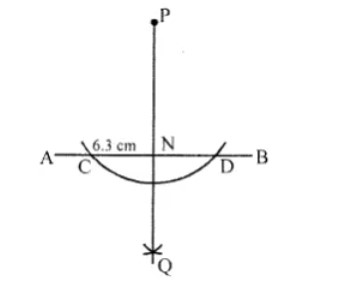
Given a line AB of 5 cm. Draw the perpendicular bisector of AB.
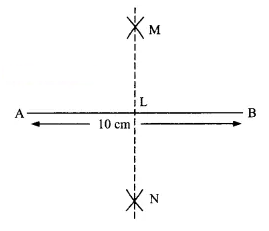
Given a line segment AB =10 cm. Draw a line perpendicular to it.
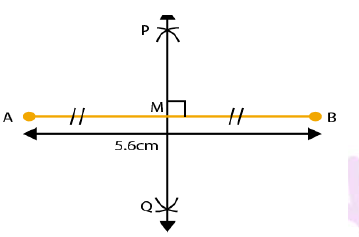
Mark two points A and B, 6.4 cm apart. Construct the line of symmetry so that the points A and B are symmetric with respect to this line.
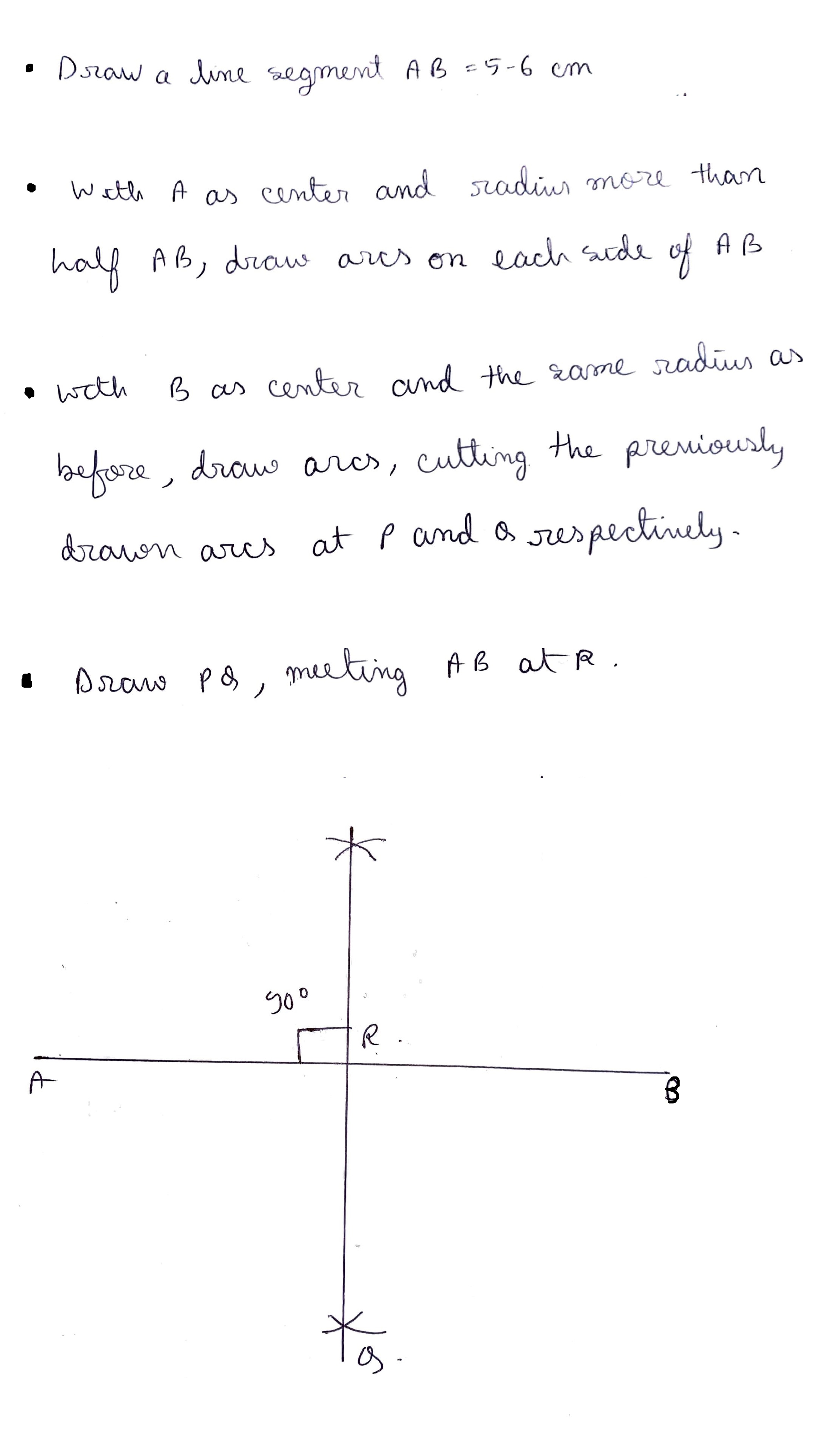
Construct perpendicular bisector of a line segment.
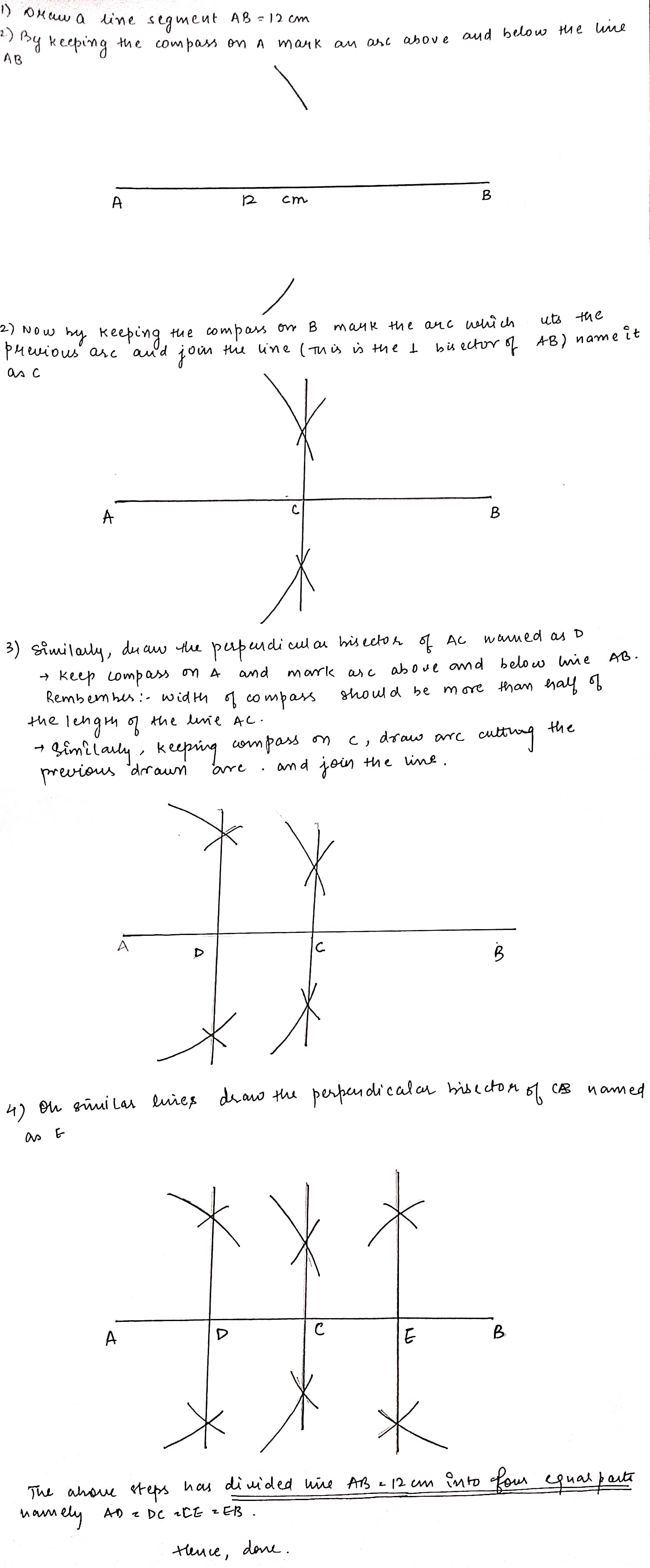
Construct a line segment perpendicular to line segment AB = 12 cm.
Construct an \angle ABC = 40^o. Draw another \angle PQR congruent to the given angle without using a protractor.
Show that of all line segments drawn from a given point, not on it, the perpendicular line segment is the shortest.
Perimeter of a circle is 10\pi. Find the radius of the circle and then draw the circle.
Draw a circle of radius 4.8cm. Take a point on the circle. Draw the tangent at that point using the tangent-chord theorem.
Draw concentric circle for the radii measuring 4 cm and 6 cm. Find out the width of circular ring.
The diameter of the circle is 8cm. Calculate the radius and draw a circle using this radius with the help of a compass. The radius of this circle is
Draw a circle of radius 4\ cm with centre O. Draw a diameter POQ. Through P or Q draw tangent to the circle.
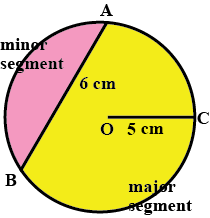
Draw a circle of radius 5 cm. In the circle, draw a chord AB = 6 cm, then shade the minor and major segments.
Using a set square and a ruler construct a line perpendicular to given line at a point on it.
Draw an angle of 40^o. Find its supplementary angle.
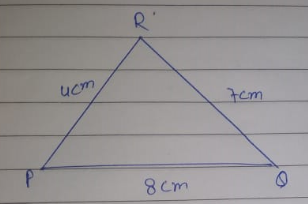
Construct \triangle PQR with PQ=4cm, \,QR =7cm and PR=8cm
If radii of two circles are 4cm and 2.8 cm. Draw figure of these circles touching each other (i) externally (ii) internally.
Construct an angle measuring 35^{o} using protractor.
Using a set square and a ruler draw a line parallel to the given line through a point 5 \text cm above it.
Draw a line segment of length 7.6 cm and divide it in the ratio 5 :Measure the two parts.
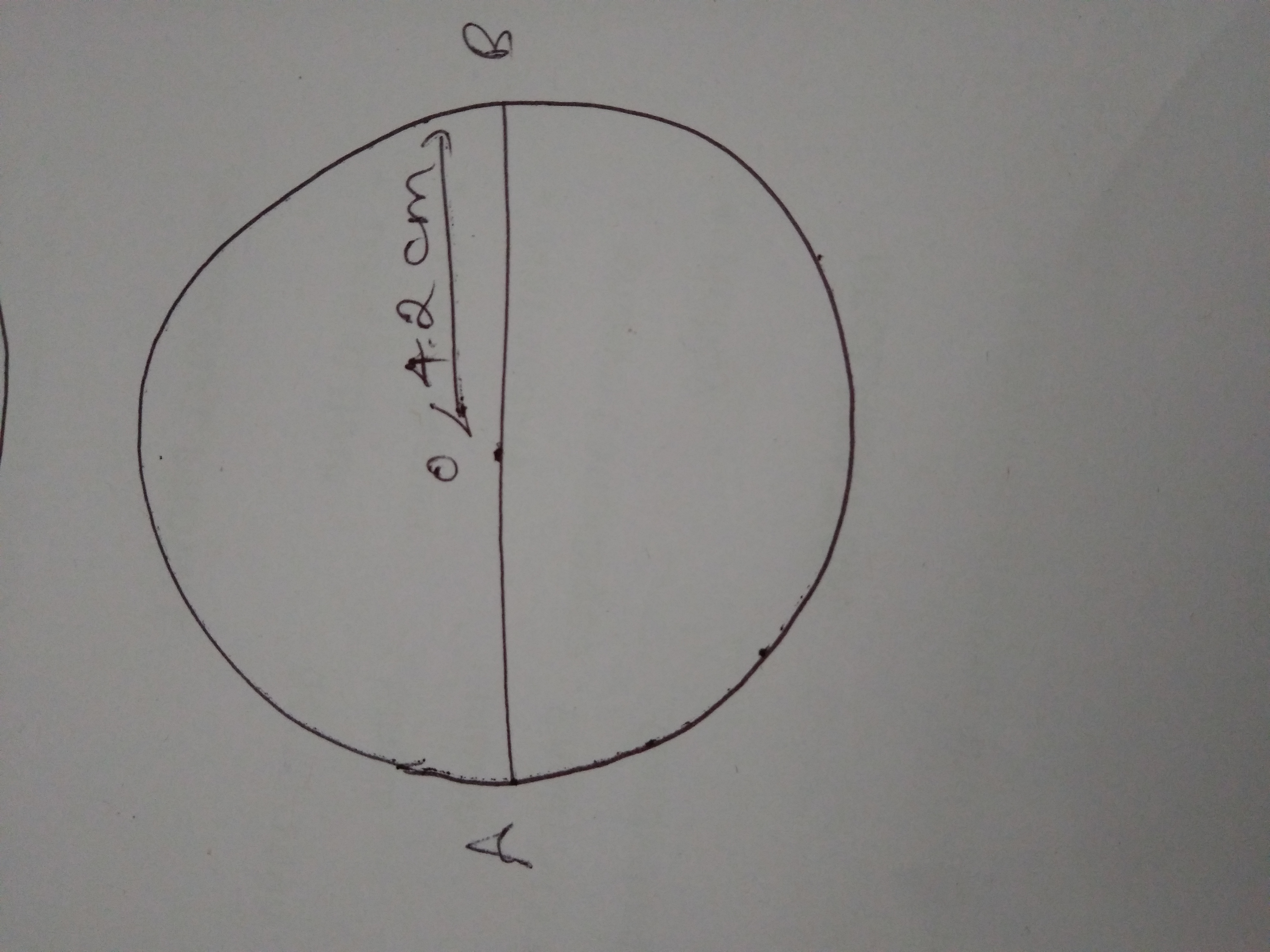
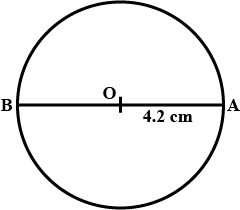
Draw a circle of radius 4.2\ cm.
Find\quad the\quad foot\quad of\quad the\quad perpendicular\quad from\quad (7,14,5)\quad to\quad 2x+4y-z=2.
Construct a line segment of length 5.6\ cm using ruler and compasses.
Draw a line segment AB using a ruler and compass, obtain a line segment of length \dfrac {3}{4}(AB).
Construct \overline{AB} of length 7.8\ cm. Find this, cut off AC of length 4.7\ cm . Measure \overline {BC}
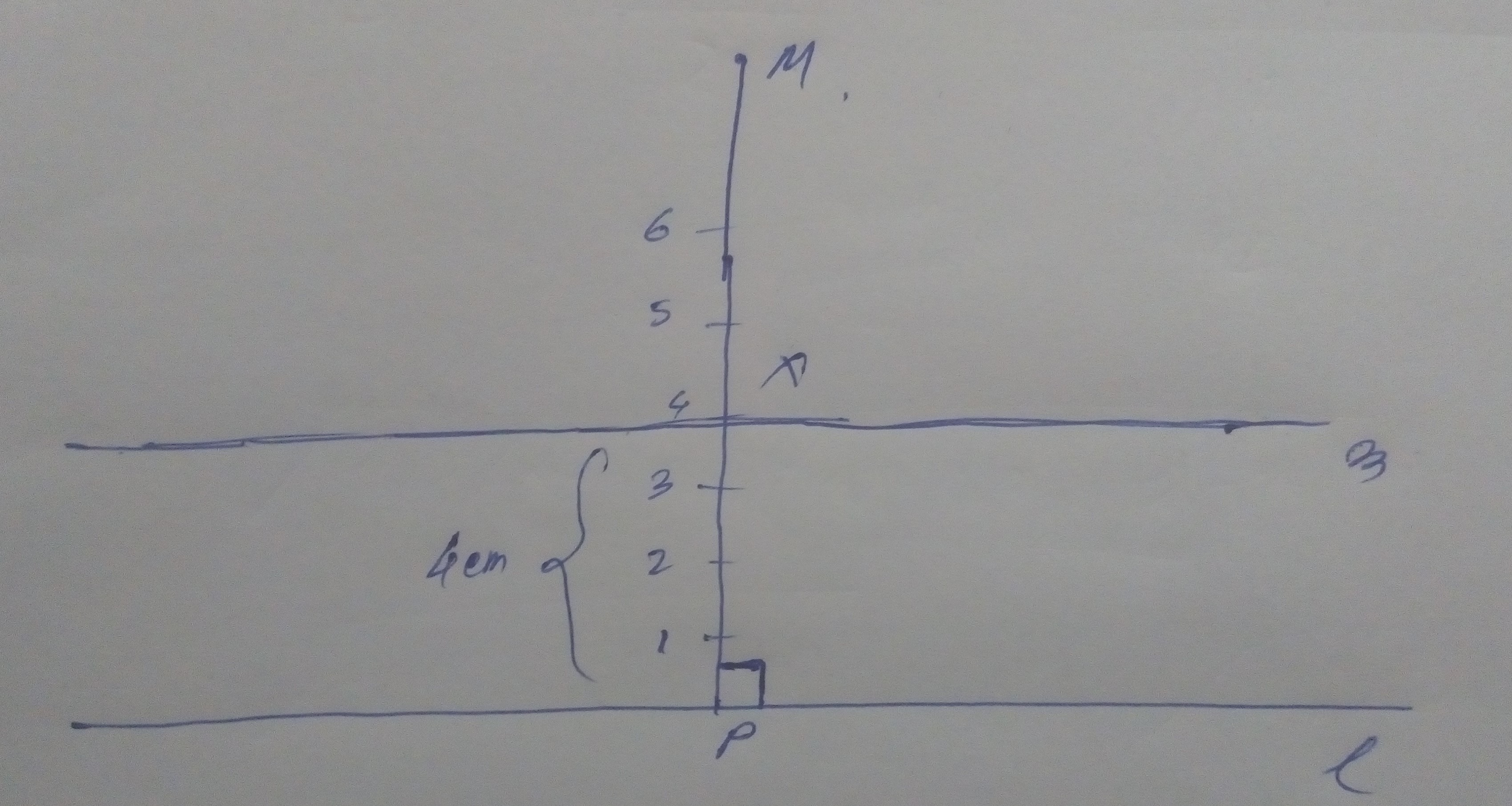
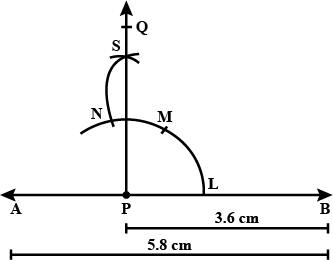
Draw a line l. Draw a perpendicular to l at any point on l.On this perpendicular choose a point X,4\ cm away from l. Through X,draw a line m parallel to l .
Find the measure of the complement of (i){115}^{\circ}\,\,(ii){25}^{\circ}\,\,(iii){15}^{\circ}\,\,(iv){35}^{\circ}.
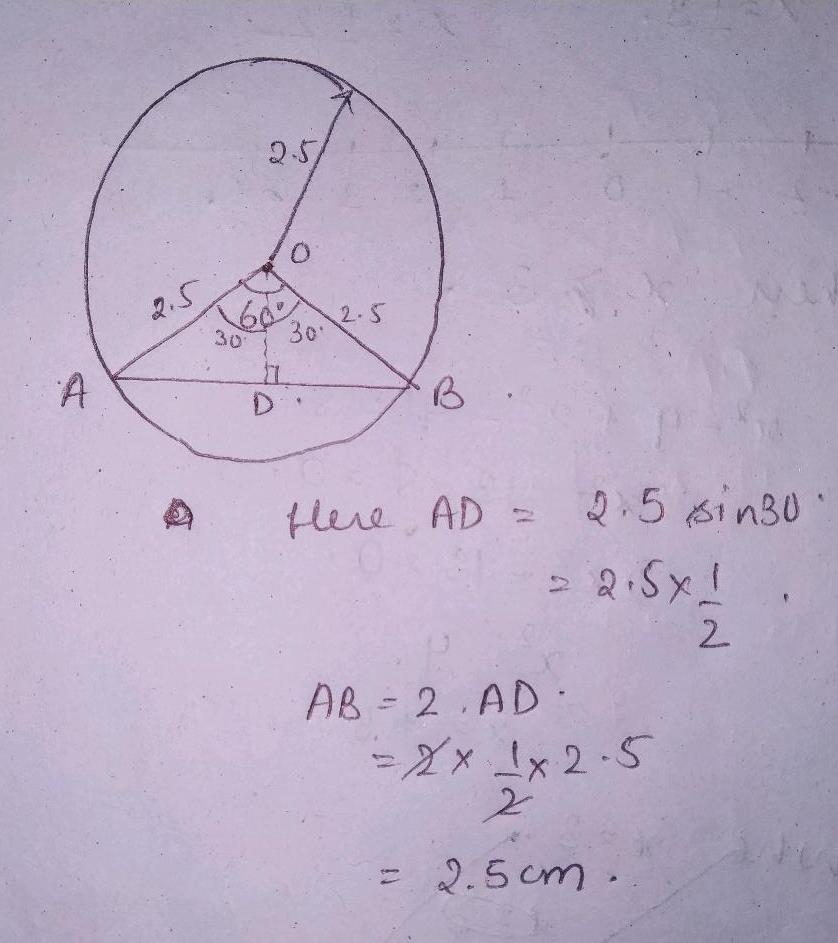
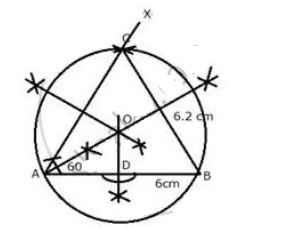
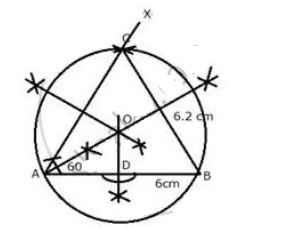
Draw a circle with centre O and radius 2.5\ cm. Draw two radii OA and OB such that \angle A O B = 60 ^ { \circ }. Measure the length of chord AB.
ABC and DBC are two isosceles triangles on the same side of BC. Prove that :
(i) DA (or AD) produced bisects BC at right angle.
(ii) \angle BDA = \angle CDA
Using your protractor, draw an angle of measure 108 ^ { \circ }.With this angle as given, draw an angle of 54 ^ { \circ }.
Draw a pair of tangents to a circle of radius 2 cm which are inclined at an angle of 90^o.
Construct the following angles and verify by measuring them by a protractor:
135^{o}
In a \Delta A B C , the sides B C = 5 , C A = 4 , A B = 3. If A ( 0,0 ) and the internal bisector of angle A meet B C in D \left( \dfrac { 12 } { 7 } , \dfrac { 12 } { 7 } \right) then in-centre of \Delta \mathrm { ABC }
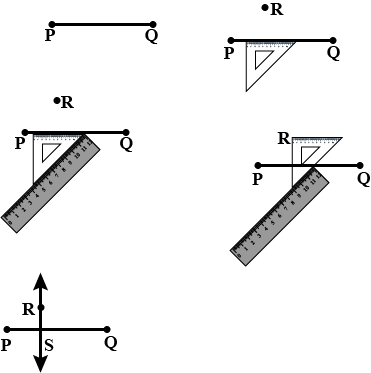
Draw any line segment AB and mark any point P on it. Using a ruler and a compass draw a perpendicular on AB through point P.
Drawn an angle of measure 30^{o} and construct its bisector.
Draw any line segment AB. Mark any point M on it. Through M, draw a perpendicular using ruler and compass.
Bisect a line of length 7 cm. Write down the steps of construction.
Draw a line, say, MN. From a point P outside it, draw a line parallel to MN using ruler and and compasses only.
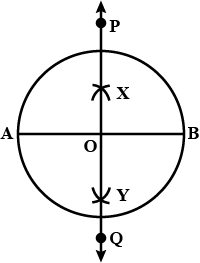
Draw a circle and any two of its (non- perpendicular) diameter. If you join the ends of these diameters, what is the figure obtained? What figure is obtained if the diameters are perpendicular to each other? How do you check the answer?
Draw a line segment PQ= 8 cm. Construct the perpendicular bisector of the line segment PQ. Let the perpendicular bisector drawn meets PQ at point R. Measure the length of PR and QR. Is PR=QR?
Draw a triangle ABC in which \angle A=120^{o} and AB=AC=3\ cm. Draw the bisectors angle A.
Draw a line segment ST, measuring 9.5\ cm.
Draw a line l . Draw a perpendicular to l at any point on l. On this perpendicular choose a point X , 4 \mathrm { cm } away from l. Through \mathrm { X } , draw a line m parallel to l.
Draw an angle of measure 147^{o} using protractor and construct its bisector.
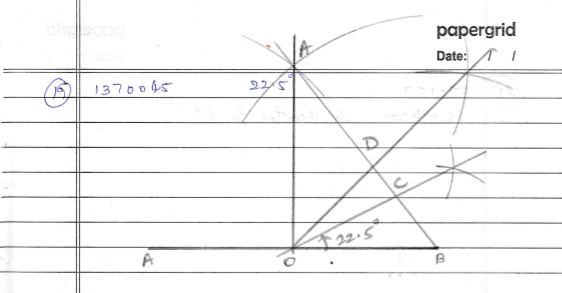
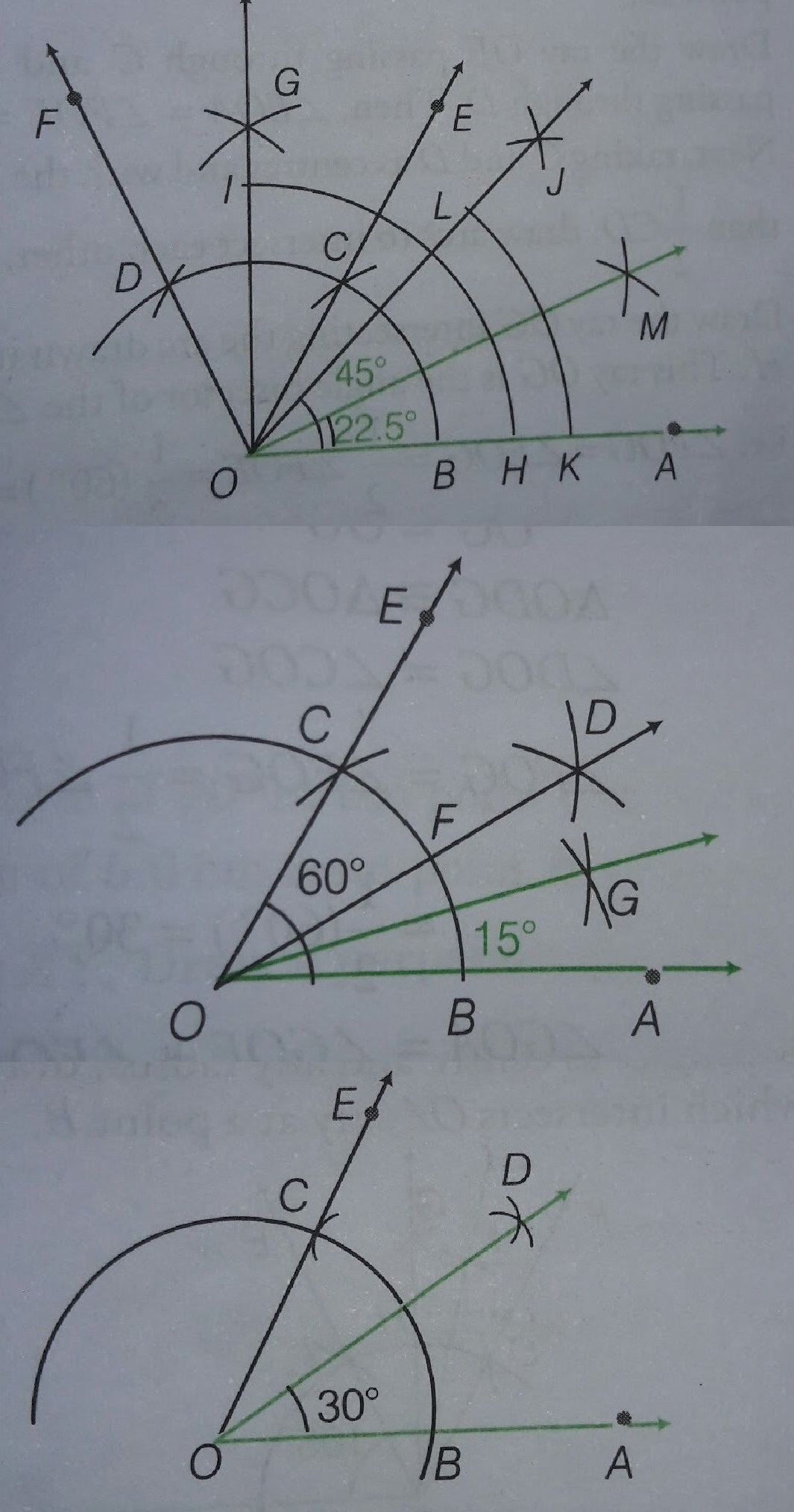
Construct an angle of measure {22.5}^{o} using compass and ruler.
Construct the following angles and verify by measuring them by a protractor.
75^{o}
Construct with ruler and compasses, angle of the following measure:
60^{o}
Construct the following angles and verify by measuring them by a protractor:
105^{o}
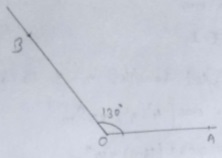
Construct the angle 130^{\circ} using protractor.
Construct the following angles and verify by measuring them by a protractor:
135^{o}
Construct the angle 115^{o} using protractor.
Construct an angle of 15^{o}.
Draw any line segment PQ. take any point R not on it .through R, draw perpendicular to PQ. (use ruler and set - square)
Draw an angle of measure 70^{\circ} with protractor. Bisect this angle using compasses and rules.
Draw an angle of measure {153}^{\circ} and divide it into four equal parts.
Draw \angle ABC = 120^\circ . Bisect the angle using the ruler and compasses only. Measure each angle so obtained and check whether the angles obtained on bisecting \angle ABC are equal or not.
Draw a line segment of length 7.3\ cm using a ruler.
Draw the perpendicular bisector of { XY } , whose length is 10.3 cm
draw a line segment of length 7.6 cm and construct its perpendicular bisector
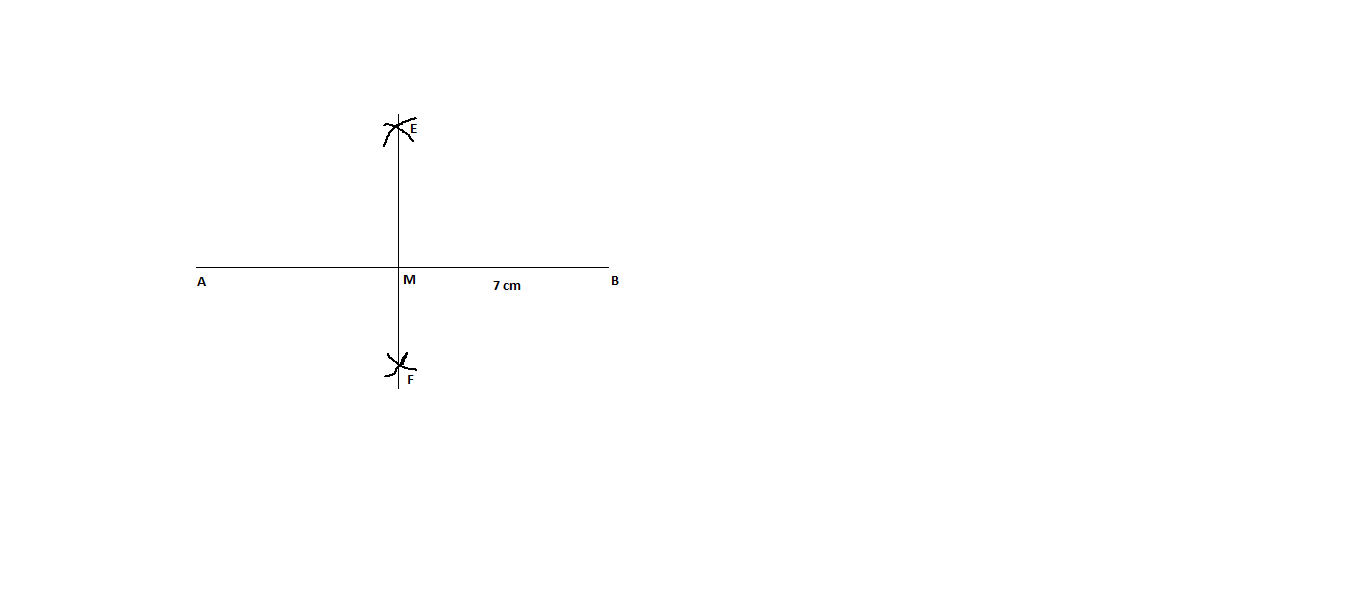
Construct a perpendicular bisector of a given line segment.
Draw an angle of { 152 }^{ o } and divide the into four equal parts.
Draw a circle of radius 3cm.At a point P on it, draw a tangent to the circle without using centre.
Construct a \Delta ABC in which CA = 6 \,cm, AB = 5 \,cm and \angle BAC = 45^o. Then construct a triangle whose sides are \dfrac{3}{5} of the corresponding sides of \Delta ABC.
Draw an angle of 40^\circ. Copy its supplementary angle.
Draw an angle and label it as \angle BAC. Construct another angle equal to \angle BAC.
Draw 105 ^\circ using rounder.
Draw any line segment \overline{PQ}. Take any point R, not on it, through R, draw a perpendicular to \overline{PQ}. (use ruler and set-square).
Draw rough sketches for the following.
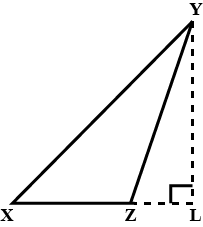
In \DeltaXYZ, YL is an altitude in the exterior of the triangle.
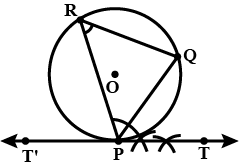
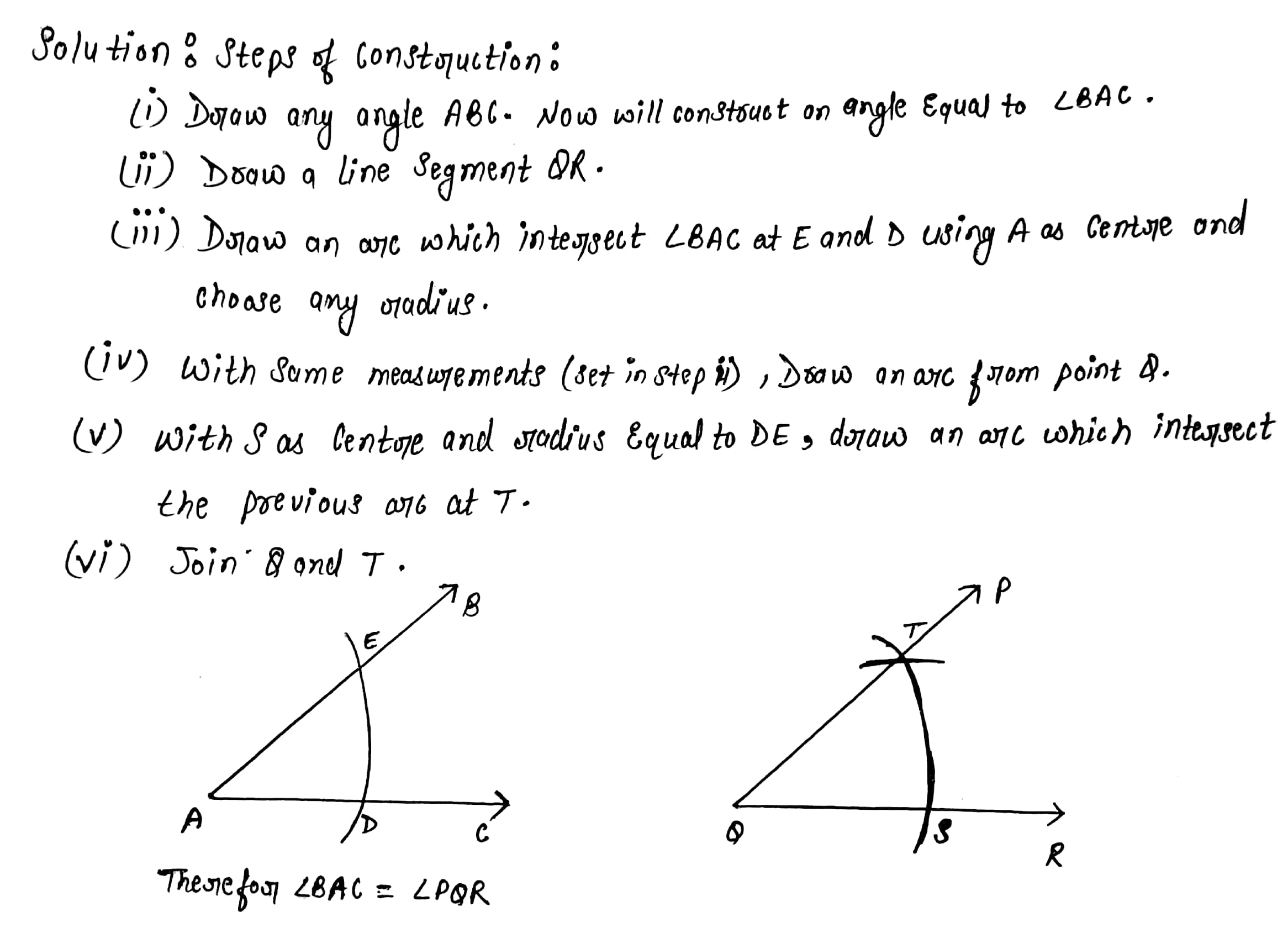
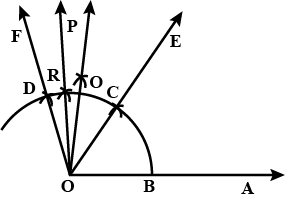
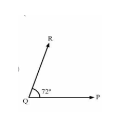
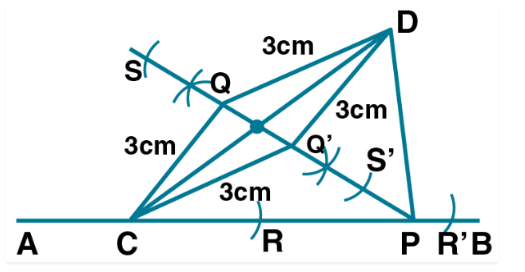
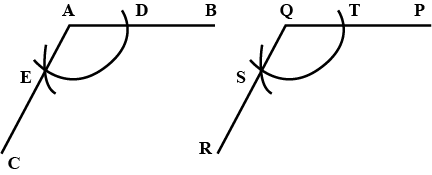
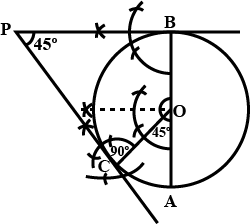
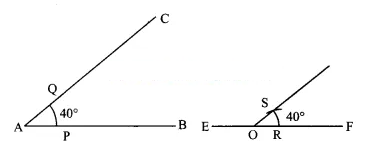
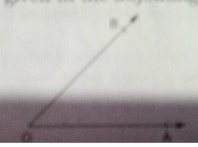
Draw an angle equal to \angle AOB given in the adjoining figure.
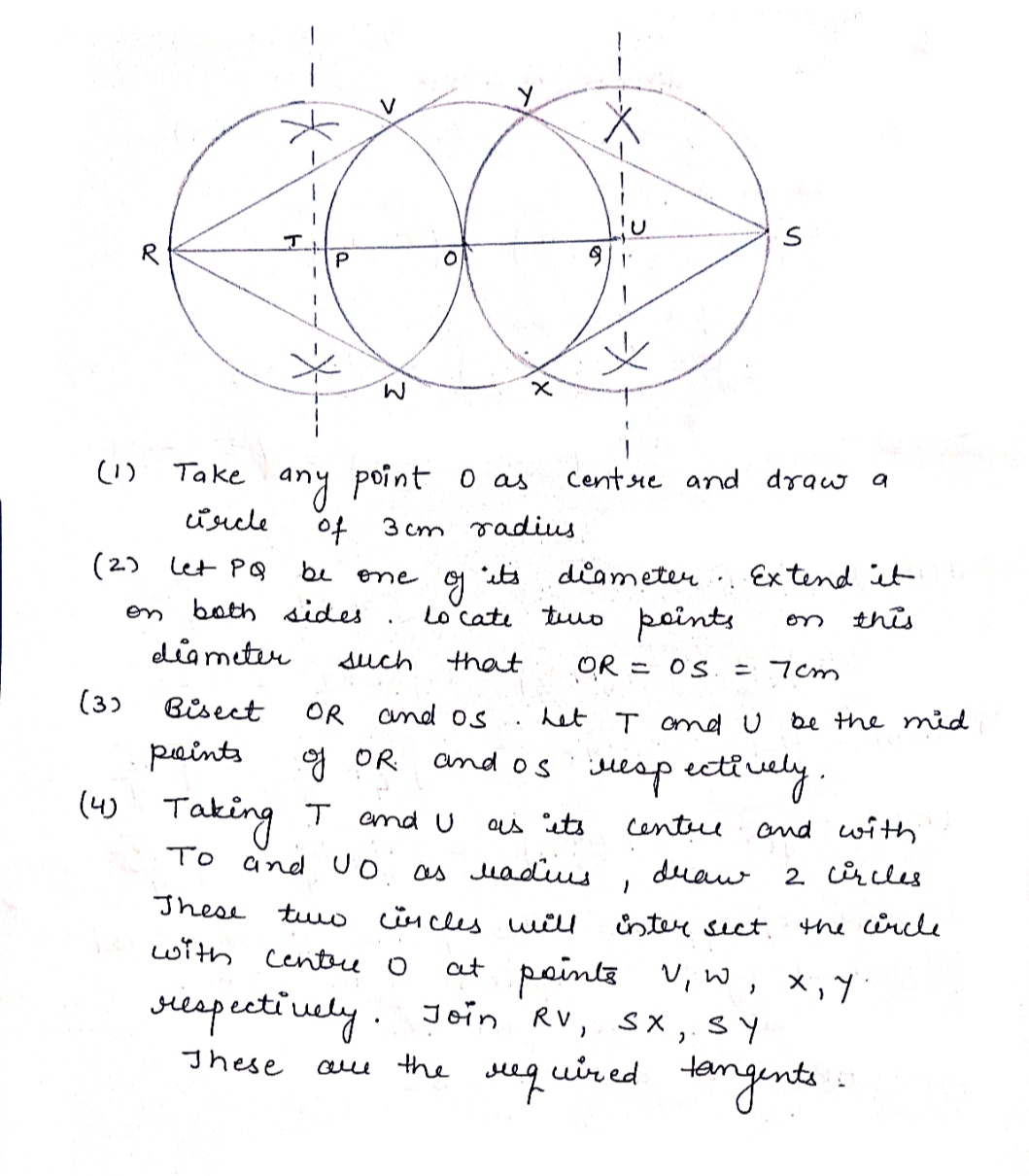
Draw a line segment AB of length 8 cm . Taking A as centre, draw a circle of radius 4 cm and taking B as centre draw another circle of radius 3 cm Construct tangents to each circle from the centre of the other circle .
Draw a line segment \bar {AB} of length 6.4 cm and construct its axis of symmetry (use ruler and compass).
The diameter of a circle is 14\ cm, find the radius.
Fill in the blanks:
A radius of a circle is a line segment with one end at ______ and the other end at _______
Draw a line segment AB and bisect it. Bisect one of the equal parts to obtain a line segment of length \dfrac{1}{2}(AB).
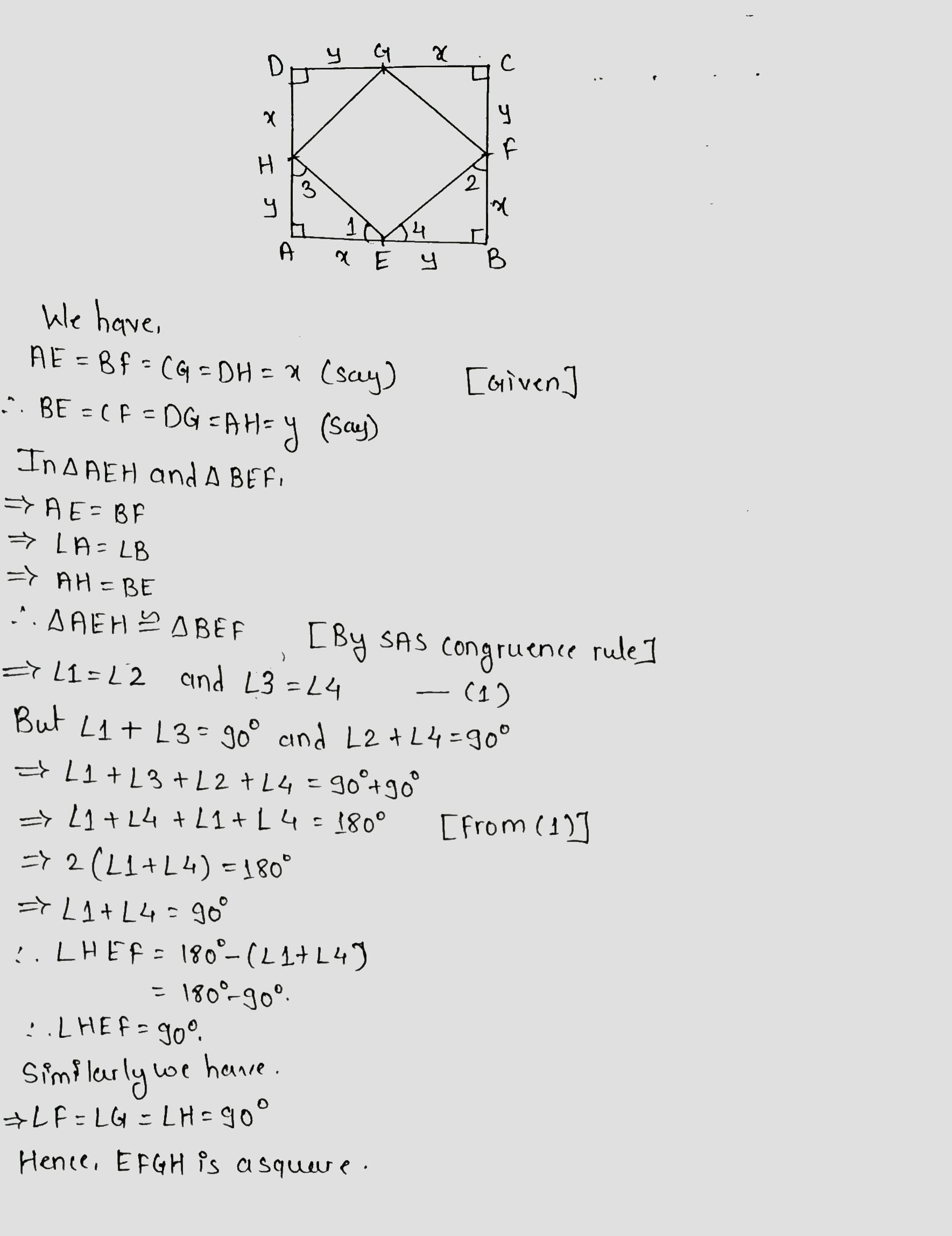
ABCD is a square E,F,G and H are points on AB, BC, CD and DA respectively, such that AE = BF = CG = DH. Prove that EFGH is a square.
Draw an angle and label it as \angle BAC. Construct another angle, equal to \angle BAC.
Using a protractor, draw the following angles.
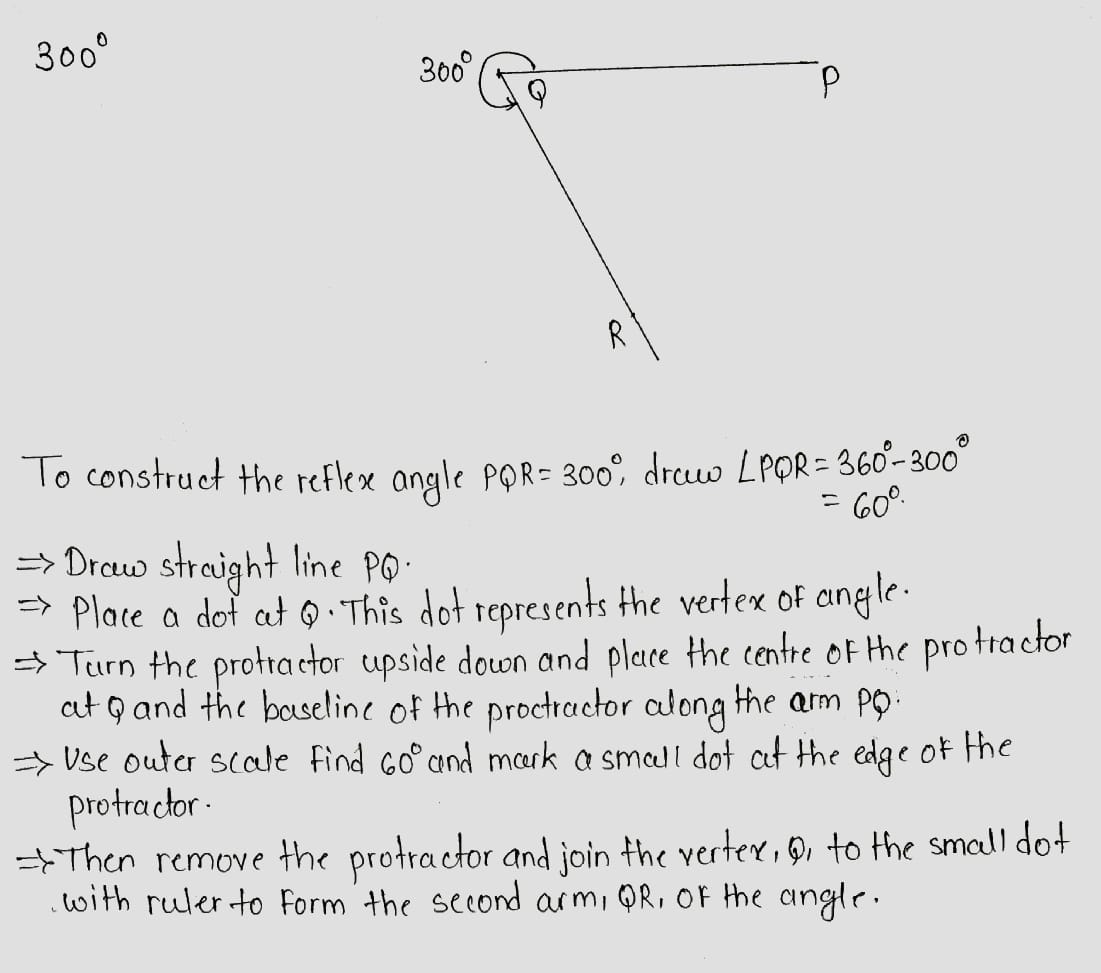
{ 300 }^{ o }
Using a protractor, draw the following angles.
{ 130 }^{ o }\quad
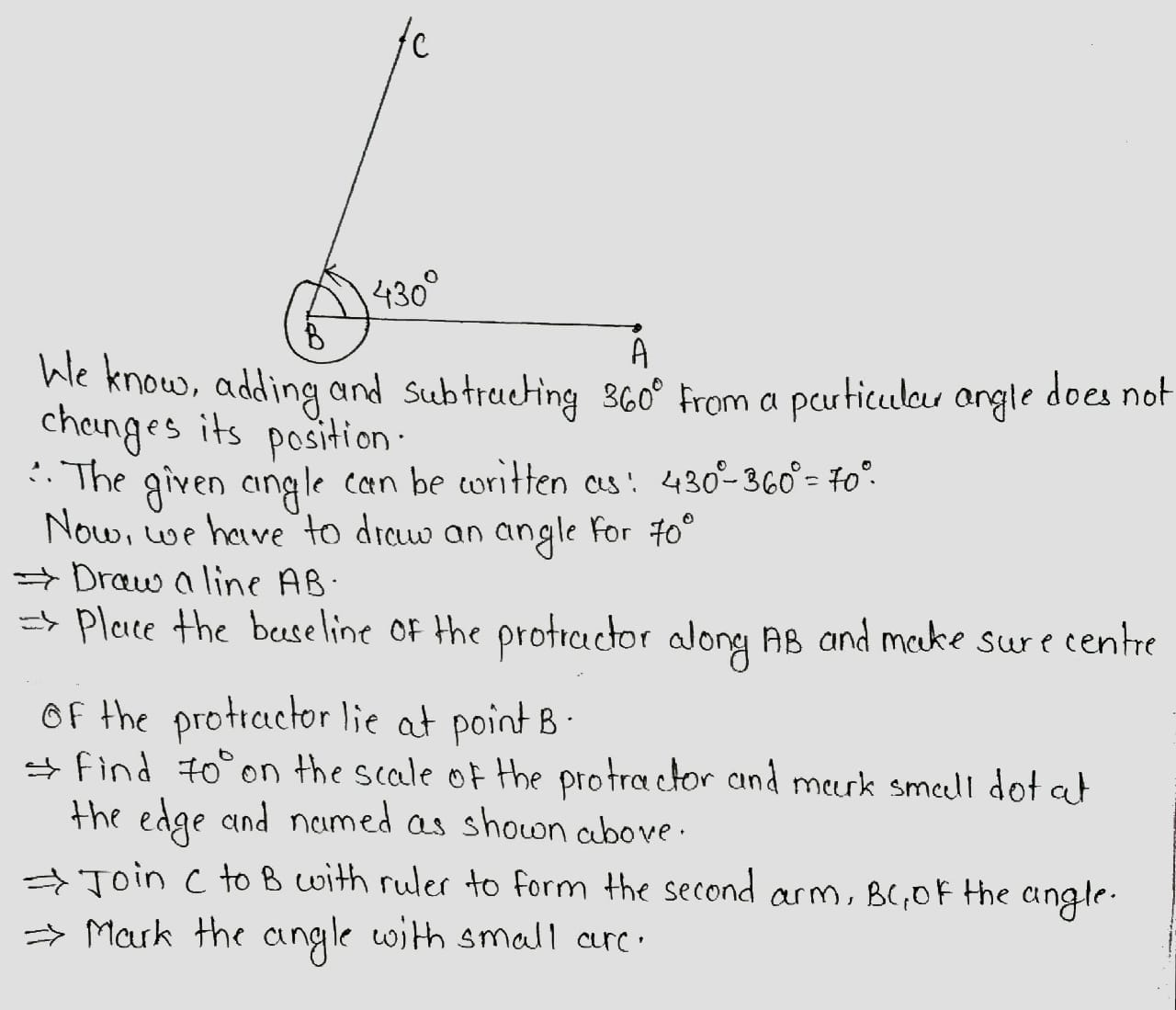
Using a protractor, draw the following angles.
{ 430 }^{ o }
Using a protractor, draw the following angles.
{ 60 }^{ o }
Draw a line PQ. Take a point R on it. Draw a line perpendicular to PQ and passing through R.(Using ruler and compasses)
Using a protractor, draw the following angles.
-{ 40 }^{ o }
Draw a circle of radius 3 cm. From a point P, 7 cm away from the centre of the circle, draw two tangents to the circle. Also, measures the triangle of the tangents.
Draw a circle of radius 4.2 cm. Draw a pair of tangents to this circle inclined to each other at an angle of 45^0.;
Construct each of the following angles, using ruler and compasses:
75^o
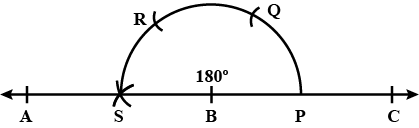
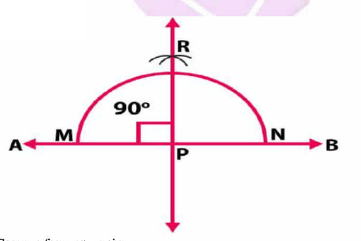
Construct an angle of 90^o using ruler and compasses and bisect it.
Draw a line segment AB=5.6\ cm and draw its perpendicular bisector. Measure the length of each part.
Construct each of the following angles, using ruler and compasses:
37.5^o
Draw a circle with centre O and radius 4 cm. Draw any diameter AB of this circle. Construct tangents to the circle at each of the two end pointsd of the diameter AB.
Draw an angle of 80^o with the help of a protractor and bisect it. Measure each part of the bisected angle.
Construct each of the following angles, using ruler and compasses:
135^o
Construct the angle whose cosine is equal to its tangent.
Construct each of the following angles with the help of a protractor:
72^{o}
Construct each of the following angles with the help of a protractor:
25^{o}
Draw a line segment AB=5.6 \ cm. Draw the perpendicular bisector of AB.
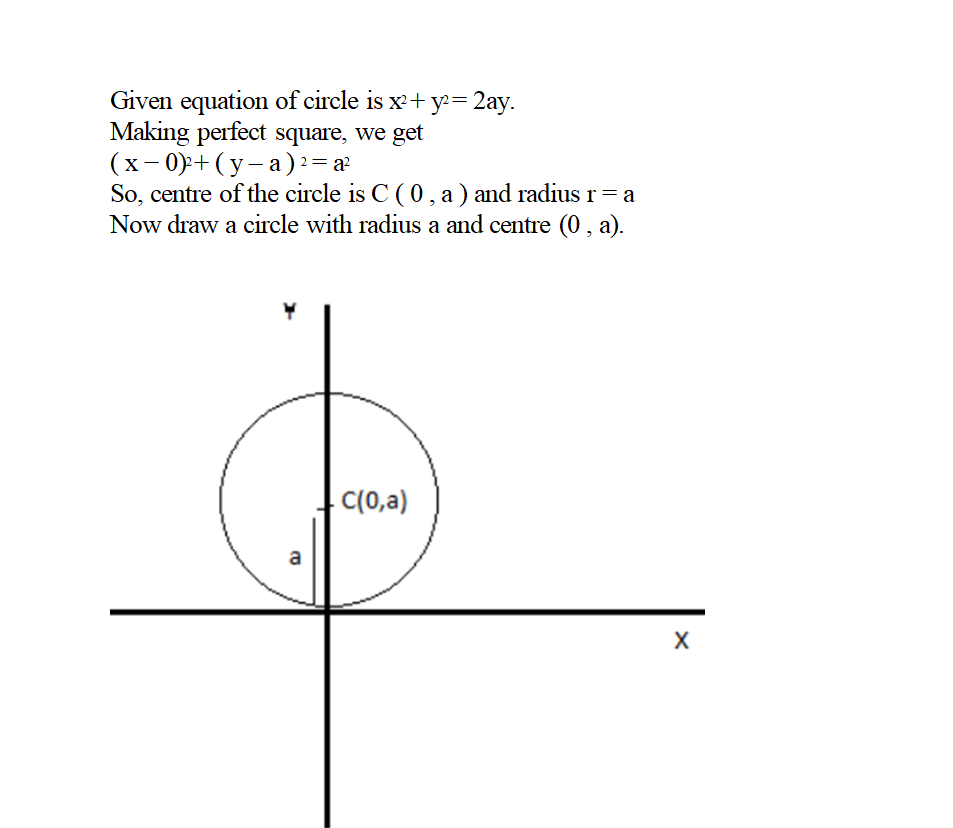
Draw the circles whose equations are x^{2} + y^{2} = 2ay.
Construct \angle AOB=85^o with the hepl of a protector. Draw a ray OX bisecting \angle AOB.
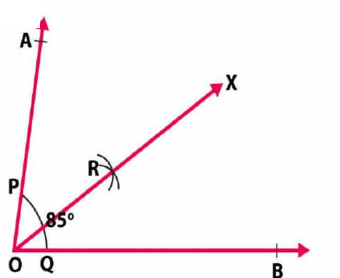
Draw an angle of 50^o with the help of a protractor. Draw a ray bisecting this angle.

Draw a line AB. Take a point P on it. Draw a line passing through P and perpendicular to AB
Construct each of the following angles, using ruler and compasses:
105^o
Construct each of the following angles, using ruler and compasses:
22.5^o
Construct each of the following angles with the help of a protractor:
180^{o}
Construct each of the following angles with the help of a protractor:
48^{o}
Using a pair of compasses construct the following angles:
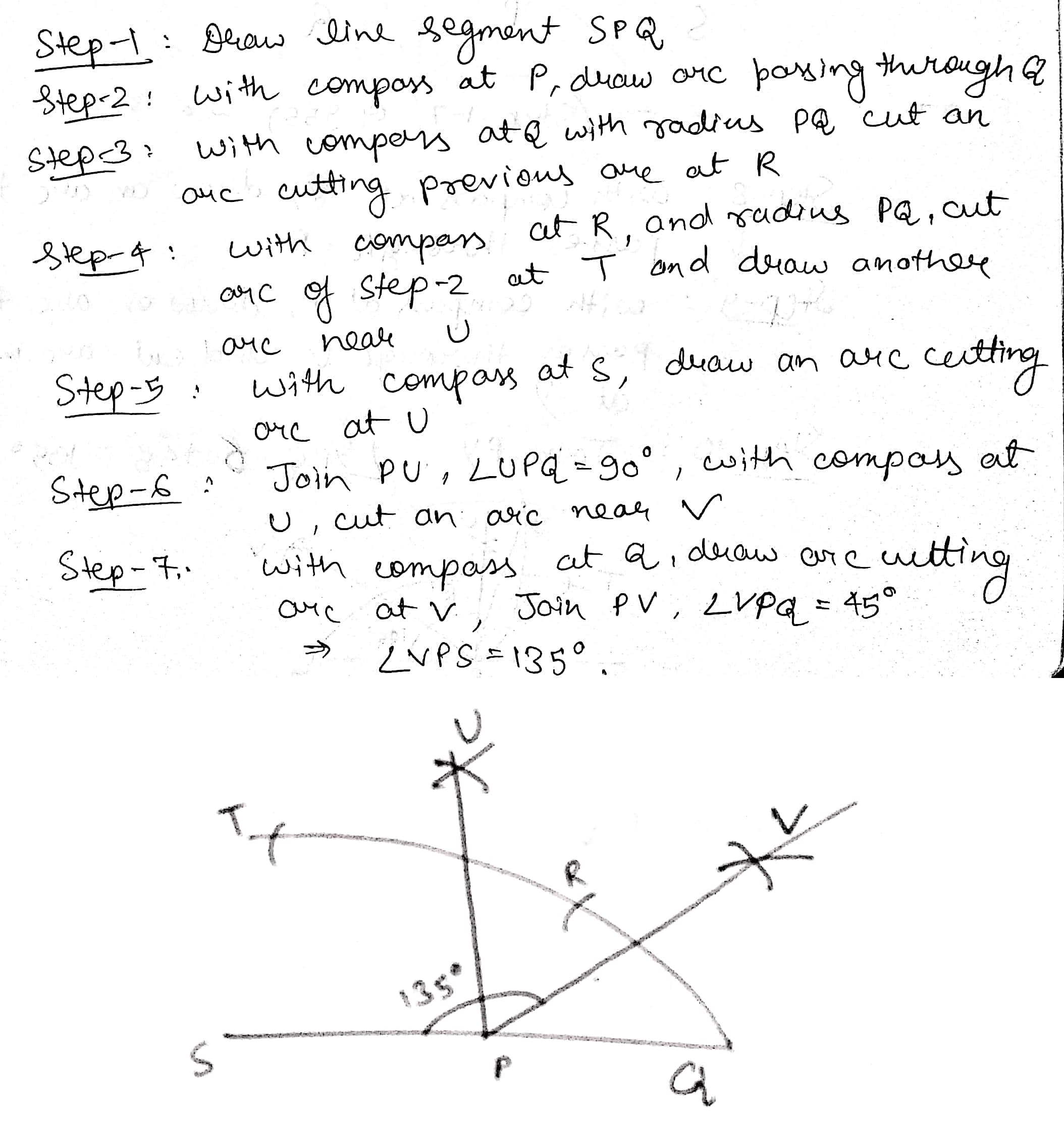
90^o
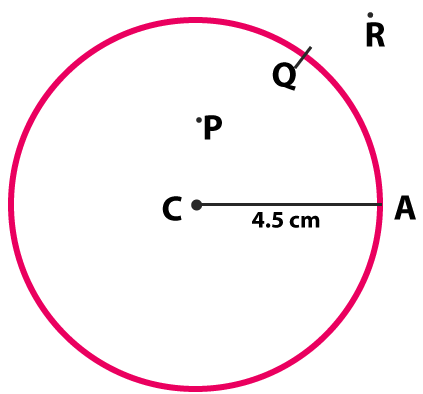
Draw a circle with centre C and radius 4.5\, cm. Mark points P, Q, R such that P lies in the interior of the circle, Q lies on the circle, and R lies in the exterior of the circle.
Construct the following angle with the help of a protractor:
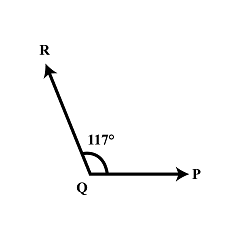
117^{o}
Using a pair of compasses construct the following angles:
60^o
Construct each of the following angles with the help of a protractor:
23^{o}
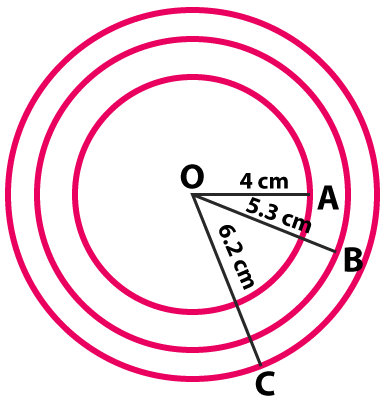
Take a point O on your notebook and draw circles of radii 4\, cm, 5.3\, cm and 6.2\, cm, each having the same centre O.
Using a pair of compasses construct the following angles:
120^o
Construct each of the following angles with the help of a protractor:
90^{o}
In the diagram, A, B and C are fixed collinear points; D is a fixed point outside the line. Locate:
Are the points P, Q, R collinear?
Without using set-squares or protractor construct:
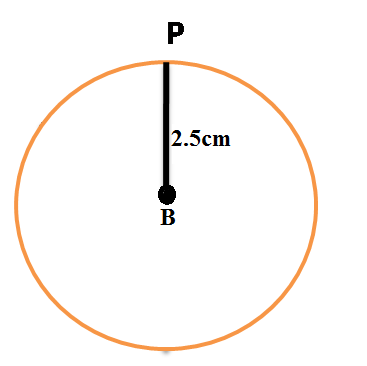
Draw the locus of a point which moves so that it is always 2.5 cm from B.
Draw a circle of radius 6 cm. From a point 10 cm away from its centre, construct a pair of tangents to the circle and measure their lengths.
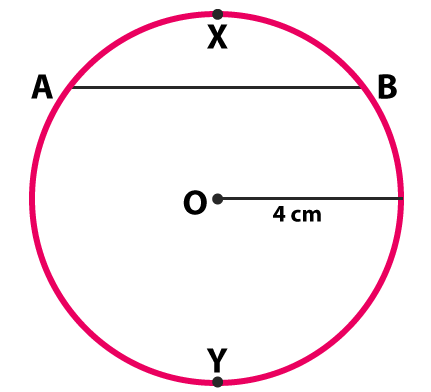
Draw a circle with centre O and radius 4\, cm. Draw a chord AB of the circle. Indicate by marking points X and Y, the minor arc AXB and the major arc AYB of the circle.
In the diagram, A, B and C are fixed collinear points; D is a fixed point outside the line. Locate:
the points S such that CS = DS and S is 4 cm away from the line CD. How many such points are possible?

In the diagram, A, B and C are fixed collinear points; D is a fixed point outside the line. Locate:
the points R on AB such that DR = 4 cm. How many such points are possible?
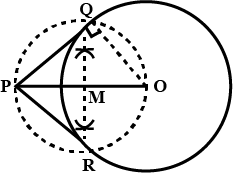
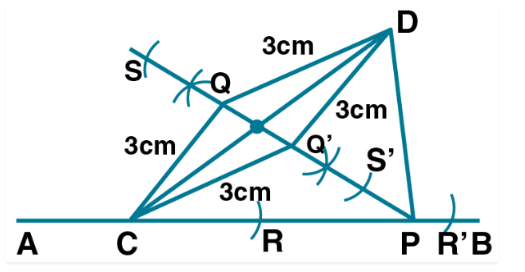
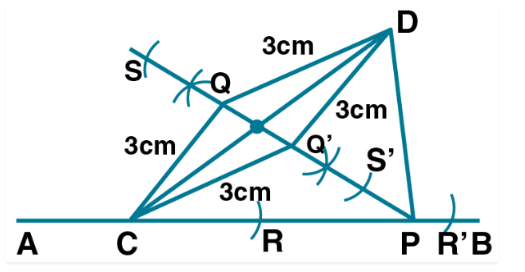
Use ruler and compasses only for this question. Draw a circle of radius 4 cm and mark two chords AB and AC of the circle of length 6 cm and 5 cm respectively.
Construct the locus of points, inside the circle, that are equidistant from AB and AC.
There are two set-squares in your geometry box. What are measures of the angles formed at their corners? Do they have any measure that is common?
A straight line AB is 8 cm long. Locate by construction the locus of a point which is:
Mark two points X and Y, which are 4 cm from AB and equidistant from A and B.
In the diagram, A, B and C are fixed collinear points; D is a fixed point outside the line. Locate:
Are the points P, Q, S collinear?
Draw an angle of 60^{\circ} using ruler and compasses and divide it into four equal parts. Measure each part.
Draw two acute angles and one obtuse angle without using a protractor. Estimate the measures of the angles. Measure them with the help of a protractor and see how much accurate is your estimate
Bisect a straight angle , Using ruler and compasses. Measure each part.
Draw an angle of 140^{o} with help of a protractor and bisect it using ruler and compasses.
Bisect a right angle, using ruler and compasses. Measure each part. Bisect each of these parts. What will be the measure of each of these parts What will be the measure of each of these parts
Draw an angle ABC of measure 45^{\circ} , using ruler and compasses. Now draw an angle DBA of measure 30 o, using ruler and compasses as shown in Fig, 9.14, What is the measure of \angle DBC
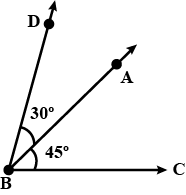
Draw an angle of 80^{\circ} with the help of a protractor . Then construct angles of (i) 40^{\circ} (ii) 160^{\circ} and (iii) 120^{\circ} .
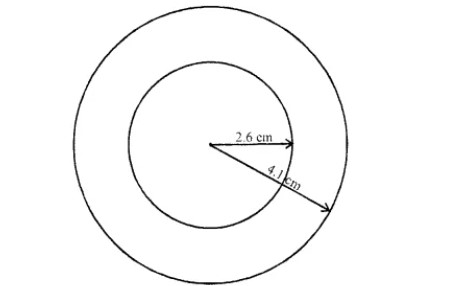
With the same centre O, draw two circles of radii 2.6 cm and 4.1 cm.
Prove that through a given point , we can draw one perpendicular to a given line.
[Hint : Use proof by contradiction].
Construct a circle of radius:
3.5 cm
Draw an angle of 110^{\circ} with the help of a protractor and bisect it.
Construct a circle of radius:
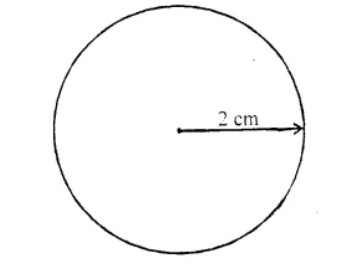
2 cm
Draw a circle of radius 3 \,cm and draw its diameter and label it as AC. Construct its perpendicular bisector and let it intersect the circle at B and D. What type of quadrilateral is ABCD?
Draw a circle of radius 4 \,cm. Construct a pair of tangents to it, the angle between which is 60^\circ. Also, justify the construction. Measure the distance between the centre of the circle and the point of intersection of tangents.
Construct \overset{\_}{AB} of length 8.3 cm. From this cut off \overset{\_}{AC} of length 5.6 cm. Measure the length of BC...
Construct a line segment of the length of 7.3 cm using ruler and compass.
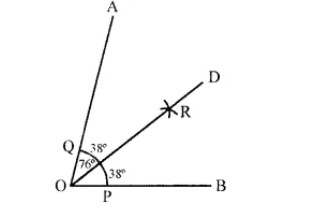
Draw \angle{AOB} = 76^\circ with the help of a protractor. Bisect this angle by using a ruler and compass. Measure the two parts by your protractor and see how accurate you are.
Draw a circle with centre C and radius 4.2 cm. Draw any chord AB. Construct the perpendicular bisector of AB and examine if it passes through C.
Draw a line segment \overline{PQ} =5.6 cm. Draw a perpendicular to it from a point outside \overline{PQ} by using ruler and compass.
Draw a line segment AB = 5.4\ \text{cm}. Construct perpendicular at A by using a ruler and compass.
Draw a line segment \overline{AB} = 6.2 cm. Draw a perpendicular to it at a point M on \overline{AB} by using ruler and compass.
By using and compass, construct an angle of 135^\circ and bisect it. Measure any one part by protractor and see how accurate you are.
Draw the perpendicular bisector of \overline{XY} whose length is 8.3 cm.
{i} Take any point P on the bisector drawn. Examine whether PX = PY.
(ii) If M is the mid-point of XY, what can you say about the lengths MX and MY?
Draw a line segment PQ = 6.8 cm. Draw a perpendicular to it from a point A outside PQ by using ruler and Compass.
Draw the plan for the information given below:
Scale 20 m=1cm
| Metre To C | ||
To D 50 To E 30 | 140 100 60 40 | 40 to B |
| From A |
Draw a circle of radius 6\ cm. In case circle, draw a chord AB=6\ cm. Assign a special name to \triangle AOB
In your note-book copy the following angles using ruler and a pair compass only.
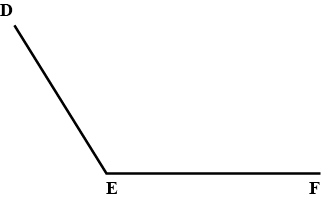
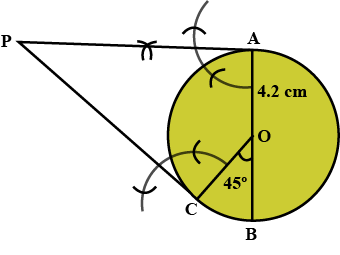
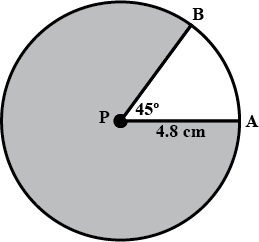
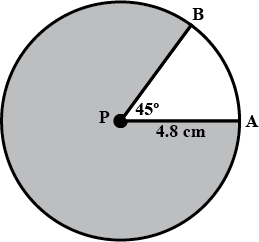
Draw a circle of radius 4.8\ cm and mark its centre as P
Draw radii PA and PB such that \angle APM=45^o
In your note-book copy the following angles using ruler and a pair compass only.
Draw a circle of radius 3.6\ cm. In the circle, draw a chord AB=5\ cm. Now shade the minor segment of the circle.
Draw a circle of radius 4.8\ cm and mark its center as PDraw radii PA and PB such that \angle APM=45^o
Shade the major sector of the circle.
Shade the major sector of the circle.
Draw a circle of radius 3 \,cm . Draw any diameter of the circle. AT the end points of the diameter of the circle , draw tangents to the circle. Any they parallel ?
Draw a circle of radius 4.2\ cm. Mark its centre as O. Take a point A on the circumference of the circle. Join AO and extend it till it meets point B on the circumference of the circle,
Assign a special name to AB
Draw a circle of radius 6\ cm. In case circle, draw a chord AB=6\ cm. Write the measure of angle AOB
Using ruler and compasses, construct the following angles:
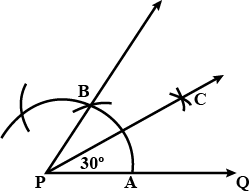
30^o
In your note-book copy the following angles using ruler and a pair compass only.
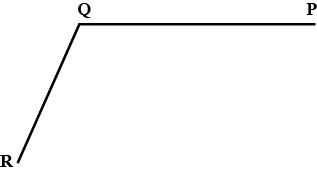
Draw a line segment OP = 8 \,cm. Use set-square to construct \angle POQ = 90^\circ; such that OQ = 6 \,cm. Join P and Q; then measure the length of PQ.
Draw a line segment OA = 5 \,cm. Use set-square to construct angle AOB = 60^\circ, such that OB = 3 \,cm. Join A and B; then measure the length of AB.
Using ruler and compasses, construct the following angles:
75^o
Using ruler and compasses, construct the following angles:
15^o
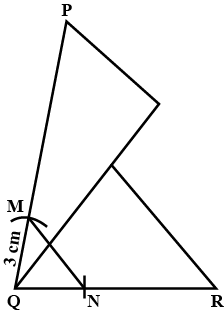
Draw a line AB = 6 \,cm. Construct angle ABC = 60^\circ. Then draw the bisector of angle ABC.
Draw \angle ABC = 120^\circ. Bisect the angle using ruler and compasses. Measure each angle so obtained and check whether or not the new angles obtained on bisecting \angle ABC are equal.
Draw \angle PQR = 75^\circ by using set-squares. On PQ mark a point M such that MQ = 3 \,cm. On QR mark a point N such that QN = 4 \,cm. Join M and N. Measure the length of MN.
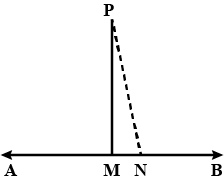
Draw a line segment AB = 7 \text{ cm}. Mark a point AB such that AP = 3 \text{ cm}. Draw perpendicular on to AB at point P.
Using ruler and compasses only,Construct a triangle ABC with a following data:Base\ AB=6\ cm,\ BC=6.2\ cm and \angle CAB=60^oProve that AD = BD
Construct a triangle ABC with a following data:
Base\ AB=6\ cm,\ BC=6.2\ cm and \angle CAB=60^o
Prove that AD = BD
Using ruler and compasses only,
Construct a triangle ABC with a following data:Base\ AB=6\ cm,\ BC=6.2\ cm and \angle CAB=60^oDraw a perpendicular from O to AB which meets AB in D .
Construct an angle of 75^o and then bisect it.
Using ruler and compasses, construct the following angles:
165^o
Draw a line segment PQ=6 cm . Mark a point A in PQ so that AP=2 cm . At point A , construct angle QAR=60^o .
Using ruler and compasses, construct the following angles:
180^o
Draw a line segment of length 6.4\;cm. Draw its perpendicular bisector.
Draw a line segment AM=5.8 cm. Mark a point P in AB such that PB=3.6 cm At P , draw perpendicular to AB .
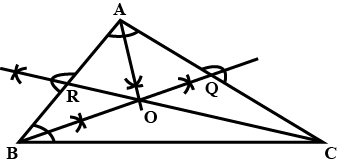
The bisector of angle A and B a scalene triangle ABC meet at O
OR and OQ are draw perpendicular to AB and CA respectively . What is the relation between OR and OQ ?
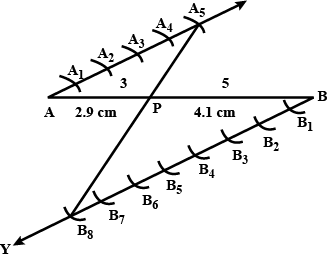
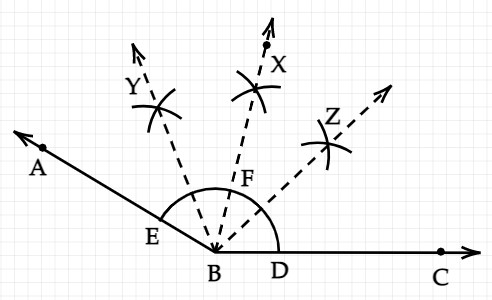
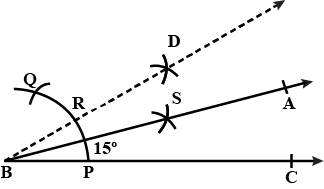
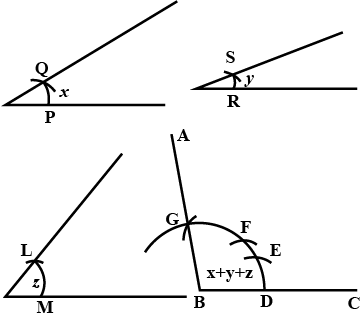
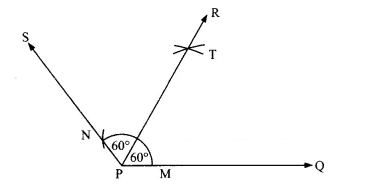
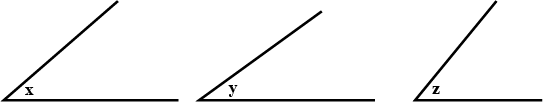
Given below are the angles x,y and z.
Without measuring these angles construct:
\angle ABC=x+y+z
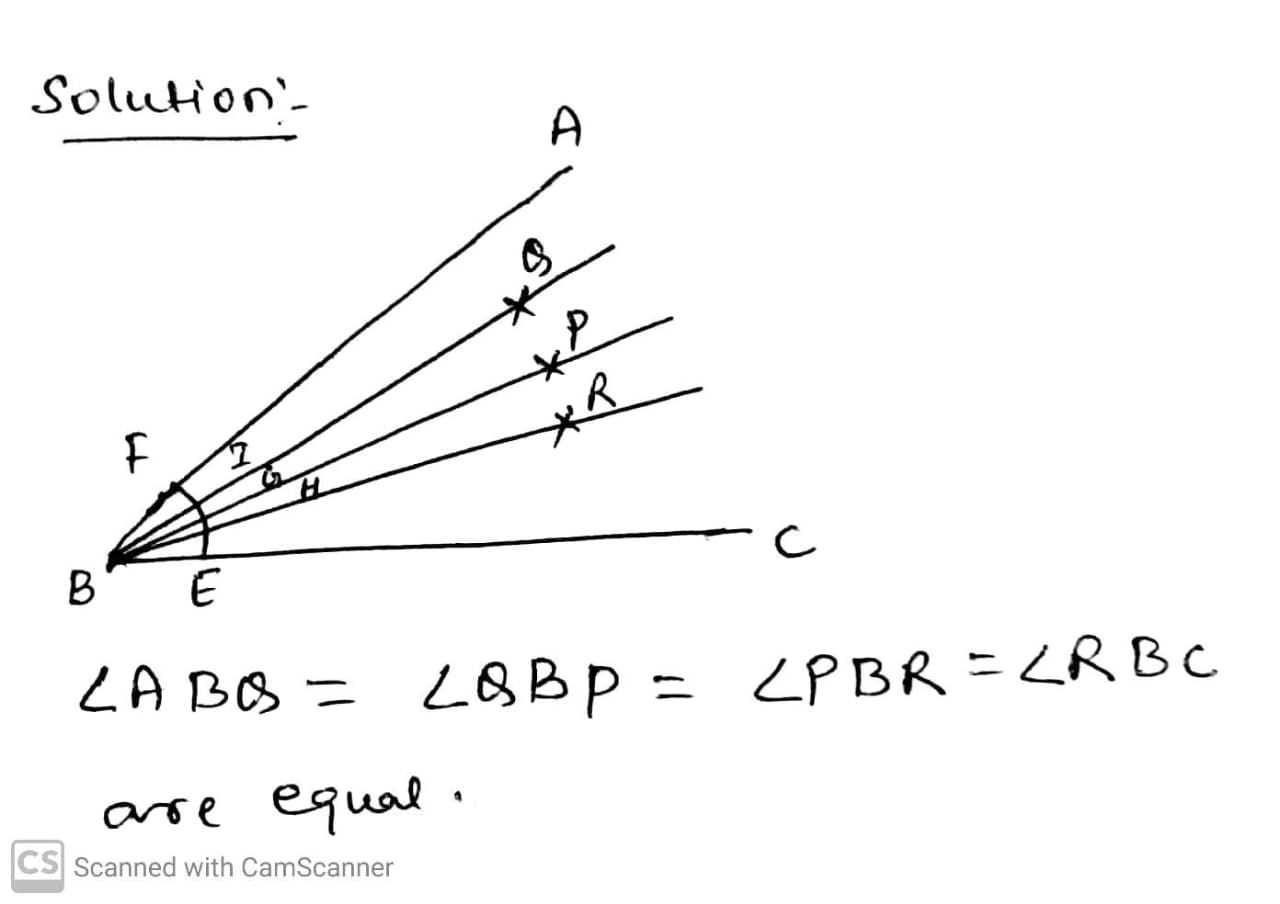
Draw angle ABC of any suitable measure.
Are the angles ABQ and QBC equal?
In each of the following, draw perpendicular through point P to the line segment AB.

Construct angle ABC=90^{\circ}. Draw BP, the bisector of angle ABC. State the measure of angle PBC.
Draw a line segment PQ=4.8cm. Construct the perpendicular bisector of PQ.
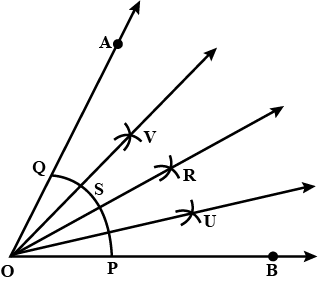
Draw angle ABC of any suitable measure.
(i) Draw BP, the bisector of angle ABC.
(ii) Draw BR, the bisector of angle PBC and draw BQ, the bisector of angle ABP
(iii) Are the angles ABQ,QBP,PBR and RBC equal?
(iv) Are the angles ABQ and QBC equal?
In each of the following, draw perpendicular through point P to the line segment AB.

In each of the following, draw perpendicular through point P to the line segment AB.

Draw angle ABC of any suitable measure.
Are the angles ABQ,QBP,PBR and RBC equal?
Draw a line segment AB=6.2\ cm Mark a point P, in AB such that BP=4\ cm through point P draw perpendicular to AB.
Construct an angle PQR=80^o. Draw a line parallel to PQ at a distance of 3cm from it and another line parallel to QR at a distance of 3.5cm from it. Mark the point of intersection of these parallel lines as A.
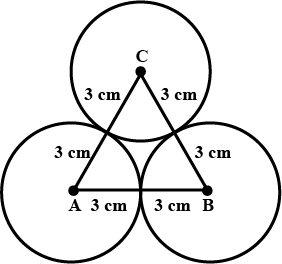
Draw circles with centers A, B and C each of radius 3 cm, such that each circle touches the other two circles.
Draw a circle of radius 3.6 cm. Draw a tangent to the circle at any point on it without using the centre.
Draw a circle of radius 2.7 cm. Draw a tangent to the circle at any point on it.
Draw rough diagrams of two angles such that they have Four points in common.
Draw rough diagrams of two angles such that they have One point in common.
Draw a line segment A\ B=5.5cm Mark a point P, such that P\ A=6cm and P\ B=4.8cm. From the point P draw perpendicular to AB.
Draw a circle of diameter 6.4 cm. Take a point R at a distance equal to its diameter from the centre. Draw tangents from point R .
Draw rough diagrams of two angles such that they have Two points in common.
Construct an angle of 45^0 at the initial point of a given ray and justify the construction.
Construct the following angles and verify by measuring them by a protractor :
(i) 75^0
Draw a line segment of length 9.5\,cm and construct its perpendicular bisector.
Construct the following angles and verify by measuring them by a protractor :
(i) 135^0
Construct an angle of 90^0 at the initial point of a given ray and justify the construction.
Construct the angles of the following measurements:
(i) 22 \frac{1}{2}^0
Construct an angle of the 30^0 measurement.
Draw rough diagrams of two angles such that they have One ray in common.
Construct the following angles and verify by measuring them by a protractor :
(i) 105^0
Construct the angles of the following measurements
(i) 15^0
Draw a circle with centre C and radius 3.4\,cm. Draw any chord \overline {AB}. Construct the perpendicular bisector of \overline {AB} and examine if it passes through C, if \overline {AB} happens to be a diameter.
Draw a line segment of length 6 cm. From a point P outside the line, draw a perpendicular on this line.
Construct \angle ABC = 120^o. Through A draw a line parallel to BC.
To a circle of radius 4.5, draw two tangents such that the angle between them is 45^\circ, also verify it.
Draw a line segment AB = 10 cm. Bisect this line segment and verify by measuring the lengths of the two segments.
Construct an angle 120^o. Bisect angle and verify by measuring the two angles.
Draw an angle of 40^o using protractor. Now with the help of ruler and compass, construct an angle to this angle.
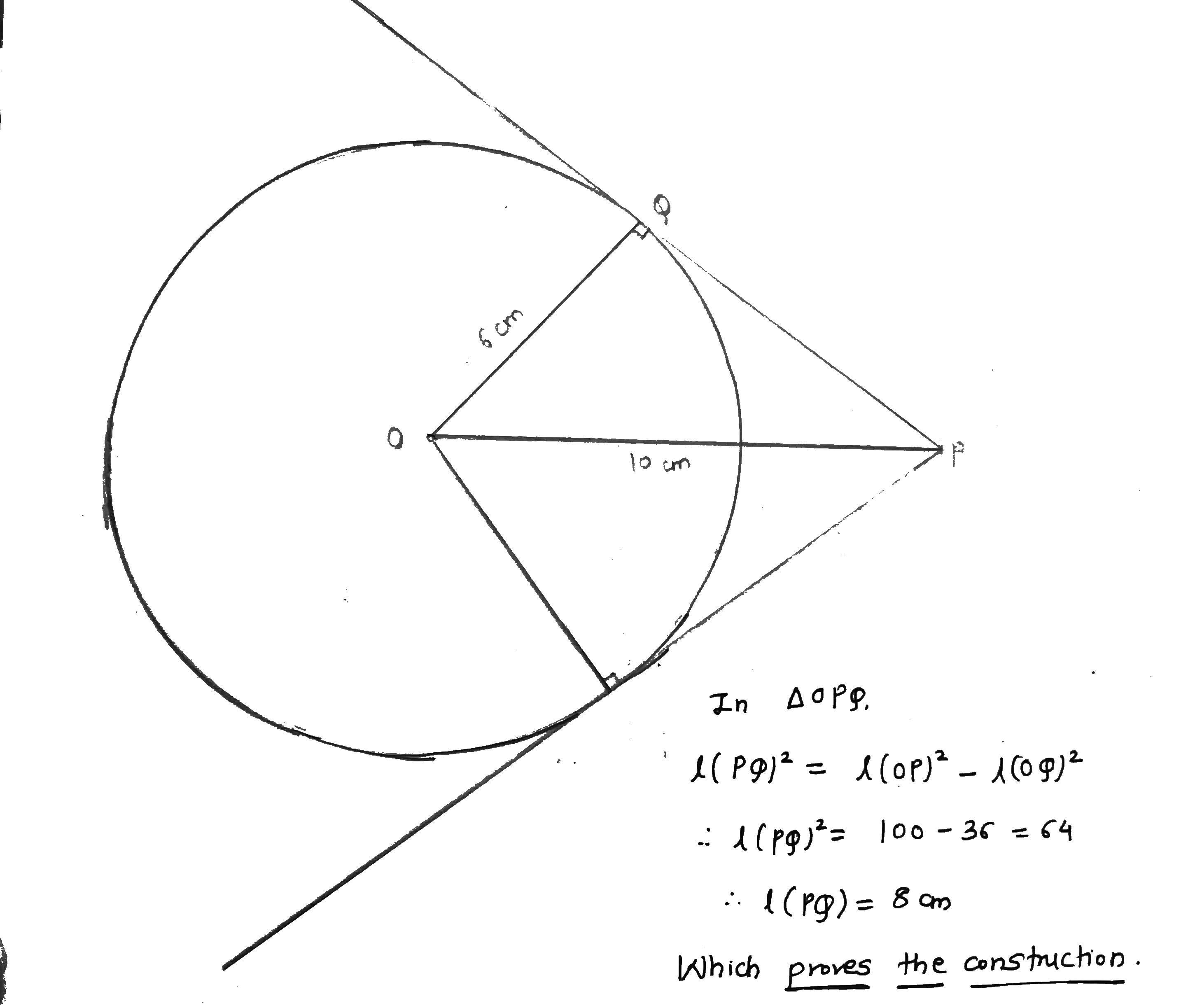
Draw a circle of radius 2.5 cm. Take a point a part 6.5 cm from its center and from this point draw the tangents to the circle. Measure the length of tangent also find the length of tangents by calculation.
Draw a line segment AB of length 85 cm. Taking A and B as centers and radii respectively 5 cm and 3 cm draw two circle. From the center of each circle draw the tangents to the other circle.
With the same center O, draw two circles of radii 4 cm and 2.5 cm.
draw BN=12 CM . divide the line segment BN in four equal parts with the help of compasses a ruler
Construct the angles of the following measurements:
30 ^ { \circ }
15 ^ { \circ }
22.5 ^ { \circ }
Show that of all line segments drawn from a given point not on it. the perpendicular line segment is the shortest.
If the sum of the distance of point from two perpendicular lines in a plane is 1, then prove that its locus is a square.
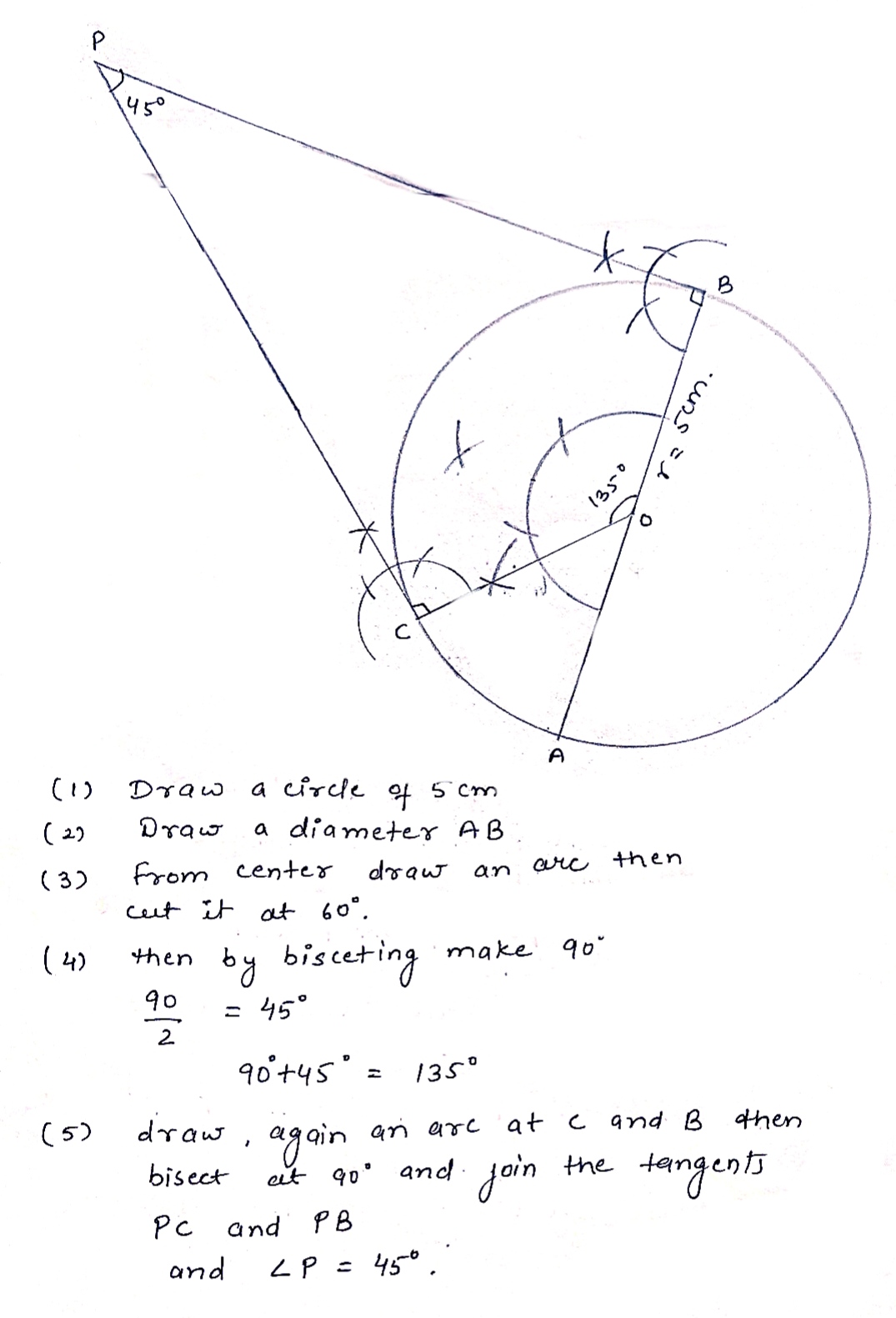
Draw a circle of radius 4\ cm. Draw two tangents to the circle such that they include an angle of 135^{o}.
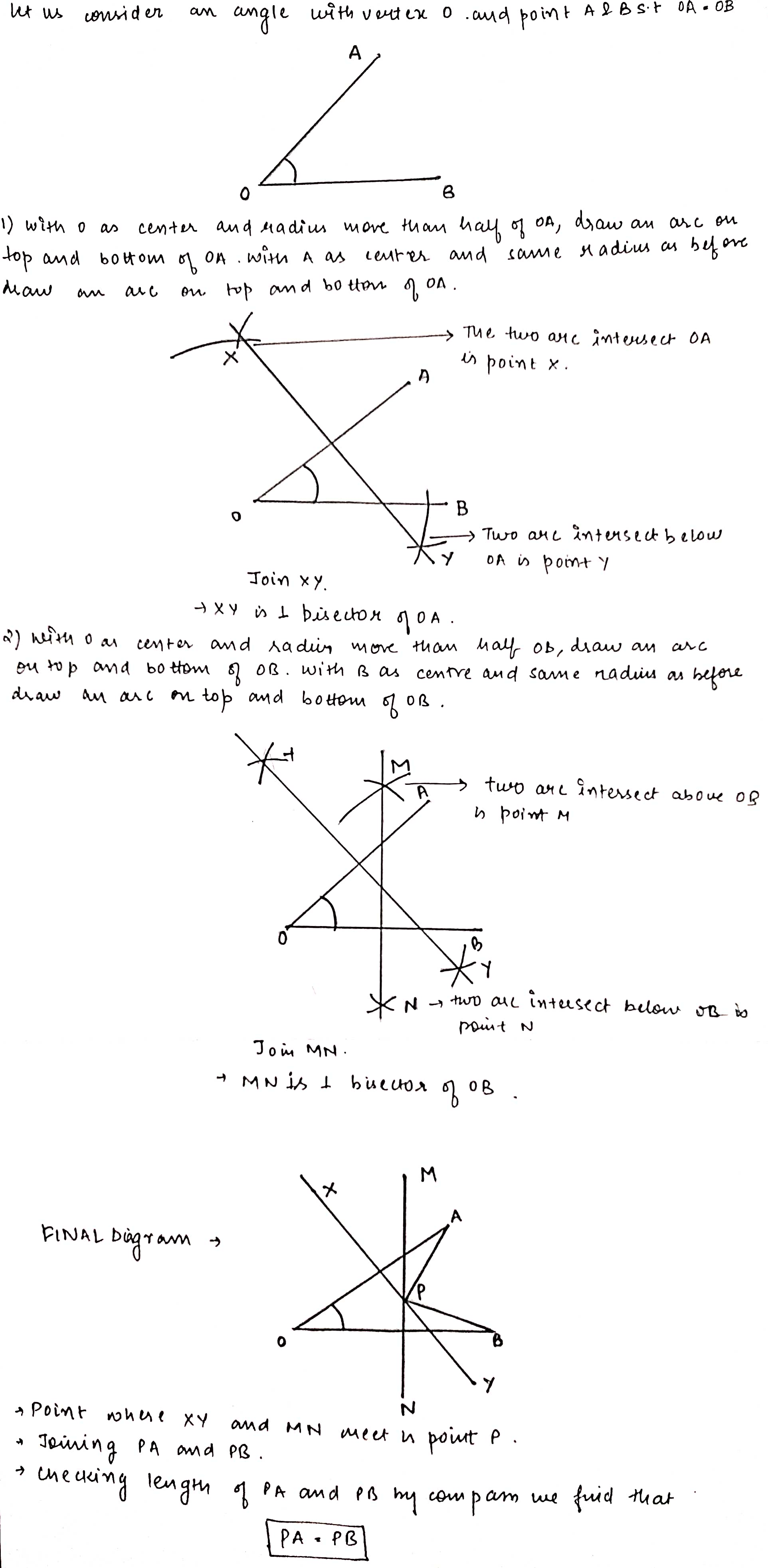
draw any angle with vertex o.take a point A on one of its arms and B on another such that OA=OB. draw the perpendiculr bisectors of \bar { OA } and\bar { OB } let them meet at P.Is PA=PB
Draw a circle of radius 6 cm. From a point 10 cm away from, its centre , construct the pair of tangents to the circle and measure their length
Draw circles for the following radius.
i) 3.5 cm ii) 4.3 cm iii) 4.5 cm
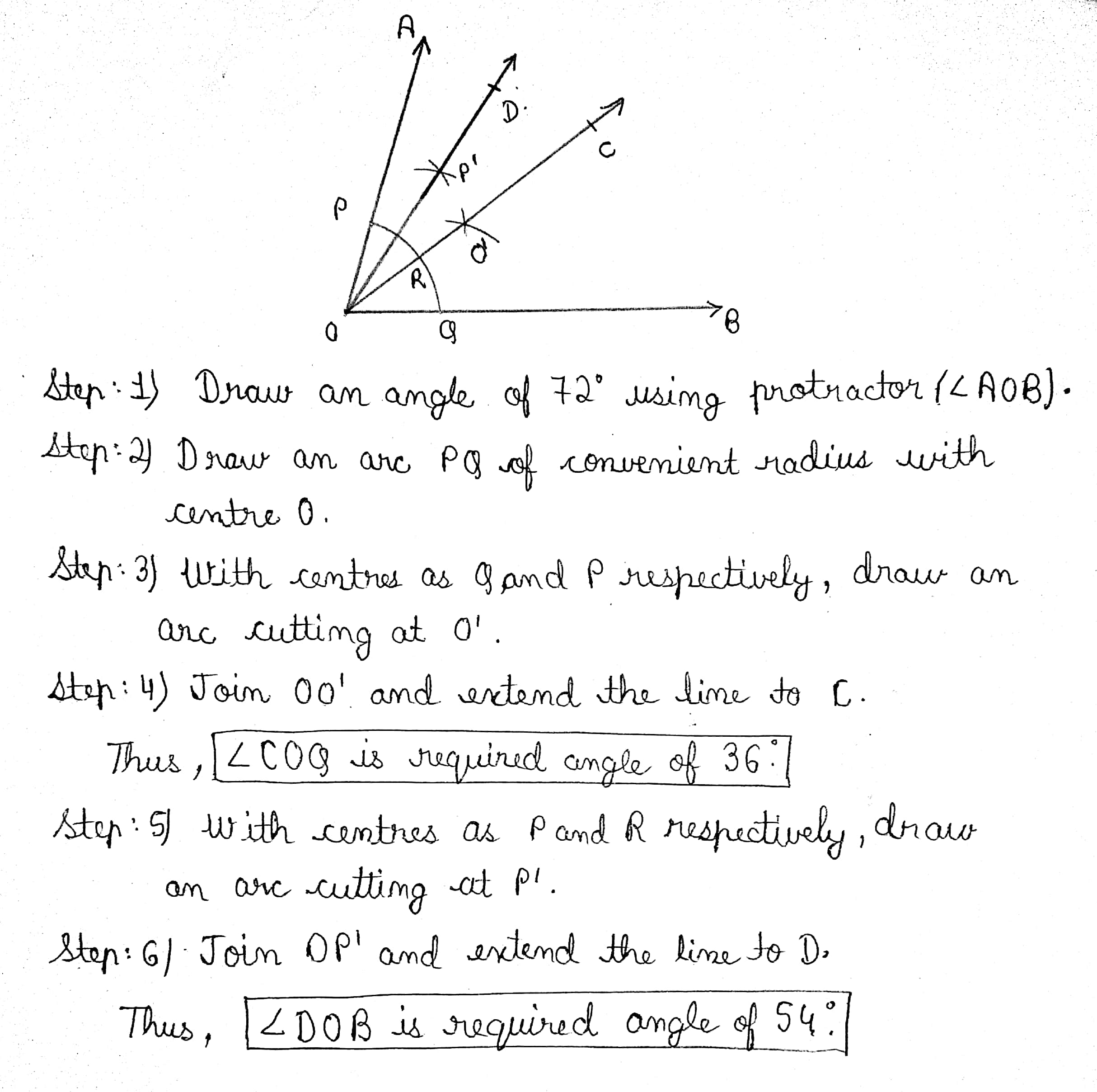
Using a protractor, draw an angle of measure 72^{o}. With this angle as given, draw angles of measure 36^{o} and 54^{o}.
Take a point on your notebook and draw circle of radii 4\ cm,\ 3\ cm and 6.5\ cm, each having the same centre O.
Draw a line segment AB = 5.6 cm. Draw the right bisector of AB.
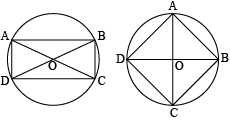
Draw a circle with centre O and any radius. Draw AC and BD two perpendicular diameter on the circle. Join AB, BC, CD and DA.
Construct the angles of the following measurements:
15^{o}
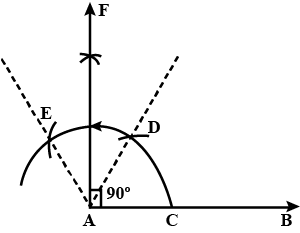
Using ruler and compass, construct a right angle.
Construct the angles of the following measurements:
75^{o}
Construct the angles of the following measurements:
105^{o}
Draw a circle of radius 3 cm. Take two points P and Q on one of its extended diameter each at a distance of 7 cm from its centre. Draw tangents to the circle from these two points P and Q. Give the justification of the construction.
Using a protractor, draw the following angles.
{ -400 }^{ o }
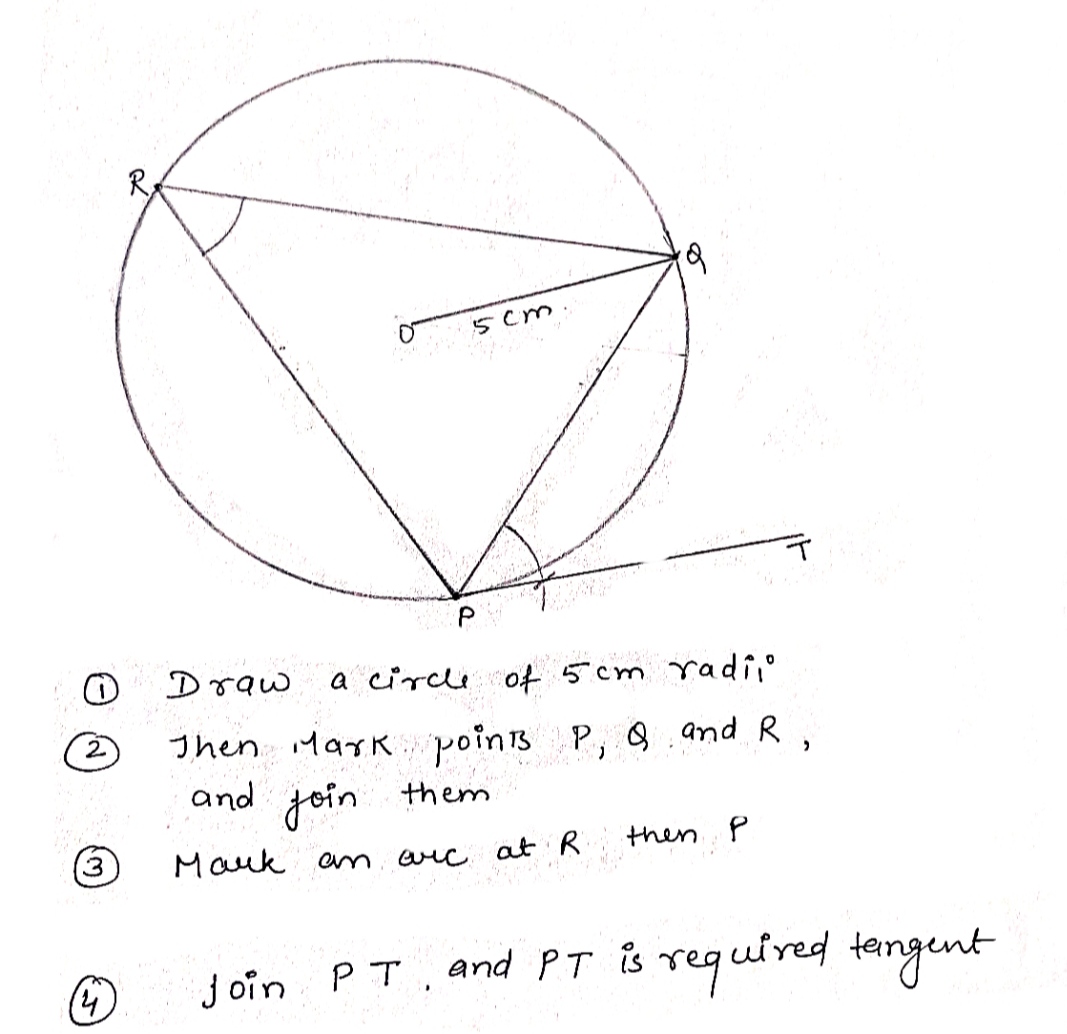
Draw a circle radius 5\,cm . Take a point P on the circle. Draw the tangent of the circle at point P without using the center of the circle.
Draw a circle radius 5 \,cm . Draw any line through the center of the circle. Draw a tangent to the circle making an angle of 45^{\circ} with the line . What is the length of the tangent ?
Using a protractor, draw the following angles.
{ -310 }^{ o }
Using a protractor, draw the following angles.
{ -220 }^{ o }
Class 6 Maths Extra Questions
- Algebra Extra Questions
- Basic Geometrical Ideas Extra Questions
- Data Handling Extra Questions
- Decimals Extra Questions
- Fractions Extra Questions
- Integers Extra Questions
- Knowing Our Numbers Extra Questions
- Mensuration Extra Questions
- Playing With Numbers Extra Questions
- Practical Geometry Extra Questions
- Ratio And Proportion Extra Questions
- Symmetry Extra Questions
- Understanding Elementary Shapes Extra Questions
- Whole Numbers Extra Questions