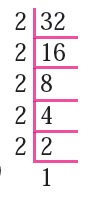
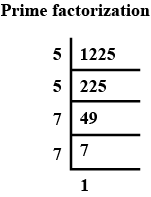
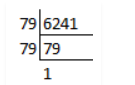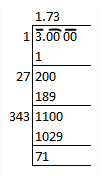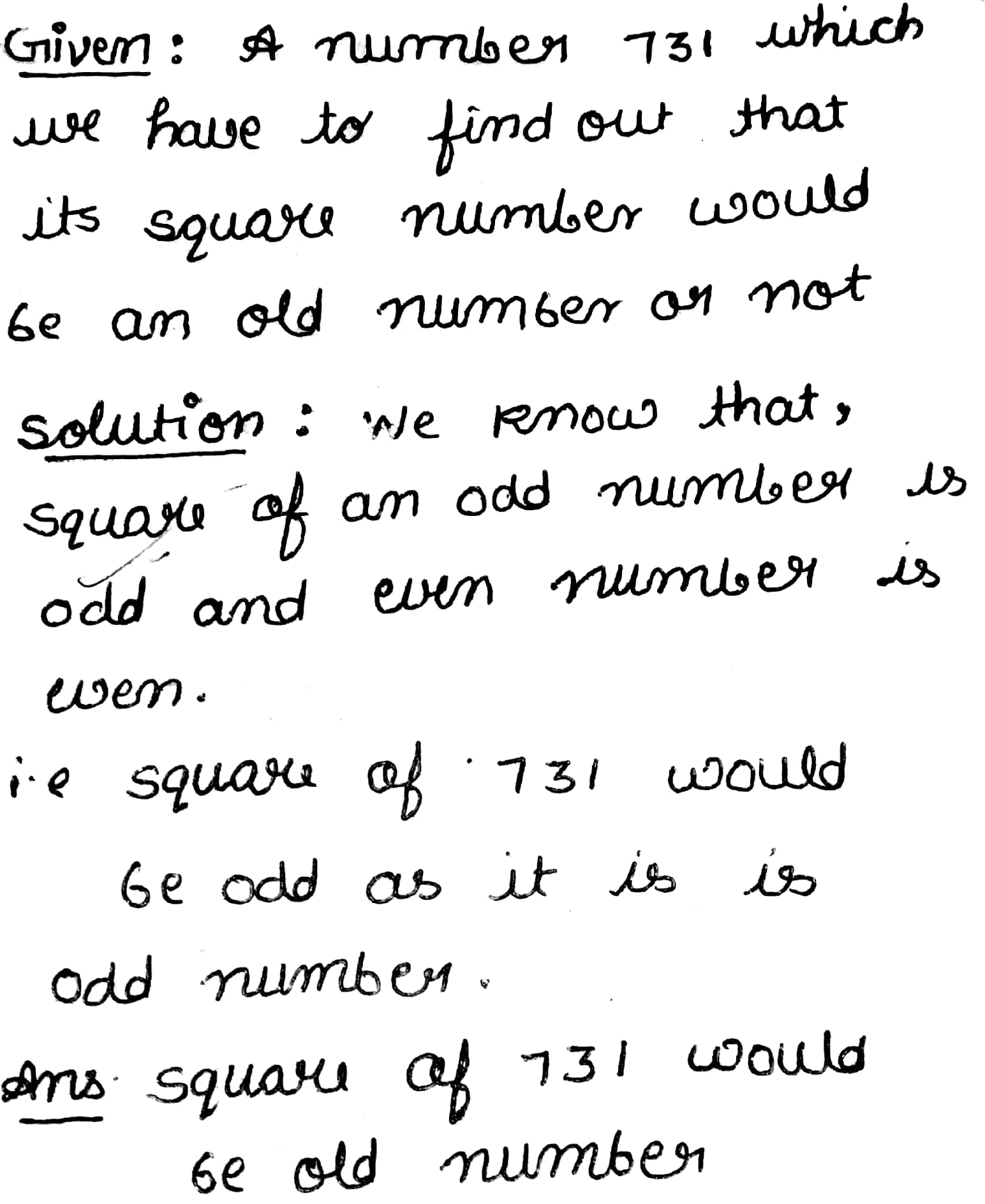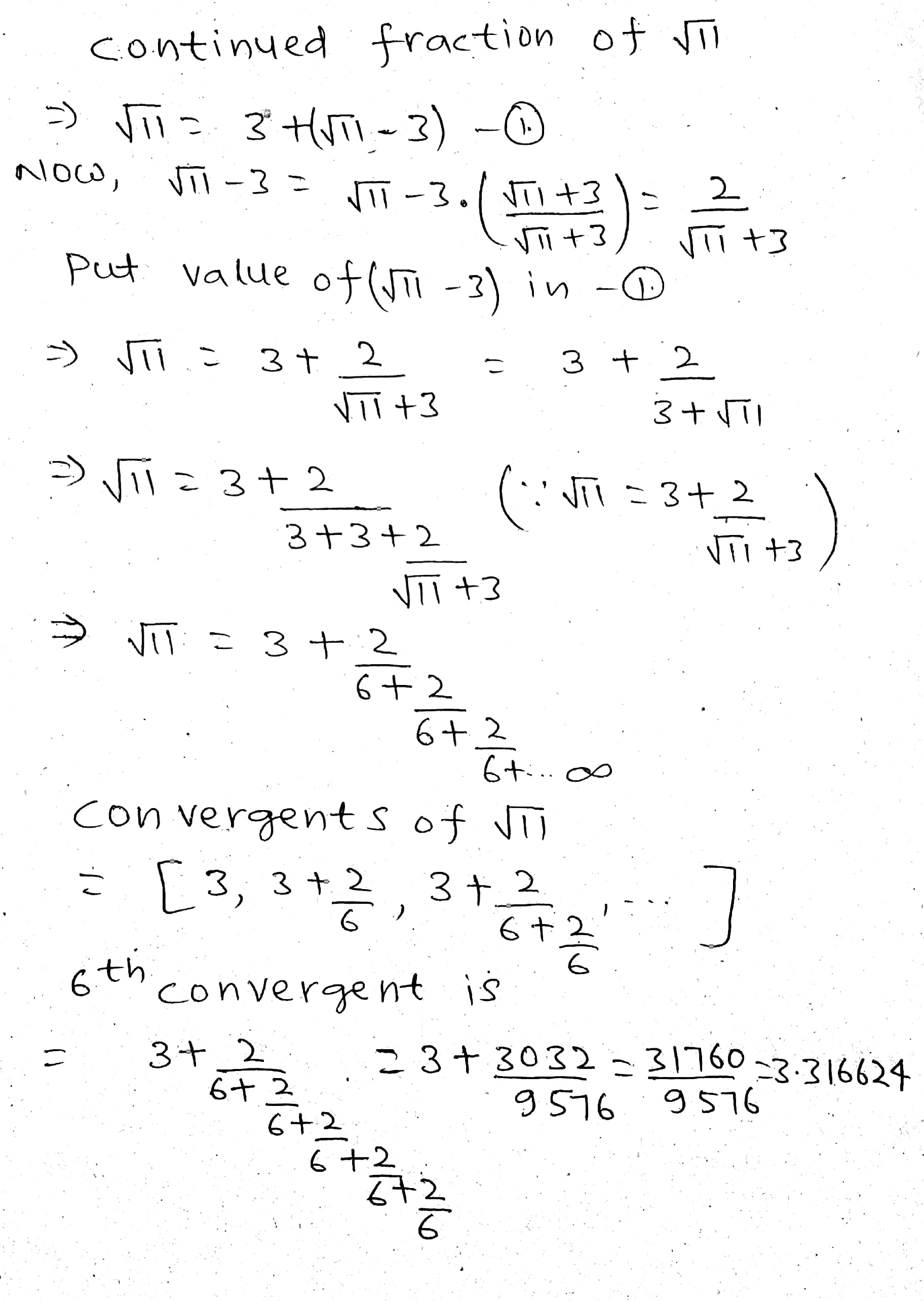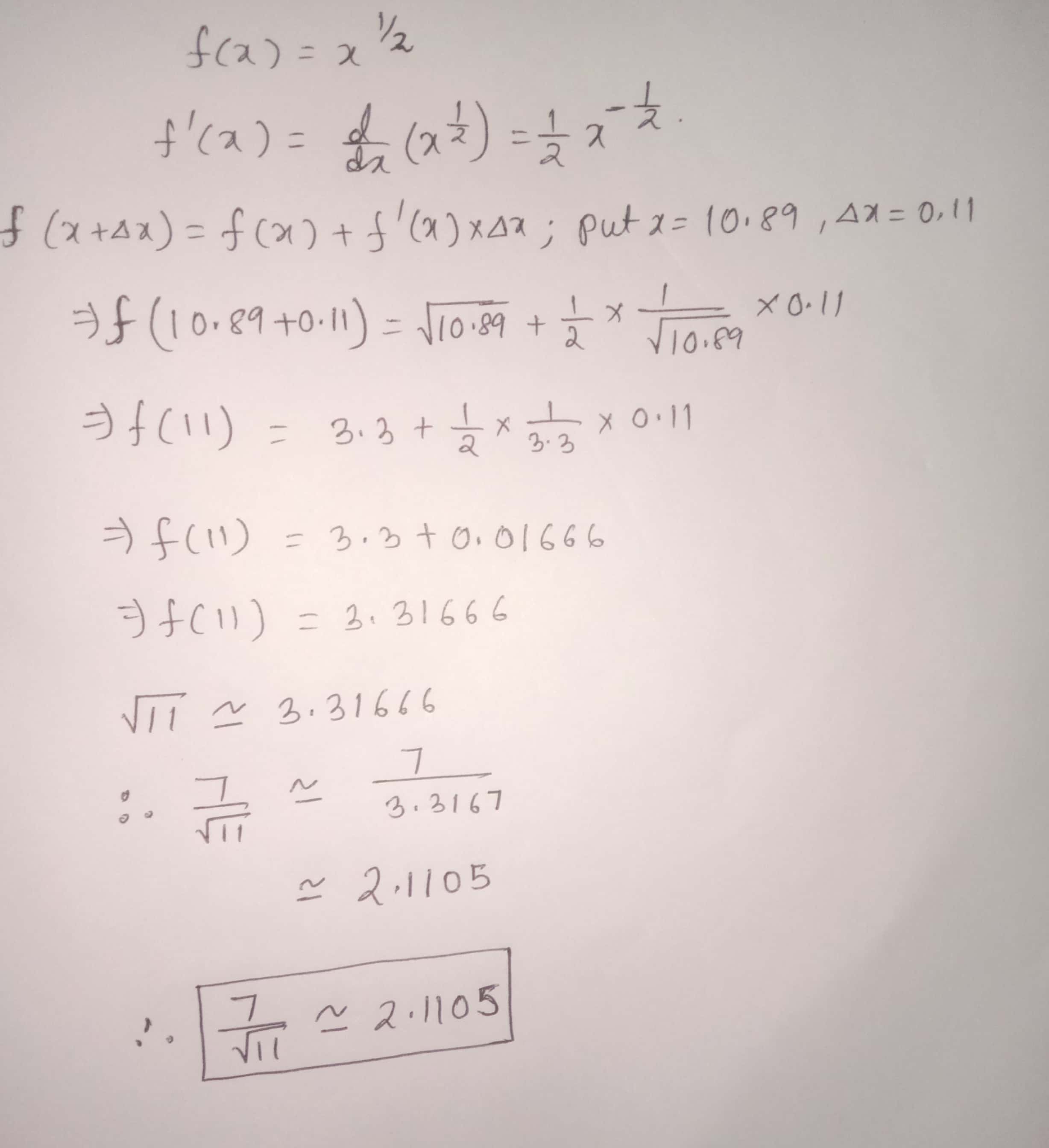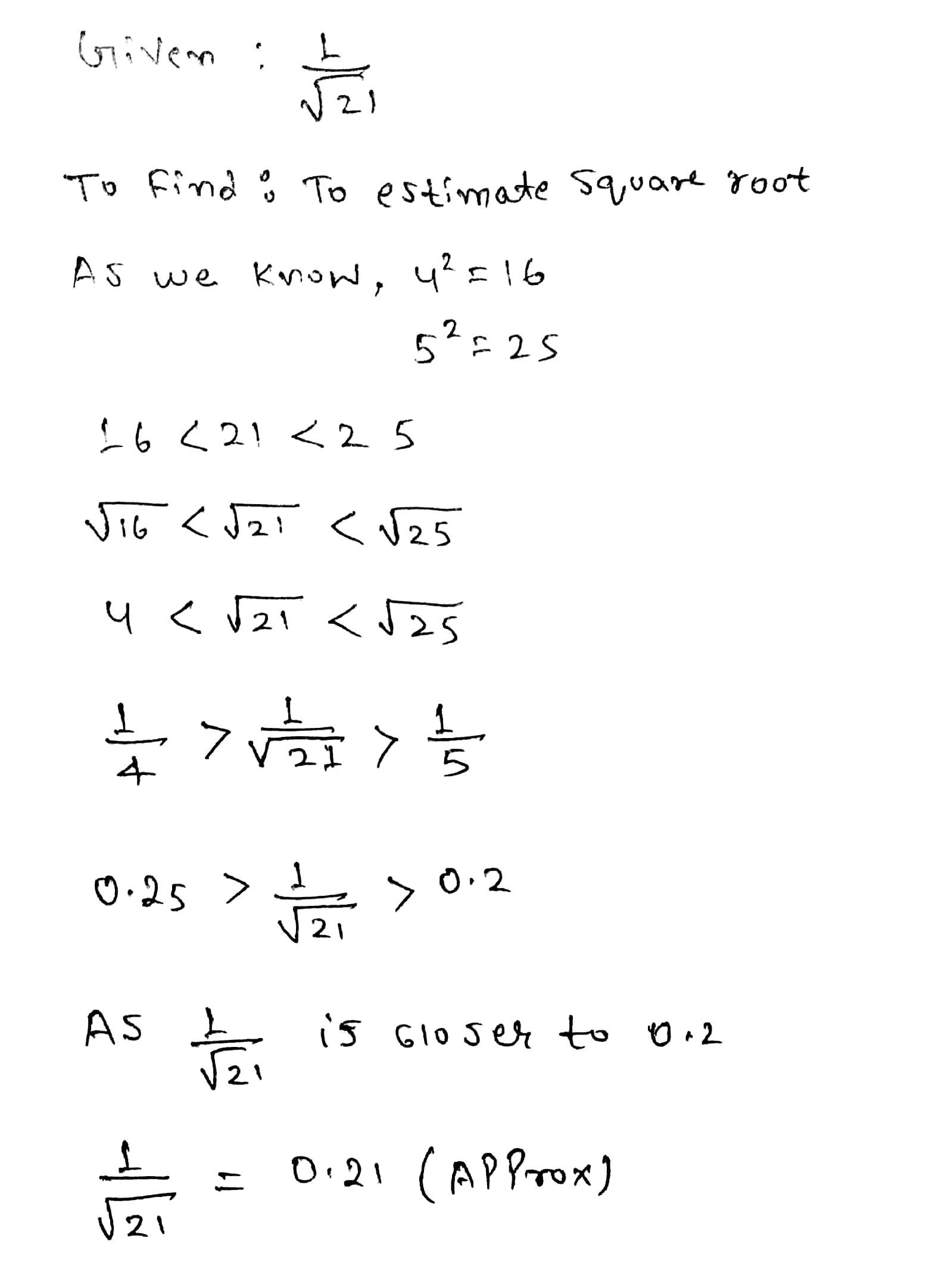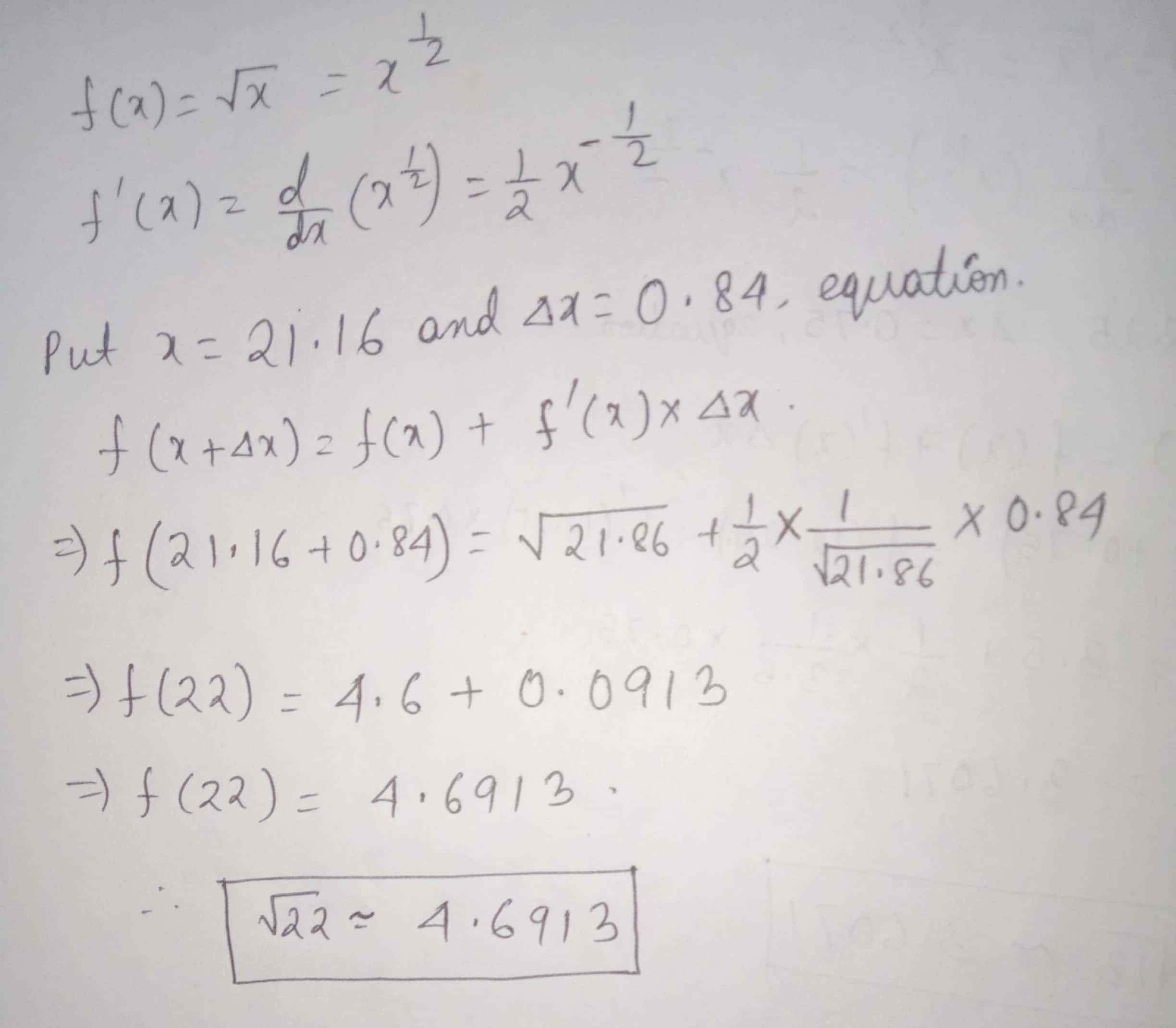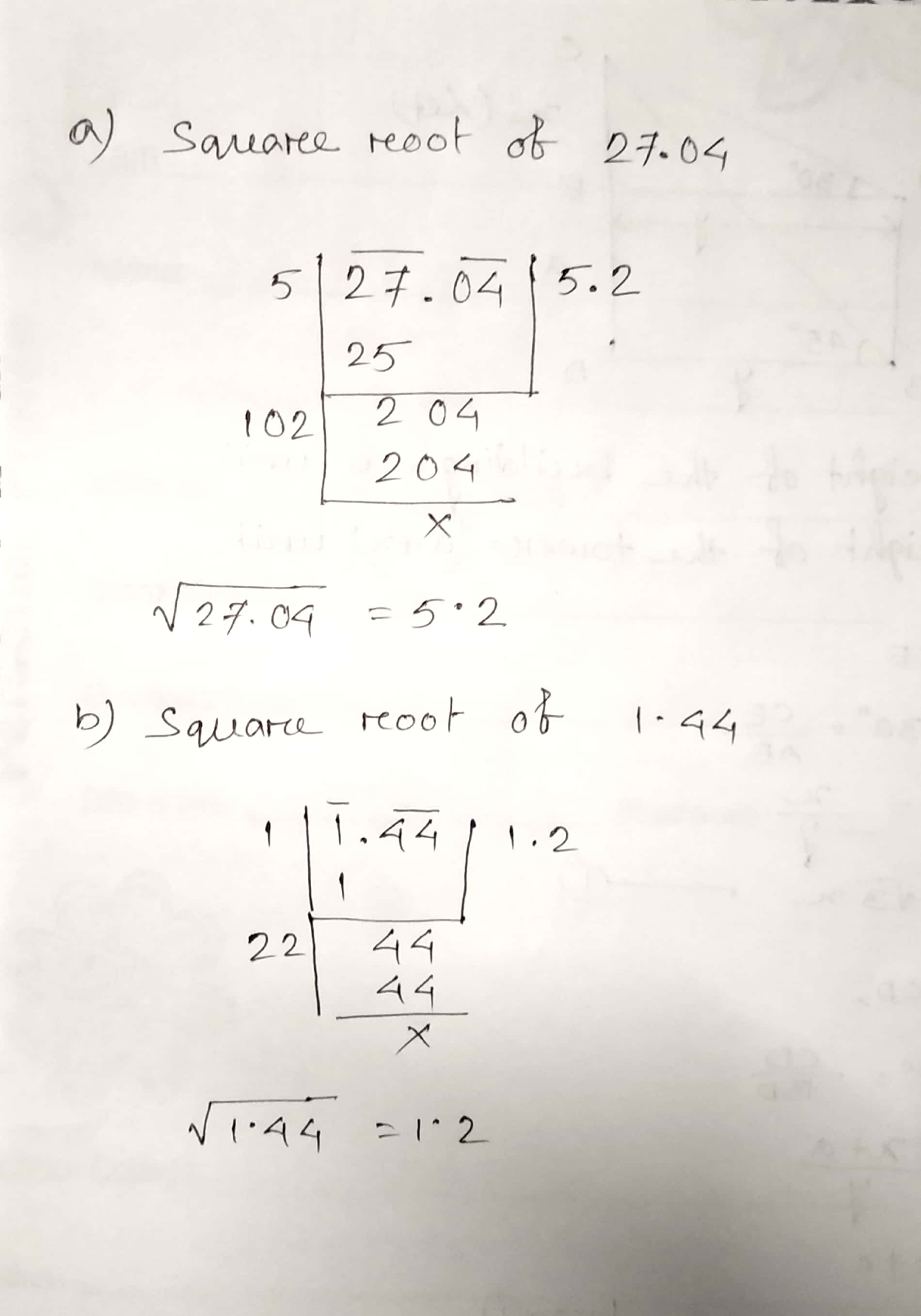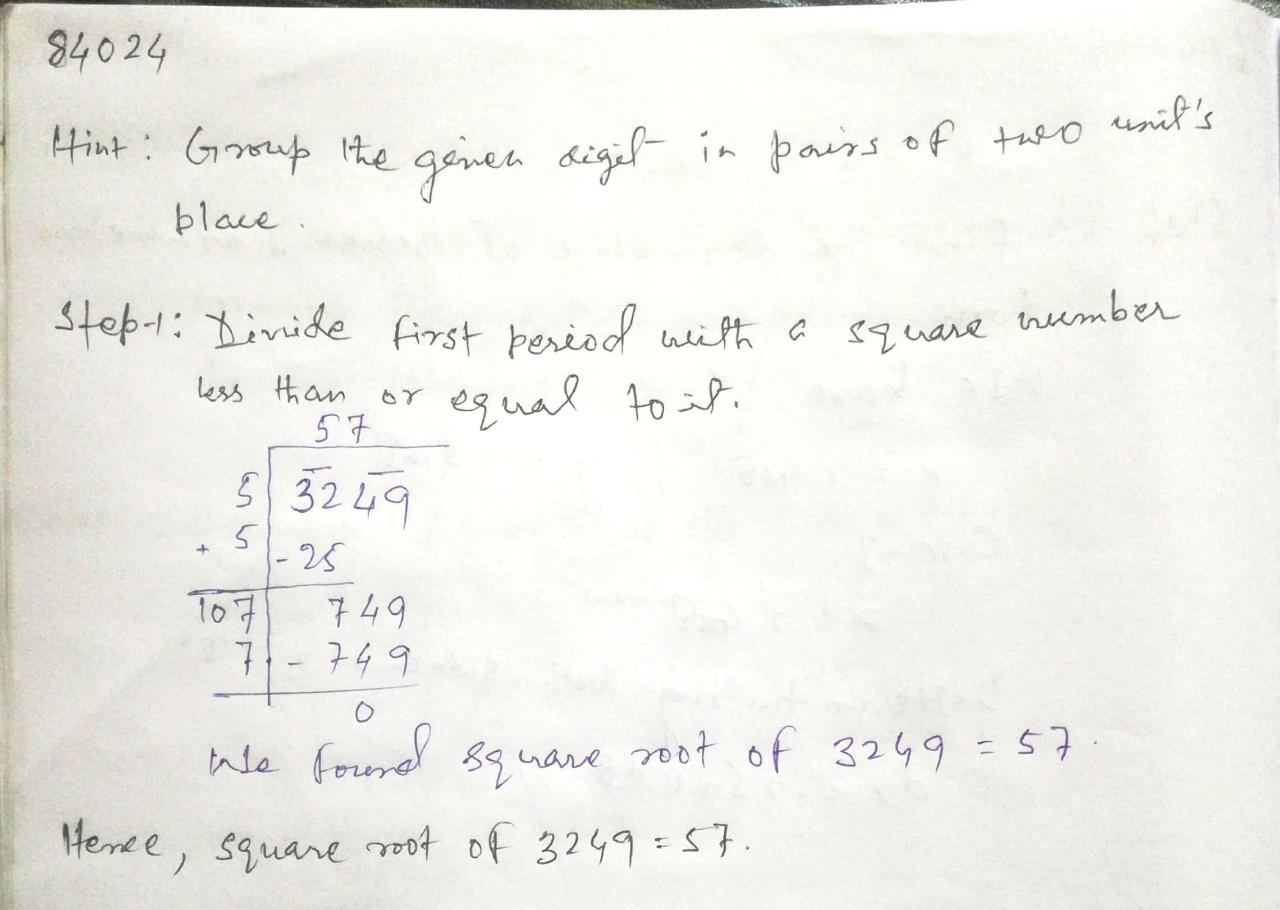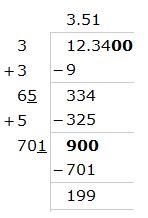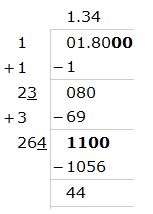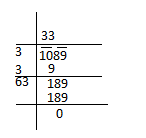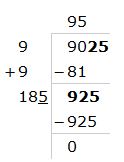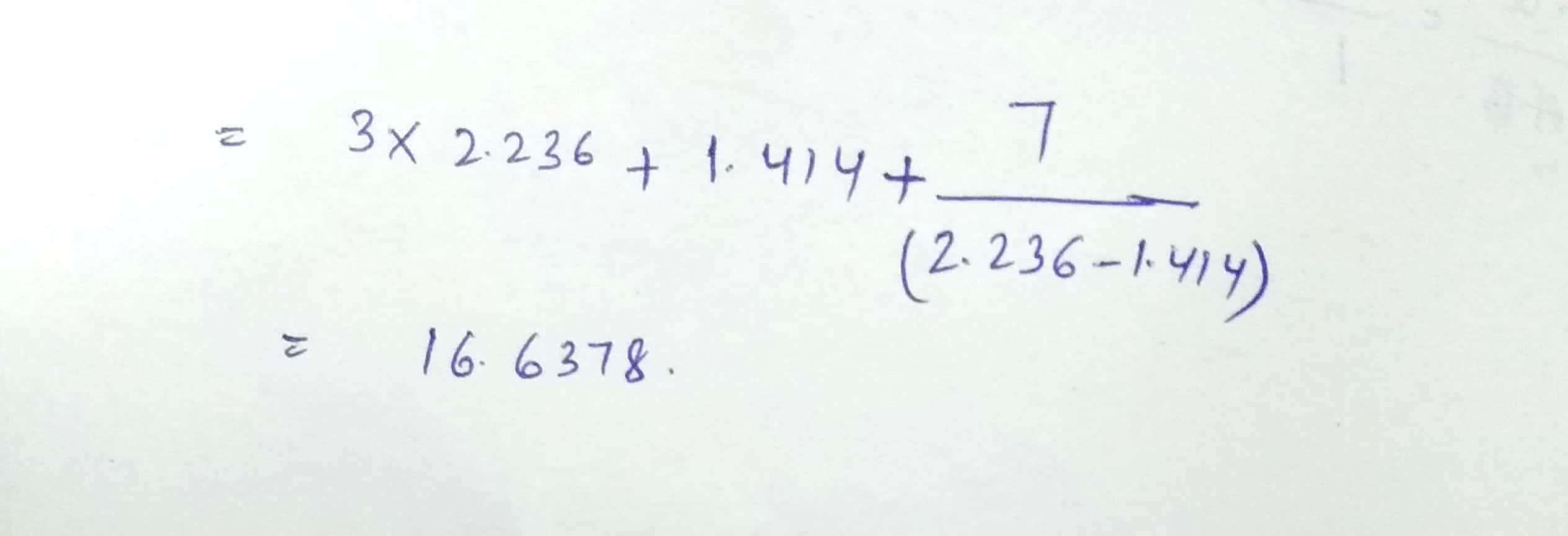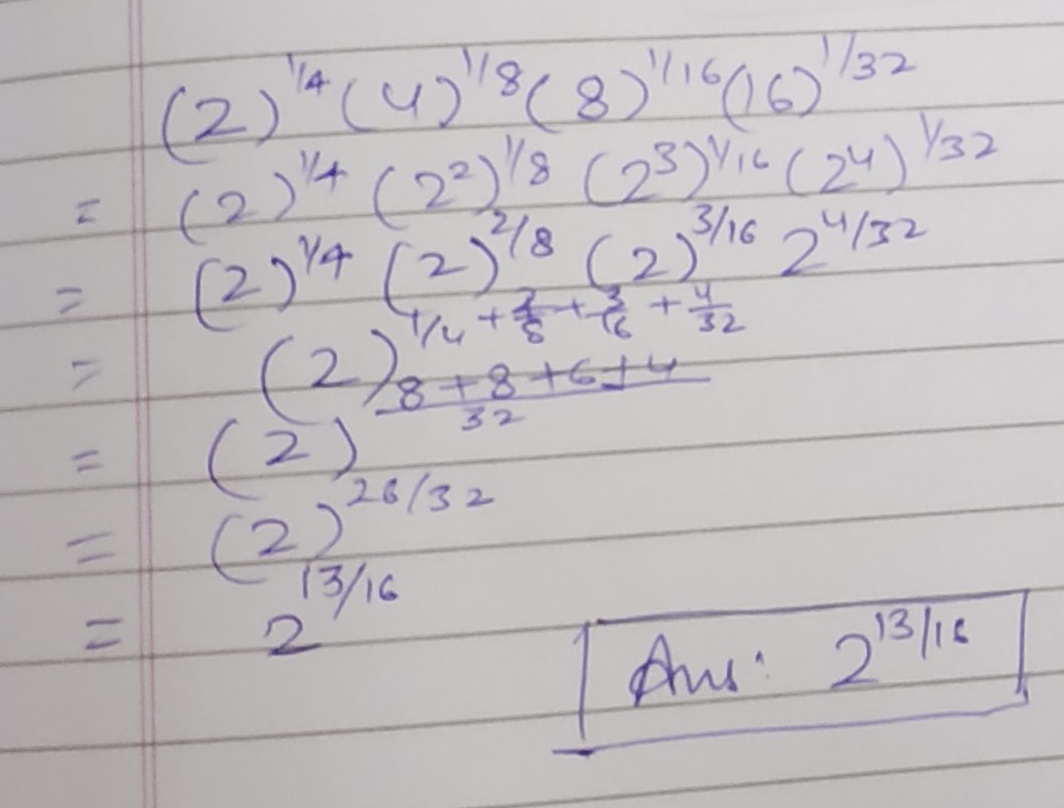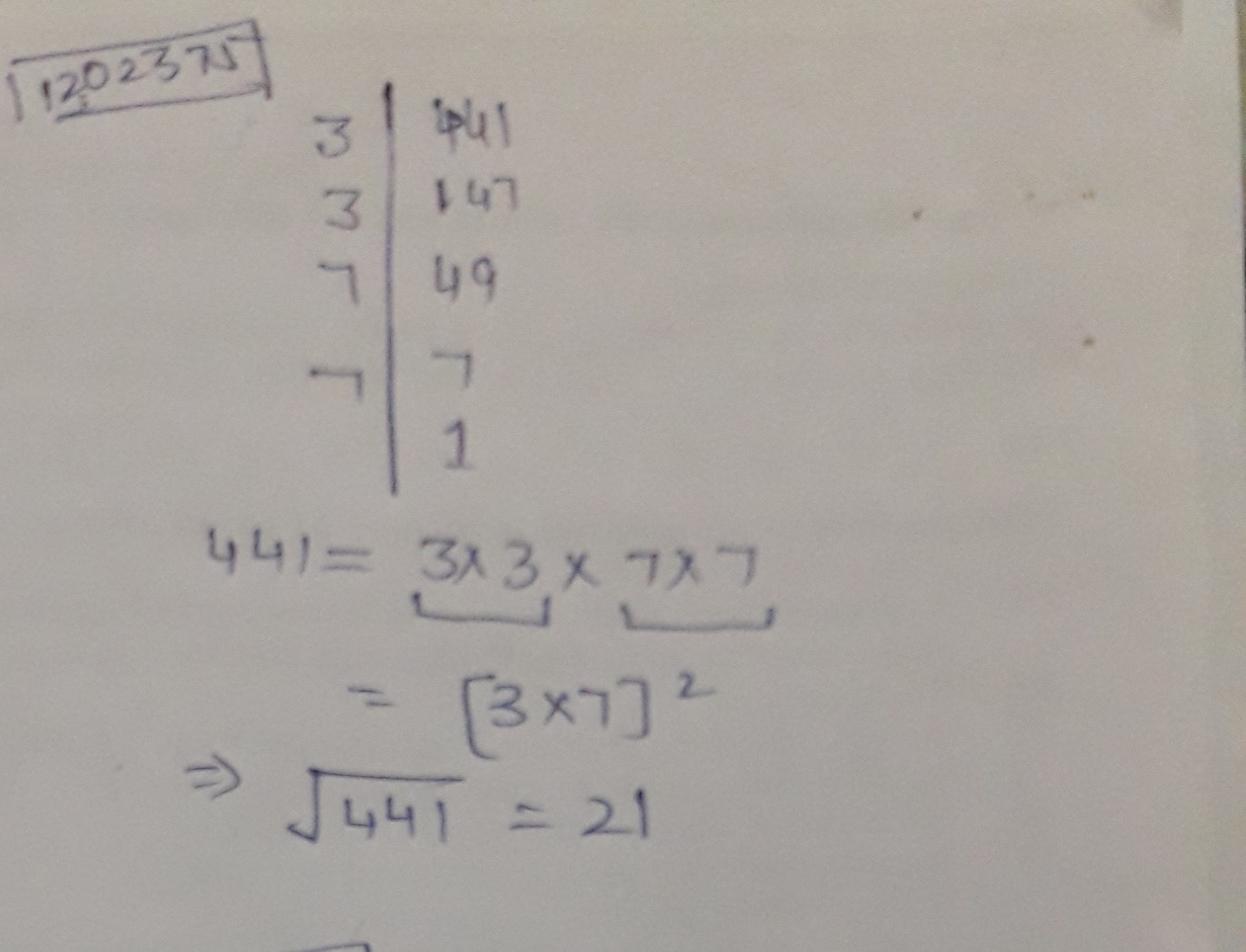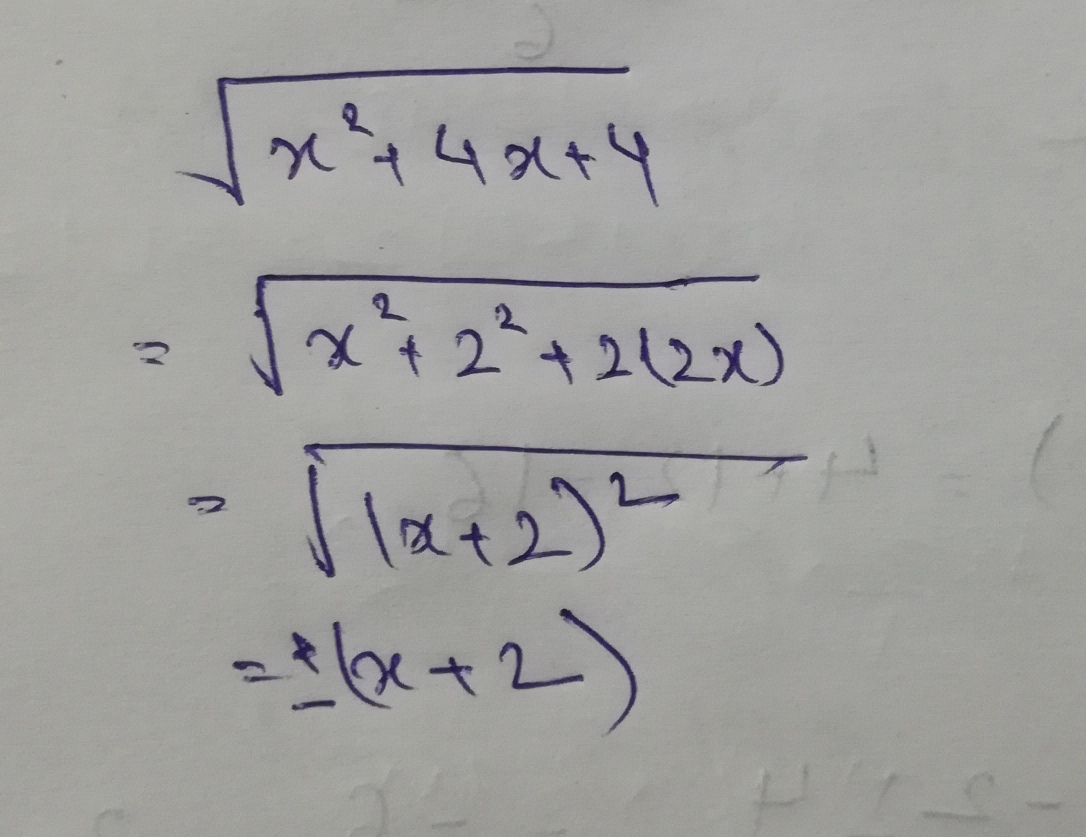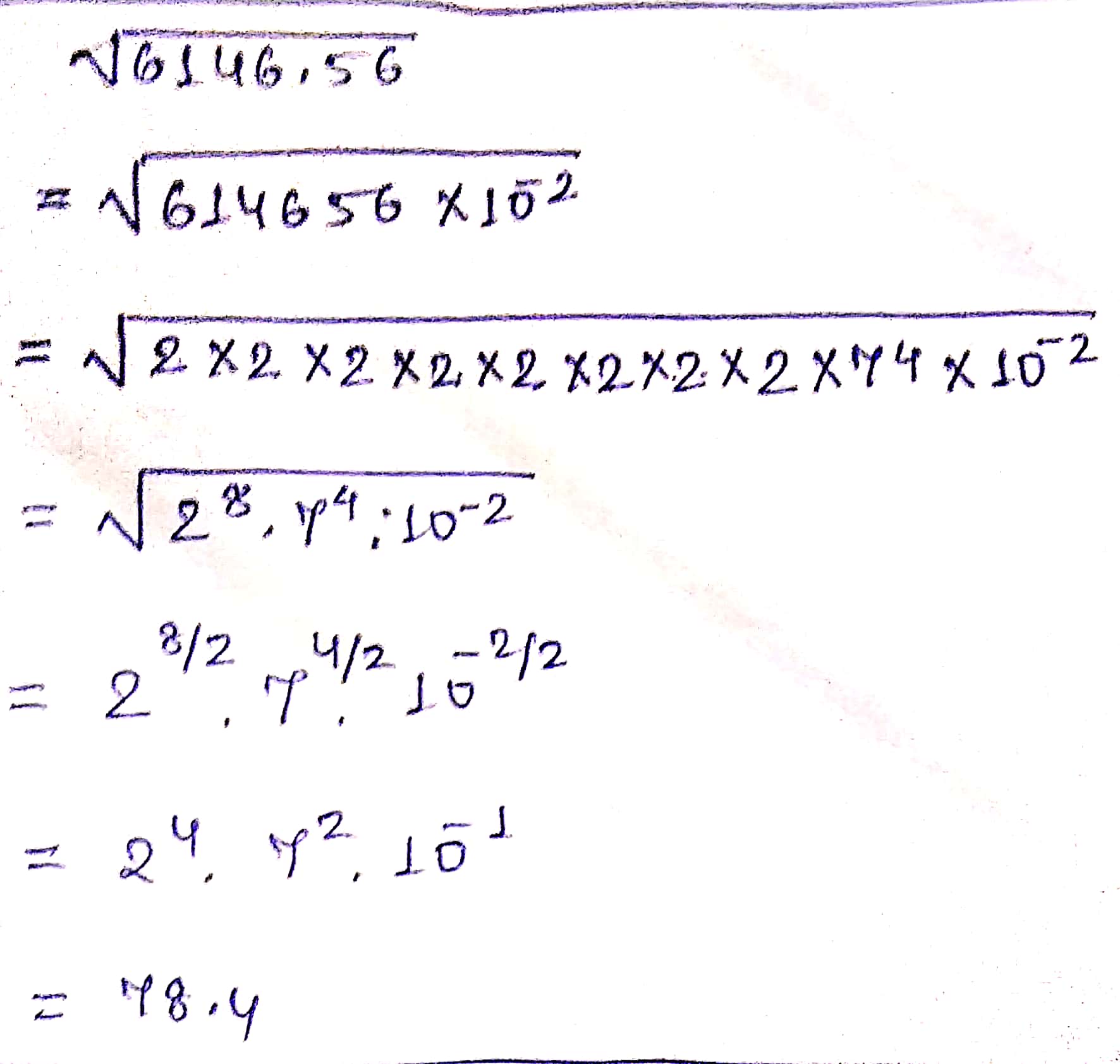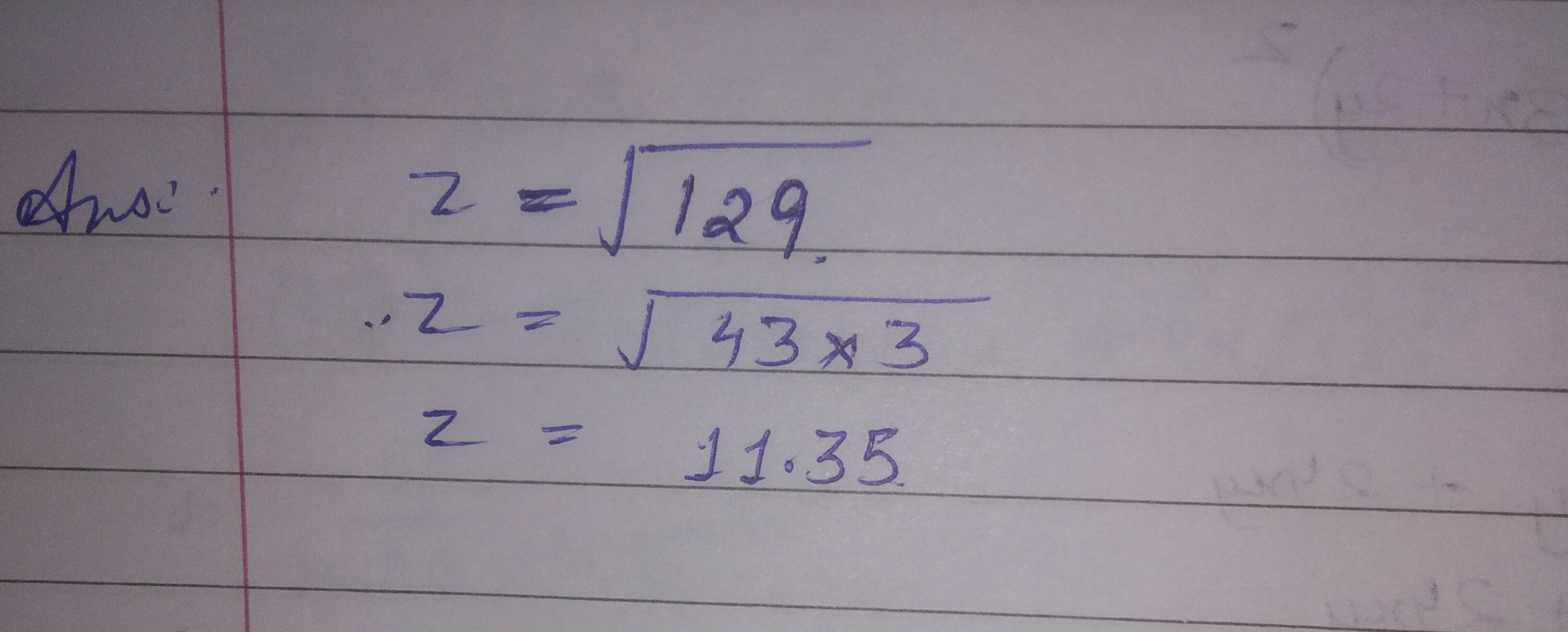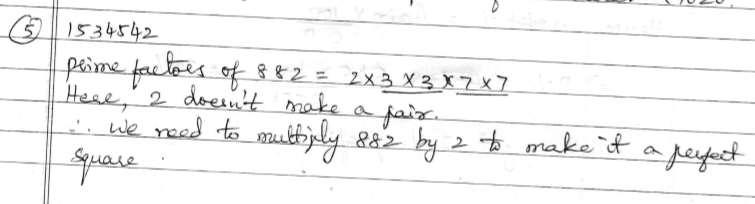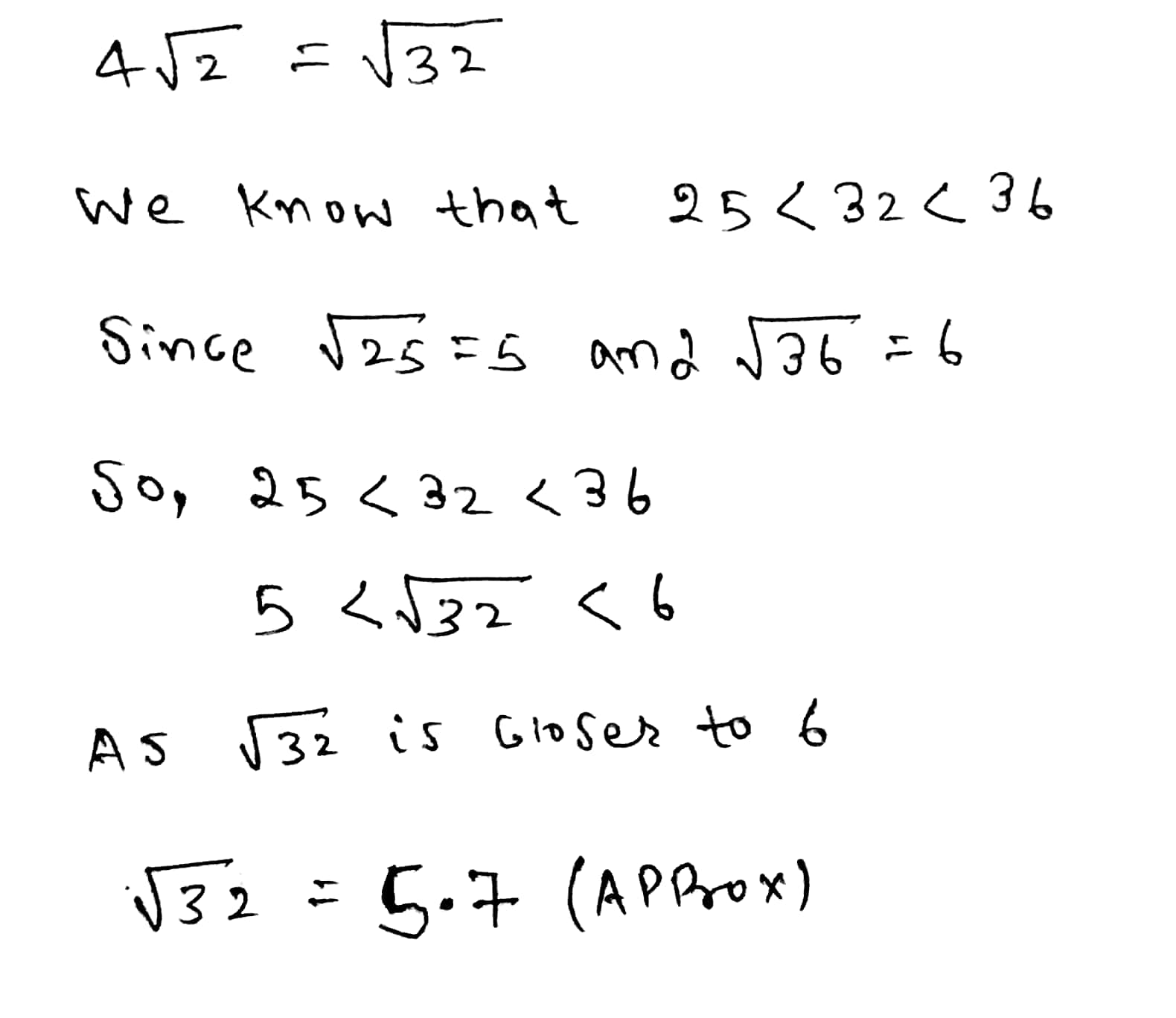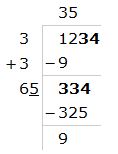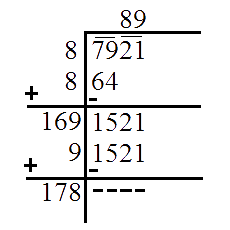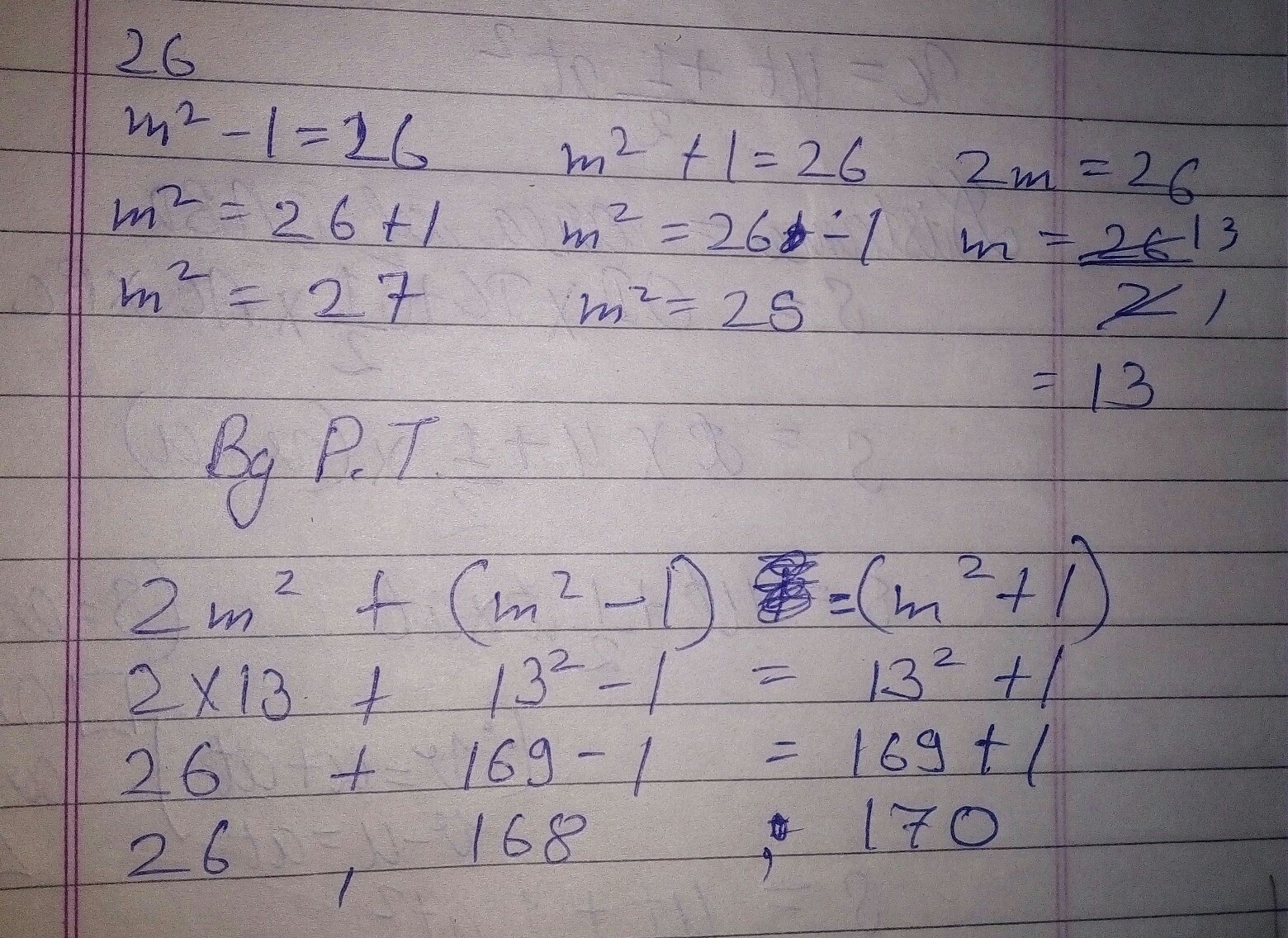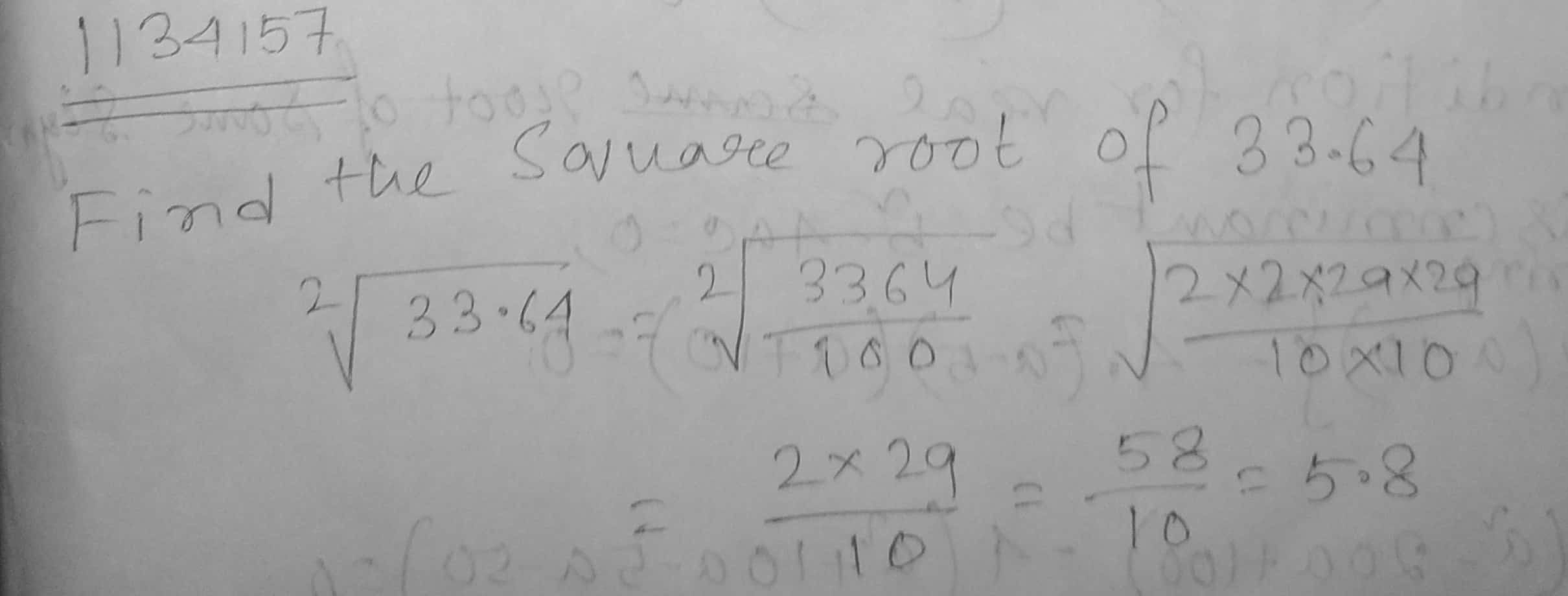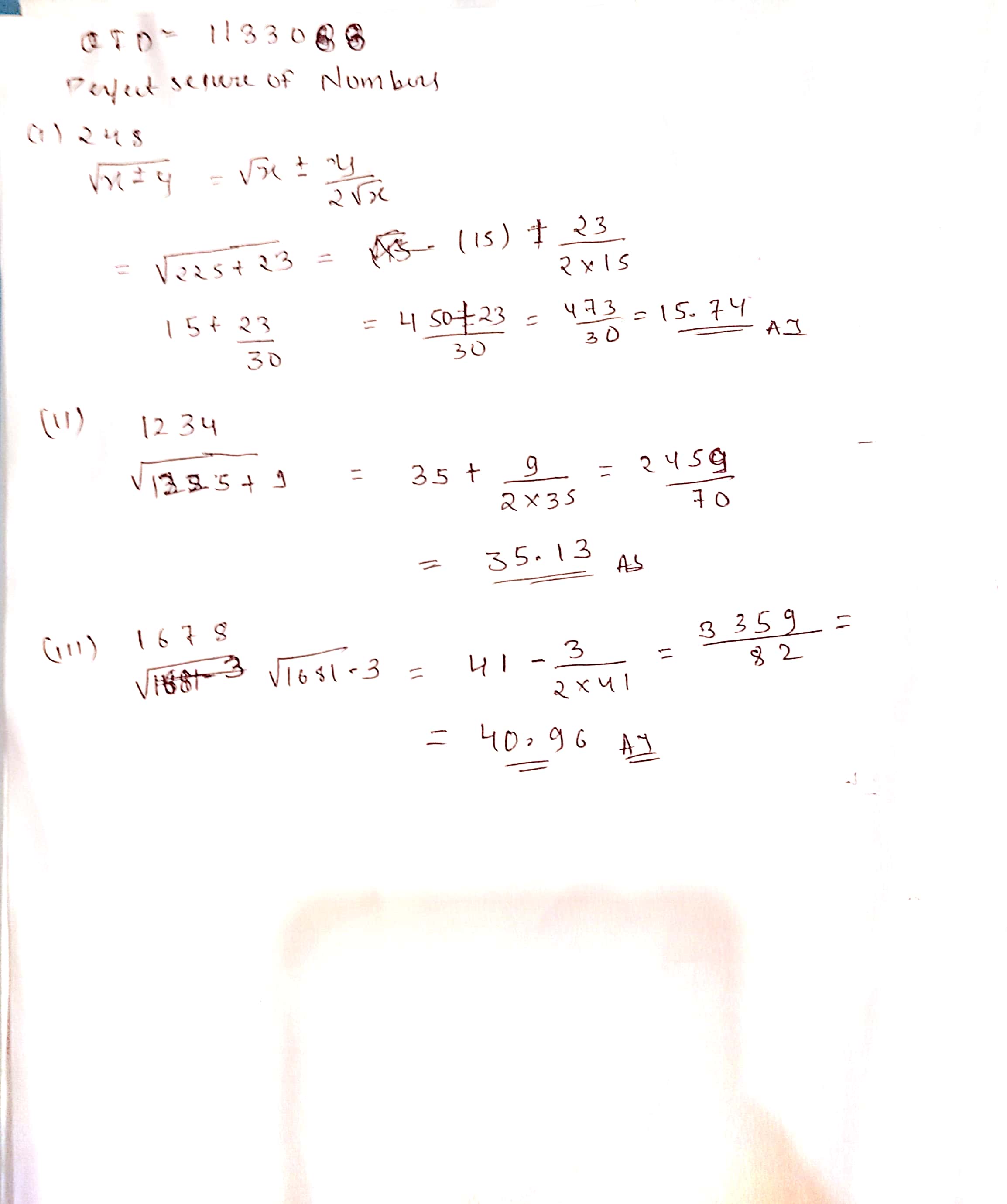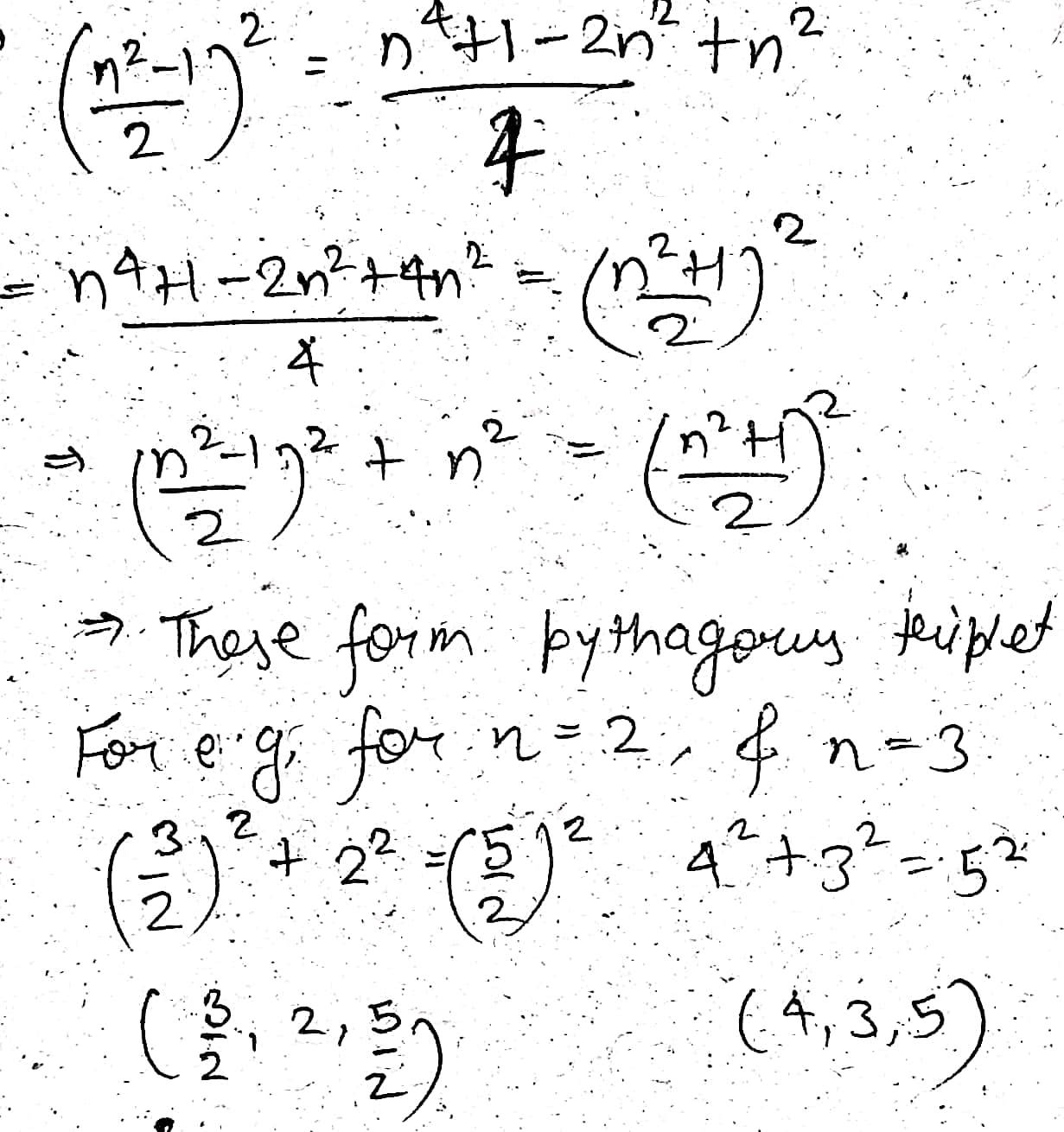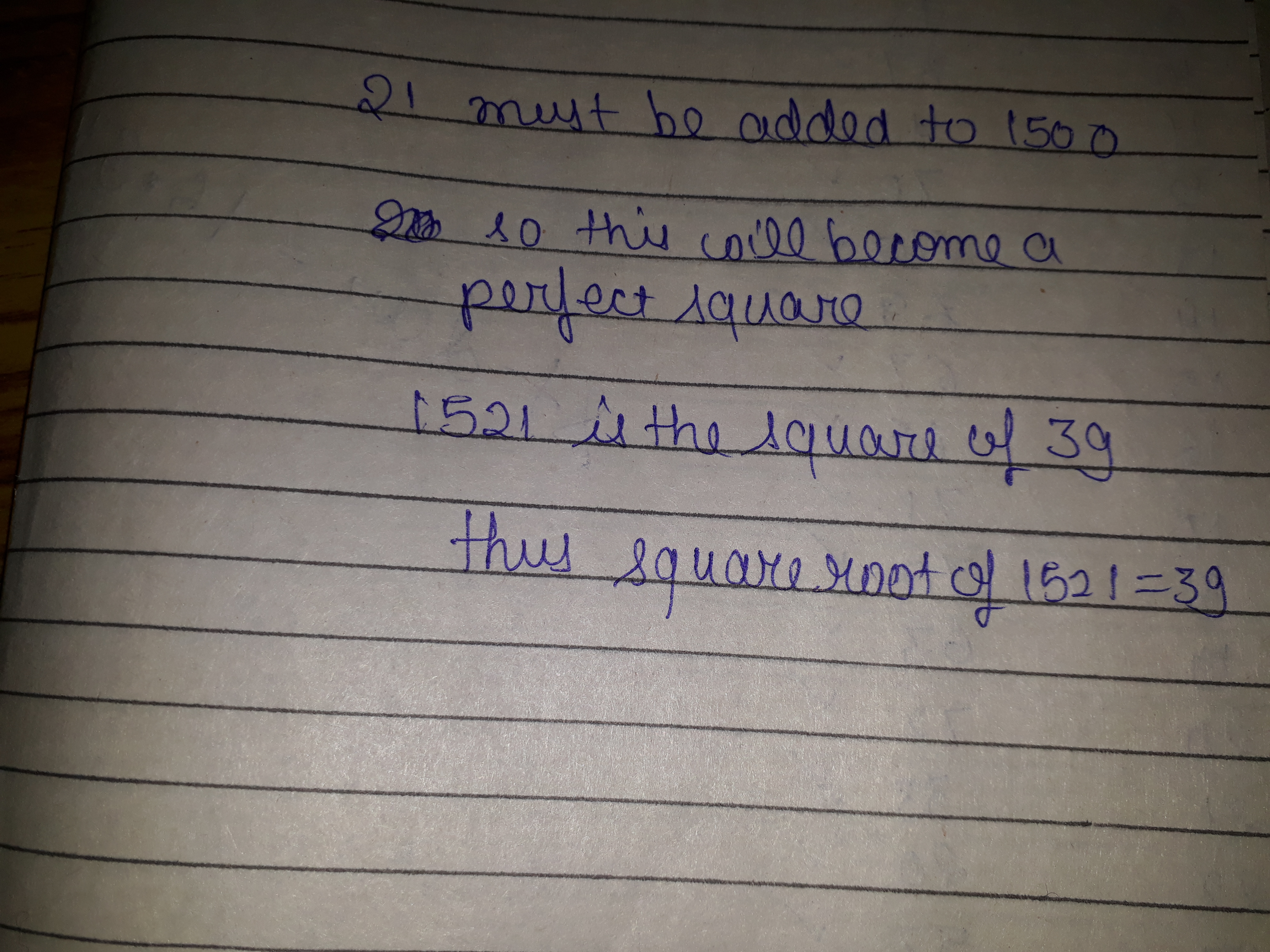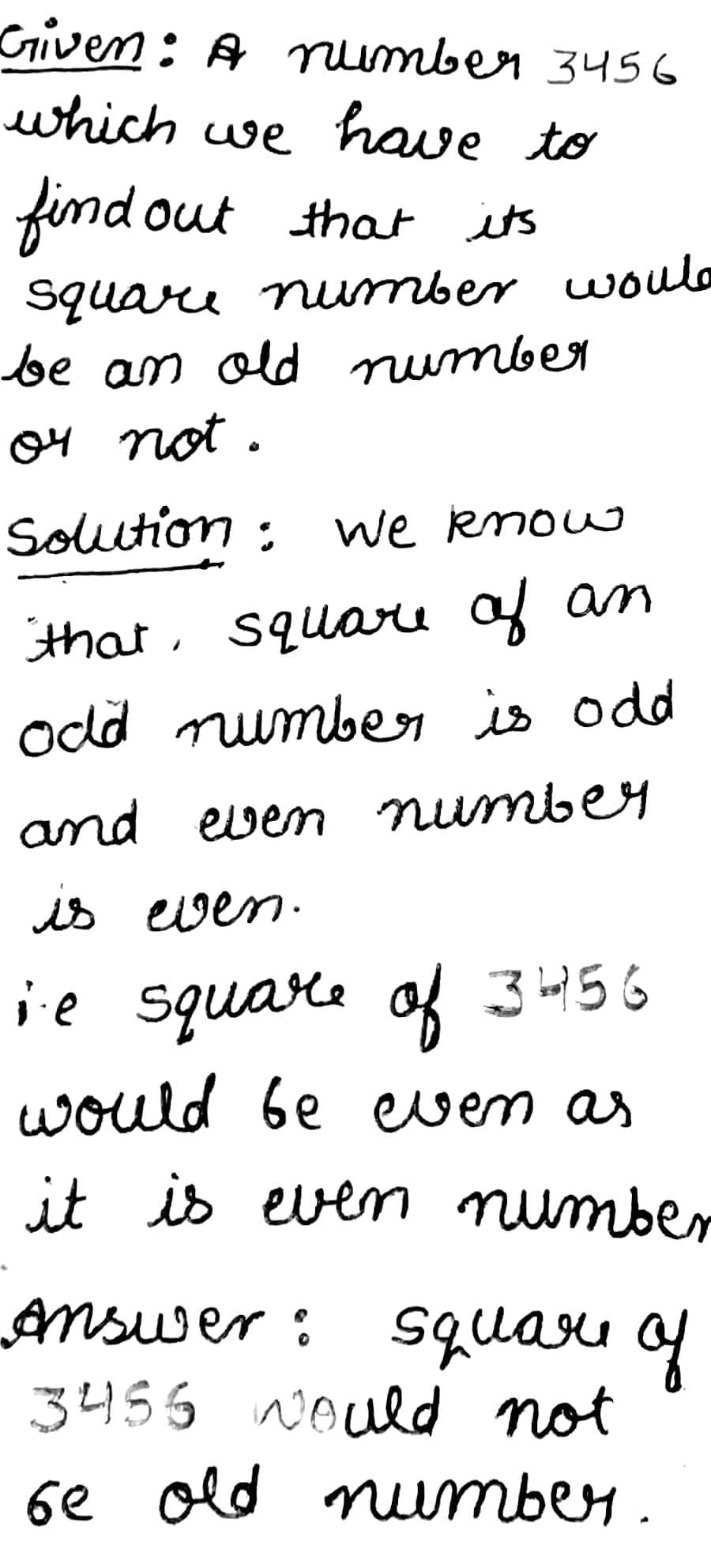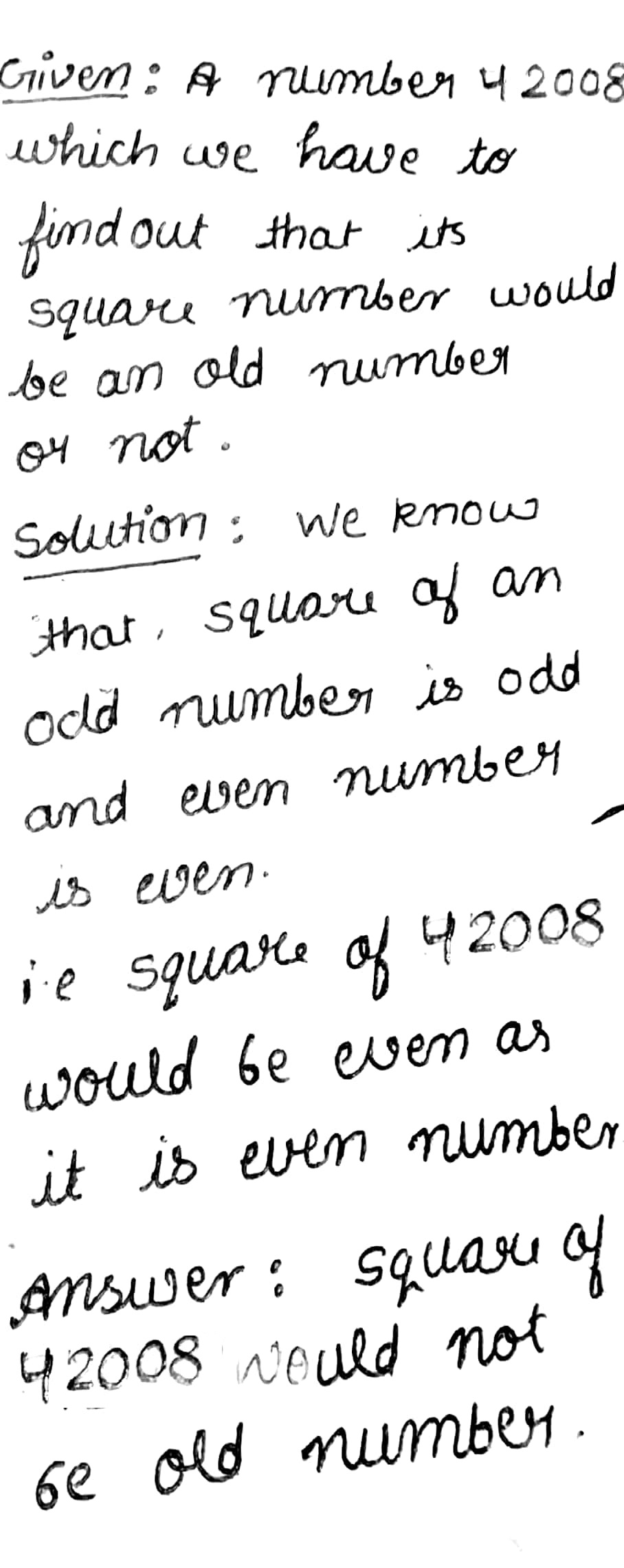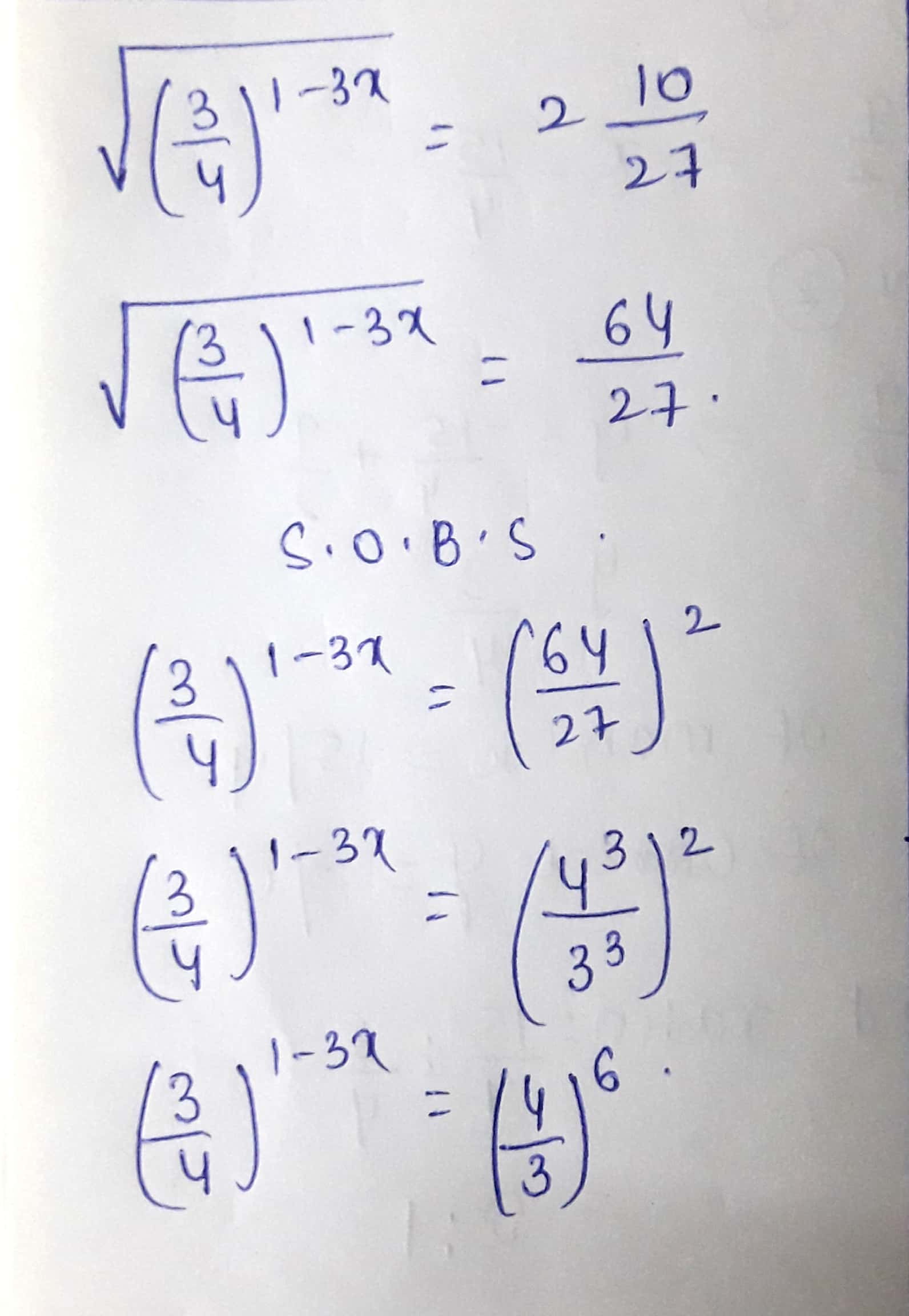Squares And Square Roots - Class 8 Maths - Extra Questions
Find the square root of the following numbers correct to two decimal places:
5.462
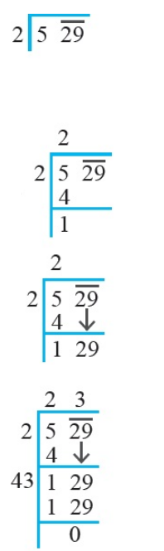
Find the square root of the following decimal numbers by division method:
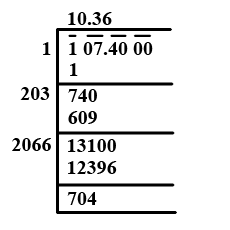
18.4041
Find the square root of the following numbers by prime factorization method:
1849
Find the square root of the following numbers correct to two decimal places:
645.8
Find the square root of the following numbers correct to two decimal places:
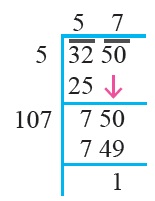
107.45
Find the square root of the following numbers by prime factorization method:
6241
Find the square root of the following decimal numbers by division method:
5.774409
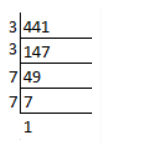
Find the square root of the following numbers by prime factorization method:
441
Find the square root of the following numbers by prime factorization method:
784
Find the square root of the following numbers by prime factorization method:
4356
Find the square root of the following numbers correct to two decimal places:
3
Find the square root of the following numbers by prime factorization method:
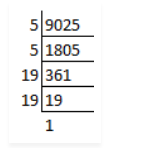
9025
Find the square root of the following number by prime factorization method:
8281
Which of the following natural numbers are perfect squares? Give reasons in support of your answer.
5488
Which of the following natural numbers are perfect squares? Give reasons in support of your answer.
243
Find the square root of the following numbers by prime factorization method:
0.0064
Show that each of the following numbers is a perfect square. Also, find the number whose square is the given number.
7056
Find numbers from 100 to 400 that end with 0,1,4,5,6 or 9, which are perfect squares.
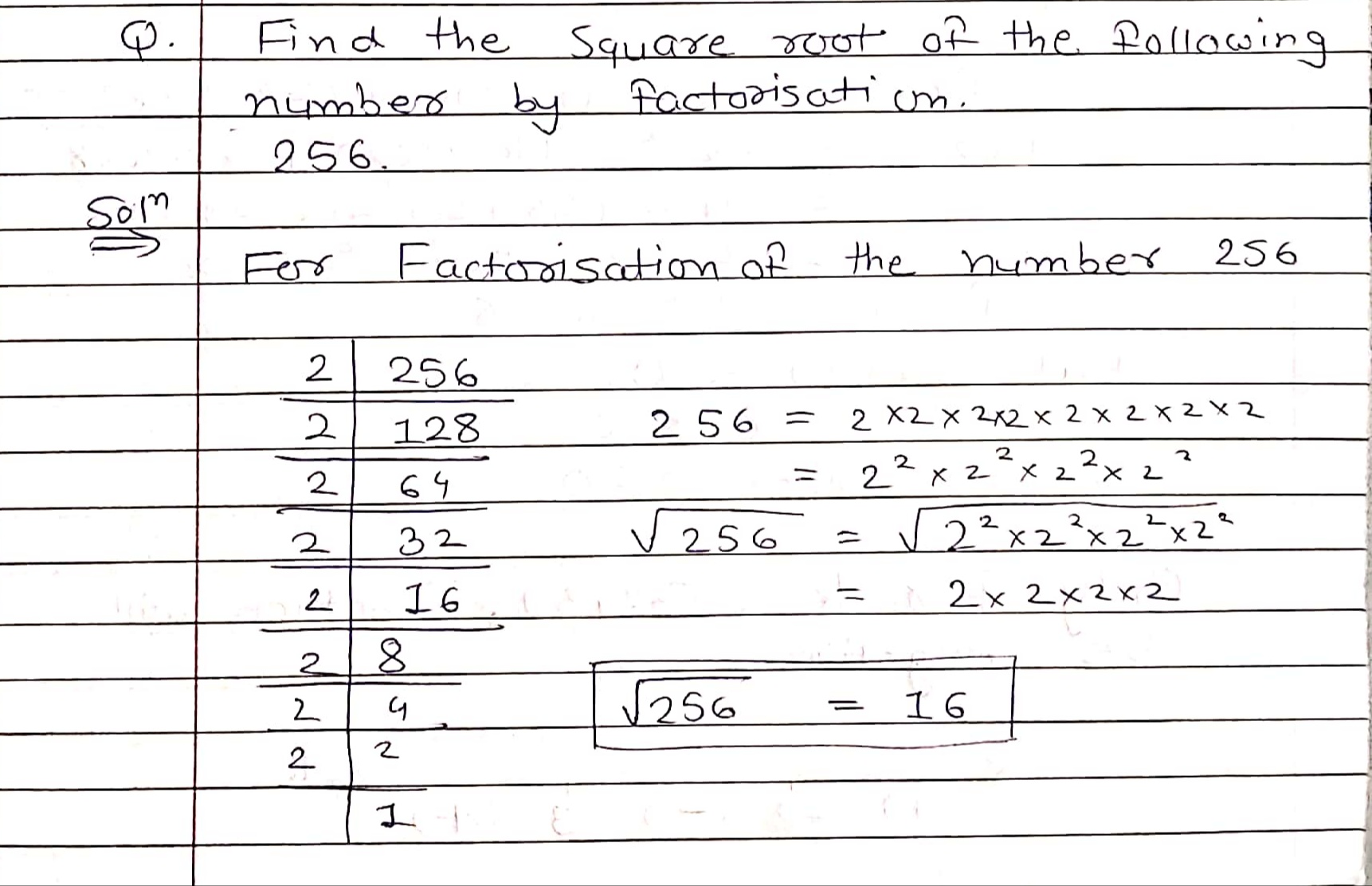
Find the square root of the following numbers by factorization 256
Find the smallest positive integer with which one has to multiply 847 to get a perfect square.
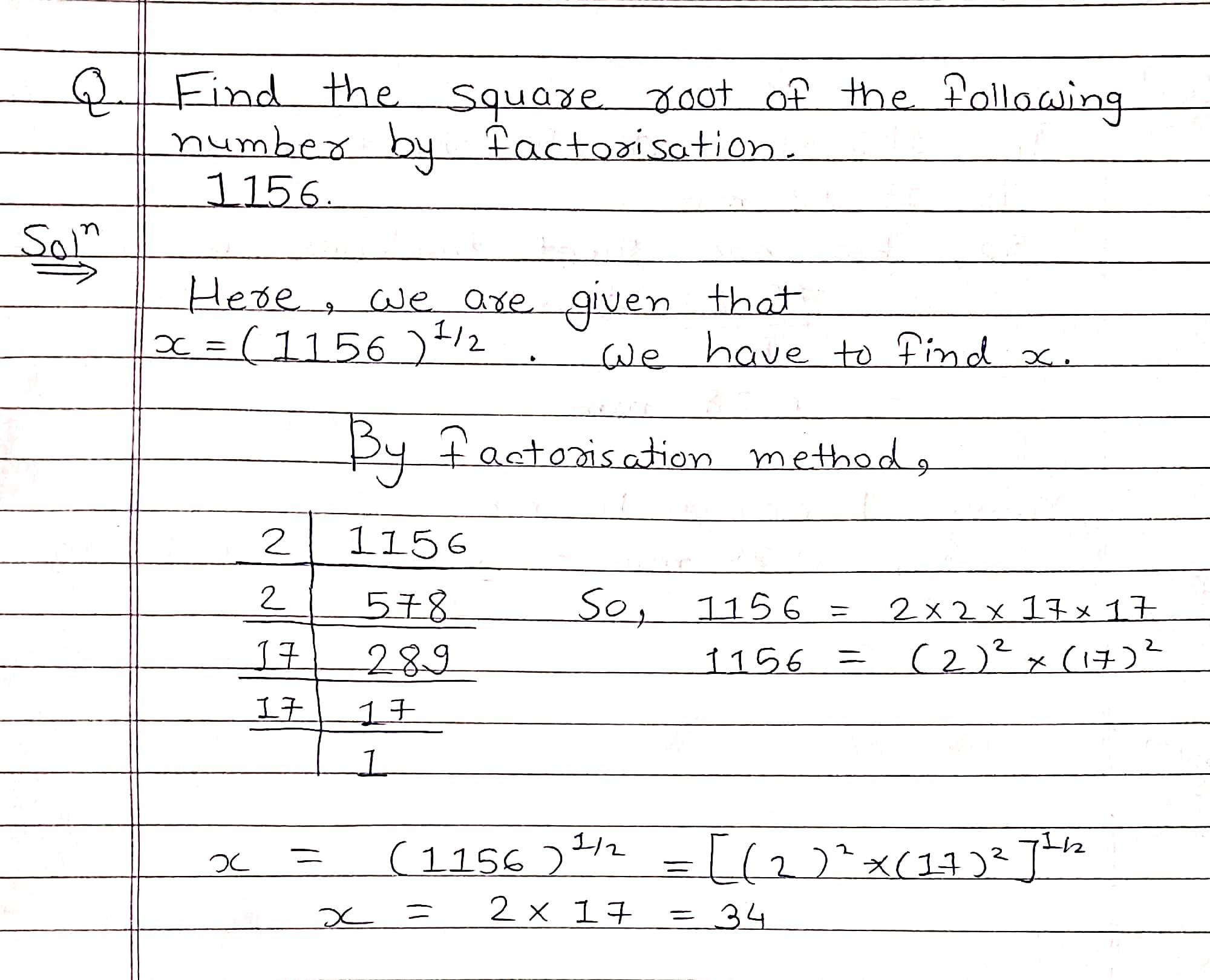
Find the square root by prime factorization method 1156
Find the square root of 10404 by prime factorization method.
Simplify: √169×√361
Simplify: √1764−√1444
Simplify: √1360+9
Find the square root by prime factorization method 13225
Simplify √2704+√144+√289
Simplify: √225−√25
Simplify √100+√36
Find the largest perfect square factor of 48
Find the largest perfect square factor in the number 1352.
Find the smallest positive integer with which one has to multiply 450 to get a perfect square.
Find the smallest positive integer with which one has to multiply to 1445 to get a perfect square.
Find the smallest positive integer with which 1352 be multiplied to get a perfect square.
Find the nearest integer to the square root of 232.
Find the nearest integer to the square root of 824.
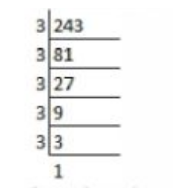
Find the largest perfect square factor in 729
If the area of a square is 90 cm2, what is its side-length, rounded to the nearest integer?
A square piece of land has area 112 m2. What is the closest integer which approximates the perimeter of the land?
Find the square root of 0.017424.
Find the square root of the following numbers by the factorisation numbers : 82944
Explain with reason whether the given number is perfect square or not: 2433
Find whether the square of the given number is even or odd: 8204
Explain with reason whether the given number is perfect square or not: 6098
What will be the units digit of the square of the given number:8742
Find the square root of 1296 by Prime Factorization
How many numbers lie between the square of the given numbers: 25 and 26
Find the square roots of the given number by Prime factorization method: 441
Explain with reason whether the given number is perfect square or not: 4592
Find the square root of
24+4√15−4√21−2√35
Express the following square numbers as the sum of two odd numbers.i)81 ii)144
A society collected Rs. 9,216 wherein, each member contributed as many rupees as there were members in the society. Find the number of members.
Find the smallest whole number by which 1458 should be multiplied, so as to get a perfect square number.
For the following number, find the smallest whole number by which it should be multiplied so as to get a perfect square number.
252
Find the least number that must be added to 1300 so as to get a perfect square. Also find the square root of the perfect square.
Find the square root of each of the following by Long division method:
(i) 2304 (ii) 4489
(iii) 3481 (iv) 529
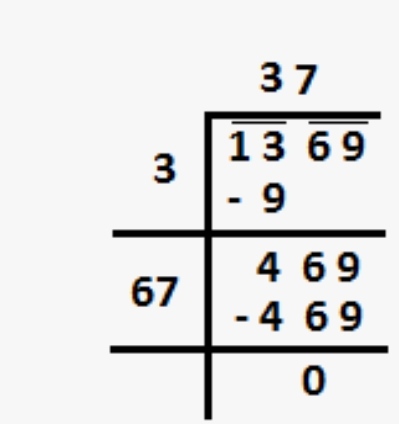
(v) 3249 (vi) 1369
(vii) 5776 (viii) 7921
(ix) 576 (x) 3136
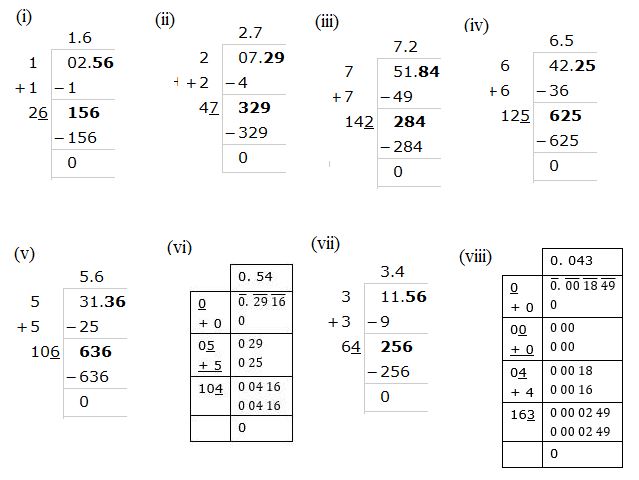
Find the square root of the following decimal numbers:
(i) 2.56 (ii) 7.29
(iii) 51.84 (iv) 42.25
(v) 31.36 (vi) 0.2916
(vii) 11.56 (viii) 0.001849
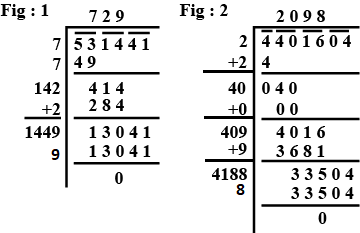
Find the square root of the following numbers by long division method:
531441
4401604
Find the square root of each of the following numbers by using the method of prime factorization:
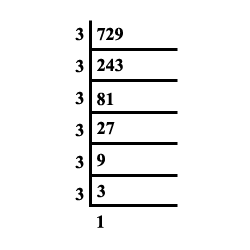
729
Find the square root of each of the following numbers by using the method of prime factorization:
4096
Find the square root of each of the following numbers by using the method of prime factorization:
7056
Find the square root of each of the following numbers by using the method of prime factorization:
2025
Find the square root of each of the following numbers by using the method of prime factorization:
8100
Write a Pythagorean triplet whose smallest number is
20
Write a Pythagorean triplet whose smallest number is
14
Find the least square number which exactly divisible by each of the numbers 8,12,15 and 20
Find the largest number of 3 digits which is perfect square.
Evaluate:
√576
Write a Pythagorean triplet whose number is
63
Find the square root of the following decimal numbers by division method:
42.25
Find the square root of each of the following by division method:
106929
Write a Pythagorean triplet whose number is
8
Find the square root of 1764 by the prime factorisation method.
Evaluate :
√92416
Find the unit digit in the square of the numbers 81 and 79.
Show that the following number is not perfect square:
22453
The square of which of the following numbers would be an odd number?
731
Show that the following number is not a perfect square. Give reason.
8948
Show that the following number is not perfect square:
4058
Show that the following number is not a perfect square. Give reason.
333333
What will be the units digit of the squares of the following numbers?
977
What will be the units digit of the squares of the following number?
53924
What will be the units digit of the squares of the following numbers?
4583
What will be the units digit of the squares of the following numbers?
52698
What will be the units digit of the squares of the following numbers?
12796
What will be the units digit of the squares of the following numbers?
99880
Check whether the following triplet is Pythagorean?(18,80,82)
What will be the unit's digit of the square of the following number?
78367
What will be the units digit of the squares of the following numbers?
52
What will be the units digit of the squares of the following numbers?
55555
The number 1023 is a perfect square.Type 1 if above statement is true, otherwise 0.
Write five numbers which you cannot decide whether they are square just by looking at the unit’s digit.
The number 1027 is a perfect square.Type 1 if above statement is true, otherwise 0.
Find the square root of the following number by division method.2304.
Find the square root of the following number by factorisation.
1156
Check whether the following triplet is Pythagorean?(12,35,38)
The number 1027 is a perfect square.Type 1 if above statement is true, otherwise 0.
Type 1 if above statement is true, otherwise 0.
Find the square root of each of the following by long division method:
1471369
Write the prime factorization of the following number and hence find its square root:
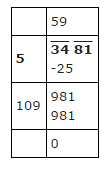
5929
Express the following surds as continued fraction, and find the sixth convergent to each
√11
Estimate the square root : 1√33
Estimate the value of square root : √14
Give reason to show that none of the numbers given below is a perfect square:
360
Find the square root of 5929 by the prime factorisation method.
Find the square root of the number 729 by the prime factorisation method.
The difference of two perfect squares is a perfect square.
If true then enter 1 and if false then enter 0
The product of two perfect squares is a perfect square.
If true then enter 1 and if false then enter 0
The number of digits in a perfect square is even.
If true then enter 1 and if false then enter 0
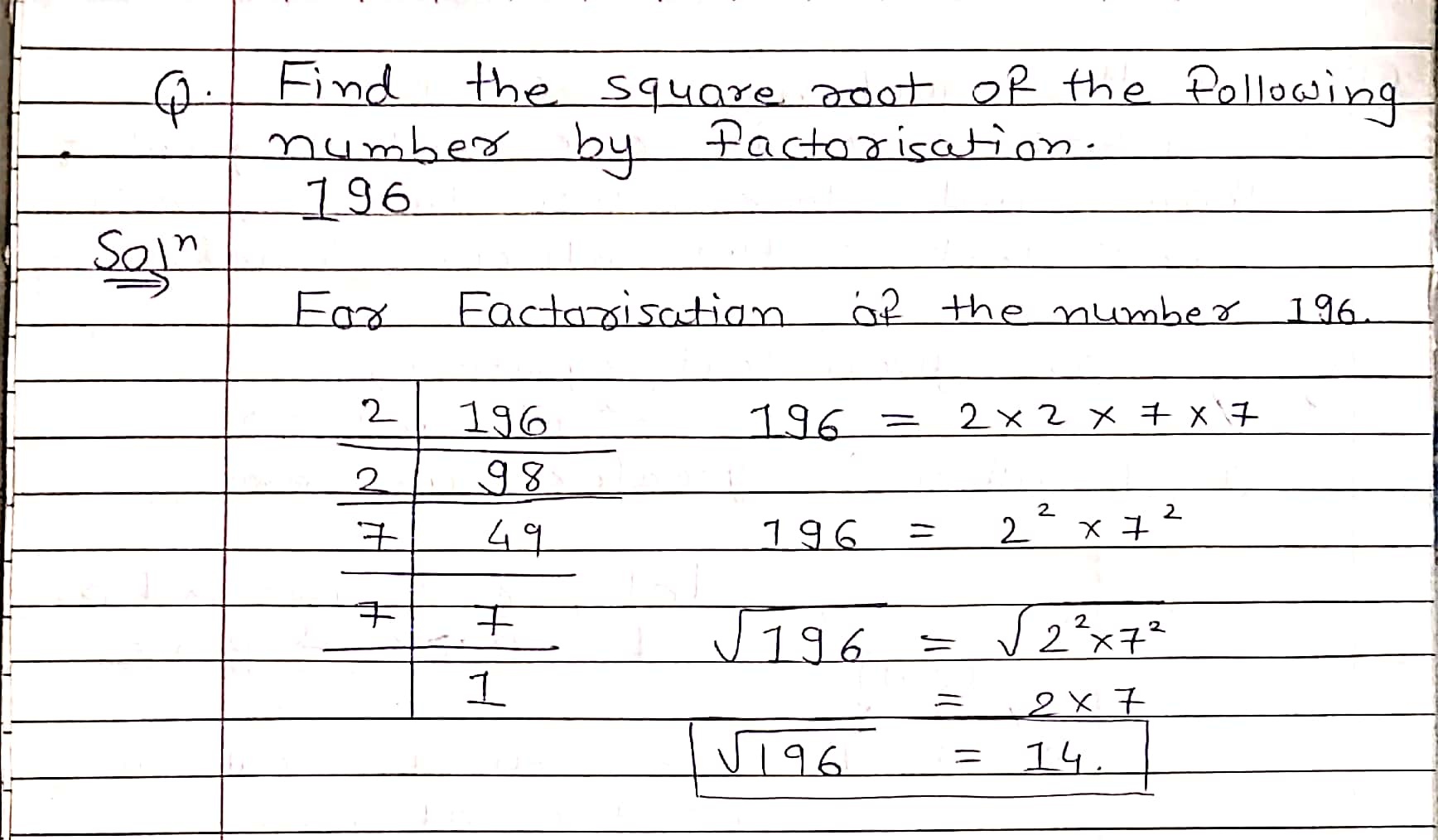
Find the square root of the 196 by factorisation.
Find the square root of the following in the form of a binomial surd.
8+2√7.
Find the greatest number of three digit which is a perfect square.
Estimate the square root : 7√11
Estimate the square root : 1√21
Express the following surds as continued fraction, and find the sixth convergent to each:
√5
Find limits of the error when 20865 is taken for √17
Estimate the value of square root : √22
Find the square root of the following in the form of a binomial surd.
14+6√5.
Give reason to show that none of the numbers given below is a perfect square:
5963
Give reason to show that none of the numbers given below is a perfect square:
64000
Give reason to show that none of the numbers given below is a perfect square:
8457
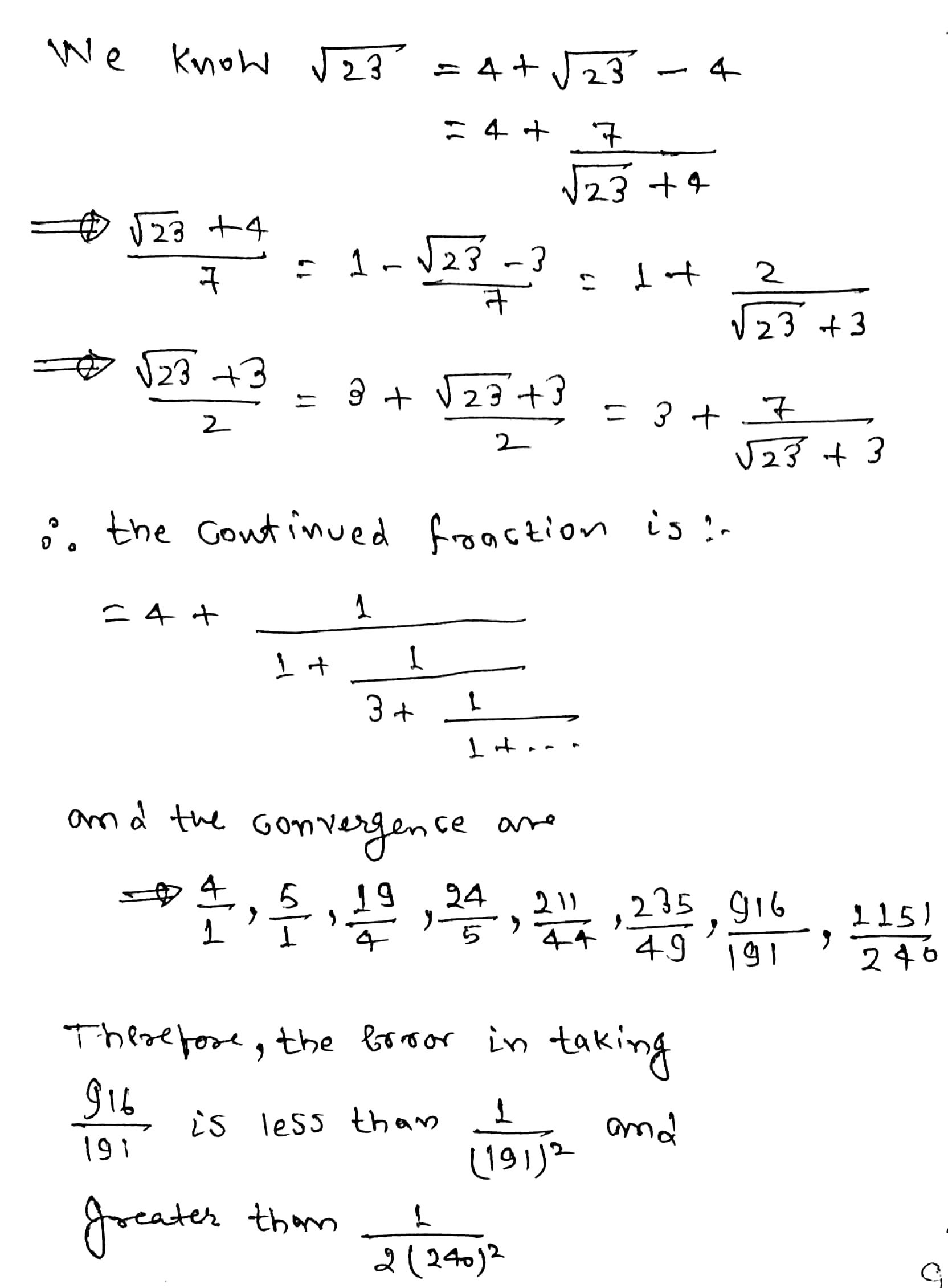
Find limits of the error when 916191 is taken for √23.
Which of the following squares are squares of even number?
196
Give reason to show that none of the numbers given below is a perfect square:
9468
Give reason to show that none of the numbers given below is a perfect square:
5372
Fill in the blanks to make the statements true.
The square of 0.7 is _________.
Fill in the blanks to make the statements true.
The digit at the ones place of 572 is _________.
Fill in the blanks to make the statements true.
The square root of 24025 will have _______ digits.
Write a Pythagorean triplet whose number is
80
Find the square of the following number containing 5 in unit's place:
305
Find the square root of each of the following by division method:
167281
Find the square of the following number containing 5 in unit's place:
525
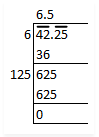
Find the square root of 13.69?
Write two Pythagorean triplets each having one of the numbers as 5.
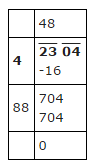
Find the square root of the following by long division method.
a) 27.04
b) 1.44
Write the first five square numbers.
Write five number which you can decide by looking at their one's digit that they are not square numbers.
The following numbers are obviously not perfect. Give reason.
5298
Find the square root of the following decimal numbers by division method:
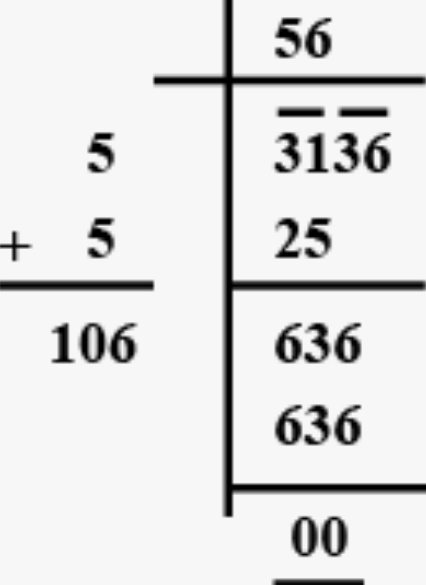
51.84
Find the square root of 2304 by long division method.
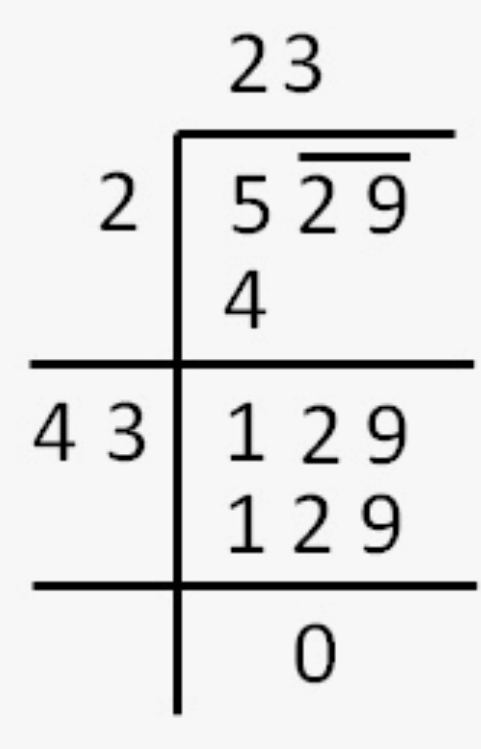
Find the square root of 529 by long division method.
Find the square root of 3481 by long division method.
Find the square root of 400 by prime factorisation method.
Find the smallest positive integer with which one has to divide 336 to get a perfect square.
What could be the possible 'one's' digits of the square root of the following number?
657666025
Solve : 3√25×6×30×6
Find:
√4096
Find the square root of 9216
If √2=1.414, then find the value of 11−√2
Find the least number which must be subtracted to get a perfect square from 1000.
Find the square root of a number 529 by prime factorisation method.
For each of the following numbers, find the smallest whole number by which it should be divided so as to get a perfect square number. Also, find the square root of the square number obtained.
(i) 252 (ii) 2925 (iii) 396 (iv) 2645 (v) 2800 (vi) 1620
Find √96.04.
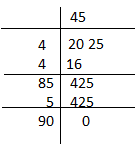
Find the square root of 2025.
Find the square root of 42.25 using division method.
Find the square root of 8281
Find the square root of 7744 by the prime factorisation method.
What will be the unit digit of the square of 3853?
What will be the unit digit of the square 12796?
What will be the unit digit of the square of 799?
What will be the unit digit of the square of 52698?
What will be the unit digit of the square of 81?
What will be the unit digit of the square of 26387?
What will be the unit digit of the square of 272?
What will be the unit digit of the square of 99880?
Find the square root of 1369 by long division method.
Find the square root of 4489 by long division method.
Find the square root of 5776 by long division method.
Find the square root by long-division method:
3249
For the following number, find the smallest whole number by which it should be divided so as to get a perfect square. Also, find the square root of the square number so obtained.
252
2925
One of the following number is not a perfect square, state the number.
2453,2500
What will be the unit digit of the square of the number 26387?
What will be the unit digit of the square of the number 12796?
What will be the unit digit of the square of the number 52698?
What will be the unit digit of the square of the number 272?
State whether 1335 is a perfect square or not.
Find the square root of the following decimal numbers
(i)2.56(ii)7.29(iii)51.84(iv)42.25(v)31.36
Find the square root of 1057.
Is 676 a perfect square? If yes, explain why?
The following numbers are obviously not perfect squares. Give reason.
(i) 1057
(ii) 23453
(iii) 7928
(iv) 222222
(v) 64000
(vi) 89722
(vii) 222000
(viii) 505050
Make a list of all perfect squares from 1 to 500.
Find the smallest integer larger than 1 which is a perfect square as well as a perfect cube.
Find the least number to be added to 9300 to get a perfect square.
Find the square root of the following numbers by the factorisation numbers : 19881
Find the square root of 70.56.
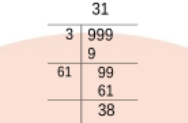
Find the least number to be subtracted from 2011 to get a perfect square.
Find the square root of the following numbers by the factorisation numbers : 34596
Find the square root of the following numbers by the factorisation numbers : 76176
Find the approximation of √3.11 both from below and above to 3 decimal places.
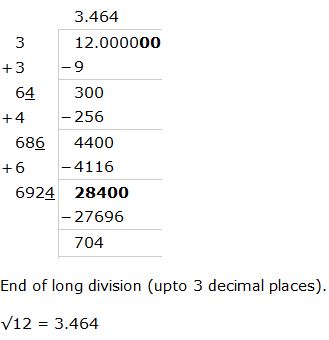
Find the square root of the following number correct to 2 decimal places: 12
Round off following number to 3 decimal places : 14.56789
Round off following number to 3 decimal places : 1.5678
Find the square root of the following number correct to 2 decimal places: 234.234
Find the square root of the following number correct to 2 decimal places: 12.34
Round off following number to 3 decimal places : 2.84671
Find the square root of the following number correct to 2 decimal places: 1.8
Round off following numbers to 3 decimal places : 3.3333567
Find the square roots of the given number by Prime factorization method: 784
Which of the following numbers are perfect squares?
(i) 121 (ii) 1136 (iii) 256 (iv) 321 (v) 600
Find the square root of the following numbers by the factorization method:
(i) 729 (ii) 400
(iii) 1764 (iv) 4096
(v) 7744 (vi) 9604
(vii) 5929 (viii) 9216
(ix) 529 (x) 8100
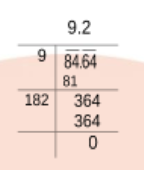
Find the square root of the given decimal number: 84.64
Find the square roots of the given number by Prime factorization method: 7056
Find the square root of the given number by division method: 1089
Find the square root of the given number by division method: 2304
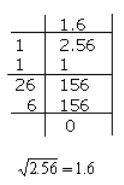
Find the square root of the given decimal number: 2.56
Find the square root of each expression given below:
(i) 3×3×4×4
(ii) 2×2×5×5
(iii) 3×3×3×3×3×3
(iv) 5×5×11×11×7×7
Write down the unit digits of the following:
(i) 782
(ii) 272
(iii) 412
(iv) 352
(v) 422
Find the square roots of the given number by Prime factorization method: 4096
Find the square root of the given number by division method: $$9025$$
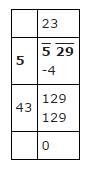
Find the square root of 5929
Find the least number by which 384 must be divided to make it a perfect square
Write down the unit digits of the squares of the following numbers:
(i) 24 (ii) 78 (iii) 35
Find the square root of 529 using long division method
Find the least number by which 200 must be multiplied to make it a perfect square
By observing the units digits, which of the numbers 3136,867 and 4413 cannot be perfect squares?
Find the square root of 169
Find the square root of 64
Find √3969 by the long division method
Find the square root of 12.25
Find the square root of
6+√12−√24−√8
Solve the following equation.16x2−24x+9=0
Verify the validity of the identity
(a+b+c)2=a2+b2+c2+2ab+2bc+2ca
for the following given values.
a=2 b=4 c=6
a=5 b=−2 c=3
a=−2 b=3 c=−4
Find the square root of the following in the form of a binomial surd.
n+√n2−1.
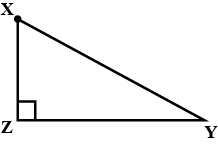
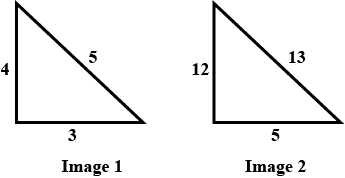
Triangle XYZ is right-angled at vertex Z. Calculate the length of YZ, if XY=13 and XZ=12
Find the least number, which must be subtracted from 3250 to make it a perfect square
Find the square root of −7−24√−1.
Find a square of a natural number between 75 and 90.
Check if the following number is perfect square.
1243
Evaluate: √32+√48√8+√12
Check if the following number is perfect square.
3300
Find the square root of a given number by prime factorization method.
3136
Find the value of 3√5+√2+7√5−√2√5=2.236 and √2=1.414 ?
Find the value of √3
Number of solutions of 2x+3x+4x=5x is
A General wishes to draw up his 7500 soldiers in the form of a largest square. After arranging, he found out that some of them are left out. How many soldiers were left out?
Find the square root of 11+2√30
Solve the problem:-
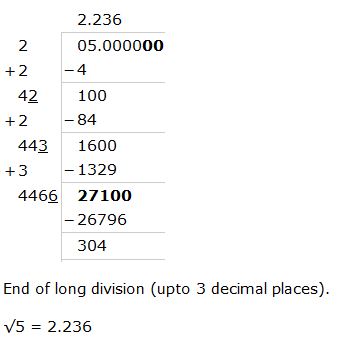
√5=?
Find the square root of (x+1x)2−4(x−1x).
Prove the followings,i) (a+b)2+(a−b)2=2(a2+b2)ii) (a+b)2−(a−b)2=4ab
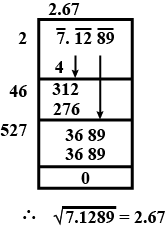
Find square root of =7.1289 by long division method.
Find the greatest five digit number which is a perfect square. Also, find the square root of the number so obtained.
Find the square root of 361201 by long division method
Find the smallest number by which 2400 is to be multiplied to get a perfect square and also find the square root of the resulting number.
Find whether the square of the following numbers are even or odd?
2826.
Find square root of 625 by long divison method.
Find the square of −1213
Solve:25(2x+y)2−16(x−y)2
Rajini says 1 sq m=1002 sq cm. Do you agree? Explain.
If x=52,y=34 then find the value of (x2+y1)(x2−y1).
If √15625=125, then find the value of √15625+√15625+√0.015625
Write a Pythagorean triplet whose one member is (i)6(ii)14(iii)16(iv)18
Find the square root of 529
Find the square roots of 6084 by division method.
What could be the possible 'one's' digits of the square root of the following number?
99856
Find the square roots of the following decimal numbers.
i) 2.56 ii) 18.49 iii) 68.89 iv) 84.64
What could be the possible 'one's' digits of the square root of the following numbers?
9801
Simplify: (2)14.(4)18.(8)116.(16)132.
Evaluate : (172−82)12
Simplify √10151225.
Which of 772,822,1612,1092 will end with the digit 1?
Solve √444
simplify √98
A rational number whose square is 8164 is ______ .
Square of −1317 is_____
The approximate value of √50 is
Solve:-
2√6−√53√5−2√6
Find the square roots of the following numbers by prime factorization method.
441
Find the square roots of the following numbers by prime factorization method.
784
Find the square roots of the following numbers by prime factorization method.
4096
Find the square roots of the following numbers by prime factorization method.
7056
Find the square of the following :
712
Find √1276
Find:
5√25+√25−5√25=.......
Find the square root of (x2+4x+4).
Solve : 20√15
What is the square root of 18?
Solve
52+62+72+...+202
Find the last 4 digit no which is a perfect square.
Expression the following as the product of expression through prime factorization
144
Identify with reason whether the following are Pythagoras triplet 24,70,74.
Evaluate : √5.38×0.47
Evaluate: √616225
Find answer
√5x×√5x=
Find the square roots of 11918
Simplify:√35.87×0.09140.0578
Evaluate :
√28094096
Find the following .
√6146.56
Evaluate (x−1x)2−(x+1x)2
√784 by long division method
√129=z. Find the value of z.
Write the Pythagorean triplet whose one member is
(i)16
(ii)14
What is the value of 32+42?
Find the smallest number by which 882 be multiplied so that the product becomes a perfect square.
√0.6
Estimate the square root : 4√10
Estimate the square root : 3√5
Estimate the square root : 2√3
Estimate the square root : 4√2
Write down the cube root of 64
√401
Find the smallest whole number by which 1458 should be multiplied so as to get a perfect square number.
2025 plants are to be planted in a garden in such a way that each row contains as many plants as the number of rows. Find the number of plants in each row.
Determine all pairs of positive integers (m, n) for which 2m+3n is a perfect square.
A man, after a tour, finds that he had spent every day as many rupees as the number of days he had been on tour. How long did his tour last, if he had spent in all Rs. 1,296?
Out of 745 students, maximum are to be arranged in the school field for a P.T. display, such that the number of rows is equal to the number of columns. Find the number of rows if 16 students were left out after the arrangement.
State true or false.
The square root of 739.461 correct to two decimal places is 27.193.
Find the square root of 4356 by prime factorization method.
The following numbers are not perfect squares. Give reason
1057
23453
7928
What will be the unit digit of the squares of the following numbers?
81
272
799
Evaluate √7056.
Find the greatest 4-digit number, which is a perfect square. Find its square root.
Estimate the square root of 140 to the nearest integer.
5929 students are sitting in an auditorium in such a manner that there are as many students in a row as there are rows in the auditorium. How many rows are there in the auditorium?
If ABCxCBA=65125 where A,B and C are single digits the A+B+C= ?
Find the square root of a number 400 by the prime factorisation method.
For the following number, find the smallest whole number by which it should be multiplied so as to get a perfect square number.
2028
Find the square root of a number 4096 by prime factorisation method.
Find the square root of the following number by the prime factorisation method.
9216
Find the smallest whole number by which 180 should be multiplied, so as to get a perfect square number.
Find the smallest whole number by which 768 should be multiplied, so as to get a perfect square number.
Find the square root of a number 1764 by the prime factorisation method.
For the following number, find the smallest whole number by which it should be multiplied so as to get a perfect square number.
1008
Find the smallest whole number by which 2645 should be multiplied so as to get a perfect square number.
Find the smallest whole number by which 252 should be divided, so as to get a perfect square.
Find the smallest whole number by which 396 should be multiplied, so as to get a perfect square number.
Find the square root of the number 729 by the prime factorisation method.
Find the square root of 7744.
Find the square of the integer just greater than the square root of the following number:
540
Find the square of the integer just less than the square root of the following number:
5500
For each of the following numbers find the smallest whole number by which it should be multiplied so as to get a perfect square number. Also find the square root of the square number so obtained .
(i) 252 (ii) 180(iii) 1008 (iv) 2028 (v) 1458 (vi) 768
(i) 252
Find the least number that must be subtracted from 5607 so as to get a perfect square. Also, find the square root of the perfect square.
State whether 7927 is a perfect square or not.
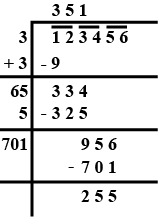
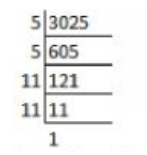
Find the square root of 33453.
Find the square root of 22222
State whether 23453 is a perfect square or not.
Find the square root of 1058.
Estimate square root of 447.
Using prime factorization method, find the square root of the following:
(i) 390625 (ii) 119025 (iii) 193600
Find the square root of the following numbers:
(i) 1444 (ii) 1849 (iii) 5776 (iv) 7921
Find the smallest number that must be added to get a perfect square.
(i) 2361 (ii) 4931 (iii) 18265 (iv) 390700
Find the square roots of the following numbers correct to two places of decimal:
(i) 1.7 (ii) 23.1 (iii) 5 (iv) 20 (v) 0.1
Find the least numbers which must be subtracted from the following numbers so as to leave a perfect square:
(i) 2361 (ii) 4931 (iii) 18265 (iv) 390700
Write true (T) or false (F) for the following statements:
(i) √0.9=0.3
(ii) If a is a natural number, then √a is a rational number.
(iii) If a is negative, then a2 is also negative.
(iv) If p and q are perfect squares, then √pq is a rational number.
(v) The square root of a prime number may be obtained approximately, but never exactly.
What is the Pythagorean Triples using the values, m=3 and n=5?
Find the Pythagorean Triples using the values, m=4 and n=8.
What is the Pythagorean Triples using the values, m=1 and n=5?
Find the Pythagorean Triples using the values, m=2 and n=3.
Find whether the following lengths are Pythagorean triples: 3,7 and 1.
Find whether the following lengths are Pythagorean triples: 6,1 and 7.
Check if the following lengths are Pythagorean triples: 8,15 and 17.
What is the Pythagorean Triples using the values, m=0 and n=2?
Check if the following lengths are Pythagorean triples: 60,61 and 11.
Determine if the following lengths are Pythagorean triples: 9,40 and 41.
Evaluate using long division method: √6241
Evaluate using long division method: √7056
Find the square root of 9.61 using long division method.
Find the square root of 79.21 using long division method.
What is the square root of 96.04 using long division method?
Find the square root of 2.89 using long division method.
For each of the following number, find the smallest whole number by which it should be multiplied so as to get a perfect square number. Also find the square root of the square number so obtained.
(i) 252 (ii) 180 (iii) 1008 (iv) 2028 (v) 1458 (vi) 768
How many numbers lie between squares of the following numbers ?
(i) 12 and 13
(ii)25 and 26
(iii) 99 and 100
What will be the unit digit of the squares of the following number ?
(i) 81 (ii) 272 (iii) 799 (iv) 3853 (v) 1234
(vi) 26387 (vii) 52698 (viii) 99880 (ix) 12796 (x) 55555
Without doing calculation, find the numbers which are surely not perfect squares.
(i)153 (ii)257 (iii)408 (iv)441
The square of which of the following number will be odd numbers?
(i) 431
(ii) 2826
(iii) 7779
(iv) 82004
What could be possible one's digit of the square root of each of the following numbers?
(i) 9801
(ii) 99856
(iii) 998001
(iv) 657666025
Find the square root of the following number by prime factorisation method
(i) 729
(ii) 400
(iii) 1764
(iv) 4096
(v) 7744
(vi) 9604
(vii) 5929
(viii) 9216
(ix) 529
(x) 8100
Let n be a product of four consecutive positive integers then n is never a perfect square
Find square root of each of the following no. by division method
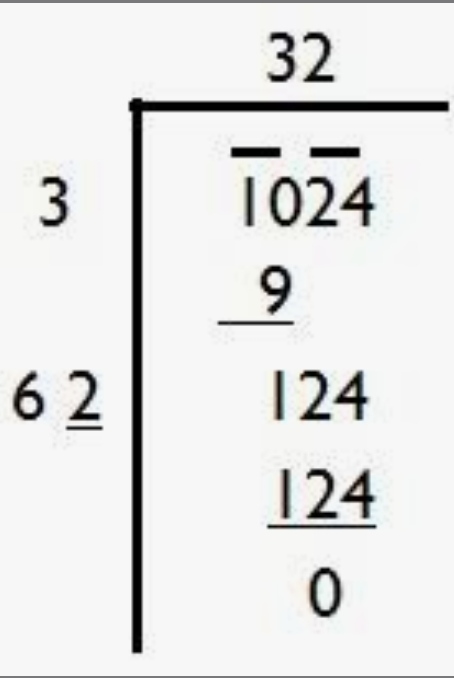
(i) 2304 (ii) 4489 (iii) 3481 (iv) 529 (v) 3249 (vi) 1369 (vii) 5776 (viii) 7921 (ix) 576 (x) 1024 (xi) 3136 (xii) 900
Identify the perfect squares among the following numbers:
1,2,3,8,36,49,65,67,71,81,169,625,125,900,100,1000,100000
Find the number of digits in the square root of each of the following number.
(i) 64 (ii) 144 (iii) 4489 (iv) 27225 (v) 390625
Find the least number which must be added from each of the following so as to get a perfect square. Also find the square root of the perfect square so obtained.
(i) 525 (ii) 1750 (iii) 252 (iv) 1825 (v) 6412
Write 3-digit numbers ending with 0,1,4,5,6,9 one for each digit, but none of them is a perfect square.
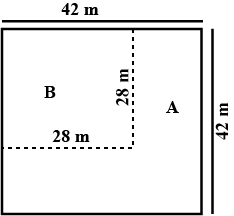
A square yard has area 1764 m2. From a corner of this yard, another square part of area 784 m2 is taken out for public utility. The remaining portion is divided in to 5 equal square parts. What is the perimeter of each of these equal parts?
Find the largest perfect square factor of each of 11280
Find the smallest square no. which is divisible by each of the numbers 4,9 and 10.
Find the square root of the following numbers by the factorisation numbers : 1296
Find the nearest integer to the square root of 728.
Find the square root of 19.5364
Find the square root of 2002225.
Find the nearest integer to the square root of 1729.
The nearest integer to the square root of 600 is ___.
Find the square root of 96721.
Find the square root of 5329 by division method
A square-field has area 3.32,929m2. It has to be fenced using barbed wire, The barbed wire should go round the field 5 times. The barbed wire is available in bundles of 100 m length. The vendor sells the wire only in complete bundles. How many bundles have to be bought?
Find the square root of the following numbers: 184.96
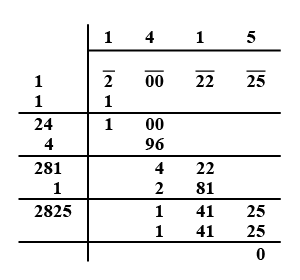
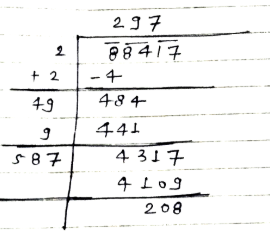
Find the least number to be added to get a perfect square: 88417
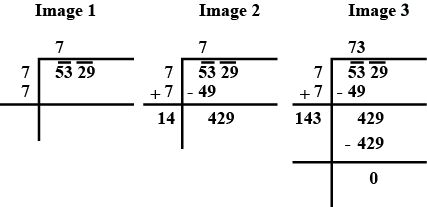
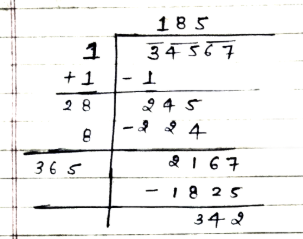
Find the least number to be subtracted from the following number to get a perfect square: 34567
Find the square root of the following number by division method: 186624
Find the least number to be added to get a perfect square: 6200
Find the consecutive perfect squares between which the following numbers lie: 56789
Find the consecutive perfect squares between which the following numbers lie: 4567
Find the square root of the following number by division method: 28224
Find the least number to be subtracted from 1234 to get a perfect square.
Check whether the following numbers form Pythagorean triplet.
6,8,10
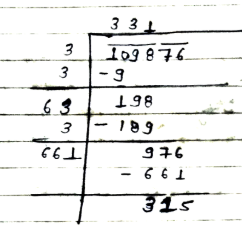
Find the least number to be subtracted from the following numbers to get a perfect square: 109876
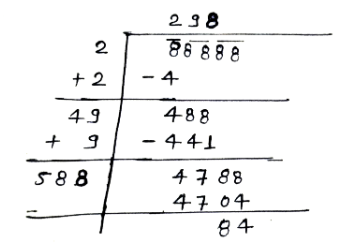
Find the consecutive perfect squares between which the following numbers lie: 88888
How many digits are there after the decimal points in the following : (1.234)2
Find the square root of the following numbers using division method: 651.7809
If a is the side of an equilateral triangle, then its area is given by √34a2. Given that the area of an equilateral triangle is 5√3 units, find its side correct to 4 decimal ploaces
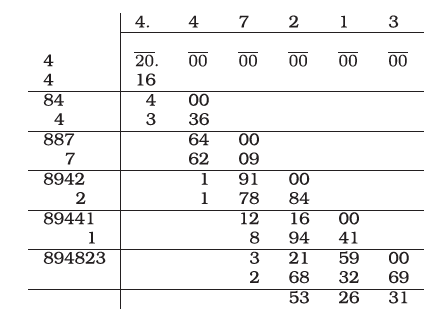
Find approximations from below to 4 decimal places to the square root of the following number: 5
A square garden has area 900 m2. Additional land measuring equal area, surrounding it, has been added to it. If the resulting plot is also in the form of a square, what is its side correct to 2 decimal places?
Find the consecutive perfect squares between which the following numbers lie: 123456
Find the square root of 3 and also correct it to 4 decimal places.
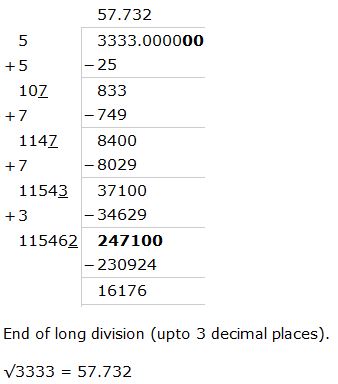
Find the square root of the following number correct to 3 decimal places: 3333
How many digits are there after the decimal points in the following : (3.16)2
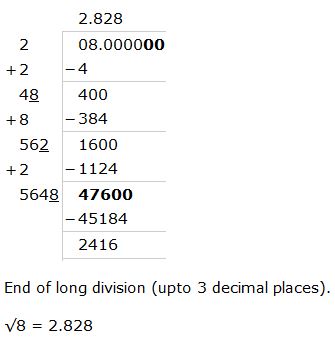
Find approximations from below to 4 decimal places to the square root of the following number: 8
Explain with reason whether the given number is perfect square or not: 257
Find the value of
(26+15√3)23−(26+15√3)−23
Which of the following numbers are perfect squares?3,5,23,25,36,26,40
To find the value of 4√−64a4.
√4x4−12x3+25x2−24x+16=?
Solve the following equations.
x√1+x2+x√1−x2=0
Find the square root of
5−√10−√15+√6
Write a Pythagorean triplet whose one member is 61416
Find the square root of a given number by prime factorization method.
2304
Which of the following are Pythagorean triplets?
i) (9, 8, 10)
ii) (4, 3, 5)
iii) (6, 8, 10)
Show that each of the following number is a perfect square. In each case find the number whose square is the given number.
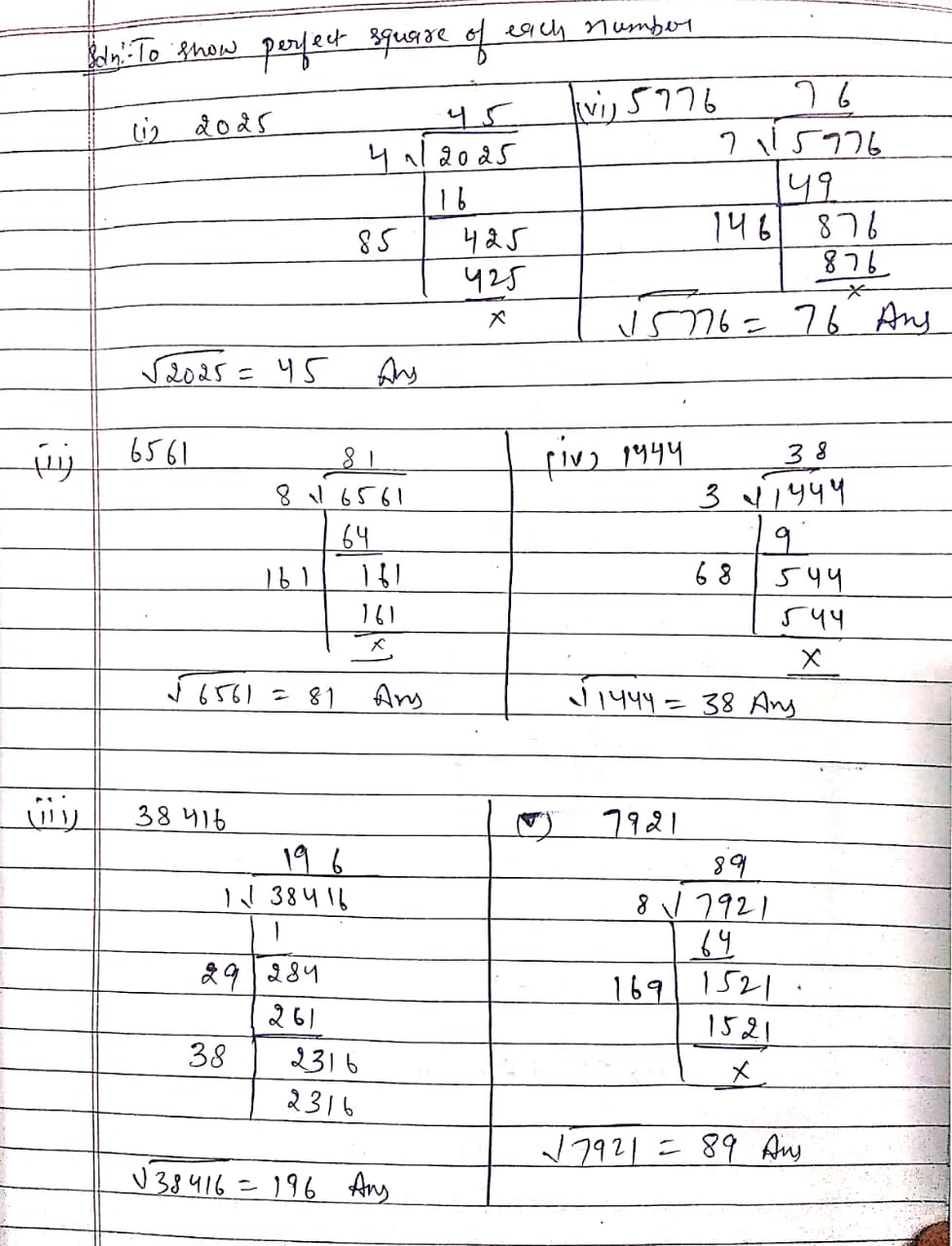
(i) 2025 (ii) 6561 (iii) 38416 (iv) 7921 (v) 1444 (vi) 5776
Find the square root of a given number by the prime factorization method.
900
Suppose m and n are any two numbers. If m2−n2,2mn and m2+n2 are the three sides of a triangle, then show that it is a right angled triangle and hence write any two pairs of Pythagorean triplet
Find the square root of 2025
√√50+√48=k(√3+√2), then k =
A student writes the formula √ab=√a√b. Then substitutes a = -1 and b = -1 finds 1 = -Explain where he is wrong.
solve: √2+√2+√2+√2...........
What is the smallest natural number that should be multiplied with 840 to make it a perfect square?
Find the fifty-sixth square number.
Find smallest No: by which 3645 must be ÷ to make it a per.square
√1806336=
Find the value:√8+√773+√116+√2+√520+81
(1035+x) chairs are placed in an auditorium in such way that the number of rows is equal to number of columns. Find the least value of x.
Find whether the square of the following numbers are even or odd?
431.
Find the square root of the following number by the prime factorisation method.
5625
Find the square roots of the following numbers by Prime factorization method.(i)441(ii)784(iii)4096(iv)7056
Find whether the square of the following numbers are even or odd?
9999
Find the smallest number by which 7776 is to be divided to get a perfect square.
Find the smallest number by which 3645 must be multiplied to get a perfect square.
Find whether the square of the following numbers are even or odd?
17779
Find whether the square of the following numbers are even or odd?
8204
Find the square root of the following number by the prime factorisation method.
11764
Find the square root of the following number by the prime factorisation method.
4096
Find the largest number of 6 digits which is a perfect square.
Find the square root of the following number by factorisation.
256
Find the square root of the following number by the prime factorisation method.
1936
Find the square root of the following number by the prime factorisation method.
7744
Find the square root of the following number by the prime factorisation method.
614
Find the square root of the following number by the prime factorisation method.
4356
Find the square root of the following number by the prime factorisation method.
1521
Find the square root of the following number by the prime factorisation method.
5929
Three-eighth of the students of a class opted for visiting an old age home. Sixteen students opted for having a nature walk. Square root of total number of students in the class opted for tree plantation in the school. The number of students who visited the old age home is same as the number of students who went for a nature walk and did tree plantation. Find the total number of students. What values are inculcated in students through such activities?
Find the square root of the following number by factorisation.
13225
Find the square root of the following number by the prime factorisation method.
289144
Estimate the value of the following numbers to the nearest whole number
(i) √97
(ii) √250
(iii) √780
Find the square root of the following number by factorisation.
10404
Find the square root of the following number by factorisation.
196
Write a Pythagorean triplet whose one member is 14.
Find the square root by long-division method:
7921
Find the square root of the following number by long division method.
1024
Find the square root of the following number by division method.
1369
Find the square root of the following number by division method.
3249
Write a Pythagorean triplet whose one member is 16.
Find the square root of the following number by long division method.
576
Find the square root of the following number by Division method.
3481
Find the square root of the following number by long division method.
529
Find the square root of following surds:
a+√a2−b2
Find the square root of the following number by Division method.
4489
Find the square root of the following number by long division method.
3136
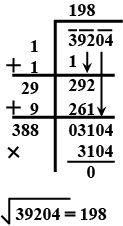
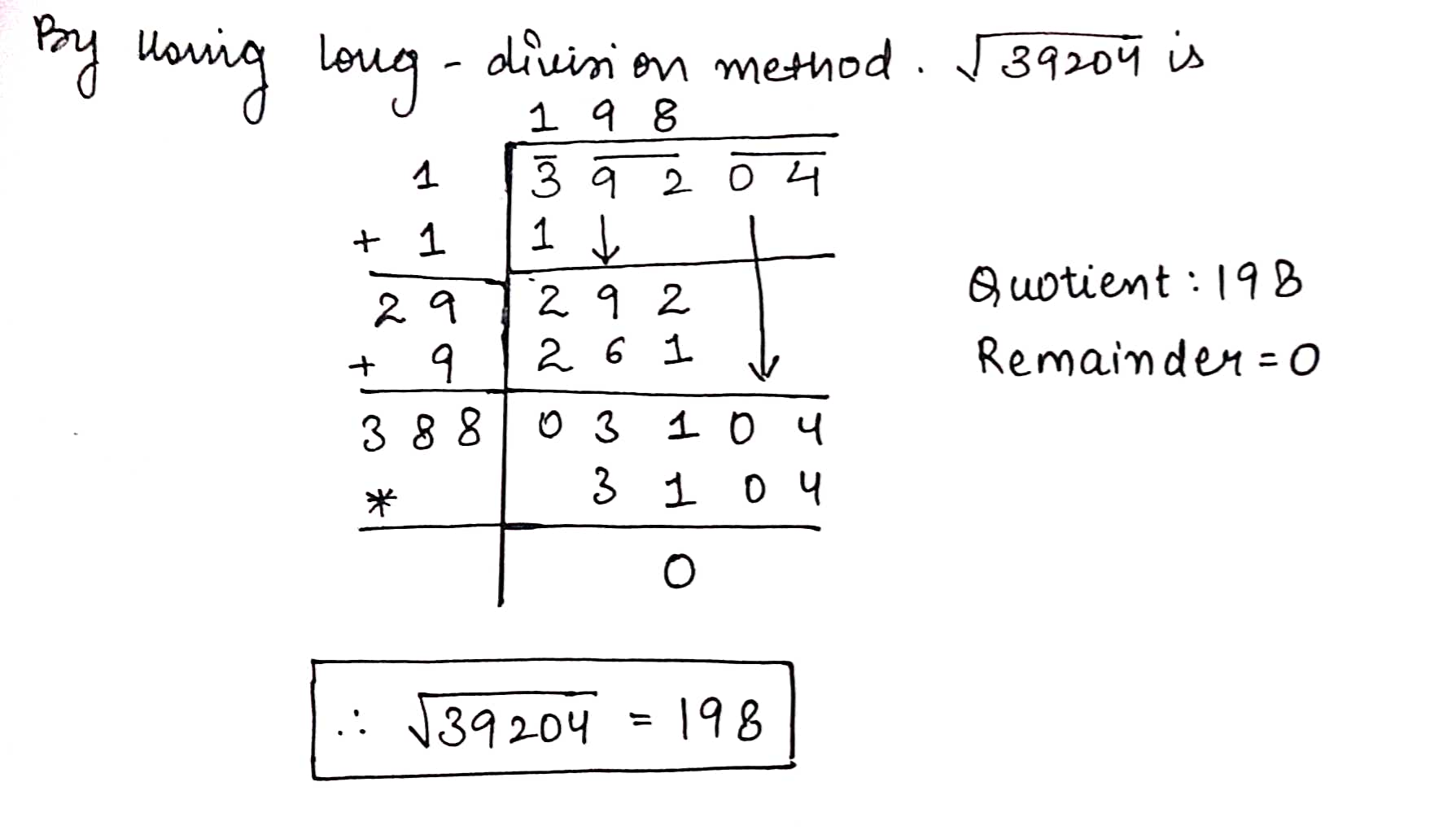
Find the √39204
Find the value of √73441 and evaluate √734.41×√7.3441
The students of 8th class of a school donate Rs.2401 in all for Prime Minister National Relief Fund. Each student donates as many rupees as the no. of students in the class. Find the no. of students in the class.
What will be the unit digit of the squares of the following numbers?
(i)81(ii)272(iii)799(iv)243(v)26387(vi)52698(vii)2796(viii)55555
Find the square root of the following number by long division method.
900
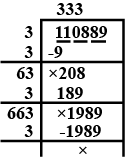
Find the value of √110889 and hence find the value of √11.0889+√1108.89
Mr. Khanna has 1200 plants. He wants to plant these in such a way that the number of rows and the number of column remain the same.
a) What is the minimum number of plants he needs more for this purpose ?
b) Which value is depicted by Mr. Khanna ?
What will be the unit digit of the square of the following numbers?
81
Find the square root by long-division method:
5776.
Find the value of √2401 using prime factorization method.
Find the square root of following surd :
7+√48
Estimate the value of the given number to the nearest whole number: √112 ?
Find the square root of:
245 correct to two places of decimal
Find the least number which must be added to 4931 to make it a perfect square ?
Find the square root of 256.
Find the square root of 1024. Hence find the value of √10.24+√0.1024+√10240000.
What will be the unit digit of the square of the following numbers?
1234
What will be the unit digit of the square of the following numbers?
55555
What will be the unit digit of the square of the following numbers?
26387
Find the smallest number by which 98 should be multiplied to make it a perfect square.
What will be the unit digit of the square of the following numbers?
52698
What will be the unit digit of the square of the following numbers?
99880
Write a phythogorean triphlets whose one of the member is 26.
What will be the unit digit of the square of the following numbers?
799
What will be the unit digit of the square of the following numbers?
3853
Find the square root of the following in the form of a binomial surd.
12+2√35.
Find the square root of: 33.64
Find the square root of the following in the form of a binomial surd.
2+23√5.
Find the square root of following surds:
8−3√7
What is the closest perfect square root of following numbers?
(i) 248 (ii) 1234 (iii) 1678.
Solve √2×(9×109)(1.6×10−19)(47)(1.6×10−19)(1.67×10−27)(2.5×10−14)
Do the following numbers make Pythagorean triplets?
(i) 8, 10, 17
(ii) 8, 10, 12
How many square tiles of side 9cm will be needed to fit in a square floor of a bathroom of side 720cm? Find the cost of tilling at the rate of Rs.7.50 per tile.
Find the least number of 4 digits which is a perfect square. Find the square roots of this number.
Solve : √1+ex1−ex
Value ϕ√∞
A group of boys collected 256 marbles for some project. The contribution of each boy was equal to the number of boys in the group.How many boys were in the group?
Find the square roots of the following perfect squares using the long division method :
a) 357096609
b) 159.7696
Estimate each root, to the nearest whole number.
(a) √171
(b) √35
(c) √407
(d) √292
Solve the term:-
12, 32, 52, 72, ......
If √6=2.55, then the value of √23+3√32 is
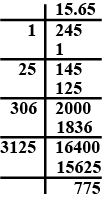
What is a Pythagorean triplet? Give some examples.
If ′n′ is odd no & n>1, them prove that (n,n2−12,n2+12) is a Pythagorean, triplet. Write two Pythagorean triplet taking suitable value of ′n′.
How many non-perfect square numbers lie between n2 and (n+1)2?
Find the square root of 97344 by prime factorization method.
Find the value of √8+√289:
In n is an odd number and n > 1, then prove that (n,n2−12,n2+12) is a Pythagorean triplet. Write two Pythagorean triplet making suitable value of n.
what will be the units digits of the square of the following number? 39, 297, 5125, 7286
Find the least number that must be added to 1500 so as to get a perfect square. Also find the square root of the perfect square.
Check whether the following numbers form Pythagorean triplet
9,10,11
Check whether the following numbers form Pythagorean triplet:-
2,3,4
Is 2352 a perfect square? If not, find the smallest number that should be multiplied to 2352 to make a perfect square. Find the square root of the new numbers.
Solve:√27x+1=9x−2
Find the square root of 12+2√35.
Check whether the following numbers form a Pythagorean triplet.
8,15,17
Find the pythagorean triplet whose smallest number is 16
Solve:
√6√6√6
Expression the following as the product of expression through prime factorization
36
Expression the following as the product of expression through prime factorization
1125
Express the following as the product of expression through prime factorization
288
Which of the following triplets are Pythagorean ?
(3,4,5),(6,7,8),(10,24,26),(2,3,4).
Square root of 375 is -
Find the least number that should be subtracted from 1,300 to get perfect square.
by what smallest number should 10584 be multiplied so that product may be a perfect square ? also find the square root of the new number.
Find the square root of the following decimal fraction :
0.09
Simplify:
3√45−√125+√200−81√30
Simplify :-
√9×63×24
625 is a perfect square number.If above statement is true, type 1 otherwise 0.
Which of the following numbers are perfect square?
2500
576 is a perfect square number.If above statement is true, type 1 otherwise 0.
Find the square root of 1296 by prime factorization method.
Simplify :√41−√21+√19−√9
Evaluate: (6+√27)−(3+√3)+(1−2√3)
941 is a perfect square number.If above statement is true, type 1 otherwise 0.
Find the Pythagorean triplets from among the following set of numbers.
3,4,5.
484 is a perfect square number.If above statement is true, type 1 otherwise 0.
961 is a perfect square number.If above statement is true, type 1 otherwise 0.
Which of the following numbers are perfect square?
11,12,16,32,36,50,64,79,81,111,121
Using prime factorization method, find which of the following numbers are perfect square?
189,225,2048,343,441,2916,11025,3549
Find the number whose square is the given following number:
4761
The following number is not perfect square. Give reason.
45743
Find the number whose square is the given number.
1156
Find the number whose square is the given number.
14641
Find the greatest number of two digits which is a perfect square.
The following number is a perfect square. Give reason.
1547
Find the least number of three digits which is perfect square.
Find the number whose square is the given number:
2025
The square of which of the following numbers would be an odd number?
3456
Show that the following number is not perfect square:
9327
The square of which of the following numbers would be an odd number?
5559
Show that the following number is not perfect square:
743522
The square of the following number would be an odd number?
42008
The number 1027 is a perfect square.Type 1 if above statement is true, otherwise 0.
Check whether the following triplet is Pythagorean?(10,24,26)
Check whether the following triplet is Pythagorean?(14,48,51)
Check whether the following triplet is Pythagorean?(16,63,65)
By just examining the units digits, can you tell whether the following can be whole square?
1024
Find the square root of the following number by prime factorization:
11664
Find the square root of the following number by prime factorization:
47089
Find the square root of the following number by prime factorization:
7056
Find the square root of the following number by prime factorization:
196
Find the square root of the following number by prime factorization:
8281
Using the prime factorization method, find if the following number is a perfect square:
441
Give reason to show that none of the numbers given below is a perfect square:
2500000
Find the square root of the following number by prime factorization:
4096
Find the square root of the following number by prime factorization:
1764
Find the square root of the following number by prime factorization:
1156
Find the square root of the following number by prime factorization:
441
Find the square root of the following number by prime factorization:
529
Find the least number of 4 digits which is a perfect.
Write the prime factorization of the following number and hence find its square root:
7056
Find the square root of the following by prime factorization:
27225
Find the square root of the following by long division method:
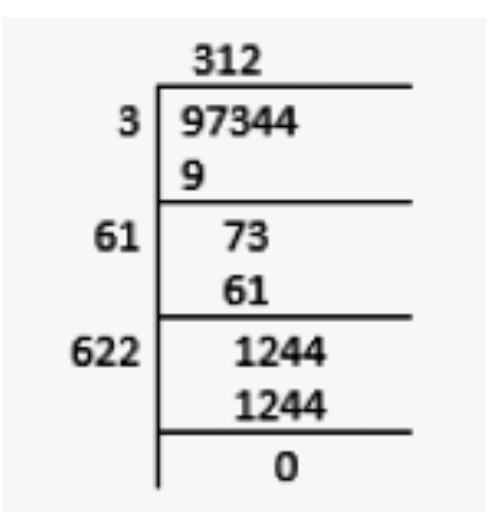
97344
Find the square root of the following by long division method:
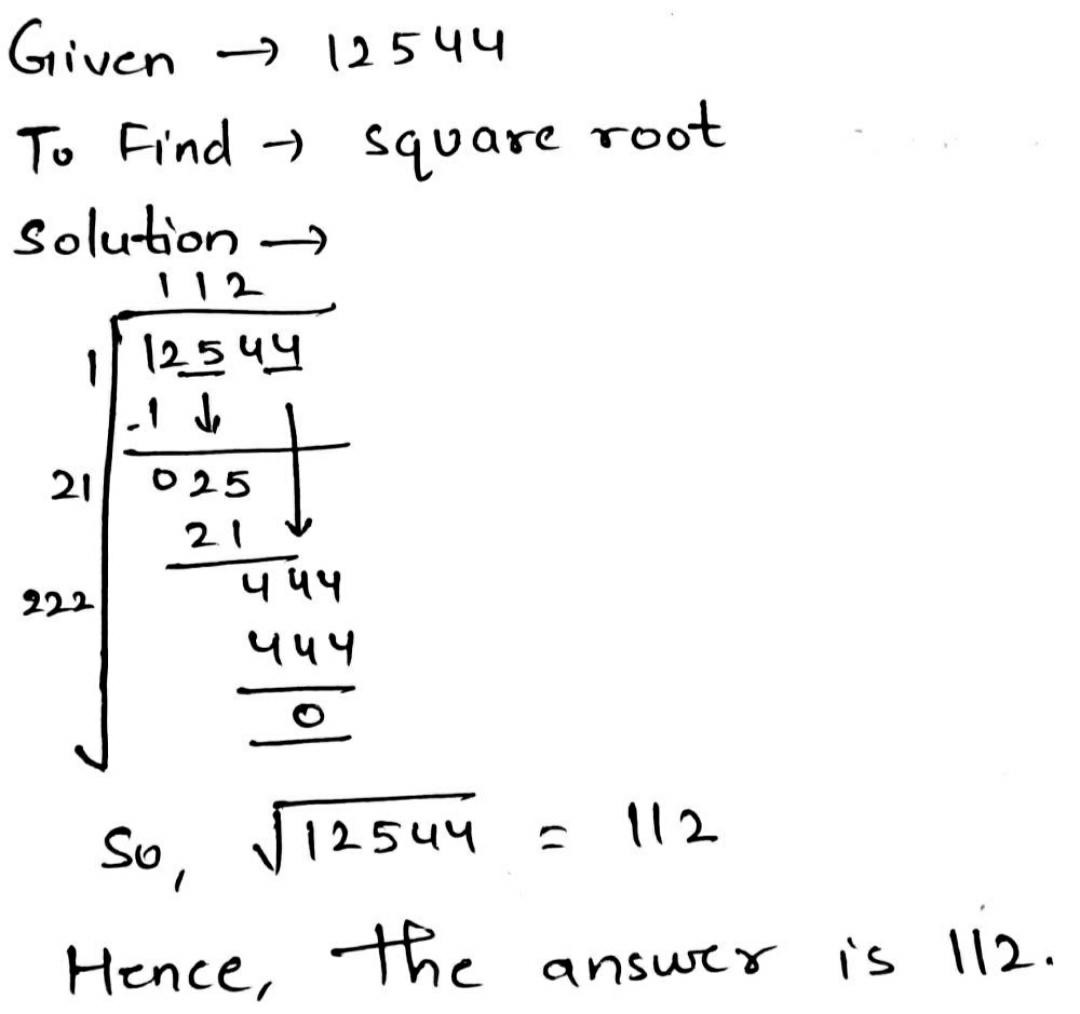
12544
Write the prime factorization of the following number and hence find its square root:
7744
Find the square root of the following by prime factorization:
24336
Write the prime factorization of the following number and hence find its square root:
9604
Using the prime factorization method, find if the following number is a perfect square:
9075
Using the prime factorization method, find if the following number is a perfect square:
1176
Which of the following squares are squares of even number?
900
Which of the following squares are squares of even number?
324
Using the prime factorization method, find if the following number is a perfect square:
11025
Using the prime factorization method, find if the following number is a perfect square:
4225
Using the prime factorization method, find if the following number is a perfect square:
5625
Which of the following are squares of even number?
441
Using the prime factorization method, find if the following number is a perfect square:
576
Which of the following are squares of even number?
625
Using the prime factorization method, find if the following number is a perfect square:
1089
Find the square root of each of the following numbers by using the method of prime factorization:
9216
Find the square root of each of the following numbers by using the method of prime factorization:
225
Find the square root of each of the following numbers by using the method of prime factorization:
1296
Find the square root of each of the following numbers by using the method of prime factorization:
441
By what least number should the given number be multiplied to get a perfect square number ? Also find the number, whose square is the new number.
9075
By what least number should the given number be multiplied to get a perfect square number ? In the case, find the number whose square is the new number.
2925
By what least number should the given number be multiplied to get a perfect square number ? In the case, find the number whose square is the new number.
3332
By what least number should the given number be multiplied to get a perfect square number ? In the case, find the number whose square is the new number.
2156
Find the smallest number by which 252 must be multiplied to get a perfect square. Also, find the square root of the perfect square so obtained.
By what least number should the given number be multiplied to get a perfect square number ? In the case, find the number whose square is the new number.
3675
Find the square root of each of the following numbers by using the method of prime factorization:
11025
Find the square root of each of the following numbers by using the method of prime factorization:
17424
Write a Pythagorean triplet whose smallest number is
6
Find the square root of each of the following numbers by using the method of prime factorization:
15876
By what least number should the given number be divided to get a perfect square number?
In the case, find the number of square is the new number
9075
By what least number should the given number be divided to get a perfect square number?
In the case, find the number of square is the new number
3380
By what least number should the given number be divided to get a perfect square number?
In the case, find the number of square is the new number
4851
Write a Pythagorean triplet whose smallest number is
16
By what least number should 2475 be multiplied to get a perfect square number ? In the case, find the number whose square is the new number.
By what least number should the given number be multiplied to get a perfect square number? Also, find the number whose square is the new number.
7623
By what least number should the given number be divided to get a perfect square number?
In the case, find the number of square is the new number
1575
By what least number should the given number be multiplied to get a perfect square number ? In the case, find the number whose square is the new number.
3380
Evaluate:
√6241
Evaluate:
√1444
Write a Pythagorean triplet whose number is
15
By what least number should the given number be divided to get a perfect square number?
In the case, find the number of square is the new number
7776
By what least number should the given number be divided to get a perfect square number?
In the case, find the number of square is the new number
4056
By what least number should the given number be divided to get a perfect square number?
In that case, find the new number which is a square number.
8820
By what least number should the given number be divided to get a perfect square number?
In the case, find the number of square is the new number
4500
Evaluate:
√4489
Evaluate:
√10404
Evaluate: √9025
Evaluate:
√7056
Evaluate:
√14161
Fill in the blanks to make the statements true.
There are ________ perfect squares between 1 and 100.
The unit digit in the square of 1294 is _______.
Fill in the blanks:
The square of an even number is.......... .
Fill in the blanks:
The square of an odd number is.......... .
Using prime factorisation, find the square roots of
a) 11025
b) 4761
Find the square root of the following by long division method.
a) 1369
b) 5625
Show that 500 is not a perfect square.
Write the Pythagorean triplet whose one of the numbers is 4.
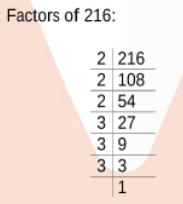
By what smallest number should 216 be divided so that the quotient is a perfect square? Also find the square root of the quotient.
Are 176 a perfect square? If not, find find the smallest number by which it should be multiplied to get a perfect square.
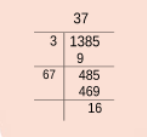
What is the least number that should be subtracted from 1385 to get a perfect square? Also find the square root of the perfect square.
A perfect square number has four digits, none of which is zero. The digits from left to right have values that are: even, even. odd, even. Find the number.
Find the greatest number of three digits which is a perfect square.
Find the decimal fraction which when multiplied by itself 84.64.
Find the least number of four digits that is a perfect square.
Find the least square number which is exactly divisible by 3, 4, 5, 6 and 8.
A decimal number is multiplied by itself. If the product is 51.84, find the number.
What is the least number that should be added to 6200 to make it a perfect square?
Find the smallest square number divisible by each one of the numbers 8, 9 and 10.
What will be the unit digit of the squares of the following numbers?
951
What will be the unit digit of the squares of the following numbers?
7625
The following numbers are obviously not perfect. Give reason.
567
What will be the unit digit of the squares of the following numbers?
5124
What will be the unit digit of the squares of the following numbers?
502
What will be the unit digit of the squares of the following numbers?
329
What will be the unit digit of the squares of the following numbers?
12796
What will be the unit digit of the squares of the following numbers?
95628
What will be the unit digit of the squares of the following numbers?
99880
What will be the unit digit of the squares of the following numbers?
68327
What will be the unit digit of the squares of the following numbers?
643
Find the square root of each of the following by division method:
2401
The following numbers are obviously not perfect. Give reason.
74000
Find the square root of each of the following by division method:
4489
The following numbers are obviously not perfect. Give reason.
2453
The square of which of the following numbers would be an odd number or an even number? Why?
8267
The following numbers are obviously not perfect. Give reason.
46292
The square of which of the following numbers would be an odd number or an even number? Why?
37916
The square of the following number would be an odd number or an even number? Why?
573
The square of which of the following numbers would be an odd number or an even number? Why?
4096
Find the smallest four- digit number which is a perfect square.
Find the least number which must be added to each of the following numbers to make them a perfect square. Also, find the square root of the perfect square number so obtained:
8000
Find the square root of the following numbers correct to two decimal places:
2
Find the square root of the following numbers by prime factorization method:
171336
Find the square root of the following numbers by prime factorization method:
1.96
Find the square root of the following numbers by prime factorization method:
967121
Find the square root of the following numbers by prime factorization method:
8836
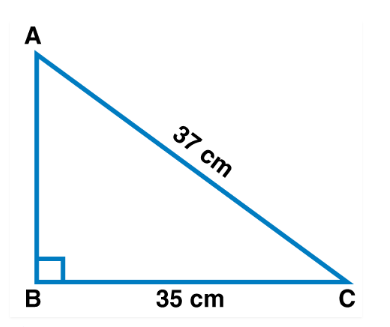
In a right triangle ABC,∠B=90o
If AC=37 cm,BC=35 cm, find AB
Show that each of the following numbers is a perfect square. Also, find the number whose square is the given number.
3025
Which of the following natural numbers are perfect squares? Give reasons in support of your answer.
1024
check if the following natural number is a perfect square? Give reasons in support of your answer.
729
Show that each of the following numbers is a perfect square. Also, find the number whose square is the given number.
1764
Show that each of the following numbers is a perfect square. Also, find the number whose square is the given number.
1296
Find the smallest number by which 2592 be multiplied so that the product is a perfect square.
Find the smallest perfect square divisible by 3,4,5 and 6.
Find the square root of:
4761
Identify , with reason , if the following is a pythagoran triplet .
(4,9,12)
Identify , with reason , if the following is a pythagoran triplet .
(3,5,4)
Identify, with reason, if the following is a Pythagorean triplet.
(5,12,13)
By splitting into prime factors, find the square root of:
396900
Find the square root of:
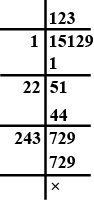
15129
Show that each of the following numbers is a perfect square. Also, find the number whose square is the given number.
3969
Find the smallest number by which 10368 be divided so that the result is a perfect square. Also, find the square root of the resulting numbers.
Divide, and write the answer in simplest form.
√310÷√5
Divide and write in simplest form.
√54÷√27
Identify , with reason , if the following is a pythagoran triplet .
(24,70,74)
Write the digit in units place when the following numbers are squared.
4,5,9,24,17,76,34,52,33,2319,18,3458,
Identify , with reason , if the following is a pythagoran triplet .
(10,24,27)
Identify , with reason , if the following is a pythagoran triplet .
(11,60,61)
Find the sum of the digits of
(111111111)2
Can 1010 be written as a difference of two perfect squares ?
What is the least perfect square which leaves the remainder 1 when divided by 7 as well as by 11 ?
What are the remainders when a perfect cube is divided by 7 ?
Find the value of √30 upto 4 places of decimal.
√0.0037
Write all the numbers from 400 to 425 which end in 2,3,7 or 8. Check if any of these in a perfect square.
What is the objective of the school administration behind such an arrangement?
Find the square root of perfect square number 10329796.
A natural number n is chosen strictly between two consecutive perfect squares. The smaller of these two squares is obtained by subtracting k from n and the larger one is obtained by adding l to n. Prove that nkl is a perfect square.
Find the square root of the following.
10.0489
Find the √39204.
Prove that 13−√8−1√8−√7+1√7−√6−1√6−√5+1√5−2=5
What will be the unit digit of the square of the following numbers?
272
What will be the unit digit of the square of the following numbers?
12796
Find the square root of following surd :
6+4√2
Find the square root of following surds:
5+√21
Solve:
√(34)1−3x=21027
By what smallest number must 180 be multiplied so that it becomes a perfect square? Also, find the square root of the number so obtained.
Find the least number which must be added to each of the following numbers to make them a perfect square. Also find the square root's of the perfect square number so obtained.
1750
Square root of √5−√21.
√244=?
Verify that the following numbers represent Pythagorean triplet.
27,36,45.
Find the value of :
√256cm2
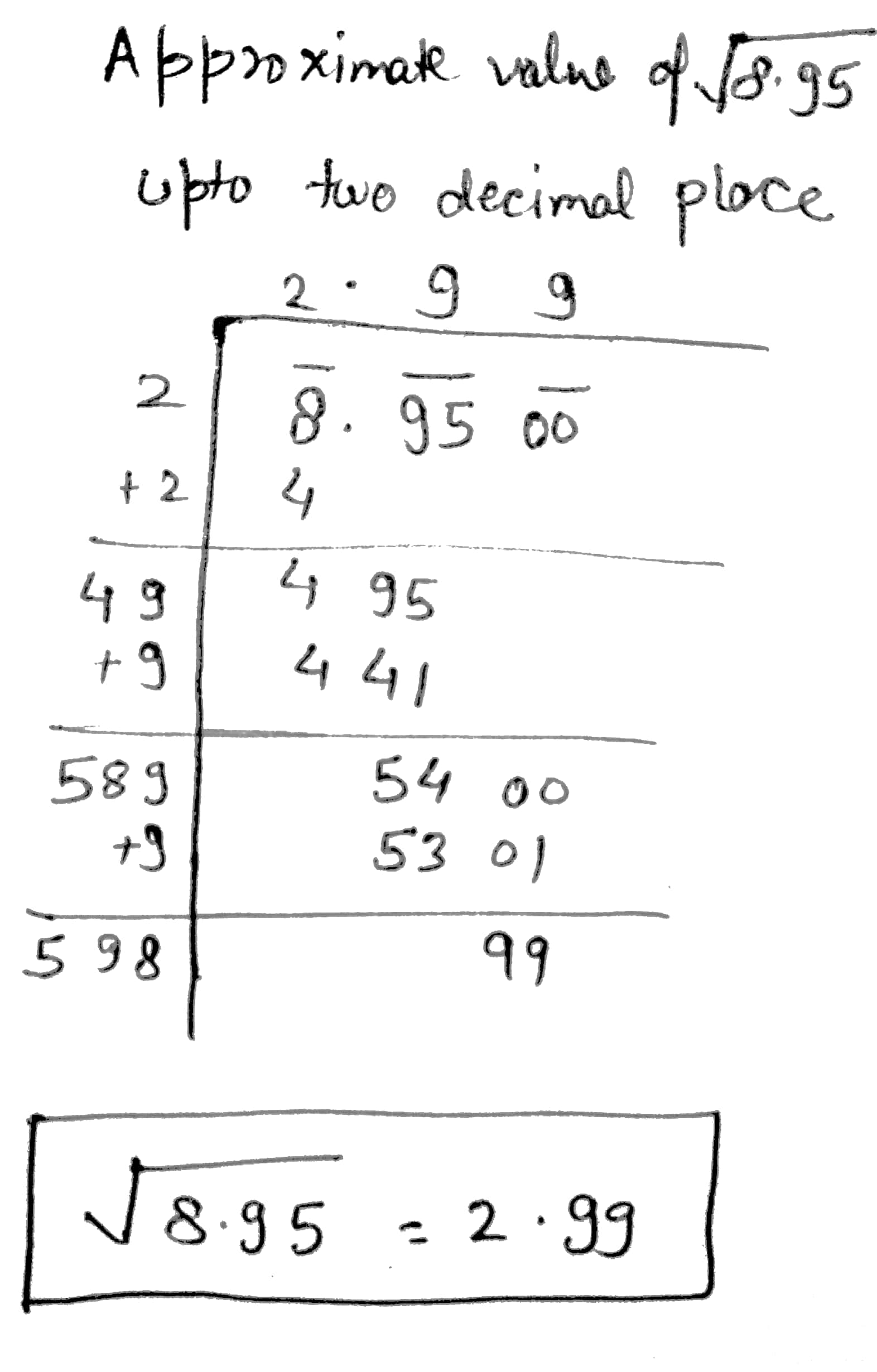
Find the approximate value of √8.95
Find the least 8 digit number which is a perfect square.
Verify that the following numbers represent Pythagorean triplet.
12,35,37.
Verify that the following numbers represent Pythagorean triplet.
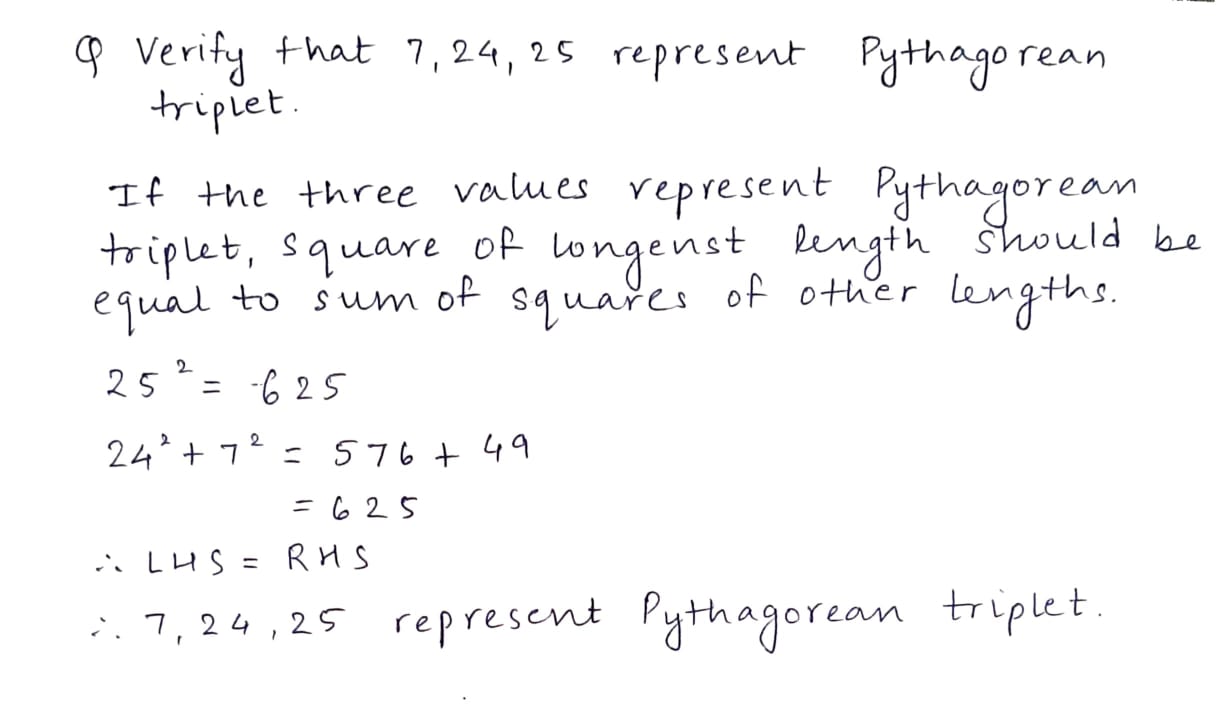
7,24,25.
Find the smallest number by which 2925 must be multiplied to get a perfect square. Also, find the square root of the perfect square so obtained.
Estimate square root : √13
Verify that the following numbers represent Pythagorean triplet.
15,36,39.
Class 8 Maths Extra Questions
- Algebraic Expressions And Identities Extra Questions
- Comparing Quantities Extra Questions
- Cubes And Cube Roots Extra Questions
- Data Handling Extra Questions
- Direct And Inverse Proportions Extra Questions
- Exponents And Powers Extra Questions
- Factorisation Extra Questions
- Introduction To Graphs Extra Questions
- Linear Equations In One Variable Extra Questions
- Mensuration Extra Questions
- Playing With Numbers Extra Questions
- Practical Geometry Extra Questions
- Rational Numbers Extra Questions
- Squares And Square Roots Extra Questions
- Understanding Quadrilaterals Extra Questions
- Visualising Solid Shapes Extra Questions