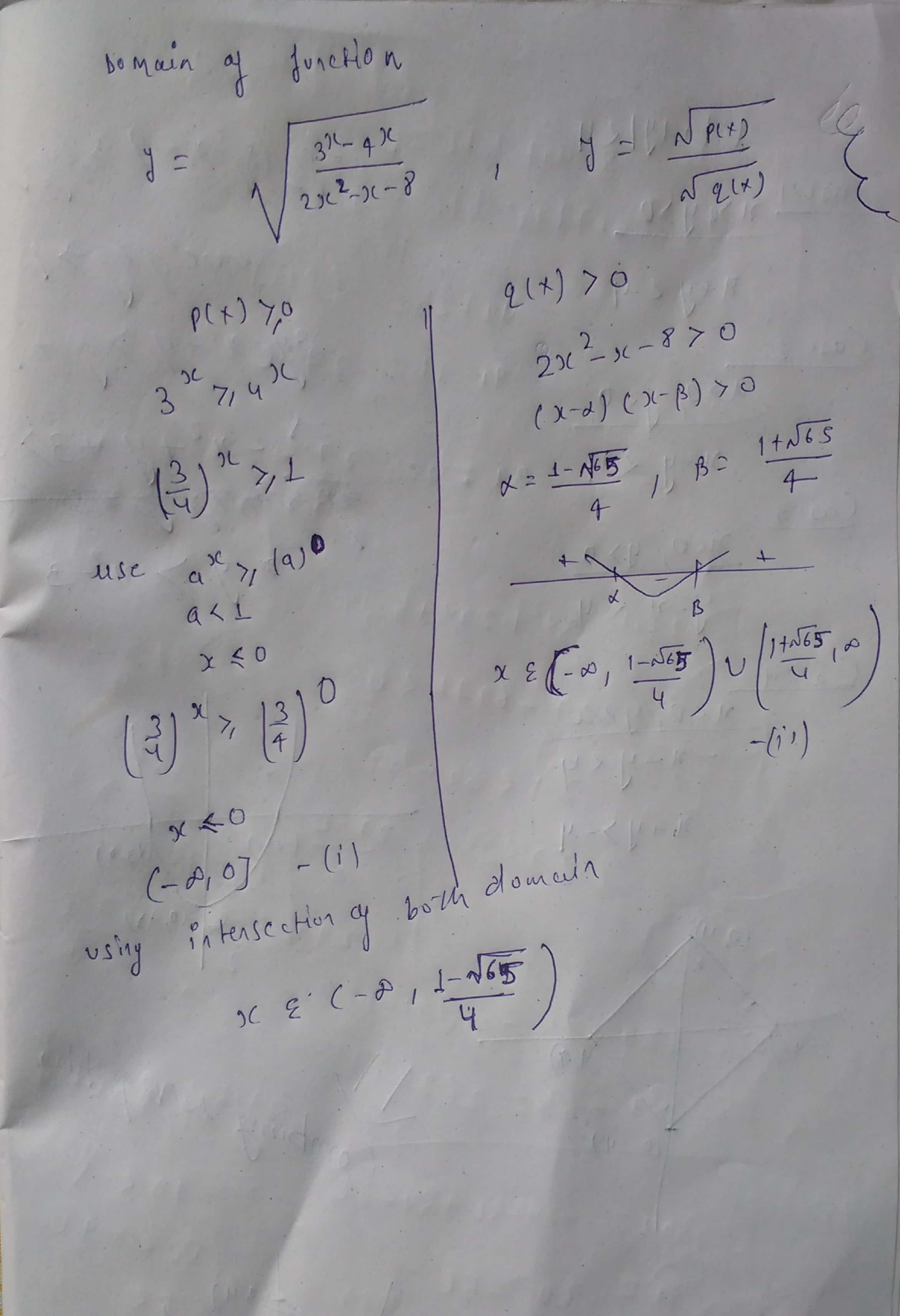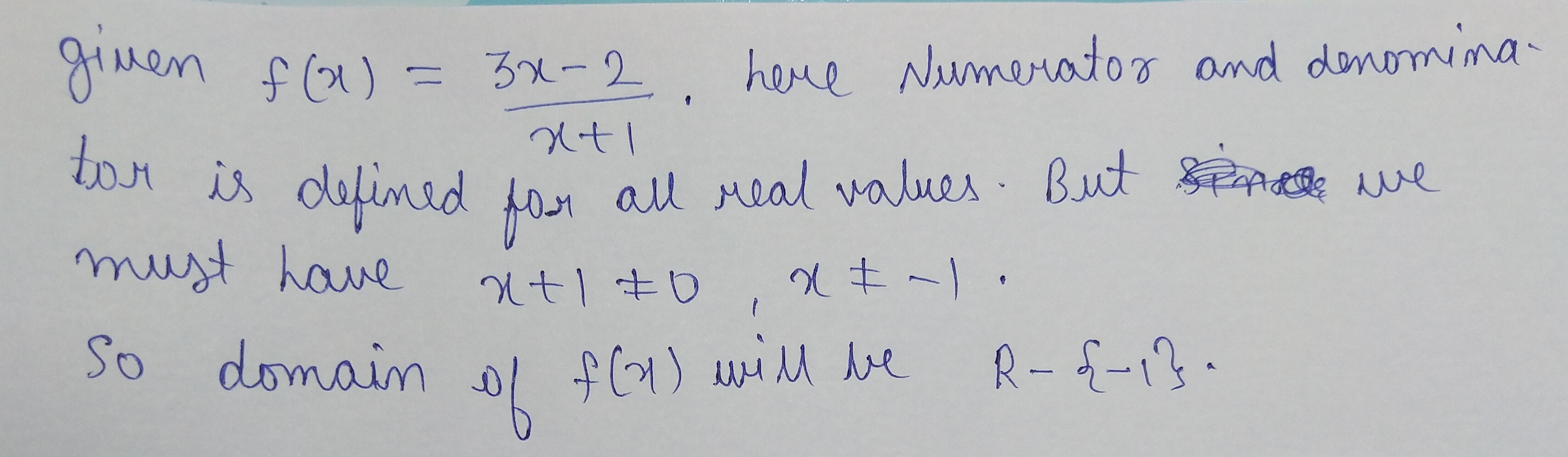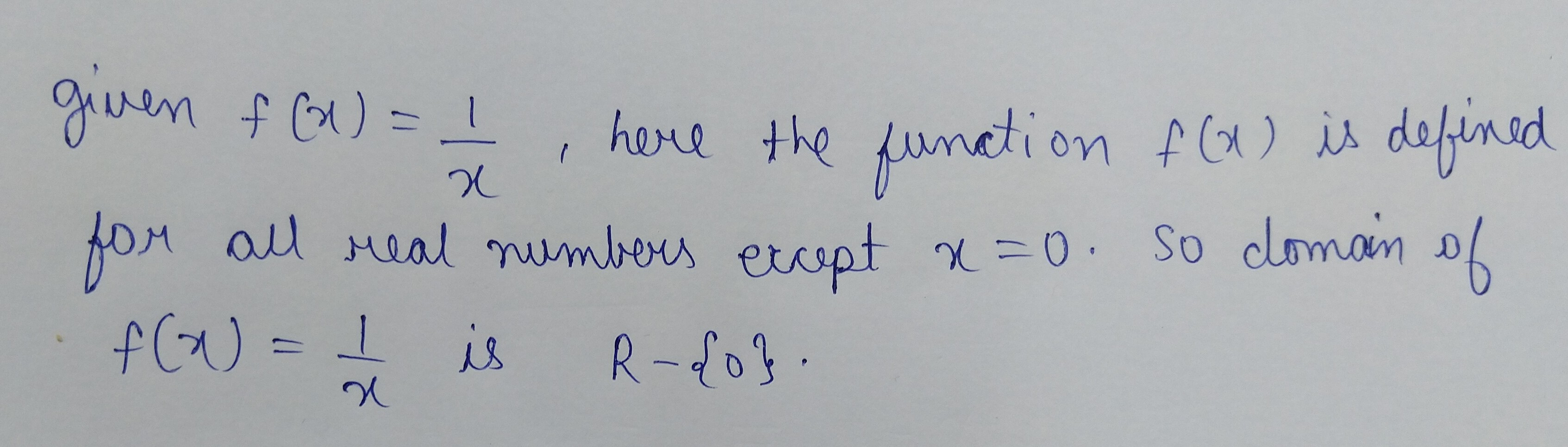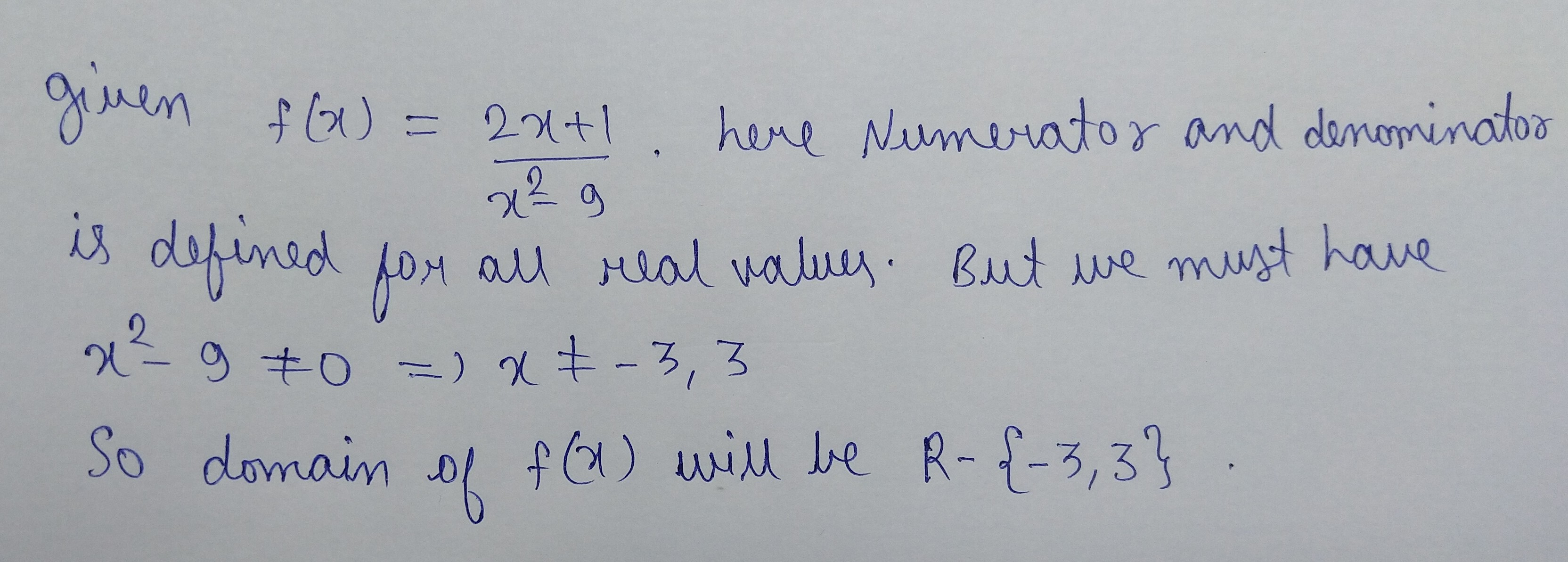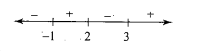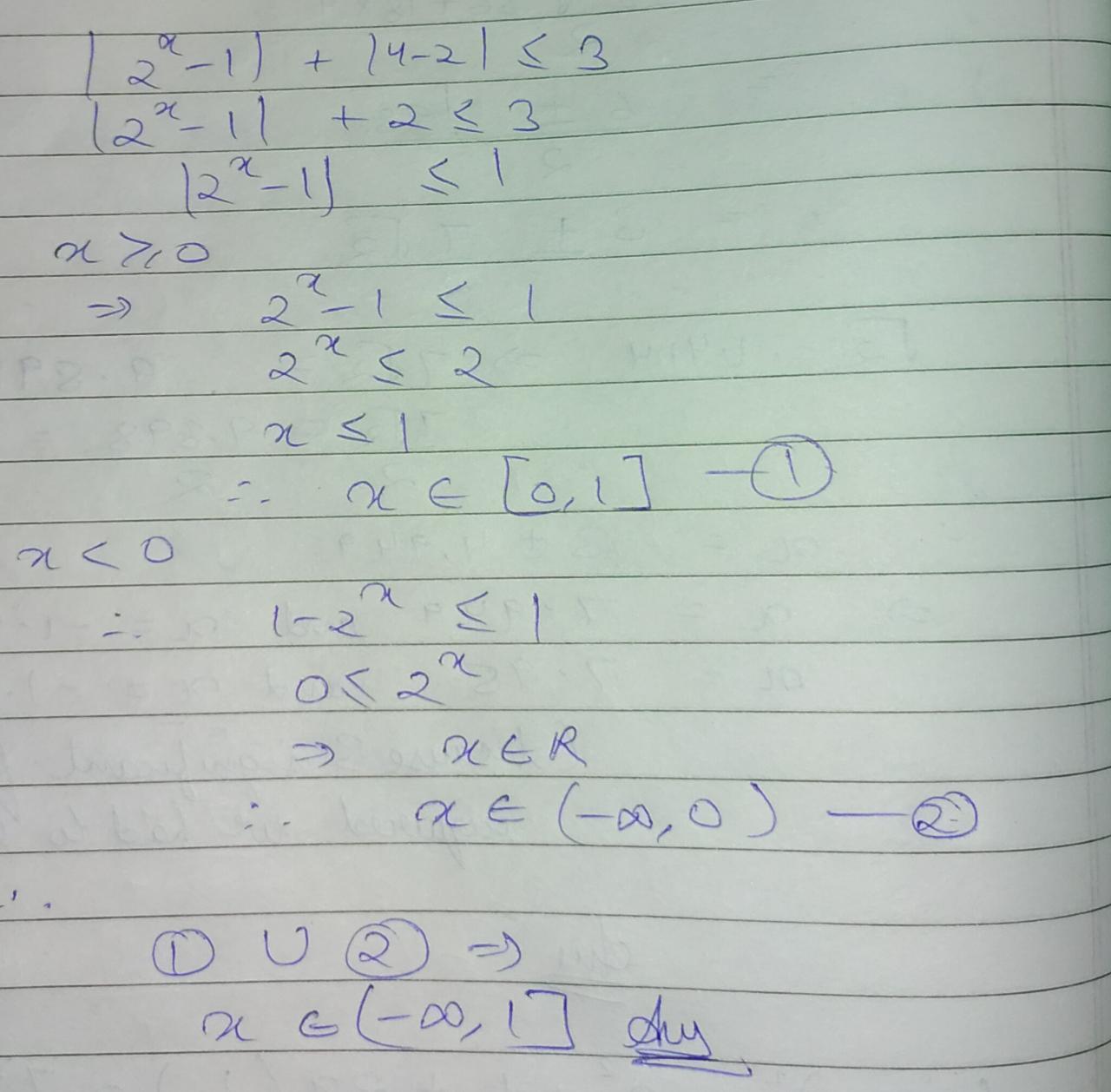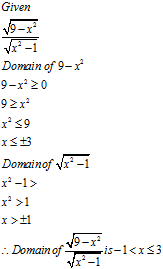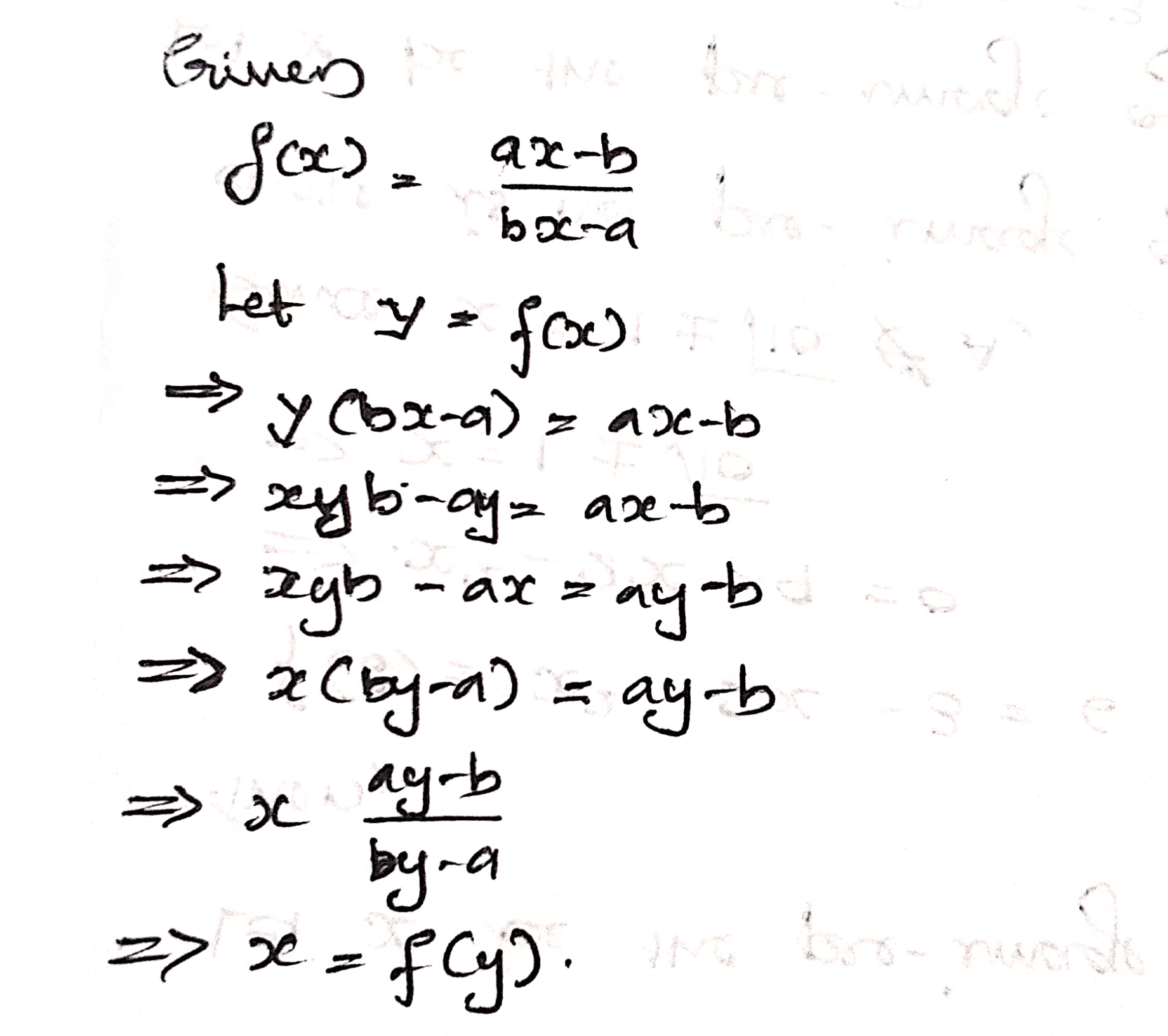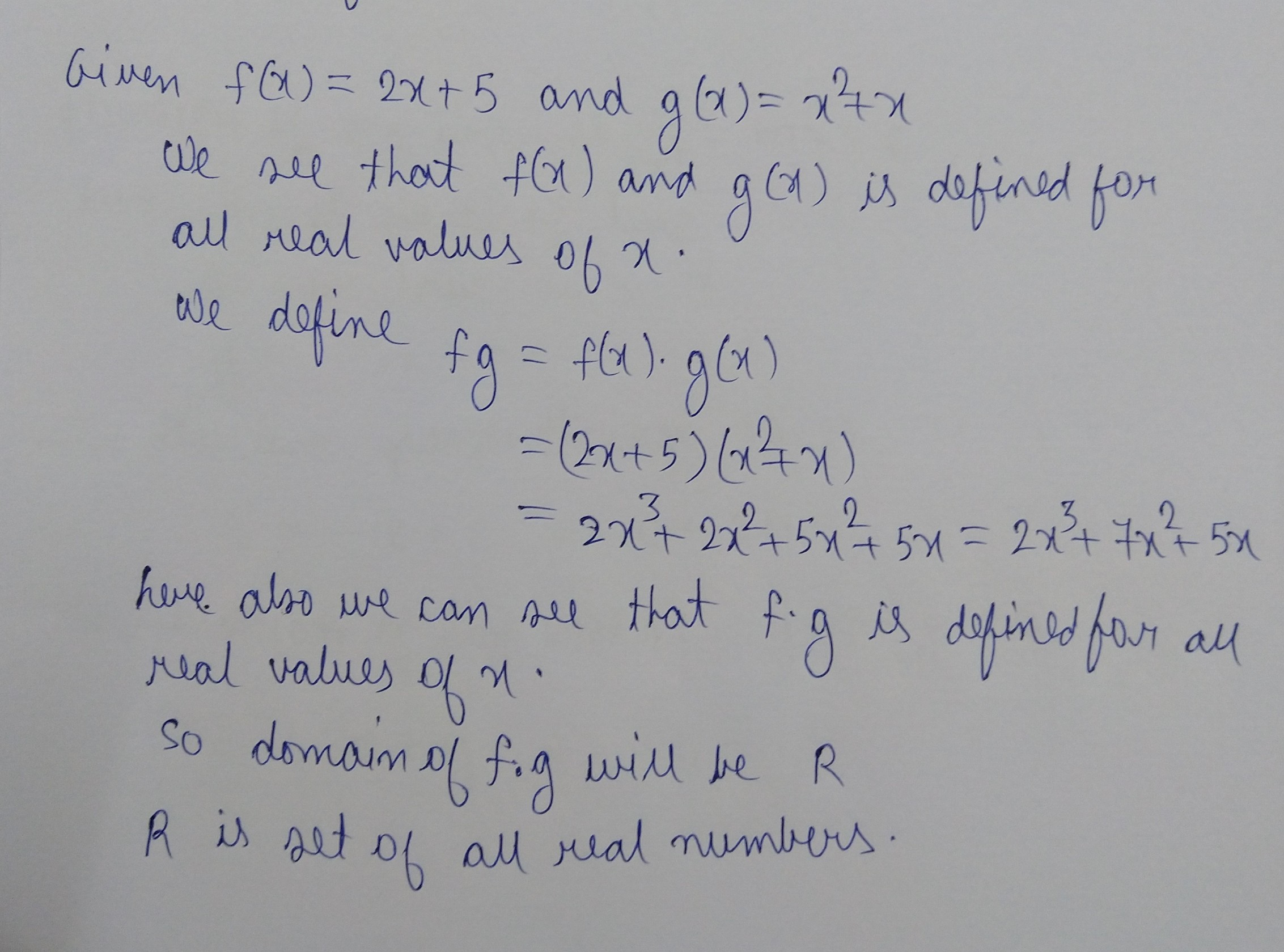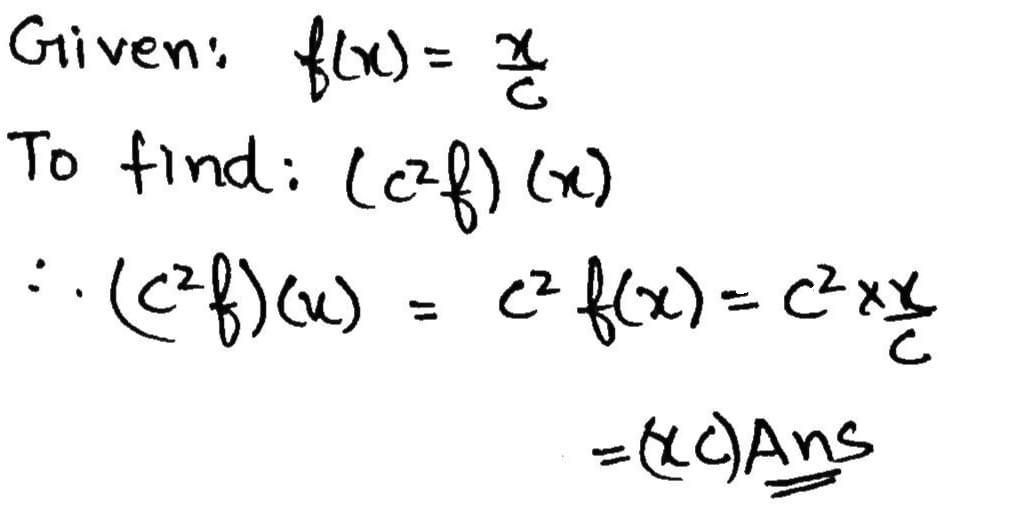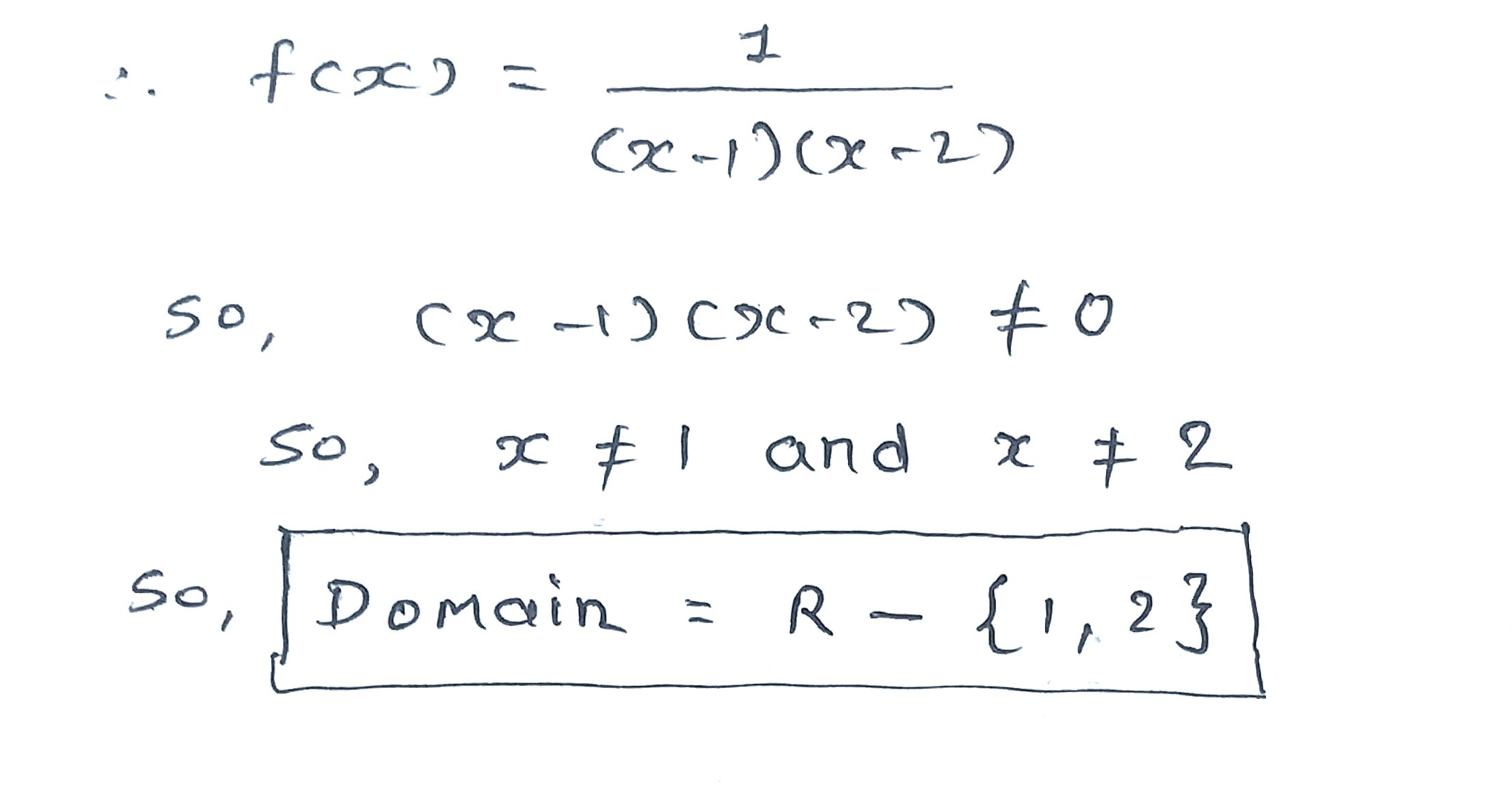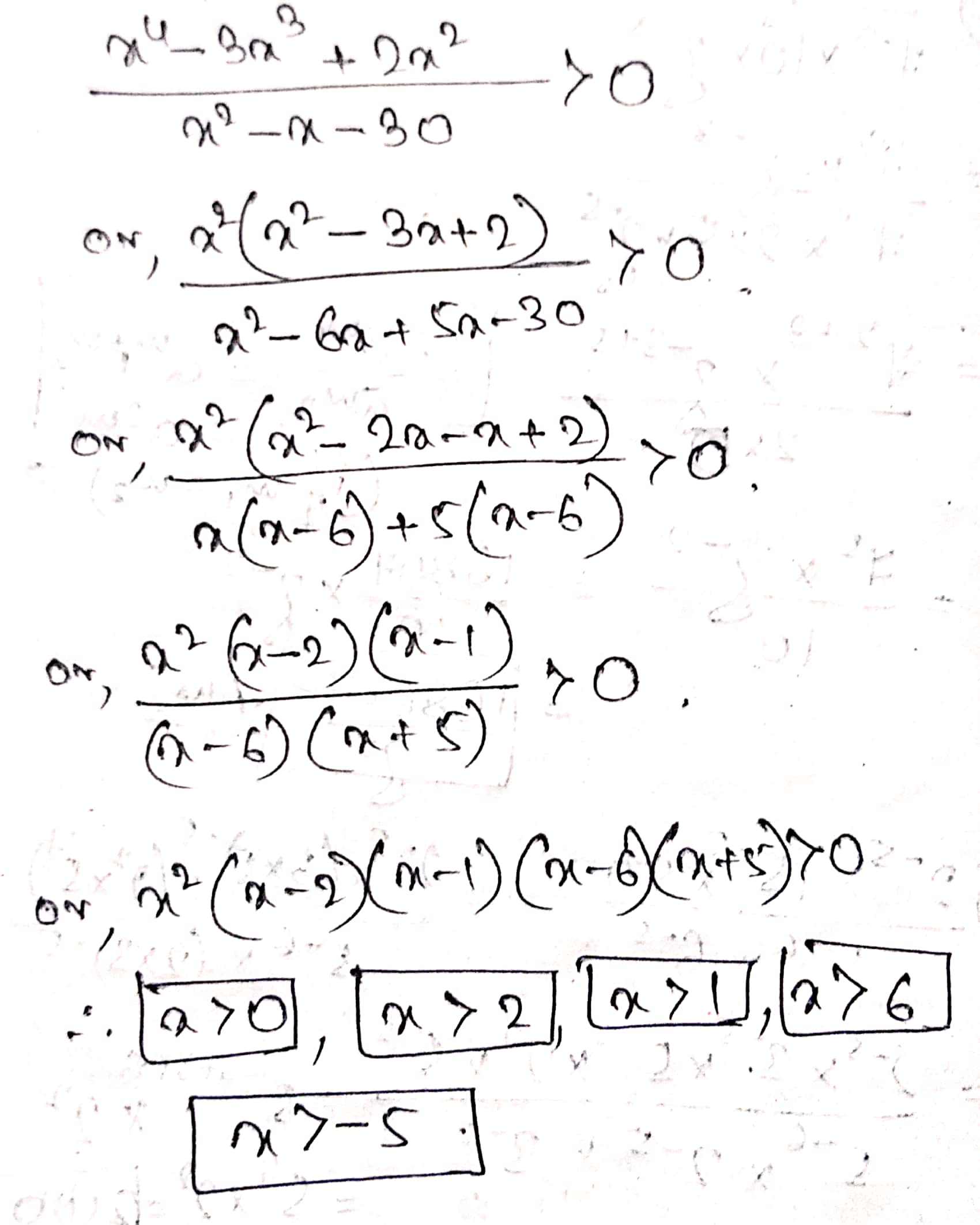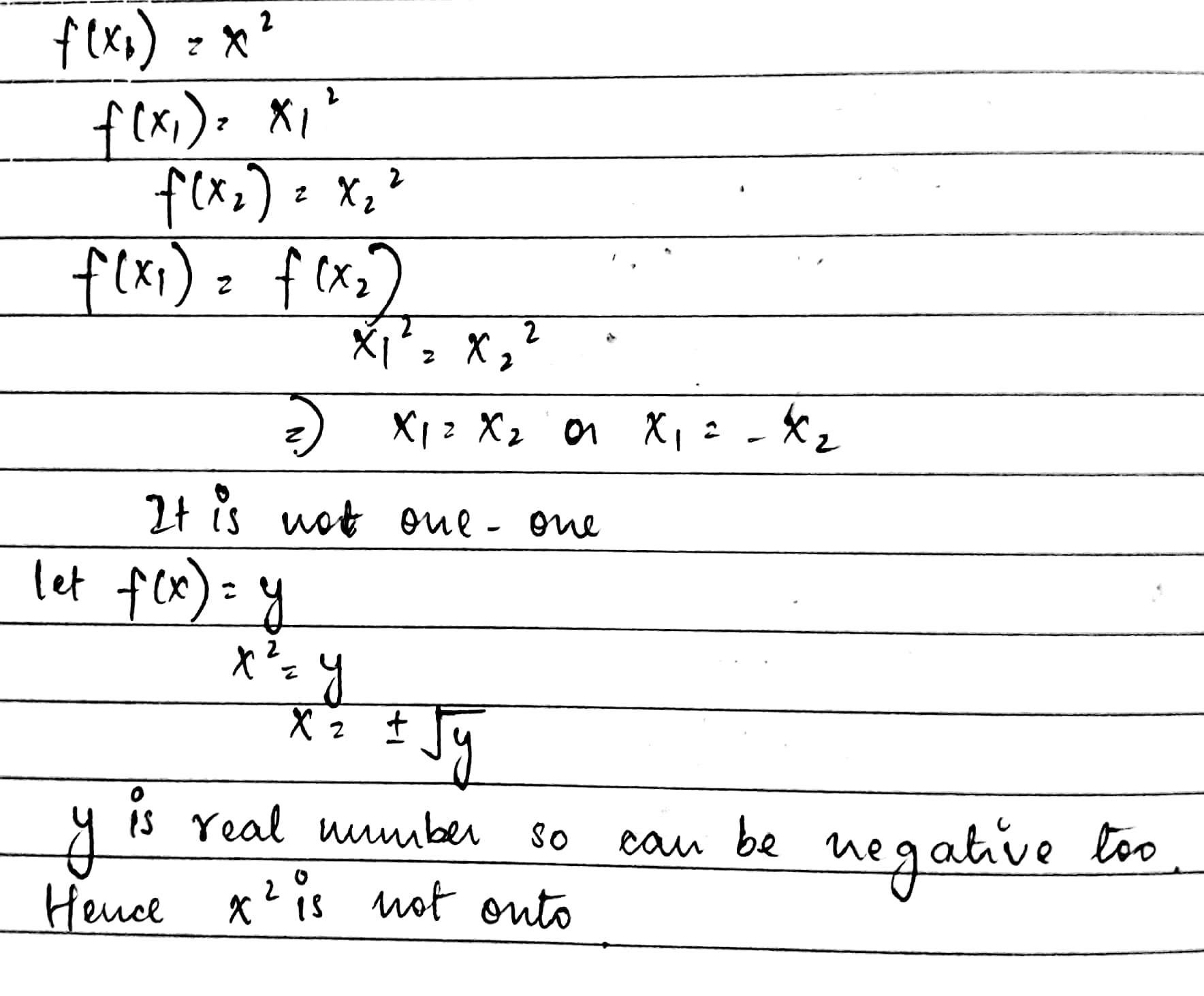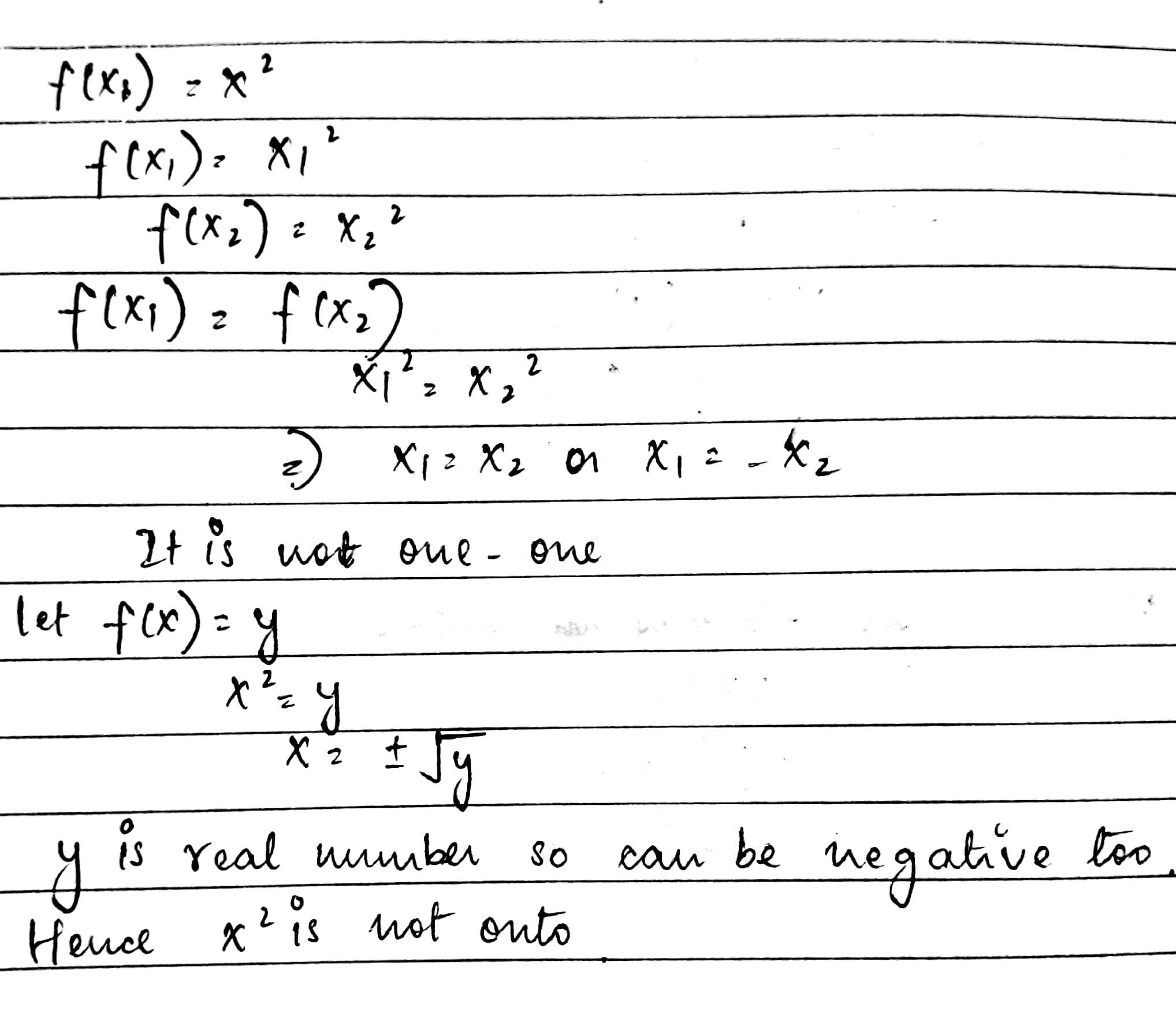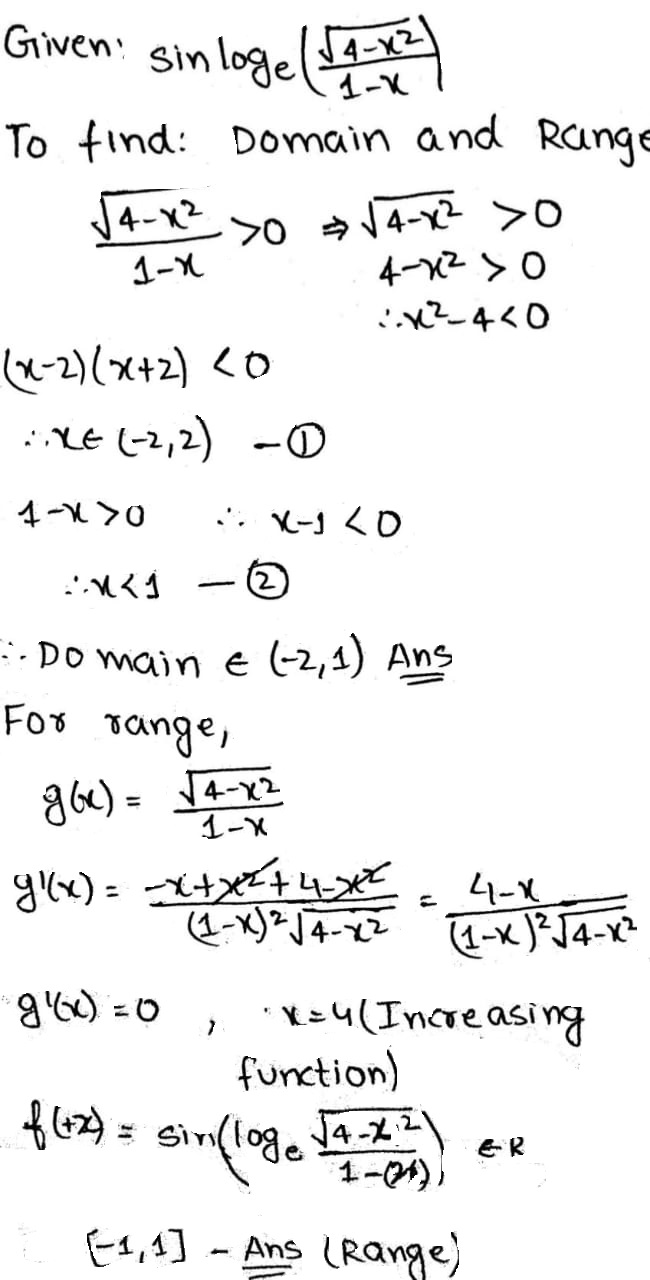Functions - Class 11 Commerce Applied Mathematics - Extra Questions
If f(x)=√x+2+1log10(1−x). Find the domain of definition of f(x).
Find the domains of definition of the following functions.
y=√6x−x2−55x−2−1.
Let f(x)=√x+2+1log10(1−x). Then find the domain of f(x).
Find the domains of definition of the following functions.
y=√3x−4x2x2−x−8.
If f(x)=2x+33x−2
Prove that fof is an identity function
Find the domain and range of :-
(i) √x−1
(ii) (x−1)
A relation R is defined on the set Z of integers as follows: (x, y)∈R′x2+y2=25
Express R and R−1 as the sets of ordered pairs and hence find their respective domains.
Find the domain and range of f(x)=√x−5
The domain of f(x)=√sin−1(log2x) is
Find the domain of the function:f(x)=1√x2−4x.
Find the domain of the function f(x)=x2√(a−x)(x−b), b>a.
Solve:
x√1+x2+log(x+√x2+1).
Find the domain of the following
(i)f(x)=√[x2]−1+√3−[x2]
Find the domain of function √25−x2
Find the domain of the following real functions :
(i)f(x)=√16−x2
The domain of Function √9−x2
The value of f(3) if f(x)=1x2−x
Find the Domain of the following function:
y=x√1+x
Find the domain for the following functions :
(a)y=1−log10x (b)y=1√x2−4x
Find the domain of y=√x−25x+5
Find the domain of the function f(x)=1√2x2+5x+2.
Find the domain and range of the real function f(x)=11−x2
Find the domain of
f(x)=√4−9x2
Find the domain and the inverse of the following functions:
f(x)=11−x2
Find the domain of f(x)=√9−x2
Find f(5) if f(x)=x2+3x+2
Find the domain and the range of the following function:
f(x)=x−32x+1
The domain of function √x2−4 is
Find the domain and the inverse of the following functions:
f(x)=x21+x2
Find the domain & range of the following function.
f(x)=x2−3x+2x2+x−6
Find the domain of each of the following real value functions real variable:
f(x)=1√x2−1
Find the domain of each of the following real valued functions of real variable:
f(x)=3x−2x+1
Find the domain of each of the following real valued functions of real variable:
f(x)=1x
Find the domain of each of the following real value functions real variable:
f(x)=√x−2
Find the domain of:
sec−1x−tan−1x.
Find the domain of each of the following real valued functions of real variable:
f(x)=2x+1x2−9
Find the domain of each of the following real valued functions of real variable:
f(x)=1x−7
Given A={2,3,4},B={2,5,6,7}. Construct an example of each of the following.
a mapping from A to B which is not injective.
Given A={2,3,4},B={2,5,6,7}. Construct an example of each of the following.
a mapping from A to B.
If the mappings, f and g are given by f={(1,2),(3,5),(4,1)} and g={(2,3),(5,1),(1,3)}.
Give an example of a map
which is not one-one but onto.
Give an example of a map
which is one-one but not onto.
Give an example of a map
which is neither one-one but nor onto.
Given A={2,3,4},B={2,5,6,7}. Construct an example of each of the following.
an injective mapping from A to B.
If domain for y=cos−1(1−2|x|3)+log|x−1|x is x∈(a,b)∪(c,d). Find a+b+c+d
Let y=√(x+1)(x−3)x−2.
Find all the real values of x for which y takes real values.
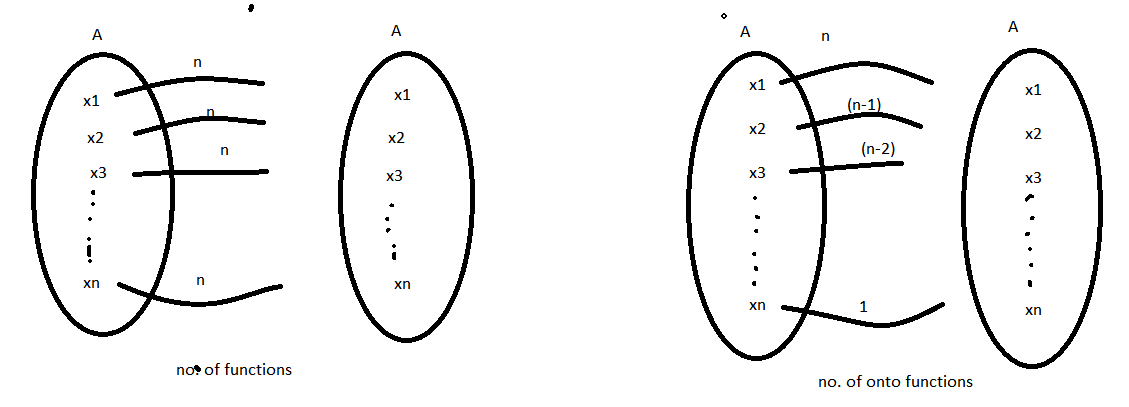
Find the number of all onto functions from the set {1,2,3,....,n} to itself
Find the domain of the real valued square root function f given below
f(x)=√(2x)
If the domain of the real valued function f given below
f(x)=√−x+1x+3 is (−∞,−x)∪(−x,1].Find x
Find the domain of the real valued rational function f given below
f(x)=1/(x3+x2−2x)
If the domain of f(x)=√2x+1 is [−1m,∞).Find m
The domain of the real valued sine function f(x)=2sin(x−1) is (−∞,∞). If true enter 1 else0.
Find the domain of the real valued exponential function f given below
f(x)=e(x−4)
Show that, if f:R−{75}→R−{35} is defined by f (x) = 3x+45x−7 and g:R−{35}→R−{75} is defined by g(x) = 7x+45x−3,
then log = lA and gof = lB, where lA and lB are called identity functions on sets A and B.
Find the domains of definition of the following functions.
y=√sinx+√16−x2.
Let A be a set of n distinct elements. Then, the total number of distinct functions from A to A is .... and out of these .... are onto functions.
Let f:D→R, where D is the domain of f. Find the inverse of f, if it exists
f(x)=1−2−x
f(x)=(4−(x−7)3)1/5
f(x)=ℓn(x+√1+x2)
Find the domains of definition of the following functions.
y=x√x2−5x+6.
Let f:N→N be defined by f(n)={n+12,if n is oddn2,if n is even.n∈N
State whether the function f is bijective. Justify your answer.
If :R→R defined by f(x)=1+x2, then show that f is neither 1−1 nor onto
Find the domain of f(x)=√([x]−1)+√(4−[x])
(where[] represents the greater integer function)
show that f:A→B and g:B→C are onto, then gof:A→ is also onto:
Let f:[α,∞)→[x,∞),f(x)=x2−6x+5 then find three possible value of ′α′ if f(x) is onto.
Let f(x)=√2−x−x2 and g(x)=cosx. Check given statement is true or not?
I. Domain of f((g(x))2) = Domain of f(g(x))
Find the domain of f(x)=√sinx+√16−x2.
Find the domain of f(x)=log10log2logπ/2(tan−1x)−1
Let f:N→Y be a function defined by f(x)=4x2+12x+15, where Y= range of f. Show thatf is invertible and find the inverse of f.
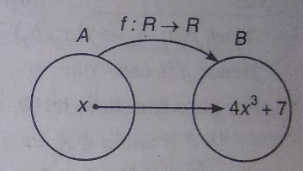
If f:R→R be the function defined by f(x)=4x3+7, then show that f is a bijection.
Find the domain for which the function f(x)=3x2−2x and g(x)=9x−6 are equal.
Evaluate:∫sin2xcos2xdx
Simplify 11+am−n+11+an−m
Find the domain of
f(x)=√([x]−1)+√(4−[x])
(where [ ] represents the greatest integer function).
Find the domain of f(x)=√x+2+log10(1−x)
The domain of definition of the function y(x) given by 2x+2y=2 is
If f:R→S,defined by f(x)=sinx−√3cosx+1, is onto, then find the set S ?
Domain of f(x)=x2+3x+5x2−5x+4 is
Show that f:N→N = x−1, if x is even is both one-one and onto
Let f:R→R be defined by i) f(x)=x+1 ii) f(x)=x+|x|. Determine whether or not f is onto.
The domain of 1√x−x2+√3x−1−2x2 is:
The domain of the function x√x2−3x+2 is
If f(x+y)=f(xy)∀x,yϵR then prove that f is a constant function.
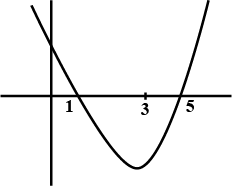
The domain of the function√x2−5x+6+√2x+8−x2is
The domain of f(x)=x2−8x−2
f(x) and g(x) are linear function such that for all x, f(g(x)) and g(f(x)) are Identity functions. If f(0) = 4 and g(5) = 17, compute f(2006)
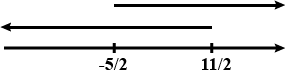
Solve: |x−1|x+2<1
Find the domain of f(x)=√cos(sinx)
Find the domain x3−5x+3x2−1
Find domain of the function f(x)=√4−√5+2x.
Find the solution of the inequation |2x−1|+|4−2|≤3
Find the largest possible domain for the real valued function given by f(x)=√9−x2√x2−1.
Find the largest possible domain for the real-valued function given byf(x)=√9−x2√x2−1
Write the domain of the real function f(x)=√[x]−x.
Write the domain of the real function f(x)=√x−[x].
If f:A→B and g:B→C are onto functions, then show that g∘f is an onto function.
Write the domain of the real function f(x)=1√[x]−x.
Write the domain of the real function f defined by f(x)=√25−x2.
Find the domain of each of the following real value functions real variable:
f(x)=√9−x2
Find the domain and range of each of the following real value functions:
f(x)=ax−bcx−d
Find the domain and range of each of the following real value functions:
f(x)=ax−bbx−a
Find f(g(x)).f(x)=5x2−2x+3 and g(x)=4x2+7x−5.
Let f(x)=2x+5 and g(x)=x2+x. Describe f−g
Find the domain in each case.
Let f(x)=2x+5 and g(x)=x2+x. Describe fg
Find the domain in each case.
Find the domain and range of each of the following real value functions:
f(x)=√x−3
Let f(x)=2x+5 and g(x)=x2+x. Describe f/g
Find the domain in each case.
Let f(x)=2x+5 and g(x)=x2+x. Describe f+g
Find the domain in each case.
Give an example of a function which is onto but not one-one.
Let f={(0,−5),(1,−2),(2,1),(3,4),(4,7)} be linear function from Z into Z. Write an expression for f.
Let f:R→R:f(x)=xc,where c is constant.
Find (c2f)(x)
Let f:R→R:f(x)=xc,where c is constant.
Find (1cf)(x)
Let f:R→R:f(x)=xc,where c is constant.
Find (cf)(x).
Are the following set of ordered pairs function? If so, examine whether the mapping is injective or surjective.
{(x,y):x is a person ,y is the mother of x}.
Are the following set of ordered pairs function? If so, examine whether the mapping is injective or surjective.
{(a,b):a is a person ,b is an ancestor of a}.
The function 't' which maps temperature in degree Celsius into temperature in degree Fahrenheit is defined by t(c)=9C5+32. Find
(i) t (0)
The function 't' which maps temperature in degree Celsius into temperature in degree Fahrenheit is defined by t(c)=9C5+32. Find
(ii) t (28)
The function 't' which maps temperature in degree Celsius into temperature in degree Fahrenheit is defined by t(c)=9C5+32. Find
(iii) t (-10)
The function ′t′ which maps temperature in degree Celsius into temperature in degree Fahrenheit is defined by t(c)=9C5+32. Find the value of C, when t(C)=212.
Is the function sin(sin−1n) bijective? Which is defined on [−1,1] to [−1,1]
Write the domain and range of the function f(x)=x3+1
Examine which of the following is / are functions :
{( 1 ,a)(2 , b)( 1 ,b)(2 ,a)}
Find the domain and range of the following real function:
f(x)=|x−1|
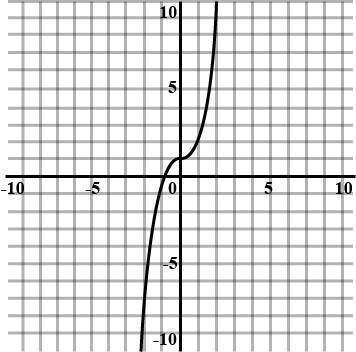
Define an identity function and draw its graph also find its domain and range.
Examine which of the following is / are functions :
{(a , 0)(b ,0), (c ,1)( d , 1)}
Examine which of the following is / are functions :
{(1 ,2),(2 ,3),(3 , 4), ( 2 , 1)}
Define a constant function and draw its graph also find its domain and range.
Examine which of the following is / are functions :
{( a ,a)(b , b)( c ,c)}
Examine which of the following is / are functions :
{( a ,b)}
Examine which of the following is / are functions :
\left \{ ( x ,y): x , y \in R , y^{2} = x \right \}
Examine which of the following is / are functions :
\left \{ (x , y) : x , y \in R , x = y^{3} \right \}
Examine which of the following is / are functions :
{( 4 , 1)(4,2),(4 , 3), ( 4 , 4)}
Examine which of the following is / are functions :
{( 1, 4)(2 , 4),(3 , 4), ( 4 , 4 )}
Examine which of the following is / are functions :
\left \{ ( x , y) : x , y \in R , x^{2} = y \right \}
A function R\rightarrow R is defined by \displaystyle f\left ( x \right )=\frac{\alpha x^{2}+6x-8}{\alpha +6x-8x^{2}}. If f is an onto function for \alpha \in [P, Q] then P + Q is
Find the domain of the real valued linear function f given below
f(x)=x+1
Find the domain of the real valued linear function f given as below:
f(x)=\dfrac{(x-1)}{(x-3)}
Find the domain of the real valued logarithmic function f given below
f(x)=\ln({x}^{2}-9)
Indicate the domain of definition of the function and simplify the given expression.
\displaystyle\, y = x\frac{1 + 2(x + 4)^{-0.5}}{2 - (x + 4)^{0.5}} + (x + 4)^{0.5} + 4(x + 4)^{-0.5}
Let f=\left\{(1,1),(2,3),(0,-1),(-1,3)\right\} be a linear function from z to z , find f(x) where x is the set of integers.
Find the domain of \sqrt[3]{5x-3}
Find the domain of \cfrac{1}{((x-1)(x-2)}.
The domain of the real-valued function f\left( x \right) =\log_{10}{\sqrt{\cfrac{(3-x)(x+2)}{(x+1)(x-4)}}} does not contain the intervals (-\infty,-5) and (5,6).
find the domain of x\dfrac{x^4-3x^3+2x^2}{x^2-x-30} > 0.
Give an example of a function which is neither one-one nor onto.
Show that the function f:R\rightarrow R:f(x)=x^2 is neither one-one nor onto.
Show that the function f: R\rightarrow R:f(x)=\sin x is neither one-one nor onto.
Let X = \{ 1, 2, 3 ,4\}, Y = \{1,5,9, 11, 15 , 16 \} and f = \{ (1,5) , (2,9), ( 3,1), (4,5), 92, 11)\}. Are the following true?
(i) f is a relation from X to Y.
(ii) f is function from X to Y. Justify your answer in each case.
Number of integers in the domain of function, satisfying f(x) + f(x^{-1}) = \dfrac{x^3 + 1}{x}, is
If f ( x + \frac {1}{x} ) = (x^2 +\frac {1}{x^2} ) for all x \epsilon R - \left\{0 \right\} then write an expression for f(x) .
If f(x) = sin log_e \left ( \frac{\sqrt{4 - x^2}}{1 - x} \right ), then the domain of f(x) is_________ and its range is _______________. (IIT-JEE, 1985)
Class 11 Commerce Applied Mathematics Extra Questions
- Basics Of Financial Mathematics Extra Questions
- Circles Extra Questions
- Descriptive Statistics Extra Questions
- Differentiation Extra Questions
- Functions Extra Questions
- Limits And Continuity Extra Questions
- Logarithm And Antilogarithm Extra Questions
- Mathematical And Logical Reasoning Extra Questions
- Number Theory Extra Questions
- Numerical Applications Extra Questions
- Permutations And Combinations Extra Questions
- Probability Extra Questions
- Relations Extra Questions
- Sequences And Series Extra Questions
- Set Theory Extra Questions
- Straight Lines Extra Questions
- Tangents And Its Equations Extra Questions