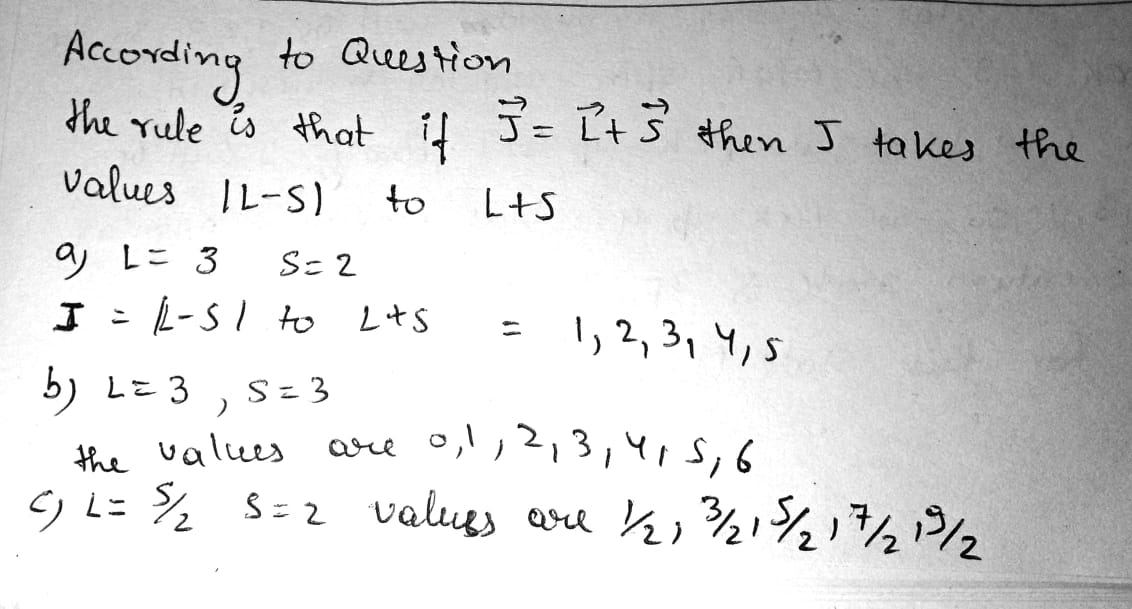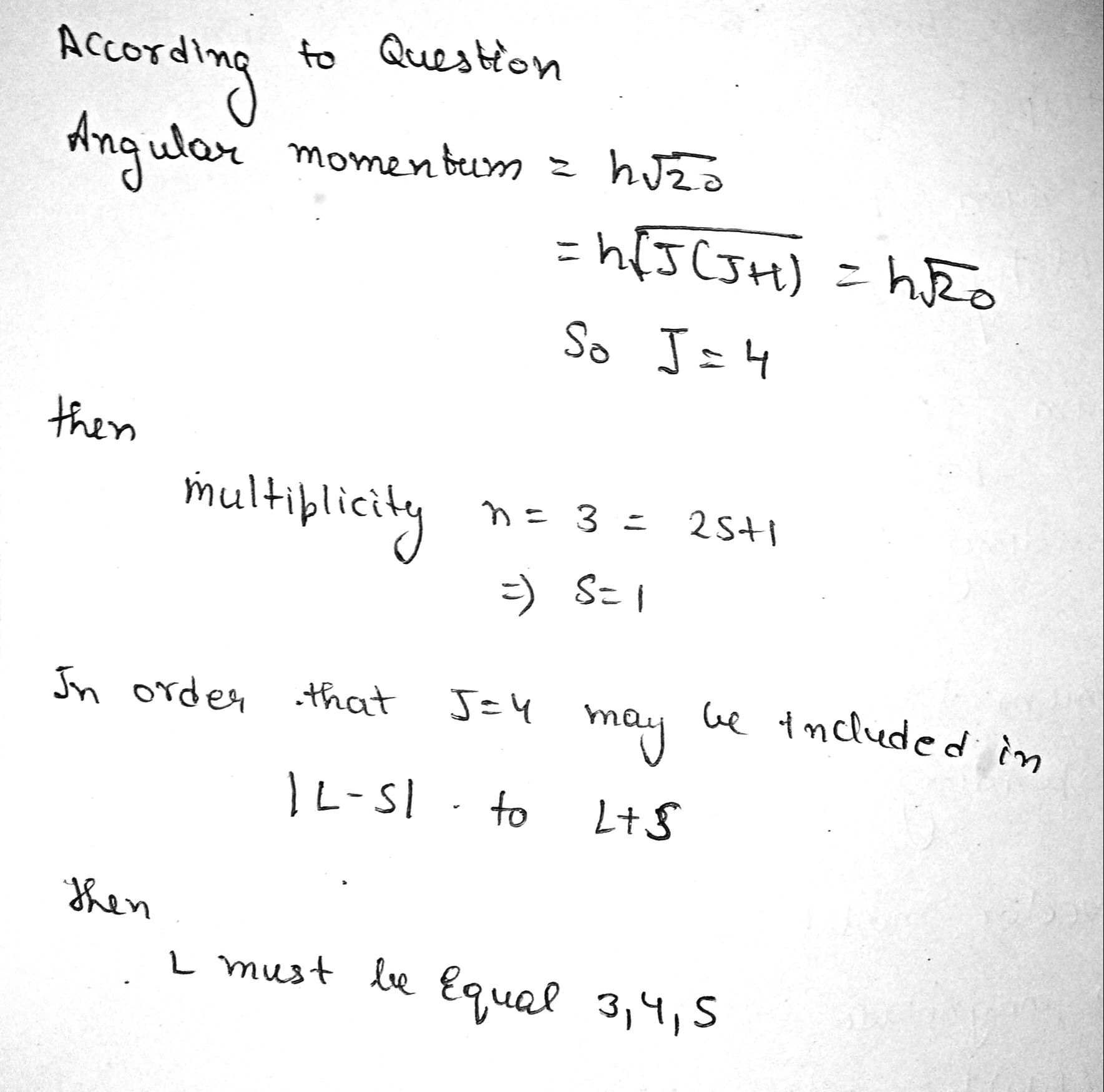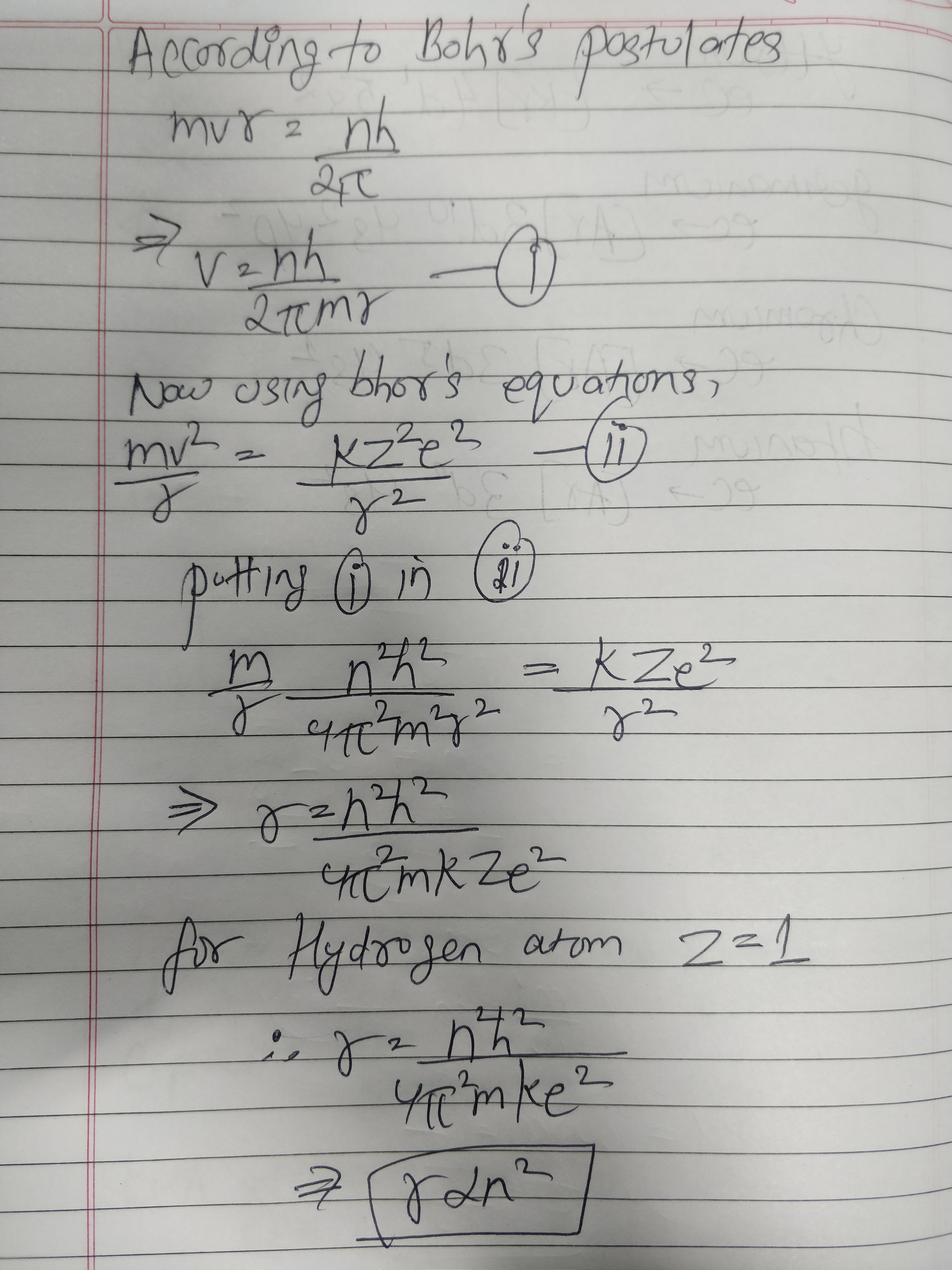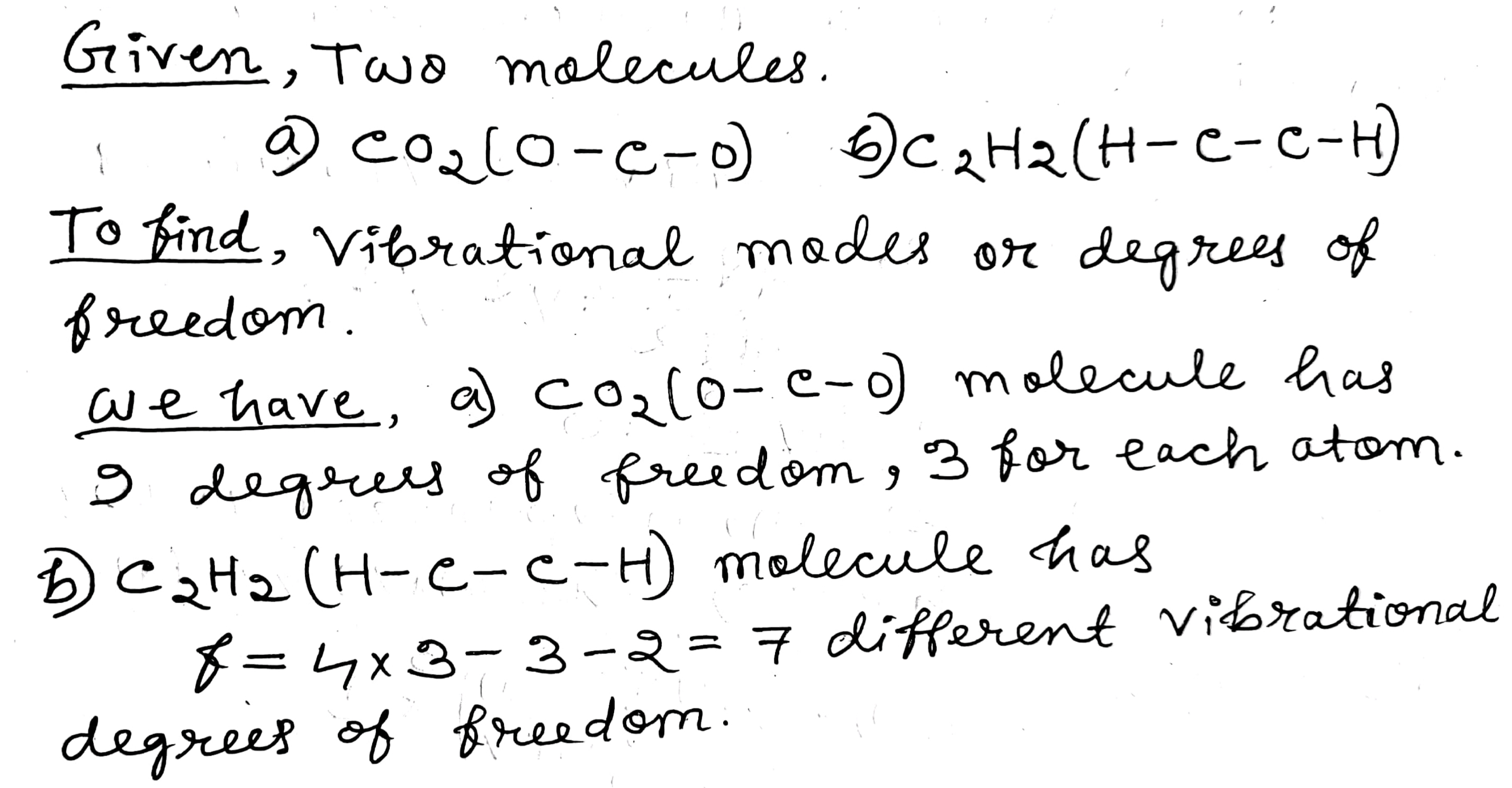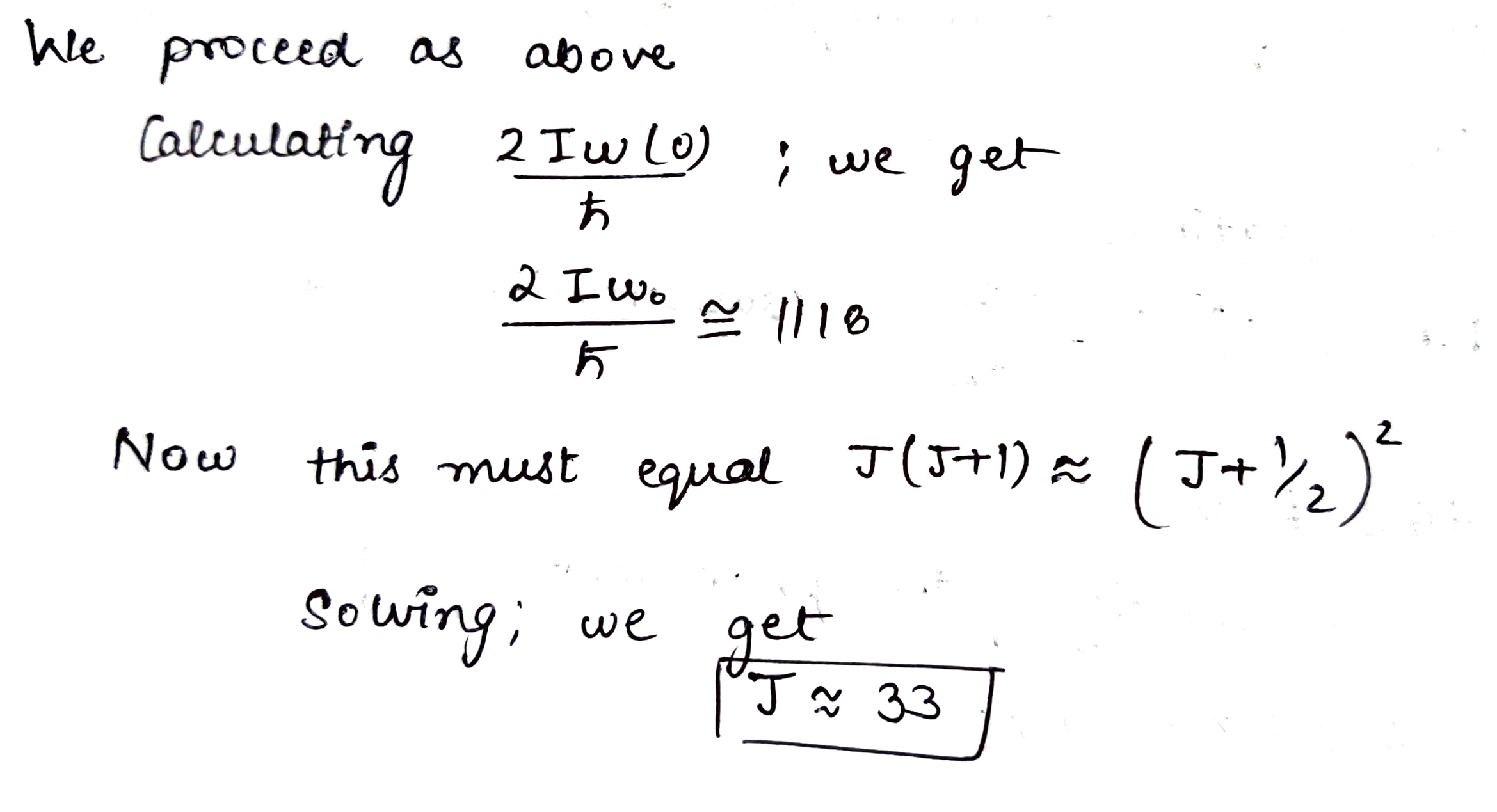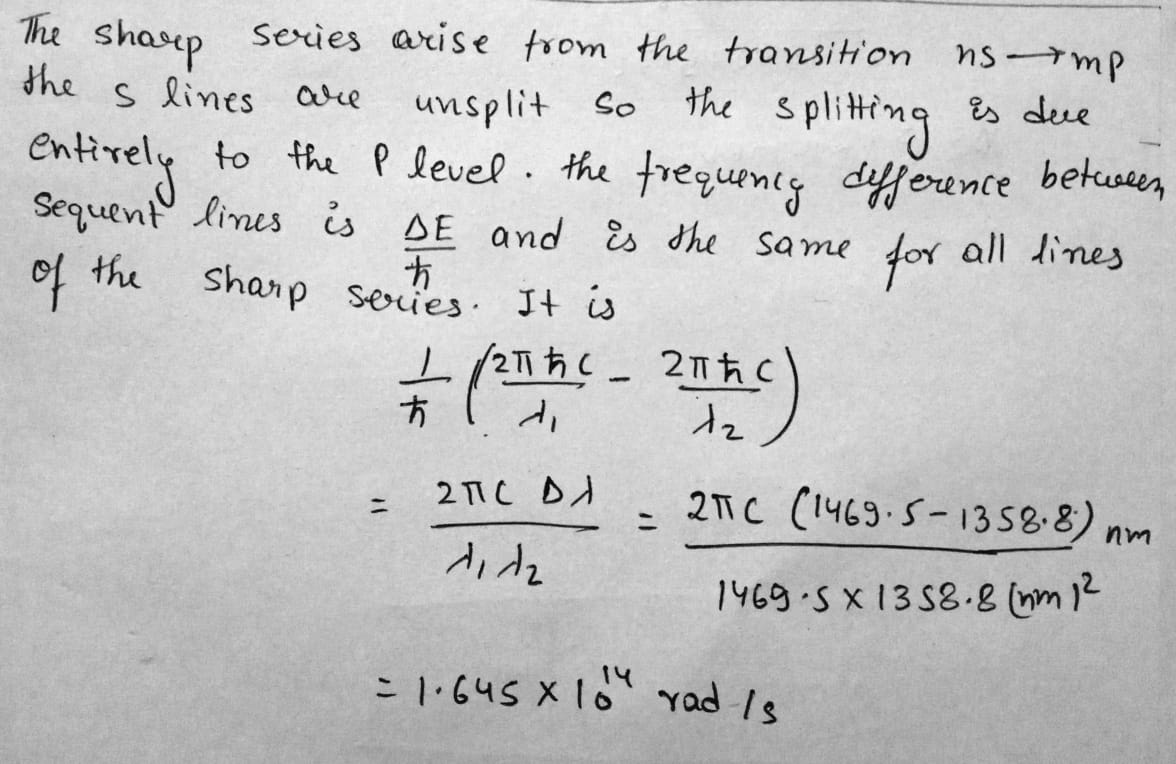Class 12 Medical Physics - Atoms

A narrow beam of protons with kinetic energy $$T = 1.4 \, MeV$$ falls normally on a brass foil whose mass thickness $$ \rho d = 1.5 \, mg/cm^2 $$. The weight ratio of copper and zinc in the foil is equal to $$ 7 : 3 $$ respectively. Find the fraction of the protons scattered through the angles exceeding $$ \theta_{0} = 30^{\circ} $$.

The relevant fraction can be immediately can be immediately written down (see 6.12 (b)) (Note that the projectiles are protons)
$$ \dfrac{\Delta N}{N} = \left (\dfrac{e^{2}}{(4 \pi \varepsilon_{0}) 2 T} \right )^{2} \pi \cot^{2} \dfrac{\theta_{0}}{2} \cdot (n_{1} Z^{2}_{1} + n_{2} Z^{2}_{2}) $$

Here $$n_{1} (n_{2}) $$ is the number of $$Z_{n} (Cu) $$ nuclei per $$cm^{2} $$ of the foil and $$Z_{1} (Z_{2}) $$ is the atomic number of $$Zn(Cu)$$. Now

$$n_{1} = \dfrac{\rho d N_{A}}{M_{1}} = 0.7 , n_{2} = \dfrac{\rho d N_{A}}{M_{2}} = 0.3 $$

Here $$M_{1} , M_{2} $$ are the mass number of $$Z_{n}$$ and $$Cu$$.
Then, substituting the values $$Z_{1} = 30, Z_{2} = 29 , M_{1} = 65.4 , M_{2} = 63.5 $$, we get
$$ \dfrac{\Delta N}{N} = 1.43 \times 10^{-3} $$

A uniform magnetic field B exists in a region. An electron projected perpendicular to the field goes in a circle. Assuming Bohr's quantization rule, calculate the radius of $$n$$th orbit and the minimum possible speed of electron.

According to NBohr's quantization rule
$$mvr = \dfrac{nh}{2\pi}$$'r' is less when 'n' has least value i.e. 1
or, $$mv = \dfrac{nh}{2\pi R}$$ ....(1)
Again, $$r = \dfrac{mv}{qB}$$, or, $$mv = rqB$$ ....(2)
From (1) and (2)
$$rqB = \dfrac{nh}{2\pi r}$$ [q = e]
$$\Rightarrow r^2 = \dfrac{nh}{2\pi eB} \Rightarrow r = \sqrt{h/2\pi eB}$$ [here n = 1]
b)
For the radius of nth orbit, $$r = \sqrt{\dfrac{nh}{2\pi eB}}$$.
c)
$$mvr = \dfrac{nh}{2\pi}, r = \dfrac{mv}{qB}$$
Substituting the value of 'r' in(1)$$mv \times \dfrac{mv}{qB} =\dfrac{nh}{2\pi}$$
$$\Rightarrow m^2v^2 = \dfrac{nheB}{2\pi}$$ [n =1, q = e]$$\Rightarrow v^2 =\dfrac{heB}{2\pi\m^2}\Rightarrow or v = \sqrt{\dfrac{heB}{2\pi\m^2}}$$.

A $$100\space eV$$ electron collides with a stationary helium ion $$(He^+)$$ in its ground state and excites to a higher level. After the collision, $$He^+$$ ion emits two photons in succession with wavelengths $$1085\mathring{A}$$ and $$304\mathring{A}$$. Find the principal quantum number of the excited state. Also, calculate the energy of the electron after the collision. Given $$h = 6.63\times10^{-34}J\space s$$.

Energy of $$He^+$$ in ground state $$=\cfrac{-13.6}{1^2}\times 4 \\ =-54.4$$

Total energy before collision $$=100-54.4 \\ =45.6eV$$

From conservation of energy:

$$45.6=E+\cfrac { hc }{ 1085 } +\cfrac { hc }{ 304 } \\ E=45.6-11.42-40.78\\ E=45.6-52.2\\ E=-6.6\\ \cfrac { -13.6 }{ { n }^{ 2 } } \times 4=-6.6\\ { n }^{ 2 }=8.2\\ n\approx 2$$

Principal quantum number = 2

The electron in a hydrogen atom makes a transition $$n_1\rightarrow n_2$$, where $$n_1$$ and $$n_2$$ are the principal quantum numbers of the two energy states. Assume Bohr's model to be valid. The time period of the electron in the initial state is eight times that in the final state. What are the possible values of $$n_1$$ and $$n_2$$?

Here, $$Z= 1$$. We can derive the expression for the time period of electron in $$n^{th}$$ orbit as
$$\quad T_n = \displaystyle\frac{1}{f_n} \propto n^3$$
Hence
$$\displaystyle\frac{T_1}{T_2} = \displaystyle\frac{n_1^3}{n_2^3}$$. As $$T_1 = 8T_2$$, therefore we get

$$\quad 8 = \left(\displaystyle\frac{n_1}{n_2}\right)^3 \Rightarrow n_1 = 2n_2$$

Thus, the possible values of $$n_1$$ and $$n_2$$ are $$n_1 = 2, n_2 = 1; n_1= 4, n_2=2;n_1=6, n_2 = 3$$ and so on.

During Rutherford's experiment, generally, the thin foil of heavy atoms like gold was used and bombarded by the $$\alpha$$-particles. If the thin foil of light atoms like aluminium etc. is used, what difference would have been observed?

The nucleus of heavy elements like gold has a large positive charge and the nucleus of light elements like aluminium have a small positive charge. The repulsion between alpha particles and heavy nuclei is much larger than the repulsion between alpha particles and light nuclei. So if a lighter element like aluminium is used instead of gold, the number of alpha particles deflected will be much less in comparison to the deflections observed when gold was used.

Using Bohr's postulates derive the expression for the frequency of radiation submitted when electron in hydrogen atom undergoes transition from higher energy state (quantum number $$n_{i}$$) to the lower state, $$(n_{f})$$
when electron in hydrogen atom jumps from energy state $$n_i=4 \;to\; n_f=3,2,1$$. Identify the special series to which the emission lines holding belong.

In the hydrogen atom:
Radius of electron orbit,
$$\displaystyle r=\frac{n^{2}h^{2}}{4\pi^{2}kme^{2}}$$.........(i)

Kinetic energy of electron:
$$\displaystyle E_{k}=\frac{1}{2}mv^{2}=\frac{ke^{2}}{r}$$

Using equation (i), we get:
$$\displaystyle E_{k}=\frac{he^{2}4\pi^{2}kme^{2}}{n^{2}h^{2}}$$

$$\displaystyle =\frac{2\pi^{2}k^{2}me^{4}}{n^{2}h^{2}}$$

Potential energy:
$$\displaystyle E_{p}=\frac{-k(e)\times(e)}{r}=\frac{-ke^{2}}{r}$$

Using equation (i) we get:
$$\displaystyle E_{p}=-ke^{2}\times \frac{4\pi^{2}kme^{2}}{n^{2}h^{2}}$$

$$\displaystyle =-\frac{4\pi^{2}k^{2}me^{4}}{n^{2}h^{2}}$$

Total energy of electron:
$$\displaystyle E=\frac{2\pi^{2}k^{2}me^{4}}{n^{2}h^{2}}-\frac{4\pi^{2}k^{2}me^{4}}{n^{2}h^{2}}$$

$$\displaystyle =-\frac{2\pi^{2}k^{2}me^{4}}{n^{2}h^{2}}$$

$$\displaystyle=\frac{2\pi^{2}k^{2}me^{4}}{h^{2}}\times \left[\frac{1}{n^{2}}\right]$$

Now, according to bohr's frequency condition when electron in hydrogen atom uindergoes transition from higher energy state to the lower energy state $$(n_{f})$$ is.
$$hv=E_{m}-E_{nf}$$

$$\displaystyle hv=-\frac{2\pi^{2}k^{2}me^{4}}{h^{2}}\times \frac{1}{n^{2}_{i}}-\left[\frac{-2\pi^{2}k^{2}me^{4}}{h^{2}}\times \frac{1}{n^{2}_{i}}\right]$$

$$\displaystyle hv=\frac{2\pi^{2}k^{2}me^{4}}{h^{2}}\times \left[\frac{1}{n^{2}_{i}}-\frac{1}{n^{2}_{i}}\right]$$

$$\displaystyle v=\frac{2\pi^{2}k^{2}me^{4}}{h^{3}}\times \left[\frac{1}{n^{2}_{i}}-\frac{1}{n^{2}_{i}}\right]$$

$$\displaystyle v=\frac{c2\pi^{2}k^{2}me^{4}}{ch^{3}}\times \left[\frac{1}{n^{2}_{i}}-\frac{1}{n^{2}_{i}}\right]$$

$$\displaystyle \frac{2\pi^{2}k^{2}me^{4}}{ch^{3}}=R=$$Rydberg constant

$$R=1.097\times 10^7m-1$$

Thus, $$\displaystyle v=Rc\times \left[\frac{1}{n^{2}_{i}}-\frac{1}{n^{2}_{i}}\right]$$

Now higher state, $$n_{i}=4$$
Lower state, $$n_{f}=3,2,1$$

For the transition:
$$n_{i}=4\;to\;n_{f}=3,\rightarrow Paschen\;Series$$
$$n_{i}=4\;to\;n_{f}=2,\rightarrow Balmer\;Series$$
$$n_{i}=4\;to\;n_{f}=1,\rightarrow Lyman\;Series$$

State the postulates of Bohr atom model. Obtain an expression for the radius of the $$n$$th orbit of hydrogen atom.

The postulates of Bohr's atom model are:
(i) An electron cannot revolve round the nucleus in all possible orbits. The electrons can revolve round the nucleus only in those allowed or permissible orbits for which the angular momentum of the electron is an integral multiple of $$\dfrac { h }{ 2\pi }$$ (where $$h$$ is Planck's constant $$-6.626\times { 10 }^{ -34 }Js$$). These orbits are called stationary orbits or non-radiating orbits and an electron revolving in these orbits does not radiate any energy.
If $$m$$ and $$v$$ are the mass and velocity of the electron in a permitted orbit of radius $$r$$, then angular momentum of electron $$=mvr=\dfrac { nh }{ 2\pi }$$, where $$n$$ is called principal quantum number and has the integral values $$1, 2, 3...$$ This is called Bohr's quantization condition.
(ii) An atom radiates energy, only when an electron jumps from a stationary orbit of higher energy to an orbit of lower energy. If the electron jumps from an orbit of energy $${ E }_{ 2 }$$ to an orbit of energy, $${ E }_{ 1 }$$, a photon of energy $$hv={ E }_{ 2 }-{ E }_{ 1 }$$ is emitted. This condition is called Bohr's frequency condition.
Consider an atom whose nucleus has a positive charge $$Ze$$, where $$Z$$ is the atomic number that gives the number of protons in the nucleus and $$e$$, the charge of the electron which is numerically equal to that of proton. Let an electron revolve around the nucleus in the $${ n }^{ th }$$ orbit of radius $${ r }_{ n }$$
By Coulomb's law, the electrostatic force of attraction between the nucleus and the electron $$=\dfrac { 1 }{ 4\pi { \varepsilon }_{ 0 } } \cdot \dfrac { \left( Ze \right) \left( e \right) }{ { r }_{ n }^{ 2 } }$$ ...(1)
where $${ \varepsilon }_{ 0 }$$ is the permittivity of the free space.
Since, the electron revolves in a circular orbit, it experiences a centripetal force,
$$\dfrac { m{ v }_{ n }^{ 2 } }{ { r }_{ n } } =m{ r }_{ n }{ \omega }_{ n }^{ 2 }$$ ...(2)
where $$m$$ is the mass of the electron $${ v }_{ n }$$ and $${ \omega }_{ n }$$ are the linear velocity and angular velocity of the electron in the $${ n }^{ th }$$ orbit respectively.
The necessary centripetal force is provided by the electrostatic force of attraction.
For equilibrium, from equations (1) and (2),
$$\dfrac { 1 }{ 4\pi { \varepsilon }_{ 0 } } \cdot \dfrac { Z{ e }^{ 2 } }{ { r }_{ n }^{ 2 } } =\dfrac { m{ v }_{ n }^{ 2 } }{ { r }_{ n } }$$ ...(3)
$$\dfrac { 1 }{ 4\pi { \varepsilon }_{ 0 } } \cdot \frac { Z{ e }^{ 2 } }{ { r }_{ n }^{ 2 } } =m{ r }_{ n }{ \omega }_{ n }^{ 2 }$$ ...(4)
From equation (4),
$${ \omega }_{ n }^{ 2 }=\dfrac { Z{ e }^{ 2 } }{ 4\pi { \varepsilon }_{ 0 }m{ r }_{ n }^{ 3 } }$$ ...(5)
The angular momentum of an electron in $${ n }^{ th }$$ orbit is,
$$L=m{ v }_{ n }{ r }_{ n }=m{ r }_{ n }^{ 2 }{ \omega }_{ n }$$ ....(6)
By Bohr's first postulate, the angular momentum of the electron
$$L=\dfrac { nh }{ 2\pi }$$ ...(7)
where $$n$$ is an integer and is called the principal quantum number.
From equations (6) and (7),
$$m{ r }_{ n }^{ 2 }{ \omega }_{ n }=\dfrac { nh }{ 2\pi }$$
(or) $${ \omega }_{ n }=\dfrac { nh }{ 2\pi m{ r }_{ n }^{ 2 } }$$
Squaring both sides,
$${ \omega }_{ n }^{ 2 }=\dfrac { { n }^{ 2 }{ h }^{ 2 } }{ 4{ \pi }^{ 2 }{ m }^{ 2 }{ r }_{ n }^{ 2 } }$$ ....(8)
From equations (5) and (8),
$$\dfrac { Z{ e }^{ 2 } }{ 4\pi { \varepsilon }_{ 0 }m{ r }_{ n }^{ 3 } } =\dfrac { { n }^{ 2 }{ h }^{ 2 } }{ 4{ \pi }^{ 2 }{ m }^{ 2 }{ r }_{ n }^{ 2 } }$$
(or) $${ r }_{ n }=\dfrac { { n }^{ 2 }{ h }^{ 2 }{ \varepsilon }_{ 0 } }{ \pi mZ{ e }^{ 2 } }$$ ....(9)
From equation (9), it is seen that the radius of the $${ n }^{ th }$$ orbit is proportional to the square of the principal quantum number. Therefore, the radii of the orbits are in the ratio $$1 : 4 : 9...$$
For hydrogen atom, $$Z=1$$
$$\therefore$$ from equation (9),
$${ r }_{ n }=\dfrac { { n }^{ 2 }{ h }^{ 2 }{ \varepsilon }_{ 0 } }{ \pi m{ e }^{ 2 } }$$ ...(10)
Substituting the known values in the above equation we get,
$${ r }_{ n }={ n }^{ 2 }\times 0.53\mathring { A }$$
If $$n=1$$, $${ r }_{ 1 }=0.53\mathring { A } $$
This is called Bohr radius.

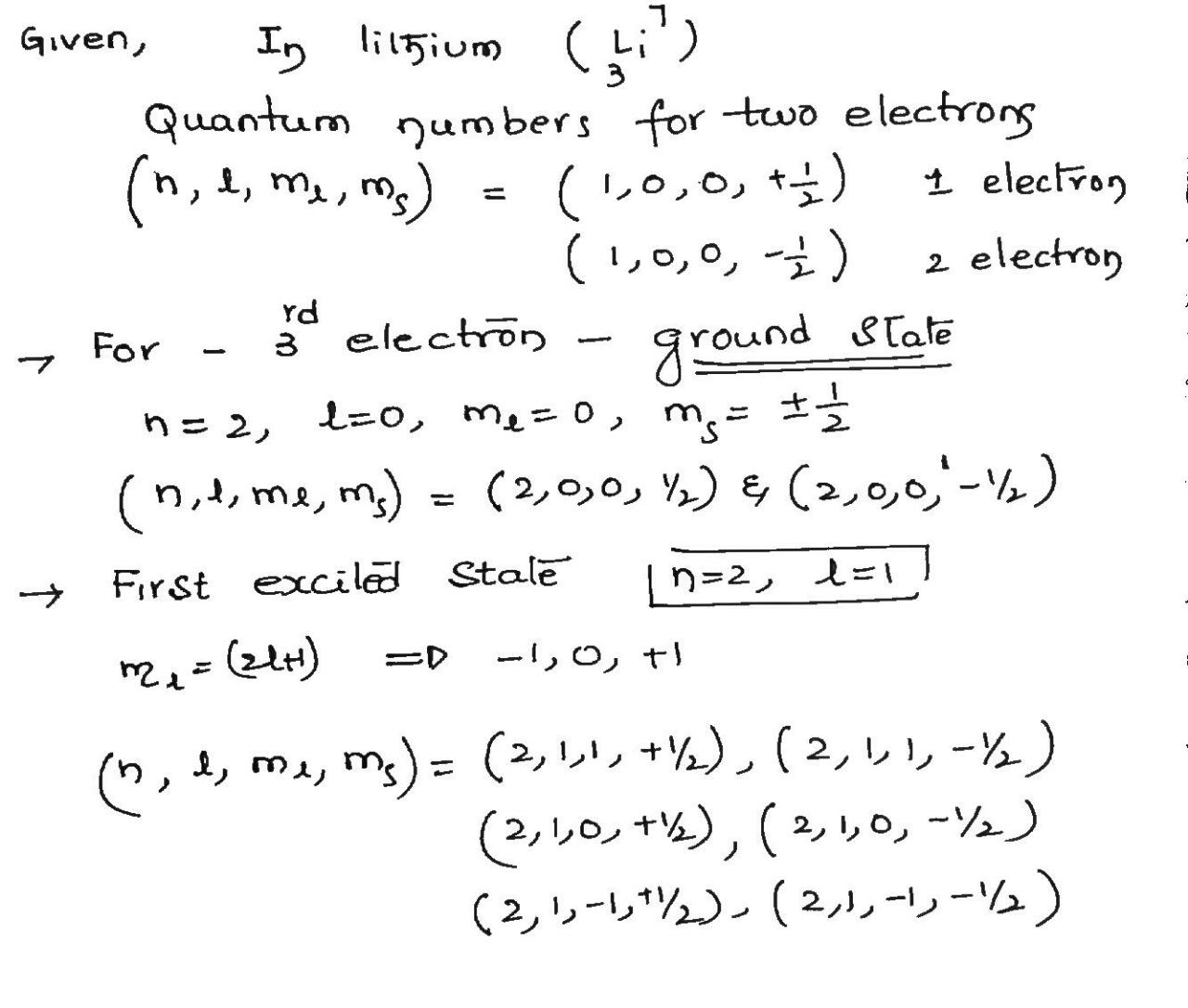
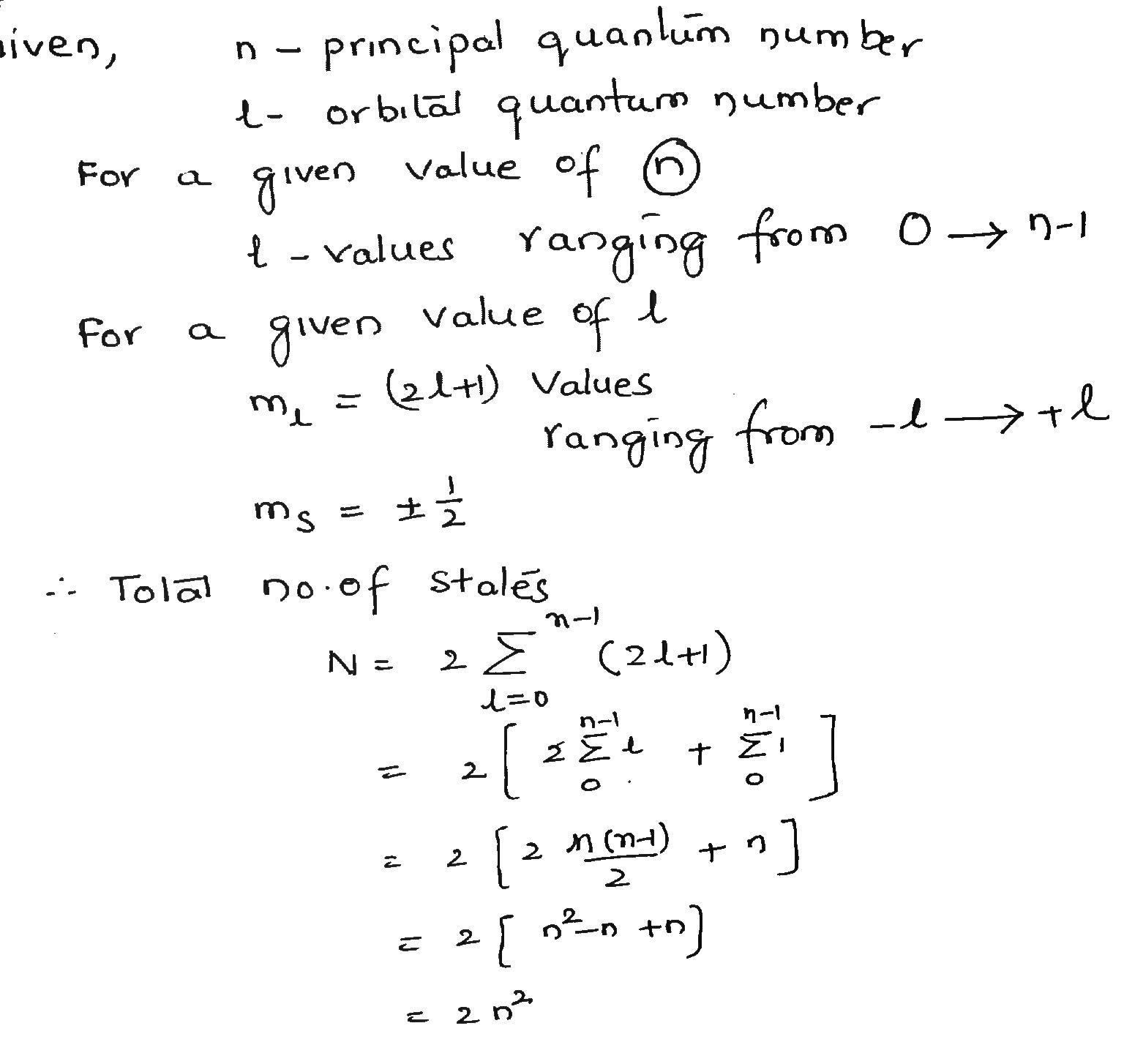
(a) How many sub-shells are associated with n = 4 ?
(b) How many electrons will be present in the sub-shells having $$m_s$$ value of -1/2 for n = 4 ?

(a) Four sub-shells are associated with n = 4. These include 4s, 4p, 4d and 4f.
(b) Sixteen electrons will be present in the sub-shells having $$m_s$$ value of -1/2 for n = 4.
Also, sixteen electrons will be present in the sub-shells having $$m_s$$ value of +1/2 for n = 4.

State two ways of providing energy to a metal to emit electrons from its surface.

The electron emission from the surface of a metal is possible only if sufficient additional energy is supplied from some external source. This external energy may come from a variety of sources such as:

( i ) Thermionic emission. In this method, the metal is heated to sufficient temperature (about 2500ºC) to enable the free electrons to leave the metal surface. The number of electrons emitted depends upon the temperature. The higher the temperature, the greater is the emission of electrons. This type of emission is employed in vacuum tubes.

( ii ) Photo-electric emission. In this method, the energy of light falling upon the metal surface is transferred to the free electrons within the metal to enable them to leave the surface. The greater the intensity ( i.e. brightness) of light beam falling on the metal surface, the greater is the photo-electric emission.

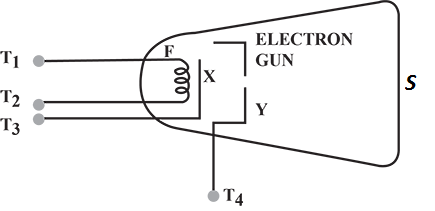
Diagram shows an electron gun inside a cathode ray tube. Label the screen with the letter S.

Electron guns are devices often used by cathode ray tube displays in order to produce light on a phosphorus-coated glass screen. The electron gun generates a beam of electrons which is targeted at the screen.

The beam can be deflected on all three axes by the image driver with the help of either a magnetic or an electric field, thus creating an image pattern on the screen. Electrons are being absorbed into the phosphorus layer, exciting it in order to emit photon particles of light.

How is a cathode ray tube used to convert an electric signal into a visual signal ?

the electron beam is directed towards the phosphorus screen and strikes it causing the light to be emitted in a small area or spot in proportion to the intensity of electron beam. In a TV or CRT display the electrical signal that controls the beam intensity corresponds to the desired picture information and referenced as the video signal.

State two uses of a cathode ray tube.

Before LCD or Plasma television, the CRT was used to create a moving image.
CRT based oscilloscopes.

Name one device in which the cathode ray tube is used.

Cathode-ray tube are used in electron microscope, invented in 1928 by Ernst Ruska. The electron microscope uses a stream of electrons to magnify an image. Because electrons have a very small wavelength, they can be used to magnify objects that are too small to be resolved by visible light.

What is the effect on the thermion beam in a cathode ray tube if the anode voltage value is reduced but remains above zero?

If the anode voltage is reduced in a cathode ray tube, the emitted electrons are less attracted and accelerated and if the rate of attraction of electrons is less than the rate of emission of electrons,then a space charge will develope around the cathode.

Can we use a screen coated with barium platinocyanide in a cathode ray tube? If so what effects do we observe on the screen?

Yes, we can use screen coated with barium plantiocyanide in a cathode ray tube. It is a fluroscent material and can be coated on the screen to produce fluroscence when thermions strike it. We can observe that the screen glows with bluish white glow.

Name spectral series of hydrogen which lies in the ultraviolet region of electromagnetic spectrum.

Lyman series of hydrogen atom lies in the ultraviolet region, Balmer series lies in visible region, Paschen series lies in near infrared region whereas Bracket, Pfund as well as Humphrey series lie in far infrared region of electromagnetic spectrum.

Name a common device where a hot cathode ray tube is used.

Cathode Ray Tube is a device that produces an image when electron beam strikes the phosphorescent surface. Cathode ray tubes are found in TVs and computers.

Draw a simplified labelled diagram of a hot cathode ray tube.

The given diagram shows hot cathode ray tube.

(i) In a cathode ray tube what is the function of anode?
(ii) State the energy conversion taking place in a cathode ray tube.
(iii) Write one use of cathode ray tube.

(i) The function of anode in a cathode ray tube is to accelerate the electrons and also focus them in a fine energetic beam.
(ii) In a cathode ray tube electrical energy is converted into light energy.
(iii) To check the waveform of varying electrical signals.

Name the three main parts of a Cathode Ray Tube.

The three main parts of a Cathode ray tube are:

(i) Electron gun: It gives out a fine beam of electrons.
(ii) Deflecting system: It deflects the electron beam.
(iii) Fluorescent screen: It is the part of the cathode ray tube on which the visual pattern of electron beam is obtained.

Name a material which exhibits fluorescence when cathode rays fall on it.

Zinc Sulphide exhibits fluorescence when cathode rays fall on it.

Answer the following question based on a hot cathode ray tube. Name the charged particles.

The charged particles in the hot cathode ray tube are fast moving electrons in the form of a beam which is produced by the thermionic emission effect from a metal surface.

Define Bohr's orbit or stationary orbit.

1310786_1138858_ans_6c6c082f695c40b9b54f2b6a433d58ae.jpg

Explain different types of spectral lines?

1310739_1138953_ans_75fd8b01573e4a25951b4b0d4043839f.jpg

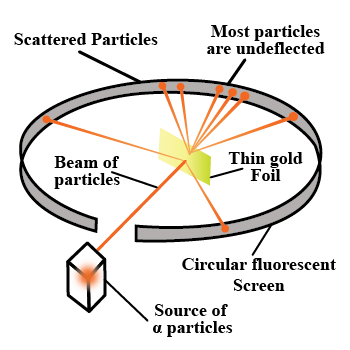
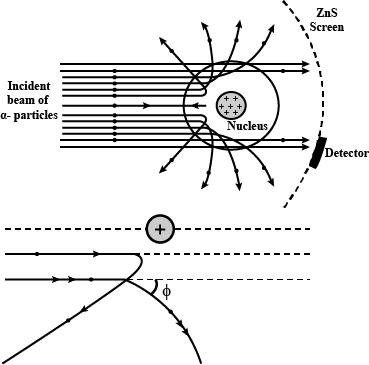
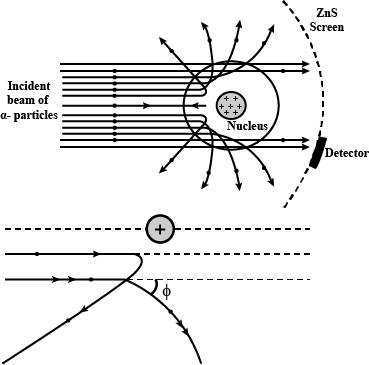
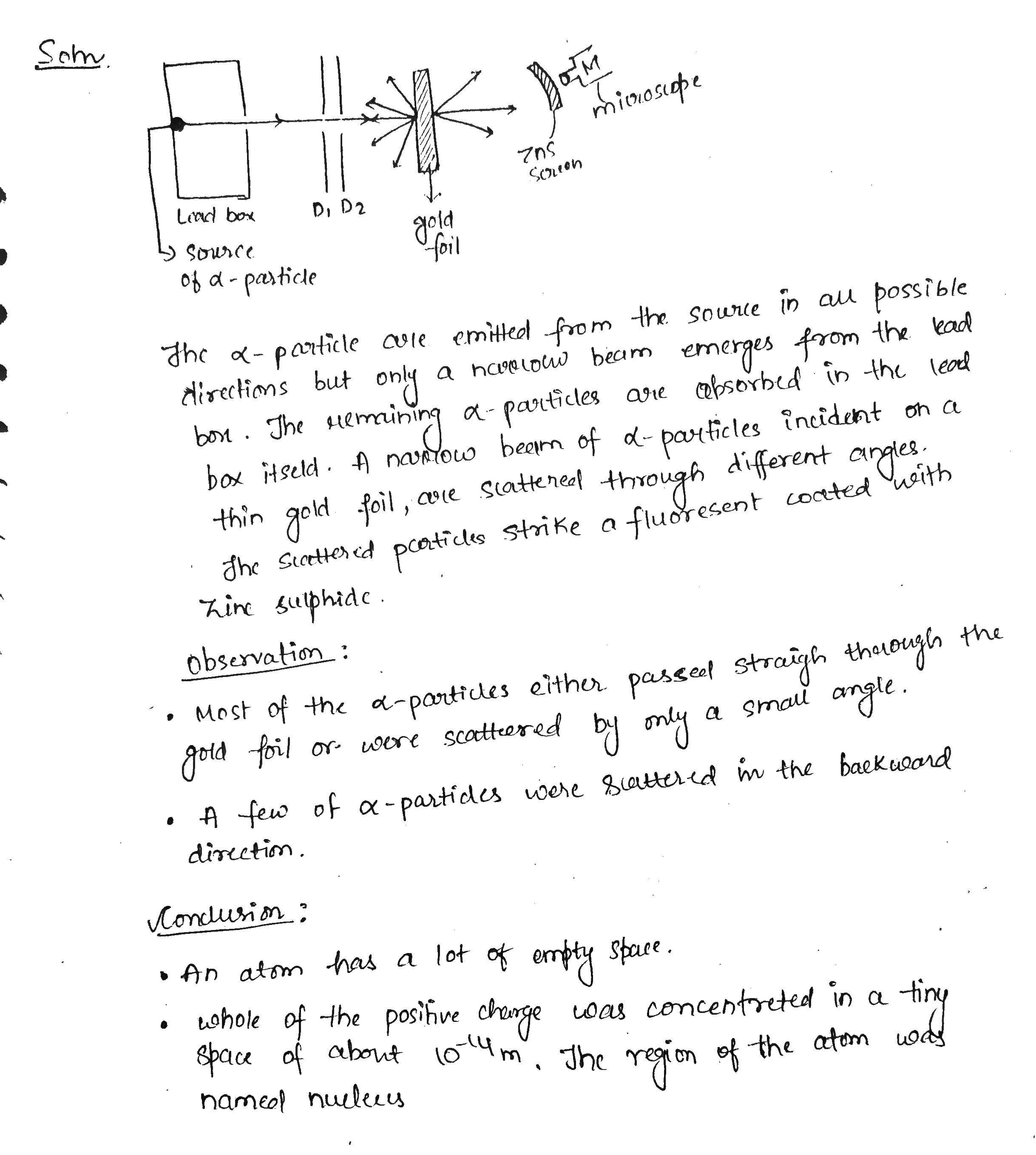
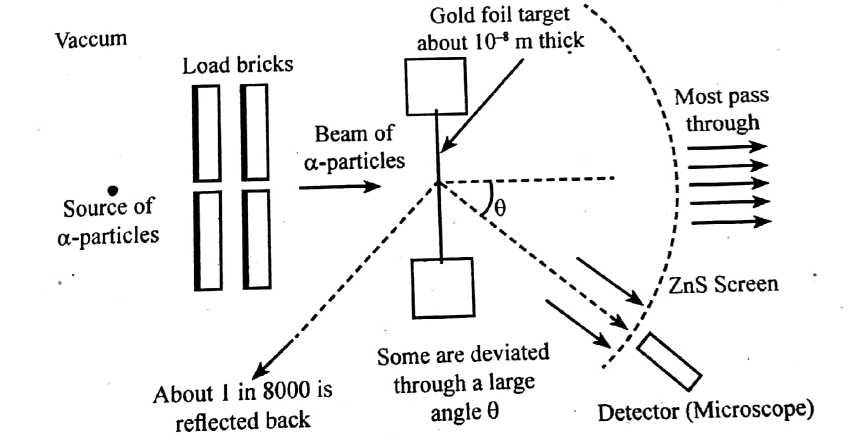
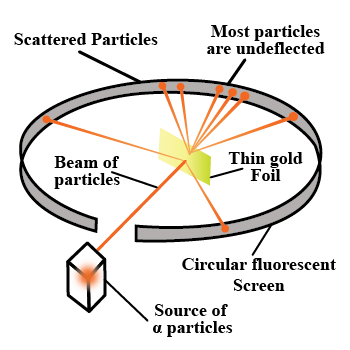
With the help of neat diagram, describe the Geiger-Marsden experiment.

1310872_1139239_ans_b0379a87a7e5442a9245d71a75c69ddf.jpg

What is photon?

A photon is a bundle of energy of electromagnetic wave. For a frequency of wave v, energy of a photon
E=hv.

In a cathode ray tube, why is the filament made of tungsten?

Because tungsten has a high melting point. This allows it to be heated red/white hot without melting. This high temperature results in the thermionic emission (boiling off) of electrons which are needed for the CRT to work.
Tungsten also has a low vapour pressure at high temperatures, so it won't evaporate too quickly, and it is relatively cheap.

Diagram shows an electron gun inside a cathode ray tube. You have two batteries one of 6 V and other of 1000 V. Show on the diagram how to connect these batteries between the terminals $$T_1,\, T_2,\, T_3,\, and\, T_4$$ in order to produce an electron beam from the gun.

The $$6V$$ battery should be connected between $$T_1$$ and $$T_2$$ with positive terminal on either side(doesn't matter).

But the $$1000V$$ battery goes in between the terminals $$T_3$$ and $$T_4$$ with positive on $$T_4$$ terminal since it is the anode so that the electrons are accelerated towards the electron gun.

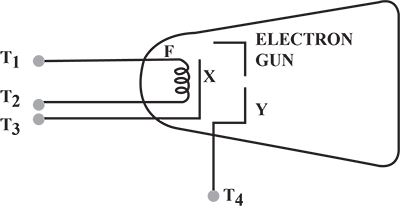
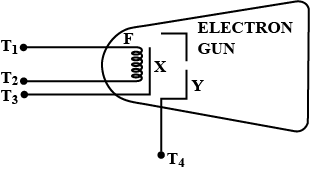
In a cathode ray tube, why is the cathode coated with thorium and carbon?

A tungsten filament on which a mixture of thorium and carbon are coated is known as thoriated tungsten. The advantage in the emitter is that heat is produced by passing electric current through tungsten filament whereas thermions are emitted from thorium. The work function of thorium is $$2.6 eV$$ and corresponding to a temperature of about $$2000 K$$. If the temperature is increased beyond $$2000 K$$, the rate of thermionic emission also increases.

An electron in $$nth$$ excited state in a hydrogen atom comes down to first excited state by emitting ten different wavelengths. Find the value of $$n$$ (an integer).

First excited state means $$n = 2$$ orbit and thus $$nth$$ excited state means $$(n+1)th$$ orbit.

As the electron comes down to $$n_1=2$$ orbit, thus number of specteral line is given by- $$N = \dfrac{[n_2 - n_1] [(n_2 - n_1) +1 ]}{2}$$

$$\therefore 10 = \dfrac{[n_2 -2][(n_2 - 2) +1]}{2} \implies n_2 = 6$$ orbit

Thus $$n_2 = n+1 \implies n =5$$

Thus the transition talkes place from $$5th$$ excited state OR $$6th$$ orbit.

Diagram shows an electron gun inside a cathode ray tube. Draw a pair of plates $$P_1\, and\, P_2$$ to apply the electric field in the diagram and show the deflection of the particles emitted by X in between the plates $$P_1\, and P_2$$.

The electricity is applied in vertically downward direction. i.e From plate $$P_1$$ to $$P_2$$. Since force exerted on the charged particle has same direction as that of electric field (if the charged particle is positive) i.e in case of protons and the opposite force exerted on the charged particle if the charged particle is negative.

Draw a neat and well labelled diagram of Rutherford's $$\alpha-$$ particle scattering experiment.

The radius of the innermost electron orbit of a hydrogen atom is $$5.3 \times 10^{-11}$$ m. Calculate the radius of n = 3 orbit of the same atom.

Radius of orbit (r)

$$r=\dfrac {n^2h^2\epsilon_0}{Z\pi me^2}=0\cdot 529\times \dfrac {n^2}{Z}A^0$$

For Hydrogen , $$\displaystyle r_n=n^2r_1$$
For $$n=3$$ ,

$$r_3=3^2\times 5.3 \times 10^{-11}=47.7 \times 10^{-11} m$$

Obtain the first Bohrs radius and the ground state energy of a muonic hydrogen atom [i.e., an atom in which a negatively charged muon $$(\mu ^-)$$ of mass about $$207m_e$$ orbits around a proton].

The charge of the muon $$m=207m_e$$,

Bohr radius is given as:

$$r_e=1/m_e$$

We know that for the first Bohr orbit

$$r_e=0.53\times 10^{-10}m$$

So, at equilibrium, $$mr=m_er_e$$

The radius pf the muonic hydrogen atom

$$r=2.56\times^{-13}m$$

We also know that,

$$E_e\propto m_e;\ \ E_e=-13.6eV$$

$$\Rightarrow \dfrac{E_e}{E}=\dfrac{m_e}{m}\Rightarrow E=-2.81\ keV$$

Suppose you are given a chance to repeat the alpha-particle scattering experiment using a thin sheet of solid hydrogen in place of the gold foil. (Hydrogen is a solid at temperatures below 14 K.) What results do you expect?

In the alpha-particle scattering experiment, if a thin sheet of solid hydrogen is used in place of a gold foil, then the scattering angle would not be large enough. This is because the mass of hydrogen $$(1.67 ×10^{-27})$$ is less than the mass of incident $$\alpha$$−particles $$(6.64 ×10^{-27})$$. Thus, the mass of the scattering particle is more than the target nucleus (hydrogen). As a result, the $$\alpha$$−particles would not bounce back if solid hydrogen is used in the $$\alpha$$−particle scattering experiment.

In the study of Geiger-Marsdon experiment on scattering of $$\alpha-particles$$ by a this foil of gold, draw the trajectory of $$\alpha-particles$$ in the coulomb field of target nucleus. Explain briefly how one gets the information on the size of the nucleus from this study:
From the relation $$R = R_{0}A^{1/3}$$, where $$R_{0}$$ is constant and A is the mass number of the nucleus, show that nuclear matter density is independently of A

The trajectory of $$\alpha$$ - particles in the gold foil experiment is shown in the attached figure. As most of the particles are undeflected, this suggests that the nucleus occupies only a small volume of the total atom.

Let $$m$$ be the mass of the nucleon and $$R$$ be the radius of the nucleus.

Mass of the nucleus is $$M = mA$$

Volume of the nucleus is $$V = \dfrac{4}{3} \pi R^3$$

$$V = \dfrac{4}{3} \pi (R_oA^{1/3})^3$$

$$V = \dfrac{4}{3} \pi R_o^3 A$$

Density of the nucleus is $$\rho = \dfrac{M}{V}$$

$$\rho = \dfrac{mA}{\frac{4}{3}\pi R_o^3 A}$$

$$\rho = \dfrac{3m}{4 \pi R_o^3}$$

Hence, nuclear density is independent of A.

With the help of a neat labelled diagram, describe the Geiger- Marsden experiment. What is mass defect ?
The photoelectric work function for a metal surface is 2.3 eV. If the light of wavelength $$ 6800\ A^\circ$$ is incident on the surface of metal find threshold frequency and incident frequency. Will there be an emission of photoelectrons or not ?
[Velocity of light c = $$3 \times 10^8 m/s$$, Planck's constant, $$h=6\cdot 63 \times 10^{-34 }Js$$]

Ginger-Marsden experiment: The setup of the Geiger-Marsden experiment is as shown below.
In this experiment, a narrow beam of $$\alpha$$-particles from a radioactive source was incident on a gold foil. These scattered $$\alpha$$-particles were detected by the detector fixed on a rotating stand. The detector used had a zinc sulphide screen and a microscope. The $$\alpha$$-particles produced scintillations n the screen which could be observed through a microscope. This entire setup a enclosed in an evacuated chamber.
They observed the number of $$\alpha$$-particles as a function of scattering angle. Now, the scattering angle is the deviation $$(\theta)$$ of a-particles from its original direction.
They observed that most a-particles passed undeviated and only a few $$(\sim 0.14%)$$ scattered by more than$$1^o$$ Few were deflected slightly and only a few (1 in 8000) deflected by more than $$90^o$$. Some particles even bounced back with 1800.
Mass defect : It is observed that the mass of a nucleus is smaller than the sum of the masses of the constituent nucleons in the free state. The difference between the actual mass of the nucleus and the sum of the masses of constituent nucleons is called mass defect.
The mass defect is
$$[Zm_p + (A- Z)m] - M$$
where Z is the, atomic number (number of protons), A is the mass number, (A - Z) is the mass of neutrons, $$m_p$$ is the mass of a proton, m is the mass of a neutron and $$M_n$$ is the measured mass of a nucleus.
Problems:

Given : $$\phi _0=2.3 eV=2.3 \times 1.6 \times 10^{-19}J=3.68 \times 10^{-19}J$$

$$\lambda =6800 A=6800 \times 10^{-10}m; c=3 \times 10^8 m/s;$$

$$h=6.63 \times 10^{-34}Js$$

We know that the incident is given as

$$v=\dfrac{c}{\lambda }$$

$$\therefore v=\dfrac{3 \times 10^8}{6800 \times 10^{-10}}=4.41 \times 10^{14}Hz$$

Now, if the incident frequency is greater than the threshold frequency, then photoelectrons will be emitted from the metal surface. The threshold frequency is given from work function as

$$v_0=\dfrac{\phi _0}{h}$$

$$\therefore v_0=\dfrac{3.68 \times 10^{-19}}{6.63 \times 10^{-34}}=5.55\times 10^{14}Hz$$

Since, $$v < v_0$$ photoelectron will not be emitted.

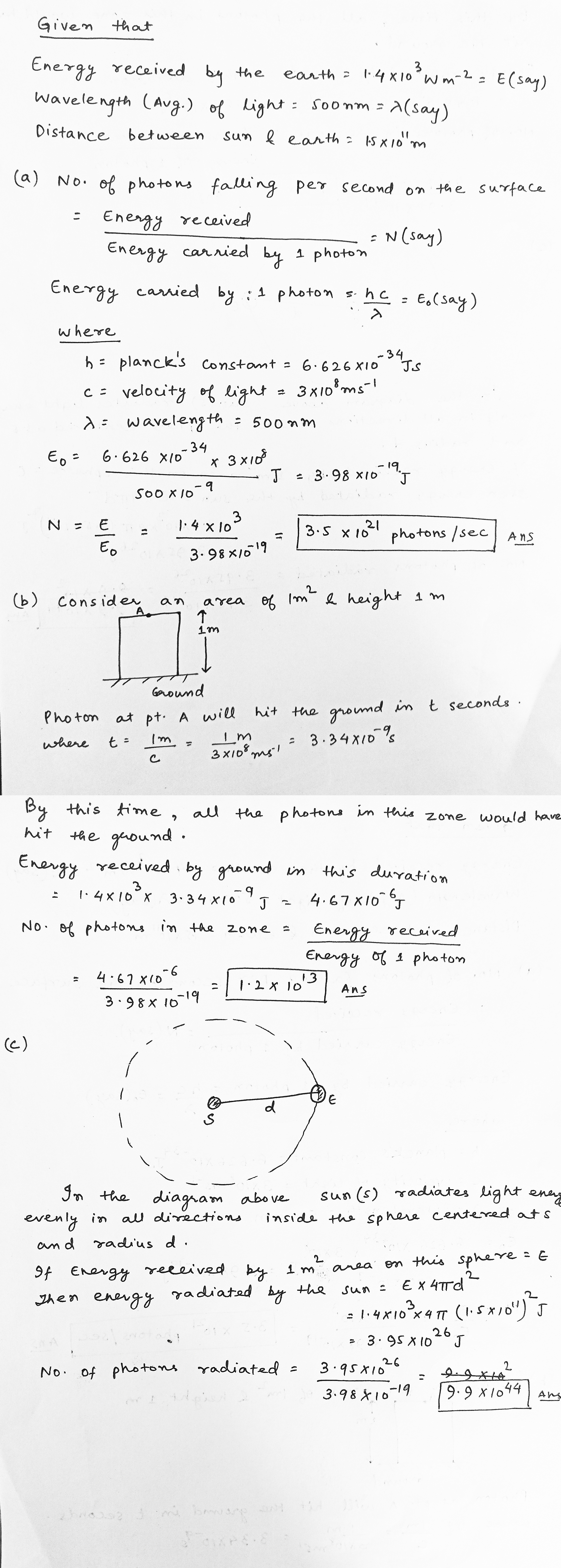
When the sun is directly overhead, the surface of the earth receives $$1.4\times {10}^{3}W$$ $${m}^{-2}$$ of sunlight. Assume that the light is monochromatic with average wavelength $$500nm$$ and that no light is absorbed in between the sun and the earth's surface. The distance between the sun and the earth is $$1.5\times {10}^{11}m$$ (a) Calculate the number of photons falling per second on each square metre of earth's surface directly below the sun (b) How many photons are there in each cubic metre near the earth's surface at any instant? (c) How many photons does the sun emit per second?

1595046_874219_ans_4fa8a9ada9284b33a5c705466106afc3.jpg

The earth revolves around the sun due to gravitational attraction. Suppose that the sun and the earth are point particles with their existing masses and that Bohr's quantization rule for angular momentum is valid in the case of gravitation. (a) Calculate the minimum radius the earth can have for its orbit. (b) What is the value of the principal quantum number n for the present?

We have,

Mass of the Earth $$= Me=6.0$$ X $$10^{24}kg$$

Mass of the Sun $$= Ms=2.0$$ X $$10^{30} kg$$

Distance between Earth and Sun $$=1.2$$ X $$10^{11}m$$

Also we have the equations,

$$mvr=\frac{nh}{2\pi}$$ or $$m^2 v^2 r^2= \frac{n^2 h^2}{4 \pi^2}$$ .....(1)

$$\frac{G Me Ms}{r^2}=\frac{Me v^2}{r}$$ or $$v^2= \frac{G Ms}{r}$$ .....(2)

Dividing (1) and (2), we get

$${Me}^2 r =\frac{n^2 h^2}{4 \pi^2 G Ms}$$

For $$n=1$$

Radius of the Earth, $$r=\sqrt{\frac{h^2}{4 \pi^2 G Ms Me^2}}=2.29$$ X $$10^{-138}m$$

Using Bohr's atomic model, derive an equation for radius of orbit of an electron.

$$mvr=\dfrac { nh }{ 2\pi } \quad \longrightarrow \left( 1 \right) $$

$$\dfrac { { mv }^{ 2 } }{ r } =\dfrac { K{ ze }^{ 2 } }{ { r }^{ 2 } } \quad \longrightarrow \left( 2 \right) $$

$$\Rightarrow r=\dfrac { Kz{ e }^{ 2 } }{ { mv }^{ 2 } } $$ (from (2))

$$v=\dfrac { nh }{ 2\pi \times mr } $$ (from (1))

Put $$'v'$$ $$'m'$$ $$'r'$$ :-

$$r=\dfrac { K{ ze }^{ 2 }\times { \left( 2\pi \right) }^{ 2 }{ m }^{ 2 }{ r }^{ 2 } }{ m\times { \left( nh \right) }^{ 2 } } $$

$$r=\dfrac { m{ \left( nh \right) }^{ 2 } }{ { Kze }^{ 2 }\left( { 4\pi }^{ 2 } \right) { m }^{ 2 } } $$

$$\boxed { r=\left( \dfrac { { h }^{ 2 } }{ mK{ ze }^{ 2 }4{ \pi }^{ 2 } } \right) \dfrac { { n }^{ 2 } }{ z } } x$$

In a process a neutron which is initially at rest, decays into a proton, an electron and an antineutrino. The ejected electron has a momentum of $$ p_1 = 2.4 \times 10^{-26} kg m/s $$ and the antineutrino has $$ p_2 = 7.0 \times 10^{-27} kg m/s $$ . Find the recoil speed of the proton if the electron and the antineutrino are ejected (a) along the same direction (b) in mutually perpendicular directions (Mass of the proton $$m_p = 1.67 \times 10^{-27} $$ )

(a)

Let the velocity of the proton is $${V_p}$$.

The momentum is given as,

$$1.67 \times {10^{ - 27}} \times {V_p} = 2.4 \times {10^{ - 26}} + 7 \times {10^{ - 27}}$$

$${V_p} = 18.56\;{\rm{m/s}}$$

Thus, the recoil speed of proton is $$18.56\;{\rm{m/s}}$$.

(b)

The total momentum of electron and antineutrino is given as,

$$p = \sqrt {{{\left( {24} \right)}^2} + {{\left( 7 \right)}^2}} \times {10^{ - 27}}$$

$$p = 25 \times {10^{ - 27}}\;{\rm{kgm/s}}$$

The recoil speed of proton is given as,

$$1.67 \times {10^{ - 27}} \times {V_p} = 25 \times {10^{ - 27}}$$

$${V_p} = 14.97\;{\rm{m/s}}$$

Thus, the recoil speed of proton is $$14.97\;{\rm{m/s}}$$.

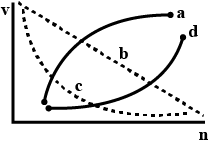
Which of the following curves may represent the speed of the electron in a hydrogen atom as a function of the principal quantum number n?

1870468_1724201_ans_0083691d8d8b402eb18a3e858baa0d8e.jpg

In an experiment on $$\alpha$$-particle scattering by a thin foil of gold, draw a plot showing the number of particles scattered versus the scattering angle $$\theta$$. Why is it that a very small fraction of the particles are scattered at $$\theta > 90^\circ$$?

A small fraction of the alpha particles scattered at angle $$\theta > 90^\circ$$ is due to the reason that if impact parameter ‘b’ reduces to zero, coulomb force increases, hence alpha particles are scattered at angle $$\theta > 90^\circ$$, and only one alpha particle is scattered at angle $$180^\circ$$.
1682481_1785370_ans_75799527b7fd4773abb982a249329f77.png

1682481_1785370_ans_75799527b7fd4773abb982a249329f77.png

Describe the Geiger-Marsden experiment. What are Its observations and conclusions?

At the suggestion of Rutherford, in

19111911 times the size of atom.

(iii) The negative charges (electrons) do not influence the scattering process. This implies that nearly the whole mass of the atom is concentrated in the nucleus.
1682393_1785604_ans_384473c63b3747e6bc70c9e359bd3b89.png

1682393_1785604_ans_384473c63b3747e6bc70c9e359bd3b89.png

Show that if the 63 electrons in an atom of europium were assigned to shells according to the logical sequence of quantum numbers, this element would be chemically similar to sodium.

1770976_1778647_ans_6acf0f09873e42aaa344e481e642fd40.jpg

(A correspondence principle problem.) Estimate (a) the quantum number $$l$$ for the orbital motion of Earth around the Sun and (b) the number of allowed orientations of the plane of Earths orbit. (c) Find $$ \theta_{min}$$ the half-angle of the smallest cone that can be swept out by a perpendicular to Earths orbit as Earth revolves around the Sun.

1770943_1778664_ans_cd168201ac5d4dba9ca0563d4723a931.jpg

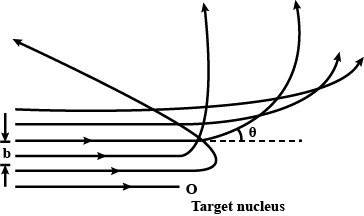
The trajectories, traced by different $$\alpha$$-particles, in the Geiger-Marsden experiment were observed as shown in the figure.
(i) What names are given to the symbols b and $$\theta$$ shown here?
(ii) What can we say about the values of b for (i) $$\theta = 0^\circ$$ (ii) $$\theta = 0^\circ$$ = p radians?

(i} The symbol ‘b’ represents the impact parameter and ‘

θθ radians, the impact parameter ’b’ will be minimum and represent the nuclear size.

The spectrum of a star in the visible and the ultraviolet region was observed and the wavelength of some of the lines that could be identified was found to be:
$$824\, \overset{\circ}{A}, 970\, \overset{\circ}{A}, 1120\, \overset{\circ}{A}, 2504\, \overset{\circ}{A}, 5173\, \overset{\circ}{A}, 6100\, \overset{\circ}{A}$$
Which of these lines cannot belong to the hydrogen atom spectrum? (Given Rydbergconstant $$R = 1.03 \times 10^7\,m^{-1}$$ and $$\dfrac{1}{R}= 970 \,\overset{\circ}{A}$$. Support your answer with suitable calculations.

For hydrogen atom. the wavenumber (i.e., reciprocal of wavelength) of the emitted radiation is given by

$$\overset{-}{v}=\dfrac{1}{\lambda}=R\Bigg(\dfrac{1}{{n_2}^2}-\dfrac{1}{{n_2}^2}\Bigg)$$

$$\therefore\,\lambda=\dfrac{\dfrac{1}{R}}{\Bigg(\dfrac{1}{{n_2}^2}-\dfrac{1}{{n_1}^2}\Bigg)}=\dfrac{970\,A^\circ}{\Bigg(\dfrac{1}{{n_2}^2}-\dfrac{1}{{n_1}^2}\Bigg)}$$

For the Lyman series of hydrogen spectrum, we take $$n_2=1$$. Hence the permitted values of A can be given as:

$$A=\dfrac{970\,A^\circ}{3/4},\dfrac{970\,A^\circ}{8/9},\dfrac{970\,A^\circ}{15/16}\,...\dfrac{970\,A^\circ}{1}$$ (taking $$n_1 = 2, 3, 4, ...\, 2$$)

$$= 1293.3 \,A^\circ, 1091 \,A^\circ, 1034.6\, A^\circ, ......... \,970\,A^\circ$$

For the Balmer series of hydrogen spectrum, we take $$m_2 = 2$$. Hence the possible values of A can be given as:

$$A=\dfrac{970\,A^\circ}{5/36},\dfrac{970\,A^\circ}{3/16},\dfrac{970\,A^\circ}{21/100}\,...\dfrac{970\,A^\circ}{1/4}$$ (taking $$n_1 = 2, 3, 4, ...\, 2$$)

$$=698\, A^\circ, 5173.3\, A^\circ, 4619 \, A^\circ, ......... 3880 \, A^\circ$$

Hence $$A = 824 \, A^\circ, 1120 \, A^\circ, 2504 \, A^\circ. 6100 \, A^\circ,$$ of the given lines, cannot belong to the hydrogen atom spectrum.

Answer the following question, which help you understand the difference between Thomson's model and Rutherford's model better.
In which model is it completely wrong to ignore multiple scattering for the calculation of a average angle of scattering of a particles by a thin foil?

On the basis of Thomson's atom model, the scattering angle of a $$\alpha$$-particles due to single collision will be very small. It is because, mass of atom is distributed all over it. The observed scattering angle can be explained only by considering multiple scattering. Therefore, it is wrong to ignore multiple scattering in Thomson's atom model.
Since the mass of the atom is concentrated in the form of nucleus in Ruther ford's atom model, most of the scattering comes through a single collision and multiple scattering can be ignored.

Answer the following question, which help you understand the difference between Thomson's model and Rutherford's model better.
Keeping other factors fixed, it is found experimentally that for small thickness $$t$$, the number of $$\alpha$$-particles scattered at moderate angles is proportional to $$t$$. What clue does this linear dependence on $$t$$ provide?

The chance of single collision increases linearly with the number of targeted atoms and hence linearly with thickness. That the number of $$\alpha$$-particles scattered at moderate angles is proportional to $$t$$, suggests that the scattering is predominantly due to single collision.

Describe Rutherford's experiment on scattering of $$\alpha$$-particles. How do it help in discovery of nucleus ?

1882454_1840944_ans_c50edc16b1a94a39b70d4f3631609fc4.jpg

The only partially filled subshell of a certain atom contains three electrons, the basic term of the atom having $$L = 3$$. Using the Hund rules, write the spectral symbol of the ground state of the given atom.

1879389_1855544_ans_ffb97483d3d0478b9ae39274168ddeb3.jpeg

How many and which values of the quantum number J can an atom possess in the state with quantum numbers S and L equal respectively to
(a) $$2$$ and $$3$$; (b) $$3$$ and $$3$$; (c) $$5/2$$ and $$2$$?

1880401_1855504_ans_3612490f6230438db859a980fd08e867.jpeg

Write the spectral designation of the term whose degeneracy is equal to seven and the quantum numbers L and S are interrelated as $$L = 3S$$.

1879971_1855535_ans_b100ae01d6d64b86b638e0cef5081cde.jpeg

Write the spectral symbols for the terms of a two-electron system consisting of one p electron and one d electron.

1880018_1855528_ans_55b0cfdd5976439a8a9b1ba476db0ba0.jpeg

Write the spectral designations of the terms of the hydrogen atom whose electron is in the state with principal quantum number $$n = 3$$.

1880403_1855501_ans_19eb18213e6b405494b462cf32f502a7.jpeg

An atom is in the state whose multiplicity is three and the total angular momentum is $$ h\sqrt{20} $$. What can the corresponding quantum number L be equal to ?

1880166_1855516_ans_ea20a889e66f4788ab4f934635c16e10.jpeg

An atom possessing the total angular momentum $$h\sqrt{6} $$ is in the state with spin quantum number $$S = 1$$. In the corresponding vector model the angle between the spin momentum and the total angular momentum is $$ \theta = 73.2^{\circ} $$. Write the spectral symbol for the term of that state.

1880066_1855524_ans_bbd47282512f481f805016e36e205917.jpeg

For an HF molecule find the number of rotational levels located between the zeroth and first excited vibrational levels assuming rotational states to be independent of vibrational ones. The natural vibration frequency of this molecule is equal to $$7.79\times 10^{14}$$ rad/s, and the distance between the nuclei is $$91.7\,pm$$.

1971090_1855593_ans_58b475a8bdc148b186d70d978941bce4.jpeg

A system comprises an atom in $$^{2}P_{3/2} $$ state and a d electron. Find the possible spectral terms of that system.

1879998_1855529_ans_5df8b815cd3d4a508e22b286c21f318d.jpeg

Find the ratio of the number of atoms of gaseous sodium in the state $$3P$$ to that in the ground state $$3S$$ at a temperature $$ T=2400\,K$$. The spectral line corresponding to the transition $$ 3P \rightarrow 3S$$ is known to have the wavelength $$ \lambda = 589\,nm$$.

1879382_1855547_ans_62c3f4d010544c98ae08dad6bde0c1c3.jpeg

(i) State bohr's quantization condition for defining stationary orbits. How does de broglie hypothesis explain the stationary orbits?
(ii) Find the relation between the three wavelengths $${ \lambda }_{ 1 }$$,$${ \lambda }_{ 2 }$$ and $${ \lambda }_{ 3 }$$ from the energy level diagram shown below.

(i) Bohr's Quantization Rule:

Of all possible circular orbits allowed by the classical theory, the electrons are permitted to circulate only in those orbits in which the angular momentum of an electron is an integral multiple of $$\dfrac{h}{2\pi}$$, where $$h$$ is Plank's constant.

Therefore, for any permitted orbit,

$$L=mvr=\dfrac{nh}{2\pi}$$ ; $$n=1,2,3, ........$$

Where $$L$$, $$m$$, and $$v$$ are the angular momentum, mass and the speed of the electron respectively. $$r$$ is the radius of the permitted orbit and $$n$$ is positive integer called principal quantum number.

The above equation is Bohr's famous quantum condition. When an electron of mass $$m$$ is confined to move on a line of length $$l$$ with velocity $$v$$, the de-Broglie wavelength $$\lambda$$ associated with electron is:

$$\lambda=\dfrac{h}{mv}=\dfrac{h}{P}$$

Where $$P$$ is Linear momentum

$$P=\dfrac{h}{\lambda}=\dfrac{h}{2l/n}=\dfrac{nh}{2l}$$

When electron revolves in a circular orbit of radius $$r$$ then $$2l=2\pi r$$

Therefore, $$P=\dfrac{nh}{2\pi r}$$ or $$P$$ X $$r$$ $$=\dfrac{nh}{2\pi}$$

(ii) Using Rydberg's formula for spectra of hydrogen atom, we have

$$\dfrac{1}{\lambda_1}=R(\dfrac{1}{n_2^2}-\dfrac{1}{n_3^2})$$ .....(1)

$$\dfrac{1}{\lambda_2}=R(\dfrac{1}{n_1^2}-\dfrac{1}{n_2^2})$$ .....(2)

$$\dfrac{1}{\lambda_3}=R(\dfrac{1}{n_1^2}-\dfrac{1}{n_3^2})$$ .....(3)

Now adding (1) and (2), we get

$$\dfrac{1}{\lambda_1} + \dfrac{1}{\lambda_2}=R(\dfrac{1}{n_1^2}-\dfrac{1}{n_3^2})=\dfrac{1}{\lambda_3}$$

That is, $$\dfrac{1}{\lambda_1}+\dfrac{1}{\lambda_2}=\dfrac{1}{\lambda_3}$$

Hence, the relation between 3 wavelengths from the energy level diagram is obtained.

Show that the radius of the orbit in hydrogen atoms varies as $$\displaystyle { n }^{ 2 }$$, where n is the principal quantum number of the atom.

The centripetal acceleration of the electron in a Bohr's atom is due to the Coulomb attraction of electron towards nucleus.

Thus, $$\dfrac{mv^2}{r}=\dfrac{1}{4\pi\epsilon_0}\dfrac{Ze^2}{r^2}$$

Also Bohr's postulate states, $$mvr=\dfrac{nh}{2\pi}$$

Substituting $$v$$ from second equation into the first gives,
$$r\propto n^2$$

(a) Using Bohr's second postulate of quantization of orbital angular momentum show that the circumference of the electron in the $$n^{th}$$ orbital state in hydrogen atom is n times the de Broglie wavelength associated with it.
(b) The electron in hydrogen atom in initially in the third excited state.What is the maximum number of spectral lines which can be emitted.When it finally moves to the ground state?

(i) According to Bohr's second postulate, we have

$$mvr_n=\dfrac{nh}{2\pi}$$

$$\implies$$ $$2 \pi r_n= \dfrac{nh}{mv}$$

But as per de-Broglie hypothesis

$$\dfrac{h}{mv}=\dfrac{h}{p}=\lambda$$

Therefore, $$2 \pi r_n=n \lambda$$ ; where $$\lambda$$ is the de-Broglie wavelength

(ii) Given, electron in the hydrogen atom is in the third excited state.

For third excited state, $$n=4$$

For ground state$$n=1$$

Now, total number of possible spectra lines are given by,

$$N=\dfrac{n(n-1)}{2}$$

$$N=\dfrac{4(4-1)}{2}$$

$$N=6$$

So, the maximum number of spectral line is $$6$$ .

Passing down to the ground state, excited $$Ag^{109}$$ nuclei emit either gamma quanta with energy $$87\,keV$$ or K conversion electrons whose binding energy is $$26\,keV$$. Find the velocity of these electrons.

In internal conversion, the total energy is used to knock out K electrons. The K.E. of these electrons is energy available-B.E. of K electrons
$$ = (87 - 26) = 61 \, keV $$
The total energy including rest mass of electrons is $$0.511 + 0.061 = 0.572 \,MeV $$
The momentum corresponding to this energy is
$$ \sqrt{(0.572)^{2} - (0.511)^{2}}/c = 0.257 \, MeV/c $$.

The velocity is then $$ \dfrac{c^{2}p}{E} = c \times \dfrac{0.257}{0.572} = 0.499 \,c $$

On the basis of Bohr's theory, derive an expression for the radius of the of the $$n^{th}$$ orbit of an electron of hydrogen atom.

Let e, m and v be respectively the charge, mass and velocity of the electron and r the radius of the orbit. The positive charge on the nucleus is Ze, where Z is the atomic number (in case of hydrogen atom Z = 1). As the centripetal force is provided by the electrostatic force of attraction. We have
$$\displaystyle \frac{mv^2}{r}=\frac{1}{4 \pi \varepsilon_0}\frac{(Ze)\times e}{r^2}$$
$$\displaystyle mv^2=\frac{Ze^2}{4 \pi \varepsilon _0\,\, r}$$ ....(i)
From the first postulate, the angular momentum of the electron is
$$\displaystyle mvr=n \frac{h}{2 \pi}$$ ....(ii)
where n (= 1, 2, 3, ...) is quantum number. Squaring eq. (ii) and dividing by eq. (i), we
get
$$\displaystyle r=n^2 \frac{h^2\varepsilon _0}{\pi mZe^2}$$
$$Z=1$$
Since
$$\displaystyle r=n^2 \frac{h^2\varepsilon _0}{\pi me^2}$$

Obtain an expression for the radius of Bohr orbit for $$H$$-atom.

From Bohr's second postulate,

$$L_n = mv_nr_n = \dfrac{nh}{2\pi}$$---1

where $$n$$ is an integer, $$r_n$$ is the radius of nth possible orbit and $$v_n$$ is the speed of moving electron in the $$n^{th}$$ orbit.

the relation between $$v_n$$ and $$r_n$$ is

$$v_n=\dfrac{e}{4\pi\epsilon_0mr_n}$$

Substituting value of $$v_n$$ from eqn 1 in above eqn, and solving for $$r$$ we get ,

$$r=\dfrac { { n }^{ 2 }{ h }^{ 2 } }{ 4{ \pi }^{ 2 }m{ e }^{ 2 } } \times \dfrac { 1 }{ z }$$

or, $$ r=0.529\times \dfrac { { n }^{ 2 } }{ z } \mathring { A }$$

Angstroms, where $$ n=$$ principal quantum number of orbit and $$Z=$$ atomic number.

Calculate the radius of second Bohr orbit in hydrogen atom from the given data.
Mass of electron= $$9.1\times { 10 }^{ -31 }Kg$$
Charge on the electron$$=1.6\times { 10 }^{ -19 }C$$
Planck's constant=$$6.63\times { 10 }^{ -34 }J-s$$
Permitivitty of free space = $$\quad 8.85\times { 10 }^{ -12 }{ C }^{ 2 }/N{ m }^{ 2 }\quad $$

$$r=\cfrac { { n }^{ 2 }{ h }^{ 2 }{ \varepsilon }_{ o } }{ \pi mZ{ e }^{ 2 } } $$
for hydrogen $$Z=1$$
$$\therefore$$ $$r=\cfrac { { n }^{ 2 }{ h }^{ 2 }{ \varepsilon }_{ o } }{ \pi m{ e }^{ 2 } } $$
$$\Rightarrow \cfrac { { 2 }^{ 2 }\times \left( 6.63\times { 10 }^{ -34 } \right)^2 \times \left( 8.85\times { 10 }^{ -12 } \right) }{ 3.14\times 9.1\times { 10 }^{ -31 }\times \left( 1.6\times { 10 }^{ -19 } \right)^2 } $$
$$=\cfrac { 1556.07\times { 10 }^{ -80 } }{ 73.15\times { 10 }^{ -69 } } =21.3\times { 10 }^{ -11 }m$$

Find the value of energy of electron in eV in the third Bohr orbit of hydrogen atom.
(Rydberg's constant $$\left(R\right)=1.097 \times {10}^{7}{m}^{-1}$$
Planck's constant $$\left(h\right)=6.63 \times {10}^{-34}J-s$$,
Velocity of light in air $$\left(c\right)=3 \times {10}^{8}{m}/{s}$$).

Energy of electron in nth Bohr orbit of H-atom $$E_n = \dfrac{-13.6}{n^2}$$ eV

Electron energy in third orbit, $$E_3 = \dfrac{-13.6}{3^2} = -1.51$$ eV

What conclusion was drawn by Rutherford based on Geiger-Marsden's experiment on scattering of alpha particles?

Conclusion made by Rutherford are:

$$(1)$$ Atom is hollow from inside,

$$(2)$$ The whole of the positive charge of atom must be concentrated in a very small space,

$$(3)$$ The positive charge in the atom is concentrated in an extremely small space at the centre of an atom. This space is called nucleus,

$$(4)$$ Coulomb's law holds for atomic distances also.

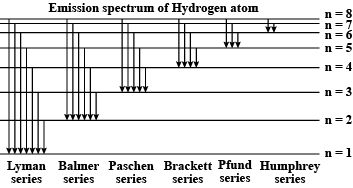
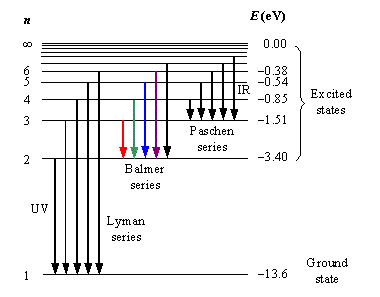
Explain the spectral series of hydrogen atom (diagram not necessary).

Whenever an electron in a hydrogen atom jumps from a higher energy level to the lower energy level, the difference in energies of the two levels is emitted as radiation of a particular wavelength. It is called a spectral line. As the wavelength of the spectral line depends upon the two orbits (energy levels) between which the transition of electron takes place, various spectral lines are obtained. The different wavelengths constitute spectral series which are the characteristic of the atoms emitting them. The following are the spectral series of hydrogen atom :
(i) Lyman series : When the electron jumps from any of the outer orbits to the first orbit, the spectral lines emitted are in the ultraviolet region of the spectrum and they are said to form a series called Lyman series (figure).
Here, $${ n }_{ 1 }=1$$, $${ n }_{ 2 }=2,3,4...$$
The wave number of the Lyman series is given by,
$$\overline { v } =R\left( 1-\dfrac { 1 }{ { n }_{ 2 }^{ 2 } } \right)$$
(ii) Balmer series : When the electron jumps from any of the outer orbits to the second orbit, we get a spectral series called the Balmer series. All the lines of this series in hydrogen have their wavelength in the visible region. Here $${ n }_{ 1 }=2$$, $${ n }_{ 2 }=3,4,5...$$
The wave number of the Balmer series is,
$$\overline { v } =R\left( \dfrac { 1 }{ { 2 }^{ 2 } } -\dfrac { 1 }{ { n }_{ 2 }^{ 2 } } \right) =R\left( \dfrac { 1 }{ 4 } -\dfrac { 1 }{ { n }_{ 2 }^{ 2 } } \right)$$
The first line in this series $$\left( { n }_{ 2 }=3 \right)$$, is called the $${ H }_{ \alpha }$$-line, the second $$\left( { n }_{ 2 }=4 \right)$$, the $${ H }_{ \beta }$$-line and so on.
(iii) Paschen series : This series consists of all wavelength which are emitted when the electron jumps from outermost orbits to the third orbit. Here $${ n }_{ 2 }=4,5,6...$$ and $${ n }_{ 1 }=3$$. This series is in teh infrared region with the wave number given by
$$\overline { v } =R\left( \dfrac { 1 }{ { 3 }^{ 2 } } -\dfrac { 1 }{ { n }_{ 2 }^{ 2 } } \right) =R\left( \dfrac { 1 }{ 9 } -\dfrac { 1 }{ { n }_{ 2 }^{ 2 } } \right)$$
(iv) Brackett series : The series obtained by the transition of the electron from $${ n }_{ 2 }=5, 6...$$ to $${ n }_{ 1 }=4$$ is called Brackett series. The wavelengths of these lines are in the infrared region. The wave number is,
$$\overline { v } =R\left( \dfrac { 1 }{ { 4 }^{ 2 } } -\dfrac { 1 }{ { n }_{ 2 }^{ 2 } } \right) =R\left( \dfrac { 1 }{ 16 } -\dfrac { 1 }{ { n }_{ 2 }^{ 2 } } \right)$$
(v) Pfund series : The lines of the series are obtained when the electron jumps from any state $${ n }_{ 2 }=6,7...$$ to $${ n }_{ 1 }=5$$. This series also lies in the infrared region. The wave number is,
$$\overline { v } =R\left( \dfrac { 1 }{ { 5 }^{ 2 } } -\dfrac { 1 }{ { n }_{ 2 }^{ 2 } } \right) =R\left( \dfrac { 1 }{ 25 } -\dfrac { 1 }{ { n }_{ 2 }^{ 2 } } \right) $$

When a vapour is excited at low pressure by passing an electric current through it, a spectrum is obtained.
a) Draw a spectral series of emission lines in hydrogen.
b) Name the different series of hydrogen atom.
c) In which region Lyman series is located.

Spectral series of emission lines in hydrogen atom is shown above indicating the names of the respective series.

Lyman series is located in ultraviolet (UV) region.

Answer the following question based on a hot cathode ray tube. State the approximate voltage used to heat the filament.

Approximately, $$6$$ volts is used to heat the filament so that the filament emits electrons from its surface via thermionic emission effect.

The electron in the hydrogen atom is moving with a speed of $$2.3 \times 10^6 m/s$$ in an orbit of radius $$0.53 \mathring{A}$$. Calculate the period of revolution of electron. ($$\pi = 3.142$$)

$$v=2.3\times10^6m/s$$

$$r=0.53 A^o=53\times10^{-8}m$$

$$T=?$$

time taken by the electron to complete one revolution$$=T=\cfrac{circumference}{speed}$$

$$\therefore T=\cfrac{2\pi r}{v}=\cfrac{2\times3.142 \times53\times10^{-8}}{2.3\times10^6}=144.8\times10^{-14}sec$$

Draw the diagram representing the schematic arrangement of the Geiger-Marsdon experimental setup tor the alpha particle scattering.

Geiger-Marsdon experimental set up-

(a) Write the $$\beta-decay$$ of tritium in symbolic from.
(b) Why is it experimentally found difficult to detect neutrinos in this process?

a) $$_{1}^{3}\textrm{T} \rightarrow _{2}^{3}\textrm{He}^{1+}+ \overline{e}+\overline{v_{e}}$$

b) Neutrions are difficult to detect experimentally in beta decay because they are uncharged particles with almost no mass. Also neutrons interact very weakly with matter. So they are very difficult to detect.

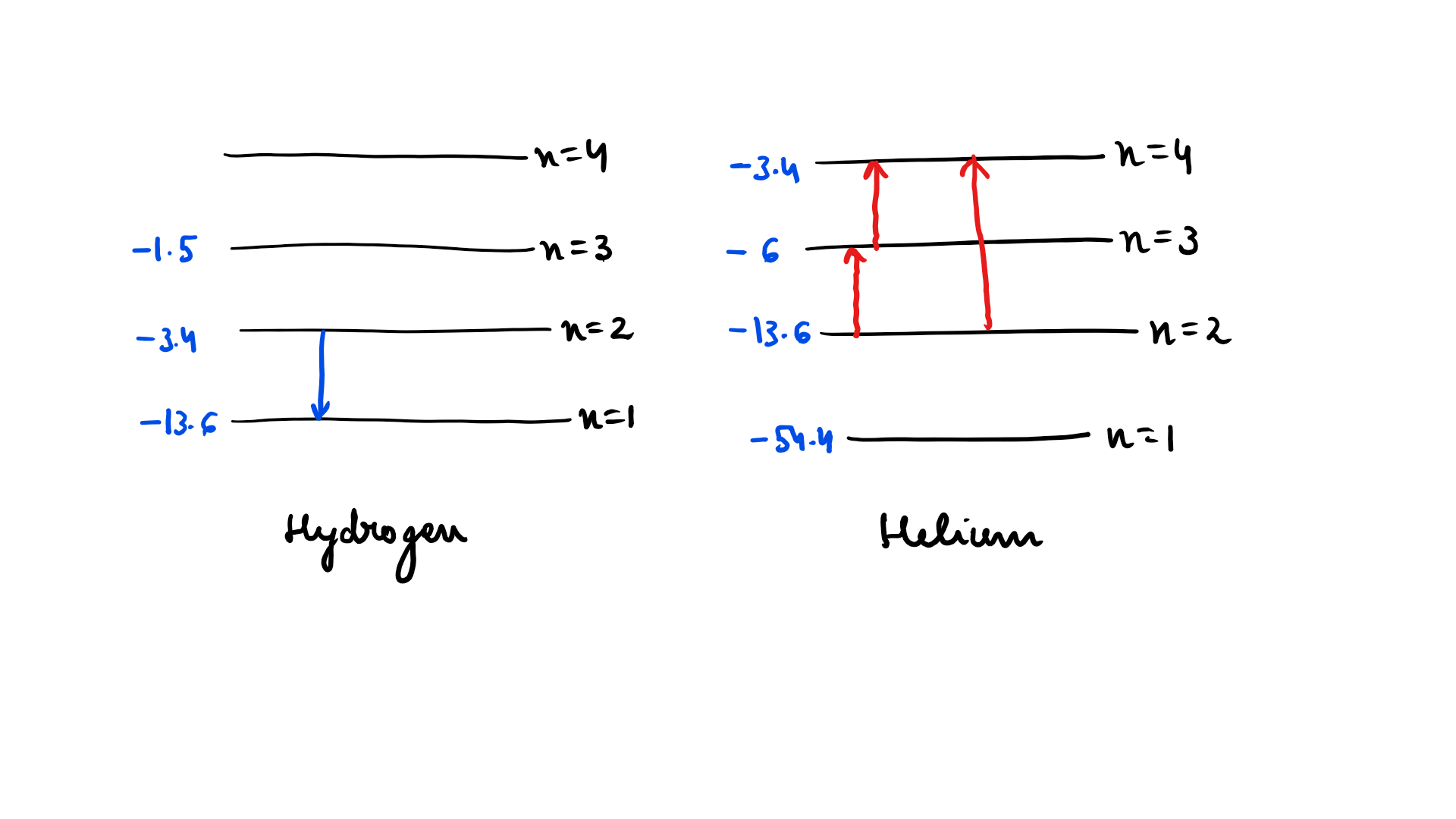
Radiation coming from transitions $$n=2$$ to $$n=1$$ of hydrogen atoms falls on helium ions in $$n=1$$ and $$n=2$$ states. What are the possible transition of helium ions as they absorb energy from the radiation ?

By Bohr's model, energy in nth orbit is given by $$ \dfrac{-13.6 Z^{2}}{n^{2}}$$ where Z is the atomic number of the atom. Using the given formula, energies of different states are obtained as shown in the image. Now when transition is made from n=2 to n=1, a photon of 13.6-3.4=10.2 eV energy is released.

When this falls on helium atom:

When it falls on n=1 state, the minimum energy required to make a transition is 54.5-13.6=40.2 eV. Hence non transition is possible with this photon,

When it falls on n=2 state, a transition can directly be made from n=2 to n=4 as the energy required for this is 10.2 eV only. Or it can first make a transition to n=3 state and then n=4.

1040078_1025749_ans_86f2afbf407f4d87811167ebc3a31292.png

In a mixture of H - $$He^+ $$ gas ($$He^+$$ is singly ionized He atom). H atoms and $$He^+$$ ions are excited to their respective first excited states. Subsequently, H atoms transfer their total excitation energy to $$He^+$$ ions (by collisions ). Assume that the Bohr model of is atom exactly valid.

Energy released by H atom

$$\Delta E_H=\dfrac{3}{4}\times13.6\ eV$$

Let $$He^+$$ goes to nth state at the end

So energy required by $$He^+$$ ions is

$$\Delta E_{He}=13.6\times4\left(\dfrac{1}{4}-\dfrac{1}{n^2}\right)\ eV$$

As $$\Delta E_H=\Delta_{He}$$

$$\dfrac{3}{4}\times13.6=13.6\times4\left(\dfrac{1}{4}-\dfrac{1}{n^2}\right)$$

$$\dfrac{1}{4}-\dfrac{1}{n^2}=\dfrac{3}{16}$$

$$\dfrac{1}{n^2}=\dfrac{1}{4}-\dfrac{3}{16}$$

$$\dfrac{1}{n^2}=\dfrac{4-3}{16}$$

$$\dfrac{1}{n^2}=\dfrac{1}{16}$$

$$\dfrac{1}{n}=\dfrac{1}{4}$$

$$n=4$$

A radiation of wavelength $$\lambda$$ is incident on a metal surface having $$ 620 nm$$ threshold wavelength. The stopping potential of the electron emitted is $$4.9 V$$. What is the value of $$\lambda$$ (in nm)?

1321508_1038665_ans_d993f5fc44da4598aece4d8d6dc066e0.jpg

Describe Rutherford's atomic model.

Rutherford's model shows that an atom is a mostly empty space with electrons orbiting a fixed positively charged nucleus.

A proton and an alpha particle having the same kinetic energy are allowed to pass through a uniform magnetic field perpendicular to the direction of their motion. Compare the radii of the paths of proton and alpha particle.

a proton has a mass= m & charge = q

mass of an alpha particlem' = 4m & charge q' = 2q

K.e of proton = K.e of alpha particle

$$0.5mv^2=0.5m'{v'}^2$$

$$v^2=4{v'}^2$$

$$\dfrac{v}{v'}=\sqrt{4}=2$$

radius of charged particle in uniform magnetic field is $$r=\dfrac{mv}{qB}$$

$$\dfrac{r}{r'}=\dfrac{m}{m'}\times \dfrac{v}{v'} \times \dfrac{q'}{q}=\dfrac{1}{4}\times \dfrac{2}{1} \times \dfrac{2q}{q}=\dfrac{1}{1}$$

What is the wavelength of light emitted when the electron in hydrogen atom undergoes transition from an energy level with n=4 to an energy level with n=2 ? What is the colour of the radiation ?

We have,

Energy level $$n=4$$ and $$n=2$$

According to the Balmer series

Wave no $$=RH [\dfrac{1}{n_1^2}-\dfrac{1}{n_2^2}]$$

Here $$n_1=2, n_2=4$$

Putting the value then we get,

Wave number $$=109678(\dfrac{1}{2^2}-\dfrac{1}{4^2})$$

$$=\dfrac{109678\times3}{16}$$

Also,

$$\lambda=\dfrac{1}{wave no}$$

Therefore,

$$ \lambda=\dfrac{16}{109678\times3}=486nm$$

The energy of a photon is equal to $$3$$ kilo $$eV$$. Calculate its linear momentum.

Momentum of photon $$ = mv= \dfrac{h\nu}{c} = \dfrac{3 keV}{3 \times 10^8}$$

$$= \dfrac{3 \times 10^3 \times 1.6 \times 10^{-19}}{3 \times 10^8} = 1.6 \times 10^{-24} \; \;\;kg\;m/s$$

which an electron in hydrogen atom jumps from the third excited state to the ground state

We know,

$$\lambda = \dfrac h p = \dfrac {h}{\sqrt{2mK.E}}$$

So, $$\dfrac {\lambda _1 }{\lambda _2} = \sqrt{\dfrac {KE_2}{KE_1}}$$

Also we know that

$$KE_n = -13.6 \dfrac {Z^2}{n^2} $$

put it above expression

$$\dfrac {\lambda_1}{\lambda_2} = \dfrac {n_1}{n_2}$$

so, ratio of wavelength of Ground state{n = 1 }nto 3rd excited state { n = 4}

$$\dfrac {\lambda_{n=1}}{\lambda_{n=4}} = \dfrac 1 4 $$

Hence, answer is 1 : 4

A beam of light having wavelengths distributed uniformly between 450 nm to 550 nm passes through a sample of hydrogen gas. Which wavelength will have the least intensity in the transmitted beam?

The energies associated with 450 nm radiation$$=\frac{1242}{450}=2.76\ ev$$

Energy associated with 550 nm radiation$$\frac{1242}{550}=2.26\ ev$$

The light comes under the visible range

Thus,

$$n_1=2,\ n_2=3,4,5.......$$

$$E_2-E_3=13.6(1/2^2-1/3^2)=1.9\ ev$$

$$E_2-E_4=13.6(1/2^2-1/4^2)=2.55\ ev$$

$$E_2-E_5=13.6(1/2^2-1/5^2)=2.856\ ev$$

Only $$E_2-E_4$$ comes in the range of energy provided. So the wavelength corresponding to that energy will be absorbed.

$$\lambda=\dfrac{1242}{2.55}=487.05\ nm=487\ nm$$

Therefore, 487 nm wavelength will be absorbed.

What is the effective mass of photon having wavelength $$\lambda$$?

$$\textbf {Hint :}$$ Use energy of photon, $$E=\dfrac{hc}{\lambda}$$

$$\textbf{Step 1: }$$In the case of the photon, all its energy is kinetic, which is $$E=\dfrac{hc}{\lambda}$$

$$\Rightarrow \dfrac{1}{2}mc^2= \dfrac{hc}{\lambda}$$

$$\Rightarrow m= \dfrac{2h}{c\lambda}$$

The wavelength of $$H_\alpha$$ line Balmer's series of hydrogen spectrum is $$6563 \ \mathring{A}$$. Find the:
(i) Wavelength of $$H_ \gamma$$ (gamma) line of Balmer series.
(ii) Shortest wavelength of Bracket series.

1353284_1089894_ans_211a97387fa8474fa88a891978a12fce.jpg

A hydrogen atom in a state having a binding energy of $$0.85\ eV$$ makes transition to a state with excitation energy $$10.2\ eV$$
Identify the quantum numbers $$n$$ of the upper and the lower energy states involved in the transition.

Initially, the electron is at $$n_{1}=4$$ shell

As, $$\dfrac{13.6}{n^{2}}= B.E= 0.85, n_{1}=4$$

After deexcitation, it is at $$n_{2}=2$$ shell.

As,

Excitation Energy = $$13.6-\dfrac{13.6}{n_{2}^{2}}= 10.2 eV$$

So, the wavelength of emitted photon is,

$$\dfrac{he}{\lambda }=\left [ \dfrac{13.6}{n_{2}^{2}}-\dfrac{13.6}{n_{1}^{2}} \right ]1.6\times 10^{-19}$$

$$\lambda = \dfrac{6.6\times 10^{-34}\times 3\times 10^{-8}\times 16}{1.6\times 10^{-19}\times 13.6\times 3}$$

$$\lambda = 4852 \mathring{A} $$

1112444_1115448_ans_a771495b58d1484d82645ef222d1766b.jpg

The transition from the state $$n=4$$ to $$n=3$$ in hydrogen-like atom results in ultraviolet radiation. Infrared radiation will be obtained in the transition from?

IR corresponds to the least value of $$\left( \dfrac { 1 }{ { n }_{ 1 }^{ 2 } } -\dfrac { 1 }{ { n }_{ 2 }^{ 2 } } \right) $$ from paschen and pfund series the transition corresponds to $$5\longrightarrow 4$$

A photon collides with a stationary hydrogen atom in the ground state inelastically. The energy of the colliding photon is $$10.2$$ eV. After a time interval of the order of a microsecond, another photon collides with same hydrogen atom with an energy of $$15$$ eV. What will be observed by the detector?

1328144_1105150_ans_38cfbf2090714da797257acd00c9201d.jpg

Show that angular speed of electron in $${n}^{th}$$ Bohr's orbit is equal to :
$$ =\dfrac { \pi { me }^{ 4 } }{ { 2 }\epsilon _{ 0 }^{ 2 }{ h }^{ 3 }{ n }^{ 3 } }$$ or frequency of revolution, $$f=\dfrac { \pi { me }^{ 4 } }{ { 2 }\epsilon _{ 0 }^{ 2 }{ h }^{ 3 }{ n }^{ 3 } }$$

1310357_1138341_ans_af55c5b05e1040b891772c83ed9a4252.jpg

Obtain the expression for radius of $${n}^{th}$$ Bohr orbit and show that the radius is proportion to square of the principal quantum number.

1309977_1138334_ans_237f431f1f9a4d2f8f76ba65a42f17f5.jpg

Using expression for energy of electron, obtain the Bohr's formula for hydrogen spectral line.

1310688_1138789_ans_fe70bc03be41461a8bb209d08974c08d.jpg

In a sample of hydrogen-like atoms all of which are in the ground state, a photon beam containing photons of various energies is passed. In the absorption spectrum, five dark lines are observed. The number of bright lines in the emission spectrum will be (Assume that all transitions take place)

Absorption spectrum is observed when a photon is observed and a electron

Jumps to a higher state. Controversy emission spectrum is observed when

electron comes to lower state emitting a photon

On considering all possible transition states, thus no. of observation

and emission lines will be same.

1104849_1180100_ans_59e011da315a4aa19786062ca7a12c53.JPG

Using postulates of Bohr's theory of hydrogen atom, show that radii of orbits increases as $$n^2$$.

The capacity of the first cell is $$2$$
the capacity of second cell is $$8$$
$$8$$
$$8$$
$$8$$
$$8$$
$$8$$
formula for calculating is $$2n^2$$

The angular speed of electron in the nth orbit of hydrogen atom is

We know that the velocity of electron in the $$n^{th}$$ orbit is $$v_n=r_n\omega_n$$

$$\omega_n=$$ angular velocity

$$\omega_n=\dfrac{v_n}{r_n}$$

but $$v_n=\dfrac{z e^2}{2\in_onn}$$

$$\Rightarrow \omega_n=\dfrac{z e^2}{2\in_onn}\propto \dfrac{1}{r_n}$$

$$\therefore \omega_n\propto \dfrac{1}{n}$$.

Describe the drawback of Rutherford's model of atom.

1) Rutherford proposed that electrons revolve at high speed in circular orbits around a positively charged nucleus.

So according to Rutherford's model of atom, the electron would eventually fall into the nucleus. The result of this would be that the atom would collapse.

2) Rutherford proposed that electrons revolve around the nucleus in the ﬁxed orbits. However, he did not specify the orbits and the number of electrons in each orbit.

Find the radius of a $$He^+$$ ion in the state $$n=1$$.

$$n=1$$,

$$r=\dfrac { { E }_{ 0 }{ h }^{ 2 }{ n }^{ 2 } }{ \pi mZ{ e }^{ 2 } } $$

$$r=\dfrac { 0.53{ n }^{ 2 } }{ Z } { A }^{ o }=\dfrac { 0.53\times 1 }{ 2 } { A }^{ o }=0.265{ A }^{ o }$$

How many times does the electron go round the first orbit in a second?

The frequency of electron is given by

$$f=\dfrac{v}{2\pi r}$$

$$v=$$ velocity with which the electron are moving

$$r=$$ radius of the hydrogen atom

$$v=2-2\times 10^6$$ m/s

Radius of orbit$$=0.5287\times 10^{-10}$$m

$$f=\dfrac{2.2\times 10^6}{2\times 3.14\times 0.5287\times 10^{-10}}$$

$$=6.6\times 10^{15}$$Hz.

$$T=\dfrac{1}{f}=\dfrac{1}{6.6\times 10^{15}}=0.15\times 10^{-15}$$sec.

Find the approximate number of molecules contained in a vessel of volume 7 liters at $$0^{\circ}C$$ at

Given, pressure , P= 1.3 × 10^5 Pascal = 1.283 atm

volume, V = 7 litres

temperature, T = 0°C = 273K

we know from ideal gas law,

PV = nRT

n = RT/PV

= 0.082 × 273/(1.283 × 7) [ R = 0.082 L.atm/mol/k]

= 2.49

hence, number of mole = 2.49

so, number of molecules = mole × 6.023 × 10²³

= 2.49 × 6.023 × 10²³

= 14.99 × 10²³ ≈ 1.5 × 10²⁴

hence, number of molecules = 1.5 × 10²⁴

If the atom $$_{100}Fm^{215}$$ follows Bohr's model and the radius of the 5th orbit of this atom is p times the Bohr radius, then the value of p is :

$$\begin{array}{l} r={ a_{ \circ } }\left( { \frac { { { n^{ e } } } }{ z } } \right) \, \, \, \, \, \, \, \, \, \, \, \, { a_{ \circ } }=bohr\, \, radius \\ \Rightarrow r={ a_{ \circ } }\left( { \frac { { 25 } }{ { 100 } } } \right) =p{ a_{ \circ } } \\ \Rightarrow p=\frac { 1 }{ 4 } \end{array}$$

Find the ratio of diameter of electron in 1st Bohr orbit to that in 4th bohr orbit

diameter$$=\dfrac{{1.06{n^2}}}{{Z\,ev}}$$

So ratio$$=\dfrac{1}{{{4^2}}} = \dfrac{1}{{16}}$$

We get, $$1:16$$

Write three conclusions made by Rutherford based on the alpha particle scattering experiment.

Conclusions made by Rutherford based on the alpha particle scattering experiment are :

There is a positively charged centre in an atom called the nucleus. Nearly all the mass of an atom resides in the nucleus.
The electrons revolve around the nucleus in circular paths.
The size of the nucleus is very small as compared to the size of the atom.

What conclusion was drawn by Rutherford from his $$\alpha$$ -particle scattering experiment?

Conclusion of Rutherford's scattering experiment:

1) Most of the space inside the atom is empty because most of the $$\alpha$$-particles passed through the gold foil without getting deflected.

2) Very few particles were deflected from their path, indicating that the positive charge of the atom occupies very little space.

3) A very small fraction of $$\alpha$$-particles were deflected by very large angles, indicating that all the positive charge and mass of the gold atom were concentrated in a very small volume within the atom.

The Bohr's radius is given by $$a_{0}=\dfrac{\varepsilon_{0}h^{2}}{\pi me^{2}}$$. Verify that the RHS has dimensions of length.

$${a}_{\circ}=\dfrac{{\epsilon}_{\circ}{h}^{2}}{\pi m{e}^{2}}$$

$$=\dfrac{{A}^{2}{T}^{2}{\left[M{L}^{2}{T}^{-1}\right]}^{2}}{{L}^{2}ML{T}^{-2}M{\left[AT\right]}^{2}}$$

$$=\dfrac{\left[{M}^{2}{L}^{4}{T}^{-2}\right]}{\left[{M}^{2}{L}^{3}{T}^{-2}\right]}$$

$$=\left[L\right]$$

$$\therefore {a}_{\circ}$$ has dimensions of length.

What do you think would be the observation if the $$\alpha$$ particle scattering experiment is carried out using a foil of a metal other than gold?

If the $$\alpha$$-scattering experiment is carried out using a foil of a metal rather than gold, there would be no change in the observation.

In the $$\alpha$$-scattering experiment, a gold foil was taken because gold is malleable and a thin foil of gold can be easily made.

It is difficult to make such foils from other metals.

What is the Rutherford's atomic model?

Ernest Rutherford performed an experiment to know the arrangement of electrons within an atom. In this experiment, fast-moving alpha particles were made to fall on a thin gold foil(100 nm thickness). The $$\alpha$$-particles are doubly charged helium ions. Since they have a mass of 4 u, the fast-moving $$\alpha$$-particles have a considerable amount of energy. It was expected that $$\alpha$$-particles would be deflected by subatomic particles in the gold atom. Since $$\alpha$$-particles were much heavier than protons, he did not expect to see large deflections.

But, the $$\alpha$$-particle scattering experiment gave totally unexpected results.
The following observations were made:
(i) Most of the fast-moving $$\alpha$$-particles passed straight through the gold foil means most of the space in an atom is empty.
(ii) Some of the particles were deflected by the foil by small angles means there is some positive charge present.
(iii) Very few particles deflected at large angles or deflected back. Moreover, very few particles deflected at $$180^o$$. Therefore, he concluded that the positively charged particles covered a small volume of an atom in comparison to the total volume of an atom.

Enlist the conclusions drawn by Rutherford from his $$\alpha$$ ray scattering experiment.

Rutherford concluded from the $$\alpha$$-particle scattering experiment that:–

(i) Most of the space inside the atom was empty because most of the $$\alpha$$-particles passed through the gold foil without any deflection.
(ii) Very few particles were deflected from their path, indicating that the positive charge occupied very little space inside the atom.
(iii) A very small fraction of $$\alpha$$-particles were deflected by $$180^o$$, indicating that the positive charge and the mass of the gold atom is concentrated in a very small volume within the atom.

From the data, he also calculated that the radius of the nucleus was about 105 times smaller than the radius of the atom.

Explain emission and absorption spectra

Types of spectra
Definition for emission and absorption spectrum Explanation for three types of emission spectra (appearance and example)
(i) continuous emission
(ii) line emission
(iii) band emission
Explanation for three types of absorption spectra (appearance and example)
(i) continuous absorption
(ii) line absorption
(iii) band absorption

What will happen if instead of goldfoil, aluminium foil is used in the experiment of Rutherford's experiment of $$\alpha $$ scattering particle?

1359564_1585734_ans_45802c00e8854eacb8789c925d891504.jpeg

A hydrogen atom emits ultraviolet radiation of wavelength $$102.5 n m.$$ What are the quantum numbers of the states involved in the transition ?

As the light emitted lies in ultraviolet range the line lies in hyman series.
$$\dfrac{1}{\lambda} = R \Big( \dfrac{1}{n_1^2} - \dfrac{1}{n_2^2} \Big)$$

$$\implies \dfrac{1}{102.5 \times 10^{-9}} = 1.1 \times 10^7 (1/1^2 - 1/n_2^2)$$

$$\implies \dfrac{10^9}{102.5} = 1.1 \times 10^7 (1 - 1/n_2^2) \implies \dfrac{10^2}{102.5} = 1.1 \times 10^7 (1 - 1/n_2^2)$$

$$\implies 1 - \dfrac{1}{n_2^2} = \dfrac{100}{102.5 \times 1.1} \implies \dfrac{1}{n_2^2} = \dfrac{1-100}{1102.5 \times 1.1}$$

$$\implies n_2 = 2.97 = 3$$

Explain the conclusions of Rutherford's model of Atom.

Rutherford's atomic model is the model which described the atom as a tiny, dense, positively charged core called a nucleus, in which nearly all the mass is concentrated, around which the light, negative constituents, called electrons, circulate at some distance, much like planets revolving around the Sun.

Find the maximum Coulomb force that can act on the electron due to the nucleus in a hydrogen atom.

$$F = \dfrac{q_1q_2}{4\pi \varepsilon_0 r^2}$$
[Smallest dist. between the electron and nucleus in the radius of first Bohr's orbit]

So, the force obtained will be:
$$= \dfrac{(1.6 \times 10^{-19}) \times (1.6 \times 10^{-19}) \times 9 \times 10^0}{(0.53 \times 10^{-10})^2} $$

$$= 82.02 \times 10^{-9} $$

$$= 8.202 \times 10^{-8} = 8.3 \times 10^{-8} N$$

Find the radius and energy of a $$He^+$$ ion in the states (a) n = 1, (b) n = 4 and (c) n = 10.

a)
$$n = 1, \ r = \dfrac{\in_0 h^2 n^2}{\pi mZe^2} = \dfrac{0.53 n^2}{Z} \mathring{A} = \dfrac{0.53 \times 1}{2} = 0.265 \mathring{A}$$

$$E = \dfrac{-13.6 z^2}{n^2} = \dfrac{-13.6 \times 4 }{1} = -54.4 \ eV $$

b)
$$n = 4, \ r = \dfrac{0.53 \times 16}{2} = 4.24 A$$
$$E = \dfrac{-13.6 \times 4}{164} = -3.4 eV$$

c)
$$n = 10, \ r = \dfrac{0.53 \times 100}{2} = 26.5 A$$

$$E = \dfrac{-13.6 \times 4}{100} = -0.544 eV$$

(a) How many l values are associated with $$n =3$$ ? (b) How many $$m_l$$ values are associated with $$ l= 1$$?

(a) For a given value of the principal quantum number n, the orbital quantum number l ranges from 0 to n – 1. For $$ n = 3$$, there are three possible values: 0, 1, and 2.
(b) For a given value of l, the magnetic quantum number $$m_l$$ ranges from −l to +l . For l = 1, there are three possible values: – 1, 0, and +1.

An electron, trapped in a one-dimensional infinite potential
well $$250\ pm$$ wide, is in its ground state. How much energy must it
absorb if it is so jump up to the state with $$n=4?$$

We first note that since
$$h=6.626 \times 10^{-34}\ J.s$$ and $$ c=2.998 \times 10^{8}\ m/s,$$
$$hc=\dfrac{(6.626 \times 10^{-34}\ J.s)(2.998 \times 10^8\ m/s)}{(1.602 \times 10^{-19}\ J.eV)(10^{-9}\ m/nm)}=1240eV.nm$$.
Using the $$mc^2$$ value for an electron from Table $$(511 \times 10^3\ eV)$$, Eq. can berewritten as
$$E_n=\dfrac{n^2h^2}{8mL^2}=\dfrac{n^2(hc)^2}{8(mc^2)L^2}$$The nergy to be absorbed is therefore
$$\triangle E=E_4-E_1=\dfrac{(4^2-1^2)h^2}{8m_eL^2}=\dfrac{15(hc)^2}{8(m_ec^2)L^2}=\dfrac{15 (1240eV.nm)^2}{8(5.11 \times 10^3eV)(0.250nm)^2}=90.3eV$$.

A hydrogen atom is excited from its ground state to the state with $$n=4$$;
what are the highest

The greatest energy is

$$E_{4\rightarrow 1}=12.8\ eV$$

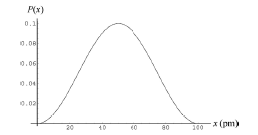
An electron is trapped in a one dimensional infinite potential well that is $$100\ pm$$ wide; the electron is in its ground state. What is the probability that you can detect the electron in an interval of width $$\Delta x=5.0\ pm$$ centered at $$x=$$
$$25\ pm$$

The probability that the electron is found in any interval is given by $$P=\displaystyle\int |\psi|^{2}dx$$. where the integral is over the interval. If the interval width $$\Delta x$$ is small, the probability can be approximated by $$P=|\psi|^{2}\Delta x$$, where the wave functions is evaluated for the center of the interval say. For an electron trapped in an infinite well of width $$L$$, the ground state probability density is
$$|\psi|^{2}=\dfrac{2}{L}\sin^{2}\left(\dfrac{\pi x}{L}\right)$$
$$P=\left(\dfrac{2\Delta x}{L}\right)\sin^{2}\left(\dfrac{\pi x}{L}\right)$$
We take $$L=100\ pm, x=25\ pm$$, and $$\Delta x=5.0\ pm$$. Then,
$$P=\left[\dfrac{2(5.0\ pm)}{100\ pm}\right]\sin^{2}\left[\dfrac{\pi(25\ pm)}{100\ pm}\right]=0.050$$.
1700974_1775298_ans_c5cb78a1bc904fb5b17f9962f5b36cff.png

1700974_1775298_ans_c5cb78a1bc904fb5b17f9962f5b36cff.png

A one dimensional infinite well of length $$200\ pm$$ contains an electron in its third excited state. We position an electron detector probe of width $$2.00\ pm$$ so that it is centered on a point of maximum probability density.
What is the probability of detection by the probe?

The position of maximum probability density corresponds to the center of the well $$x=L/2=(200\ pm)/2=100\ pm$$
The probability of detection at $$x$$ is given by Eq $$39-11$$:

$$p(x)=\pi s_{n}^{2}(x)dx=\left[\sqrt{\dfrac{2}{L}}\sin \left(\dfrac{n\pi}{L}x\right)\right]^{2}dx=\dfrac{2}{L}\sin^{2}\left(\dfrac{n\pi}{L}x\right)dx$$

For $$n=3, L=200\ pm$$ and $$dx=2.00\ pm$$ (width of the probe), the probability of detection at $$x=L/2=100\ pm$$ is

$$p(x=L/2)=\dfrac{2}{L}\sin^{2}\left(\dfrac{3\pi}{L}.\dfrac{L}{2}\right)dx=\dfrac{2}{L}\sin^{2}\left(\dfrac{3\pi}{2}\right)dx=\dfrac{2}{L}dx=\dfrac{2}{200\ pm}(2.00\ pm)=0.020$$

Which scientist concluded that the size of the nucleus is very small as compared to the size of an atom?

Rutherford through his gold foil experiment concluded that the size of the nucleus is very small ($$10^5$$ times small ) as compared to the size of an atom.

How many electron states are there in the following shells: $$(a) n ! 4, (b) n ! 1, (c) n ! 3, (d) n ! 2$$?

For a given quantum number n there are n possible values of l, ranging from 0 to n – 1. For each l the number of possible electron states is $$ N_l = 2(2l + 1) $$ . Thus, the total number of possible electron states for a given n is

$$N_n = \overset {n-1} {\underset {n=1} {\Sigma}} N_l = 2 \overset{n-1}{\underset{n=1}{\Sigma}} (2l + 1) = 2n^2 $$.

(a) In this case $$n = 4$$, which implies $$N_n = 2(4^2) =32$$
(b) Now $$ n=1 $$, so $$N_n = 2(2^2) =8$$
(c) Here $$ n = 3$$, and we obtain $$N_n = 2( 3^2)= 18$$
(d) Finally, $$n=2 \rightarrow N_n =2(2^2) = 8$$

A hydrogen atom is excited from its ground state to the state with $$n=4$$
How many different energies are possible

There are $$6$$ possible energies associated with the transitions $$4\rightarrow 3, 4\rightarrow 2,$$

$$ 4\rightarrow 1, 3\rightarrow 2, 3\rightarrow 1$$

and $$2\rightarrow 1$$

What are the frequency range of the Lyman series?

Weknow,

For the Lyman series.

$$\Delta f=\dfrac{2.998\times 10^{8}\ m/s}{91.4\times 10^{-9}\ m}-\dfrac{2.998\times 10^{8}\ m/s}{122\times 10^{-19}m}$$

$$=8.2\times 10^{14}Hz$$

How many electron states are in these subshells:$$ (a) n = 4, l = 3; (b) n = 3, l = 1 ; (c) n = 4, l = 1 ; (d) n = 2, l =0$$ ?

For a given quantum number l there are (2 l + 1) different values of $$m_l$$ . For each given $$m_l$$ the electron can also have two different spin orientations. Thus, the total number of electron states for a given l is given by $$N_l = 2(2 l + 1)$$.
(a) Now $$ l = 3$$, so $$N_l = 2(2 \times 3 + 1) = 14$$ .
(b) In this case, $$l = 1$$ ,which means $$N_l = 2(2 \times 1 + 1) = 6$$.
(c) Here $$ l = 1$$, so $$N_l = 2(2 \times 1 + 1) = 6$$.
(d) Now $$l = 0$$, so $$ N_l = 2(2 \times 0 + 1) = 2$$.

An electron in a multielectron atom has $$m_l = +4$$ . For this electron, what are (a) the value of l , (b) the smallest possible value of n, and (c) the number of possible values of $$m_s$$?

(a) using the table following table, we find $$l = [m_l]_{max} = 4$$.

(b) The smallest possible value of n is $$ n ={l_{max }+1} \geq {l + 1} = 5$$ .

1710692_1778276_ans_d3faddf101cc4e0f96fb7e9307aa1ba1.png

State Bohrs quantization condition for defining stationary orbits.

Quantum Condition: The stationary orbits are those in which angular momentum of the electron is an integral multiple of $$\frac{h}{2\pi}$$ i.e.,
$$L=mvr=\dfrac{nh}{2\pi}$$, $$n=1,2,3, \,...$$
Integer n is called the principal quantum number. This equation is called Bohr's quantum condition

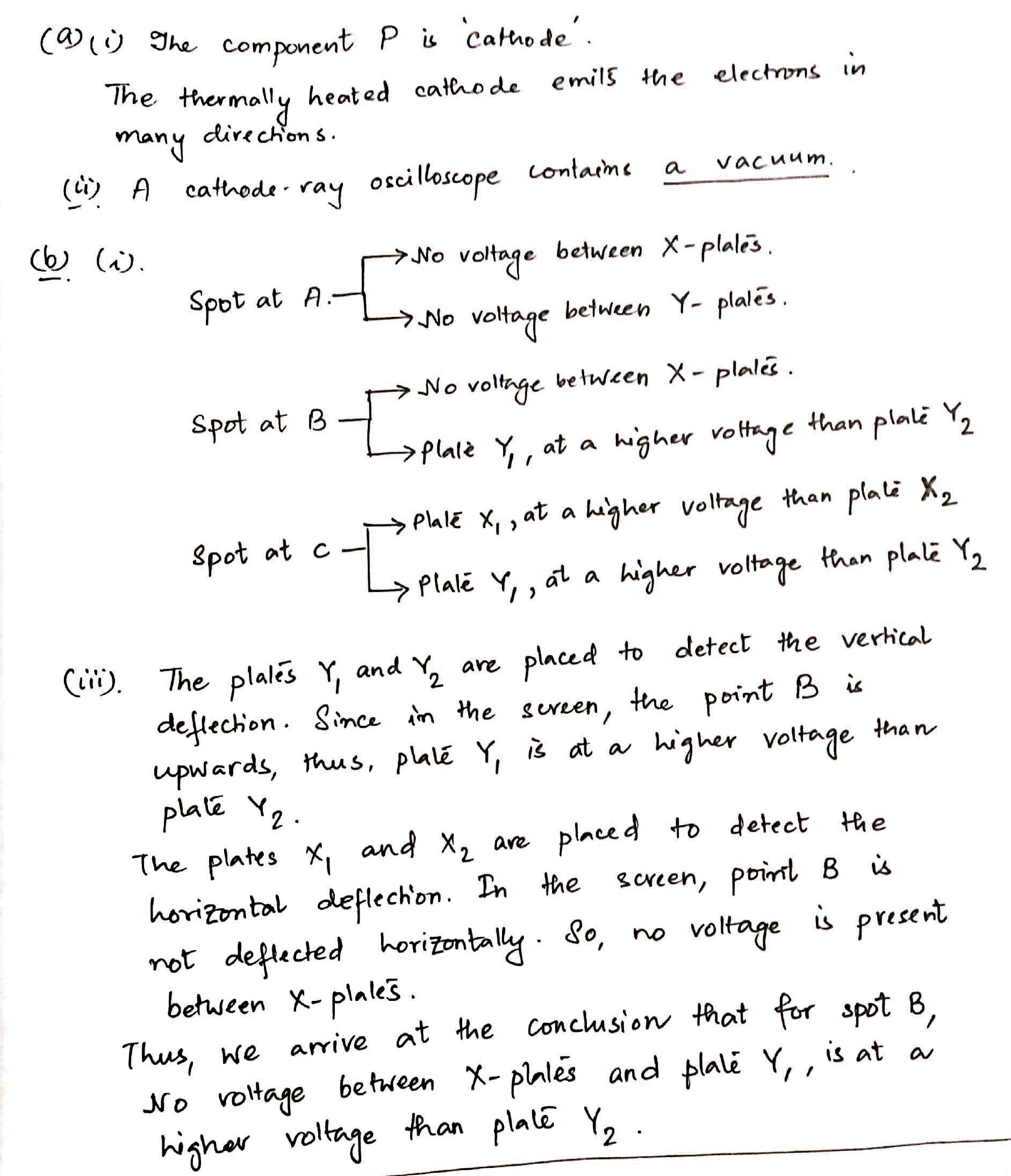
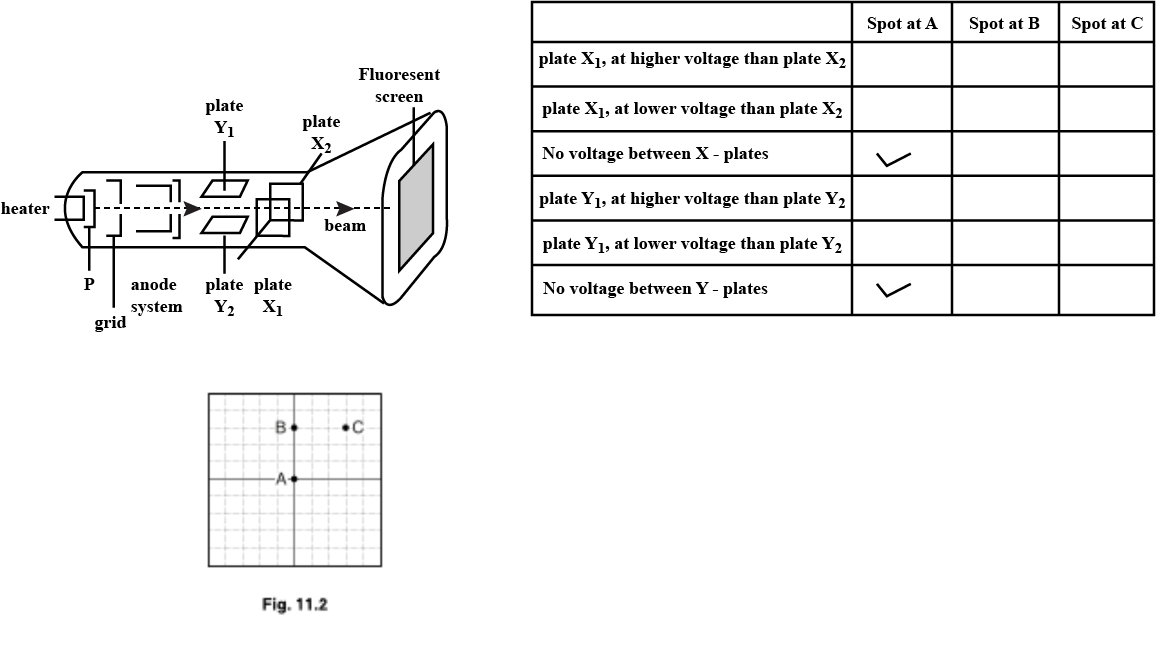
Figure shows the main components of a cathode-ray oscilloscope.
(a) (i) State the function of component P.
(ii) Tick one box to complete the sentence correctly.
A cathode-ray oscilloscope contains
$$\square$$ air at about five times normal atmospheric pressure.
$$\square$$ air at about normal atmospheric pressure.
$$\square$$ air at about one fifth of normal atmospheric pressure.
$$\square$$ a vacuum.
$$\square$$ neon gas.

(b) Fig. $$11.2$$ shows the front view of the screen of the cathode-ray oscilloscope.
With no voltage applied between the X-plates or between the Y-plates, the spot is at A.
(i) Places two ticks in each of the each of the table to describe the voltage across the plates when the spot is at points B and C. the column for the spot at A has been completed as an example.
(iii) Explain your answers for the spot at point B.

1924167_1913135_ans_665ca84df5b849d788789ea7970c4431.jpg

An electron is in a state with $$ n = 3$$. What are (a) the number of possible values of l , (b) the number of possible values of $$m_l$$, (c) the number of possible values of $$m_s$$, (d) the number of states in the $$n = 3$$ shell, and (e) the number of subshells in the $$ n = 3$$ shell?

(a) For $$n = 3$$ there are 3 possible values of l : 0, 1, and 2.
(b) We interpret this as asking for the number of distinct values for $$m_l$$ (this ignores the multiplicity of any particular value). For each $$l$$ there are $$2l +l$$ possible values of $$m_l$$ . Thus the number of possible's $$m_l$$ for $$l = 2$$ is $$(2 l + 1) =5$$. Examining the $$l = 1$$ and $$ l= 0$$ cases cannot lead to any new (distinct) values for $$m_l$$ , so the answer is 5.
(c) Regardless of the values of n, l and $$m_l$$ , for an electron there are always two possible values of $$m_s:± \frac{1}{2 }$$.
(d) The population in the $$n = 3$$ shell is equal to the number of electron states in the shell, or $$2n^2 = 2(3^2) = 18$$.
(e) Each subshell has its own value of A . Since there are three different values of $$l$$ for n= 3, there are three subshells in the $$n = 3$$ shell.

In the subshell $$ l = 3$$ , (a) what is the greatest (most positive) $$m_l $$ value, (b) how many states are available with the greatest $$ m_l $$ value, and (c) what is the total number of states available in the subshell?

(a) For $$ l = 3$$, the greatest value of $$m_l$$ is $$ m_l= 3$$ .

(b) Two states $$(m_s = ± \frac {1}{2}
)$$are available for $$m_l = 3$$ .

(c) Since there are 7 possible values for $$m_l : {+3}, {+2}, {+1},{0}, {–1},{ – 2},{ – 3}$$, and two possible
values for $$m_s$$, the total number of state available in the subshell $$l = 3$$ is 14.

What is the ratio of radii of the orbits corresponding to the first excited state and ground state in a hydrogen atom?

Given the bohr's radius

$$r_n=\dfrac{\epsilon_0h^2n^2}{\pi me^2} \,\propto \,n^2$$
For I excited state, n = 2
For ground state, n = 1
$$\therefore \frac{r_2}{r_1}$$$$=\frac{4}{1}$$

Write the expression for Bohr's radius in the hydrogen atom.

Bohr's radius, $$r_1=\dfrac{\epsilon_0h^2}{\pi me^2}=0.529\times 10^{-10}m$$

The radius of the innermost electron orbit of a hydrogen atom is $$5.1 \times 10^{-11} m$$.What is the radius of the orbit in the second excited state?

In-ground state, $$n = 1$$
In the second excited state, $$n = 3$$
As $$r_n\,\propto\,n^2$$
$$\therefore \frac{r_3}{r_1}=(\frac{3}{1})^2=9$$
$$r_3=9r_1=9\times 5.1\times10^{-11}\,m=3.77\times10^{-10}m$$

Which part of atom was discovered by Rutherford.

$$\text {nucleus}$$

Ernest Rutherford discovered the nucleus of the atom in 1911.

In Rutherford's $$\alpha$$-particle scattering experiment he found that most of the $$\alpha$$-particles pass straight without any deflection through gold foil and very few of the $$\alpha$$-particles were deflected, From this experiment, he concluded that

1) Most of the space of the atom is empty because most of the $$\alpha$$- particles pass straight through the gold foil.

2) As very few $$\alpha$$-particles were deflected indicates that positive charge is concentrated in a very small space.

On the basis of the above experimental observation, he concluded that there is a positively charged center in an atom called a $$\text{nucleus}$$ which nearly has the whole mass of the atom.

Electric Potential and Potential Energy Due to Point Charges(35)
In 1911, Ernest Rutherford and his assistants Geigerand Marsden conducted an experiment in which they scattered alpha particles (nuclei of helium atoms) from thin sheets of gold. An alpha particle, having charge $$+2_e$$ and mass $$6.64 \times 10^{-27} \,kg$$, is a product of certain radio active decays. The results of the experiment led Rutherford to the idea that most of an atoms mass is in a very small nucleus, with electrons in orbit around it. (This is the planetary model of the atom, which well study in Chapter 42.) Assume an alpha particle, initially very far from a stationary gold nucleus, is fired with a velocity of $$2.00 \times 10^7 \,m/s$$ directly toward the nucleus $$(charge +79_e)$$. What is the smallest distance between the alpha particle and the nucleus before the alpha particle reverses direction? Assume the gold nucleus remains stationary.

1974799_1861545_ans_b3cbe7b95dbf438da2e2c24fca74be44.jpg

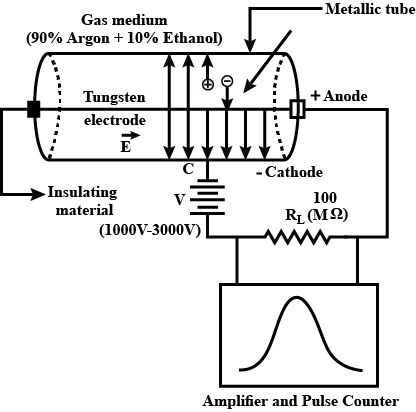
Explain the construction and working of a Geiger-Muller Counter.

Construction

A cylindric metallic tube is filled with $$90\%$$ Argon and $$10\%$$ ethanol and a tungsten electrode is connected as shown in the figure. And further electrode is connected to a external circuit which contains high voltage battery and circuit is completed by connecting battery to metallic tube. This creates an electricfield directed towards an electric field from tungsten electrode.

Working

Whenever a nucleus enters the metallic tube, it leads to creation of a positive and an electron.
In the electric field $$\vec{E}$$ electron accelerates towards tungsten electrode while positive ion moves towards metallic tube. When electrons accelerates it knocks out some other electrons from other atom's orbit resulting electrons do the same creating an avalanche effect.

All electrons move towards anode and complete the circuit giving potential difference across $$R_L$$

And recombine with +ve ions collected at point C, which completes the circuit. The signal generated across $$R_L$$ is amplified and sent to pulse counter. It Scouts this as 1 nucleus detected. During this period if any other nucleus enters metallic tube it is not counted, this is called dead period.

1709040_1811768_ans_ec638b1a8ace438ebd3dca7d216e11d7.png

How was it shown that atom has empty space?

Rutherford experimented by allowing a stream of the alpha particles to pass through a very thin gold foil. He observed that most of the alpha particles passed through the metal foil without deviating from their path. This goes to show that an atom has a large empty space.

Explain Rutherford-Soddy law.

Rutherford Soddy Law of Radioactive Decay

In $$1902$$, Rutherford and Soddy studied the disintegrating of many radioactive substance and found the following conclusion regarding radioactive decay known as Rutherford and Soddy Rules. According to:

1. Radioactivity is a nuclear phenomonon and the rate of emission of radioactive ray cannot be controlled by physical or chemical process that mean neither can it be extended nor can it be reduced.

2. The nature of the disintegration of radioactive substance is statistical, this is, it very difficult to say which nucleus will be disintegrated and which particle will emit $$\alpha, \beta$$ and $$\gamma$$ With the emission of $$\alpha, \beta$$ and $$\gamma$$ rays in the process of disintegration one element change into another new element, its chemical and radioactive quantities are completely new.

3. At any time the rate of decay of radioactive atom is proportional to the number of atoms present at that time.

Let the number of atoms present at any given time $$t$$ is $$N$$ and at the time $$t+\Delta t$$ this number decreases $$N-\Delta N$$ to its value then, the rate of decay of atoms is $$-\dfrac{\Delta N}{\Delta t}$$. Therefore, according to the law of Rutherford and Soddy.

If at the time $$\Delta t$$ the nucleus $$\Delta N$$ will be disintegrated then the rate of disintegration,

$$\dfrac{-dN}{dt}\propto N$$

$$\dfrac{-dN}{dt}=\lambda N$$

or $$dN=-\lambda Ndt$$ .......(1)

Now, arranging equation $$(1)$$ again,

$$\dfrac{dN}{N}=-\lambda dt$$

Integrating both the sides, we have

$$\displaystyle\int_{N_{0}}^{N}\dfrac{dN}{N}=-\lambda \int_{0}^{t}dt$$

$$\ln N-\ln N_{0}=-\lambda t$$ or $$\ln \dfrac{N}{N_{0}}=-\lambda t$$

Taking the exponential on both the sides, we get

$$\dfrac{N}{N_{0}}=e^{-\lambda t}$$ or $$N=N_{0}e^{-\lambda t}$$ ....(2)

Where $$N_{0}$$ is the number of active nuclei at time $$t=0$$. From equation $$(2)$$, it is apparent that the number of nuclei to be decayed decreases exponentially. It is shown in figure $$15.5$$

Number of nuclei decayed in time $$t$$,

$$N_{0}-N=N_{0}-N_{0}e^{-\lambda t}=N_{0}(1-e^{-\lambda t})$$ ....(3)

Thus, fraction of decayed nuclei in time $$t$$,

$$\dfrac{N_{0}-N}{N_{0}}=1-e^{\lambda t}$$

1758481_1836772_ans_a5b247a716bc492fa378bf054a5546b7.png

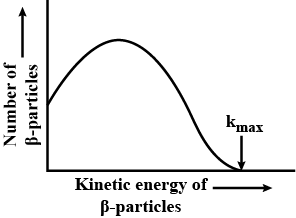
Out of which, $$\alpha$$ and $$\beta$$ rays, has wide spectrum?

$$\alpha$$ particles has wide spectrum.

Out of which, $$\alpha$$ and $$\beta$$ rays, has wide spectrum ?

1882997_1836746_ans_03fa7e3da50c4e2a9130e8aa6dca2e23.jpg

What does it mean when we say that $$\beta$$-ray spectrum is a continuous energy spectrum ?

A process in which a nucleus spontaneously emits an electron or a positron is called $$\beta-$$ decay. The atomic number $$(Z)$$ of daughter nucleus increases by unity, but the mass $$(A)$$ remains unchanged.

Symbolically ($$\beta^{-}$$ decay is represented as:

$$\underset{Z}{A}X\rightarrow \ ^{A}_{Z+1}Y+\ ^{0}_{-1}e+\bar{v}$$

An example of $$\beta^{-}$$ decay is

$$\ ^{234}_{90}Th\rightarrow \ ^{234}_{91}Pa+\ ^{0}_{-1}e+\bar{v}$$

Here, we can see that charge and the number of nucleus are conserved in this type of decay. Antineutrino $$(\bar{V})$$ is a neutral particle whose rest mass is very small.Total charge before reaction is $$+90e$$ charge after reaction $$-91\ e+(-e)+090e$$. As electrons and anti-neutrino both are not nucleons. Thus, the number of nucleons remains the same, $$234$$.

It is surprising that nucleus emits electrons (positrons) and neutrino because it has only neutrons and protons. In the previous chapter we have seen that an atom emits photons, we never say that photons are present in an atom. We say that photons are formed in the emission process. Similarly, in the $$\beta-$$ decay, electrons (positrons) and antineutrino (neutrino) are formed. They are formed during the emission process.

We can also write.

$$n\rightarrow p+\bar{e}+\bar{V}$$

Here, we can see that nucleus do not vary in this process.

We can calculate disintegration energy in $$\beta^{-}$$ decay. Let the nuclear masses of $$X$$ and $$Y$$ be $$m_{x}$$ and $$m_{y}$$ and mass of electron is $$m_{e}$$, then mass lost

$$\Delta m=m_{x}-[m_{y}\times m_{e}]$$ ..........(2)

Where antineutrino is considered to be massless. Adding and subtracting $$Zme$$ on the right side of equation $$(2)$$, we get

$$\Delta m=(m_{x}+Zm_{e})-[m_{y}+(Z+1)m_{e}]$$

or, $$\Delta m=M_{x}-M_{y}$$ .........(3)

Where, $$M_x$$ and $$M_y$$ the atomic masses of $$X$$ and $$Y$$ respectively, here, disintegration energy,

$$Q=\Delta mc^{2}=(M_x-M_y)c^{2}$$ ..........(4)

The emitted $$\beta-$$ rays a range to energy varying from zero to a maximum value. The distribution of kinetic energies of $$\beta-$$ rays during $$\beta-$$ decay is shown in figure $$15.9$$.

In positive $$\beta(\beta^{+})$$ decay, a positron and a neutrino are emitted from the nucleus. The symbolic representation of such a decay is

$$\ ^{A}_{Z}X\rightarrow \ ^{A}_{Z-1}Y+\ ^{0}_{1}e^{+}+v$$ ......(5)

An example of such a decay is:

$$\ ^{23}_{11}Na\rightarrow \ ^{23}_{10}Ne+\ ^{0}_{1}e^{+}+v$$

Here mass number $$A$$ is unchanged where as atomic reduces by unity. Like antineutrino, neutrino $$v$$ is chargeless and almost massless. Neutrino and positrons are also formed during this,

$$p\rightarrow n+e^{+}+v $$ ........(6)

In $$\beta^{+}$$ decay, like $$\beta^{-}$$ decay, there is conservation of number of nucleons and charge. The disintegration energy for $$\beta^{+}$$ decay,

$$Q=(M_{x}-M_{y}-2m_{e})c^{2} ........ (15.31)$$

In some $$P$$ decay, the parent nucleus accepts an electrons and proton combines with it to form a neutron and emits a neutrino. Symbolic representation for such a decay is:

$$\ ^{A}_{Z}X+\ ^{0}_{-1}e\rightarrow \ ^{A}_{Z-1}Y+v$$

An example of such a process is:

$$\ ^{64}_{29}Cu+\ ^{0}_{-1}e\rightarrow \ ^{64}_{28}Ni+v$$

and $$Q$$ value for such a process,

$$Q=(M_{x}-M_{y})c^{2}$$

From $$\beta$$ decay, we get to know that neutron and proton are not fundamental particles. It is note worthy that the process $$n\rightarrow p+e^{-}+v$$ is possible both in the cases: inside and outside the nucleus, i.e. an isolated neutron can decay to from a proton. However the process $$p\rightarrow n+e^{+}+v$$ is not possible outside the nucleus. As the mass of neutron is more than that of proton. Thus, it is not possible to decay an isolated proton into a neutron.

1758497_1836777_ans_b38ecf6d8f31455a8194f501afb0acc6.png

Choose the correct alternatives from the clues given at the end of the each statement:
The positively charged part of the atom possess most of the mass in ..................
(Rutherford's model / both the models)

The positively charged part of the atom possesses most of the mass in both the models.

The radius of $$1^{st}$$ electron orbit of hydrogen atom is $$5.3\times 10^{-11}m$$. What is the radius of $$2^{nd}$$ orbit?

Radius of $$n^{th}$$ orbit, $$r_{n}\propto n^{2}$$
$$\dfrac{r_{2}}{r_{1}}=\dfrac{2^{2}}{1^{2}}$$
$$\Rightarrow \dfrac{r_{2}}{r_{1}}=4$$
$$\therefore r_{2}=4r_{1}=4\times 5.3\times10^{-11}$$

$$=2.12\times10^{-10}m$$

What is the ratio of radii of the orbits corresponding to first excited state and ground state of hydrogen atom?

Radius of $$n^{th}$$ orbit, $$r_{n}\propto n^{2}$$
The ground state corresponds to $$n=1$$ and the first excited state corresponds to $$n=2$$
$$\therefore \dfrac{r_{2}}{r_{1}}=\dfrac{2^{2}}{1^{2}}$$
$$\dfrac{r_{2}}{r_{1}}=4$$

Suppose you are given a chance to repeat the alpha-particle scattering experiment using a thin sheet of solid hydrogen in place of the gold foil. (Hydrogen is a solid at temperatures below $$14K$$.) What results do you expect?

The nucleus of hydrogen contains only one proton. Its mass is $$1.67\times 10^{-27}kg$$. The mass of an alpha-particle is $$6.64\times 10^{-27}kg$$. When the alpha particles are incident on a thin sheet of solid hydrogen [in place of the gold foil], even in a head-on collision, the alpha-particles will not be scattered back. It is because the scattering particle is more massive than the target particle.

In accordance with the Bohr's model, find the quantum number that characteristics the earth's revolution around the sun in an orbit of radius $$1.5\times 10^{11}m$$ with orbital speed $$3\times10^{4}m/s$$. (Mass of earth = $$6.0\times10^{24}$$).

Here, $$m=6.0\times 10^{24}kg$$, $$r=1.5\times10^{n}m$$ and $$v=3\times10^{4}ms^{-1}$$
The angular momentum of the earth,
$$mvr=6.0\times 10^{24}\times 3\times10^{4}\times1.5\times10^{11}=2.7\times 10^{40}kg$$ $$ms^{-1}$$
In accordance with the Bohr's model,
$$mvr=n\dfrac{h}{2\pi}$$
or, $$n=mvr\times\dfrac{2\pi}{h}=\dfrac{2.7\times10^{40}\times2\pi}{6.62\times 10^{-34}}\approx 10^{74}$$.

Choose the correct alternatives from the clues given at the end of the each statement:
An atom has a nearly continuous mass distribution in a ............. but has a highly non-uniform mass distribution in.
(Thomson's model / Rutherford's model)

An atom has a nearly continuous mass distribution in Thomson’s model, but has a highly non-uniform mass distribution in Rutherford’s model.

When a beryllium metal is bombarded with an alpha particle, a new element carbon is produced. But when a gold plate is bombarded with an alpha particle, no new element is produced. Why?

The atomic number of gold is 79. Therefore, the number of proton in gold nucleus is 79. This high number of protons is responsible for deflection of alpha particle which is positively charged. Hence, the alpha particle can not strike the nucleus of gold. On the other hand, the atomic number of beryllium is 4. Thus, the alpha particle can strike the Be nucleus without being much deflected. This bombardment will generate new element - carbon.

$$^9_4Be + \ ^4_2He \to ^{12}_6C + \ ^1_0n$$

Why the atomic spectrum of an element is called the finger print of that element?

The atomic spectrum of an element is called the finger print because one can identify the element looking at the spectrum. The spectrum of two different elements can not be exactly similar just like the finger print of two different persons.

Based on Rutherford’s model of an atom, which sub-atomic particles are present in the nucleus of an atom?

Rutherford suggested that there is a positively charged centre in

an atom called the nucleus. Nearly all the mass and all of the positive charge of an atom resides in the nucleus. The subatomic particle which has charge, as well as considerable mass, is a proton. Hence, based on Rutherford's model of an atom, proton is the sub-atomic particle present in the nucleus of an atom.

Consider the possible vibrations modes in the following linear molecules:
(a) $$CO_2 (O - C - O) $$; (b) $$C_2H_2 (H - C - C - H) $$

1971227_1855655_ans_9b185e2e04ce4336998b96ec613c1eb6.jpg

Is it possible for a hydrogen $$(_1^1 H)$$ nucleus to emit an alpha particle? Give a reason for your answer.

$$\alpha -particle\, {_2^4{He}}$$

$$\therefore$$ Hydrogen has less $$A$$ and $$Z$$ as compared to helium, so, it cannot emit alpha particle.

Calculate for a hydrogen atom and a $$He^+$$ ion:
(a) the radius of the first Bohr orbit and the velocity of an electron moving along it;
(b) the kinetic energy and the binding energy of an electron in the ground state;
(c) the ionization potential, the first excitation potential and the wavelength of the resonance line $$(n' = 2 \rightarrow n = 1) $$.

1880416_1854426_ans_f321f3d4d5be48668e8fe2e793ded9ae.jpg

Evaluate how many lines there are in a true rotational spectrum of CO molecules whose natural vibration frequency is $$ \omega = 4.09 \times 10^{14}\,s^{-1}$$ and moment of inertia $$I = 1.44 \times 10^{-39} \, g\cdot cm^{2}$$.

1971219_1855594_ans_821c7464fc60491b8de94b539b2f7efb.jpg

The difference between $$(n+2)^{th}$$ Bohr radius and $$n^{th}$$ Bohr radius is equal to the $$(n-2)^{th}$$ Bohr radius. The value of $$n$$ is:

$$r=\dfrac {n^2h^2\epsilon_0}{Z\pi me^2}=0\cdot 529\times \dfrac {n^2}{Z}A^0$$

As $$r_n \propto n^2$$ so, $$r_{n+2}=k(n+2)^2; r_n=kn^2$$ and $$r_{n-2}=k(n-2)^2$$

Here, $$k(n+2)^2-kn^2=k(n-2)^2$$
$$n^2+4n+4-n^2=n^2-4n+4$$
$$n^2=8n \Rightarrow n=8$$

The difference between $$\left ( n+2 \right )^{th}$$ Bohr radius and $$n^{th}$$ Bohr radius is equal to the $$\left ( n-2 \right )^{th}$$ Bhor radius. The value of $$n$$ is ?

Radius of $$nth$$ Bohr orbit is given by

$$r_n = (\dfrac{h^2 \epsilon_o}{\pi m e^2})n^2$$

Hence,

$$r_{n}\propto n^{2}$$
Thus we get $$r_{n+2}= k\left (n+2 \right )^{2}$$
and $$r_{n}=kn^{2}$$
and $$r_{n-2}= k\left (n-2 \right )^{2}$$
$$\therefore \ \ \left ( n+2 \right )^{2}-n^{2}=\left ( n-2 \right )^{2}$$
$$ \Rightarrow n=8$$

(a) Using Bohr's postulates, obtain the expression for total energy of the electron in the $${n}^{th}$$ orbit of hydrogen atom.
(b) What is the significance of negative sign in the expression for the energy?
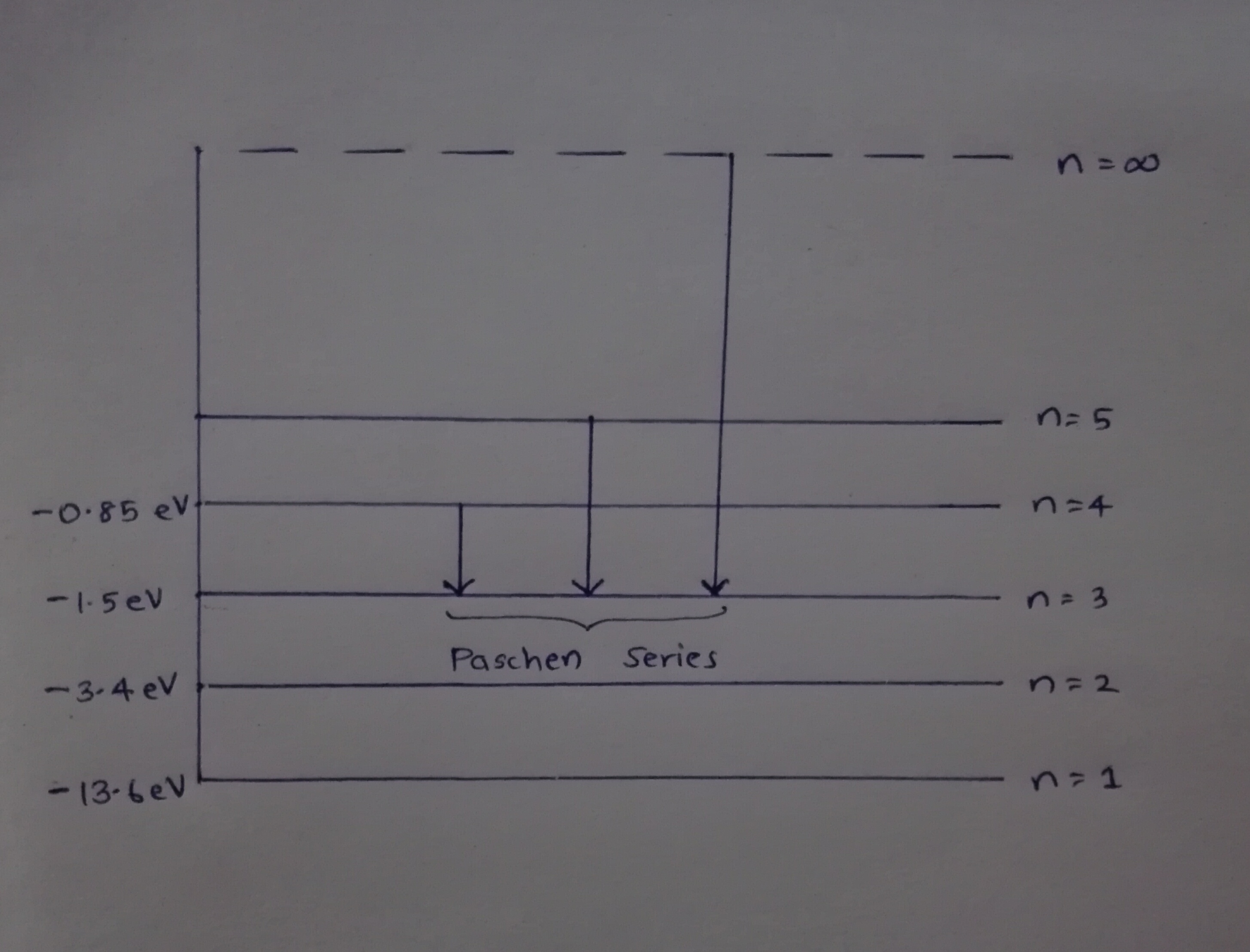
(c) Draw the energy level diagram showing how the line spectra corresponding to Paschen series occur due to transition between energy levels.

(a) According to Bohr's postulates, in a hydrogen atom, a single electron revolves around a nucleus of charge$$+e$$. For an electron moving with a uniform speed in a circular orbit on a given radius, the centripetal force is provided by the coulomb force of attraction between the electron and the nucleus.

Therefore,

$$\frac{mv^2}{r}=\frac{1(Ze)(e)}{4\pi \epsilon_0 r^2}$$

$$\implies$$ $$mv^2=\frac{1}{4\pi \epsilon_0 } \frac{Ze^2}{r}$$ ....(1)

So,

Kinetic Energy, $$ K.E=\frac12 mv^2$$

$$K.E=\frac{1}{4\pi \epsilon_0} \frac{Ze^2}{r}$$

Potential Energy is given by, $$P. E=\frac{1}{4\pi \epsilon_0} \frac{(Ze)(-e)}{r}$$

Therefore total energy is given by, $$E=K.E+P.E$$

$$E=\frac{1}{4 \pi \epsilon_0}\frac{Ze^2}{2r}+(-\frac{1}{4\pi \epsilon_0} \frac{Z e^2}{r})$$

$$E=-\frac{1}{4 \pi \epsilon_0} \frac{Ze^2}{2r}$$

For, $$n^{th}$$ orbit, $$E$$ can be written as $$E_n$$,

$$E_n=-\frac{1}{4\pi \epsilon_0} \frac{Ze^2}{2r_n}$$ ......(2)

Now, using Bohr's postulate for quantization of angular momentum, we have

$$mvr=\frac{nh}{2 \pi}$$

$$\implies$$ $$v= \frac{nh}{2 \pi mr}$$

Putting the values of $$v$$ in equation (1), we get

$$\frac{m}{r}$$ $$(\frac{nh}{2 \pi mr})^2=\frac{1}{4 \pi \epsilon_0} \frac{Ze^2}{r^2}$$

$$=-\frac{mZ^2 e^4}{8 \epsilon_0 h^2 n^2}$$

$$\implies$$ $$\frac{\epsilon_0 h^2 n^2}{\pi m Z e^2}$$

Now putting a]value of $$r_n$$ in equation (2), we get

$$E_n=-\frac{1}{4 \pi \epsilon_0} \frac{Ze^2}{2(\frac{\epsilon_0 h^2 n^2}{ \pi m})}$$

$$=-\frac{mZ^2e^4}{8 \epsilon_0 h^2 n^2}$$

$$\implies$$ $$E_n=-\frac{Z^2 Rhc}{n^2}$$, where $$R=\frac{m e^4}{8 \epsilon_0^2 c h^3}$$

where R is the Rydberg constant.

(b) Negative sign shows that electron remain bound with the nucleus.

$$\triangle E = \frac{me^4}{8 \epsilon_0^2 h^2} (\frac{1}{n_f^2}-\frac{1}{n_i^2})$$

The fraction occupied by nucleus in an atom is _________.

According to Rutherford, the fraction occupied by the nucleus of the atom is $$\dfrac{1}{10^{5}}$$. Which is a very small portion of the atom.

Define intensity of radiation on the basis of photon picture of light. Write its S.I. unit.

The intensity of radiation can be defined as the energy related with photons emitted from a unit surface area in unit time. Its S.I. units is watt per steradian. ($$W{sr}^{-1}$$)

Using Bohr's postulates of the atomic model, derive the expression for radius of n$$^{th}$$ electron orbit. Hence obtain the expression for Bohr's radius.

Centripetal force is provided by the electrostatic force of attraction.

$$\dfrac{mv^2}{r} = \dfrac{e^2}{4 \pi \varepsilon_o r^2}$$

From bohr's second postulate.

$$L_n = m v_n r_n = \dfrac{nh}{2 \pi} $$

Eliminating $$v_n$$ from above equations.

$$r_n = \dfrac{n^2 h^2\varepsilon_o}{\pi m e^2}$$

Bohr's radius is the radius of the innermost orbit. Hence, $$n =1$$

$$r_1 = \dfrac{h^2 \varepsilon_o}{\pi m e^2}$$

Rutherford's experiment of scattering of $$\alpha-$$particles showed for the first time that the atom has ________.

Answer: $$Nucleus$$

Based on his observations of $$\alpha-$$ray scattering experiment, Rutherford proved that the atom consists of small dense portion at the center, the nucleus, that is positively charged.

True or False
Rutherford's atomic model was successful in explaining stability of the atom.

The stability of the atom was explained by Bohr's atomic model.

Rutherford proposed an atomic model based on the alpha scattering experiment but it could not explain the stability of the atom.

The Bohr model consists of the following principles:

Electrons assume only certain orbits around the nucleus.
Each orbit has an energy associated with it. For example, the orbit closest to the nucleus has an energy $$E1$$, the next closest $$E2$$, and so on.
Light is emitted when an electron jumps from a higher orbit to a lower orbit and absorbed when it jumps from a lower to a higher orbit.
The energy and frequency of light emitted or absorbed is given by the difference between the two-orbit energies
With these conditions, Bohr was able to explain the stability of atoms as well as the emission spectrum of hydrogen.

Therefore, the given statement is false.

In Rutherford's $$\alpha$$-ray scattering experiment method:
(1) Some rays were completely deflected back.
(2) Most of the rays passed straight.
Give reasons for the above observations.

In Rutherford's $$\alpha $$-ray scattering experiment some of the rays were completely deflected due to the presence of an unknown mass in the center which was later discovered as the nucleus.

Most of the $$\alpha$$- particles passed straight through the foil without suffering any deflection. This shows that most of the space inside the atom is empty or hollow.

In a cathode ray tube, state:
(i) the purpose of covering cathode by thorium and carbon.
(ii) the purpose of the fluorescent screen.
(iii) how is it possible to increase the rate of emission of electrons.

(i) The purpose of covering cathode by thorium and carbon is to decrease the work function.

(ii) When electron beam on striking the screen gives a bright spot on it due to the fluorescence of the material coated on it.

(iii) On increasing the filament current, the rate of emission of electrons from the cathode will increase.

$$(i)$$ Why is a cathode ray tube evacuated to a low pressure?
$$(ii)$$ What happens if the negative potential is changed on a grid?

$$(i)$$ The cathode ray tube is evacuated to a low pressure so that the electrons can move without being obstructed by air molecules.
$$(ii)$$ The variation of the negative potential on the grid affects the number of electrons reaching the anode and striking the screen changed which changes the brightness of pattern on the screen.

Wavelengths of the first lines of the Lyman series, Paschen series and Balmer series in hydrogen spectrum are denoted by $$\lambda _L ,\lambda _P \,\, and \,\,\lambda _B$$ respectively. Arrange these wavelength in increasing order.

From the figure, we can write the wavelengths in increasing order as: $$\lambda _L <\lambda _B <\lambda _P$$

Explain emission and absorption spectra.

(i) Emission spectra: When the light emitted directly from a source is examined with a spectrometer, the emission spectrum is obtained. Every source has its own characteristics emission spectrum.
The emission spectrum is of three types. They are,
(a) Continuous spectrum, (b) Line spectrum and (c) Band spectrum.
(a) Continuous spectrum: It consists of unbroken luminous bands of all wavelengths containing all the colours from violet to red. These spectra depend only on the temperature of the source and is independent of the characteristic of the source.
Incandescent solids, liquids, carbon arc, electric filament lamps, etc., give continuous spectra.
(b) Line spectrum: Line spectra are sharp lines of definite wavelengths. It is the characteristic of the emitting substance. It is used to identify the gas.
Atoms in the gaseous state, i.e., free excited atoms emit line spectrum. The substance in atomic state such as sodium vapour lamp, mercury in mercury vapour lamp and gases in discharge tube give line spectra (Fig.)
(c) Band spectrum: It consists of number of bright bands with a sharp edge at one end but fading out at the other end.
Band spectra are obtained from molecules. It is the characteristics of the molecule. Calcium or barium salts in a bunsen flame and gases like carbondioxide, ammonia and nitrogen in molecular state in the discharge tube give band spectra. When the bands are examined with high resolving power spectrometer, each band is found to be made of a large number of fine lines, very close to each other at the sharp edge but spaced out at the other end. Using band spectra the molecular structure of the substance can be studied.

(ii) Absorption spectra: When the light emitted from a source is made to pass through an absorbing material and then examined with a spectrometer, the obtained spectrum is called absorption spectrum. It is characteristic of the absorbing substance.
Absorption spectra is also of three types. They are,
(a) Continuous absorption spectrum, (b) Line absorption spectrum and (c) Band absorption spectrum.
(a) Continuous absorption spectrum: A pure green glass plate when placed in the path of white light, absorbs everything except green and gives continuous absorption spectrum.
(b) Line absorption spectrum: When light from the carbon are is made to pass through sodium vapour and then examined by a spectrometer, a continuous spectrum of carbon arc with two dark lines in the yellow region is obtained as shown in figure.
(c) Band absorption spectrum: If white light is allowed to pass through iodine vapour or dilute solution of blood or chlorophyll or through certain solutions of organic and inorganic compounds, dark bands on continuous bright background are obtained. The band absorption spectra are used for making dyes.

Explain the different types of spectral series in a hydrogen atom.

According to Bohr, when an electron from its initial stationary orbit with $$n=n_i$$ jumps to another (lower) stationary orbit with $$n=n_f$$, it emits energy equal to the difference between the energy of the two stationary orbits in the form of small packets of light known as photons. For every transition of the electron, there is a line in the spectrum and there are different types of spectral series formed. He gave the spectrum of Hydrogen ion which has one electron only, so this spectrum cannot be applied for atoms with more than one electron. The following are the series in the hydrogen spectrum: –

a) Lyman Series: – when the electron jumps from any higher stationary orbit to first stationary orbit, the spectral lines falls in the Lyman series. For Lyman series, $$n_i=1$$.

Now putting $$n_f=1$$ in the relation given by Bohr,$$w=R_h(\dfrac{1}{1^2}-\dfrac{1}{n_i^2})$$, $$n_i=2,3,4....$$

b) Balmer Series: – when the electron jumps from any higher stationary orbit to the second stationary orbit with $$n_f=2$$, the spectral lines falls in the Balmer Series. Here, $$n_i=2$$

$$w=R_h(\dfrac{1}{2^2}-\dfrac{1}{n_i^2})$$, $$n_i=3,4,5....$$

c) Paschen Series: – when the electron falls from any higher stationary orbit to third stationary orbit with $$n=3$$, the spectral lines falls in the Paschen Series. Here $$n_f=3$$

$$w=R_h(\dfrac{1}{3^2}-\dfrac{1}{n_i^2})$$, $$n_i=4,5,6....$$

d) Brackett Series: – when the electron jumps from any higher stationary orbit to fourth stationary orbit with n=4, the spectral lines fall in Bracket Series. Here, $$n_f=4$$

$$w=R_h(\dfrac{1}{4^2}-\dfrac{1}{n_i^2})$$, $$n_i=5,6,7....$$

e) Pfund Series: – when the electron from any higher stationary orbit jumps to fifth stationary orbit with n=5, the spectral lines falls in Pfund Series. Here, $$n_f=5$$

$$w=R_h(\dfrac{1}{5^2}-\dfrac{1}{n_i^2})$$, $$n_i=6,7,8....$$

The short wavelength limit for the Lyman series of the hydrogen spectrum is $$913.4\overset{o}{A}$$. Calculate the short wavelength limit for Balmer series of the hydrogen spectrum.

Rydberg formula for wavelength for the hydrogen spectrum is given by

$$\dfrac{1}{\lambda}=R[1/n_1^2-1/n_2^2]$$

For short wavelength of Lyman series, $$\dfrac{1}{913.4}=R[1/1^2-1/\infty^2]$$

or $$R=(1/913.4)A^{-1}$$

For short limit of wavelength for Balmer series, $$\dfrac{1}{\lambda_b}=R[1/2^2-1/\infty^2]$$

or $$\lambda_b=4/R=4(913.4)=3653.6 A^o=365.36 nm$$

Calculate the shortest and longest wavelengths of Balmer series of hydrogen atom. Given $$R=1.097\times 10^7m^{-1}$$.

Wavelength of photon emitted due to transition in H-atom $$\dfrac{1}{\lambda} = R \bigg( \dfrac{1}{n_1^2} - \dfrac{1}{n_2^2} \bigg)$$

Shortest wavelength is emitted in Balmer series if the transition of electron takes place from $$n_2 = \infty$$ to $$n_1 = 2$$.

$$\therefore$$ Shortest wavelength in Balmer series $$\dfrac{1}{\lambda_s} = R \bigg( \dfrac{1}{2^2} - \dfrac{1}{\infty} \bigg)$$

Or $$\lambda_s = \dfrac{4}{R}$$

$$\implies$$ $$\lambda_s = \dfrac{4}{1.097 \times 10^{7}} = 364.6 nm$$

Longest wavelength is emitted in Balmer series if the transition of electron takes place from $$n_2 = 3$$ to $$n_1 = 2$$.

$$\therefore$$ Longest wavelength in Balmer series $$\dfrac{1}{\lambda_L} = R \bigg( \dfrac{1}{2^2} - \dfrac{1}{3^2} \bigg)$$

Or $$\lambda_s = \dfrac{36}{5R}$$

$$\implies$$ $$\lambda_s = \dfrac{36}{5\times 1.097 \times 10^{7}} = 656.3 nm$$

Write the different types of Hydrogen Spectral series. The Lyman series of Hydrogen spectrum lies in the ultraviolet region. Why?

Hydrogen spectral lines:

1. Lyman series ($$n=1$$)

2. Balmer series ($$n=2$$)

3. Paschen series ($$n=3$$)

4. Brackett series ($$n=4$$)

5. Pfund series ($$n=5$$)

Where $$n$$ corresponds to final energy level in the following equation:

$$\dfrac{1}{\lambda}=RZ(\dfrac{1}{n^2} - \dfrac{1}{m^2})$$

where, $$\lambda$$ is wavelength

$$R$$ is Rydberg constant

$$Z$$ is atomic number

$$m$$ is initial energy level

From the above equation, in Lyman series longest wavelength corresponding to $$m=2$$ is 121.57nm and shortest wavelength corresponding to $$m=\infty$$ is 91.18nm. Therefore, the entire range of Lyman series lies in ultraviolet region.

Name the phenomenon which shows the quantum nature of electromagnetic radiation.

Photoelectric effect shows the quantum nature of electromagnetic radiation.

What is the nuclear radius of $$_{ 4 }^{ }{ { Be }^{ 8 } }$$ nucleus?

Radius of a nucleus $$=r=r_0(A)^\dfrac{1}{3}$$

Here, $$A=8$$ and $$r_0=1.25\times 10^{-15}m$$

$$\therefore r=1.25\times 10^{-15}\times (8)^\dfrac{1}{3}=2.5\times 10^{-15}m$$

By assuming Bohr's postulates derive an expression for radius of $$n^{th}$$ orbit of electron, revolving round the nucleus of hydrogen atom.

Let the electron of mass $$m$$ revolves around a nucleus (H-atom) in an orbit of radius $$r_n$$ with linear velocity $$v_n$$.

As the electron revolves in an stationary orbit, thus centrifugal force acting on the electron is balanced by the coulombic force i.e. $$F_{centrifugal} = F_{coulomb}$$

$$\therefore$$ $$\dfrac{mv_n^2}{r_n} = \dfrac{ke^2}{r_n^2}$$ where $$k = \dfrac{1}{4\pi \epsilon_o} = 9\times 10^9$$

$$\implies$$ $$r_n = \dfrac{ke^2}{mv_n^2}$$

Also we use $$mv_nr_n = \dfrac{nh}{2\pi}$$

Eliminating $$v_n$$ from both equations, we get $$r_n = \dfrac{ke^2}{m}.\dfrac{4\pi^2m^2 r_n^2}{n^2 h^2}$$

$$\implies$$ $$r_n = \dfrac{h^2 n^2}{4\pi^2 m ke^2} $$

Putting $$m = 9.1\times 10^{-31} kg$$, $$h = 6.626 \times 10^{-34} Js$$ and $$e = 1.6\times 10^{-19} C$$

We get radius of $$n^{th}$$ Bohr orbit $$r_n = 0.529$$ $$ n^2$$ $$A^o$$

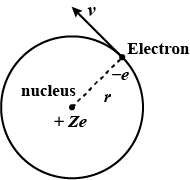
If the Bohr model of hydrogen atom, the electron is treated as a particle going in a circle with the centre at the proton. The proton itself is assumed to be fixed in an inertial frame. The centripetal force is provided by the Coloumb attraction. In the ground state, the electron goes round the proton in a circle of radius $$5.3 \times 10^{-11} m $$ . Find the speed of the electron in the ground state. Mass of the electron $$ = 9.1 \times 10^{-31} kg $$ and charge of the electron $$ = 1.6 \times 10^{-19} C $$

Electron revolves around the proton in a circle having proton at the center.

Centripetal force is provided by coulomb attraction

$$r=5.3\times { 10 }^{ -11 }m$$

$$m=$$ mass of electron $$=9.1\times { 10 }^{ -3 }kg$$

charge of electron $$=1.6\times { 10 }^{ -19 }C$$

For the particular charge particle, the centripetal force is balanced by the electrostatic force.

Centripetal force = Electrostatic force

$$\cfrac { m{ v }^{ 2 } }{ r } =k\cfrac { { q }^{ 2 } }{ { r }^{ 2 } } $$

$$\Rightarrow { v }^{ 2 }=\cfrac { k{ q }^{ 2 } }{ rm } =\cfrac { 9\times { 10 }^{ 9 }\times 1.6\times 1.6\times { 10 }^{ -38 } }{ 5.3\times { 10 }^{ -11 }\times 9.1\times { 10 }^{ -31 } }$$

$$ =\cfrac { 23.04 }{ 48.23 } \times { 10 }^{ 13 }$$

$$\Rightarrow { v }^{ 2 }=4.077\times { 10 }^{ 13 }=4.7\times { 10 }^{ 12 }$$

$$\Rightarrow v=\sqrt { 4.7\times { 10 }^{ 12 } } =2.2\times { 10 }^{ 6 }m/sec$$

The terminology of different parts of the electromagnetic spectrum is given in the text. Use the formula $$E=hv$$ (for energy of a quantum of radiation photon) and obtain the photon energy in units of $$eV$$ for different parts of the electromagnetic spectrum. In what ways are the different scales of photon energies that you obtain related to the sources of electromagnetic radiation.?

Energy of photon, $$E=hv$$

This implies, $$E = h\dfrac{c}{\lambda }$$

Where,

$$\begin{array}{l} h=6.62\times { 10^{ -34 } }js \\ c=3\times { 10^{ 8 } }m{ s^{ -1 } } \end{array}$$

If wave length $$\lambda $$ is in meter and energy is in joule then, we will divide $$E$$ by $$1.6 \times {10^{ - 19}}$$ to convert into $$eV$$ (Electron volt).

$$\therefore E = \dfrac{{hc}}{{\lambda \times 1.6 \times {{10}^{ - 19}}}}eV$$

$$(1)$$ For $$y-rays$$ wave length ranges from $${10^{ - 10}}m$$ to less that $${10^{ - 14}}m$$

Therefore,

$$\begin{array}{l} Energy=\dfrac { { 6.62\times { { 10 }^{ -34 } }\times 3\times { { 10 }^{ 8 } } } }{ { { { 10 }^{ -10 } }\times 1.6\times { { 10 }^{ -19 } } } } eV \\ =12.4\times { 10^{ 3 } }eV\approx { 10^{ 4 } }eV \end{array}$$

Thus,

$$\lambda = {10^{ - 10}}m,\,\,energy = {10^4}eV$$ and

$$\lambda = {10^{ - 14}}m,\,\,energy = {10^8}eV$$

Energy of $$y-rays$$ ranges between $${10^4}$$ to $${10^8}$$ $$eV$$

$$(2)$$ For $$X-rays$$ wave length ranges from $${10^{ - 8}}m$$ to $${10^{ - 7}}m$$

For $$\lambda ={ 10^{ - } }^{ 8 }$$

Therefore,

$$\begin{array}{l} Energy=\dfrac { { 6.62\times { { 10 }^{ -34 } }\times 3\times { { 10 }^{ 8 } } } }{ { { { 10 }^{ -8 } }\times 1.6\times { { 10 }^{ -19 } } } } eV \\ =12.4\approx { 10^{ 2 } }eV \end{array}$$

$$\lambda ={ 10^{ - } }^{ 13 }m,\, \, energy={ 10^{ 7 } }eV$$

$$(3)$$ For violet radiation $$\lambda $$ ranges from $$4 \times {10^{ - 7}}$$ to $$6 \times {10^{ - 10}}$$

Therefore,

for $$\lambda =4\times { 10^{ -7 } }$$

$$\begin{array}{l} Energy=\dfrac { { 6.62\times { { 10 }^{ -34 } }\times 3\times { { 10 }^{ 8 } } } }{ { 4\times { { 10 }^{ -7 } }\times 1.6\times { { 10 }^{ -19 } } } } eV \\ =3.1eV\approx { 10^{ 10 } }eV \end{array}$$

$$\lambda = 6 \times {10^{ - 10}}m$$ , Energy $$ = {10^3}eV$$

Energy of ultraviolet radiation vary between $${10^{10}}\,to\,{10^3}eV$$.

$$(4)$$ For visible radiations wave length range from $$4 \times {10^{ - 7}}m\,\,to\,7 \times {10^{ - 7}}m$$

Therefore,

For $$\lambda = 4 \times {10^{ - 7}}m$$, and Energy $$ = {10^{10}}eV$$

$$\begin{array}{l} Energy=\dfrac { { 6.62\times { { 10 }^{ -34 } }\times 3\times { { 10 }^{ 8 } } } }{ { 7\times { { 10 }^{ -7 } }\times 1.6\times { { 10 }^{ -19 } } } } eV \\ =1.77eV\approx { 10^{ 0 } }eV \end{array}$$

$$(5)$$ For infrared radiation $$\lambda $$ range from $$7 \times {10^{ - 7}}m\,\,to\,7 \times {10^{ - 14}}m$$

Therefore,

$$\lambda = 7 \times {10^{ - 7}},\,energy = {10^0}eV$$

For

$$\lambda = 7 \times {10^{ - 4}},\,energy = \dfrac{1}{{1000}}times$$

the other order of $${10^{ - 3}}eV$$

$$(6)$$ For micro waves $$\lambda $$ ranges from $$1 mm$$ to $$0.3 m$$

For $$\lambda = 1mm\,\,or\,{10^{ - 3}}$$

energy is equal to

$$\begin{array}{l} Energy=\dfrac { { 6.62\times { { 10 }^{ -34 } }\times 3\times { { 10 }^{ 8 } } } }{ { { { 10 }^{ -3 } }\times 1.6\times { { 10 }^{ -19 } } } } eV \\ =1.24\times { 10^{ -3 } }eV\approx { 10^{ -3 } }eV \end{array}$$

For

$$\lambda = 0.3m,\,Energy = 4.1 \times {10^{ - 6}}eV \approx {10^{ - 6}}eV.$$

$$(7)$$ For Radio waves $$\lambda $$ ranges from $$1m$$ to few $$km$$

For $$\lambda = 1m$$

Energy is equal to

$$\begin{array}{l} =\dfrac { { 6.62\times { { 10 }^{ -34 } }\times 3\times { { 10 }^{ 8 } } } }{ { { { 10 }^{ 0 } }\times 1.6\times { { 10 }^{ -19 } } } } eV \\ =1.24\times { 10^{ -6 } }eV\approx { 10^{ -6 } }eV \end{array}$$

Energy for $$\lambda $$ of the order of few $$km\approx { 10^{ -6 } }eV$$

The Energy of a photon that a source produces indicates the spacing of relevant energy levels of the source

Find the shortest wavelength in Paschen series if, the longest wavelength in Balmer series is 6563 $$A^0$$

Given: Longest wavelength of Balmer series $$= 6563A^o$$

To find: Shortest wavelength of Paschen series $$=?$$

Soln:

We know that

$$\dfrac{1}{\lambda} = RZ^2\left(\dfrac{1}{n^2} - \dfrac{1}{m^2}\right)$$

For Balmer series

$$\dfrac{1}{\lambda} = RZ^2\left(\dfrac{1}{2^2} - \dfrac{1}{3^2}\right) \Rightarrow\dfrac{1}{6563} = RZ^2\left(\dfrac{1}{4} - \dfrac{1}{9}\right)$$

$$\Rightarrow \dfrac{1}{6563}=RZ^2 \left(\dfrac{9-4}{36}\right) $$

$$\Rightarrow RZ^2 = \dfrac{36}{5\times 6563}$$ ...(1)

For paschen series

Shortest wavelength when $$(m=\infty)$$

$$\dfrac{1}{\lambda_{min}} = RZ^2\left(\dfrac{1}{3^2} - \dfrac{1}{\infty}\right) $$

$$\dfrac{1}{\lambda_{min}} = \dfrac{36}{5\times 6563} \times\dfrac{1}{9}$$

$$\lambda_{min} = 8203.75A^o$$

Explain Rutherford's $$\alpha$$-ray scattering experiment with a neat diagram.

Rutherford passed beams of alpha particles through a thin gold foil and noted how the alpha particles scattered from the foil.

Observations of Rutherford's alpha ray scattering experiment:

1. Most of the $$\alpha$$-particles passed straight through the gold foil without any deviation.

2. Some of the $$\alpha$$-particles were deflected by the foil by some angles.

3. Interestingly one out of every 12,000 alpha particles appeared to rebound.

Conclusion of Rutherford's scattering experiment:

1. Most of the space inside the atom is empty because most of the $$\alpha$$-particles passed through the gold foil without getting deflected.

2. Very few particles were deflected from their path, indicating that the positive charge of the atom occupies very little space.

3. A very small fraction of $$\alpha$$-particles were deflected by very large angles, indicating that all the positive charge and mass of the gold atom were concentrated in a very small volume within the atom.

961952_1023597_ans_b7e25ab2bd2f4d6aa7fec0d04b73288c.png

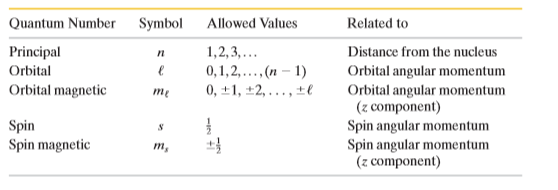
Explain the significance of quantum number?

Quantum numbers are important because they can be used to determine the electron configuration of an atom and the probable location of the atom's electrons. Quantum numbers are also used to determine other characteristics of atoms, such as ionization energy and the atomic radius.

What are the postulates of Rutherford? What are his drawbacks ?

$$\text{Postulates of Rutherford's atomic model:}$$

(1) +ve charge is concentrated in the center of the atom called nucleus.

(2) Electron revolved around the nucleus in circular paths called orbits.

(3) The nucleus is much smaller in size than the atom.

$$\text{Drawbacks of Rutherford's atomic model:}$$

(1) The orbital of the electron is not expected to be stable.

(2) According to Rutherford's model, electrons while moving in their orbit would give up energy. This would make them slow down gradually and move towards the nucleus. Electron will follow a spiral path and then fall into the nucleus. Ultimately the atom would collapse. But in reality the atom is stable.

Using Bohr's postulates, derive the expression for the total energy of the electron revolving in nth orbit of hydrogen atom. Find the wavelength of $$ H_{\alpha} $$ line, given the value of Rybderg constant, $$ R = 1.1 \times 10^7m^{-1} $$

We know,

$$mvr=\dfrac{nh}{2n}$$ (i) [Bhor's theorem]

Also,

$$\dfrac{mv^{2}}{r}=\dfrac{k Ze^{2}}{r^{2}}$$ - (ii)

We know in circular motion about electrostatic field

$$PE=-2KE$$

$$TE=PE+KE=-2KE+KE$$

$$TE=-KE$$

$$KE=\dfrac{mv^{2}}{2}$$

From (i) and (ii), we have

$$r=\dfrac{nh}{2nmv}$$

So, $$mv^{2}=\dfrac{k ze^{2}}{r}=\dfrac{k ze^{2}}{\dfrac{nh}{2nmv}}$$

$$\Rightarrow mv^{2}=\dfrac{2nmv kze^{2}}{nh}$$

$$\Rightarrow v=\dfrac{2nkze^{2}}{nh}$$

So, $$\dfrac{mv^{2}}{2}=\dfrac{m}{2}\left[\dfrac{2nkze^{2}}{nh}\right]^{2}$$

$$\dfrac{mv^{2}}{2}=\dfrac{2n^{2}k^{2}z^{2}e^{2}m}{n^{2}h^{2}}$$

$$\dfrac{mv^{2}}{2}=\left[\dfrac{2n^{2}k^{2}e^{4}m}{h^{2}}\right]\left(\dfrac{z^{2}}{n^{2}}\right)$$

So, $$TE=-KE=\dfrac{-mv^{2}}{2}=\left[\dfrac{-2n^{2}k^{2}e^{4}m}{h^{2}}\right]\left(\dfrac{z^{2}}{n^{2}}\right)$$

We know,

For $$H\alpha$$ line,

$$n_{1}=2$$ and $$n_{2}=3$$

$$\dfrac{1}{\lambda}=R(z^{2})\left[\dfrac{1}{n_{1}^{2}}-\dfrac{1}{n_{2}^{2}}\right]$$

$$\dfrac{1}{\lambda}=(1.1\times 10^{7})(1)\left[\dfrac{1}{4}-\dfrac{1}{9}\right]$$

$$\dfrac{1}{\lambda}=0.513\times 10^{7}=1.53\times 10^{8}m^{-1}$$

$$\lambda =\dfrac{1}{153\times 10^{8}}=65.35\ A$$

A hydrogen atom in a state having a binding energy of $$0.85\ eV$$ makes transition to a state with excitation energy $$10.2\ eV$$
Find the wavelength of the emitted radiation.

From the energy data we see that the $$H$$ atom transists from binding energy of $$0.85$$ ev to exitation energy of $$10.2$$ ev $$=$$ Binding Energy of $$–3.4$$ ev.

So, $$n = 4$$ to $$n = 2$$

We know that $$\dfrac{1}{\lambda}=1.097\times {10}^{7}\left(\dfrac{1}{4}-\dfrac{1}{16}\right)$$

Wavelength of the emitted radiation$$=\lambda=\dfrac{16}{1.097\times 3\times {10}^{7}}=4.8617\times{10}^{-7}=487$$nm

An electron in hydrogen atom stays in 2nd orbit for $$10 ^ { - 8 }$$ . How many, revolutions will it make till it jumps to the ground state ?

As the $$e^{\circleddash}$$ has to jump from $$2^{nd}$$ orbit to $$1^{st}$$ orbit

$$v=\dfrac{\Delta E}{h}=\dfrac{E_2-E_1}{h}$$

$$v=\dfrac{(-3.4)-(-13.6)}{6.63\times 10^{-34}}$$

$$v=\dfrac{10.2\times 1.6\times 10^{-19}}{6.63\times 10^{-34}}$$

$$v=2.46\times 10^{15}$$Hz

$$\Rightarrow$$ It means that in $$1$$ sec $$e^{\circleddash}$$ completes $$2.46\times 10^{15}$$ recolutions.

Then, in $$10^{-8}s$$ the electron will undergo

$$2.46\times 10^{15}\times 10^{-8}$$ revolutions

$$2.46\times 10^7$$ revolutions.

1205990_1400029_ans_f6f640e52f66462b93f3262d871d2150.png

An electron in a multielectron atom is known to have the quantum number $$l = 3$$. What are its possible $$n, m_l, and m_s $$ quantum numbers?

1770971_1778646_ans_c09082820e3b45779e7339576eedc1b7.jpg

For a helium atom in its ground state, what are quantum numbers $$ ( n,\ l,\ m_l,\ $$ and $$m_s ) $$ for the (a) spin-up electron and (b) spin-down electron?

1753609_1778333_ans_b3262cb90d954c23807b2da93003d79b.jpg

A recently named element is darmstadtium (Ds), which has 110 electrons. Assume that you can put the 110 electrons into the atomic shells one by one and can neglect any electron electron interaction. With the atom in ground state, what is the spectroscopic notation for the quantum number l for the last electron?

1753102_1778332_ans_096e53891d54416da842a1e0a1784644.jpg

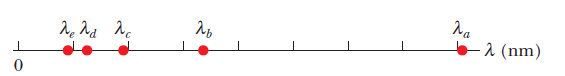
An electron is trapped in a one-dimensional infinite well
and is in its first excited state. Fig. indicates the five longest
wavelengths of light that the electron could absorb in transitions
from this initial state via a single photon absorption: $$\lambda_a=80.78\ nm,\lambda_b=33.66\ nm, \lambda_c=19.23\ nm, \lambda_d=12.62\ nm, $$ and $$\lambda_e=8.98\ nm$$.
What is the width of the potential well?

The frequency of the light that will excite the electron from the state with quantum
number $$n_i$$ to the state with quantum number $$n_f$$ is
$$f=\dfrac{\triangle E}{h}=\dfrac{h}{8mL^2}(n_f^2 - n_i^2)$$
and the wavelength of the light is $$\lambda =\dfrac{c}{f}=\dfrac{8mL^2c}{h((n_f^2 - n_i^2))}$$
The width of the well is$$L=\sqrt{\dfrac{\lambda hc(n_f^2 - n_i^2)}{8mc^2}}$$
The longest wavelength shown in Fig. is $$\lambda = 80.78\ nm$$, which corresponds to ajump from $$n_i=2$$ to $$n_f=3$$. Thus, the width of the well is
$$L=\sqrt {\dfrac{\lambda hc(n_f^2 - n_i^2)}{8mc^2} } = \sqrt{\dfrac{(80.78\ nm)(1240\ eV.nm)(3^2-2^2)}{8(511 \times 10^3\ eV)}}=0.350nm=350\ pm$$

Two of the three electrons in a lithium atom have quantum numbers $$ (n, l, m_l, m_s )$$ of $$(1,0,0, \frac {+1}{2})$$and $$(1,0,0, \frac {-1}{2}) $$. What quantum numbers are possible for the third electron if the atom is (a) in the ground state and (b) in the first excited state?

1755703_1778330_ans_f6209412c5f24d4381c52528f695f04f.jpg

Show that the number of states with the same quantum number n is $$2n^2$$.

1758025_1778331_ans_e3635a22cf1343288b4f28832be6b553.jpg

How many electron State are there in a shell defined by the quantum number $$n= 5$$?

For a given quantum number $$n$$ there are n possible values of $$l$$ , ranging from $$0$$ to $$n −1$$.
For each $$l$$ the number of possible electron states is $$ N_l = 2(2 l + 1)$$. Thus the total
number of possible electron states for a given $$n$$ is .
$$N_n = \overset{n-1}{\underset{l=0}{\Sigma}} \ N_l= 2 \overset{n-1}{\underset{l=0}{\Sigma}} \ (2l+1)= 2n^2 $$
Thus, in this problem, the total number of electron states is $$ N_n=2n^2=2(5)^2=50 $$

A narrow beam of alpha particles with kinetic energy $$1.0\ MeV $$ falls normally on a platinum foil $$1.0\ \mu m $$ thick. The scattered particles are observed at an angle of $$60^{\circ}$$ to the incident beam direction by means of a counter with a circular inlet area $$1.0\ cm^2 $$ located at the distance $$10\ cm$$ from the scattering section of the foil. What fraction of scattered alpha particles reaches the counter inlet ?

From the formula (6.1 b) of the book
$$ \dfrac{dN}{N} = n \left ( \dfrac{Ze^2}{(4 \pi \varepsilon_0) 2T} \right )^2 \dfrac{d \Omega}{\sin^{4} \dfrac{\theta}{2}} $$

We have put $$ q_{1} = 2e , q_{2} = -\!\!\!\! Z \, e $$ here. Also $$n$$ = no. of Pt nuclei in the foil per unit area
$$ = (A\ t\ \rho) \cdot \dfrac{N_A}{A_{Pt}} \cdot \dfrac{1}{A} = \dfrac{N_{A} \rho\ t}{A_{Pt}} $$
$$ \downarrow $$ $$ \downarrow $$
mass of no. of nuclei
the foil per unit mass

Using the values $$A_{Pt} = 195 , \rho = 21.5 \times 10^{3} \, kg/m^{3} $$
$$N_{A} = 6.023 \times 10^{26} $$ / kilo mole
We get $$ n = 6.641 \times 10^{22} \, per \, m^{2} $$
Also $$ d \Omega = \dfrac{dS_n}{r^2} = 10^{-2} Sr $$
Substituting we get $$ \dfrac{dN}{N} = 3.36 \times 10^{-5} $$

The gravitational attraction between electron and proton in a hydrogen atom is weaker than the coulomb attraction by a factor of about $$10^{-10}$$. An alternative way of looking at this fact is to estimate the radius of the first Bohr orbit of a hydrogen atom if the electron and proton were bound by gravitational attraction. You will find the answer interesting.

Let $$m$$ and $$m$$ be the masses of electron and proton respectively. If the elctron and proton were bound only by gravitational attraction, then
$$\dfrac{m_{e}v^{2}}{r}=\dfrac{G.m_{e}m_{p}}{r^{2}}$$
Or, $$v=\sqrt{\frac{G.m_{p}}{r}}$$
According to Bihr's quantistion condition, angular momentum of the electron.
$$m_{e}vr=n\dfrac{h}{2\pi}$$
$$\Rightarrow m_{e}\times\sqrt{\dfrac{G.m_{p}}{r}}\times r=n\dfrac{h}{2\pi}$$
$$\Rightarrow r=\dfrac{n^{2}h^{2}}{4\pi ^{2}Gm_{e}^{2}m_{p}}$$
Therefore, radius of first Bohr orbit of the $$H$$ atom,
$$r_{1}=\dfrac{1^{2}\times(6.62\times10^{-34})^{2}}{4\pi ^{2}\times6.67\times10^{-11}(9.1\times 10^{-31})^{2}\times1.67\times 10^{-27}}$$
$$=1.2\times10^{29}m$$.

Answer the following question, which help you understand the difference between Thomson's model and Rutherford's model better.
Is the probability of backward scattering (i.e, scattering of $$\alpha$$-particles at angles greater than $$90^{0}$$) predicted by Thomson's model much less, about the same, or much greater than that predicted by Rutherford's model?

Thomson's model predicts the backward scattering of a $$\alpha$$-particles much less than that predicted by Rutherford's model. The reason is that in Thomson's model, positive charge is uniformly distributed over whole of the nucleus instead of being concentrated in a small part.

The first line of the sharp series of atomic cesium is a doublet with wavelengths $$1358.8$$ and $$1469.5\,nm$$. Find the frequency intervals (in rad/s units) between the components of the sequent lines of that series.

1880404_1855500_ans_0ed1ec68f053423dacd2a30ec4f82fcb.jpeg

Find the effective cross section of a uranium nucleus corresponding to the scattering of alpha particles with kinetic energy $$T = 1.5\ MeV$$ through the angles exceeding $$ \theta_{0} = 60^{\circ} $$.

From the Rutherford scattering formula
$$ \dfrac{d \sigma}{d \Omega} = \left ( \dfrac{Ze^{2}}{(4 \pi \varepsilon_{0}) 2T} \right )^{2} \dfrac{1}{\sin^{4} \dfrac{\theta}{2}} $$

or $$ d \sigma = \left ( \dfrac{Ze^{2}}{(4 \pi \varepsilon_{0}) 2T} \right )^{2} \dfrac{2 \pi \sin \theta d \theta}{\sin^{4} \dfrac{\theta}{2}} $$

$$ = \left ( \dfrac{Ze^{2}}{(4 \pi \varepsilon_{0}) T} \right )^{2} \pi \dfrac{\cos \dfrac{\theta}{2} d \theta}{\sin^{3} \dfrac{\theta}{2}} $$

Then integrating from $$ \theta = \theta_{0} $$ to $$ \theta = \pi $$ we get required cross section

$$\displaystyle \Delta \sigma = \left ( \dfrac{Ze^{2}}{(4 \pi \varepsilon_{0})T} \right )^{2} \pi \int_{\theta_{0}}^{\pi} \dfrac{\cos \dfrac{\theta}{2} d \theta}{\sin^{3} \dfrac{\theta}{2}} $$

$$ = \left (\dfrac{Ze^{2}}{(4 \pi \varepsilon_{0}) 2T} \right )^{2} \cot^{2} \dfrac{\theta_{0}}{2} $$.

For $$U$$ nucleus $$Z = 92 $$ and we get on putting the values
$$ \Delta \sigma = 737 b = 0.737 \, kb$$
$$(1b = 1 \, bar = 10^{-28} m^{2}) $$.

A narrow beam of alpha particles with kinetic energy $$T = 0.50\ MeV $$ falls normally on a golden foil whose mass thickness is $$ \rho d = 1.5 mg/cm2$$. The beam intensity is $$I_{0} = 5.0 \times 10^{5} $$ particles per second. Find the number of alpha particles scattered by the foil during a time interval $$ \tau = 30\ min$$ into the angular interval
(a) $$59-61^{\circ} $$ ; (b) over $$ \theta_{0} = 60^{\circ} $$.

we known that
$$dN = I_{0} \tau \dfrac{\rho \cdot d \cdot N_{A}}{A_{Au}} \left ( \dfrac{Z\ e^{2}}{(4 \pi \varepsilon_{0}) 2 T} \right )^{2} \dfrac{2 \pi \, \sin \theta \, d \theta}{\sin^{4} \dfrac{\theta}{2}}$$

(we have used $$ d \Omega = 2 \pi \sin \theta d\ \theta $$ and $$ N = I_{0}I $$)
From the data
$$ d \theta = 2^{\circ} = \dfrac{2}{57.3} $$ radian
Also $$ Z_{Au} = 79 , A_{Au} = 197 $$. Putting the values we get
$$dN = 1.63 \times 10^{6} $$

(b) This number is
$$\displaystyle N(\theta_{0}) = I_{0} \tau \left ( \dfrac{\rho\ d N_{A}}{A_{Au}} \right ) \left ( \dfrac{Ze^{2}}{(4 \pi \varepsilon_{0})2T} \right )^{2} \, 4 \pi \int_{\theta_{0}}^{\pi} \dfrac{\cos \dfrac{\theta}{2} d \theta}{\sin^{3} \dfrac{\theta}{2}} $$

The integral is
$$ \displaystyle 2 \int_{\sin \dfrac{\theta_{0}}{2}}^{1} \dfrac{dx}{x^{2}} = \dfrac{1}{2} \left [\dfrac{-1}{2x^{2}} \right ]^{1}_{\sin \dfrac{\theta_{0}}{2}} = \cot^{2} \dfrac{\theta_{0}}{2} $$

Thus $$N(\theta_{0}) = \pi\ n\ d \left (\dfrac{Ze^{2}}{(4 \pi \varepsilon_{0}) T} \right )^{2} I_{0} \tau \cot^{2} \dfrac{\theta_{0}}{2} $$

where $$n$$ is the concentration of nuclei in the foil $$ (n = \rho N_{1}/A_{Au}) $$
Substitution gives
$$N(\theta_{0}) = 2.02 \times^{7} $$

A narrow beam of alpha particles with kinetic energy $$T = 0.50\ MeV$$ and intensity $$I = 5.0 \times 10^5 $$ particles per second falls normally on a golden foil. Find the thickness of the foil if at a distance $$r = 15\ cm $$ from a scattering section of that foil the flux density of scattered particles at the angle $$\theta = 60^{\circ} $$ to the incident beam is equal to $$J = 40$$ particles/$$(cm^2 \cdot s)$$.

A scattered flux density of $$J$$ (particles per unit area per second) equals $$ \dfrac{J}{\dfrac{1}{r^2}} = r^2 J $$ particles scattered per unit time per steradian in the given direction. Let n = concentration of the gold nuclei in the foil. Then

$$ n = \dfrac{N_{A} \rho}{A_{Au}} $$
and the number of $$Au$$ nuclei per unit area of the foil is$$nd$$ where $$d$$ = thickness of the foil.
Then from Eqn. (6.16) (note that $$ n \rightarrow nd$$ here)

$$r^{2} J = dN = nd I \left ( \dfrac{Z e^{2}}{(4 \pi \varepsilon_{0}) 2T} \right )^2 cosec^{4} \dfrac{\theta}{2} $$

Here $$I$$ is the number of $$ \alpha $$ falling on the foil per second
Hence $$ d = \dfrac{4 T^{2} r^{2} J}{nI \left ( \dfrac{Ze^{2}}{4 \pi \varepsilon_{0}} \right )^2} \sin^{4} \dfrac{\theta}{2} $$

Using $$ Z = 79 , A_{Au} = 196 , \rho = 19.3 \times 10^{3} kg/m^{3} N_{A} = 6.023 \times 10^{26} $$ / kilo mole and other data from the problem we get

$$ d = 1.47 \mu m $$

A narrow beam of protons with velocity $$ \nu = 6 \times 10^{6} m/s $$ falls normally on a silver foil of thickness $$ d = 1.0\, \mu m $$. Find the probability of the protons to be scattered into the rear hemisphere $$ (\theta > 90^{\circ}) $$

The requisite probability can be written easily by analog
It is
$$\displaystyle P = \dfrac{N(\pi / 2)}{I_{0} \tau} = nd \left ( \dfrac{Ze^2}{(4 \pi \varepsilon_0) 2mv^2} \right )^2 4 \pi \int_{\pi/2}^{x} \dfrac{\cos \theta/2 \, d \theta}{\sin^3 \dfrac{\theta}{2}} $$

The integral is unity. Thus.

$$ P = \pi n d \left ( \dfrac{Ze^2}{(4 \pi \varepsilon_{0}) mv^2} \right )^2 $$

Substitution gives using

$$ n = \dfrac{\rho_{Ag} N_{A}}{A_{Ag}} = \dfrac{10.5 \times 10^3 \times 6.023 \times 10^{26}}{108} , P = 0.006 $$

The effective cross section of a gold nucleus corresponding to the scattering of monoenergetic alpha particles within the angular interval from $$ 90^{\circ} $$ to $$180^{\circ} $$ is equal to $$ \Delta \sigma= 0.50\ kb$$. Find:
(a) the energy of alpha particles;
(b) the differential cross section of scattering $$ d \sigma / d \Omega $$ (kb/sr) corresponding to the angle $$ \theta = 60^{\circ} $$.

From the formula
$$ \Delta \sigma = \left ( \dfrac{Ze^{2}}{(4 \pi \varepsilon_{0})2 T} \right )^{2} \pi \cot^{2} \dfrac{\theta_{0}}{2}$$

or $$ T = \dfrac{Ze^{2}}{4 \pi \varepsilon_{0}} \cot^{\theta_{0}}{2} \sqrt{\dfrac{\pi}{\Delta \sigma}} $$

Substituting the values with $$Z = 79 $$ we get $$ (\theta_{0} = 90^{\circ}) $$

$$ T = 0.903 \,MeV$$
(b) The differential scattering cross section is
$$ \dfrac{d \sigma}{d \Omega} = C \,cosec^{4} \dfrac{\theta}{2} $$

where $$ \Delta \sigma (\theta > \theta_{0}) = 4 \pi C \cot^{2} \dfrac{\theta_{0}}{2} $$

Thus from the given data
$$C = \dfrac{500}{4 \pi} b = 39.79\, b/sr $$

So $$ \dfrac{d \sigma}{d \Omega} (\theta = 60^{\circ}) = 39.79 \times 16 \, b/sr = 0.637\, kb/sr $$

A narrow beam of alpha particle with kinetic energy $$ T = 900\, keV$$ falls normally on a golden foil incorporating $$ n = 1.1 \times 10^{19} $$ nuclei / $$cm^2$$. Find the fraction of alpha particles scattered through the angles $$ \theta < \theta_{0} = 20^{\circ} $$.

Because of the $$ {\text{cosec}}^4\dfrac{\theta}{2} $$ dependence of the scattering , the number of particles (or fraction) scattered through $$ \theta < \theta_{0} $$ cannot be calculated directly. But we can write this fraction as
$$P (\theta_{0}) = 1 - Q(\theta_{0}) $$
where $$ Q (\theta_0)$$ is the fraction of particles scattered through $$ \theta \geq \theta_0$$. This fraction has been calculated before and is (see the results of 6.12(b))

$$ Q (\theta_0) = \pi n \left ( \dfrac{Ze^2}{(4 \pi \varepsilon_0) T} \right )^2 \cot^2 \dfrac{\theta_0}{2} $$
where $$n$$ here is number of nuclei/cm$$^{2}$$. Using the data we get

$$ Q = 0.4 $$
Thus $$P(\theta_0) = 0.6 $$

A particle of mass $$m$$ moves along a circular orbit in a centro-symmetrical potential field $$ U(r) = \dfrac{kr^2}{2}$$. Using the Bohr quantization condition, find the permissible orbital radii and energy levels of that particle.

We have the following equation (we ignore reduced mass effects),thus we know that
$$ \dfrac{m v^{2}}{r} = kr $$

also, $$\displaystyle m\ v\ r = n -\!\!\!_h $$
so, $$ \ v = \sqrt{m\ k} r $$

and $$ r = \sqrt{\dfrac{n -\!\!\!_h}{\sqrt{m\ k}}} $$

and $$ v = \sqrt{n -\!\!\!_h \sqrt{m\ k}}/ m $$

The energy levels are $$E_{n} = \dfrac{1}{2} m\ v^{2} + \dfrac{1}{2} k\ r^{2} $$

$$ = \dfrac{1}{2} \dfrac{n -\!\!\!_h \sqrt{m\ k}}{m} + \dfrac{1}{2} k \dfrac{n -\!\!\!_h}{\sqrt{m k}} $$

$$ = n -\!\!\!_h \sqrt{\dfrac{k}{m}} $$

Atoms - Class 12 Medical Physics - Extra Questions

A uniform magnetic field B exists in a region. An electron projected perpendicular to the field goes in a circle. Assuming Bohr's quantization rule, calculate the radius of $$n$$th orbit and the minimum possible speed of electron.

During Rutherford's experiment, generally, the thin foil of heavy atoms like gold was used and bombarded by the $$\alpha$$-particles. If the thin foil of light atoms like aluminium etc. is used, what difference would have been observed?

State the postulates of Bohr atom model. Obtain an expression for the radius of the $$n$$th orbit of hydrogen atom.

(a) How many sub-shells are associated with n = 4 ? (b) How many electrons will be present in the sub-shells having $$m_s$$ value of -1/2 for n = 4 ?

State two ways of providing energy to a metal to emit electrons from its surface.

Diagram shows an electron gun inside a cathode ray tube. Label the screen with the letter S.

How is a cathode ray tube used to convert an electric signal into a visual signal ?

State two uses of a cathode ray tube.

Name one device in which the cathode ray tube is used.

What is the effect on the thermion beam in a cathode ray tube if the anode voltage value is reduced but remains above zero?

Can we use a screen coated with barium platinocyanide in a cathode ray tube? If so what effects do we observe on the screen?

Name spectral series of hydrogen which lies in the ultraviolet region of electromagnetic spectrum.

Name a common device where a hot cathode ray tube is used.

Draw a simplified labelled diagram of a hot cathode ray tube.

(i) In a cathode ray tube what is the function of anode? (ii) State the energy conversion taking place in a cathode ray tube. (iii) Write one use of cathode ray tube.

Name the three main parts of a Cathode Ray Tube.

Name a material which exhibits fluorescence when cathode rays fall on it.

Answer the following question based on a hot cathode ray tube. Name the charged particles.

Define Bohr's orbit or stationary orbit.

Explain different types of spectral lines?

With the help of neat diagram, describe the Geiger-Marsden experiment.

What is photon?

In a cathode ray tube, why is the filament made of tungsten?

Diagram shows an electron gun inside a cathode ray tube. You have two batteries one of 6 V and other of 1000 V. Show on the diagram how to connect these batteries between the terminals $$T_1,\, T_2,\, T_3,\, and\, T_4$$ in order to produce an electron beam from the gun.

In a cathode ray tube, why is the cathode coated with thorium and carbon?

An electron in $$nth$$ excited state in a hydrogen atom comes down to first excited state by emitting ten different wavelengths. Find the value of $$n$$ (an integer).

Diagram shows an electron gun inside a cathode ray tube. Draw a pair of plates $$P_1\, and\, P_2$$ to apply the electric field in the diagram and show the deflection of the particles emitted by X in between the plates $$P_1\, and P_2$$.

Draw a neat and well labelled diagram of Rutherford's $$\alpha-$$ particle scattering experiment.

The radius of the innermost electron orbit of a hydrogen atom is $$5.3 \times 10^{-11}$$ m. Calculate the radius of n = 3 orbit of the same atom.

Obtain the first Bohrs radius and the ground state energy of a muonic hydrogen atom [i.e., an atom in which a negatively charged muon $$(\mu ^-)$$ of mass about $$207m_e$$ orbits around a proton].

Suppose you are given a chance to repeat the alpha-particle scattering experiment using a thin sheet of solid hydrogen in place of the gold foil. (Hydrogen is a solid at temperatures below 14 K.) What results do you expect?

Using Bohr's atomic model, derive an equation for radius of orbit of an electron.

Which of the following curves may represent the speed of the electron in a hydrogen atom as a function of the principal quantum number n?

In an experiment on $$\alpha$$-particle scattering by a thin foil of gold, draw a plot showing the number of particles scattered versus the scattering angle $$\theta$$. Why is it that a very small fraction of the particles are scattered at $$\theta > 90^\circ$$?

Describe the Geiger-Marsden experiment. What are Its observations and conclusions?

Show that if the 63 electrons in an atom of europium were assigned to shells according to the logical sequence of quantum numbers, this element would be chemically similar to sodium.

Answer the following question, which help you understand the difference between Thomson's model and Rutherford's model better.In which model is it completely wrong to ignore multiple scattering for the calculation of a average angle of scattering of a particles by a thin foil?

Describe Rutherford's experiment on scattering of $$\alpha$$-particles. How do it help in discovery of nucleus ?

The only partially filled subshell of a certain atom contains three electrons, the basic term of the atom having $$L = 3$$. Using the Hund rules, write the spectral symbol of the ground state of the given atom.

How many and which values of the quantum number J can an atom possess in the state with quantum numbers S and L equal respectively to(a) $$2$$ and $$3$$; (b) $$3$$ and $$3$$; (c) $$5/2$$ and $$2$$?

Write the spectral designation of the term whose degeneracy is equal to seven and the quantum numbers L and S are interrelated as $$L = 3S$$.

Write the spectral symbols for the terms of a two-electron system consisting of one p electron and one d electron.

Write the spectral designations of the terms of the hydrogen atom whose electron is in the state with principal quantum number $$n = 3$$.

An atom is in the state whose multiplicity is three and the total angular momentum is $$ h\sqrt{20} $$. What can the corresponding quantum number L be equal to ?

An atom possessing the total angular momentum $$h\sqrt{6} $$ is in the state with spin quantum number $$S = 1$$. In the corresponding vector model the angle between the spin momentum and the total angular momentum is $$ \theta = 73.2^{\circ} $$. Write the spectral symbol for the term of that state.

A system comprises an atom in $$^{2}P_{3/2} $$ state and a d electron. Find the possible spectral terms of that system.

Find the ratio of the number of atoms of gaseous sodium in the state $$3P$$ to that in the ground state $$3S$$ at a temperature $$ T=2400\,K$$. The spectral line corresponding to the transition $$ 3P \rightarrow 3S$$ is known to have the wavelength $$ \lambda = 589\,nm$$.

(i) State bohr's quantization condition for defining stationary orbits. How does de broglie hypothesis explain the stationary orbits?(ii) Find the relation between the three wavelengths $${ \lambda }_{ 1 }$$,$${ \lambda }_{ 2 }$$ and $${ \lambda }_{ 3 }$$ from the energy level diagram shown below.

Show that the radius of the orbit in hydrogen atoms varies as $$\displaystyle { n }^{ 2 }$$, where n is the principal quantum number of the atom.

Passing down to the ground state, excited $$Ag^{109}$$ nuclei emit either gamma quanta with energy $$87\,keV$$ or K conversion electrons whose binding energy is $$26\,keV$$. Find the velocity of these electrons.

On the basis of Bohr's theory, derive an expression for the radius of the of the $$n^{th}$$ orbit of an electron of hydrogen atom.

Obtain an expression for the radius of Bohr orbit for $$H$$-atom.

Find the value of energy of electron in eV in the third Bohr orbit of hydrogen atom.(Rydberg's constant $$\left(R\right)=1.097 \times {10}^{7}{m}^{-1}$$Planck's constant $$\left(h\right)=6.63 \times {10}^{-34}J-s$$,Velocity of light in air $$\left(c\right)=3 \times {10}^{8}{m}/{s}$$).

What conclusion was drawn by Rutherford based on Geiger-Marsden's experiment on scattering of alpha particles?

Explain the spectral series of hydrogen atom (diagram not necessary).

When a vapour is excited at low pressure by passing an electric current through it, a spectrum is obtained.a) Draw a spectral series of emission lines in hydrogen.b) Name the different series of hydrogen atom.c) In which region Lyman series is located.

Answer the following question based on a hot cathode ray tube. State the approximate voltage used to heat the filament.

The electron in the hydrogen atom is moving with a speed of $$2.3 \times 10^6 m/s$$ in an orbit of radius $$0.53 \mathring{A}$$. Calculate the period of revolution of electron. ($$\pi = 3.142$$)

Draw the diagram representing the schematic arrangement of the Geiger-Marsdon experimental setup tor the alpha particle scattering.

(a) Write the $$\beta-decay$$ of tritium in symbolic from.(b) Why is it experimentally found difficult to detect neutrinos in this process?

Radiation coming from transitions $$n=2$$ to $$n=1$$ of hydrogen atoms falls on helium ions in $$n=1$$ and $$n=2$$ states. What are the possible transition of helium ions as they absorb energy from the radiation ?

A radiation of wavelength $$\lambda$$ is incident on a metal surface having $$ 620 nm$$ threshold wavelength. The stopping potential of the electron emitted is $$4.9 V$$. What is the value of $$\lambda$$ (in nm)?

Describe Rutherford's atomic model.

A proton and an alpha particle having the same kinetic energy are allowed to pass through a uniform magnetic field perpendicular to the direction of their motion. Compare the radii of the paths of proton and alpha particle.

What is the wavelength of light emitted when the electron in hydrogen atom undergoes transition from an energy level with n=4 to an energy level with n=2 ? What is the colour of the radiation ?

The energy of a photon is equal to $$3$$ kilo $$eV$$. Calculate its linear momentum.

which an electron in hydrogen atom jumps from the third excited state to the ground state

A beam of light having wavelengths distributed uniformly between 450 nm to 550 nm passes through a sample of hydrogen gas. Which wavelength will have the least intensity in the transmitted beam?

What is the effective mass of photon having wavelength $$\lambda$$?

The wavelength of $$H_\alpha$$ line Balmer's series of hydrogen spectrum is $$6563 \ \mathring{A}$$. Find the:(i) Wavelength of $$H_ \gamma$$ (gamma) line of Balmer series.(ii) Shortest wavelength of Bracket series.

A hydrogen atom in a state having a binding energy of $$0.85\ eV$$ makes transition to a state with excitation energy $$10.2\ eV$$Identify the quantum numbers $$n$$ of the upper and the lower energy states involved in the transition.

The transition from the state $$n=4$$ to $$n=3$$ in hydrogen-like atom results in ultraviolet radiation. Infrared radiation will be obtained in the transition from?

Show that angular speed of electron in $${n}^{th}$$ Bohr's orbit is equal to :$$ =\dfrac { \pi { me }^{ 4 } }{ { 2 }\epsilon _{ 0 }^{ 2 }{ h }^{ 3 }{ n }^{ 3 } }$$ or frequency of revolution, $$f=\dfrac { \pi { me }^{ 4 } }{ { 2 }\epsilon _{ 0 }^{ 2 }{ h }^{ 3 }{ n }^{ 3 } }$$

Obtain the expression for radius of $${n}^{th}$$ Bohr orbit and show that the radius is proportion to square of the principal quantum number.

Using expression for energy of electron, obtain the Bohr's formula for hydrogen spectral line.

In a sample of hydrogen-like atoms all of which are in the ground state, a photon beam containing photons of various energies is passed. In the absorption spectrum, five dark lines are observed. The number of bright lines in the emission spectrum will be (Assume that all transitions take place)

Using postulates of Bohr's theory of hydrogen atom, show that radii of orbits increases as $$n^2$$.

The angular speed of electron in the nth orbit of hydrogen atom is

Describe the drawback of Rutherford's model of atom.

(a) How many sub-shells are associated with n = 4 ?
(b) How many electrons will be present in the sub-shells having $$m_s$$ value of -1/2 for n = 4 ?

(i) In a cathode ray tube what is the function of anode?
(ii) State the energy conversion taking place in a cathode ray tube.
(iii) Write one use of cathode ray tube.

Answer the following question, which help you understand the difference between Thomson's model and Rutherford's model better.
In which model is it completely wrong to ignore multiple scattering for the calculation of a average angle of scattering of a particles by a thin foil?

How many and which values of the quantum number J can an atom possess in the state with quantum numbers S and L equal respectively to
(a) $$2$$ and $$3$$; (b) $$3$$ and $$3$$; (c) $$5/2$$ and $$2$$?

(i) State bohr's quantization condition for defining stationary orbits. How does de broglie hypothesis explain the stationary orbits?
(ii) Find the relation between the three wavelengths $${ \lambda }_{ 1 }$$,$${ \lambda }_{ 2 }$$ and $${ \lambda }_{ 3 }$$ from the energy level diagram shown below.

Find the value of energy of electron in eV in the third Bohr orbit of hydrogen atom.
(Rydberg's constant $$\left(R\right)=1.097 \times {10}^{7}{m}^{-1}$$
Planck's constant $$\left(h\right)=6.63 \times {10}^{-34}J-s$$,
Velocity of light in air $$\left(c\right)=3 \times {10}^{8}{m}/{s}$$).

When a vapour is excited at low pressure by passing an electric current through it, a spectrum is obtained.
a) Draw a spectral series of emission lines in hydrogen.
b) Name the different series of hydrogen atom.
c) In which region Lyman series is located.

(a) Write the $$\beta-decay$$ of tritium in symbolic from.
(b) Why is it experimentally found difficult to detect neutrinos in this process?

The wavelength of $$H_\alpha$$ line Balmer's series of hydrogen spectrum is $$6563 \ \mathring{A}$$. Find the:
(i) Wavelength of $$H_ \gamma$$ (gamma) line of Balmer series.
(ii) Shortest wavelength of Bracket series.

A hydrogen atom in a state having a binding energy of $$0.85\ eV$$ makes transition to a state with excitation energy $$10.2\ eV$$
Identify the quantum numbers $$n$$ of the upper and the lower energy states involved in the transition.

Show that angular speed of electron in $${n}^{th}$$ Bohr's orbit is equal to :
$$ =\dfrac { \pi { me }^{ 4 } }{ { 2 }\epsilon _{ 0 }^{ 2 }{ h }^{ 3 }{ n }^{ 3 } }$$ or frequency of revolution, $$f=\dfrac { \pi { me }^{ 4 } }{ { 2 }\epsilon _{ 0 }^{ 2 }{ h }^{ 3 }{ n }^{ 3 } }$$

An electron, trapped in a one-dimensional infinite potential
well $$250\ pm$$ wide, is in its ground state. How much energy must it
absorb if it is so jump up to the state with $$n=4?$$

A hydrogen atom is excited from its ground state to the state with $$n=4$$;
what are the highest

An electron is trapped in a one dimensional infinite potential well that is $$100\ pm$$ wide; the electron is in its ground state. What is the probability that you can detect the electron in an interval of width $$\Delta x=5.0\ pm$$ centered at $$x=$$
$$25\ pm$$

A one dimensional infinite well of length $$200\ pm$$ contains an electron in its third excited state. We position an electron detector probe of width $$2.00\ pm$$ so that it is centered on a point of maximum probability density.
What is the probability of detection by the probe?

A hydrogen atom is excited from its ground state to the state with $$n=4$$
How many different energies are possible

Choose the correct alternatives from the clues given at the end of the each statement:
The positively charged part of the atom possess most of the mass in ..................
(Rutherford's model / both the models)

Choose the correct alternatives from the clues given at the end of the each statement:
An atom has a nearly continuous mass distribution in a ............. but has a highly non-uniform mass distribution in.
(Thomson's model / Rutherford's model)